Study on the Degradation Law of Artificial Joint Surfaces with Natural Morphologies under Quasi-Static Cyclic Shearing
Abstract
:1. Introduction
2. Materials and Methods
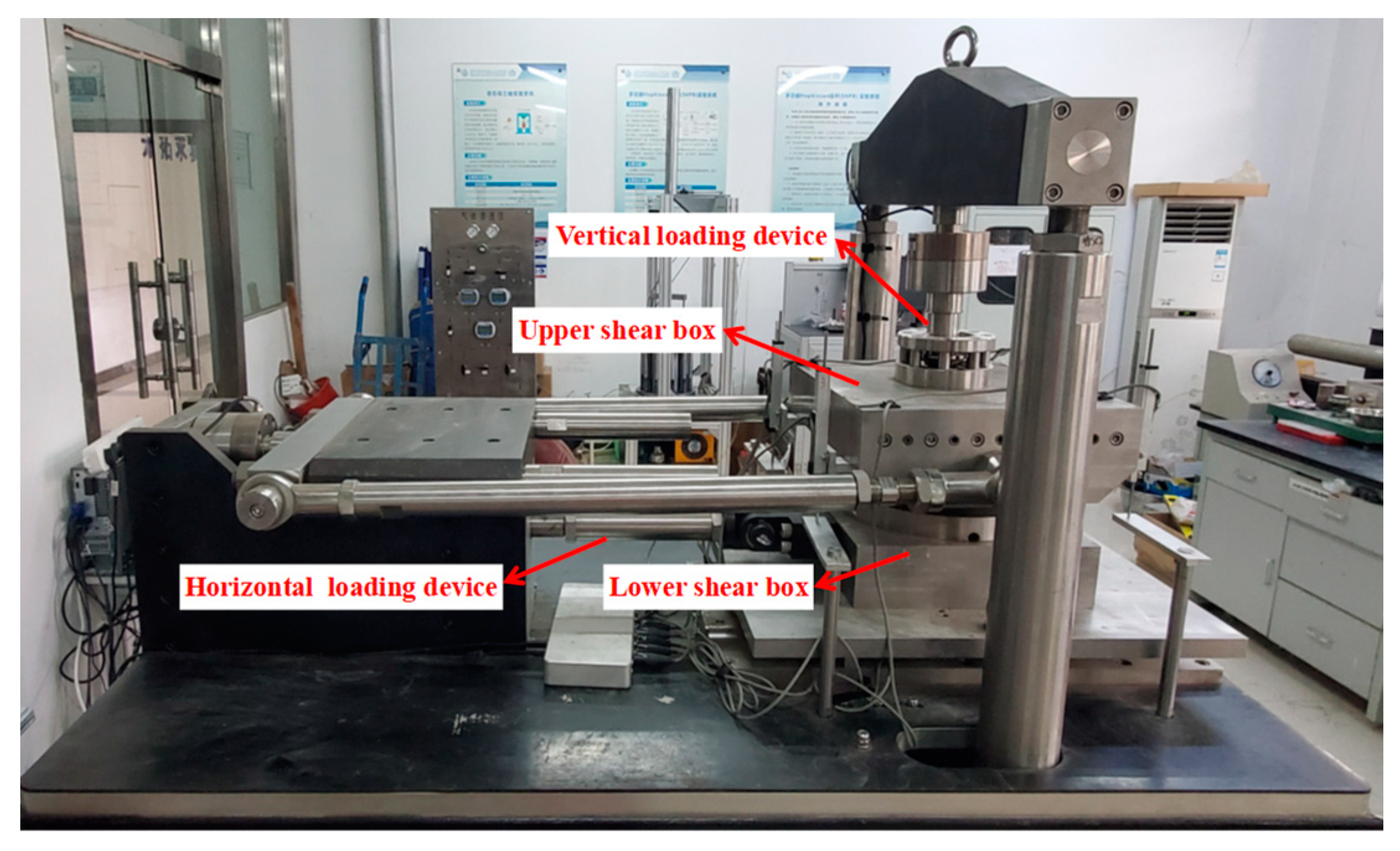
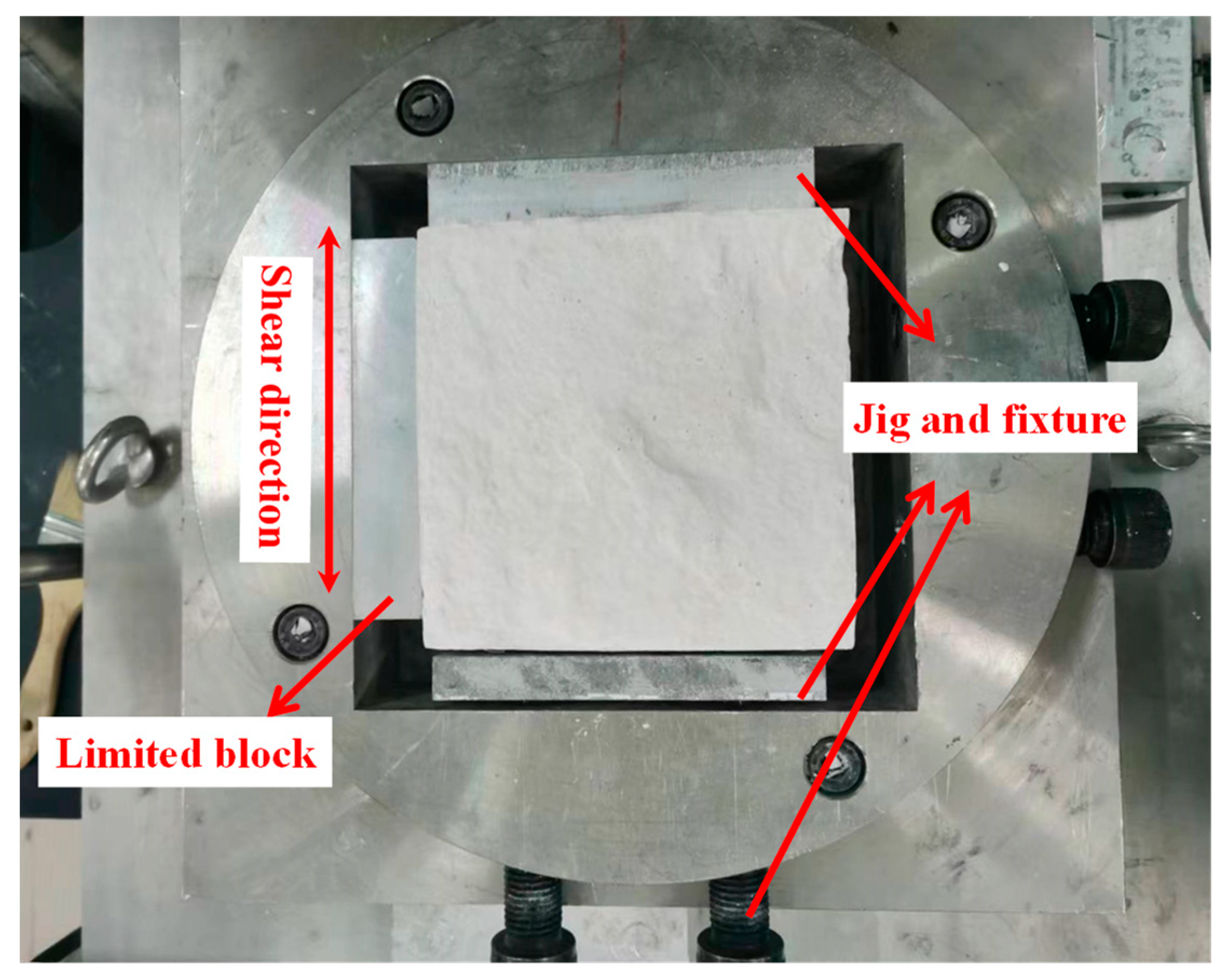
2.1. Test Apparatus
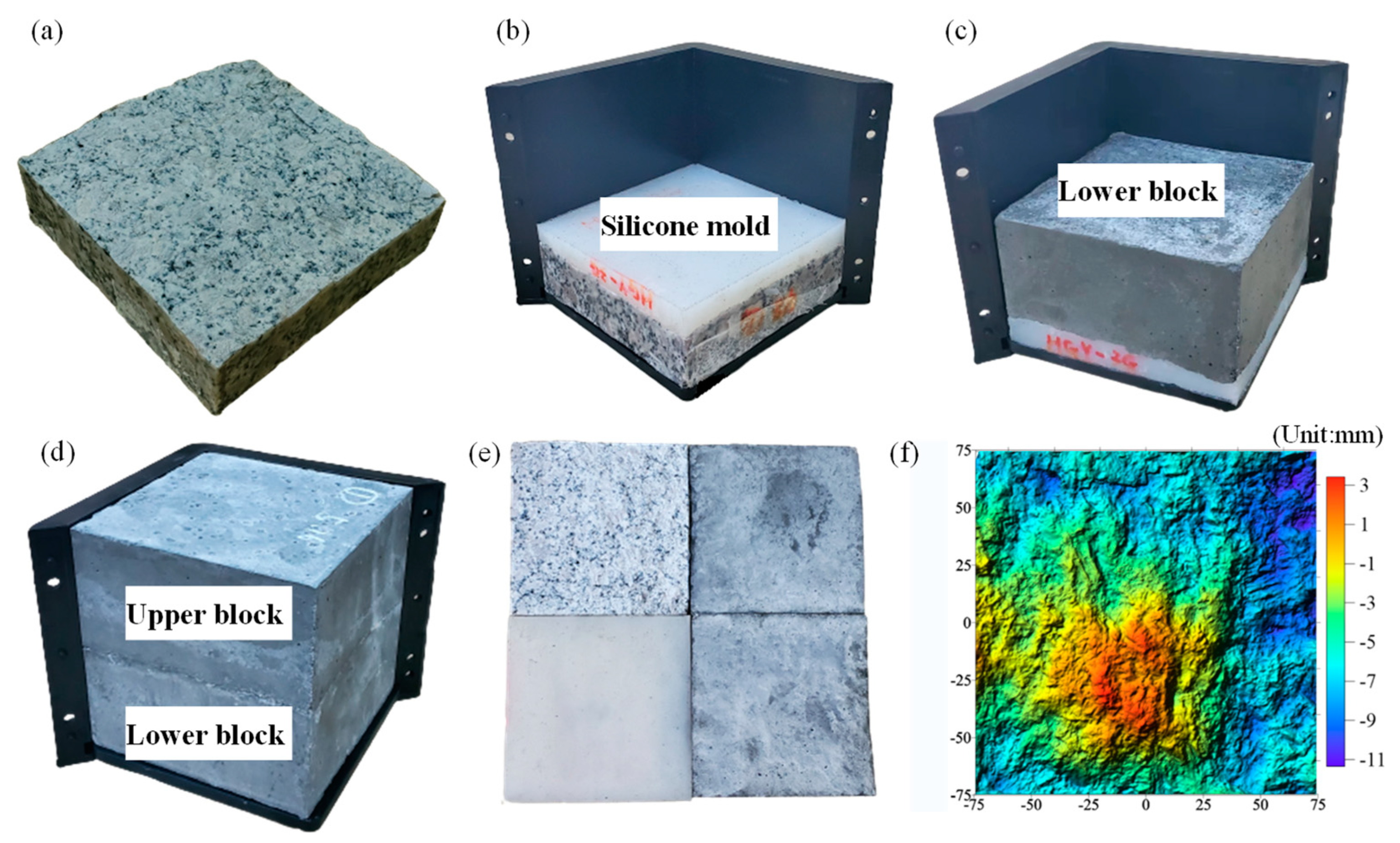
2.2. Specimen Preparation
2.3. Test Conditions
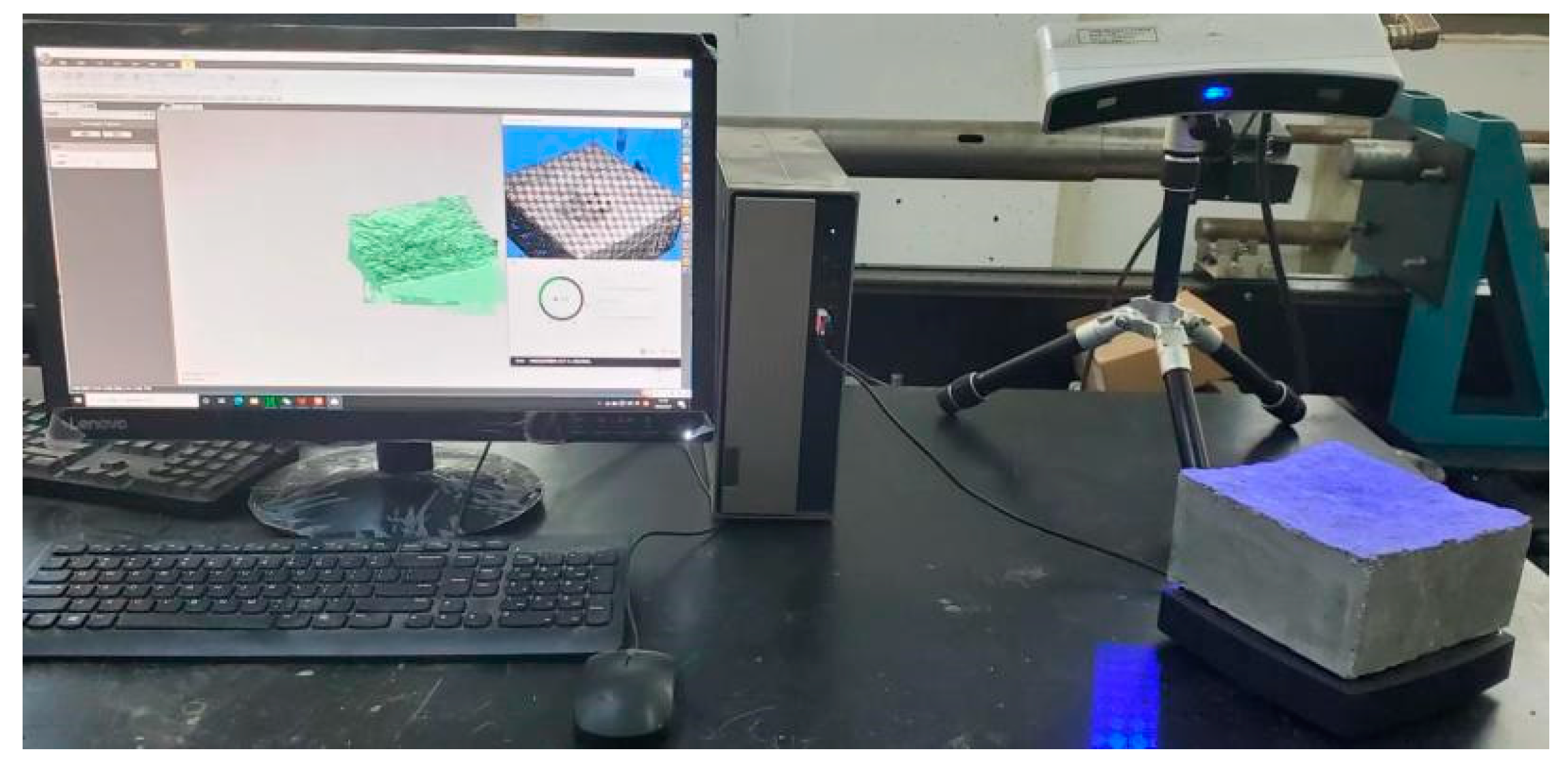
3. Characterization of 3D Joint Surfaces
3.1. Joint Morphology Quantification
3.2. Joint Surface Damage Calculation
4. Test Results and Discussion
4.1. Degree of Surface Roughness Degradation
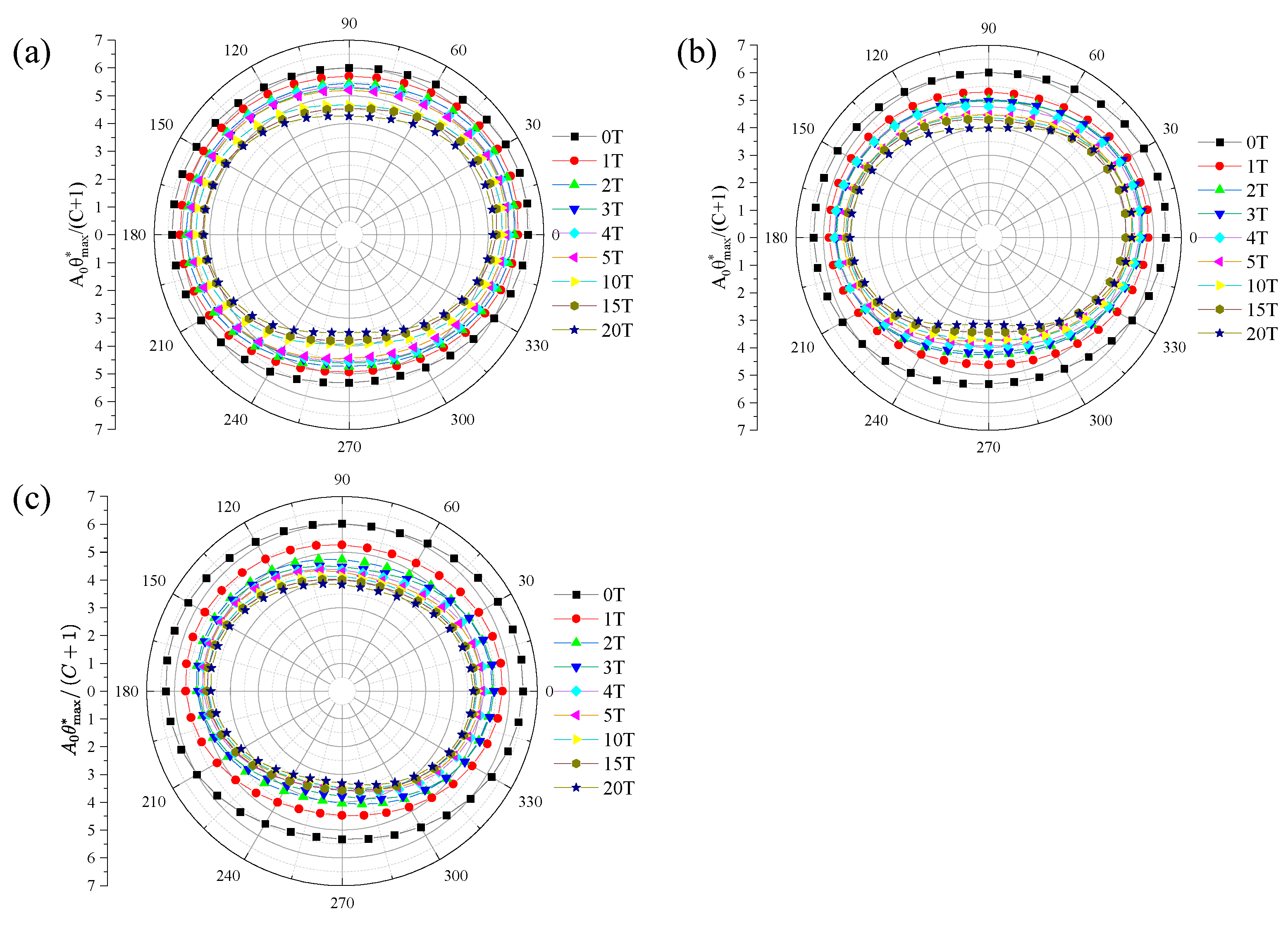
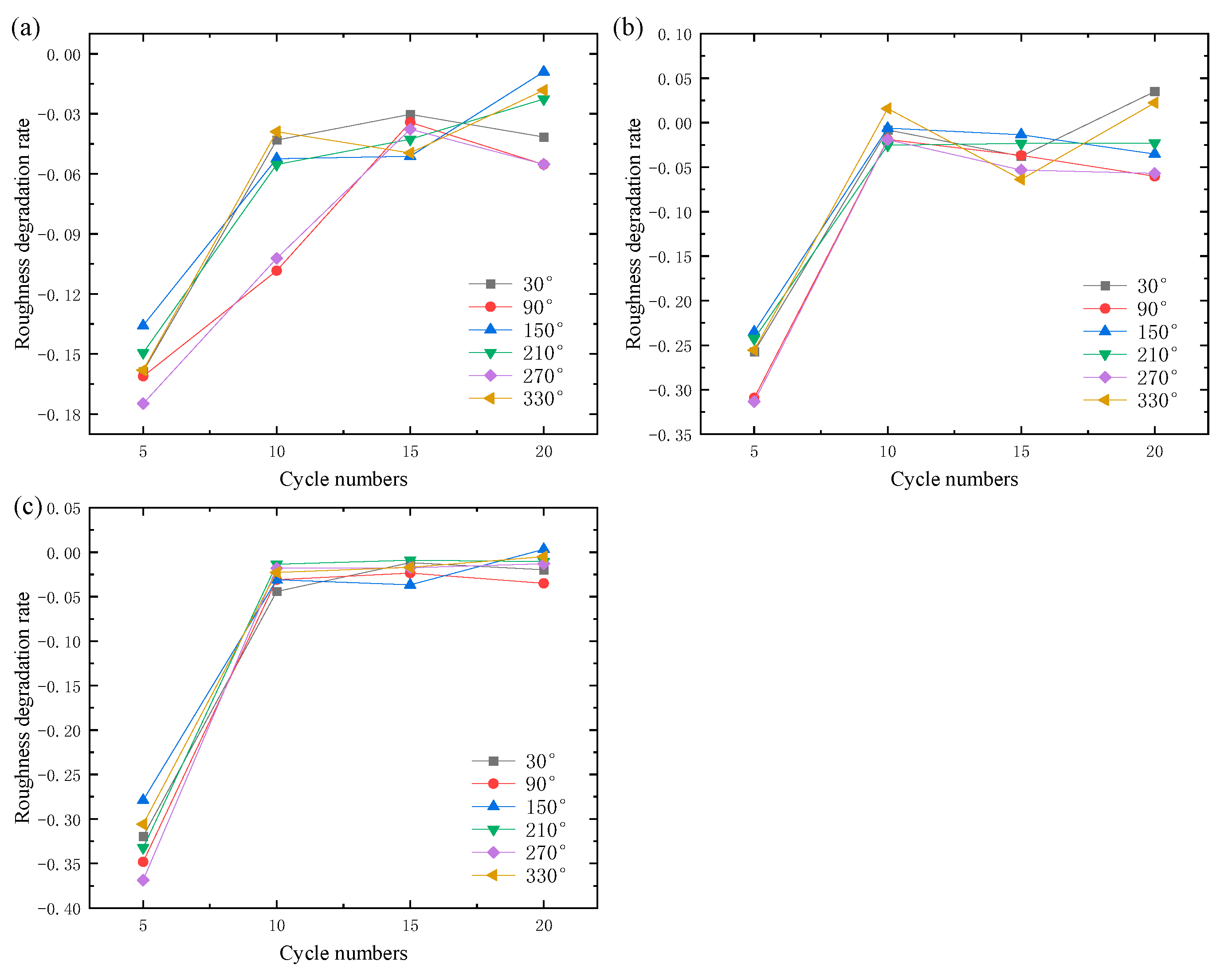
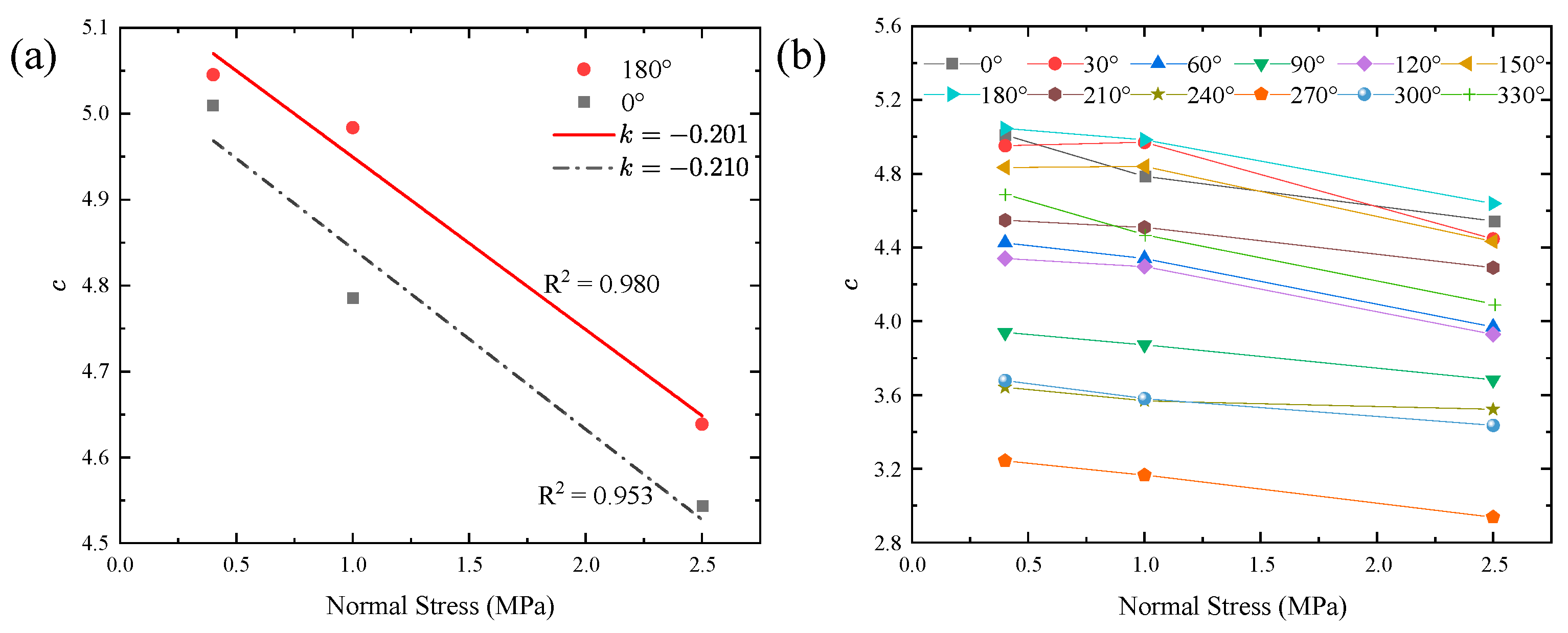
4.2. Surface Roughness Degradation Anisotropy
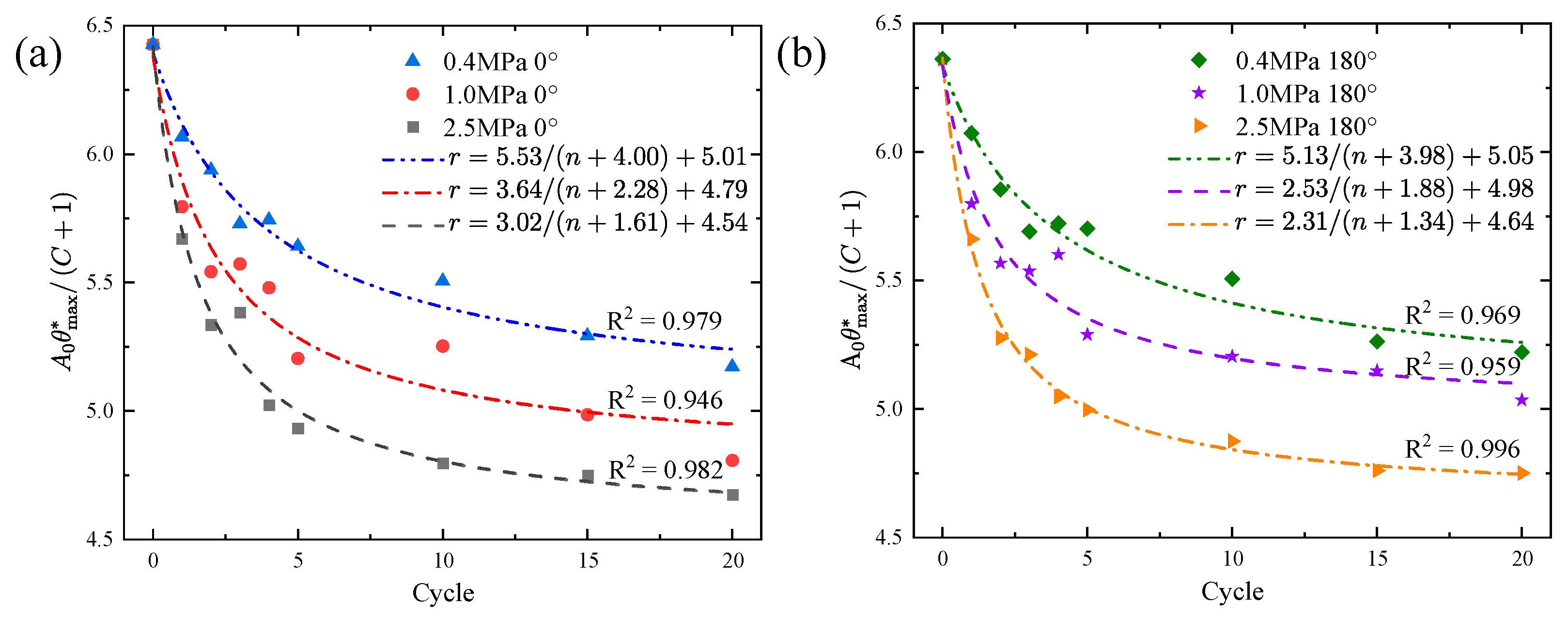
4.2.1. Analysis of the Roughness Degradation along the Shear Direction
4.2.2. Analysis of the Roughness Degradation Perpendicular to the Shear Direction
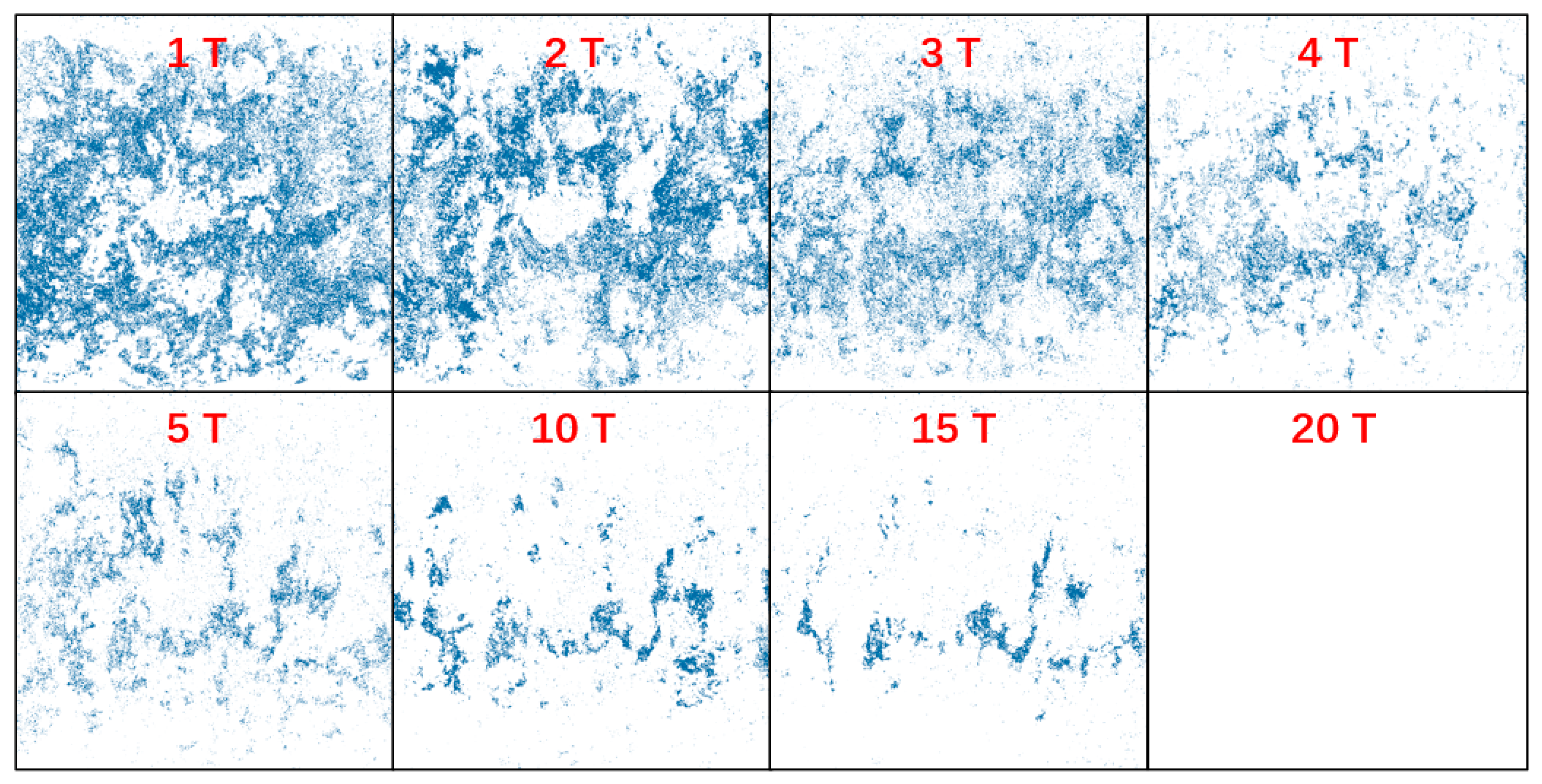
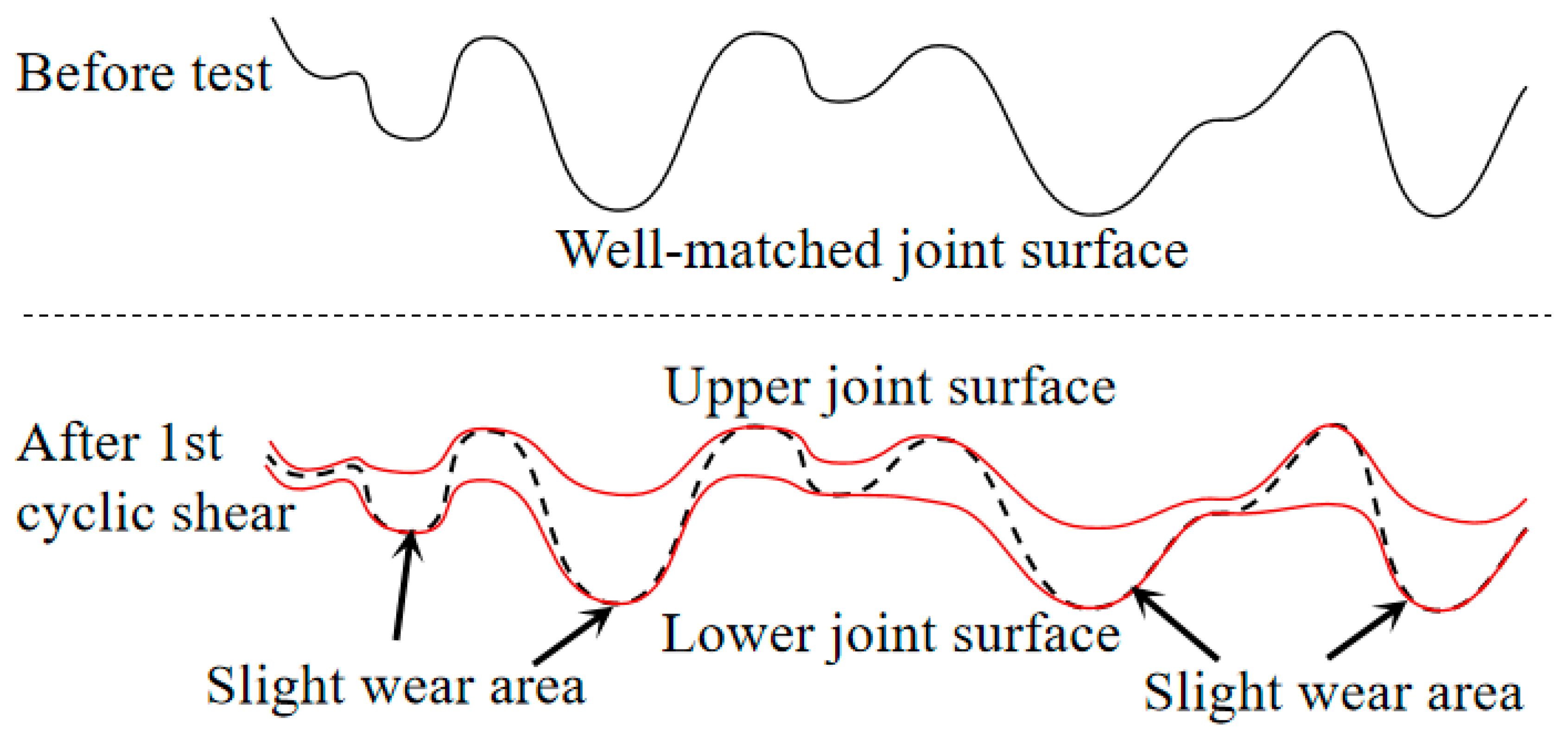
4.3. Analysis of the Joint Surface Wear
5. Conclusions
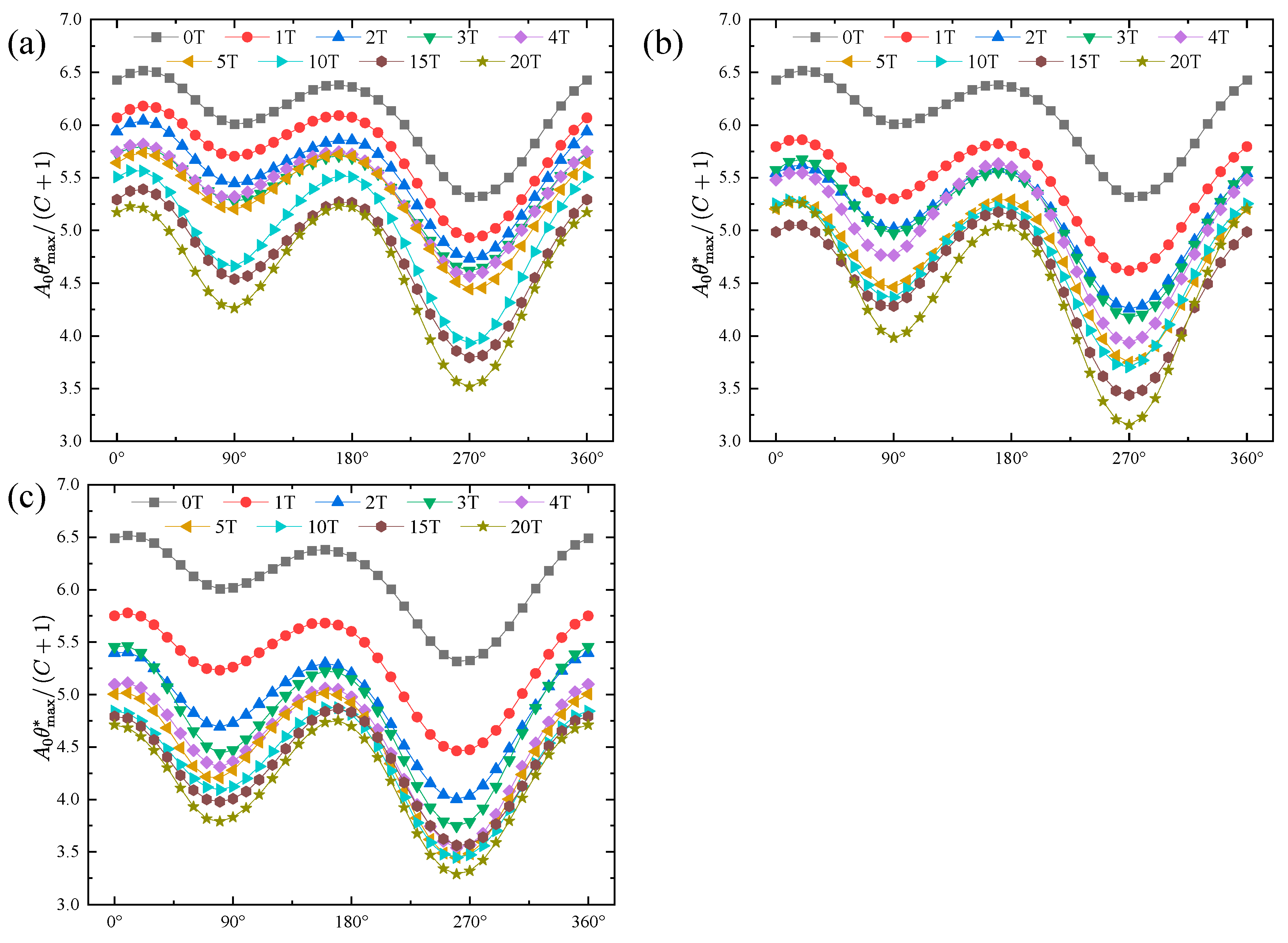
- The polar plot of the 3D roughness parameter of the joint surfaces analyzed in 36 directions demonstrates that, as the number of shear cycles increases, the polar curve of the joint surface roughness shrinks to the original direction. That is, it gradually develops from a circular to an elliptical shape, with the shear direction as the minor axis.
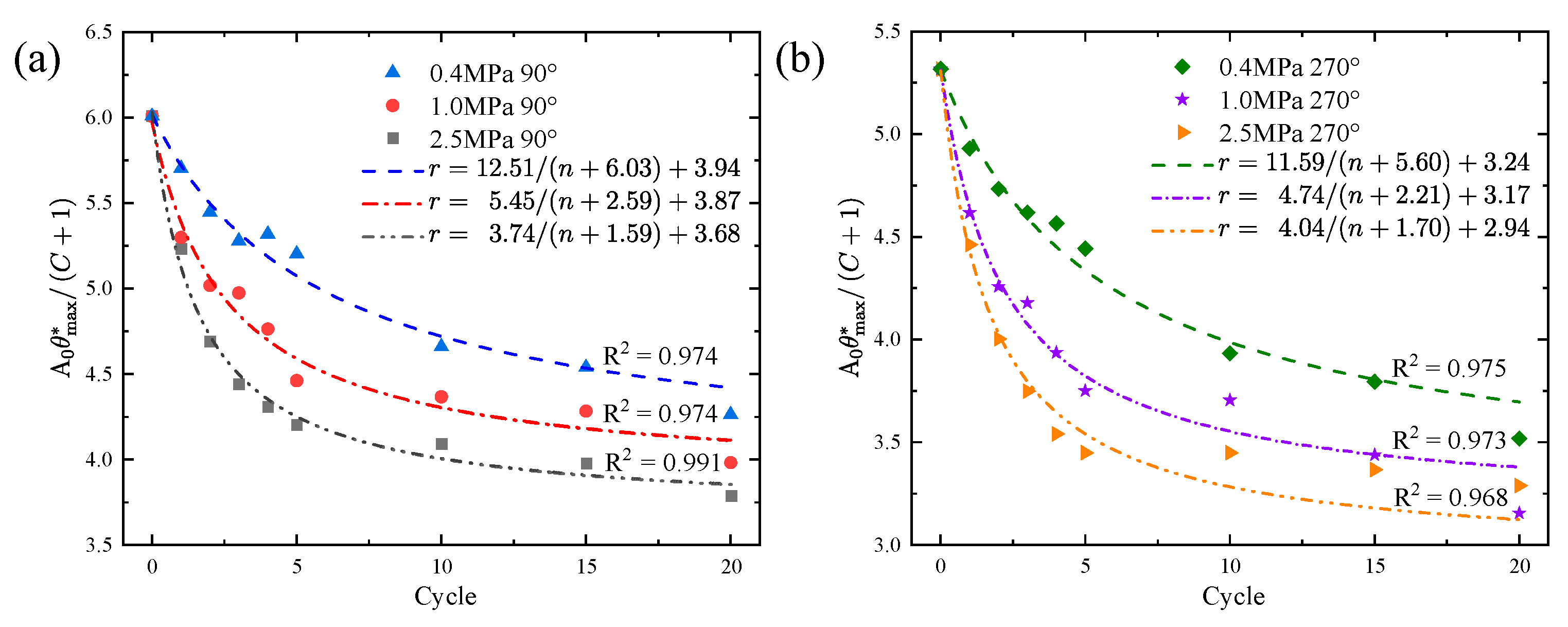
- The roughness degradation rate and the shear cycles are mutually interrelated with the normal stress level. A larger normal stress results in faster degradation rates and smaller residual roughness parameter values. The roughness degradation rate is approximately uniform with the shear cycles under low normal stress (0.4 MPa). When the normal stress increases, the roughness first degenerates quickly and then slowly.
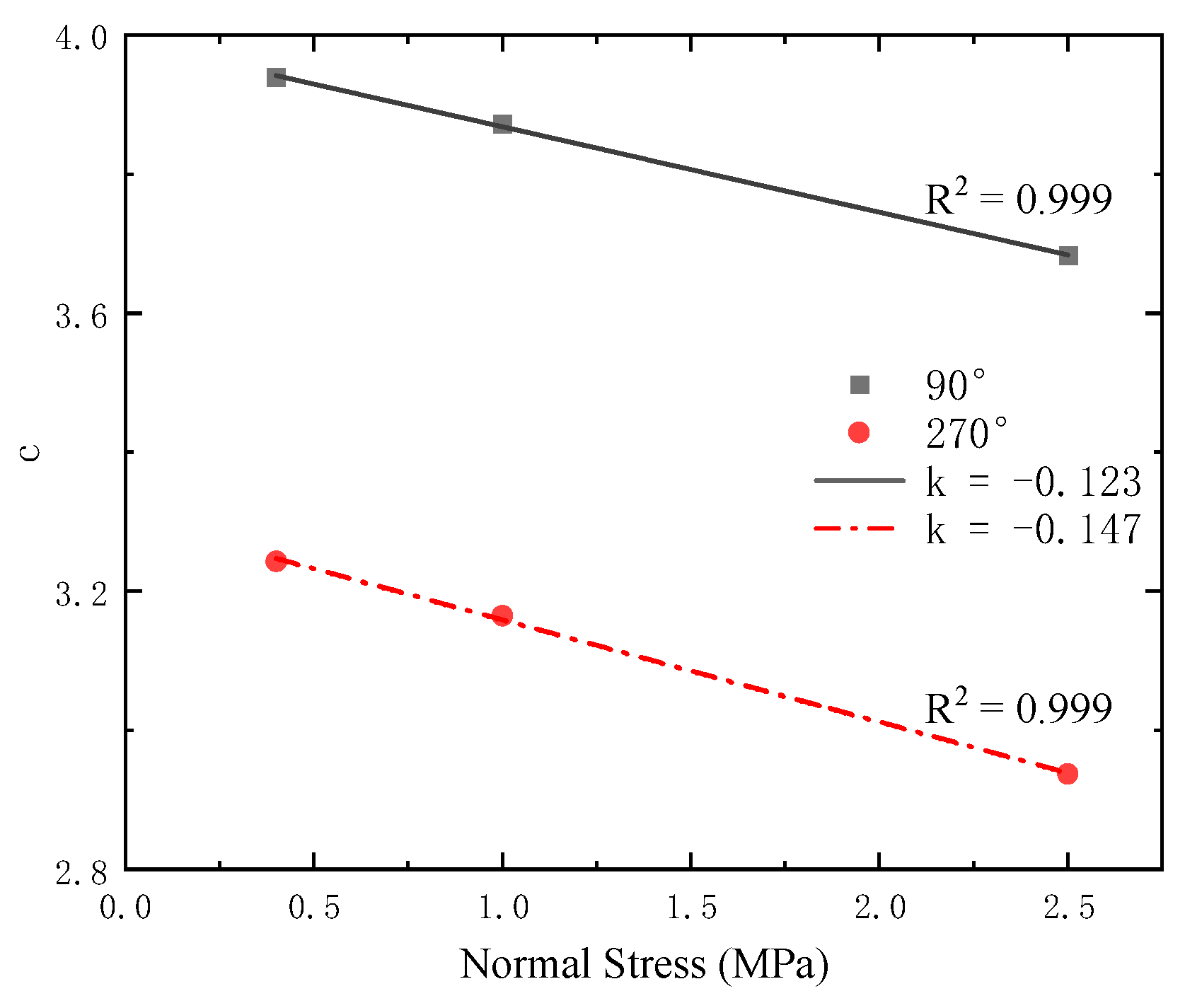
- Influenced by the cyclic shear direction, the 3D roughness of the joint surfaces degenerates anisotropically, and the most serious damage is sustained along the shear direction. An RDF () under cyclic shearing is proposed, and the residual roughness is linearly related to normal stress. The joint roughness after cyclic shears with different normal stresses can be predicted.
- The wear area ratio of the specimen’s lower block surfaces becomes severer as the number of shear cycles increases, and the increment rate of is first fast and then slow. A fitting formula of the joint surface wear under cyclic shearing is proposed, and it could aid in the assessment of the cumulative degree of wear in jointed rock masses.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Jing, L.; Stephansson, O.; Nordlund, E. Study of Rock Joints under Cyclic Loading Conditions. Rock Mech. Rock Eng. 1993, 26, 215–232. [Google Scholar] [CrossRef]
- Huang, X.; Haimson, B.C.; Plesha, M.E. An investigation of the mechanics of rock joints—Part I. Laboratory investigation. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1993, 30, 257–269. [Google Scholar] [CrossRef]
- Kana, D.D.; Fox, D.J.; Hsiung, S.M. Interlock/friction model for dynamic shear response in natural jointed rock. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1996, 33, 371–386. [Google Scholar] [CrossRef]
- Fox, D.J.; Kana, D.D.; Hsiung, S.M. Influence of interface roughness on dynamic shear behavior in jointed rock. Int. J. Rock Mech. Min. Sci. 1998, 35, 923–940. [Google Scholar] [CrossRef]
- Homand, F.; Belem, T.; Souley, M. Friction and degradation of rock joint surfaces under shear loads. Int. J. Numer. Anal. Methods Geomech. 2001, 25, 973–999. [Google Scholar] [CrossRef]
- Lee, H.S.; Park, Y.J.; Cho, T.F. Influence of asperity degradation on the mechanical behavior of rough rock joints under cyclic shear loading. Int. J. Rock Mech. Min. Sci. 2001, 38, 967–980. [Google Scholar] [CrossRef]
- Jafari, M.K.; Hosseini, K.A.; Pellet, F. Evaluation of shear strength of rock joints subjected to cyclic loading. Soil Dyn. Earthq. Eng. 2003, 23, 619–630. [Google Scholar] [CrossRef]
- Xiao-Ming, Z.; Li, H.B.; Bo, L. Study of mechanical characteristics of simulated rock joints with second-order asperity under cyclic shear loading. Rock Soil Mech. 2014, 35, 371–379. [Google Scholar]
- Mirzaghorbanali, A.; Nemcik, J.; Aziz, N. Effects of Cyclic Loading on the Shear Behaviour of Infilled Rock Joints Under Constant Normal Stiffness Conditions. Rock Mech. Rock Eng. 2014, 47, 1373–1391. [Google Scholar] [CrossRef]
- Liu, B.; Li, H.B.; Liu, Y.Q. Experimental study of deformation behavior of rock joints under cyclic shear loading. Rock Soil Mech. 2013, 34, 2475. [Google Scholar]
- Xu, B.; Liu, X.R.; Zhou, X.H. Investigation on the macro-meso fatigue damage mechanism of rock joints with multiscale asperities under pre-peak cyclic shear loading. Soil Dyn. Earthq. Eng. 2021, 151, 16. [Google Scholar] [CrossRef]
- Dang, W.G.; Konietzky, H.; Fruhwirt, T. Cyclic Frictional Responses of Planar Joints Under Cyclic Normal Load Conditions: Laboratory Tests and Numerical Simulations. Rock Mech. Rock Eng. 2020, 53, 337–364. [Google Scholar] [CrossRef]
- Ferrero, A.M.; Migliazza, M.; Tebaldi, G. Development of a New Experimental Apparatus for the Study of the Mechanical Behaviour of a Rock Discontinuity Under Monotonic and Cyclic Loads. Rock Mech. Rock Eng. 2010, 43, 685–695. [Google Scholar] [CrossRef]
- Greenwood, J.A. A Unified Theory of Surface-Roughness. Proc. R. Soc. Lond. Ser. A-Math. Phys. Eng. Sci. 1984, 393, 133–157. [Google Scholar]
- Mulvaney, D.J.; Newland, D.E. Identification of surface-roughness. Proc. Inst. Mech. Eng. Part C-J. Mech. Eng. Sci. 1985, 199, 281–286. [Google Scholar] [CrossRef]
- Barton, N. Review of a new shear-strength criterion for rock joints. Eng. Geol. 1973, 7, 287–332. [Google Scholar] [CrossRef]
- Belem, T.; Homand-Etienne, F.; Souley, M. Quantitative parameters for rock joint surface roughness. Rock Mech. Rock Eng. 2000, 33, 217–242. [Google Scholar] [CrossRef]
- Grasselli, G.; Egger, P. Constitutive law for the shear strength of rock joints based on three-dimensional surface parameters. Int. J. Rock Mech. Min. Sci. 2003, 40, 25–40. [Google Scholar] [CrossRef]
- Grasselli, G. Manuel Rocha Medal recipient - Shear strength of rock joints based on quantified surface description. Rock Mech. Rock Eng. 2006, 39, 295–314. [Google Scholar] [CrossRef]
- Sun, F.T.; Jiang, Q.R.; She, C.X. Research on Three-dimensional Roughness Characteristics of Tensile Granite Joint. In Proceedings of the International Conference on Civil, Architectural and Hydraulic Engineering (ICCAHE 2012), Zhangjiajie, China, 10–12 August 2012. [Google Scholar]
- Tatone, B.S.; Grasselli, G. A method to evaluate the three-dimensional roughness of fracture surfaces in brittle geomaterials. Rev. Sci. Instrum. 2009, 80, 125110. [Google Scholar] [CrossRef]
- Chen, S.J.; Zhu, W.C.; Yu, Q.L. Characterization of Anisotropy of Joint Surface Roughness and Aperture by Variogram Approach Based on Digital Image Processing Technique. Rock Mech. Rock Eng. 2016, 49, 855–876. [Google Scholar] [CrossRef]
- Tang, H.M.; Ge, Y.F.; Wang, L.Q. Study on Estimation Method of Rock Mass Discontinuity Shear Strength Based on Three-Dimensional Laser Scanning and Image Technique. J. Earth Sci. 2012, 23, 908–913. [Google Scholar] [CrossRef]
- Shen, H.; Liu, Y.; Li, H. Rate-Dependent Characteristics of Damage and Roughness Degradation of Three-Dimensional Artificial Joint Surfaces. Rock Mech. Rock Eng. 2022, 55, 2221–2237. [Google Scholar] [CrossRef]
- Wang, G.; Zhang, X.P.; Jiang, Y.J. Rate-dependent mechanical behavior of rough rock joints. Int. J. Rock Mech. Min. Sci. 2016, 83, 231–240. [Google Scholar] [CrossRef]
- Gui, Y.; Xia, C.; Ding, W. A New Method for 3D Modeling of Joint Surface Degradation and Void Space Evolution Under Normal and Shear Loads. Rock Mech. Rock Eng. 2017, 50, 2827–2836. [Google Scholar] [CrossRef]
- Hong, E.-S.; Kwon, T.-H.; Song, K.-I. Observation of the Degradation Characteristics and Scale of Unevenness on Three-dimensional Artificial Rock Joint Surfaces Subjected to Shear. Rock Mech. Rock Eng. 2015, 49, 3–17. [Google Scholar] [CrossRef]
- Xie, H.P.; Wang, J.A.; Xie, W.H. Fractal effects of surface roughness on the mechanical behavior of rock joints. Chaos Solitons Fractals 1997, 8, 221–252. [Google Scholar] [CrossRef]
- Gentier, S.; Riss, J.; Archambault, G. Influence of fracture geometry on shear behavior. Int. J. Rock Mech. Min. Sci. 2000, 37, 161–174. [Google Scholar] [CrossRef]

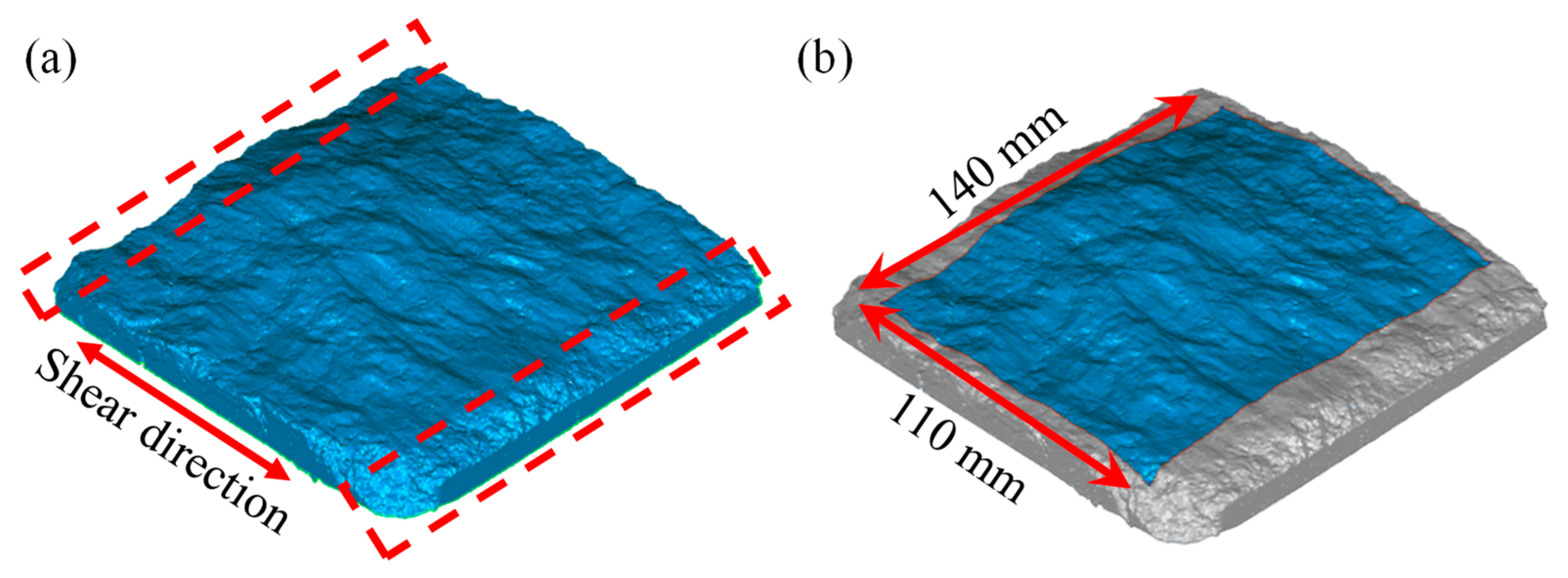
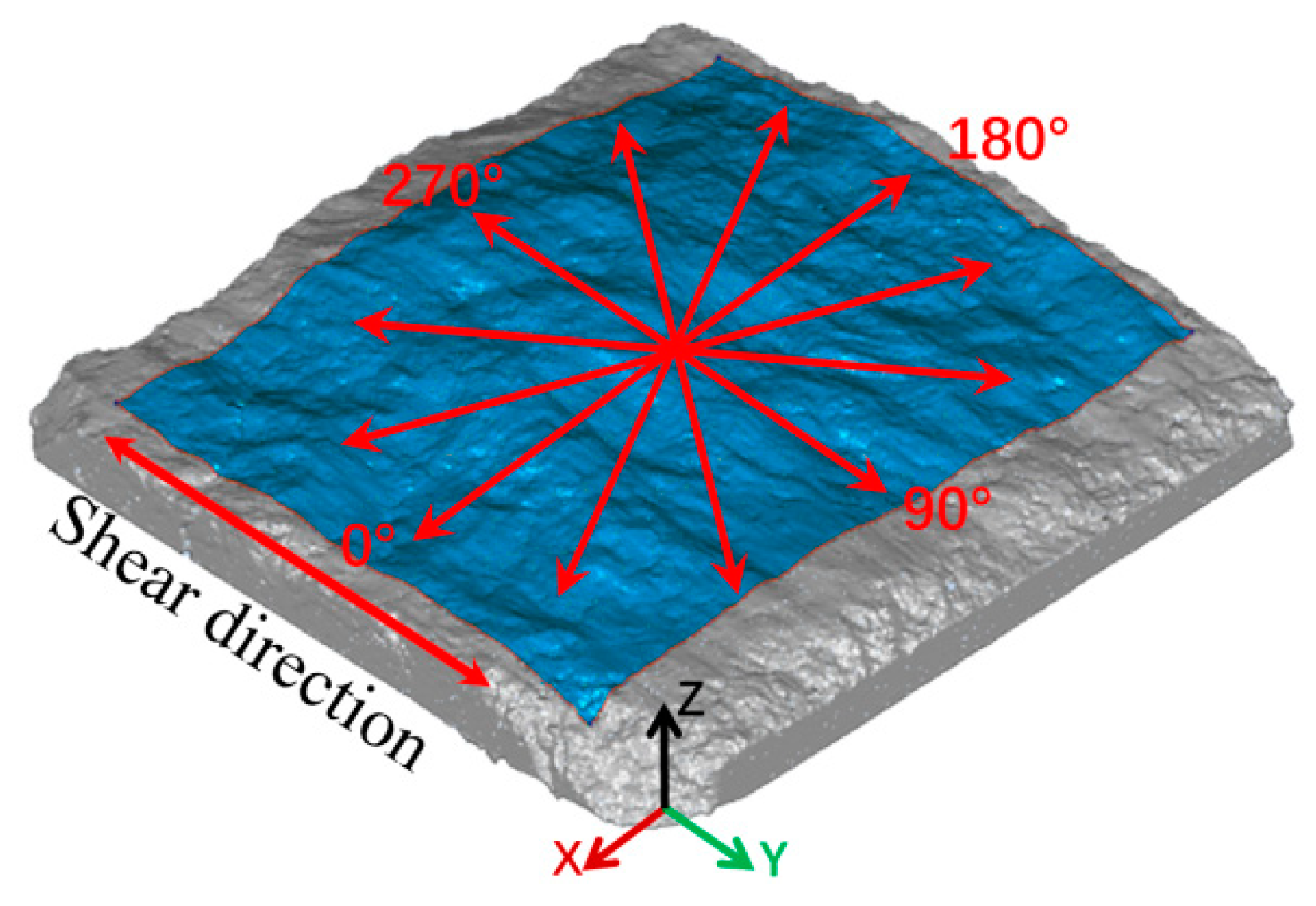
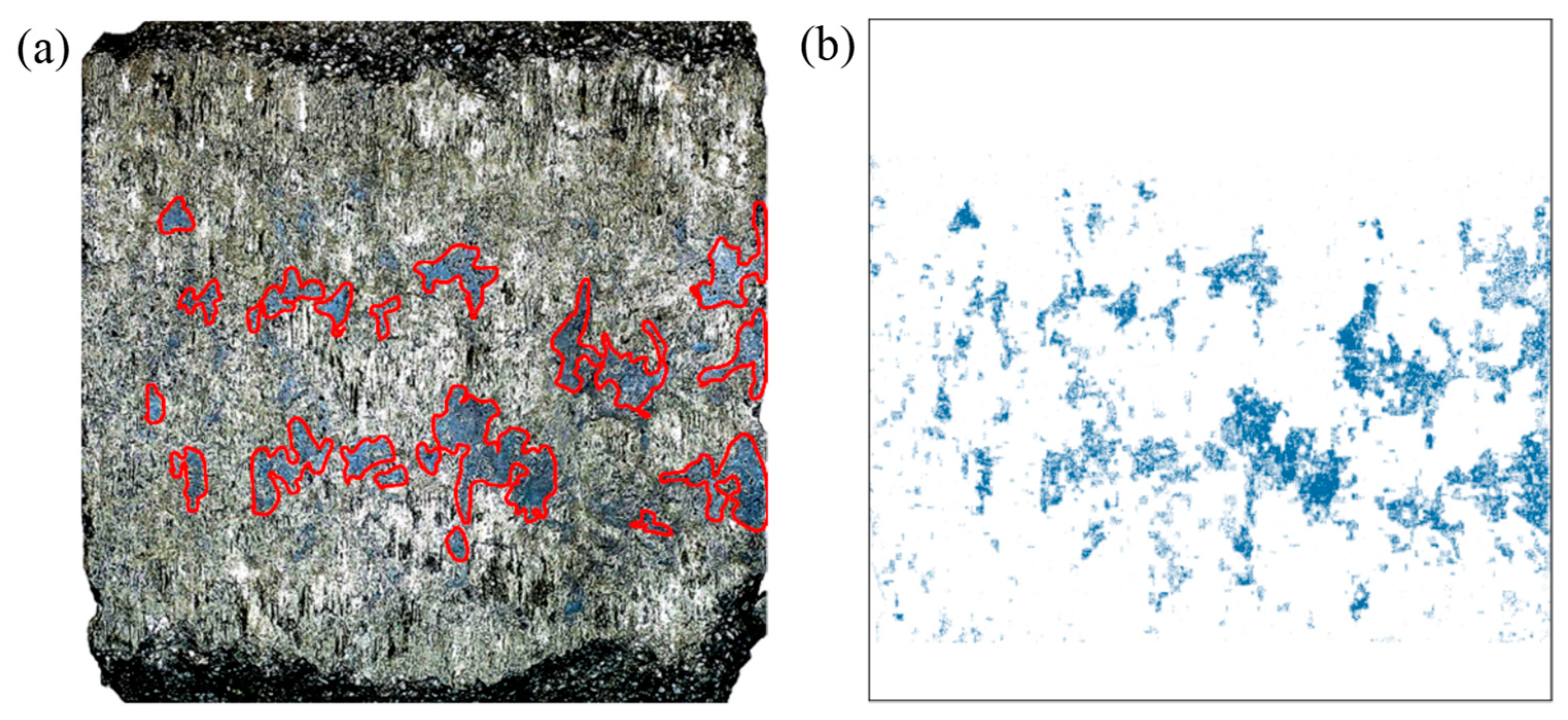


| Model Material | Grouting Cement |
|---|---|
| Density (kg/m3) | 2323 |
| Young’s modulus (GPa) | 11.54 |
| Uniaxial compressive strength (MPa) | 49.82 |
| Cohesion (MPa) | 18.94 |
| Friction angle (°) | 35.5 |
| Poisson’s ratio | 0.12 |
| Sample Number | Normal Stress (MPa) | Frequency (Hz) | Shear Displacement (mm) | Cyclic Shear Number * |
|---|---|---|---|---|
| H1, H2, H3, H4, H5, H6, H7, and H8 | 2.5 | 1/120 | 6 | 1, 2, 3, 4, 5 10, 15, and 20 |
| H9, H10, H11, H12, H13, H14, H15, and H16 | 1.0 | 1, 2, 3, 4, 5 10, 15, and 20 | ||
| H17, H18, H19, H20, H21, H22, H23, and H24 | 0.4 | 1, 2, 3, 4, 5 10, 15, and 20 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, Y.; Liu, B.; Shen, H.; Li, H. Study on the Degradation Law of Artificial Joint Surfaces with Natural Morphologies under Quasi-Static Cyclic Shearing. Appl. Sci. 2023, 13, 1441. https://doi.org/10.3390/app13031441
Zhao Y, Liu B, Shen H, Li H. Study on the Degradation Law of Artificial Joint Surfaces with Natural Morphologies under Quasi-Static Cyclic Shearing. Applied Sciences. 2023; 13(3):1441. https://doi.org/10.3390/app13031441
Chicago/Turabian StyleZhao, Yan, Bo Liu, Hui Shen, and Haibo Li. 2023. "Study on the Degradation Law of Artificial Joint Surfaces with Natural Morphologies under Quasi-Static Cyclic Shearing" Applied Sciences 13, no. 3: 1441. https://doi.org/10.3390/app13031441
APA StyleZhao, Y., Liu, B., Shen, H., & Li, H. (2023). Study on the Degradation Law of Artificial Joint Surfaces with Natural Morphologies under Quasi-Static Cyclic Shearing. Applied Sciences, 13(3), 1441. https://doi.org/10.3390/app13031441






