Magnetic Field Analysis and Optimization of the Gauge of Hybrid Maglev Needles
Abstract
1. Introduction
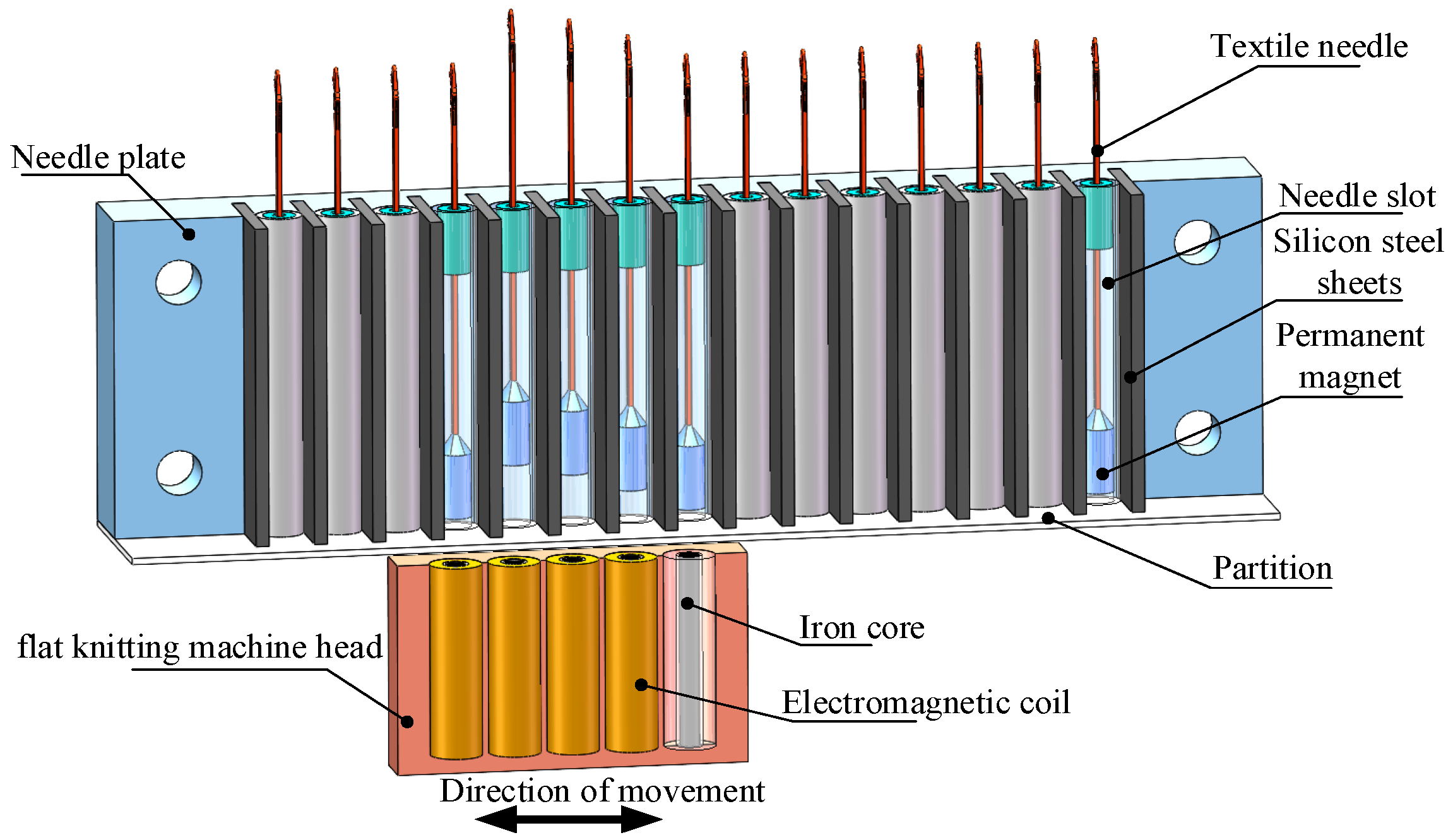
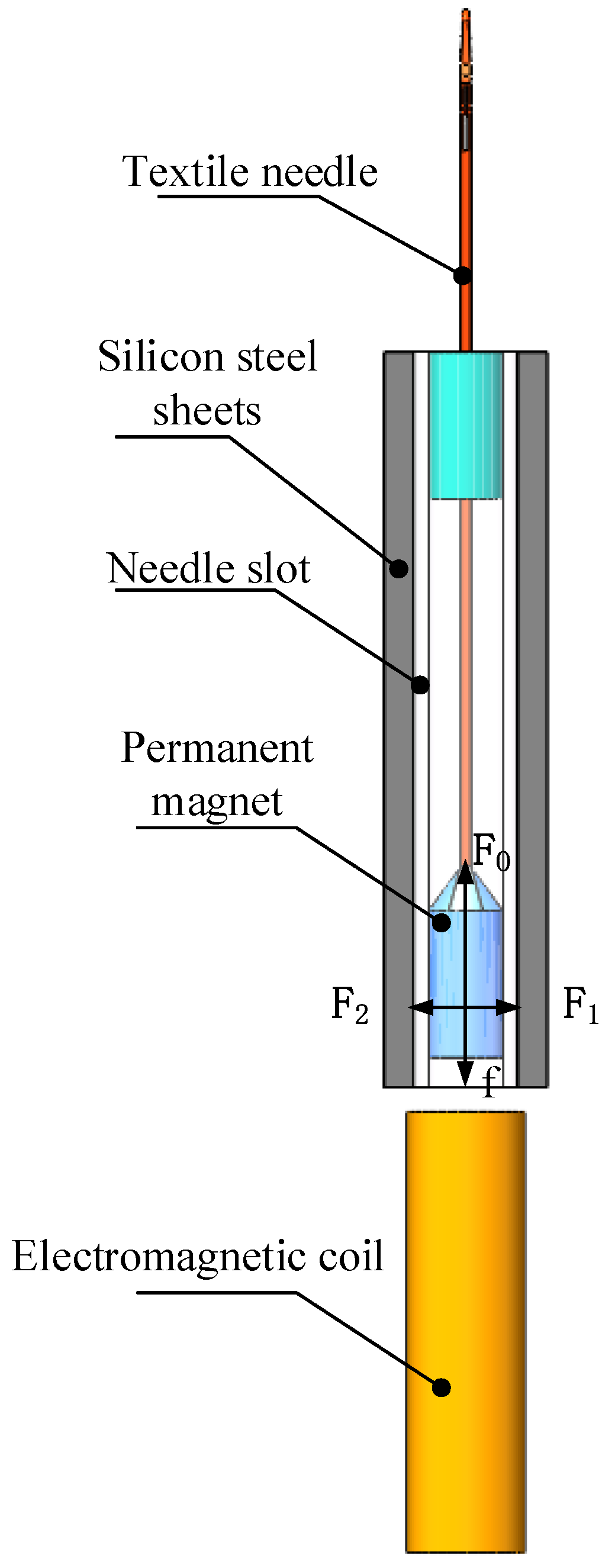
2. Driving Structure of Hybrid Maglev Needle
3. Performance Analysis of Hybrid Maglev Needle Drive
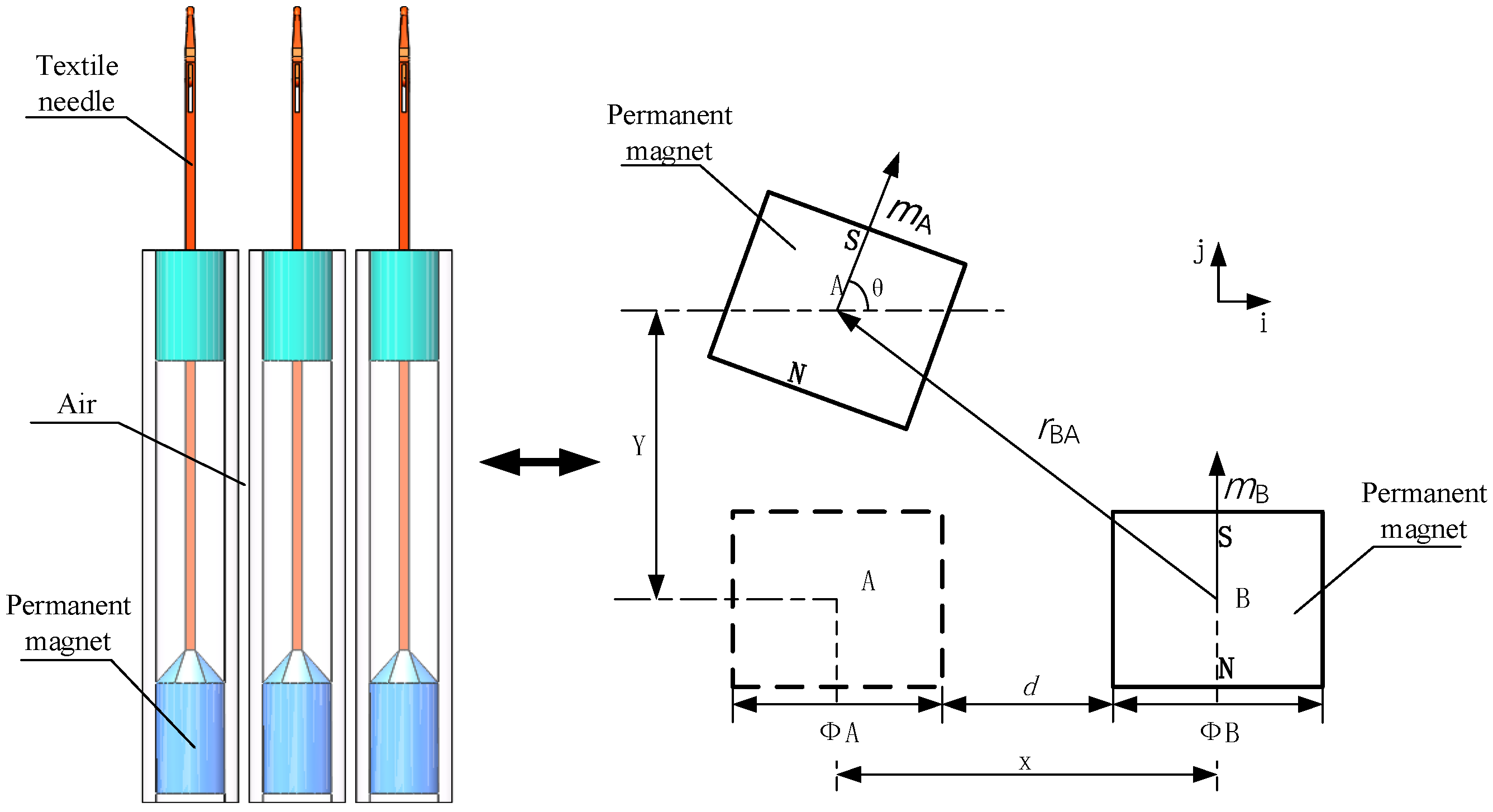
3.1. Mathematical Model of the Magnetic Force for Knitting Needle Gauge
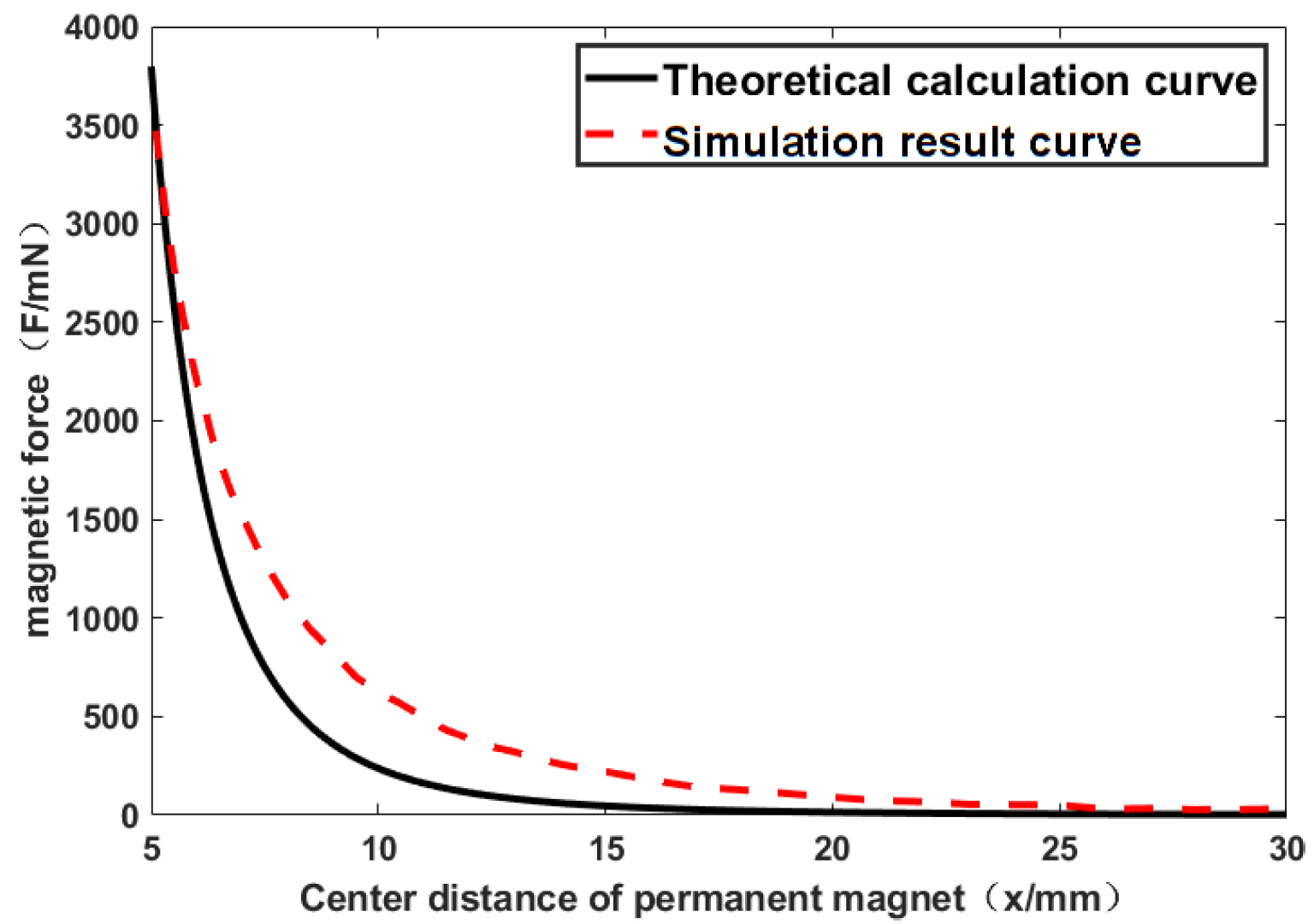
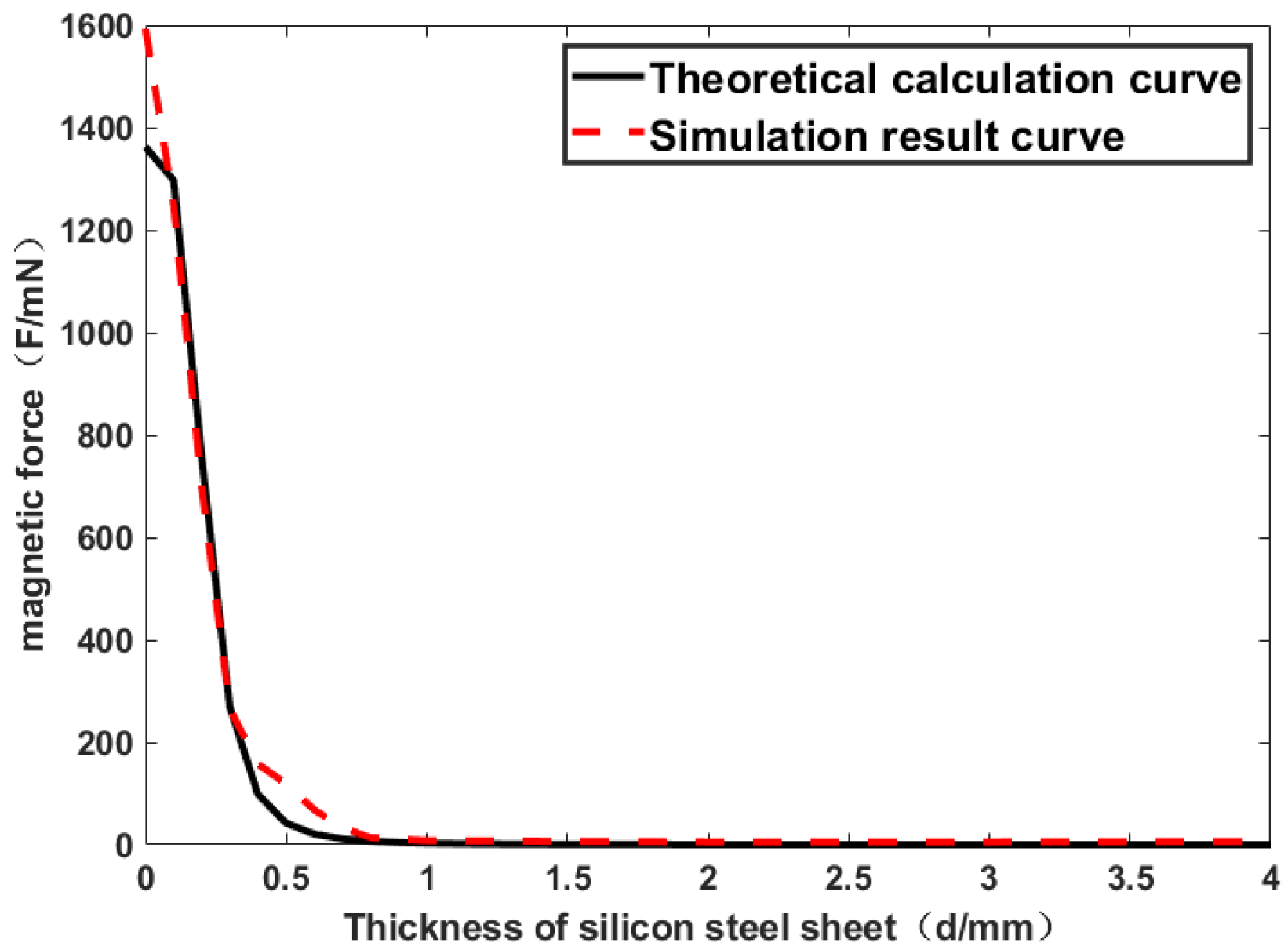
3.2. Validation of Mathematical Models
4. Optimization of Hybrid Maglev Needle Drive
4.1. Structural Optimization of Hybrid Maglev Needle Drive
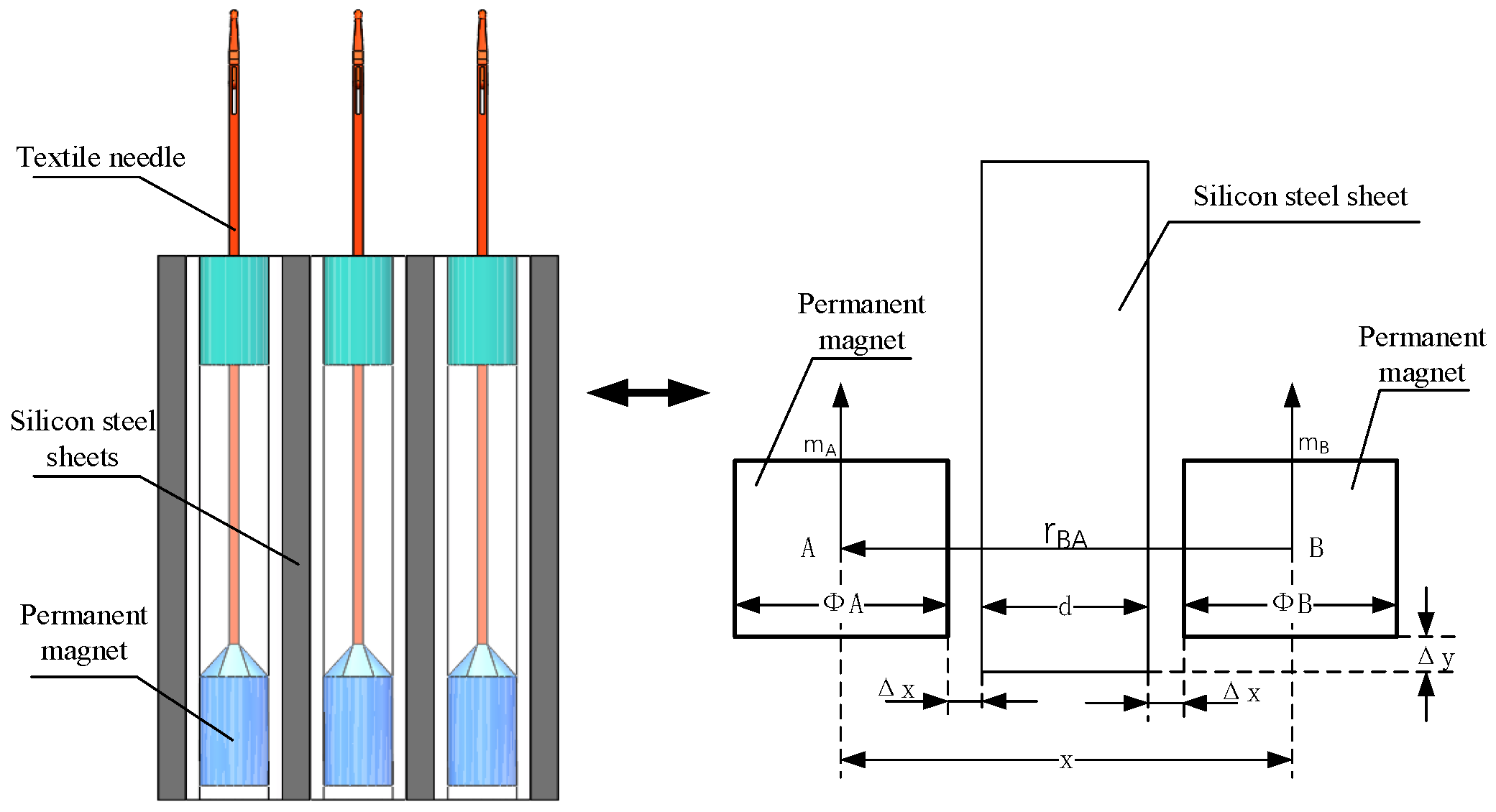
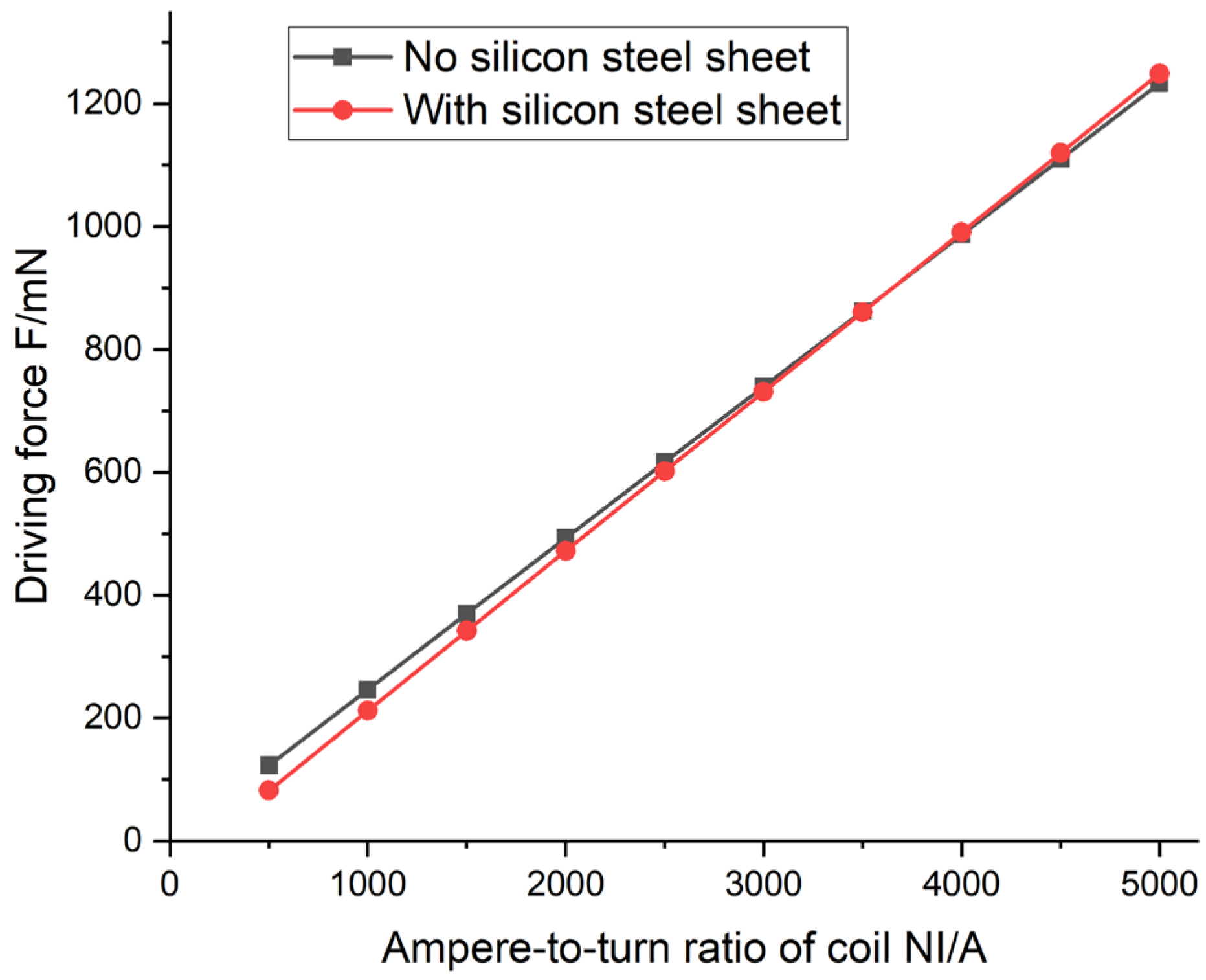
4.2. Magnetic Force Change of Knitting Needle Gauge with Silicon Steel Shield
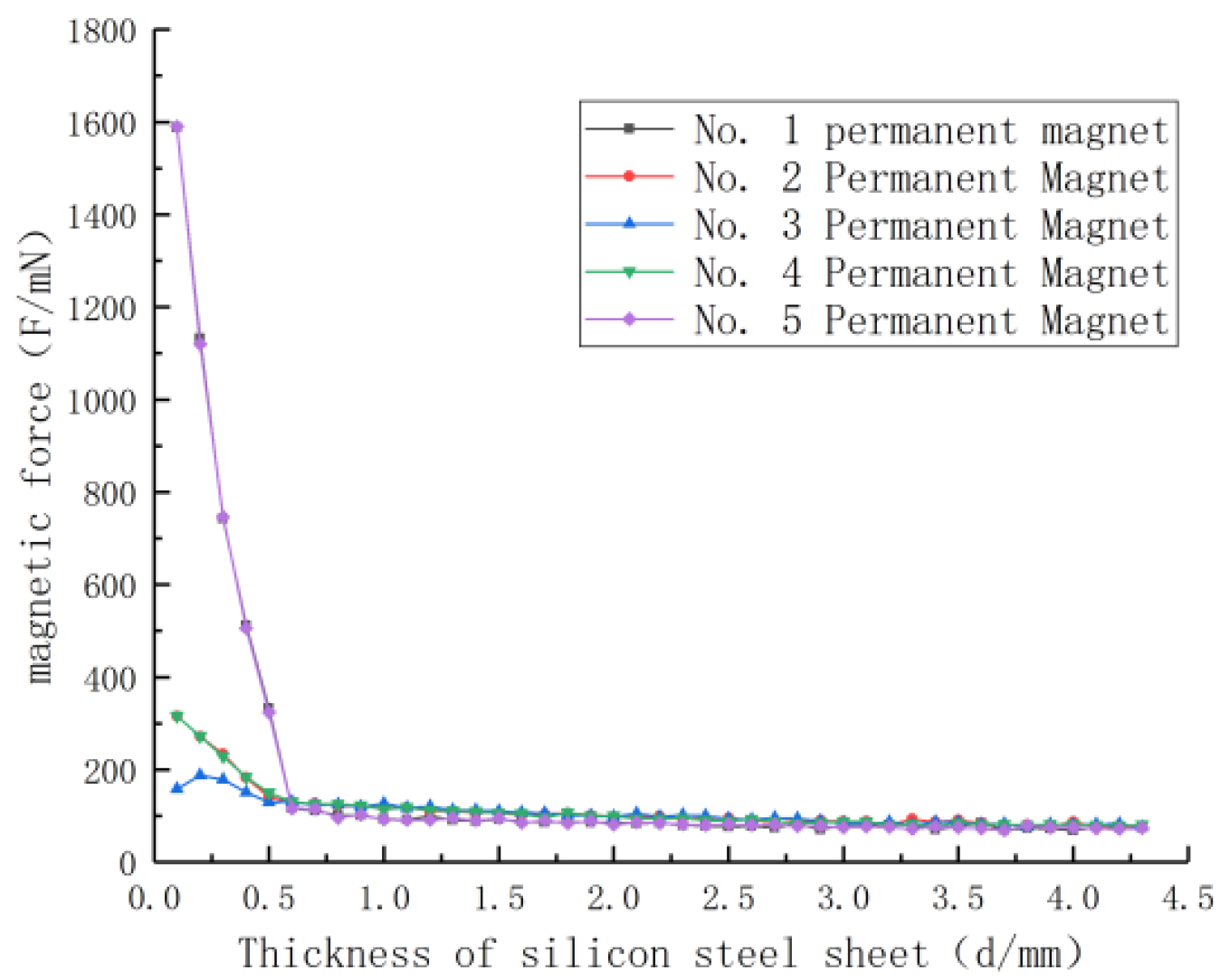
4.3. Analysis of Silicon Steel Thickness and Magnetic Field Shielding
5. Experimental Verification
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- He, Z.; Xu, J.; Tran, K.P.; Thomassey, S.; Zeng, X.; Yi, C. Modeling of textile manufacturing processes using intelligent techniques: A review. Int. J. Adv. Manuf. Technol. 2021, 116, 39–67. [Google Scholar] [CrossRef]
- Zhao, C.; Song, G.L.; Xu, L. Optimal Design of Computerized Flat Knitting Machine Cam Curves Based on UG and ANSYS/LS-DYNA. Appl. Mech. Mater. 2014, 529, 410–414. [Google Scholar] [CrossRef]
- Liu, Z.; Xu, G. Design and simulation of Lorentz force actuated maglev knitting needle actuator. J. Text. Res. 2021, 42, 159–165. [Google Scholar]
- Xiong, T.; Yin, W.; Li, D.; Zhang, C.; Zuo, X. Research on Electromagnetic Force of Hybrid Electromagnetic Levitation Needle Driving System. In Proceedings of the International Conference on Electronics, Electrical and Information Engineering 2021, ICEEIE 2021, Changsha, China, 13–16 August 2021; Volume 2136. [Google Scholar]
- Zuo, X.; Zhang, C.; Xiong, T.; Yin, W.; Li, M.; Zhang, C.; Wu, X.; Zhu, L. Maglev weft knitting needle driving modeling and PID controller design. J. Text. Inst. 2021, 113, 1715–1722. [Google Scholar] [CrossRef]
- Vokoun, D.; Beleggia, M. Forces between arrays of permanent magnets of basic geometric shapes. J. Magn. Magn. Mater. 2014, 350, 174–178. [Google Scholar] [CrossRef]
- Du, Z.; Zhan, T.; Ruan, J.; Huang, G.; Zhang, Y.; Yao, Y.; Liu, K.; Huang, D.; Guan, W. Research on Electromagnetic Performance Affected by Shielding Enclosure of a Coil Launcher. IEEE Trans. Plasma Sci. 2013, 41. [Google Scholar] [CrossRef]
- Singhasathein, A.; Pruksanubal, A. The Shielding Factor of the Steel Mesh for High-Intensity Magnetic Field Protection. In Proceedings of the 7th International Electrical Engineering Congress, iEECON 2019, Hua Hin, Thailand, 6–8 March 2019. [Google Scholar]
- Jagatheesan, K.; Ramasamy, A.; Das, A.; Basu, A. Electromagnetic shielding effectiveness of carbon/stainless steel/polypropylene hybrid yarn-based knitted fabrics and their composites. J. Text. Inst. 2018, 109, 1445–1457. [Google Scholar] [CrossRef]
- Nakashima, Y.; Kimura, T.; Sasada, I. Magnetic Field Leakage From a 45 -Angle Magnetic Shell and a Reduction Method for a High-Performance Magnetic Shield. IEEE Trans. Magn. 2006, 42, 3545–3547. [Google Scholar] [CrossRef]
- Campion, S.; Rueda, J.A.; Ruffini, R.; Xue, S.S. Magnetic field screening in strong crossed electromagnetic fields. Phys. Lett. B 2021, 820, 136562. [Google Scholar] [CrossRef]
- Ahmad, A.F.; Aziz, S.A.; Abbas, Z.; Obaiys, S.J.; Matori, K.A.; Zaid MH, M.; Raad, H.K.; Aliyu, U.S. Chemically Reduced Graphene Oxide-Reinforced Poly(Lactic Acid)/Poly(Ethylene Glycol) Nanocomposites: Preparation, Characterization, and Applications in Electromagnetic Interference Shielding. Polymers 2019, 11, 661. [Google Scholar] [CrossRef] [PubMed]
- Boyvat, M.; Hafner, C.V. Magnetic field shielding by metamaterials. Prog. Electromagn. Res. 2013, 136, 647–664. [Google Scholar] [CrossRef]
- Yin, Y.; Zhou, B.; Yin, K.; Tang, J.; Ning, X.; Han, B.; Fang, J. Comprehensive influence of modulated and bias magnetic fields on an atomic magnetometer. Meas. Sci. Technol. 2021, 32. [Google Scholar] [CrossRef]
- Kandasaamy, P.V.; Rameshkumar, M. Electromagnetic interference shielding effectiveness of sol-gel coating on Cu-plated fabrics. J. Text. Inst. 2021, 112, 855–863. [Google Scholar] [CrossRef]
- Camacho, J.M.; Sosa, V. Alternative method to calculate the magnetic field of permanent magnets with azimuthal symmetry. Rev. Mex. De Fis. E 2013, 59, 8–17. [Google Scholar]


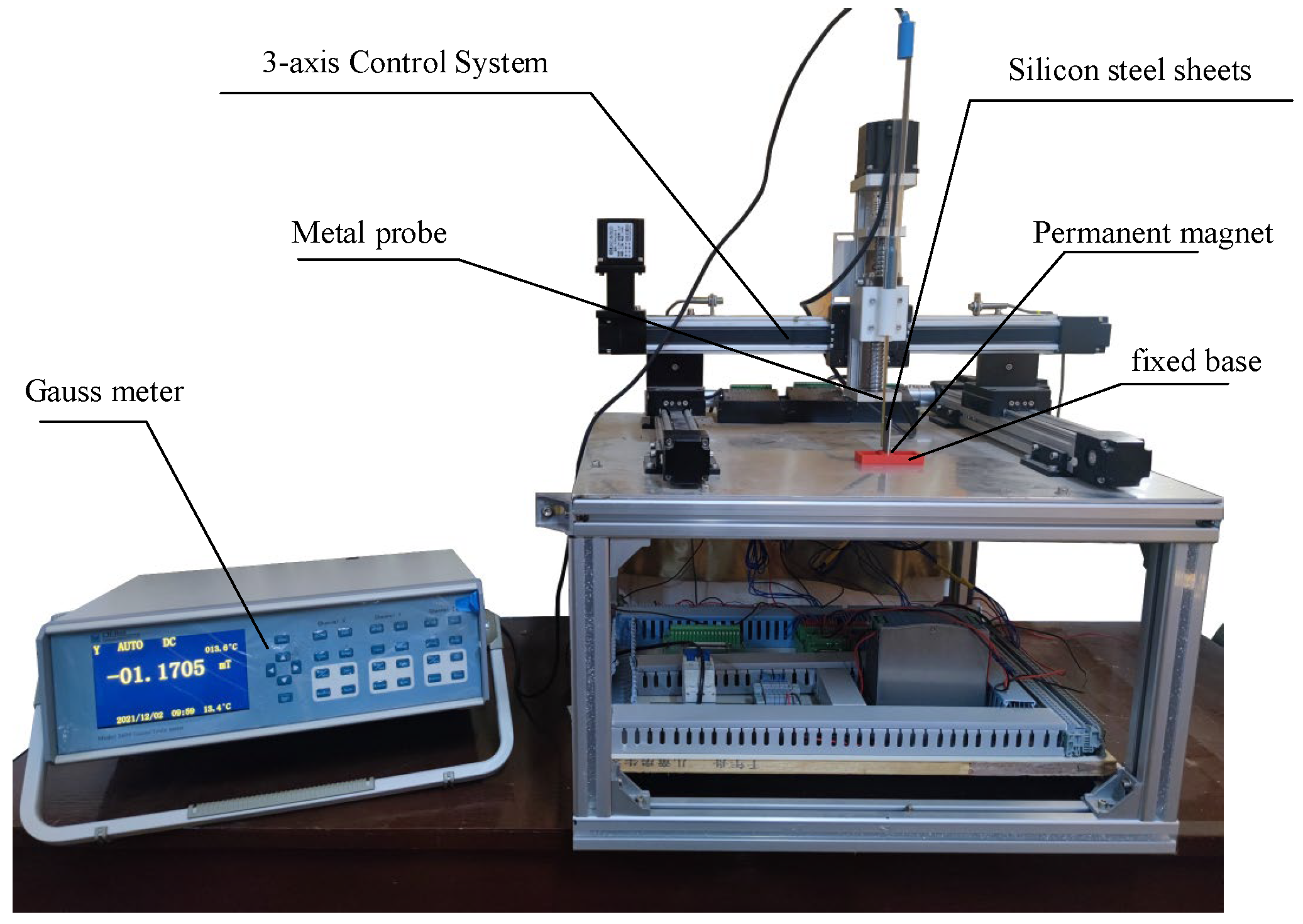
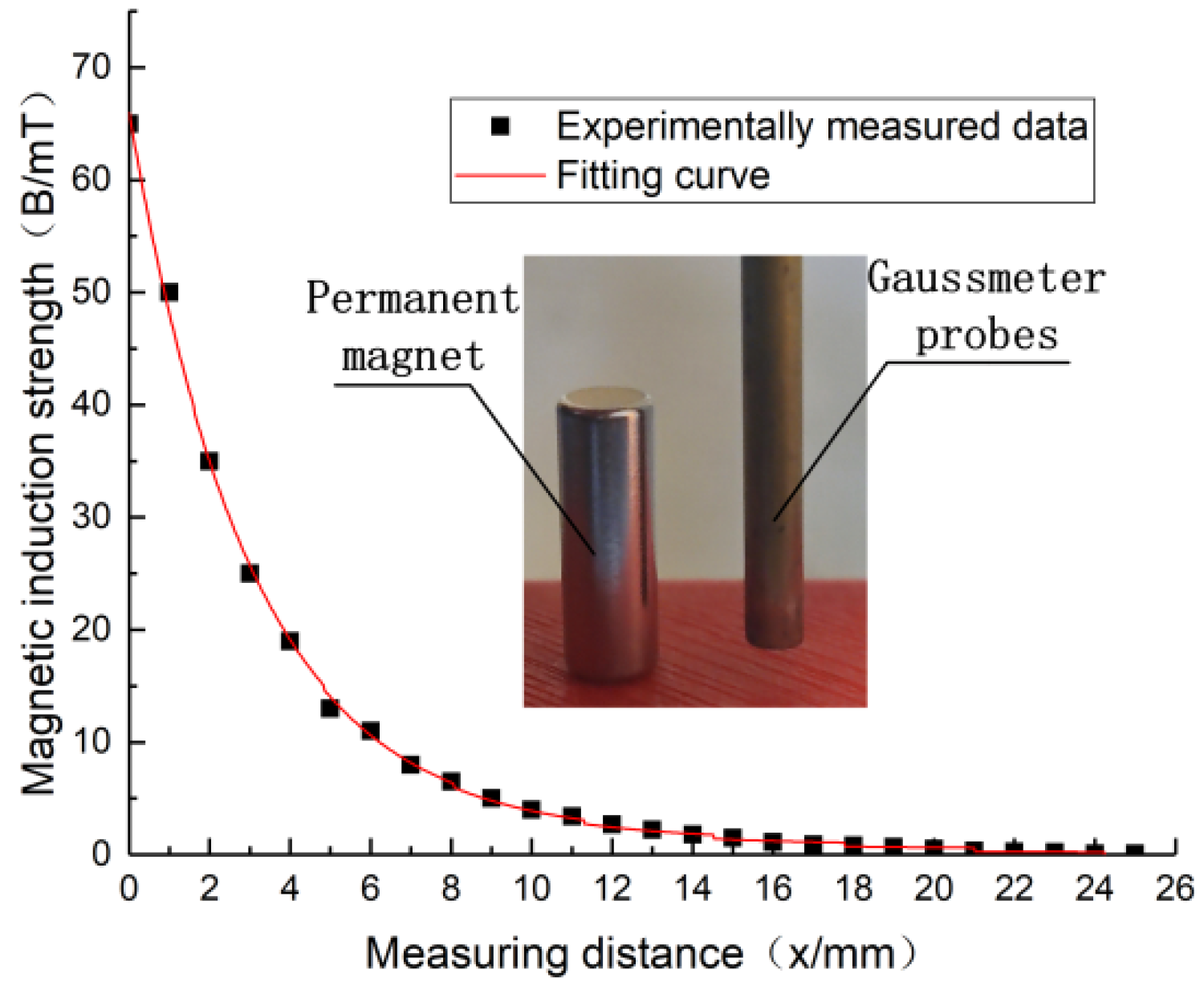


| Parameter | Value | Unit |
|---|---|---|
| Permanent magnet material | NdFe35 | |
| Permanent magnet shape | cylinder | |
| Relative permeability of permanent magnet | 1.0997 | |
| Bulk conductivity of permanent magnet | 6.25 × 105 | siemens/m |
| Young’s modulus of permanent magnet | 1.45 × 1011 | N/m2 |
| Permanent magnet diameter | 5 | mm |
| Permanent magnet height | 15 | mm |
| Residual magnetic induction/ | 1.15 | T |
| Vacuum permeability/ | 4π × 10−7 | N/A2 |
| Center distance of permanent magnet/x | 5–15 | mm |
| Silicon steel sheet material | DW310 | |
| Silicon steel sheet width | 20 | mm |
| Relative permeability of silicon steel/ | 8000 | |
| Silicon steel sheet thickness/d | 0–4 | mm |
| Thickness of needle slot/ | 1 | mm |
| Parameter | Value | Unit |
|---|---|---|
| Silicon steel sheet thickness/d | 1 | mm |
| Needle slot | 1 | mm |
| Coil material | copper | |
| Core material | Steel_1008 | |
| Core diameter | 2 | mm |
| Coil diameter | 10 | mm |
| Coil height | 50 | mm |
| Core height | 50 | mm |
| Ampere-to-turn ratio of coil | 500–5000 | A |
| Parameter | Value | Unit |
|---|---|---|
| Silicon steel sheet thickness/d | 0–4.3 | mm |
| Relative initial position/ | 2 | mm |
| Number of permanent magnet arrays | 5 | |
| Number of silicon steel arrays | 6 |
| Parameter | Before Optimization | After Optimization | Unit |
|---|---|---|---|
| Silicon steel sheet thickness/d | 0 | 1 | mm |
| Gauge of hybrid maglev needles | 8 | 8 | mm |
| Force on permanent magnet No. 1 | 1783 | 6 | mN |
| Force on permanent magnet No. 2 | 462 | 2 | mN |
| Force on permanent magnet No. 3 | 2 | 5 | mN |
| Force on permanent magnet No. 4 | 454 | 2 | mN |
| Force on permanent magnet No. 5 | 1786 | 2 | mN |
| Maximum difference of force | 1783 | 4 | mN |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xiong, T.; Peng, Y.; Zuo, X.; Zhang, C.; Zhang, C.; Zhang, L.; Li, H. Magnetic Field Analysis and Optimization of the Gauge of Hybrid Maglev Needles. Appl. Sci. 2023, 13, 1257. https://doi.org/10.3390/app13031257
Xiong T, Peng Y, Zuo X, Zhang C, Zhang C, Zhang L, Li H. Magnetic Field Analysis and Optimization of the Gauge of Hybrid Maglev Needles. Applied Sciences. 2023; 13(3):1257. https://doi.org/10.3390/app13031257
Chicago/Turabian StyleXiong, Tao, Yi Peng, Xiaoyan Zuo, Chengjun Zhang, Chi Zhang, Libin Zhang, and Hongjun Li. 2023. "Magnetic Field Analysis and Optimization of the Gauge of Hybrid Maglev Needles" Applied Sciences 13, no. 3: 1257. https://doi.org/10.3390/app13031257
APA StyleXiong, T., Peng, Y., Zuo, X., Zhang, C., Zhang, C., Zhang, L., & Li, H. (2023). Magnetic Field Analysis and Optimization of the Gauge of Hybrid Maglev Needles. Applied Sciences, 13(3), 1257. https://doi.org/10.3390/app13031257







