The Study of the Influence of the Tracing Speed on the Result of Surface Roughness Measurement Using the Tactile Method

Abstract

This work concerns the problem of measuring surface roughness using the tactile method. The result of such a measurement is influenced by many factors, for example, the applied measurement parameters or changes in ambient conditions, e.g., temperature. The authors attempted to determine the influence of the tracing speed of the result of the profilometer tip on the roughness measurement of surfaces characterized by different types of irregularities. In the experiment, samples manufactured using various machining techniques were measured. Eleven selected roughness parameters were observed, including the most well-known ones, such as Ra, Rz, or Rt. The results obtained by the authors allowed them to determine which of the analyzed parameters are the most sensitive to changes in the tracing speed of the measuring tip, which is of great importance in the aspect of surface roughness measurements in industrial conditions. Owing to the obtained results, it is possible to determine in which cases it is possible to increase the speed of tip travel without reducing the reliability of the obtained results of surface roughness measurement.

1. Introduction

Surface roughness measurements are a very important area of metrology of geometrical quantities. In general, surface roughness measurements can be divided into contactless and tactile [1,2,3]. Currently, a very dynamic development of contactless methods can be observed. The main reason is that contactless methods are faster than tactile methods. Furthermore, they allow the surface roughness measurement of materials susceptible to mechanical damage [4,5]. Nevertheless, contactless methods are also not without drawbacks. For example, after measurement, there are usually a large number of unmeasured points on the tested surface. They are also sensitive to external factors, such as possible contaminants in the environment in which measurement is carried out. For this reason, tactile instruments, despite their limitations, are still widely used in industry [6,7,8].

When measuring roughness using the tactile method, the measuring tip moves on the surface to be measured. Due to irregularities on the surface, the measuring tip vibrates. These vibrations are amplified and converted into a signal on the basis of which surface quality is assessed. This solution was inspired by observing the behavior of the turntable needle on the surface of a gramophone record during sound playback. The principle of using needle vibrations to assess surface roughness was probably first put into practice in 1919 by P. Tomlinson of the National Physics Laboratory (UK) [9]. Tactile profilometers can be divided according to the type of transducer used. The most common technical solutions are profilometers with inductive, piezoelectric, and laser interference transducers. The most accurate profilometers are those equipped with a laser interference transducer.

Several researchers are engaged in methods for assessing and predicting surface roughness from various points of view. These works concern, for example, predicting surface roughness based on the analysis of the machining parameters [10] or the impact of the parameters of various types of manufacturing technologies on the surface quality of the product [11,12].

As mentioned previously, intensive research on the measurement of roughness by non-contact methods is currently underway. He et al. in their paper [13] propose the use of image segmentation to measure the surface roughness of helical gears. Other optical methods used to measure surface roughness described in the scientific literature are the analysis of the colors of surface images [14] or multi-parameter modeling learning [15].

In the measurement of surface roughness using optical methods, techniques based on the phenomenon of light scattering on the measured surface are also used [16]. Another interesting method of analyzing the surface irregularities of products is the application of signal decomposition using a wavelet transform for this purpose [17,18,19,20].

Due to the development of optical methods, the question arises regarding their accuracy in relation to the traditional tactile method. Therefore, some researchers have conducted studies comparing the results of measuring the same surface using the tactile method and selected optical methods [21,22]. In the scientific literature, one can also find papers on the adaptation of AFM to nanoscale surface roughness in nanoscale [23]. Since surface irregularities are divided into classes: i.e., form deviations, waviness, and roughness, many research centers are working on the development of new methods for the filtration of measurement data [24,25]. Researchers are also investigating the influence of selected factors and parameters on the result of surface roughness measurement. For example, the paper [26] describes the results of research on the influence of measurement noise, sampling interval, and digital filtering methods.

One of the main parameters of surface roughness measurement using the tactile method is the tracing speed of the measuring tip. It is generally accepted that this speed should be as low as possible. However, contemporary industry often requires measurements to be as short as possible (especially in the case of in-process measurements). Nevertheless, a too-high tracing speed can lead to significant measurement errors. This is due to the risk of losing contact between the tip and the surface to be measured (a so-called “tip flight” phenomenon).

Taking into account this problem, the authors conducted research on the influence of the tracing speed of the measuring tip on the measurement results of the selected surface roughness parameters. Avrinth Davinci et al., in their paper [27], dealt with this problem, but the research was very limited. Firstly, the measurements were carried out on only one sample, which was the UKAS Reference Specimen 112/1534. Secondly, only a small range of parameters was observed, including Ra, Rq, and Rz for 2D measurements and their equivalents Sa, Sq, and Sz for 3D measurements.

This was the reason why the authors of this article conducted studies to assess the impact of the tracing speed for a large number of samples with different types of irregularities. In this work, 11 roughness parameters were observed describing various features. The main aim of this study was to prove that the effect of tracing speed is different for different surface roughness parameters. The detailed objective of this research was to determine which of the analyzed parameters are less sensitive and which are more sensitive to changes in the tip travel speed. Compared to other papers examining the influence of tracing speed on roughness measurement results, this article is distinguished primarily by a large number of tested surfaces and a large number of analyzed roughness parameters.

The research methodology is presented in the second section. The third section contains the results of the research. The fourth section presents a discussion of the results. The last section of this work contains a summary and final conclusions.

2. Materials and Methods

2.1. Experimental Setup

In order to investigate the influence of the tracing speed of the tip on the obtained measurement results in relation to the nature of the measured surface measurements of comparative roughness standards were carried out. For this purpose, a set of comparative roughness standards presented in Figure 1 was used along with the parameters summarized in

Table 1. Roughness parameters of standards used in the experiments.

Machining Type	Symbol of the Standard	Nominal Parameters
		Ra, μm	Rz, μm
Flat lapping	FL 0.05	0.05	0.55
	FL 0.1	0.1	1.0
	FL 0.2	0.2	1.6
Reaming	R 0.4	0.4	3.0
	R 0.8	0.8	6.0
	R 1.6	1.6	10.0
Grinding	G 0.05	0.05	0.55
	G 0.1	0.1	1.0
	G 0.2	0.2	1.6
	G 0.4	0.4	3.0
	G 0.8	0.8	6.0
	G 1.6	1.6	10.0
Horizontal milling	HM 0.4	0.4	2.5
	HM 0.8	0.8	4.0
	HM 1.6	1.6	8.0
	HM 3.2	3.2	16
	HM 6.3	6.3	32
	HM 12.5	12.5	50
Vertical milling	VM 0.4	0.4	2.5
	VM 0.8	0.8	4.0
	VM 1.6	1.6	8.0
	VM 3.2	3.2	16
	VM 6.3	6.3	32
	VM 12.5	12.5	50
Turning	T 0.4	0.4	2.5
	T 0.8	0.8	4.0
	T 1.6	1.6	8.0
	T 3.2	3.2	16
	T 6.3	6.3	32
	T 12.5	12.5	50

.

Measurements of the surface roughness of the standards were performed on the Taylor Hobson Form Talysurf PGI 1230 tactile profilometer (Taylor Hobson Ltd., Leicester, UK), shown in Figure 2.

The PGI 1230 tactile profilometer is a high-quality instrument for the analysis of the primary profile, waviness, and surface roughness on a micro and nano scale. The transducer in the PGI 1230 system is the so-called phase-grating interferometer.

The instrument table is made of granite and equipped with an active vibration isolation system. The operation of this system is based on the fact that when vibrations are detected, the system generates a damping wave. The damping wave combined with the vibration waves from the outside eliminates them. The instrument is equipped with a motorized table for measuring the surface topography of the samples. The table is positioned using an incremental scale with a resolution of 0.1 μm. The instrument is controlled using the appropriate software options or manually using a special control panel.

The measuring tip can move from point to point or along a previously defined track, usually along a straight line or along an arc. The lowest pressure value of the measuring head is 1 mN, and a constant pressure value is ensured by electronic control systems.

In the work [1], the measurement uncertainty of the instrument was estimated, which for the most commonly used amplitude parameters was 85 nm.

The instrument’s interference transducer provides a vertical tip displacement resolution of 0.8 nm in a range of 12.5 mm. The horizontal positioning accuracy of the tip is less than 1 μm. The maximum measurement length is 200 mm when the sampling interval is 0.125 μm. The maximum straightness deviation of the tip over a length of 200 mm is 0.2 μm. The instrument is placed in a specially designed cabin, which isolates the measuring system from contamination and weather influences.

The instrument’s control computer is equipped with Talymap Platinum ver. 6.2 software, utilized for measurements as well as the comprehensive analysis of the results obtained.

2.2. Experiment

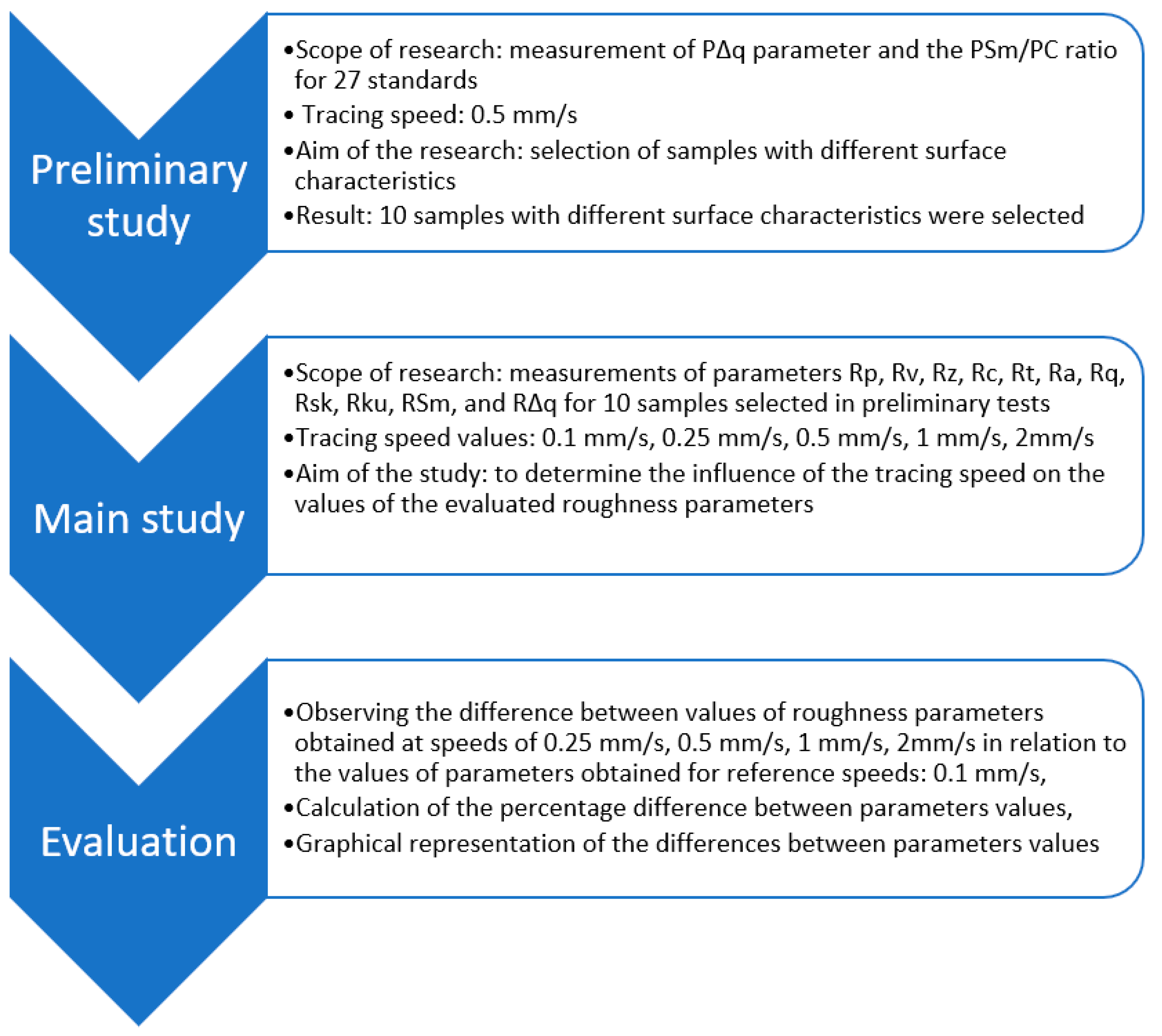
The experimental studies were divided into two stages: preliminary and main studies. Preliminary measurements were aimed at determining the nature of the irregularities of individual standards by determining the PΔq parameter and the PSm/PC ratio. Preliminary measurements were carried out on 27 of 30 standards, excluding three standards with a nominal value of Ra = 12.5 μm. They were conducted at a tip tracing speed of v = 0.5 mm/s. As a result of the analysis of the results obtained, standards were selected for further research covering the entire range of variation of the root mean square slope of the primary profile PΔq, and at the same time representing surfaces subjected to various types of machining.

The next stage of the research was to carry out measurements of selected standards with the following tracing speeds of the measuring tip: 0.1; 0.25; 0.5; 1.0; and 2.0 mm/s. Measurements were carried out within an area of X = 4 mm; Y = 0.1 mm with sampling intervals of ΔX = 0.125 μm and ΔY = 10 μm, i.e., each measurement contained 10 profiles. The following roughness parameters were observed: Rp, Rv, Rz, Rc, Rt, Ra, Rq, Rsk, Rku, RSm, and RΔq.

The flow chart of the experiment is shown in Figure 3.

Based on the measurements, the average values of the roughness parameters were calculated following the application of Gaussian filters with cut-off wavelengths of λs = 2.5 μm and λc = 0.8 mm for all measured standards, except those marked as HM 3.2, HM 1.6, and HM 6.3, for which Gaussian filters with cut-off wavelengths of λs = 2.5 μm and λc = 2.5 mm were used.

3. Results

As mentioned above, the first stage of experimental research was a preliminary study aimed at determining the character of the irregularities of measured standards. The results of these measurements are shown in Figure 4.

The primary profiles of the standards were characterized by values of the PΔq parameter in the range of 2.4 to 19.6°.

As a result of the analysis of the results obtained, standards were selected for further research, covering the entire range of variation of the root mean square slope of the primary profile PΔq, and at the same time representing surfaces subjected to various types of machining. The standards selected for further measurements are marked with appropriate symbols in Figure 4. The results obtained from the measurement of the selected standards are summarized in

Table 2. List of standards selected for further research.

Machining Type	Symbol of the Standard	PΔq, °	PSm, μm	Pc, μm	PSm/Pc
Horizontal milling	HM 3.2	2.4	2113	14.4	146
	HM 1.6	2.6	763	3.7	205
	HM 6.3	4.2	3659	37.6	97
Reaming	R 1.6	7.0	199	4.2	47
Vertical milling	VM 1.6	7.6	182	4.9	37
Flat lapping	FL 0.2	9.1	21	0.7	29
Turning	T0.8	9.8	68	3.0	23
Grinding	G 0.4	11.1	26	1.1	23
	G 0.8	14.6	42	2.3	18
	G 1.6	19.6	52	4.2	12

.

After the preliminary studies, the main part of the research was carried out. As previously mentioned, the standards selected for the study were measured using five tracing speeds, with the speed range ranging from 0.1 mm/s to 2 mm/s. The sampling intervals in the X and Y directions and the observed roughness parameters are given in the previous subsection. Ten measurements of each standard were taken to calculate the average value of each of the observed parameters.

To illustrate the magnitude of changes in individual parameters as a function of the tracing speed, colored markings according to the scale shown in Figure 5 were used.

Figure 6, Figure 7, Figure 8, Figure 9, Figure 10, Figure 11, Figure 12, Figure 13, Figure 14 and Figure 15 show the average values of the roughness parameters of individual standards for the reference value of the tracing speed of v = 0.1 mm/s and the percentage change in the parameter values for the remaining values of the tracing speed.

4. Discussion

The analysis of the results obtained shows that the parameter most sensitive to changes in the tracing speed, regardless of the nature of the measured surface, is the root mean square slope of the roughness profile RΔq. Its values increase with increasing tracing speed, reaching a change of more than 300% in relation to the reference speed of v = 0.1 mm/s in extreme cases.

It should also be noted that the parameter whose values decrease with increasing tracing speed in most cases is the mean spacing of the profile elements RSm.

The largest changes in the values of the roughness profile parameters in response to changes in the tracing speed were observed for the surface of the standard FL 0.2 surface (the surface after flat lapping) and G 0.4 surface (the surface after grinding). These are surfaces with a random profile, characterized by large values of slope angle of the profile peaks and valleys, small distances between profile elements, and at the same time, small values of the height of the grooves. Figure 16 shows the nature of the changes in the individual parameters of the roughness profile as a function of the tracing speed for standard G 0.4.

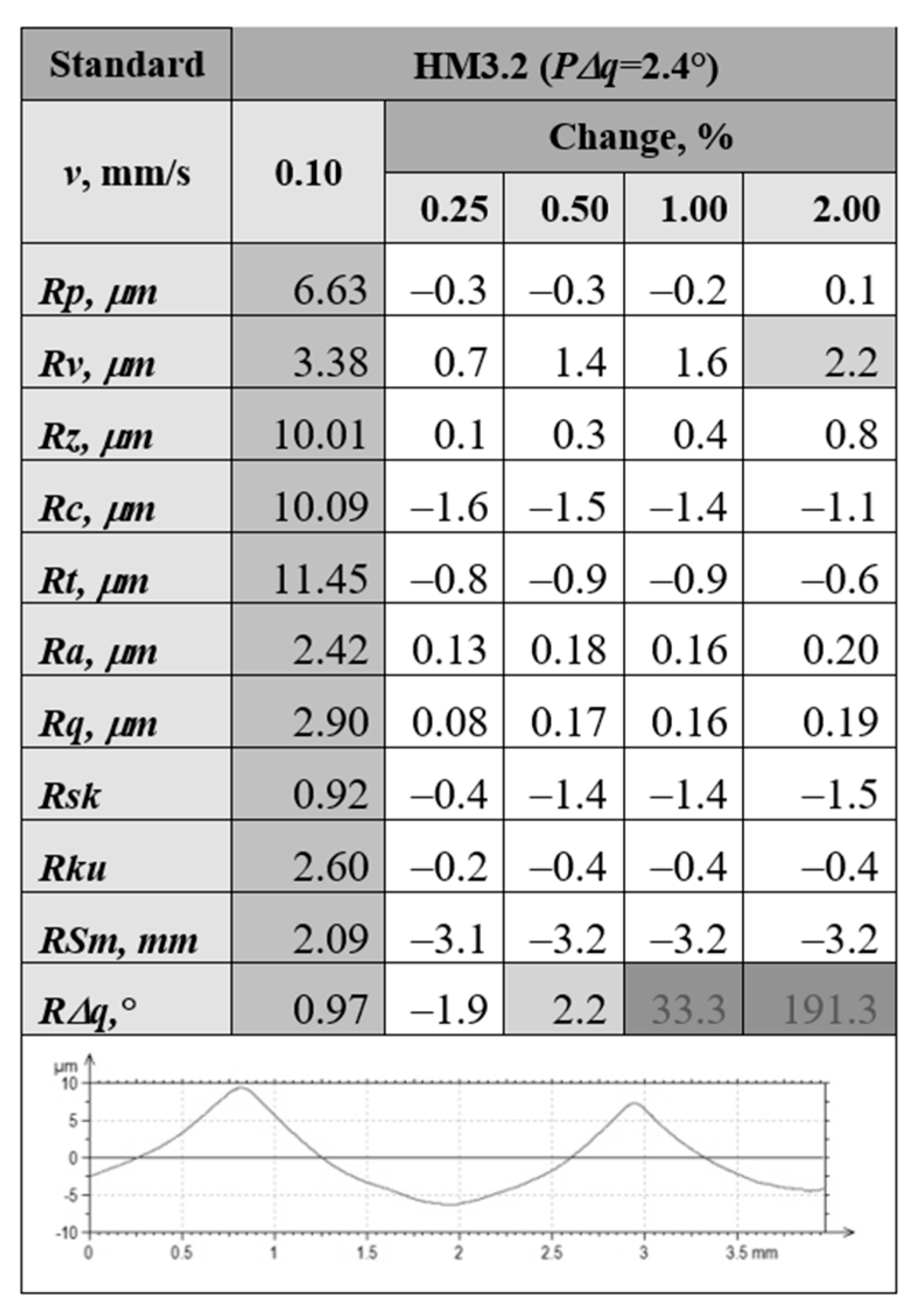
The smallest changes in the roughness profile parameters in response to changes in the tracing speed were observed for the surface of standard HM 3.2 (the surface after horizontal milling). This is a surface with a periodic profile characterized by small values of the slope angle, large distances between the profile elements, and at the same time, large height irregularity values. For this surface, the only significant changes were observed for the parameter RΔq. Figure 17 shows the nature of the changes in the individual parameters of the roughness profile as a function of the tracing speed for the HM 3.2 standard.

The comparison of profile fragments measured at tracing speeds of v = 0.25, 0.50, 1.0, and 2.0 mm/s with the profile measured at the reference value of a tracing speed of v = 0.10 mm/s for standard G 0.4 (the surface after grinding) is shown in Figure 18.

The red color shows the profile obtained for the reference tracing speed of v = 0.10 mm/s.

Figure 19 shows a fragment of the standard profile HM 3.2 (surface after horizontal milling) measured at different tracing speeds.

5. Conclusions

Despite the ongoing dynamic development of non-contact methods, tactile surface roughness measurements are still widely used both in scientific research and in industrial practice. One of the fundamental parameters of tactile measurement is the tracing speed of the measuring tip on the measured surface. In the case of a too-high tracing speed, the tip may lose physical contact with the measured surface, which results in very significant measurement errors. Therefore, contact instrument operators usually use low tracing speeds. Unfortunately, this is not beneficial considering the requirements of contemporary manufacturing processes, where it is often necessary to shorten the measurement time. This is why, in tactile measurements, the tracing speed should be selected in such a way that, on the one hand, it allows reliable results to be obtained and, on the other hand, it does not cause the unnecessary prolongation of the measurement time. The papers published so far described the influence of the tracing speed only for one selected surface and for a small number of roughness parameters. Therefore, the authors of this article decided to conduct research that would describe the influence of the tracing speed for various surfaces and many different roughness parameters.

The results presented in this article indicate that the influence of tracing speed on the measurement results of individual roughness parameters may differ significantly for surfaces of different natures.

• Surfaces engineered via horizontal milling.

For horizontally milled (HM) samples, it was found that the influence of the tracing speed on the obtained results is relatively small for most parameters, except for the RΔq and RSm. For the RSm parameter in the case of two standards, the error values of the parameter determination exceeded 2% for each speed higher than the reference speed. For the standard HM1.6, these values ranged from −3.1% to −3.2%. On the other hand, for the standard HM 6.3, the percentage error ranged from 2.8 to 3%. For the last of the HM standards (HM 1.6), there was no significant influence of the sensor speed on the measurement results of the RSm parameter. In the case of HM standards, another parameter that has been shown to be extremely sensitive to changes in tracing speed is RΔq. For each of the measured samples, the error values of the RΔq parameter were extremely large. Values ranged from 0–1.9% to 191% for the HM 3.2 sample, from 8.3% to 325.6% for the HM 1.6 sample, and from 1.1% to 63.3% for the HM 6.3 sample.

Other parameters turned out to be much less sensitive to changes in tracing speed. However, the results presented show that at the tracing speed of v = 2 mm/s, the percentage error value exceeds 2% for some parameters; in particular, the Rv parameter.

2.

The surface engineered via vertical milling.

In the case of this surface, it is noticeable that the parameter most sensitive to the change in tracing speed is RΔq. In this case, the value of the percentage error of the determination exceeded the assumed threshold of 2% at a tracing speed of $0.5 \mathrm{m}\mathrm{m}/\mathrm{s}$, while at $v=2 \mathrm{m}\mathrm{m}/\mathrm{s}$, the value of this error was as high as 89.5%. Other parameters turned out to be much less sensitive to the increase in tracing speed. However, it was shown that for the maximum speed of $v=2 \mathrm{m}\mathrm{m}/\mathrm{s}$, the error value exceeded the threshold of 2% for all parameters tested except Ra, Rq, and Rku.

3.

The surface engineered via reaming.

As in the case of the previous standard, the parameter most sensitive to the change in tracing speed for the surface engineered through reaming is RΔq. In the case of RΔq, the percentage error of the determination exceeded the assumed threshold of 2% at a speed of $v=0.5 \mathrm{m}\mathrm{m}/\mathrm{s}$, and at $v=2 \mathrm{m}\mathrm{m}/\mathrm{s}$, the error amounted to as much as 60.6%. Among the other parameters, the RSm parameter turned out to be the most sensitive to the increase in tracing speed. For RSm, the threshold value was exceeded at a speed of 1 mm/s, while at a speed of 2 mm/s, the determination error was −12%. Other parameters turned out to be much less sensitive to the increase in tracing speed. However, it was shown that at the maximum speed, the error value exceeded the threshold of 2% for all parameters, except Rp, Rz, Rc, and Rt.

4.

The surface engineered via flat lapping.

In the case of the surface engineered using flat lapping, the roughness parameters proved to be very sensitive to the increase in tracing speed. At a speed of $v=0.25 \mathrm{m}\mathrm{m}/\mathrm{s}$, the error of determining six parameters exceeded the threshold value. At a speed of $v=0.5 \mathrm{m}\mathrm{m}/\mathrm{s}$, the errors of only two parameters did not exceed 2%, and at $v=1 \mathrm{m}\mathrm{m}/\mathrm{s}$, only the RSm parameter remained within the allowed limits. At a speed of $v=2 \mathrm{m}\mathrm{m}/\mathrm{s}$, the error of each of the parameters exceeded the threshold value, in many cases very significantly. For example, for the Rp and RΔq parameters, the error was greater than 100%.

5.

The surface engineered via turning.

In the case of turned surface, the roughness parameters were found to be moderately sensitive to an increase in tracing speed. Errors in the determination of roughness parameters exceeded the threshold value of 2% at speed $v=0.5 \mathrm{m}\mathrm{m}/\mathrm{s}$ for two parameters: Rt and RΔq. At speed $v=1 \mathrm{m}\mathrm{m}/\mathrm{s}$ the threshold value was exceeded for five parameters. At speed $v=2 \mathrm{m}\mathrm{m}/\mathrm{s}$ The error of each of the parameters exceeded the threshold value. In the case of Rqd, the error was very significant and reached 71%.

6.

Surfaces engineered via grinding.

In the case of ground surfaces, the roughness parameters tend to be more sensitive to the increase in the tracing speed, particularly for surfaces with smaller irregularities. In the case of the G 0.4 standard, it was observed that at the speed of $v=0.25 \mathrm{m}\mathrm{m}/\mathrm{s}$, the error threshold for the Rsk parameter was exceeded. With an increase in speed to $v=0.5 \mathrm{m}\mathrm{m}/\mathrm{s}$, the threshold value was exceeded for six parameters, with nine parameters exceeded the threshold at $v=1 \mathrm{m}\mathrm{m}/\mathrm{s}$. At the maximum speed of $v=2 \mathrm{m}\mathrm{m}/\mathrm{s}$, errors of all parameters exceeded the threshold value, with some parameters exhibiting a very significant exceedance. For example, for Rp the error was 81.5%, and for RΔq, it was more than 116%.

In the case of two consecutive ground standards at a speed of $v=0.25 \mathrm{m}\mathrm{m}/\mathrm{s}$, the error threshold for one of the parameters was exceeded. With a further increase in speed to the value of $v=0.5 \mathrm{m}\mathrm{m}/\mathrm{s}$, the error rate exceeded 2% for three parameters in the case of the standard G 0.8, and for two parameters in the case of the standard G 1.6. At a speed of $v=1 \mathrm{m}\mathrm{m}/\mathrm{s},$ the threshold value was exceeded for five parameters for G 0.8 and six parameters for G 1.6. The maximum speed of $v=2 \mathrm{m}\mathrm{m}/\mathrm{s}$ resulted in all parameters exceeding the threshold value. The highest errors were obtained in the case of the Rp, RSm, and RΔq parameters. For RΔq, the errors exceeded 80%.

Summarizing the analysis of the results obtained for individual standards, it can be stated that the possibility of increasing the tracing speed in the roughness measurement using the tactile method depends on the shape of irregularities of the measured surface and what roughness parameters are to be observed. On the basis of the obtained results, one can conclude that:

• High values of the tracing speed, e.g., 1 mm/s or 1.5 mm/s, can be used for periodic profiles, such as milled and turned surfaces, when vertical and amplitude parameters are observed (e.g., Ra, Rq, etc.).
• For random surfaces, low values of the tracing speed should be used (0.1 mm/s or 0.25 mm/s), especially for smooth surfaces.
• If the object of interest is the RΔq parameter, we should avoid increasing the tracing speed, regardless of whether the surface is periodic or random.
• Care must also be taken with the RSm parameter when increasing the tracing speed. However, this parameter is much less sensitive to an increase in tracing speed than RΔq.

In the near future, the authors plan to perform another series of experiments on more samples and observe the effect of the tracing speed on more roughness parameters in order to analyze this problem with the use of statistical tools.