Application of Bonded-Block Models to Rock Failure Analysis
Abstract
:1. Introduction
2. Discrete Element Modeling of Rock Fracture
2.1. Bonded-Particle Models
2.2. Bonded-Block Models
3. Generation and Application of Bonded-Block Models
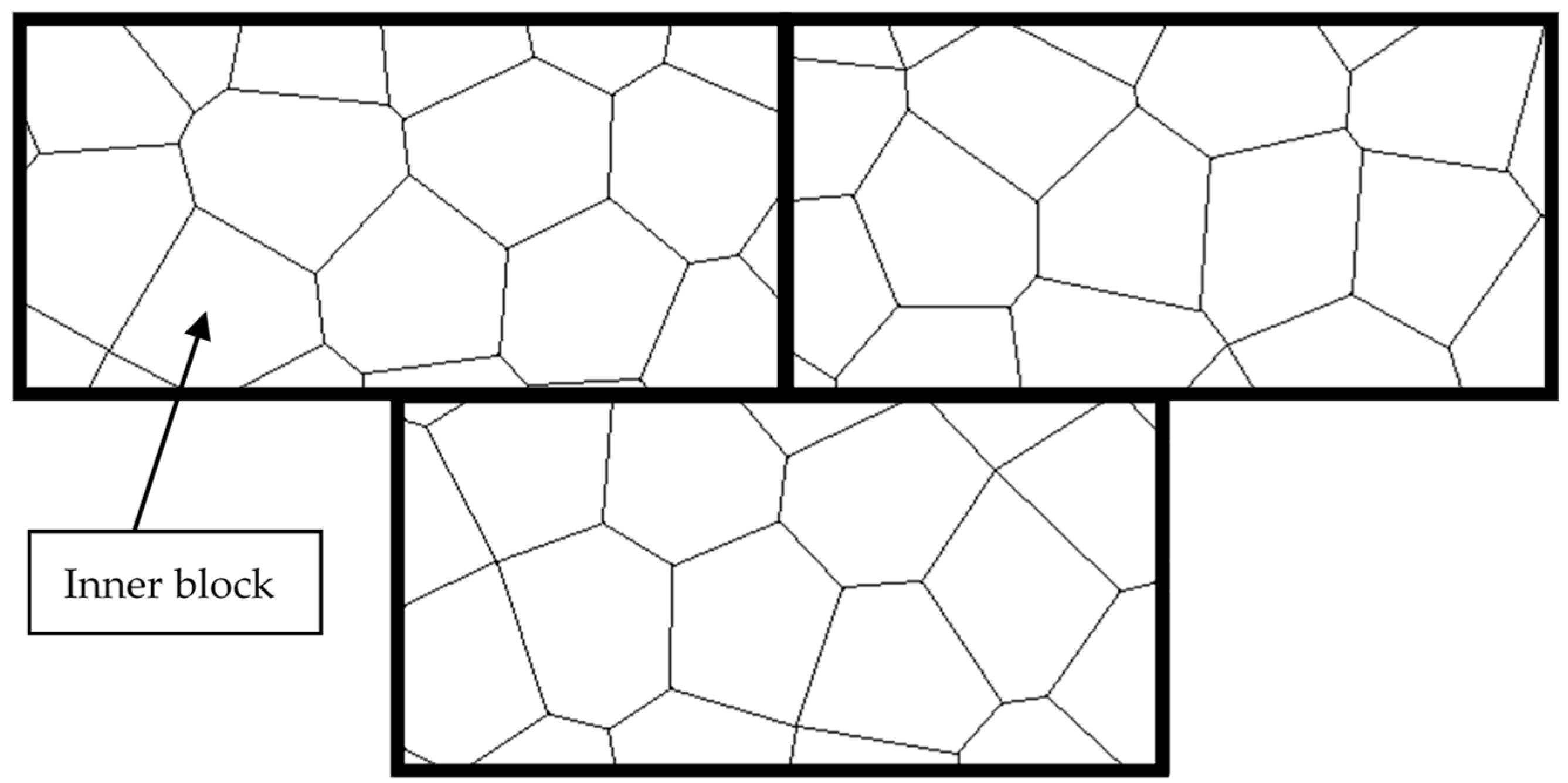
3.1. Modeling Assumptions and Block Patterns
3.2. Mechanical Micro- and Macro-Properties
3.3. Constitutive Model with Post-Peak Softening for Inner-Block Bonds
3.4. Solution Methods
4. Analysis of Underground Structures
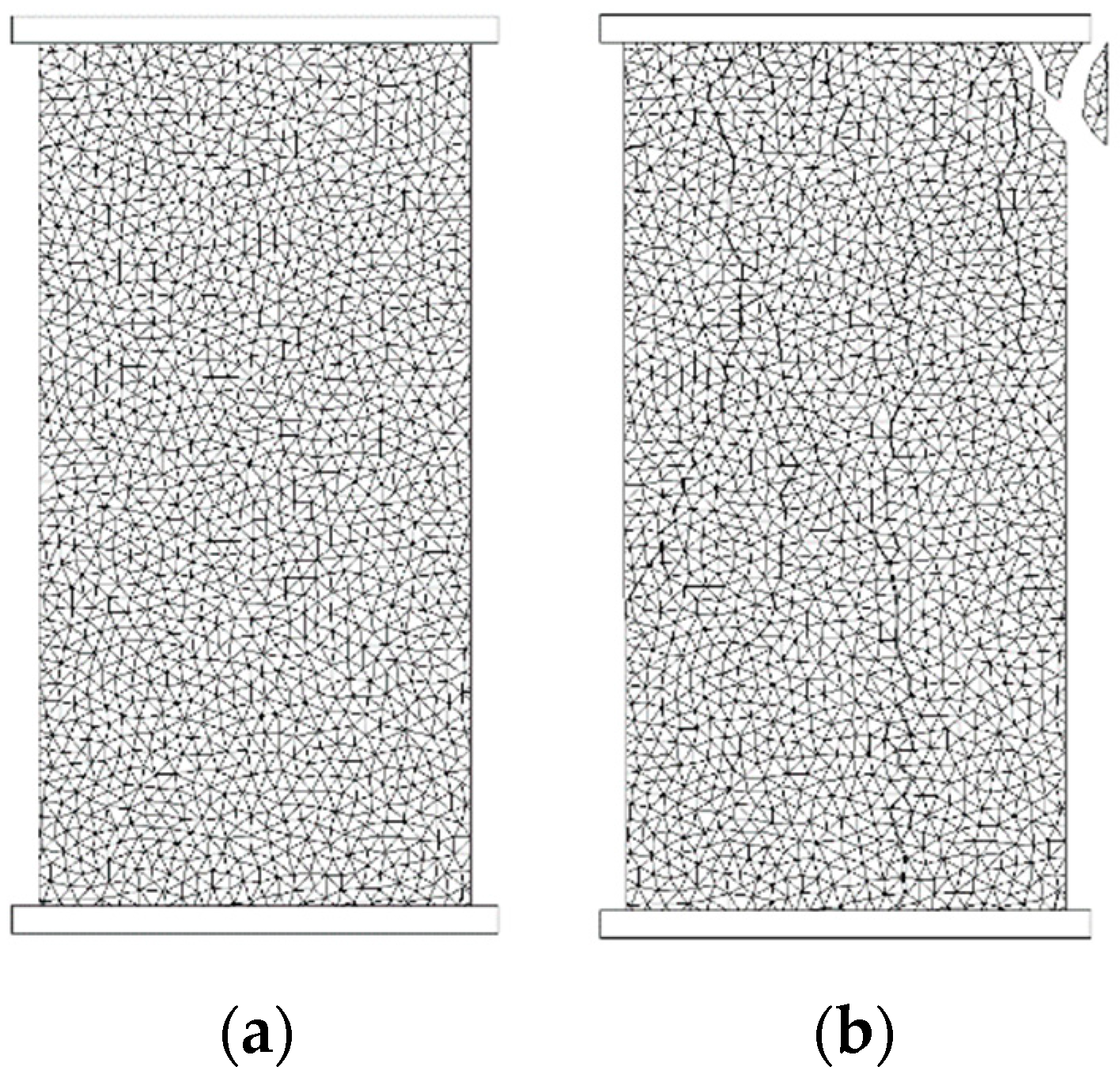
4.1. Example Problem
4.2. Selection of Micro-Parameters
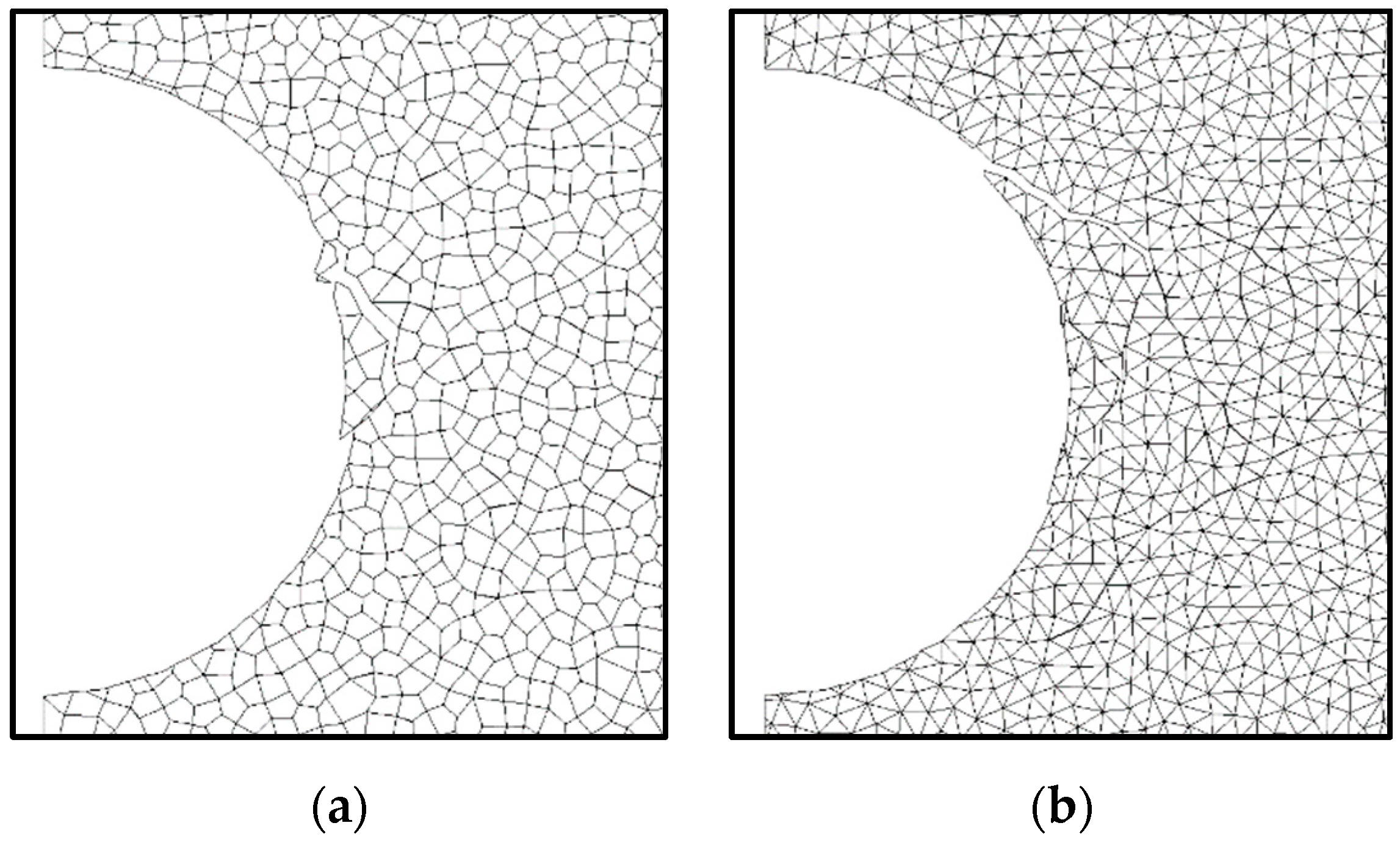
4.3. Underground Opening Model
5. Concluding Remarks
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Cundall, P.A. A computer model for simulating progressive large scale movements in blocky rock systems. In Proceedings of the Symposium Rock Fracture (ISRM), Nancy, France, 4–6 October 1971; Volume 1. Paper II-8. [Google Scholar]
- Cundall, P.A. A discontinuous future for numerical modelling in geomechanics? Proc. Inst. Civ. Eng.-Geotech. Eng. 2001, 149, 41–47. [Google Scholar] [CrossRef]
- Potyondy, D.O.; Cundall, P.A. A bonded-particle model for rock. Int. J. Rock Mech. Min. Sci. 2004, 41, 1329–1364. [Google Scholar] [CrossRef]
- Mas Ivars, D.; Pierce, M.E.; Darcel, C.; Reyes-Montes, J.; Potyondy, D.O.; Young, R.P.; Cundall, P.A. The synthetic rock mass approach for jointed rock mass modelling. Int. J. Rock Mech. Min. Sci. 2011, 48, 219–244. [Google Scholar] [CrossRef]
- Lan, H.; Martin, C.D.; Hu, B. Effect of heterogeneity of brittle rock on micromechanical extensile behavior during compression loading. J. Geophys. Res. 2010, 115, B01202. [Google Scholar] [CrossRef]
- Garza-Cruz, T.V.; Pierce, M. A 3DEC Model for Heavily Veined Massive Rock Masses. In Proceedings of the 48th US Rock Mechanics/Geomechanics Symposium, Minneapolis, MN, USA, 1–4 June 2014; American Rock Mechanics Association: Alexandria, VA, USA, 2014; pp. 14–7660. [Google Scholar]
- Lisjak, A.; Grasselli, G. A review of discrete modeling techniques for fracturing processes in discontinuous rock masses. J. Rock Mech. Geotech. Eng. 2014, 6, 301–314. [Google Scholar] [CrossRef]
- Lemos, J.V. Rock failure analysis with discrete elements. In Numerical Methods in Geotechnical Engineering IX; Cardoso, A., Borges, J., Costa, P., Gomes, A., Marques, J., Vieira, C., Eds.; Taylor & Francis: London, UK, 2018; Volume 1, pp. 13–22. [Google Scholar]
- Yoon, J.S.; Hazzard, J. Coupled Fracture Modelling with Distinct Element Methods. In Modelling Rock Fracturing Processes Theories, Methods and Applications; Shen, B., Stephansson, O., Rinne, M., Eds.; Springer: Berlin/Heidelberg, Germany, 2020; pp. 227–254. [Google Scholar]
- Li, Y.; Zhao, G.; Jiao, Y.; Yan, G.; Wang, X.; Shen, L.; Yang, L.; Liang, Z.; Li, W.; Zhou, X.; et al. A benchmark study of different numerical methods for predicting rock failure. Int. J. Rock Mech. Min. Sci. 2023, 166, 105381. [Google Scholar] [CrossRef]
- Cho, N.; Martin, C.D.; Sego, D.C. A clumped particle model for rock. Int. J. Rock Mech. Min. Sci. 2007, 44, 997–1010. [Google Scholar] [CrossRef]
- Yoon, J.S.; Jeon, S.; Zang, A.; Stephansson, O. Bonded particle model simulation of laboratory rock tests for granite using particle clumping and contact unbonding. In Continuum and Distinct Element Numerical Modeling in Geomechanics—2011; Sainsbury, D., Hart, R., Detournay, C., Nelson, M., Eds.; Itasca: Minneapolis, MN, USA, 2011; Paper 08-05. [Google Scholar]
- Scholtès, L.; Donzé, F.-V. A DEM model for soft and hard rocks: Role of grain interlocking on strength. J. Mech. Phys. Solids 2013, 61, 352–369. [Google Scholar] [CrossRef]
- Potyondy, D.O. The bonded-particle model as a tool for rock mechanics research and application: Current trends and future directions. Geosyst. Eng. 2014, 17, 342–369. [Google Scholar] [CrossRef]
- Ma, Y.; Huang, H. DEM analysis of failure mechanisms in the intact Brazilian test. Int. J. Rock Mech. Min. Sci. 2018, 102, 109–119. [Google Scholar] [CrossRef]
- Azevedo, N.M.; Candeias, M.; Gouveia, F. A Rigid Particle Model for Rock Fracture Following the Voronoi Tessellation of the Grain Structure: Formulation and Validation. Rock Mech. Rock Eng. 2015, 48, 535–557. [Google Scholar] [CrossRef]
- Potyondy, D.O.; Ivars, D.M. Simulating spalling with a flat-jointed material. In Applied Numerical Modeling in Geomechanics—2020; Billaux, D., Hazzard, J., Nelson, M., Schöpfer, M., Eds.; Itasca: Minneapolis, MN, USA, 2020; Paper 03-01. [Google Scholar]
- Brady, B.H.G.; Lemos, J.V.; Cundall, P.A. Stress measurement schemes for jointed and fractured rock. In Proceedings of the International Symposium in Rock Stress and Rock Stress Measurements, Stockholm, Sweden, 1–3 September 1986; Centec Publisher: Lulea, Sweden; pp. 167–176. [Google Scholar]
- Barla, G.; Bonini, M.; Cammarata, G. Stress and seepage analyses for a gravity dam on a jointed granitic rock mass. In Numerical Modeling of Discrete Materials in Geotechnical Engineering; Konietzky, H., Ed.; Balkema: Rotterdam, The Netherlands, 2004; pp. 263–268. [Google Scholar]
- Lemos, J.V. Representation of rock discontinuities in safety analysis of large dams. In Rock Engineering and Rock Mechanics: Structures in and on Rock Masses; Alejano, R., Perucho, Á., Olalla, C., Jiménez, R., Eds.; Taylor & Francis Group: London, UK, 2014; pp. 29–38. [Google Scholar]
- Alejano, L.R.; Ferrero, A.M.; Ramirez-Oyanguren, P.; Fernandez, M.I.A. Comparison of limit-equilibrium, numerical and physical models of wall slope stability. Int. J. Rock Mech. Min. Sci. 2011, 48, 16–26. [Google Scholar] [CrossRef]
- Brideau, M.-A.; Stead, D. Controls on Block Toppling Using a Three-Dimensional Distinct Element Approach. Rock Mech. Rock Eng. 2010, 43, 241–260. [Google Scholar] [CrossRef]
- Christianson, M.; Board, M.; Rigby, D. UDEC simulation of triaxial testing of lithophysal tuff. In Proceedings of the 41st US Symposium on Rock Mechanics, Golden, CO, USA, 17–21 June 2006; American Rock Mechanics Association: Alexandria, VA, USA, 2006. [Google Scholar]
- Damjanac, B.; Board, M.; Lin, M.; Kicker, D.; Leem, J. Mechanical degradation of emplacement drifts at Yucca Mountain—A modeling case study. Part II: Lithophysal rock. Int. J. Rock Mech. Min. Sci. 2007, 44, 368–399. [Google Scholar] [CrossRef]
- Damjanac, B.; Fairhurst, C. Evidence for a Long-Term Strength Threshold in Crystalline Rock. Rock Mech. Rock Eng. 2010, 43, 513–531. [Google Scholar] [CrossRef]
- Herbst, M.; Konietzky, H.; Walter, K. 3D microstructural modeling. In Continuum and Distinct Element Numerical Modeling in Geo-Engineering; Hart, R., Detournay, C., Cundall, P., Eds.; Itasca: Minneapolis, MN, USA, 2008; pp. 435–441. [Google Scholar]
- Kazerani, T.; Zhao, J. Micromechanical parameters in bonded particle method for modeling of brittle material failure. Int. J. Num. Analyt. Meth. Geomech. 2010, 34, 1877–1895. [Google Scholar] [CrossRef]
- Chen, W.; Konietzky, H.; Tan, X.; Frühwirt, T. Pre-failure damage analysis for brittle rocks under triaxial compression. Comput. Geotech. 2016, 74, 45–55. [Google Scholar] [CrossRef]
- Xu, T.; Fu, T.; Heap, M.; Meredith, P.; Mitchell, T.; Baud, P. Mesoscopic Damage and Fracturing of Heterogeneous Brittle Rocks Based on Three-dimensional Polycrystalline Discrete Element Method. Rock Mech. Rock Eng. 2020, 53, 5389–5409. [Google Scholar] [CrossRef]
- Wang, F.; Konietzky, H.; Herbst, M.; Chen, W. Mechanical responses of grain-based models considering different crystallographic spatial distributions to simulate heterogeneous rocks under loading. Int. J. Rock Mech. Min. Sci. 2022, 151, 105036. [Google Scholar] [CrossRef]
- Lorig, L.; Potyondy, D.; Varun, J. Quantifying excavation-induced rock mass damage in large open pits. In Slope Stability 2020; Dight, P.M., Ed.; Australian Centre for Geomechanics: Perth, Australia, 2020. [Google Scholar]
- Ghazvinian, E.; Diederichs, M.S.; Quey, R. 3D random Voronoi grain-based models for simulation of brittle rock damage and fabric-guided micro-fracturing. J. Rock Mech. Geotech. Eng. 2014, 6, 506–521. [Google Scholar] [CrossRef]
- Müller, C.; Frühwirt, T.; Haase, D.; Schlegel, R.; Konietzky, H. Modeling deformation and damage of rock salt using the discrete element method. Int. J. Rock Mech. Min. Sci. 2018, 103, 230–241. [Google Scholar] [CrossRef]
- Kazerani, T.; Yang, Z.Y.; Zhao, J. A Discrete Element Model for Predicting Shear Strength and Degradation of Rock Joint by Using Compressive and Tensile Test Data. Rock Mech. Rock Eng. 2012, 45, 695–709. [Google Scholar] [CrossRef]
- Gao, F.Q.; Stead, D. The application of a modified Voronoi logic to brittle fracture modelling at the laboratory and field scale. Int. J. Rock Mech. Min. Sci. 2014, 68, 1–14. [Google Scholar] [CrossRef]
- Munjiza, A. The Combined Finite-Discrete Element Method; John Wiley & Sons Ltd.: Chichester, UK, 2004. [Google Scholar]
- Lisjak, A.; Grasselli, G.; Vietor, T. Continuum-discontinuum analysis of failure mechanisms around un-supported circular excavations in anisotropic clay shales. Int. J. Rock Mech. Min. Sci. 2014, 65, 96–115. [Google Scholar] [CrossRef]
- Rasmussen, L.L.; Min, K.-B. Developments to the Bonded Block Modeling technique for Discrete Element, simulation of transversely isotropic rock. Int. J. Rock Mech. Min. Sci. 2023, 170, 105518. [Google Scholar] [CrossRef]
- Shen, B.; Barton, N. Rock fracturing mechanisms around underground openings. Geomech. Eng. 2018, 16, 35–47. [Google Scholar]
- Eberhardt, E.; Stead, D.; Coggan, J.S. Numerical analysis of initiation and progressive failure in natural rock slopes—The 1991 Randa rockslide. Int. J. Rock Mech. Min. Sci. 2004, 41, 69–87. [Google Scholar] [CrossRef]
- Shao, L.; Mao, J.; Zhao, L.; Li, T. A three-dimensional deformable spheropolyhedral-based discrete element method for simulation of the whole fracture process. Eng. Fract. Mech. 2022, 263, 108290. [Google Scholar] [CrossRef]
- Chen, W.; Konietzky, H.; Abbas, S.M. Numerical simulation of time-independent and -dependent fracturing in sandstone. Eng. Geol. 2015, 193, 118–131. [Google Scholar] [CrossRef]
- Lisjak, A.; Kaifosh, P.; He, L.; Tatone, B.S.A.; Mahabadi, O.K.; Grasselli, G. A 2D, fully-coupled, hydro-mechanical, FDEM formulation for modelling fracturing processes in discontinuous, porous rock masses. Comput. Geotech. 2017, 81, 1–18. [Google Scholar] [CrossRef]
- Du, J.; Huang, X.; Yang, G.; Xue, L.; Wu, B.; Zang, M.; Zhang, X. UDEC Modelling on Dynamic Response of Rock Masses with Joint Stiffness Weakening Attributed to Particle Crushing of Granular Fillings. Rock Mech. Rock Eng. 2023, 56, 1823–1841. [Google Scholar] [CrossRef]
- Wang, F.; Konietzky, H.; Pang, R.; Zou, Y.; Pang, B.; Ismael, M. Grain-Based Discrete Element Modeling of Thermo-Mechanical Response of Granite under Temperature. Rock Mech. Rock Eng. 2023, 56, 5009–5027. [Google Scholar] [CrossRef]
- Radakovic-Guzina, Z.; Damjanac, B.; Lam, T.; Kasani, H.A. DEM-Based Methodology for Simulation of Long-Term Geomechanical Performance of a Placement Room in a Deep Geological Repository. Rock Mech. Rock Eng. 2022, 56, 2737–2761. [Google Scholar] [CrossRef]
- Lisjak, A.; Mahabadi, O.; Ha, J.; Mas Ivars, D. Analysis of thermo-mechanical damage around tunnel and deposition boreholes of an underground nuclear waste disposal facility at the Forsmark site (Sweden) by 3D coupled FDEM simulations. Int. J. Rock Mech. Min. Sci. 2023, 171, 105586. [Google Scholar] [CrossRef]
- Garza-Cruz, T.; Bouzeran, L.; Pierce, M.; Jalbout, A.; Ruest, M. Evaluation of ground support design at Eleonore Mine via Bonded Block Modelling. In Ground Support 2019: Proceedings of the Ninth International Symposium on Ground Support in Mining and Underground Construction; Hadjigeorgiou, J., Hudyma, M., Eds.; Australian Centre for Geomechanics: Perth, Australia, 2019; pp. 341–356. [Google Scholar]
- Lavoie, T.; Eberhardt, E.; Pierce, M.E. Numerical modelling of rock mass bulking and geometric dilation using a bonded block modelling approach to assist in support design for deep mining pillars. Int. J. Rock Mech. Min. Sci. 2022, 156, 105145. [Google Scholar] [CrossRef]
- Keneti, A.; Pouragha, M.; Sainsbury, B.-A. Review of design parameters for discontinuous numerical modelling of excavations in the Hawkesbury Sandstone. Eng. Geol. 2021, 288, 106158. [Google Scholar] [CrossRef]
- Sinha, S.; Walton, G. Modeling the behavior of a coal pillar rib using Bonded Block Models with emphasis on ground-support interaction. Int. J. Rock Mech. Min. Sci. 2021, 148, 104965. [Google Scholar] [CrossRef]
- Sunkpal, M.; Sherizadeh, T.; Guner, D. Quantifying coal rib stability and support requirements using bonded block modeling and reliability analysis based on the random set theory. Int. J. Rock Mech. Min. Sci. 2023, 163, 105332. [Google Scholar] [CrossRef]
- West, I.; Walton, G. Quantitative Evaluation of the Effects of Input Parameter Heterogeneity on Model Behavior for Bonded Block Models of Laboratory Rock Specimens. Rock Mech. Rock Eng. 2023, 56, 7129–7146. [Google Scholar] [CrossRef]
- Sarhosis, V.; Lemos, J.V. A detailed micro-modelling approach for the structural analysis of masonry assemblages. Comput. Struct. 2018, 206, 66–81. [Google Scholar] [CrossRef]
- Pulatsu, B.; Erdogmus, E.; Lourenço, P.B.; Quey, R. Simulation of uniaxial tensile behavior of quasi-brittle materials using softening contact models in DEM. Int. J. Fract. 2019, 217, 105–125. [Google Scholar] [CrossRef]
- Lemos, J.V.; Sarhosis, V. Discrete element bonded-block models for detailed analysis of masonry. Infrastructures 2022, 7, 31. [Google Scholar] [CrossRef]
- Prakash, P.R.; Pulatsu, B.; Lourenço, P.B.; Azenha, M.; Pereira, J.M. A meso-scale discrete element method framework to simulate thermo-mechanical failure of concrete subjected to elevated temperatures. Eng. Fract. Mech. 2020, 239, 107269. [Google Scholar] [CrossRef]
- Bolander, J.E.; Elias, J.; Cusatis, G.; Nagai, K. Discrete mechanical models of concrete fracture. Eng. Fract. Mech. 2021, 257, 108030. [Google Scholar] [CrossRef]
- Atkinson, B.K.; Meredith, P.G. Experimental fracture mechanics data for rocks and minerals. In Fracture Mechanics of Rock; Atkinson, B.K., Ed.; Academic Press: London, UK, 1987; pp. 477–525. [Google Scholar]
- Pijaudier-Cabot, G.; Hajimohammadi, A.; Nouailletas, O.; La Borderie, C.; Padin, A.; Mathieu, J.P. Determination of the fracture energy of rocks from size effect tests: Application to shales and carbonate rocks. Eng. Fract. Mech. 2022, 271, 108630. [Google Scholar] [CrossRef]
- Pérez-Rey, I.; Muñoz-Ibáñez, A.; González-Fernández, M.A.; Muñiz-Menéndez, M.; Penabad, M.H.; Estévez-Ventosa, X.; Delgado, J.; Alejano, L.R. Size effects on the tensile strength and fracture toughness of granitic rock in different tests. J. Rock Mech. Geotech. Eng. 2023, 15, 2179–2192. [Google Scholar] [CrossRef]
- Pulatsu, B. Coupled elasto-softening contact models in DEM to predict the in-plane response of masonry walls. Comput. Part. Mech. 2023, 10, 1759–1770. [Google Scholar] [CrossRef]
- Itasca. UDEC—Universal Distinct Element Code: Theory and Background; Version 6.0; Itasca Consulting Group: Minneapolis, MN, USA, 2014. [Google Scholar]




Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lemos, J.V. Application of Bonded-Block Models to Rock Failure Analysis. Appl. Sci. 2023, 13, 12207. https://doi.org/10.3390/app132212207
Lemos JV. Application of Bonded-Block Models to Rock Failure Analysis. Applied Sciences. 2023; 13(22):12207. https://doi.org/10.3390/app132212207
Chicago/Turabian StyleLemos, José V. 2023. "Application of Bonded-Block Models to Rock Failure Analysis" Applied Sciences 13, no. 22: 12207. https://doi.org/10.3390/app132212207
APA StyleLemos, J. V. (2023). Application of Bonded-Block Models to Rock Failure Analysis. Applied Sciences, 13(22), 12207. https://doi.org/10.3390/app132212207




