Study on Asymmetric Support of Anchor Cable with C-Shaped Tube in Inclined Coal Seam Roadway
Abstract
:1. Introduction
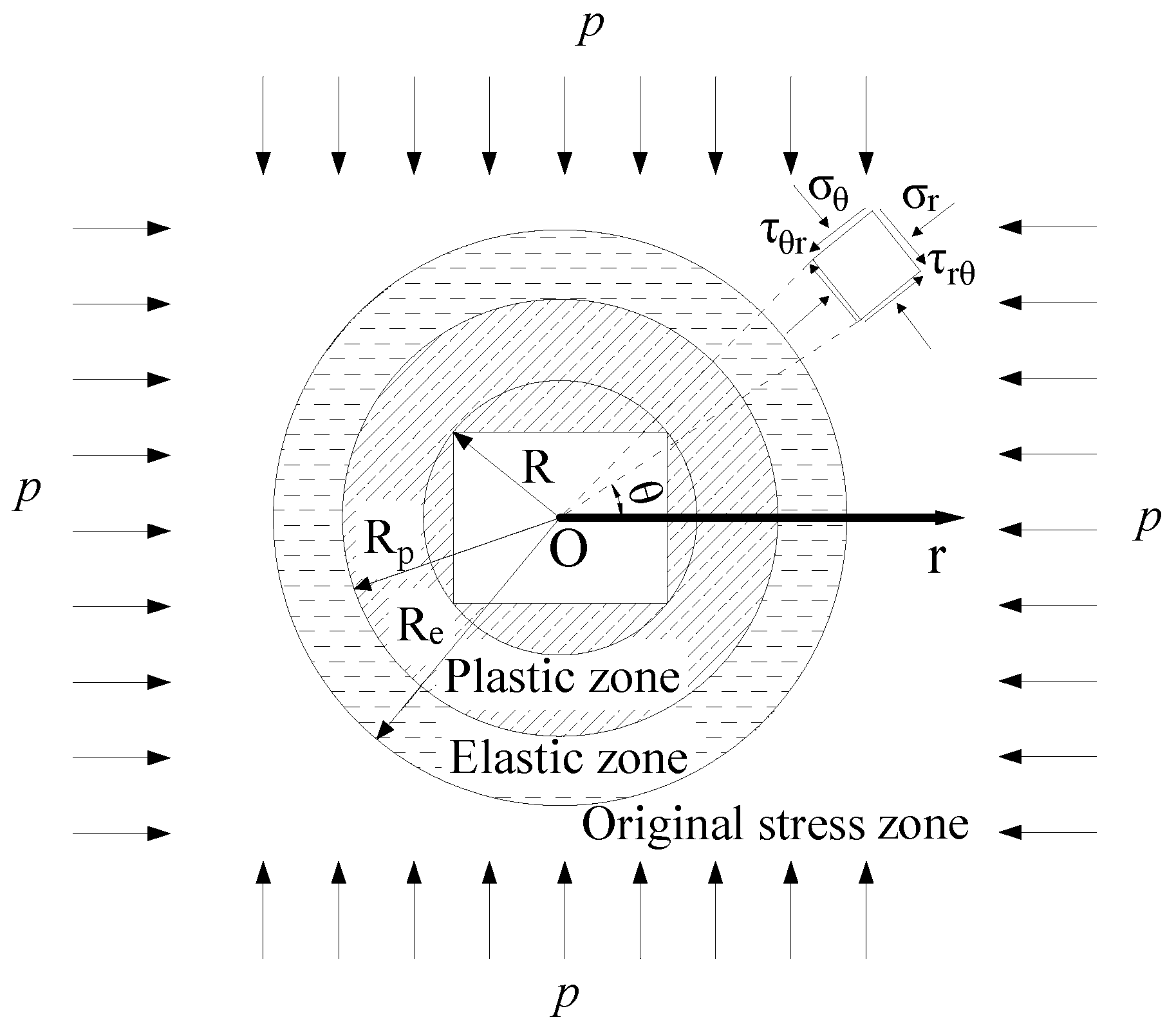
2. Research on the Mechanical Characteristics of Anchor Cable with C-Shaped Tube
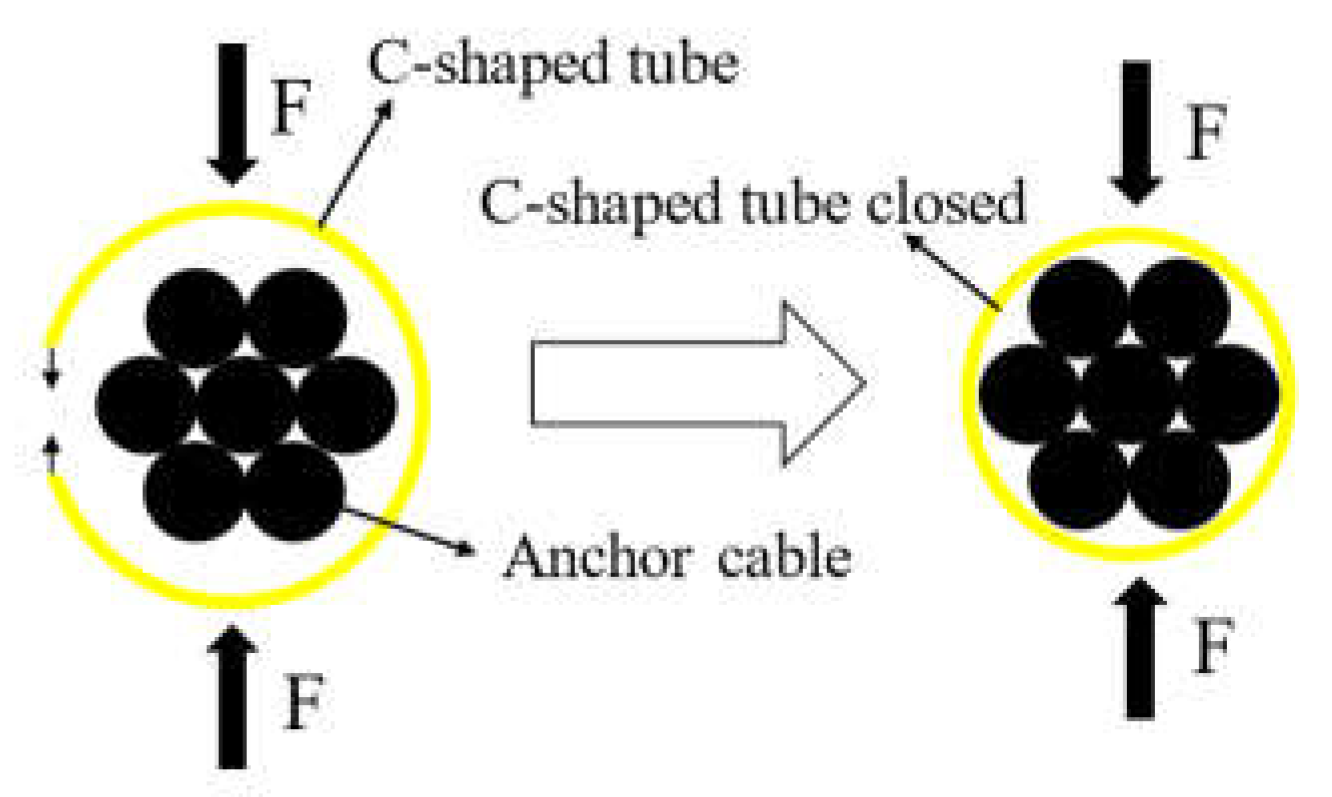
2.1. An Introduction
2.2. A Brief Analysis of the Shear Strength of ACC Anchored Joint Plane
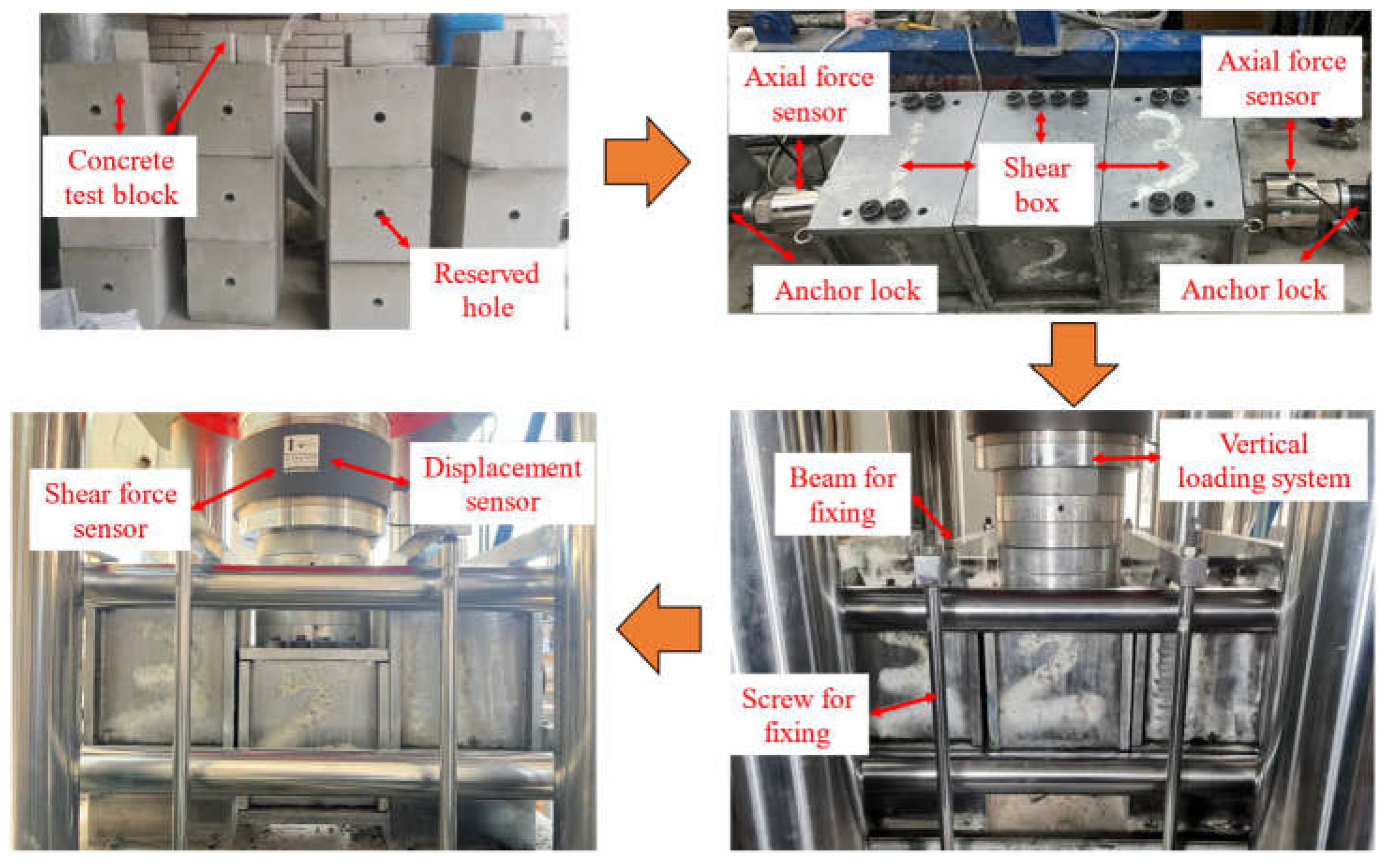
2.3. Preparation for Test
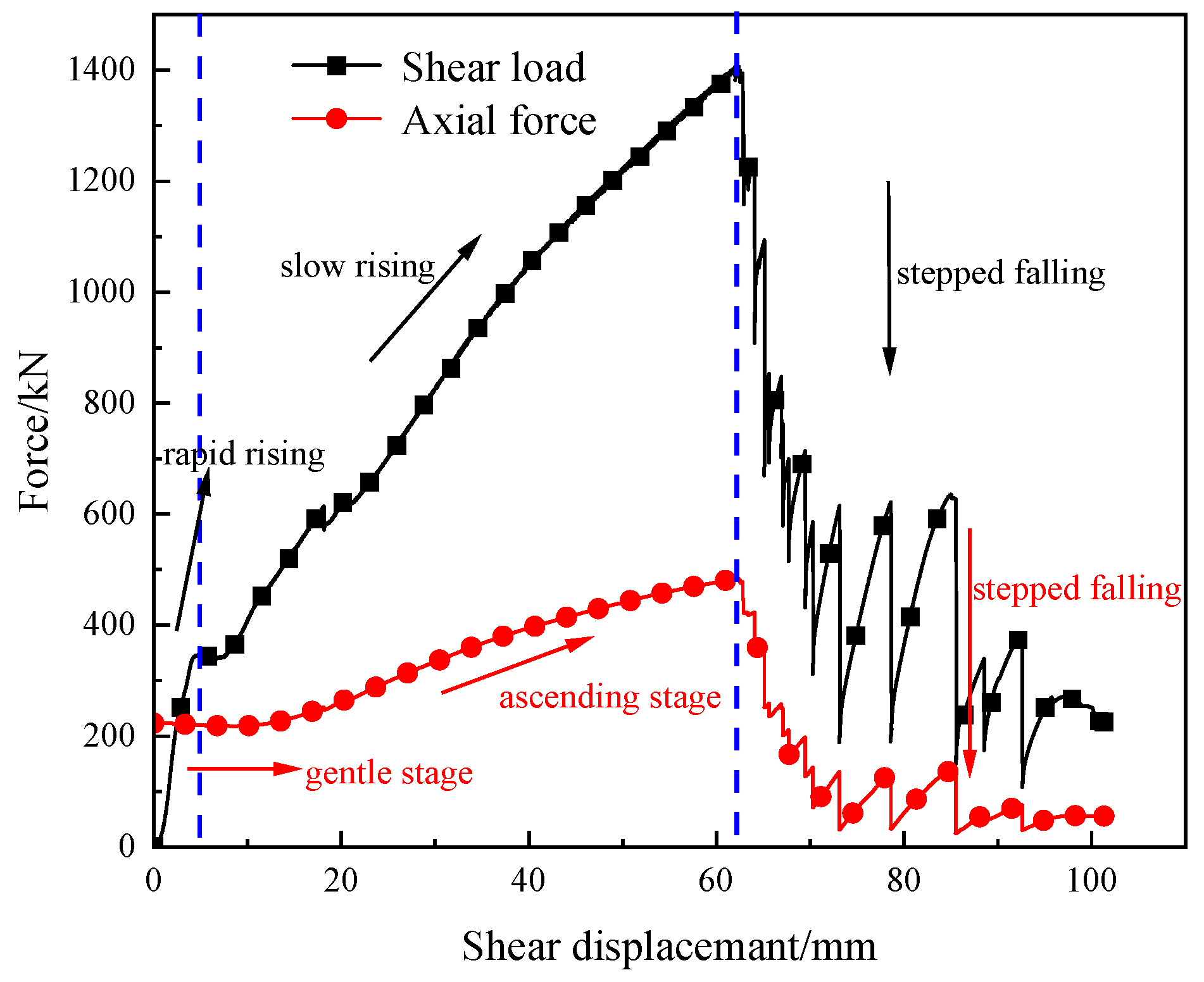
2.4. Analysis of the Outcomes
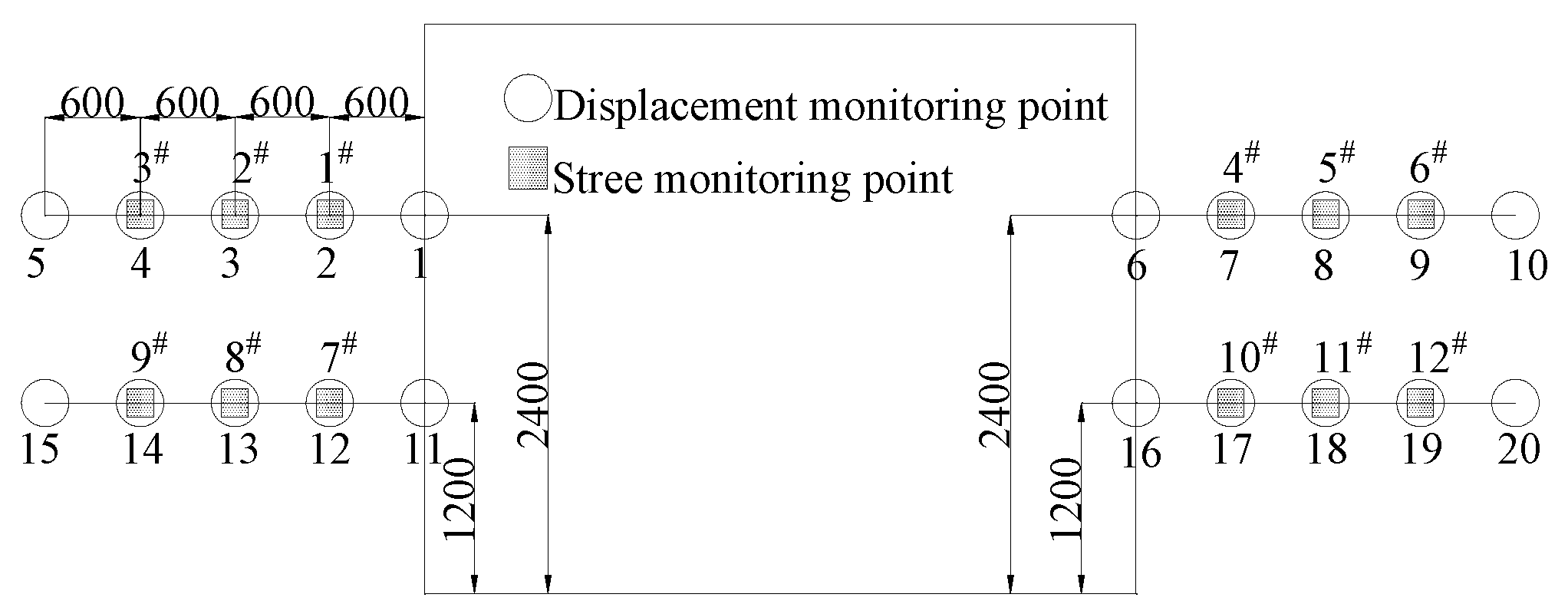
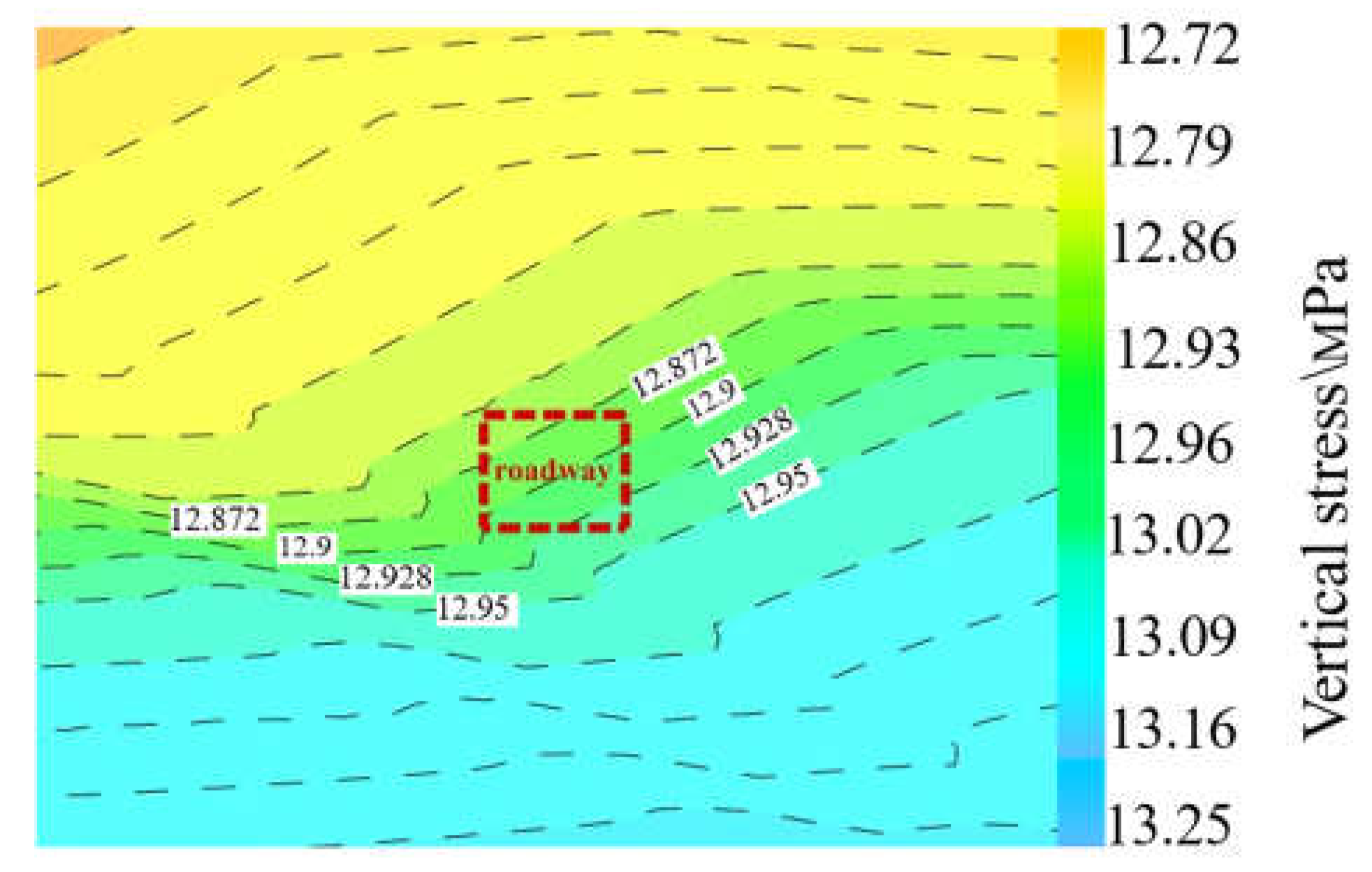
3. Roadway Deformation Law in Inclined Coal Seam
3.1. Initial Support Scheme
3.2. Building Numerical Models
3.3. Asymmetric Deformation Law of the Initial Support System
4. Design of Anchor Cable with C-Shaped Tube Supporting Reinforcement Based on Asymmetric Deformation of the Roadway
4.1. Brief Analysis of Roadway Deformation
4.2. Supporting Reinforcement Scheme
- ①
- A Φ 21.8 mm × 10,300 mm ACC: it consists of a 21.8 mm × 10,300 mm anchor cable and a 2000 mm C-shaped tube.
- ②
- A Φ 21.8 mm × 4300 mm ACC: it consists of a 21.8 mm × 4300 mm anchor cable and a 2000 mm C-shaped tube.
- (1)
- Supporting reinforcement Scheme 1
- (2)
- Supporting reinforcement Scheme 2
- (3)
- Supporting reinforcement Scheme 3
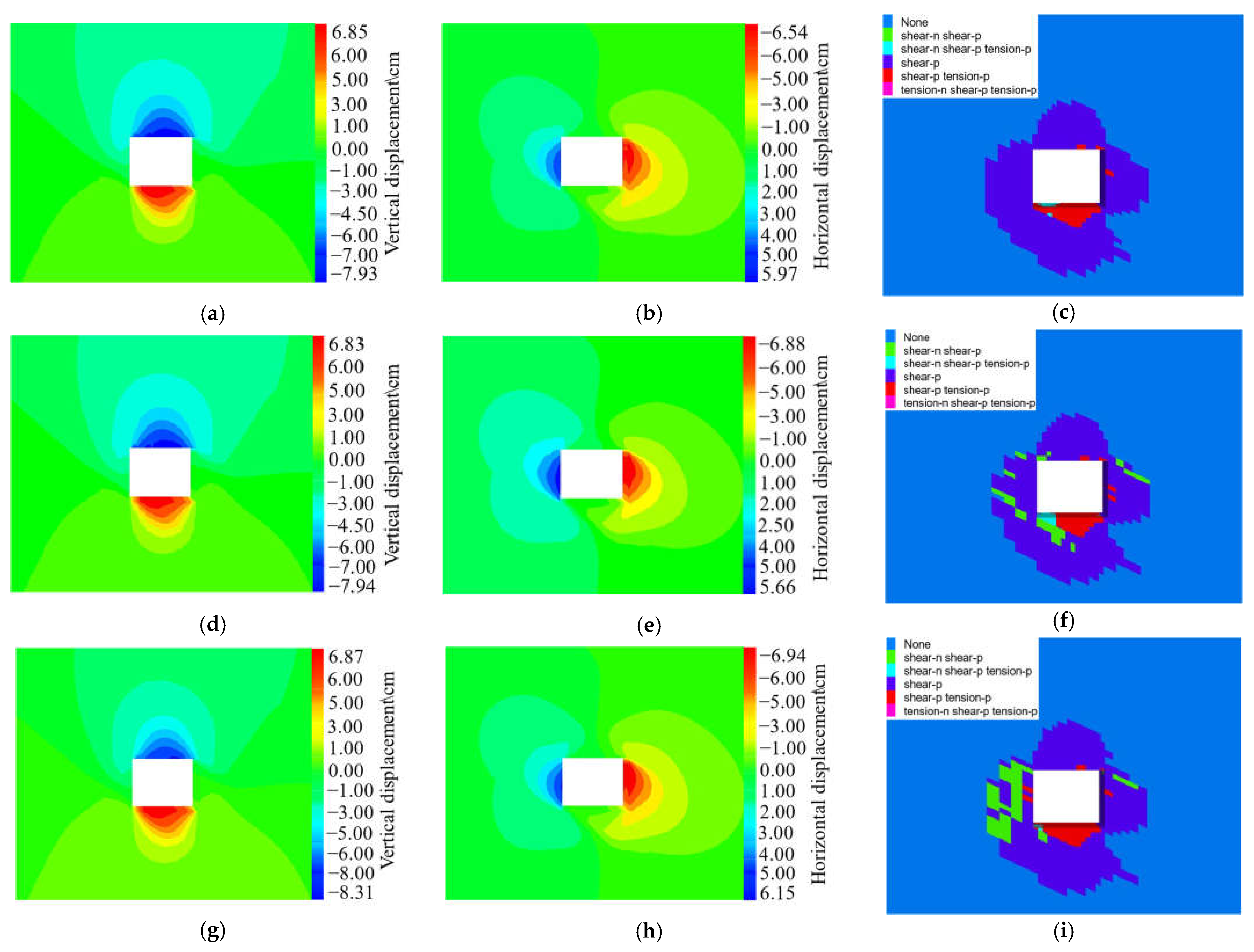
5. Support Effect of Different Support Schemes
5.1. Comparison of Supporting Effect between Asymmetric Scheme and Symmetrical Scheme
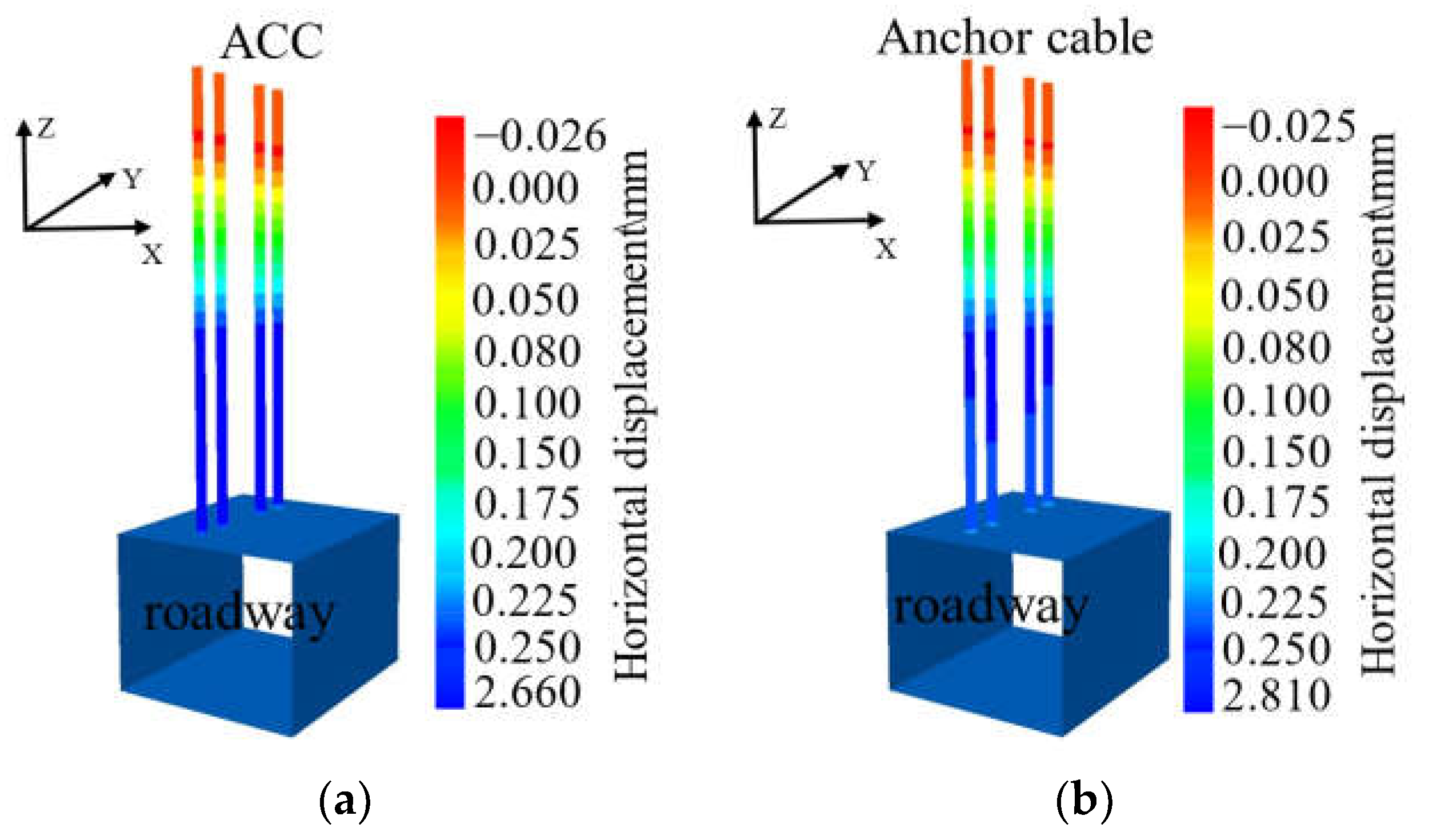
5.2. Comparison of ACC and Anchor Cable Support Effect
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Feng, G.; Wang, P. Simulation of Recovery of Upper Remnant Coal Pillar While Mining the Ultra-close Lower Panel Using Longwall Top Coal Caving. Int. J. Min. Sci. Technol. 2020, 30, 55–61. [Google Scholar] [CrossRef]
- Zhou, Y.; Yu, X. Rock of a Fully Mechanized Caving Face in Gently Inclined Extra-Thick Coal Seams. Appl. Sci. 2022, 12, 9057. [Google Scholar]
- Mo, D.; Li, Z. A Spatial Analysis of China’s Coal Flow. Energy Policy 2012, 48, 358–368. [Google Scholar] [CrossRef]
- Chen, D.; Sun, C.; Wabf, L. Collapse Behavior and Control of Hard Roofs in Steeply Inclined Coal Seams. Bull. Eng. Geol. Environ. 2021, 80, 1489–1505. [Google Scholar] [CrossRef]
- Ma, B.; Wang, F.; Liu, H.; Yin, D.; Xia, Z. Mechanical Properties of Rock-Coal-Rock Composites at Different Inclined Coal Seam Thicknesses. Front. Phys. 2022, 10, 3389. [Google Scholar]
- Wang, H.; Wu, Y.; Jiao, J.; Cao, P. Stability Mechanism and Control Technology for Fully Mechanized Caving Mining of Steeply Inclined Extra-Thick Seams with Variable Angles. Min. Metall. Explor. 2021, 38, 1047–1057. [Google Scholar]
- Eremin, M.; Peryshkin, A.; Esterhuizen, G.; Pavlova, L.; Fryanov, V. Numerical Analysis of Pillar Stability in Longwall Mining of Two Adjacent Panels of an Inclined Coal Seam. Appl. Sci. 2022, 12, 11028. [Google Scholar] [CrossRef]
- Ta, X.; Wan, Z.; Zhang, Y.; Shi, P.; Wei, Z.; Sun, X.; Jia, L. Field and Numerical Investigation on the Coal Pillar Instability of Gob-Side Entry in Gently Inclined Coal Seam. Adv. Civ. Eng. 2021, 2021, 4901670. [Google Scholar] [CrossRef]
- Rong, H.; Pan, L.; Li, X.; Wang, M.; Qu, Z.; Lu, M.; Guo, K. Analysis on Influence Factors of Roadway Instability in High-Stress, Steeply Inclined Extra-Thick Coal Seam. Adv. Civ. Eng. 2021, 2021, 4676685. [Google Scholar] [CrossRef]
- Wu, G.; Jia, S.; Chen, W.; Yuan, J.; Yu, H.; Zhao, W. An Anchorage Experimental Study on Supporting a Roadway in Steeply Inclined Geological Formations. Tunn. Undergr. Space Technol. 2018, 82, 125–134. [Google Scholar] [CrossRef]
- Xiong, X.; Dai, J.; Chen, X. Analysis of Stress Asymmetric Distribution Law of Surrounding Rock of Roadway in Inclined Coal Seam: A Case Study of Shitanjing No. 2 Coal Seam. Adv. Civ. Eng. 2020, 2020, 8877172. [Google Scholar] [CrossRef]
- Xiong, X.; Dai, J.; Ouyang, Y.; Shen, P. Experimental Analysis and Control Technology of Deformation and Failure Mechanism of Inclined Coal Seam Roadway using Non-contact DIC Technique. Sci. Rep. 2021, 11, 20930. [Google Scholar] [CrossRef]
- Xiong, X.; Dai, J.; Chen, X.; Ouyang, Y. Complex Function Solution for Deformation and Failure Mechanism of Inclined Coal Seam Roadway. Sci. Rep. 2022, 12, 7147. [Google Scholar] [CrossRef]
- Liu, P.; Gao, L.; Zhang, P.; Wu, G.; Wang, C.; Ma, Z.; Kong, D.; Kang, X.; Han, S. A Case Study on Surrounding Rock Deformation Control Technology of Gob-Side Coal-Rock Roadway in Inclined Coal Seam of a Mine in Guizhou, China. Processes 2022, 10, 863. [Google Scholar] [CrossRef]
- Das, A.J.; Mandal, P.K.; Bhattacharjee, R.; Tiwari, S.; Kushwaha, A.; Roy, L.B. Evaluation of stability of underground workings for exploitation of an inclined coal seam by the ubiquitous joint model. Int. J. Rock Mech. Min. Sci. 2017, 93, 101–114. [Google Scholar] [CrossRef]
- Das, A.J.; Mandal, P.K.; Paul, P.S.; Sinha, R.K.; Tewari, S. Assessment of the Strength of Inclined Coal Pillars through Numerical Modelling based on the Ubiquitous Joint Model. Rock Mech. Rock Eng. 2019, 52, 3691–3717. [Google Scholar] [CrossRef]
- Das, A.J.; Mandal, P.K.; Paul, P.S.; Sinha, R.K. Generalised Analytical Models for the Strength of the Inclined as well as the Flat Coal Pillars using Rock Mass Failure Criterion. Rock Mech. Rock Eng. 2019, 52, 3921–3946. [Google Scholar] [CrossRef]
- Das, A.J.; Paul, P.S.; Mandal, P.K.; Kumar, R.; Tewari, S. Investigation of Failure Mechanism of Inclined Coal Pillars: Numerical Modelling and Tensorial Statistical Analysis with Field Validations. Rock Mech. Rock Eng. 2021, 52, 3263–3289. [Google Scholar] [CrossRef]
- Jiang, L.; Yang, Z.; Li, G. Research on the Reasonable Coal Pillar Width and Surrounding Rock Supporting Optimization of Gob-Side Entry under Inclined Seam Condition. Adv. Civ. Eng. 2021, 10, 1155. [Google Scholar] [CrossRef]
- Zhao, Y.; Feng, X.; Jiang, Q.; Han, Y.; Zhou, Y.; Guo, H.; Kou, Y.; Shi, Y. Large Deformation Control of Deep Roadways in Fractured Hard Rock Based on Cracking-Restraint Method. Rock Mech. Rock Eng. 2021, 54, 2559–2580. [Google Scholar] [CrossRef]
- Li, X.; Wu, B.; Zhu, Y.; Guo, J.; Li, Z. Numerical Study on Deformation and Failure Characteristics of Rectangular Roadway. Geofluids 2023, 2023, 3792978. [Google Scholar] [CrossRef]
- Gao, F.; Stead, D.; Kang, H. Simulation of Roof Shear Failure in Coal Mine Roadways Using an Innovative UDEC Trigon Approach. Comput. Geotech. 2014, 64, 33–41. [Google Scholar] [CrossRef]
- Tahmasebinia, F.; Zhang, C.; Canbulat, I.; Vardar, O.; Saydam, S. Numerical and analytical simulation of the structural behaviour of fully grouted cable bolts under impulsive loading. Int. J. Min. Sci. Technol. 2018, 28, 807–811. [Google Scholar] [CrossRef]
- Tahmasebinia, F.; Yang, D.; Feghali, P.; Skrzypkowski, K. Structural Evaluation of Cable Bolts under Static Loading. Appl. Sci. 2023, 13, 1326. [Google Scholar] [CrossRef]
- Tahmasebinia, F.; Yang, D.; Feghali, P.; Skrzypkowski, K. A Numerical Investigation to Calculate Ultimate Limit State Capacity of Cable Bolts Subjected to Impact Loading. Appl. Sci. 2023, 13, 15. [Google Scholar] [CrossRef]
- Mirzaghorbanali, A.; Rasekh, H.; Aziz, N.; Yang, G.; Khaleghparast, S.; Nemcik, J. Shear Strength Properties of Cable Bolts Using a New Double Shear Instrument, Experimental Study, and Numerical Simulation. Tunn. Undergr. Space Technol. 2017, 70, 240–253. [Google Scholar] [CrossRef]
- Li, X.; Aziz, N.; Mirzaghorbanali, A.; Nemcik, J. Behavior of Fiber Glass Bolts, Rock Bolts and Cable Bolts in Shear. Rock Mech. Rock Eng. 2016, 49, 2723–2735. [Google Scholar] [CrossRef]
- Wang, K.; Zhao, Y.; Hu, Z.; Nie, Y. Shear Test of Pre-stressed Anchor Block and Fracture Mechanism Analysis of Anchor Cable. Rock Mech. Rock Eng. 2022, 56, 589–601. [Google Scholar] [CrossRef]
- Li, D.; Masoumi, H.; Hagan, P.; Saydam, S. Experimental and Analytical Study on the Mechanical Behaviour of Cable Bolts Subjected to Axial Loading and Constant Normal Stiffness. Int. J. Rock Mech. Min. Sci. 2019, 113, 83–91. [Google Scholar] [CrossRef]
- Spang, K.; Egger, P. Action of fully-grouted bolts in jointed rock and factors of influence. Rock Mech. Rock Eng. 1990, 23, 201–229. [Google Scholar] [CrossRef]
- Aziz, N.; Rasekh, H.; Mirzaghorbanali, A.; Yang, G.; Khaleghparast, S.; Nemcik, J. An Experimental Study on the Shear Performance of Fully Encapsulated Cable Bolts in Single Shear Test. Rock Mech. Rock Eng. 2018, 51, 2207–2221. [Google Scholar] [CrossRef]
- Li, X.; Aziz, N.; Mirzaghorbanali, A.; Nemcik, J. Comparison of the shear test results of a cable bolt on three laboratory test apparatuses. Tunn. Undergr. Space Technol. 2017, 61, 82–89. [Google Scholar] [CrossRef]
- Aziz, N.; Mirzaghorbanali, A.; Anzanpour, S.; Rastegarmanesh, A.; Khaleghparat, S.; Nemcik, J.; On, J.; Si, G. Angle Shear Testing of 15.2 mm Seven Wire Cable Bolt. Rock Mech. Rock Eng. 2022, 55, 3919–3937. [Google Scholar] [CrossRef]
- Shan, R.; Zhang, S.; Huang, P.; Liu, W. Research on Full-Section Anchor Cable and C-Shaped Tube Support System of Deep Layer Roadway. Geofluids 2021, 2021, 5593601. [Google Scholar] [CrossRef]
- Shan, R.; Huang, P.; Yuan, H.; Meng, C.; Zhang, S. Research on the Full-section Anchor Cable and C-shaped Tube Support System of Mining Roadway in Island Coal Gaces. J. Asian Archit. Build. Eng. 2022, 43, 602–614. [Google Scholar]
- Tong, X.; Shan, R.; Liu, N.; Liu, D.; Wei, Y. Experimental study on the shear performance of an anchor cable with a C-shaped tube anchored flat structural plane. Sci. Rep. 2023, 13, 797. [Google Scholar] [CrossRef]
- Pellet, F.L.; Egger, P.H. Analytical model for the mechanical behaviour of bolted rock joints subjected to shearing. Rock Soil Mech. 1996, 29, 73–97. [Google Scholar] [CrossRef]
- Shan, R.; Tong, X.; Huang, P.; Yuan, H.; Bao, Y.; Liu, N. Research on the anchor cable combined with the c-shaped tube and the mechanical properties. Rock Soil Mech. 2022, 43, 602–614. [Google Scholar]
- Ferrero, A.M. The shear strength of reinforced rock joints. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1995, 35, 595–605. [Google Scholar] [CrossRef]
- Liu, C.; Li, Y. Analytical Study of the Mechanical Behavior of Fully Grouted Bolts in Bedding Rock Slopes. Rock Mech. Rock Eng. 2017, 50, 2413–2423. [Google Scholar] [CrossRef]
- Kang, H.; Yin, B.; Gao, F.; Lv, H. Database and characteristics of underground in-situ stress distribution in Chinese coal mines. J. China Coal Soc. 2019, 44, 23–33. [Google Scholar]
- Yuan, W.; Chen, J. Analysis of plastic zone and fracture zone in roadways in softened rock layers. J. China Coal Soc. 1986, 10, 77–86. [Google Scholar]









| Number | Material Type | Design Prestress (kN) | Actual Prestress (kN) |
|---|---|---|---|
| S1 | ACC | 100 | 100 |
| S2 | Anchor cable | 100 | 100 |
| S3 | ACC | 200 | 223 |
| S4 | Anchor cable | 200 | 211 |
| Density (kg/m3) | Bulk Modulus (GPa) | Shear Modulus (GPa) | Tensile Strength (MPa) | Cohesion (MPa) | Friction Angle (°) | |
|---|---|---|---|---|---|---|
| Coal | 1370 | 1.25 | 0.49 | 0.18 | 1.4 | 25 |
| Mudstone | 2300 | 1.4 | 1.5 | 0.49 | 0.9 | 27 |
| Sandy mudstone | 2860 | 1.25 | 0.76 | 0.49 | 0.7 | 25 |
| Limestone | 2690 | 2.3 | 1.2 | 0.62 | 1.2 | 29 |
| Emod (GPa) | Xcarea (mm2) | Per (mm) | Cs_sk (MPa) | Cs_scoh (MN/m) | Cs_nk (MPa) | Cs_ncoh (MN/m) | Cs_sfric (MPa) | Nu | |
|---|---|---|---|---|---|---|---|---|---|
| pile | 200 | 4.02 | 100 | 13 | 13 | 13 | 0 | 25 | 0.3 |
| Type | Anchor Cable | Bolt |
|---|---|---|
| Diameter (mm) | 21.8 | 22 |
| Xcaera (mm2) | 373 | 380 |
| Emod (GPa) | 200 | 200 |
| Breaking load (kN) | 550 | 216 |
| Gr-per (mm) | 100 | 100 |
| Gr-k (MN/m/m) | 17.5 | 17.5 |
| Gr_coh (MN/m) | 0.44 | 0.44 |
| Distance from Roadway Surface | The Side Is 2.4 m High | The Side Is 1.2 m High | ||
|---|---|---|---|---|
| Right Displacement/cm | Left Displacement/cm | Right Displacement/cm | Left Displacement/cm | |
| 0 | −7.83 | 6.2 | −7.13 | 6.59 |
| 0.6 | −6.85 | 4.94 | −6.33 | 5.09 |
| 1.2 | −5.26 | 3.67 | −5.49 | 3.27 |
| 1.8 | −3.11 | 2.57 | −3.48 | 1.98 |
| 2.4 | −2.5 | 2.57 | −2.81 | 1.98 |
| Initial Support Scheme | Scheme 1 | Scheme 2 | Scheme 3 | |
|---|---|---|---|---|
| Roof/cm | −9.23 | −7.93 | −7.94 | −8.31 |
| Right side/cm | −7.93 | −6.54 | −6.88 | −6.94 |
| Left side/cm | 6.77 | 5.97 | 5.66 | 6.15 |
| Plastic zone volume/m3 | 457.8 | 330.9 | 370.3 | 384.6 |
| Distance from Roadway Surface | Scheme 1 | Scheme 2 | Scheme 3 | |||
|---|---|---|---|---|---|---|
| Right Displacement/cm | Left Displacement/cm | Right Displacement/cm | Left Displacement/cm | Right Displacement/cm | Left Displacement/cm | |
| 0 | −6.09 | 5.29 | −6.5 | 4.99 | −6.42 | 5.43 |
| 0.6 | −5.41 | 4.27 | −5.62 | 3.99 | −5.68 | 4.39 |
| 1.2 | −4.24 | 3.11 | −4.43 | 3.05 | −4.43 | 3.22 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shan, R.; Li, T.; Liu, W.; Chen, Y.; Shi, S.; Li, G. Study on Asymmetric Support of Anchor Cable with C-Shaped Tube in Inclined Coal Seam Roadway. Appl. Sci. 2023, 13, 8088. https://doi.org/10.3390/app13148088
Shan R, Li T, Liu W, Chen Y, Shi S, Li G. Study on Asymmetric Support of Anchor Cable with C-Shaped Tube in Inclined Coal Seam Roadway. Applied Sciences. 2023; 13(14):8088. https://doi.org/10.3390/app13148088
Chicago/Turabian StyleShan, Renliang, Tianwen Li, Weijun Liu, Ye Chen, Shuguo Shi, and Gengzhao Li. 2023. "Study on Asymmetric Support of Anchor Cable with C-Shaped Tube in Inclined Coal Seam Roadway" Applied Sciences 13, no. 14: 8088. https://doi.org/10.3390/app13148088
APA StyleShan, R., Li, T., Liu, W., Chen, Y., Shi, S., & Li, G. (2023). Study on Asymmetric Support of Anchor Cable with C-Shaped Tube in Inclined Coal Seam Roadway. Applied Sciences, 13(14), 8088. https://doi.org/10.3390/app13148088






