Analysis of the Mechanical Properties and Study of Influential Factors of Different Materials in Tape Spring
Abstract
1. Introduction
2. Theoretical Analysis
3. Numerical Analysis
4. Conclusions
- (1)
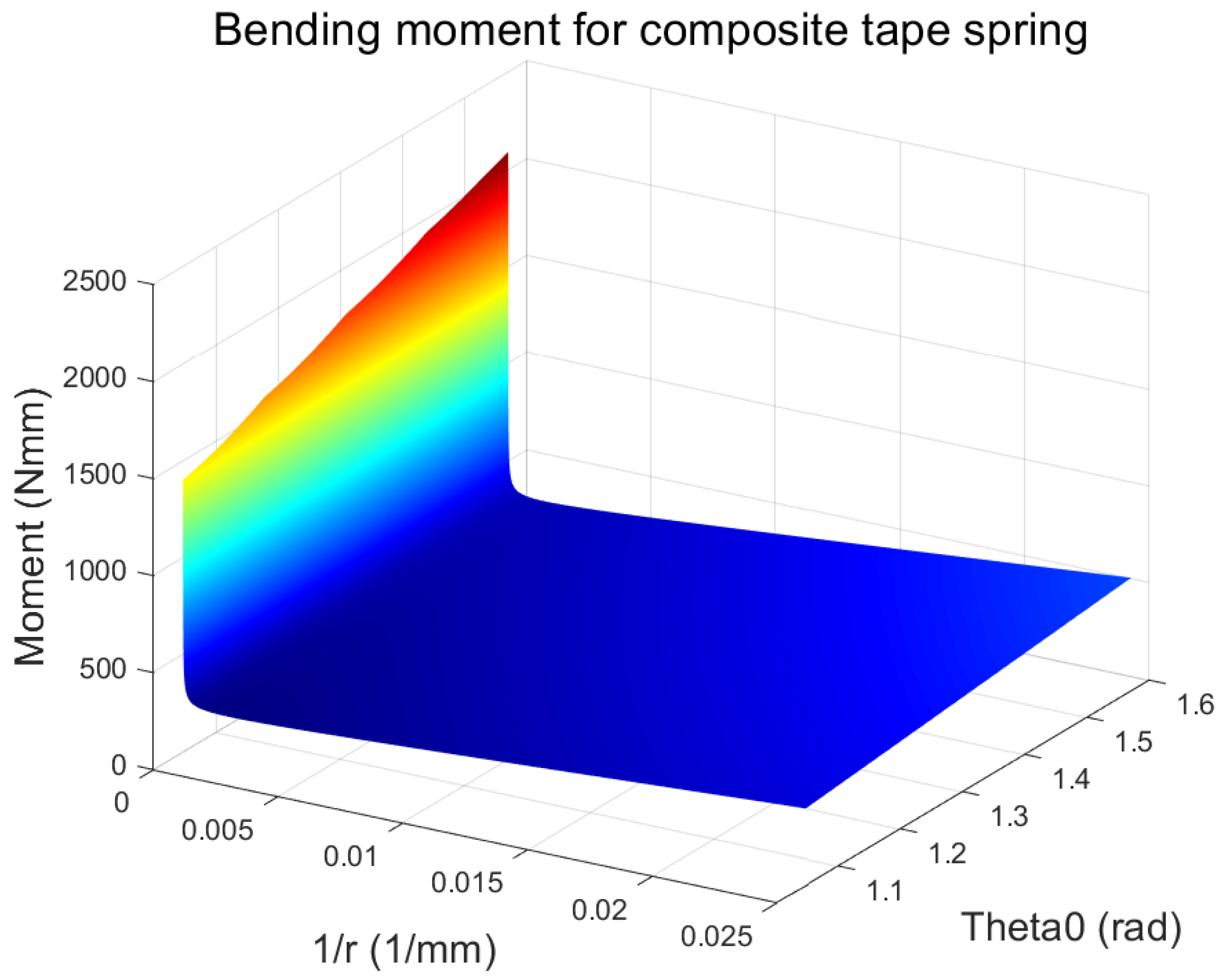
- Based on classical laminated plate shell theory, considering the nonlinearity of geometric equations, a nonlinear control equation for the folding and bending of composite laminated tape springs was established, and precise expressions for the folding and bending displacement and bending moment were obtained. The influence of laminate thickness and the central angle of the section on the performance of composite material springs was investigated. It was found that increasing the laminate thickness significantly improves the performance of the composite material spring. Similarly, the performance of the composite material spring shows a linear improvement with an increase in the central angle of the section.
- (2)
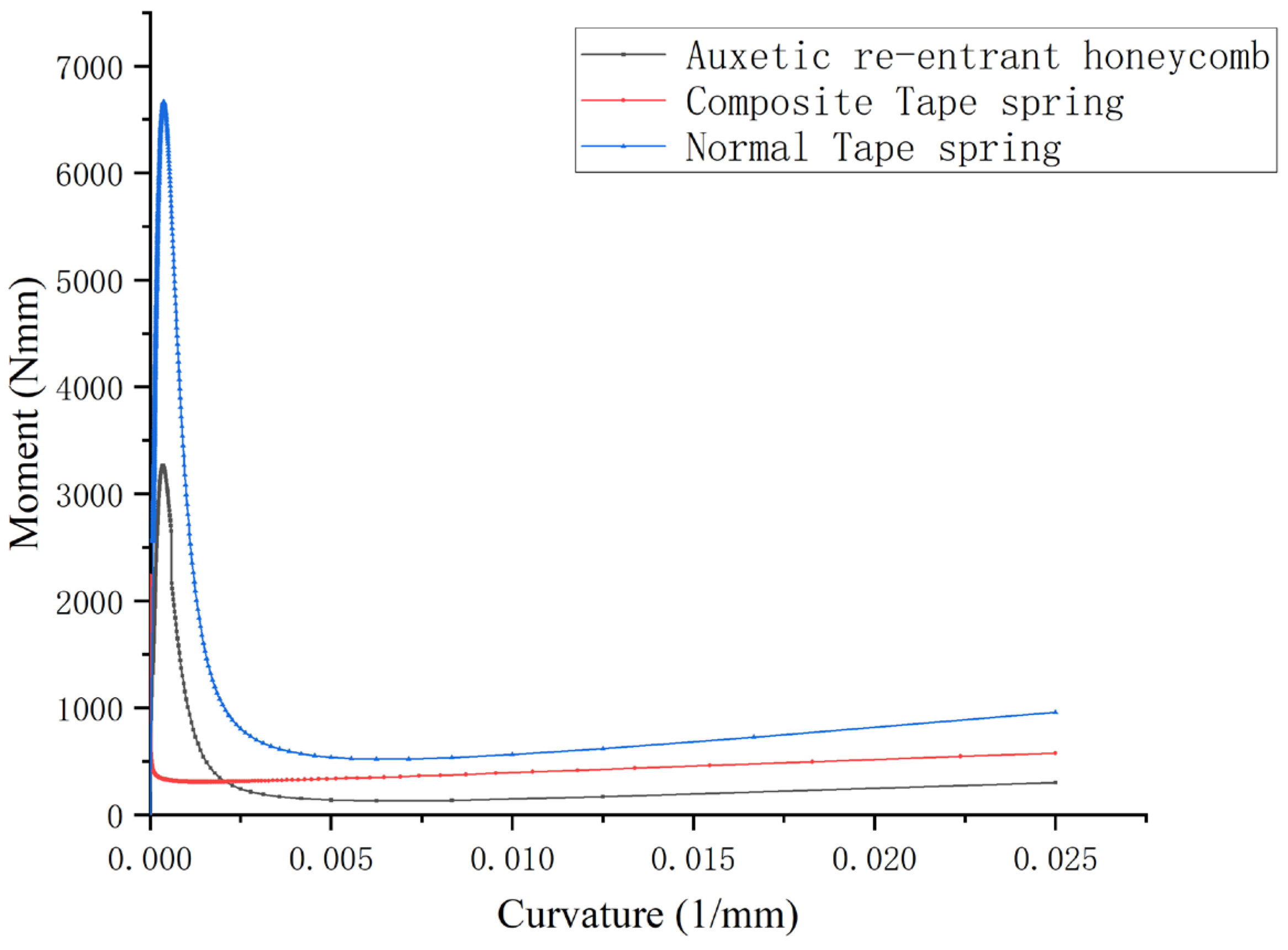
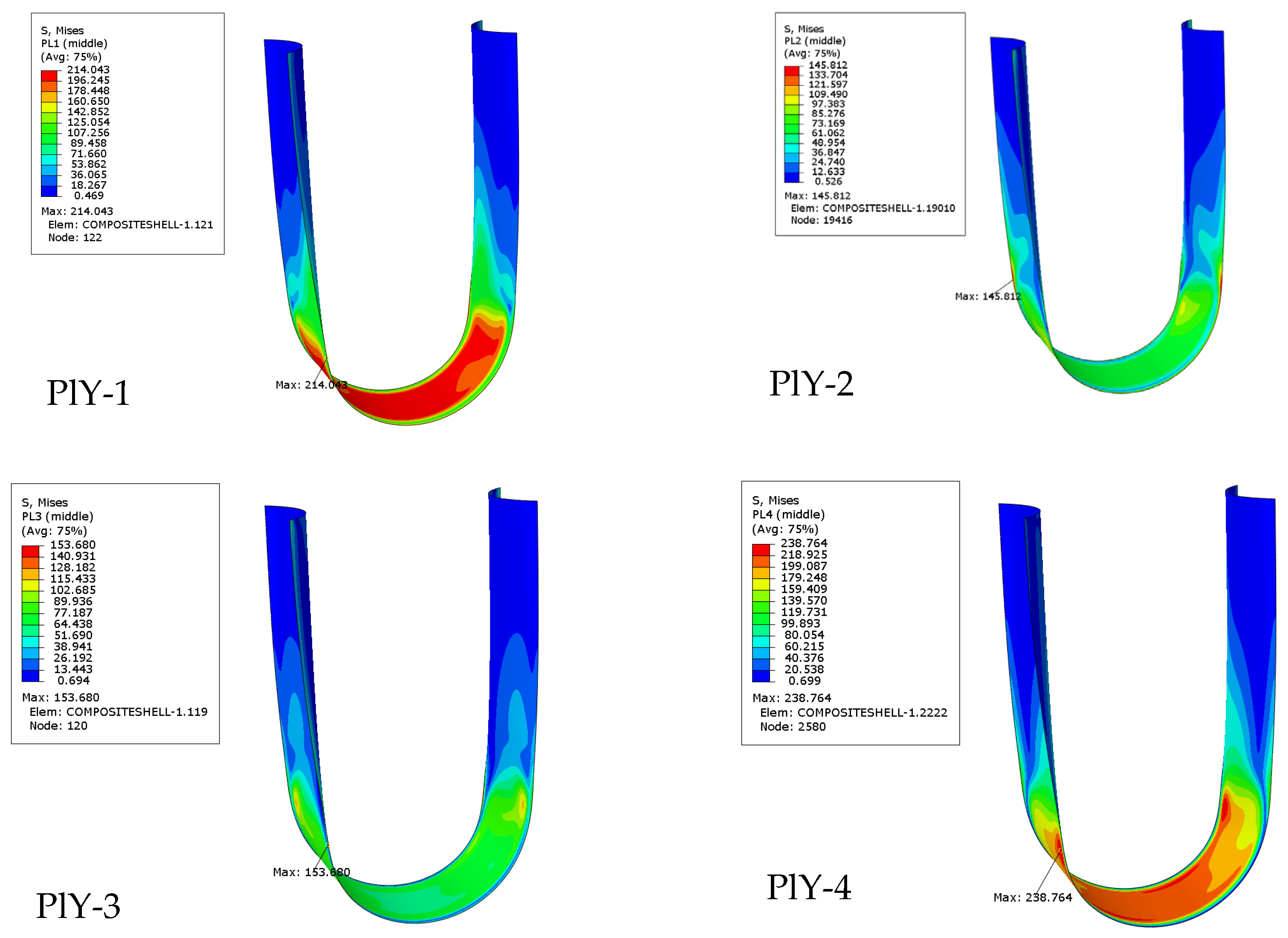
- Finite element numerical analysis is carried out on the [−45 45]s laminated composite tape spring, and the correctness of the theoretical derivation is proved by comparing the curvature radius-bending moment change relationship curve.
- (3)
- The mechanical properties of traditional spring structures, negative Poisson ratio honeycomb structures, and composite laminated spring structures are compared with previous work. In terms of critical bending moment, the unit mass critical bending moment performance of the composite laminated tape spring is close to that of the ordinary tape spring and much lower than that of the negative Poisson ratio honeycomb structure tape spring. In terms of steady-state bending moment, the composite laminated spring is better than the ordinary tape spring and the negative Poisson ratio honeycomb structure tape spring.
- (1)
- Comparative analysis between composite material springs and negative Poisson ratio honeycomb springs: In further studies, we will conduct an in-depth analysis of the mechanical performance factors that affect the tape spring structure. We aim to identify the key mechanical parameters that influence the mechanical performance of the tape springs and explore more optimal structures. By achieving superior mechanical performance with lighter mass, we aim to contribute to the lightweight design of space-deployable structures.
- (2)
- Composite tape springs are subjected to extreme temperature conditions in practical applications. Therefore, in the subsequent research, it is necessary to consider the influence of the temperature field in the constitutive equations.
Author Contributions
Funding
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Seffen, K.; You, Z.; Pellegrino, S. Folding and deployment of curved tape springs. Int. J. Mech. Sci. 2000, 42, 2055–2073. [Google Scholar] [CrossRef]
- Kim, K.-W.; Park, Y. Solar array deployment analysis considering path-dependent behavior of a tape spring hinge. J. Mech. Sci. Technol. 2015, 29, 1921–1929. [Google Scholar] [CrossRef]
- Eigenmann, M.; Schmalbach, M.; Schiller, M.; Schmidt, T.; Scolamiero, L. Ultra-light deployment mechanism (UDM) for sectioned large deployable antenna reflectors. In Proceedings of the 14th European Space Mechanisms & Tribology Symposium—ESMATS 2011, Konstanz, Germany, 28–30 September 2009. [Google Scholar]
- Ye, H.; Zhang, Y.; Yang, Q.; Zhang, B. Quasi-static analysis and multi-objective optimization for tape spring hinge. Struct. Multidiscip. Optim. 2019, 60, 2417–2430. [Google Scholar] [CrossRef]
- Gu, W.; Zhang, J.; Pan, L.; Qu, Y.; Choi, J.-H.; Zhu, X. Coupling Effect of Nonlinear Stiffness of Tape Spring Hinges and Flexible Deformation of Panels during Orbit Maneuvers. Aerospace 2022, 9, 30. [Google Scholar] [CrossRef]
- Footdale, J.N.; Murphey, T.W. Mechanism design and testing of a self-deploying structure using flexible composite tape springs. In Proceedings of the 42nd Aerospace Mechanism Symposium 2014, Baltimore, MD, USA, 14–16 May 2014. [Google Scholar]
- Seffen, K.; Pellegrino, S. Deployment of a Rigid Panel by Tape-Springs; Cambridge University Department of Engineering: Cambridge, UK, 1997. [Google Scholar]
- Seffen, K.A. Analysis of Structures Deployed by Tape-Springs. Ph.D. Thesis, University of Cambridge, Cambridge, UK, 1997. [Google Scholar]
- Seffen, K.; Pellegrino, S. Deployment dynamics of tape springs. Proc. R. Soc. Lond. A 1999, 45, 1003–1048. [Google Scholar] [CrossRef]
- Walker, S.J.; Aglietti, G.S. An investigation of tape spring fold curvature. In Proceedings of the 6th International Conference on Dynamics and Control of Systems and Structures in Space, Liguria, Italy, 18–22 July 2004; Citeseer: Princeton, NJ, USA, 2004. [Google Scholar]
- Chang, W.; Cao, D.; Lian, M. Simulation and analysis of tape spring for deployed space structures. In Proceedings of the Young Scientists Forum 2017, Shanghai, China, 24–26 November 2017. [Google Scholar] [CrossRef]
- Lan, X.; Wang, X.; Lu, H.; Liu, Y.; Leng, J. Shape recovery performances of a deployable hinge fabricated by fiber-reinforced shape-memory polymer. Proc. SPIE 2009, 7289, 728910. [Google Scholar] [CrossRef]
- Oberst, S.; Tuttle, S.; Griffin, D.; Lambert, A.; Boyce, R. Experimental validation of tape springs to be used as thin-walled space structures. J. Sound Vib. 2018, 419, 558–570. [Google Scholar] [CrossRef]
- Yang, H.; Liu, R.; Wang, Y.; Deng, Z.; Guo, H. Experiment and multiobjective optimization design of tape-spring hinges. Struct. Multidiscip. Optim. 2015, 51, 1373–1384. [Google Scholar] [CrossRef]
- Wang, B.; Seffen, K.A.; Guest, S.D. Folded strains of a bistable composite tape-spring. Int. J. Solids Struct. 2021, 233, 111221. [Google Scholar] [CrossRef]
- Elsheikh, A. Bistable morphing composites for energy-harvesting applications. Polymers 2022, 14, 1893. [Google Scholar] [CrossRef] [PubMed]
- Kajihara, S.; Yokozeki, T.; Aoki, T. Dimension effects on the stowability and self-deployment behavior of CFRP bistable open-section semi-cylindrical beam. Compos. Struct. 2023, 307, 116628. [Google Scholar] [CrossRef]
- Yang, H.; Lu, F.; Guo, H.; Liu, R. Design of a new N-shape composite ultra-thin deployable boom in the post-buckling range using response surface method and optimization. IEEE Access 2019, 7, 129659–129665. [Google Scholar] [CrossRef]
- Yao, Y.; Li, G.; Ning, X. Ultra-thin, Ultra-lightweight Structural Electronic Skin for Measurements of Tape-spring Hinge Behavior. In Proceedings of the AIAA Scitech 2021 Forum, Virtual Event, 11–21 January 2021. [Google Scholar] [CrossRef]
- Yang, Y.; Wang, F.; Liu, J. Application of Honeycomb Structures in Key Components of Space Deployable Structures. Adv. Mater. Sci. Eng. 2022, 2022, 4756272. [Google Scholar] [CrossRef]
- Yee, J.; Soykasap, O.; Pellegrino, S. Carbon fibre reinforced plastic tape springs. In Proceedings of the 45th AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics & Materials Conference, Palm Springs, CA, USA, 19–22 April 2004. [Google Scholar] [CrossRef]
- Yang, Y.; Wang, F.; Liu, J. The Exact Solution of the Bending Moment in the Folding Process of Negative Poisson’s Ratio Honeycomb Tape Spring and Multi-Objective Optimization Design. Aerospace 2023, 10, 390. [Google Scholar] [CrossRef]
- Olsson, R.; André, A.; Hellstrm, P. Analytical modelling and FE simulation of impact response and damage growth in a thin-ply laminate. In Proceedings of the 20th International Conference on Composite Materials, Copenhagen, Denmark, 19–24 July 2015. [Google Scholar]


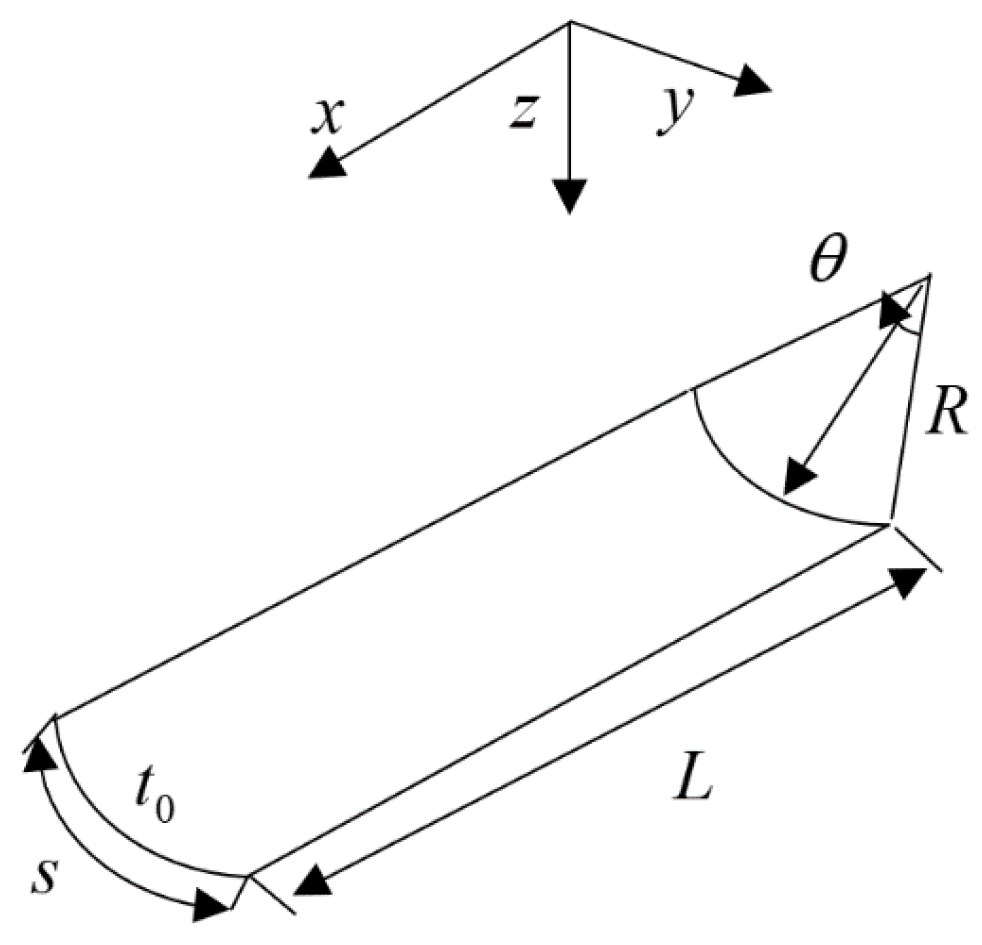
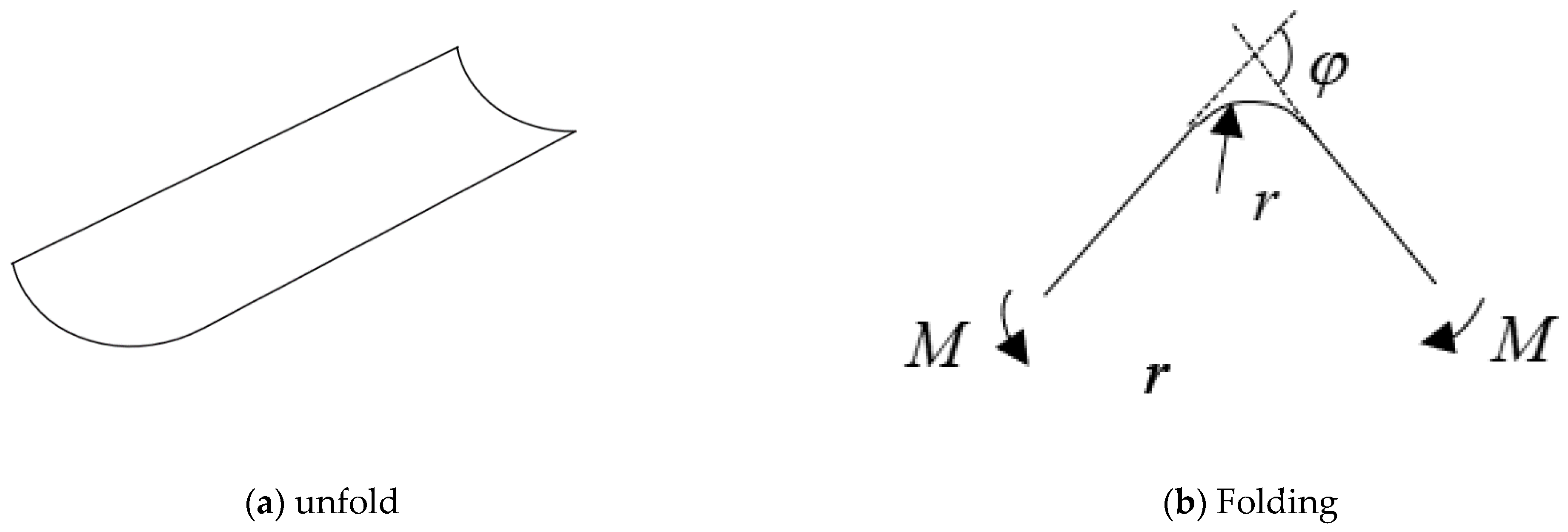

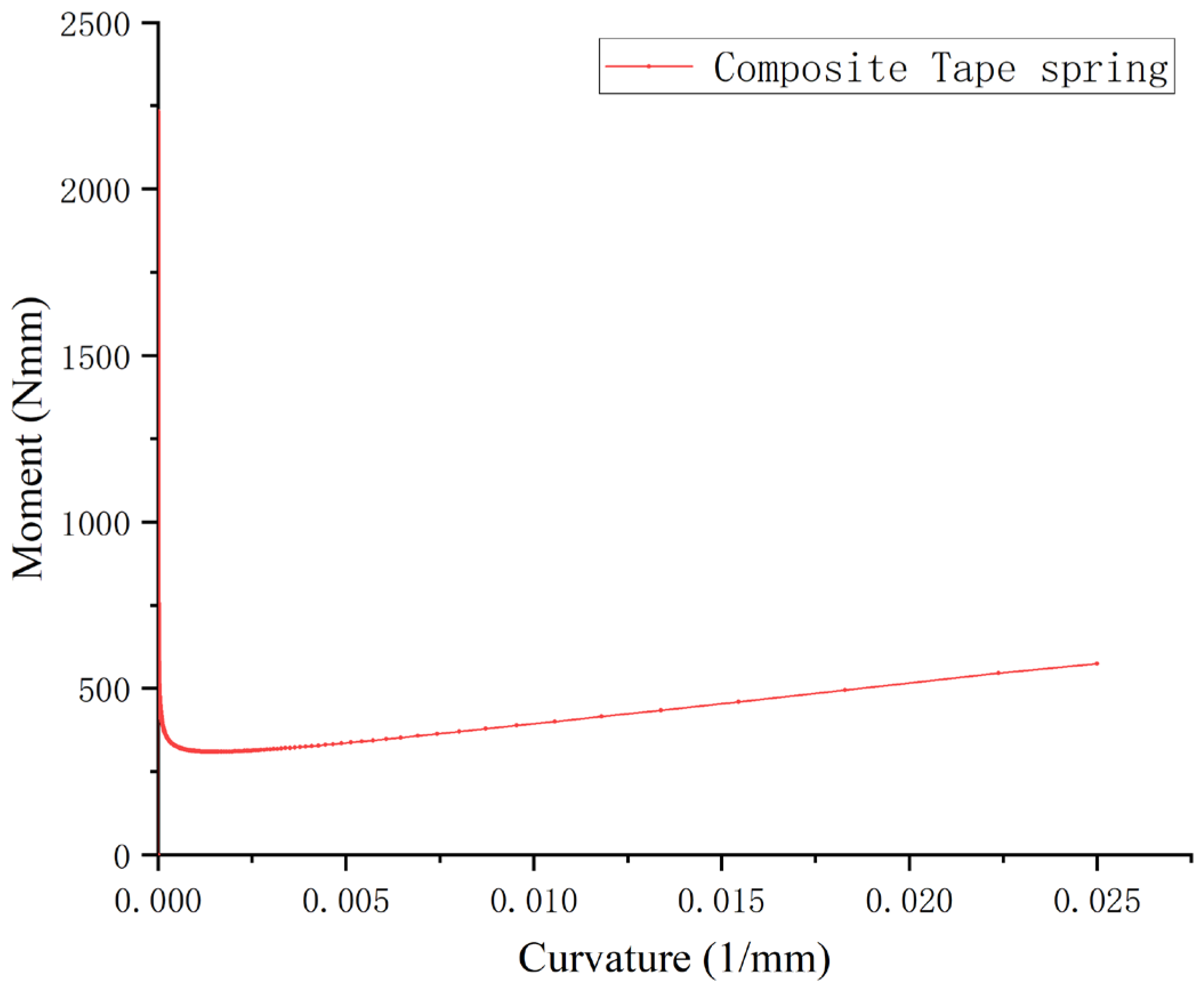
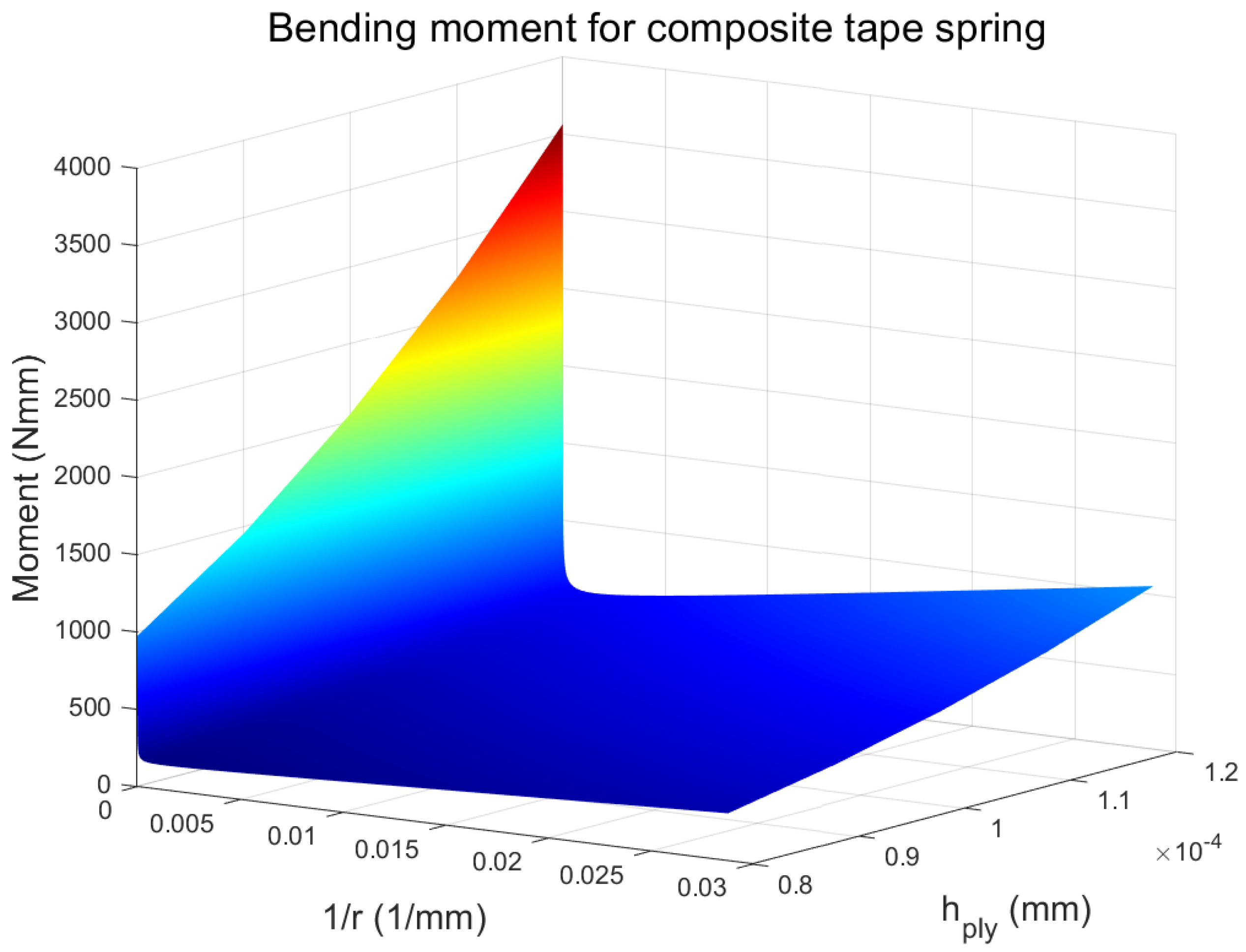

| Parameter | Value |
|---|---|
| Length L/mm | 350 |
| Radius R/mm | 35 |
| Central angle θ/(°) | 90 |
| Parameter | Value |
|---|---|
| Elastic modulus E1 | 50 GPa |
| Elastic modulus E2 | 7 GPa |
| Shear modulus G12 | 6.2 GPa |
| Poisson’s ratio u | 0.3 |
| Ply Thickness (mm) | Steady-State Bending Moment (Nmm) | Critical Bending Moment (Nmm) |
|---|---|---|
| 0.08 | 996.6 | 318.8 |
| 0.09 | 1487.2 | 454.6 |
| 0.1 | 2091.0 | 625.2 |
| 0.11 | 2801.6 | 832.7 |
| 0.12 | 3637.2 | 1083.5 |
| Central Angles (°) | Steady-State Bending Moment (Nmm) | CRITICAL Bending Moment (Nmm) |
|---|---|---|
| 60 | 1403.3 | 424.7 |
| 65 | 1520.6 | 457.6 |
| 70 | 1637.2 | 491.3 |
| 75 | 1754.1 | 523.5 |
| 80 | 1871.0 | 559.0 |
| 85 | 1987.9 | 591.2 |
| 90 | 2091.0 | 625.2 |
| Critical Bending Moment (Nmm) | Steady-State Bending Moment (Nmm) | Unit Mass Critical Bending Moment (Nmm/g) | Unit Mass Steady-State Bending Moment (Nmm/g) | |
|---|---|---|---|---|
| Normal tape spring | 6664.5 | 956.5 | 166.2 | 17.7 |
| Composite tape spring | 2249.0 | 625.8 | 166.6 | 46.4 |
| Auxetic re-entrant honeycomb | 2998.7 | 277.4 | 269.8 | 25.3 |
| Location | Boundary Conditions |
|---|---|
| Centroid of the cross-section A | U1 = U2 = UR2 = UR3 = 0, UR1 = 1.57 |
| Centroid of the cross-section B | U1 = U2= U2 = UR2 = UR3 = 0, UR1 = −1.57 |
| Property | Value (MPa) |
|---|---|
| Fiber tensile strength (Xt) | 2390 |
| Fiber compressive strength (Xc) | 1272 |
| Matrix tensile strength (Yt) | 50 |
| Matrix compressive strength (Yt) | 146 |
| Shear strength of the unidirectional ply (S) | 57 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, Y.; Wang, F.; Liu, J. Analysis of the Mechanical Properties and Study of Influential Factors of Different Materials in Tape Spring. Appl. Sci. 2023, 13, 6315. https://doi.org/10.3390/app13106315
Yang Y, Wang F, Liu J. Analysis of the Mechanical Properties and Study of Influential Factors of Different Materials in Tape Spring. Applied Sciences. 2023; 13(10):6315. https://doi.org/10.3390/app13106315
Chicago/Turabian StyleYang, Yang, Fan Wang, and Jieshan Liu. 2023. "Analysis of the Mechanical Properties and Study of Influential Factors of Different Materials in Tape Spring" Applied Sciences 13, no. 10: 6315. https://doi.org/10.3390/app13106315
APA StyleYang, Y., Wang, F., & Liu, J. (2023). Analysis of the Mechanical Properties and Study of Influential Factors of Different Materials in Tape Spring. Applied Sciences, 13(10), 6315. https://doi.org/10.3390/app13106315






