One-Dimensional Optimization Design of Supercritical Carbon Dioxide Radial Inflow Turbine and Performance Analysis under Off-Design Conditions
Abstract
:1. Introduction
2. One-Dimensional Optimization Design
2.1. Thermo-Physical Property Calculation
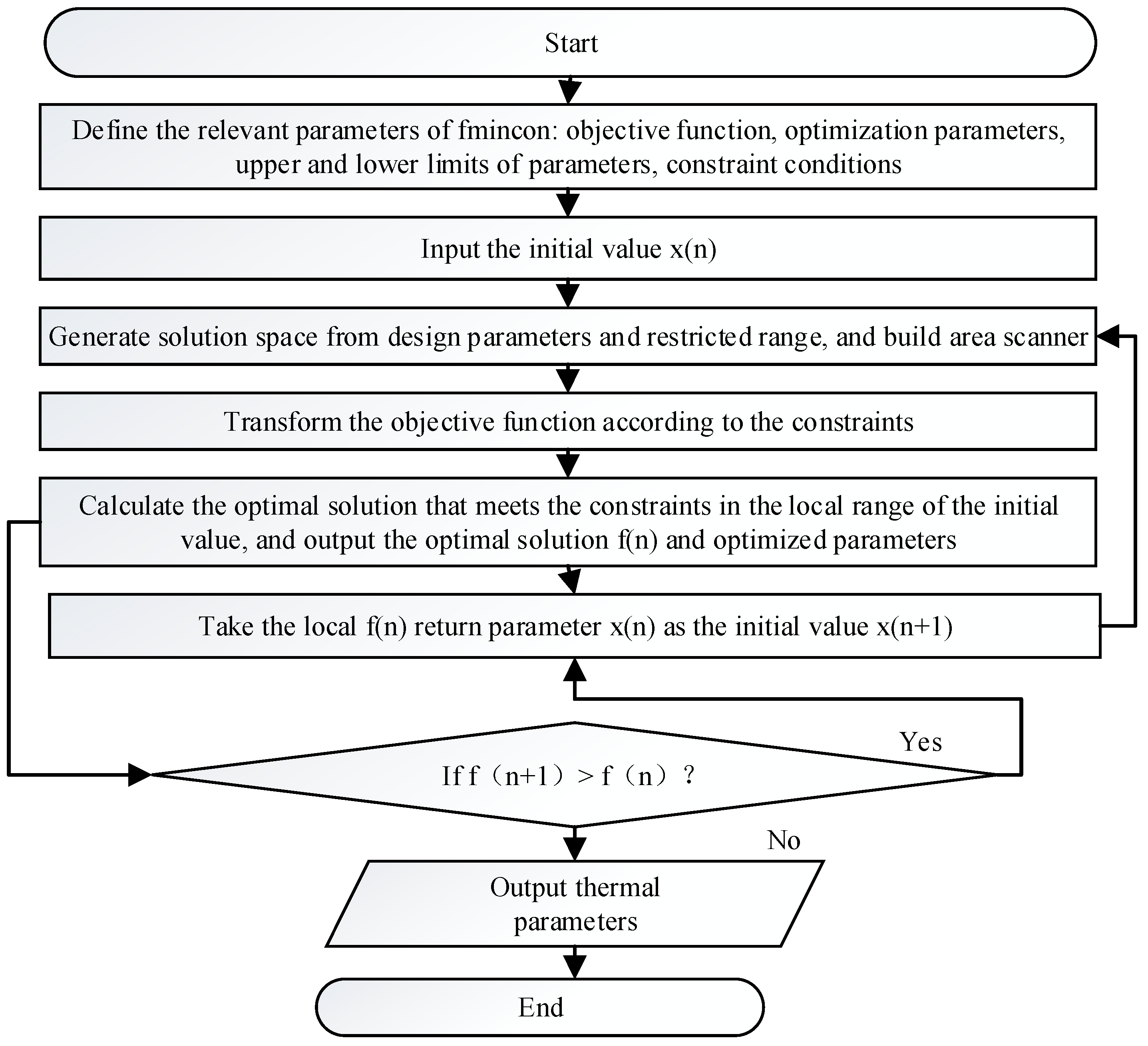
2.2. Optimization Algorithm
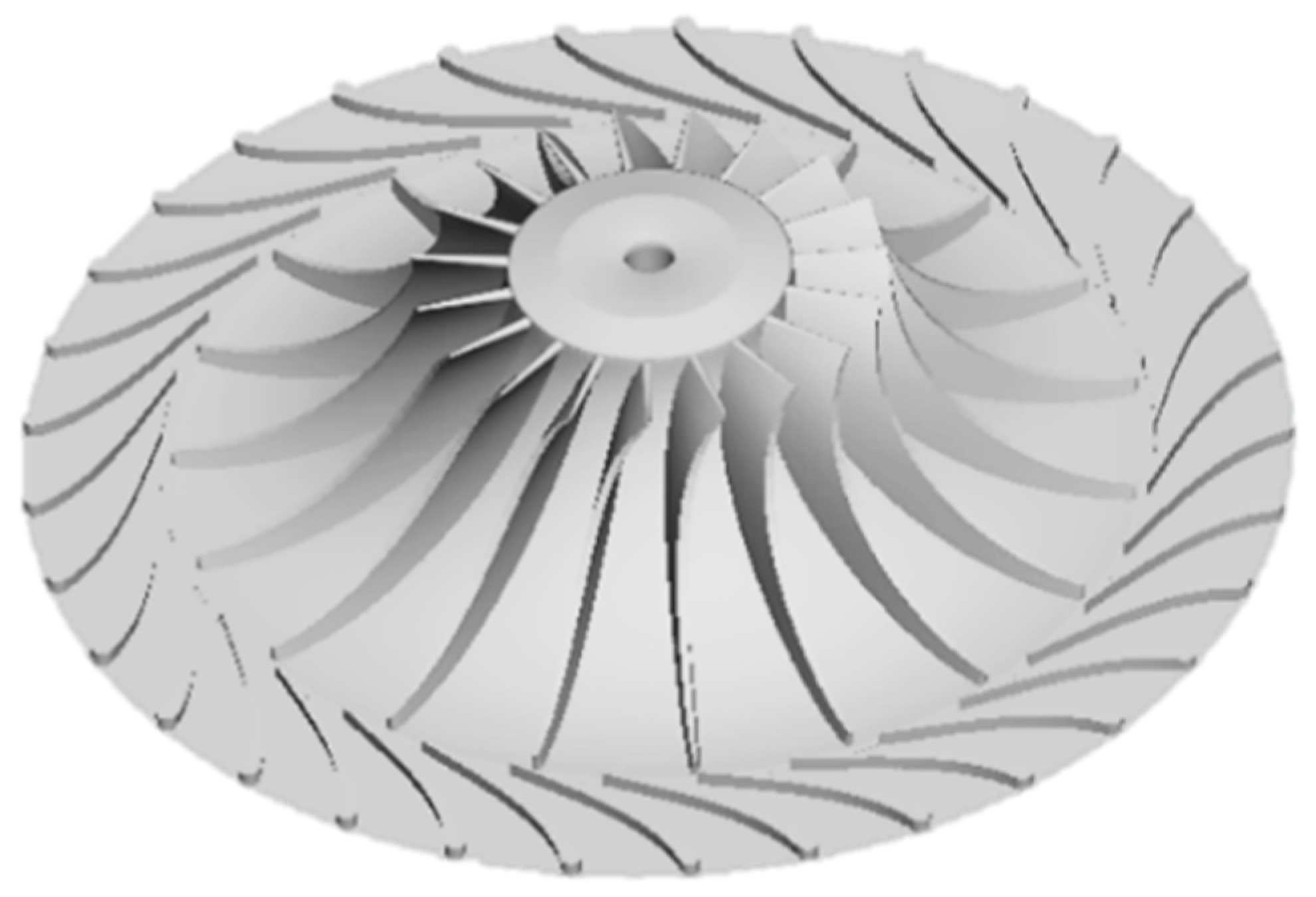
2.3. One-Dimensional Design Method
2.4. Design Constraints and Optimization Function
2.5. Optimization Result
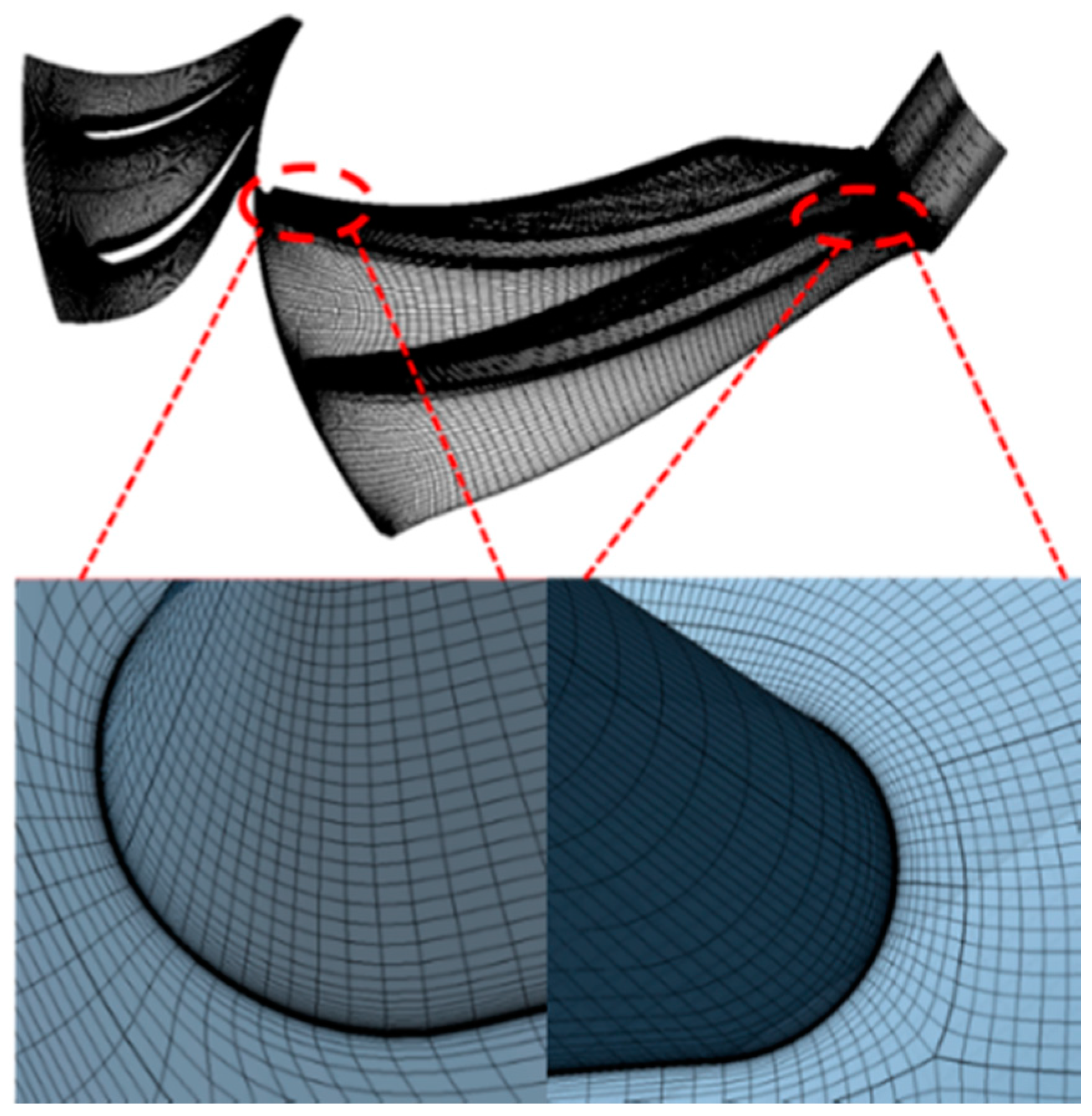
3. Numerical Methodology and Setup
4. Numerical Result Analysis and Discussion
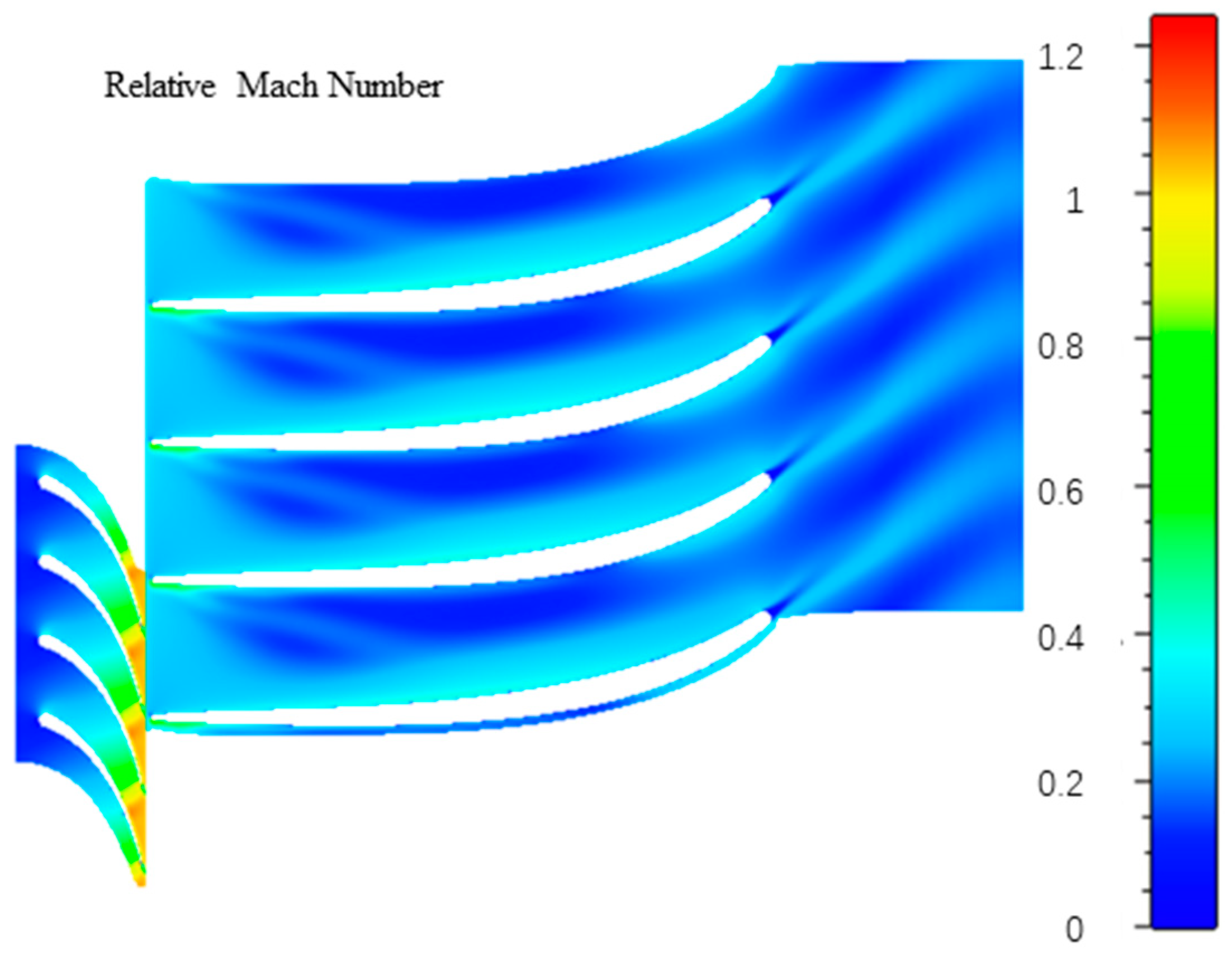
4.1. Design Working Condition
4.2. Off-Design Working Conditions
5. Conclusions
- (1)
- The developed one-dimensional optimization design method is validated by comparing the initial and optimum designs. The total-to-static efficiency for the optimum design is 85.30%, with an absolute improvement of 1.54%.
- (2)
- The performance deviations between the three-dimensional prediction result and the one-dimensional design data are lower than 2%. Under the design working condition, the total-to-static efficiency is close to the maximum value at the nominal rotational speed. The static pressure, temperature, enthalpy, and entropy distribute uniformly in the nozzle and rotor blade flow passages, demonstrating good performance for the current radial inflow turbine under the designed working conditions.
- (3)
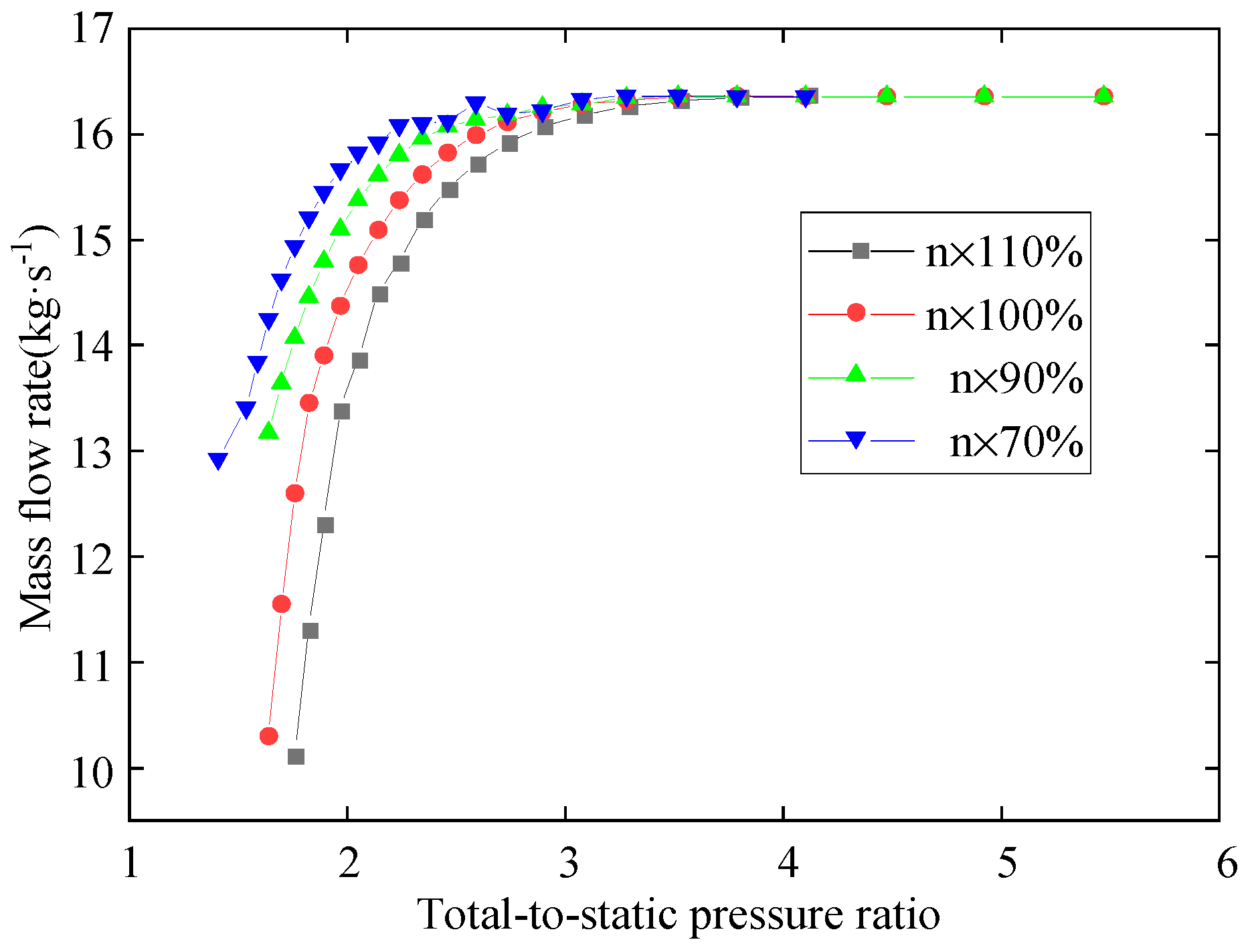
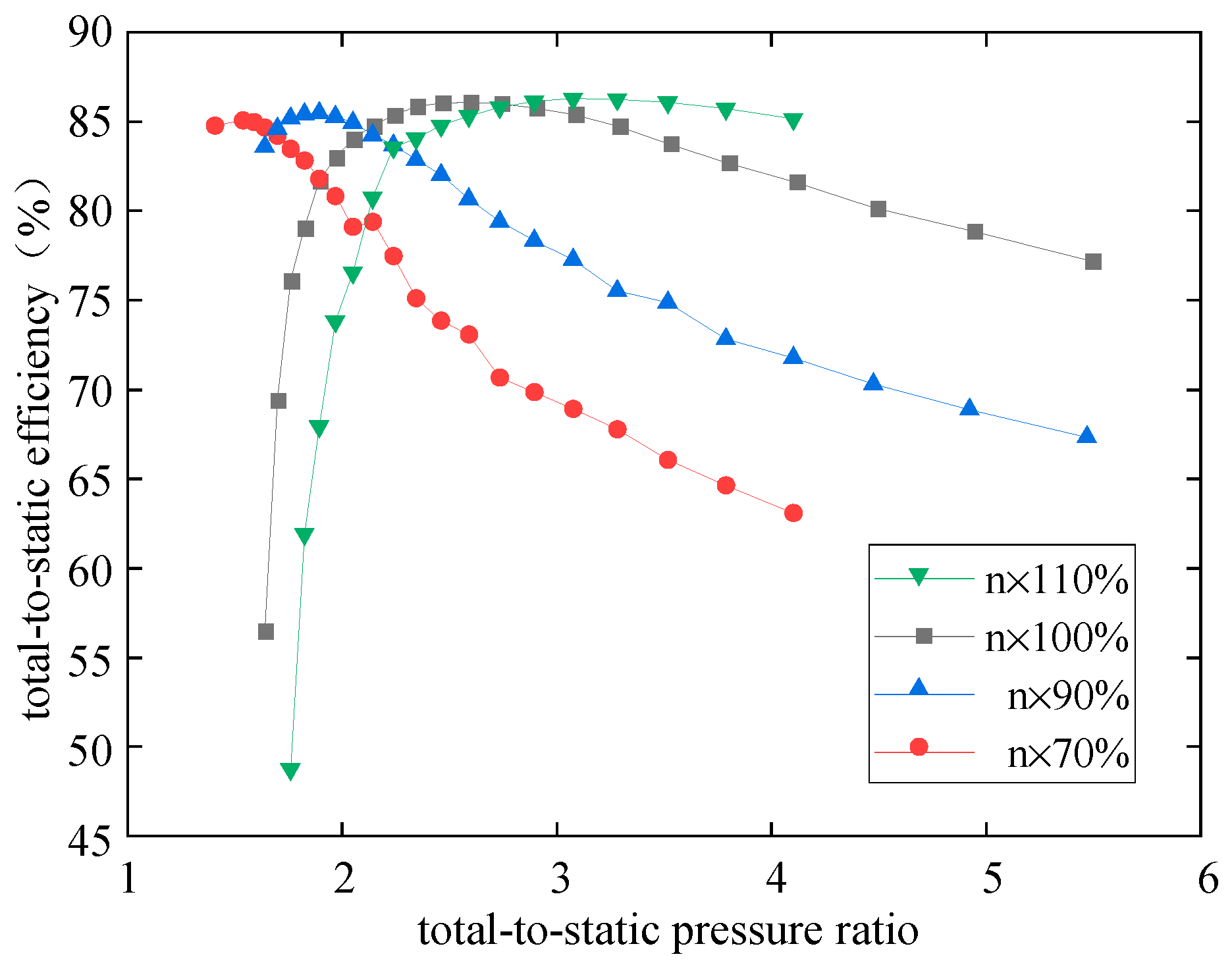
- The performance analysis revealed that the total-to-static efficiency is higher than 75% at 80–110% nominal rotational speed.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Dostal, V. A Supercritical Carbon Dioxide Cycle for Next-Generation Nuclear Reactors. Ph.D. Thesis, Massachusetts Institute of Technology, Cambridge, UK, 2004. [Google Scholar]
- Iverson, B.D.; Conboy, T.M.; Pasch, J.J.; Kruizenga, A.M. Supercritical CO2 Brayton cycles for solar-thermal energy. Appl. Energy 2013, 111, 957–970. [Google Scholar] [CrossRef] [Green Version]
- Sun, L.; Wang, Y.; Wang, D.; Xie, Y. Parametrized analysis and multi-objective optimization of supercritical CO2 (s-CO2) power cycles coupled with parabolic trough collectors. Appl. Sci. 2020, 10, 3123. [Google Scholar] [CrossRef]
- Ruiz-Casanova, E.; Rubio-Maya, C.; Pacheco-Ibarra, J.J.; Ambriz-Díaz, V.M.; Romero, C.E.; Wang, X. Thermodynamic analysis and optimization of supercritical carbon dioxide Brayton cycles for use with low-grade geothermal heat sources. Energy Convers. Manag. 2022, 216, 112978. [Google Scholar] [CrossRef]
- Liang, Y.; Bian, X.; Qian, W.; Pan, M.; Ban, Z.; Yu, Z. Theoretical analysis of a regenerative supercritical carbon dioxide Brayton cycle/organic Rankine cycle dual loop for waste heat recovery of a diesel/natural gas dual-fuel engine. Energy Convers. Manag. 2019, 197, 111845. [Google Scholar] [CrossRef]
- Wu, P.; Ma, Y.; Gao, C.; Liu, W.; Shan, J.; Huang, Y.; Wang, J.; Zhang, D.; Ran, X. A review of research and development of supercritical carbon dioxide Brayton cycle technology in nuclear engineering applications. Nucl. Eng. Des. 2019, 368, 110767. [Google Scholar] [CrossRef]
- Sashadri, L.; Raja, H.N.; Kumar, P.; Nassar, A.; Giri, G.; Moroz, L. Supercritical carbon dioxide turbomachinery options for kilowatt to gigawatt level power generation. In Proceedings of the ASME 2019 Gas Turbine India Conference, Tamil Nadu, India, 5–6 December 2020. Volume 2: Combustion, Fuels, and Emissions; Renewable Energy: Solar and Wind; Inlets and Exhausts; Emerging Technologies: Hybrid Electric Propulsion and Alternate Power Generation; GT Operation and Maintenance; Materials and Manufacturing (Including Coatings, Composites, CMCs, Additive Manufacturing), Analytics and Digital Solutions for Gas Turbines/Rotating Machinery. [Google Scholar]
- Pasch, J.; Conboy, T.; Fleming, D.; Rochau, G. Supercritical CO2 Recompression Brayton Cycle: Completed Assembly Description; Report: SAND2012-9546; Sandia National Laboratories: Albuquerque, NM, USA; Livermore, CA, USA, 2012. [Google Scholar]
- Pasch, J.; Conboy, T.; Fleming, D.; Carlson, M.; Rochau, G. Steady state supercritical carbon dioxide recompression closed Brayton cycle operating point comparison with predictions. In Proceedings of the ASME Turbo Expo 2014: Turbine Technical Conference and Exposition, Düsseldorf, Germany, 16–20 June 2014. Volume 3B: Oil and Gas Applications; Organic Rankine Cycle Power Systems; Supercritical CO2 Power Cycles, Wind Energy. [Google Scholar]
- Utamura, M.; Hasuike, H.; Yamamoto, T. Demonstration test plant of closed cycle gas turbine with supercritical CO2 as working fluid. Strojarstvo 2010, 52, 459–465. [Google Scholar]
- Cho, J.; Choi, M.; Baik, Y.J.; Lee, G.; Ra, H.S.; Kim, B.; Kim, M. Development of the turbomachinery for the supercritical carbon dioxide power cycle. Int. J. Energy Res. 2016, 40, 587–599. [Google Scholar] [CrossRef]
- Saeed, M.; Berrouk, A.S.; Burhani, B.M.; Alatyar, A.M.; Wahedi, Y.F.A. Turbine design and optimization for a supercritical CO2 cycle using a multifaceted approach based on deep neural network. Energies 2021, 14, 7807. [Google Scholar] [CrossRef]
- Zhou, A.; Song, J.; Li, X.; Ren, X.; Gu, C. Aerodynamic design and numerical analysis of a radial inflow turbine for the supercritical carbon dioxide Brayton cycle. Appl. Therm. Eng. 2018, 132, 245–255. [Google Scholar] [CrossRef]
- Lv, G.; Yang, J.; Shao, W.; Wang, X. Aerodynamic design optimization of radial-inflow turbine in supercritical CO2 cycles using a one-dimensional model. Energy Convers. Manag. 2018, 165, 827–839. [Google Scholar] [CrossRef]
- Lee, S.; Yaganegi, G.; Mee, D.J.; Guan, Z.; Gurgenci, H. Part-load performance prediction model for supercritical CO2 radial inflow turbines. Energy Convers. Manag. 2021, 235, 113964. [Google Scholar] [CrossRef]
- Persky, R.; Sauret, E. Loss models for on and off-design performance of radial inflow turbomachinery. Appl. Ther. Eng. 2019, 150, 1066–1077. [Google Scholar] [CrossRef]
- Keep, J.A.; Jahn, I.H.J. Numerical loss investigation of a small scale, low specific speed supercritical CO2 radial inflow turbine. J. Eng. Gas Turbines Power 2019, 141, 091003. [Google Scholar] [CrossRef]
- Yang, J.; Zhao, F.; Zhang, M.; Liu, Y.; Wang, X. Numerical analysis of labyrinth seal performance for the impeller backface cavity of a supercritical CO2 radial inflow turbine. Comput. Model. Eng. Sci. 2021, 126, 935–953. [Google Scholar] [CrossRef]
- Shu, S.Z.; Zhu, L.; Ke, X.L.; Jiang, Z.K. Principle of Turbomachinery, 3rd ed.; Tsinghua University Press: Beijing, China, 1991; pp. 150–172. [Google Scholar]
- Lemmon, E.W.; Mclinden, M.O.; Huber, M.L. NIST Standard Reference Database 23, Version 10.0, Reference Fluid Thermodynamic and Transport Properties-REFPROP; National Institute of Standards and Technology (NIST): Boulder, CO, USA, 2018. [Google Scholar]
- Chen, Y.; Cheng, Z.; Huang, Z. Optimal design of lifting mechanism of linkage hydraulic lifting dam based on matlab. J. Phys. Conf. Ser. 2021, 1948, 012128. [Google Scholar] [CrossRef]
- Li, Y.S.; Lu, G. Radial Inflow Turbine and Centrifugal Compressor, 1st ed.; China Machine Press: Beijing, China, 1987; pp. 34–80. [Google Scholar]
- Ameli, A.; Uusitalo, A.; Turunen-Saaresti, T.; Backman, J. Numerical sensitivity analysis for supercritical CO2 radial turbine performance and flow field. Energy Procedia 2017, 129, 1117–1124. [Google Scholar] [CrossRef]
- Lou, L.; Du, W.; Wang, S.T. Effect of turbulence model on the performance of a SCO2 radial turbine. Heat Transf. Res. 2020, 51, 173–192. [Google Scholar]




| Design Variable | Value Region |
|---|---|
| Velocity ratio xa | 0.63~0.72 |
| Reaction degree Ω | 0.36~0.5 |
| Nozzle velocity coefficient φ | 0.92~0.97 |
| Rotor inlet absolute flow angle α1 (°) | 14~25 |
| Rotor exit relative flow angle β2 (°) | 20~45 |
| Wheel diameter ratio | 0.35~0.55 |
| Rotor blade velocity coefficient ψ | 0.75~0.90 |
| Constrained Variable | Value |
|---|---|
| Incidence angle at rotor inlet i1 (°) | −20~+10 |
| Mach number at rotor inlet | ≤1.4 |
| Absolute flow angle at rotor exit (°) | 85~95 |
| Relative shroud diameter at rotor exit | ≤0.85 |
| Relative hub diameter at rotor exit | ≥0.12 |
| Relative blade height at rotor inlet | 0.02~0.17 |
| Parameter | Specification |
|---|---|
| Mass flow rate (kg/s) | 16.0 |
| Rotating speed (r/min) | 42,000 |
| Inlet total pressure (MPa) | 24.6 |
| Inlet total temperature (K) | 823 |
| Outlet static pressure (MPa) | 8.5 |
| Output power (MW) | 2.1 |
| Parameter | Initial Value | Optimum Value |
|---|---|---|
| Velocity ratio xa | 0.650 | 0.662 |
| Reaction degree Ω | 0.470 | 0.483 |
| Nozzle velocity coefficient φ | 0.95 | 0.97 |
| Rotor inlet absolute flow angle α1 (°) | 16.0 | 16.0 |
| Rotor exit relative flow angle β2 (°) | 21.4 | 25.0 |
| 0.430 | 0.417 | |
| Rotor blade velocity coefficient ψ | 0.85 | 0.90 |
| Rotor inlet relative flow angle β1 (°) | 85.55 | 85.00 |
| Rotor exit absolute flow angle α2 (°) | 78.0 | 75.0 |
| Nozzle exit diameter (mm) | 170.3 | 170.4 |
| Rotor inlet diameter (mm) | 163.8 | 166.8 |
| Rotor inlet blade height (mm) | 3.0 | 3.3 |
| Rotor exit hub diameter (mm) | 43.1 | 48.6 |
| Rotor exit shroud diameter (mm) | 89.8 | 85.6 |
| Specific speed | 13.76 | 13.84 |
| Output power (MW) | 2.056 | 2.118 |
| Total-to-static efficiency ηs (%) | 83.76 | 85.30 |
| Gas Type | Resolution | Total-to-Static Efficiency |
|---|---|---|
| Perfect gas | / | 82.82% |
| Real gas | 101 × 101 | 85.78% |
| 201 × 201 | 85.77% | |
| 301 × 301 | 85.77% |
| Parameter | Design | Prediction | Error (%) |
|---|---|---|---|
| Total-to-static expansion ratio | 2.896 | 2.896 | 0.00 |
| Mass flow rate (kg/s) | 16 | 16.213 | 1.33 |
| Output power (MW) | 2.118 | 2.135 | 0.80 |
| Total-to-static efficiency (%) | 85.30 | 85.77 | 0.47 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tong, Z.; Song, Z.; Zhang, C.; Xing, H.; Sun, Y. One-Dimensional Optimization Design of Supercritical Carbon Dioxide Radial Inflow Turbine and Performance Analysis under Off-Design Conditions. Appl. Sci. 2022, 12, 3882. https://doi.org/10.3390/app12083882
Tong Z, Song Z, Zhang C, Xing H, Sun Y. One-Dimensional Optimization Design of Supercritical Carbon Dioxide Radial Inflow Turbine and Performance Analysis under Off-Design Conditions. Applied Sciences. 2022; 12(8):3882. https://doi.org/10.3390/app12083882
Chicago/Turabian StyleTong, Zhiting, Zedong Song, Chao Zhang, Hao Xing, and Yuxuan Sun. 2022. "One-Dimensional Optimization Design of Supercritical Carbon Dioxide Radial Inflow Turbine and Performance Analysis under Off-Design Conditions" Applied Sciences 12, no. 8: 3882. https://doi.org/10.3390/app12083882
APA StyleTong, Z., Song, Z., Zhang, C., Xing, H., & Sun, Y. (2022). One-Dimensional Optimization Design of Supercritical Carbon Dioxide Radial Inflow Turbine and Performance Analysis under Off-Design Conditions. Applied Sciences, 12(8), 3882. https://doi.org/10.3390/app12083882






