Effect of Dynamic Loading Conditions on Maximizing Energy Dissipation of Metallic Dampers
Abstract
:1. Introduction
2. Design Concept and Optimization Approaches
2.1. Design Concept of a Prismatic Metallic Damper
2.2. Optimization Approach
2.3. Static and Dynamic Optimization
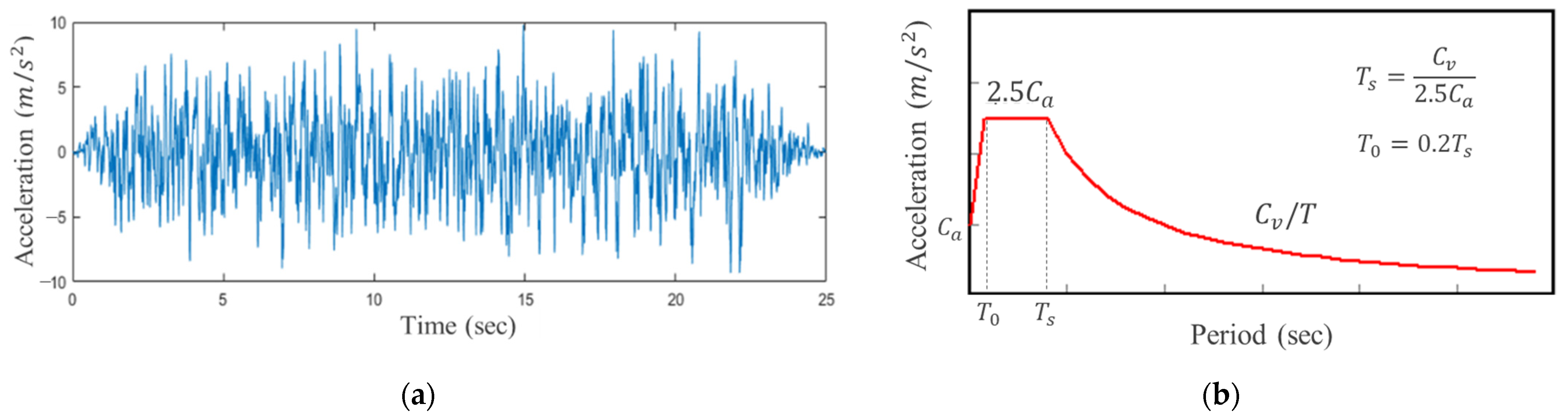
3. Dynamic Analysis of Static Optimization
3.1. Design Variables
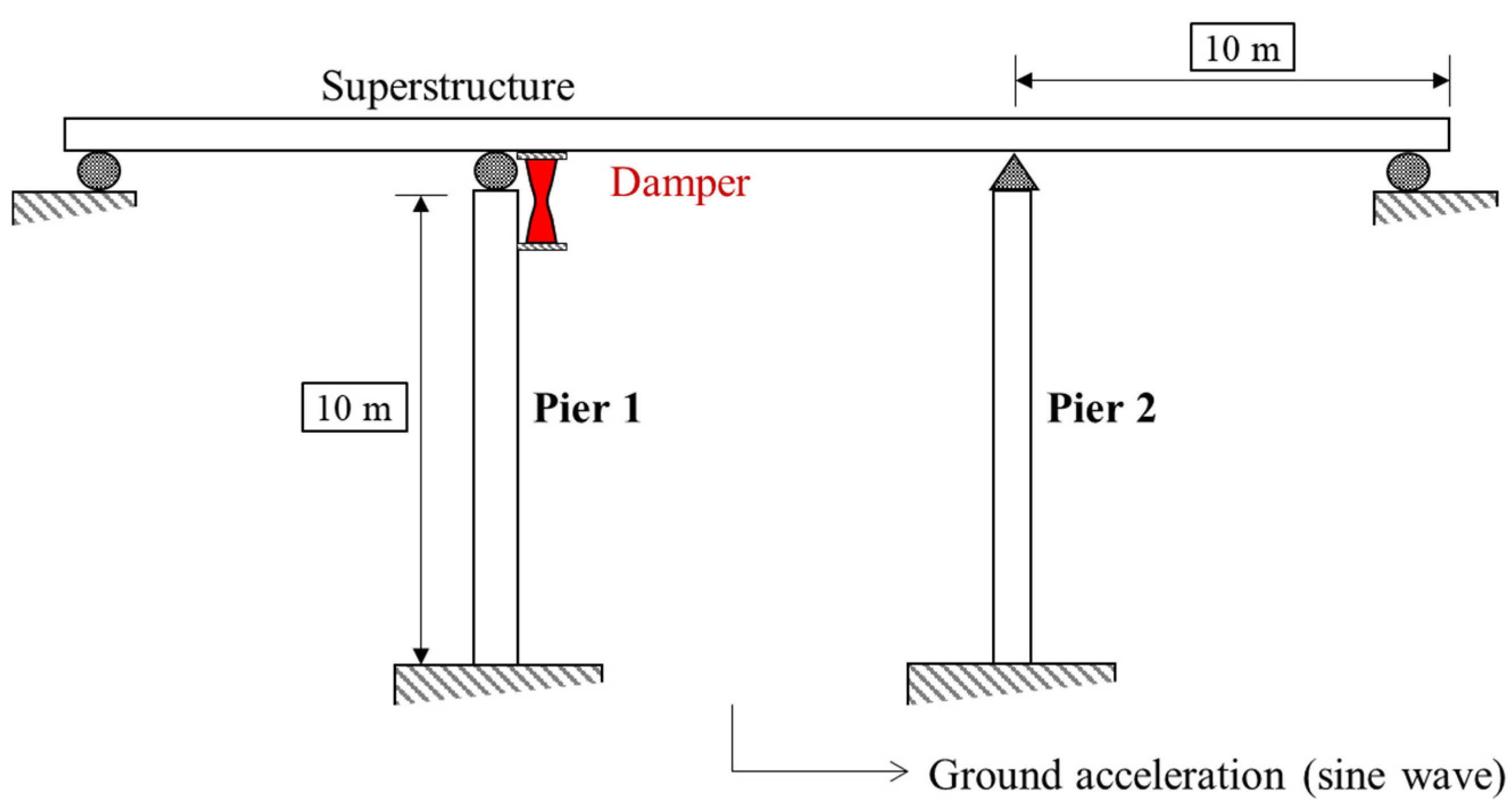
3.2. Finite Element Model
3.3. Static Optimization Results
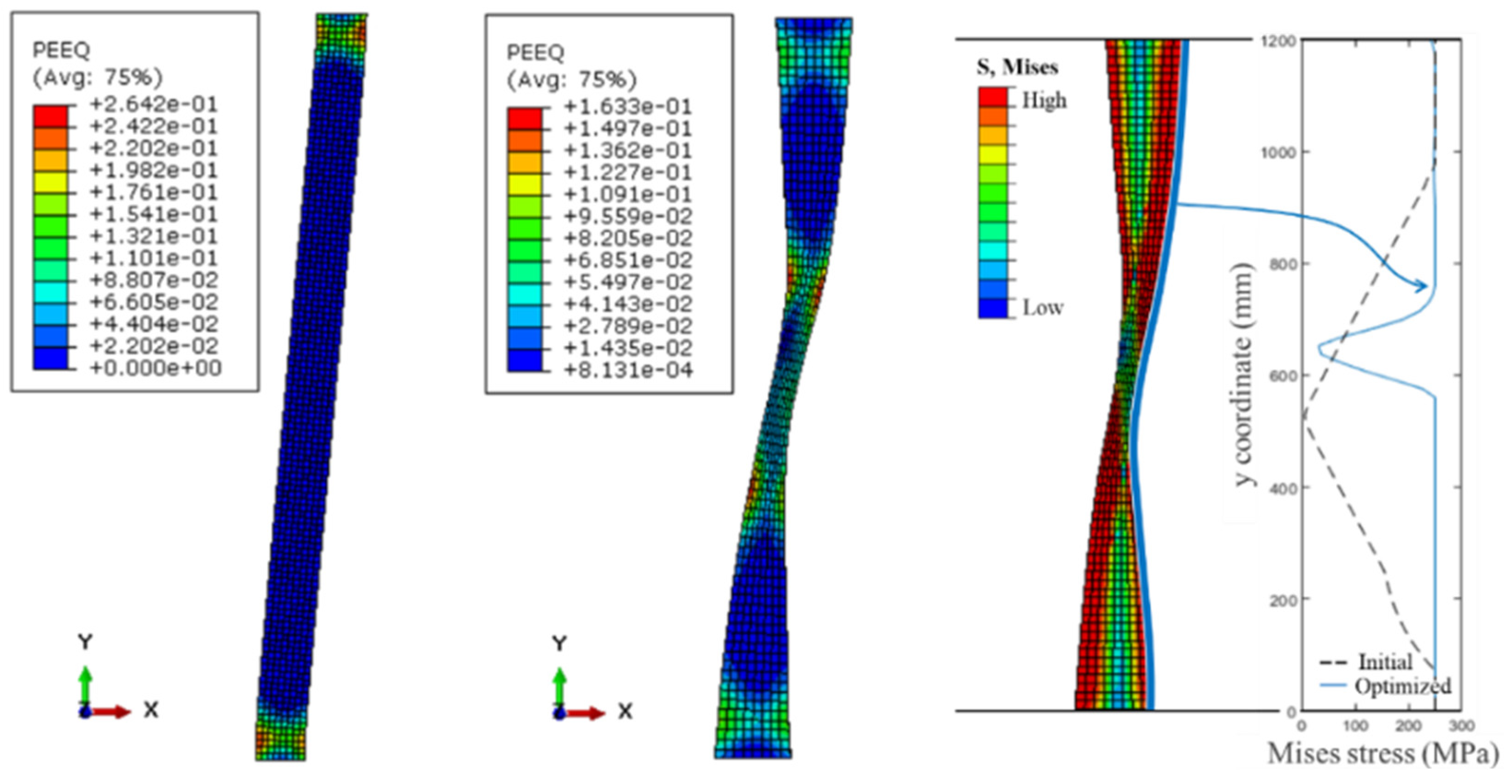
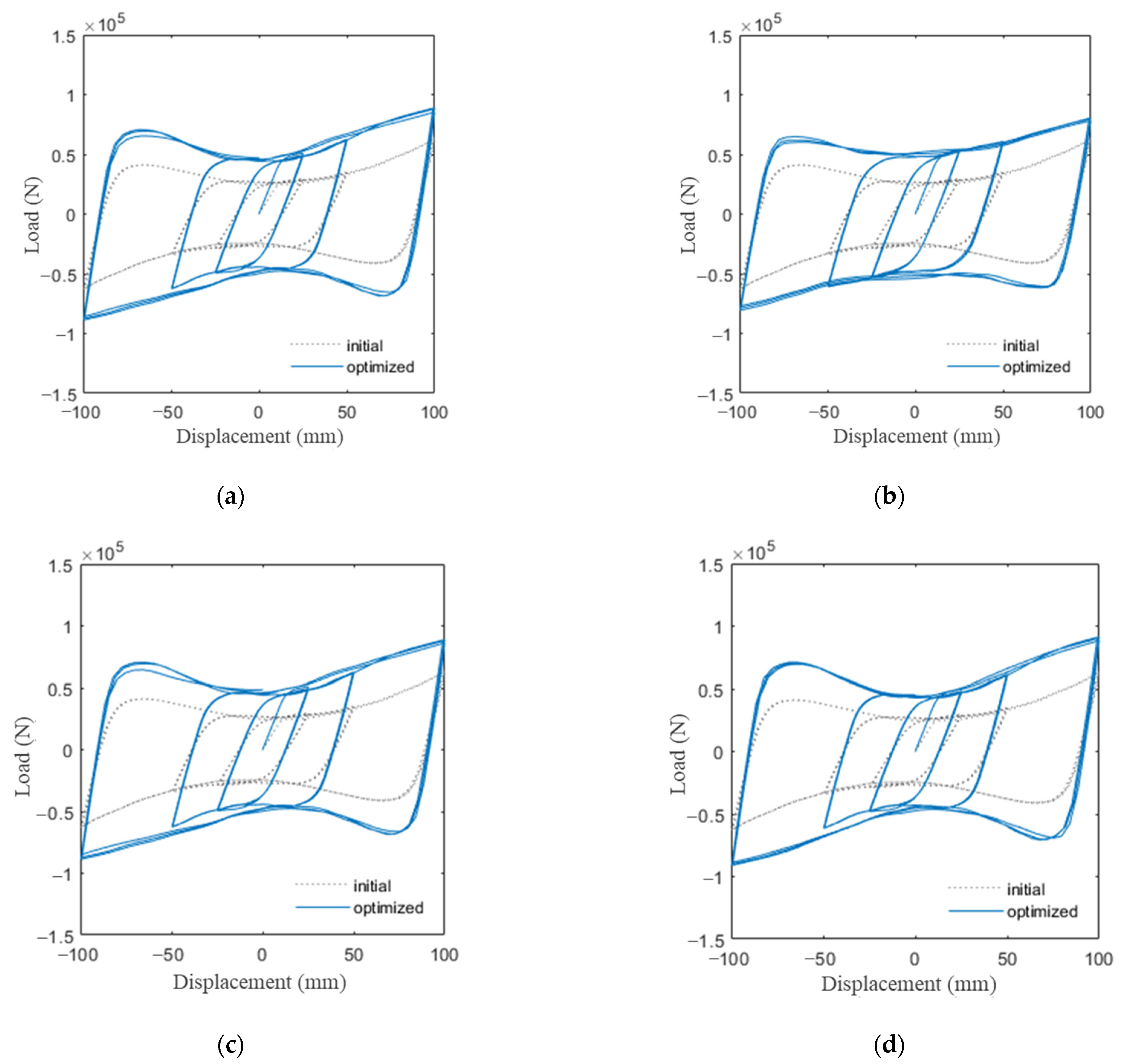
3.4. Dynamic Analysis of the Statically Optimized Damper
4. Dynamic Optimization
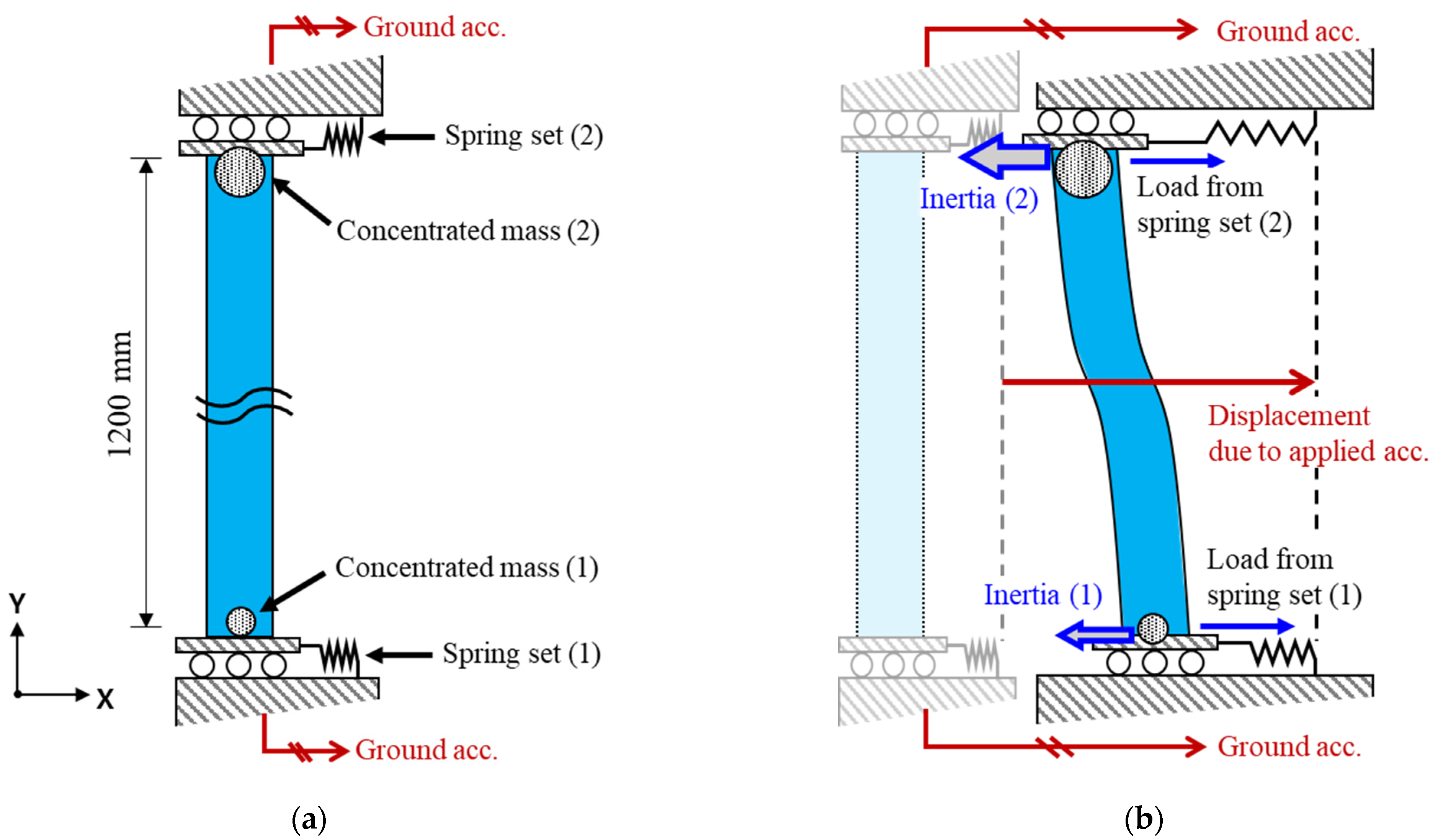
4.1. An Analytical Model for Dynamic Optimization
4.2. Dynamic Optimization Results
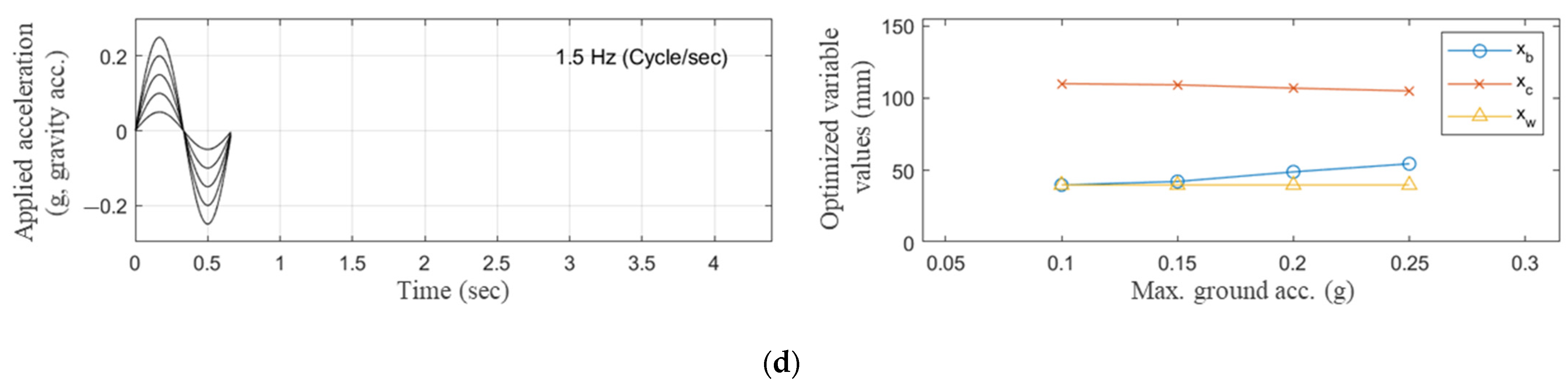
4.3. Effect of the Excitation Frequencies to the Dynamic Optimization
4.4. Seismic Analysis of the Optimized Results
5. Conclusions
- The optimized shape obtained through the static analysis showed an hourglass shape in which the width of both ends was increased, and the width of the center was reduced. This trend was similar to the results of previous studies. However, in the static analysis, it was difficult to determine the different optimal shapes for all stroke sizes, or to determine an appropriate virtual stroke for optimization.
- Through optimization with static analysis, the stiffness of the damper increased. Accordingly, the optimized shape dissipated a large amount of energy when the damper was deformed due to large earthquakes. In the case of small earthquakes, however, the optimized damper may undergo less deformation and dissipate only a small amount of energy. Identical results were obtained using the structural prototype bridge FE model.
- Thus, further optimization based on a dynamic analysis was carried out to determine the optimal shapes that dissipate the maximum amount of energy during relatively small earthquakes. To generalize the optimization method based on the dynamic analysis, an ideal analytical model was proposed with the spring set and concentrated mass.
- Through the proposed analytical model, an additional optimized shape for small earthquake accelerations was developed. The optimized shape at low acceleration and high frequency exhibited a slight reduction in width at both ends, unlike previous results. The new shape could lead to a greater amount of energy dissipation for small seismic loading.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Lee, C.-H.; Ju, Y.K.; Min, J.-K.; Lho, S.-H.; Kim, S.-D. Non-uniform steel strip dampers subjected to cyclic loadings. Eng. Struct. 2015, 99, 192–204. [Google Scholar] [CrossRef]
- Shu, G.; Li, Z. Parametric identification of the Bouc-Wen model by a modified genetic algorithm: Application to evaluation of metallic dampers. Earthq. Struct. 2017, 13, 397–407. [Google Scholar]
- Mota-Páez, S.; Escolano-Margarit, D.; Benavent-Climent, A. Seismic Response of RC Frames with a Soft First Story Retrofitted with Hysteretic Dampers under Near-Fault Earthquakes. Appl. Sci. 2021, 11, 1290. [Google Scholar] [CrossRef]
- Gandelli, E.; De Domenico, D.; Quaglini, V. Cyclic engagement of hysteretic steel dampers in braced buildings: A parametric investigation. Bull. Earthq. Eng. 2021, 19, 5219–5251. [Google Scholar] [CrossRef]
- Li, Z.; Hu, Y.; Tu, X. Wind-Induced Response and Its Controlling of Long-Span Cross-Rope Suspension Transmission Line. Appl. Sci. 2022, 12, 1488. [Google Scholar] [CrossRef]
- Song, Y.; Zhang, M.; Øiseth, O.; Rønnquist, A. Wind deflection analysis of railway catenary under crosswind based on nonlinear finite element model and wind tunnel test. Mech. Mach. Theory 2022, 168, 104608. [Google Scholar] [CrossRef]
- Deng, K.; Pan, P.; Wang, C. Development of crawler steel damper for bridges. J. Constr. Steel Res. 2013, 85, 140–150. [Google Scholar] [CrossRef]
- Pan, P.; Yan, H.; Wang, T.; Xu, P.; Xie, Q. Development of steel dampers for bridges to allow large displacement through a vertical free mechanism. Earthq. Eng. Eng. Vib. 2014, 13, 375–388. [Google Scholar] [CrossRef]
- Salem Milani, A.; Dicleli, M. Systematic development of a new hysteretic damper based on torsional yielding: Part I—Design and development. Earthq. Eng. Struct. Dyn. 2016, 45, 845–867. [Google Scholar] [CrossRef]
- Yamazaki, S.; Usami, T.; Nonaka, T. Developing a new hysteretic type seismic damper (BRRP) for steel bridges. Eng. Struct. 2016, 124, 286–301. [Google Scholar] [CrossRef]
- Zheng, J.; Zhang, C.; Li, A. Experimental investigation on the mechanical properties of curved metallic plate dampers. Appl. Sci. 2020, 10, 269. [Google Scholar] [CrossRef] [Green Version]
- Gonzalez-Sanz, G.; Escolano-Margarit, D.; Benavent-Climent, A. A New Stainless-Steel Tube-in-Tube Damper for Seismic Protection of Structures. Appl. Sci. 2020, 10, 1410. [Google Scholar] [CrossRef] [Green Version]
- Deng, K.; Pan, P.; Sun, J.; Liu, J.; Xue, Y. Shape optimization design of steel shear panel dampers. J. Constr. Steel Res. 2014, 99, 187–193. [Google Scholar] [CrossRef]
- Deng, K.; Pan, P.; Su, Y.; Xue, Y. Shape optimization of U-shaped damper for improving its bi-directional performance under cyclic loading. Eng. Struct. 2015, 93, 27–35. [Google Scholar] [CrossRef]
- Zhang, Z.; Ou, J.; Li, D.; Zhang, S. Optimization design of coupling beam metal damper in shear wall structures. Appl. Sci. 2017, 7, 137. [Google Scholar] [CrossRef] [Green Version]
- Nakashima, M.; Saburi, K.; Tsuji, B. Energy input and dissipation behaviour of structures with hysteretic dampers. Earthq. Eng. Struct. Dyn. 1996, 25, 483–496. [Google Scholar] [CrossRef]
- Teruna, D.R.; Majid, T.A.; Budiono, B. Experimental study of hysteretic steel damper for energy dissipation capacity. Adv. Civ. Eng. Mater. 2015, 2015, 631726. [Google Scholar] [CrossRef] [Green Version]
- Xu, Y.H.; Li, A.Q.; Zhou, X.D.; Sun, P. Shape optimization study of mild steel slit dampers. In Advanced Materials Research; Trans Tech Publications Ltd.: Bäch, Switzerland, 2011; pp. 2434–2438. [Google Scholar]
- Lagaros, N.D. Design Optimization of Active and Passive Structural Control Systems; IGI Global: Hershey, PA, USA, 2012. [Google Scholar]
- Golzan, S.B.; Langlois, S.; Legeron, F.P. Implementation of a simplified method in design of hysteretic dampers for isolated highway bridges. J. Bridge Eng. 2017, 22, 04016127. [Google Scholar] [CrossRef]
- Romo, L.; Benavent-Climent, A.; Morillas, L.; Escolano, D.; Gallego, A. Health monitoring of a new hysteretic damper subjected to earthquakes on a shaking table. Earthq. Struct. 2015, 8, 485–509. [Google Scholar] [CrossRef]
- Ohsaki, M.; Nakajima, T. Optimization of link member of eccentrically braced frames for maximum energy dissipation. J. Constr. Steel Res. 2012, 75, 38–44. [Google Scholar] [CrossRef] [Green Version]
- Aydin, E. A simple damper optimization algorithm for both target added damping ratio and interstorey drift ratio. Earthq. Struct. 2013, 5, 83–109. [Google Scholar] [CrossRef]
- Ghabraie, K.; Chan, R.; Huang, X.; Xie, Y.M. Shape optimization of metallic yielding devices for passive mitigation of seismic energy. Eng. Struct. 2010, 32, 2258–2267. [Google Scholar] [CrossRef] [Green Version]
- Steinbuch, R. Evolutionary Optimization of Passive Compensators to Improve Earthquake Resistance. In Design Optimization of Active and Passive Structural Control Systems; IGI Global: Hershey, PA, USA, 2013; pp. 250–273. [Google Scholar]
- Mohammadi, R.K.; Mirjalaly, M.; Mirtaheri, M.; Nazeryan, M. Comparison between uniform deformation method and Genetic Algorithm for optimizing mechanical properties of dampers. Earthq. Struct. 2018, 14, 001–010. [Google Scholar]
- He, H.; Wang, X.; Zhang, X. Energy-dissipation performance of combined low yield point steel plate damper based on topology optimization and its application in structural control. Adv. Mater. Sci. Eng. 2016, 2016, 5654619. [Google Scholar] [CrossRef] [Green Version]
- Denimal, E.; Renson, L.; Wong, C.; Salles, L. Topology optimisation of friction under-platform dampers using moving morphable components and the efficient global optimization algorithm. Struct. Multidiscip. Optim. 2022, 65, 56. [Google Scholar] [CrossRef]
- Kim, Y.-C.; Mortazavi, S.J.; Farzampour, A.; Hu, J.-W.; Mansouri, I.; Awoyera, P.O. Optimization of the Curved Metal Damper to Improve Structural Energy Dissipation Capacity. Buildings 2022, 12, 67. [Google Scholar] [CrossRef]
- Mathworks. Optimization Toolbox: User’s Guide (r2018a); Mathworks: Natick, MA, USA, 2018. [Google Scholar]
- Yoon, J.-H.; Park, J.-W.; Lim, Y.-M.; Yoon, G.-H. Shape Optimization of Uniaxial Vibrating Metal Damper. J. Comput. Struct. Eng. Inst. Korea 2017, 30, 313–318. [Google Scholar] [CrossRef]
- Hilber, H.M.; Hughes, T.J.; Taylor, R.L. Improved numerical dissipation for time integration algorithms in structural dynamics. Earthq. Eng. Struct. Dyn. 1977, 5, 283–292. [Google Scholar] [CrossRef] [Green Version]
- ABAQUS. Abaqus User Manual; ABAQUS: Providence, RI, USA, 2020. [Google Scholar]
- Uniform Building Code. International Building Code; International Code Council: Washington, DC, USA, 1997. [Google Scholar]
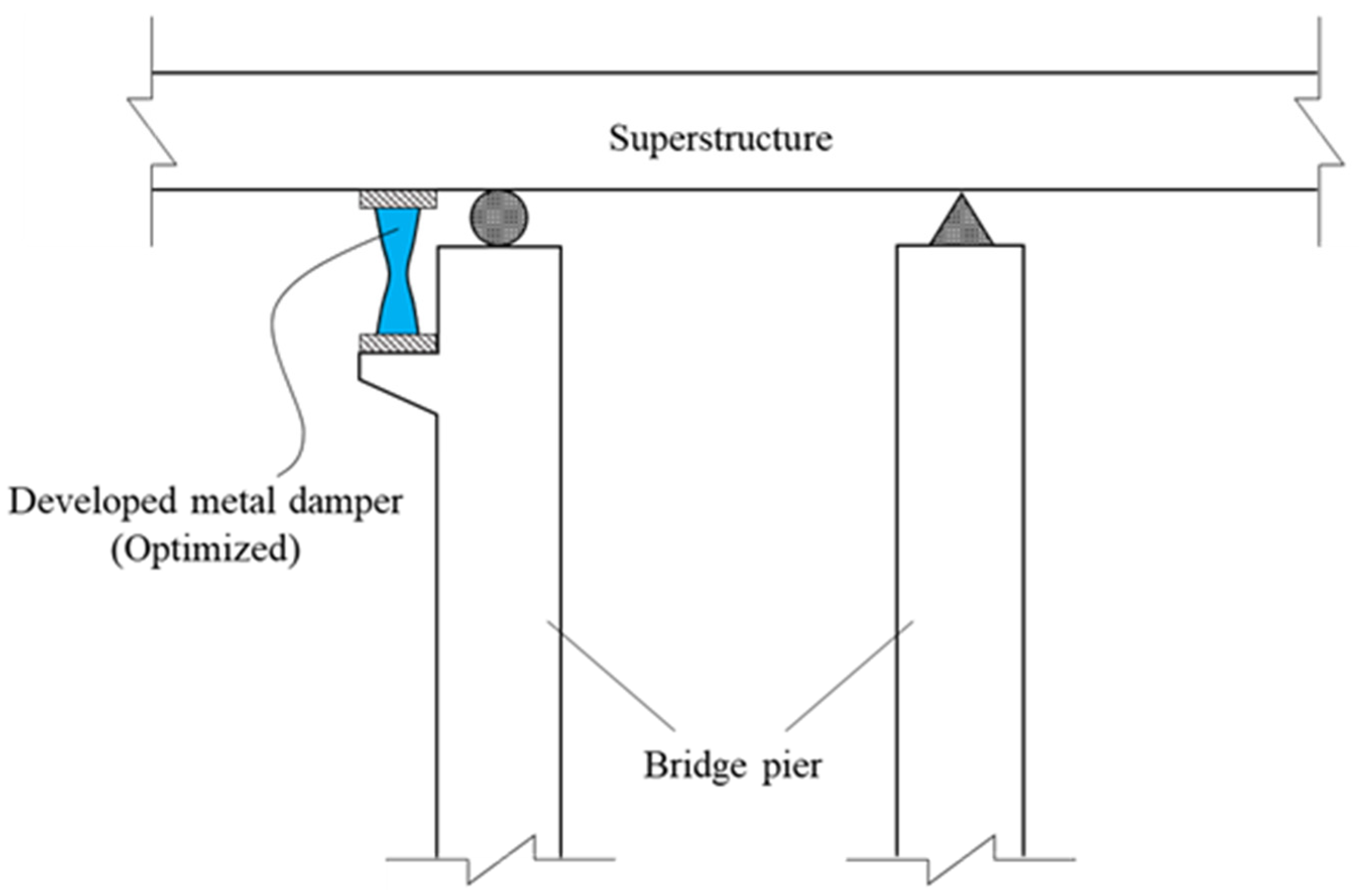
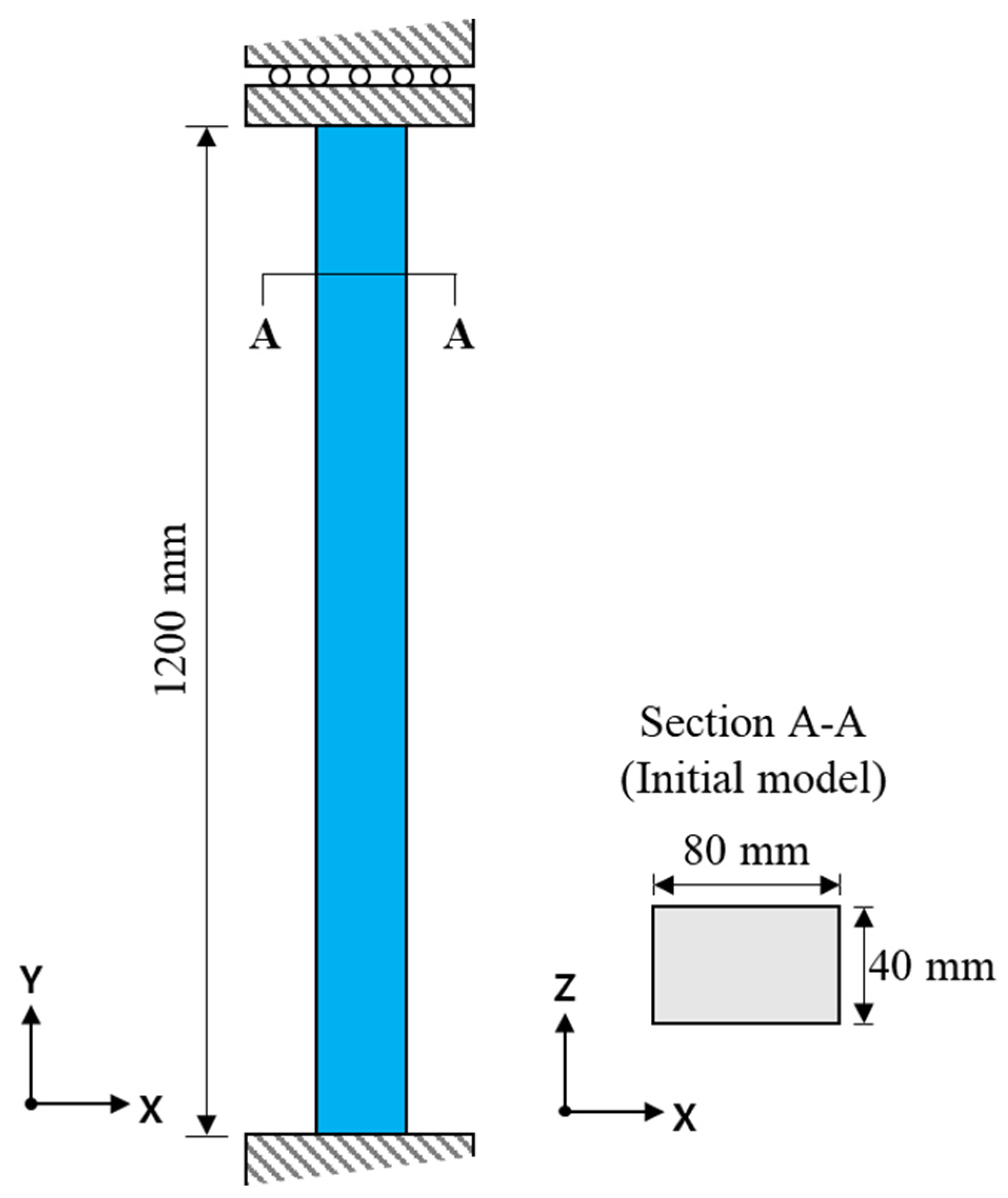
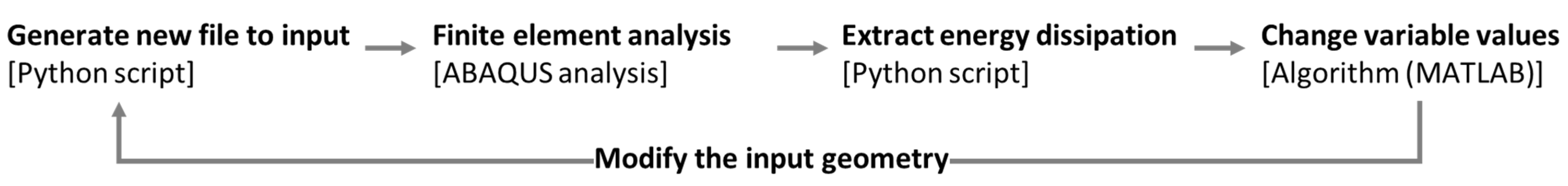
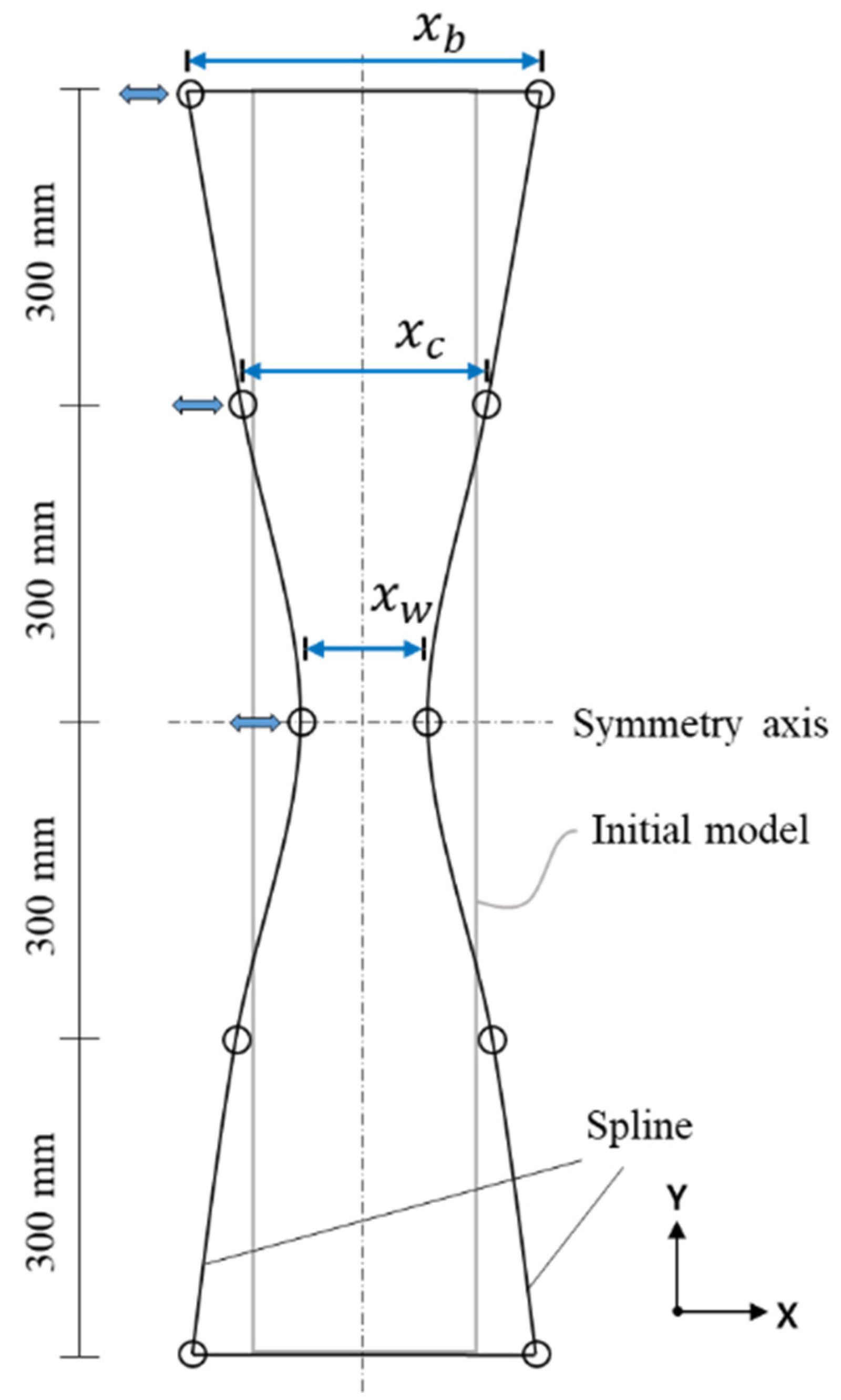

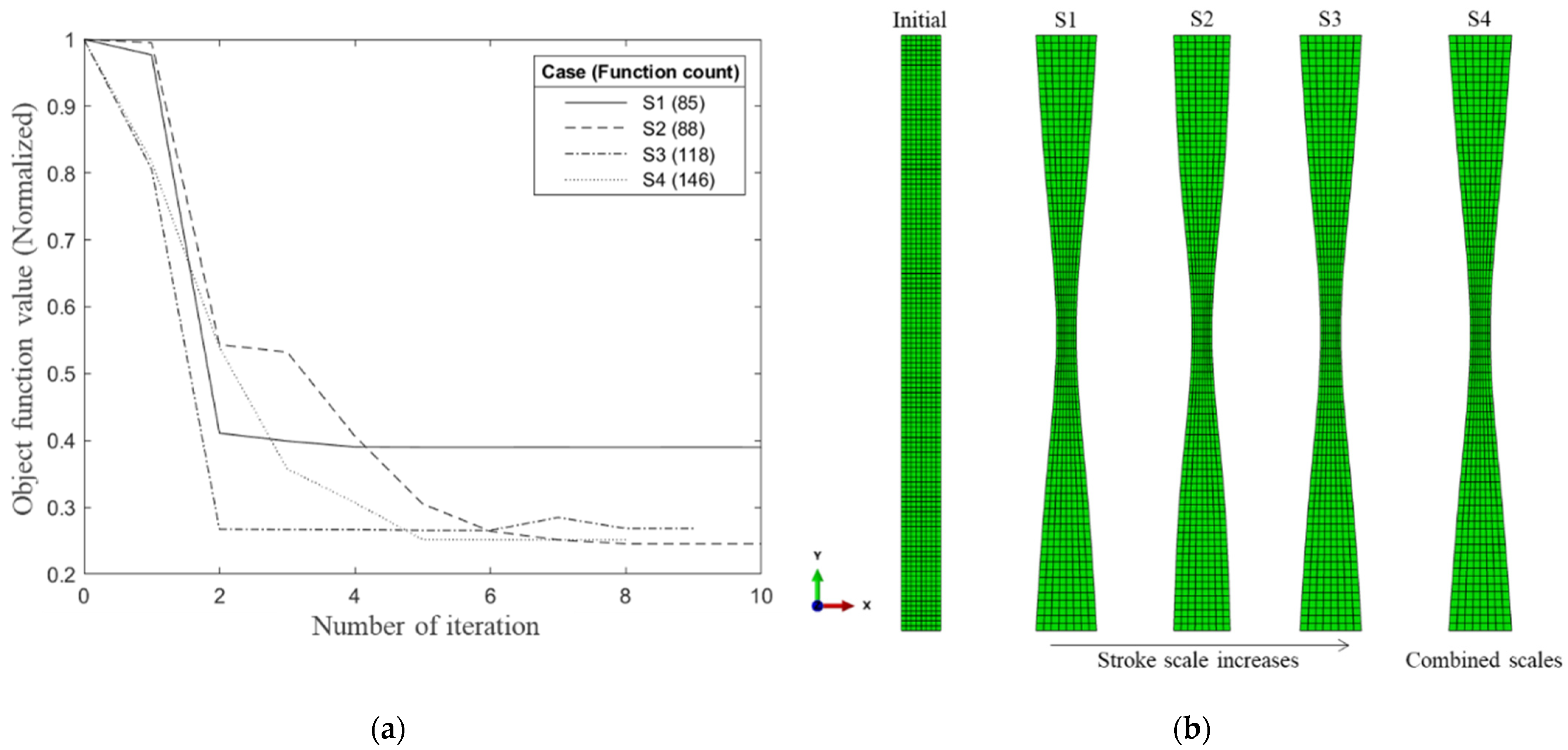



| Cases | Initial | S1 | S2 | S3 | S4 |
|---|---|---|---|---|---|
| Optimized Stroke size | 25 mm | 50 mm | 100 mm | Max. 100 mm * | |
| [mm] | 80 | 123.37 | 113.87 | 123.23 | 126.61 |
| [mm] | 80 | 81.34 | 84.60 | 81.42 | 80.10 |
| [mm] | 80 | 40.00 | 40.00 | 40.00 | 40.00 |
| ] | 3.840 | 3.840 | 3.839 | 3.840 | 3.836 |
| Elastic stiffness [MN/m] | 2.314 | 3.705 | 3.730 | 3.709 | 3.672 |
| ALLPD [kN·m] | 53.76 | 93.99 | 91.01 | 93.91 | 93.64 |
| Improvement [%] | +74.9 | +69.3 | +74.7 | +74.2 | |
| Structural Model Analysis | Initial | Optimized (Case S1) | Initial | Optimized (Case S1) |
|---|---|---|---|---|
| Dynamic Analysis (Max. 0.1 g) | Dynamic Analysis (Max. 0.2 g) | |||
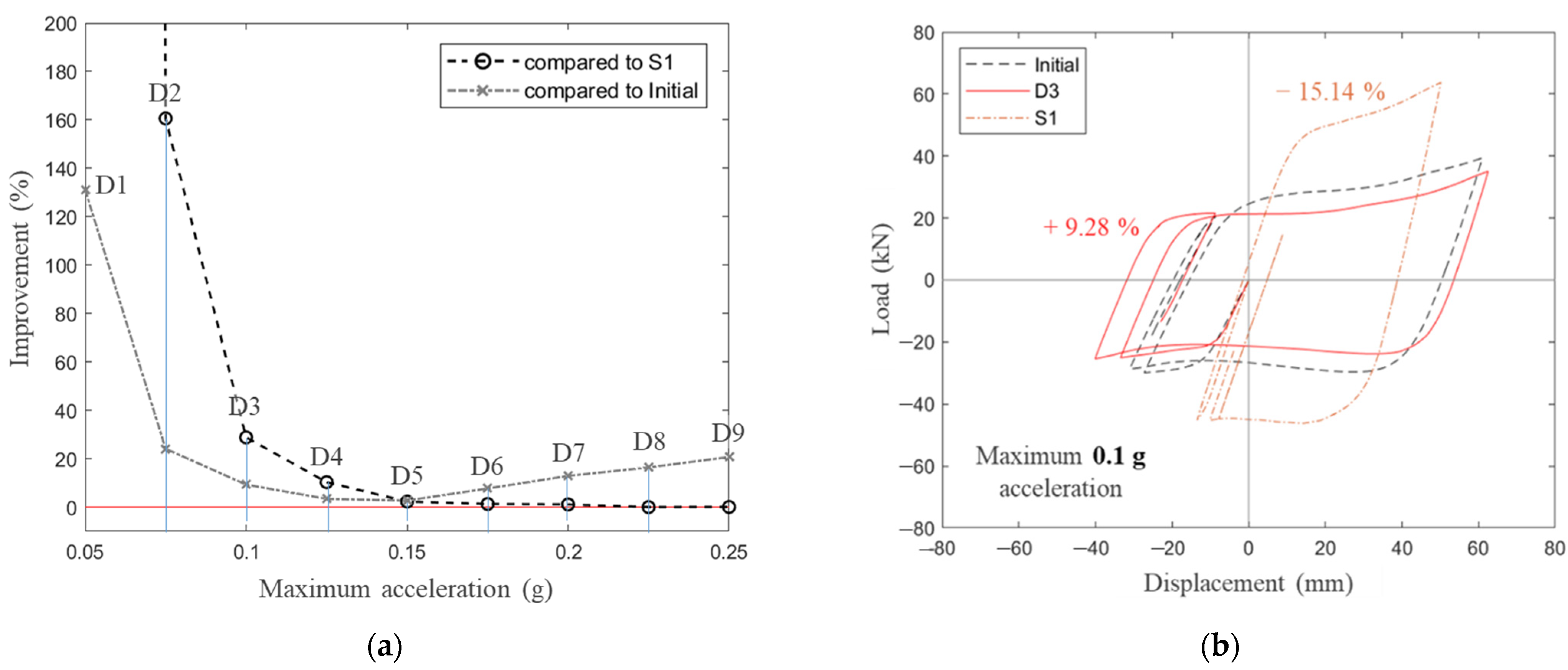
| ALLPD [kN·m] | 4.82 | 4.61 | 18.91 | 21.27 |
| Rate of change | 4.42% | +12.5% | ||
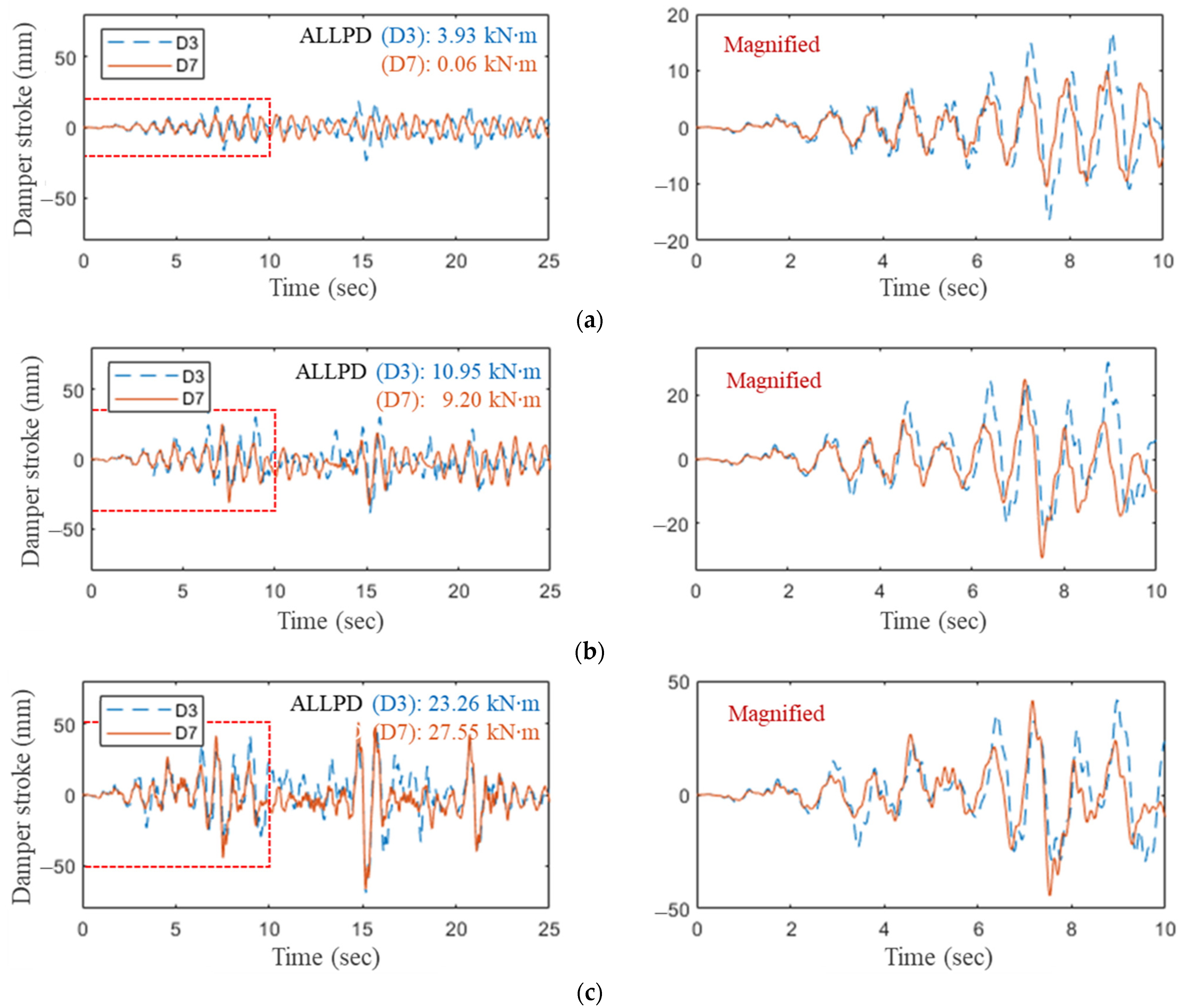
| Case | D1 | D2 | D3 | D4 | D5 | D6 | D7 | D8 | D9 |
|---|---|---|---|---|---|---|---|---|---|
| Max. acc. | 0.05 g | 0.075 g | 0.1 g | 0.125 g | 0.15 g | 0.175 g | 0.2 g | 0.225 g | 0.25 g |
| [mm] | 40.46 | 55.86 | 65.32 | 73.05 | 152.15 | 149.51 | 137.04 | 135.42 | 123.19 |
| [mm] | 109.84 | 104.56 | 101.31 | 98.66 | 71.44 | 72.17 | 76.62 | 77.20 | 81.36 |
| [mm] | 40.00 | 40.00 | 40.00 | 40.00 | 40.03 | 40.47 | 40.00 | 40.00 | 40.08 |
| ] | 3.839 | 3.840 | 3.840 | 3.840 | 3.839 | 3.835 | 3.839 | 3.840 | 3.838 |
| Elastic stiffness [MN/m] | 1.277 | 2.105 | 2.567 | 2.890 | 3.264 | 3.305 | 3.554 | 3.580 | 3.703 |
| ALLPD [kN·m] | 0.96 | 2.35 | 4.38 | 6.97 | 10.08 | 13.98 | 18.42 | 23.39 | 29.01 |
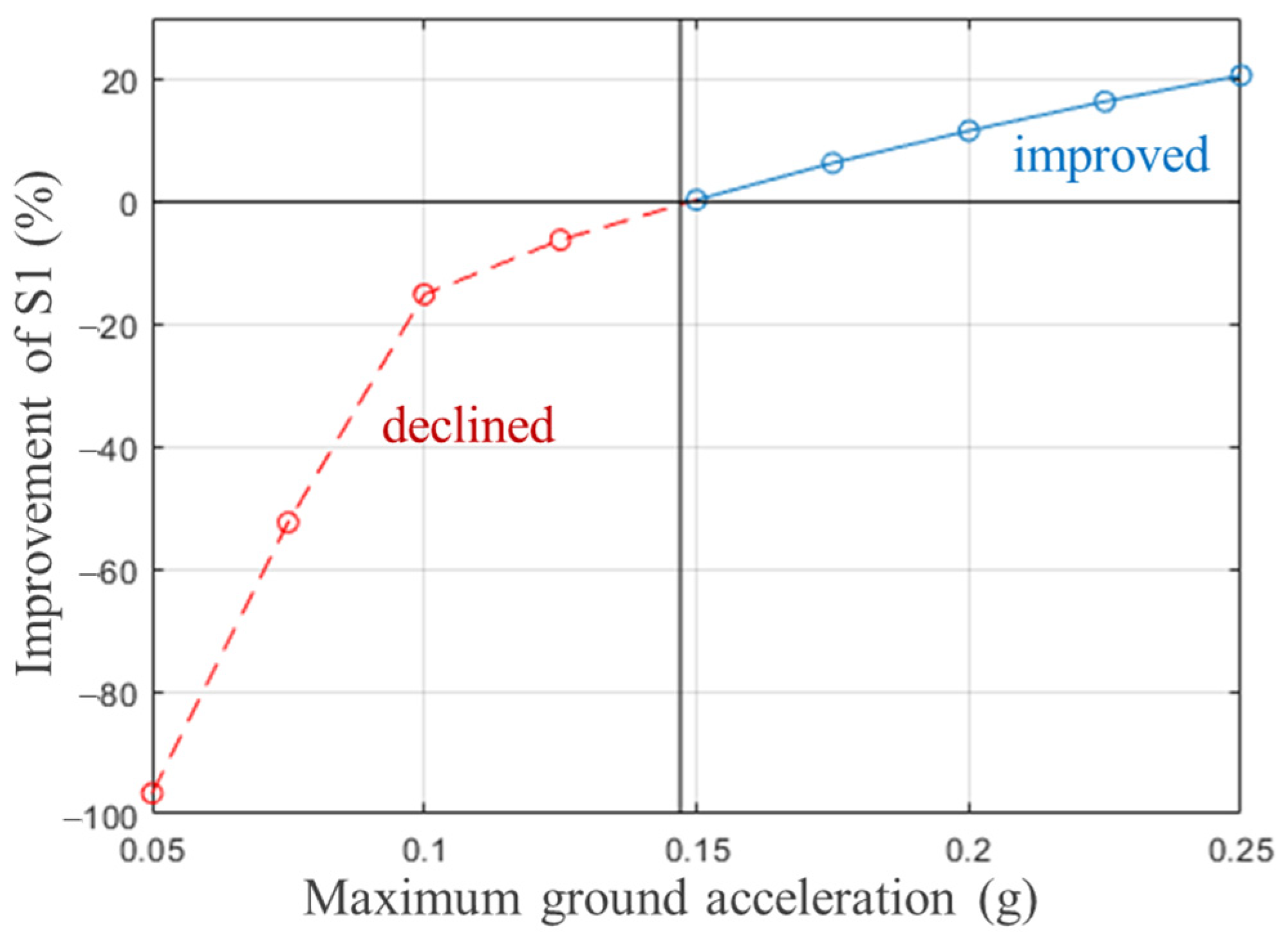
| Improvement | |||||||||
| to Initial | +131.1% | +24.1% | +9.3% | +3.4% | +2.7% | +7.7% | +12.9% | +16.4% | +20.7% |
| to S1 | +6762.0% | +160.6% | +28.8% | +10.3% | +2.3% | +1.3% | +1.1% | +0.01% | +0.07% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Park, J.W.; Yoon, J.-H.; Yoon, G.-H.; Lim, Y.M. Effect of Dynamic Loading Conditions on Maximizing Energy Dissipation of Metallic Dampers. Appl. Sci. 2022, 12, 3086. https://doi.org/10.3390/app12063086
Park JW, Yoon J-H, Yoon G-H, Lim YM. Effect of Dynamic Loading Conditions on Maximizing Energy Dissipation of Metallic Dampers. Applied Sciences. 2022; 12(6):3086. https://doi.org/10.3390/app12063086
Chicago/Turabian StylePark, Ji Woon, Ji-Hoon Yoon, Gil-Ho Yoon, and Yun Mook Lim. 2022. "Effect of Dynamic Loading Conditions on Maximizing Energy Dissipation of Metallic Dampers" Applied Sciences 12, no. 6: 3086. https://doi.org/10.3390/app12063086
APA StylePark, J. W., Yoon, J.-H., Yoon, G.-H., & Lim, Y. M. (2022). Effect of Dynamic Loading Conditions on Maximizing Energy Dissipation of Metallic Dampers. Applied Sciences, 12(6), 3086. https://doi.org/10.3390/app12063086






