Discretization Approach for the Homogenization of Three-Dimensional Solid-Solid Phononic Crystals in the Quasi-Static Limit: Density and Elastic Moduli
Abstract
:1. Introduction
2. General Approach to Homogenization
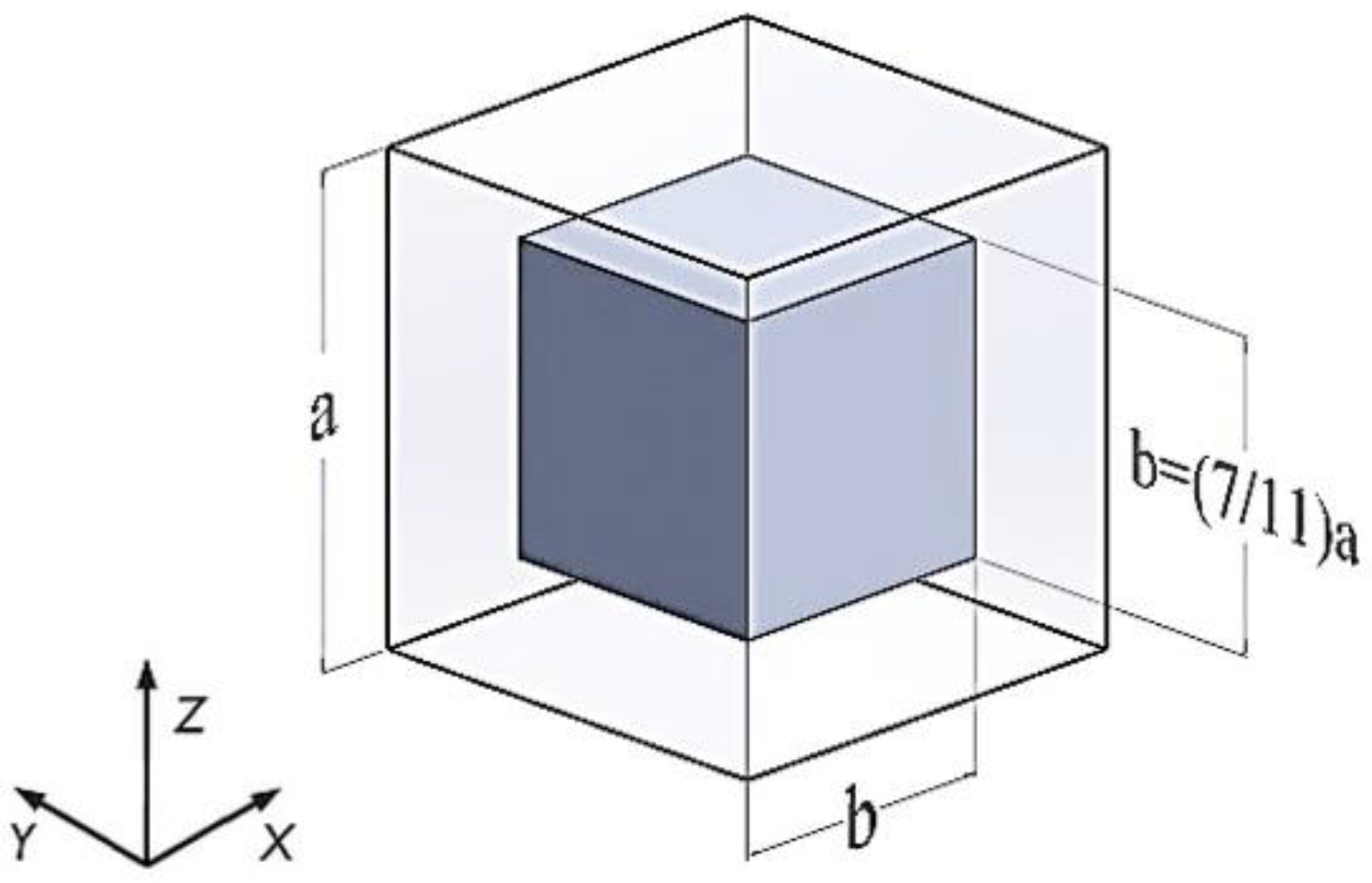
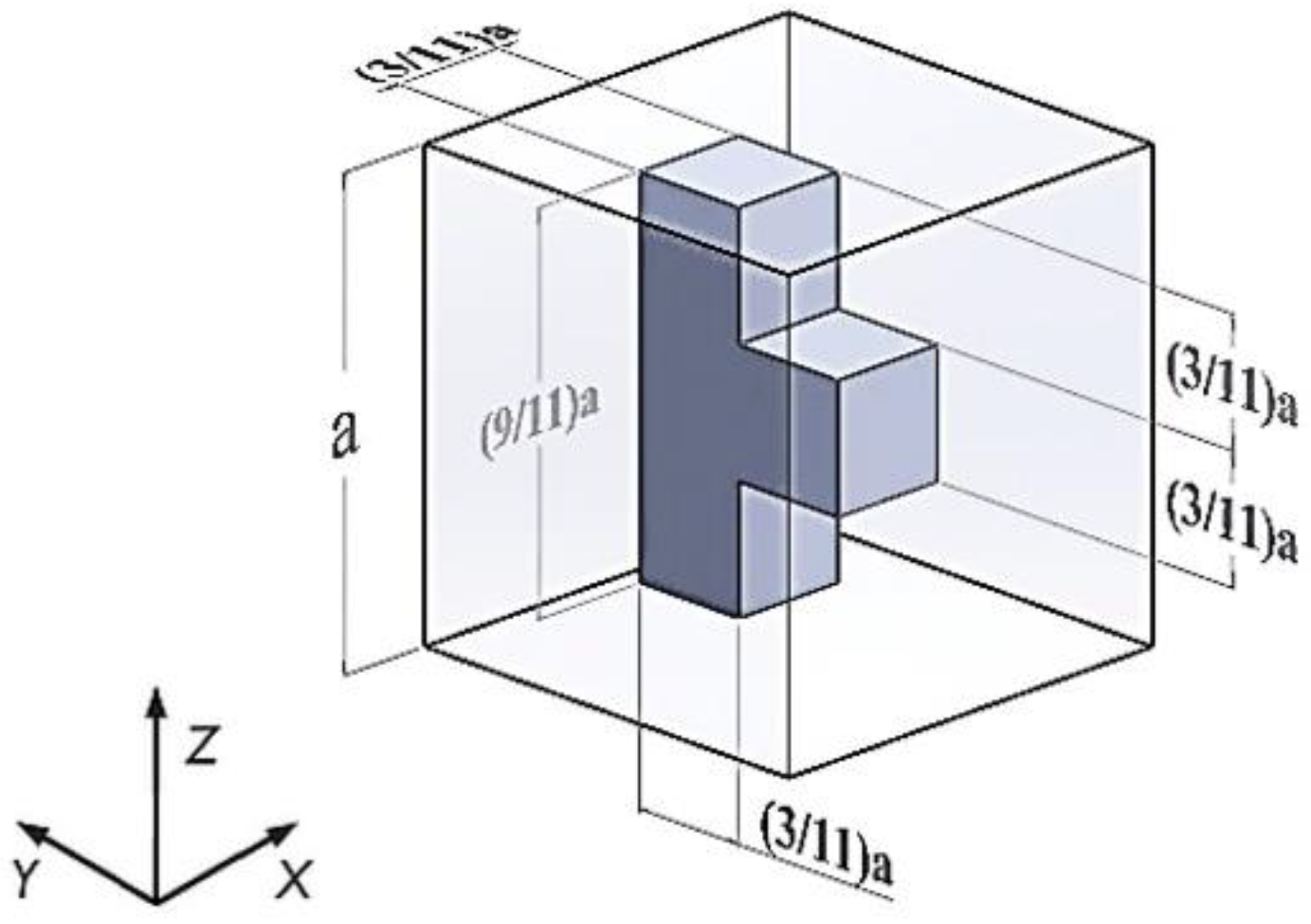
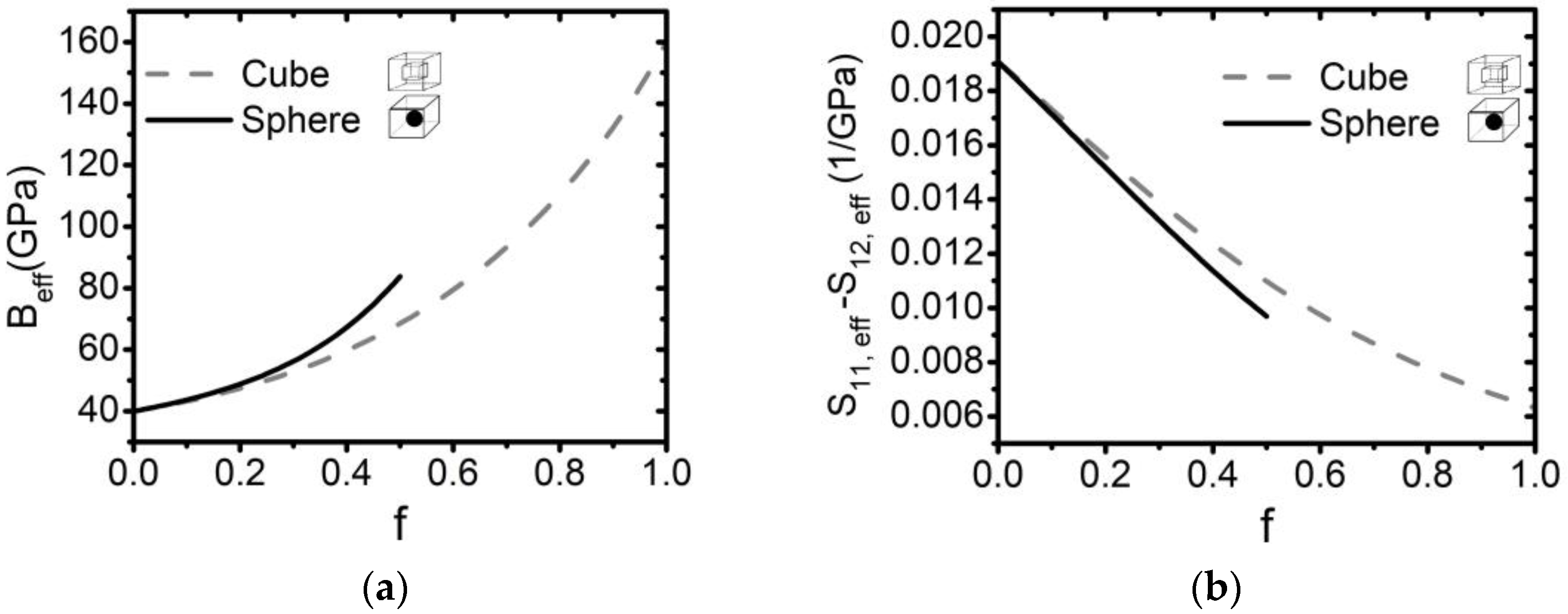
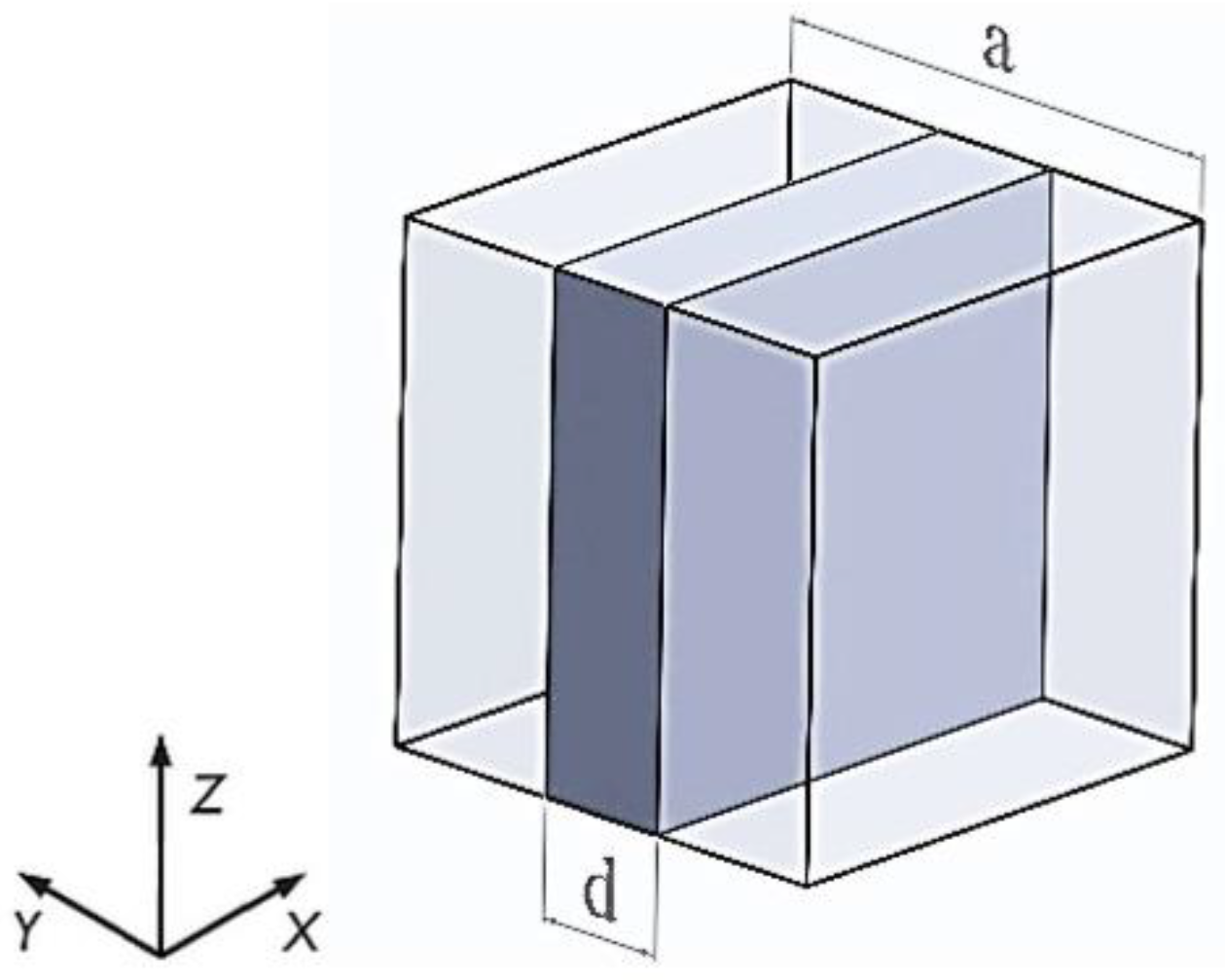
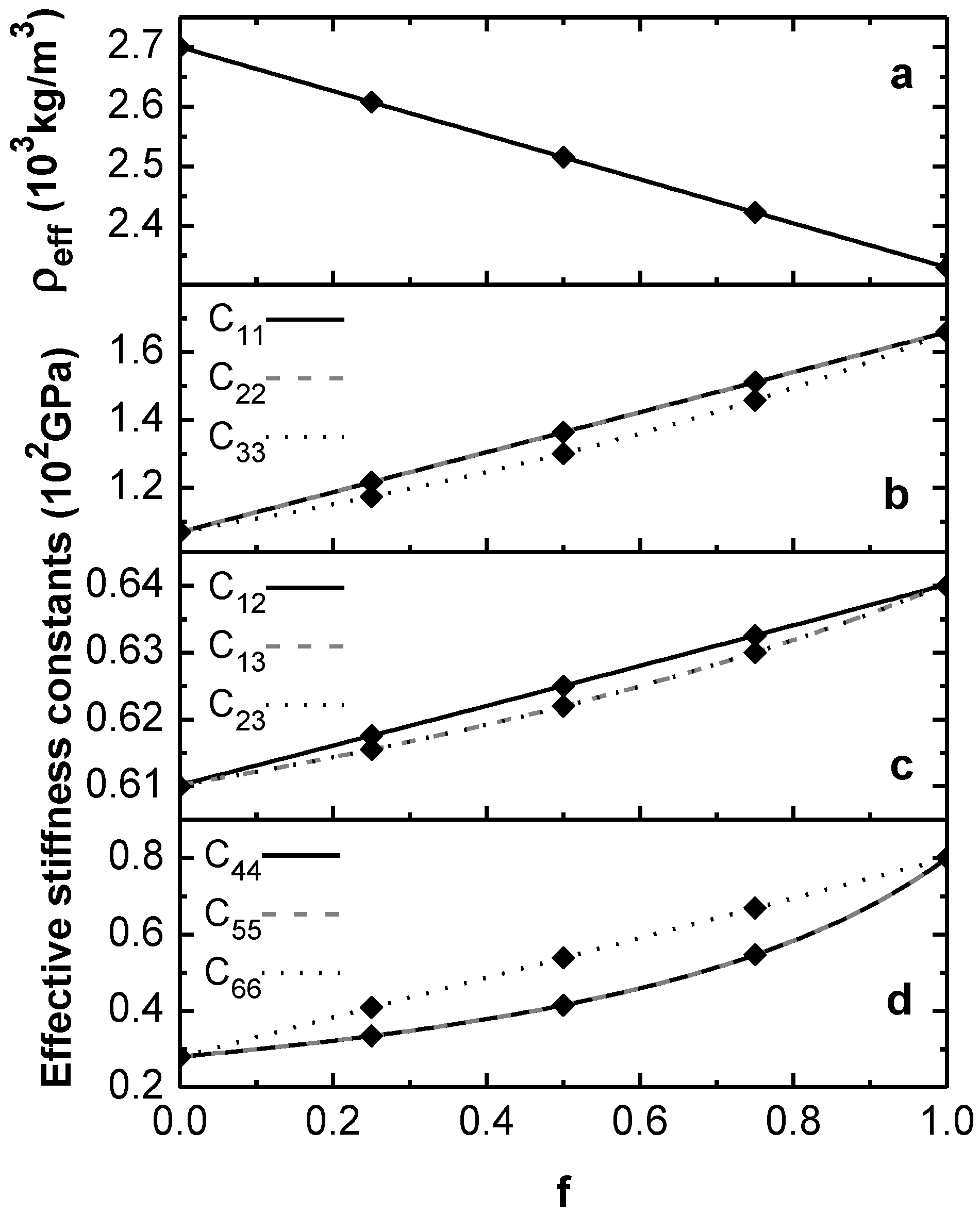
3. Numerical Results and Discussion
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Hashin, Z.; Shtrikman, S. A Variational Approach to the Theory of the Effective Magnetic Permeability of Multiphase Materials. J. Appl. Phys. 1962, 33, 3125. [Google Scholar] [CrossRef]
- Milton, G.W. Bounds on the Electromagnetic, Elastic, and Other Properties of Two-Component Composites. Phys. Rev. Lett. 1981, 46, 542. [Google Scholar] [CrossRef]
- Berryman, J.G. Long-wavelength propagation in composite elastic media I. Spherical inclusions. J. Acoust. Soc. Am. 1980, 68, 1809. [Google Scholar] [CrossRef]
- Sheng, P.; Callegri, A.J. Differential effective medium theory of sedimentary rocks. Appl. Phys. Lett. 1984, 44, 738. [Google Scholar] [CrossRef]
- Kushwaha, M.S.; Halevi, P.; Dobrzynski, L.; Djafari, R. Acoustic band structure of periodic elastic composites. Phys. Rev. Lett. 1993, 71, 2022. [Google Scholar] [CrossRef]
- Ni, Q.; Cheng, J. Long wavelength propagation of elastic waves in three-dimensional periodic solid-solid media. J. Appl. Phys. 2007, 101, 073515. [Google Scholar] [CrossRef]
- Ni, Q.; Cheng, J.-C. Homogenization of Three-Dimensional Periodic Solid-Solid Elastic Composites. Chin. Phys. Lett. 2007, 24, 747–750. [Google Scholar]
- Zhou, X.-W.; Zou, X.-Y.; Wang, T.-H.; Cheng, J.-C. Effective velocity of 2D phononic crystals with rectangular lattice. Ultrasonics 2010, 50, 577–582. [Google Scholar] [CrossRef]
- Liu, J.; Wu, Y.; Li, F.; Zhang, P.; Liu, Y.; Wu, J. Anisotropy of homogenized phononic crystals with anisotropic material. Europhys. Lett. 2012, 98, 36001. [Google Scholar] [CrossRef]
- Flores Méndez, J.; Salazar Villanueva, M.; Ambrosio Lázaro, R.C.; Calixto Sirene, B.; Mota González, M.L.; Candia García, F. Plane Wave-Perturbative Method for Evaluating the Effective Speed of Sound in 1D Phononic Crystals. Adv. Mater. Sci. Eng. 2016, 2016, 3017835. [Google Scholar] [CrossRef] [Green Version]
- Krokhin, A.A.; Arriaga, J.; Gumen, L.N. Speed of Sound in Periodic Elastic Composites. Phys. Rev. Lett. 2003, 91, 264302. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kafesaki, M.; Penciu, R.S.; Economou, E.N. Air Bubbles in Water: A Strongly Multiple Scattering Medium for Acoustic Waves. Phys. Rev. Lett. 2000, 84, 6050. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Cervera, F.; Sanchis, L.; Sánchez-Pérez, J.V.; Martínez-Sala, R.; Rubio, C.; Meseguer, F.; López, C.; Caballero, D.; Sánchez-Dehesa, J. Refractive Acoustic Devices for Airborne Sound. Phys. Rev. Lett. 2002, 88, 023902. [Google Scholar] [CrossRef] [PubMed]
- Mei, J.; Liu, Z.; Wen, W.; Sheng, P. Effective Mass Density of Fluid-Solid Composites. Phys. Rev. Lett. 2006, 96, 024301. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Torrent, D.; Håkansson, A.; Cervera, F.; Sánchez-Dehesa, J. Homogenization of Two-Dimensional Clusters of Rigid Rods in Air. Phys. Rev. Lett. 2006, 96, 204302. [Google Scholar] [CrossRef]
- Torrent, D.; Sánchez-Dehesa, J. Anisotropic mass density by two-dimensional acoustic metamaterials. New J. Phys. 2008, 10, 023004. [Google Scholar] [CrossRef]
- Laude, V.; Iglesias Martínez, J.A.; Wang, Y.F.; Kadic, M. Effective anisotropy of periodic acoustic and elastic composites. J. Appl. Phys. 2021, 129, 215106. [Google Scholar] [CrossRef]
- Li, J.; Chan, C.T. Double-negative acoustic metamaterial. Phys. Rev. E 2004, 70, 055602(R). [Google Scholar] [CrossRef] [Green Version]
- Dong, H.W.; Zhao, S.D.; Wei, P.; Cheng, L.; Wang, Y.S.; Zhang, C. Systematic design and realization of double-negative acoustic metamaterials by topology optimization. Acta Mater. 2019, 172, 102–120. [Google Scholar] [CrossRef] [Green Version]
- Wang, T.; Wang, L.M.; Ma, Z.D.; Hulbert, G.M. Elastic analysis of auxetic cellular structure consisting of re-entrant hexagonal cells using a strain-based expansion homogenization method. Mater. Des. 2018, 160, 284–293. [Google Scholar] [CrossRef]
- Yuan, S.Q.; Shen, F.; Bai, J.M.; Chu, C.K.; Wei, J.; Zhou, K. 3D soft auxetic lattice structures fabricated by selective laser sintering: TPU powder evaluation and process optimization. Mater. Des. 2017, 120, 317–327. [Google Scholar] [CrossRef]
- Hou, S.Y.; Li, T.T.; Jia, Z.; Wang, L.F. Mechanical properties of sandwich composites with 3d-printed auxetic and non-auxetic lattice cores under low velocity impact. Mater. Des. 2018, 160, 1305–1321. [Google Scholar] [CrossRef]
- Eringen, A.C. Microcontinuum Field Theories I: Foundations and Solids, 1st ed.; Springer: New York, NY, USA, 1999. [Google Scholar]
- Duan, S.Y.; Wen, W.B.; Fang, D.N. A predictive micropolar continuum model for a novel three-dimensional chiral lattice with size effect and tension-twist coupling behavior. J. Mech. Phys. Solids 2018, 121, 23–46. [Google Scholar] [CrossRef]
- Kaczmarczyk, L.; Pearce, C.J.; Bicanic, N. Studies of Microstructural Size Effect and Higher-order Deformation in Second-order Computational Homogenization. Comput. Struct. 2010, 88, 1383–1390. [Google Scholar] [CrossRef]
- Reis, F.D.; Ganghoffer, J.F. Construction of micropolar continua from the asymptotic homogenization of beam lattices. Comput. Struct. 2012, 112–113, 354–363. [Google Scholar] [CrossRef]
- Dirrenberger, J.; Forest, S.; Jeulin, D.; Colin, C. Homogenization of periodic auxetic materials. Procedia Eng. 2011, 10, 1847–1852. [Google Scholar] [CrossRef]
- Ha, C.S.; Hestekin, E.; Li, J.H.; Plesha, M.E.; Lakes, R.S. Controllable thermal expansion of large magnitude in chiral negative Poisson’s ratio lattices. Phys. Status Solidi B 2015, 252, 1431–1434. [Google Scholar] [CrossRef]
- Tian, Y.; Zhang, W.; Tan, Z.; Cho, C. Chiral edge states for phononic crystals based on shunted piezoelectric materials. Extrem. Mech. Lett. 2022, 50, 101568. [Google Scholar] [CrossRef]
- Chen, Y.; Frenzel, T.; Guenneau, S.; Kadic, M.; Wegener, M. Mapping acoustical activity in 3D chiral mechanical metamaterials onto micropolar continuum elasticity. J. Mech. Phys. Solids 2020, 137, 103877. [Google Scholar] [CrossRef]
- Airoldi, A.; Bettini, P.; Zazzarini, M.; Scarpa, F. Failure and energy absorption of plastic and composite chiral honeycombs. WIT Trans. Built Environ. 2012, 126, 101–114. [Google Scholar]
- Mohammad, M.; Amanul, S.; Behnam, A.; Barthelat, F. Toughness by segmentation: Fabrication, testing and micromechanics of architectured ceramic panels for impact applications. Int. J. Solids Struct. 2019, 158, 52–65. [Google Scholar]
- Ma, Y.H.; Scarpa, F.; Zhang, D.Y.; Zhu, B.; Chen, L.L.; Hong, J. A nonlinear auxetic structural vibration damper with metal rubber particles. Smart Mater. Struct. 2013, 22, 084012. [Google Scholar] [CrossRef]
- Baravelli, E.; Ruzzene, M. Internally resonating lattices for bandgap generation and low-frequency vibration control. J. Sound Vib. 2013, 332, 6562–6579. [Google Scholar] [CrossRef]
- Huang, K.X.; Shui, G.S.; Wang, Y.Z.; Wang, Y.S. Elastic wave scattering by a pair of parallel semi-infinite cracks in mechanical metamaterials with multi resonators. Int. J. Fract. 2021, 232, 199–212. [Google Scholar] [CrossRef]
- Huang, K.X.; Shui, G.S.; Wang, Y.Z.; Wang, Y.S. Enhanced Fracture Resistance Induced by Coupling Multiple Degrees of Freedom in Elastic Wave Metamaterials with Local Resonators. J. Elast. 2021, 144, 33–53. [Google Scholar] [CrossRef]
- Huang, K.X.; Shui, G.S.; Wang, Y.Z.; Wang, Y.S. Discrete scattering and meta-arrest of locally resonant elastic wave metamaterials with a semi-infinite crack. Proc. R. Soc. A 2021, 477, 20210356. [Google Scholar] [CrossRef]
- Flores-Méndez, J.; Pérez-Rodríguez, F. Metasolid with anisotropic mass density. Europhys. Lett. 2013, 103, 54001. [Google Scholar] [CrossRef]
- Rayne, J.A.; Chandrasekhar, B.S. Elastic Constants of Iron from 4.2° to 300° K. Phys. Rev. 1961, 122, 1714. [Google Scholar] [CrossRef]
- Chandrasekhar, B.S.; Rayne, J.A. Elastic Constants of Indium from 1.4° to 300°K. Phys. Rev. 1961, 124, 1011. [Google Scholar] [CrossRef]
- Crandall, S.H.; Dahl, N.C.; Lardner, T.J.; Sivakumar, M.S. An Introduction to the Mechanics of Solids (In SI Units), 3rd ed.; Tata McGraw Hill Education Private Limited: Nueva Delhi, India, 2012; p. 259. [Google Scholar]
- Kittel, C. Introduction to Solid State Physics, 8th ed.; Wiley: New York, NY, USA, 2004; pp. 21–84. [Google Scholar]
- Castillero, J.B.; Otero, J.A.; Ramos, R.R.; Bourgeat, A. Asymptotic homogenization of laminated piezocomposite materials. Int. J. Solids Struct. 1998, 35, 527–541. [Google Scholar] [CrossRef]
- Bravo-Castillero, J.; Rodríguez-Ramos, R.; Mechkour, H.; Otero, J.A.; Cabanas, J.H.; Sixto, L.; Sabina, F.J. Homogenization and effective properties of periodic thermomagnetoelectroelastic composites. J. Mech. Mater. Struct. 2009, 4, 819–836. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Flores Méndez, J.; Pinón Reyes, A.C.; Heredia Jiménez, A.H.; Ambrosio Lázaro, R.C.; Morales-Sánchez, A.; Moreno Moreno, M.; Luna-López, J.A.; Severiano Carrillo, F.; Meraz Melo, M.A. Discretization Approach for the Homogenization of Three-Dimensional Solid-Solid Phononic Crystals in the Quasi-Static Limit: Density and Elastic Moduli. Appl. Sci. 2022, 12, 2987. https://doi.org/10.3390/app12062987
Flores Méndez J, Pinón Reyes AC, Heredia Jiménez AH, Ambrosio Lázaro RC, Morales-Sánchez A, Moreno Moreno M, Luna-López JA, Severiano Carrillo F, Meraz Melo MA. Discretization Approach for the Homogenization of Three-Dimensional Solid-Solid Phononic Crystals in the Quasi-Static Limit: Density and Elastic Moduli. Applied Sciences. 2022; 12(6):2987. https://doi.org/10.3390/app12062987
Chicago/Turabian StyleFlores Méndez, J., A. C. Pinón Reyes, Aurelio H. Heredia Jiménez, Roberto C. Ambrosio Lázaro, A. Morales-Sánchez, M. Moreno Moreno, J. A. Luna-López, F. Severiano Carrillo, and M. A. Meraz Melo. 2022. "Discretization Approach for the Homogenization of Three-Dimensional Solid-Solid Phononic Crystals in the Quasi-Static Limit: Density and Elastic Moduli" Applied Sciences 12, no. 6: 2987. https://doi.org/10.3390/app12062987
APA StyleFlores Méndez, J., Pinón Reyes, A. C., Heredia Jiménez, A. H., Ambrosio Lázaro, R. C., Morales-Sánchez, A., Moreno Moreno, M., Luna-López, J. A., Severiano Carrillo, F., & Meraz Melo, M. A. (2022). Discretization Approach for the Homogenization of Three-Dimensional Solid-Solid Phononic Crystals in the Quasi-Static Limit: Density and Elastic Moduli. Applied Sciences, 12(6), 2987. https://doi.org/10.3390/app12062987








