A Unified Nonlinear Elastic Model for Rock Material
Abstract
1. Introduction
2. Common Nonlinear Elastic Models
2.1. Nonlinear Elastic Models of Joints
2.2. Nonlinear Elastic Models of Joints
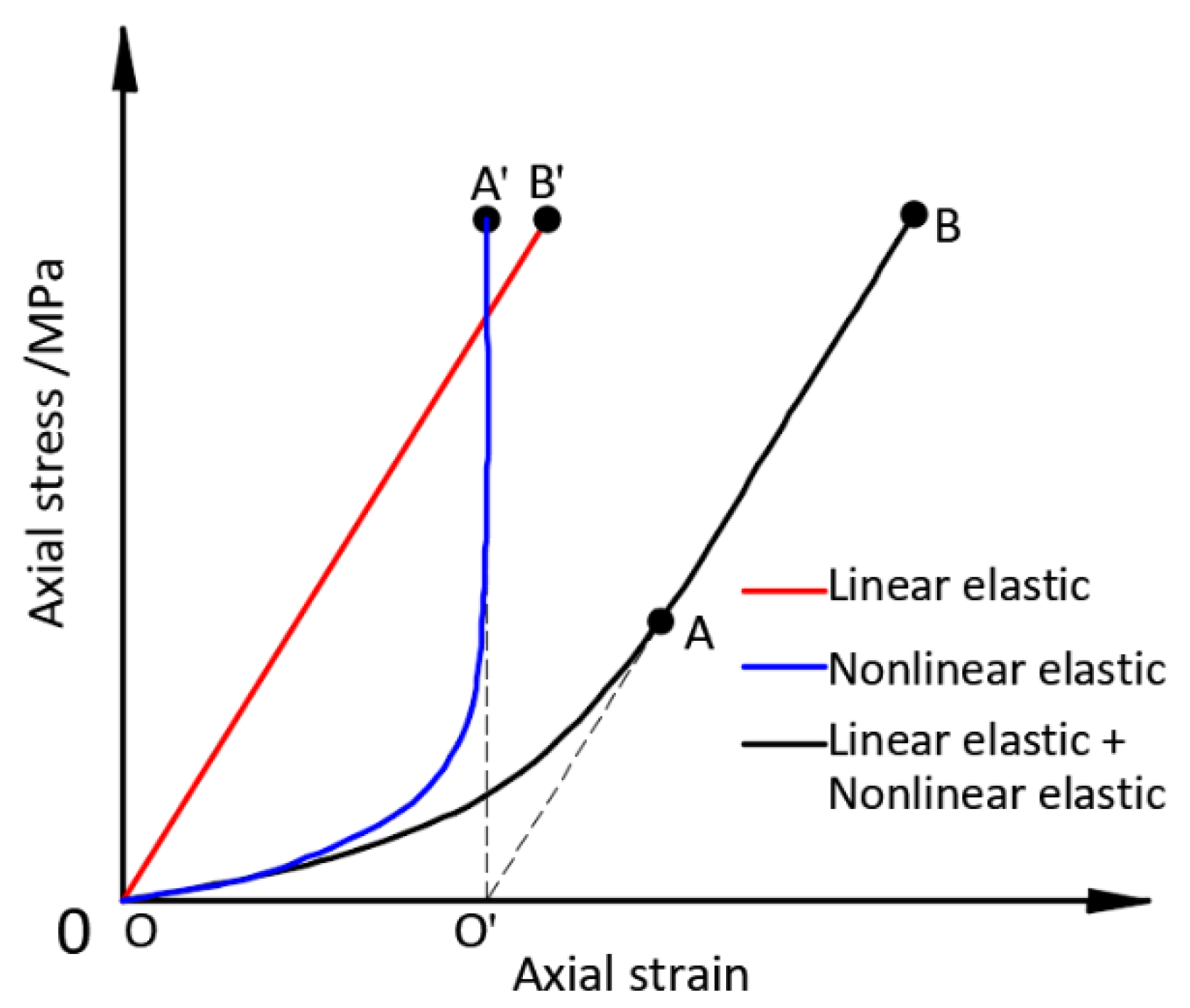
3. A New Unified Nonlinear Elastic Model
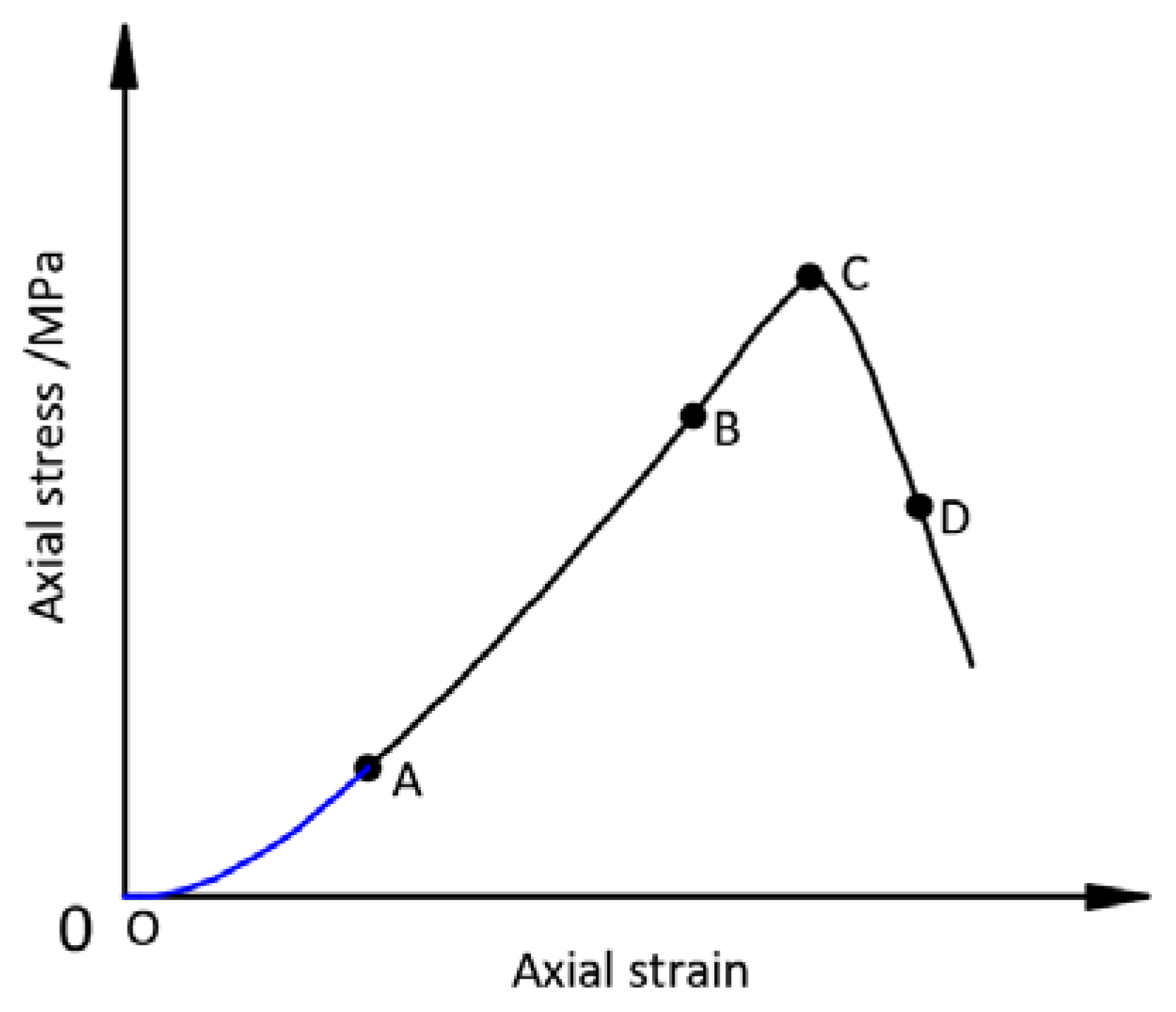
4. The Characteristics of the New Model
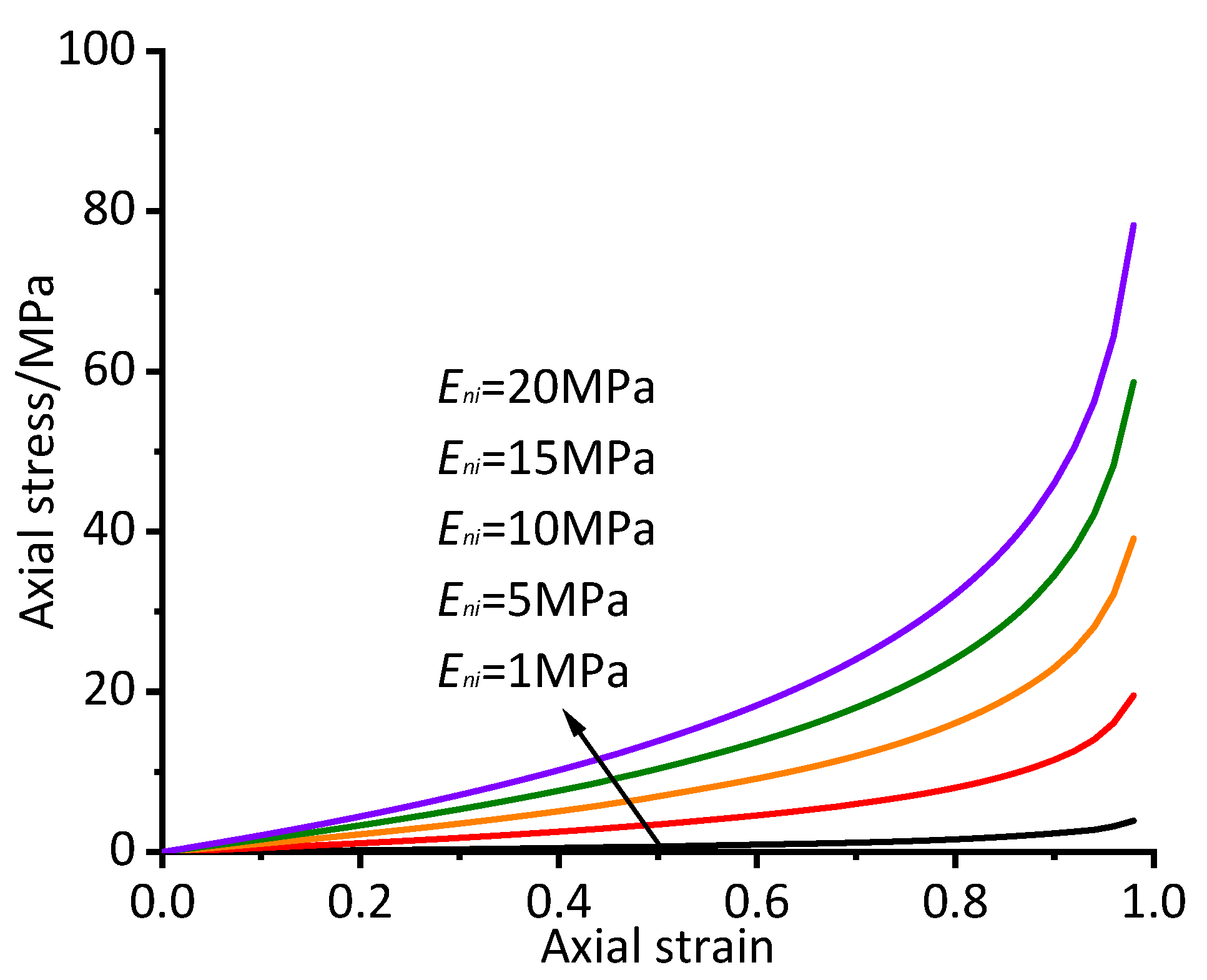
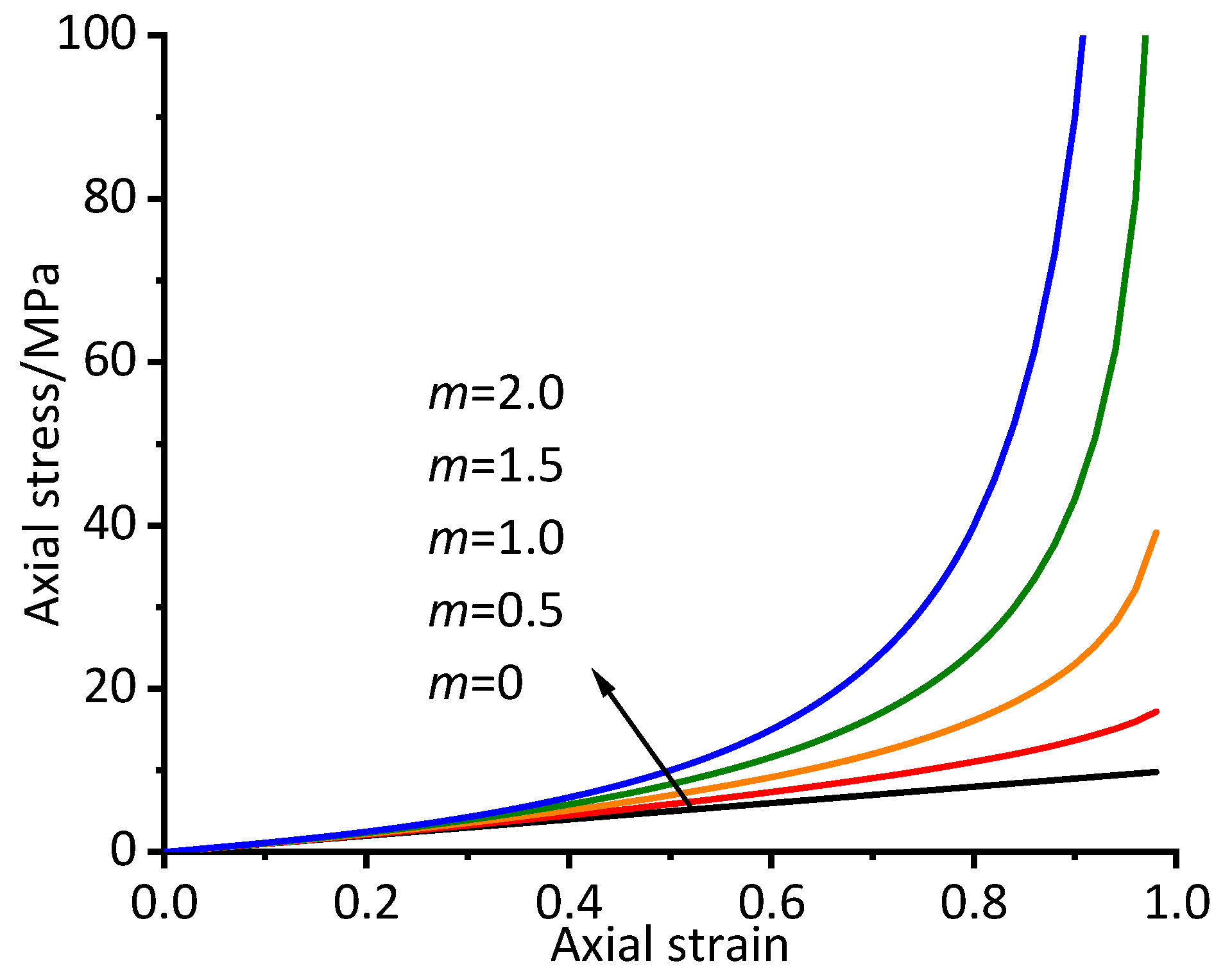
4.1. Sensitivity Analysis of the Parameters
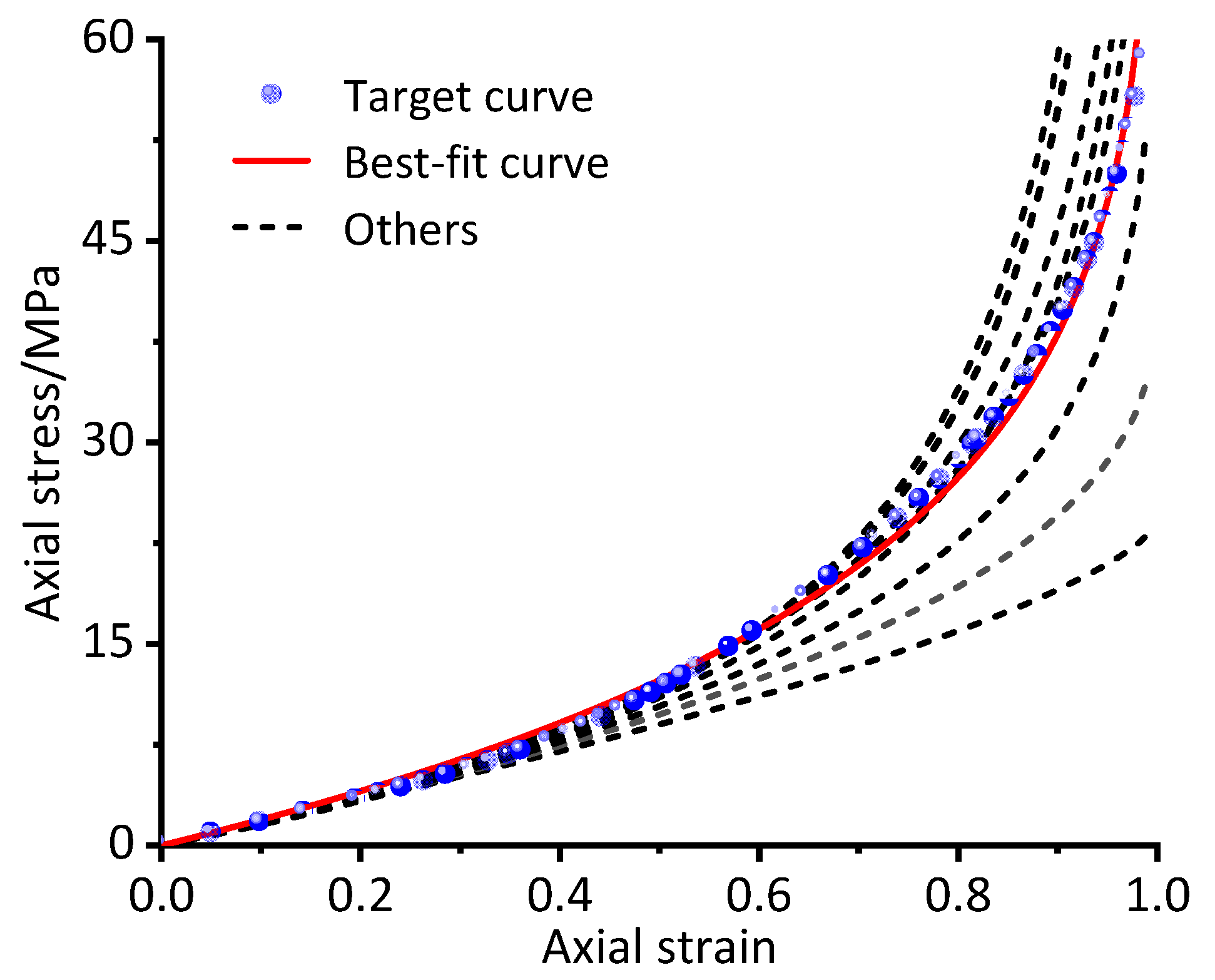
4.2. Approximating to a Single Stress‒Strain Curve
5. Experimental Validation of the New Model
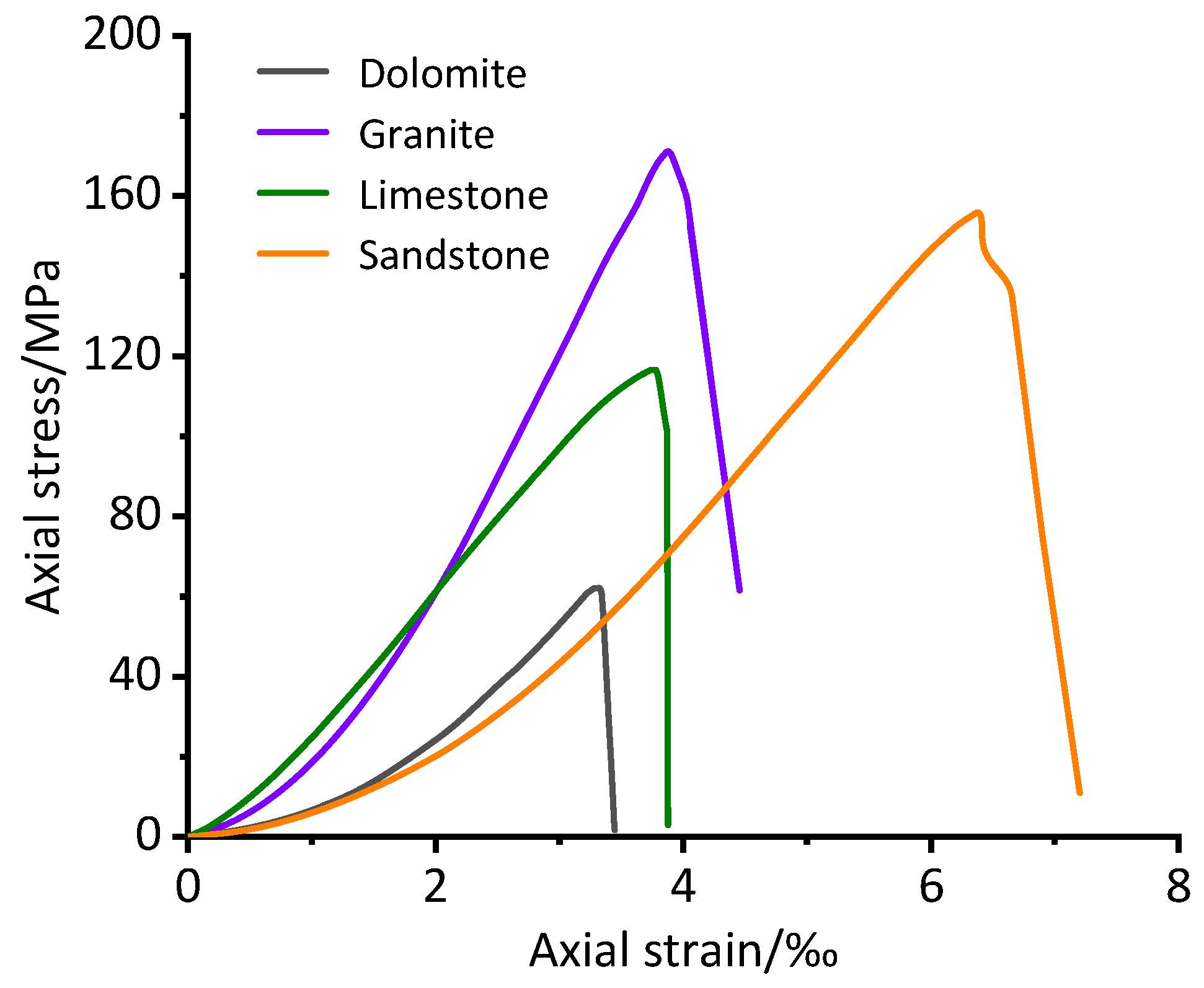
5.1. Experimental Methods
5.2. Experimental and Fitting Results
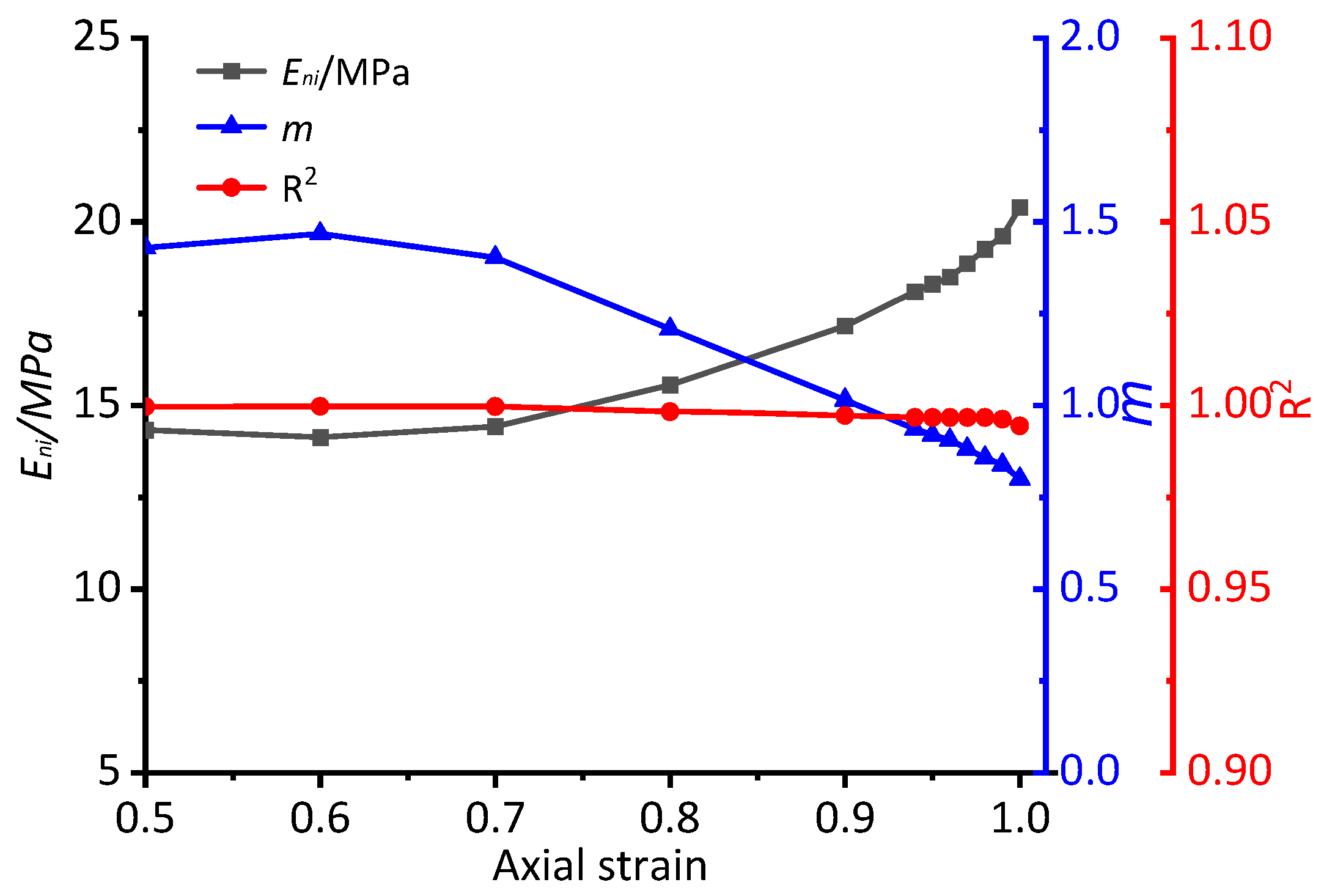
5.3. The m Value Range of the Rock Material
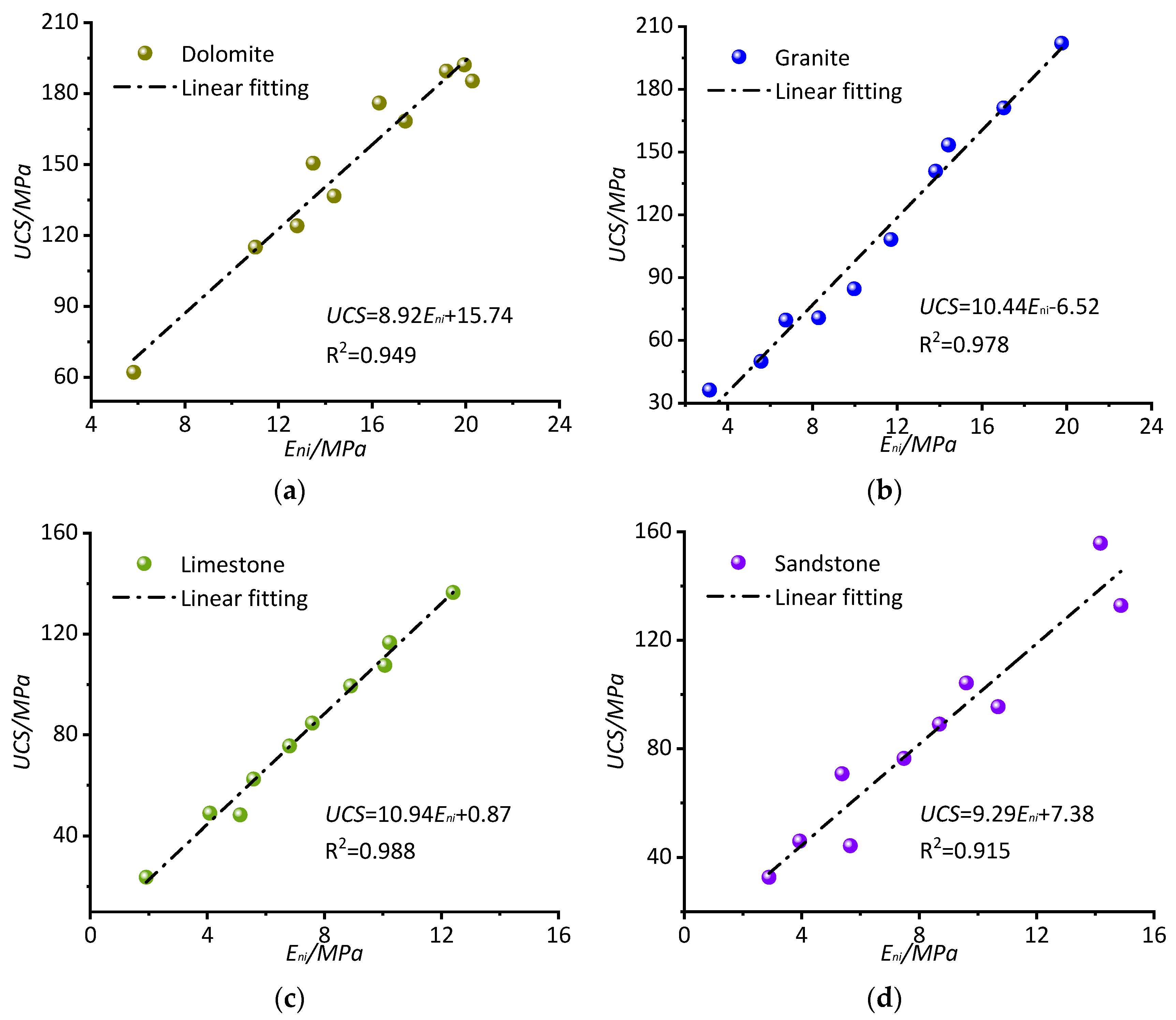
5.4. The Range of Eni Values for the Rock Material
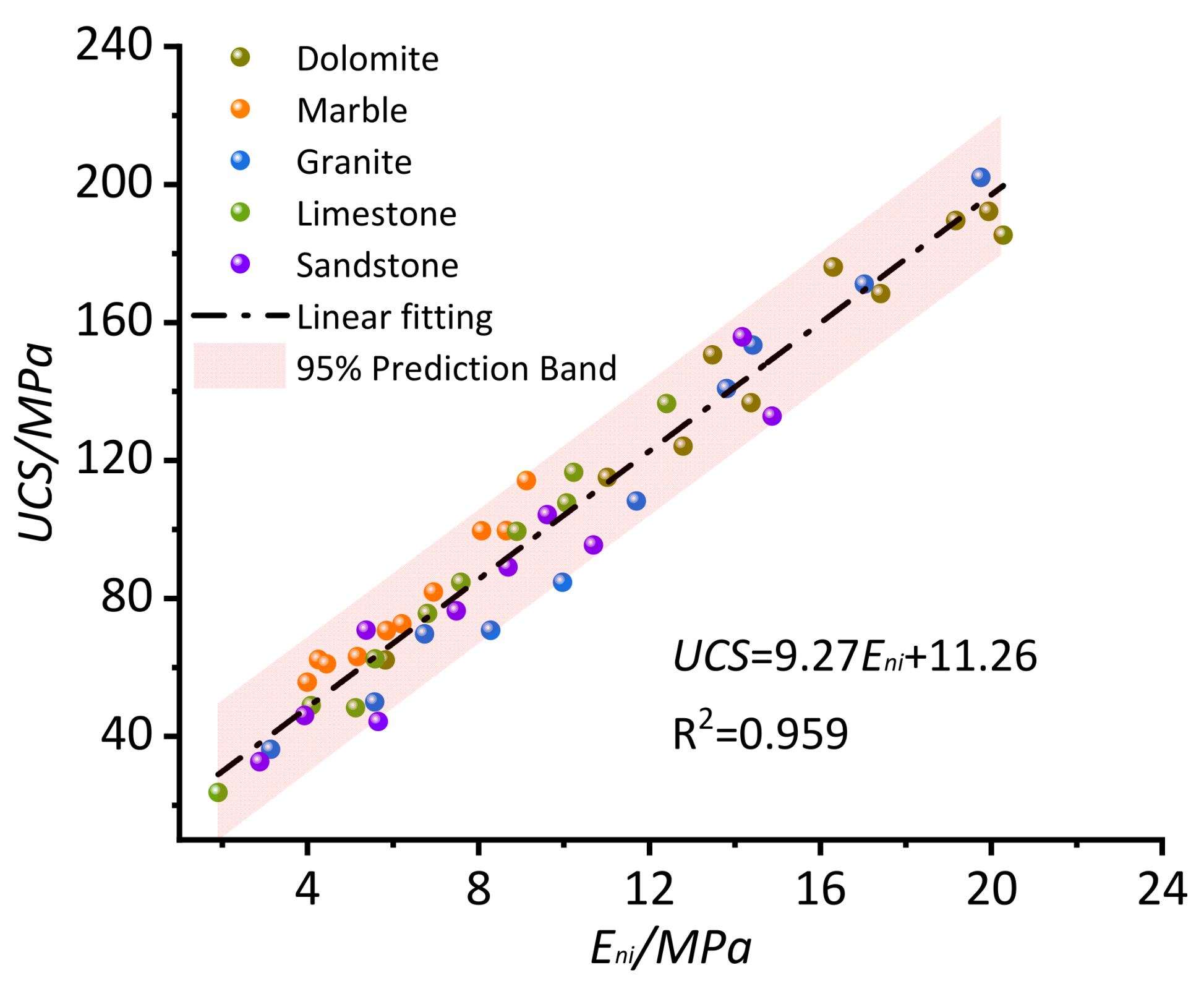
6. Application of the New Model
7. Summary and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Chen, F.; He, C.; Deng, J. Concept of high geostress and its qualitative and quantitative Definitions. Rock Soil Mech. 2015, 36, 971–980. [Google Scholar] [CrossRef]
- Shehata, W.M. Geohydrology of Mount Vemon Canyon Area. Ph.D. Thesis, Colorado School of Mines, Golden, CO, USA, 1971. [Google Scholar]
- Goodman, R.E. Methods of Geological Engineering in Discontinuous Rocks; West Group: New York, NY, USA, 1976; pp. 472–490. [Google Scholar]
- Bandis, S.C.; Lumsden, A.C.; Barton, N.R. Fundamentals of rock joint deformation. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1983, 20, 249–268. [Google Scholar] [CrossRef]
- Barton, N.R.; Bandis, S.C.; Bakhtar, K. Strength deformation and conductivity coupling of rock joints. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1985, 22, 121–140. [Google Scholar] [CrossRef]
- Malama, B.; Kulatilake, P.H.S.W. Models for normal fracture deformation under compressive loading. Int. J. Rock Mech. Ming Sci. 2003, 40, 893–901. [Google Scholar] [CrossRef]
- Swan, G. Determination of stiffness and other joint properties from roughness measurement. Rock Mech. Rock Eng. 1983, 16, 19–38. [Google Scholar] [CrossRef]
- Sun, Z.; Gerrard, C.; Stephansson, O. Rock joint compliance tests for compression and shear loads. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1985, 22, 197–213. [Google Scholar] [CrossRef]
- Xia, C.; Yue, Z.; Tham, L.G.; Lee, C.; Sun, Z. Quantifying topography and closure deformation of rock joints. Int. J. Rock Mech. Min. Sci. 2003, 40, 197–220. [Google Scholar] [CrossRef]
- Yu, J.; Zhao, X.; Zhao, W.; Li, X.; Guan, Y. Improved nonlinear elastic constitutive model for normal deformation of rock fractures. Chin. J. Geotech. Eng. 2008, 30, 1316–1321. [Google Scholar] [CrossRef]
- Rong, G.; Huang, K.; Zhou, C.; Wang, X.; Peng, J. A new constitutive law for the nonlinear normal deformation of rock joints under normal load. Sci. China Technol. Sci. 2012, 42, 402–414. [Google Scholar] [CrossRef]
- Zhao, Y.; Zhang, L.; Wang, W.; Liu, Q.; Tang, L.; Cheng, G. Experimental Study on Shear Behavior and a Revised Shear Strength Model for Infilled Rock Joints. Int. J. Geomech. 2020, 20, 04020141. [Google Scholar] [CrossRef]
- Xie, S.; Lin, H.; Chen, Y. New constitutive model based on disturbed state concept for shear deformation of rock joints. Arch. Civ. Mech. Eng. 2022, 23, 26. [Google Scholar] [CrossRef]
- Martin, C.D. The Strength of Massive Lac du Bonnet Granite Around Underground Openings. Ph.D. Thesis, University of Manitoba, Winnipeg, MB, Canada, 1993. [Google Scholar]
- Duncan, J.M.; Chang, C.Y. Non-linear analysis of stress and strain in soils. J. Soil Mech. Found. Div. 1970, 96, 1629–1653. [Google Scholar] [CrossRef]
- Puzrin, A.M.; Burland, J.B. A logarithmic stress–strain function for rocks and soils. Geotechnique 1996, 46, 157–164. [Google Scholar] [CrossRef]
- Shibuya, S. A non-linear stress–stiffness model for geomaterials at small to intermediate strains. Geotech. Geol. Eng. 2002, 20, 333–369. [Google Scholar] [CrossRef]
- Palchik, V. Simple stress–strain model of very strong limestones and dolomites for engineering practice. Geomech. Geophys. Geo-Energy Geo-Resour. 2019, 5, 345–356. [Google Scholar] [CrossRef]
- Davarpanah, M.; Somodi, G.; Kovács, L.; Vásárhelyi, B. Experimental determination of the mechanical properties and deformation constants of mórágy granitic rock formation (hungary). Geotech. Geol. Eng. 2020, 38, 3215–3229. [Google Scholar] [CrossRef]
- Cai MK, P.K.; Kaiser, P.K.; Tasaka, Y.; Maejima, T.; Morioka, H.; Minami, M. Generalized crack initiation and crack damage stress thresholds of brittle rock masses near underground excavations. Int. J. Rock Mech. Min. Sci. 2004, 41, 833–847. [Google Scholar] [CrossRef]
- Corkum, A.G.; Martin, C.D. The mechanical behaviour of weak mudstone (Opalinus Clay) at low stresses. Int. J. Rock Mech. Min. Sci. 2007, 44, 196–209. [Google Scholar] [CrossRef]
- Yang, H.; Lin, H.; Chen, Y.; Wang, Y.; Zhao, Y.; Yong, W.; Gao, F. Influence of wing crack propagation on the failure process and strength of fractured specimens. Bull. Eng. Geol. Environ. 2022, 81, 71. [Google Scholar] [CrossRef]
- Zhang, X.; Lin, H.; Wang, Y.; Zhao, Y. Creep damage model of rock mass under multi-level creep load based on spatio-temporal evolution of deformation modulus. Arch. Civ. Mech. Eng. 2021, 21, 71. [Google Scholar] [CrossRef]
- Zhu, Z.; Tian, H.; Wang, R.; Jiang, G.; Dou, B.; Mei, G. Statistical thermal damage constitutive model of rocks based on Weibull distribution. Arab. J. Geosci. 2021, 14, 495. [Google Scholar] [CrossRef]
- Jiang, Y.; Xian, X.; Su, J. Research on distortion of singlerock and constitutive relation. Rock Soil Mech. 2005, 26, 941–945. [Google Scholar] [CrossRef]
- Zhang, W.; Chen, C.; Yu, M. Study of mechanical properties and constitutive relation of weathered sandstone. Rock Soil Mech. 2009, 30, 33–36. [Google Scholar] [CrossRef]
- Wang, Q.; Zhu, W.; Xu, T.; Liu, H.; Jia, J. Numerical analysis on nonlinear deformation behavior of rock considering compaction of pores. Sci. Sin. Technol. 2018, 48, 565–574. [Google Scholar] [CrossRef][Green Version]
- Ma, Q.; Qin, Y.; Zhou, T.; Yang, X. Constitutive model of rock compaction stage based on contact theory. J. Cent. South Univ. (Sci. Technol.) 2019, 50, 1941–1948. [Google Scholar] [CrossRef]
- Liu, H.; Rutqvist, J.; Berryman, J.C. On the relationship between stress and elastic strain for porous and fractured rock. Int. J. Rock Mech. Min. Sci. 2009, 46, 289–296. [Google Scholar] [CrossRef]
- Zhao, Y.; Liu, H. An elastic strain-stress relationship for porous rock under anisotropic stress conditions. Rock Mech. Rock Eng. 2012, 45, 389–399. [Google Scholar] [CrossRef]
- Peng, J.; Rong, G.; Zhou, C.; Wei, J.; Li, X. A study of crack closure effect of rocks and its quantitative model. Rock Soil Mech. 2016, 37, 126–132. [Google Scholar] [CrossRef]
- Zhang, C.; Cao, W.; Xu, Z.; He, M. Initial macro-deformation simulation and determination method of micro-crack closure stress for rock. Rock Soil Mech. 2018, 39, 1281–1288+1301. [Google Scholar] [CrossRef]
- Liu, S.; Wang, D.; Yin, G.; Wei, J.; Li, X. A double hook-statistical damage constitutive model of coal under temperature impact. J. Min. Saf. Eng. 2019, 36, 1025–1033. [Google Scholar] [CrossRef]
- Cao, W.; Tan, X.; Zhang, C.; He, M. A constitutive model to simulate the full deformation and failure process for rocks considering initial compression and residual strength behaviors. Can. Geotech. J. 2019, 56, 649–661. [Google Scholar] [CrossRef]
- Zhao, X. Microstructure and Time Dependent Fracture of Hard Brittle Rock Based on Distinct Element. Ph.D. Thesis, Wuhan University, Wuhan, China, 2022. [Google Scholar]
- Del Greco, O.; Ferrero, A.M.; Oggeri, C. Experimental and analytical interpretation of the behaviour of laboratory tests on composite specimens. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1993, 30, 1539–1543. [Google Scholar] [CrossRef]
- Zuo, J.; Chen, Y.; Sun, Y.; Jiang, G.; Wang, J. Investigation on whole failure nonlinear model for deep coal-rock combined bodies. J. Min. Sci. Technol. 2017, 2, 17–24. [Google Scholar] [CrossRef]
- Bieniawski, Z.T.; Bernede, M.J. Suggested methods for determining the uniaxial compressive strength and deformability of rock materials: Part 1. Suggested method for determining deformability of rock materials in uniaxial compression. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1979, 16, 138–140. [Google Scholar] [CrossRef]
- Ocak, I. Estimating the modulus of elasticity of the rock material from compressive strength and unit weight. J. -South Afr. Inst. Min. Metall. 2008, 108, 621–626. [Google Scholar] [CrossRef]
- Morland, L.W. Continuum model of regularly jointed mediums. J. Geophys. Res. 1974, 79, 357–362. [Google Scholar] [CrossRef]
- Salamon, M.D.G. Elastic moduli of a stratified rock mass. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1968, 5, 519–527. [Google Scholar] [CrossRef]





| Type of Rock | UCS/MPa | Eni/MPa | m | Coefficients of Determination R2 |
|---|---|---|---|---|
| D01 | 62.15 | 5.82 | 0.9117 | 0.9951 |
| D02 | 115.06 | 11.01 | 0.8911 | 0.9977 |
| D03 | 124.10 | 12.79 | 0.9039 | 0.9792 |
| D04 | 150.59 | 13.48 | 0.9626 | 0.9935 |
| D05 | 136.74 | 14.38 | 0.8759 | 0.9917 |
| D06 | 176.04 | 16.30 | 0.9216 | 0.9907 |
| D07 | 168.37 | 17.41 | 0.9389 | 0.9922 |
| D08 | 189.53 | 19.17 | 0.8630 | 0.9817 |
| D09 | 192.21 | 19.94 | 0.9478 | 0.9976 |
| D10 | 185.34 | 20.28 | 0.8880 | 0.9927 |
| G01 | 36.24 | 3.14 | 0.6508 | 0.9939 |
| G02 | 49.95 | 5.57 | 0.7621 | 0.9840 |
| G03 | 69.71 | 6.74 | 0.8872 | 0.9859 |
| G04 | 70.76 | 8.28 | 0.7335 | 0.9998 |
| G05 | 84.64 | 9.97 | 0.7789 | 0.9934 |
| G06 | 108.23 | 11.70 | 0.8899 | 0.9977 |
| G07 | 140.88 | 13.81 | 0.9517 | 0.9861 |
| G08 | 153.39 | 14.42 | 0.9488 | 0.9758 |
| G09 | 171.11 | 17.03 | 0.9455 | 0.9846 |
| G10 | 202.02 | 19.76 | 0.9919 | 0.9951 |
| L01 | 23.72 | 1.91 | 0.8868 | 0.9907 |
| L02 | 48.96 | 4.09 | 0.9927 | 0.9904 |
| L03 | 48.28 | 5.12 | 0.9867 | 0.9850 |
| L04 | 62.50 | 5.58 | 0.8569 | 0.9850 |
| L05 | 75.60 | 6.81 | 1.0282 | 0.9733 |
| L06 | 84.66 | 7.58 | 0.8631 | 0.9943 |
| L07 | 99.42 | 8.90 | 0.8684 | 0.9897 |
| L08 | 107.62 | 10.07 | 0.8406 | 0.9888 |
| L09 | 116.59 | 10.23 | 0.8039 | 0.9948 |
| L10 | 136.49 | 12.40 | 0.8981 | 0.9870 |
| S01 | 32.69 | 2.88 | 1.0102 | 0.9954 |
| S02 | 46.07 | 3.93 | 1.0529 | 0.9868 |
| S03 | 70.80 | 5.37 | 0.9518 | 0.9947 |
| S04 | 44.31 | 5.66 | 0.8245 | 0.9940 |
| S05 | 89.10 | 8.69 | 0.9203 | 0.9853 |
| S06 | 76.43 | 7.48 | 1.0095 | 0.9762 |
| S07 | 104.29 | 9.61 | 1.0199 | 0.9775 |
| S08 | 95.49 | 10.69 | 0.9901 | 0.9780 |
| S09 | 155.85 | 14.17 | 1.0273 | 0.9916 |
| S10 | 132.84 | 14.87 | 1.0529 | 0.9922 |
| Type of Rock | Best-Fit Range | Range When R2 Values Exceed 0.95 | Range When R2 Values Exceed 0.80 |
|---|---|---|---|
| Dolomite | 0.86~0.96 | 0.58~1.20 | 0.08~1.46 |
| Granite | 0.65~0.99 | 0.28~1.23 | 0.00~1.47 |
| Limestone | 0.80~1.03 | 0.51~1.22 | 0.04~1.52 |
| Sandstone | 0.82~1.05 | 0.49~1.26 | 0.00~1.32 |
| Type of Rock | Range of Eni/MPa |
|---|---|
| Dolomite | 5.82~20.28 |
| Granite | 3.14~19.76 |
| Limestone | 1.91~12.40 |
| Sandstone | 2.88~14.87 |
| Type of Rock | Linear Fitting y = ax + b | Coefficients of Determination R2 | |
|---|---|---|---|
| a | b | ||
| Dolomite | 8.92 | 15.74 | 0.950 |
| Granite | 10.44 | −6.52 | 0.978 |
| Limestone | 10.94 | 0.87 | 0.988 |
| Sandstone | 9.29 | 7.38 | 0.915 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, C.; Chen, S.; Zhang, Y.; Lin, H.; Wang, Y. A Unified Nonlinear Elastic Model for Rock Material. Appl. Sci. 2022, 12, 12725. https://doi.org/10.3390/app122412725
Chen C, Chen S, Zhang Y, Lin H, Wang Y. A Unified Nonlinear Elastic Model for Rock Material. Applied Sciences. 2022; 12(24):12725. https://doi.org/10.3390/app122412725
Chicago/Turabian StyleChen, Chong, Shenghong Chen, Yihu Zhang, Hang Lin, and Yixian Wang. 2022. "A Unified Nonlinear Elastic Model for Rock Material" Applied Sciences 12, no. 24: 12725. https://doi.org/10.3390/app122412725
APA StyleChen, C., Chen, S., Zhang, Y., Lin, H., & Wang, Y. (2022). A Unified Nonlinear Elastic Model for Rock Material. Applied Sciences, 12(24), 12725. https://doi.org/10.3390/app122412725








