Least-Squares Reverse Time Migration of Primary and Internal Multiple Predicted by the High-Order Born Modeling Method
Abstract
1. Introduction
2. Method
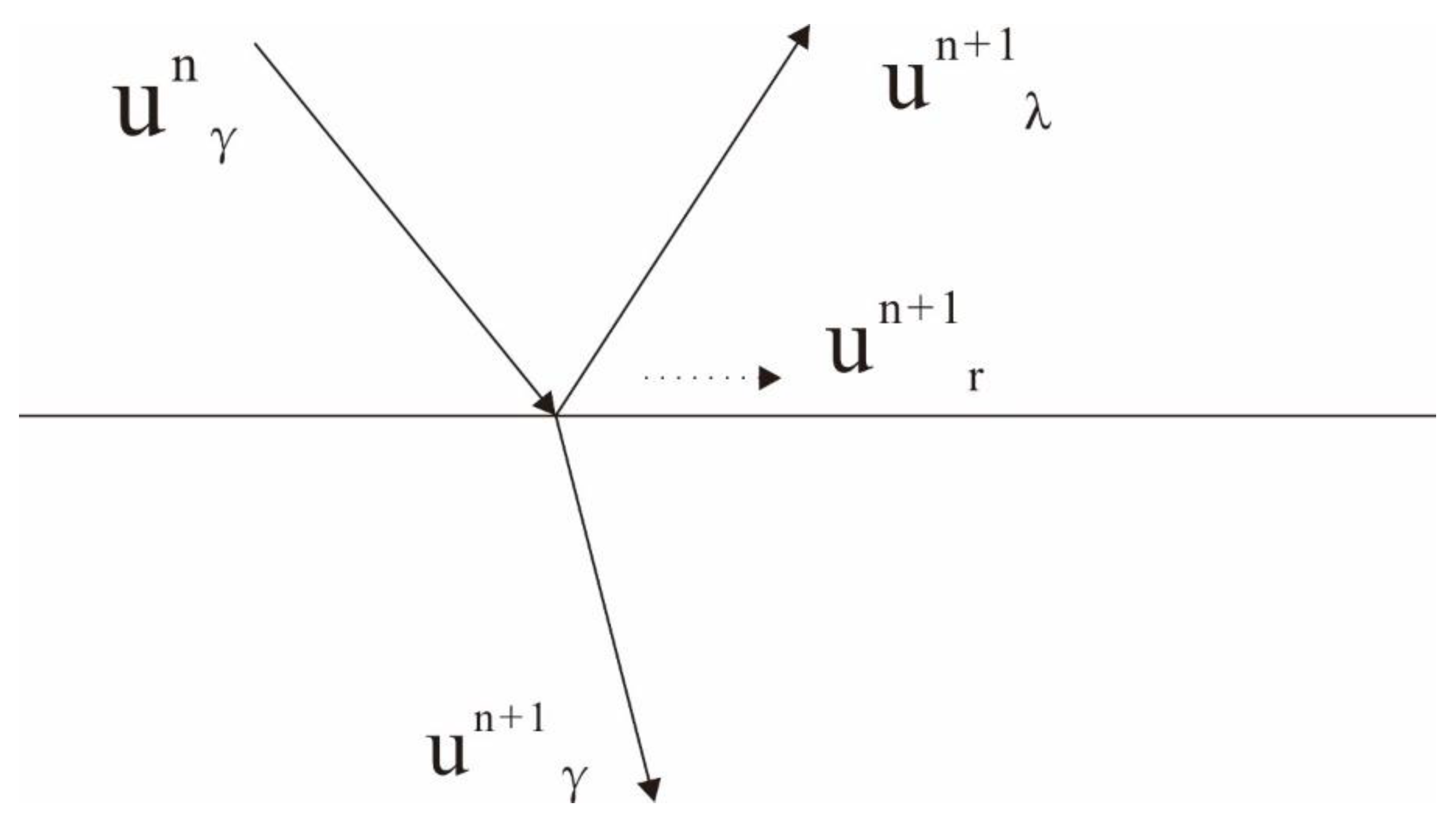
2.1. Multiples and Artifacts Analysis
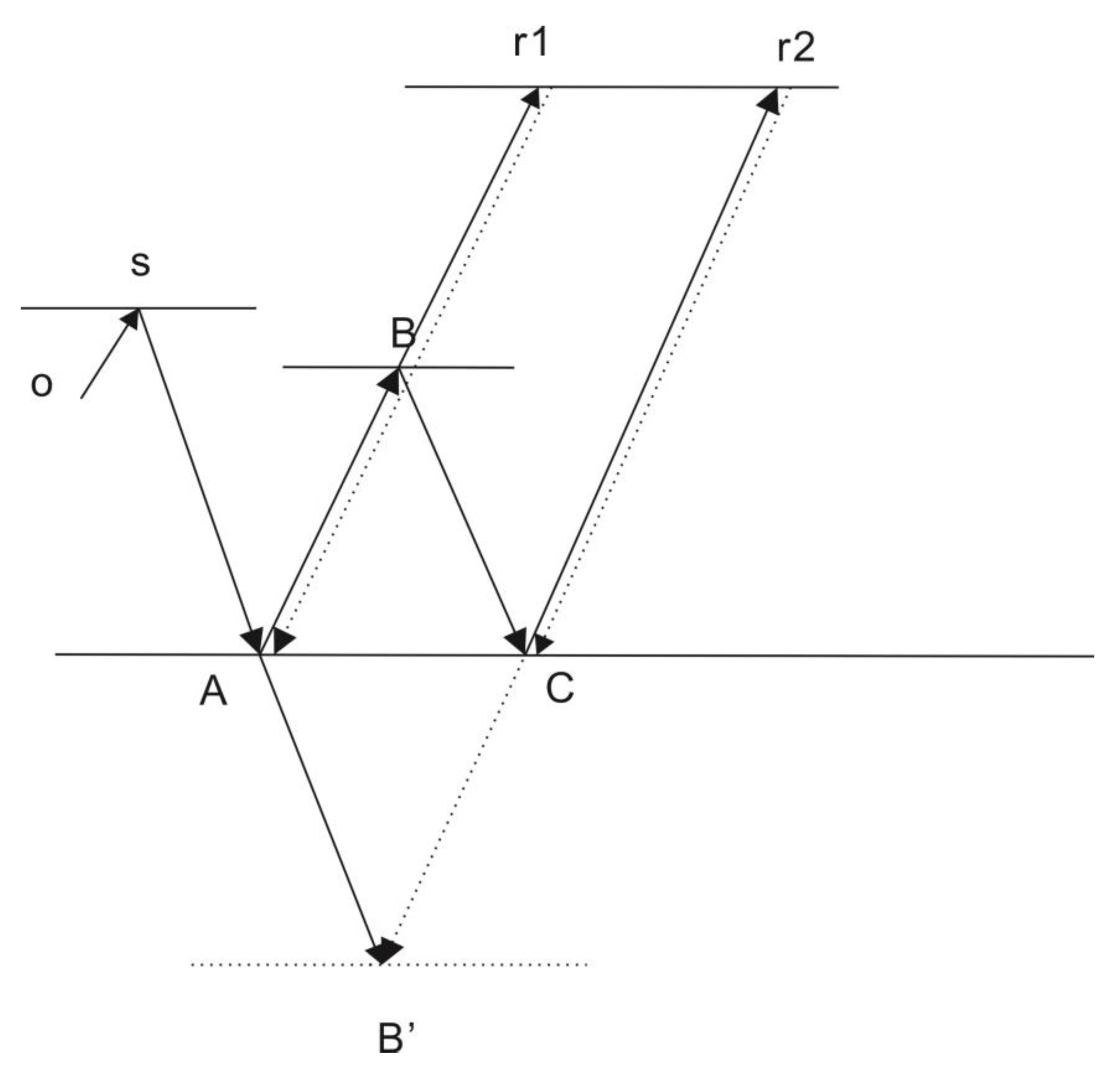
2.2. Internal Multiple Prediction Using High-Order Born Modeling
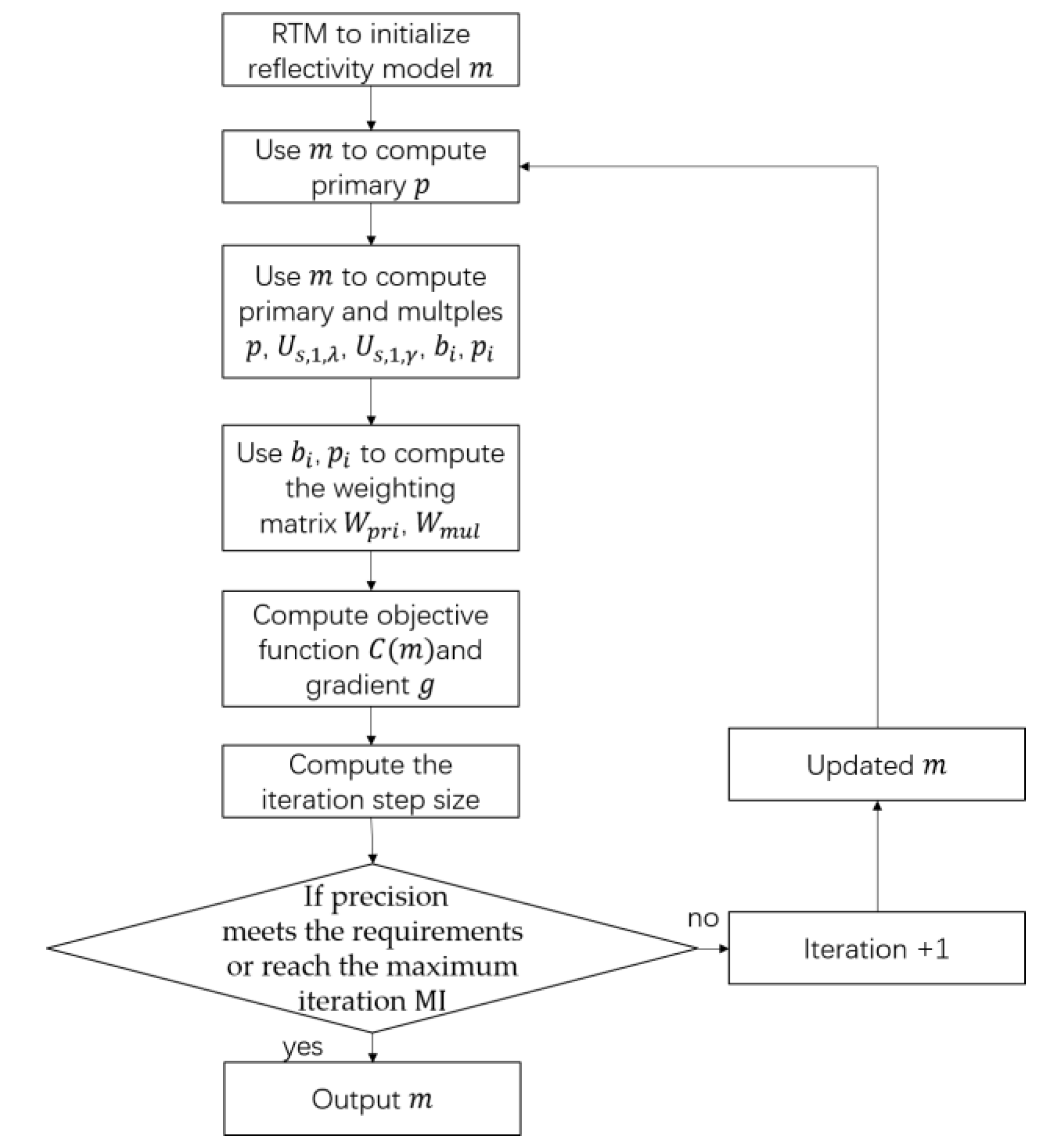
2.3. LSRTM Image of Primary and Internal Multiples
3. Numerical Tests
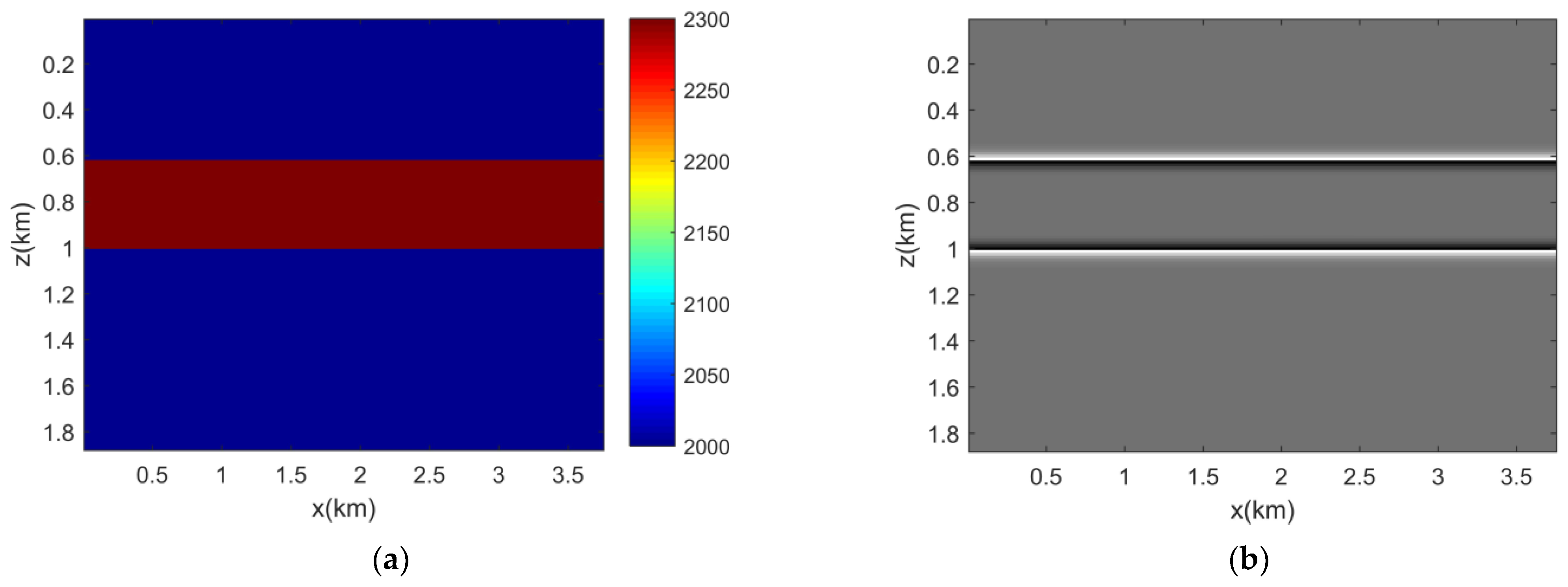
3.1. Examples of Three-Layer Model
3.2. Examples of Two-Dimensional Salt Hill Model
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
References
- Hampson, D. Inverse Velocity Stacking for Multiple Elimination. In Proceedings of the 56th Annual International Meeting, SEG Expanded Abstracts, Houston, TX, USA, 2–6 November 1986; pp. 422–424. [Google Scholar]
- Schneider, W.A.; Backus, M.M. Dynamic correlation analysis. Geophysics 1968, 33, 105–126. [Google Scholar] [CrossRef]
- Taner, M.T.; Koehler, F. Velocity spectra-digital computer derivation and applications of velocity functions. Geophysics 1969, 34, 859–881. [Google Scholar] [CrossRef]
- Robinson, E.A. Predictive decomposition of seismic trace. Geophysics 1957, 22, 767–778. [Google Scholar] [CrossRef]
- Backus, M.M. Water reverberation: Their nature and elimination. Geophysics 1959, 24, 233–261. [Google Scholar] [CrossRef]
- Ryu, J.V. Decomposition (DECOM) approach applied to wave field analysis with seismic reflection records. Geophysics 1982, 47, 869–883. [Google Scholar] [CrossRef]
- Yilmaz, O. Seismic Data Processing; Electronic Edition; Society of Exploration Geophysicists: Tulsa, OK, USA, 1987. [Google Scholar]
- Foster, D.J.; Mosher, C.C. Suppression of multiple reflections using the Radon transform. Geophysics 1992, 57, 386–395. [Google Scholar] [CrossRef]
- Jakubowicz, H. Wave equation prediction and removal of interbed multiples. In Proceedings of the 68th Annual International Meeting, SEG Expanded Abstracts, New Orleans, LA, USA, 13–18 September 1998; pp. 1527–1530. [Google Scholar]
- Verschuur, D.J.; Berkhout, A.J. Removal of internal multiples with the common- focus-point (CFP) approach: Part 2—Application strategies and data examples. Geophysics 2005, 70, V61–V72. [Google Scholar] [CrossRef]
- Ikelle, L.T. A construct of internal multiples form surface data only: The concept of virtual seismic events. Geophysics 2006, 164, 383–393. [Google Scholar]
- Ikelle, L.T. Scattering diagrams in seismic imaging: More insights into the construction of virtual events and internal multiples. J. Appl. Geophys. 2009, 67, 150–170. [Google Scholar] [CrossRef]
- Yuan, S.; Wang, S.; Yuan, F.; Liu, Y. The influence of errors in the source wavelet on inversion-based surface-related multiple attenuation. Geophys. Prospect. 2018, 66, 55–73. [Google Scholar] [CrossRef]
- Carvalho, F.M.; Weglein, A.B.; Stolt, R.H. Examples of a non-linear inversion method based on the T matrix of scattering theory: Application to multiple suppression. In Proceedings of the 61st Annual International Meeting, SEG Expanded Abstracts, Houston, CA, USA, 10–14 November 1991; pp. 1319–1322. [Google Scholar]
- Carvalho, F.M.; Weglein, A.B.; Stolt, R.H. Non-linear inverse scattering for multiple suppression: Application to real data, Part I. In Proceedings of the 62nd Annual International Meeting, SEG Expanded Abstracts, New Orleans, LA, USA, 25–29 October 1992; pp. 1093–1095. [Google Scholar]
- Carvalho, F.M. Free-Surface Multiple Reflection Elimination Method Based on Non-Linear Inversion of Seismic Data. Ph.D. Dissertation, Universidade Federal da Bahia, Salvador, Brazil, 1992. (In Portuguese). [Google Scholar]
- Weglein, A.B.; Gasparotto, E.A.; Carvalho, P.M.; Stolt, R.H. An inverse-scattering series method for attenuating multiples in seismic reflection data. Geophysics 1997, 62, 1975–1989. [Google Scholar] [CrossRef]
- Weglein, A.B. Multiple attenuation: An overview of recent advances and the road ahead. Lead. Edge 1999, 18, 40–44. [Google Scholar] [CrossRef]
- Matson, K.H. The relationship between seattering theory and the Primaries and multiples of refiection seismic data. J. Seism. Explor. 1996, 5, 63–78. [Google Scholar]
- Matson, K.H. An inverses scattering series method for attenuating elastic multiples from multicomponent land ocean bottom seismic data. Ph.D. Dissertation, Dept. EOAS., British Columbia Univ. B.C., Vancouver, BC, Canada, 1997. [Google Scholar]
- Ikelle, L.T. Using even terms of the scattering series for deghosting and multiple attenuation of ocean-bottom cable data. Geophysics 1999, 64, 579–592. [Google Scholar] [CrossRef]
- Ramírez, A.C.; Weglein, A.B. An inverse scattering internal multiple elimination method: Beyond attenuation, a new algorithm, and initial tests. In Proceedings of the 75th Annual International Meeting, SEG Expanded Abstracts, Houston, TX, USA, 6–11 November 2005; pp. 2115–2118. [Google Scholar]
- Pica, A.; Laurie, D. Wave equation based internal multiple modeling in 3D. In Proceedings of the 78th Annual International Meeting, SEG Expanded Abstracts, Las Vegas, NV, USA, 9–14 November 2008; pp. 2476–2480. [Google Scholar]
- van der Neut, J.; Wapenaar, K. Adaptive overburden elimination with the multidimensional Marchenko equation. Geophysics 2016, 81, T265–T284. [Google Scholar] [CrossRef]
- Berkhout, A.J. Combining full wavefield migration and full waveform inversion. In Proceedings of the 81st Annual International Meeting, SEG Expanded Abstracts, San Antonio, TX, USA, 18–23 September 2011; pp. 3321–3325. [Google Scholar]
- Berkhout, A.J. Combining full wavefield migration and full waveform inversion, a glance into the future of seismic imaging. Geophysics 2012, 77, S43–S50. [Google Scholar] [CrossRef]
- Berkhout, A.J. Review Paper: An outlook on the future of seismic imaging, Part II: Full-Wavefield Migration. Geophys. Prospect. 2014, 62, 931–949. [Google Scholar] [CrossRef]
- Whitmore, N.D. Iterative depth migration by backward time propagation. In Proceedings of the 53rd Annual International Meeting, SEG Expanded Abstracts, Las Vegas, NV, USA, 11–15 September 1983; pp. 382–385. [Google Scholar]
- Moradpouri, F.; Moradzadeh, A.; Pestana, R.C. Seismic reverse time migration using a new wave-field extrapolator and a new imaging condition. Acta Geophys. 2016, 64, 1673–1690. [Google Scholar] [CrossRef]
- Moradpouri, F.; Moradzadeh, A.; Pestana, R.N.C.; Ghaedrahmati, R.; Soleimani, M. An improvement in wave-field extrapolation and imaging condition to suppress RTM artifacts. Geophysics 2017, 82, S403–S409. [Google Scholar] [CrossRef]
- Soleimani, M. Challenges of seismic imaging in complex media around Iran, from Zagros overthrust in the southwest to Gorgan Plain in the northeast. Lead. Edge 2017, 36, 499–506. [Google Scholar] [CrossRef]
- Moradpouri, F. A new approach in reverse time migration for properly imaging complex geological media. Acta Geophys. 2021, 69, 529–538. [Google Scholar] [CrossRef]
- Tarantola, A. Linearized Inversion of Seismic Reflection Data. Geophys. Prospect. 1984, 32, 998–1015. [Google Scholar] [CrossRef]
- Dai, W.; Wang, X.; Schuster, G.T. Least-squares migration of multisource data with a deblurring filter. Geophysics 2011, 76, R135–R146. [Google Scholar] [CrossRef]
- He, R.; Schuster, G. Least-squares migration of both primaries and multiples. In Proceedings of the 73rd Annual International Meeting, SEG Expanded Abstracts, Dallas, TX, USA, 26–31 October 2003; pp. 1035–1038. [Google Scholar]
- Liu, Y.; Xu, C.; Jin, D.; He, R.; Sun, H.; Zheng, R. Reverse time migration of multiples. In Proceedings of the 81st Annual International Meeting, SEG Expanded Abstracts, San Antonio, TX, USA, 18–23 September 2011; pp. 3326–3331. [Google Scholar]
- Liu, Y.; Xu, C.; Jin, D.; He, R.; Sun, H.; Zheng, R. Reverse time migration of multiples for subsalt imaging. Geophysics 2011, 76, WB209–WB216. [Google Scholar] [CrossRef]
- Zhang, D.; Schuster, G.T. Least-squares reverse time migration of multiples. Geophysics 2014, 79, S11–S21. [Google Scholar] [CrossRef]
- Liu, X.; Liu, Y.; Khan, M. Fast least-squares reverse time migration of VSP free-surface multiples with dynamic phase-encoding schemes. Geophysics 2018, 83, S321–S332. [Google Scholar] [CrossRef]
- Li, Z.; Li, Z.; Li, Q.; Li, Q.; Sun, M.; Hu, P.; Li, L. Least-squares reverse time migration of multiples in visco-acoustic media. Geophysics 2020, 85, S285–S297. [Google Scholar] [CrossRef]
- Wong, M.; Biondi, B.; Ronen, S. Imaging with primaries and free-surface multiples by joint least-squares reverse time migration. Geophysics 2015, 80, S223–S235. [Google Scholar] [CrossRef]
- Liu, X.; Liu, Y. Plane-wave domain least-squares reverse time migration with free-surface multiples. Geophysics 2018, 83, S477–S487. [Google Scholar] [CrossRef]
- Qu, Y.; Huang, C.; Liu, C.; Li, Z. Full-Path Compensated Least-Squares Reverse Time Migration of Joint Primaries and Different-Order Multiples for Deep-Marine Environment. IEEE Trans. Geosci. Remote Sens. 2021, 59, 7109–7121. [Google Scholar] [CrossRef]
- Liu, Y.; Liu, X.; Osen, A.; Shao, Y.; Hu, H.; Zheng, Y. Least-squares reverse time migration using controlled-order multiple reflections. Geophysics 2016, 81, S347–S357. [Google Scholar] [CrossRef]
- Li, C.; Huang, J.; Li, Z.; Yu, H.; Wang, R. Least-squares migration with primary- and multiple-guided weighting matrices. Geophysics 2019, 84, S171–S185. [Google Scholar] [CrossRef]
- Chen, R.; Han, L.; Zhang, P.; Dong, S.; Yin, Y. Internal multiple prediction using high-order born modeling for LSRTM. Acta Geophys. 2022, 70, 1491–1505. [Google Scholar] [CrossRef]
- Nocedal, J. Updating quasi-Newton matrices with limited storage. Math. Comput. 1980, 35, 773–782. [Google Scholar] [CrossRef]
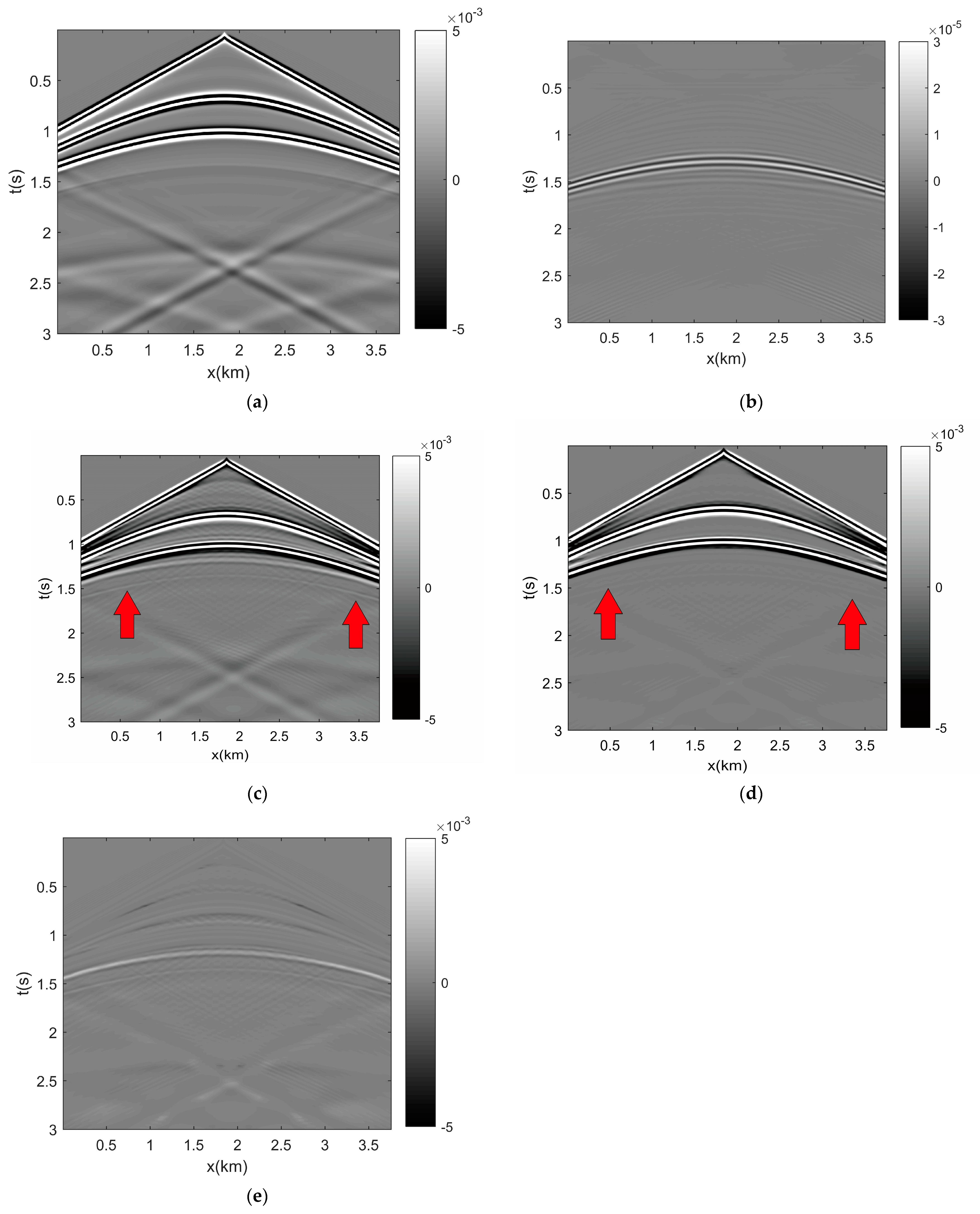

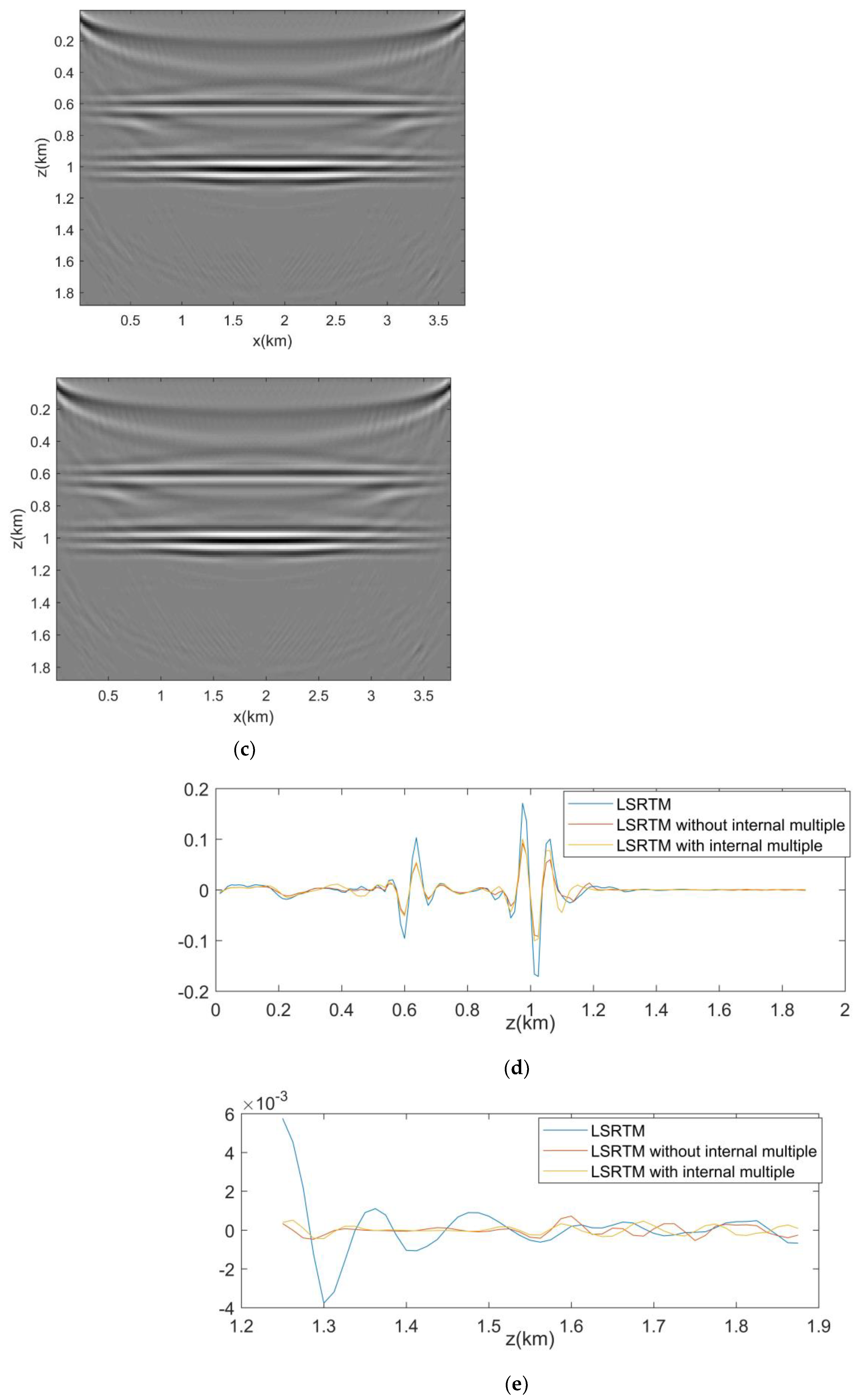
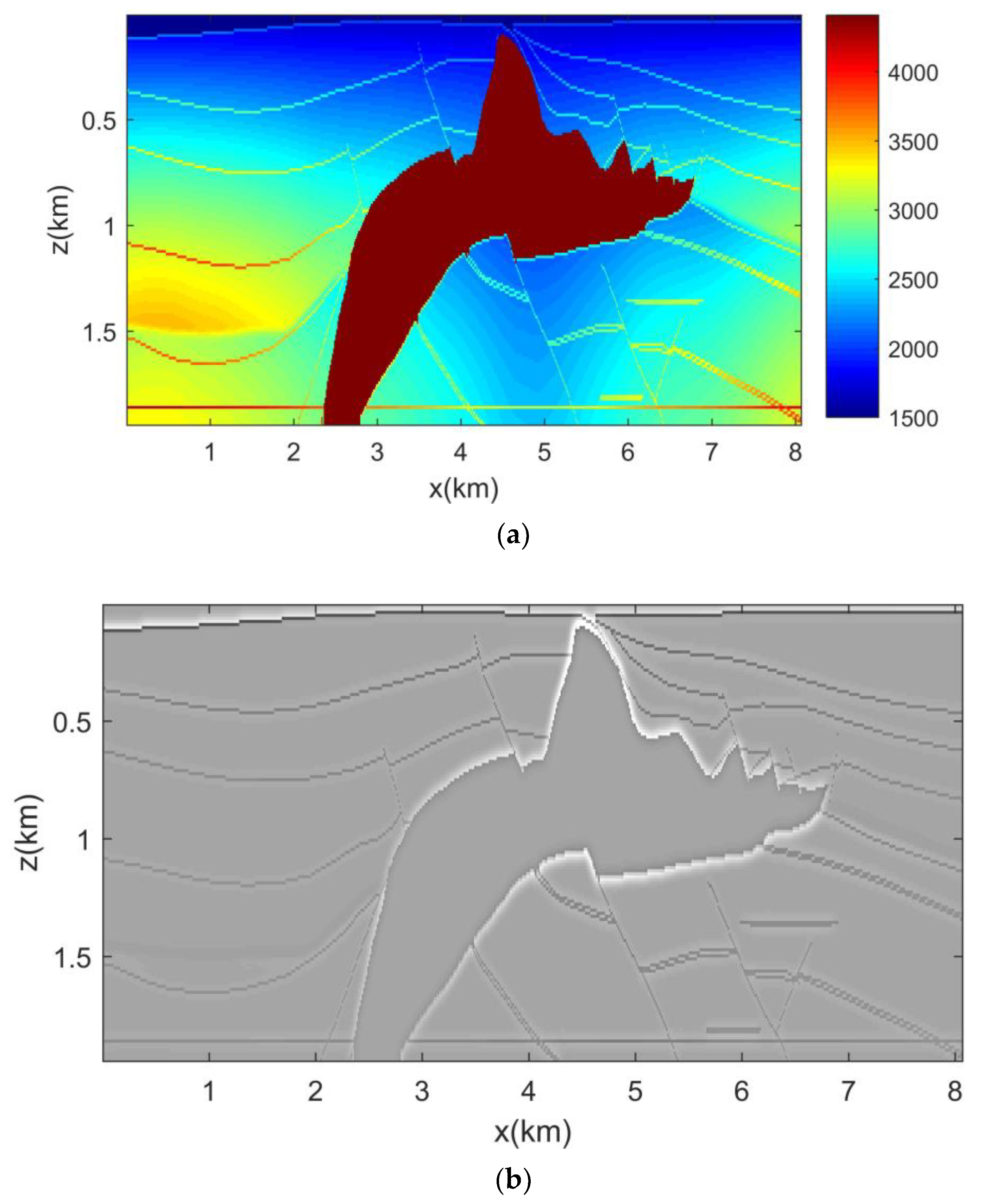
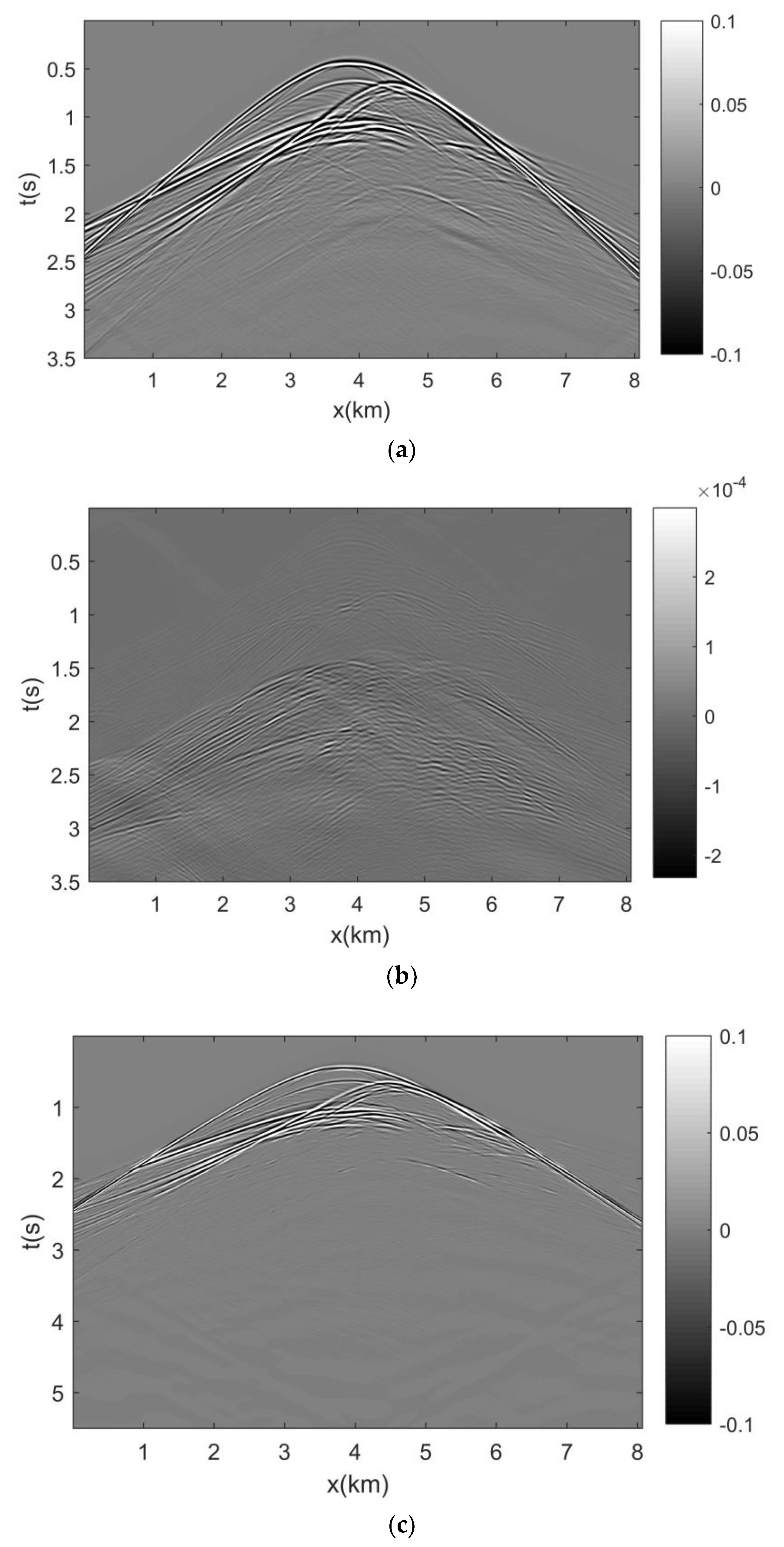
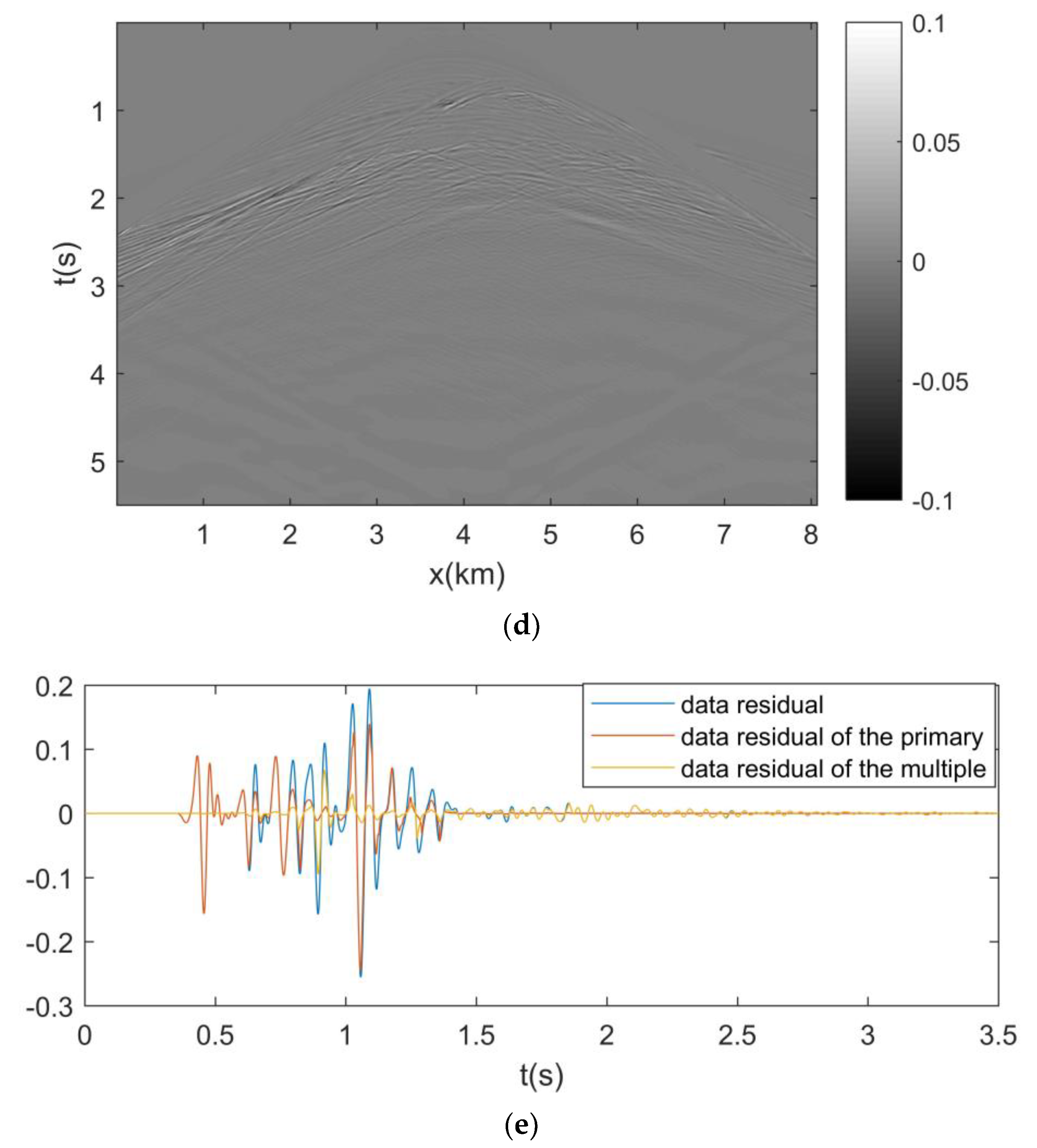
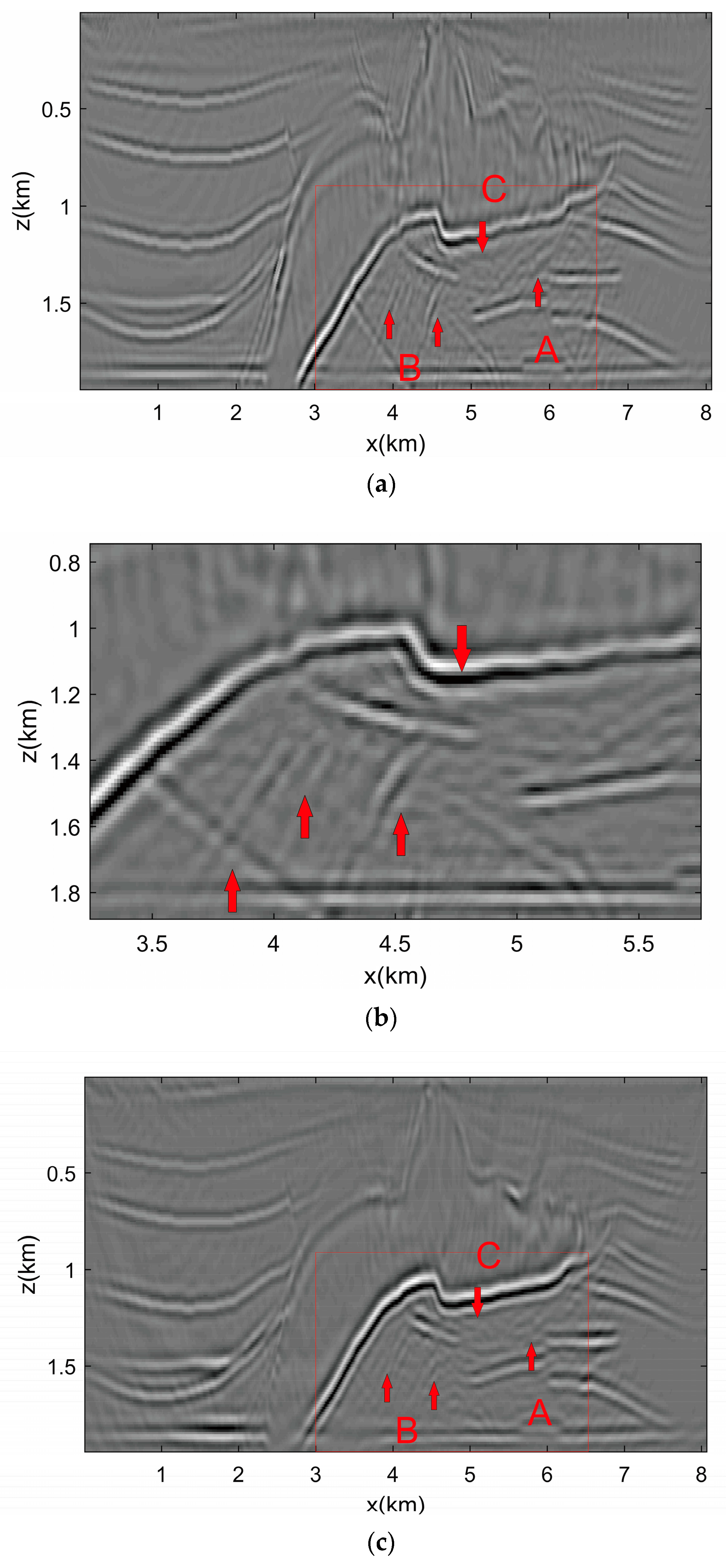
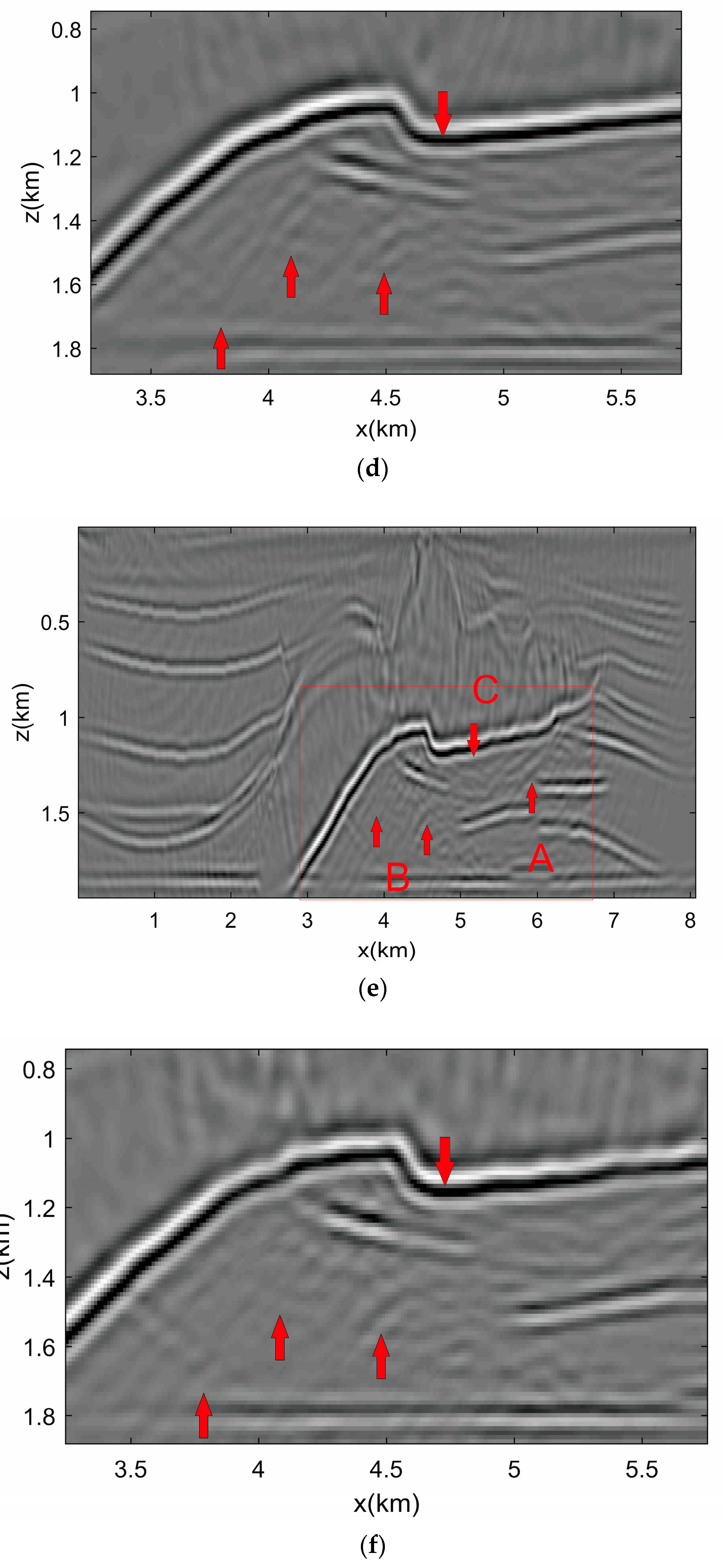
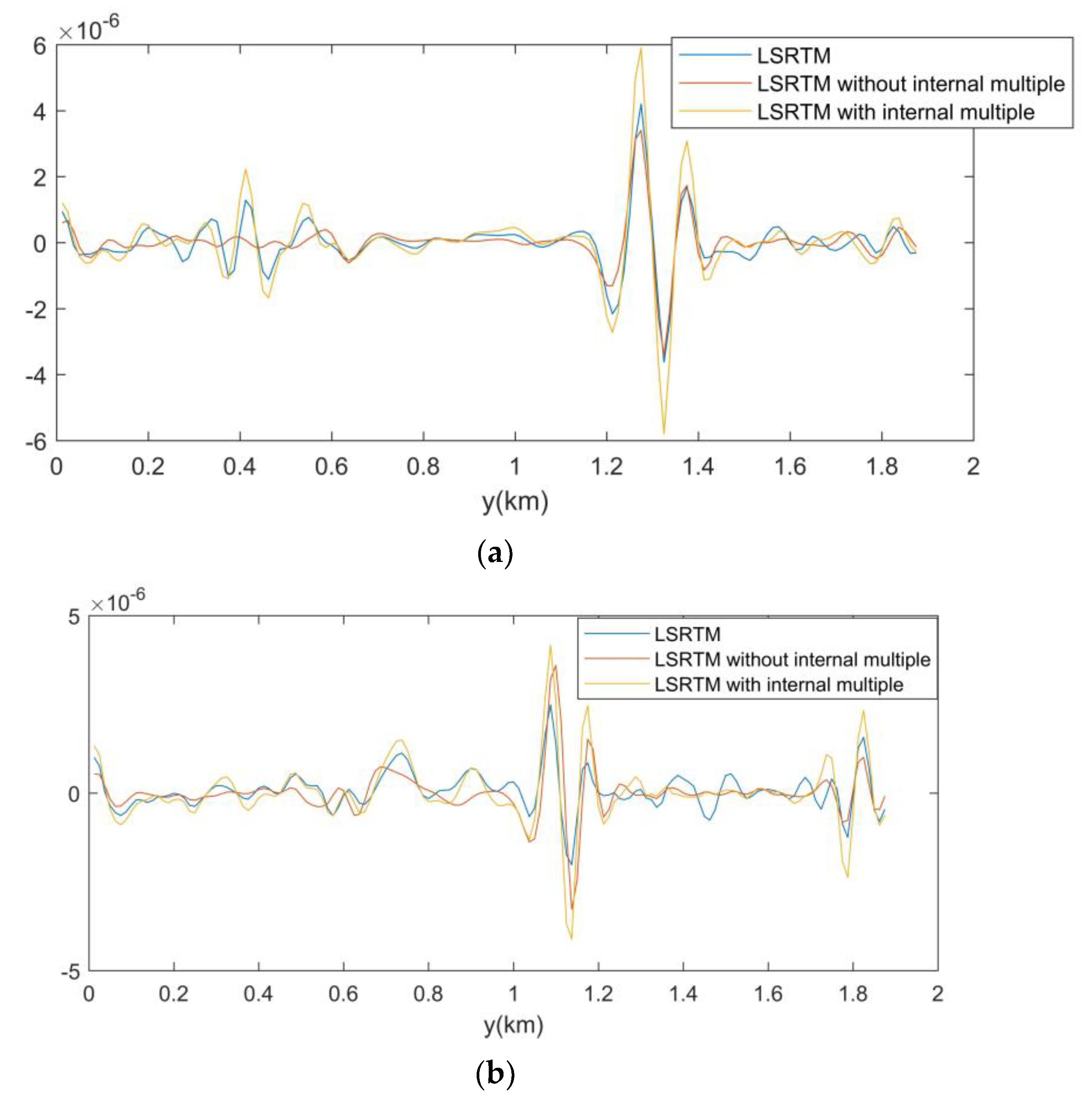
| The Model | Three-Layer Model | Two-Dimensional Salt Hill Model |
|---|---|---|
| The data and multiple of single shot | 1. the 30th shot; 2. shows the primary and the internal multiple used in RWLSRTM | 1. the 35th shot; 2. shows the primary and the internal multiple used in RWLSRTM |
| Comparison of LSRTM with or without internal multiple | 1. The artifacts can be eliminated by the LSRTM with or without internal multiple | 1. The artifacts can be eliminated by the LSRTM with or without internal multiple; 2. the useful structure is damaged in LSRTM without an internal multiple while it is compensated in LSRTM with the internal multiple |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, R.; Han, L.; Zhang, P. Least-Squares Reverse Time Migration of Primary and Internal Multiple Predicted by the High-Order Born Modeling Method. Appl. Sci. 2022, 12, 10657. https://doi.org/10.3390/app122010657
Chen R, Han L, Zhang P. Least-Squares Reverse Time Migration of Primary and Internal Multiple Predicted by the High-Order Born Modeling Method. Applied Sciences. 2022; 12(20):10657. https://doi.org/10.3390/app122010657
Chicago/Turabian StyleChen, Ruiding, Liguo Han, and Pan Zhang. 2022. "Least-Squares Reverse Time Migration of Primary and Internal Multiple Predicted by the High-Order Born Modeling Method" Applied Sciences 12, no. 20: 10657. https://doi.org/10.3390/app122010657
APA StyleChen, R., Han, L., & Zhang, P. (2022). Least-Squares Reverse Time Migration of Primary and Internal Multiple Predicted by the High-Order Born Modeling Method. Applied Sciences, 12(20), 10657. https://doi.org/10.3390/app122010657





