Buckling Analysis of a Composite Honeycomb Reinforced Sandwich Embedded with Viscoelastic Damping Material
Abstract
1. Introduction
2. Mathematical Modeling
3. Methodologies and Theoretical Derivation
3.1. Critical Buckling Equation Determination
3.2. Equivalent Effective Modulus Determination
3.3. Homogenous Asymptotic Methodologies
4. Numerical Analysis
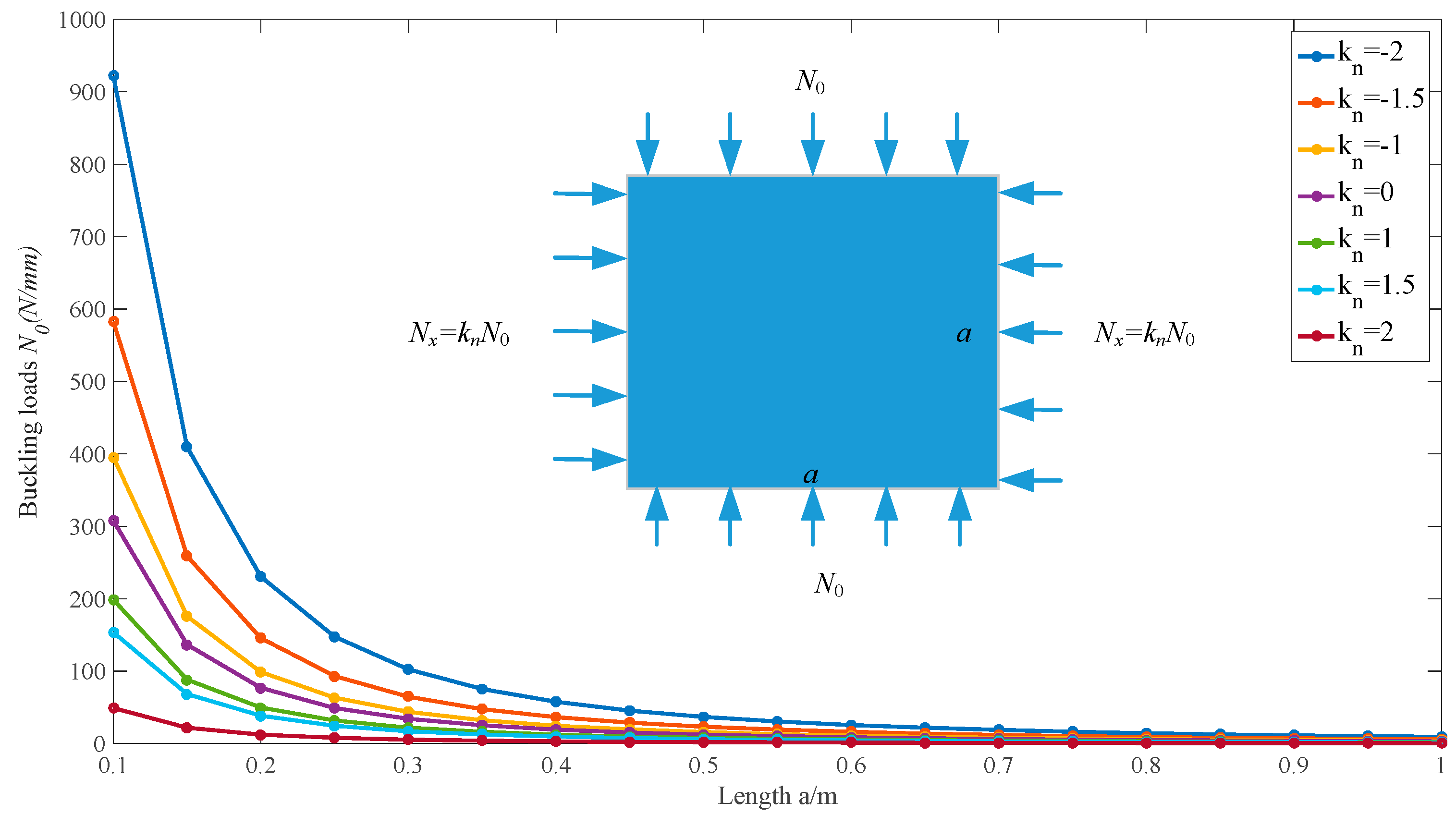
4.1. Critical Dynamic Buckling Load Affected by the Load Parameter kn
4.2. Critical Buckling Load Affected by the Length/Width Geometry Parameter
4.3. Critical Buckling Load Affected by the Load Frequency
4.4. Critical Buckling Load Affected by the Core Layer’s Thickness
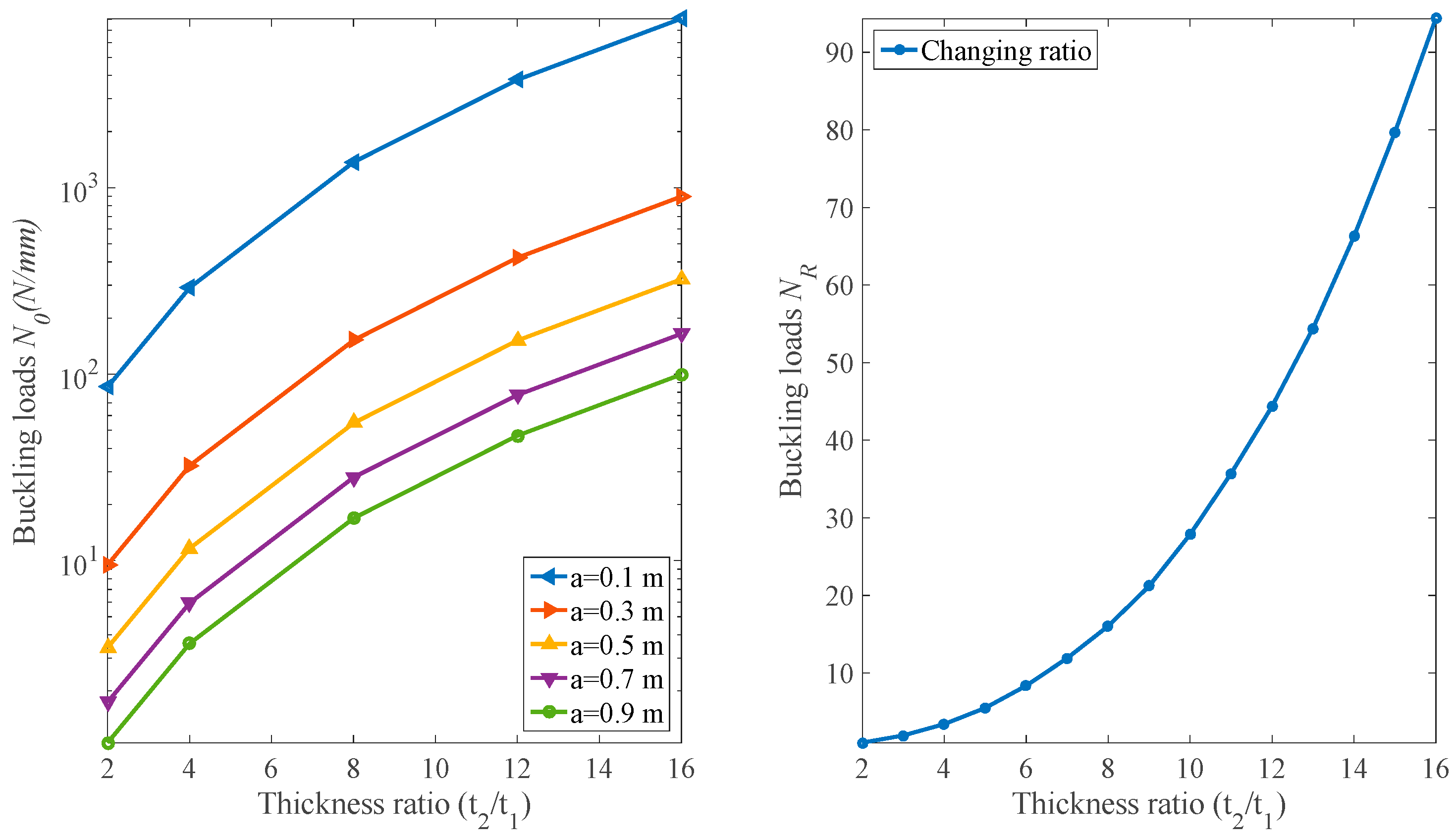
4.5. Critical Buckling Load Affected by the Honeycomb Reinforcement’s Thickness
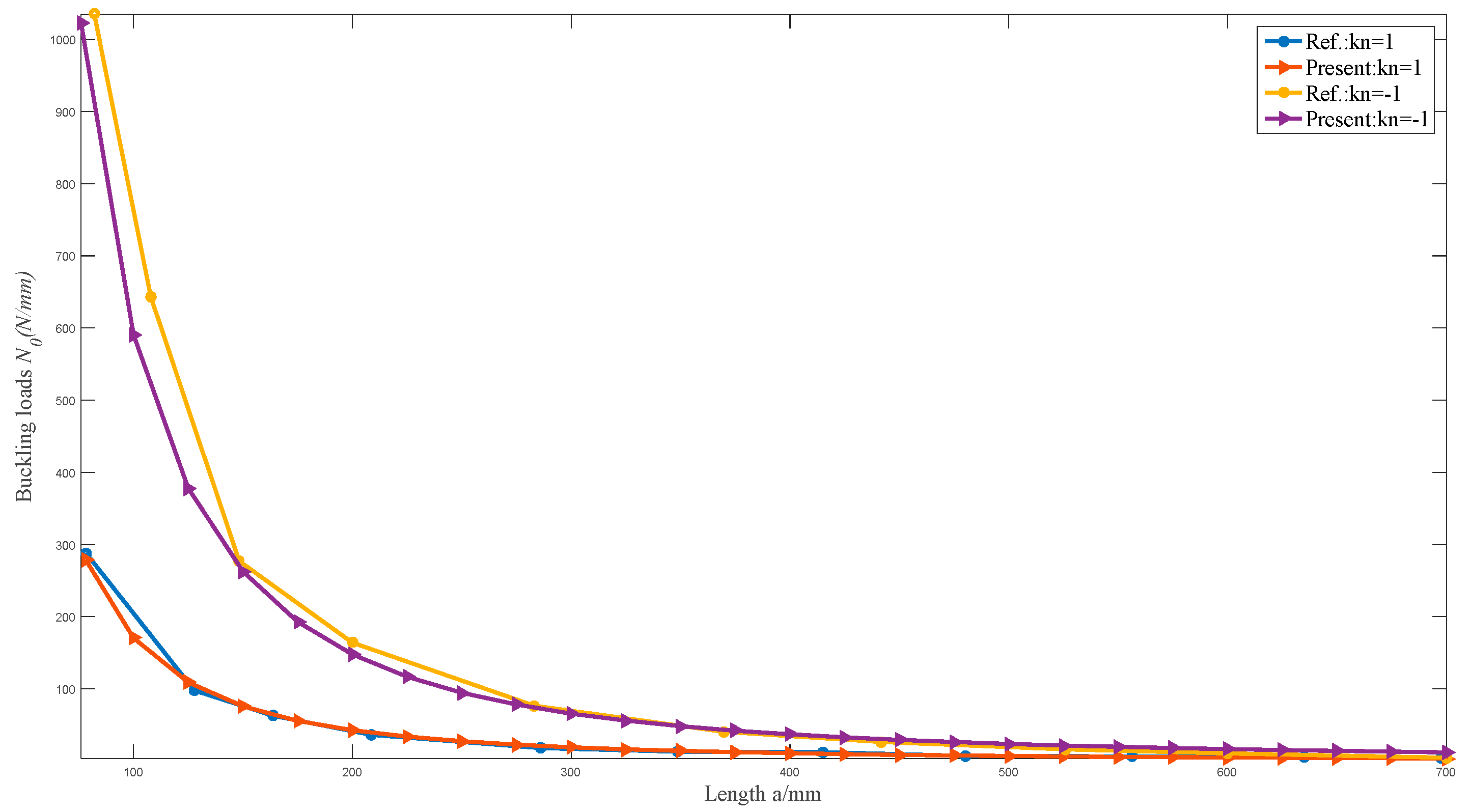
5. Validation
6. Conclusions and Discussion
- (1)
- The physical parameters, such as the elastic modulus and shear modulus of the viscoelastic material, are affected by the load’s frequencies; accordingly, the buckling loads of the composite structure are influenced by the load’s frequency. The trend of the buckling loads affected by the loading frequency is the same as the composite structure’s dynamic equivalent effective stiffness, and the original phenomenon can be traced back to the behaviors of viscoelastic materials.
- (2)
- Buckling loads are affected by the geometry parameters of the composite sandwich structure directly. The buckling load is significantly increased by increasing by the width of the composite structure, but it is decreased sharply by increasing the length of the sandwich structure. This phenomenon shows the same results as those of the buckling analysis of classical beams and plates.
- (3)
- The composite structure’s buckling load is influenced by the honeycomb reinforcement layer’s thickness and the honeycomb’s width. The main reason is that the equivalent stiffness of the composite sandwich structure is changed by the honeycomb reinforcement’s width and height. It should be noted that, by comparing it to the honeycomb reinforcement layer’s thickness, the honeycomb’s width affects the structure’s buckling loads to a relatively smaller degree.
Author Contributions
Funding
Conflicts of Interest
References
- Pearson, C.; Delatte, N. Collapse of the Quebec bridge, 1907. J. Perform. Constr. Facil. 2006, 20, 84–91. [Google Scholar] [CrossRef]
- Turvey, G.J.; Marshall, I.H. Buckling and Postbuckling of Composite Plates; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2012. [Google Scholar]
- Wang, C.M.; Wang, C.Y. Exact Solutions for Buckling of Structural Members; CRC Press: Boca Raton, FL, USA, 2004. [Google Scholar]
- Sayyad, A.S.; Ghugal, Y.M. Bending, buckling and free vibration of laminated composite and sandwich beams: A critical review of literature. Compos. Struct. 2017, 171, 486–504. [Google Scholar] [CrossRef]
- Namdar, Ö.; Darendeliler, H. Buckling, postbuckling and progressive failure analyses of composite laminated plates under compressive loading. Compos. Part B Eng. 2017, 120, 143–151. [Google Scholar] [CrossRef]
- Kolahchi, R.; Zarei, M.S.; Hajmohammad, M.H.; Naddaf Oskouei, A. Visco-nonlocal-refined Zigzag theories for dynamic buckling of laminated nanoplates using differential cubature-Bolotin methods. Thin-Walled Struct. 2017, 113, 162–169. [Google Scholar] [CrossRef]
- Ho-Huu, V.; Do-Thi, T.D.; Dang-Trung, H.; Vo-Duy, T.; Nguyen-Thoi, T. Optimization of laminated composite plates for maximizing buckling load using improved differential evolution and smoothed finite element method. Compos. Struct. 2016, 146, 132–147. [Google Scholar] [CrossRef]
- Ehsani, A.; Rezaeepazhand, J. Stacking sequence optimization of laminated composite grid plates for maximum buckling load using genetic algorithm. Int. J. Mech. Sci. 2016, 119, 97–106. [Google Scholar] [CrossRef]
- Huang, L.; Sheikh, A.H.; Ng, C.-T.; Griffith, M.C. An efficient finite element model for buckling analysis of grid stiffened laminated composite plates. Compos. Struct. 2015, 122, 41–50. [Google Scholar] [CrossRef]
- Chen, Q.; Qiao, P. Buckling analysis of laminated plate structures with elastic edges using a novel semi-analytical finite strip method. Compos. Struct. 2016, 152, 85–95. [Google Scholar] [CrossRef]
- Arshid, E.; Amir, S.; Loghman, A. Bending and buckling behaviors of heterogeneous temperature-dependent micro annular/circular porous sandwich plates integrated by FGPEM nano-Composite layers. J. Sandw. Struct. Mater. 2021, 23, 3836–3877. [Google Scholar] [CrossRef]
- Gunawan, D.; Suryoatmono, B. Numerical study on lateral-torsional buckling of honeycomb beam. Procedia Eng. 2017, 171, 140–146. [Google Scholar] [CrossRef]
- Southward, T.; Mallinson, G.; Jayaraman, K.; Horrigan, D. Buckling of disbonds in honeycomb-core sandwich beams. J. Sandw. Struct. Mater. 2008, 10, 195–216. [Google Scholar] [CrossRef]
- Li, C.; Shen, H.-S.; Wang, H. Thermal post-buckling of sandwich beams with functionally graded negative Poisson's ratio honeycomb core. Int. J. Mech. Sci. 2019, 152, 289–297. [Google Scholar] [CrossRef]
- Arefi, M.; Najafitabar, F. Buckling and free vibration analyses of a sandwich beam made of a soft core with FG-GNPs reinforced composite face-sheets using Ritz Method. Thin-Walled Struct. 2021, 158, 107200. [Google Scholar] [CrossRef]
- Qiu, C.; Guan, Z.; Guo, X.; Li, Z. Buckling of honeycomb structures under out-of-plane loads. J. Sandw. Struct. Mater. 2020, 22, 797–821. [Google Scholar] [CrossRef]
- Ohno, N.; Okumura, D.; Niikawa, T. Long-wave buckling of elastic square honeycombs subject to in-plane biaxial compression. Int. J. Mech. Sci. 2004, 46, 1697–1713. [Google Scholar] [CrossRef]
- Miller, W.; Smith, C.W.; Evans, K.E. Honeycomb cores with enhanced buckling strength. Compos. Struct. 2011, 93, 1072–1077. [Google Scholar] [CrossRef]
- López Jiménez, F.; Triantafyllidis, N. Buckling of rectangular and hexagonal honeycomb under combined axial compression and transverse shear. Int. J. Solids Struct. 2013, 50, 3934–3946. [Google Scholar] [CrossRef]
- Zhou, X.Q.; Yu, D.Y.; Shao, X.Y.; Wang, S. Asymptotic homogenization analysis of the dynamics properties of periodically and orthogonally stiffened composite laminates. Compos. Part B Eng. 2016, 105, 116–131. [Google Scholar] [CrossRef]
- Usta, F.; Türkmen, H.S.; Scarpa, F. High-velocity impact resistance of doubly curved sandwich panels with re-entrant honeycomb and foam core. Int. J. Impact Eng. 2022, 165, 104230. [Google Scholar] [CrossRef]
- Meng, H.; Galland, M.A.; Ichchou, M.; Xin, F.X.; Lu, T.J. On the low frequency acoustic properties of novel multifunctional honeycomb sandwich panels with micro-perforated faceplates. Appl. Acoust. 2019, 152, 31–40. [Google Scholar] [CrossRef]
- Zhou, X.; Yu, D.; Zhang, S. Homogenous asymptotic analysis on vibration energy dissipation characteristics of periodical honeycomb reinforced composite laminate filled with viscoelastic damping material. Compos. Struct. 2021, 258, 113307. [Google Scholar] [CrossRef]
- Zhou, X.Q.; Zhang, S.Q.; Lin, W.W. Sound radiation characteristics analysis for the honeycomb reinforced laminated structures with viscoelastic material fillers through the asymptotic homogenous method. Compos. Struct. 2020, 245, 112266. [Google Scholar] [CrossRef]
- Du, B.; Chen, L.; Wu, W.; Liu, H.; Zhao, Y.; Peng, S.; Guo, Y.; Zhou, H.; Chen, L.; Li, W.; et al. A novel hierarchical thermoplastic composite honeycomb cylindrical structure: Fabrication and axial compressive properties. Compos. Sci. Technol. 2018, 164, 136–145. [Google Scholar] [CrossRef]
- Eipakchi, H.; Nasrekani, F.M. Vibrational behavior of composite cylindrical shells with auxetic honeycombs core layer subjected to a moving pressure. Compos. Struct. 2020, 254, 112847. [Google Scholar] [CrossRef]
- Cong, P.H.; Long, P.T.; Van Nhat, N.; Duc, N.D. Geometrically nonlinear dynamic response of eccentrically stiffened circular cylindrical shells with negative poisson’s ratio in auxetic honeycombs core layer. Int. J. Mech. Sci. 2019, 152, 443–453. [Google Scholar] [CrossRef]
- Zhou, X.Q.; Yu, D.Y.; Shao, X.Y.; Zhang, S.Q.; Wang, S. Research and applications of viscoelastic vibration damping materials: A review. Compos. Struct. 2016, 136, 460–480. [Google Scholar] [CrossRef]
- Zhou, X.Q.; Yu, D.Y.; Shao, X.Y.; Wang, S.; Zhang, S.Q. Asymptotic analysis for composite laminated plate with periodically fillers in viscoelastic damping material core. Compos. Part B Eng. 2016, 96, 45–62. [Google Scholar] [CrossRef]
- Zhou, X.Q.; Yu, D.Y.; Shao, X.; Zhang, S.Q.; Wang, S. Research on the low frequency band gap properties of periodically composite stiffened thin-plate with fillers. Thin-Walled Struct. 2016, 108, 41–55. [Google Scholar] [CrossRef]
- Guesmia, A.; Messaoudi, S.A. General energy decay estimates of Timoshenko systems with frictional versus viscoelastic damping. Math. Methods Appl. Sci. 2009, 32, 2102–2122. [Google Scholar] [CrossRef]
- Saravanan, C.; Ganesan, N.; Ramamurti, V. Vibration and damping analysis of multilayered fluid filled cylindrical shells with constrained viscoelastic damping using modal strain energy method. Comput. Struct. 2000, 75, 395–417. [Google Scholar] [CrossRef]
- Suhr, J.; Koratkar, N.; Keblinski, P.; Ajayan, P. Viscoelasticity in carbon nanotube composites. Nat. Mater. 2005, 4, 134. [Google Scholar] [CrossRef] [PubMed]
- Jung, W.-Y.; Aref, A.J. A combined honeycomb and solid viscoelastic material for structural damping applications. Mech. Mater. 2003, 35, 831–844. [Google Scholar] [CrossRef]
- Aumjaud, P.; Smith, C.W.; Evans, K.E. A novel viscoelastic damping treatment for honeycomb sandwich structures. Compos. Struct. 2015, 119, 322–332. [Google Scholar] [CrossRef]
- Gibson, R.F. Principles of Composite Material Mechanics; CRC Press: Boca Raton, FL, USA, 2016. [Google Scholar]
- Kassapoglou, C. Design and Analysis of Composite Structures: With Applications to Aerospace Structures; John Wiley & Sons: Hoboken, NJ, USA, 2013. [Google Scholar]
- Timoshenko, S.; Woinowsky-Krieger, S.; Woinowsky-Krieger, S. Theory of Plates and Shells; McGraw-Hill: New York, NY, USA, 1959. [Google Scholar]
- Reddy, J.N. Mechanics of Laminated Composite Plates and Shells: Theory and Analysis; CRC Press: Boca Raton, FL, USA, 2003. [Google Scholar]
- Cai, Y.; Xu, L.; Cheng, G. Novel numerical implementation of asymptotic homogenization method for periodic plate structures. Int. J. Solids Struct. 2014, 51, 284–292. [Google Scholar] [CrossRef]
- Smith, P.A.; Wood, J.R. Poisson’s ratio as a damage parameter in the static tensile loading of simple crossply laminates. Compos. Sci. Technol. 1990, 38, 85–93. [Google Scholar] [CrossRef]
- Halpin, J.C.; Thomas, R.L. Ribbon Reinforcement of Composites. J. Compos. Mater. 1968, 2, 488–497. [Google Scholar] [CrossRef]
- Halpin, J.C.; Jerine, K.; Whitney, J.M. The Laminate Analogy for 2 and 3 Dimensional Composite Materials. J. Compos. Mater. 1971, 5, 36–49. [Google Scholar] [CrossRef]
- Affdl, J.; Kardos, J. The Halpin-Tsai equations: A review. Polym. Eng. Sci. 1976, 16, 344–352. [Google Scholar] [CrossRef]
- Hill, R. Elastic properties of reinforced solids: Some theoretical principles. J. Mech. Phys. Solids 1963, 11, 357–372. [Google Scholar] [CrossRef]
- Xu, X.; Ma, J.; Lim, C.W.; Chu, H. Dynamic local and global buckling of cylindrical shells under axial impact. Eng. Struct. 2009, 31, 1132–1140. [Google Scholar] [CrossRef]









| a | 0.1 | 0.2 | 0.3 | 0.4 | 0.5 | 0.6 | 0.7 | 0.8 | 0.9 | 1 | |
|---|---|---|---|---|---|---|---|---|---|---|---|
| kn | −2 | 921.69 | 230.42 | 102.41 | 57.61 | 36.87 | 25.60 | 18.81 | 14.4 | 11.38 | 9.22 |
| −1.5 | 582.62 | 145.66 | 64.74 | 36.41 | 23.30 | 16.18 | 11.89 | 9.10 | 7.19 | 5.83 | |
| −1 | 395.41 | 98.85 | 43.93 | 24.71 | 15.82 | 10.98 | 8.07 | 6.18 | 4.88 | 3.95 | |
| 0 | 307.70 | 76.93 | 34.19 | 19.23 | 12.31 | 8.55 | 6.28 | 4.81 | 3.80 | 3.08 | |
| 1 | 198.55 | 49.64 | 22.06 | 12.41 | 7.94 | 5.52 | 4.05 | 3.10 | 2.45 | 1.99 | |
| 1.5 | 153.49 | 38.37 | 17.05 | 9.59 | 6.14 | 4.26 | 3.13 | 2.40 | 1.89 | 1.53 | |
| 2 | 49.170 | 12.29 | 5.46 | 3.07 | 1.97 | 1.37 | 1.00 | 0.77 | 0.61 | 0.49 | |
| a | 0.1 | 0.2 | 0.3 | 0.4 | 0.5 | 0.6 | 0.7 | 0.8 | 0.9 | 1 | |
|---|---|---|---|---|---|---|---|---|---|---|---|
| ka | 2 | 553.83 | 138.46 | 61.540 | 34.61 | 22.15 | 15.38 | 11.30 | 8.65 | 6.84 | 5.54 |
| 4 | 1198.17 | 299.54 | 133.13 | 74.89 | 47.93 | 33.28 | 24.45 | 18.72 | 14.79 | 11.98 | |
| 6 | 2193.74 | 548.44 | 243.75 | 137.11 | 87.75 | 60.94 | 44.77 | 34.28 | 27.08 | 21.94 | |
| 8 | 3574.88 | 893.72 | 397.21 | 223.43 | 143.00 | 99.30 | 72.96 | 55.86 | 44.13 | 35.75 | |
| 10 | 5346.98 | 1336.75 | 594.11 | 334.19 | 213.88 | 148.53 | 109.12 | 83.55 | 66.01 | 53.47 | |
| a | 0.1 | 0.2 | 0.3 | 0.4 | 0.5 | 0.6 | 0.7 | 0.8 | 0.9 | 1 | |
|---|---|---|---|---|---|---|---|---|---|---|---|
| 2 | 85.38 | 21.35 | 9.49 | 5.34 | 3.42 | 2.37 | 1.74 | 1.33 | 1.05 | 0.85 | |
| 4 | 290.83 | 72.71 | 32.31 | 18.18 | 11.63 | 8.08 | 5.94 | 4.54 | 3.59 | 2.91 | |
| 8 | 1368.22 | 342.06 | 152.02 | 85.51 | 54.73 | 38.01 | 27.92 | 21.38 | 16.89 | 13.68 | |
| 12 | 3785.82 | 946.45 | 420.65 | 236.61 | 151.43 | 105.16 | 77.26 | 59.15 | 46.74 | 37.86 | |
| 16 | 8053.88 | 2013.47 | 894.88 | 503.37 | 322.16 | 223.72 | 164.36 | 125.84 | 99.43 | 80.54 | |
| a | 0.1 | 0.2 | 0.3 | 0.4 | 0.5 | 0.6 | 0.7 | 0.8 | 0.9 | 1 | |
|---|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 299.73 | 74.93 | 33.3 | 18.73 | 11.99 | 8.33 | 6.12 | 4.68 | 3.7 | 3 | |
| 1.75 | 441.04 | 110.26 | 49 | 27.56 | 17.64 | 12.25 | 9 | 6.89 | 5.44 | 4.41 | |
| 2.5 | 737.25 | 184.31 | 81.92 | 46.08 | 29.49 | 20.48 | 15.05 | 11.52 | 9.1 | 7.37 | |
| 3.25 | 1060.38 | 265.1 | 117.82 | 66.27 | 42.42 | 29.46 | 21.64 | 16.57 | 13.09 | 10.6 | |
| 4 | 1445.42 | 361.36 | 160.6 | 90.34 | 57.82 | 40.15 | 29.5 | 22.58 | 17.84 | 14.45 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Qi, D.; Sun, Q.; Zhang, S.; Wang, Y.; Zhou, X. Buckling Analysis of a Composite Honeycomb Reinforced Sandwich Embedded with Viscoelastic Damping Material. Appl. Sci. 2022, 12, 10366. https://doi.org/10.3390/app122010366
Qi D, Sun Q, Zhang S, Wang Y, Zhou X. Buckling Analysis of a Composite Honeycomb Reinforced Sandwich Embedded with Viscoelastic Damping Material. Applied Sciences. 2022; 12(20):10366. https://doi.org/10.3390/app122010366
Chicago/Turabian StyleQi, Dezhong, Qiang Sun, Sanqiang Zhang, Yuanfang Wang, and Xiaoqiang Zhou. 2022. "Buckling Analysis of a Composite Honeycomb Reinforced Sandwich Embedded with Viscoelastic Damping Material" Applied Sciences 12, no. 20: 10366. https://doi.org/10.3390/app122010366
APA StyleQi, D., Sun, Q., Zhang, S., Wang, Y., & Zhou, X. (2022). Buckling Analysis of a Composite Honeycomb Reinforced Sandwich Embedded with Viscoelastic Damping Material. Applied Sciences, 12(20), 10366. https://doi.org/10.3390/app122010366









