Flutter Reliability Analysis of Xiangshan Harbor Highway Cable-Stayed Bridges in Service
Abstract
:1. Introduction
2. The Flutter Analysis Framework for Flexible Bridge
2.1. Overview
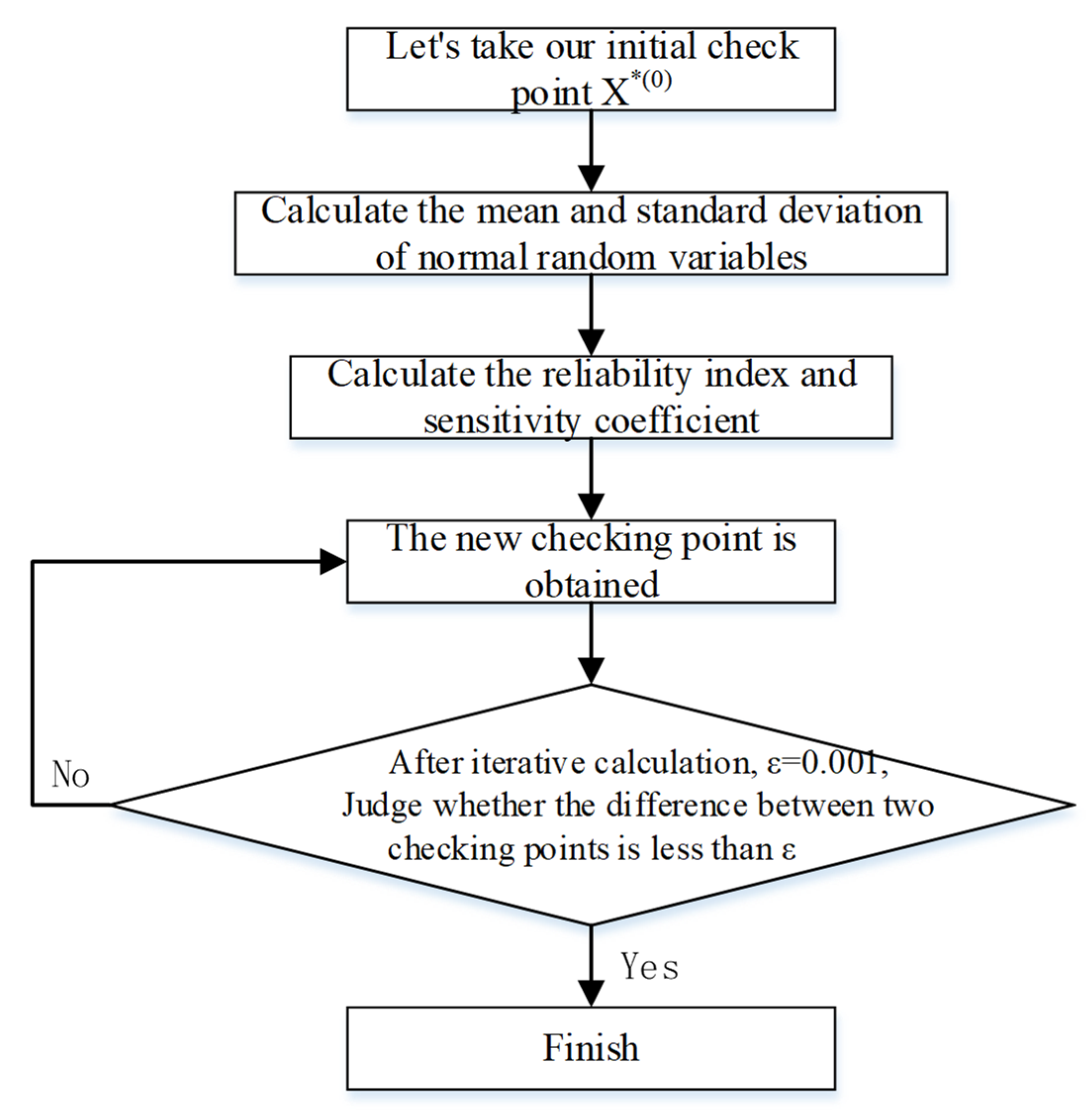
2.2. Reliability Analysis Method and Process
2.3. Determination of Functional Function and Parameters of Wind-Induced Flutter Reliability Analysis
2.3.1. Establishment of Flutter Reliability Functional Function
2.3.2. Wind Speed Probability Distribution Function at Bridge Site
2.3.3. Parameters Distribution in Functional Function
3. Case Analysis—Critical Wind Speed Solution and Analysis of the Influence Factors of Flutter for Xiangshan Harbor Bridge
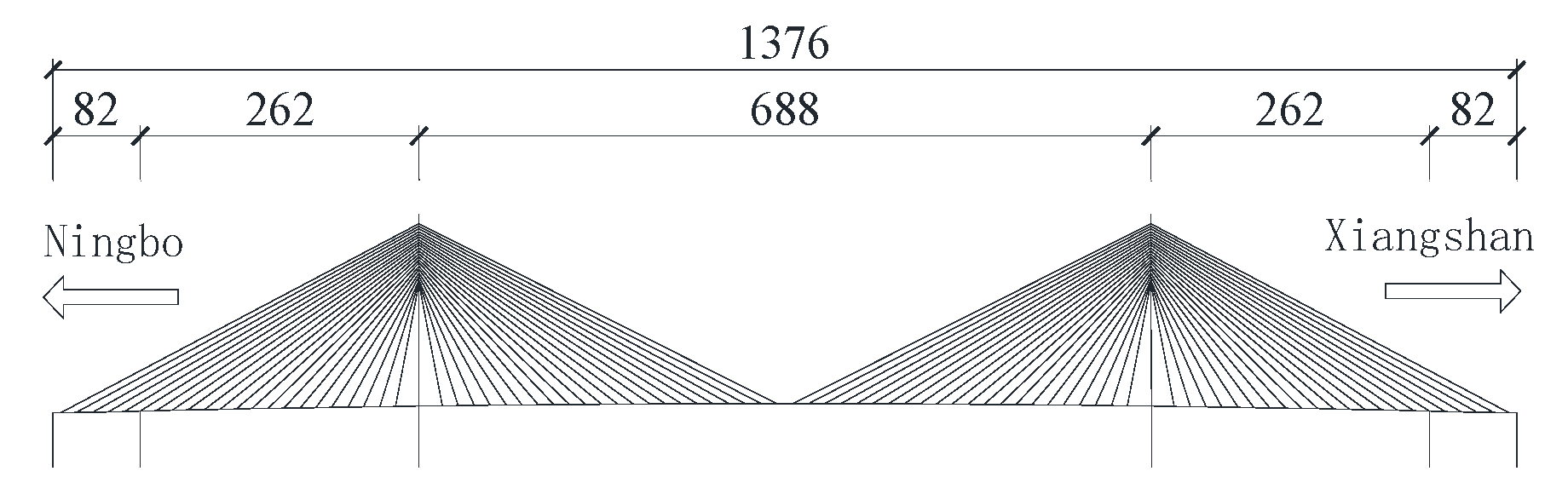
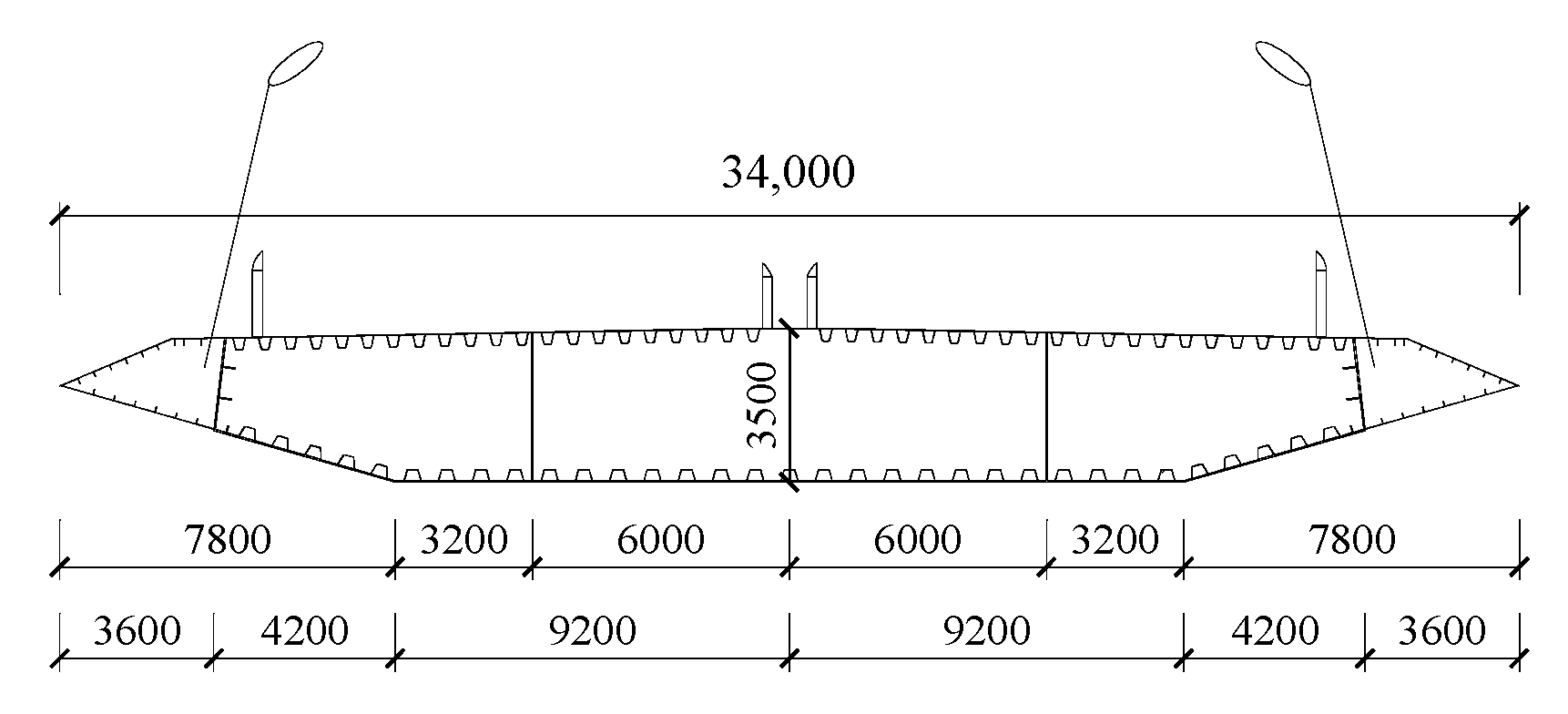
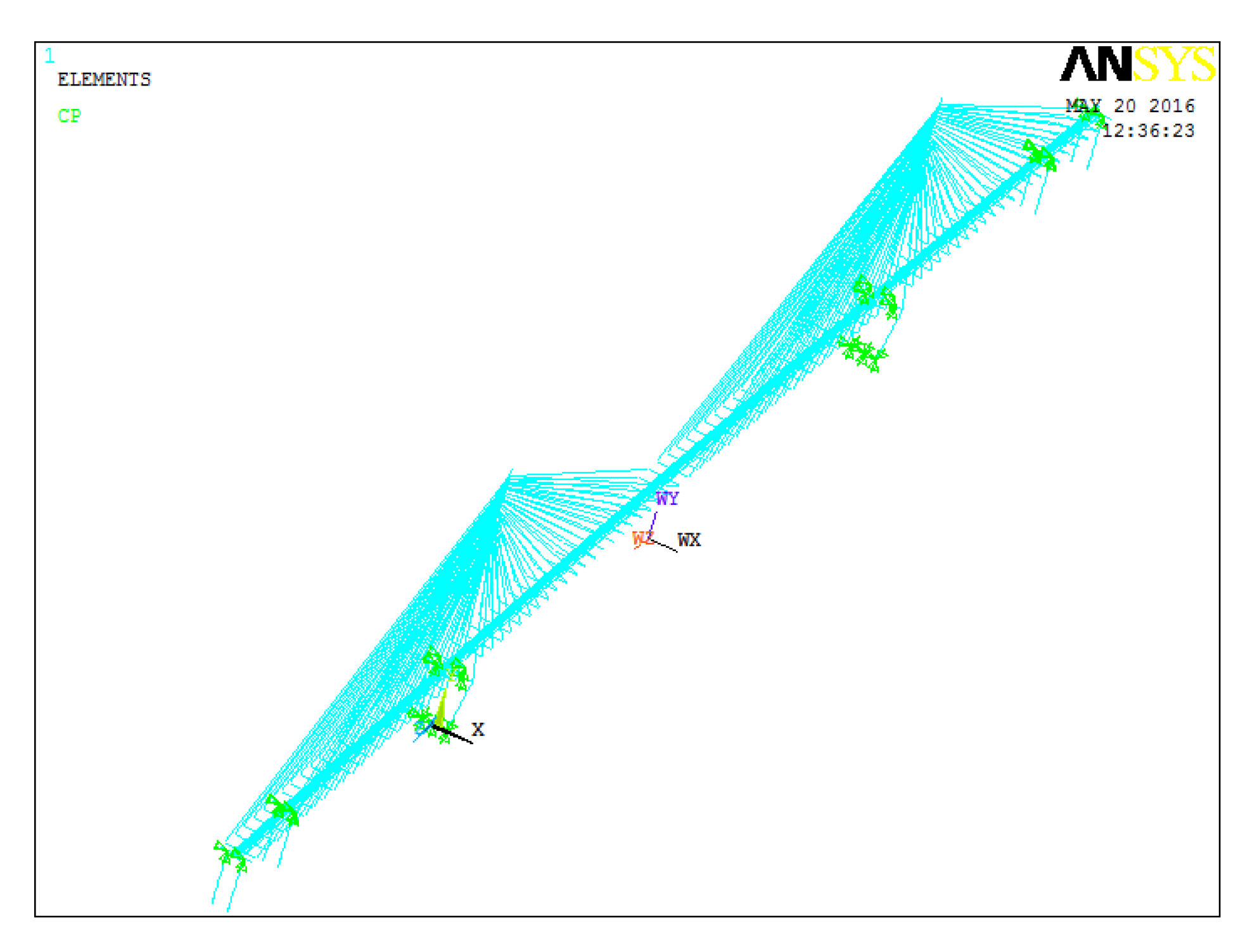
3.1. Establishment of Bridge Finite Element Model
3.2. Calculation of the Bridge Flutter Derivatives
3.3. Calculation of the Bridge Flutter Critical Wind Speed
- Create the corresponding target function.
- Read the flutter derivative data and the reduction wind speed data.
- Determine the initial value of these parameters to be fitted and set the search conditions d1,d2 > 0.
- Transform the initial value, then start the cycle iteration to find the optimal solution, and take the minimum residual value of the target function as the optimal result.
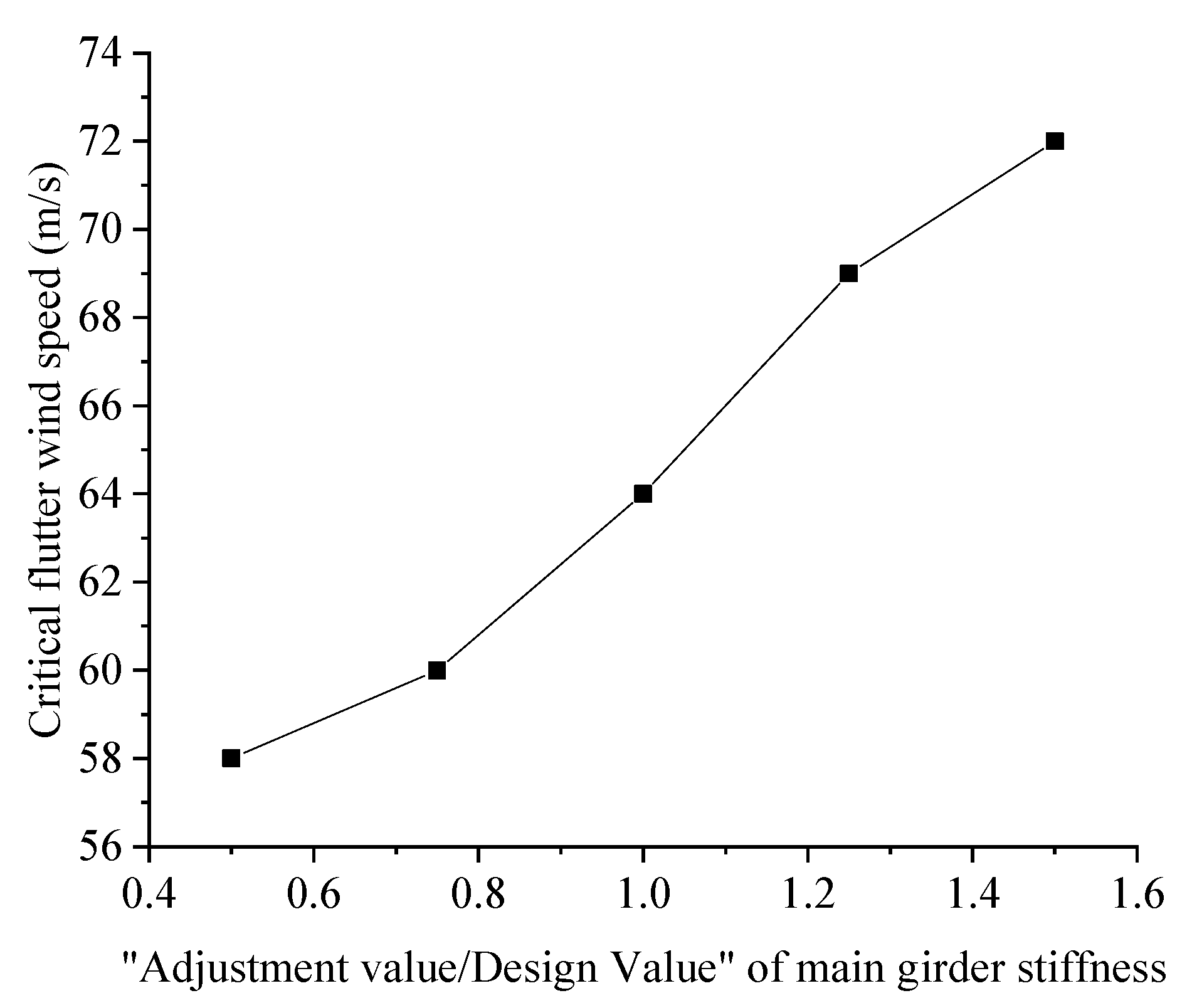
3.4. Influence of Main Beam Stiffness on Flutter Stability
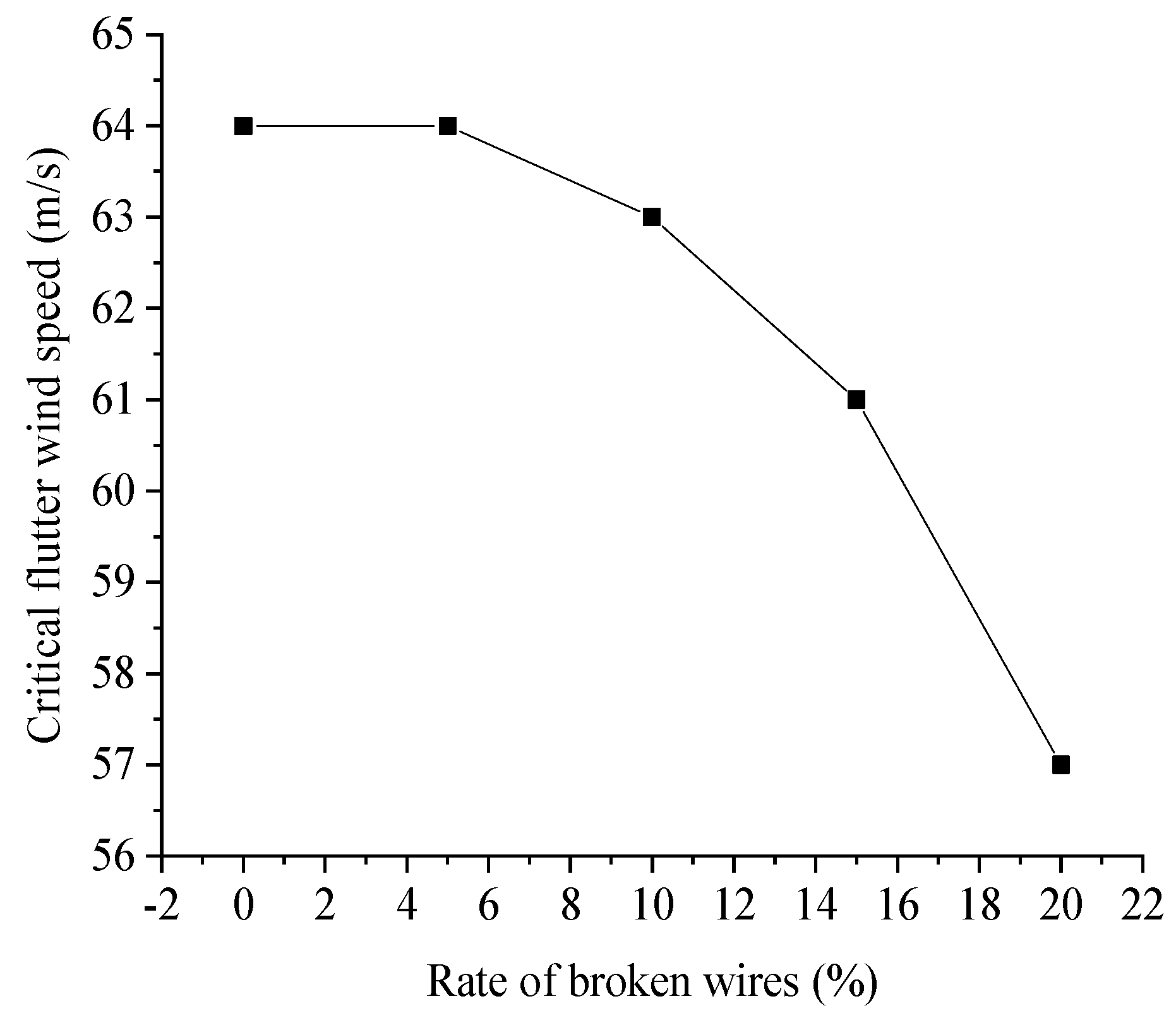
3.5. Influence of Wire Breaking Rate of Cables on Flutter Stability
3.6. Influence of Damping Ratio on Flutter Stability
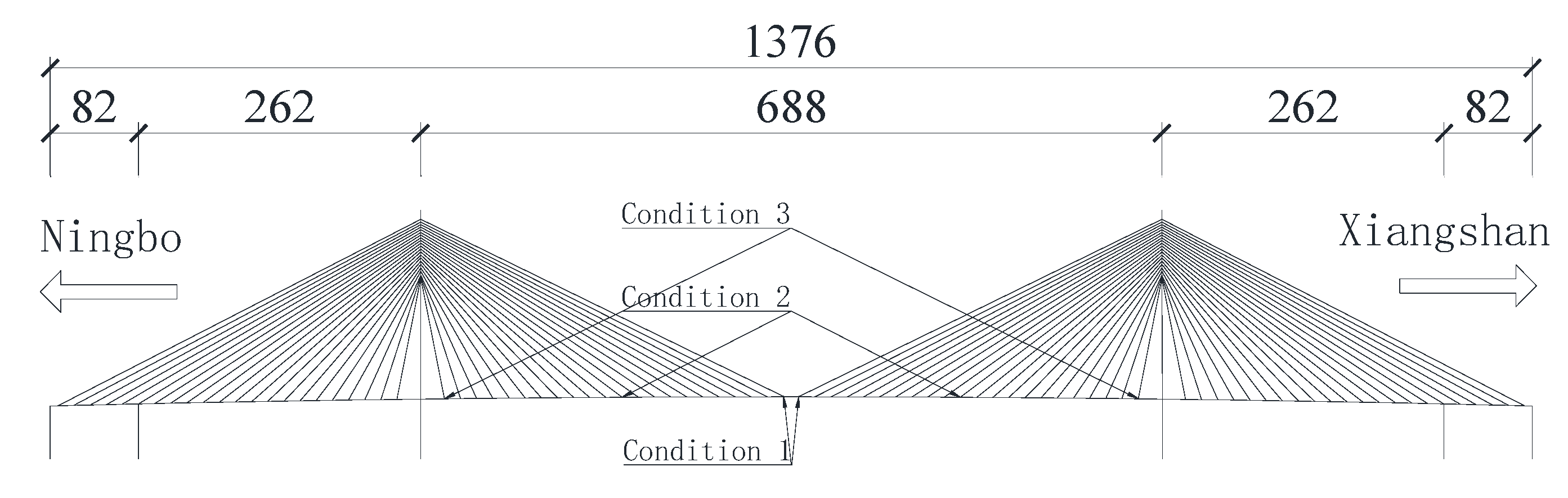
3.7. Influence of Cable Breakage Position on Flutter Stability
4. Flutter Reliability Analysis of Xiangshan Harbor Bridge
4.1. Flutter Reliability Analysis
4.2. Flutter Reliability under Different Conditions
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Abbas, T.; Kavrakov, I.; Morgenthal, G. Methods for flutter stability analysis of long-span bridges: A review. Proc. Inst. Civ. Eng. Bridge Eng. 2017, 170, 271–310. [Google Scholar] [CrossRef]
- Miyata, T.; Yamada, H. Coupled flutter estimate of a suspension bridge. J. Wind. Eng. Ind. Aerodyn. 1990, 33, 341–348. [Google Scholar] [CrossRef]
- Tanaka, H.; Yamamura, N.; Shiraishi, N. Multi-mode flutter analysis and two & three-dimensional model tests on bridges with non-analogous modal shapes. J. Struct. Eng. Earthq. Eng. 1993, 10, 71–82. [Google Scholar]
- Chen, X.; Matsumoto, M.; Kareem, A. Time domain flutter and buffeting response analysis of bridges. J. Eng. Mech. 2000, 126, 7–16. [Google Scholar] [CrossRef]
- Ge, Y.; Tanaka, H. Aerodynamic flutter analysis of cable-supported bridges by multi-mode and full-mode approaches. J. Wind. Eng. Ind. Aerodyn. 2000, 86, 123–153. [Google Scholar] [CrossRef]
- Yang, Y.; Ge, Y.; Xiang, H. Investigation on flutter mechanism of long-span bridges with 2d–3DOF method. Wind. Struct. 2007, 10, 421–435. [Google Scholar] [CrossRef]
- Hua, X.G.; Chen, Z.Q.; Ni, Y.Q.; Ko, J.M. Flutter analysis of long-span bridges using ANSYS. Wind. Struct. 2007, 10, 61–82. [Google Scholar] [CrossRef]
- Sarkar, P.P.; Caracoglia, L.; Haan, F.L., Jr.; Sato, H.; Murakoshi, J. Comparative and sensitivity study of flutter derivatives of selected bridge deck sections, Part 1: Analysis of inter-laboratory experimental data. Eng. Struct. 2009, 31, 158–169. [Google Scholar] [CrossRef]
- Xu, F. System decoupling approach for 3-dof bridge flutter analysis. J. Struct. Eng. 2015, 141, 04014168. [Google Scholar] [CrossRef]
- Cheng, J.; Cai, C.S.; Xiao, R.C.; Chen, S.R. Flutter reliability analysis of suspension bridges. J. Wind. Eng. Ind. Aerodyn. 2005, 93, 757–775. [Google Scholar] [CrossRef]
- Cheng, J.; Xiao, R. Safety factor evaluation of main cables of long-span suspension bridges based on inverse reliability method. China J. Highw. Transp. 2007, 20, 58–61. [Google Scholar]
- Seo, D.-W.; Caracoglia, L. Estimation of torsional-flutter probability in flexible bridges considering randomness in flutter derivatives. Eng. Struct. 2011, 33, 2284–2296. [Google Scholar] [CrossRef]
- Seo, D.-W.; Caracoglia, L. Exploring the impact of “climate change” on lifetime replacement costs for long-span bridges prone to torsional flutter. J. Wind. Eng. Ind. Aerodyn. 2015, 140, 1–9. [Google Scholar] [CrossRef]
- Mannini, C.; Bartoli, G. Aerodynamic uncertainty propagation in bridge flutter analysis. Struct. Saf. 2015, 52, 29–39. [Google Scholar] [CrossRef]
- Rizzo, F.; Caracoglia, L. Examination of experimental errors in Scanlan derivatives of a closed-box bridge deck. Wind. Struct. 2018, 26, 231–251. [Google Scholar]
- Rizzo, F.; Caracoglia, L.; Montelpare, S. Predicting the flutter speed of a pedestrian suspension bridge through examination of laboratory experimental errors. Eng. Struct. 2018, 172, 589–613. [Google Scholar] [CrossRef]
- Ge, Y. Study on Wind Vibration Reliability Theory and Application of Bridge Structure; Tongji University: Shanghai, China, 1997. [Google Scholar]
- Ge, Y.; Xiang, H. Random finite element analysis of bridge flutter. China Civ. Eng. J. 1999, 32, 27–32. [Google Scholar]
- Ge, Y.; Xiang, H.; Tanaka, H. Application of a reliability analysis model to bridge flutter under extreme winds. J. Wind. Eng. Ind. Aerodyn. 2000, 86, 155–167. [Google Scholar] [CrossRef]
- Zhou, Z.; Ge, Y.; Du, B. Stochastic finite element method for probabilistic evaluation of bridge flutter. Eng. Mech. 2007, 24, 98–104. [Google Scholar]
- Cheng, J.; Xiao, R. Improved response surface method for flutter reliability analysis of long-span suspension bridges. China Civ. Eng. J. 2006, 39, 69–73. [Google Scholar]
- Canor, T.; Caracoglia, L.; Denol, V. Application of random eigenvalue analysis to assess bridge flutter probability. J. Wind. Eng. Ind. Aerodyn. 2015, 140, 79–86. [Google Scholar] [CrossRef]
- Kusano, I.; Baldomir, A.; Jurado, J.Á.; Hernández, S. Reliability based design optimization of long-span bridges considering flutter. J. Wind. Eng. Ind. Aerodyn. 2014, 135, 149–162. [Google Scholar] [CrossRef]
- Kusano, I.; Baldomir, A.; Jurado, J.Á.; Hernández, S. The importance of correlation among flutter derivatives for the reliability based optimum design of suspension bridges. Eng. Struct. 2018, 173, 416–428. [Google Scholar] [CrossRef]
- Baldomir, A.; Kusano, I.; Hernandez, S.; Jurado, J.A. A reliability study for the messina bridge with respect to flutter phenomena considering uncertainties in experimental and numerical data. Comput. Struct. 2013, 128, 91–100. [Google Scholar] [CrossRef]
- Argentini, T.; Pagani, A.; Rocchi, D.; Zasso, A. Monte Carlo analysis of total damping and flutter speed of a long span bridge: Effects of structural and aerodynamic uncertainties. J. Wind. Eng. Ind. Aerodyn. 2014, 128, 90–104. [Google Scholar] [CrossRef]
- Wu, X. Flutter stability evaluation of long-span suspension bridges based on finite element reliability. J. Xi’an Univ. Technol. 2020, 36, 100–106. [Google Scholar]
- Ma, T. Parameter analysis of flutter stability based on segment model of bridge. Struct. Eng. 2019, 35, 69–75. [Google Scholar]
- Fang, G.; Cao, J.; Yang, Y.; Zhao, L.; Cao, S.; Ge, Y. Experimental uncertainty quantification of flutter derivatives for a pk section girder and its application on probabilistic flutter analysis. J. Bridge Eng. 2020, 25, 04020034. [Google Scholar] [CrossRef]
- Ji, X.; Huang, G.; Zhao, Y.G. Probabilistic flutter analysis of bridge considering aerodynamic and structural parameter uncertainties. J. Wind. Eng. Ind. Aerodyn. 2020, 201, 104168. [Google Scholar] [CrossRef]
- Ji, X.; Zhao, Y.G.; Lu, Z.H. Uncertainty analysis of bridge flutter considering dependence and sampling error in flutter derivative measurements. Eng. Struct. 2021, 246, 113051. [Google Scholar] [CrossRef]
- Wang, B.; Tang, G.; Tang, J.; Wang, W. State-of-the-art review of reliability in bridge engineering in 2020. J. Civ. Environ. Eng. 2021, 43, 222–227. [Google Scholar]
- Xu, Z.; Ma, L. Structural Dynamics; Science Press: Beijing, China, 2007. [Google Scholar]
- Scanlan, R.H.; Tomko, J.J. Airfoil and bridge deck flutter derivatives. J. Soil Mech. Found. Div. 1971, 97, 1717–1737. [Google Scholar] [CrossRef]
- Qing, Q. Time-Domain Analysis and Program Implementation of Bridge Flutter; Hunan University: Changsha, China, 2011. (In Chinese) [Google Scholar]
- Li, Q.C.; Lin, Y.K. New stochastic theory for bridge stability in turbulent flow. II. J. Eng. Mech. 1995, 121, 102–116. [Google Scholar] [CrossRef]
- Lin, Y.K.; Yang, J.N. Multimode bridge response to wind excitations. J. Eng. Mech. 1983, 109, 586–603. [Google Scholar] [CrossRef]
- Bao, J.; Hu, X.; Xing, M.; Zhao, S. Comparative study of latin hypercube and traditional selective sampling in reliability analysis. J. North China Inst. Sci. Technol. 2021, 18, 81–84. [Google Scholar]
- JTG/T 3360-01-2018; Wind-resistant Design Specification for Highway Bridges. People’s Communications Press: Beijing, China, 2018.
- Xu, Y. Wind Effects on Cable-Supported Bridges; John Wiley & Sons: New York, NY, USA, 2013. [Google Scholar]
- Tang, J.; Xu, J. Load Test Report of the Main Bridge of Xiangshan Harbor Bridge; Zhejiang Jiaoke Engineering Inspection Co., Ltd.: Hangzhou, China, 2012; Volume 24–27, pp. 94–99. [Google Scholar]
- Lan, C. Theoretical study on fatigue performance of parallel steel cable. J. Shenyang Jianzhu Univ. 2009, 25, 56–60. [Google Scholar]
- GB 50153-2008; Unified Standard for Reliability Design of Engineering Structures. China Architecture Press: Beijing, China, 2008.


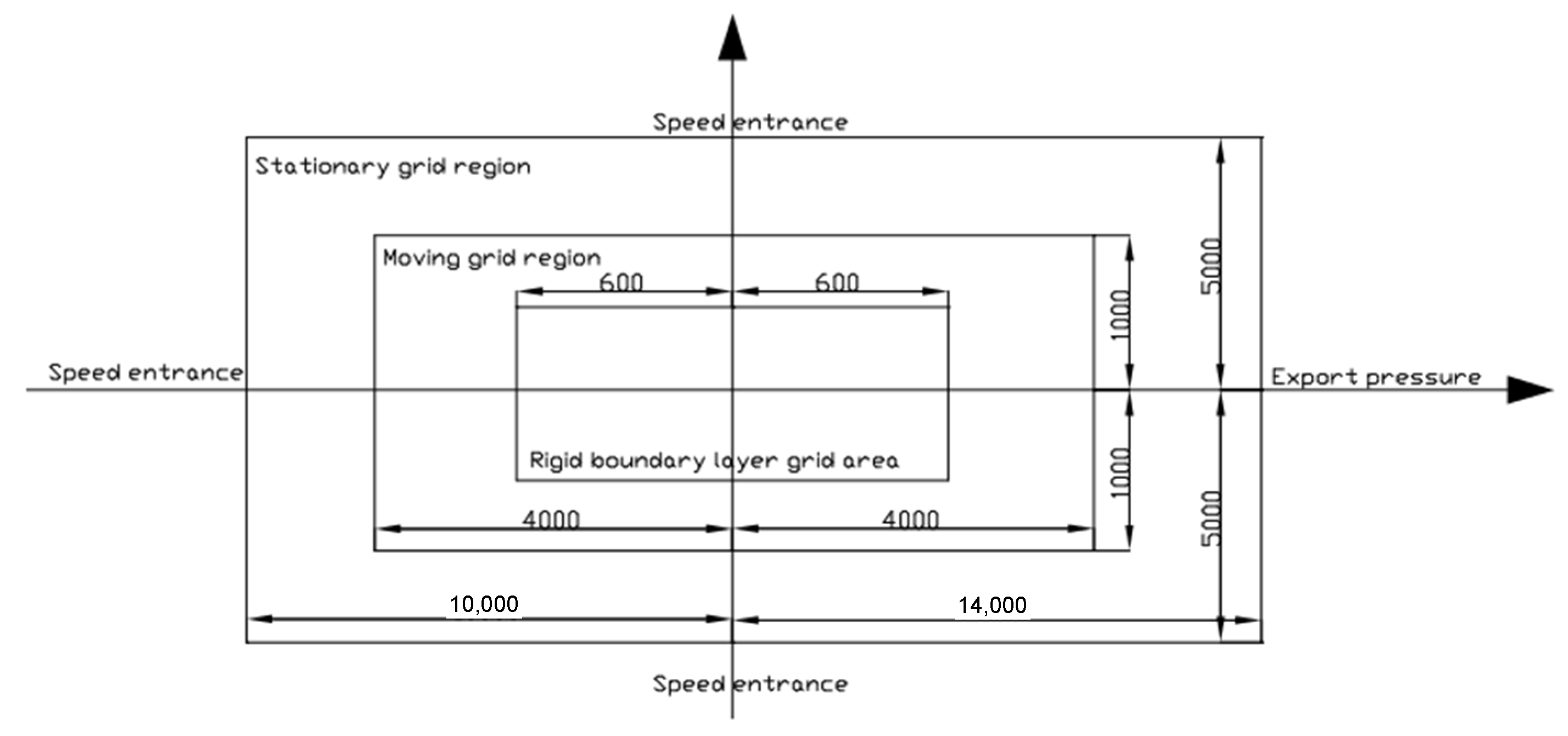
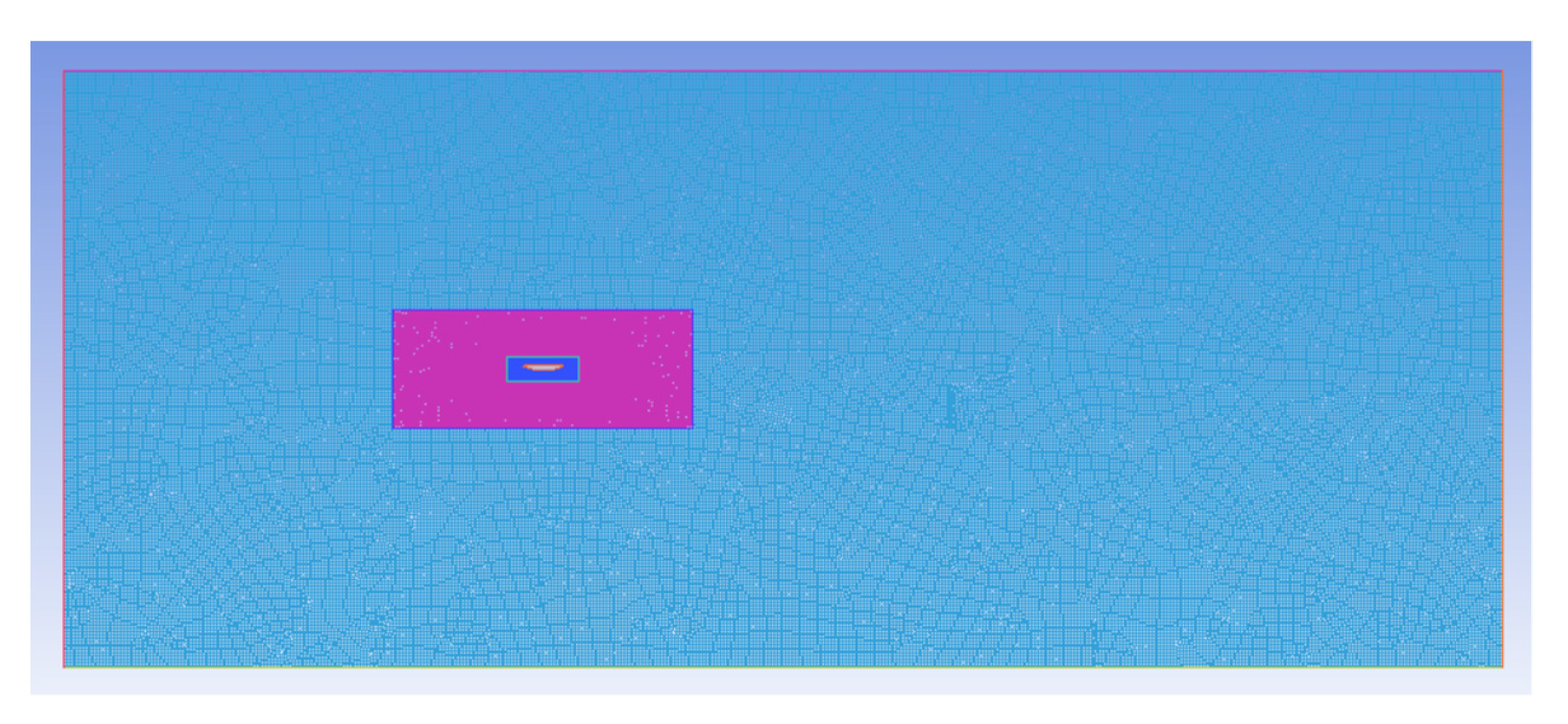

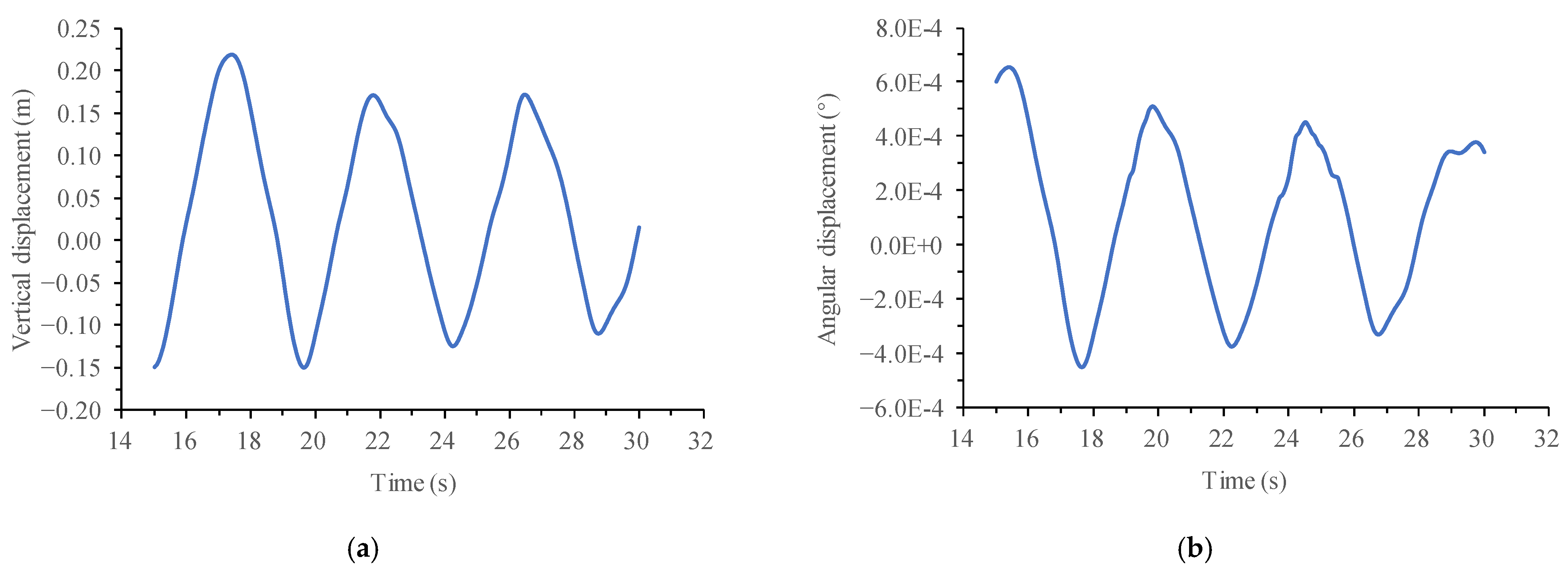
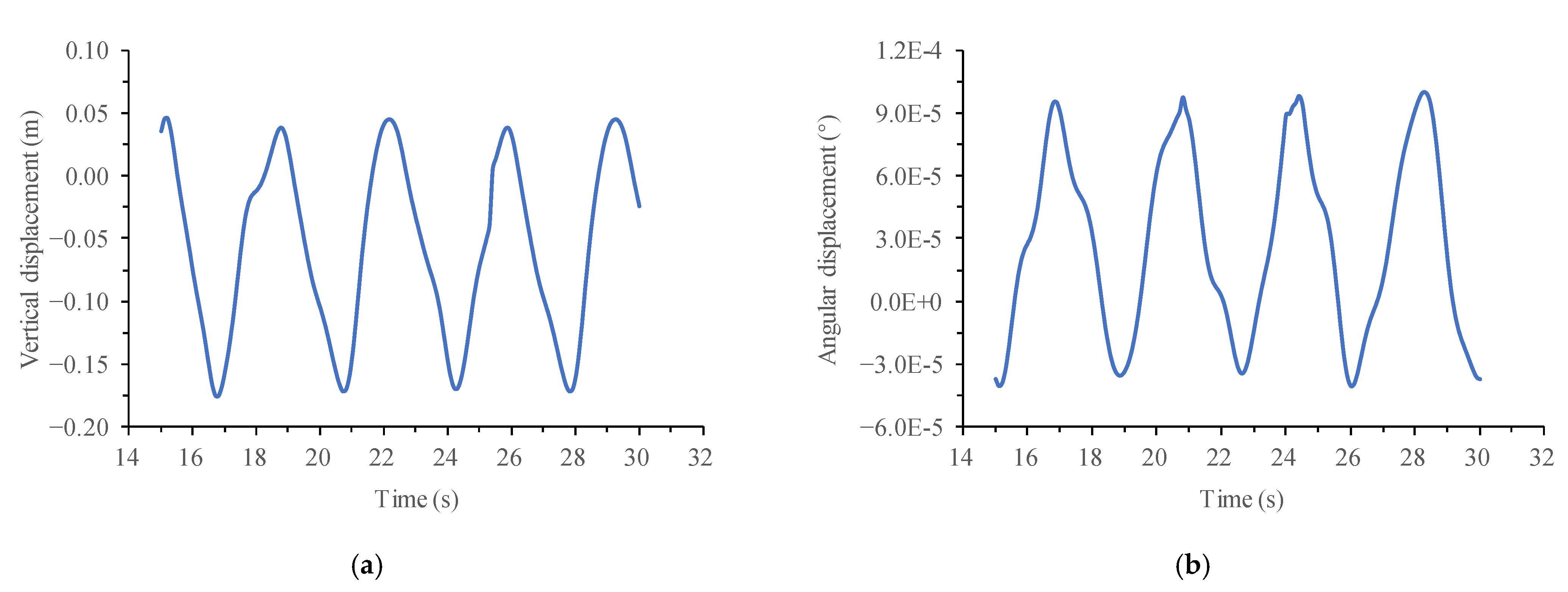


| Year/Month | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 1987 | 12.7 | 13.0 | 13.0 | 9.3 | 9.3 | 15.7 | 14.3 | 10.3 | 9.0 | 11.3 | 12.3 | 12.7 |
| 1988 | 13.0 | 11.3 | 9.0 | 10.7 | 8.3 | 10.0 | 17.3 | 9.7 | 8.7 | 10.3 | 9.7 | 13.0 |
| 1989 | 11.0 | 12.0 | 10.3 | 8.3 | 8.0 | 13.3 | 13.0 | 110.0 | 10.3 | 10.7 | 8.7 | 11.0 |
| 1990 | 9.3 | 9.7 | 12.0 | 9.7 | 16.0 | 15.0 | 17.3 | 15.0 | 10.3 | 12.7 | 10.7 | 9.3 |
| 1991 | 11.0 | 11.0 | 8.7 | 9.7 | 7.7 | 10.7 | 9.7 | 9.3 | 9.3 | 8.0 | 11.3 | 11.0 |
| 1992 | 11.3 | 12.0 | 16.0 | 11.3 | 9.0 | 10.1 | 12.9 | 14.3 | 9.0 | 10.4 | 10.0 | 11.3 |
| 1993 | 10.3 | 9.5 | 12.5 | 8.4 | 14.3 | 7.6 | 13.0 | 9.1 | 10.0 | 11.0 | 11.8 | 10.3 |
| 1994 | 11.4 | 9.2 | 11.7 | 9.7 | 10.7 | 11.6 | 13.6 | 8.3 | 15.4 | 7.8 | 9.0 | 11.4 |
| 1995 | 10.7 | 10.0 | 10.4 | 9.3 | 8.9 | 10.7 | 14.1 | 8.7 | 8.3 | 14.1 | 9.1 | 10.7 |
| 1996 | 9.0 | 11.9 | 11.6 | 10.0 | 7.8 | 11.0 | 13.0 | 9.9 | 7.7 | 11.9 | 10.4 | 9.0 |
| 1997 | 10.8 | 9.1 | 10.2 | 10.5 | 9.9 | 11.3 | 18.7 | 8.9 | 8.1 | 10.6 | 8.5 | 10.8 |
| 1998 | 11.3 | 14.8 | 10.0 | 8.8 | 7.7 | 8.7 | 10.0 | 17.3 | 11.2 | 7.8 | 9.9 | 11.3 |
| 1999 | 10.2 | 11.1 | 9.2 | 9.0 | 9.4 | 10.5 | 7.5 | 9.6 | 9.7 | 10.3 | 9.6 | 10.2 |
| 2000 | 9.2 | 11.9 | 11.9 | 10.8 | 8.1 | 13.6 | 15.9 | 14.1 | 8.5 | 10.6 | 9.5 | 9.2 |
| 2001 | 11.5 | 10.0 | 9.1 | 9.3 | 9.5 | 9.2 | 11.6 | 10.6 | 10.1 | 11.1 | 10.2 | 11.5 |
| 2002 | 8.1 | 10.6 | 11.9 | 8.1 | 8.4 | 15.5 | 10.6 | 9.5 | 9.6 | 9.4 | 8.8 | 8.1 |
| 2003 | 10.1 | 10.8 | 11.2 | 7.8 | 6.8 | 9.4 | 8.1 | 8.4 | 8.8 | 9.0 | 9.4 | 10.1 |
| 2004 | 8.8 | 11.1 | 10.2 | 8.1 | 8.9 | 11.9 | 11.7 | 10.6 | 8.6 | 9.0 | 11.5 | 8.8 |
| 2005 | 10.4 | 11.6 | 9.2 | 8.1 | 8.1 | 10.4 | 15.7 | 19.7 | 9.2 | 7.1 | 10.5 | 10.4 |
| 2006 | 8.4 | 10.9 | 10.7 | 13.7 | 10.3 | 13.4 | 7.8 | 9.4 | 7.0 | 10.4 | 9.8 | 8.4 |
| 2007 | 8.7 | 9.3 | 11.9 | 11.3 | 7.3 | 11.5 | 9.7 | 13.3 | 10.0 | 8.0 | 8.9 | 8.7 |
| 2008 | 9.0 | 8.2 | 12.9 | 9.2 | 8.3 | 12.6 | 11.1 | 10.1 | 5.7 | 7.1 | 9.8 | 9.0 |
| 2009 | 8.9 | 8.9 | 10.9 | 7.4 | 8.8 | 8.9 | 9.5 | 10.1 | 6.2 | 8.7 | 7.6 | 8.9 |
| 2010 | 8.5 | 9.5 | 9.7 | 7.6 | 6.3 | 8.7 | 8.1 | 8.3 | 8.9 | 6.4 | 10.6 | 8.5 |
| 2011 | 6.1 | 7.2 | 7.6 | 8.3 | 9.5 | 7.3 | 13.5 | 9.0 | 7.1 | 8.3 | 8.0 | 6.1 |
| 2012 | 9.3 | 8.2 | 11.7 | 8.0 | 6.6 | 6.9 | 14.9 | 9.4 | 8.6 | 9.5 | 10.2 | 9.3 |
| 2013 | 7.8 | 8.4 | 11.4 | 8.2 | 10.9 | 9.9 | 11.1 | 8.0 | 8.4 | 7.8 | 7.9 | 7.8 |
| 2014 | 6.9 | 7.9 | 7.5 | 7.4 | 5.8 | 8.7 | 7.7 | 11.9 | 8.7 | 6.1 | 9.9 | 6.9 |
| 2015 | 7.8 | 8.1 | 8.6 | 6.8 | 6.7 | 12.5 | 9.5 | 6.5 | 6.2 | 7.2 | 6.7 | 7.8 |
| 2016 | 7.7 | 8.7 | 8.7 | 9.3 | 10.5 | 6.2 | 6.2 | 8.0 | 8.6 | 10.3 | 7.8 | 7.7 |
| Number | Calculated Frequency/Hz | Measured Frequency/Hz | Error/% | Mode Shape |
|---|---|---|---|---|
| 1 | 0.129 | —— | —— | Longitudinal drift of main girder |
| 2 | 0.206 | 0.213 | 2.82 | First-order symmetrical lateral bending of main girder |
| 3 | 0.249 | 0.250 | 0.40 | First-order symmetrical vertical bending of main girder |
| 4 | 0.316 | 0.300 | 5.33 | First-order antisymmetric vertical bending of main girder |
| 5 | 0.453 | 0.438 | 3.20 | First-order antisymmetric side bending of main tower |
| 6 | 0.457 | —— | —— | First-order symmetrical side bending of main tower |
| 7 | 0.482 | —— | —— | Second-order symmetrical vertical bending of main girder |
| 8 | 0.570 | 0.525 | 9.52 | First-order antisymmetric lateral bend of main girder |
| 9 | 0.578 | 0.600 | 3.17 | Second-order antisymmetric vertical bending of main girder |
| 10 | 0.628 | —— | —— | Third-order symmetrical vertical bending of the main girder |
| Wind Speed (m/s) | Reduction Wind Speed | H1* | H2* | H3* | H4* | A1* | A2* | A3* | A4* |
|---|---|---|---|---|---|---|---|---|---|
| 2 | 1.47 | −0.31 | −0.12 | −0.05 | 0.61 | 0.07 | −0.0019 | 0.033 | −0.010 |
| 4 | 2.94 | −0.64 | −0.16 | −0.14 | 0.52 | 0.12 | −0.0078 | 0.073 | −0.002 |
| 6 | 4.41 | −0.99 | −0.30 | −0.48 | 0.43 | 0.24 | −0.021 | 0.177 | 0.008 |
| 8 | 5.88 | −1.39 | −0.36 | −0.62 | 0.32 | 0.38 | −0.040 | 0.303 | 0.011 |
| 10 | 7.35 | −1.82 | −0.41 | −1.52 | 0.30 | 0.53 | −0.089 | 0.473 | 0.012 |
| 12 | 8.82 | −1.92 | −0.44 | −2.27 | 0.24 | 0.65 | −0.12 | 0.690 | 0.044 |
| 14 | 10.29 | −2.17 | −0.47 | −3.17 | 0.18 | 0.76 | −0.16 | 0.960 | 0.051 |
| 16 | 11.76 | −2.53 | −0.52 | −4.21 | 0.07 | 0.87 | −0.21 | 1.275 | 0.061 |
| 18 | 13.24 | −2.86 | −0.56 | −5.38 | −0.03 | 0.91 | −0.27 | 1.642 | 0.067 |
| 20 | 14.71 | −3.16 | −0.63 | −6.70 | −0.12 | 1.08 | −0.33 | 2.047 | 0.079 |
| Self-Excited Force | A1 | A2 | A3 | A4 | d1 | d2 |
|---|---|---|---|---|---|---|
| Lseh | −0.1141 | −17.348 | 479.926 | −0.0286 | 30.026 | 0.4648 |
| Lseα | −1.3287 | −1.0653 | 1.2277 | 0.1474 | 1.5640 | 1.9191 |
| Mseh | 0.0144 | 0.4450 | 0.0813 | −0.0856 | 2.1176 | 2.7743 |
| Mseα | 0.4266 | −0.0361 | −0.0942 | 0.0183 | 0.3744 | 3.5885 |
| Cable Breakage Position | Condition 1 | Condition 2 | Condition 3 | Initial State |
|---|---|---|---|---|
| Critical flutter wind speed (m/s) | 53 | 60 | 63 | 64 |
| Random Parameter | Distribution Type | Mean Value | Variation Coefficient |
|---|---|---|---|
| Stiffness of main girder | Normal distribution | 1.00 | 0.05 |
| Wire breaking rate | Normal distribution | 1.00 | 0.05 |
| Damping ratio | Normal distribution | 1.00 | 0.40 |
| Cable breakage location | Normal distribution | 1.00 | 0.50 |
| Number | Stiffness of Main Girder | Wire Breaking Rate | Damping Ratio | Cable Breakage Location |
|---|---|---|---|---|
| 1 | 0.923709 | 0.966521 | 0.397121 | 1.285030 |
| 2 | 0.978303 | 0.973576 | 1.125546 | 0.552123 |
| 3 | 0.943786 | 1.005954 | 0.977282 | −0.024684 |
| 4 | 0.949115 | 0.993655 | 0.858008 | 1.106805 |
| 5 | 1.046271 | 1.012304 | 0.756266 | 1.241252 |
| 6 | 1.041689 | 0.987206 | 1.100707 | 1.716483 |
| 7 | 0.999166 | 0.923709 | 0.652328 | 1.001545 |
| 8 | 1.095844 | 0.978303 | 1.823723 | 0.635685 |
| 9 | 1.024584 | 1.053701 | 1.298578 | 1.578594 |
| 10 | 0.926544 | 1.000593 | 1.161744 | 0.715661 |
| 11 | 0.966521 | 1.024584 | 0.511513 | 1.168349 |
| 12 | 1.067997 | 1.084866 | 1.240521 | 1.631187 |
| 13 | 0.987206 | 1.021351 | 1.407390 | 0.256723 |
| 14 | 0.979184 | 0.926544 | 0.690548 | 0.850914 |
| 15 | 1.005954 | 0.959554 | 1.035235 | 1.382220 |
| 16 | 1.000593 | 1.041689 | 0.738072 | 2.011800 |
| 17 | 1.053701 | 1.095844 | 1.355190 | 0.861031 |
| 18 | 1.084866 | 1.035579 | 0.585582 | 1.808437 |
| 19 | 1.012304 | 1.016118 | 0.437287 | 1.027163 |
| 20 | 1.035579 | 0.943786 | 1.005405 | 1.090421 |
| 21 | 1.026391 | 0.903784 | 1.282456 | 2.022600 |
| 22 | 0.959554 | 1.067997 | 1.497616 | 0.796653 |
| 23 | 0.903784 | 1.061711 | 0.864946 | 0.224175 |
| 24 | 1.016118 | 0.991424 | 0.161737 | 1.345108 |
| 25 | 1.021351 | 1.026391 | 0.827167 | 0.454660 |
| 26 | 0.991424 | 0.979184 | 0.901138 | 1.402973 |
| 27 | 0.993655 | 0.949115 | 0.935695 | 0.953122 |
| 28 | 0.952276 | 1.046271 | 1.665293 | 1.508447 |
| 29 | 0.973576 | 0.999166 | 1.180937 | 1.880601 |
| 30 | 1.0617110 | 0.952276 | 1.541311 | 0.515844 |
| Number | Stiffness of Main Girder | Wire Breaking Rate | Damping Ratio | Cable Breakage Location |
|---|---|---|---|---|
| 1 | 59 | 57 | 53 | 59 |
| 2 | 60 | 57 | 59 | 60 |
| 3 | 59 | 57 | 58 | 64 |
| 4 | 59 | 57 | 57 | 55 |
| 5 | 61 | 57 | 56 | 59 |
| 6 | 61 | 57 | 59 | 63 |
| 7 | 61 | 58 | 55 | 53 |
| 8 | 61 | 57 | 65 | 60 |
| 9 | 60 | 56 | 61 | 62 |
| 10 | 60 | 57 | 59 | 59 |
| 11 | 60 | 57 | 54 | 57 |
| 12 | 61 | 56 | 60 | 62 |
| 13 | 60 | 57 | 62 | 64 |
| 14 | 60 | 58 | 55 | 57 |
| 15 | 60 | 57 | 58 | 60 |
| 16 | 60 | 56 | 56 | 64 |
| 17 | 60 | 56 | 61 | 56 |
| 18 | 61 | 56 | 54 | 64 |
| 19 | 60 | 57 | 53 | 53 |
| 20 | 60 | 58 | 58 | 54 |
| 21 | 60 | 58 | 61 | 64 |
| 22 | 59 | 56 | 62 | 58 |
| 23 | 59 | 56 | 57 | 64 |
| 24 | 60 | 57 | 50 | 60 |
| 25 | 60 | 56 | 56 | 62 |
| 26 | 60 | 57 | 57 | 60 |
| 27 | 60 | 58 | 57 | 53 |
| 28 | 60 | 56 | 64 | 61 |
| 29 | 60 | 57 | 60 | 64 |
| 30 | 61 | 57 | 63 | 60 |
| Flutter critical Wind Speed (m/s) | Stiffness of Main Girder | Wire Breaking Rate | Damping Ratio | Cable Breakage Location |
|---|---|---|---|---|
| Mean value | 60 | 57 | 58 | 59 |
| Standard deviation | 0.63 | 0.67 | 3.46 | 3.52 |
| Item | Stiffness of Main Girder | Wire Breaking Rate | Damping Ratio | Cable Breakage Location |
|---|---|---|---|---|
| Reliability index | 2.35 | 2.24 | 2.26 | 2.31 |
| Cw | 0.9493 | 0.9525 | 0.9524 | 0.9507 |
| Us | 60.0317 | 56.827 | 56.9689 | 58.5988 |
| Gv | 1.454 | 1.4503 | 1.4504 | 1.4523 |
| Ub | 39.1955 | 37.3223 | 37.4057 | 38.3604 |
| Stiffness of main girder | 0.5 | 0.75 | 1.0 | 1.25 | 1.5 |
| Reliability index | 2.28 | 2.35 | 2.48 | 2.64 | 2.73 |
| Failure probability | 0.0144 | 0.0095 | 0.0066 | 0.0042 | 0.0032 |
| Wire breaking rate | Initial state of bridge (0%) | 5% | 10% | 15% | 20% |
| Reliability index | 2.48 | 2.47 | 2.45 | 2.38 | 2.24 |
| Failure probability | 0.006 | 0.0066 | 0.0072 | 0.0087 | 0.0125 |
| Damping ratio | 0% | 0.3% | 0.5% | 1% |
| Reliability index | 1.96 | 2.26 | 2.46 | 2.73 |
| Failure probability | 0.0249 | 0.0120 | 0.0070 | 0.0032 |
| Broken cable position | Random location | Bridge tower | Main span 1/4 | Bridge span midpoint |
| Reliability index | 2.31 | 2.42 | 2.32 | 2.08 |
| Failure probability | 0.0103 | 0.0077 | 0.0101 | 0.0189 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, T.; Cui, X.; Zhang, X.; Li, H.; Zou, Y. Flutter Reliability Analysis of Xiangshan Harbor Highway Cable-Stayed Bridges in Service. Appl. Sci. 2022, 12, 8301. https://doi.org/10.3390/app12168301
Zhang T, Cui X, Zhang X, Li H, Zou Y. Flutter Reliability Analysis of Xiangshan Harbor Highway Cable-Stayed Bridges in Service. Applied Sciences. 2022; 12(16):8301. https://doi.org/10.3390/app12168301
Chicago/Turabian StyleZhang, Tian, Xinjia Cui, Xuefan Zhang, Haijiang Li, and Yunfeng Zou. 2022. "Flutter Reliability Analysis of Xiangshan Harbor Highway Cable-Stayed Bridges in Service" Applied Sciences 12, no. 16: 8301. https://doi.org/10.3390/app12168301
APA StyleZhang, T., Cui, X., Zhang, X., Li, H., & Zou, Y. (2022). Flutter Reliability Analysis of Xiangshan Harbor Highway Cable-Stayed Bridges in Service. Applied Sciences, 12(16), 8301. https://doi.org/10.3390/app12168301






