Low Reynolds Number Swimming Near Interfaces in Multi-Fluid Media
Abstract
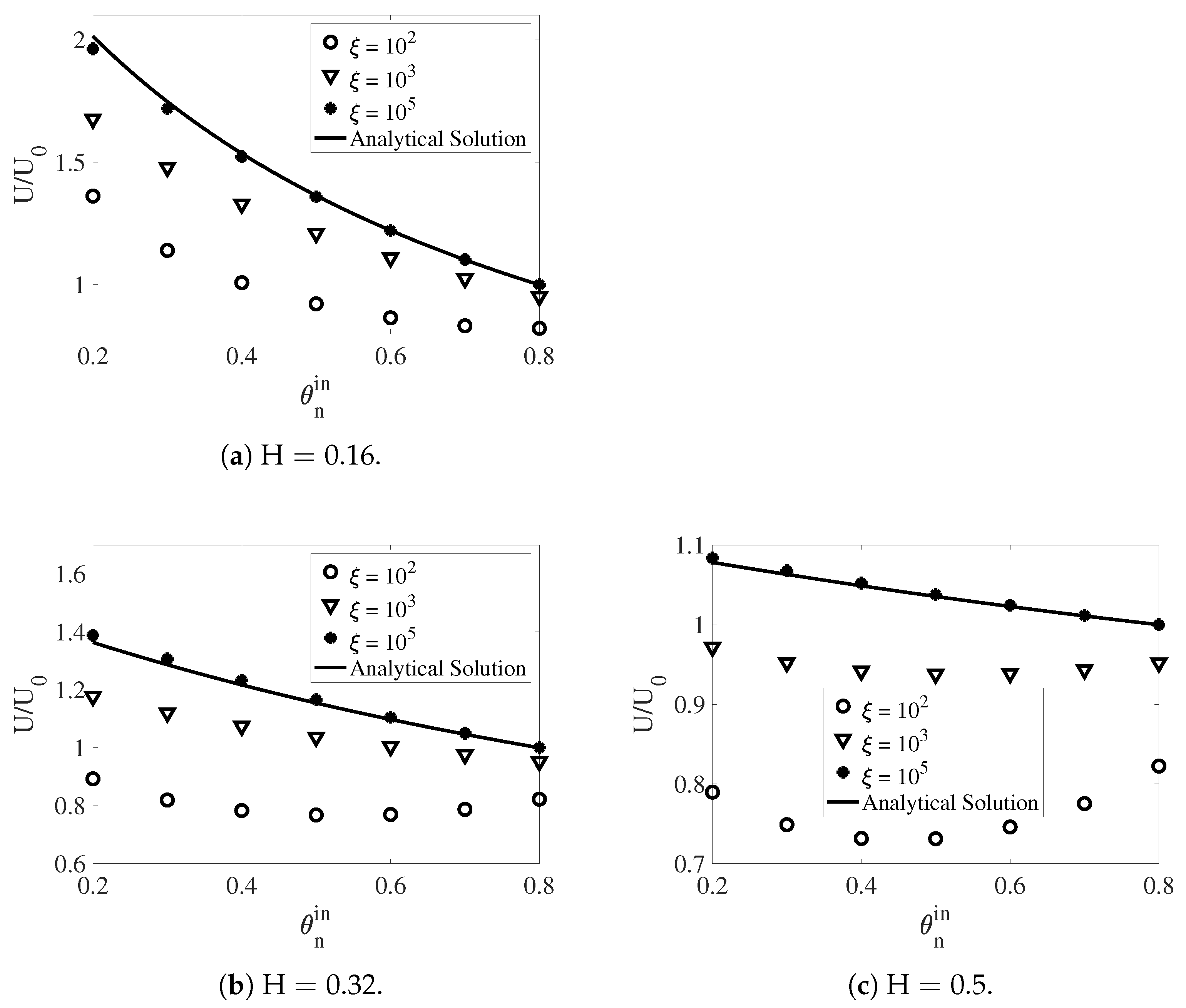
:1. Introduction
2. Model Equations and Numerical Method
2.1. Two-Fluid Mixture Model
2.2. Immersed Boundary Method for Multi-Fluid Mixture
2.3. Numerical Solutions
- Based on the geometric configurations of IB curves and at , compute the total elastic force densities and on them. Compute the Eulerian forces and by spreading to the network and to the solvent.
- Compute at from discretized version of (2).
- Repeat step 1 at next time level .
3. Results
3.1. Problem Setup
3.2. Comparison with Analytic Solutions
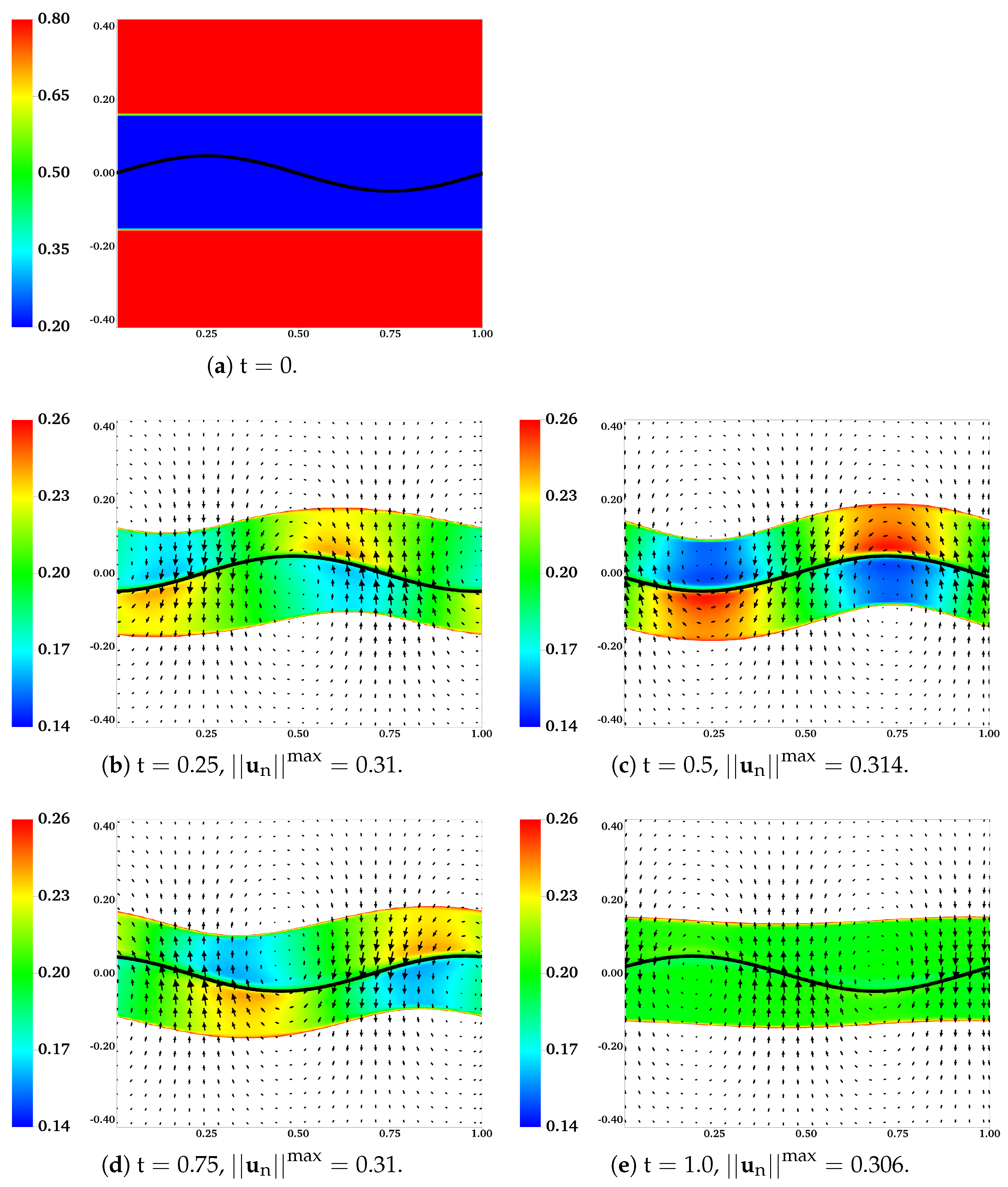
3.2.1. Swimming Near Non-Deformable Fluid Interface
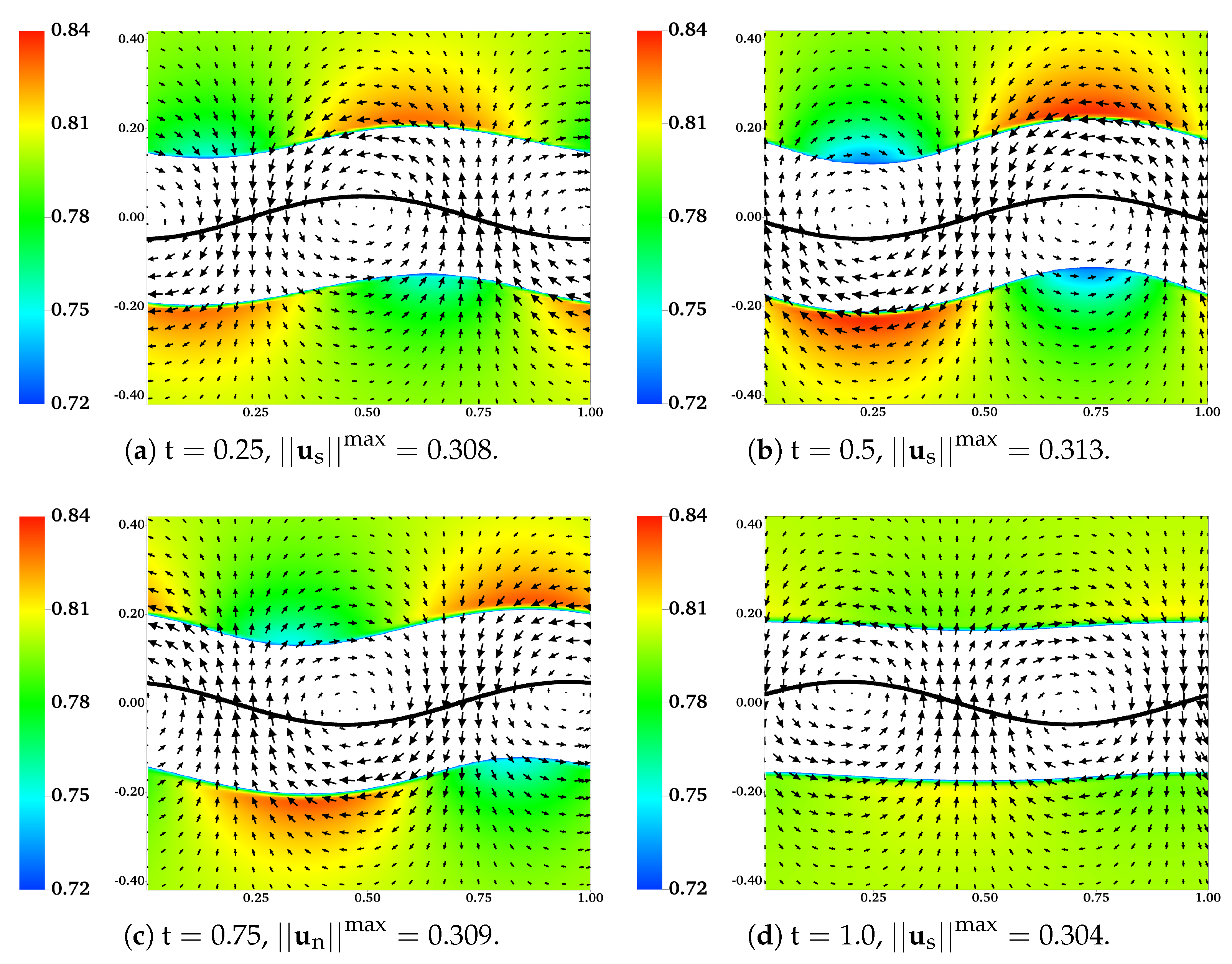
3.2.2. Swimming Near Deformable Fluid Interface
3.2.3. Force Analysis
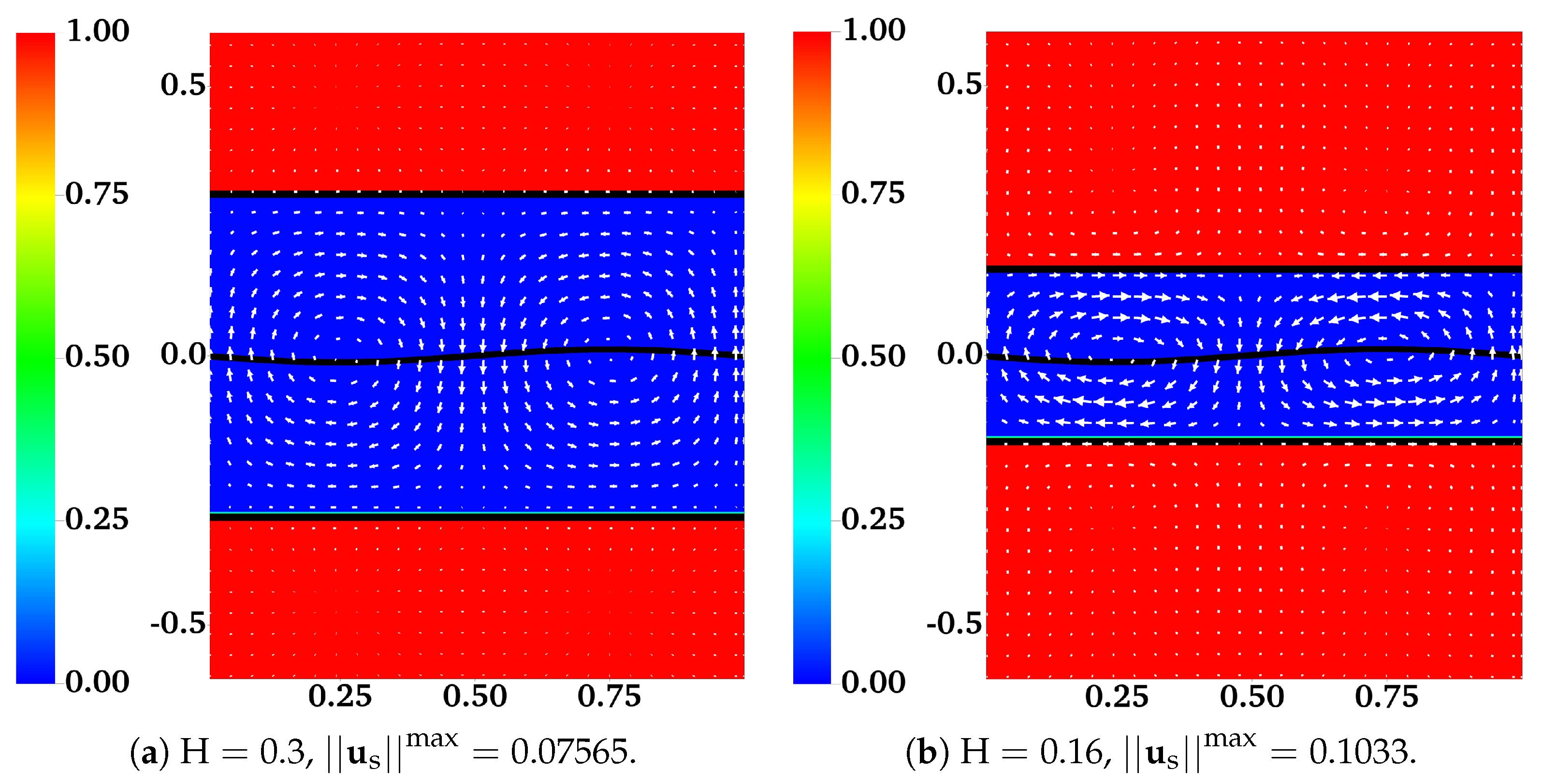
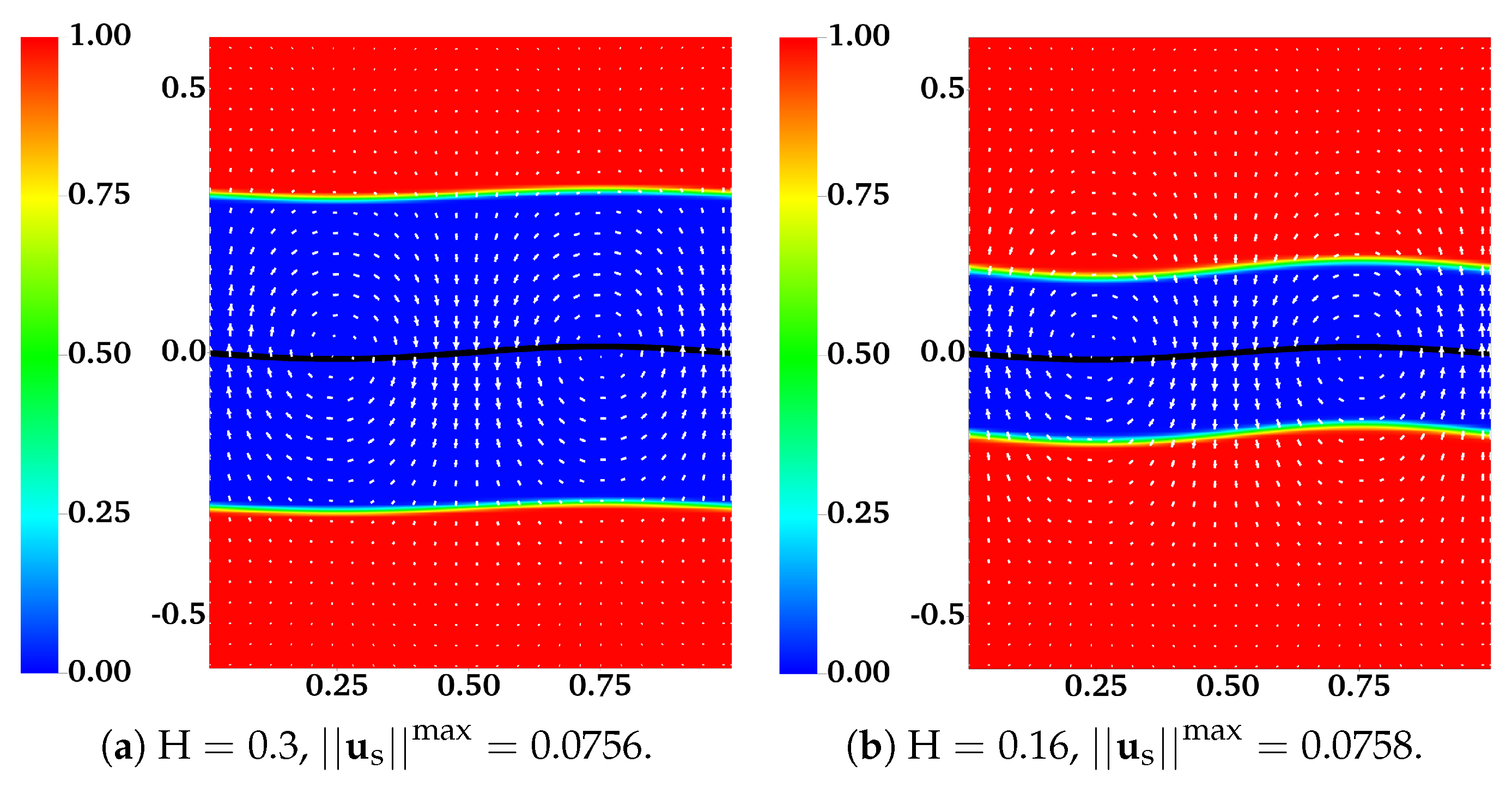
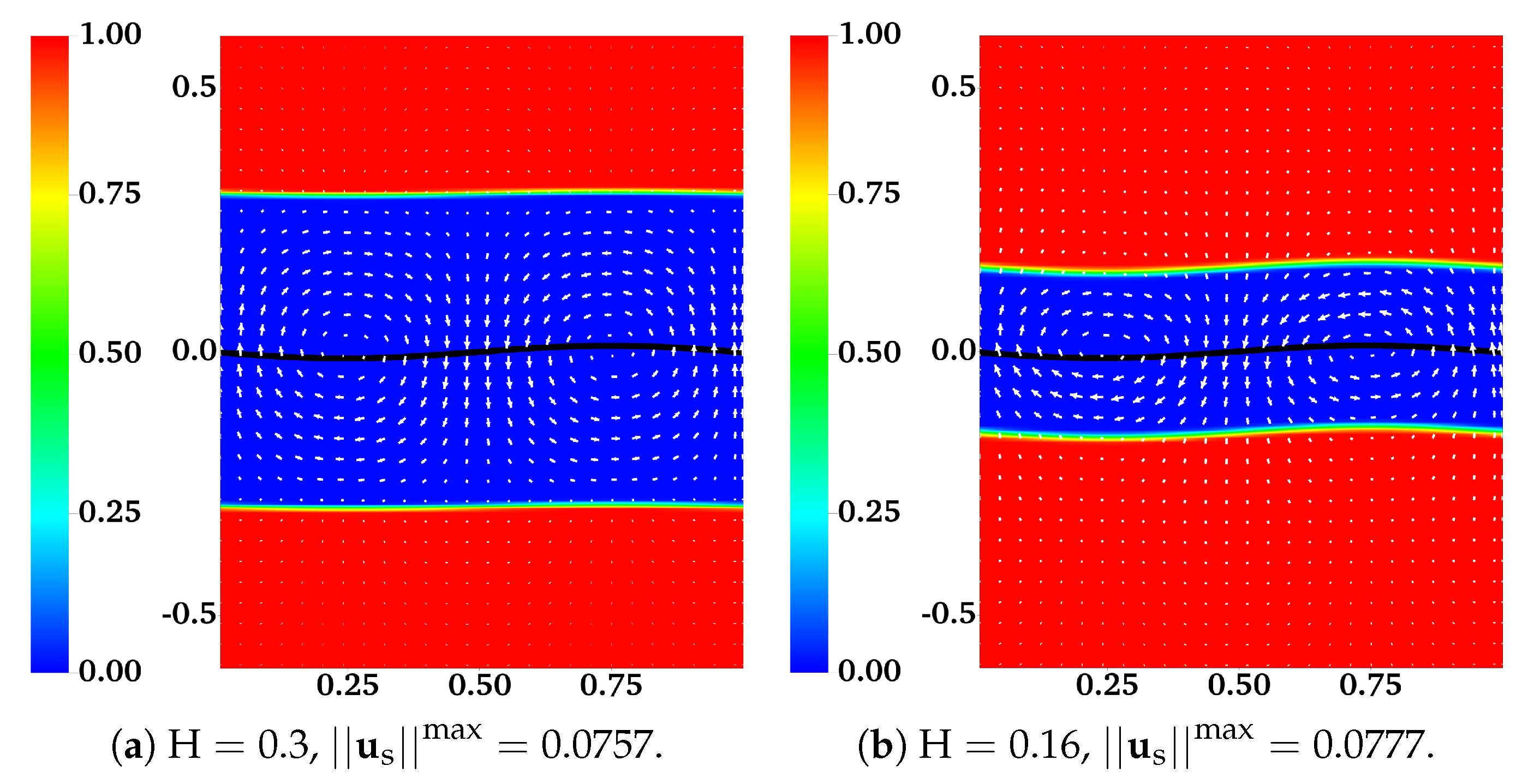
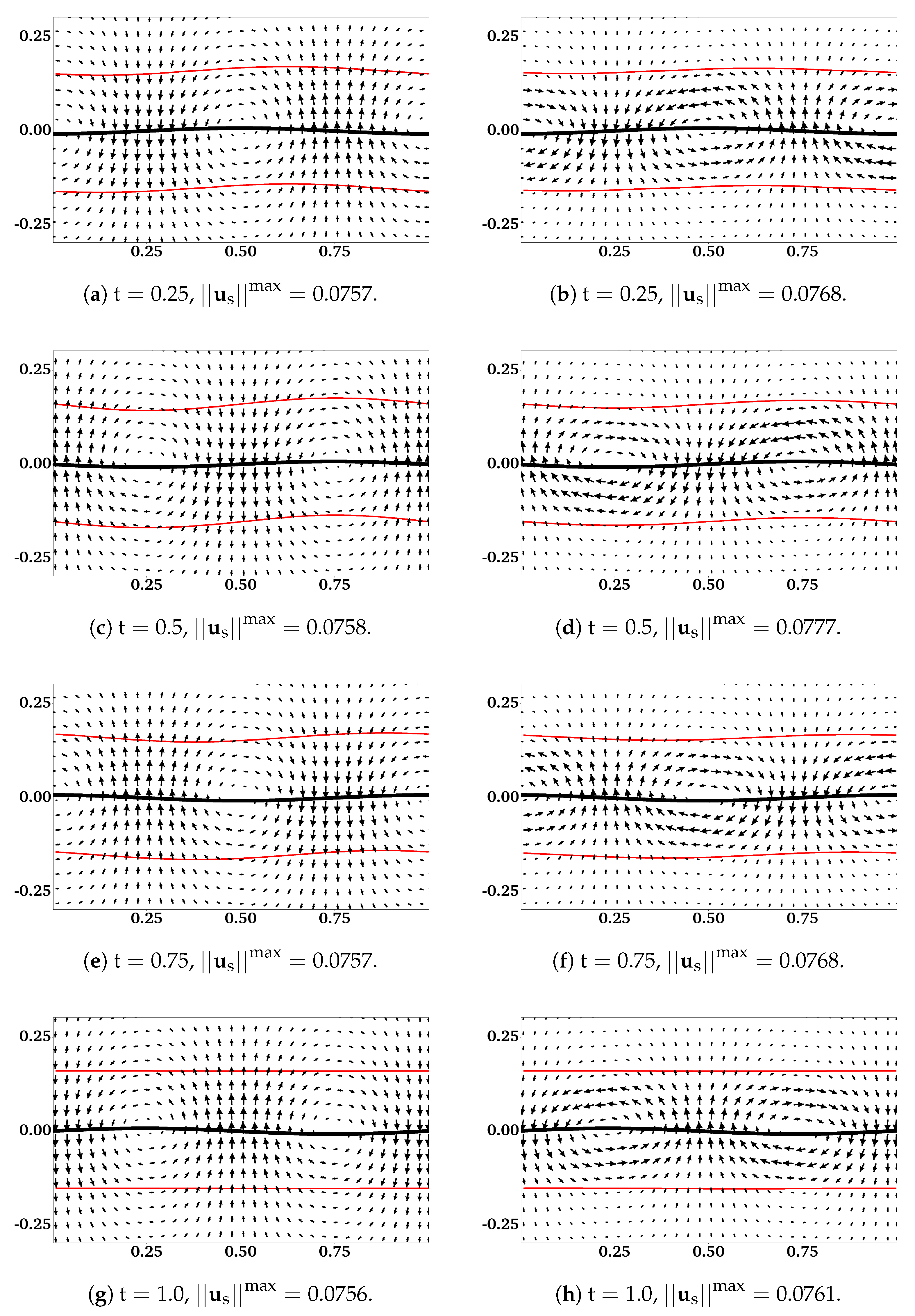
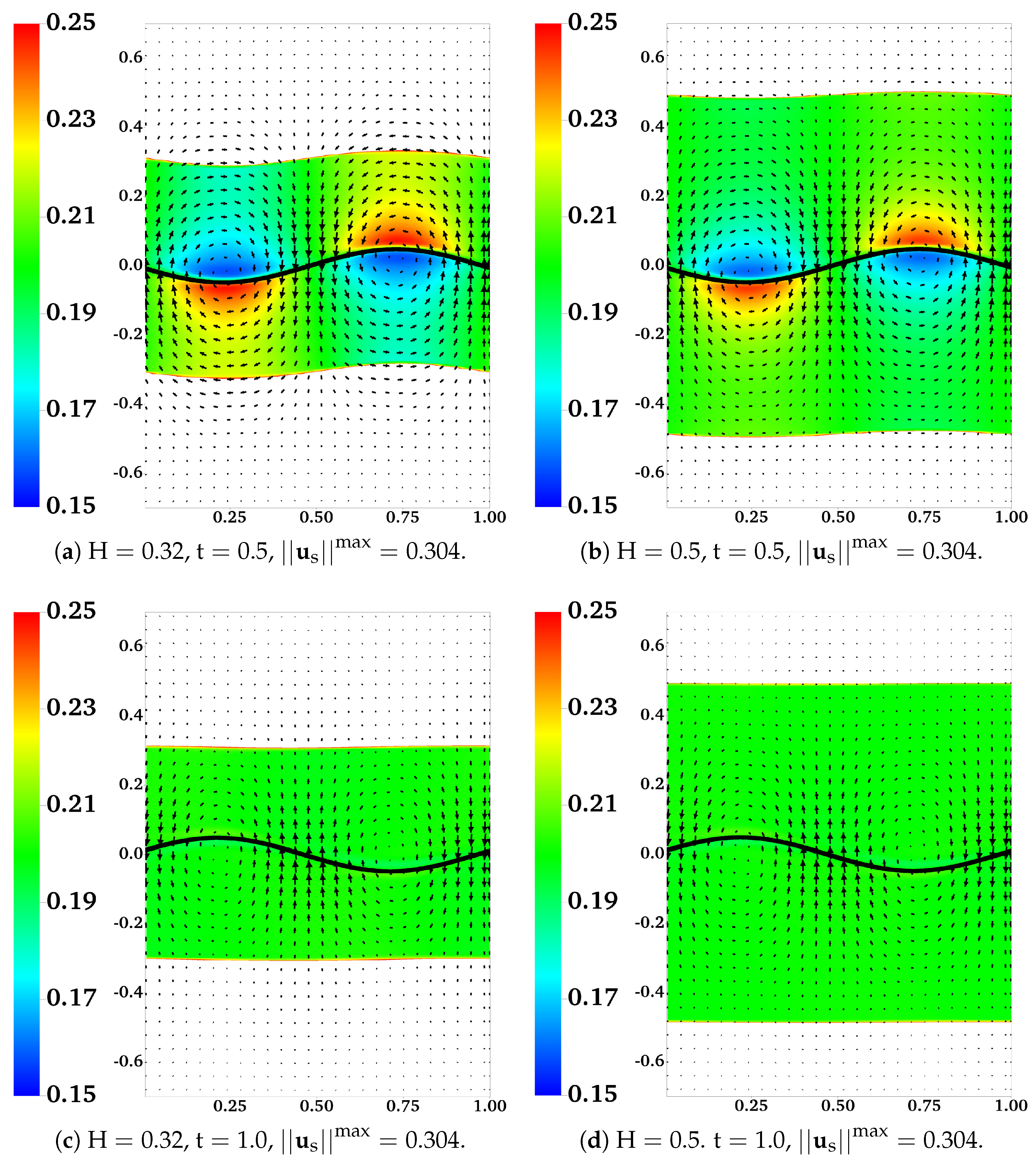
3.3. Swimming Near Deformable Interface in Fluid Mixtures
4. Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
References
- Celli, J.P.; Turner, B.S.; Afdhal, N.H.; Keates, S.; Ghiran, I.; Kelly, C.P.; Ewoldt, R.H.; McKinley, G.H.; So, P.; Erramilli, S.; et al. Helicobacter pylori moves through mucus by reducing mucin viscoelasticity. Proc. Natl. Acad. Sci. USA 2009, 106, 14321. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Patteson, A.E.; Gopinath, A.; Goulian, M.; Arratia, P.E. Running and tumbling with E. coli in polymeric solutions. Sci. Rep. 2015, 5, 15761. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Sznitman, J.; Arratia, P.E. Locomotion through complex fluids: An experimental view. In Complex Fluids in Biological Systems; Spagnolie, S.E., Ed.; Springer: New York, NY, USA, 2015; pp. 245–281. [Google Scholar]
- Elfring, G.J.; Lauga, E. Theory of locomotion through complex fluids. In Complex Fluids in Biological Systems; Spagnolie, S.E., Ed.; Springer: New York, NY, USA, 2015; pp. 283–317. [Google Scholar]
- Guy, R.D.; Thomases, B. Computational challenges for simulating strongly elastic flows in biology. In Complex Fluids in Biological Systems; Spagnolie, S.E., Ed.; Springer: New York, NY, USA, 2015; pp. 359–397. [Google Scholar]
- Lauga, E.; DiLuzio, W.R.; Whitesides, G.M.; Stone, H.A. Swimming in circles: Motion of bacteria near solid boundaries. Biophys J. 2006, 2, 400–412. [Google Scholar] [CrossRef] [Green Version]
- Boryshpolets, S.; Cosson, J.; Bondarenko, V.; Gillies, E.; Rodina, M.; Dzyuba, B.; Linhart, O. Different swimming behaviors of sterlet (Acipenser ruthenus) spermatozoa close to solid and free surfaces. Theriogenology 2013, 79, 81–86. [Google Scholar] [CrossRef]
- Gomez, S.; Godinez, F.A.; Lauga, E.; Zenit, R. Helical propulsion in shear-thinning fluids. J. Fluid Mech. 2017, 812, R3. [Google Scholar] [CrossRef] [Green Version]
- Shaik, V.A.; Ardekani, A.M. Swimming sheet near a plane surfactant-laden interface. Phys. Rev. E 2019, 99, 033101. [Google Scholar] [CrossRef] [Green Version]
- Nganguia, H.; Zhu, L.; Palaniappan, D.; Pak, O.S. Squirming in a viscous fluid enclosed by a Brinkman medium. Phys. Rev. E 2020, 101, 063105. [Google Scholar] [CrossRef]
- Dias, M.A.; Powers, T.R. Swimming near deformable membranes at low Reynolds number. Phys. Fluids 2013, 25, 101901. [Google Scholar] [CrossRef] [Green Version]
- Man, Y.; Lauga, E. Phase-separation models for swimming enhancement in complex fluids. Phys. Rev. E 2015, 92, 023004. [Google Scholar] [CrossRef] [Green Version]
- Du, J.; Guy, R.D.; Fogelson, A.L. An immersed boundary method for two-fluid mixtures. J. Comput. Phys. 2014, 262, 231–243. [Google Scholar] [CrossRef] [Green Version]
- Lee, P.; Wolgemuth, C. An immersed boundary method for two-phase fluids and gels and the swimming of C. Elegans through viscoelastic fluids. Phys. Fluids 2016, 28, 011901. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Du, J.; Fogelson, A.L. A two-phase mixture model of platelet aggregation. Math. Med. Biol. J. IMA 2018, 35, 225–256. [Google Scholar] [CrossRef] [PubMed]
- Cogan, N.; Guy, R.D. Multiphase flow models of biogels from crawling cells to bacterial biofilms. HFSP J. 2010, 4, 11–25. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Peskin, C. The immersed boundary method. Acta Numer. 2002, 11, 479–517. [Google Scholar] [CrossRef] [Green Version]
- Teran, J.; Fauci, L.; Shelley, M. Viscoelastic fluid response can increase the speed and efficiency of a free swimmer. Phys. Rev. Lett. 2010, 104, 038101. [Google Scholar] [CrossRef]
- Chrispell, L.J.; Fauci, L.; Shelley, M. An actuated elastic sheet interacting with passive and active structures in a viscoelastic fluid. Phys. Fluids 2013, 25, 013103. [Google Scholar] [CrossRef] [Green Version]
- Strychalski, W.; Guy, R.D. A computational model of bleb formation. Math. Med. Biol. J. IMA 2013, 30, 115–130. [Google Scholar] [CrossRef]
- Griffith, B.E.; Luo, X.; McQueen, D.M.; Peskin, C.S. Simulating the fluid dynamics of natural and prosthetic heart valves using the immersed boundary method. Int. J. Appl. Mech. 2009, 1, 137–177. [Google Scholar] [CrossRef]
- Fogelson, A.L.; Guy, R.D. Immersed-boundary-type models of intravascular platelet aggregation. Comput. Method Appl. Mech. Eng. 2008, 197, 2087–2104. [Google Scholar] [CrossRef] [Green Version]
- Taylor, G. Analysis of the swimming of microscopic organisms. Proc. R. Soc. A 1951, 209, 447–461. [Google Scholar]
- Unverdi, S.O.; Tryggvason, G. A front tracking method for viscous incompressible multi-fluid flows. J. Comput. Phys. 1992, 100, 25–37. [Google Scholar] [CrossRef] [Green Version]
- Du, J.; Guy, R.D.; Fogelson, A.F.; Wright, G.B.; Keener, J.P. An interface-capturing regularization method for solving the equations for two-fluid mixtures. Commun. Comput. Phys. 2013, 14, 1322–1346. [Google Scholar] [CrossRef] [Green Version]
- Lauga, E.; Powers, T.R. The hydrodynamics of swimming microorganisms. Rep. Prog. Phys. 2009, 72, 096601. [Google Scholar] [CrossRef]
- Du, J.; Keener, J.P.; Guy, R.D.; Fogelson, A.L. Low Reynolds-number swimming in viscous two-phase fluids. Phys. Rev. E 2012, 85, 036304. [Google Scholar]
- Shen, X.; Arratia, P. Undulatory swimming in viscoelastic fluids. Phys. Rev. Lett. 2011, 106, 208101. [Google Scholar] [CrossRef] [Green Version]
- Espinosa-Garcia, J.; Lauga, E.; Zenit, R. Fluid elasticity increases the locomotion of flexible swimmers. Phys. Fluids 2013, 25, 031701. [Google Scholar] [CrossRef]
- Mirbagheri, S.A.; Fu, H.C. Helicobacter pylori couples motility and diffusion to actively create a heterogeneous complex medium in gastric mucus. Phys. Rev. Lett. 2016, 116, 198101. [Google Scholar] [CrossRef] [PubMed] [Green Version]




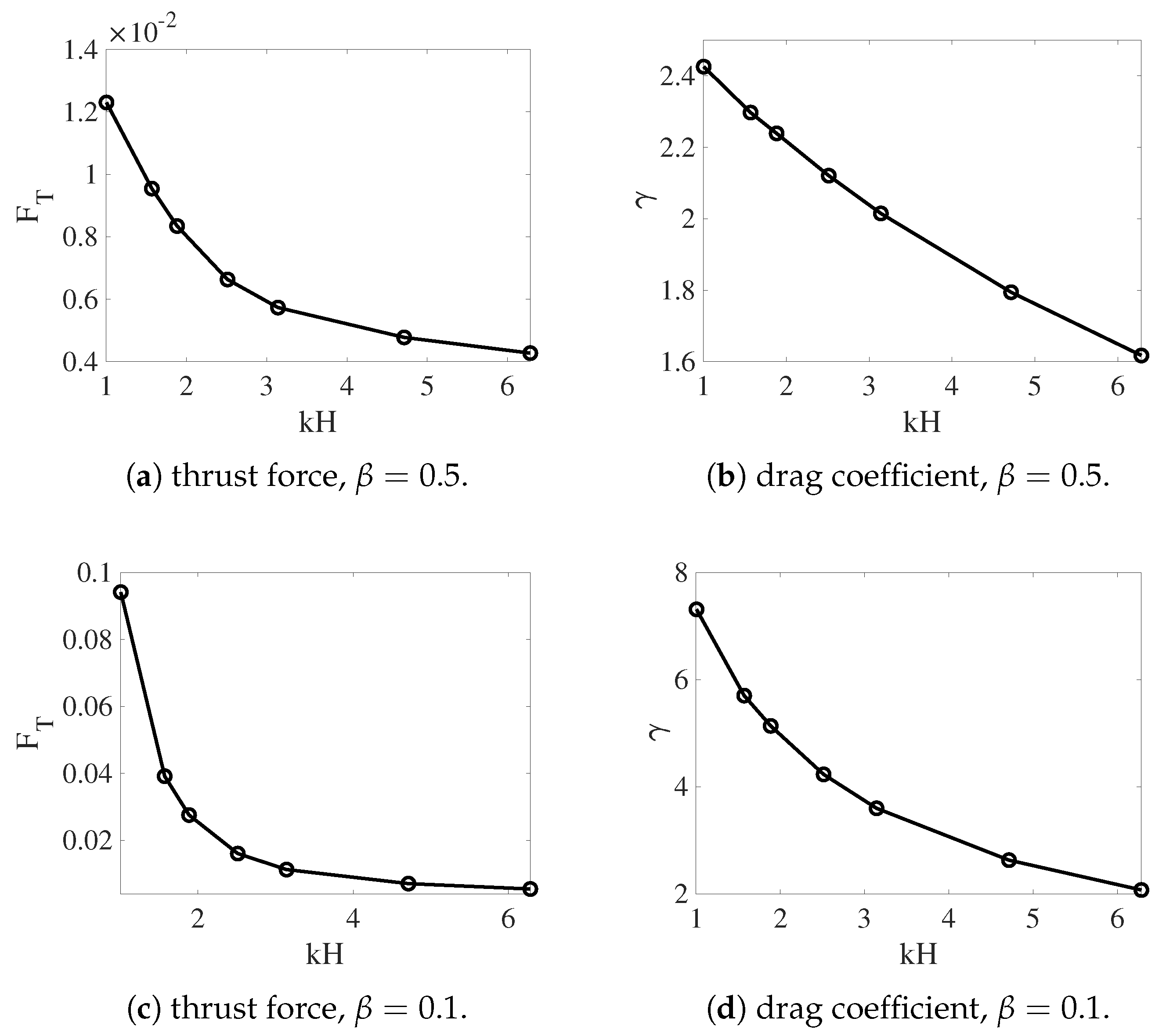

| Thrust Force () | Drag Coefficient () | Swimming Speed U | ||
|---|---|---|---|---|
| Thrust Force () | Drag Coefficient () | Swimming Speed U | ||
|---|---|---|---|---|
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cartwright, A.; Du, J. Low Reynolds Number Swimming Near Interfaces in Multi-Fluid Media. Appl. Sci. 2021, 11, 9109. https://doi.org/10.3390/app11199109
Cartwright A, Du J. Low Reynolds Number Swimming Near Interfaces in Multi-Fluid Media. Applied Sciences. 2021; 11(19):9109. https://doi.org/10.3390/app11199109
Chicago/Turabian StyleCartwright, Avriel, and Jian Du. 2021. "Low Reynolds Number Swimming Near Interfaces in Multi-Fluid Media" Applied Sciences 11, no. 19: 9109. https://doi.org/10.3390/app11199109
APA StyleCartwright, A., & Du, J. (2021). Low Reynolds Number Swimming Near Interfaces in Multi-Fluid Media. Applied Sciences, 11(19), 9109. https://doi.org/10.3390/app11199109






