Numerical Analysis of Edge Cracking in High-Silicon Steel during Cold Rolling with 3D Fracture Locus
Abstract
:1. Introduction
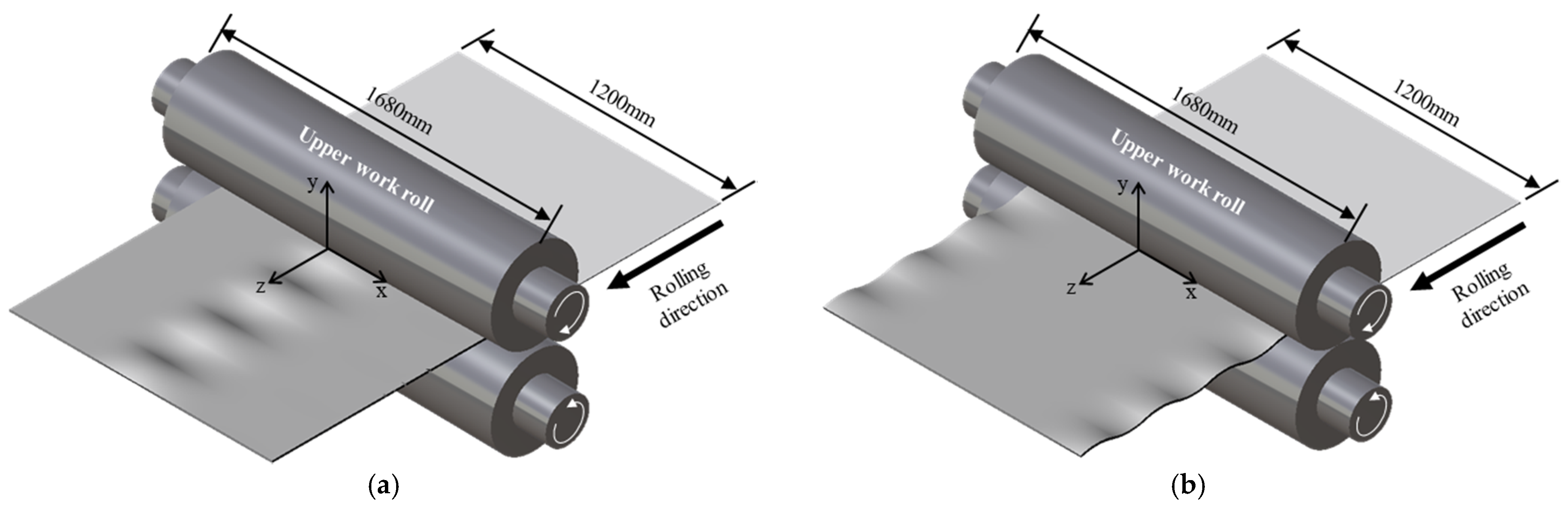
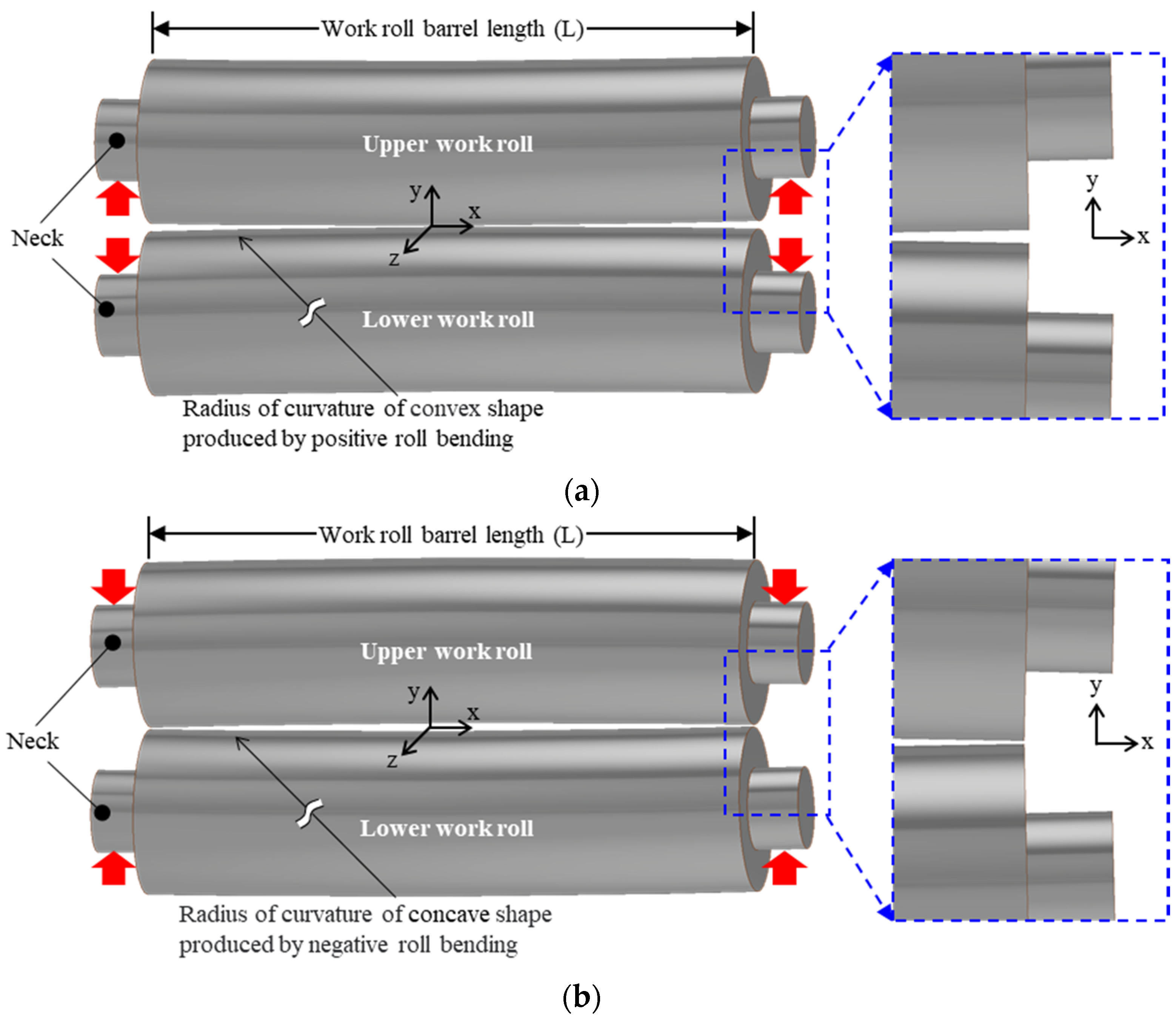
2. Description of Roll Bending
3. Experiments
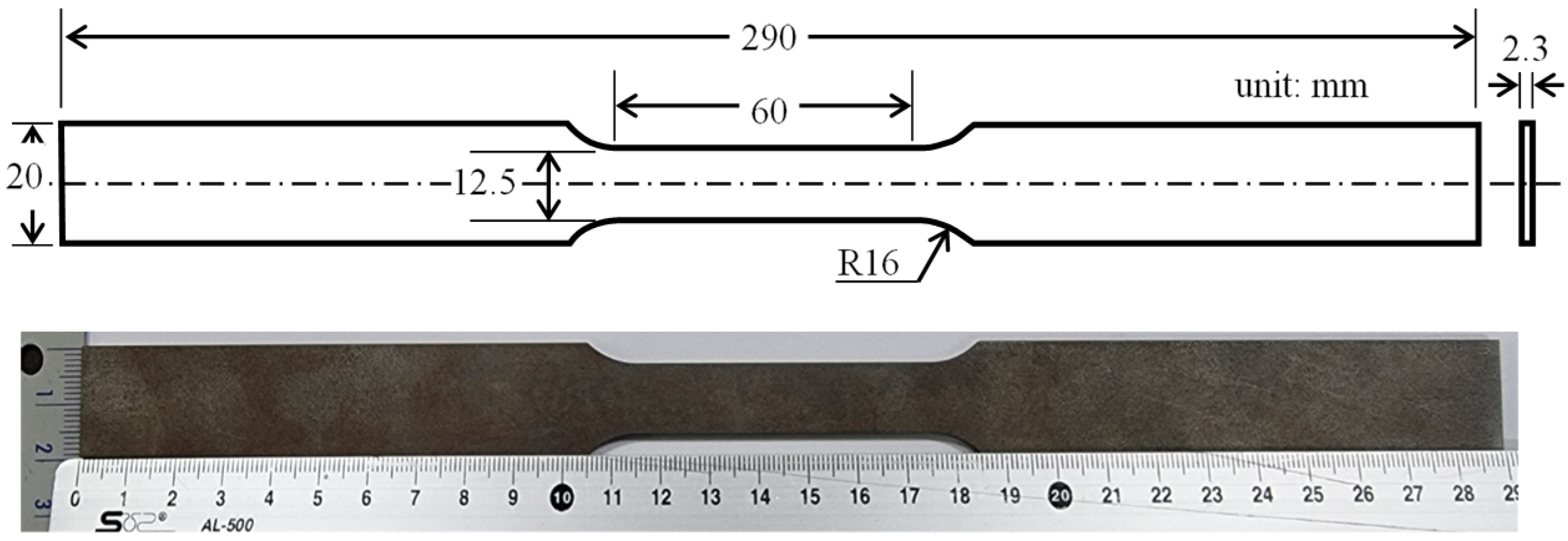
3.1. Specimens for Uniaxial Tensile Test
3.2. Specimens for Fracture Test
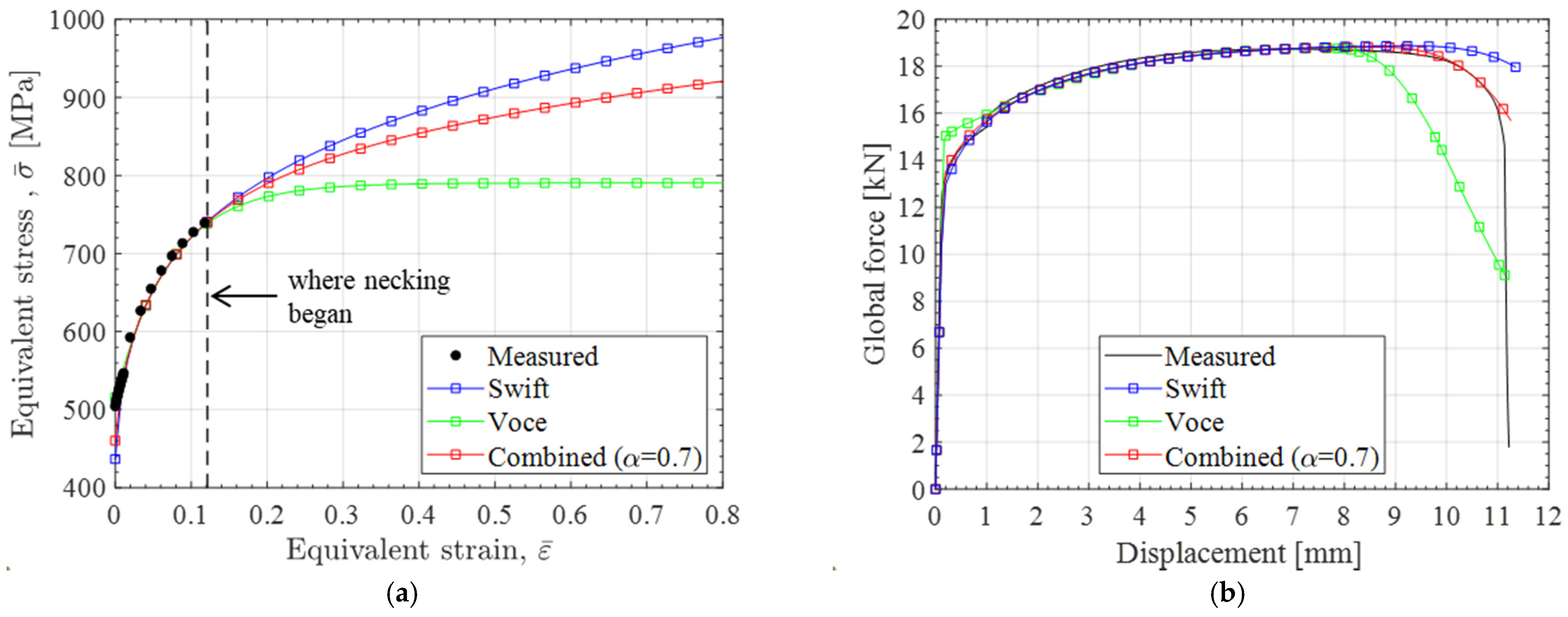
4. Stress–Strain Behavior of High Silicon Steel
5. Fracture Initiation Model
5.1. 3D Fracture Locus
5.2. Material Stiffness Degradation
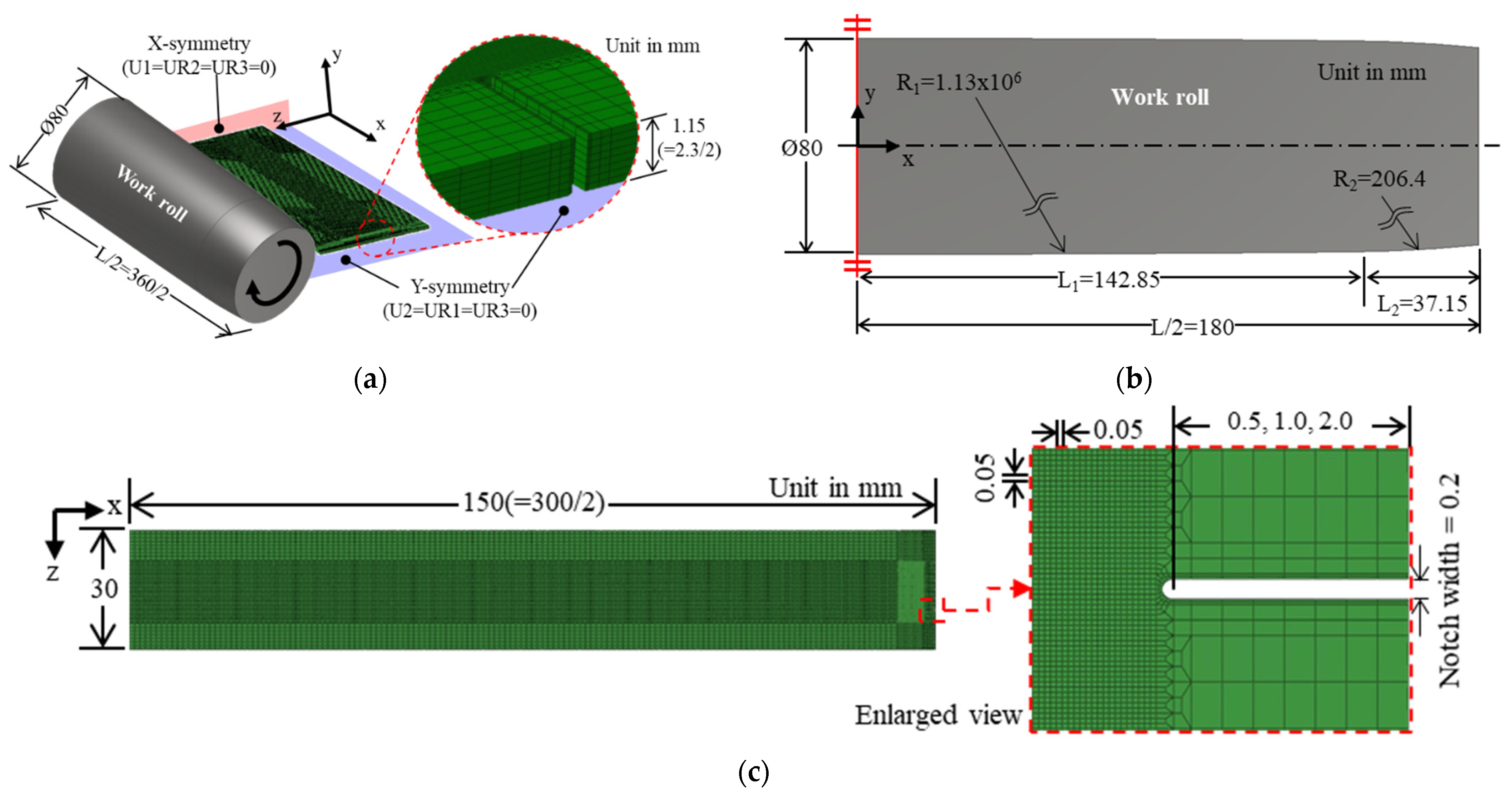
6. FE Simulation of Cold Rolling
7. Results and Discussion
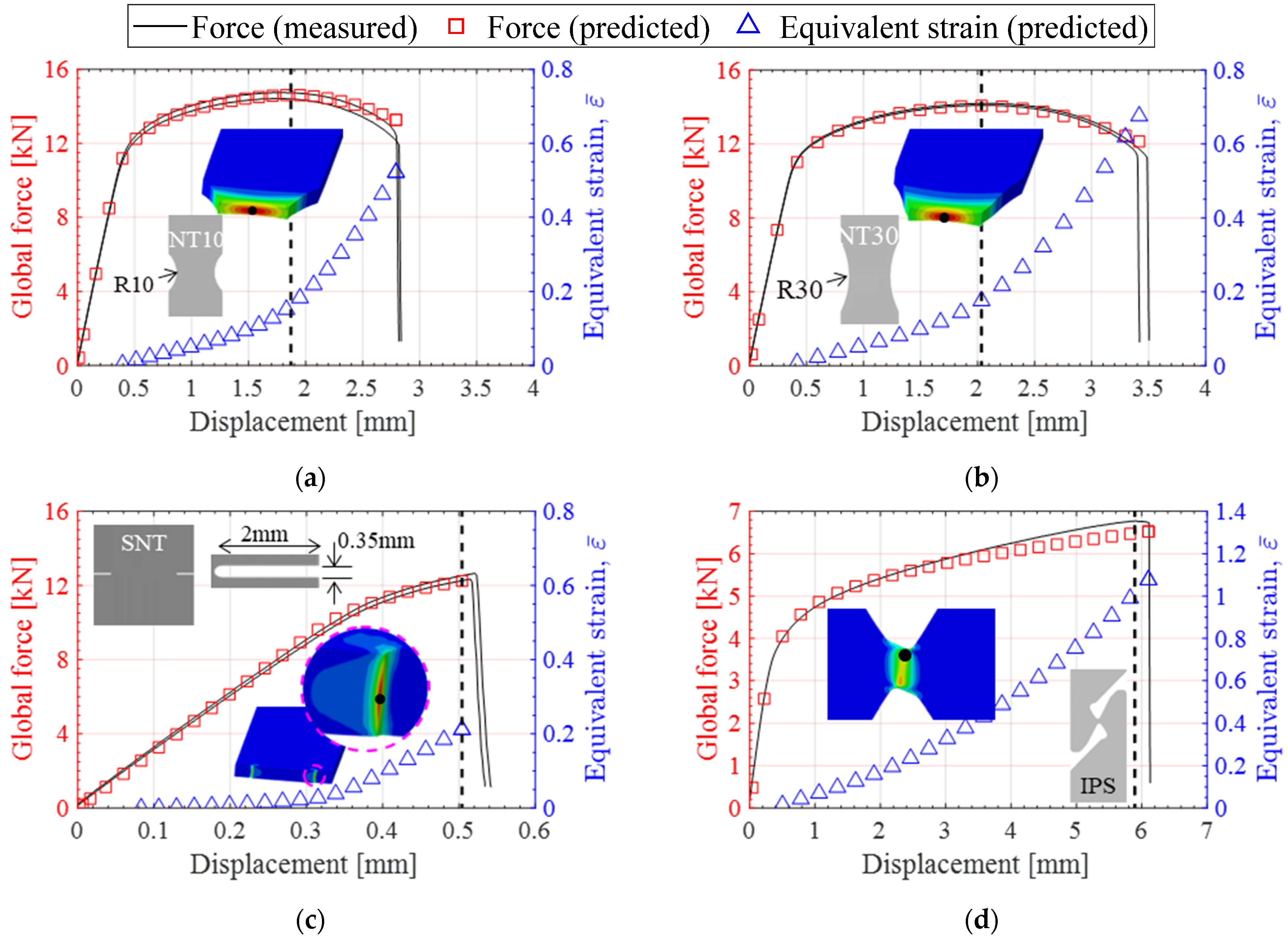
7.1. Force, Displacement, and Equivalent Strain Responses in Different Stress States
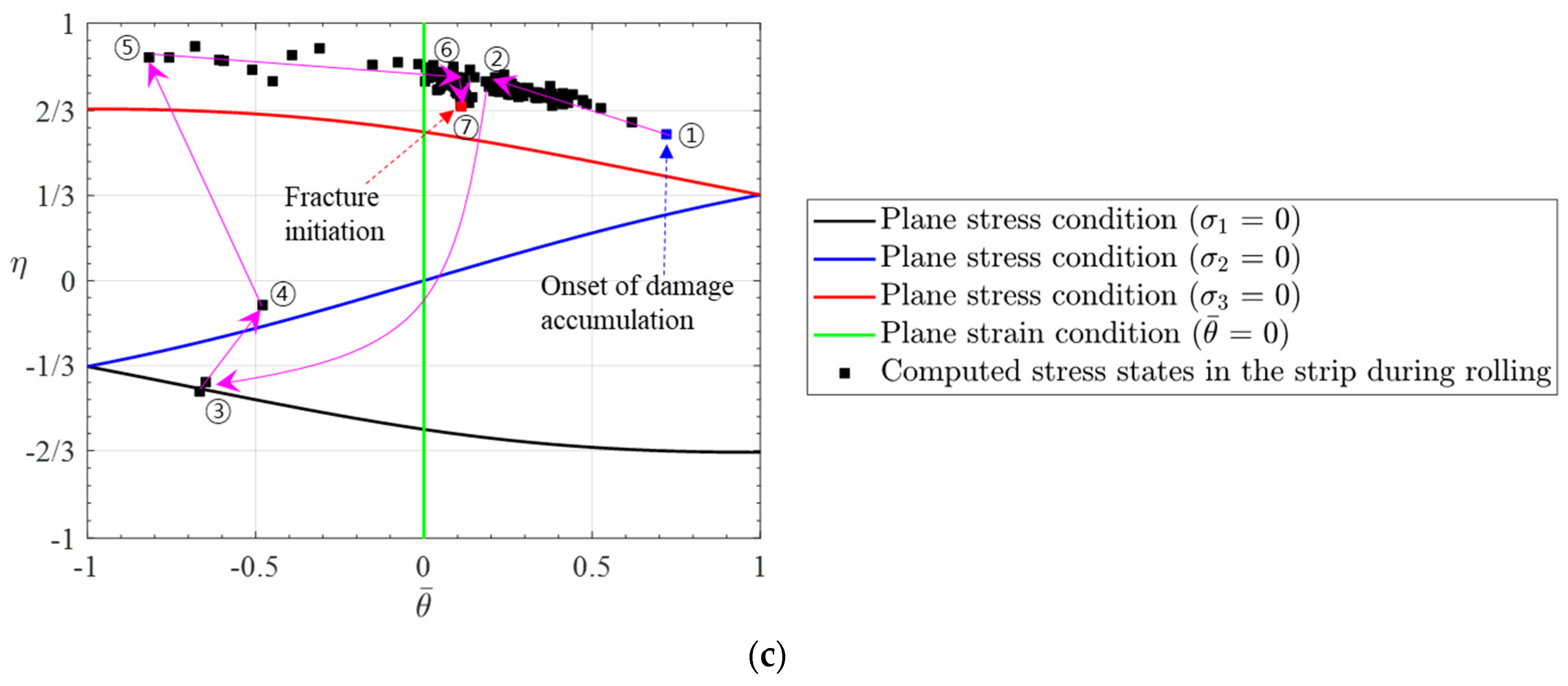
7.2. Fracture Locus
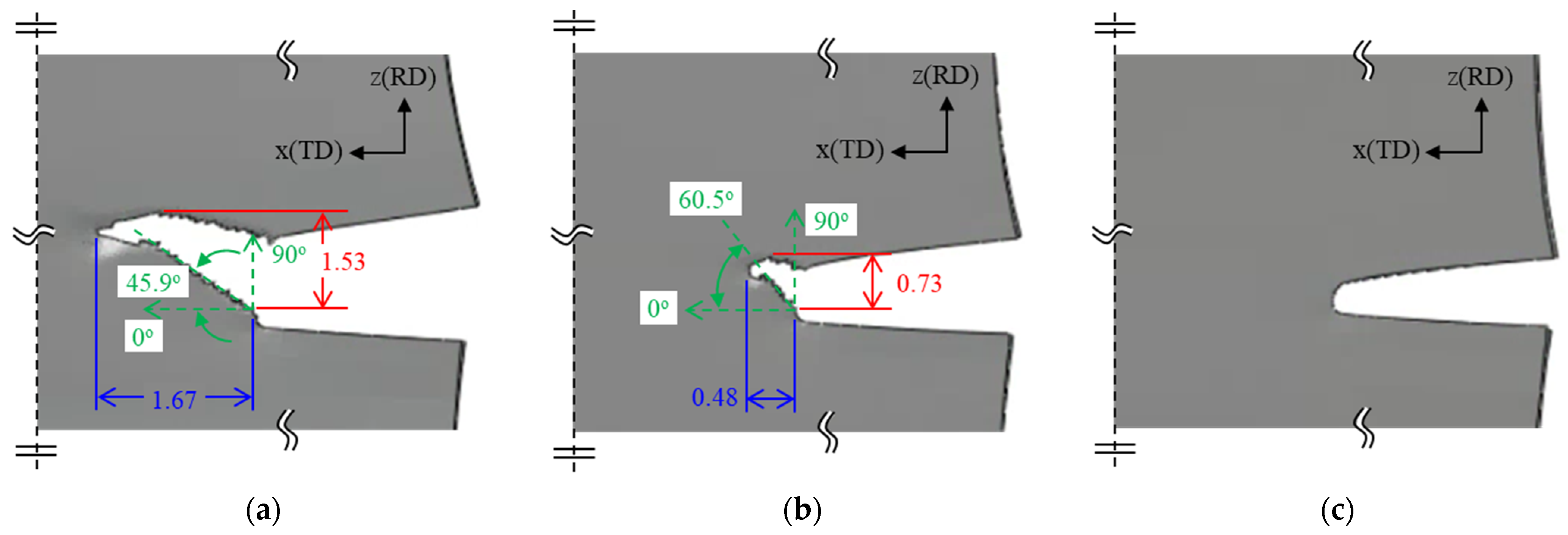
7.3. Numerical Analysis of Edge Cracking in the Strip
8. Application
9. Concluding Remarks
- 1.
- The 2D fracture locus at a high triaxiality of 13.1–22.2% overestimates the edge cracking. Therefore, edge cracking should be predicted using the 3D fracture locus that includes stress triaxiality, and Lode angle parameters varying between −0.81and 0.71 should be used.
- 2.
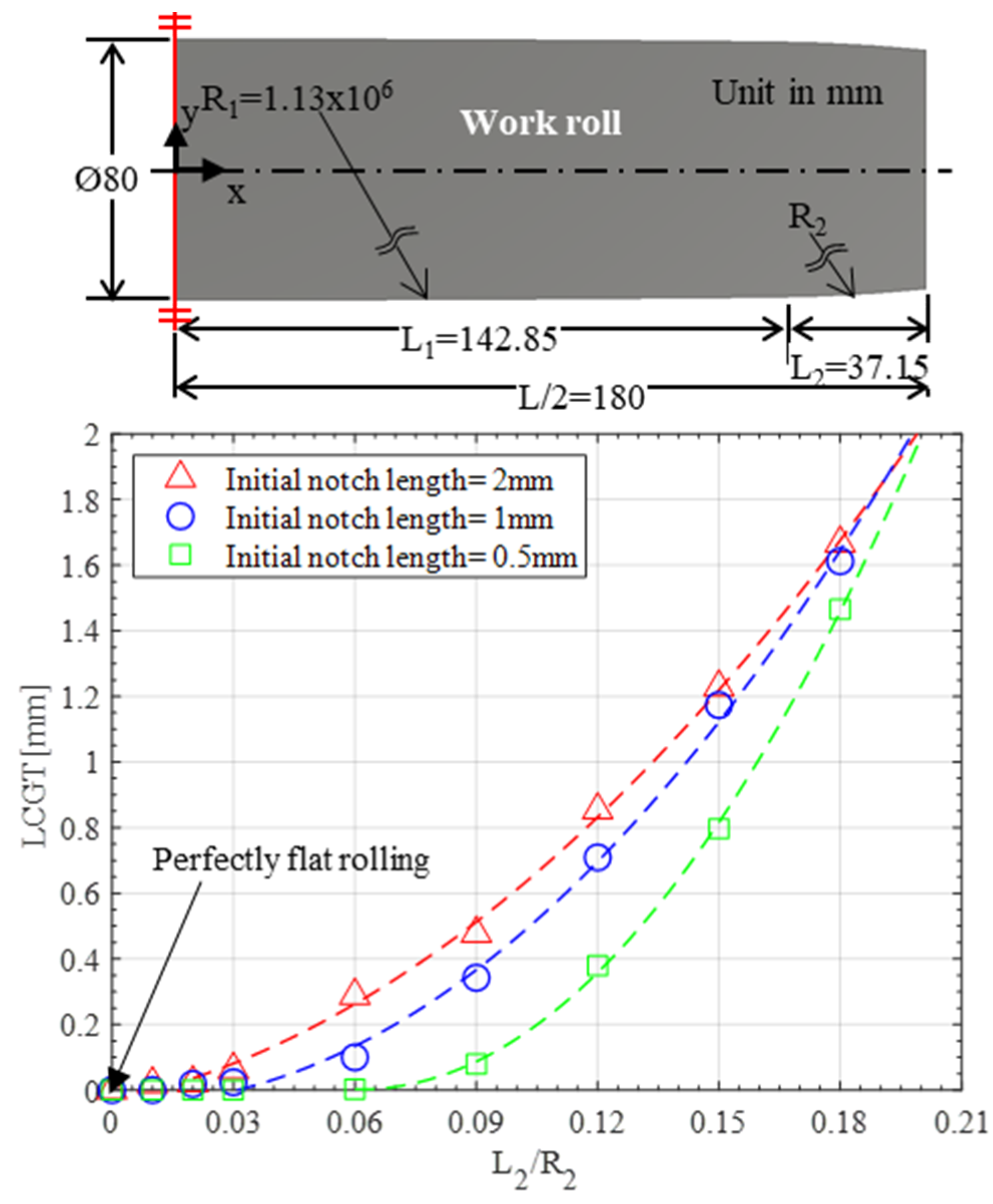
- As the initial notch length at the edges of the trimmed strip after hot rolling is shortened, the length of crack grown in the transverse direction at the edge of the strip was reduced non-linearly. This effect was greatest when the secondary roll-bending ratio was 0.12.
Author Contributions
Funding
Conflicts of Interest
References
- Hilbert, H.G.; Roemmen, H.J.; Boucke, K.E. MKW cold mill rolling silicon steel strip. Iron Steel Eng. 1976, 25, 25–31. [Google Scholar]
- Chen, A.-H.; Guo, H.-R.; Li, H.-L.; Emi, T. Formation of edge crack in 1.4% Si non-oriented electrical steel during hot rolling. J. Iron Steel Res. Int. 2014, 21, 269–274. [Google Scholar] [CrossRef]
- Lee, S.H.; Lee, K.H.; Lee, S.B.; Kim, B.M. Study of edge-cracking characteristics during thin-foil rolling of Cu-Fe-P strip. Int. J. Precis. Eng. Manuf. 2013, 14, 2109–2118. [Google Scholar] [CrossRef]
- Byon, S.M.; Lee, Y. Experimental study on the prohibition of edge cracking of high-silicon steel strips during cold rolling. Proc. Inst. Mech. Eng. Part B J. Eng. Manuf. 2011, 225, 1983–1988. [Google Scholar] [CrossRef]
- Yan, Y.; Sun, Q.; Chen, J.; Pan, H. The initiation and propagation of edge cracks of silicon steel during tandem cold rolling process based on the Gurson-Tvergaard-Needleman damage model. J. Mater. Process. Technol. 2013, 213, 598–605. [Google Scholar] [CrossRef]
- Tvergaard, V.; Needleman, A. Analysis of the cup-cone fracture in a round tensile bar. Acta Metall. 1984, 32, 157–169. [Google Scholar] [CrossRef]
- Sun, Q.; Chen, J.; Pan, H. Prediction of edge crack in cold rolling of silicon steel strip based on an extended Gurson-Tvergaard-Needleman damage model. J. Manuf. Sci. Eng. Trans. ASME 2015, 137, 1–6. [Google Scholar] [CrossRef]
- Byon, S.M.; Roh, Y.H.; Yang, Z.; Lee, Y. A roll-bending approach to suppress the edge cracking of silicon steel in the cold rolling process. Proc. Inst. Mech. Eng. Part B J. Eng. Manuf. 2021, 235, 112–124. [Google Scholar] [CrossRef]
- Johnson, G.R.; Cook, W.H. Fracture characteristics of three metals subjected to various strains, strain rates, temperatures and pressures. Eng. Fract. Mech. 1985, 21, 31–48. [Google Scholar] [CrossRef]
- Bai, Y.; Wierzbicki, T. Application of extended Mohr—Coulomb criterion to ductile fracture. Int. J. Fract. 2010, 161, 1–20. [Google Scholar] [CrossRef]
- JFE Deformation of Rolling Mill. Available online: http://www.jfe-21st-cf.or.jp/chapter_3/3b_2.html (accessed on 7 August 2021).
- Qin, S.; Beese, A.M. Multiaxial fracture of DP600: Experiments and finite element modeling. Mater. Sci. Eng. A 2020, 785, 139386. [Google Scholar] [CrossRef]
- Lian, J.; Sharaf, M.; Archie, F.; Münstermann, S. A hybrid approach for modelling of plasticity and failure behaviour of advanced high-strength steel sheets. Int. J. Damage Mech. 2013, 22, 188–218. [Google Scholar] [CrossRef]
- Abi-Akl, R.; Mohr, D. Paint-bake effect on the plasticity and fracture of pre-strained aluminum 6451 sheets. Int. J. Mech. Sci. 2017, 124–125, 68–82. [Google Scholar] [CrossRef] [Green Version]
- Mohr, D.; Marcadet, S.J. Micromechanically-motivated phenomenological Hosford-Coulomb model for predicting ductile fracture initiation at low stress triaxialities. Int. J. Solids Struct. 2015, 67–68, 40–55. [Google Scholar] [CrossRef]
- Gao, F.; Gui, L.; Fan, Z. Experimental and Numerical Analysis of an In-Plane Shear Specimen Designed for Ductile Fracture Studies. Exp. Mech. 2011, 51, 891–901. [Google Scholar] [CrossRef]
- Paredes, M.; Lian, J.; Wierzbicki, T.; Cristea, M.E.; Münstermann, S.; Darcis, P. Modeling of plasticity and fracture behavior of X65 steels: Seam weld and seamless pipes. Int. J. Fract. 2018, 213, 17–36. [Google Scholar] [CrossRef]
- Swift, H.W. Plastic instability under plane stress. J. Mech. Phys. Solids 1952, 1, 1–18. [Google Scholar] [CrossRef]
- Voce, E. The relationship between stress and strain for homogeneous deformation. J. Inst. Met. 1948, 74, 537–562. [Google Scholar]
- Mu, L.; Zang, Y.; Wang, Y.; Li, X.L.; Araujo Stemler, P.M. Phenomenological uncoupled ductile fracture model considering different void deformation modes for sheet metal forming. Int. J. Mech. Sci. 2018, 141, 408–423. [Google Scholar] [CrossRef]
- Kachanov, L.M. On creep rupture time. Izv. Acad. Nauk SSSR Otd. Techn. Nauk 1958, 8, 26–31. [Google Scholar]
- Chaboche, J.L. Anisotropic creep damage in the framework of continuum damage mechanics. Nucl. Eng. Des. 1984, 79, 309–319. [Google Scholar] [CrossRef] [Green Version]
- Lemaitre, J. Local approach of fracture. Eng. Fract. Mech. 1986, 25, 523–537. [Google Scholar] [CrossRef]
- Zhang, X.; O’Brien, D.J.; Ghosh, S. Parametrically homogenized continuum damage mechanics (PHCDM) models for composites from micromechanical analysis. Comput. Methods Appl. Mech. Eng. 2019, 346, 456–485. [Google Scholar] [CrossRef]
- Li, H.; Wang, J.; Wang, J.; Hu, M.; Peng, Y. Continuum Damage Mechanics Approach for Modeling Cumulative-Damage Model. Math. Probl. Eng. 2021, 1–12. [Google Scholar] [CrossRef]
- McClintock, F.A.; Kaplan, S.M.; Berg, C.A. Ductile fracture by hole growth in shear bands. Int. J. Fract. Mech. 1966, 2, 614–627. [Google Scholar] [CrossRef]
- Gruson, A.L. Continuum theory of ductile rupture by void nucleation and growth: Part I-Yield criteria and flow rules for porous ductile media. J. Eng. Mater. Technol. 1977, 99, 2–15. [Google Scholar] [CrossRef]
- Needleman, A.; Tvergaard, V.F. An analysis of ductile rupture in notched bars. J. Mech. Phys. Solids 1984, 32, 461–490. [Google Scholar] [CrossRef]
- Wen, H.; Mahmoud, H. New Model for Ductile Fracture of Metal Alloys. I: Monotonic Loading. J. Eng. Mech. 2016, 142, 04015088. [Google Scholar] [CrossRef]
- Jiang, W.; Li, Y.; Su, J. Modified GTN model for a broad range of stress states and application to ductile fracture. Eur. J. Mech. A/Solids 2016, 57, 132–148. [Google Scholar] [CrossRef]
- Zhu, Y.; Engelhardt, M.D. Prediction of ductile fracture for metal alloys using a shear modified void growth model. Eng. Fract. Mech. 2018, 190, 491–513. [Google Scholar] [CrossRef]
- Cockcroft, M.G.; Latham, D.J. Ductility and the Workability of Metals. J. Inst. Met. 1968, 96, 33–39. [Google Scholar]
- Oyane, M.; Sato, T.; Okimoto, K.; Shima, S. Criteria for ductile fracture and their applications. J. Mech. Work. Technol. 1980, 4, 65–81. [Google Scholar] [CrossRef]
- Bai, Y.; Wierzbicki, T. A new model of metal plasticity and fracture with pressure and Lode dependence. Int. J. Plast. 2008, 24, 1071–1096. [Google Scholar] [CrossRef]
- Lou, Y.; Huh, H.; Lim, S.; Pack, K. New ductile fracture criterion for prediction of fracture forming limit diagrams of sheet metals. Int. J. Solids Struct. 2012, 49, 3605–3615. [Google Scholar] [CrossRef] [Green Version]
- Fratini, L.; Macaluso, G.; Pasta, S. Residual stresses and FCP prediction in FSW through a continuous FE model. J. Mater. Process. Technol. 2009, 209, 5465–5474. [Google Scholar] [CrossRef]
- Marannano, G.V.; Mistretta, L.; Cirello, A.; Pasta, S. Crack growth analysis at adhesive-adherent interface in bonded joints under mixed mode I/II. Eng. Fract. Mech. 2008, 75, 5122–5133. [Google Scholar] [CrossRef]
- Rice, J.R.; Tracey, D.M. On the ductile enlargement of voids in triaxial stress fields*. J. Mech. Phys. Solids 1969, 17, 201–217. [Google Scholar] [CrossRef] [Green Version]
- Sajid, H.U.; Kiran, R. Influence of high stress triaxiality on mechanical strength of ASTM A36, ASTM A572 and ASTM A992 steels. Constr. Build. Mater. 2018, 176, 129–134. [Google Scholar] [CrossRef]
- Sajid, H.U.; Kiran, R. Post-fire mechanical behavior of ASTM A572 steels subjected to high stress triaxialities. Eng. Struct. 2019, 191, 323–342. [Google Scholar] [CrossRef]
- Kang, L.; Ge, H.; Fang, X. An improved ductile fracture model for structural steels considering effect of high stress triaxiality. Constr. Build. Mater. 2016, 115, 634–650. [Google Scholar] [CrossRef]
- Bao, Y. Prediction of Ductile Crack Formation in Uncracked Bodies. Ph.D. Thesis, Massachusetts Institute of Technology, Cambridge, MA, USA, 2003. [Google Scholar]
- Bao, Y.; Wierzbicki, T. On fracture locus in the equivalent strain and stress triaxiality space. Int. J. Mech. Sci. 2004, 46, 81–98. [Google Scholar] [CrossRef]
- Wierzbicki, T.; Bao, Y.; Lee, Y.W.; Bai, Y. Calibration and evaluation of seven fracture models. Int. J. Mech. Sci. 2005, 47, 719–743. [Google Scholar] [CrossRef]
- Talebi-Ghadikolaee, H.; Naeini, H.M.; Mirnia, M.J.; Mirzai, M.A.; Alexandrov, S.; Zeinali, M.S. Modeling of ductile damage evolution in roll forming of U-channel sections. J. Mater. Process. Technol. 2020, 283, 116690. [Google Scholar] [CrossRef]
- Saneian, M.; Han, P.; Jin, S.; Bai, Y. Fracture response of steel pipelines under combined tension and torsion. Thin-Walled Struct. 2020, 154, 106870. [Google Scholar] [CrossRef]
- Hillerborg, A.; Modéer, M.; Petersson, P.-E. Analysis of crack formation and crack growth in concrete by means of fracture mechanics and finite elements. Cem. Concr. Res. 1976, 6, 773–781. [Google Scholar] [CrossRef]
- Manual, A.U. Abaqus Theory Guide; Version 6.14; USA Dassault Systemes Simulia Corp: Johnston, RI, USA, 2014. [Google Scholar]
- Documentation, A.U. Abaqus Documentation; Version 6.14; USA Dassault Systemes Simulia Corp: Johnston, RI, USA, 2014. [Google Scholar]
- Gao, X.; Zhang, G.; Roe, C. A study on the effect of the stress state on ductile fracture. Int. J. Damage Mech. 2010, 19, 75–94. [Google Scholar] [CrossRef]
- Cao, T.S. Models for ductile damage and fracture prediction in cold bulk metal forming processes: A review. Int. J. Mater. Form. 2017, 10, 139–171. [Google Scholar] [CrossRef]
- Liu, W.; Lian, J.; Münstermann, S. Damage mechanism analysis of a high-strength dual-phase steel sheet with optimized fracture samples for various stress states and loading rates. Eng. Fail. Anal. 2019, 106, 104138. [Google Scholar] [CrossRef]



| ε0 | A1 (MPa) | A2 | B1 (MPa) | B2 (MPa) | B3 | α |
|---|---|---|---|---|---|---|
| 0.003552 | 1009 | 0.1485 | 515.5 | 274.9 | 13.75 | 0.7 |
| No. of Elements | 2 | 4 | 6 | 8 | 10 |
|---|---|---|---|---|---|
| Equivalent strain at fracture | 0.5962 | 0.5844 | 0.5786 | 0.5767 | 0.5770 |
| Specimen Type | |||
|---|---|---|---|
| Standard (dog-bone) | 0.4424 | 0.7366 | 0.7893 |
| NT10 | 0.5349 | 0.4551 | 0.5416 |
| NT30 | 0.4916 | 0.6145 | 0.6755 |
| SNT | 0.8301 | 0.2235 | 0.2116 |
| IPS | 0.0118 | 0.0305 | 1.0993 |
| K (MPa) | n | ||
|---|---|---|---|
| 976.2 | 0.1299 | 0.1226 | 558.7 |
| 0.08478 | 5.133 | 4.467 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Roh, Y.-H.; Byon, S.M.; Lee, Y. Numerical Analysis of Edge Cracking in High-Silicon Steel during Cold Rolling with 3D Fracture Locus. Appl. Sci. 2021, 11, 8408. https://doi.org/10.3390/app11188408
Roh Y-H, Byon SM, Lee Y. Numerical Analysis of Edge Cracking in High-Silicon Steel during Cold Rolling with 3D Fracture Locus. Applied Sciences. 2021; 11(18):8408. https://doi.org/10.3390/app11188408
Chicago/Turabian StyleRoh, Yong-Hoon, Sang Min Byon, and Youngseog Lee. 2021. "Numerical Analysis of Edge Cracking in High-Silicon Steel during Cold Rolling with 3D Fracture Locus" Applied Sciences 11, no. 18: 8408. https://doi.org/10.3390/app11188408
APA StyleRoh, Y.-H., Byon, S. M., & Lee, Y. (2021). Numerical Analysis of Edge Cracking in High-Silicon Steel during Cold Rolling with 3D Fracture Locus. Applied Sciences, 11(18), 8408. https://doi.org/10.3390/app11188408





