Influence of Frequency and Distance on Acoustic Emission Velocity Propagating in Various Dielectrics
Abstract
1. Introduction
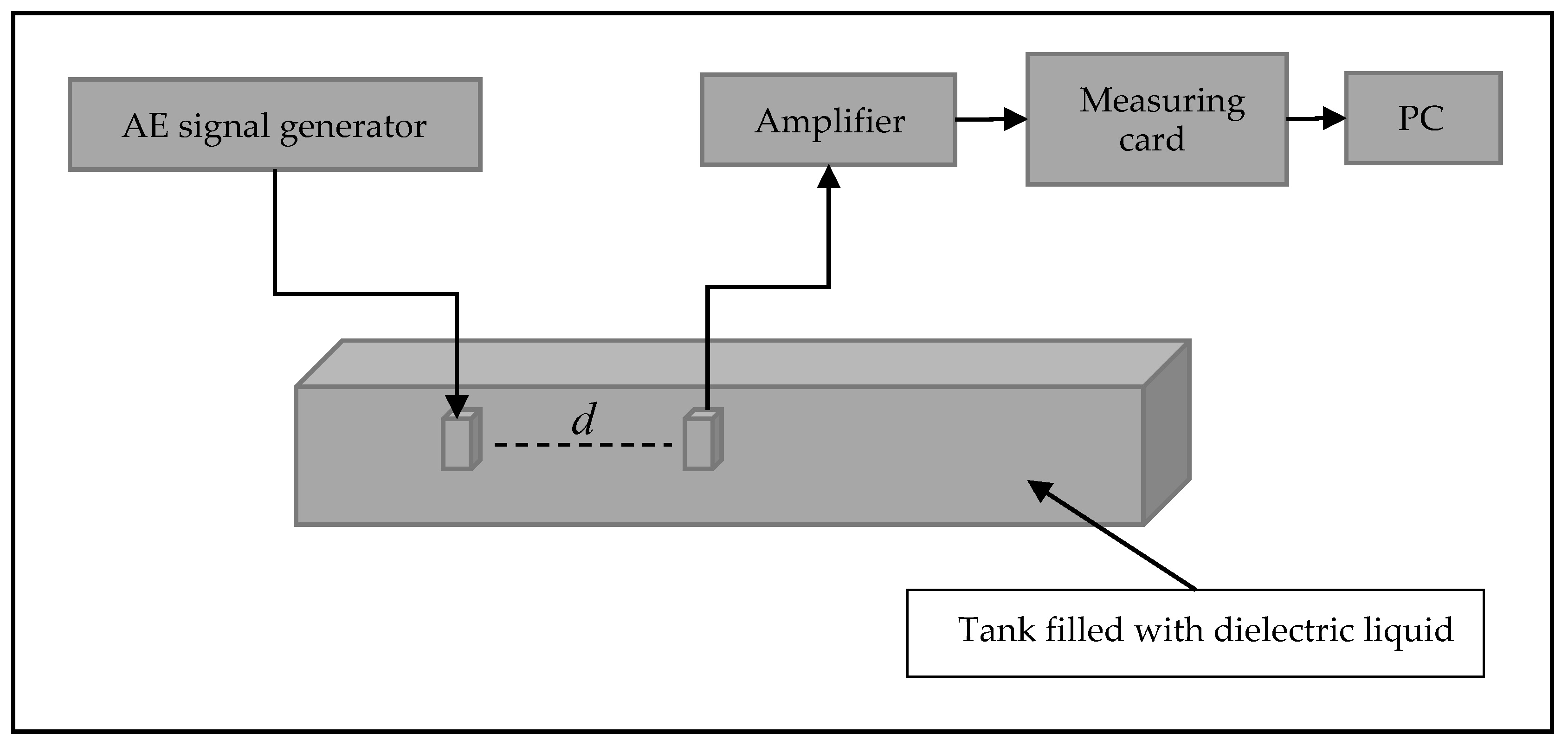
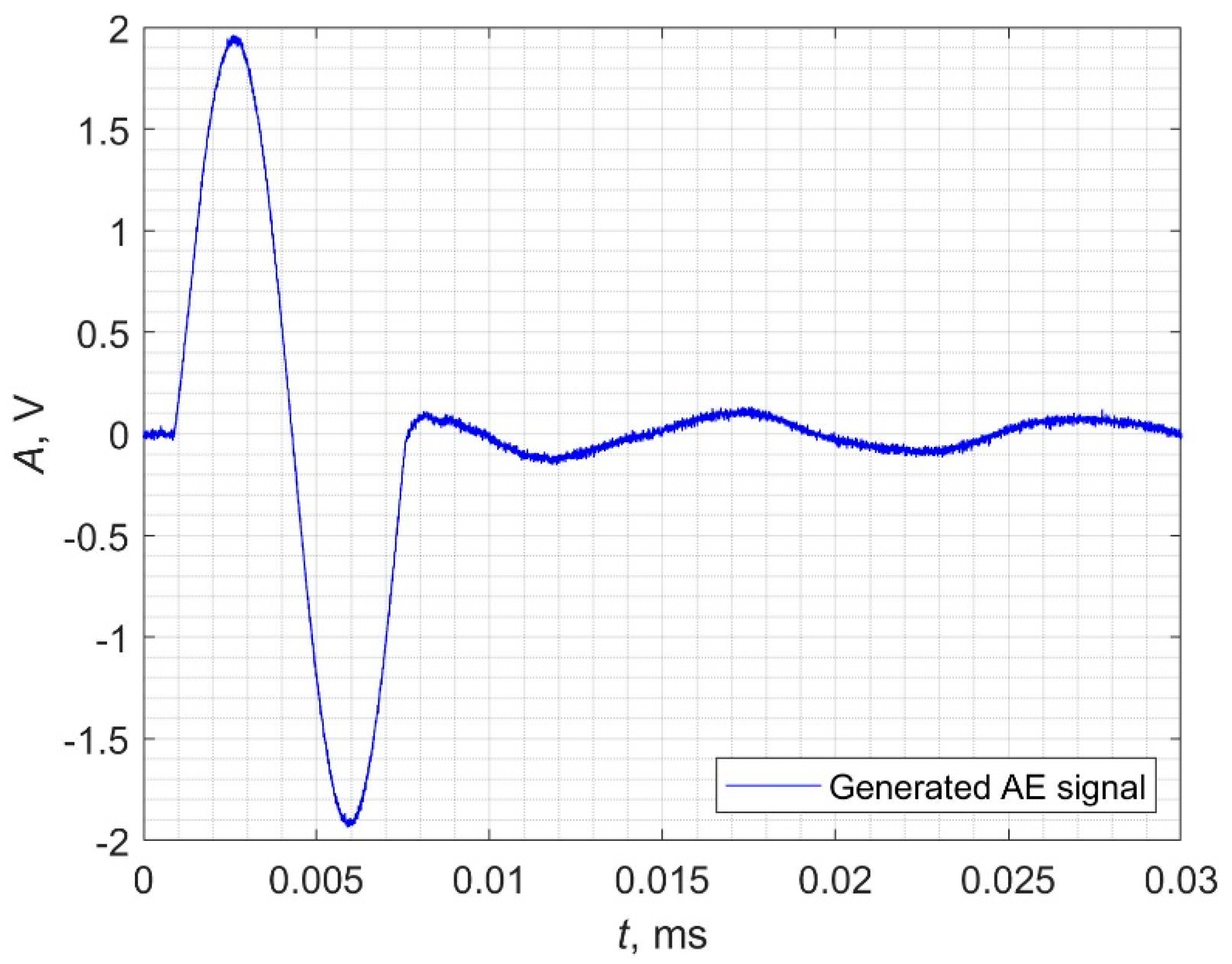
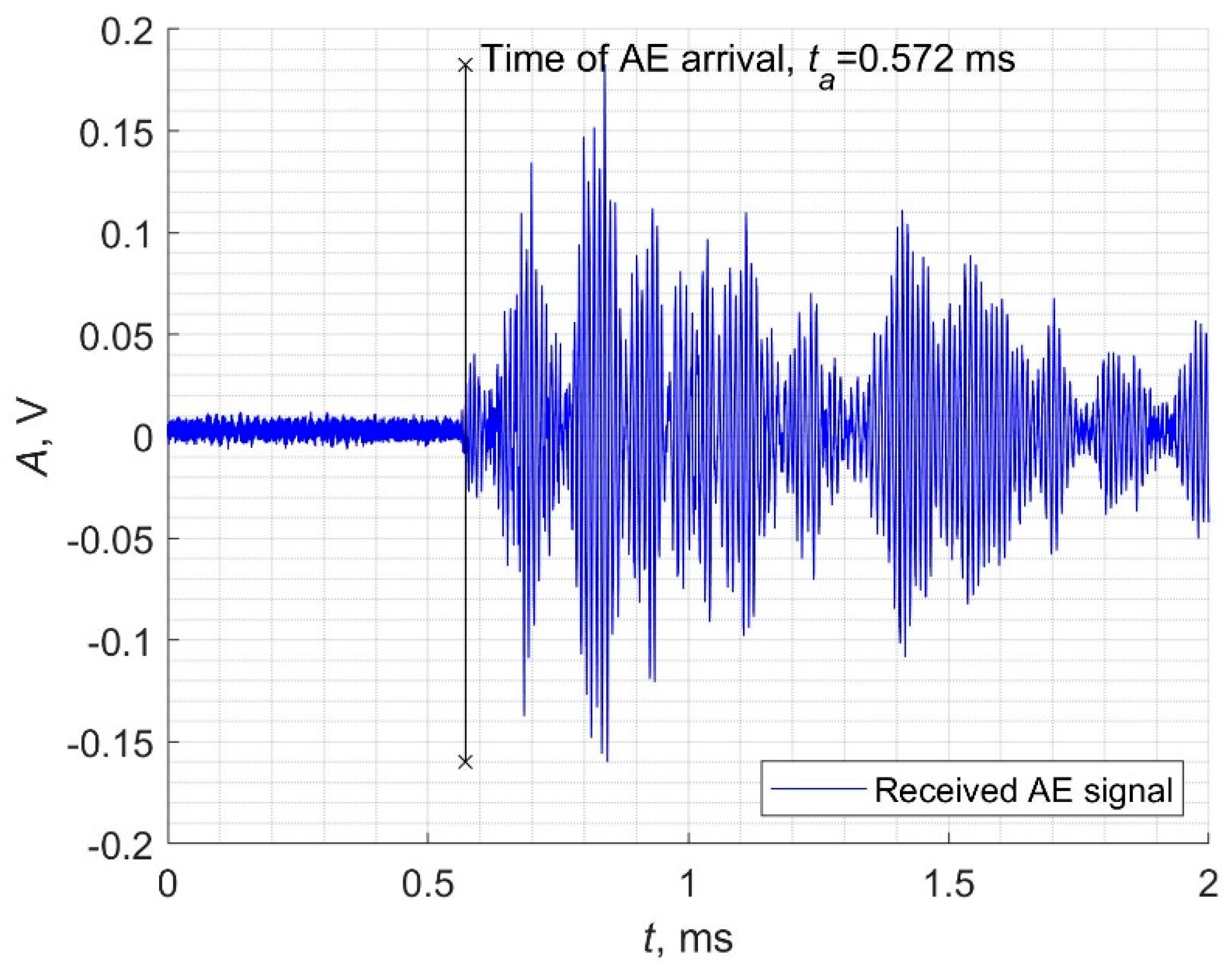
2. Materials and Methods
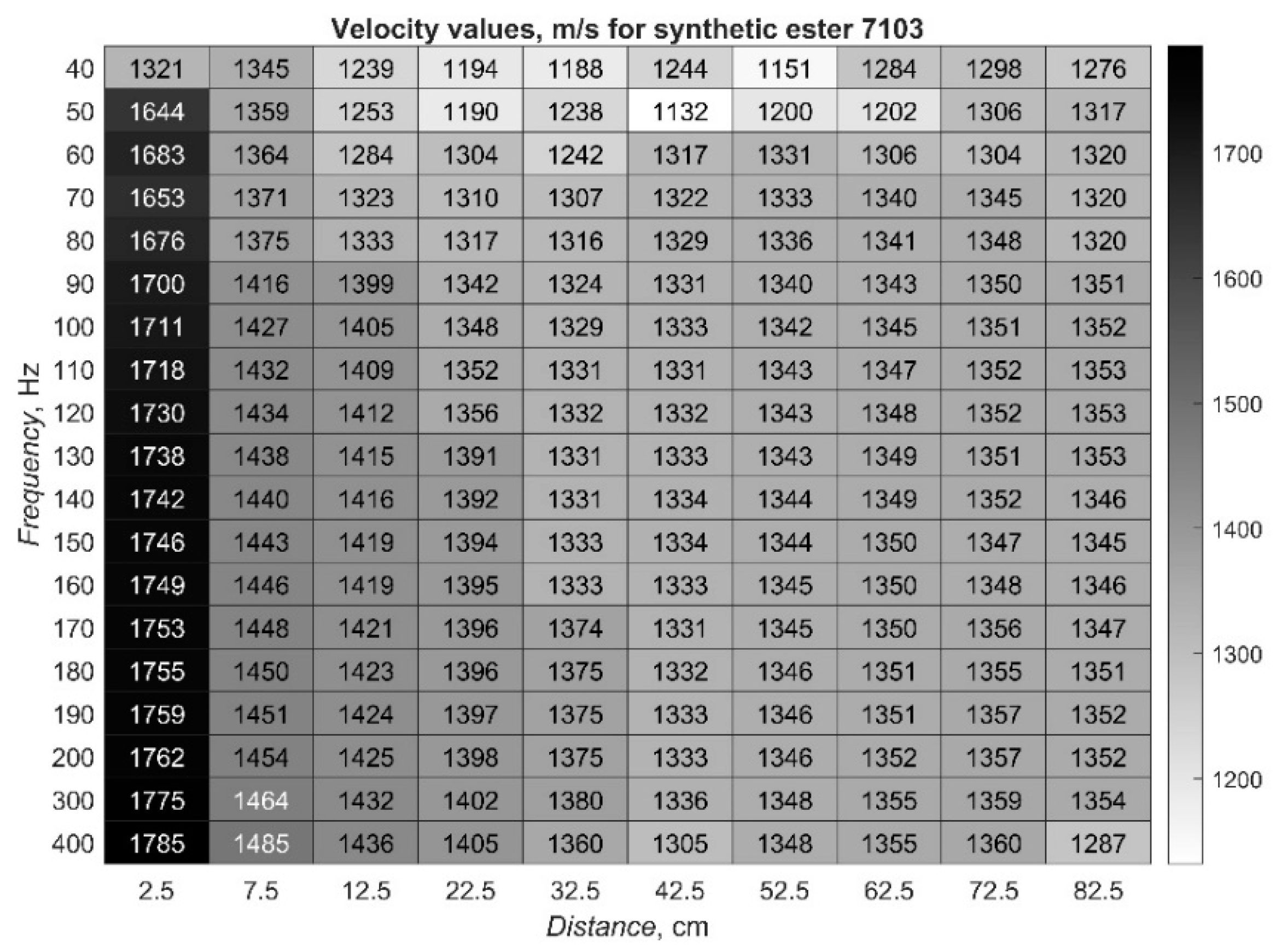
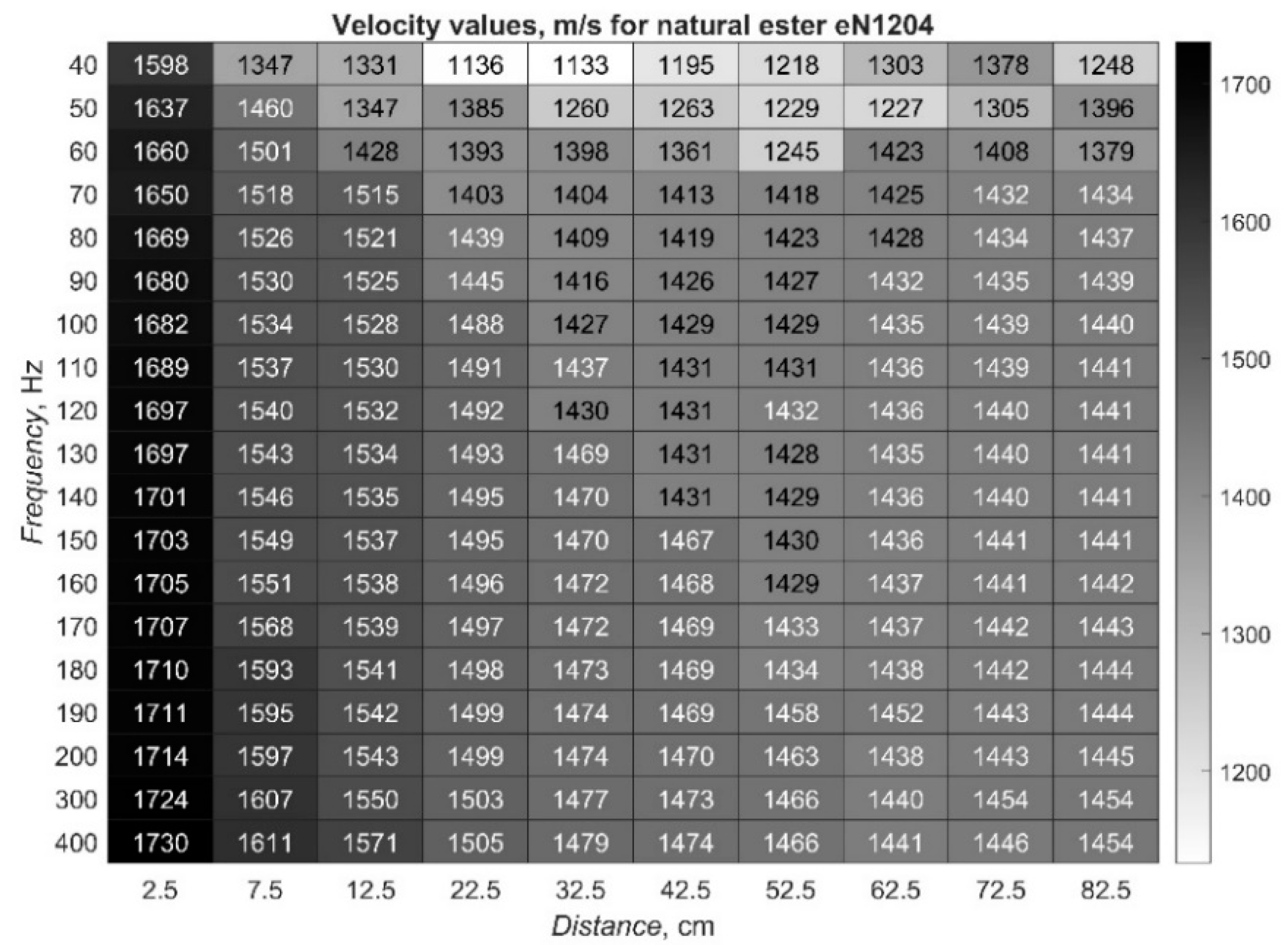
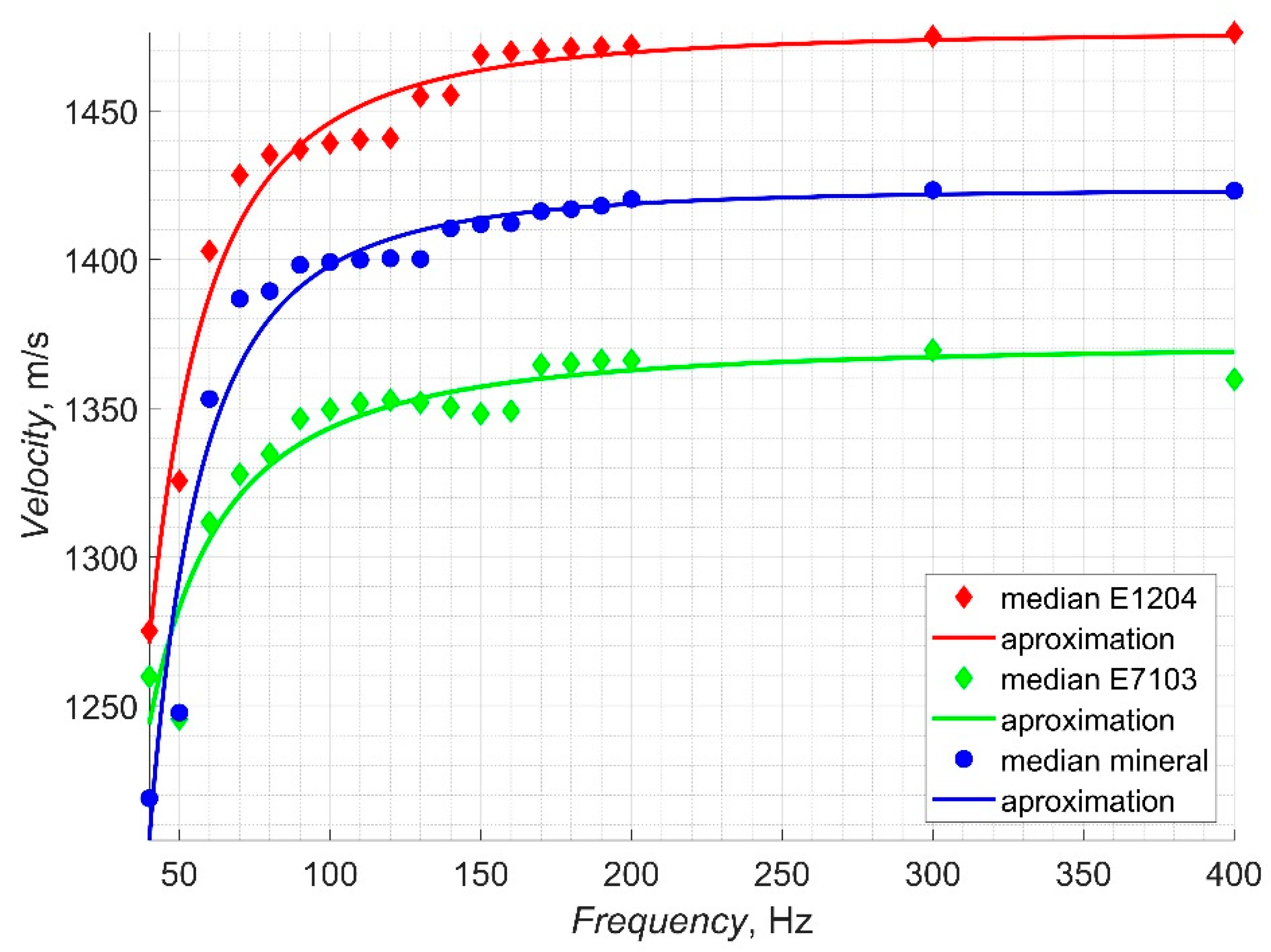
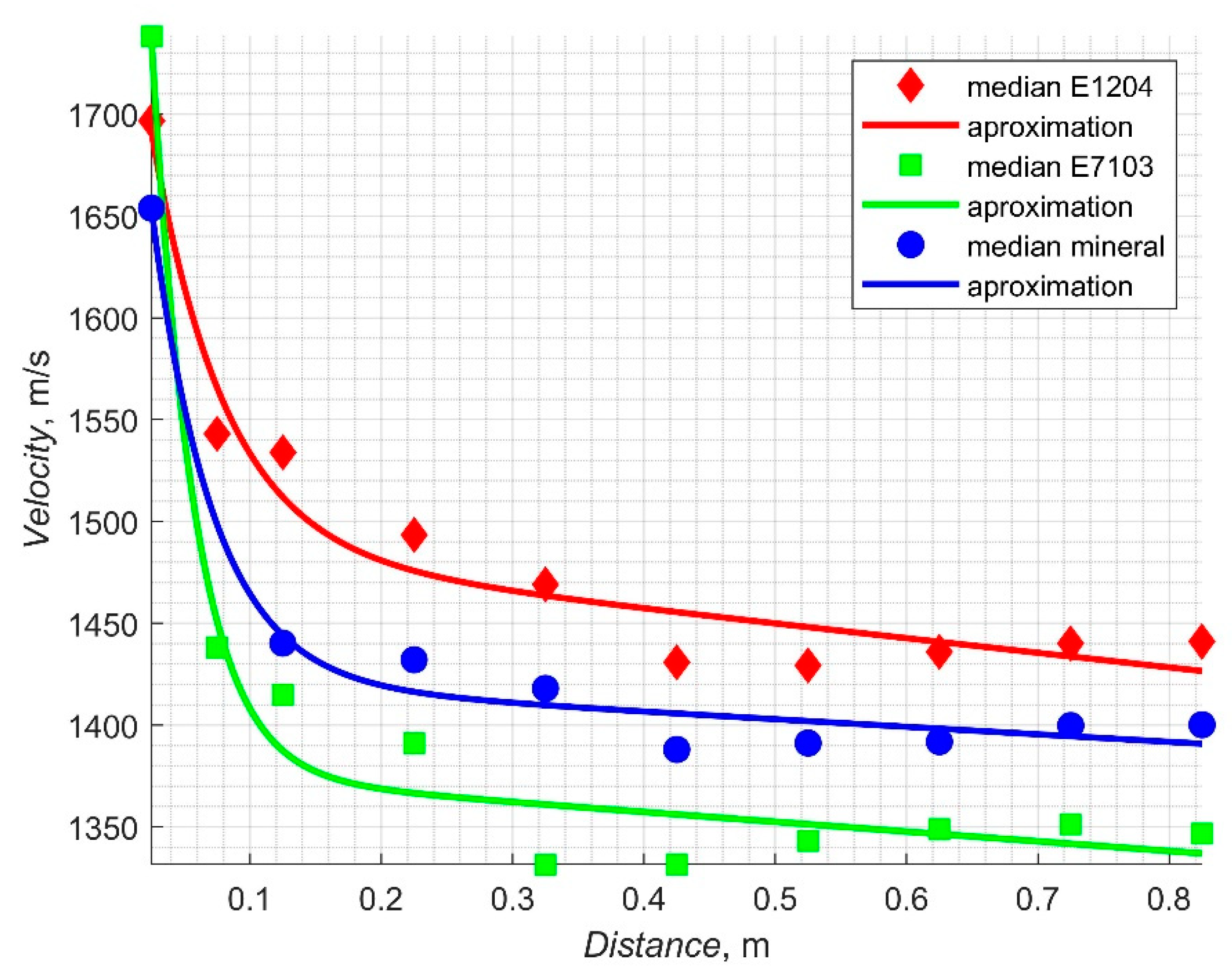
3. Results Analysis and Discussion
Funding
Conflicts of Interest
References
- Pompili, M.; Bartnikas, R. On partial discharge measurement in dielectric liquids. IEEE Trans. Dielectr. Electr Insul. 2012, 19, 1476–1481. [Google Scholar] [CrossRef]
- Pompili, M. Partial discharge measurements in dielectric liquids. In Proceedings of the 2008 IEEE International Conference on Dielectric Liquids, Poitiers, France, 30 June–3 July 2008; pp. 1–7. [Google Scholar] [CrossRef]
- Han, Y.; Song, Y.H. Condition monitoring techniques for electrical equipment: A literature survey. IEEE Power Eng. Rev. 2002, 22, 59. [Google Scholar] [CrossRef]
- Kozioł, M.; Boczar, T.; Nagi, Ł. Identification of electrical discharge forms, generated in insulating oil, using the optical spectrophotometry method. IET Sci. Meas. Technol. 2019, 13, 416–425. [Google Scholar] [CrossRef]
- Koziol, M.; Nagi, L. Analysis of Optical Radiation Spectra Emitted by Electrical Discharges, Generated by Different Configuration Types of High Voltage Electrodes. In Proceedings of the 2018 IEEE 2nd International Conference on Dielectrics (ICD), Budapest, Hungary, 1–5 July 2018; pp. 1–4. [Google Scholar] [CrossRef]
- Kozioł, M.; Nagi, Ł.; Kunicki, M.; Urbaniec, I. Radiation in the Optical and UHF Range Emitted by Partial Discharges. Energies 2019, 12, 4334. [Google Scholar] [CrossRef]
- Siegel, M.; Beltle, M.; Tenbohlen, S.; Coenen, S. Application of UHF sensors for PD measurement at power transformers. IEEE Trans. Dielectr. Electr. Insul. 2017, 24, 331–339. [Google Scholar] [CrossRef]
- Liu, Y.P.; Wang, H.B.; Chen, W.J.; Yang, Y.J.; Jian, T. Test study on corona onset voltage of UHV transmission lines based on UV detection. In Proceedings of the 2008 International Conference on High Voltage Engineering and Application, ICHVE 2008, Chongqing, China, 9–12 November 2008; pp. 387–390. [Google Scholar] [CrossRef]
- Kunicki, M. Variability of the UHF signals generated by partial discharges in mineral oil. Sensors (Switzerland) 2019, 19, 1392. [Google Scholar] [CrossRef] [PubMed]
- Kunicki, M.; Nagi, L. Correlation analysis of partial discharge measurement results. In Proceedings of the 2017 IEEE International Conference on Environment and Electrical Engineering and 2017 IEEE Industrial and Commercial Power Systems Europe (EEEIC/I&CPS Europe), Milan, Italy, 6–9 June 2017; pp. 1–6. [Google Scholar] [CrossRef]
- Boczar, T.; Witkowski, P.; Borucki, S.; Cichon, A. Solving a set of spherical equations for localization of partial discharges by acoustic emission method. Acta Phys. Pol. A 2015, 128, 299–305. [Google Scholar] [CrossRef]
- Boczar, T.; Witkowski, P. Partial discharges localization using triangular method with Rogowski coil. Prz. Elektrotechniczny 2010, 86, 70–73. [Google Scholar]
- Markalous, S.; Tenbolhen, S.; Feser, K. Detection and Location of Partial Discharges in Power Transformers using acoustic and electromagnetic signals. IEEE Trans. Dielectr. Electr. Insul. 2008, 15, 1576–1583. [Google Scholar] [CrossRef]
- Hekmati, A.; Hekmati, R. Optimum acoustic sensor placement for partial discharge allocation in transformers. IET Sci. Meas. Technol. 2017, 11, 581–589. [Google Scholar] [CrossRef]
- Antony, D.; Punekar, G.S. Noniterative Method for Combined Acoustic-Electrical Partial Discharge Source Localization. IEEE Trans. Power Deliv. 2018, 33, 1679–1688. [Google Scholar] [CrossRef]
- Wang, Q.; Kundur, D.; Yuan, H.; Liu, Y.; Lu, J.; Ma, Z. Noise Suppression of Corona Current Measurement From HVdc Transmission Lines. IEEE Trans. Instrum. Meas. 2016, 65, 264–275. [Google Scholar] [CrossRef]
- C57.127TM-2007. IEEE Guide for the Detection and Location of Acoustic Emissions from Partial Discharges in Oil-Immersed Power Transformers and Reactors; IEEE Std: Piscataway, NJ, USA, 31 August 2007. [Google Scholar] [CrossRef]
- Li, J.; Qin, L. Study on Acoustic Emission Localization of Concrete Using Modified Velocity. Adv. Civ. Eng. 2019. [Google Scholar] [CrossRef]
- Rajab, A.; Tsuchie, M.; Kozako, M.; Hikita, M.; Suzuki, T. PD properties and gases generated by palm fatty acids esters (PFAE) oil. In Proceedings of the 2016 IEEE International Conference on Dielectrics, ICD 2016, Montpellier, France, 3–7 July 2016; Volume 2, pp. 816–819. [Google Scholar] [CrossRef]
- Pompili, M.; Mazzetti, C.; Bartnikas, R. Comparative PD pulse burst characteristics of transformer type natural and synthetic ester fluids and mineral oils. IEEE Trans. Dielectr. Electr. Insul. 2009, 16, 1511–1518. [Google Scholar] [CrossRef]
- Jing, Y.; Timoshkin, I.V.; Wilson, M.P.; Given, M.J.; Macgregor, S.J.; Wang, T.; Lehr, J. Dielectric properties of natural ester, synthetic ester midel 7131 and mineral oil diala, D. IEEE Trans. Dielectr. Electr. Insul. 2014, 21, 644–652. [Google Scholar] [CrossRef]
- Wotzka, D.; Koziol, M.; Nagi, L. Measurement of acoustic emission velocity in natural ester. In Proceedings of the 20th International Scientific Conference on Electric Power Engineering, EPE 2019, Kouty nad Desnou, Czech Republic, 15–17 May 2019; Institute of Electrical and Electronics Engineers Inc.: Danvers, MA, USA, 2019. [Google Scholar] [CrossRef]
- ORLEN OIL TRAFO EN Product Brochure. Available online: https://www.orlenoil.pl/PL/NaszaOferta/Produkty/Strony/produkt.aspx?produkt=ORLEN_OIL_TRAFO_EN (accessed on 8 May 2020).
- MIDEL eN 1204 Product Brochure. Available online: https://www.midel.com/downloads/ (accessed on 8 May 2020).
- MIDEL 7131 Product Brochure. Available online: https://www.midel.com/downloads/ (accessed on 8 May 2020).
- PI Piezoelectric Discs Datasheet. Available online: https://www.piceramic.com/en/products/piezoceramic-components/disks-rods-and-cylinders/piezoelectric-discs-1206710/#downloads (accessed on 8 May 2020).
- Product Brochure—Hydrophones—Types 8103, 8104, 8105 and 8106. Available online: https://www.bksv.com/media/doc/bp0317.pdf (accessed on 8 May 2020).

| Property | Density at 20 °C (g/cm3) | Viscosity at 40 °C (mm2/s) | Water content | Breakdown Voltage (kV) |
|---|---|---|---|---|
| Test method | ISO 3675 | ISO 3104 | IEC 60814 | IEC 60156/ASTM D877/IEC 60296 |
| Natural ester | 0.92 | 37 | 50 (mg/kg) | ≥ 30 |
| Synthetic ester | 0.97 | 29 | 50 (mg/kg) | ≥ 75 |
| Mineral oil | 0.87 | 9.9 | 50 (m/m) | ≥ 69 |
| Property | Natural Ester | Synthetic Ester | Mineral Oil |
|---|---|---|---|
| Coeff. a1 | −424,600 | −53,840 | −1,182,000 |
| Coeff. a2 | −2.069 | −1.638 | −2.329 |
| Coeff. a3 | 1477 | 1372 | 1424 |
| SSE | 1565 | 2251 | 3324 |
| Rsquare | 0.968 | 0.892 | 0.942 |
| Adjrsquare | 0.965 | 0.879 | 0.935 |
| RMSE | 9.89 | 11.86 | 14.41 |
| Property | Natural Ester | Synthetic Ester | Mineral Oil |
| Coeff. b1 | 323.4 | 778.3 | −398.4 |
| Coeff. b2 | −17.86 | −30.69 | −21.47 |
| Coeff. b3 | 1487 | 1377 | 1422 |
| Coeff. b4 | −0.05 | −0.03557 | −0.02653 |
| SSE | 2615 | 3244 | 915 |
| Rsquare | 0.958 | 0.976 | 0.983 |
| Adjrsquare | 0.937 | 0.964 | 0.974 |
| RMSE | 20.87 | 23.25 | 13.53 |
© 2020 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wotzka, D. Influence of Frequency and Distance on Acoustic Emission Velocity Propagating in Various Dielectrics. Appl. Sci. 2020, 10, 3305. https://doi.org/10.3390/app10093305
Wotzka D. Influence of Frequency and Distance on Acoustic Emission Velocity Propagating in Various Dielectrics. Applied Sciences. 2020; 10(9):3305. https://doi.org/10.3390/app10093305
Chicago/Turabian StyleWotzka, Daria. 2020. "Influence of Frequency and Distance on Acoustic Emission Velocity Propagating in Various Dielectrics" Applied Sciences 10, no. 9: 3305. https://doi.org/10.3390/app10093305
APA StyleWotzka, D. (2020). Influence of Frequency and Distance on Acoustic Emission Velocity Propagating in Various Dielectrics. Applied Sciences, 10(9), 3305. https://doi.org/10.3390/app10093305





