Numerical and Experimental Study on the Flow-Induced Noise Characteristics of High-Speed Centrifugal Pumps
Abstract
1. Introduction
2. Research Object and Methods
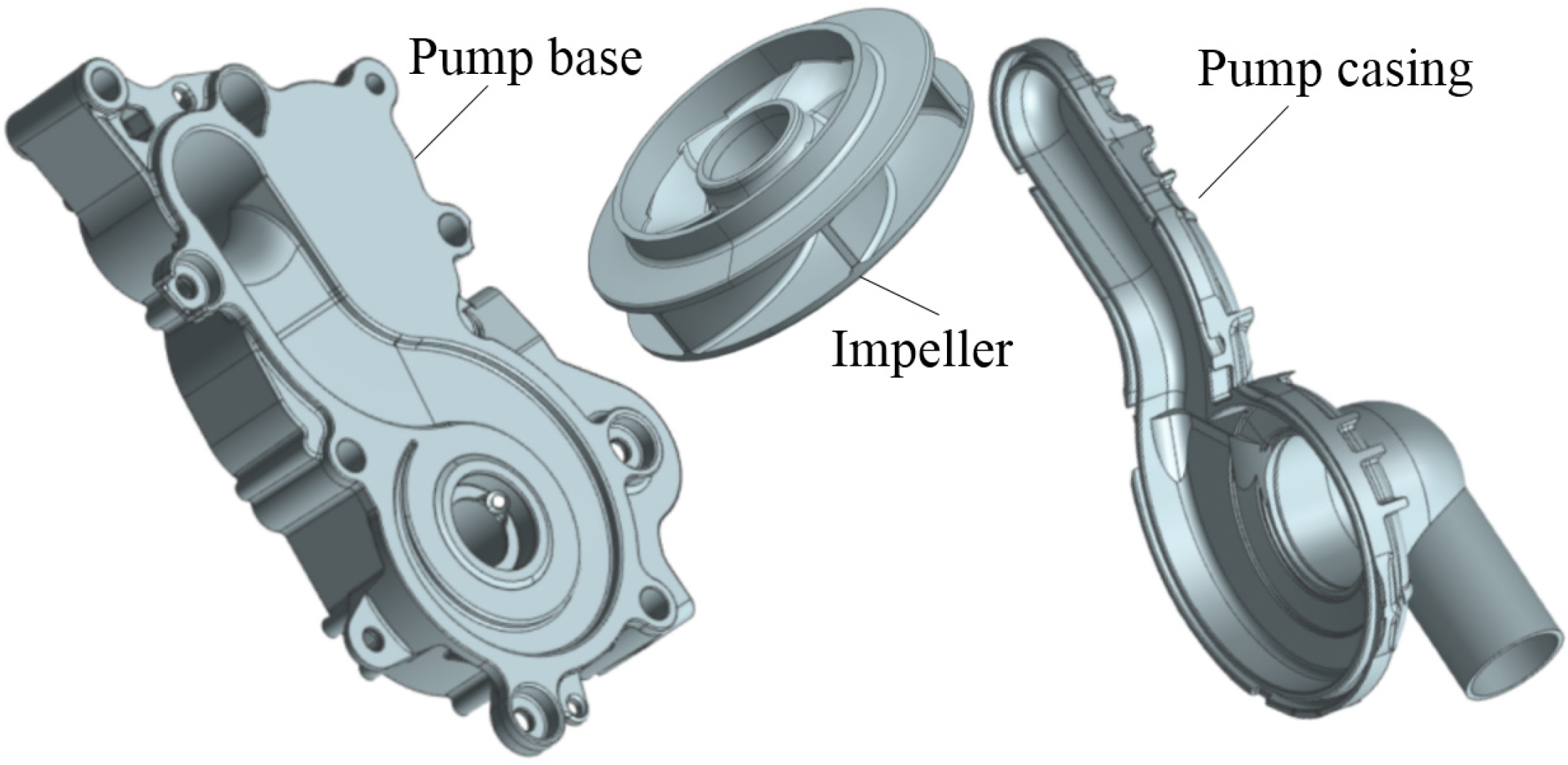
2.1. Pump Model
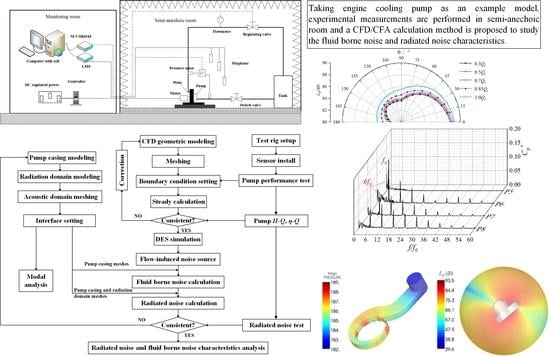
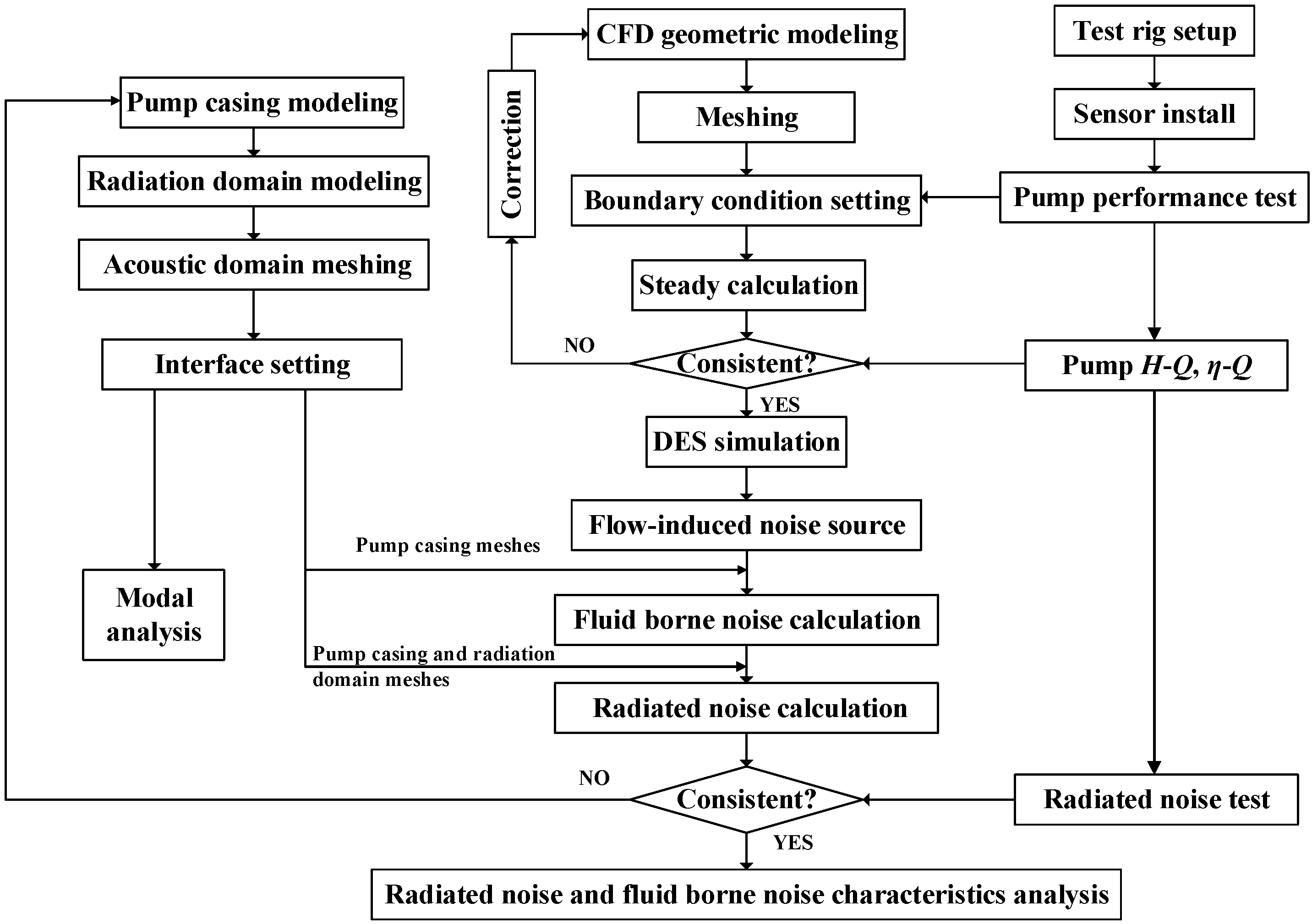
2.2. Theory of the Proposed CFD/CFA
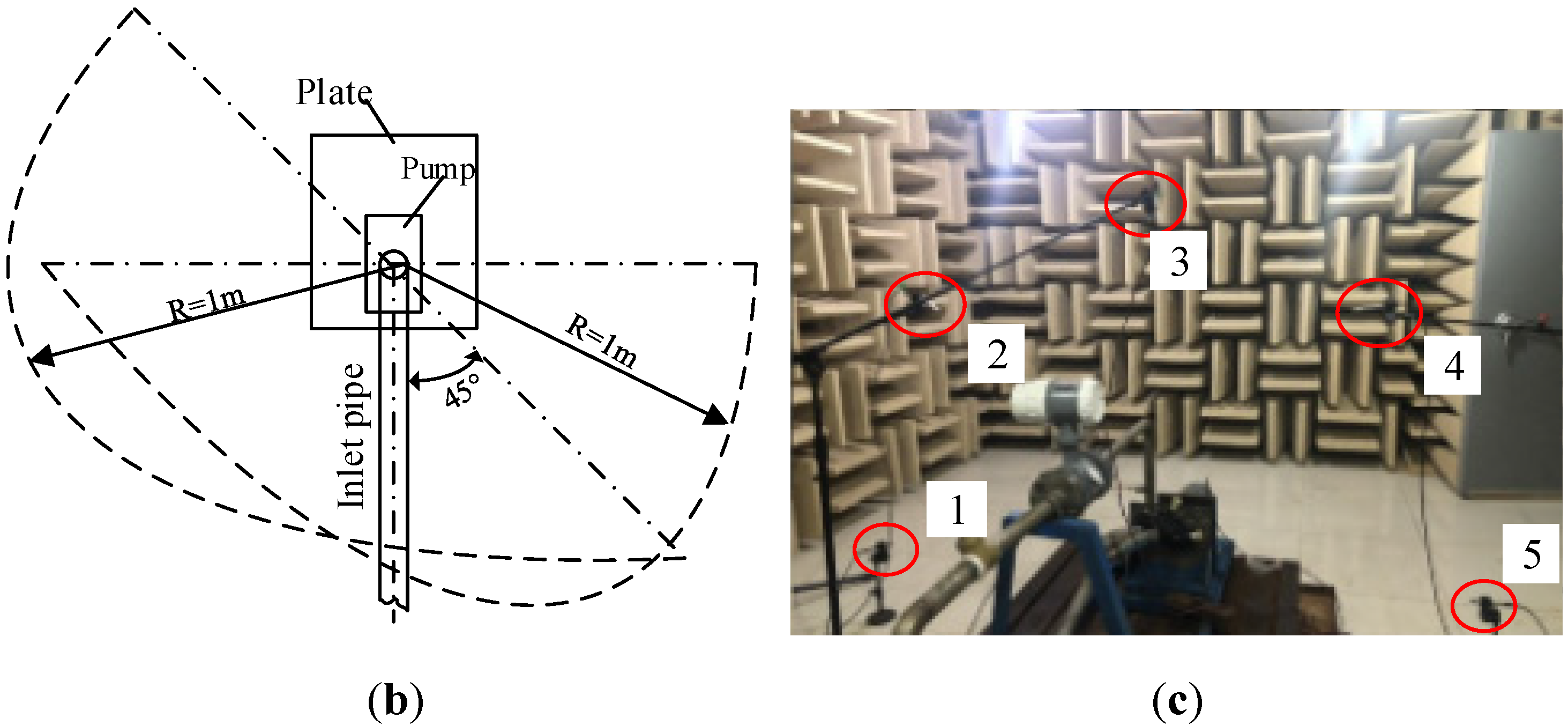
2.3. Experimental Support
2.4. Numerical Setups
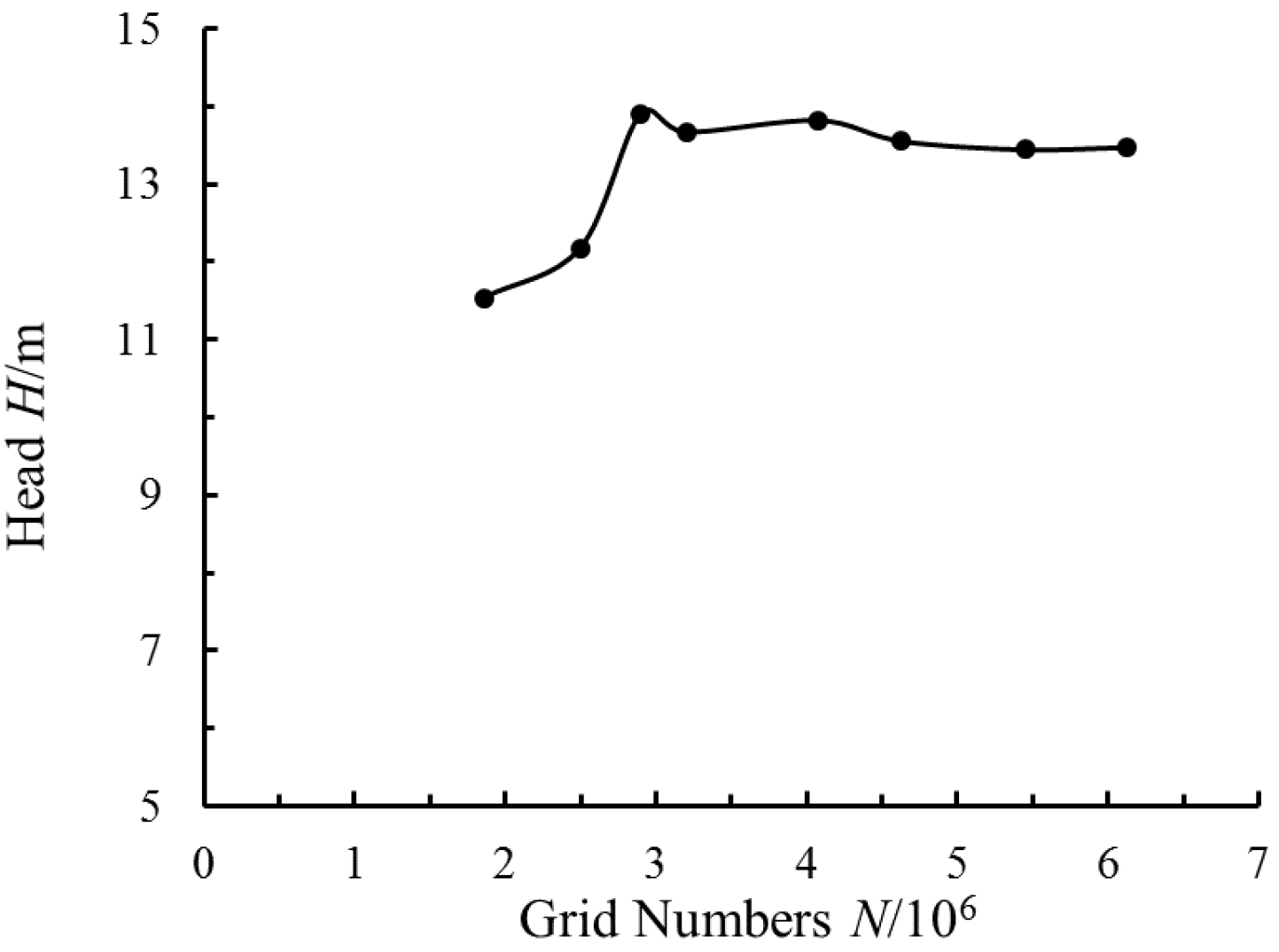
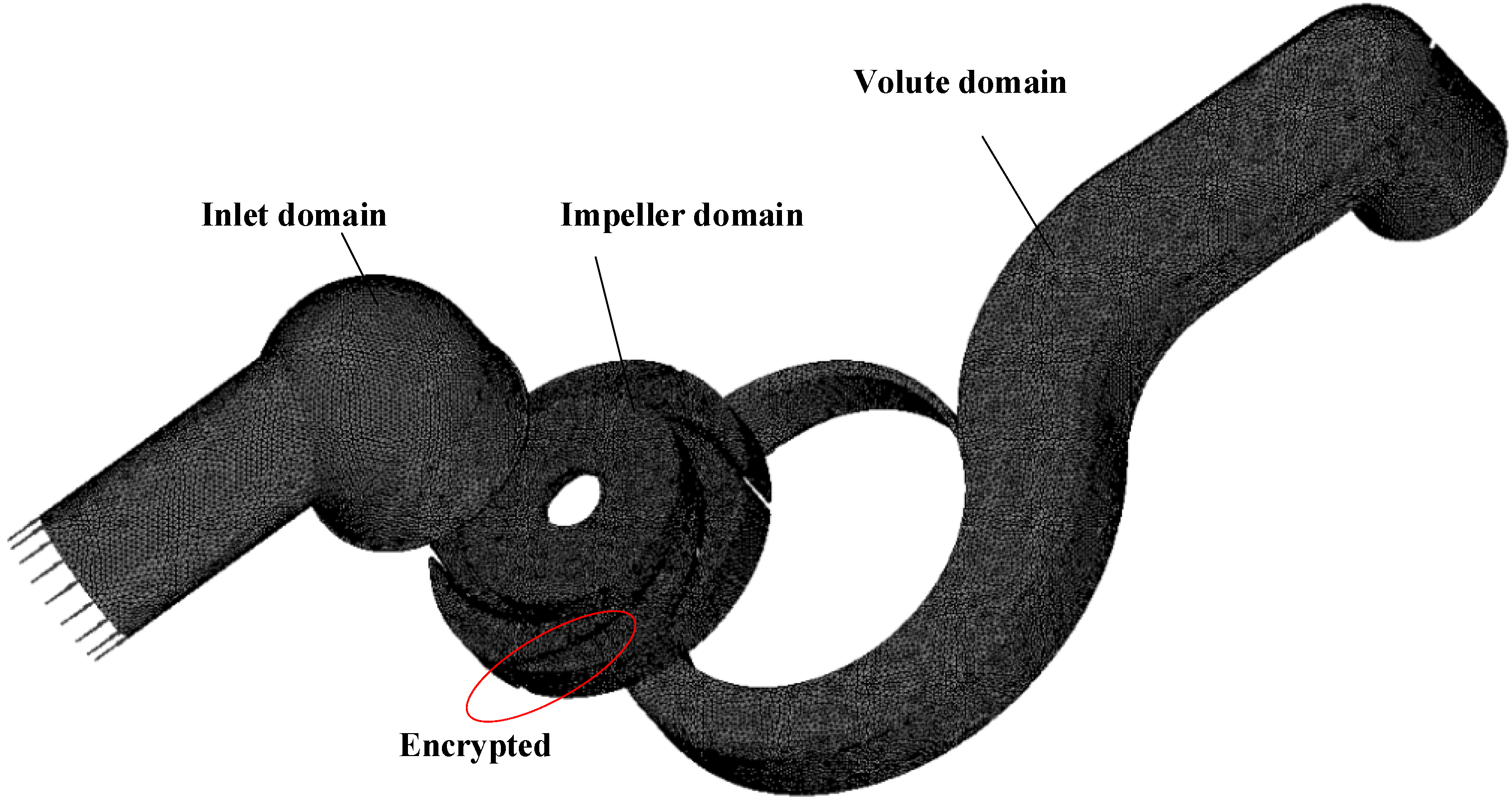
2.4.1. Computation Domain and Mesh Generation of the Flow Field
2.4.2. Boundary Conditions and Turbulence Model of the CFD
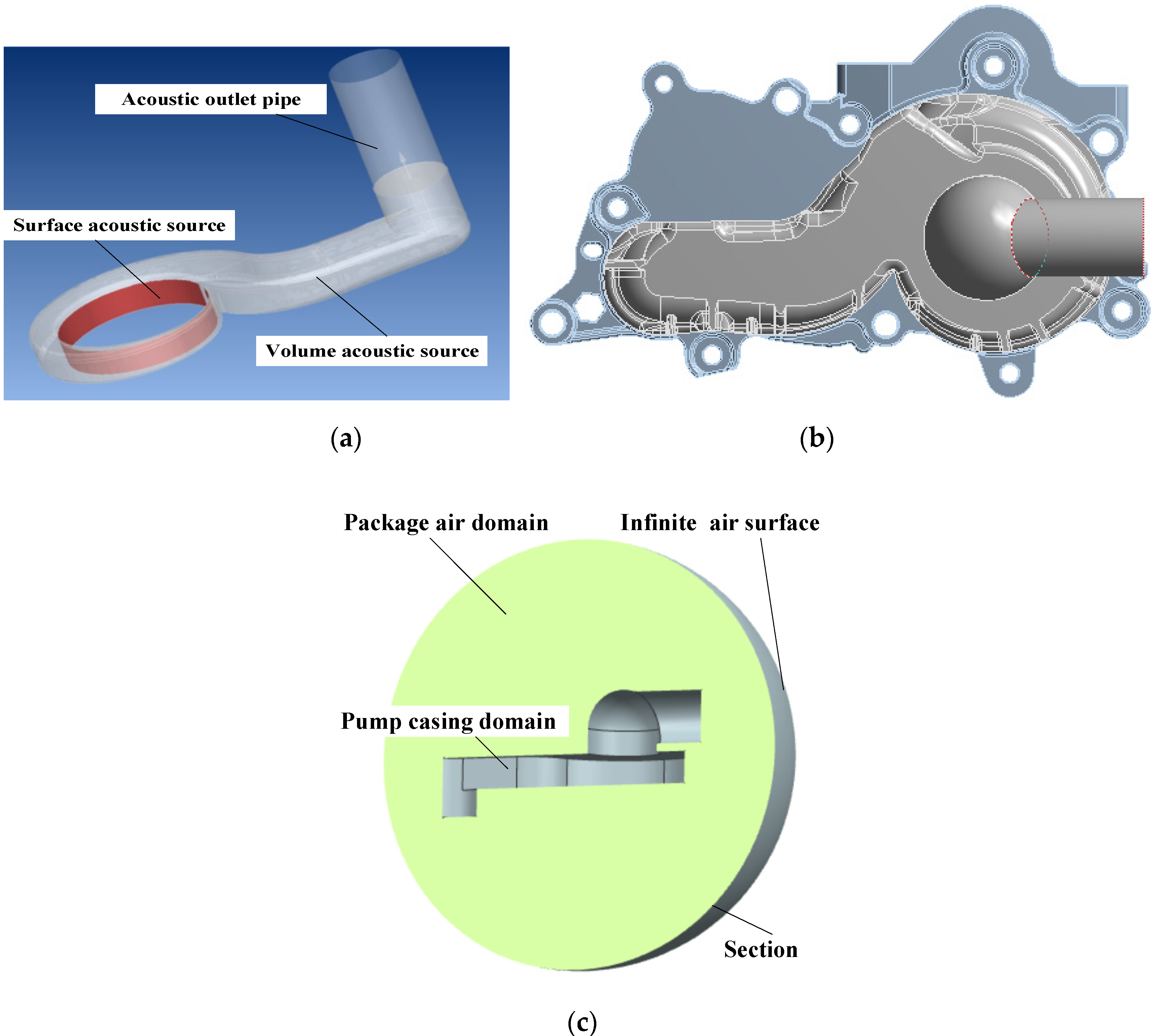
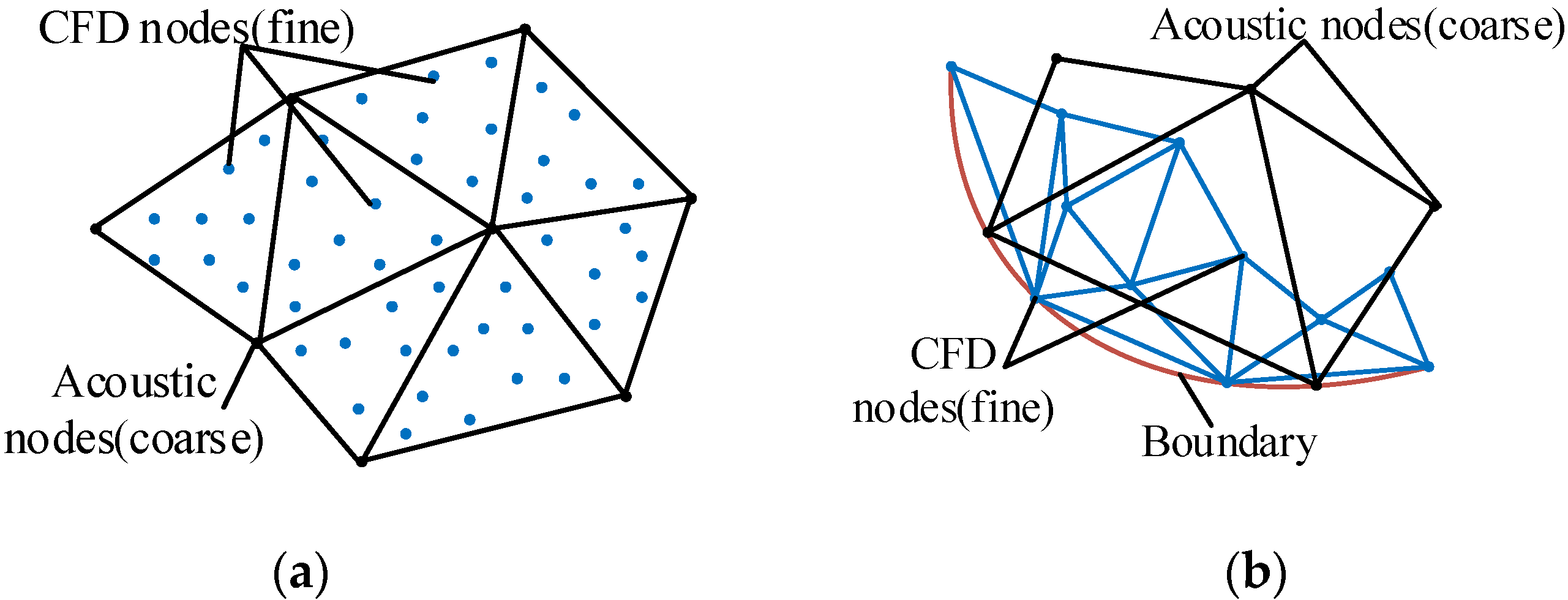
2.4.3. Computation setup of the acoustic field
3. Experimental Results
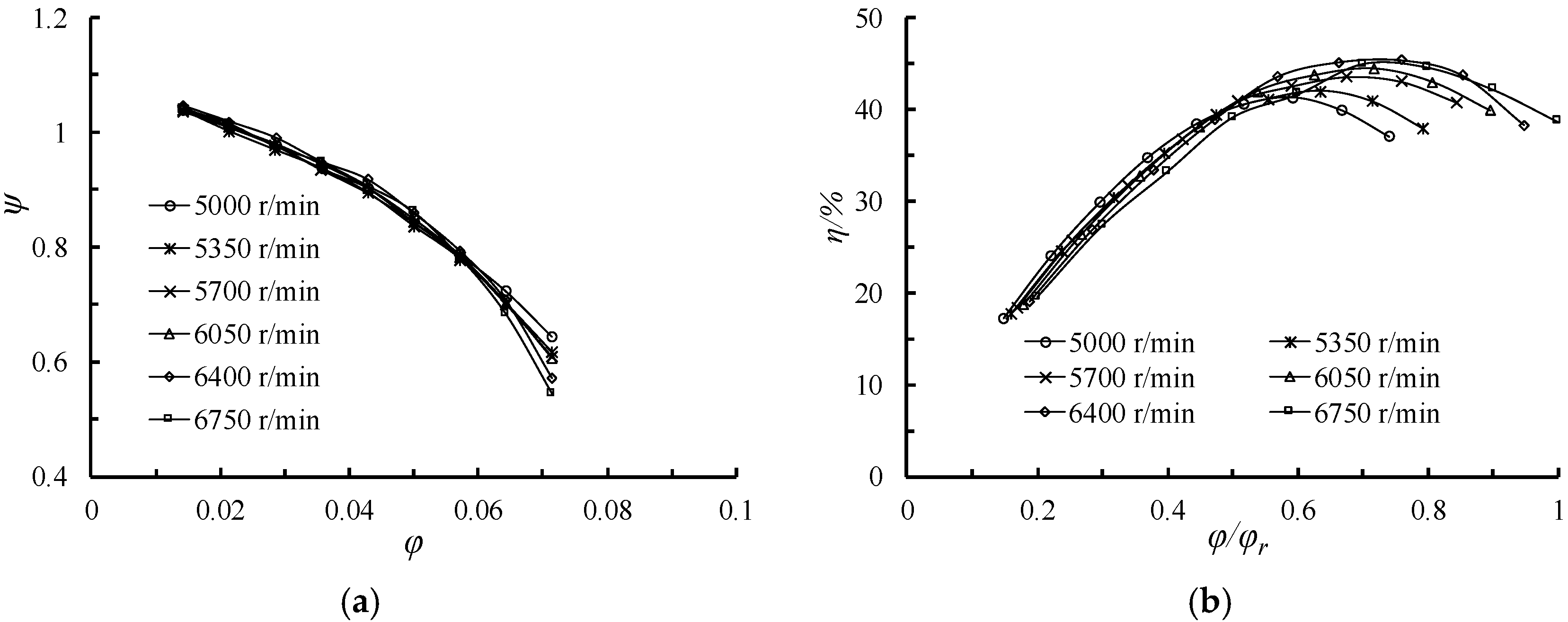
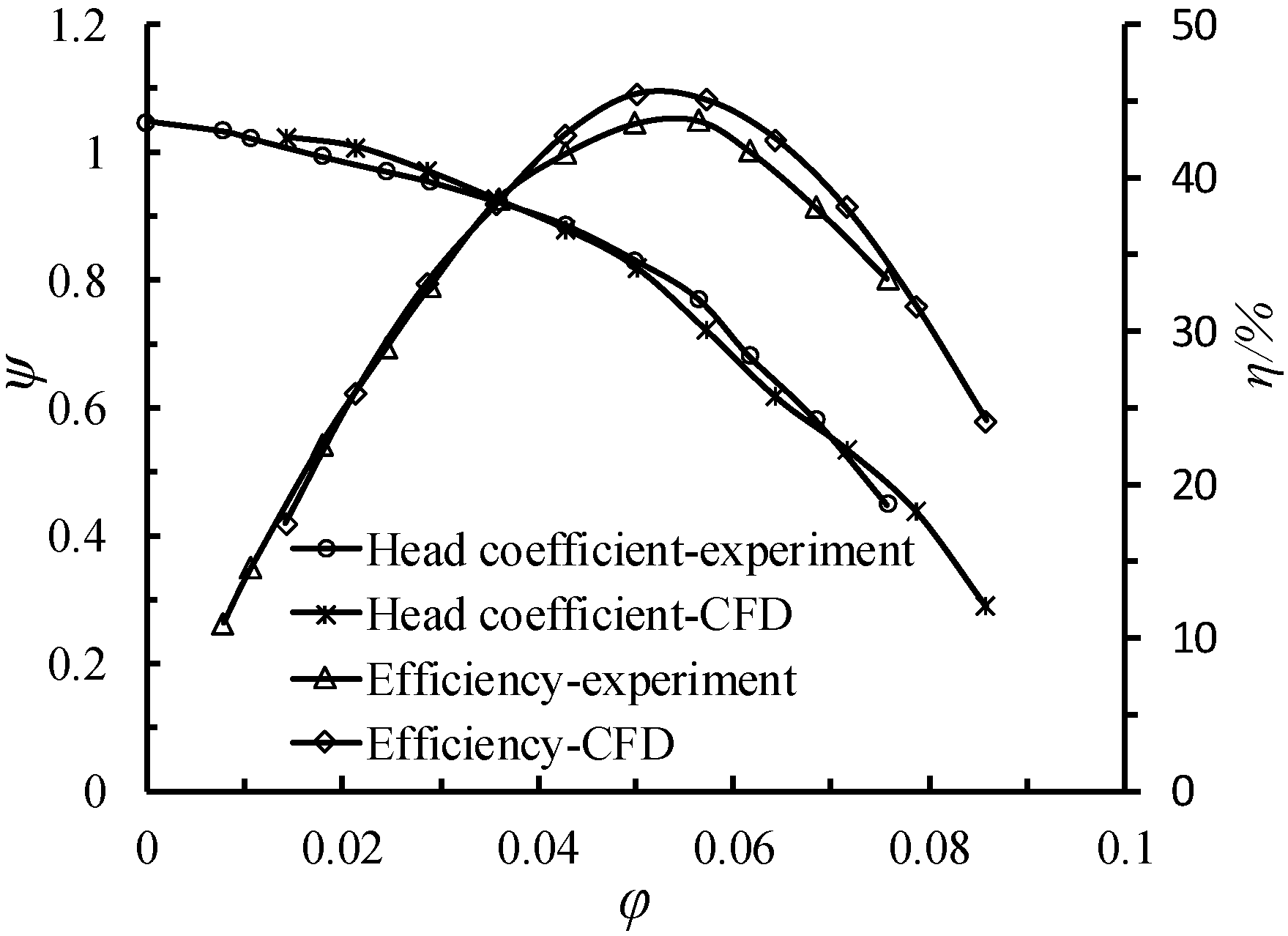
3.1. Overall Pump Performances
3.2. Analysis of Radiation Noise Characteristic
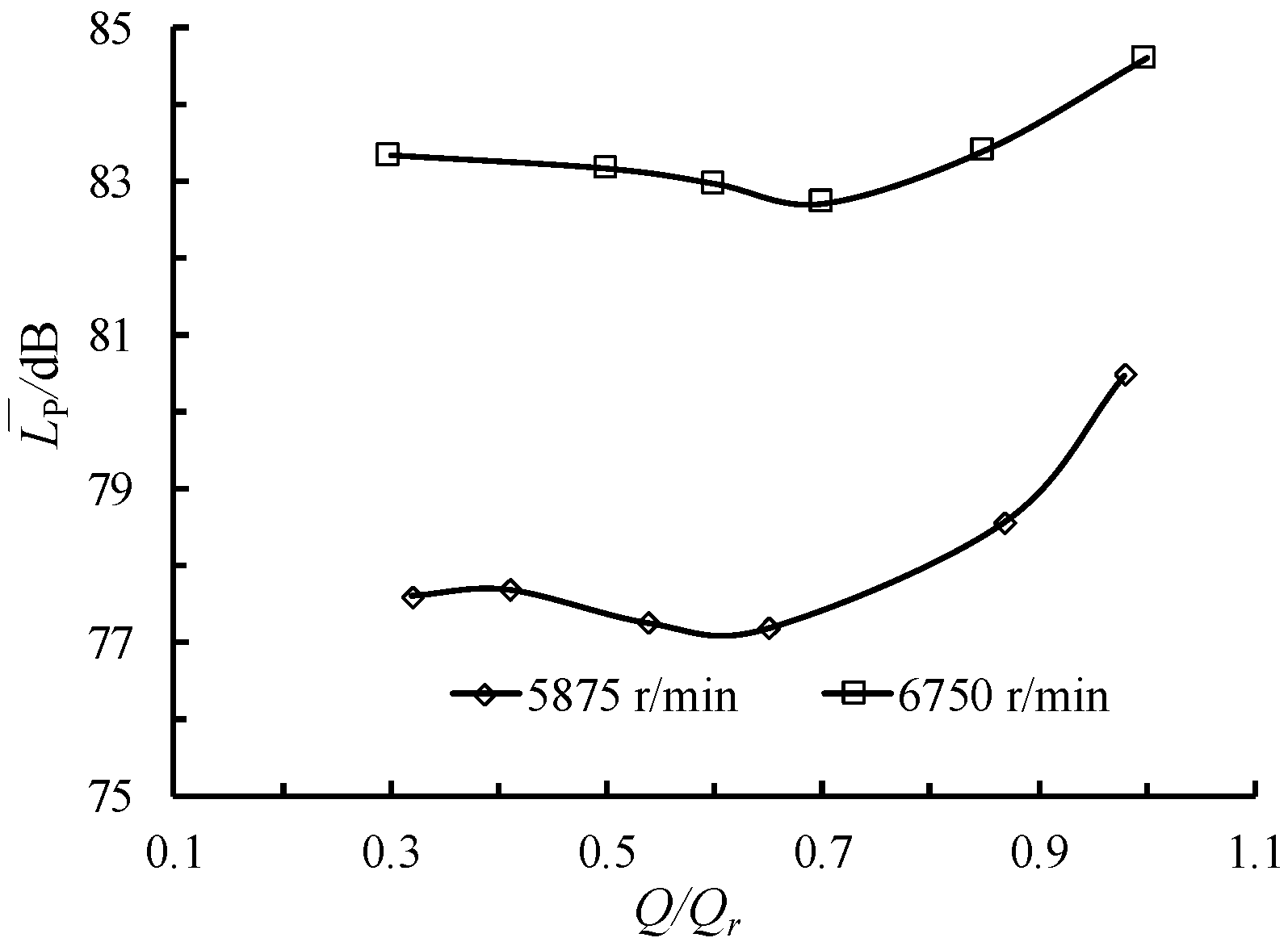
3.2.1. Sound Pressure Level at Different Flow Rates
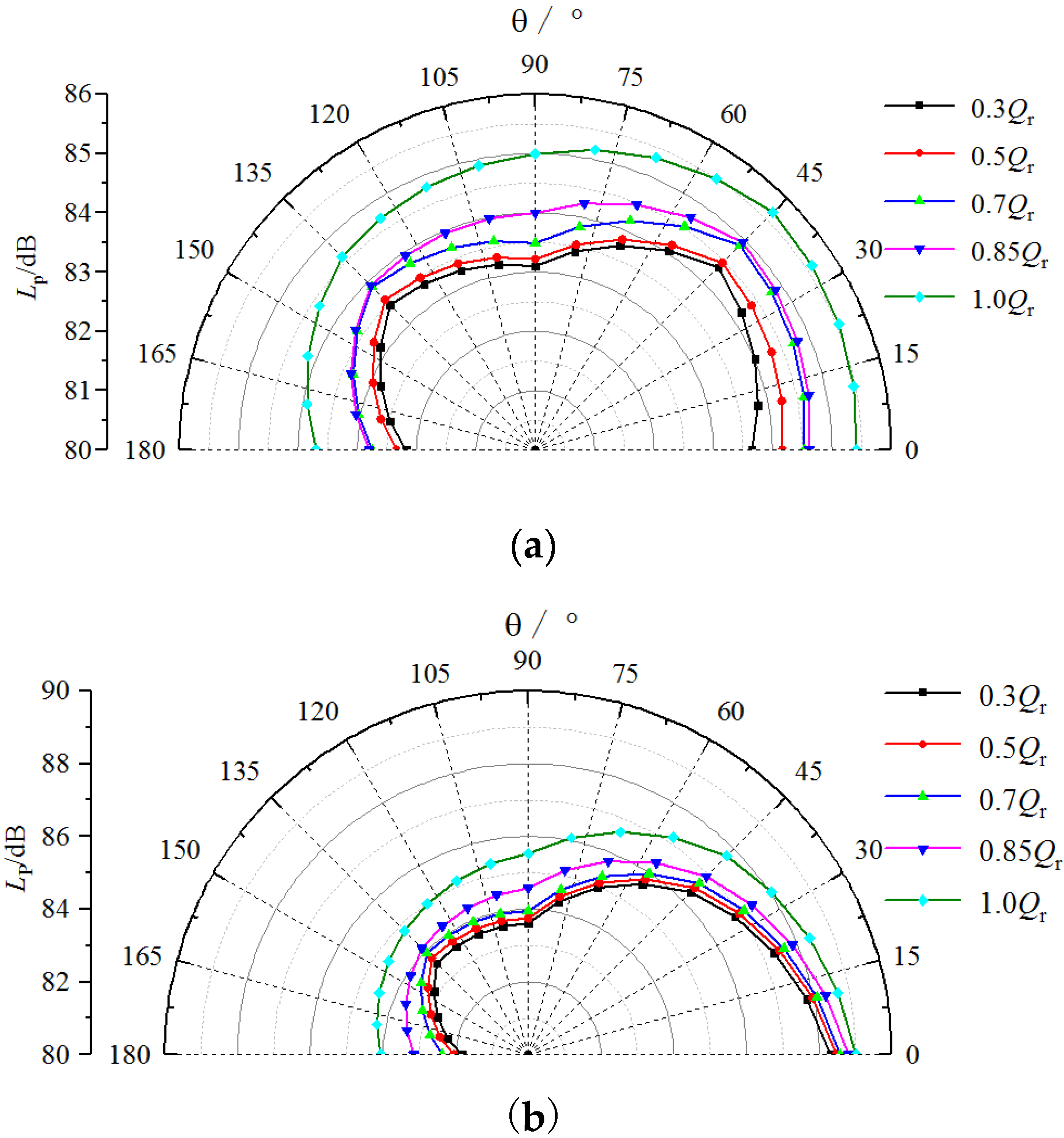
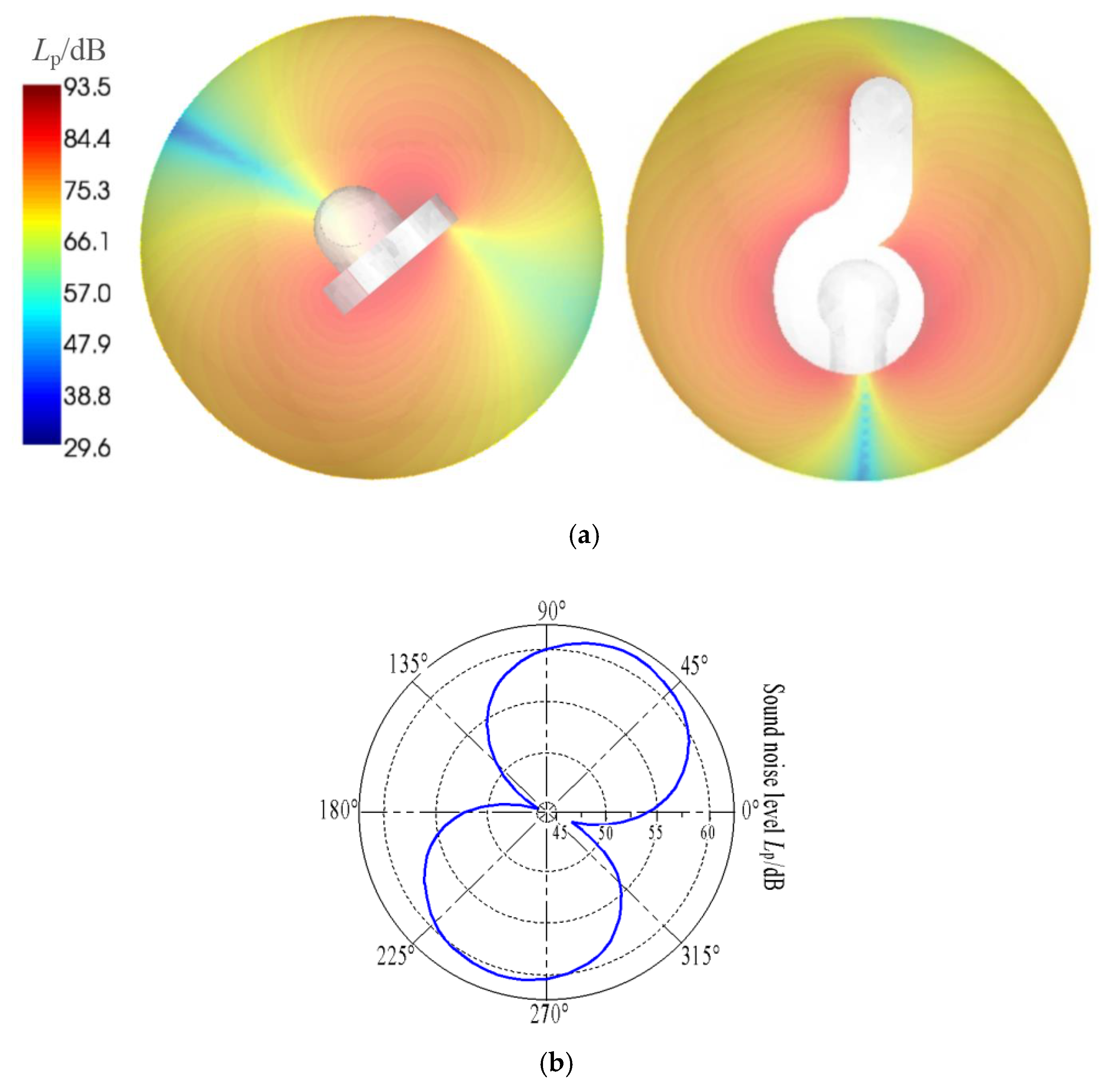
3.2.2. Acoustic Directivity at Different Flow Rates
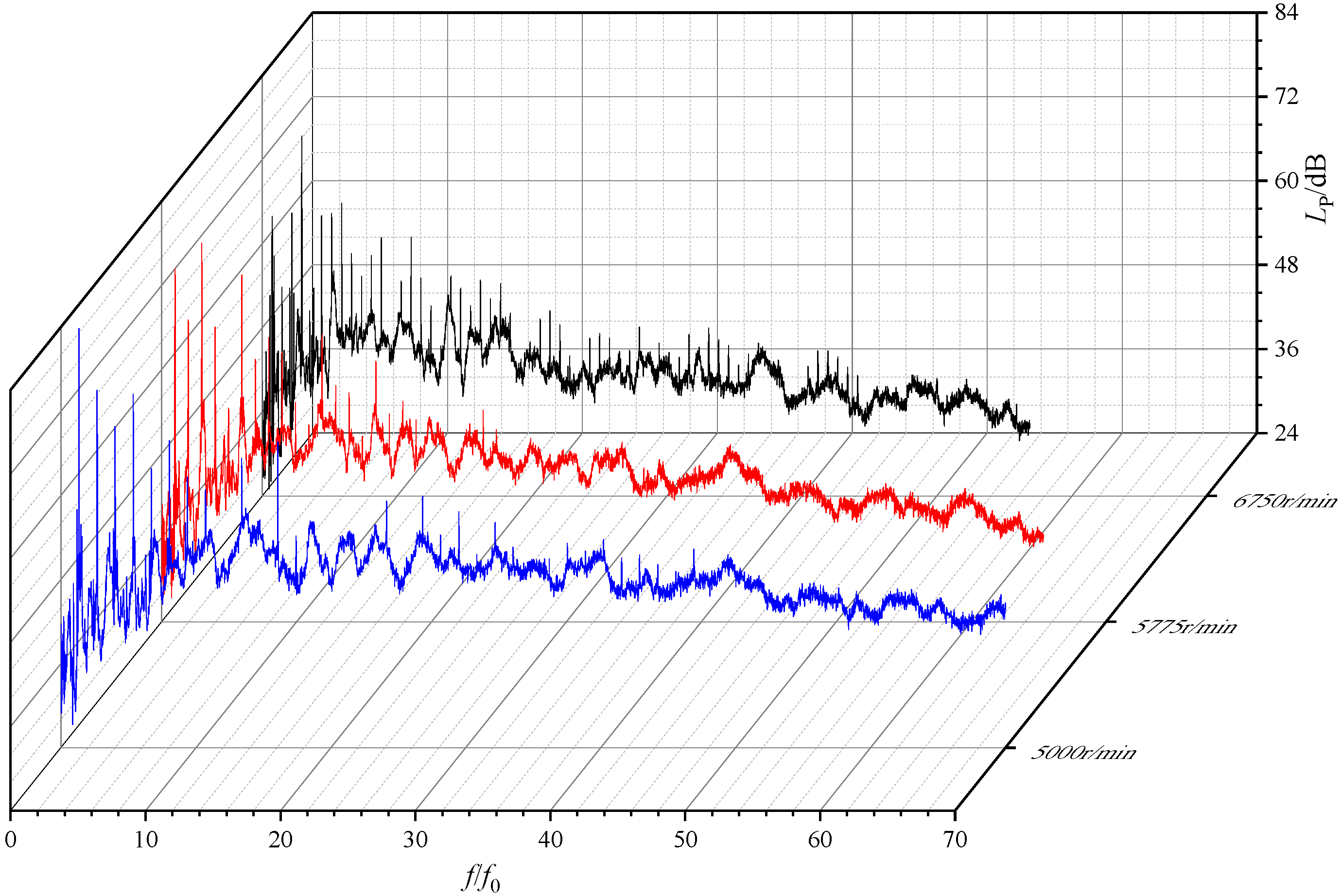
3.2.3. Frequency Domain Characteristics of the Radiate Noise
4. Numerical Results Analysis
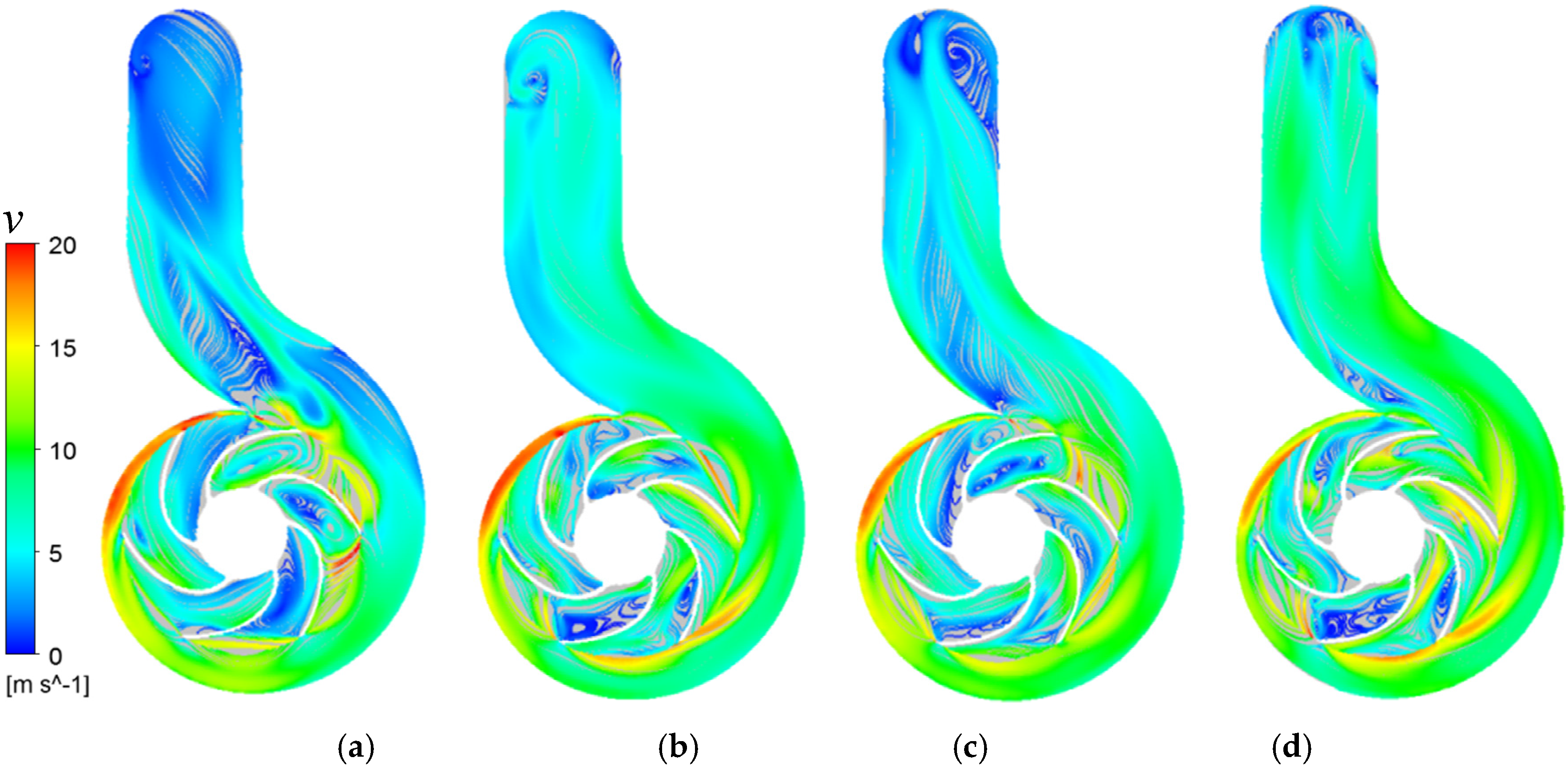
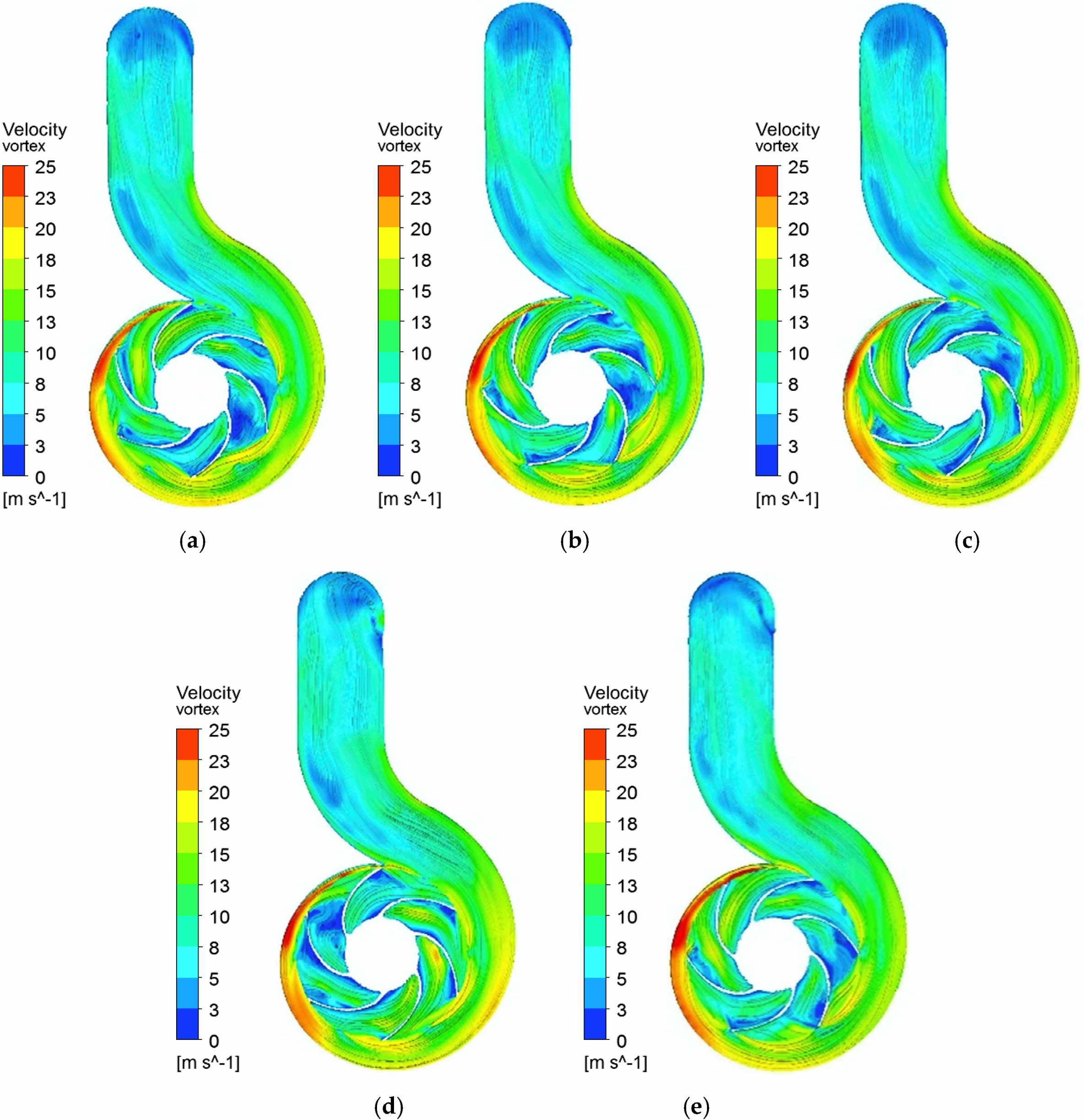
4.1. Flow Field Results Analysis
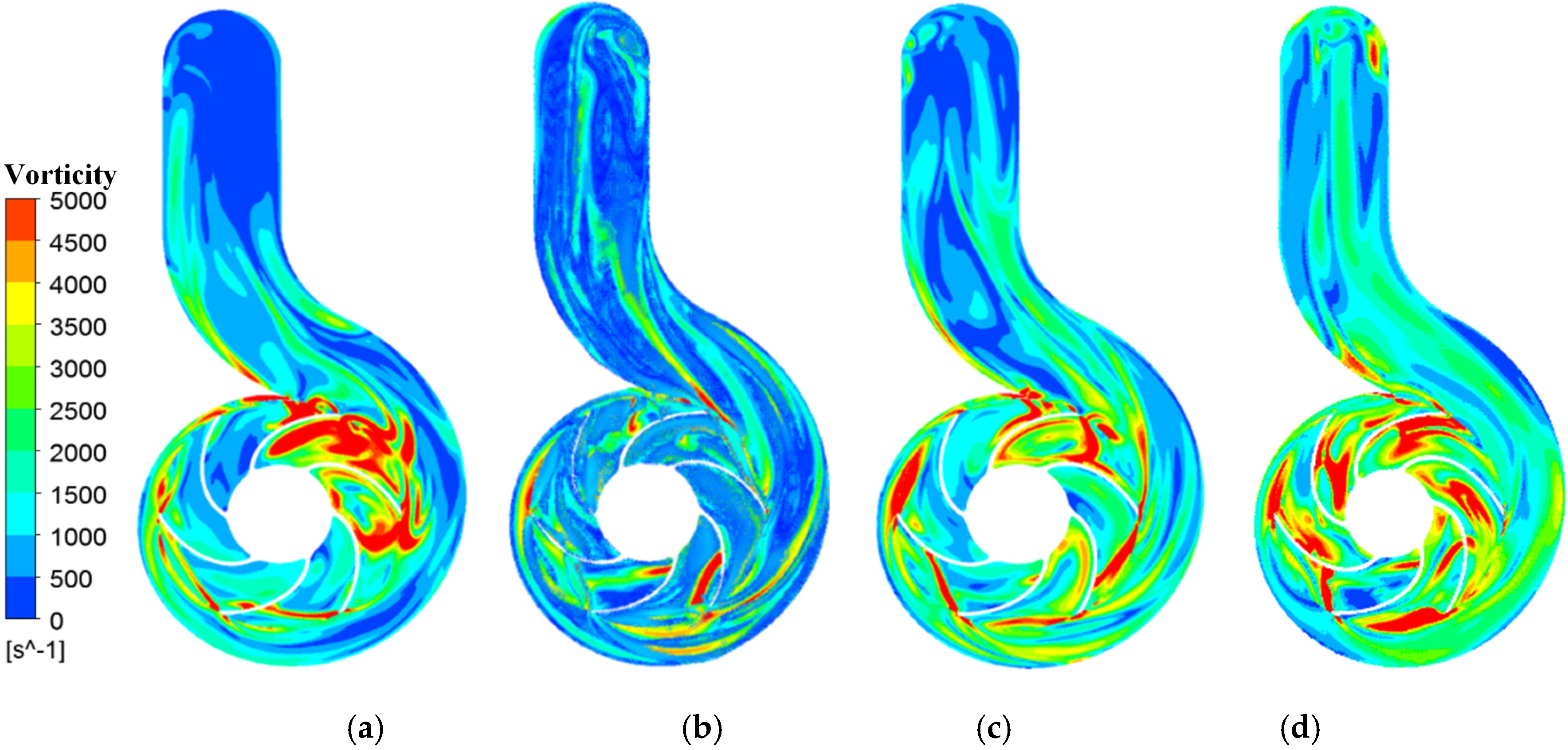
4.2. Unsteady Flow Characteristic Analysis
4.3. Modal Analysis
4.4. Acoustic Field Results Analysis
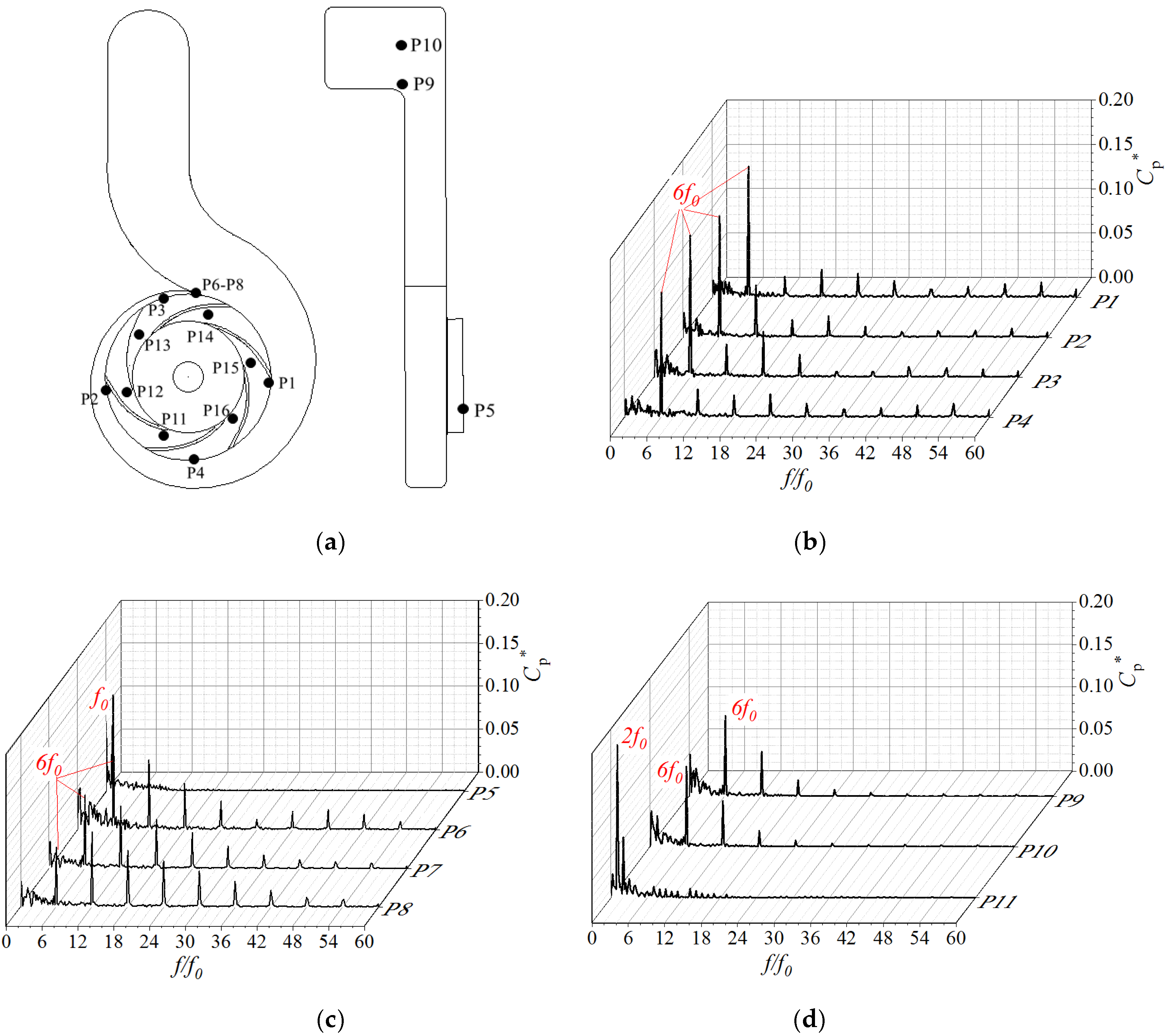
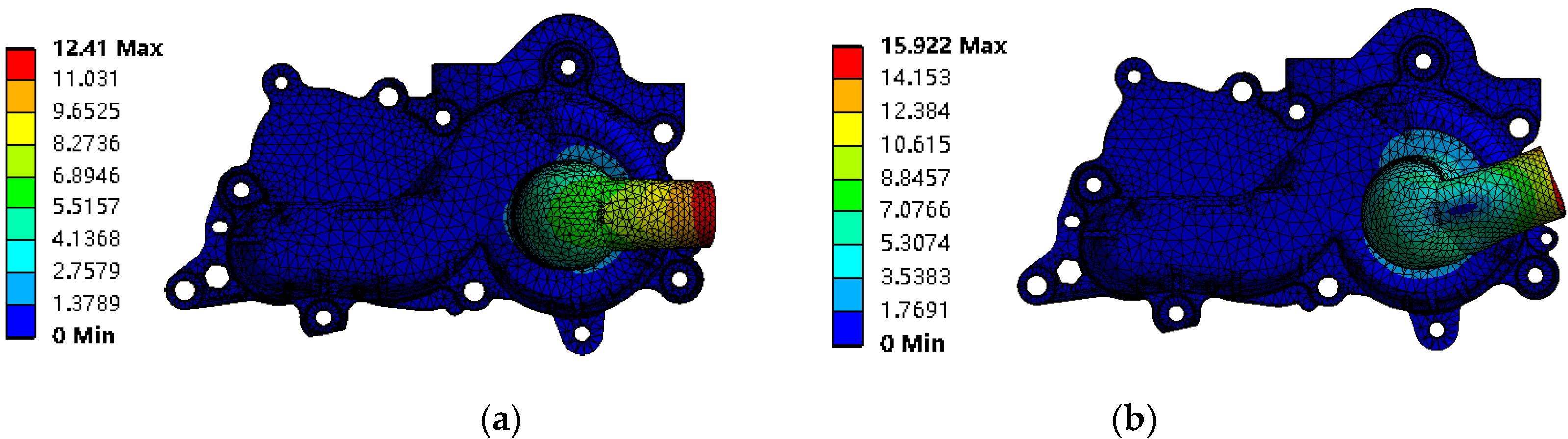
4.4.1. Fluid-Borne Noise
4.4.2. Fluid-Induced Radiated Noise
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Gülich, J.F. Centrifugal Pumps, 3rd ed.; Springer: Berlin/Heidelberg, Germany, 2014. [Google Scholar]
- Guo, C.; Gao, M.; He, S.Y. A review of the flow-induced noise study for centrifugal pumps. Appl. Sci. 2020, 10, 1022. [Google Scholar] [CrossRef]
- Wu, Y.L.; Li, S.C.; Liu, S.H.; Dou, H.S.; Qian, Z.D. Vibration of Hydraulic Machinery; Springer: Dordrecht, The Netherlands, 2013. [Google Scholar]
- Zhong, S.Y.; Huang, X. A review of aero acoustics and flow-induced noise: For beginners. Acta Aero Dyn. Sin. 2018, 36, 363–371. [Google Scholar]
- Lighthill, M. On sound generated aerodynamically: I. general theory. Proc. R. Soc. Lond. 1954, 222, 1–32. [Google Scholar]
- Si, Q.R.; Ali, A.; Yuan, J.P.; Fall, I.; Muhammad, F.Y. Flow-induced noises in a centrifugal pump: A review. Sci. Adv. Mater. 2019, 11, 909–924. [Google Scholar] [CrossRef]
- Simpson, H.C.; Macaskill, R.; Clark, T.A. Generation of hydraulic noise in centrifugal pumps. Proc. Instn. Mech. Engrs. 1966, 181, 84–108. [Google Scholar]
- Chu, S.M.; Dong, R.Y.; Katz, J. Relationship between unsteady flow pressure fluctuations and noise in a centrifugal pump. Part A: Effects of blade-tongue interactions. J. Fluid Eng.-T ASME 1995, 117, 24–29. [Google Scholar] [CrossRef]
- Chu, S.M.; Dong, R.Y.; Katz, J. Relationship between unsteady flow pressure fluctuations and noise in a centrifugal pump. Part B: Effects of blade-tongue interactions. J. Fluid Eng.-T ASME 1995, 117, 30–35. [Google Scholar] [CrossRef]
- Choi, J.S.; Mclaughlin, D.K.; Thompson, D.E. Experiments on the unsteady flow field and noise generation in a centrifugal pump impeller. J. Sound Vib. 2003, 263, 493–514. [Google Scholar] [CrossRef]
- Černetič, J. The use of noise and vibration signals for detecting cavitation in kinetic pumps. Proc. Inst. Mech. Eng. C 2009, 223, 1645–1655. [Google Scholar] [CrossRef]
- Langthjem, M.A.; Olhoff, N. A numerical study of flow-induced noise in a two-dimensional centrifugal pump, Part II. Hydroacoustics. J. Fluid Struct. 2004, 19, 369–386. [Google Scholar] [CrossRef]
- Parrondo, J.; Pérez, J.; Barrio, R.; Gonzales, J. A simple acoustic model to characterize the internal low frequency sound field in centrifugal pumps. Appl. Acoust. 2011, 72, 59–64. [Google Scholar] [CrossRef]
- Keller, J.; Barrio, R.; Parrondo, J.; Barrio, R.; Fernandez, J.; Blanco, E. Effects of the pump-circuit acoustic coupling on the blade-passing frequency perturbations. Appl. Acoust. 2014, 76, 150–156. [Google Scholar] [CrossRef]
- Mao, X.L.; Pavesi, G.; Chen, D.Y. Flow induced noise characterization of pump turbine in continuous and intermittent load rejection processes. Renew. Energy 2019, 139, 1029–1039. [Google Scholar] [CrossRef]
- Jiang, Y.Y.; Yoshimura, S.; Imai, R.; Katsura, H.; Yoshida, T.; Kato, C. Quantitative evaluation of flow-induced structural vibration and noise in turbomachinery by full-scale weakly coupled simulation. J. Fluid Struct. 2007, 23, 531–544. [Google Scholar] [CrossRef]
- Si, Q.R.; Shen, C.H.; Ali, A.; Cao, R.; Yuan, J.P.; Wang, C. Experimental and numerical study on gas-liquid two-phase flow behavior and flow induced noise characteristics of radial blade pumps. Process 2019, 7, 920. [Google Scholar] [CrossRef]
- Si, Q.R.; Wang, B.B.; Yuan, J.P.; Huang, K.L.; Lin, G.; Wang, C. Numerical and experimental investigation on radiated noise characteristics of the multistage centrifugal pump. Processes 2019, 7, 793. [Google Scholar] [CrossRef]
- Kapellos, C.S.; Papoutsis-Kiachagias, EM.; Giannakoglou, K.C.; Hartmann, M. The unsteady continuous adjoint method for minimizing flow-induced sound radiation. J. Comput. Phys. 2019, 392, 368–384. [Google Scholar] [CrossRef]
- Velarde, S.; Tajadura, R. Numerical simulation of the aerodynamic tonal noise generation in a backward-curved blades centrifugal fan. J. Sound Vib. 2006, 295, 781–786. [Google Scholar]
- Cravero, C.; Marsano, D. Numerical Prediction of Tonal Noise in Centrifugal Blowers. In Proceedings of the Turbo Expo 2018: Turbomachinery Technical Conference & Exposition, Oslo, Norway, 11–15 June 2018. [Google Scholar]
- Caro, S.; Ploumhans, P.; Gallez, X. Implementation of Lighthill’s Acoustic Analogy in a Finite/Infinite Elements Framework. In Proceedings of the 10th AIAA/CEAS Aeroacoustics Conference, Manchester, UK, 10–12 May 2004. [Google Scholar]
- International Organization for Standardization (ISO). ISO 9906: 2012 Rotodynamic Pumps-Hydraulic Performance Acceptance Tests—Grades 1, 2 and 3; ISO: Geneva, Switzerland, 2012. [Google Scholar]
- Si, Q.R.; Lu, R.; Shen, C.H.; Xia, S.J.; Sheng, G.C.; Yuan, J.P. An intelligent CFD-based optimization system for fluid machinery: Automotive electronic pump case application. Appl. Sci. 2020, 10, 366. [Google Scholar] [CrossRef]
- Huang, K.; Yuan, J.; Si, Q.; Lin, G. Numerical simulation on pressure pulsation in multistage centrifugal pump under several working conditions. J. Drain. Irrig. Mach. Eng. 2019, 37, 387–392. [Google Scholar]
- Strelets, M. Detached Eddy Simulation of Massively Separated Flows. In Proceedings of the 39th Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 8–11 January 2001. [Google Scholar]
- Si, Q.; Bois, G.; Liao, M.; Zhang, H.; Cui, Q.; Yuan, S. A comparative study on centrifugal pump designs and two-phase flow characteristic under inlet gas entrainment conditions. Energies 2020, 13, 65. [Google Scholar] [CrossRef]
- Free Field Technologies Co. MSC Actran14.1 User’s Guide; Free Field Technologies, Co.: Mont-Saint-Guibert, Belgium, 2012. [Google Scholar]
- Yang, J.; Xie, T.; Liu, X.H.; Si, Q.R.; Liu, J. Study of unforced unsteadiness in centrifugal pump at partial flow rates. J. Therm Sci. 2019. [Google Scholar] [CrossRef]


| Material | Density/(kg/m3) | Young’s Modulus/GPa | Poisson Ratio |
|---|---|---|---|
| PPS | 1500 | 11.95 | 0.4 |
| ADC | 2700 | 71 | 0.33 |
| Mode | Natural Frequency/Hz | Mode | Natural Frequency/Hz |
|---|---|---|---|
| 1st mode | 928.29 | 6th mode | 3702.2 |
| 2nd mode | 1095.7 | 7th mode | 3840.5 |
| 3rd mode | 2045.2 | 8th mode | 4767.8 |
| 4th mode | 2932.3 | 9th mode | 5089 |
| 5th mode | 3670.6 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Si, Q.; Shen, C.; He, X.; Li, H.; Huang, K.; Yuan, J. Numerical and Experimental Study on the Flow-Induced Noise Characteristics of High-Speed Centrifugal Pumps. Appl. Sci. 2020, 10, 3105. https://doi.org/10.3390/app10093105
Si Q, Shen C, He X, Li H, Huang K, Yuan J. Numerical and Experimental Study on the Flow-Induced Noise Characteristics of High-Speed Centrifugal Pumps. Applied Sciences. 2020; 10(9):3105. https://doi.org/10.3390/app10093105
Chicago/Turabian StyleSi, Qiaorui, Chunhao Shen, Xiaoke He, Hao Li, Kaile Huang, and Jianping Yuan. 2020. "Numerical and Experimental Study on the Flow-Induced Noise Characteristics of High-Speed Centrifugal Pumps" Applied Sciences 10, no. 9: 3105. https://doi.org/10.3390/app10093105
APA StyleSi, Q., Shen, C., He, X., Li, H., Huang, K., & Yuan, J. (2020). Numerical and Experimental Study on the Flow-Induced Noise Characteristics of High-Speed Centrifugal Pumps. Applied Sciences, 10(9), 3105. https://doi.org/10.3390/app10093105