Meso-Scale Failure of Freezing–Thawing Damage of Concrete under Uniaxial Compression
Abstract
Featured Application
Abstract
1. Introduction
2. Experimental Materials and Methods
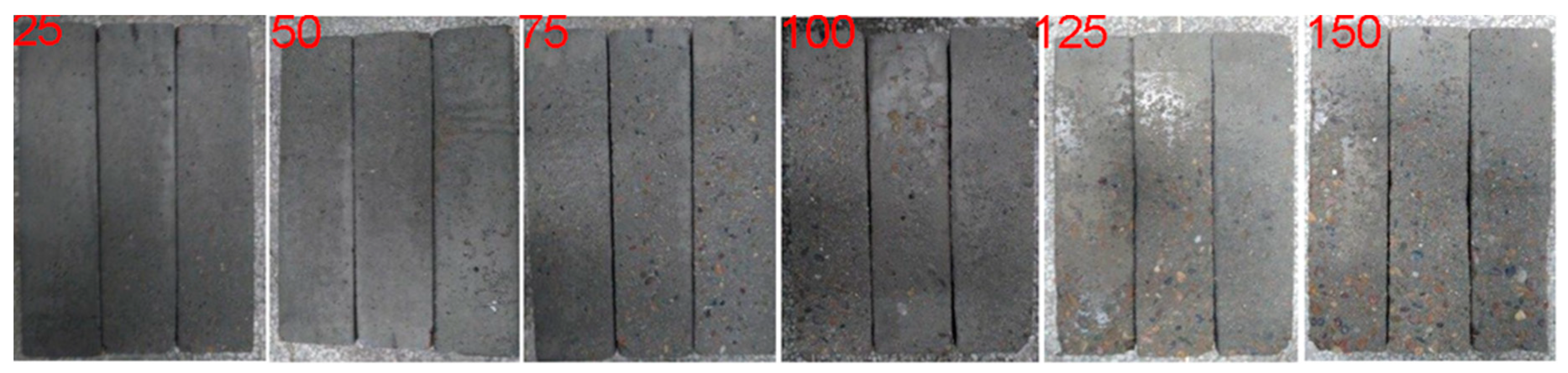
2.1. Freezing–Thawing Tests of Concrete
2.2. Damaged Layer of Frozen-Thawed Concrete
2.3. Uniaxial Compression Test of Concrete Damaged by Freezing–Thawing
3. Numerical Model of Concrete Damaged by Freezing–Thawing
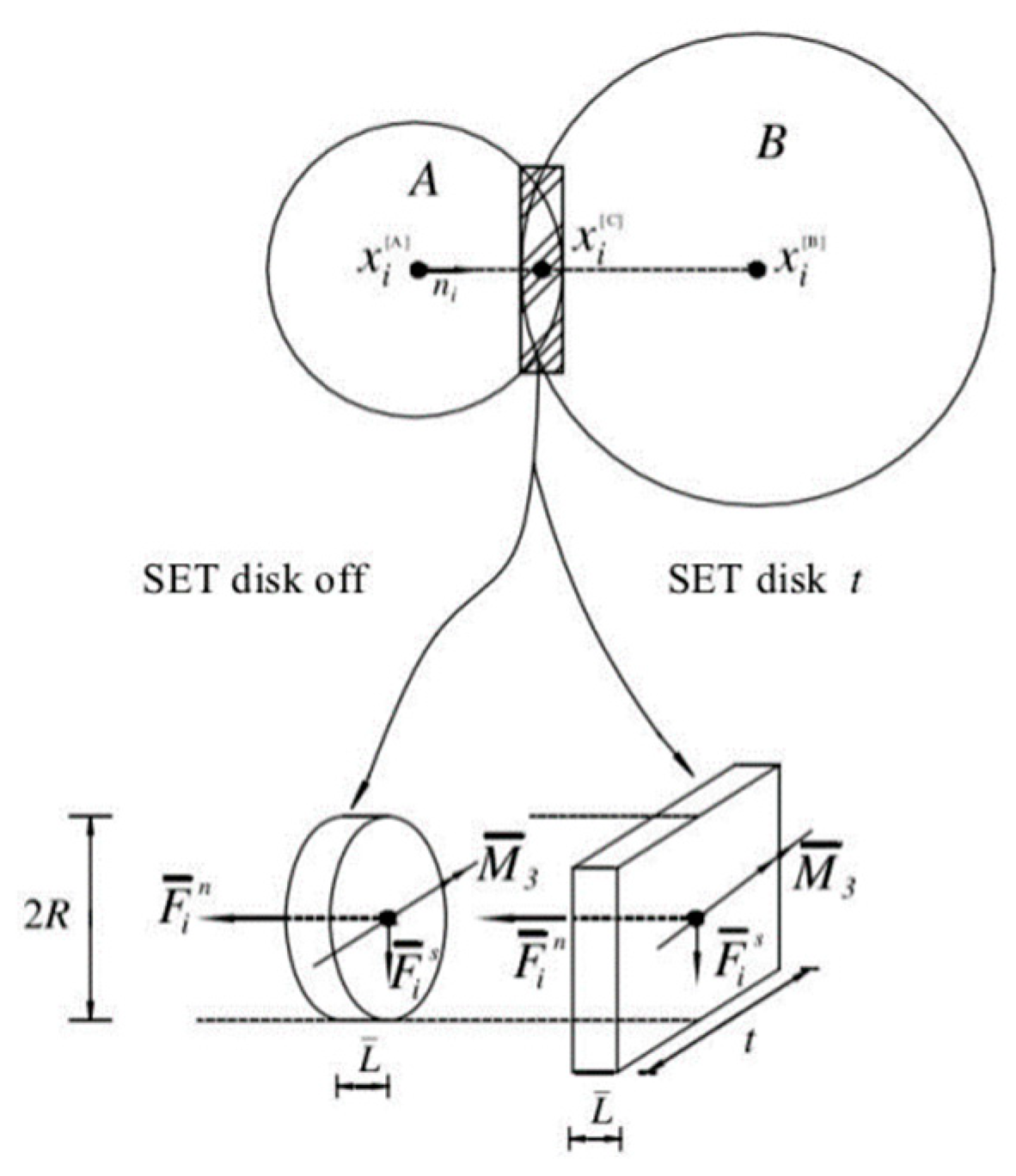
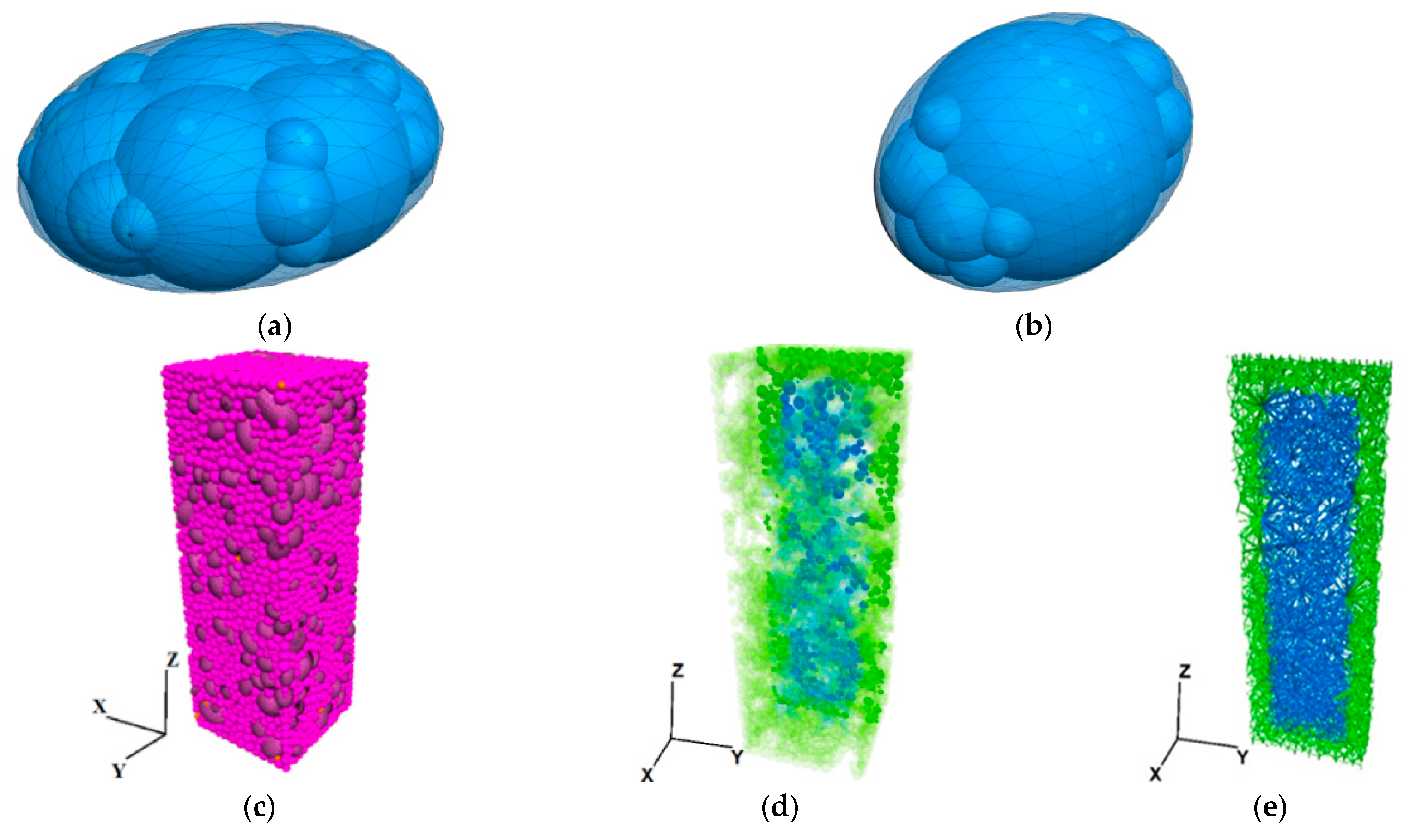
3.1. Model Setup
3.2. Calibration of Meso-Parameters
3.3. Model Validation
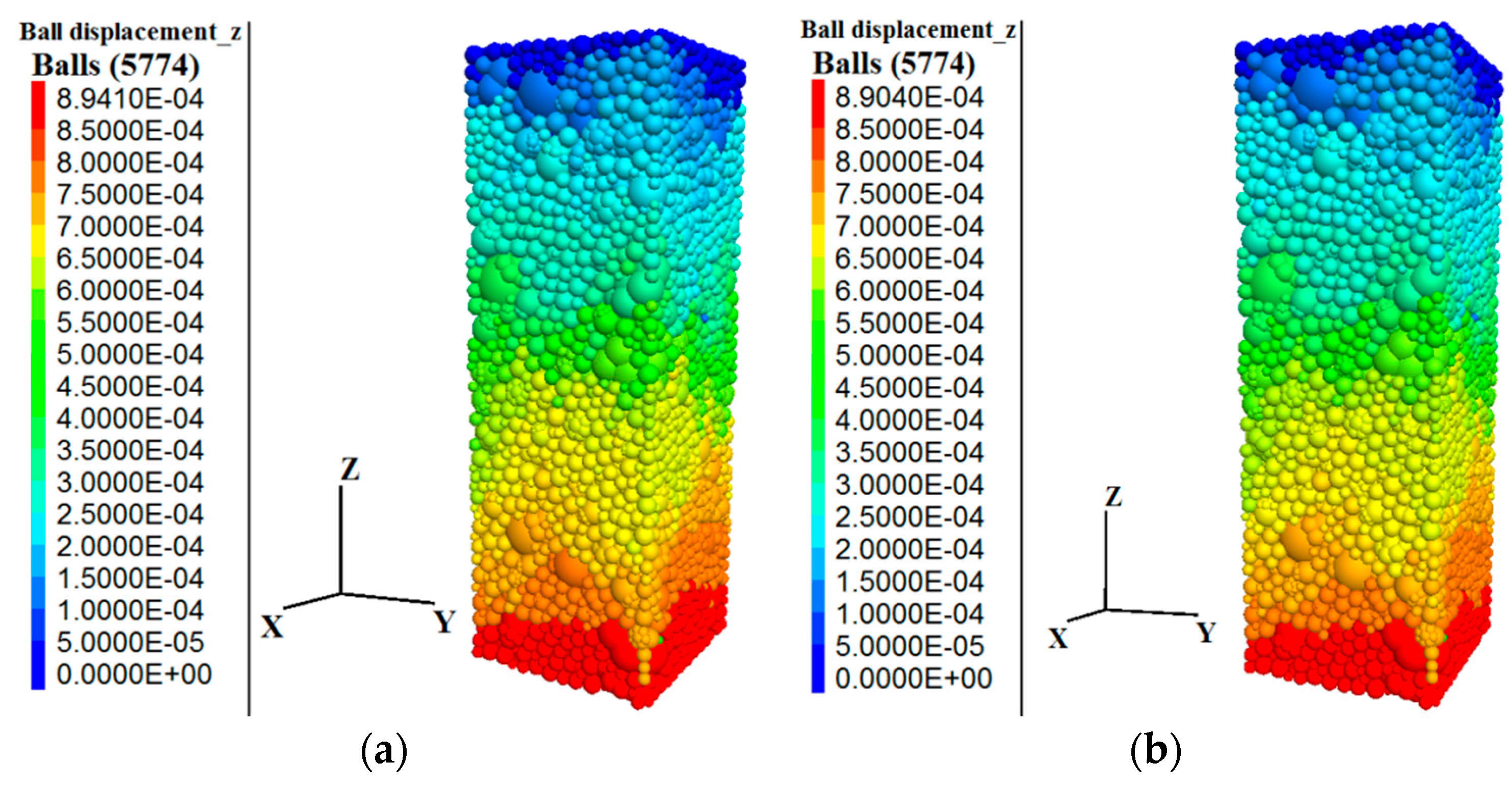
4. Numerical Simulation of Meso-Scale Failure of Freezing–Thawing Damage Concrete under Uniaxial Compression
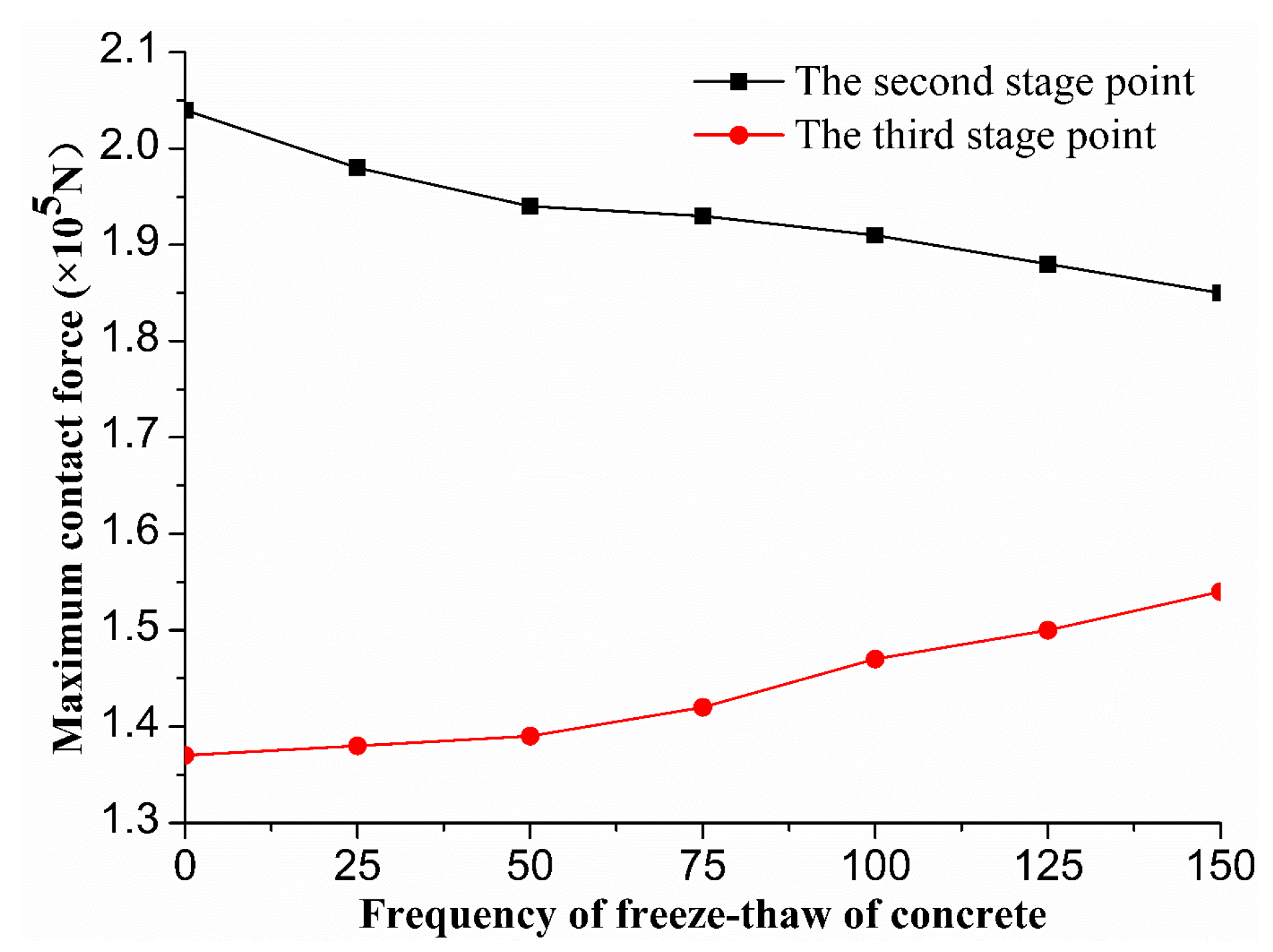
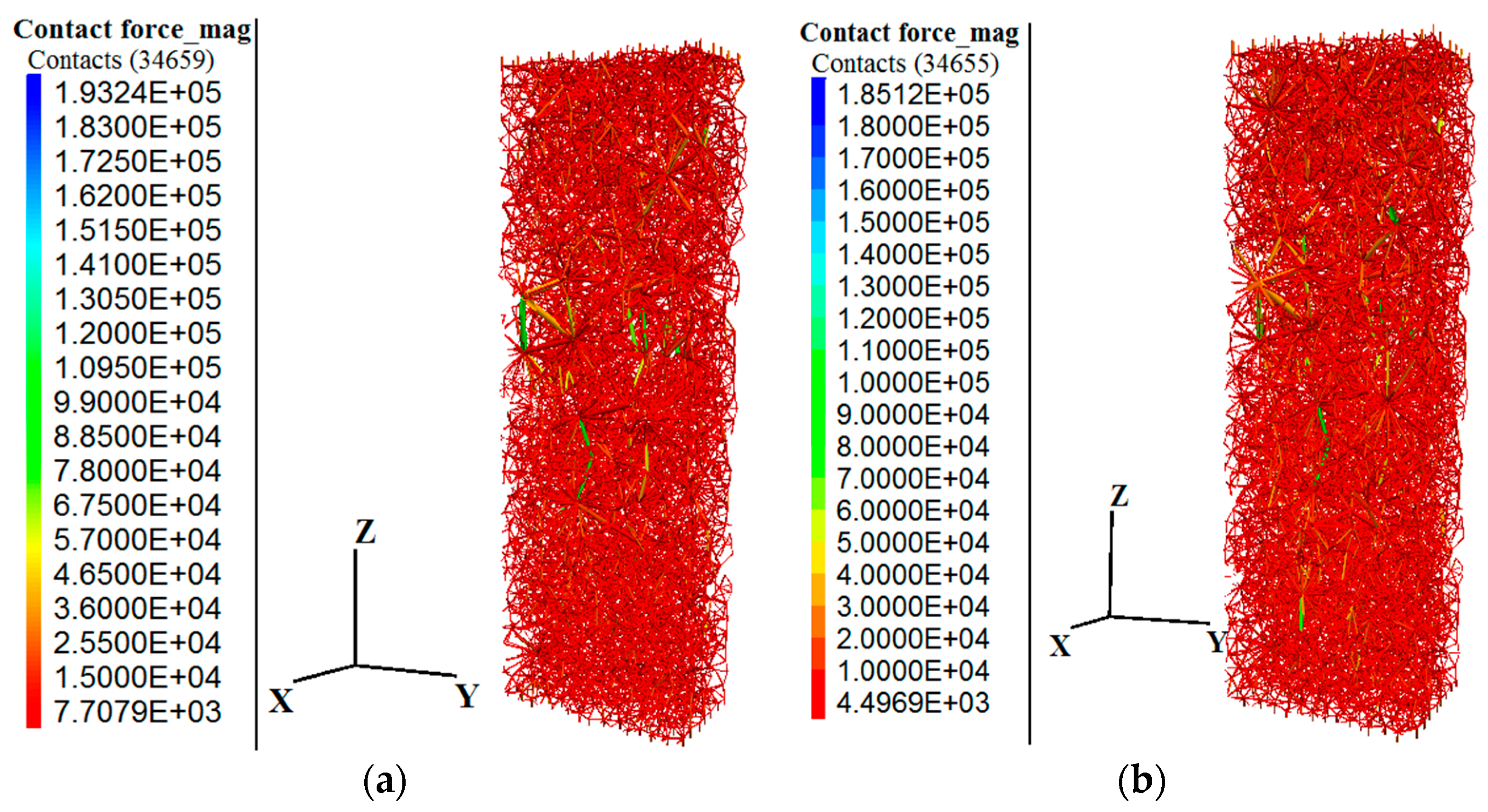
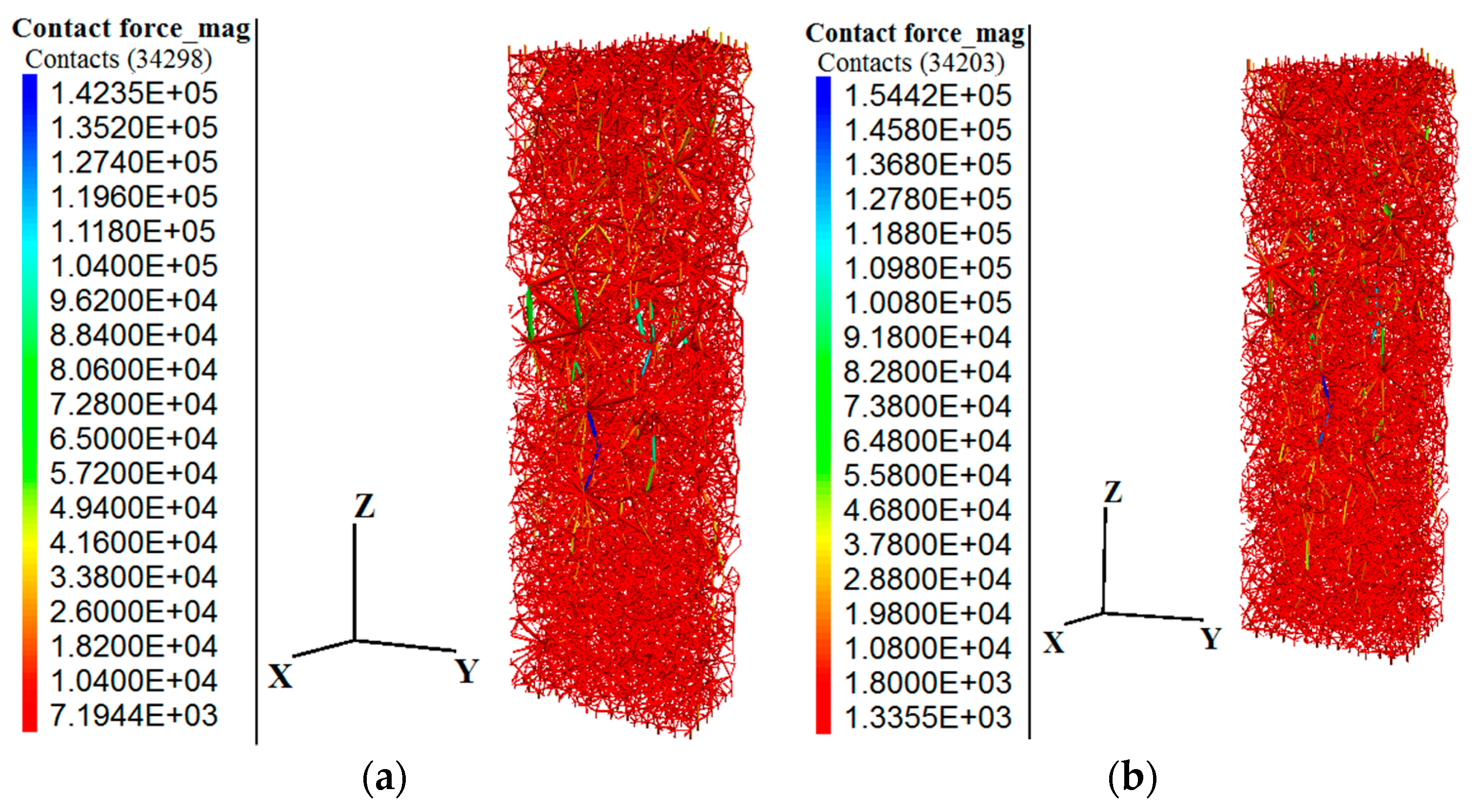
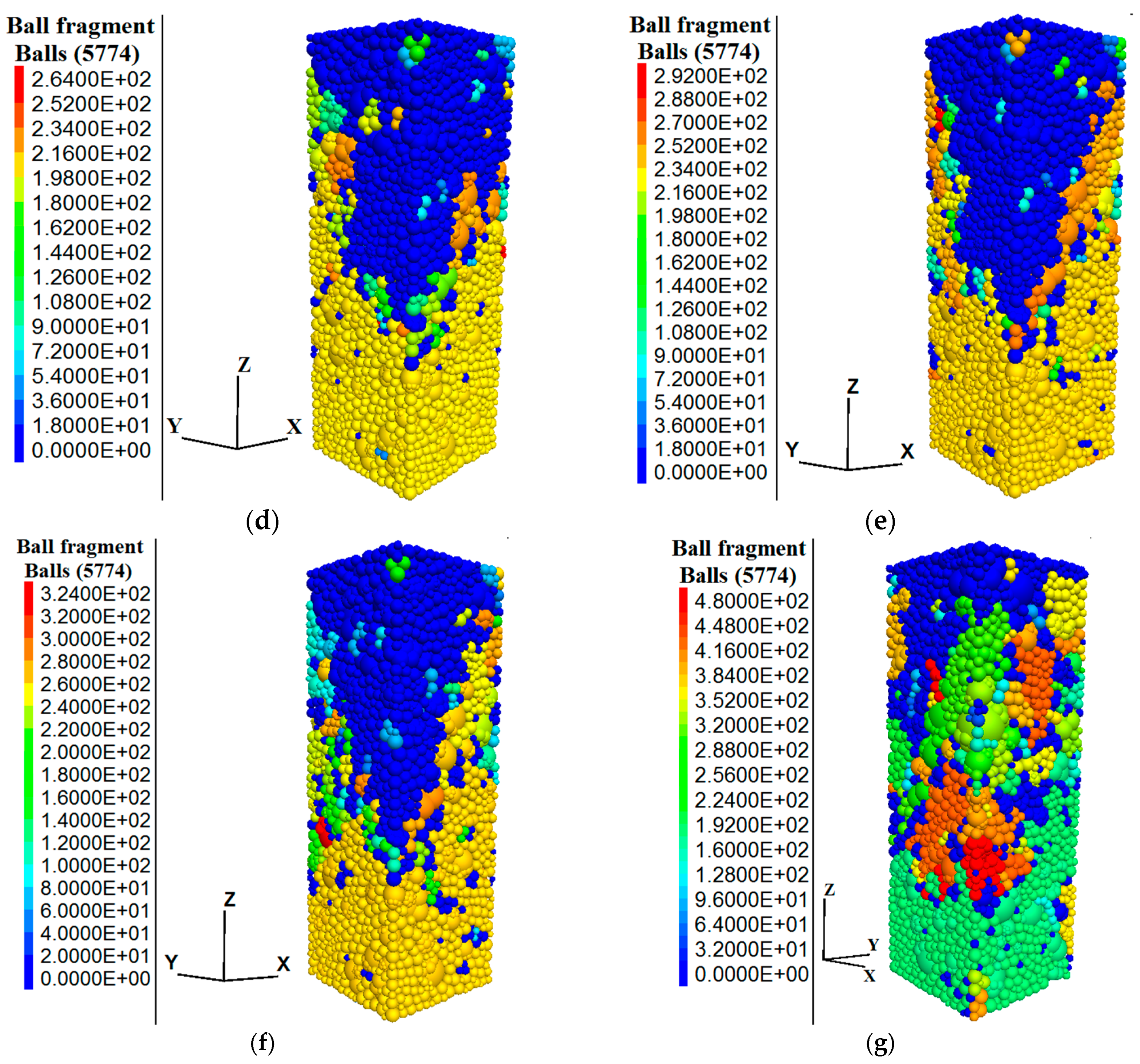
4.1. Analysis of Contact Force between Concrete Particles
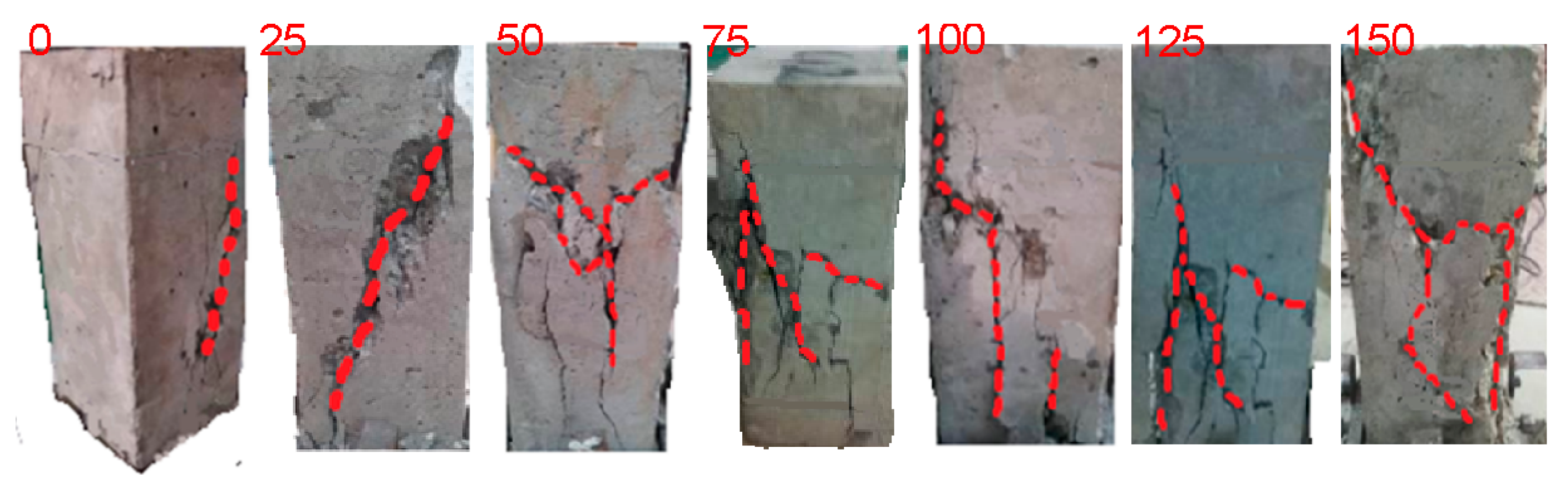
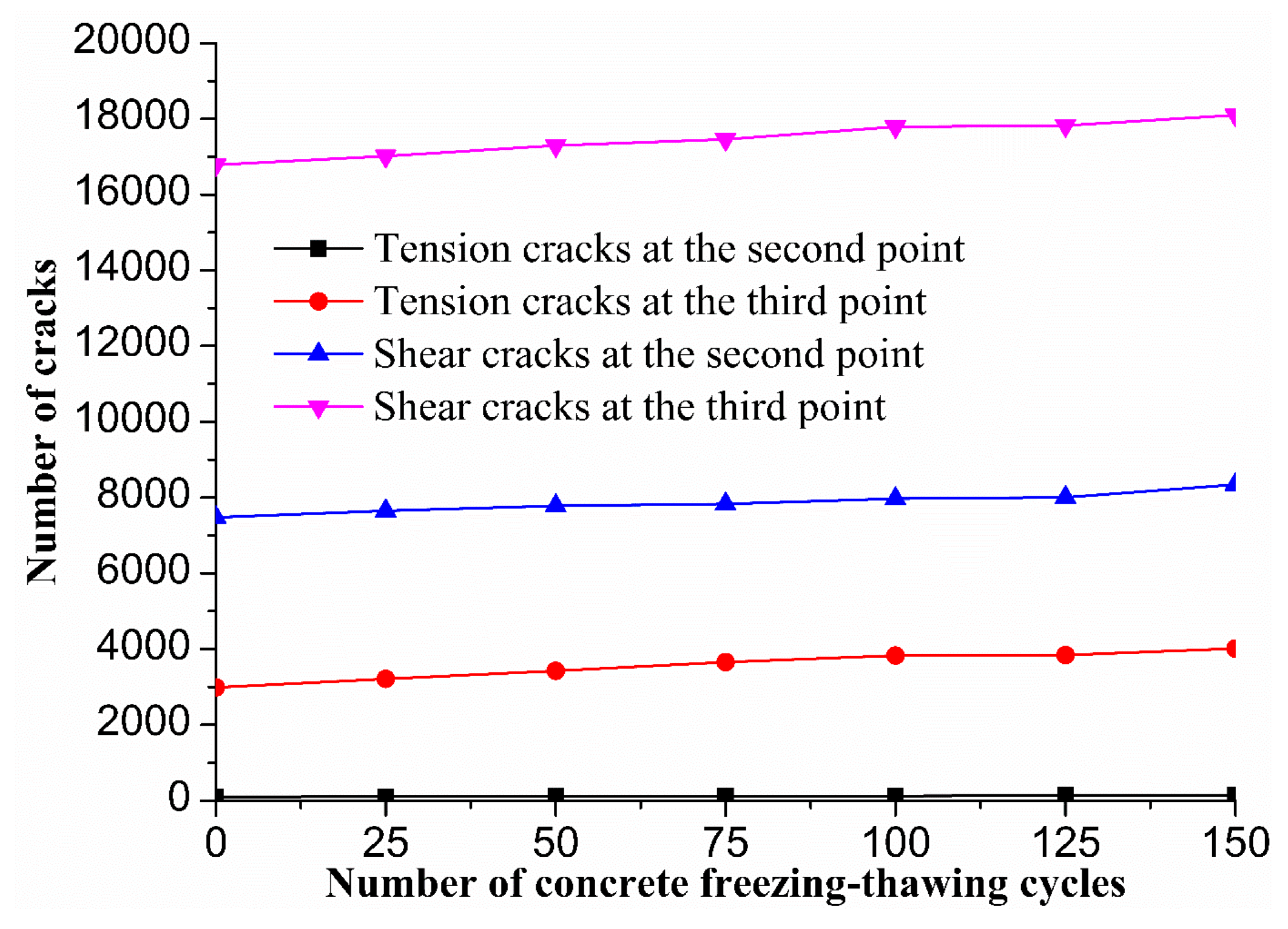
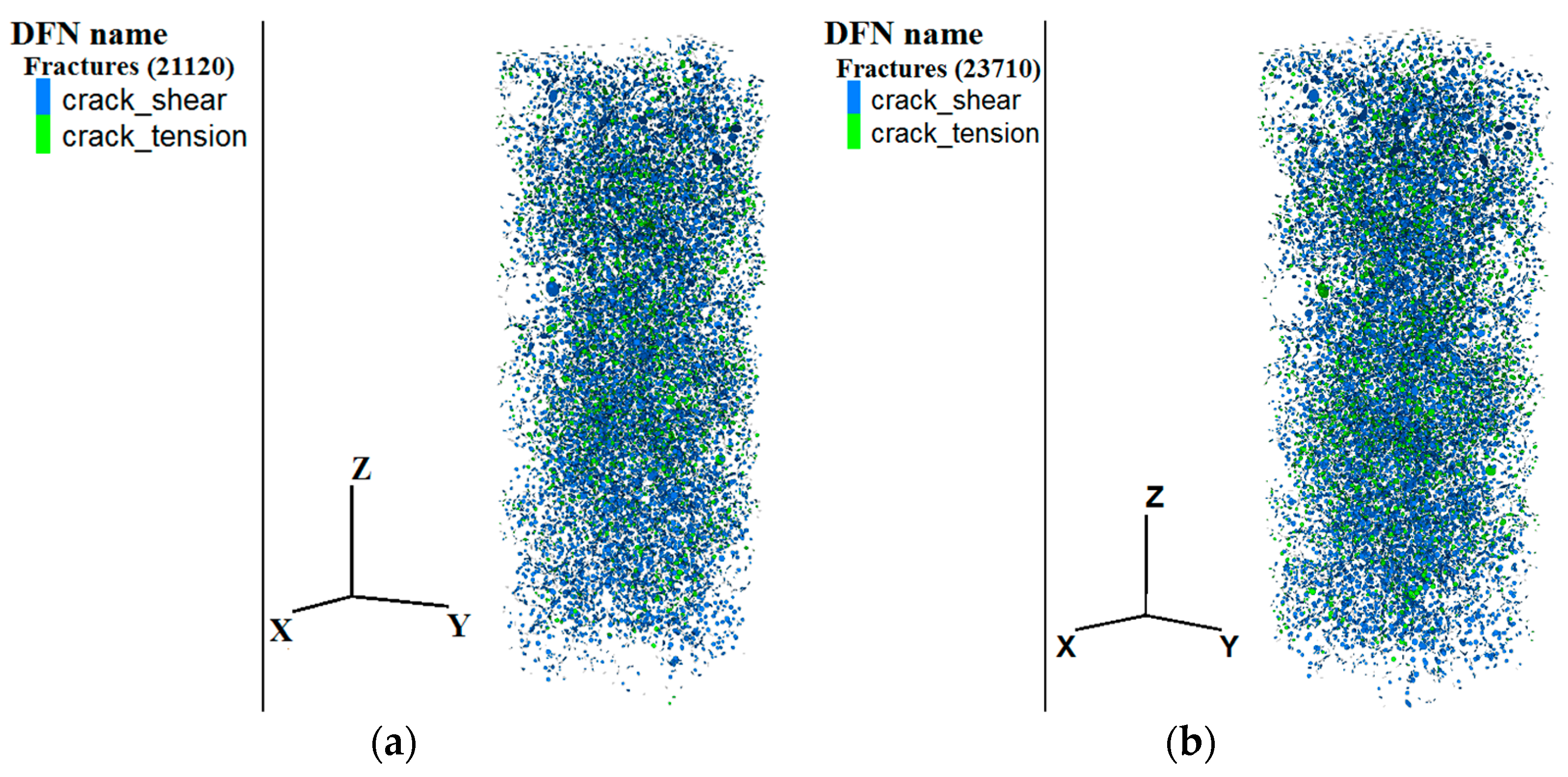
4.2. Crack Development Law of Concrete Model
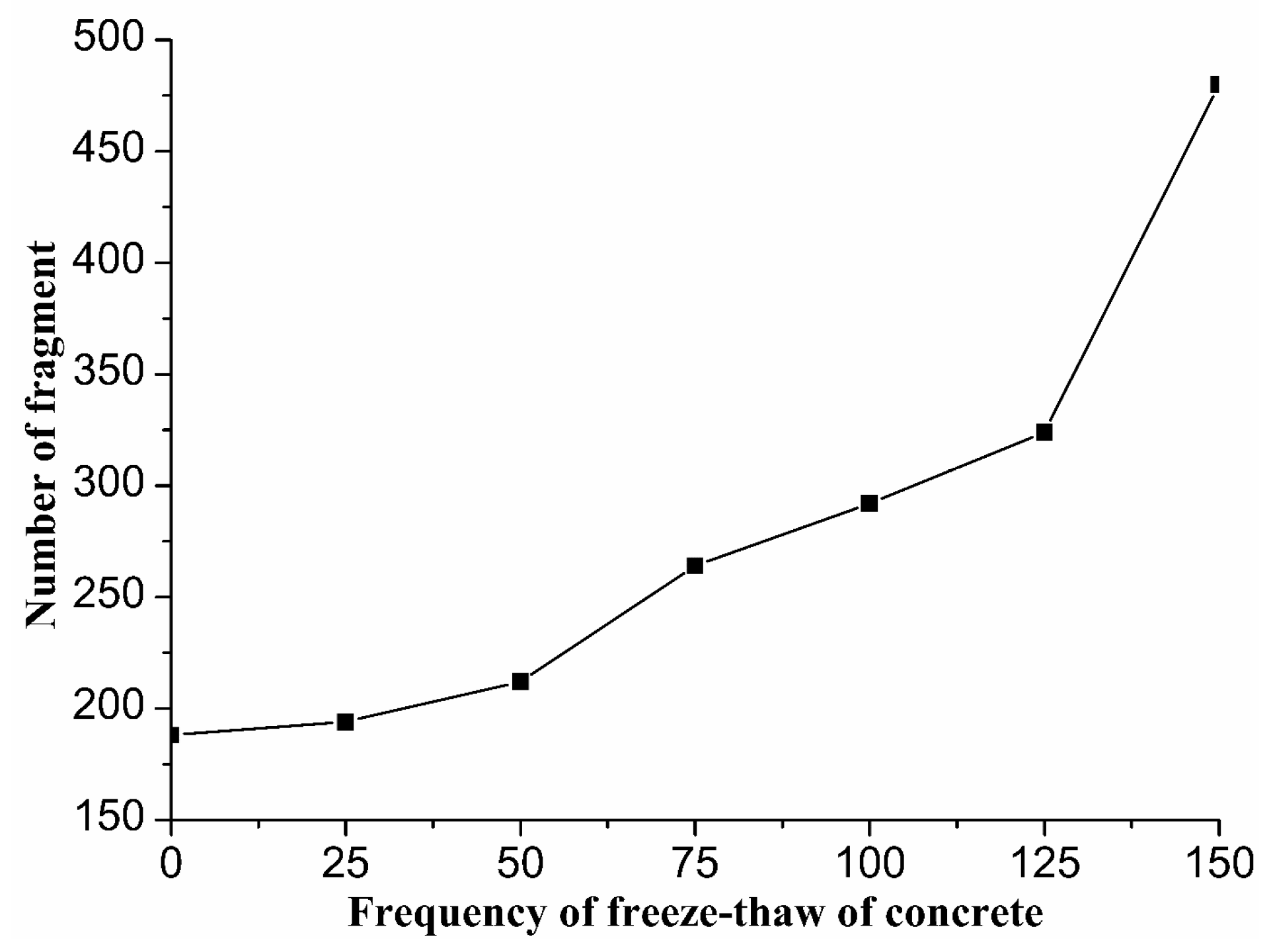
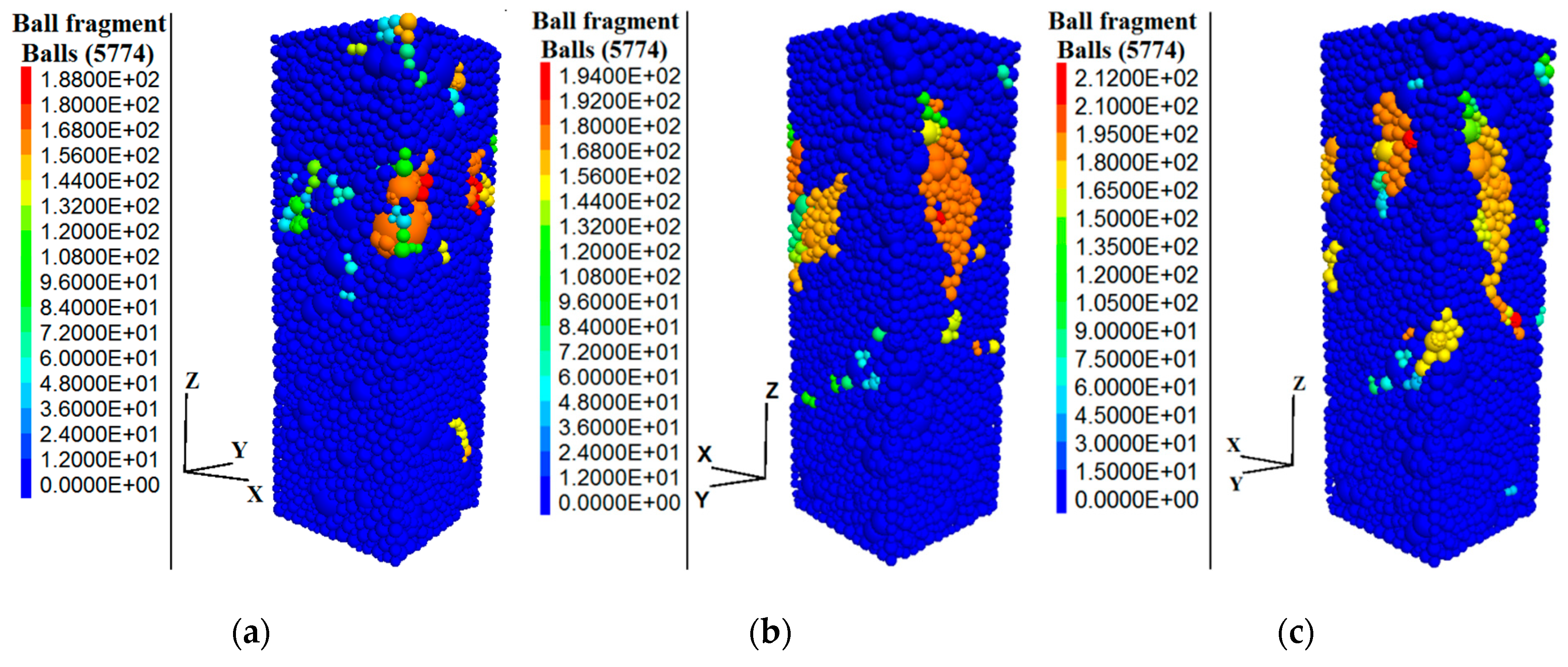
4.3. Cracking Law of Concrete Model Specimens
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Hanjari, K.Z.; Utgenannt, P.; Lundgren, K. Experimental study of the material and bond properties of frost-damaged concrete. Cem. Concr. Res. 2011, 41, 244–254. [Google Scholar] [CrossRef]
- Almahmoud, F.; Mechling, J.M. Bond strength of different strengthening systems—Concrete elements under freeze-thaw cycles and salt water immersion exposure. Constr. Build. Mater. 2014, 70, 399–409. [Google Scholar] [CrossRef]
- Li, B.; Mao, J.; Nawa, T. Mesoscopic damage model of concrete subjected to freeze-thaw cycles using mercury intrusion porosimetry and differential scanning calorimetry (MIP-DSC). Constr. Build. Mater. 2017, 147, 79–90. [Google Scholar] [CrossRef]
- Powers, T.C. A working hypothesis for further studies of frost resistance of concrete. Am. Concr. Inst. 1950, 16, 245–272. [Google Scholar]
- Powers, T.C. Freezing effect in concrete, Durability of Concrete. Am. Concr. Inst. 1975, 47, 1–12. [Google Scholar]
- Powers, T.C.; Helmuth, R.A. Theory of volume changes in hardened Portland cement paste during freezing. Proc. Highw. Res. Board 1953, 32, 285–297. [Google Scholar]
- Hanjari, K.Z.; Kettil, P.; Lundgren, K. Modelling the structural behaviour of frost-damaged reinforced concrete structures. Struct. Infrastruct. Eng. 2013, 9, 416–431. [Google Scholar] [CrossRef]
- Cao, D.F. Experimental study on tensile properties of concrete after freeze-thaw cycles. Constr. Build. Mater. 2012, 4, 42–52. [Google Scholar]
- Hasan, M.; Okuyama, H.; Sato, Y. Stress-strain model of concrete damaged by freezing and thawing cycles. J. Adv. Concr. Technol. 2004, 2, 8999. [Google Scholar] [CrossRef]
- Bogas, J.A.; Brito, J.D.; Ramos, D. Freeze–thaw resistance of concrete produced with fine recycled concrete aggregates. J. Clean. Prod. 2016, 115, 294–306. [Google Scholar] [CrossRef]
- Shang, H.S.; Song, Y.P. Experimental study of strength and deformation of plain concrete under biaxial compression after freezing and thawing cycles. Cem. Concr. Res. 2006, 36, 1857–1864. [Google Scholar] [CrossRef]
- Ma, B.; Ye, Y.H.; Sun, Y. Mechanical performance analysis of salt-frozen or freeze-thaw concrete based on damage theory. J. Build. Struct. 2009, 30, 298–302. [Google Scholar]
- Sun, W.; Mu, R.; Luo, X. Effect of chloride salt, freeze-thaw cycling and externally applied load on the performance of the concrete. Cem. Concr. Res. 2002, 32, 1859–1864. [Google Scholar] [CrossRef]
- Zhu, J.P.; Li, S.C.; Ramos, D. Mechanical property deterioration model for concrete in environment with freezing-thawing. J. Archit. Civ. Eng. 2009, 26, 62–67. [Google Scholar]
- Hasan, M.; Ueda, T.; Sato, Y. Stress-Strain Relationship of Frost-Damaged Concrete Subjected to Fatigue Loading. J. Mater. Civ. Eng. 2008, 20, 37–45. [Google Scholar] [CrossRef]
- Liu, H.K.; Li, J. Constitutive law of attacked concrete subject to freeze-thaw cycles. Constr. Build. Mater. 2011, 14, 736–741. [Google Scholar]
- Duan, A.; Tian, Y.; Dai, J.G. A stochastic damage model for evaluating the internal deterioration of concrete due to freeze–thaw action. Mater. Struct. 2014, 47, 1025–1039. [Google Scholar] [CrossRef]
- Shen, K.X. Study on Bearing Capacity of Reinforced Concrete Beams under Freeze-Thaw Cycle. Master’s Thesis, XI’AN University of Architecture and Technology, Xi’an, China, 2014. [Google Scholar]
- Yu, H.; Ma, H.; Yan, K. An equation for determining freeze-thaw fatigue damage in concrete and a model for predicting the service life. Constr. Build. Mater. 2017, 137, 104–116. [Google Scholar] [CrossRef]
- Guan, X. The Analysisi of Damage and the Research of Bearing Capacity of Reinforced Concrete Flexural Members under the Environment of Freezing and Thawing. Ph.D. Thesis, XI’AN University of Architecture and Technology, Xi’an, China, 2015. [Google Scholar]
- Chen, G.X. Test Code for Hydraulic Concrete; Ministry of Water Resources of the PRC: Beijing, China, 2006.
- Wang, Z.X. Uniaxial Test Simulation of Concrete Freeze-Thaw Damage Based on Particle Flow Discrete Element Method. Master’s Thesis, Xi’an University of Technology, Xi’an, China, 2018. [Google Scholar]
- Liu, Z.L.; Sun, W.W.; Wang, X.M. Research on numerical simulation method of projectile penetration into micro concrete based on particle dispersion model. J. Vib. Shock 2016, 35, 162–169. [Google Scholar]
- Li, Y.J.; Cui, W.; Qi, L. Numerical simulation of self compacting concrete performance based on discrete element method. Eng. J. Wuhan Univ. 2019, 52, 33–39. [Google Scholar]
- Itasca Consulting Group Inc. PFC3D User’s Manual; Version 5.0; Itasca Consulting Group: Minneapolis, MN, USA, 2017. [Google Scholar]
- Elias, J. Simulation of railway ballast using crushable polyhedral particles. Powder Technol. 2014, 264, 458–465. [Google Scholar] [CrossRef]
- Lichter, J.; Lim, K.; Potapov, A. New developments in cone crusher performance optimization. Miner. Eng. 2009, 22, 613–617. [Google Scholar] [CrossRef]
- Geng, J.Y.; Bu, J.Q.; Gao, Y. Numerical Analysis of Concrete Triaxial Compression Test Based on Discrete Element Method. J. Shijiazhuang Railw. Inst. 2015, 28, 88–91. [Google Scholar]
- Xiao, H. Simulation Of Comcrete Failure Process Using Discrete Element Method Based On 2-D Particle Flow Code. Master’s Thesis, Xiangtan University, Xiangtan, China, 2011. [Google Scholar]
- Kang, Z.; Tang, X.W.; Qin, C. End effect of concrete by meso-scale discrete element modeling. J. Harbin Inst. Technol. 2013, 45, 94–98. [Google Scholar]
- Xu, S.J.; Yin, X.T.; Ma, S.K. Numerical Test Study of Concrete Material Based on Particle Flow. J. Exp. Mech. 2009, 24, 251–258. [Google Scholar]





| Specimen Strength | Water/Binder Ratio | Sand Ratio | 1 m3 Material Content of Concrete/kg | |||||
|---|---|---|---|---|---|---|---|---|
| Water | Cement | Sand | Stone | Fly Ash | Admixture | |||
| C30 | 0.53 | 0.4 | 170 | 256 | 765 | 1150 | 64 | 9.6 |
| Number of Freezing-Thawing Cycles | 0 | 25 | 50 | 75 | 100 | 125 | 150 |
| Average Value of Test Axial Deformation (mm) | 0.897 | 0.894 | 0.894 | 0.891 | 0.890 | 0.890 | 0.889 |
| Number of Freezing–Thawing Cycles | emod/Gpa | kratio | fric | pb_emod/Gpa | pb_kratio | pb_nstrength/Mpa | pb_strength/Mpa | pb_radius |
|---|---|---|---|---|---|---|---|---|
| 0 | 40 | 0.1 | 0.6 | 40 | 0.42 | 68 | 68 | 0.5 |
| 25 | 32 | 0.1 | 0.6 | 32 | 0.42 | 64 | 64 | 0.5 |
| 50 | 30 | 0.1 | 0.6 | 30 | 0.42 | 62.25 | 62.25 | 0.5 |
| 75 | 28 | 0.1 | 0.6 | 28 | 0.42 | 61.6 | 61.6 | 0.5 |
| 100 | 26 | 0.1 | 0.6 | 26 | 0.42 | 63 | 63 | 0.5 |
| 125 | 24.5 | 0.1 | 0.6 | 24.5 | 0.42 | 61 | 61 | 0.5 |
| 150 | 26 | 0.1 | 0.6 | 26 | 0.42 | 62 | 62 | 0.5 |
| Number of Freezing–Thawing Cycles | emod/Gpa | kratio | fric | pb_emod/Gpa | pb_kratio | pb_nstrength /Mpa | pb_strength /Mpa | pb_radius |
|---|---|---|---|---|---|---|---|---|
| 0 | 40 | 0.1 | 0.6 | 40 | 0.42 | 34 | 34 | 0.5 |
| 25 | 32 | 0.1 | 0.6 | 32 | 0.42 | 32 | 32 | 0.5 |
| 50 | 30 | 0.1 | 0.6 | 30 | 0.42 | 31.25 | 31.25 | 0.5 |
| 75 | 28 | 0.1 | 0.6 | 28 | 0.42 | 30.8 | 30.8 | 0.5 |
| 100 | 26 | 0.1 | 0.6 | 26 | 0.42 | 31.5 | 31.5 | 0.5 |
| 125 | 24.5 | 0.1 | 0.6 | 24.5 | 0.42 | 30.5 | 30.5 | 0.5 |
| 150 | 26 | 0.1 | 0.6 | 26 | 0.42 | 31 | 31 | 0.5 |
| Number of Freezing–Thawing Cycles | Average Value of Test Axial Deformation (mm) | Axial Displacement of Particles (mm) | Difference Value between Numerical Model and Test in Axial Deformation (mm) | Relative Error of Numerical Model and Test in Axial Deformation (%) |
|---|---|---|---|---|
| 0 | 0.897 | 0.899 | 0.002 | 0.223 |
| 25 | 0.894 | 0.895 | 0.001 | 0.112 |
| 50 | 0.894 | 0.898 | 0.004 | 0.447 |
| 75 | 0.891 | 0.894 | 0.003 | 0.337 |
| 100 | 0.890 | 0.896 | 0.006 | 0.674 |
| 125 | 0.890 | 0.897 | 0.007 | 0.787 |
| 150 | 0.889 | 0.890 | 0.001 | 0.112 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Si, Z.; Du, X.; Huang, L.; Li, Y. Meso-Scale Failure of Freezing–Thawing Damage of Concrete under Uniaxial Compression. Appl. Sci. 2020, 10, 1252. https://doi.org/10.3390/app10041252
Si Z, Du X, Huang L, Li Y. Meso-Scale Failure of Freezing–Thawing Damage of Concrete under Uniaxial Compression. Applied Sciences. 2020; 10(4):1252. https://doi.org/10.3390/app10041252
Chicago/Turabian StyleSi, Zheng, Xiaoqi Du, Lingzhi Huang, and Yanlong Li. 2020. "Meso-Scale Failure of Freezing–Thawing Damage of Concrete under Uniaxial Compression" Applied Sciences 10, no. 4: 1252. https://doi.org/10.3390/app10041252
APA StyleSi, Z., Du, X., Huang, L., & Li, Y. (2020). Meso-Scale Failure of Freezing–Thawing Damage of Concrete under Uniaxial Compression. Applied Sciences, 10(4), 1252. https://doi.org/10.3390/app10041252




