Combining Stable Inversion and H∞ Synthesis for Trajectory Tracking and Disturbance Rejection Control of Civil Aircraft Autolanding
Abstract
1. Introduction
- (1).
- A robust autolanding controller (RAC) is designed based on synthesis, which can handle with the disturbances during landing procedure, such as windshear and noise.
- (2).
- A stable inversion (SI) based robust autolanding controller (SIRAC) is proposed to improve the RAC scheme. The SI algorithm is used to enhance the trajectory tracking ability of aircraft autolanding system, which calculates the desired input and state through the desired landing trajectory. While the disturbance rejection ability is also increased due to the integration of the SI algorithm and synthesis.
2. Dynamics Modelling and Problem Formulation
2.1. Aircraft Dynamics and Actuator Modelling
2.2. Windshear Model
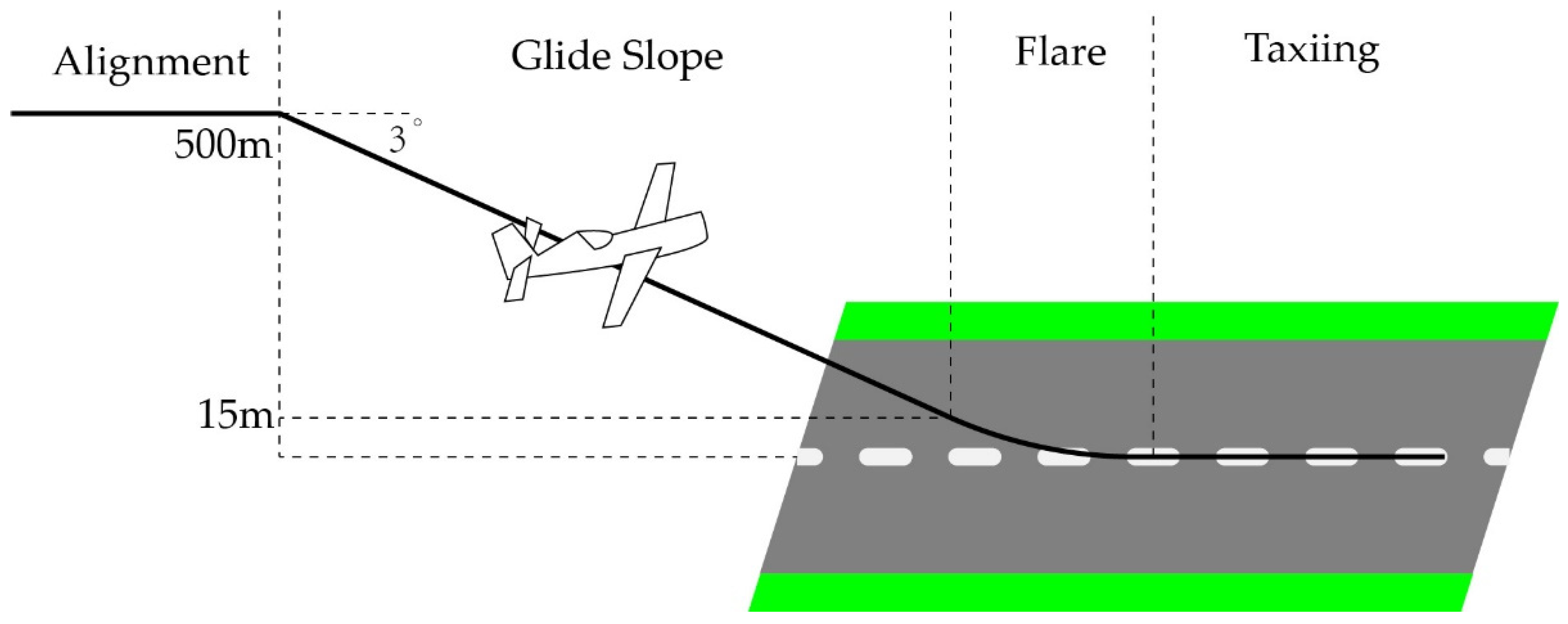
2.3. The Desired Landing Trajectory
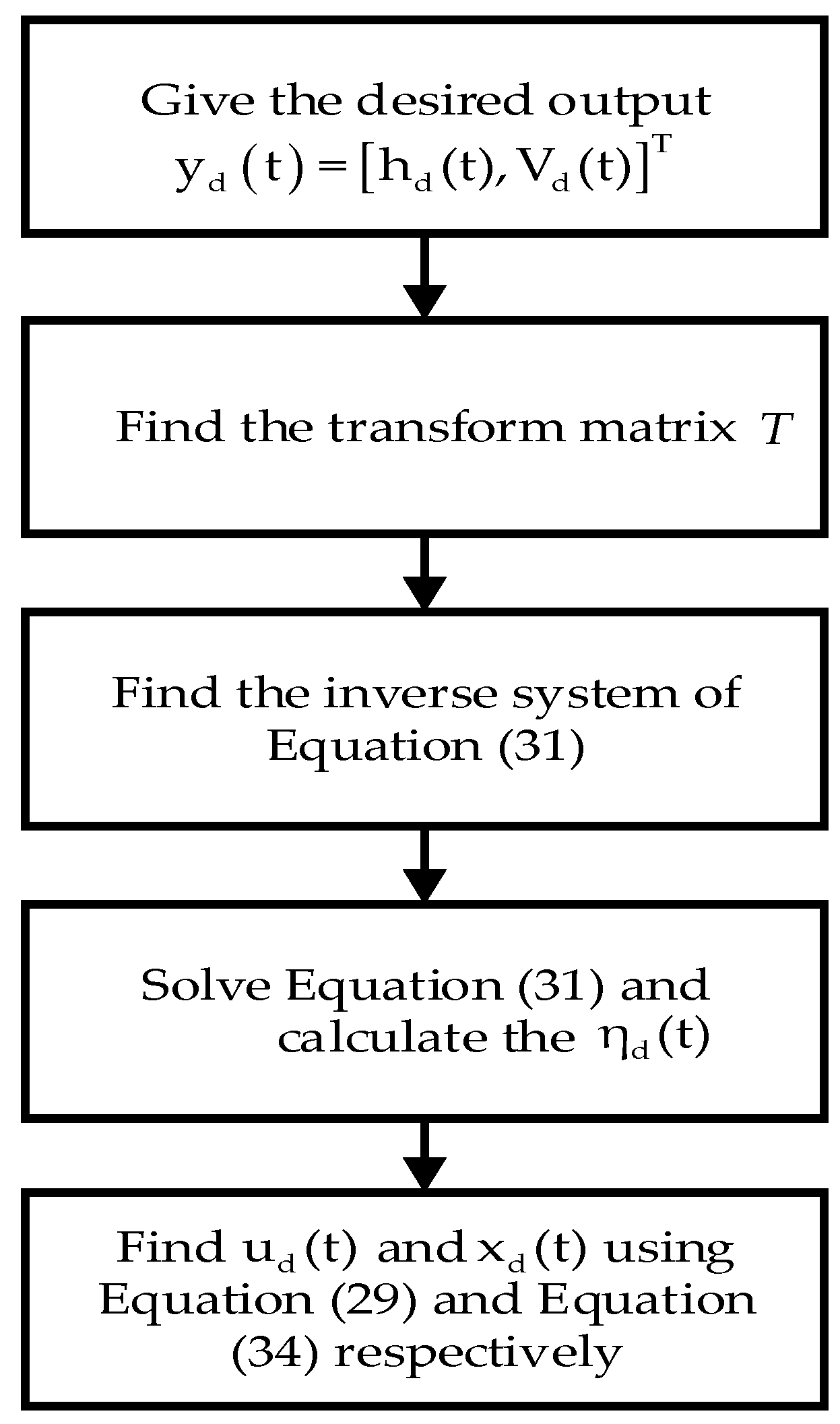
3. Design of SIRAC
3.1. The SI Algorithm
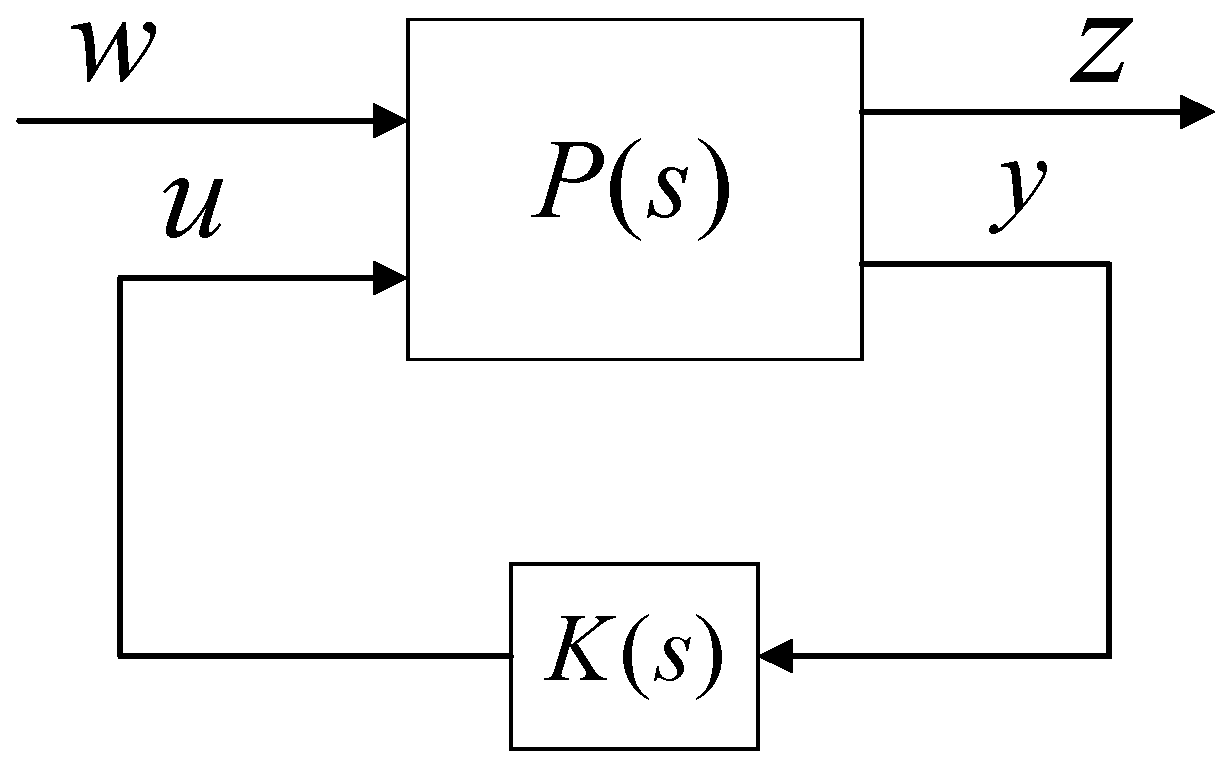
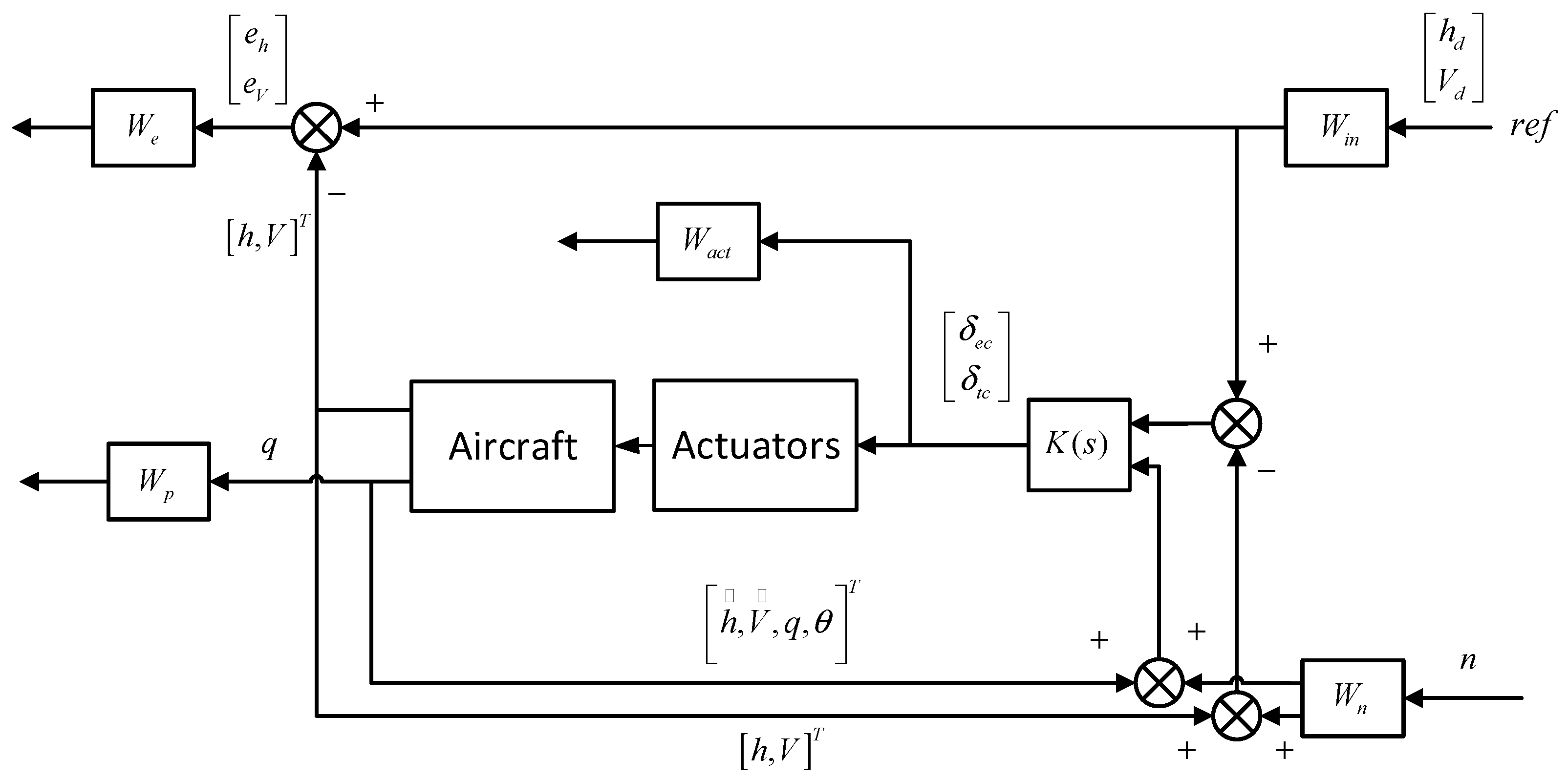
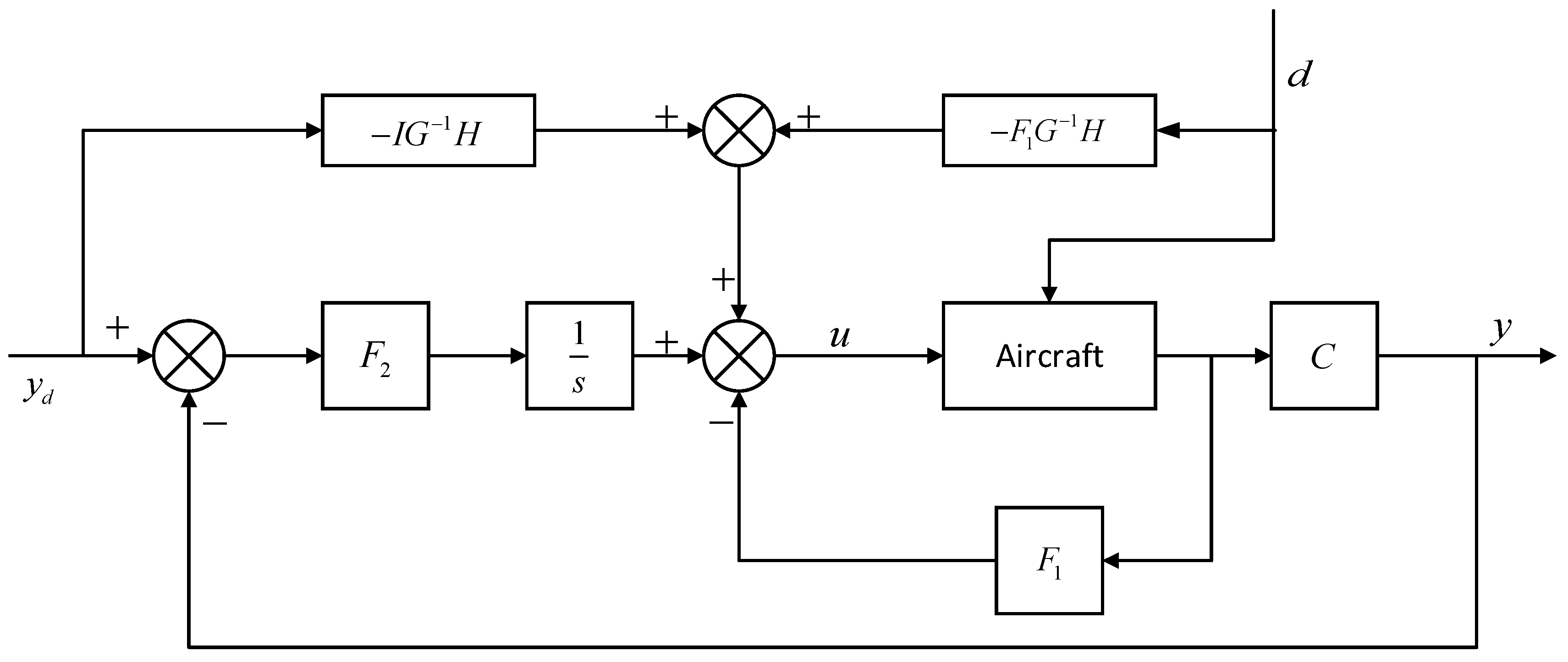
3.2. Design of RAC
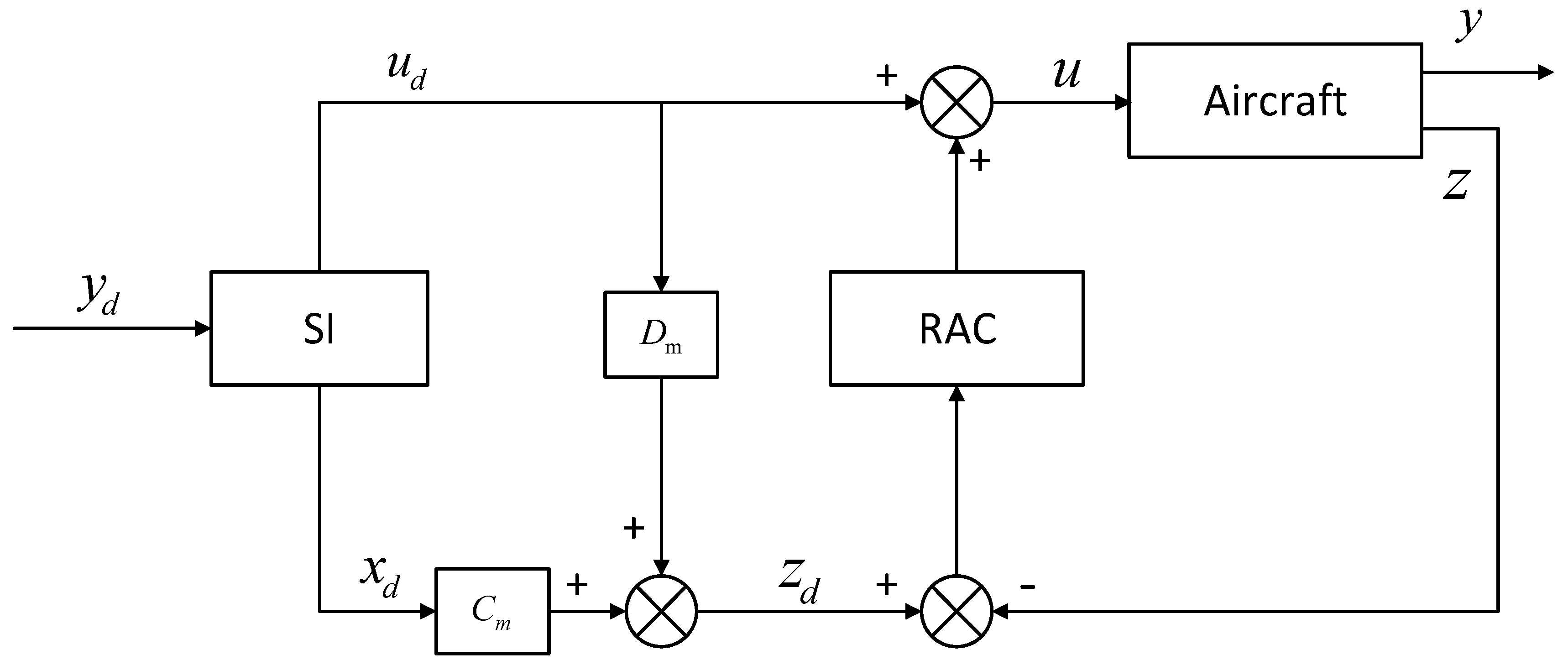
3.3. Combining the SI Algorithm and RAC
- Step 1
- Calculating .
- Step 2
- Knowing , solve Equation (29) and Equation (34) using SI algorithm to find the and .
- Step 3
- Knowing and , solve .
- Step 4
- Knowing , solve for the input of RAC: .
- Step 5
- Knowing , solve Equation (36) for the output of RAC: .
- Step 6
- Knowing , solve for the input of the aircraft: .
- Step 7
- Knowing , solve the system equation of aircraft for .
- Step 8
- Iterate over Step 3 to 7 until the landing process is done.
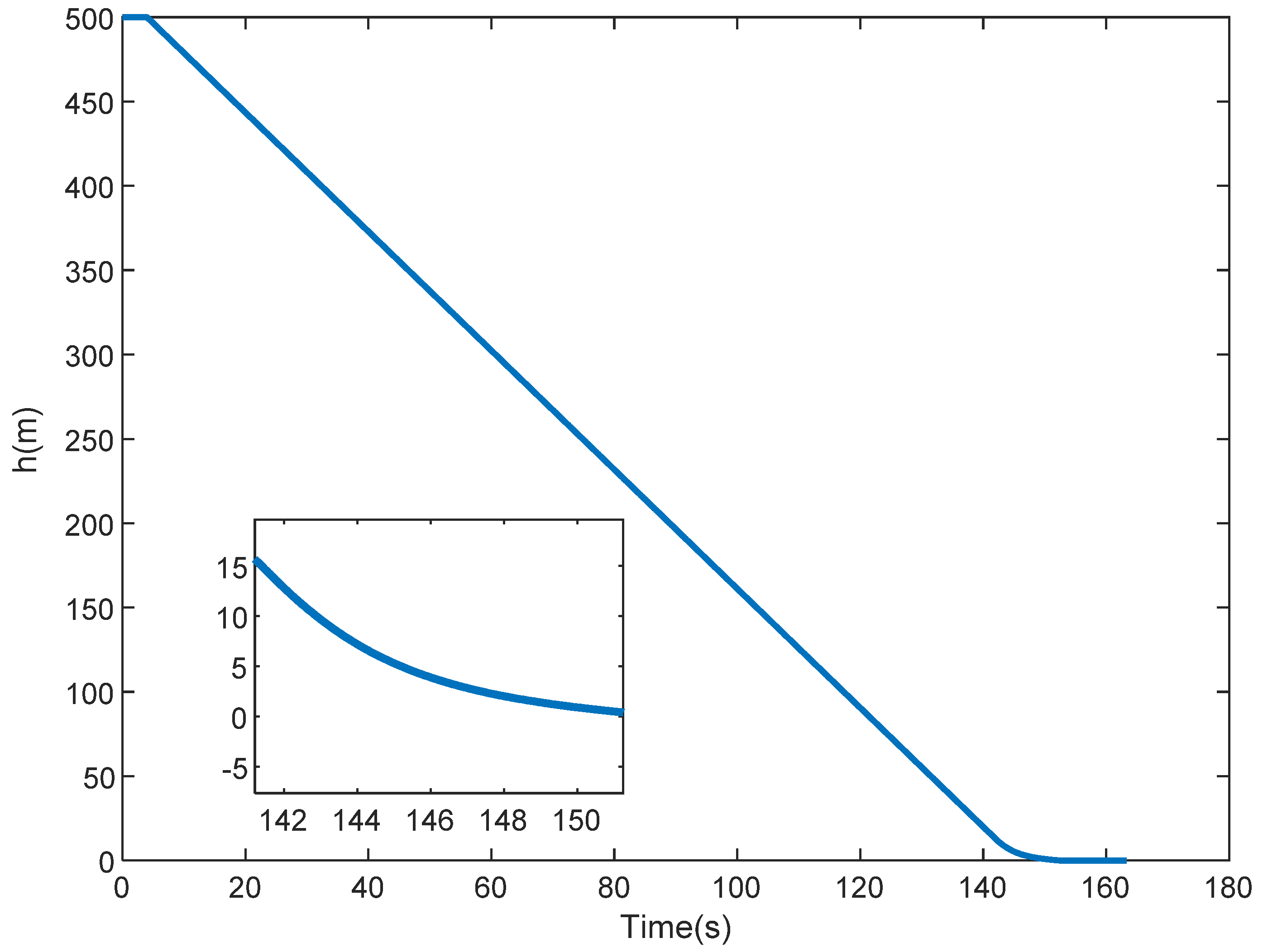
4. Simulation Analysis
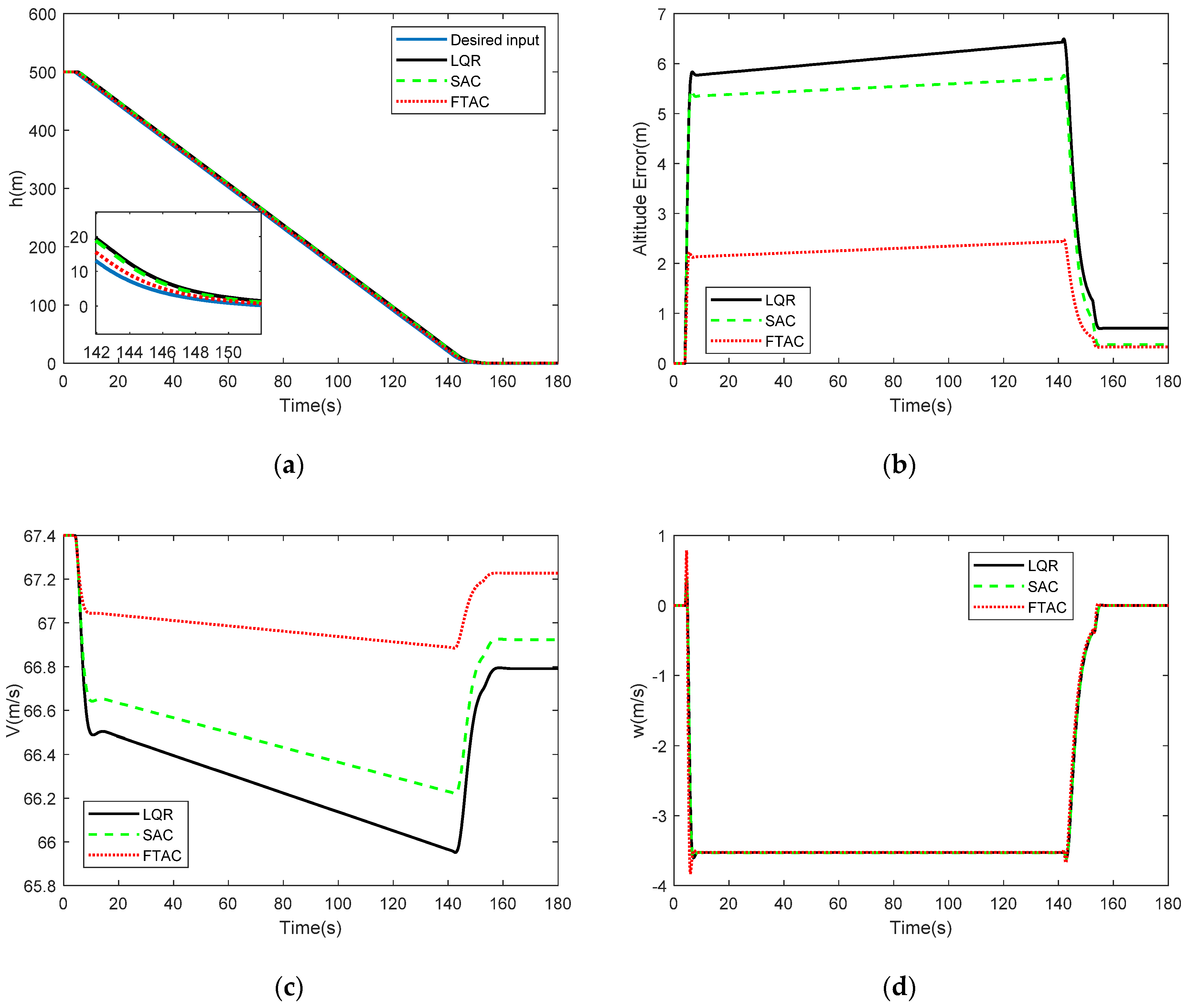
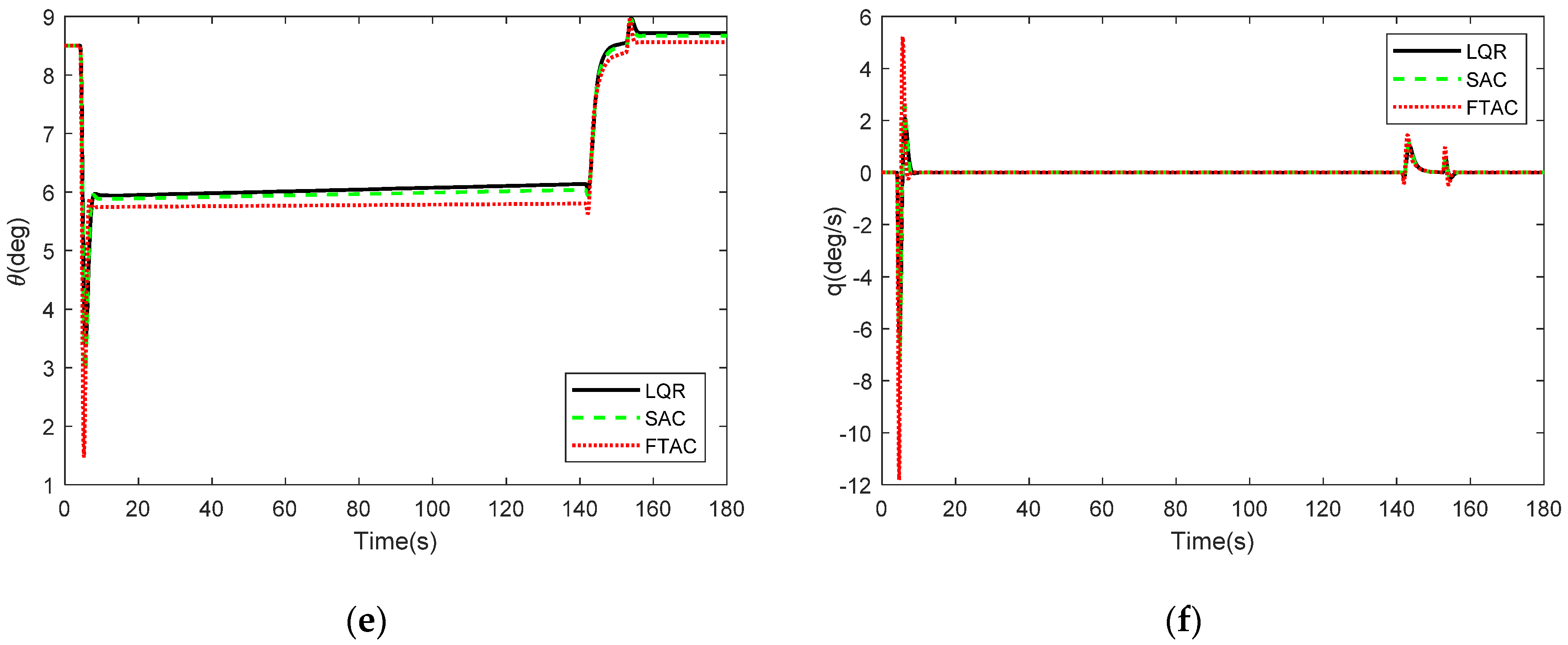
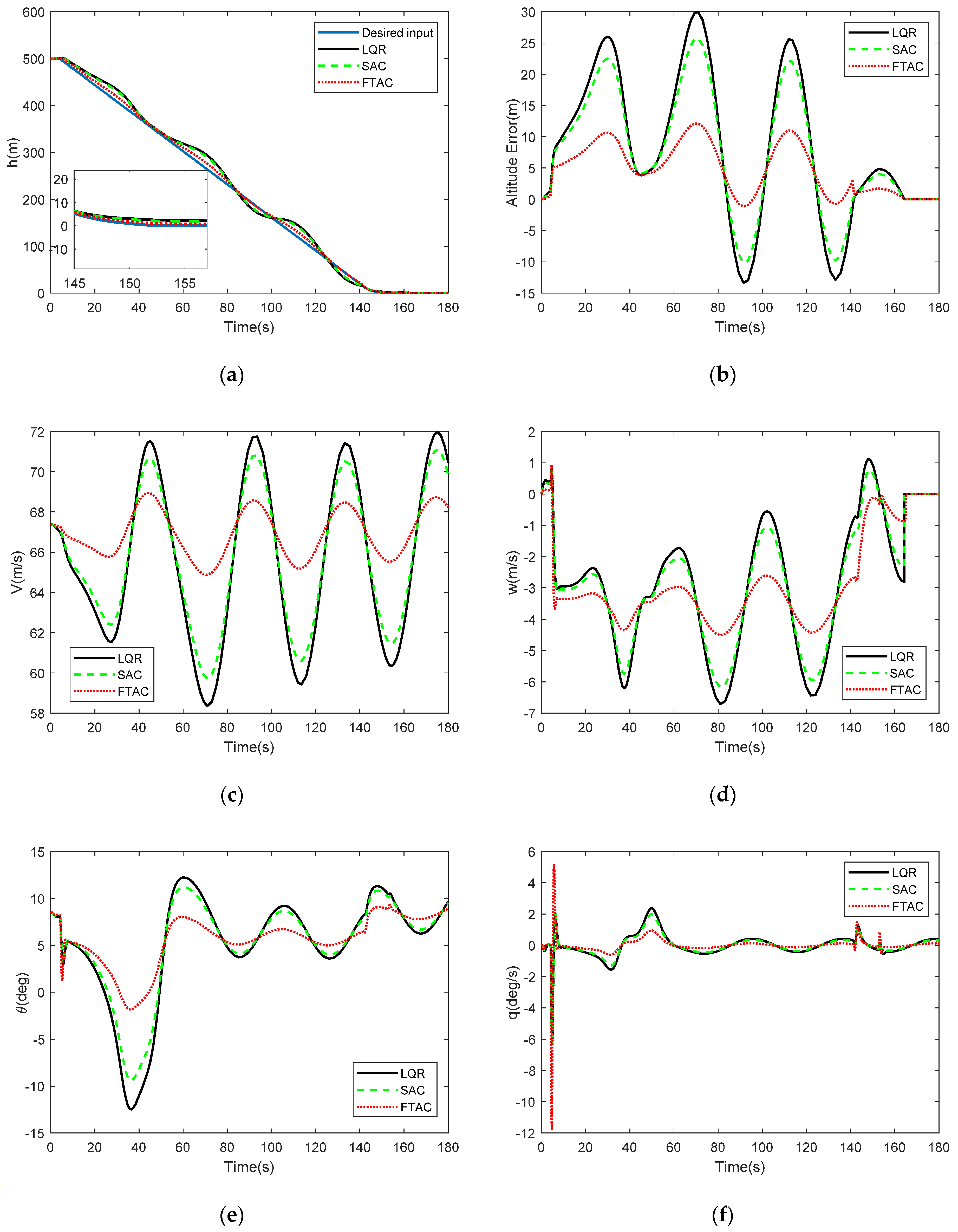
4.1. Scenario 1
4.2. Scenario 2
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Nomenclature
| aircraft mass | |
| elevator deflection angle and throttle position changing, respectively | |
| elevator command and throttle command, respectively | |
| altitude and desired altitude, respectively | |
| longitudinal speed and desired longitudinal speed, respectively | |
| angle of attack, pitch angle and flight path angle, respectively | |
| pitch rate | |
| thrust inclination angle | |
| principal moment of inertia in pitch axis | |
| pitching moment | |
| thrust, lift and drag, respectively | |
| aerodynamic coefficients of lift, drag and pitching moment, respectively | |
| angle of attack derivative of lift, drag and pitching moment, respectively | |
| elevator variation derivative of lift, drag and pitching moment, respectively | |
| angle of attack rate derivative of lift, drag and pitching moment, respectively | |
| pitch rate derivative of lift, drag and pitching moment, respectively | |
| throttle variation derivative of thrust | |
| wing reference area | |
| mean aerodynamic chord | |
| air density | |
| horizontal and vertical wind speed, respectively | |
| strengths of windshear |
References
- Siegel, D.; Hansman, R.J. Development of an Autoland System for General Aviation Aircraft. Master’s Thesis, Massachusetts Institute of Technology, Cambridge, MA, USA, 2011. [Google Scholar]
- Serra, P.; Cunha, R.; Hamel, T.; Silvestre, C.; Le Bras, F. Nonlinear image-based visual servo controller for the flare maneuver of fixed-wing aircraft using optical flow. IEEE Trans. Control Syst. Technol. 2015, 23, 570–583. [Google Scholar] [CrossRef]
- Tapia, N.D.; Simplicio, P.; Iannelli, A.; Marcos, A. Robust flare control design using structured H∞ synthesis: A civilian aircraft landing challenge. In Proceedings of the 20th IFAC World Congress, Toulouse, France, 9–14 July 2017; pp. 3971–3976. [Google Scholar]
- Sadat-Hoseini, H.; Fazelzadeh, S.A.; Rasti, A.; Marzocca, P. Final approach and flare control of a flexible aircraft in crosswind landings. J. Guid. Control Dyn. 2013, 36, 946–957. [Google Scholar] [CrossRef]
- Magni, J.F. Multimodel eigenstructure assignment in flight-control design. Aerosp. Sci. Technol. 1999, 3, 141–151. [Google Scholar] [CrossRef]
- Wang, Y.W.; Li, Q.F.; Lu, B. Automatic landing system design via multivariable model reference adaptive control. Aerosp. Syst. 2018, 1, 63–71. [Google Scholar] [CrossRef]
- Rao, D.V.; Go, T.H. Automatic landing system design using sliding mode control. Aerosp. Sci. Technol. 2014, 32, 180–187. [Google Scholar]
- Juang, J.G.; Yu, S.T. Disturbance encountered landing system design based on sliding mode control with evolutionary computation and cerebellar model articulation controller. Appl. Math. Model. 2015, 39, 5862–5881. [Google Scholar] [CrossRef]
- Lan, C.E.; Chang, R.C. Unsteady aerodynamic effects in landing operation of transport aircraft and controllability with fuzzy-logic dynamic inversion. Aerosp. Sci. Technol. 2018, 78, 354–363. [Google Scholar] [CrossRef]
- Juang, J.G.; Chio, J.Z. Fuzzy modelling control for aircraft automatic landing system. Int. J. Syst. Sci. 2007, 36, 77–87. [Google Scholar] [CrossRef]
- Tamkaya, K.; Ucun, L.; Ustoglu, I. H∞-based model following method in autolanding systems. Aerosp. Sci. Technol. 2019, 94, 105379. [Google Scholar] [CrossRef]
- Theis, J.; Ossmann, D.; Thielecke, F.; Pfifer, H. Robust autopilot design for landing a large civil aircraft in crosswind. Control Eng. Pract. 2018, 76, 54–64. [Google Scholar] [CrossRef]
- Biannic, J.M.; Roos, C. Flare control law design via multi-channel ∞ synthesis: Illustration on a freely available nonlinear aircraft benchmark. In Proceedings of the 2015 American Control Conference (ACC), Chicago, IL, USA, 1–3 July 2015; pp. 1303–1308. [Google Scholar]
- Shevchenko1, A.; Pavlov, B.; Nachinkina, G. Methods for predicting unsteady takeoff and landing trajectories of the aircraft. In Proceedings of the AIPC, Sydney, Australia, 2–5 July 2017; Volume 1798. [Google Scholar]
- Clean Sky. Available online: https://www.cleansky.eu/ (accessed on 2 February 2020).
- Devasia, S.; Chen, D.; Paden, B. Nonlinear inversion-based output tracking. IEEE Trans. Autom. Control 1996, 41, 930–942. [Google Scholar] [CrossRef]
- Chen, D.; Paden, B. Stable inversion of nonlinear non-minimum phase systems. Int. J. Control 1996, 64, 81–97. [Google Scholar] [CrossRef]
- Olivier, B.; Guaraci, J.; Robert, S. A stable inversion method for feedforward control of constrained flexible multibody systems. ASME J. Comput. Nonlinear Dyn. 2013, 9, 1–7. [Google Scholar]
- Maghzaoui, C.; Jerbi, H.; Abdelkrim, M. A MIMO time-varying system control via a stable dynamic inversion methodology: Case of an induction machine. In Proceedings of the Computational Intelligence, Communication Systems and Networks, International Conference, Bali, Indonesia, 26–28 July 2011; pp. 146–151. [Google Scholar]
- Zhang, J.; Zhang, P. Precise decoupling tracking of airspeed and altitude for UAV based on causal solution of stable inversion. Chin. J. Aeronaut. 2009, 22, 307–315. [Google Scholar]
- Mancisidor, J.; Pena-Sevillano, A.; Barcena, R.; Franco, O.; Munoa, J.; De Lacalle, L.N.L. Comparison of model free control strategies for chatter suppression by an inertial actuator. Int. J. Mechatron. Manuf. Syst. 2019, 12, 164–179. [Google Scholar]
- Bryson, A.E. Control of Spacecraft and Aircraft; Princeton University Press: Princeton, NJ, USA, 1994; pp. 137–155. [Google Scholar]
- Roskam, J. Airplane Flight Dynamics and Automatic Flight Controls: Part I; Design, Analysis and Research Corporation: Lawrence, KS, USA, 2001; Volume 1, pp. 65–182. [Google Scholar]
- McLean, D. Automatic Flight Control System; Prentice Hall: Hertfordshire, NJ, USA, 1990; pp. 537–576. [Google Scholar]
- Parkinson, B.W.; O’Connor, M.L.; Fitzgibbon, K.T. Aircraft Automatic Approach and Landing Using GPS. In Global Positioning System: Theory and Applications; American Institute of Aeronautics and Astronautics, Inc.: Reston, VA, USA, 1996; Volume 2, pp. 397–425. [Google Scholar]
- Woodfield, A.; Woods, J. Worldwide Experience of Wind Shear during 1981–1982. Technical Report; Royal Aircraft Establishment Bedford: Bedford, UK, 1983. [Google Scholar]
- Zhao, Y. Optimal Control of an Aircraft Flying through a Downburst. Ph.D. Thesis, Stanford University, Stanford, CA, USA, 1989. [Google Scholar]
- Thomas, R.; Stephen, C. Review of the Carrier Approach Criteria for Carrier-Based Aircraft Phase I. Naval Air Syst. Command. 2002, 71, 12–24. [Google Scholar]
- Wang, J.-G.; Dong, X.-M.; Xue, J.-P. Aircraft longitudinal automatic landing control law design based on stable inversion. Flight Dyn. 2011, 29, 33–36. [Google Scholar]
- Zhou, K.; Doyle, J.C.; Glover, K. Robust and Optimal Control; Prentice Hall: Bergen, NJ, USA, 1996. [Google Scholar]
- Scherer, C.; Gahinet, P.; Chilali, M. Multiobjective output-feedback control via LMI optimization. IEEE Trans. Autom. Control 1997, 42, 896–911. [Google Scholar] [CrossRef]
- Pinilla, L.S.; Rodríguez, R.L.; Gandarias, N.T.; De Lacalle, L.N.L.; Farokhad, M.R. TRLs 5–7 Advanced Manufacturing Centres, Practical Model to Boost Technology Transfer in Manufacturing. Sustainability 2019, 11, 4890. [Google Scholar] [CrossRef]

| Parameter | Value | Unit | Description |
|---|---|---|---|
| 250,000 | kg | Mass | |
| 510 | m2 | Wing reference area | |
| 8.3 | m | Mean aerodynamic chord | |
| kg·m2 | Pitch axis inertia | ||
| 0.044 | rad | Thrust inclination angle | |
| 1.71 | |||
| 5.67 | 1/rad | Angle of attack derivative for lift | |
| 0.36 | 1/rad | Elevator variation derivative for lift | |
| 6.7 | s/rad | Angle of attack rate derivative for lift | |
| 5.65 | s/rad | Pitch rate derivative for lift | |
| 0.263 | |||
| 1.13 | 1/rad | Angle of attack derivative for drag | |
| 0 | 1/rad | Elevator variation derivative for drag | |
| 0 | s/rad | Angle of attack rate derivative for drag | |
| 0 | s/rad | Pitch rate derivative for drag | |
| −0.093 | |||
| −1.45 | 1/rad | Angle of attack derivative for moment | |
| −1.40 | 1/rad | Elevator variation derivative for moment | |
| −3.3 | s/rad | Angle of attack rate derivative for moment | |
| −21.4 | s/rad | Pitch rate derivative for moment | |
| 382.572 | kN | ||
| 7801.63 | kN/rad | Throttle variation derivative for thrust |
| Parameters | Value | Unit | Description |
|---|---|---|---|
| 0 | m | Altitude | |
| 67.4 | m/s | Initial speed | |
| 1.225 | kg/m3 | Air density | |
| 0.148 | rad | Trim angle of attack | |
| 0 | rad | Trim flight path angle |
| 0.35(rad) | 0.26(rad/s) | 0.088(rad) | 0.017(rad/s) |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, X.; Sang, Y.; Zhou, G. Combining Stable Inversion and H∞ Synthesis for Trajectory Tracking and Disturbance Rejection Control of Civil Aircraft Autolanding. Appl. Sci. 2020, 10, 1224. https://doi.org/10.3390/app10041224
Wang X, Sang Y, Zhou G. Combining Stable Inversion and H∞ Synthesis for Trajectory Tracking and Disturbance Rejection Control of Civil Aircraft Autolanding. Applied Sciences. 2020; 10(4):1224. https://doi.org/10.3390/app10041224
Chicago/Turabian StyleWang, Xudong, Yuanjun Sang, and Guangrui Zhou. 2020. "Combining Stable Inversion and H∞ Synthesis for Trajectory Tracking and Disturbance Rejection Control of Civil Aircraft Autolanding" Applied Sciences 10, no. 4: 1224. https://doi.org/10.3390/app10041224
APA StyleWang, X., Sang, Y., & Zhou, G. (2020). Combining Stable Inversion and H∞ Synthesis for Trajectory Tracking and Disturbance Rejection Control of Civil Aircraft Autolanding. Applied Sciences, 10(4), 1224. https://doi.org/10.3390/app10041224





