Numerical Study on the Evolution Mechanism of the Crater under a Millisecond Laser
Abstract
1. Introduction
2. Numerical Simulation
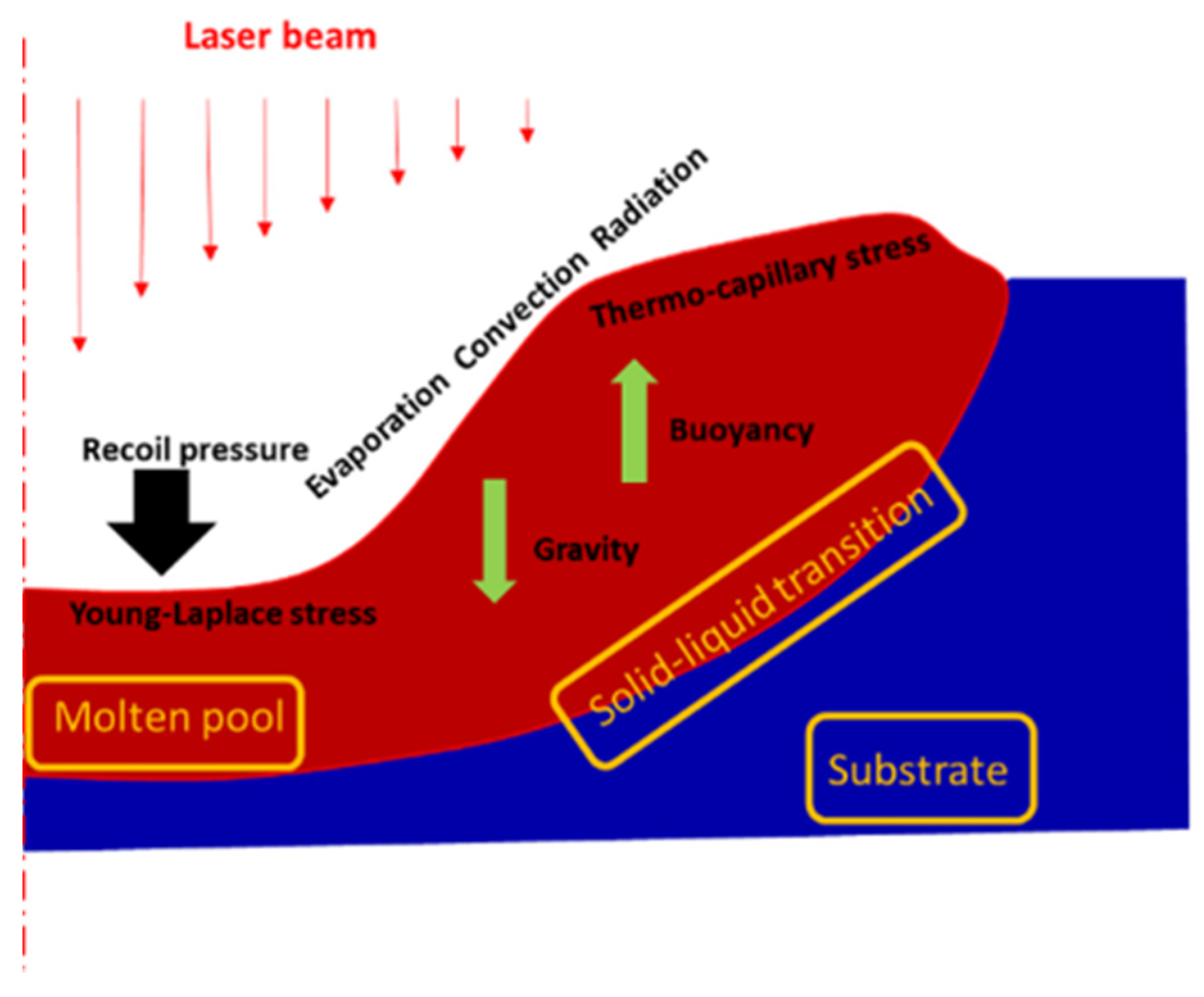
2.1. Heat Transfer Governing Equation and Boundary Conditions
2.1.1. Heat Transfer Governing Equation
2.1.2. Heat Transfer Boundary Conditions
2.2. Laminar Governing Equations and Boundary Conditions
2.2.1. Laminar Governing Equations
2.2.2. Laminar Boundary Conditions
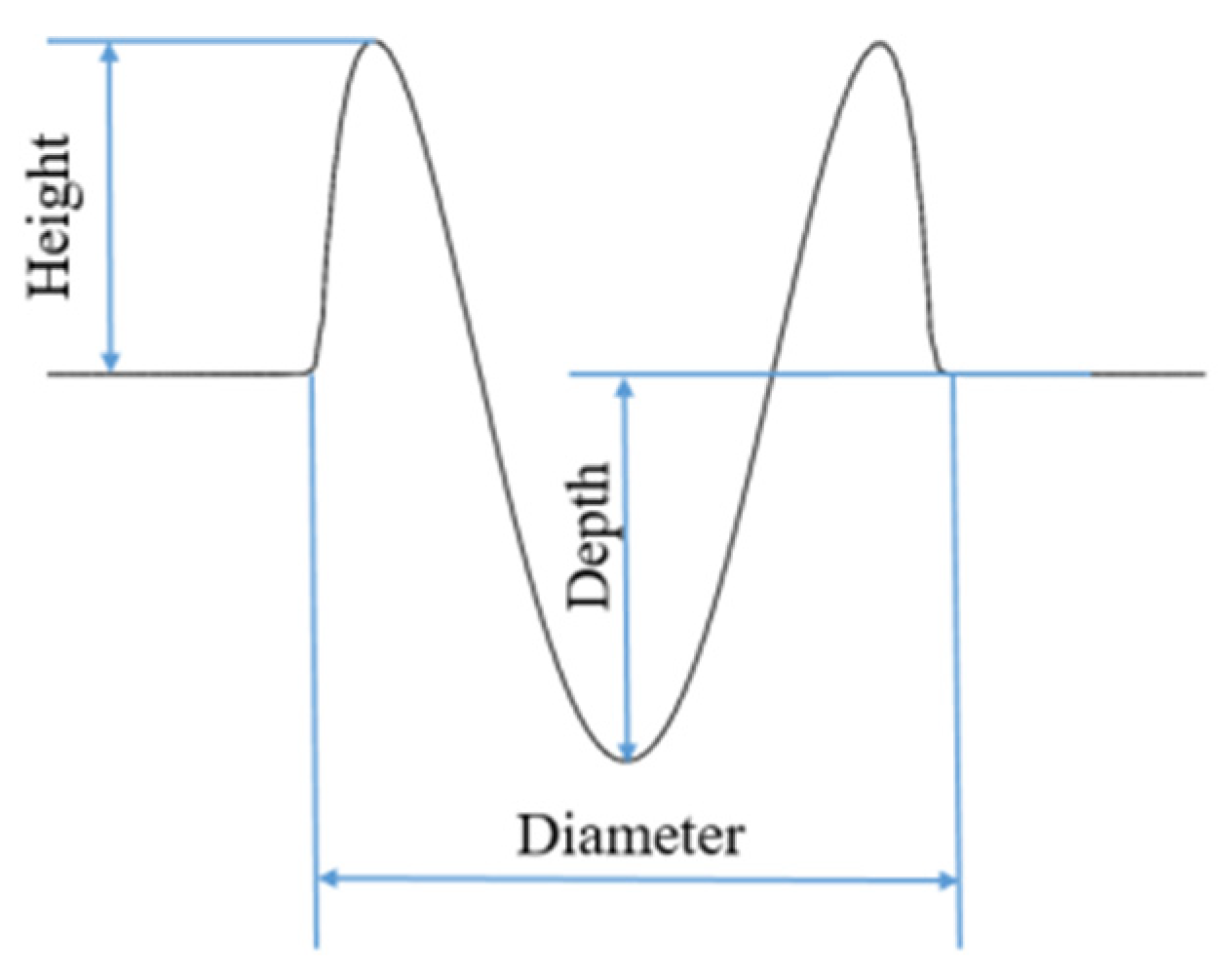
2.3. Deformation Geometry and Meshing Mesh
2.4. Experimental Method
3. Results and Discussion
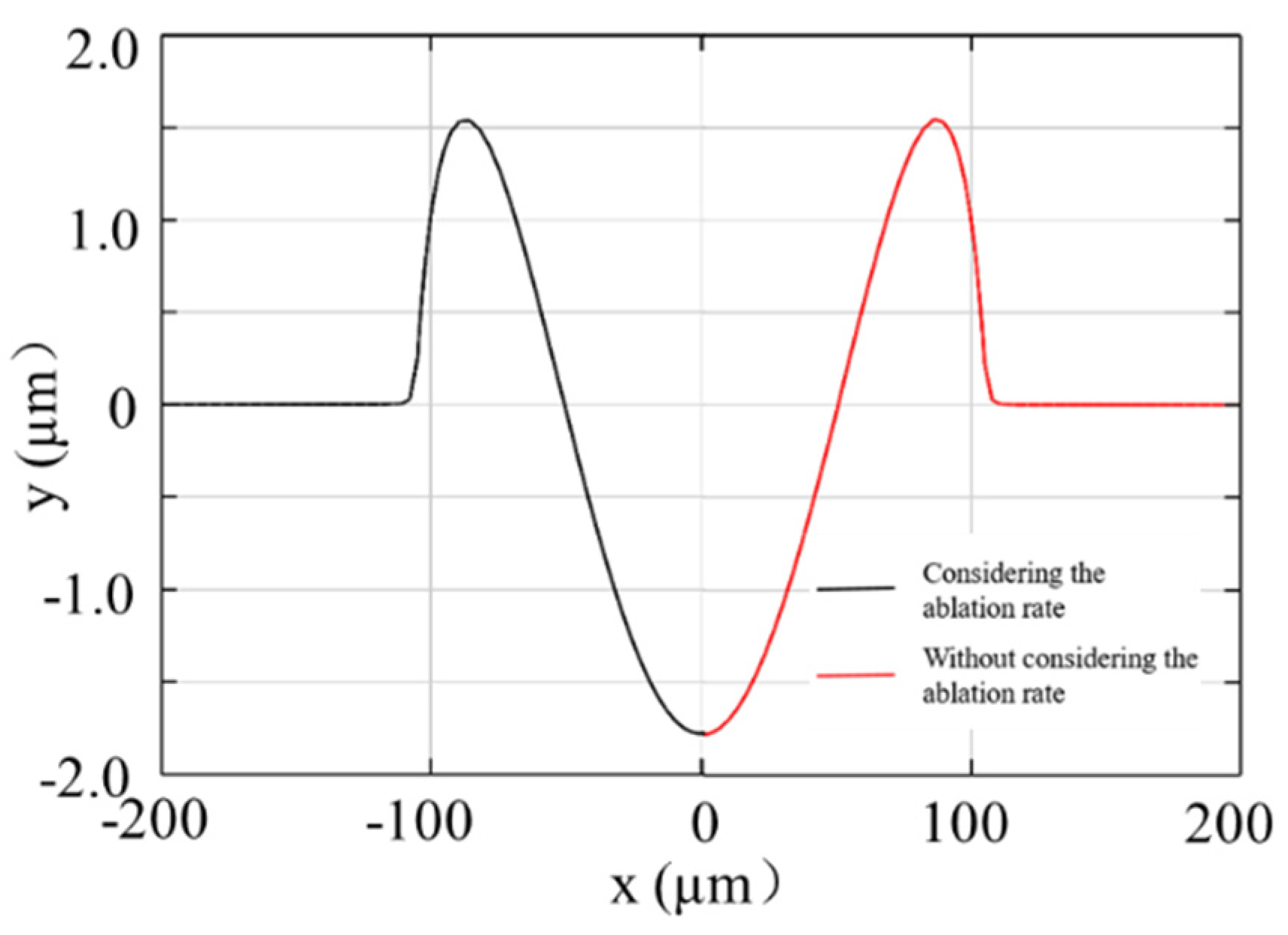
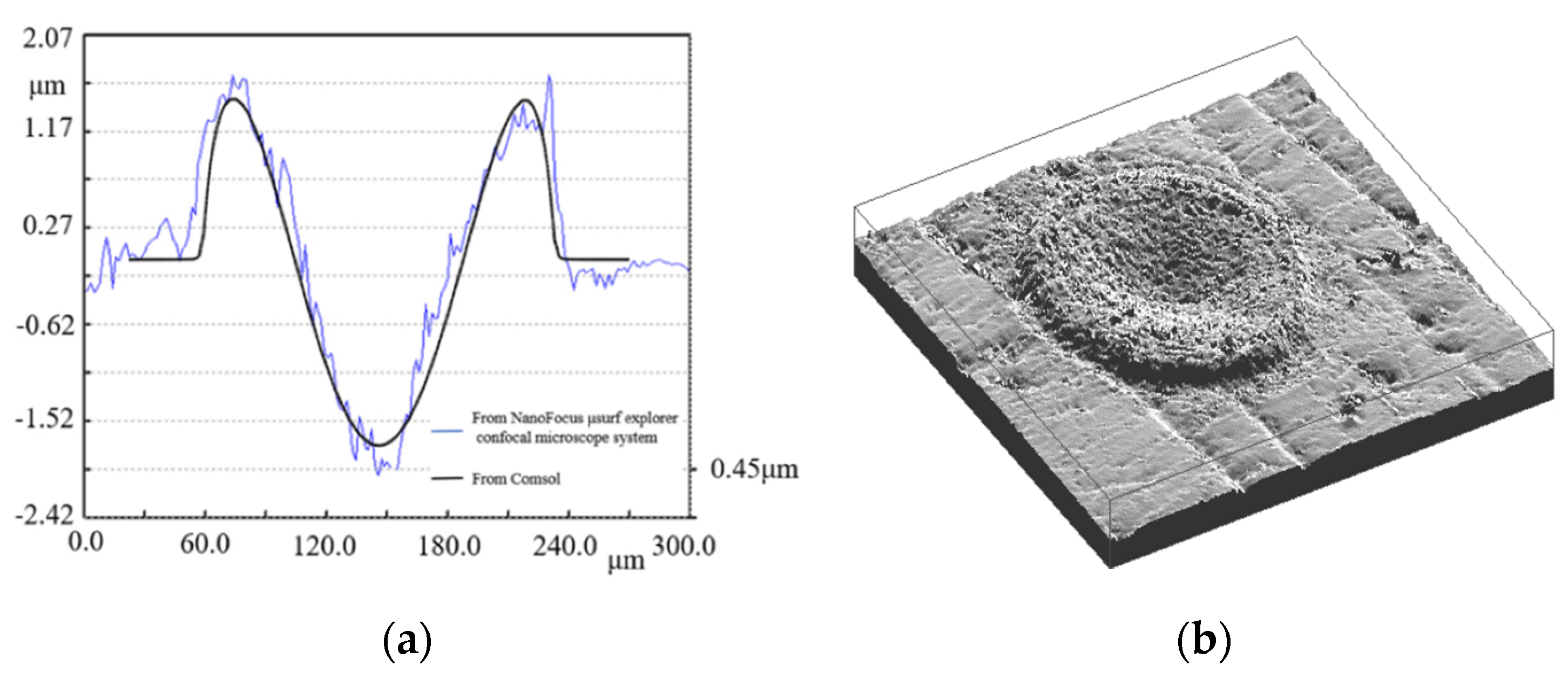
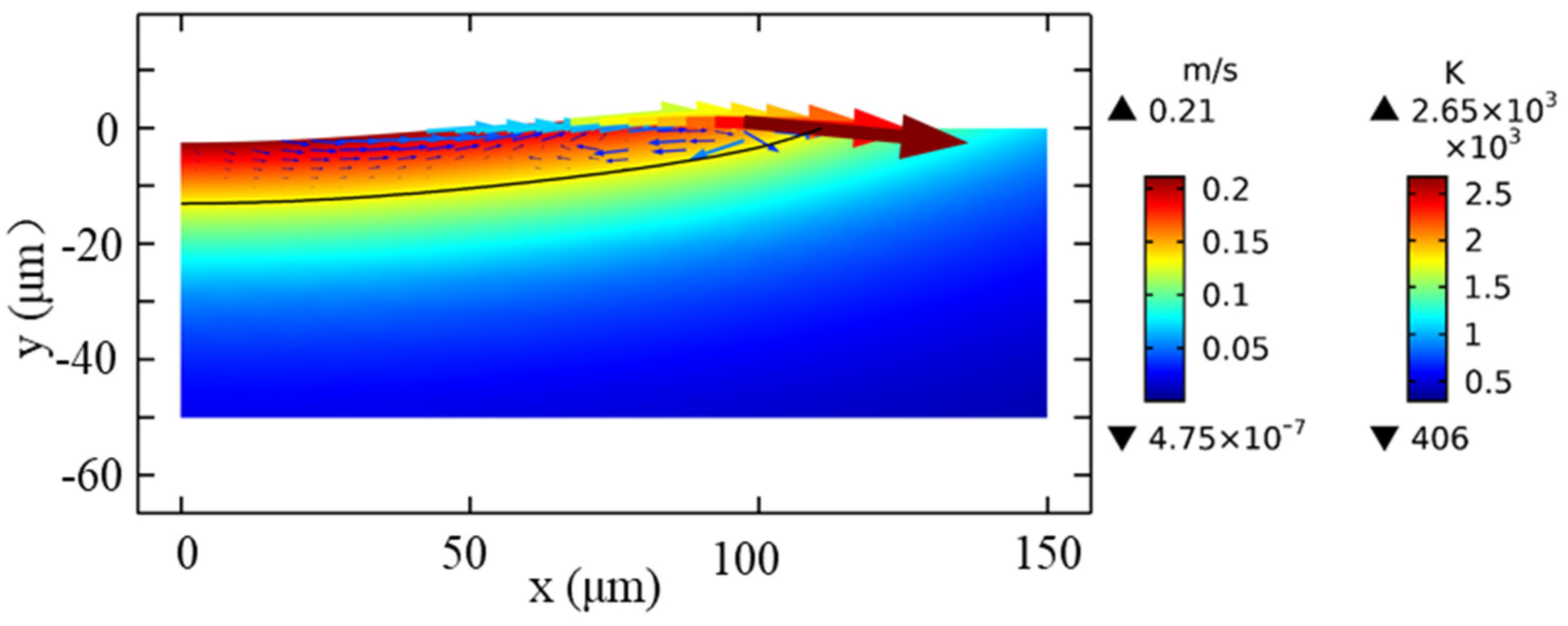
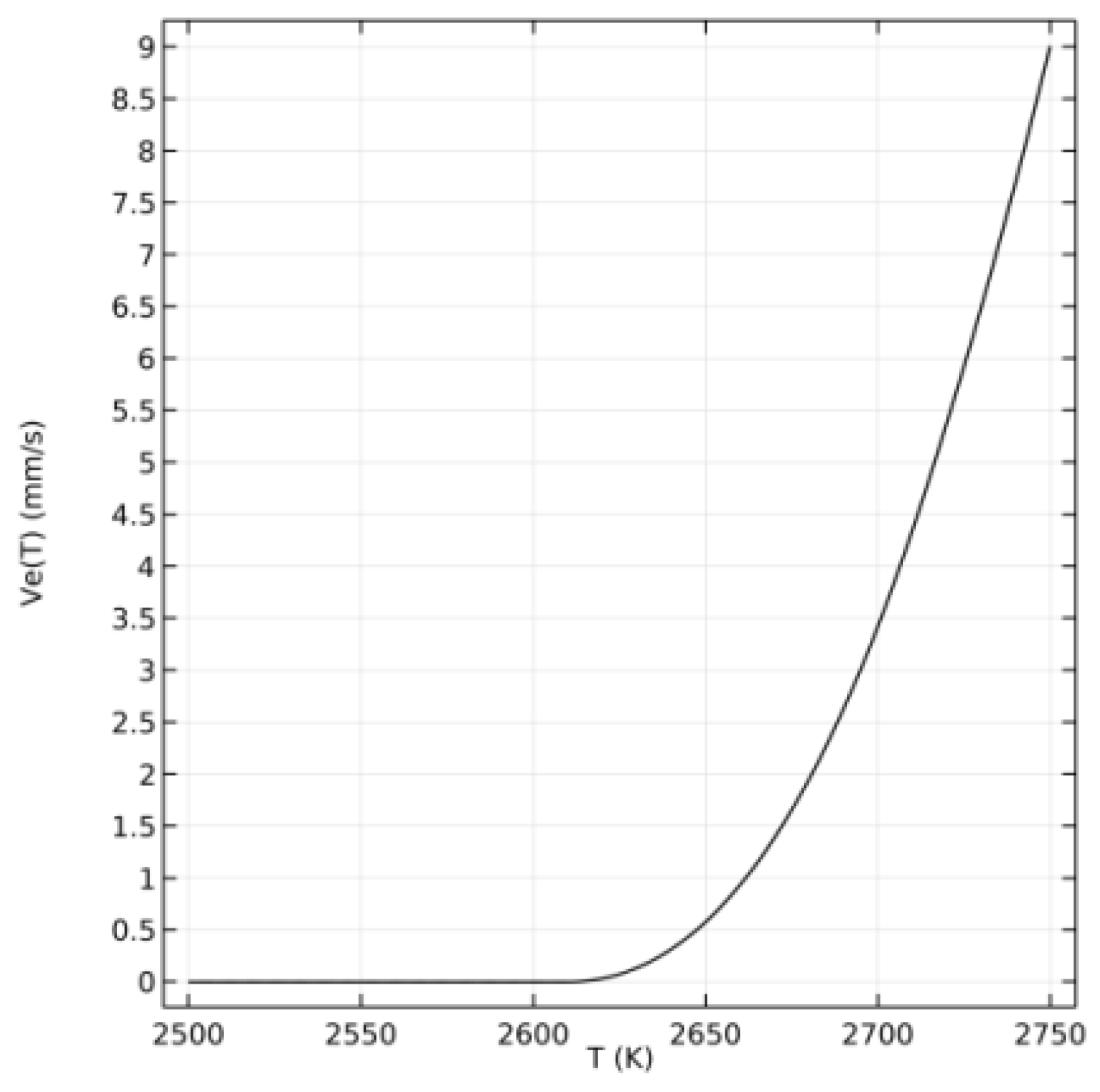
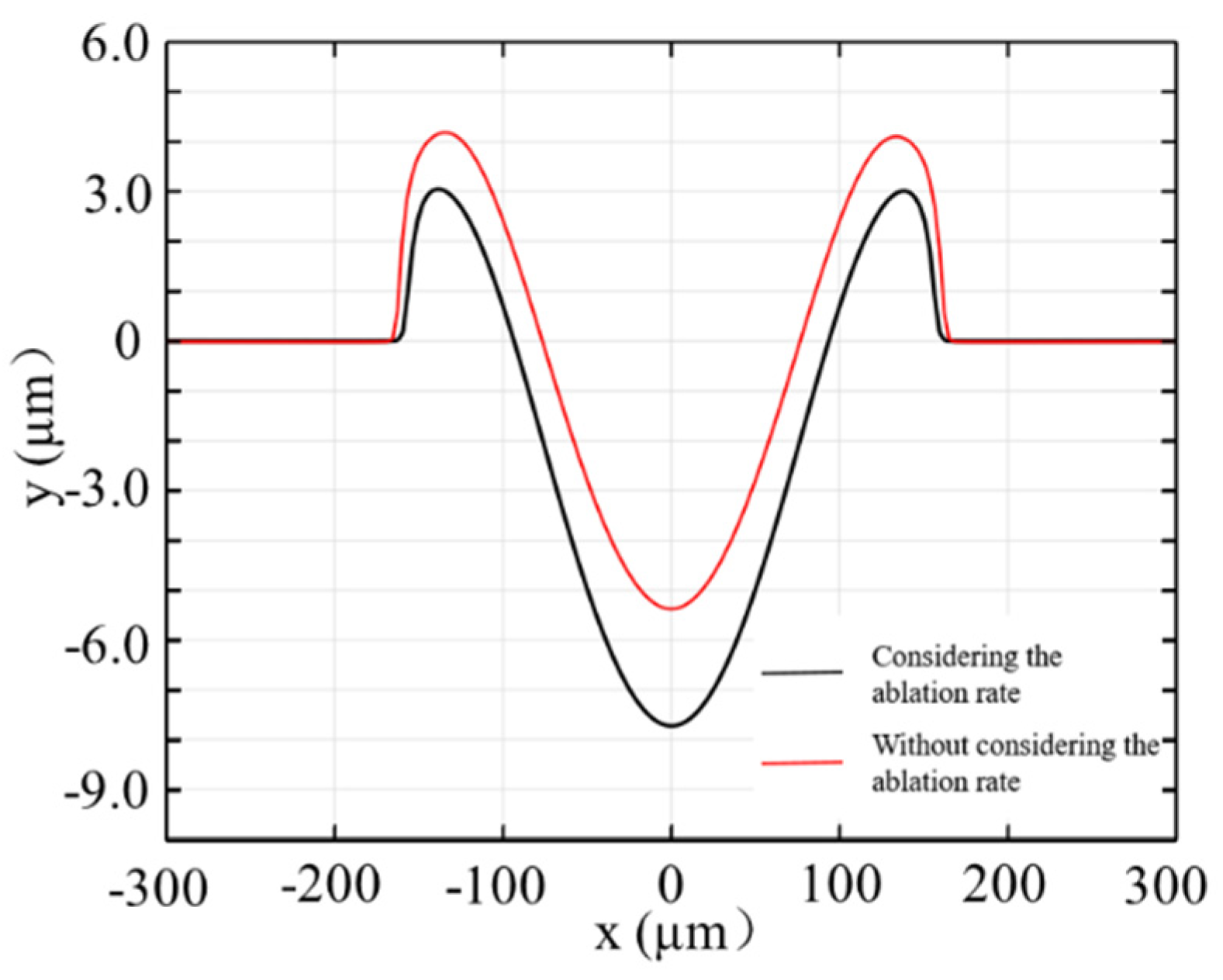
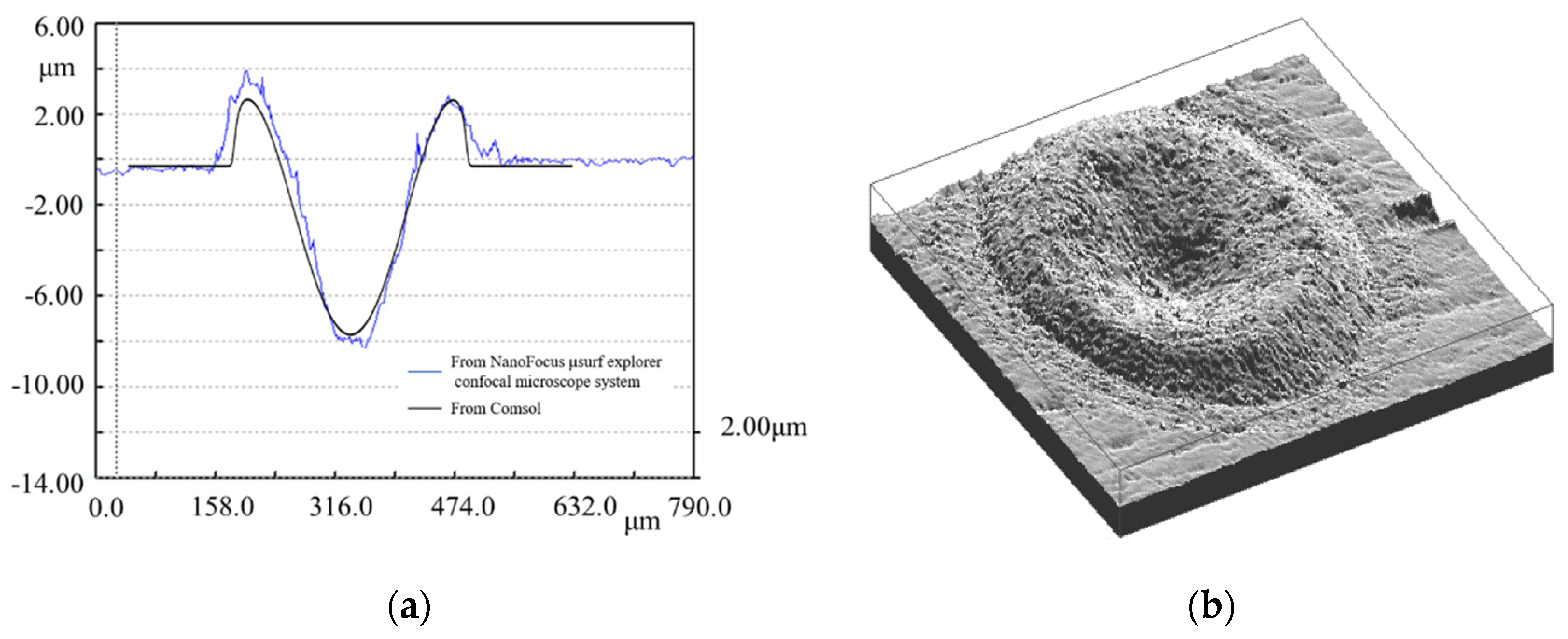
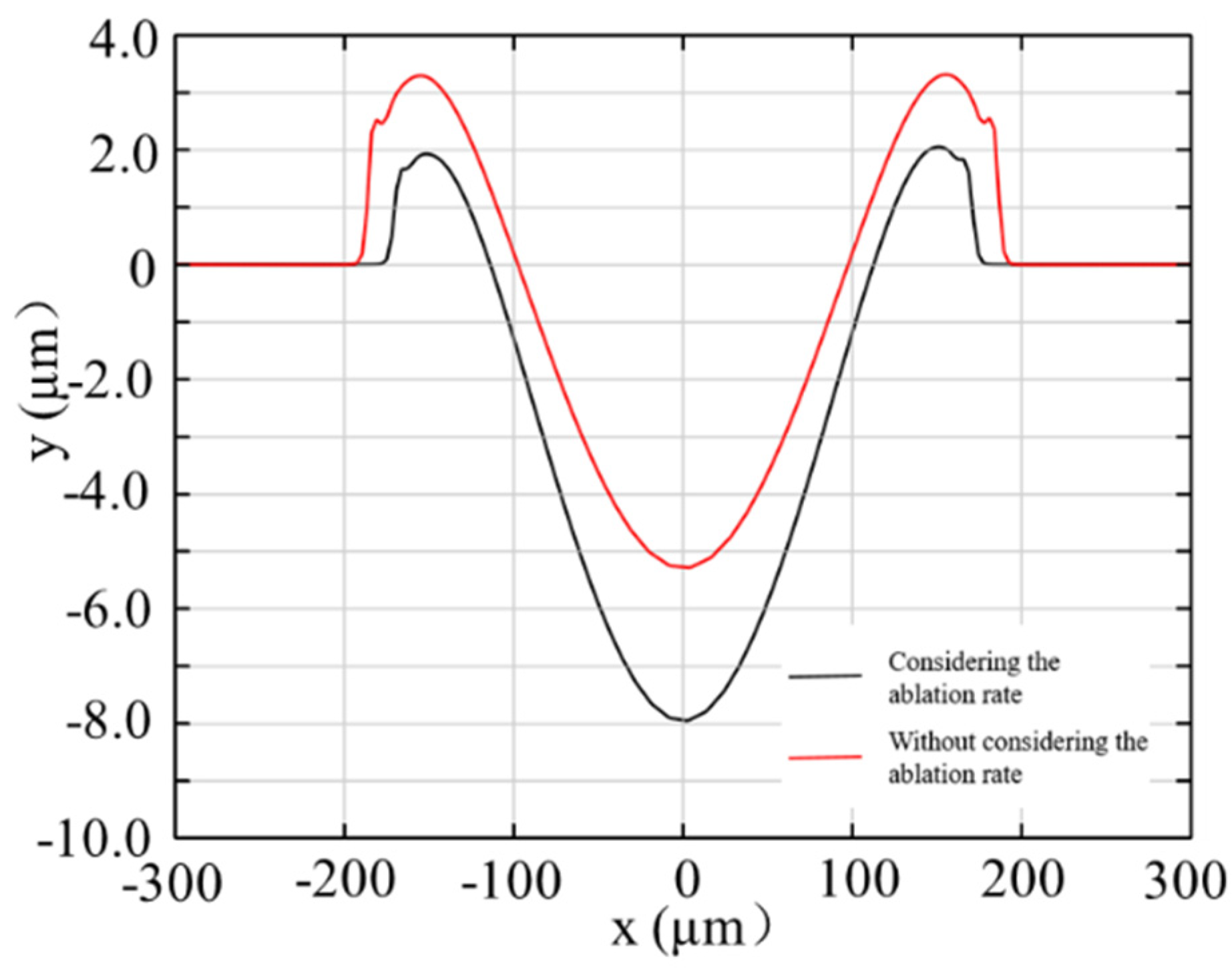
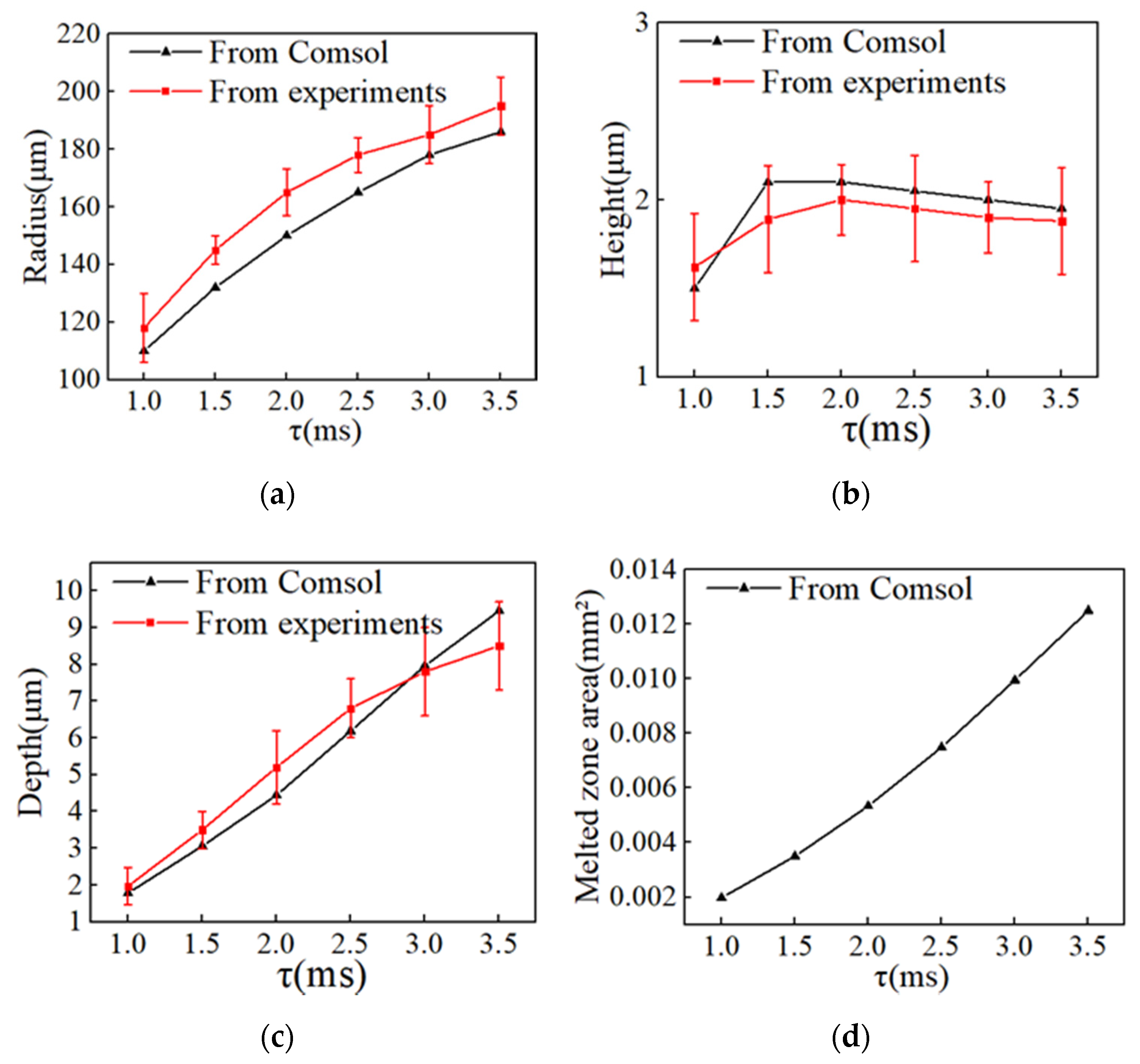
3.1. Effect of Ablation Rate on Morphology and Verification of Numerical Model
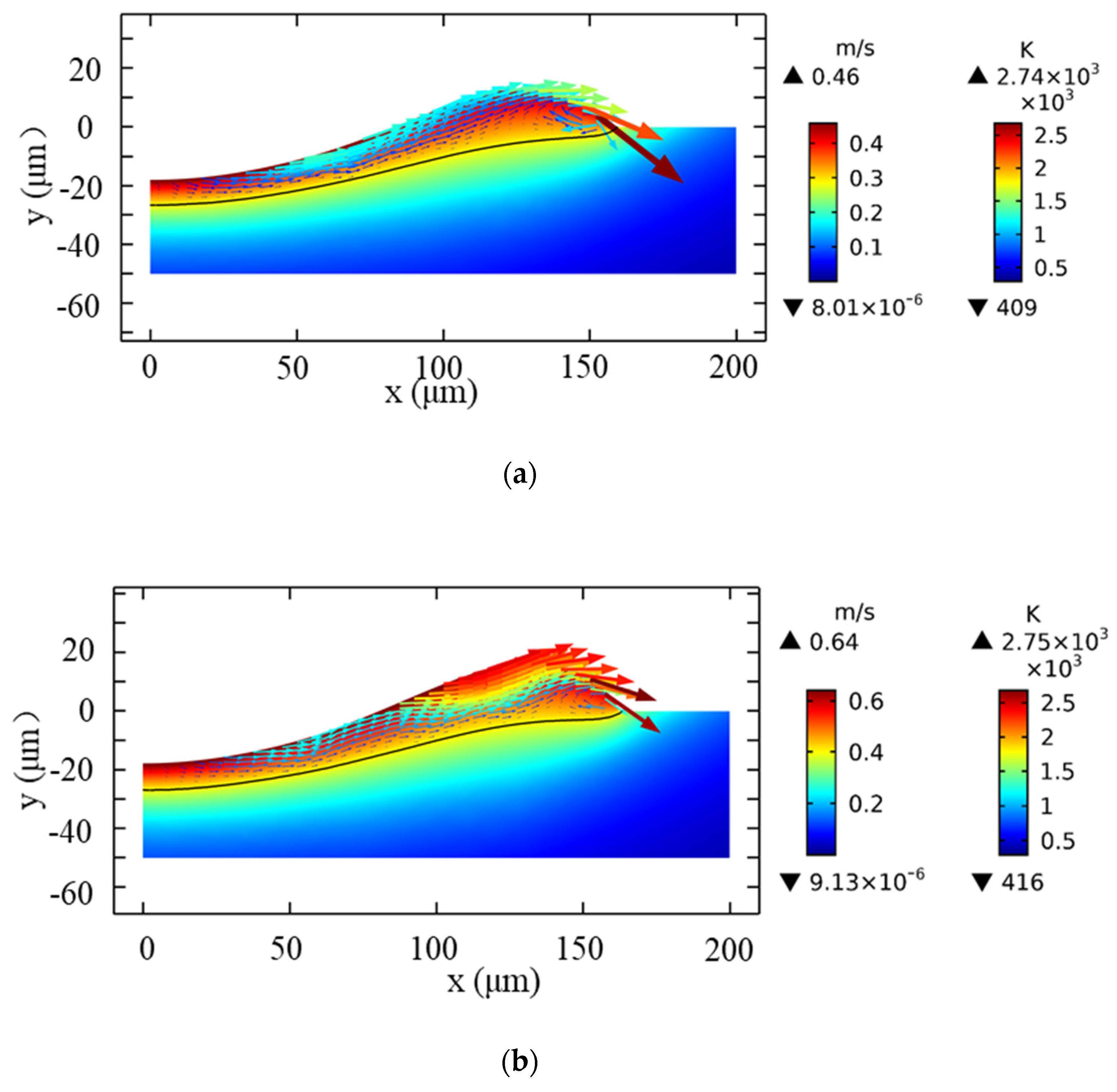
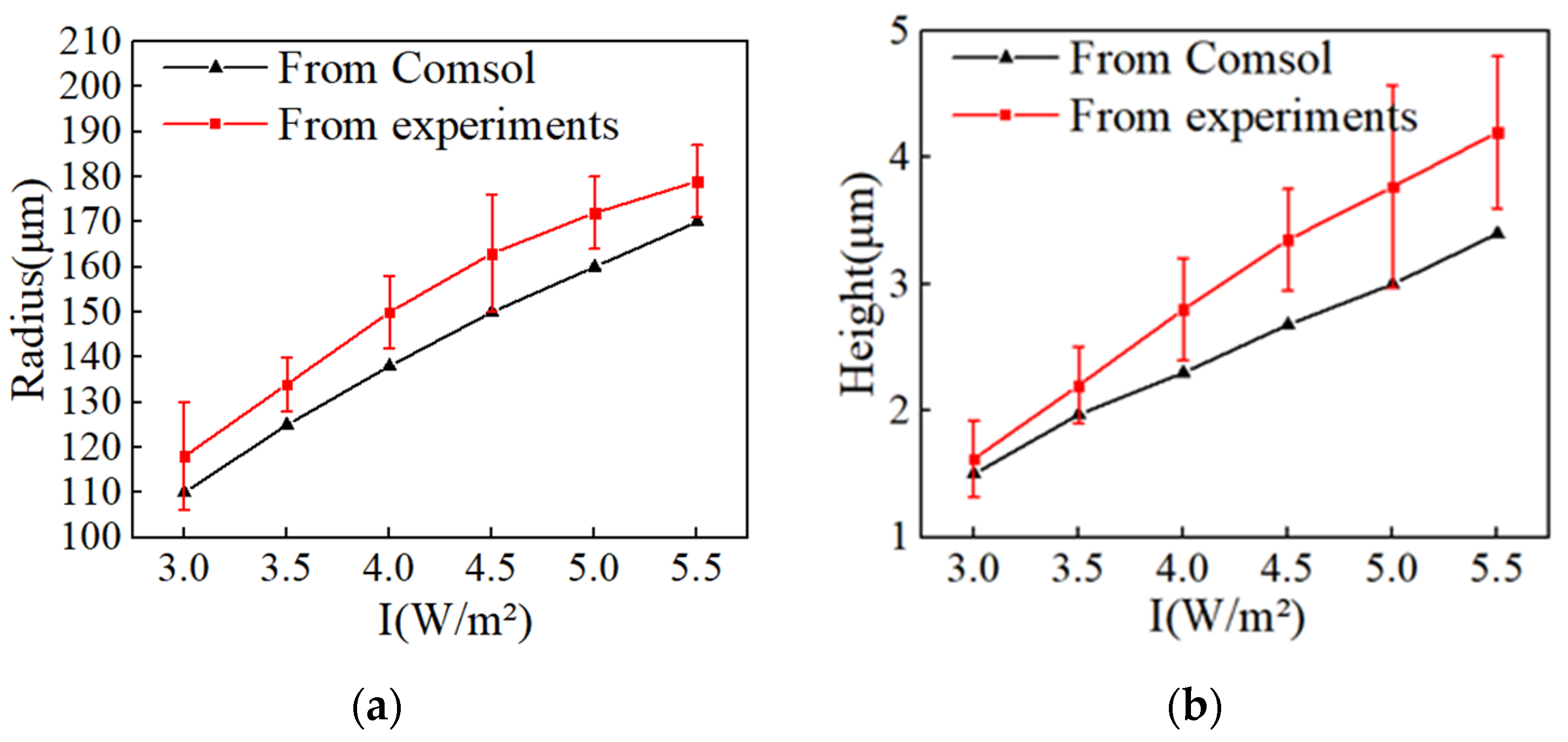
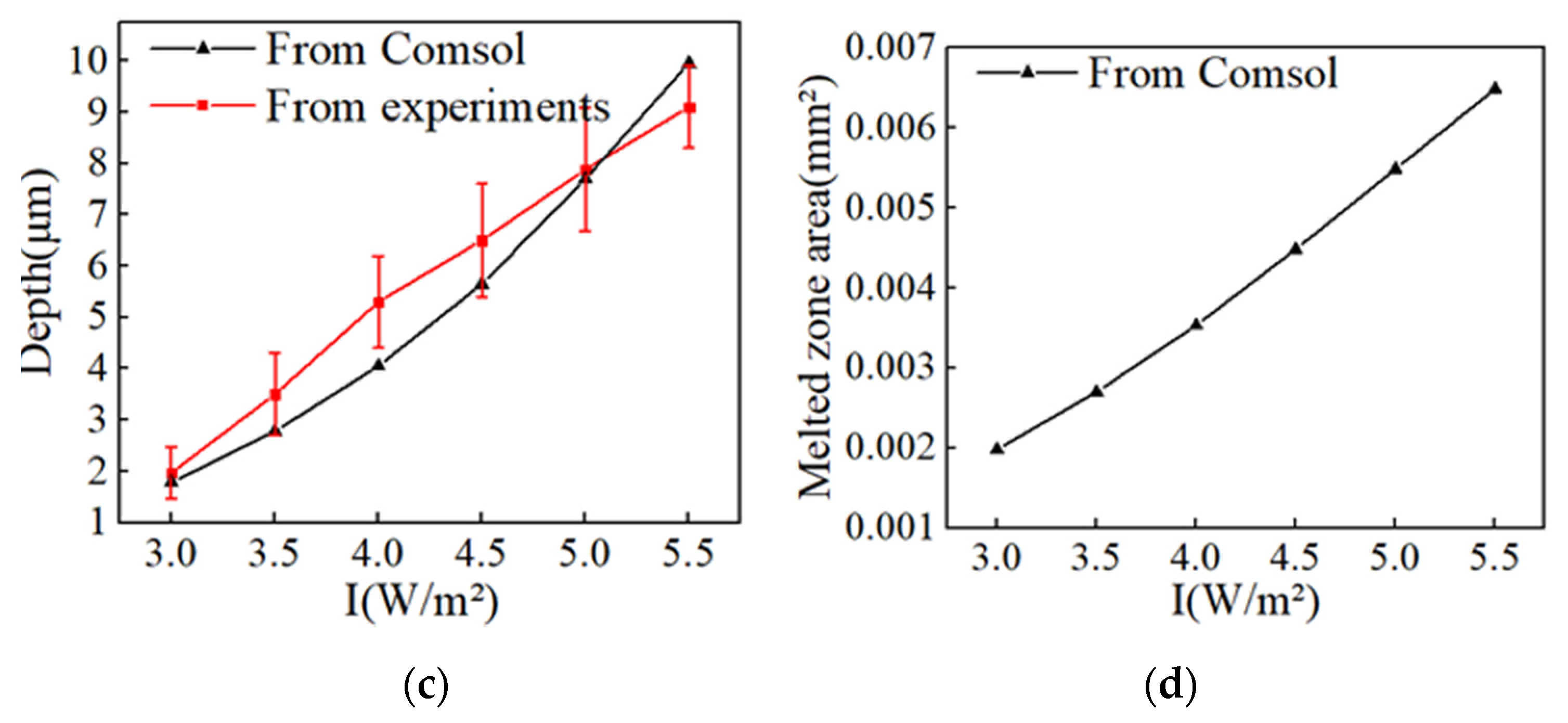
3.2. Effect of Peak Power Density on Morphology
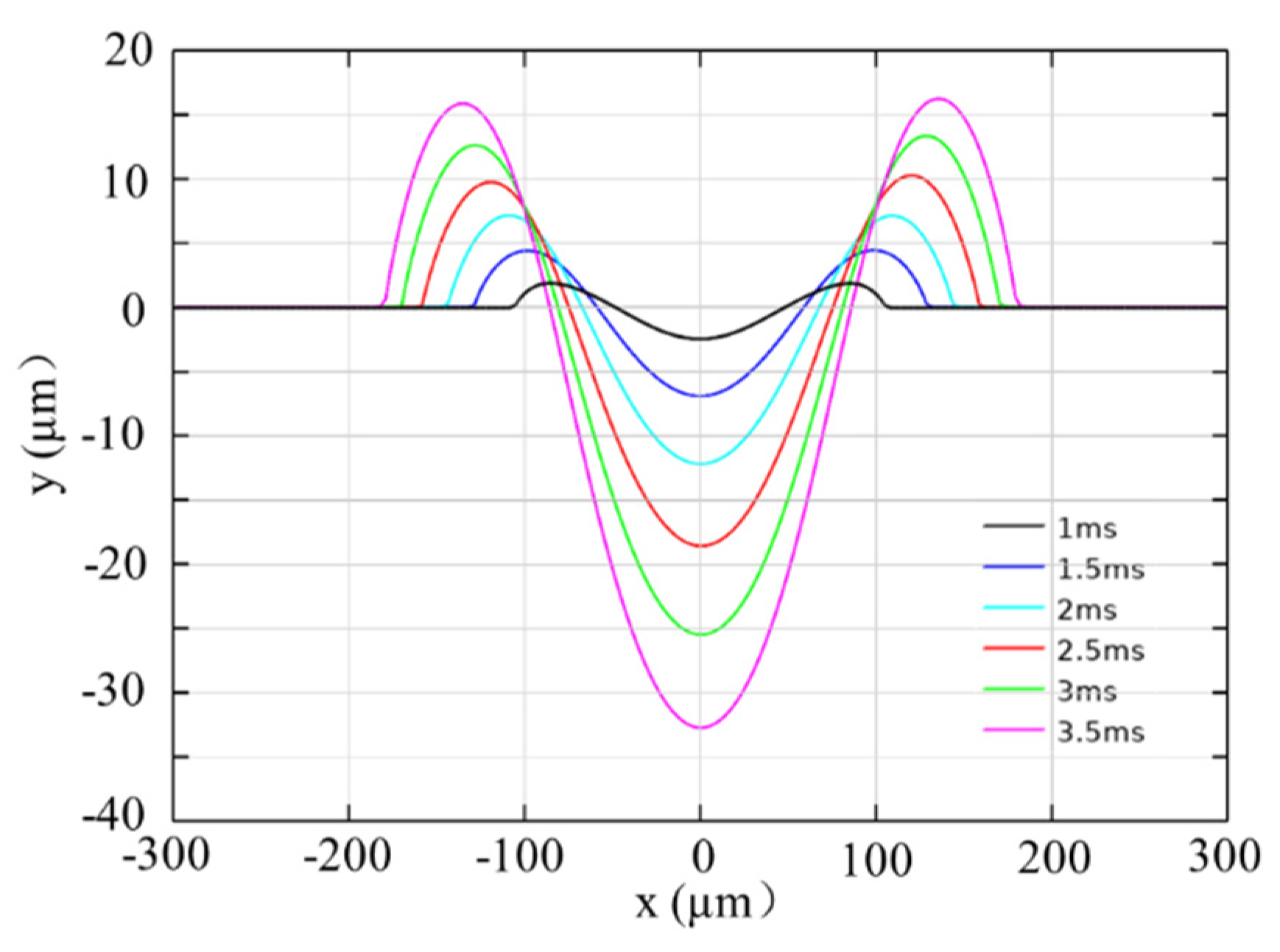
3.3. Effect of Laser Duration on Morphology
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Du, D.; He, Y.F.; Sui, B.; Xiong, L.J.; Zhang, H. Laser texturing of rollers by pulsed Nd: Yag laser. J. Mater. Process. Technol. 2005, 161, 456–461. [Google Scholar] [CrossRef]
- Hua, X.J.; Xie, X.; Yin, B.F.; Zhang, P.Y.; Ji, J.H.; Wang, H.; Fu, Y.H. Tribological performance and selflubricating mechanism of the laser textured surface filled with solid lubricant in rolling friction pair. Ind. Lubr. Tribol. 2018, 70, 371–384. [Google Scholar] [CrossRef]
- Jiang, J.F.; He, Y.H.; Yan, Y.G. Research on laser texturing process and surface quality control. Int. Symp. Laser Precis. Microfabr. 2000, 4088, 240–243. [Google Scholar]
- Fu, H.; Ji, J.H.; Fu, Y.H.; Hua, X.J. Influence of donut-shaped bump on the hydrodynamic lubrication of textured parallel sliders. J. Tribol. 2018, 140. [Google Scholar] [CrossRef]
- He, X.; Fuerschbach, P.W.; Debroy, T. Heat transfer and fluid flow during laser spot welding of 304 stainless steel. J. Phys. D Appl. Phys. 2003, 36, 1388–1398. [Google Scholar] [CrossRef]
- Traidia, A.; Roger, F.; Guyot, E. Optimal parameters for pulsed gas tungsten arc welding in partially and fully penetrated weld pools. Int. J. Therm. Sci. 2010, 49, 1197–1208. [Google Scholar] [CrossRef]
- Ding, X.P.; Wang, L.Z.; Wang, S. Comparison study of numerical analysis for heat transfer and fluid flow under two different laser scan pattern during selective laser melting. Int. J. Light Electron. Optics 2016, 127, 10898–10907. [Google Scholar] [CrossRef]
- Dai, D.H.; Gu, D.D. Influence of thermodynamics within molten pool on migration and distribution state of reinforcement during selective laser melting of Aln/Alsi10 mg composites. Int. J. Mach. Tools Manuf. 2016, 100, 14–24. [Google Scholar] [CrossRef]
- Liu, H.B.; Wan, D.P.; Hu, D.J. Numerical simulation and experimental studies of surface topography of laser textured roller. J. Comput. Theor. Nanosci. 2008, 5, 1570–1573. [Google Scholar] [CrossRef]
- Shen, H.; Pan, Y.Q.; Zhou, J.; Yao, Z.Q. Forming mechanism of bump shape in pulsed laser melting of stainless steel. J. Heat Transf. 2017, 139, 062301. [Google Scholar] [CrossRef]
- Zang, Y.N.; Ni, X.W.; Han, B. The ejection threshold of molten aluminum induced by millisecond pulsed laser. J. Appl. Phys. 2014, 116, 013104. [Google Scholar] [CrossRef]
- Zhou, J.; Shen, H.; Pan, Y.Q.; Ding, X.H. Experimental study on laser microstructures using long pulse. Opt. Lasers Eng. 2016, 78, 113–120. [Google Scholar] [CrossRef]
- Yuan, B.S.; Zhang, Y.; Zhang, W.; Dong, Y.; Jin, G.Y. The effect of spot size combination mode on ablation morphology of aluminum alloy by millisecond-nanosecond combined-pulse laser. Materials 2018, 11, 1419. [Google Scholar] [CrossRef] [PubMed]
- Sharma, S.; Mandal, V.; Ramakrishna, S.A.; Ramkumar, J. Numerical simulation of melt pool oscillations and protuberance in pulsed laser micro melting of Ss304 for surface texturing applications. J. Manuf. Process. 2019, 39, 282–294. [Google Scholar] [CrossRef]
- Shen, H.; Feng, D.; Yao, Z. Modeling of underwater laser drilling of alumina. J. Manuf. Sci. Eng. 2016, 139, 041008. [Google Scholar] [CrossRef]
- Ganesh, R.K.; Bowley, W.W.; Bellantone, R.R.; Hahn, Y. A model for laser hole drilling in metals. J. Comput. Phys. 1996, 125, 161–176. [Google Scholar] [CrossRef]
- Zhang, Y.M.; Shen, Z.H.; Ni, X.W. Modeling and simulation on long pulse laser drilling processing. Int. J. Heat Mass Transf. 2014, 73, 429–437. [Google Scholar] [CrossRef]
- Voller, V.R.; Prakash, C. A fixed grid numerical modelling methodology for convection-diffusion mushy region phase-change problems. Int. J. Heat Mass Transf. 1987, 30, 1709–1719. [Google Scholar] [CrossRef]
- Hirano, K.; Fabbro, R.; Muller, M. Experimental determination of temperature threshold for melt surface deformation during laser interaction on iron at atmospheric pressure. J. Phys. D Appl. Phys. 2011, 44, 435402. [Google Scholar] [CrossRef]
- Sharma, S.; Mandal, V.; Ramakrishna, S.A.; Ramkumar, J. Numerical simulation of melt hydrodynamics induced hole blockage in quasi-CW fiber laser micro-drilling of TiAl6V4. J. Mater. Process. Technol. 2018, 262, 131–148. [Google Scholar] [CrossRef]






| Elements | C | Si | Mn | Cr | Mo | N | Pi | S | Fe |
|---|---|---|---|---|---|---|---|---|---|
| wt% | 1 | 0.2 | 0.34 | 1.5 | 0.01 | 0.08 | 0.013 | 0.003 | Balanced |
| Property | Symbol | Value |
|---|---|---|
| Liquid phase density | 6500 | |
| Solid phase density | 7600 | |
| Liquid phase thermal conductivity | 20 | |
| Solid phase thermal conductivity | 26 | |
| Melting temperature | 1700 K | |
| Vaporizing temperature | 2610 K | |
| Latent heat of fusion | ||
| Latent heat of vaporization | ||
| Specific heat of liquid phase | 660 | |
| Specific heat of solid phase | 840 | |
| Surface tension of pure metal | 1.943 | |
| Constant in surface tension gradient | ||
| Mushy zone constant | ||
| Coefficient of heat transfer | ||
| Radiation emissivity | 0.7 | |
| Retro diffusion coefficient | 0.2 | |
| Temperature transition interval of melting | 50 K | |
| Absorption coefficient | 0.39 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhu, D.; Zhang, P.; Tian, Z.; Chen, C.; Hua, X.; Xu, S.; Xie, X. Numerical Study on the Evolution Mechanism of the Crater under a Millisecond Laser. Appl. Sci. 2020, 10, 9054. https://doi.org/10.3390/app10249054
Zhu D, Zhang P, Tian Z, Chen C, Hua X, Xu S, Xie X. Numerical Study on the Evolution Mechanism of the Crater under a Millisecond Laser. Applied Sciences. 2020; 10(24):9054. https://doi.org/10.3390/app10249054
Chicago/Turabian StyleZhu, Dongpo, Peiyun Zhang, Zhixiang Tian, Cheng Chen, Xijun Hua, Sheng Xu, and Xuan Xie. 2020. "Numerical Study on the Evolution Mechanism of the Crater under a Millisecond Laser" Applied Sciences 10, no. 24: 9054. https://doi.org/10.3390/app10249054
APA StyleZhu, D., Zhang, P., Tian, Z., Chen, C., Hua, X., Xu, S., & Xie, X. (2020). Numerical Study on the Evolution Mechanism of the Crater under a Millisecond Laser. Applied Sciences, 10(24), 9054. https://doi.org/10.3390/app10249054




