Time-Variant Seismic Fragility of Offshore Continuous Beam Bridges Based on Collapse Analysis
Abstract
1. Introduction
2. Durability Damage Analysis of Materials
2.1. Concrete Carbonation
2.2. Corrosion of Reinforcement Bar
3. Fragility Analysis
3.1. Fragility Analysis Method
3.2. System Fragility Analysis Method
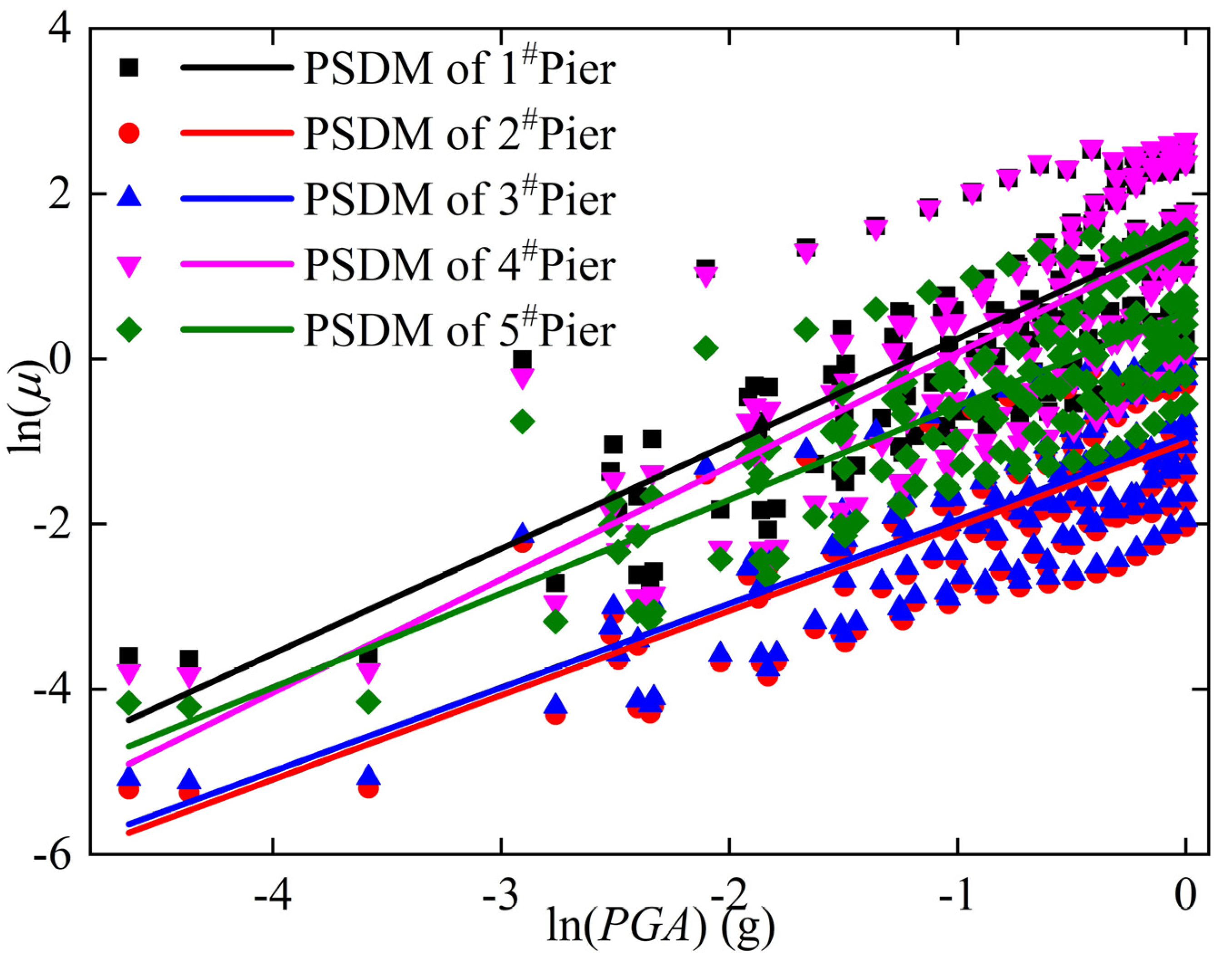
3.3. Fragility Coefficient
4. Case Study
4.1. Bridge Description
4.2. Finite Element Model
4.3. Damage Index
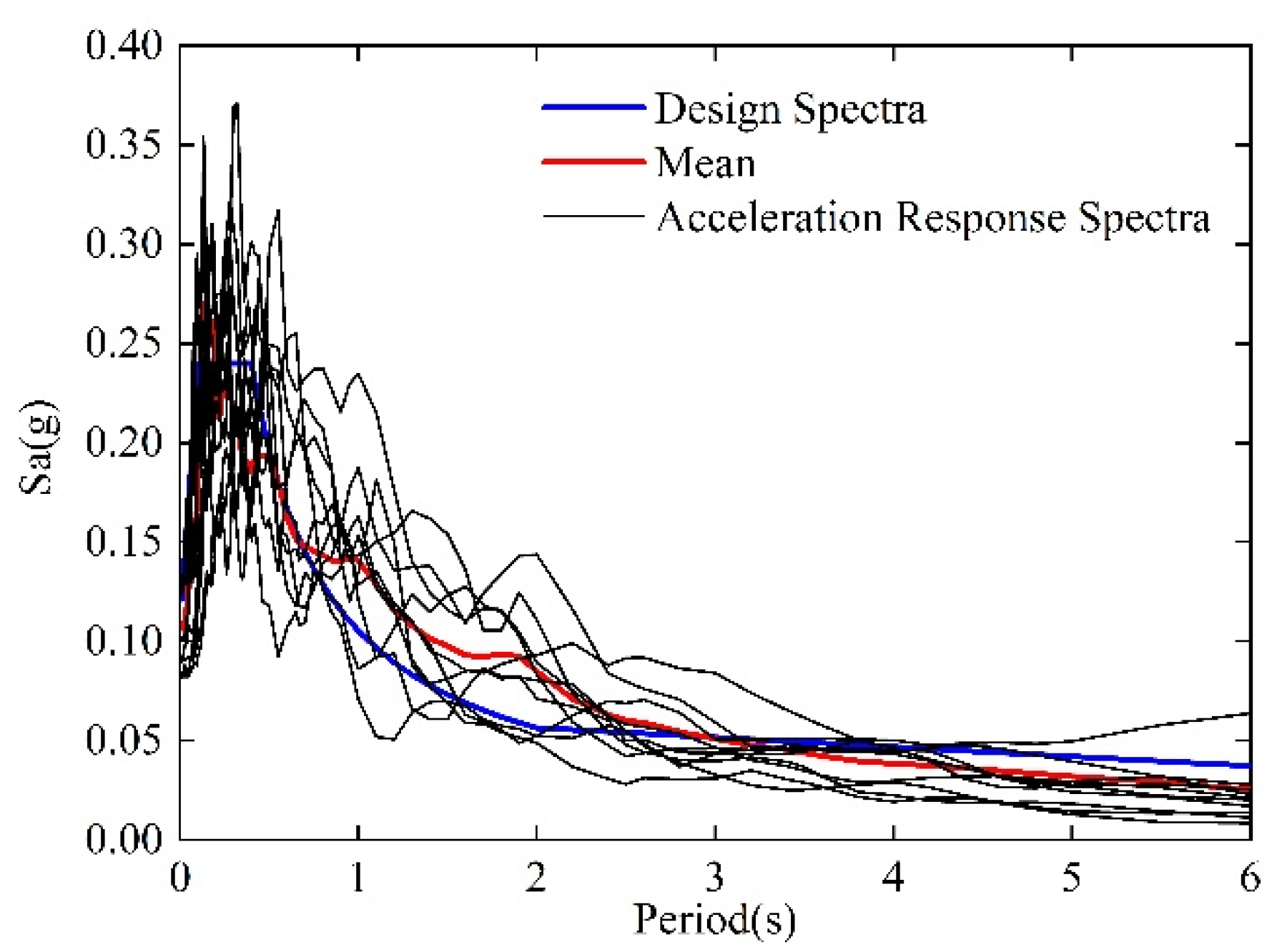
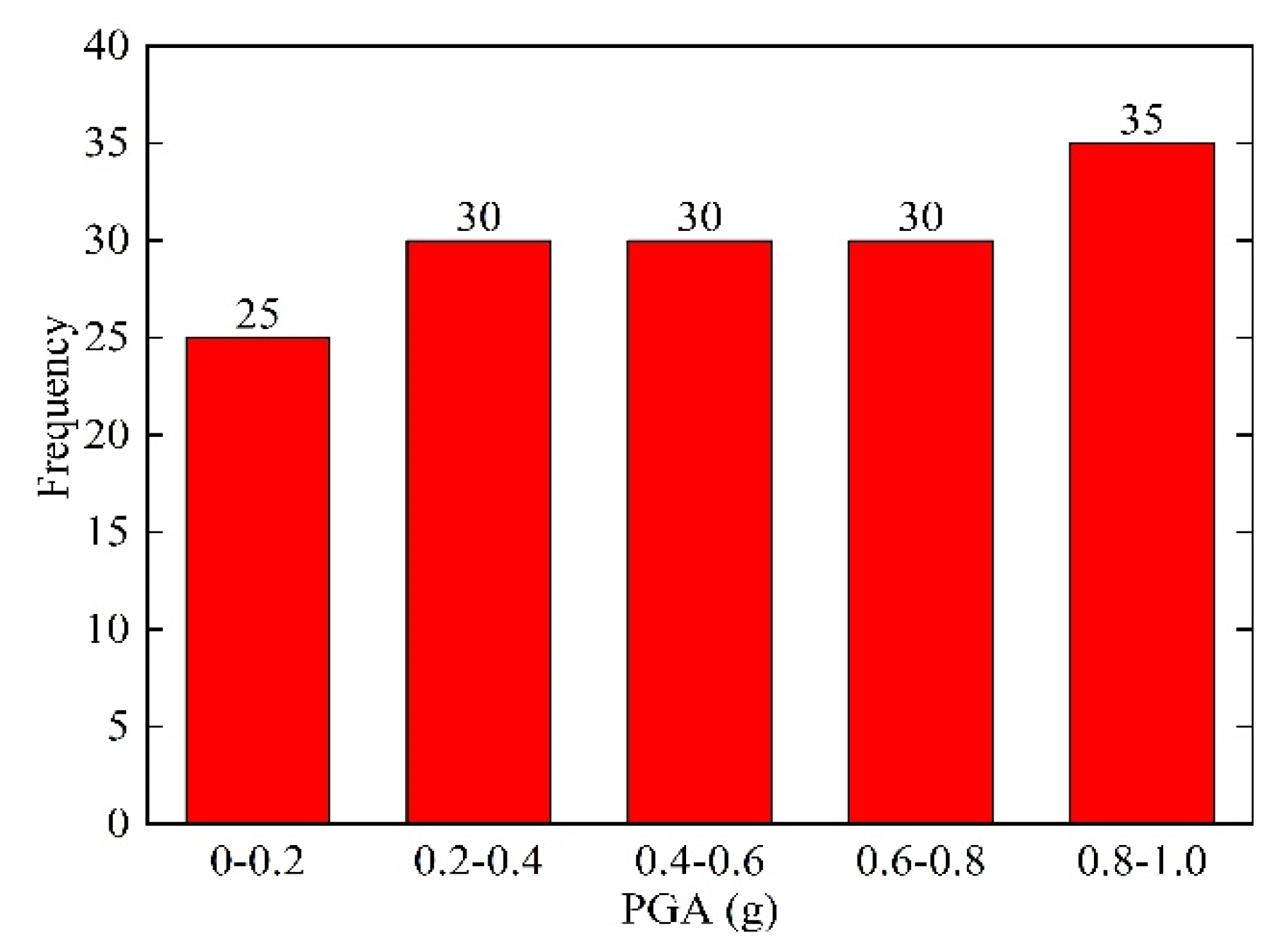
4.4. Selection of Input Ground Motions
5. Time-Variant Fragility of Components and System
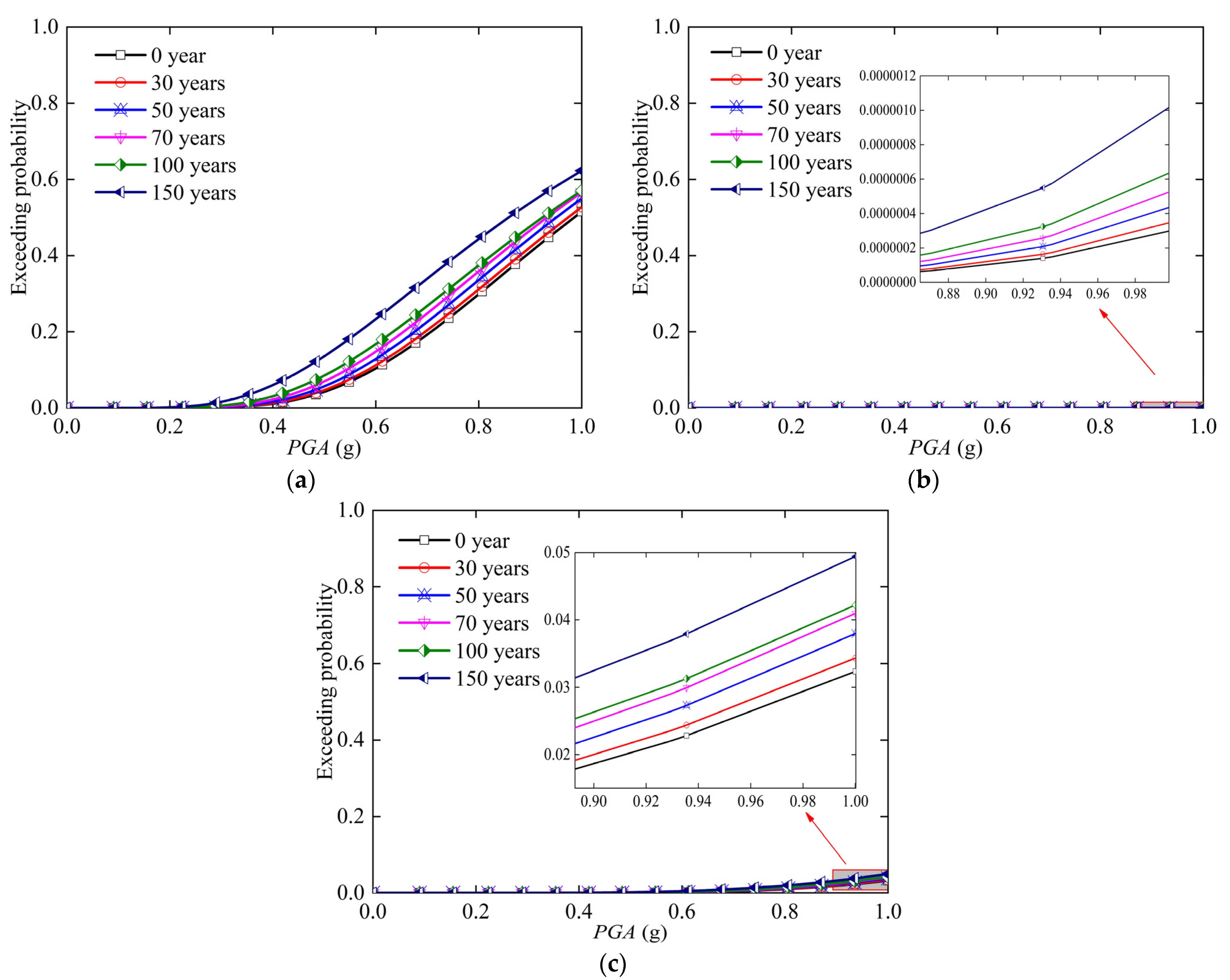
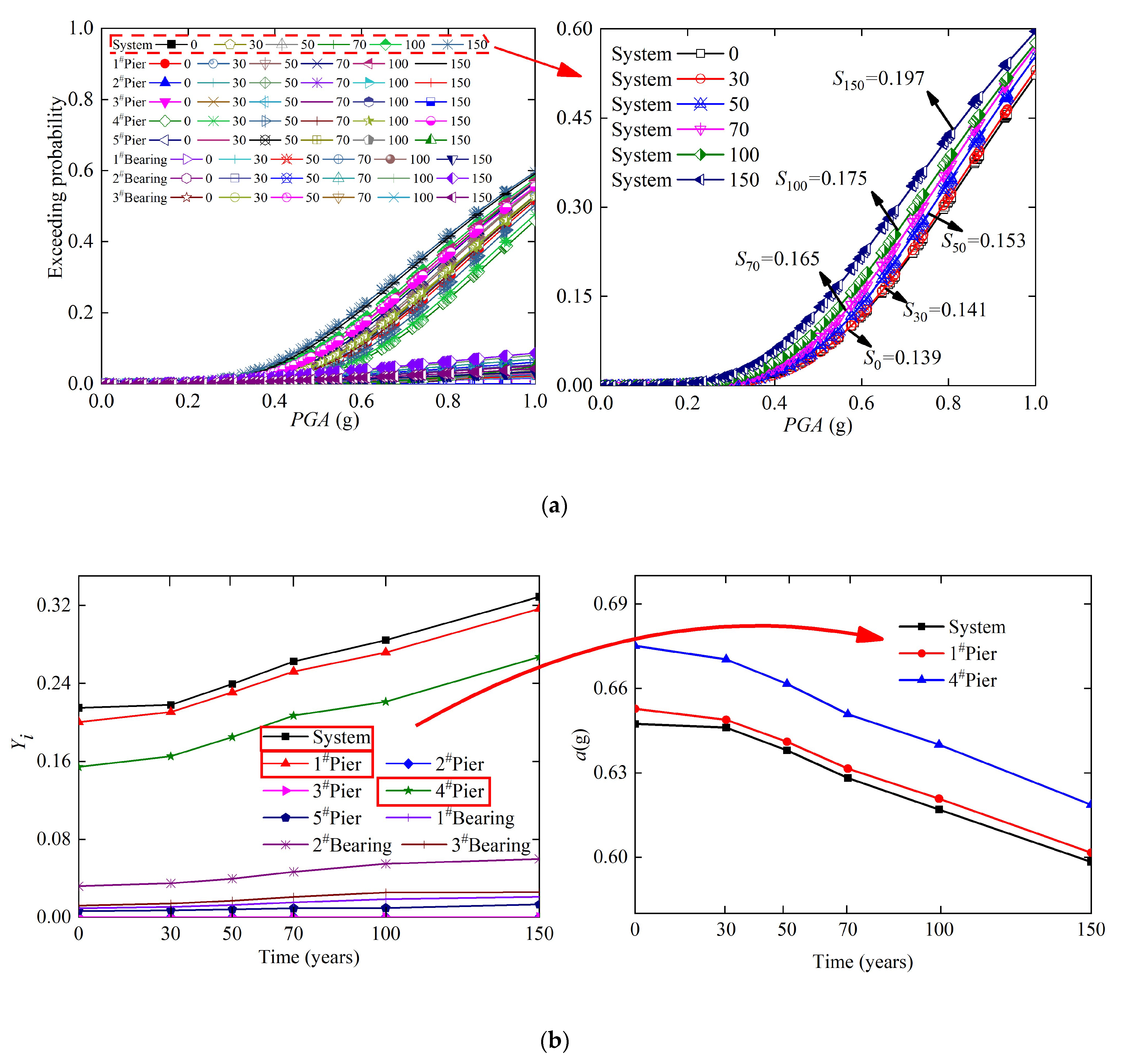
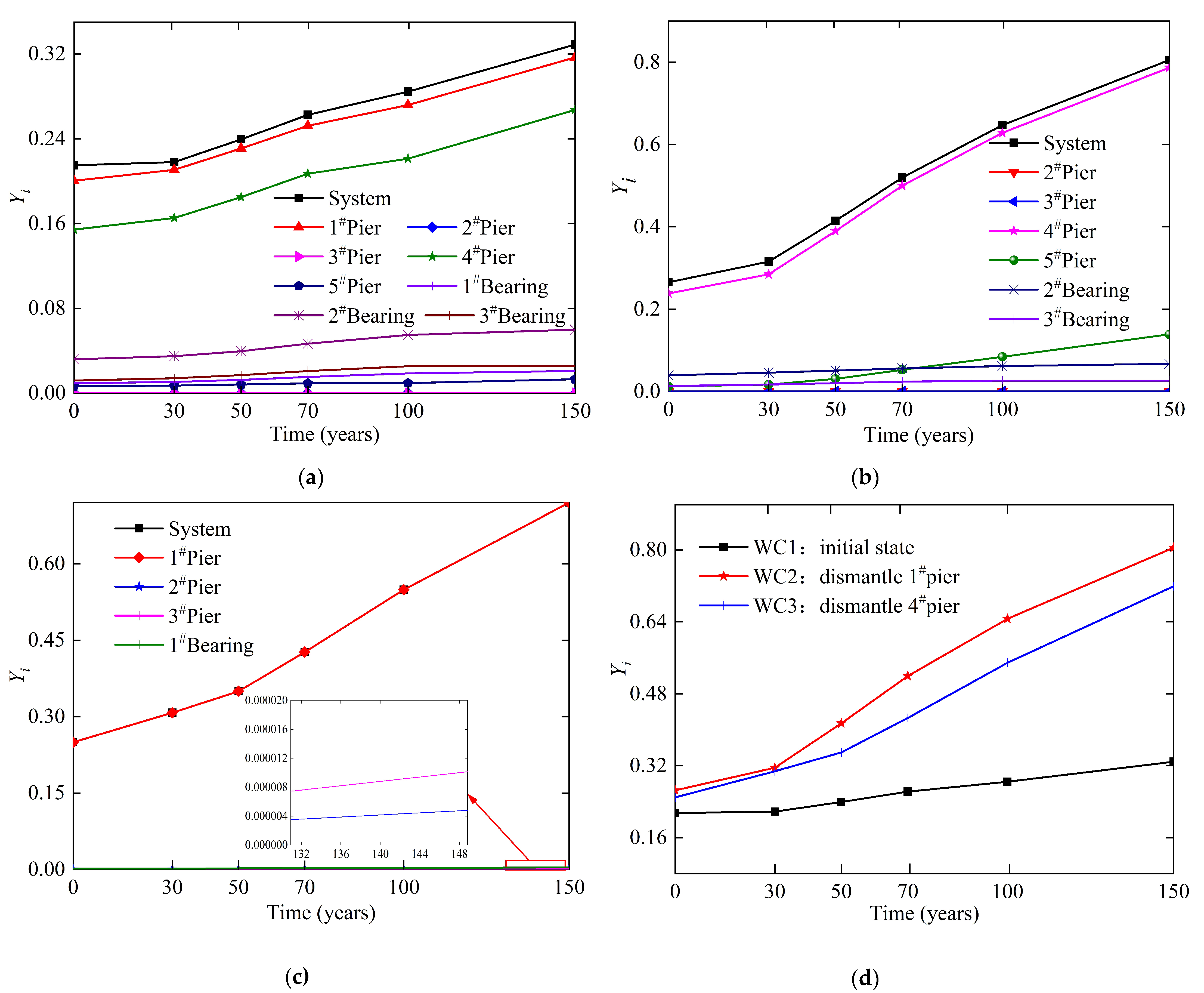
5.1. Time-Variant Fragility Analysis of Pier
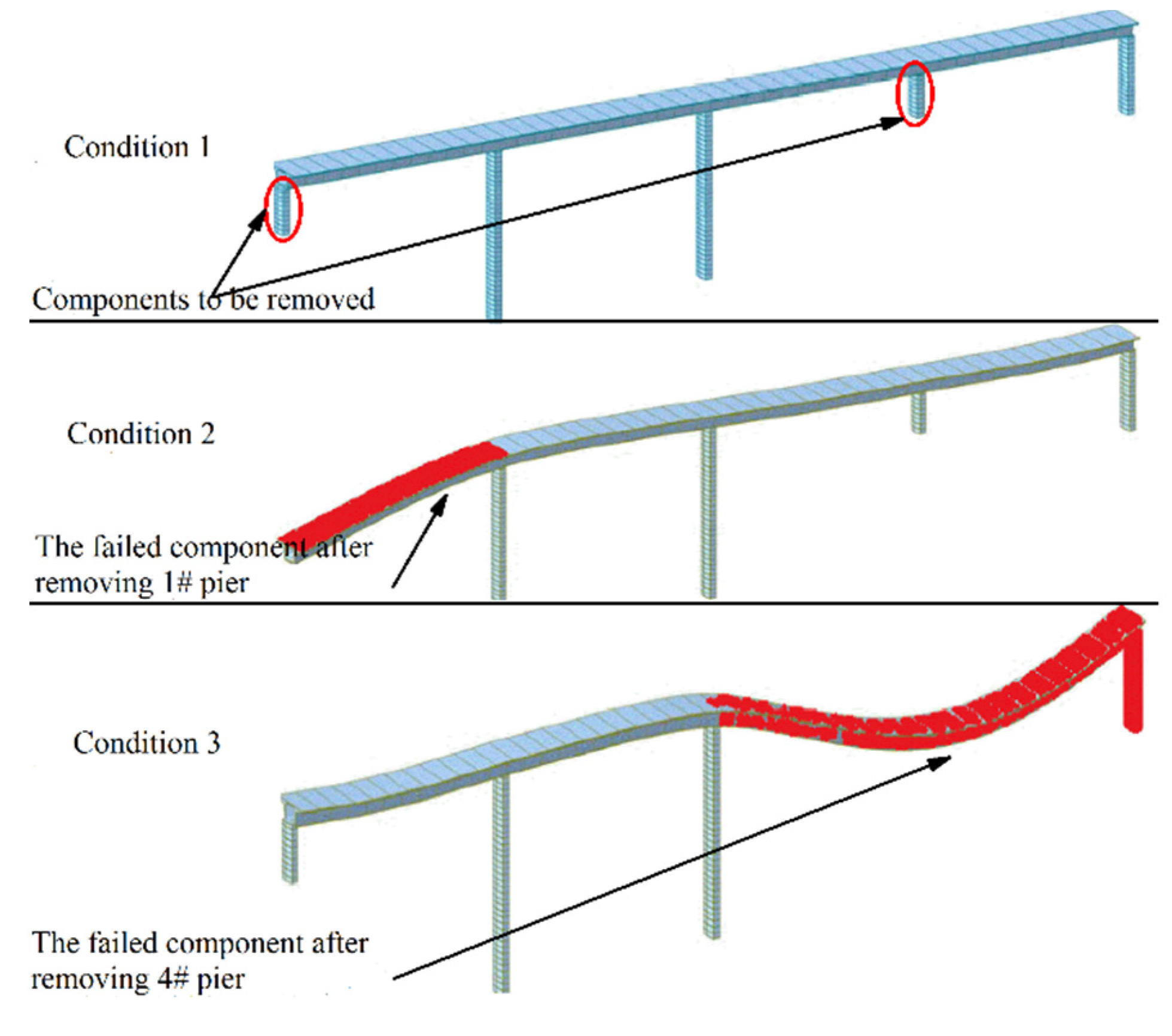
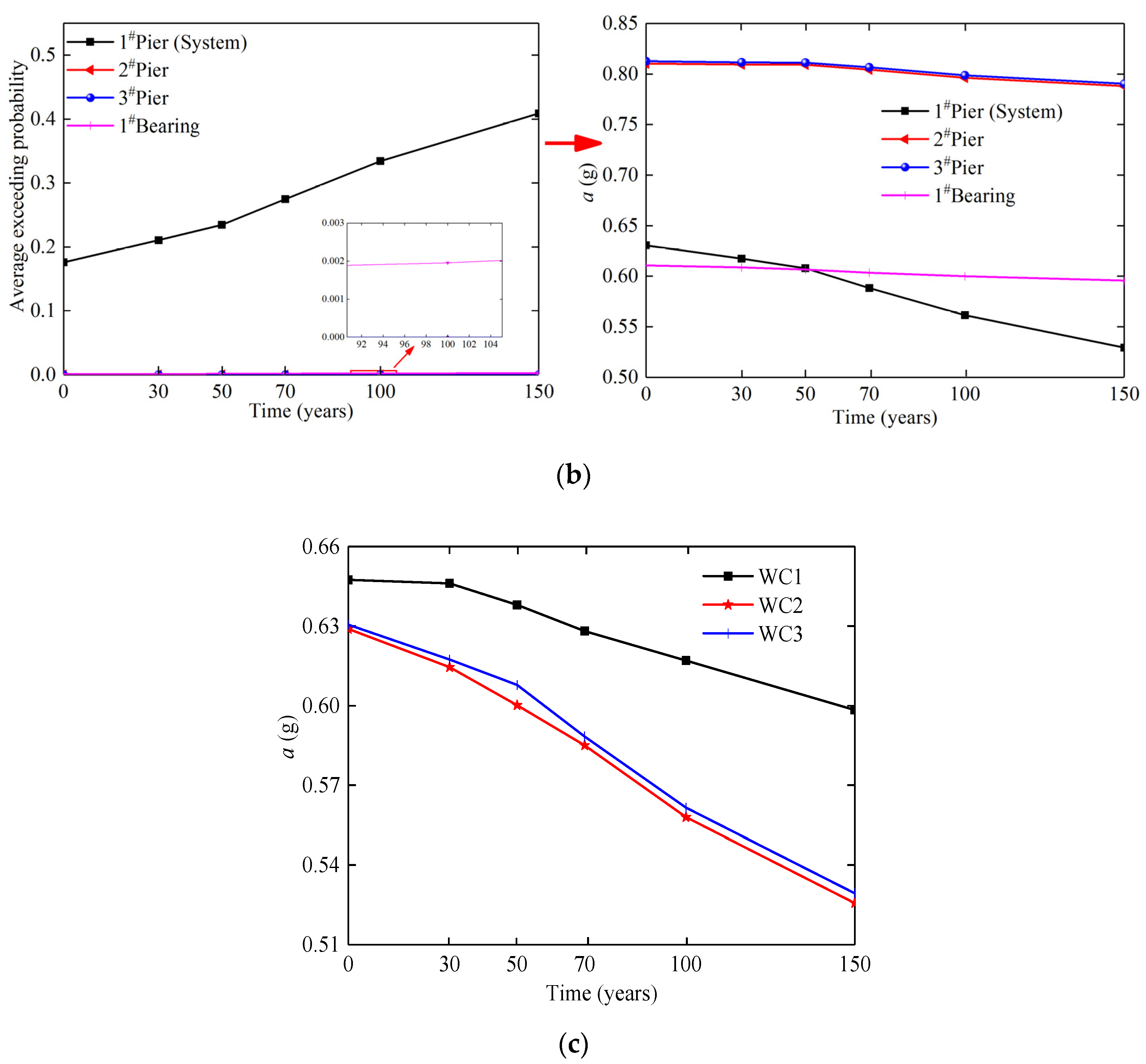
- The working condition of “component dismantle” in the bridge system does not consider the additional load on the remaining new system.
- When a component exceeds the damage index corresponding to its complete damage state, the component is considered to collapse.
5.2. Time-Variant Fragility Analysis of Bearing
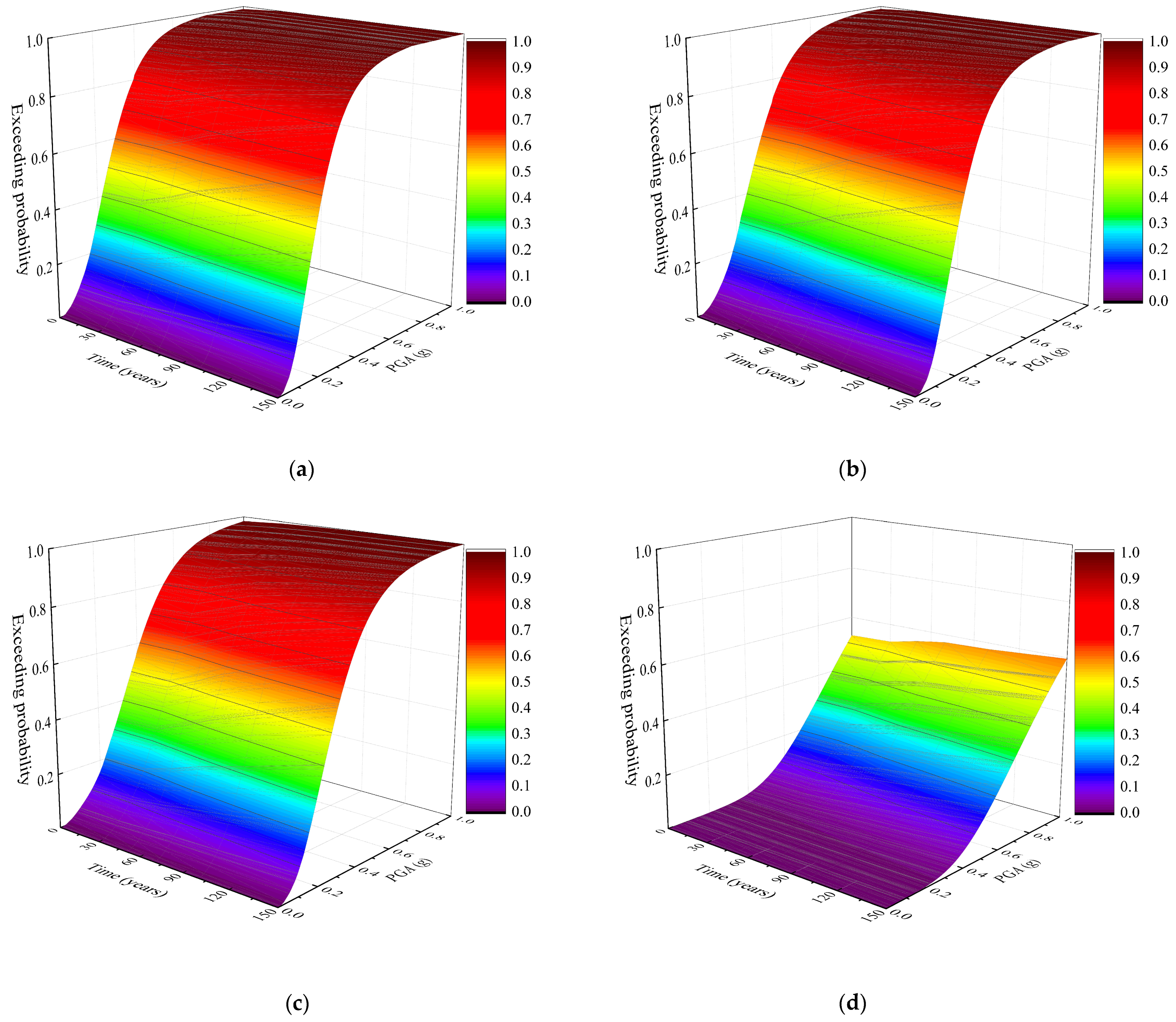
5.3. Time-Variant Fragility Analysis of System
5.4. Time-Variant Fragility Coefficient of the System
6. Conclusions
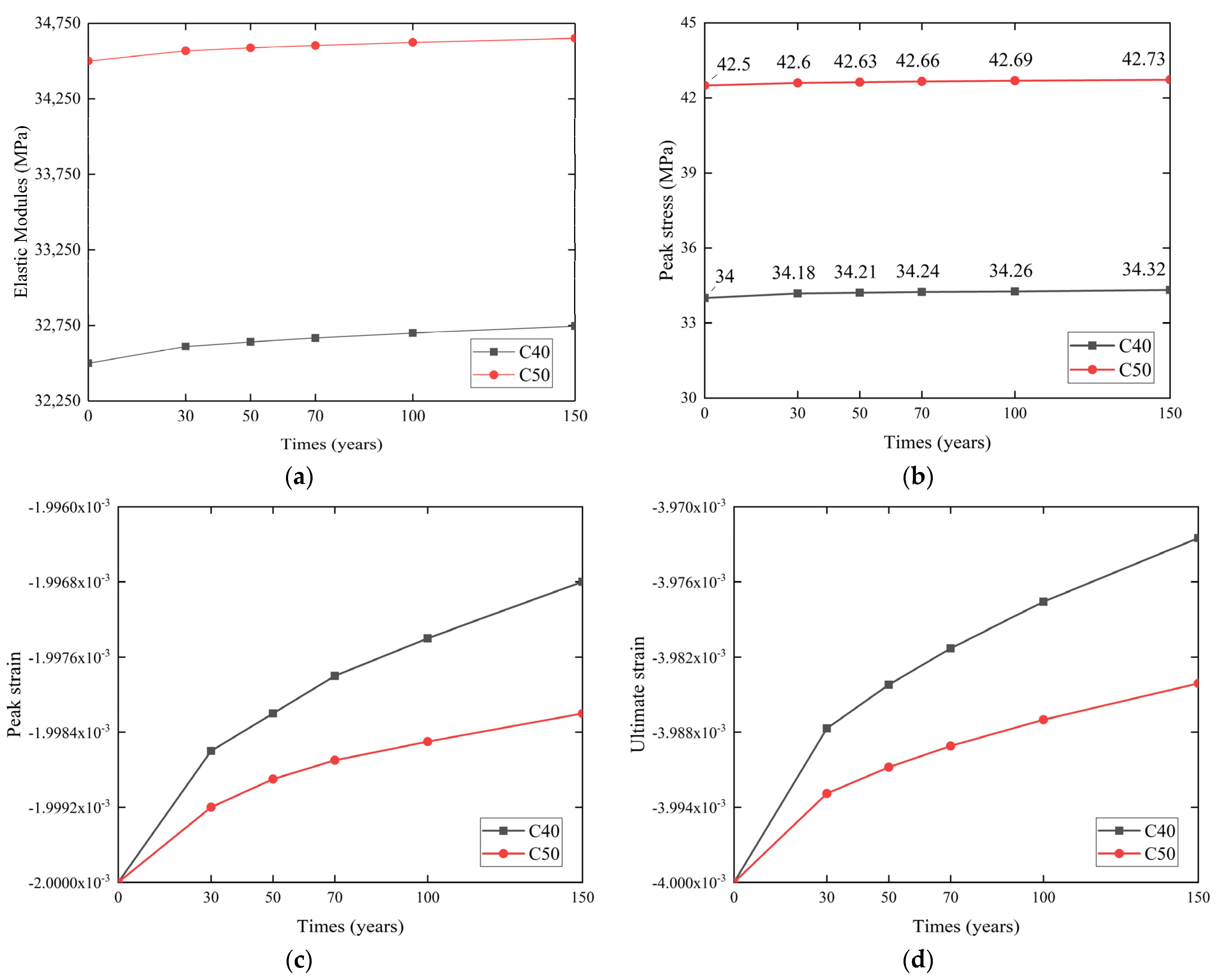
- After considering the time-varying damage of material durability, the peak stress of concrete increases with the extension of service period. On the contrary, the peak strain and limit strain of concrete decrease with the extension of service period. Moreover, the yield strength, ultimate strength, and diameter of the reinforcement decreased with the extension of service period. In addition, the stirrup damage degree was worse than the longitudinal reinforcement. As C50 concrete has better material properties than C40 concrete, the longitudinal reinforcement damage degree in 2# pier and 3# pier is lower than that of 1#, 4# and 5# piers. Taking 1# pier which is in 100 years as an example, the yield strength of longitudinal reinforcement and stirrup decreases by 2.52% and 7%, respectively, after the repair of concrete damage in the protective layer of the pier.
- When the local vibration intensity is small, the 1# pier in working condition 1 is more fragile to damage at the component level. The bridge system in working condition 2 is more fragile at the system level in small local vibration intensity. According to the time-variant fragility curves of components and bridge system, the fragility of each component and bridge system increases with the extension of the service period. Meanwhile, the exceeding probability of any component is lower than the system in same condition. Therefore, the exceeding probability of component cannot be used to replace the exceeding probability of bridge systems when assessing the seismic performance of a bridge.
- The time-varying fragility coefficient of each component and system in three working conditions are compared, among which the fragility coefficient of system are the largest values under every working conditions. When the bridge reaches the design service life, the fragility coefficient of the bridge system is 105% of 1# pier and 132% of the bridge system in 0 year. During the whole service period, both WC2 (dismantle 1# pier) and WC3 (dismantle 4# pier) will increase the fragility coefficient of the system compared with WC1 (original state). However, WC2 is the most fragile working condition in the service period of the bridge.
Author Contributions
Funding
Conflicts of Interest
References
- Val, D.V.; Stewart, M.G. Life-cycle cost analysis of reinforced concrete structures in marine environments. Struct. Saf. 2003, 25, 343–362. [Google Scholar] [CrossRef]
- Darmawan, M.S.; Stewart, M.G. Spatial time-dependent reliability analysis of corroding pretensioned prestressed concrete bridge girders. Struct. Saf. 2007, 29, 16–31. [Google Scholar] [CrossRef]
- Papadakis, V.G.; Vayenas, C.G.; Fardis, M.N. Fundamental modeling and experimental investigation of concrete carbonation. ACI Mater. J. 1991, 88, 363–373. [Google Scholar]
- Saetta, A.V.; Schrefler, B.A.; Vitaliani, R.V. The carbonation of concrete and the mechanism of moisture, heat and carbon dioxide flow through porous materials. Cem. Concr. Res. 1993, 23, 761–772. [Google Scholar] [CrossRef]
- Chang, C.F.; Chen, J.W. The experimental investigation of concrete carbonation depth. Cem. Concr. Res. 2006, 36, 1760–1767. [Google Scholar] [CrossRef]
- Cheng, Y.; Zhang, Y.; Jiao, Y.; Yang, J. Quantitative analysis of concrete property under effects of crack, freeze-thaw and carbonation. Constr. Build. Mater. 2016, 129, 106–115. [Google Scholar] [CrossRef]
- Chen, Y.; Liu, P.; Yu, Z. Effects of Environmental Factors on Concrete Carbonation Depth and Compressive Strength. Materials 2018, 11, 2167. [Google Scholar] [CrossRef]
- He, H.; Li, R.; Chen, K. Durability evolution of RC bridge under coupling action of chloride corrosion and carbonization based on DLA model. Math. Prob. Eng. 2015. [Google Scholar] [CrossRef]
- Lee, M.K.; Jung, S.H.; Oh, B.H. Effects of Carbonation on Chloride Penetration in Concrete. ACI Mater. J. 2013, 110, 559–566. [Google Scholar]
- Guo, A.; Yuan, W.; Lan, C.; Guan, X.; Li, H. Time-dependent seismic demand and fragility of deteriorating bridges for their residual service life. Bull. Earthq. Eng. 2015, 13, 2389–2409. [Google Scholar] [CrossRef]
- Hwang, H.; Liu, J.B.; Chiu, Y.H. Seismic Fragility Analysis of Highway Bridges. China Civil Eng. J. 2001, 37, 47–51. [Google Scholar]
- Choi, E.; DesRoches, R.; Nielson, B. Seismic fragility of typical bridges in moderate seismic zones. Eng. Struct. 2004, 26, 187–199. [Google Scholar] [CrossRef]
- Gardoni, P.; Rosowsky, D. Seismic fragility increment functions for deteriorating reinforced concrete bridges. Struct. Infrastruct. Eng. 2011, 7, 869–879. [Google Scholar] [CrossRef]
- Guo, X.; Wu, Y.; Guo, Y. Time-dependent seismic fragility analysis of bridge systems under scour hazard and earthquake loads. Eng. Struct. 2016, 121, 52–60. [Google Scholar] [CrossRef]
- Cui, F.; Zhang, H.; Ghosn, M.; Xu, Y. Seismic fragility analysis of deteriorating RC bridge substructures subject to marine chloride-induced corrosion. Eng. Struct. 2018, 155, 61–72. [Google Scholar] [CrossRef]
- Choe, D.-E.; Gardoni, P.; Rosowsky, D.; Haukaas, T. Probabilistic capacity models and seismic fragility estimates for RC columns subject to corrosion. Reliab. Eng. Syst. Saf. 2008, 93, 383–393. [Google Scholar] [CrossRef]
- Choe, D.-E.; Gardoni, P.; Rosowsky, D.; Haukaas, T. Seismic fragility estimates for reinforced concrete bridges subject to corrosion. Struct. Saf. 2009, 31, 275–283. [Google Scholar] [CrossRef]
- Padgett, J.E.; Ghosh, J.; Ataei, N. Sensitivity of Dynamic Response of Bridges under Multiple Hazards to Aging Parameters. In Proceedings of the Structures Congress 2010: 19th Analysis and Computation Specialty Conference, Orlando, FL, USA, 12–15 May 2010; pp. 1–12. [Google Scholar] [CrossRef]
- Ghosh, J.; Padgett, J.E. Aging Considerations in the Development of Time-Dependent Seismic Fragility Curves. J. Struct. Eng. 2010, 136, 1497–1511. [Google Scholar] [CrossRef]
- Dong, Y.; Frangopol, D.M.; Saydam, D. Time-variant sustainability assessment of seismically vulnerable bridges subjected to multiple hazards. Earthq. Eng. Struct. Dyn. 2013, 42, 1451–1467. [Google Scholar] [CrossRef]
- Nielson, B.G.; DesRoches, R. Seismic fragility methodology for highway bridges using a component level approach. Earthq. Eng. Struct. Dyn. 2007, 36, 823–839. [Google Scholar] [CrossRef]
- Liang, Y.; Yan, J.; Cheng, Z.; Chen, H.; Mao, R. Seismic Fragility Analysis of Long-Span Bridge System with Durability Degradation. CMES-Comp. Model. Eng. 2019, 121, 177–214. [Google Scholar] [CrossRef]
- Lu, S.N. Research on Seismic Robustness of Concrete Continuous Rigid Frame Bridge with High Pier in Mountain Area; Southwest Jiaotong University, Press: Chengdu, China, 2018; Available online: https://kns.cnki.net/kcms/detail/detail.aspx?dbcode=CMFD&dbname=CMFD201802&filename=1018825353.nh&v=yTkJu6SEctuEG3HmJzBBrLr3bqQIiOXsRtK%25mmd2BcrSFSQxBohL%25mmd2BNXTq4cd2zWfTVIgg (accessed on 30 November 2020).
- CECS. Code for Durability Assessment of Concrete Structures; China Association of Engineering Construction Standardization: Beijing, China, 2007. [Google Scholar]
- Sung, Y.C.; Su, C.K. Time-dependent seismic fragility curves on optimal retrofitting of neutralised reinforced concrete bridges. Struct. Infrastruct. Eng. 2011, 7, 797–805. [Google Scholar] [CrossRef]
- Vidal, T.; Castel, A.; François, R. Analyzing crack width to predict corrosion in reinforced concrete. Cem. Concr. Res. 2004, 34, 165–174. [Google Scholar] [CrossRef]
- Vu, K.A.; Stewart, M.G. Predicting the likelihood and extent of reinforced concrete corrosion-induced cracking. J. Struct. Eng. 2005, 131, 1681–1689. [Google Scholar] [CrossRef]
- Porter, K.; Kennedy, R.; Bachman, R. Creating fragility functions for performance-based earthquake engineering. Earthq. Spectra 2007, 23, 471–489. [Google Scholar] [CrossRef]
- Federal Emergency Management Agency. Earthquake Loss Estimation Methodology: User’s Manual; HAZUS99; Federal Emergency Management Agency: Washington, DC, USA, 1999.
- Shome, N.; Cornell, C.A.; Bazzurro, P.; Carballo, J.E. Earthquakes, records, and nonlinear responses. Earthq. Spectra 1998, 14, 469–500. [Google Scholar] [CrossRef]
- Cornell, C.A.; Jalayer, F.; Hamburger, R.O.; Foutch, D.A. Probabilistic basis for 2000 SAC federal emergency management agency steel moment frame guidelines. J. Struct. Eng. 2002, 128, 526–533. [Google Scholar] [CrossRef]
- Hunter, D. An upper bound for the probability of a union. J. Appl. Probab. 1976, 13, 597–603. [Google Scholar] [CrossRef]
- Ditlevsen, O. Narrow reliability bounds for structural systems. J. Struct. Mech. 1979, 7, 453–472. [Google Scholar] [CrossRef]
- Liang, Y.; Yan, J.L.; Wang, J.F.; Zhang, P.; He, B.J. Analysis on the Time-Varying Fragility of Offshore Concrete Bridge. Complexity 2019, 2019, 2739212. [Google Scholar] [CrossRef]
- Mander, J.B.; Priestley, M.J.; Park, R. Theoretical stress-strain model for confined concrete. J. Struct. Eng. 1988, 114, 1804–1826. [Google Scholar] [CrossRef]
- Zhao, J.; Sritharan, S. Modeling of strain penetration effects in fiber-based analysis of reinforced concrete structures. ACI Struct. J. 2007, 104, 133. [Google Scholar]
- Comité Euro-International Du Béton (CEB). CEB-FIP Model Code for Concrete Structures; Design Code, Press: Thomas Telford, London, 1990; Available online: https://scholar.google.com/scholar?q=Comit%C3%A9%20EuroInternational%20Du%20B%C3%A9ton%20.%20CEBFIP%20model%20code%20for%20concrete%20structures.%20Lausanne,%20Switzerland;%201990 (accessed on 30 November 2020).
- Nielson, B.G. Analytical Fragility Curves for Highway Bridges in Moderate Seismic Zones; Georgia Institute of Technology: Atlanta, GA, USA, 2005. [Google Scholar]
- Ministry of Transport of the People’s Republic of China. Guidelines for Seismic Design of Chinese Highway Bridges; People’s Communications Press: Beijing, China, 2008.

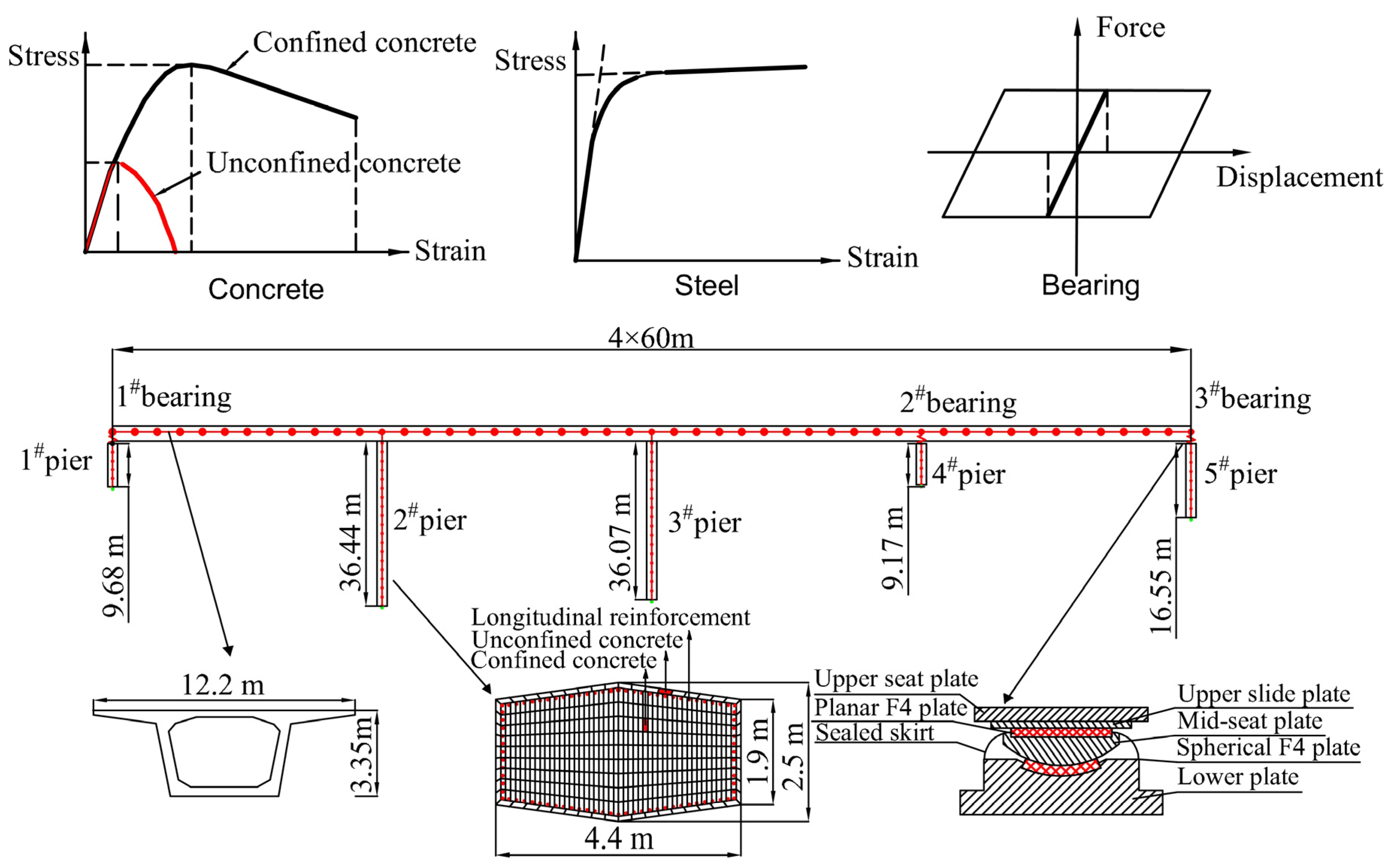



| Steel Types | Xc | w/c | ΔAs0 | ΔAs | x | t |
|---|---|---|---|---|---|---|
| Stirrup | 90 | 0.5 | 1.904π | 32π × −4π × 2 | 0.240 | 1.495 |
| Longitudinal reinforcement | 106 | 0.5 | 2.452π | 64π × −4π × 2 | 0.126 | 0.761 |
| Pier Number | Slight Damage | Moderate Damage | Extensive Damage | Complete Damage |
|---|---|---|---|---|
| 1# pier | ||||
| 2# pier | ||||
| 3# pier | ||||
| 4# pier | ||||
| 5# pier |
| Pier Number | R2 | ||
|---|---|---|---|
| 1# pier | 1.517 | 1.273 | 0.601 |
| 2# pier | −1.010 | 1.021 | 0.602 |
| 3# pier | −0.934 | 1.015 | 0.600 |
| 4# pier | 1.446 | 1.372 | 0.585 |
| 5# pier | 0.568 | 1.136 | 0.612 |
| Working Condition | Component Dismantle | Remaining Service Components of the System |
|---|---|---|
| WC1 | — | 1#, 2#, 3#, 4# and 5# pier;1#, 2# and 3# bearing |
| WC2 | Dismantle 1# pier | 2#, 3#, 4# and 5# pier; 2# and 3# bearing |
| WC3 | Dismantle 4# pier | 1#, 2# and 3# pier; 1# bearing |
| Service Period (Years) | 0 | 30 | 50 | 70 | 100 | 150 |
|---|---|---|---|---|---|---|
| 1# Bearing | 0.02108 | 0.02455 | 0.02931 | 0.03531 | 0.04258 | 0.04299 |
| 3# Bearing | 0.01621 | 0.01828 | 0.02127 | 0.02555 | 0.03076 | 0.03405 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shi, Z.; Liang, Y.; Cao, Y.; Yan, J. Time-Variant Seismic Fragility of Offshore Continuous Beam Bridges Based on Collapse Analysis. Appl. Sci. 2020, 10, 8595. https://doi.org/10.3390/app10238595
Shi Z, Liang Y, Cao Y, Yan J. Time-Variant Seismic Fragility of Offshore Continuous Beam Bridges Based on Collapse Analysis. Applied Sciences. 2020; 10(23):8595. https://doi.org/10.3390/app10238595
Chicago/Turabian StyleShi, Zhaodong, Yan Liang, Yang Cao, and Jialei Yan. 2020. "Time-Variant Seismic Fragility of Offshore Continuous Beam Bridges Based on Collapse Analysis" Applied Sciences 10, no. 23: 8595. https://doi.org/10.3390/app10238595
APA StyleShi, Z., Liang, Y., Cao, Y., & Yan, J. (2020). Time-Variant Seismic Fragility of Offshore Continuous Beam Bridges Based on Collapse Analysis. Applied Sciences, 10(23), 8595. https://doi.org/10.3390/app10238595





