Experimental Analysis of the Dynamic Stiffness in Industrial Robots
Abstract
1. Introduction
- (i)
- The indirect approach adopts the FRFs based on the experimental modal analysis.
- (ii)
- The direct approach measures the vibration displacement and the corresponding excitation force through a force sensor and high-speed camera system.
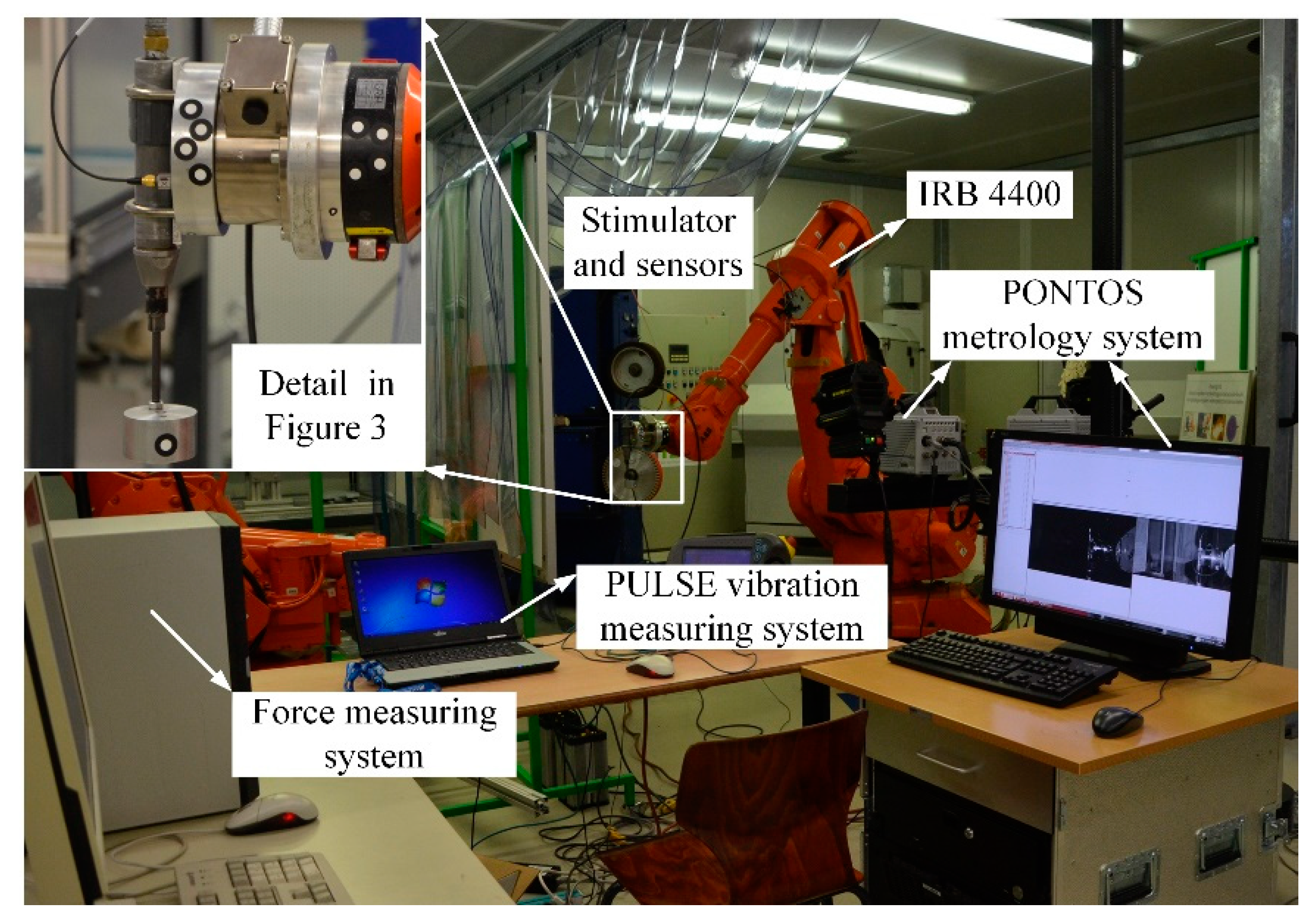
2. Measurement System
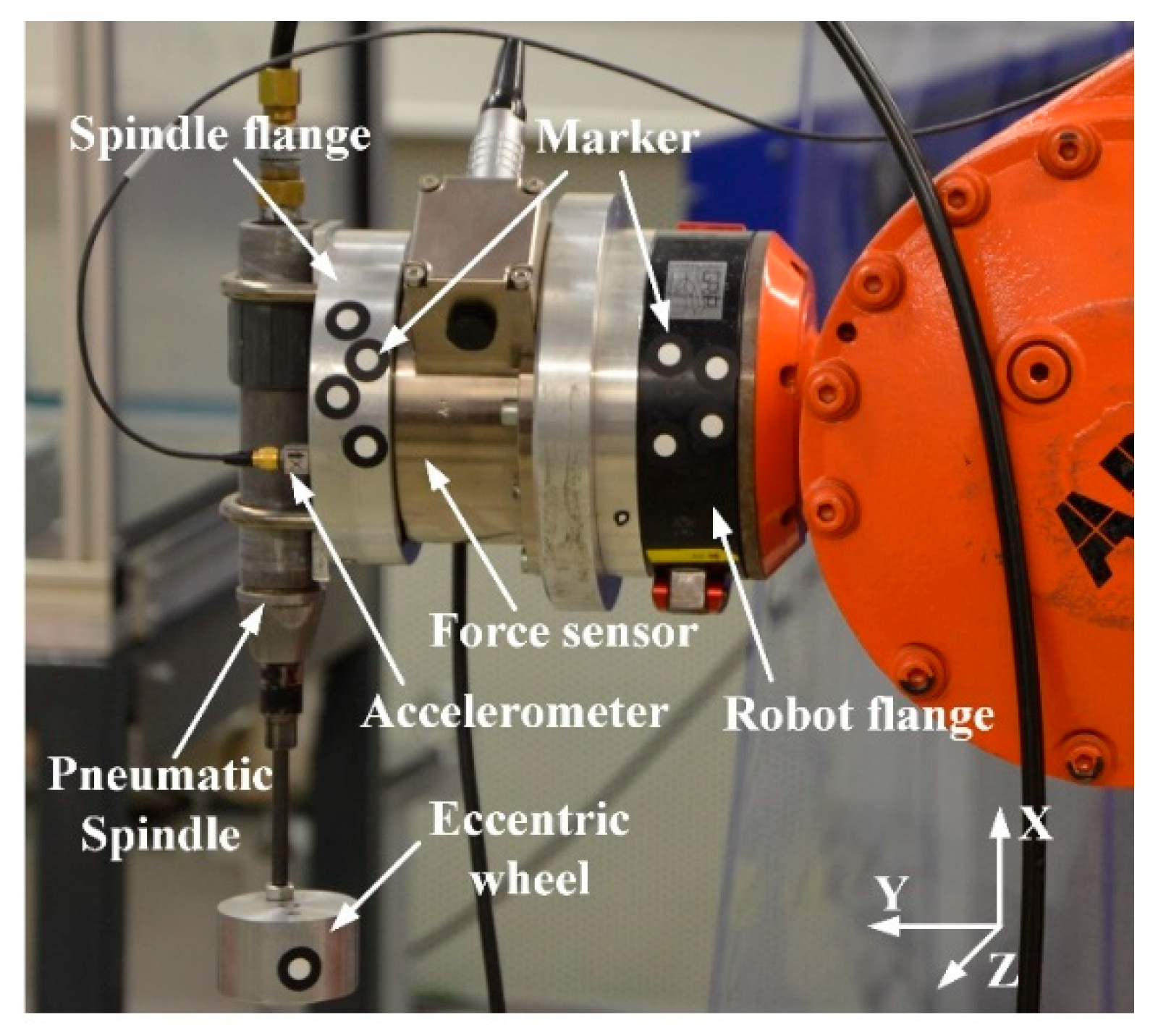
2.1. Configuration of the Experimental Modal Test
2.2. Configuration of the Experimental Modal Test
3. Experimental Procedure and Data Analysis
3.1. Dynamic Stiffness Calculation with Modal Test Data
3.2. Dynamic Stiffness Calculation with Forced Vibration Data
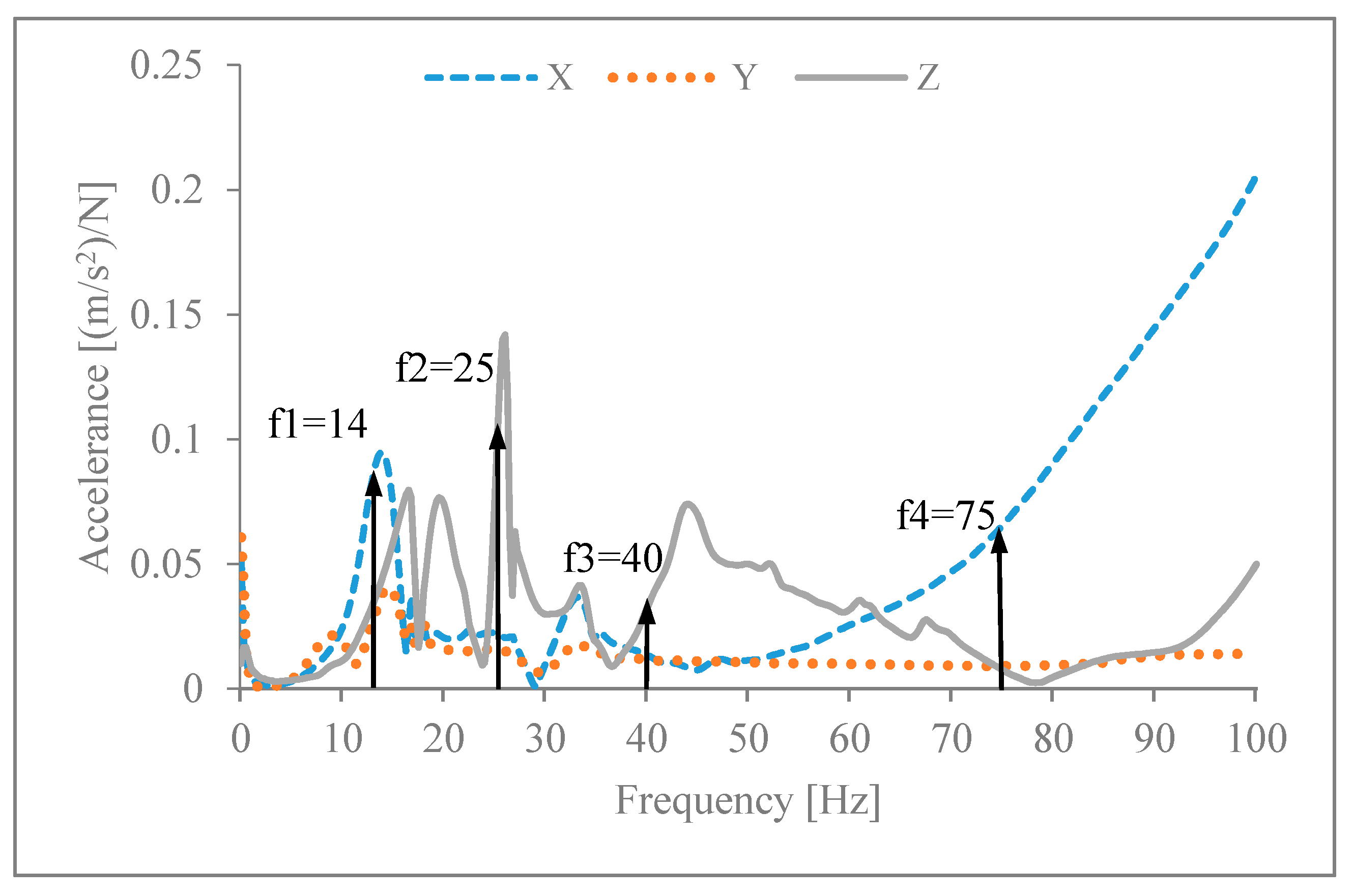
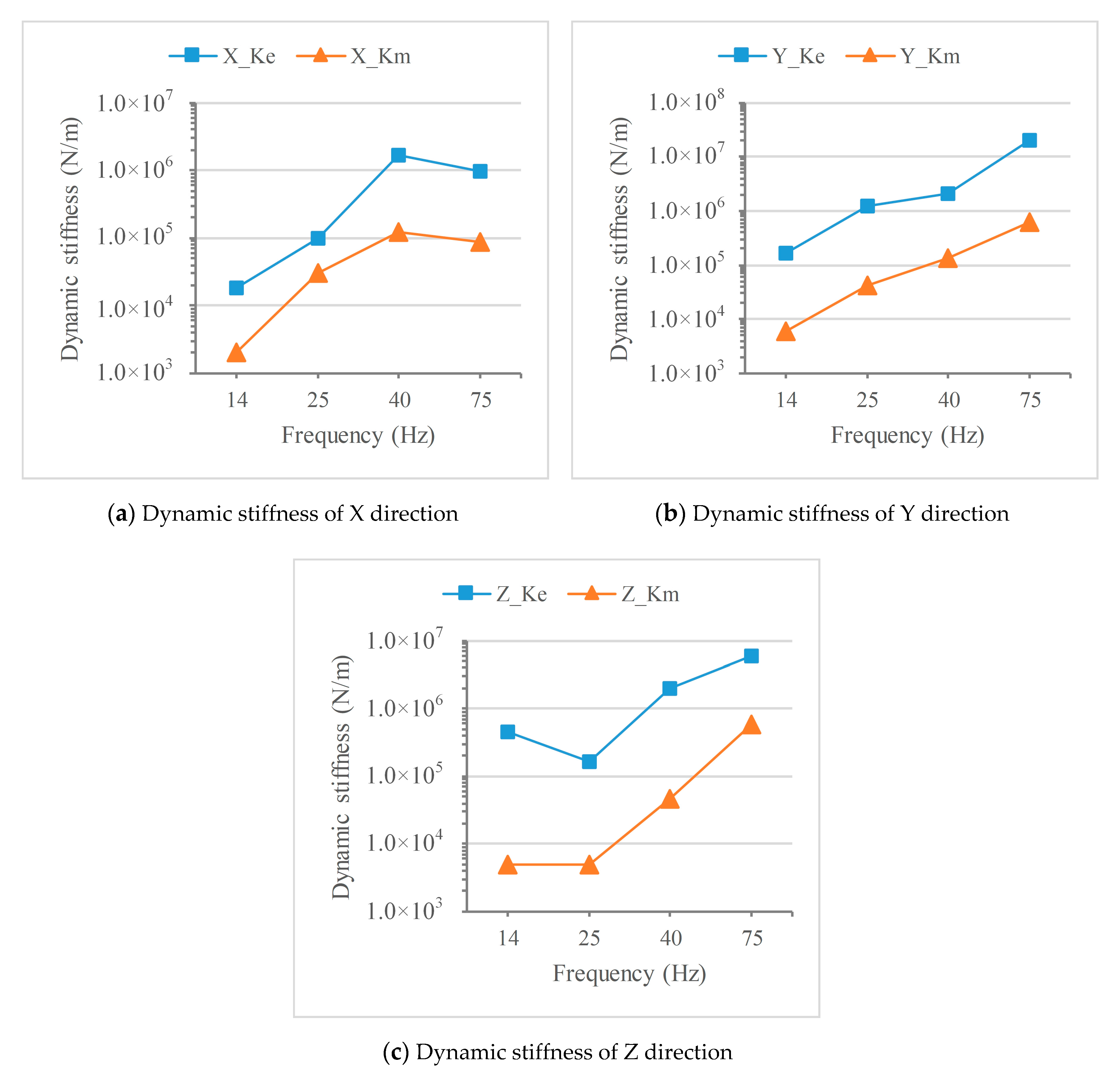
3.3. Dynamic Stiffness Analysis
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Celikag, H.; Sims, N.D.; Ozturk, E. Cartesian Stiffness Optimization for Serial Arm Robots. Procedia CIRP 2018, 77, 566–569. [Google Scholar] [CrossRef]
- Abele, E.; Weigold, M.; Rothenbücher, S. Modeling and Identification of an Industrial Robot for Machining Applications. CIRP Ann. Manuf. Technol. 2007, 56, 387–390. [Google Scholar] [CrossRef]
- Pan, Z.; Zhang, H. Improving robotic machining accuracy by real-time compensation. In Proceedings of the 2009 ICCAS-SICE, Fukuoka, Japan, 18–21 August 2009; pp. 4289–4294. [Google Scholar]
- Schneider, U.; Momeni-K, M.; Ansaloni, M.; Verl, A. Stiffness modeling of industrial robots for deformation compensation in machining. In Proceedings of the 2014 IEEE/RSJ International Conference on Intelligent Robots and Systems, Chicago, IL, USA, 14–18 September 2014; pp. 4464–4469. [Google Scholar]
- Abele, E.; Bauer, J.; Rothenbücher, S.; Stelzer, M.; Stryk, O. Von Prediction of the Tool Displacement by Coupled Models of the Compliant Industrial Robot and the Milling Process. In Proceedings of the International Conference on Process Machine Interactions, Hannover, Germany, 3–4 September 2008; pp. 223–230. [Google Scholar]
- Claudiu, B.; Mehdi, C.; Alain, G.; Jean-Yves, K. Dynamic behavior analysis for a six axis industrial machining robot. Adv. Mater. Res. 2012, 423, 65–76. [Google Scholar]
- K’Nevez, J.-Y.; Cherif, M.; Zapciu, M.; Gérard, A. Experimental Characterization of Robot Arm Rigidity in Order to Be Used in Machining Operation. In Proceedings of the 19th International Conference on Manufacturing System-ICMaS, Bucarest, Romania, 11–12 November 2010; Volume 5, pp. 153–156. [Google Scholar]
- Huckaby, J.; Christensen, H.I. Dynamic Characterization of KUKA Light-Weight Robot Manipulators; Georgia Institute of Technology: Atlanta, GA, USA, 2012. [Google Scholar]
- Abele, E.; Fiedler, U. Creating Stability Lobe Diagrams during Milling. CIRP Ann. 2004, 53, 309–312. [Google Scholar] [CrossRef]
- Matsubara, A.; Tsujimoto, S.; Kono, D. Evaluation of dynamic stiffness of machine tool spindle by non-contact excitation tests. CIRP Ann. Manuf. Technol. 2015, 64, 365–368. [Google Scholar] [CrossRef]
- Cvitanic, T.; Nguyen, V.; Melkote, S.N.; Program, P.D.; Nw, N.A.; States, U. Pose optimization in robotic machining using static and dynamic stiffness models. Robot. Comput. Integr. Manuf. 2020, 66, 101992. [Google Scholar] [CrossRef]
- Kim, S.-G.; Jang, S.-H.; Hwang, H.-Y.; Choi, Y.-H.; Ha, J.-S. Analysis of Dynamic Characteristics and Evaluation of Dynamic Stiffness of a 5-Axis Multi-tasking Machine Tool by using F.E.M and Exciter Test. In Proceedings of the 2008 International Conference on Smart Manufacturing Application, Gyeonggi-do, Korea, 9–11 April 2008; pp. 565–569. [Google Scholar]
- Karim, A.; Hitzer, J.; Lechler, A.; Verl, A. Analysis of the dynamic behavior of a six-axis industrial robot within the entire workspace in respect of machining tasks. In Proceedings of the 2017 IEEE International Conference on Advanced Intelligent Mechatronics (AIM), Munich, Germany, 3–7 July 2017; pp. 670–675. [Google Scholar] [CrossRef]
- Nguyen, V.; Cvitanic, T.; Melkote, S. Data-Driven Modeling of the Modal Properties of a Six-Degrees-of-Freedom Industrial Robot and Its Application to Robotic Milling. J. Manuf. Sci. Eng. 2019, 141, 1–12. [Google Scholar] [CrossRef]
- Bauchau, O.A. Flexible Multibody Dynamics; Solid Mechanics and Its Applications; Springer: Dordrecht, The Netherlands, 2011; Volume 176, ISBN 978-94-007-0334-6. [Google Scholar]
- Zimmermann, S.A.; Berninger, T.F.C.; Derkx, J.; Rixen, D.J. Dynamic modeling of robotic manipulators for accuracy evaluation. In Proceedings of the 2020 IEEE International Conference on Robotics and Automation (ICRA), Paris, France, 31 May–31 August 2020; pp. 8144–8150. [Google Scholar]
- Abele, E.; Bauer, J.; Hemker, T.; Laurischkat, R.; Meier, H.; Reese, S.; von Stryk, O. Comparison and validation of implementations of a flexible joint multibody dynamics system model for an industrial robot. CIRP J. Manuf. Sci. Technol. 2011, 4, 38–43. [Google Scholar] [CrossRef]
- Bruel & Kjaer. Type 3050:Multi-Purpose 4- and 6-Channel Input Modules. Available online: https://www.bksv.com/en/products/daq-data-acquisition/lan-xi-data-acquisition-system/daq-modules/type-3050 (accessed on 12 November 2020).
- Bruel & Kjaer. System Data:Software for PULSE LabShop. Available online: https://www.bksv.com/-/media/literature/Product-Data/bu0229.ashx (accessed on 12 November 2020).
- Bruel & Kjaer. Cubic Triaxial CCLD Accelerometer Types 4524, 4524-B and 4524-B-001. Available online: https://www.bksv.com/media/doc/Bp2076.pdf (accessed on 12 November 2020).
- Brüel&Kjaer. Heavy-Duty Impact Hammers: Types 8207, 8208 and 8210. Available online: https://www.bksv.com/-/media/literature/Product-Data/bp2079.ashx (accessed on 12 November 2020).
- Wu, K.; Brueninghaus, J.; Johnen, B.; Kuhlenkoetter, B. Applicability of stereo high speed camera systems for robot dynamics analysis. In Proceedings of the 2015 International Conference on Control, Automation and Robotics, Singapore, 20–22 May 2015; pp. 44–48. [Google Scholar]
- ATI Industrial Automation. F/T Sensor: Data Acquisition (DAQ) Systems. Available online: https://www.ati-ia.com/app_content/documents/9620-05-DAQ.pdf (accessed on 12 November 2020).
- ATI Indusrial Automation. F/T System Interfaces. Available online: https://www.ati-ia.com/products/ft/ft_SystemInterfaces.aspx (accessed on 12 November 2020).
- Bilošová, A. Modal Testing. 2011. Available online: https://moodle2.units.it/pluginfile.php/75129/mod_resource/content/1/EMA.pdf (accessed on 8 November 2020).
- Brandt, A.; Wiley, J. Noise and Vibration Analysis: Signal Analysis and Experimental Procedures, 1st ed.; John Wiley & Sons, Ltd.: Hoboken, NJ, USA, 2010; ISBN 978-0-470-74644-8. [Google Scholar]
- Brüel&Kjaer. Simplified Measurement of Complex Modulus. Available online: https://www.bksv.com/media/doc/BO0218.pdf (accessed on 8 November 2020).
- Bu, Y.; Liao, W.; Tian, W.; Zhang, J.; Zhang, L. Stiffness analysis and optimization in robotic drilling application. Precis. Eng. 2017, 49, 388–400. [Google Scholar] [CrossRef]
- Alici, G.; Shirinzadeh, B. Enhanced stiffness modeling, identification and characterization for robot manipulators. IEEE Trans. Robot. 2005, 21, 554–564. [Google Scholar] [CrossRef]
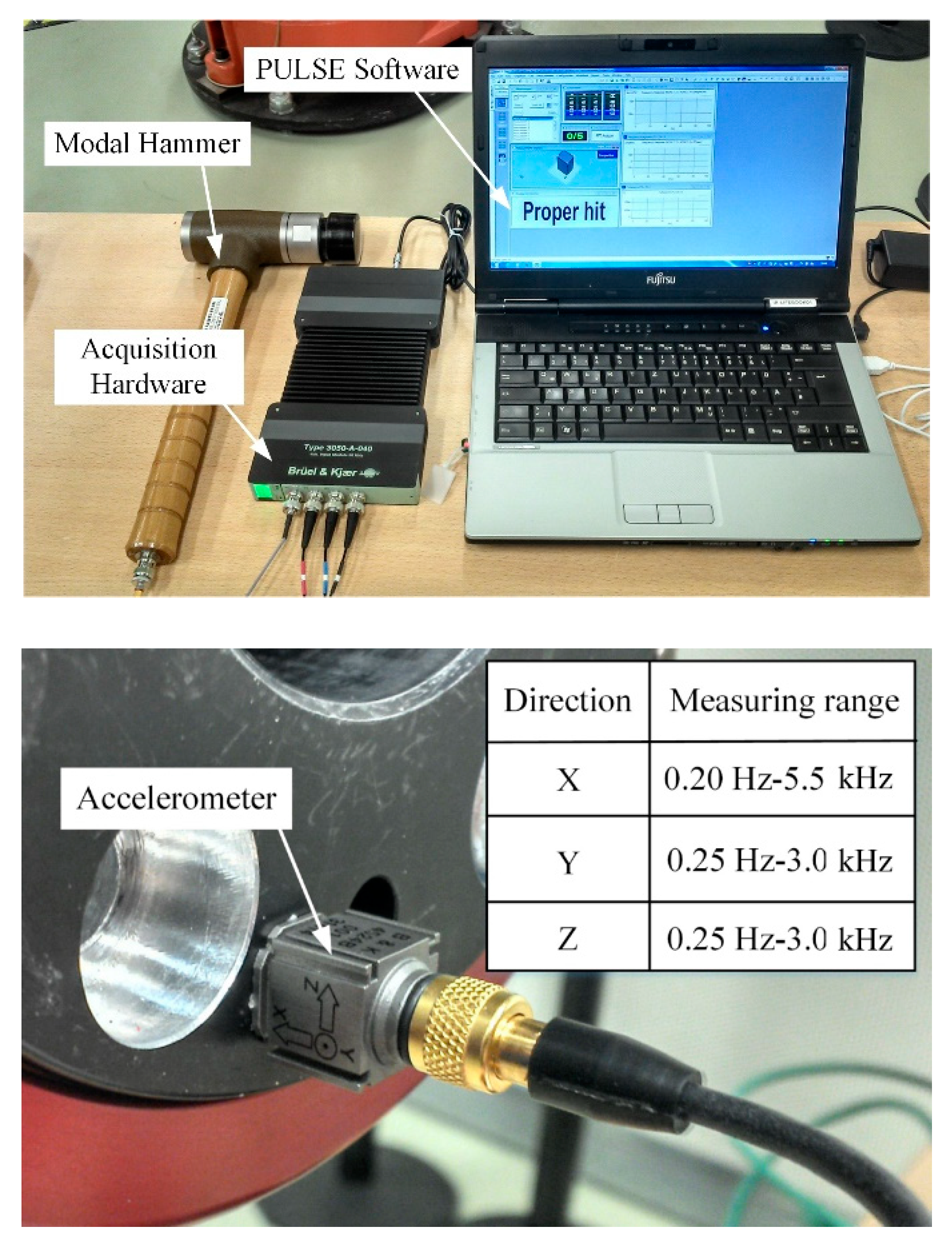

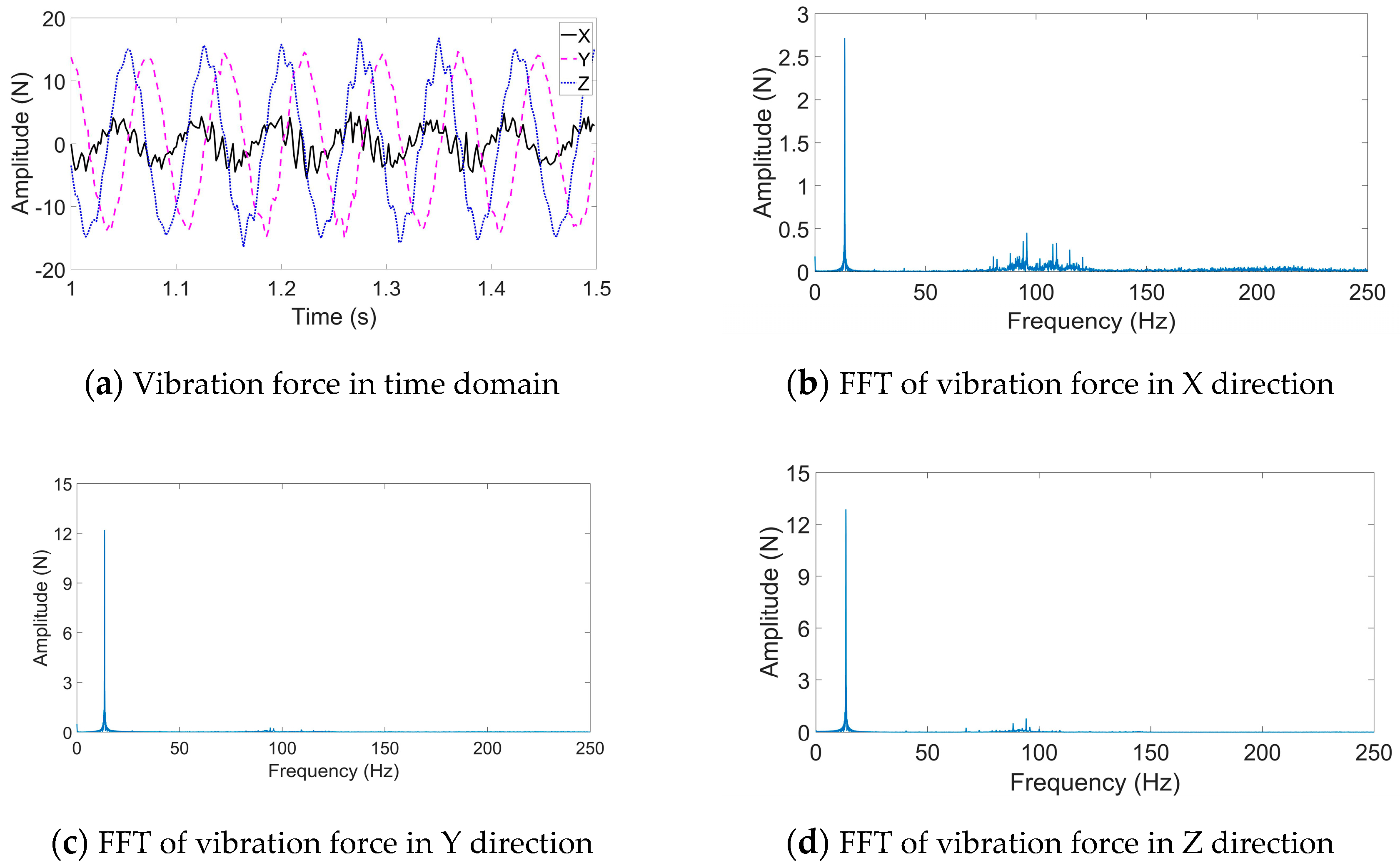
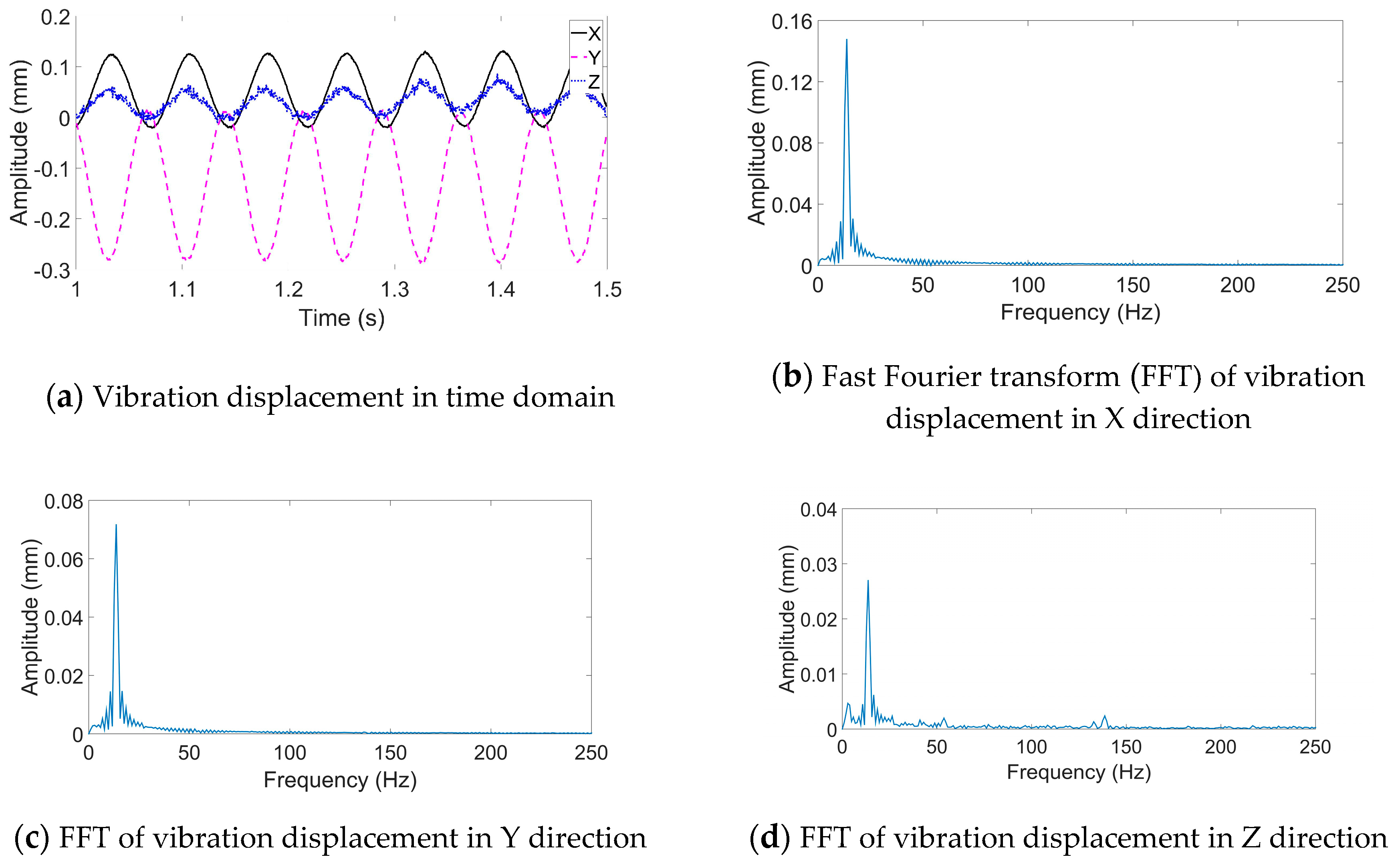
| Device | Type | Dynamic Characteristics | Unites | Value |
|---|---|---|---|---|
| Accelerometer | B&K 4524 transducer | Measuring Range | m/s2 | ±500 |
| Amplitude Response ±10% | Hz | X: 0.2 to 5500 Y: 0.25 to 3000 Z: 0.25 to 3000 | ||
| Residual Noise (1 to 6000 Hz) Broadband | mg | X: <0.4 Y: <0.2 Z: <0.2 | ||
| Transverse Sensitivity | % | <5 | ||
| Modal hammer | B&K impact hammer 8208 | Sensitivity | mV/N | 0.225 |
| Full Scale Force Range Compression | kN | 22.2 | ||
| Linear Error at Full Scale | % | <±2 |
| PONTOS System | Value |
|---|---|
| Focal length of lens | 35 mm |
| Measuring volume | 400 × 400 mm |
| Frame rate | 500 Hz |
| Resolution | 1024 × 1024 pixels |
| Type | Sensing Ranges | Measurement Uncertainty | ||
|---|---|---|---|---|
| ATI Delta IP 65 | Fx, Fy | Fz | Fx, Fy | Fz |
| 660 N | 1980 N | ±0.66 N | ±1.98 N | |
| Setting | Values |
|---|---|
| Hammer impact tip | Hard |
| Impact force | 200–300 N |
| Frequency span | 100 Hz |
| Frequency resolution | 0.125 Hz |
| Measuring time | 8 s |
| Average times | 5 |
| Frequency (Hz) | ||||||
|---|---|---|---|---|---|---|
| 14.0 | 0.018 | 0.170 | 0.454 | 0.002 | 0.006 | 0.005 |
| 25.0 | 0.100 | 1.235 | 0.162 | 0.031 | 0.042 | 0.005 |
| 40.0 | 1.678 | 2.042 | 1.953 | 0.123 | 0.137 | 0.046 |
| 75.0 | 0.982 | 19.790 | 6.054 | 0.087 | 0.606 | 0.593 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, K.; Kuhlenkoetter, B. Experimental Analysis of the Dynamic Stiffness in Industrial Robots. Appl. Sci. 2020, 10, 8332. https://doi.org/10.3390/app10238332
Wu K, Kuhlenkoetter B. Experimental Analysis of the Dynamic Stiffness in Industrial Robots. Applied Sciences. 2020; 10(23):8332. https://doi.org/10.3390/app10238332
Chicago/Turabian StyleWu, Kai, and Bernd Kuhlenkoetter. 2020. "Experimental Analysis of the Dynamic Stiffness in Industrial Robots" Applied Sciences 10, no. 23: 8332. https://doi.org/10.3390/app10238332
APA StyleWu, K., & Kuhlenkoetter, B. (2020). Experimental Analysis of the Dynamic Stiffness in Industrial Robots. Applied Sciences, 10(23), 8332. https://doi.org/10.3390/app10238332






