P-Wave Reflection Approximation of a Thin Bed and Its Application
Abstract
1. Introduction
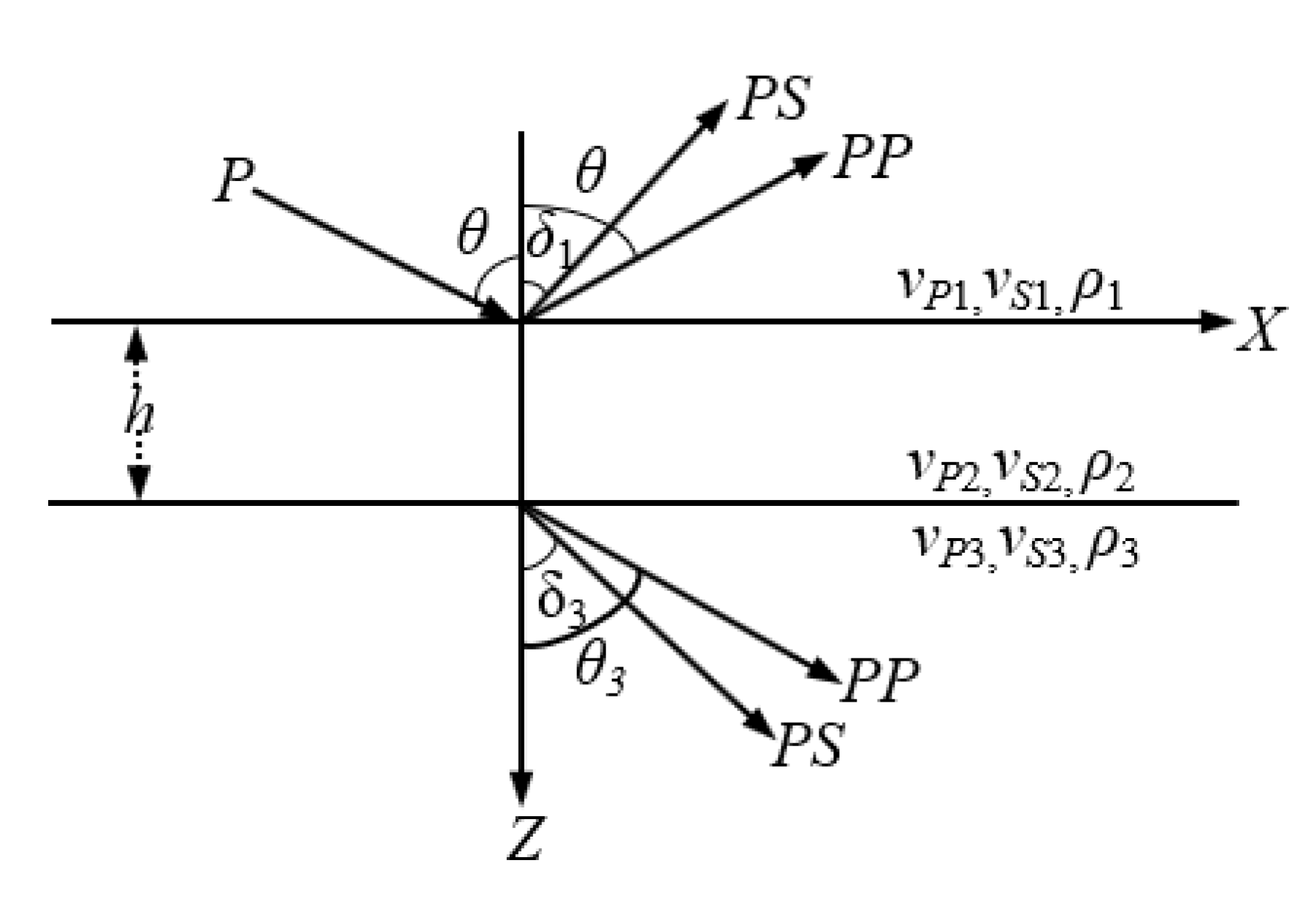
2. Theory
2.1. P-Wave Reflected Approximation of a Thin Bed
2.2. Inversion of Thin-Bed Elastic Parameters and Thickness
2.2.1. Extraction of Complex Reflection Coefficients
2.2.2. AVA Inversion of Thin-Bed Properties
3. Numerical Analysis and Application
3.1. Approximation Accuracy Analysis
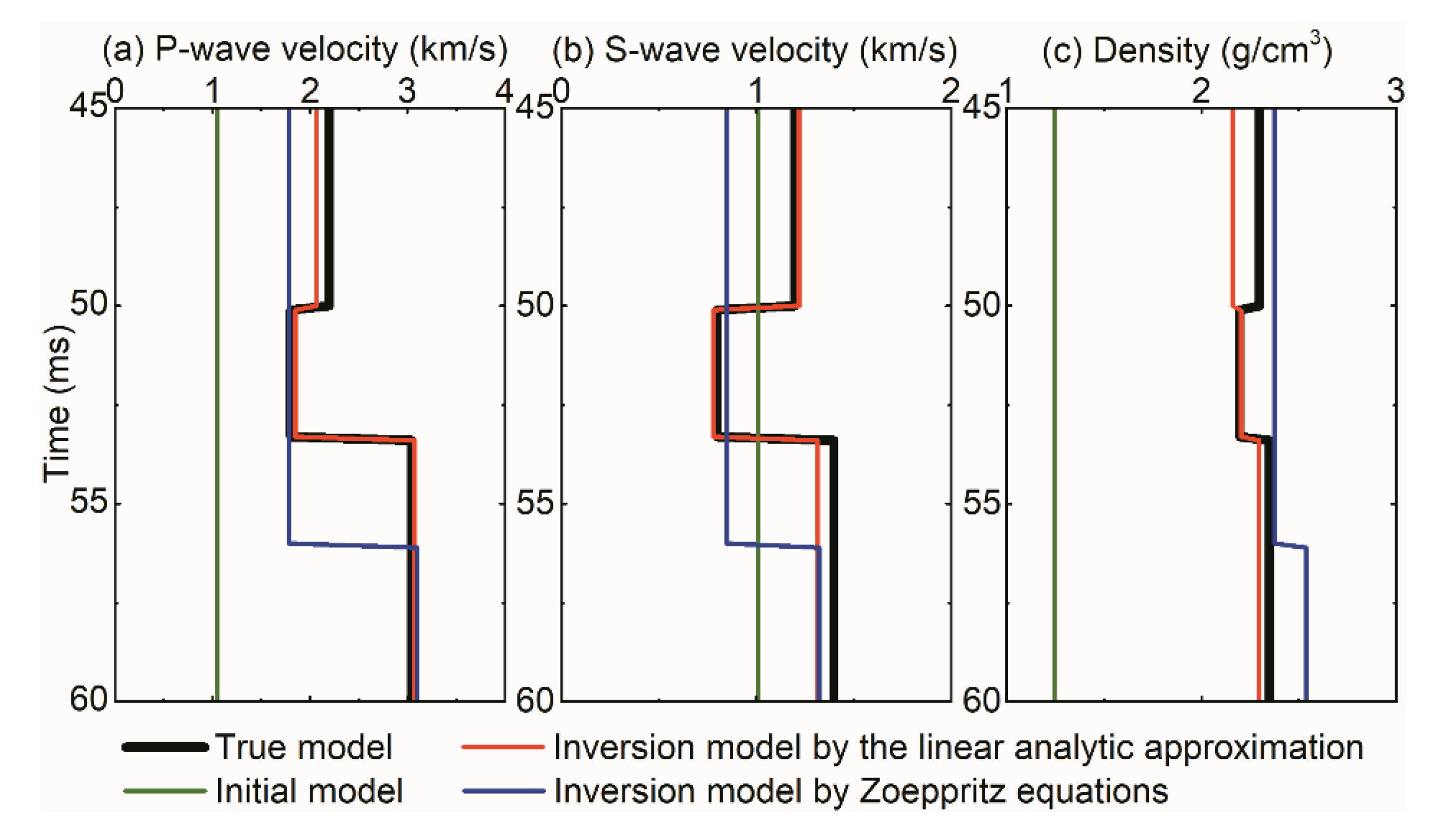
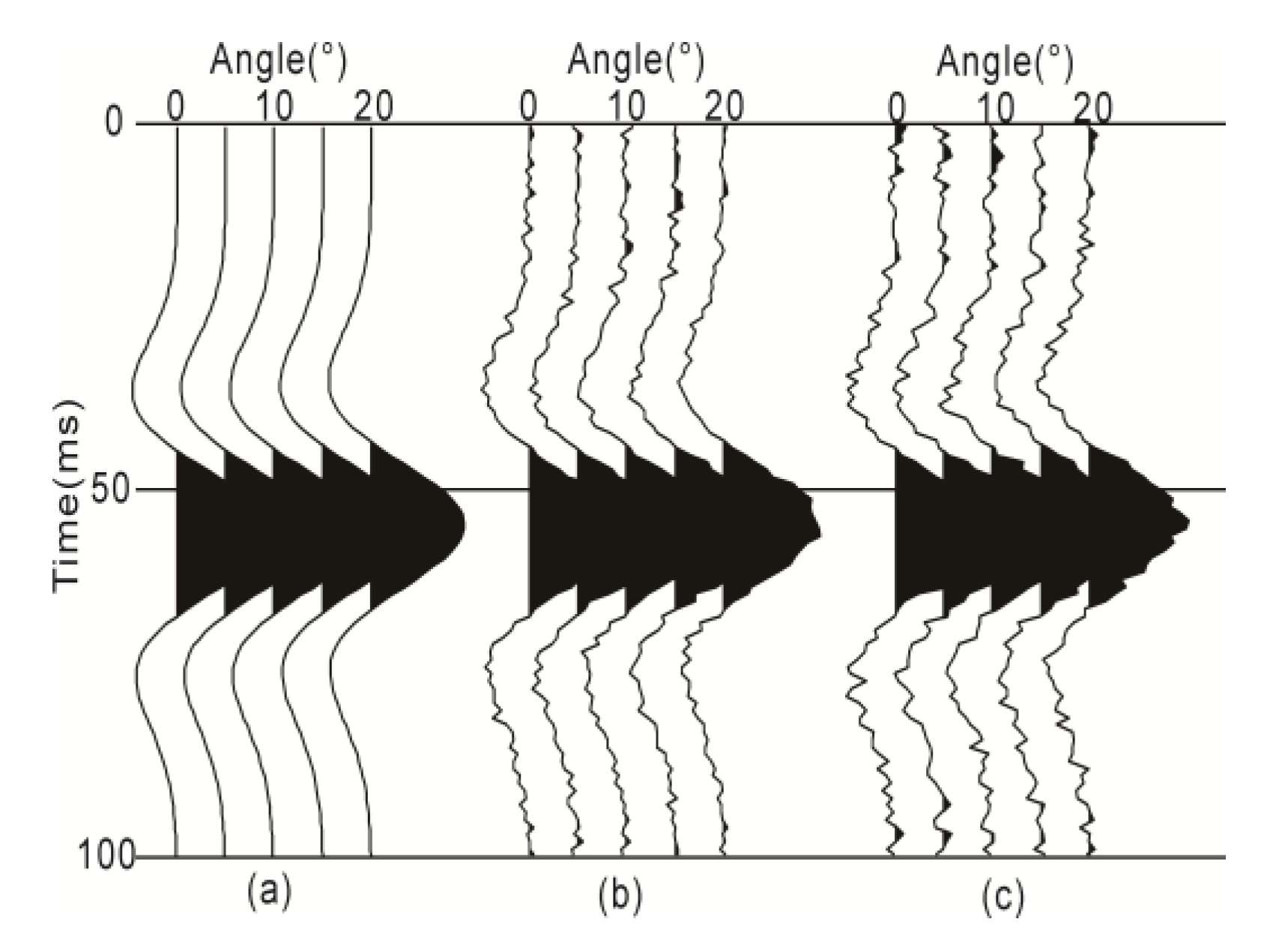
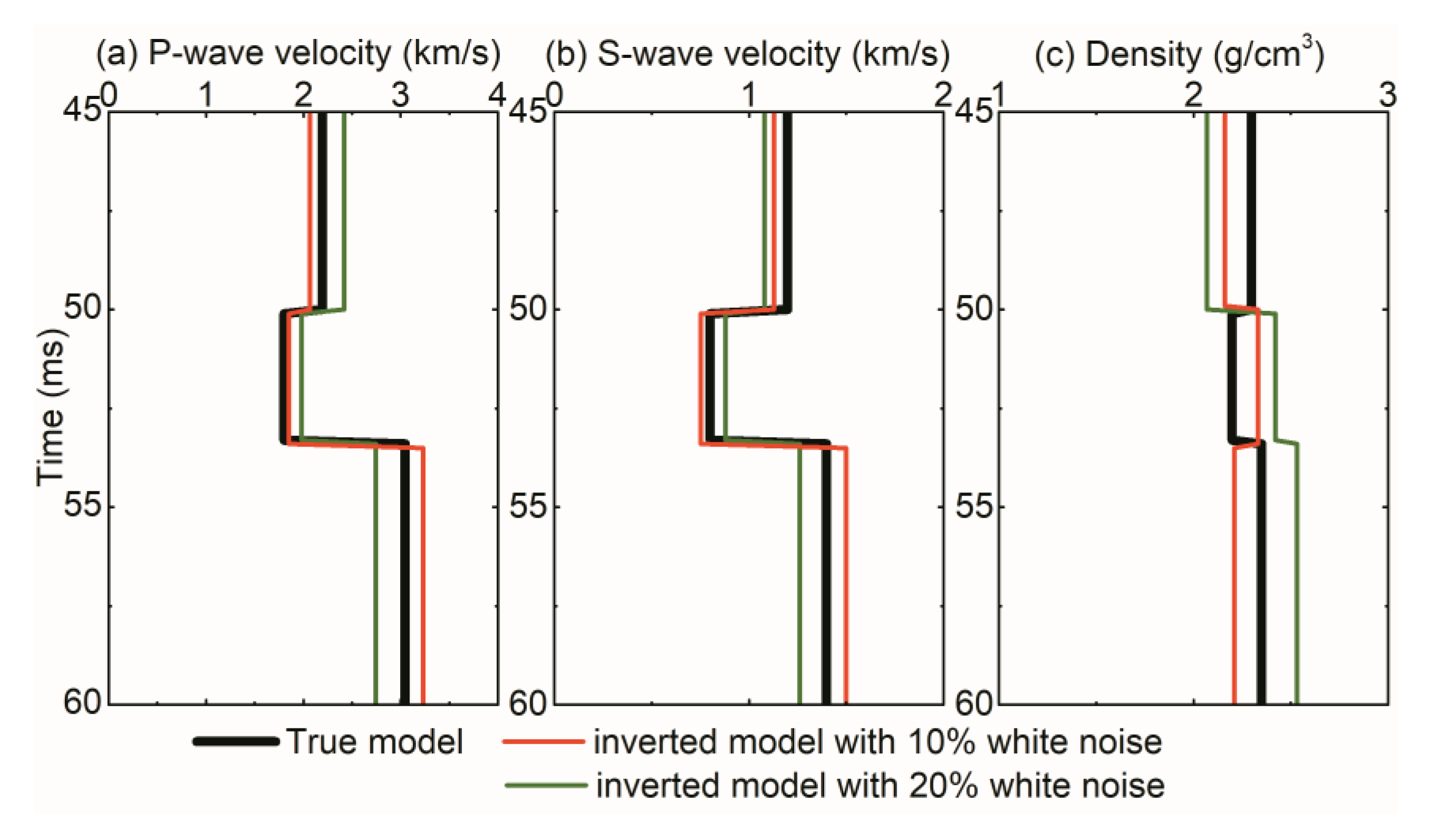
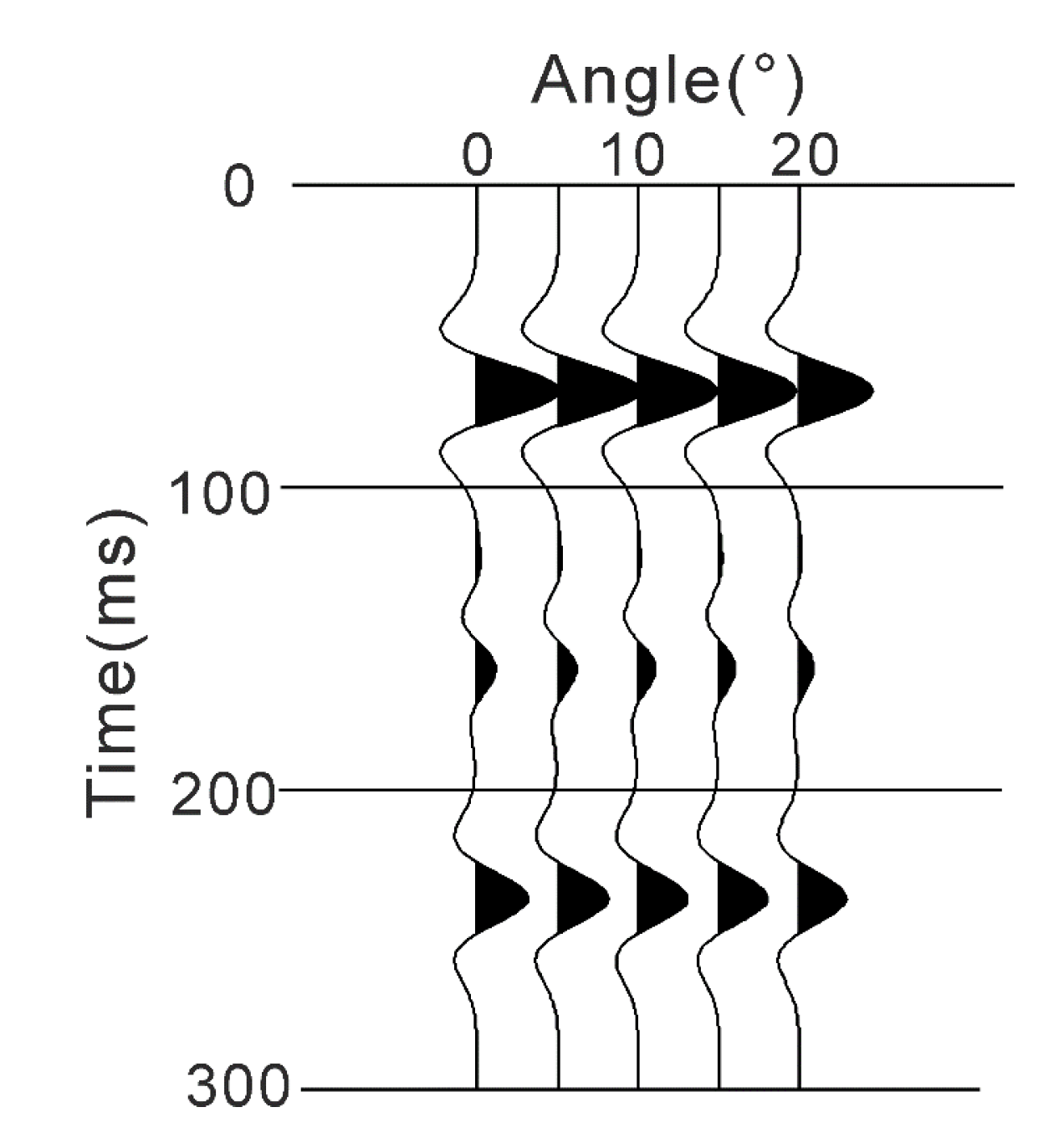
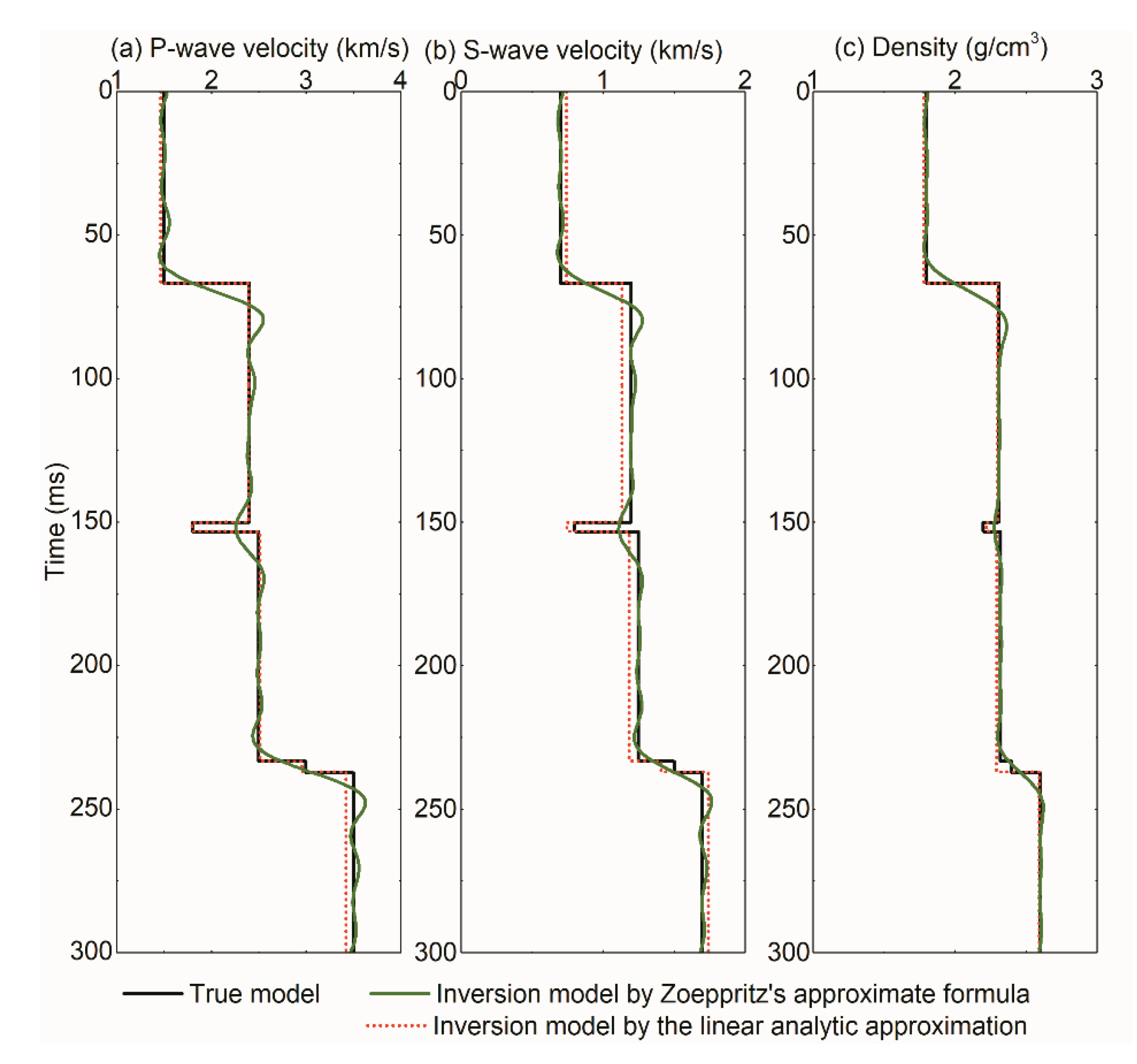
3.2. Application Examples
4. Discussion
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A
Appendix B
References
- Zeng, H.; Backus, M.M. Interpretive advantages of 90°-phase wavelets: Part 1—Modeling. Geophysics 2005, 70, C7–C15. [Google Scholar] [CrossRef]
- Almoghrabi, H.; Lange, J. Layers and bright spots. Geophysics 1986, 51, 699–709. [Google Scholar] [CrossRef]
- Juhlin, C.; Young, R. Implications of thin layers for amplitude variation with offset (AVO) studies. Geophysics 1993, 58, 1200–1204. [Google Scholar] [CrossRef]
- Widess, M.B. How thin is a thin bed? Geophysics 1973, 38, 1176–1180. [Google Scholar] [CrossRef]
- Neidell, N.S.; Poggiagliolmi, E. Stratigraphic modeling and interpretation—Geophysical principles, in Payton, C.E., Eds., Seismic stratigraphy—Application to hydrocarbon exploration. Am. Assn. Petr. Geol. Memoir 1977, 26, 389–416. [Google Scholar]
- Koefoed, O.; De Voogd, N. The linear properties of thin layers, with an application to synthetic seismograms over coal seams. Geophysics 1980, 45, 1254–1268. [Google Scholar] [CrossRef]
- De Voogd, N.; Rooijen, H.D. Thin-layer response and spectral bandwidth. Geophysics 1983, 48, 12–18. [Google Scholar] [CrossRef]
- Chung, H.; Lawton, D.C. Amplitude responses of thin beds: Sinusoidal approximation versus Ricker approximation. Geophysics 1995, 60, 223–230. [Google Scholar] [CrossRef]
- Chung, H.M.; Lawton, D.C. Frequency characteristics of seismic reflections from thin beds. Can. J. Explor. Geophys. 1995, 31, 32–37. [Google Scholar]
- Liu, Y.; Schmitt, D.R. Amplitude and AVO responses of a single thin bed. Geophysics. 2003, 68, 1161–1168. [Google Scholar] [CrossRef]
- Thomson, W.T. Transmission of Elastic Waves through a Stratified Solid Medium. J. Appl. Phys. 1950, 21, 89–93. [Google Scholar] [CrossRef]
- Haskell, N.A. The dispersion of surface waves on multilayered media. In Vincit Veritas: A Portrait of the Life and Work of Norman Abraham Haskell; Wiley: New Jersey, NJ, USA, 1990; pp. 86–103. [Google Scholar] [CrossRef]
- Brekhovskikh, L.M. Waves in Layered Media; Academic Process: Tamil Nadu, India, 1960. [Google Scholar]
- Meissner, R.; Meixner, E. Deformation of seismic wavelets by thin layers and layered boundaries. Geophys. Prospect. 1969, 17, 1–27. [Google Scholar] [CrossRef]
- Yang, C.; Wang, Y.; Lu, J. Weak impedance difference approximations of thin-bed PP-wave reflection responses. J. Geophys. Eng. 2017, 14, 1010–1019. [Google Scholar] [CrossRef]
- Kennett, B.L.N.; Kerry, N.J.; Woodhouse, J.H. Symmetries in the reflection and transmission of elastic waves. Geophys. J. Int. 1978, 52, 215–229. [Google Scholar] [CrossRef]
- Kennett, B.L.N. Seismic Wave Propagation in a Stratified Medium; Cambridge University Press: Cambridge, UK, 1983. [Google Scholar]
- Rubino, J.G.; Velis, D. Thin-bed pre-stack spectral inversion. Geophysics 2009, 74, R49–R57. [Google Scholar] [CrossRef]
- Pan, W.; Innanen, K.A. AVO/AVF analysis of thin beds in elastic media. SEG Tech. Program Expand. Abstr. 2013, 2013, 373–377. [Google Scholar]
- Yang, C.; Wang, Y.; Wang, Y. Reflection and transmission coefficients of a thin bed. Geophysics 2016, 81, N31–N39. [Google Scholar] [CrossRef]
- Yang, C.; Wang, Y.; Lu, J.; Chen, B.; Shi, L. A Low-Order Series Approximation of Thin-Bed PP-Wave Reflections. Appl. Sci. 2019, 9, 709. [Google Scholar] [CrossRef]
- Aki, K.; Richards, P.G. Quantitative Seismology—Theory and Methods; W.H. Freeman and Co: New York, NY, USA, 1980. [Google Scholar]
- Shuey, R.T. A simplification of the Zoeppritz equations. Geophysics 1985, 50, 609–614. [Google Scholar] [CrossRef]
- Smith, G.; Gidlow, P. Weighted stacking for rock property estimation and detection of gas. Geophys. Prospect. 1987, 35, 993–1014. [Google Scholar] [CrossRef]
- Taner, M.T.; Koehler, F.; Sheriff, R.E. Complex seismic trace analysis. Geophysics 1979, 44, 1041–1063. [Google Scholar] [CrossRef]
- Robertson, J.D.; Nogami, H.H. Complex seismic trace analysis of thin beds. Geophysics 1984, 49, 344–352. [Google Scholar] [CrossRef]
- Barnes, A.E. Theory of 2-D complex seismic trace analysis. Geophysics 1996, 61, 264–272. [Google Scholar] [CrossRef]
- Liu, Y.; Tinivella, U.; Liu, X. An inversion method for seafloor elastic parameters. Geophysics 2015, 80, N11–N21. [Google Scholar] [CrossRef]
- Levenberg, K. A method for the solution of certain non-linear problems in least squares. Q. Appl. Math. 1944, 2, 164–168. [Google Scholar] [CrossRef]
- Marquardt, D.W. An algorithm for least-squares estimation of non-linear inequalities. J. Soc. Ind. Appl. Math. 1963, 11, 431–441. [Google Scholar]
- Lu, J.; Yang, Z.; Wang, Y.; Shi, Y. Joint PP and PS AVA seismic inversion using exact Zoeppritz equations. Geophysics 2015, 80, R239–R250. [Google Scholar] [CrossRef]
- Lu, J.; Wang, Y.; Chen, J. Detection of Tectonically Deformed Coal Using Model-Based Joint Inversion of Multi-Component Seismic Data. Energies 2018, 11, 829. [Google Scholar] [CrossRef]
- Chen, T.; Liu, Y. Multi-component AVO response of thin beds based on reflectance spectrum theory. Appl. Geophys. 2006, 3, 27–36. [Google Scholar] [CrossRef]
- Fatti, J.L.; Smith, G.C.; Vail, P.J.; Strauss, P.J.; Levitt, P.R. Detection of gas in sandstone reservoirs using AVO analysis: A 3-D seismic case history using the Geostack technique. Geophysics 1994, 59, 1362–1376. [Google Scholar] [CrossRef]
- Pan, W.; Innanen, K.A.; Geng, Y. Elastic full-waveform inversion and parameterization analysis applied to walk-away vertical seismic profile data for unconventional (heavy oil) reservoir characterization. Geophys. J. Int. 2018, 213, 1934–1958. [Google Scholar] [CrossRef]
- Postma, G.W. Wave propagation in a stratified medium. Geophysics 1955, 20, 780–806. [Google Scholar] [CrossRef]
- Backus, G.E. Long-wave elastic anisotropy produced by horizontal layering. J. Geophys. Res. Space Phys. 1962, 67, 4427–4440. [Google Scholar] [CrossRef]
- Stovas, A.; Landrø, M.; Avseth, P. AVO attribute inversion for finely layered reservoirs. Geophysics 2006, 71, C25–C36. [Google Scholar] [CrossRef]
- Stovas, A.; Landro, M. Uncertainty in discrimination between net-to-gross and water saturation for fine-layered reservoirs. SEG Tech. Program Expand. Abstr. 2006, 25, 1698–1702. [Google Scholar] [CrossRef]
- Roganov, Y.; Stovas, A. Low-frequency wave propagation in periodically layered media. Geophys. Prospect. 2012, 60, 825–837. [Google Scholar] [CrossRef]
- An, Y.; Lu, J. Calculation of AVA Responses for Finely Layered Reservoirs. Math. Probl. Eng. 2018, 2018, 1–11. [Google Scholar] [CrossRef]
- Lavaud, B.; Kabir, N.; Chavent, G. Pushing AVO Inversion beyond Linearized Approximation. In Proceedings of the EAGE 61st Conference on European Association Geoscience and Engineering, Helsinki, Finland, 7–11 June 1999; pp. 6–49. [Google Scholar] [CrossRef]
- Kurt, H. Joint inversion of AVA data for elastic parameters by bootstrapping. Comput. Geosci. 2007, 33, 367–382. [Google Scholar] [CrossRef]
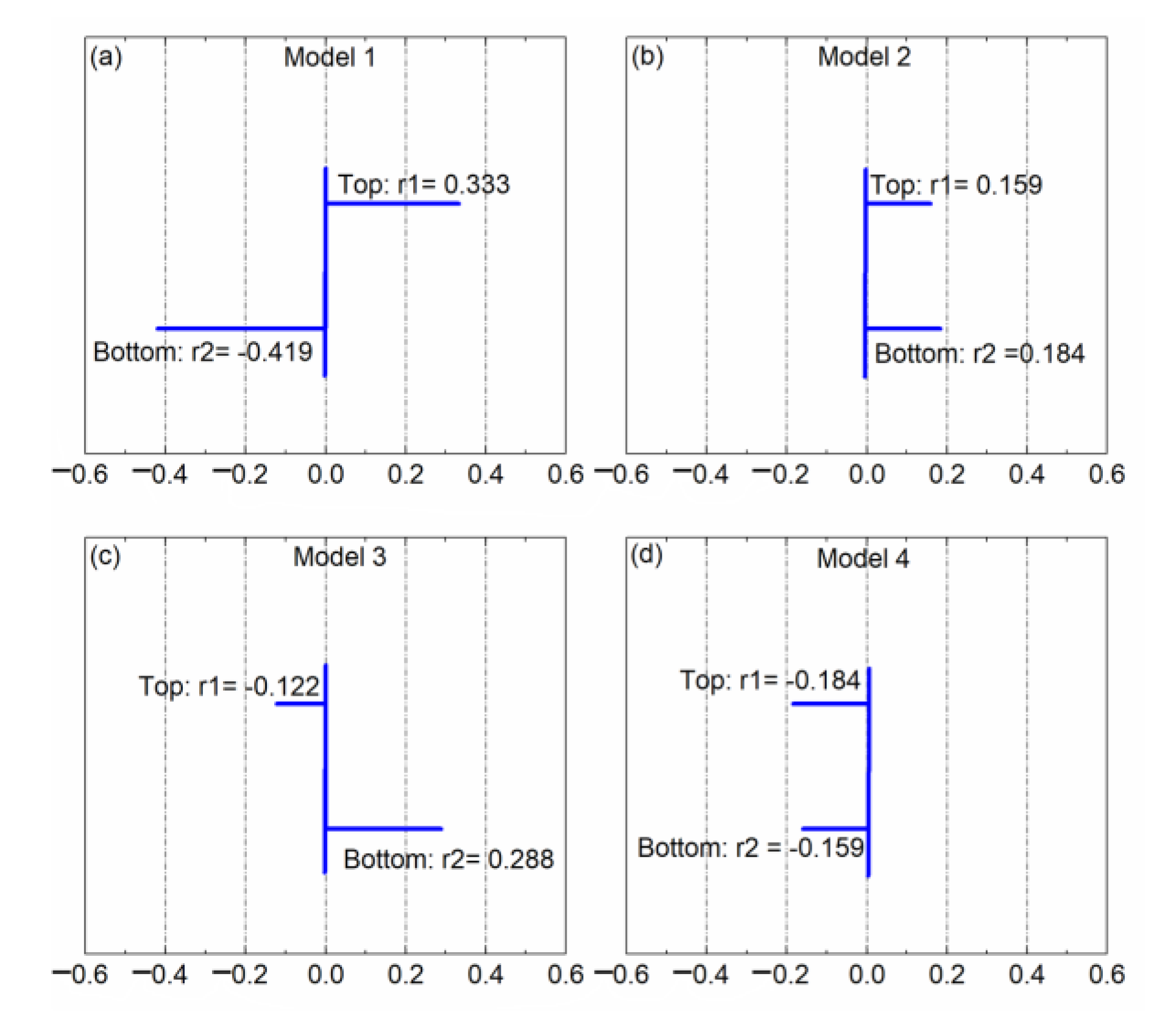
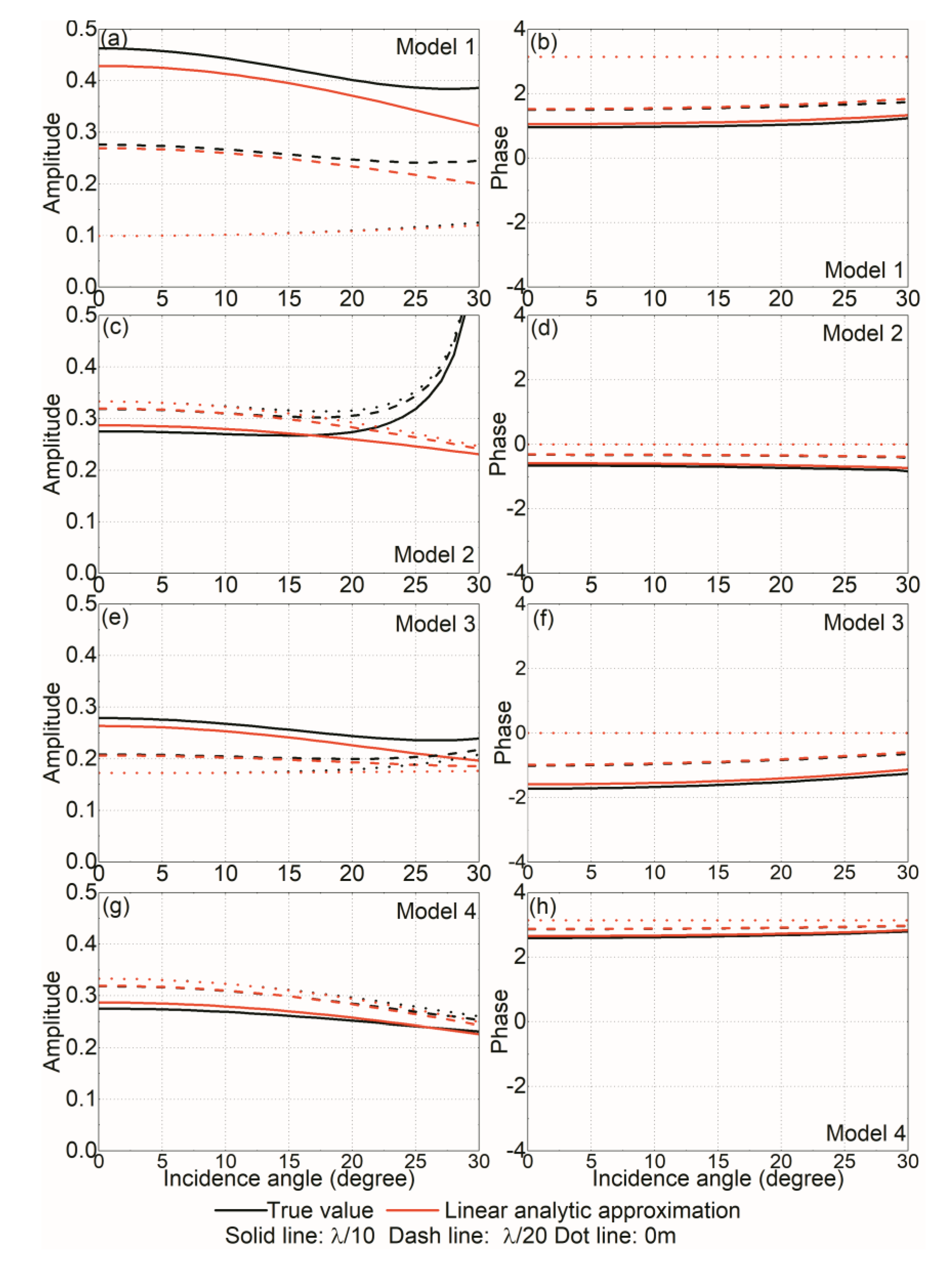



| Layer No. | vP | vS | ρ | IP | IS | |
|---|---|---|---|---|---|---|
| Model 1 | 1 | 3050 | 1525 | 2.7 | 8235 | 4117.5 |
| 2 | 6100 | 3050 | 2.7 | 16,470 | 8235 | |
| 3 | 2500 | 1525 | 2.7 | 6750 | 4117.5 | |
| Model 2 | 1 | 3050 | 1600 | 2.7 | 8235 | 4320 |
| 2 | 4200 | 2500 | 2.7 | 11,340 | 6750 | |
| 3 | 6100 | 3100 | 2.7 | 16,470 | 8370 | |
| Model 3 | 1 | 2200 | 1200 | 2.3 | 5060 | 2760 |
| 2 | 1800 | 800 | 2.2 | 3960 | 1760 | |
| 3 | 3050 | 1400 | 2.35 | 7167.5 | 3290 | |
| Model 4 | 1 | 6100 | 3100 | 2.7 | 16,470 | 8370 |
| 2 | 4200 | 2500 | 2.7 | 11,340 | 6750 | |
| 3 | 3050 | 1600 | 2.7 | 8235 | 4320 |
| Layer No. | vP | vS | ρ | Thickness | |
|---|---|---|---|---|---|
| Model 5 | 1 | 1500 | 700 | 1.80 | 50 |
| 2 | 2400 | 1200 | 2.31 | 100 | |
| 3 | 1800 | 800 | 2.20 | 3 | |
| 4 | 2500 | 1250 | 2.32 | 100 | |
| 5 | 3000 | 1500 | 2.40 | 6 | |
| 6 | 3500 | 1700 | 2.60 | ∞ |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, C.; Wang, Y.; Xiong, S.; Li, Z.; Han, H. P-Wave Reflection Approximation of a Thin Bed and Its Application. Appl. Sci. 2020, 10, 8061. https://doi.org/10.3390/app10228061
Yang C, Wang Y, Xiong S, Li Z, Han H. P-Wave Reflection Approximation of a Thin Bed and Its Application. Applied Sciences. 2020; 10(22):8061. https://doi.org/10.3390/app10228061
Chicago/Turabian StyleYang, Chun, Yun Wang, Shu Xiong, Zikun Li, and Hewei Han. 2020. "P-Wave Reflection Approximation of a Thin Bed and Its Application" Applied Sciences 10, no. 22: 8061. https://doi.org/10.3390/app10228061
APA StyleYang, C., Wang, Y., Xiong, S., Li, Z., & Han, H. (2020). P-Wave Reflection Approximation of a Thin Bed and Its Application. Applied Sciences, 10(22), 8061. https://doi.org/10.3390/app10228061






