Photosynthetic Active Radiation, Solar Irradiance and the CIE Standard Sky Classification
Abstract
Featured Application:
Abstract
1. Introduction
2. Experimental Data
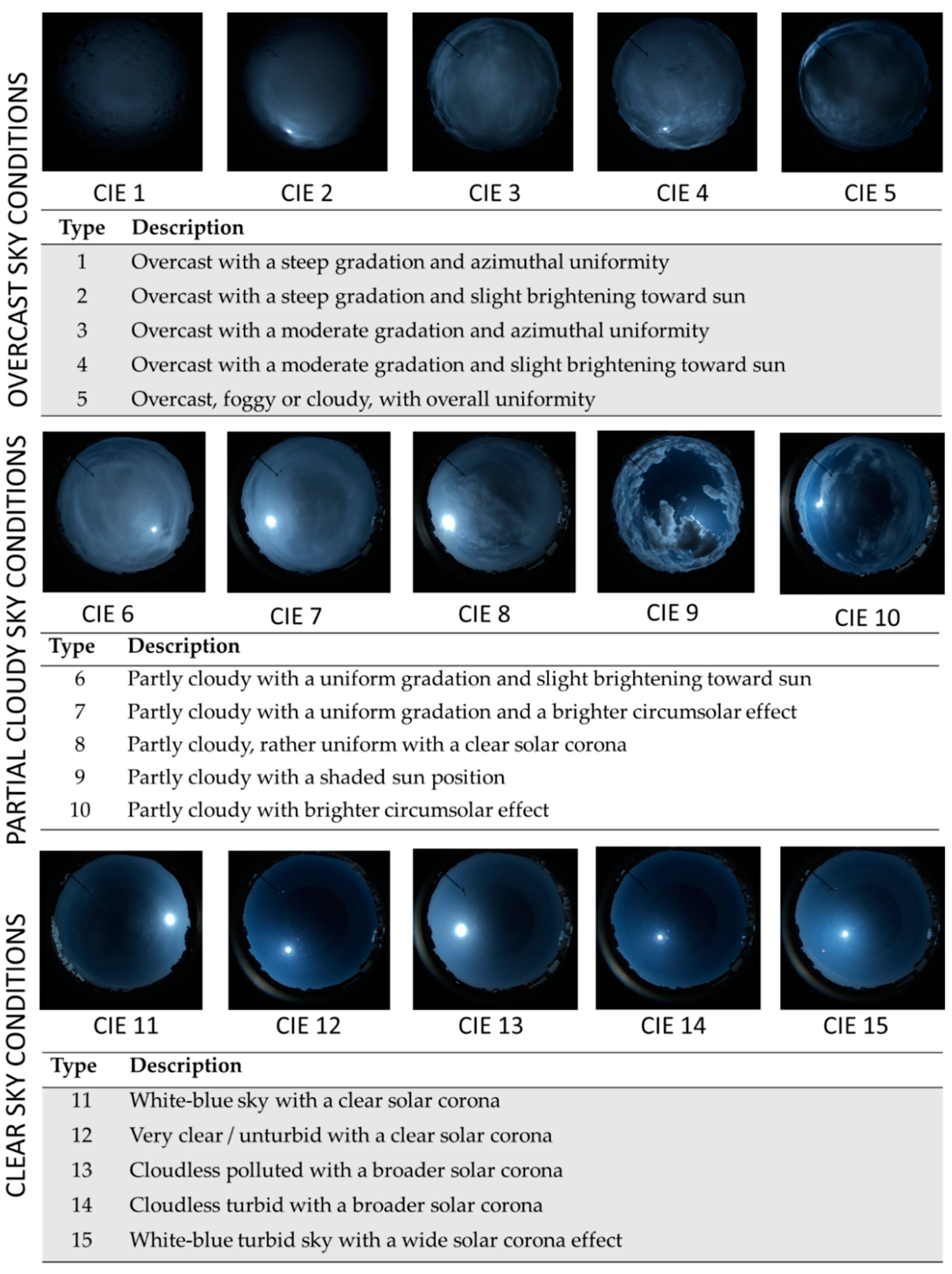
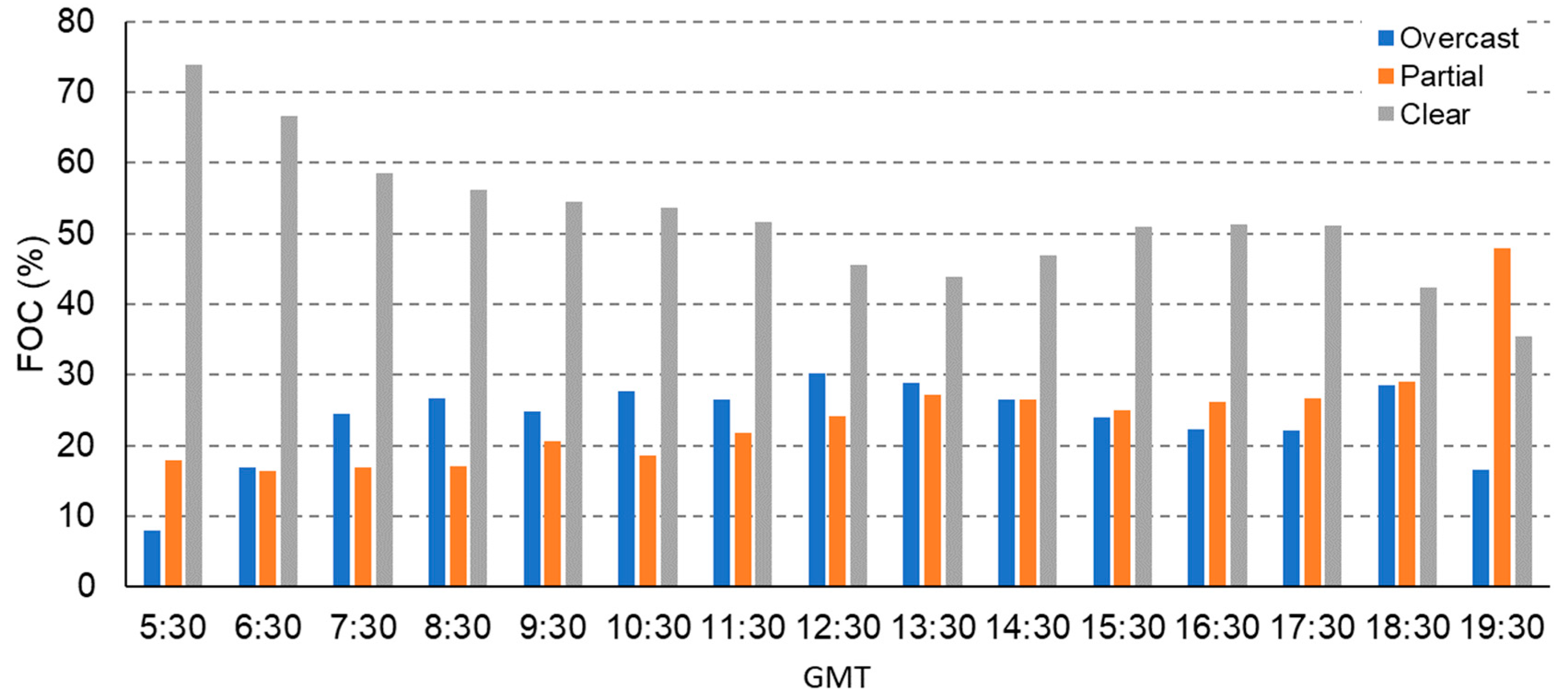
3. CIE Standard Sky Classification in Burgos between 1 April 2019 and 21 January 2020
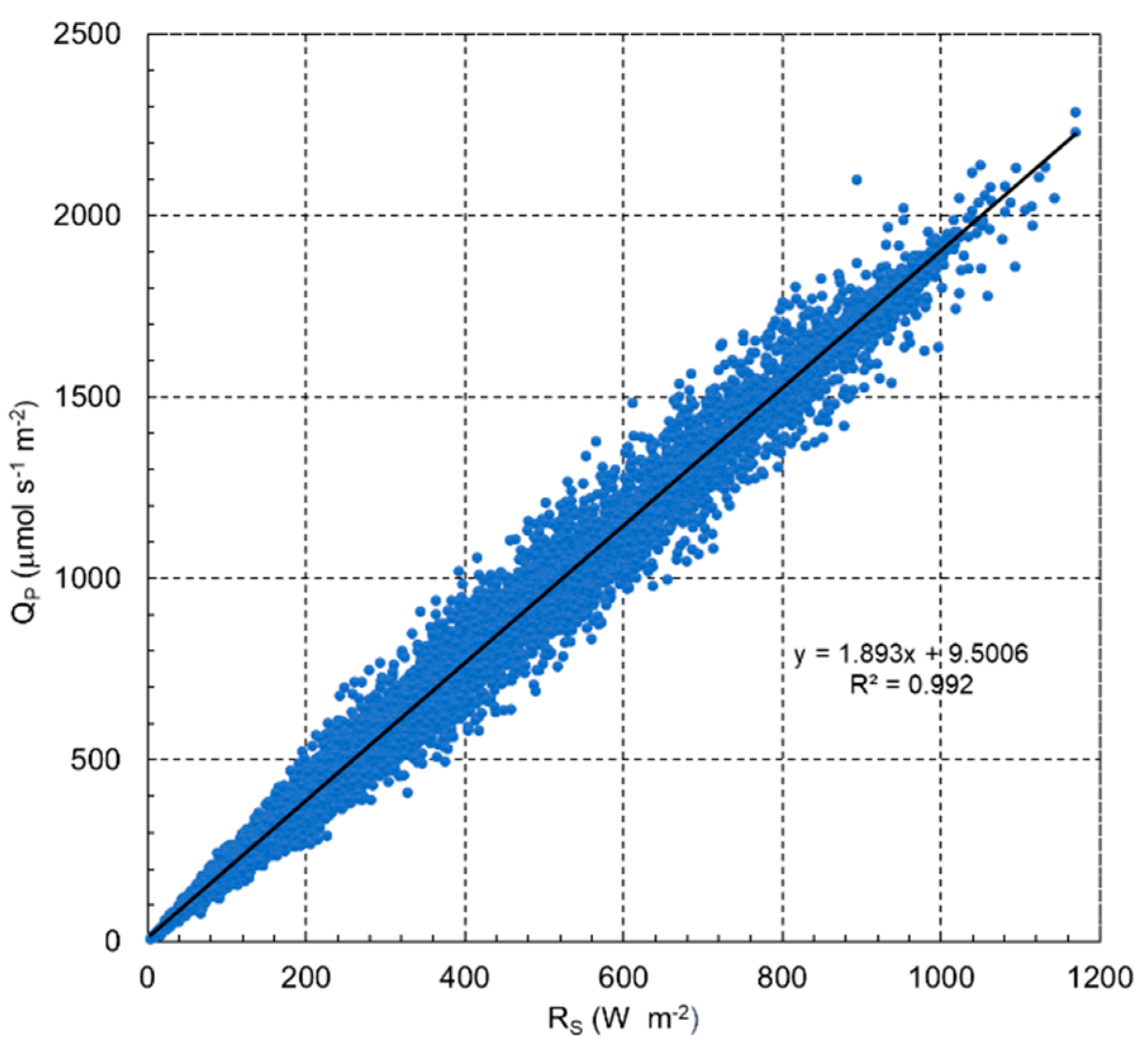
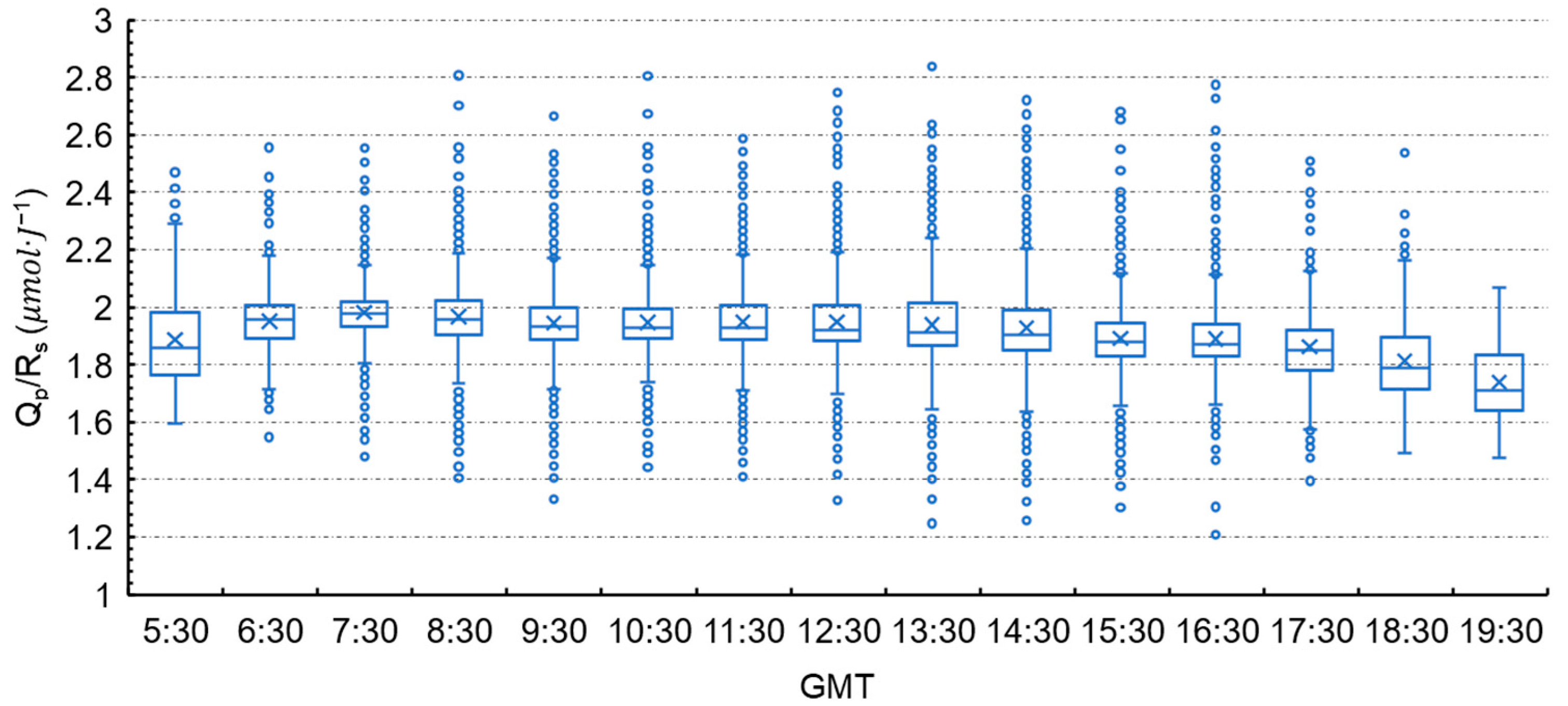
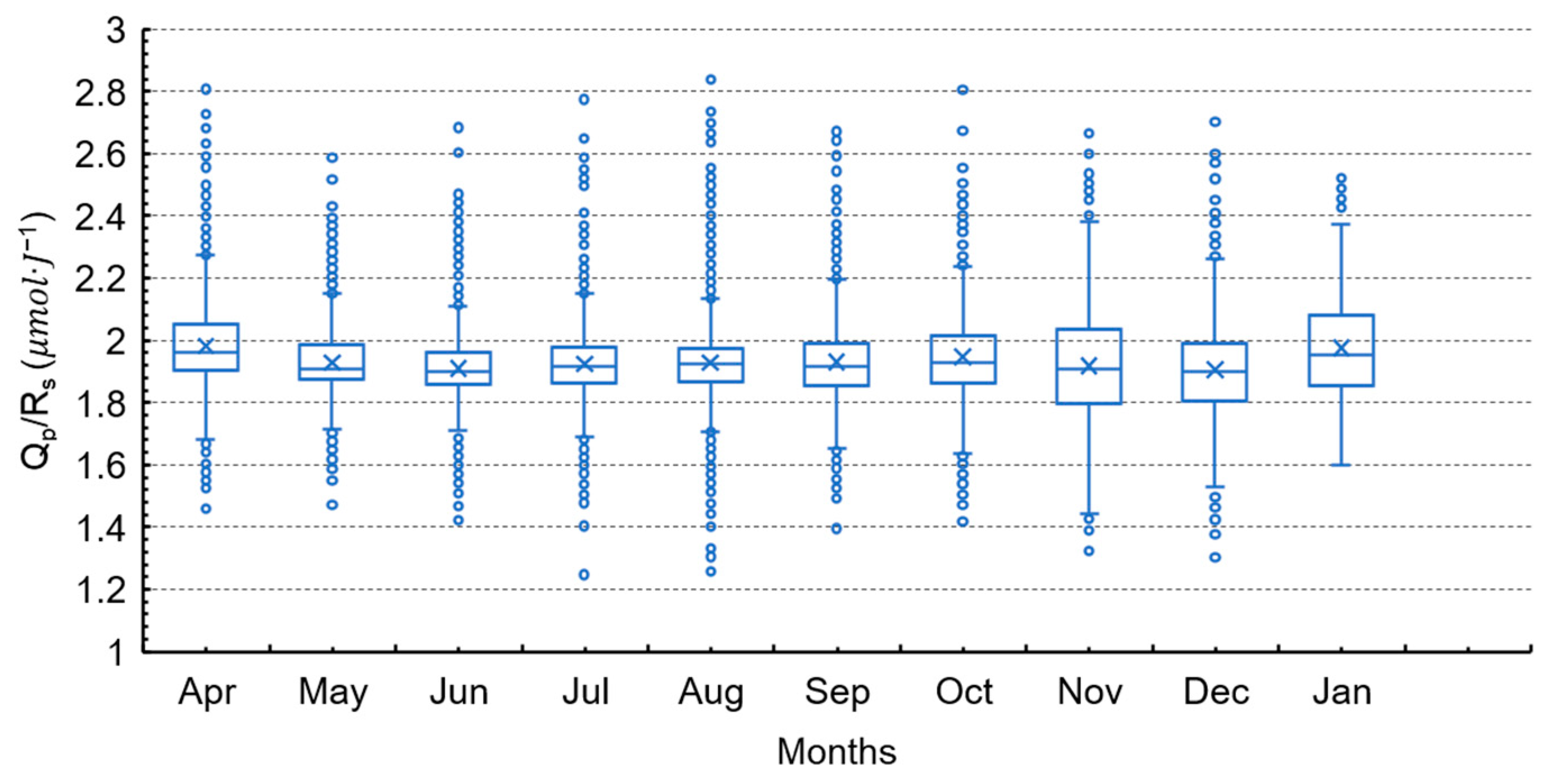
4. Temporal Variability of the Ratio
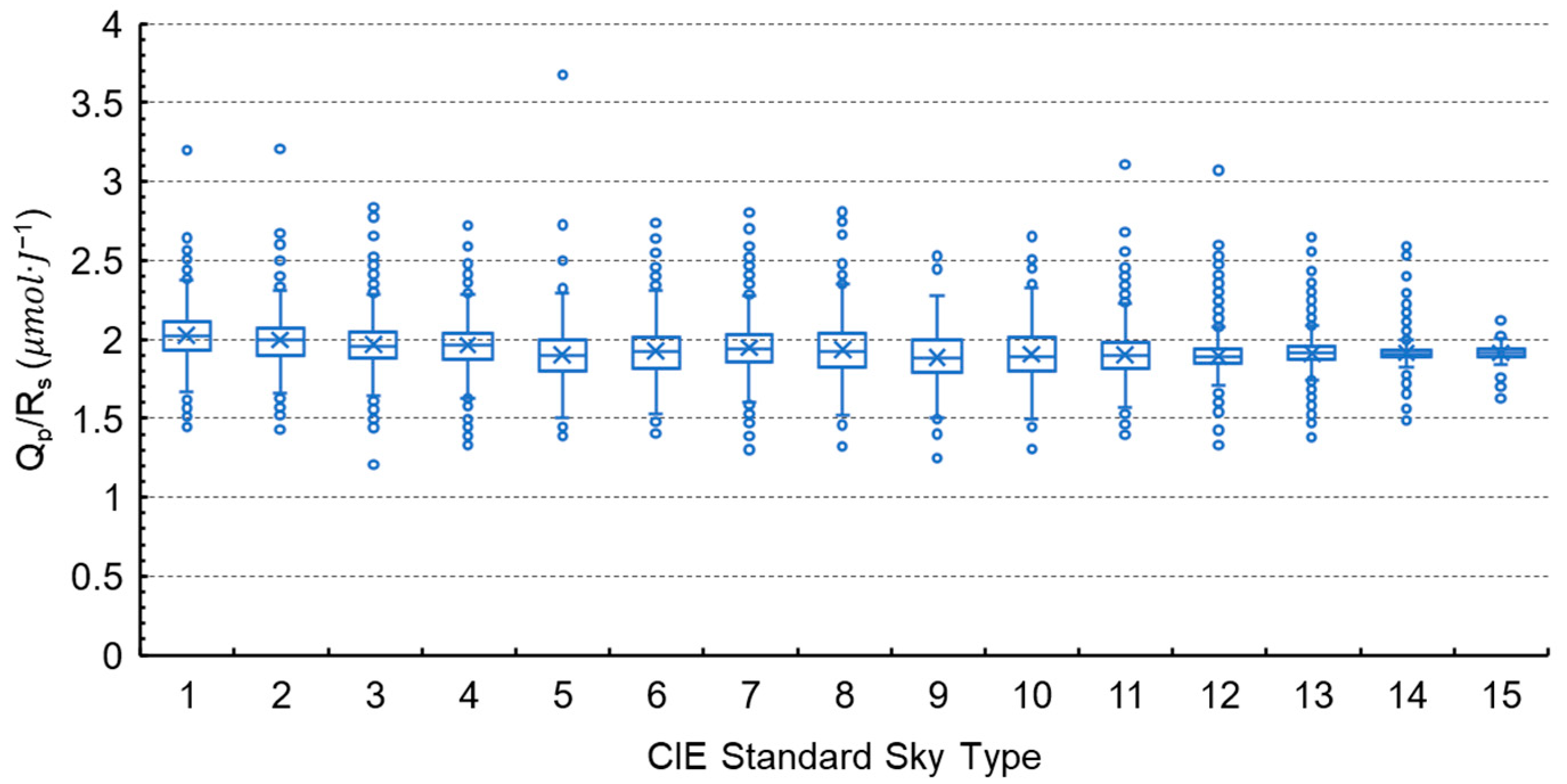
5. Variability of the Ratio with the CIE Standard Sky Types
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- McCree, K.J. The measurement of photosynthetically active radiation. Sol. Energy 1973, 15, 83–87. [Google Scholar] [CrossRef]
- Akitsu, T.; Kume, A.; Hirose, Y.; Ijima, O.; Nasahara, K.N. On the stability of radiometric ratios of photosynthetically active radiation to global solar radiation in Tsukuba, Japan. Agric. For. Meteorol. 2015, 209–210, 59–68. [Google Scholar] [CrossRef]
- Aguiar, L.J.G.; Fischer, G.R.; Ladle, R.J.; Malhado, A.C.M.; Justino, F.B.; Aguiar, R.G.; da Costa, J.M.N. Modeling the photosynthetically active radiation in South West Amazonia under all sky conditions. Theor. Appl. Climatol. 2012, 108, 631–640. [Google Scholar] [CrossRef]
- Alados, I.; Foyo-Moreno, I.; Alados-Arboledas, L. Photosynthetically active radiation: Measurements and modelling. Agric. For. Meteorol. 1996, 78, 121–131. [Google Scholar] [CrossRef]
- McCree, K.J. Test of current definitions of photosynthetically active radiation against leaf photosynthesis data. Agric. Meteorol. 1972, 10, 443–453. [Google Scholar] [CrossRef]
- Landsberg, J.J.; Waring, R.H. A generalised model of forest productivity using simplified concepts of radiation-use efficiency, carbon balance and partitioning. For. Ecol. Manag. 1997, 95, 209–228. [Google Scholar] [CrossRef]
- Kirk, J. Spectral Distribution of Photosynthetically Active Radiation in some South-eastern Australian Waters. Mar. Freshw. Res. 1979, 30, 81–91. [Google Scholar] [CrossRef]
- Pei, F.; Li, X.; Liu, X.; Lao, C. Assessing the impacts of droughts on net primary productivity in China. J. Environ. Manag. 2013, 114, 362–371. [Google Scholar] [CrossRef]
- Tsubo, M.; Walker, S. Relationships between photosynthetically active radiation and clearness index at Bloemfontein, South Africa. Theor. Appl. Climatol. 2005, 80, 17–25. [Google Scholar] [CrossRef]
- Moon, P. Proposed standard solar-radiation curves for engineering use. J. Frankl. Inst. 1940, 230, 583–617. [Google Scholar] [CrossRef]
- Monteith, J.L.; Reifsnyder, W.E. Principles of Environmental Physics. Phys. Today 1973, 27, 51–52. [Google Scholar] [CrossRef]
- Szeicz, G. Solar radiation for plant growth. J. Appl. Ecol. 1974, 11, 617–636. [Google Scholar] [CrossRef]
- McCartney, H.A. Spectral distribution of solar radiation. II: Global and diffuse. Q. J. R. Meteorol. Soc. 1978, 104, 911–926. [Google Scholar] [CrossRef]
- Al-Shooshan, A.A. Estimation of photosynthetically active radiation under an arid climate. J. Agric. Eng. Res. 1997, 66, 9–13. [Google Scholar] [CrossRef]
- Foyo-Moreno, I.; Alados, I.; Alados-Arboledas, L. A new conventional regression model to estimate hourly photosynthetic photon flux density under all sky conditions. Int. J. Climatol. 2017, 37, 1067–1075. [Google Scholar] [CrossRef]
- Bai, J. Observations and estimations of PAR and solar visible radiation in North China. J. Atmos. Chem. 2012, 69, 231–252. [Google Scholar] [CrossRef]
- Hu, B.; Wang, Y.; Liu, G. Spatiotemporal characteristics of photosynthetically active radiation in China. J. Geophys. Res. Atmos. 2007, 112. [Google Scholar] [CrossRef]
- Meek, D.W.; Hatfield, J.L.; Howell, T.A.; Idso, S.B.; Reginato, R.J. Generalized relationship between photosynthetically active radiation and solar radiation. J. Agron. 1984, 76, 939–945. [Google Scholar] [CrossRef]
- Stanhill, G.; Fuchs, M. The relative flux density of photosynthetically active radiation. J. Appl. Ecol. 1977, 14, 317–322. [Google Scholar] [CrossRef]
- Blackburn, W.J.; Proctor, J.T.A. Estimating photosynthetically active radiation from measured solar irradiance. Sol. Energy 1983, 31, 233–234. [Google Scholar] [CrossRef]
- Stigter, C.J.; Musabilha, V.M.M. The conservative ratio of photosynthetically active to total radiation in the tropics (Dar es Salaam). J. Appl. Ecol. 1982, 19, 853–858. [Google Scholar] [CrossRef]
- Rao, C.R.N. Photosynthetically active components of global solar radiation: Measurements and model computations. Arch. Meteorol. Geophys. Bioclimatol. Ser. B 1984, 34, 353–364. [Google Scholar] [CrossRef]
- Harmel, T.; Chami, M. Estimation of daily photosynthetically active radiation (PAR) in presence of low to high aerosol loads: Application to OLCI-like satellite data. Opt. Express 2016, 24, A1390–A1407. [Google Scholar] [CrossRef] [PubMed]
- Bat-Oyun, T.; Shinoda, M.; Tsubo, M. Effects of cloud, atmospheric water vapour and dust on photosynthetically active radiation and total solar radiation in a Mongolian grassland. J. Arid Land 2012, 4, 349–356. [Google Scholar] [CrossRef]
- Escobedo, J.F.; Gomes, E.N.; Oliveira, A.P.; Soares, J. Modeling hourly and daily fractions of UV, PAR and NIR to global solar radiation under various sky conditions at Botucatu, Brazil. Appl. Energy 2009, 86, 299–309. [Google Scholar] [CrossRef]
- Finch, D.A.; Bailey, W.G.; McArthur, L.J.B.; Nasitwitwi, M. Photosynthetically active radiation regimes in a southern African savanna environment. Agric. For. Meteorol. 2004, 122, 229–238. [Google Scholar] [CrossRef]
- Jacovides, C.P.; Tymvios, F.S.; Assimakopoulos, V.D.; Kaltsounides, N.A. The dependence of global and diffuse PAR radiation components on sky conditions at Athens, Greece. Agric. For. Meteorol. 2007, 143, 277–287. [Google Scholar] [CrossRef]
- Wang, L.; Gong, W.; Lin, A.; Hu, B. Analysis of photosynthetically active radiation under various sky conditions in Wuhan, Central China. Int. J. Biometeorol. 2014, 58, 1711–1720. [Google Scholar] [CrossRef]
- Udo, S.O.; Aro, T.O. Global PAR related to global solar radiation for central Nigeria. Agric. For. Meteorol. 1999, 97, 21–31. [Google Scholar] [CrossRef]
- Udo, S.O.; Aro, T.O. New empirical relationships for determining global PAR from measurements of global solar radiation, infrared radiation or sunshine duration. Int. J. Climatol. 2000, 20, 1265–1274. [Google Scholar] [CrossRef]
- Jacovides, C.P.; Timvios, F.S.; Papaioannou, G.; Asimakopoulos, D.N.; Theofilou, C.M. Ratio of PAR to broadband solar radiation measured in Cyprus. Agric. For. Meteorol. 2004, 121, 135–140. [Google Scholar] [CrossRef]
- Alados, I.; Olmo, F.J.; Foyo-Moreno, I.; Alados-Arboledas, L. Estimation of photosynthetically active radiation under cloudy conditions. Agric. For. Meteorol. 2000, 102, 39–50. [Google Scholar] [CrossRef]
- Suárez-García, A.; Díez-Mediavilla, M.; Granados-López, D.; González-Peña, D.; Alonso-Tristán, C. Benchmarking of meteorological indices for sky cloudiness classification. Sol. Energy 2020, 195, 499–513. [Google Scholar] [CrossRef]
- Alshaibani, K. Finding frequency distributions of CIE Standard General Skies from sky illuminance or irradiance. Light. Res. Technol. 2011, 43, 487–495. [Google Scholar] [CrossRef]
- Li, D.H.W.; Cheung, G.H.W. Average daylight factor for the 15 CIE standard skies. Light. Res. Technol. 2006, 38, 137–152. [Google Scholar] [CrossRef]
- Li, D.H.W.; Lam, T.N.T.; Cheung, K.L.; Tang, H.L. An analysis of luminous efficacies under the CIE standard skies. Renew. Energy 2008, 33, 2357–2365. [Google Scholar] [CrossRef]
- Li, D.H.W.; Tang, H.L.; Wong, S.L.; Tsang, E.K.W.; Cheung, G.H.W.; Lam, T.N.T. Skies classification using artificial neural networks (ANN) techniques. In Proceedings of the 6th International Conference on Indoor Air Quality, Ventilation and Energy Conservation in Buildings: Sustainable Built Environment, IAQVEC 2007, Sendai, Japan, 28–31 October 2007; pp. 61–68. [Google Scholar]
- Torres, J.L.; de Blas, M.; García, A.; Gracia, A.; de Francisco, A. Sky luminance distribution in Pamplona (Spain) during the summer period. J. Atmos. Sol. Terr. Phys. 2010, 72, 382–388. [Google Scholar] [CrossRef]
- Torres, J.L.; de Blas, M.; García, A.; Gracia, A.; de Francisco, A. Sky luminance distribution in the North of Iberian Peninsula during winter. J. Atmos. Sol. Terr. Phys. 2010, 72, 1147–1154. [Google Scholar] [CrossRef]
- Tregenza, P.R. Analysing sky luminance scans to obtain frequency distributions of CIE Standard General Skies. Light. Res. Technol. 2004, 36, 271–279. [Google Scholar] [CrossRef]
- Li, D.H.W.; Chau, N.T.C.; Wan, K.K.W. Predicting daylight illuminance and solar irradiance on vertical surfaces based on classified standard skies. Energy 2013, 53, 252–258. [Google Scholar] [CrossRef]
- Suárez-García, A.; Granados-López, D.; González-Peña, D.; Díez-Mediavilla, M.; Alonso-Tristán, C. Seasonal caracterization of CIE standard sky types above Burgos, northwestern Spain. Sol. Energy 2018, 169, 24–33. [Google Scholar] [CrossRef]
- Gueymard, C.A.; Ruiz-Arias, J.A. Extensive worldwide validation and climate sensitivity analysis of direct irradiance predictions from 1-min global irradiance. Sol. Energy 2016, 128, 1–30. [Google Scholar] [CrossRef]
- Littlefair, P.J. A comparison of sky luminance models with measured data from Garston, United Kingdom. Sol. Energy 1994, 53, 315–322. [Google Scholar] [CrossRef]
- Littlefair, P.J. The luminance distributions of clear and quasi-clear skies. In Proceedings of the CIBSE National Lighting Conference, Cambridge, UK, 27–30 March 1994; pp. 267–283. [Google Scholar]
- Kittler, R.; Perez, R.; Darula, S. A new generation of sky standards. In Proceedings of the Eighth European Lighting Conference, Amsterdam, The Netherlands, 11–14 May 1997; pp. 359–373. [Google Scholar]
- Proutsos, N.; Liakatas, A.; Alexandris, S. Ratio of photosynthetically active to total incoming radiation above a Mediterranean deciduous oak forest. Theor. Appl. Climatol. 2019, 137, 2927–2939. [Google Scholar] [CrossRef]
- Yu, X.; Guo, X. Hourly photosynthetically active radiation estimation in Midwestern United States from artificial neural networks and conventional regressions models. Int. J. Biometeorol. 2016, 60, 1247–1259. [Google Scholar] [CrossRef] [PubMed]
- Peng, S.; Du, Q.; Lin, A.; Hu, B.; Xiao, K.; Xi, Y. Observation and estimation of photosynthetically active radiation in Lhasa (Tibetan Plateau). Adv. Space Res. 2015, 55, 1604–1612. [Google Scholar] [CrossRef]
- Spiegel, M.R. Estadística, 3rd ed.; Mcgraw-Hill: Mexico city, Mexico, 2002. [Google Scholar]
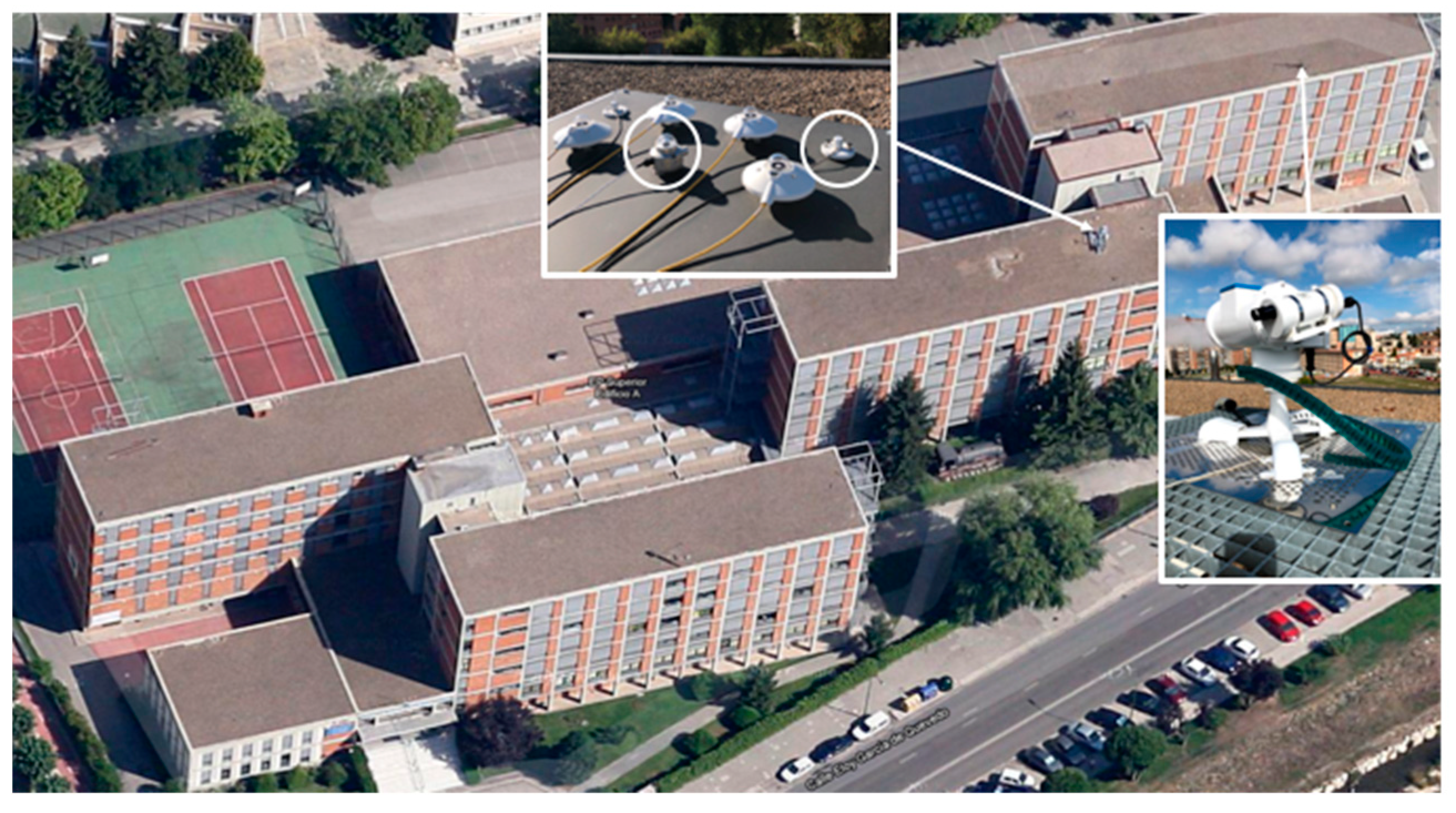





| Model | MS-321LR Sky Scanner |
|---|---|
| Dimensions (W × D × H) | 430 mm × 380 mm × 440 mm |
| Mass | 12.5 kg |
| FOV | 11° |
| Luminance | 0 to 50 kcd/m2 |
| Radiance | 0 to 300 W/m2 |
| A/D Convertor | 16 bits |
| Calibration Error | 2% |
| Model | SR11 |
|---|---|
| ISO classification | first class |
| Spectral range | 300 to 2800 nm |
| Irradiance range | 0 to 2000 W/m2 |
| Sensitivity | 15 × 10−6 V/(Wm−2) |
| Calibration uncertainty | <1.8% |
| Model | ML-020P |
|---|---|
| Measurement Range | 0–3000 μmol·s−1·m−2 |
| Spectral range | 400 to 700 nm |
| Operating temperature | −10 °C to 50 °C |
| Temperature response | ±1% |
| Sensitivity | 0.15 × 10−6 V/μmol·s−1·m−2 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
García-Rodríguez, A.; García-Rodríguez, S.; Díez-Mediavilla, M.; Alonso-Tristán, C. Photosynthetic Active Radiation, Solar Irradiance and the CIE Standard Sky Classification. Appl. Sci. 2020, 10, 8007. https://doi.org/10.3390/app10228007
García-Rodríguez A, García-Rodríguez S, Díez-Mediavilla M, Alonso-Tristán C. Photosynthetic Active Radiation, Solar Irradiance and the CIE Standard Sky Classification. Applied Sciences. 2020; 10(22):8007. https://doi.org/10.3390/app10228007
Chicago/Turabian StyleGarcía-Rodríguez, Ana, Sol García-Rodríguez, Montserrat Díez-Mediavilla, and Cristina Alonso-Tristán. 2020. "Photosynthetic Active Radiation, Solar Irradiance and the CIE Standard Sky Classification" Applied Sciences 10, no. 22: 8007. https://doi.org/10.3390/app10228007
APA StyleGarcía-Rodríguez, A., García-Rodríguez, S., Díez-Mediavilla, M., & Alonso-Tristán, C. (2020). Photosynthetic Active Radiation, Solar Irradiance and the CIE Standard Sky Classification. Applied Sciences, 10(22), 8007. https://doi.org/10.3390/app10228007






