Generating Bessel-Gaussian Beams with Controlled Axial Intensity Distribution
Abstract
1. Introduction
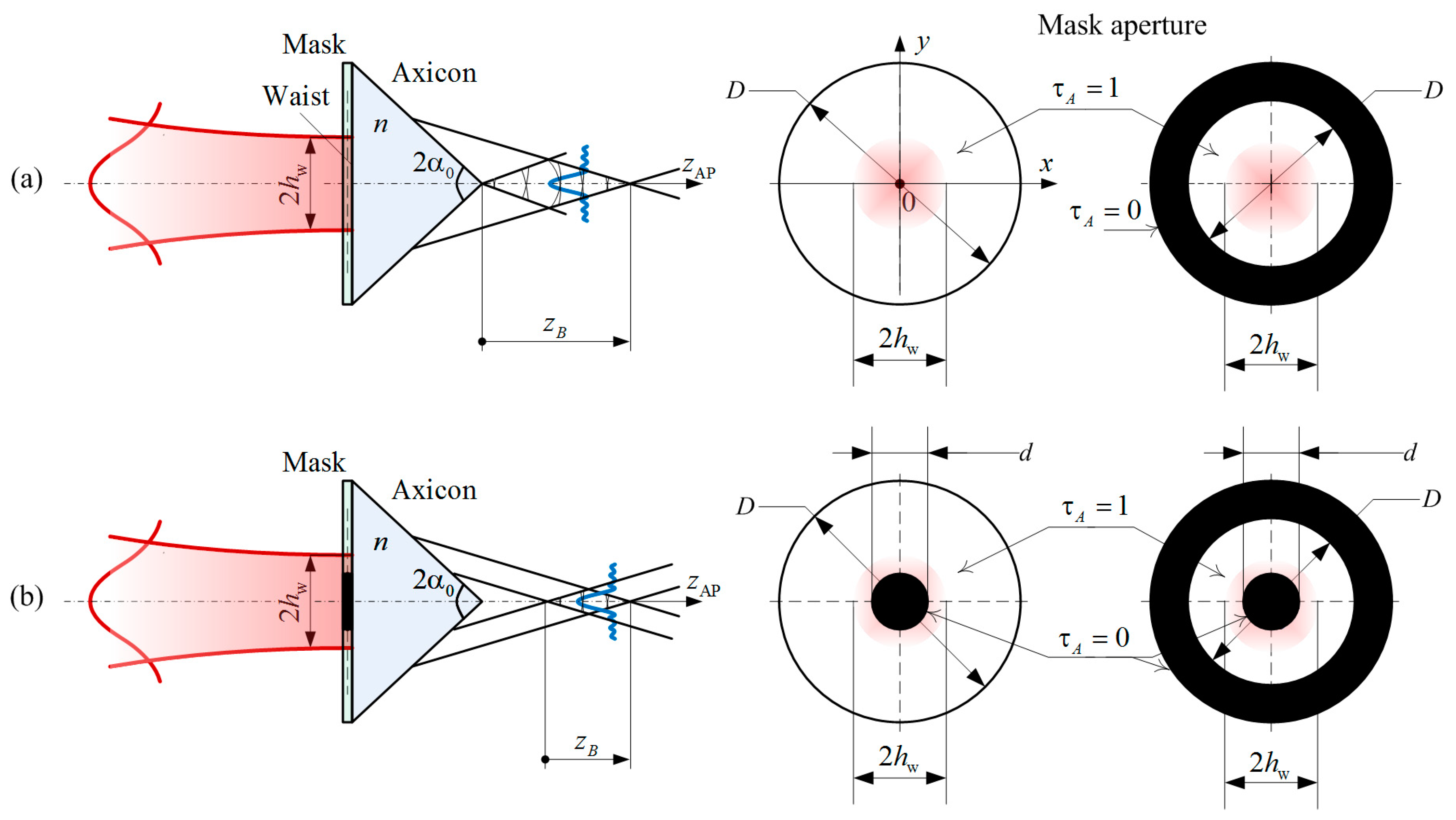
2. Modeling
3. Experimental Implementation of the Bessel-Gaussian Beam
3.1. Experimental Setup
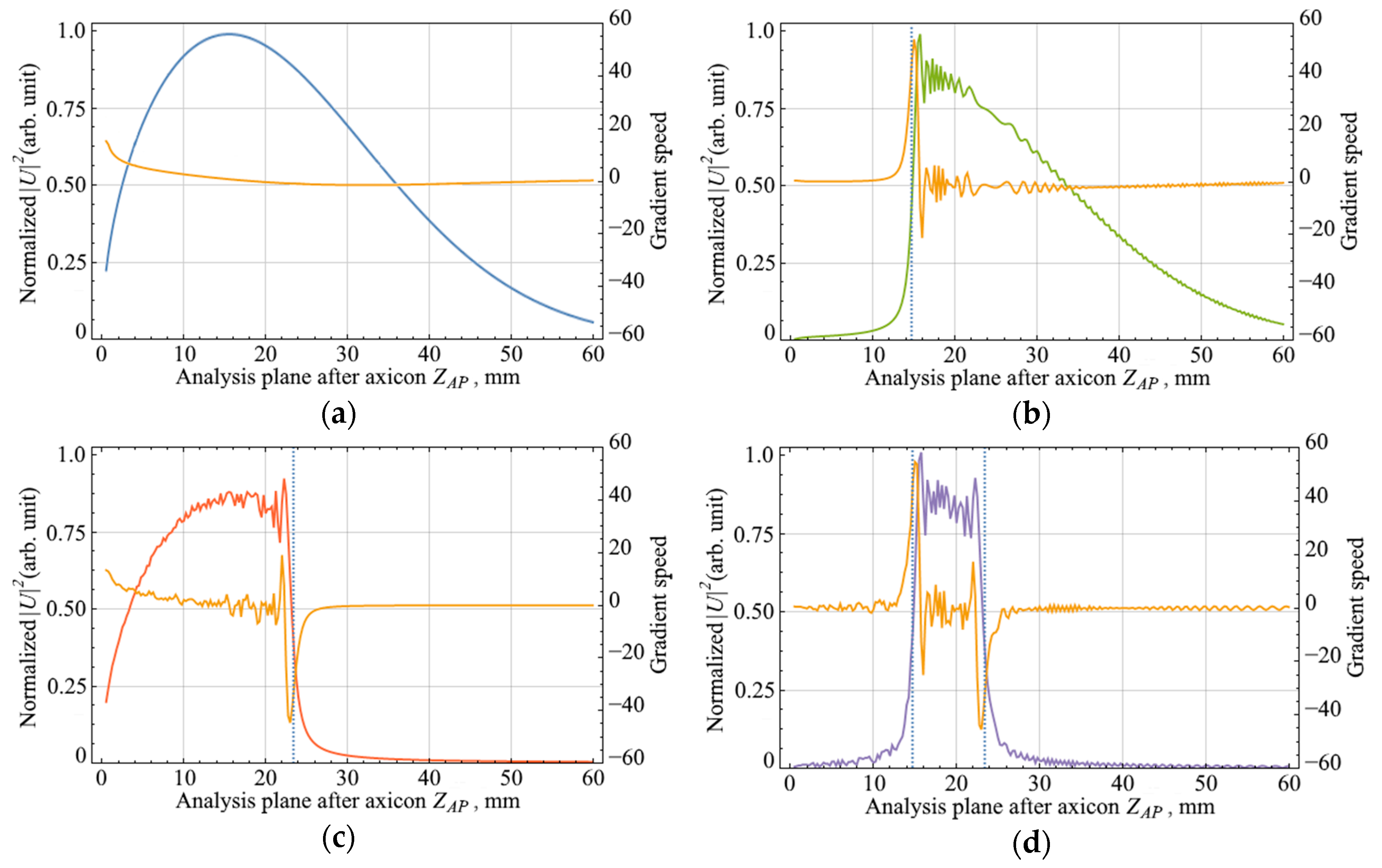
3.2. Results and Analysis
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Saraeva, I.N.; Kudryashov, S.I.; Rudenko, A.A.; Zhilnikova, M.I.; Ivanov, D.S.; Zayarny, D.A.; Simakin, A.V.; Ionin, A.A.; Garcia, M.E. Effect of fs/ps laser pulsewidth on ablation of metals and silicon in air and liquids on their nanoparticle yields. Appl. Surf. Sci. 2019, 470, 1018–1034. [Google Scholar] [CrossRef]
- Galaktionov, I.; Kudryashov, A.; Sheldakova, J.; Nikitina, A. Laser beam focusing through the scattering medium by means of adaptive optics. In SPIE Proceedings Vol. 10073: Adaptive Optics and Wavefront Control for Biological Systems III, Proceedings of the SPIE BiOS, San Francisco, CA, USA, 28–30 January 2017; Bifano, T.G., Kubby, J., Gigan, S., Eds.; SPIE—International Society for Optics and Photonics: Bellingham, WA, USA, 2017. [Google Scholar] [CrossRef]
- Saj, W.M. Light focusing on a stack of metal-insulatormetal waveguides sharp edge. Opt. Express 2009, 17, 13615–13623. [Google Scholar] [CrossRef] [PubMed]
- Stsepuro, N.G.; Krasin, G.K.; Kovalev, M.S.; Pestereva, V.N. Determination of the Point Spread Function of a Computer-Generated Lens Formed by a Phase Light Modulator. Opt. Spectrosc. 2020, 128, 1036–1040. [Google Scholar] [CrossRef]
- Simonov, A.N.; Rombach, M.C. Sharp-focus image restoration from defocused images. Opt. Lett. 2009, 34, 2111–2113. [Google Scholar] [CrossRef] [PubMed]
- Dorn, R.; Quabis, S.; Leuchs, G. Sharper Focus for a Radially Polarized Light Beam. Phys. Rev. Lett. 2003, 91, 233901. [Google Scholar] [CrossRef] [PubMed]
- Nosov, P.A.; Piskunov, D.E.; Shirankov, A.F. Combined laser variosystems paraxial design for longitudinal movement of a Gaussian beam waist. Opt. Express 2020, 28, 5105–5118. [Google Scholar] [CrossRef]
- Galkin, M.L.; Nosov, P.A.; Kovalev, M.S.; Verenikina, N.M. Calculation and analysis of the laser beam field distribution formed by a real optical system. J. Phys. Conf. Ser. 2018, 1096, 012120. [Google Scholar] [CrossRef]
- Miks, A.; Pokorny, P. Fundamental design parameters of two-component optical systems: Theoretical analysis. Appl. Opt. 2020, 59, 1998–2003. [Google Scholar] [CrossRef]
- Mcleod, J.H. The Axicon: A New Type of Optical Element. J. Opt. Soc. Am. 1954, 44, 592–597. [Google Scholar] [CrossRef]
- Yew, E.Y.S.; Sheppard, C.J.R. Tight focusing of radially polarized Gaussian and Bessel-Gauss beams. Opt. Lett. 2007, 32, 3417–3419. [Google Scholar] [CrossRef]
- Kotlyar, V.V.; Stafeev, S.S.; Porfirev, A.P. Tight focusing of an asymmetric Bessel beam. Opt. Commun. 2015, 357, 45–51. [Google Scholar] [CrossRef]
- Summers, A.M.; Yu, X.; Wang, X.; Raoul, M.; Nelson, J.; Todd, D.; Zigo, S.; Lei, S.; Trallero-Herrero, C.A. Spatial characterization of Bessel-like beams for strong-field physics. Opt. Express 2017, 25, 1646–1655. [Google Scholar] [CrossRef]
- Zhang, Z.; Zeng, X.; Miao, Y.; Fan, Y.; Gao, X. Focusing properties of vector Bessel–Gauss beam with multiple-annular phase wavefront. Optik 2018, 157, 240–247. [Google Scholar] [CrossRef]
- Dudutis, J.; Gečys, P.; Račiukaitis, G. Non-ideal axicon-generated Bessel beam application for intra-volume glass modification. Opt. Express 2016, 24, 28433–28443. [Google Scholar] [CrossRef] [PubMed]
- Mcgloin, D.; Dholakia, K. Bessel beams: Diffraction in a new light. Contemp. Phys. 2005, 46, 15–28. [Google Scholar] [CrossRef]
- Herman, R.M.; Wiggins, T.A. Production and uses of diffractionless beams. J. Opt. Soc. Am. A 1991, 8, 932–942. [Google Scholar] [CrossRef]
- Gorelick, S.; Paganin, D.; Korneev, D.; de Marco, A. Hybrid refractive-diffractive axicons for Bessel-beam multiplexing and resolution improvement. Opt. Express 2020, 28, 12174–12188. [Google Scholar] [CrossRef]
- Kharitonov, S.I.; Khonina, S.N.; Volotovskiy, S.G.; Kazanskiy, N.L. Caustics of the vortex beams generated by vortex lenses and vortex axicons. J. Opt. Soc. Am. A 2020, 37, 476–482. [Google Scholar] [CrossRef]
- Fahrbach, F.O.; Rohrbach, A. A line scanned light-sheet microscope with phase shaped self-reconstructing beams. Opt. Express 2010, 18, 24229–24244. [Google Scholar] [CrossRef]
- Chang, B.-J.; Dean, K.M.; Fiolka, R. A systematic and quantitative comparison of lattice and Gaussian light-sheets. Opt. Express 2020, 28, 27052–27077. [Google Scholar] [CrossRef]
- Chang, B.-J.; Kittisopikul, M.; Dean, K.M.; Roudot, P.; Welf, E.S.; Fiolka, R. Universal light-sheet generation with field synthesis. Nat. Methods 2019, 16, 235–238. [Google Scholar] [CrossRef] [PubMed]
- Meinert, T.; Rohrbach, A. Light-sheet microscopy with length-adaptive bessel beams. Biomed. Opt. Express 2019, 10, 670–681. [Google Scholar] [CrossRef] [PubMed]
- Xiong, B.; Han, X.; Wu, J.; Xie, H.; Dai, Q. Improving axial resolution of Bessel beam light-sheet fluorescence microscopy by photobleaching imprinting. Opt. Express 2020, 28, 9464–9476. [Google Scholar] [CrossRef]
- Yu, X.; Zhang, M.; Lei, S. Multiphoton polymerization using femtosecond bessel beam for layerless three-dimensional printing. J. Micro Nanomanufacturing 2017, 6, 010901. [Google Scholar] [CrossRef]
- Stoian, R.; Bhuyan, M.K.; Rudenko, A.; Colombier, J.-P.; Cheng, G. High-resolution material structuring using ultrafast laser non-diffractive beams. Adv. Phys. X 2019, 4, 1659180. [Google Scholar] [CrossRef]
- Chong, A.; Renninger, W.H.; Christodoulides, D.N.; Wise, F.W. Airy-Bessel wave packets as versatile linear light bullets. Nat. Photonics 2010, 4, 103–106. [Google Scholar] [CrossRef]
- Szulzycki, K.; Savaryn, V.; Grulkowski, I. Generation of dynamic Bessel beams and dynamic bottle beams using acousto-optic effect. Opt. Express 2016, 24, 23977–23991. [Google Scholar] [CrossRef]
- Monsoriu, J.A.; Furlan, W.D.; Andrés, P.; Lancis, J. Fractal conical lenses. Opt. Express 2006, 14, 9077–9082. [Google Scholar] [CrossRef]
- Herrmann, J.; Wilhelmi, B. Lasers for Ultrashort Light Pulses; North-Holland: Amsterdam, The Netherlands, 1987. [Google Scholar]
- Ustinov, A.V.; Khonina, S.N. Calculating the Complex Transmission Function of Refractive Axicons. Opt. Mem. Neural Netw. 2012, 21, 133–144. [Google Scholar] [CrossRef]
- Arlt, J.; Dholakia, K.; Soneson, J.; Wright, E.M. Optical dipole traps and atomic waveguides based on Bessel light beams. Phys. Rev. A 2001, 63, 063602. [Google Scholar] [CrossRef]
- Brzobohatý, O.; Čižmár, T.; Zemánek, P. High quality quasi-Bessel beam generated by round-tip axicon. Opt. Express 2008, 16, 12688–12700. [Google Scholar] [CrossRef] [PubMed]
- Khonina, S.N.; Ustinov, A.V.; Kovalev, A.A.; Volotovsky, S.G. Propagation of the radially-limited vortical beam in a near zone. Part I. Calculation algorithms. Comput. Opt. 2010, 34, 315–329. [Google Scholar]
- Khonina, S.N.; Ustinov, A.V.; Kovalev, A.A.; Volotovsky, S.G. Propagation of the radially-limited vortical beam in a near zone. Part II. Results of simulation. Comput. Opt. 2010, 34, 330–339. [Google Scholar]
- Levin, D. Procedures for computing one and two-dimensional integrals of functions with rapid irregular oscillations. Math. Comput. 1982, 38, 531–538. [Google Scholar] [CrossRef]
- Itô, K. Encyclopedic Dictionary of Mathematics; MIT Press: Cambridge, MA, USA, 1980; p. 1139. [Google Scholar]
- Kaplan, W. The Gradient Field; Addison-Wesley: Reading, MA, USA, 1991; pp. 183–185. [Google Scholar]
- Morse, P.M.; Feshbach, H. The Gradient. In Methods of Theoretical Physics, Part I; McGraw-Hill: New York, NY, USA, 1953; pp. 31–32. [Google Scholar]




Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Stsepuro, N.; Nosov, P.; Galkin, M.; Krasin, G.; Kovalev, M.; Kudryashov, S. Generating Bessel-Gaussian Beams with Controlled Axial Intensity Distribution. Appl. Sci. 2020, 10, 7911. https://doi.org/10.3390/app10217911
Stsepuro N, Nosov P, Galkin M, Krasin G, Kovalev M, Kudryashov S. Generating Bessel-Gaussian Beams with Controlled Axial Intensity Distribution. Applied Sciences. 2020; 10(21):7911. https://doi.org/10.3390/app10217911
Chicago/Turabian StyleStsepuro, Nikita, Pavel Nosov, Maxim Galkin, George Krasin, Michael Kovalev, and Sergey Kudryashov. 2020. "Generating Bessel-Gaussian Beams with Controlled Axial Intensity Distribution" Applied Sciences 10, no. 21: 7911. https://doi.org/10.3390/app10217911
APA StyleStsepuro, N., Nosov, P., Galkin, M., Krasin, G., Kovalev, M., & Kudryashov, S. (2020). Generating Bessel-Gaussian Beams with Controlled Axial Intensity Distribution. Applied Sciences, 10(21), 7911. https://doi.org/10.3390/app10217911






