Effect of Solid Particles on Droplet Size Applying the Time-Shift Method for Spray Investigation
Abstract
1. Introduction
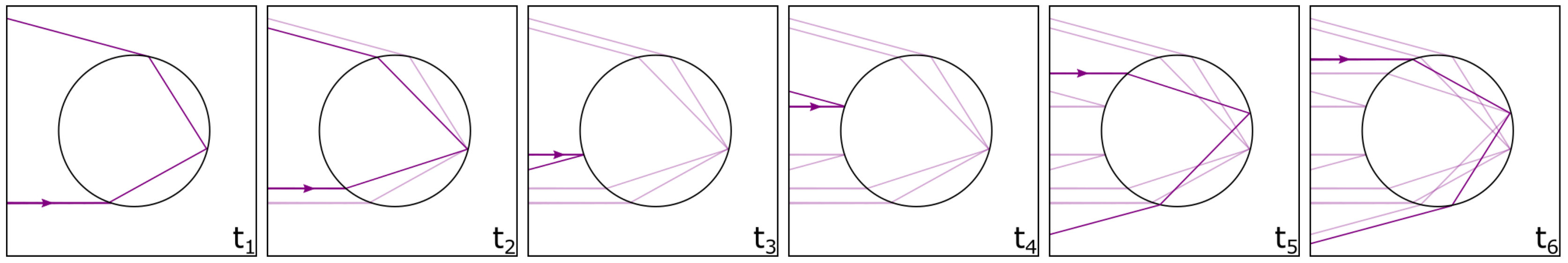
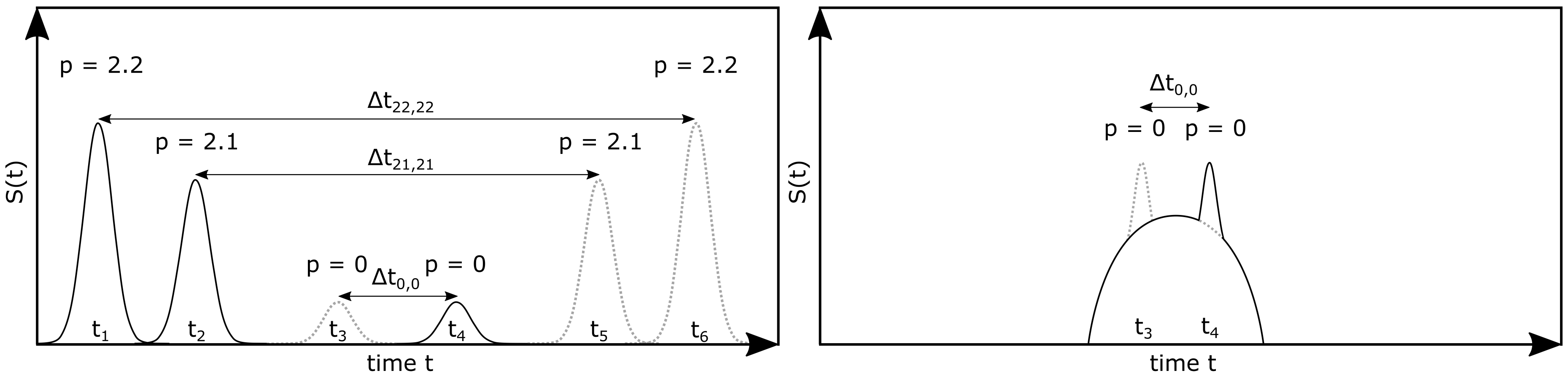
2. Theoretical Background
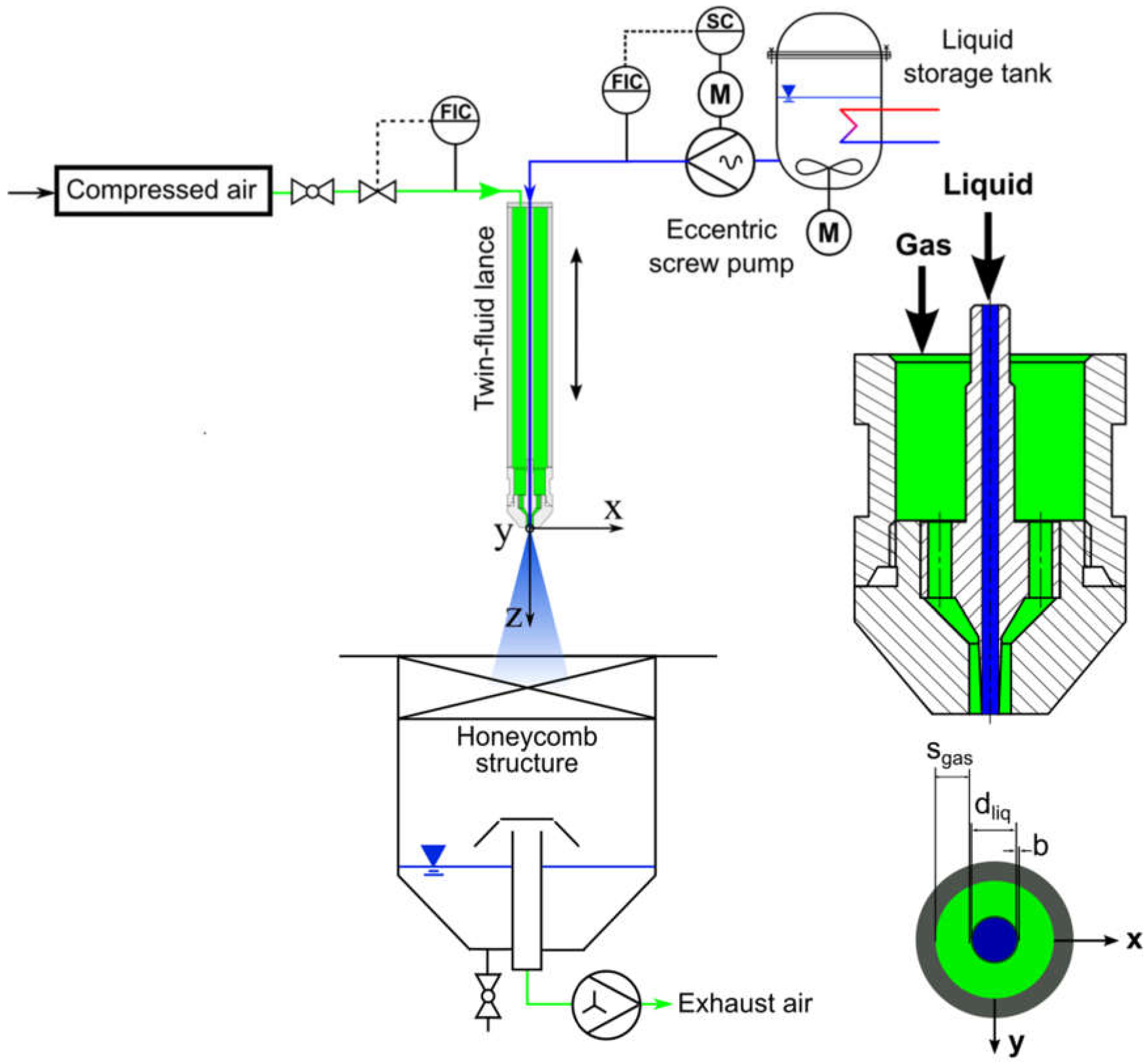
3. Experimental Setup and Measurement Techniques
4. Results and Discussion
4.1. Rheological Characterization
4.2. Spray Characterization
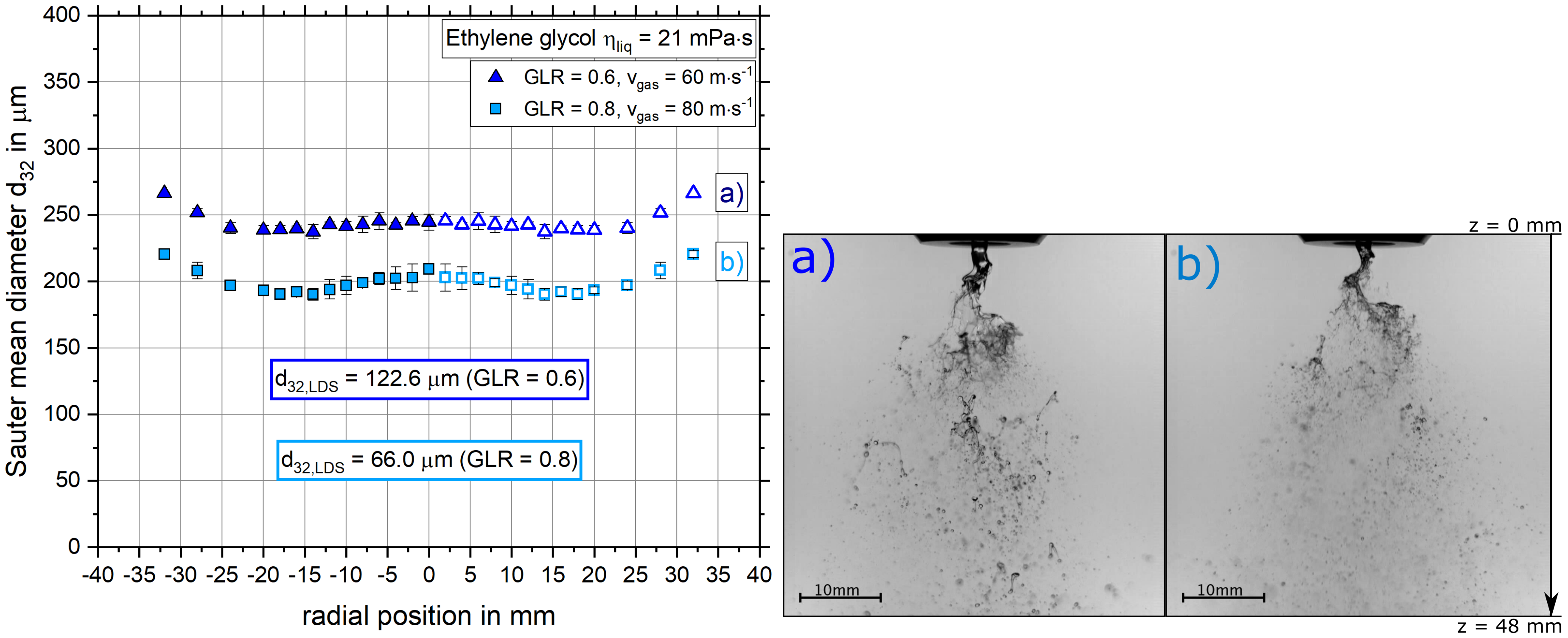
4.2.1. The Influence of Gas Velocity on the Radial Sauter Mean Diameter Distribution and Primary Breakup
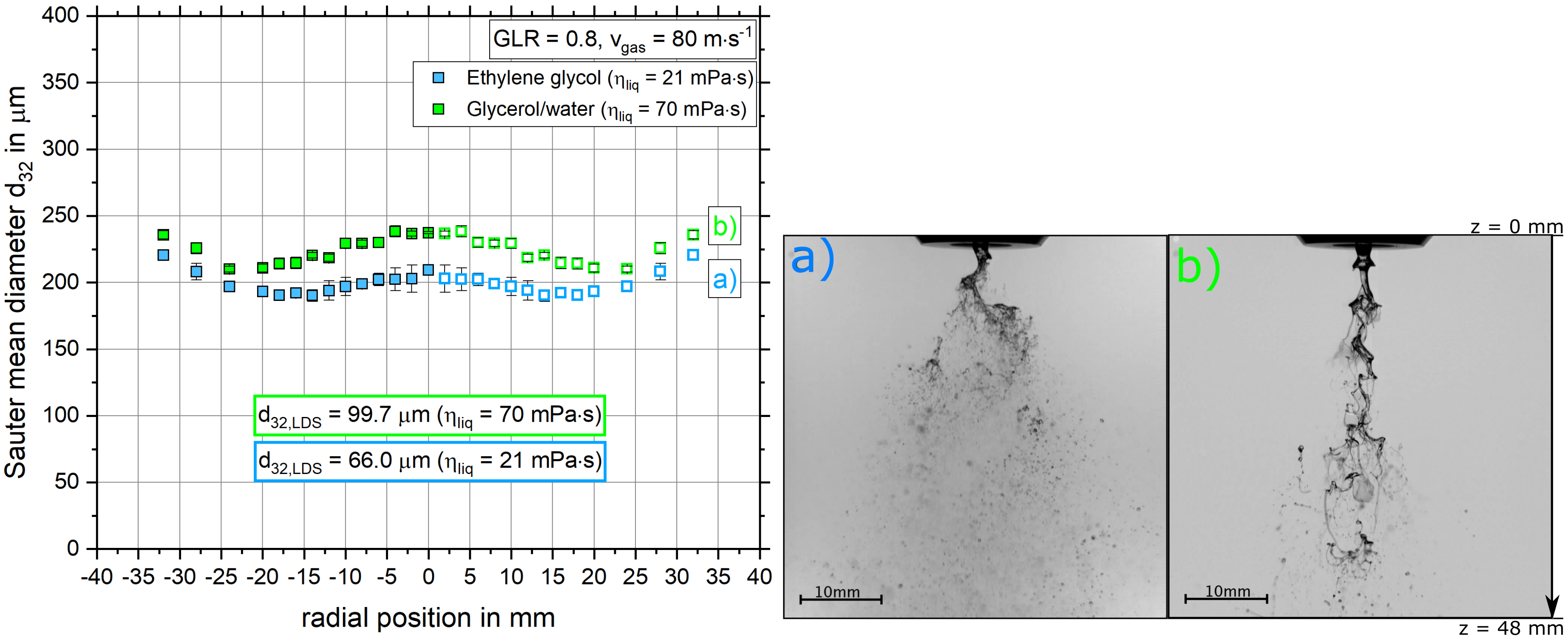
4.2.2. The Influence of Dynamic Viscosity on the Sauter Mean Diameter Distribution and Primary Breakup
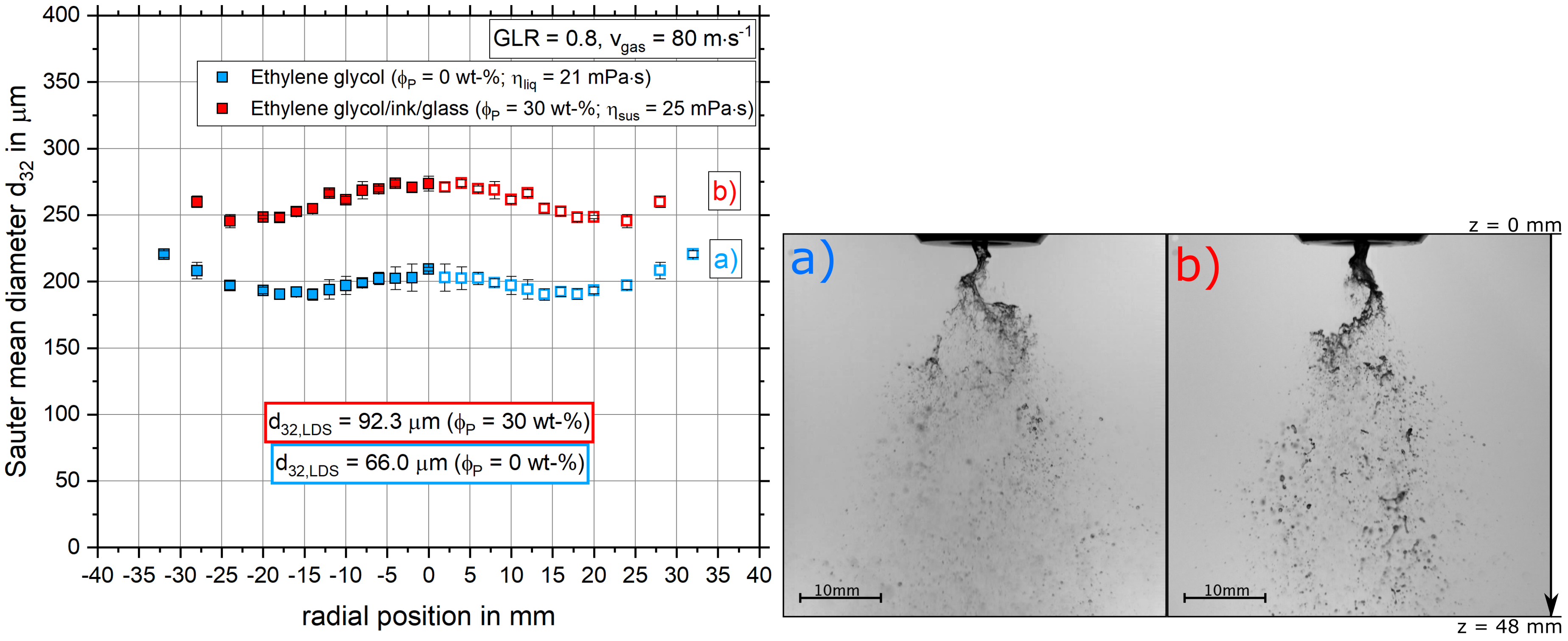
4.2.3. The Influence of Varying Particle Fractions on the Sauter Mean Diameter Distribution and Primary Breakup
4.3. Conclusions
- Newtonian flow behavior of the suspensions was achieved by suspending round glass beads of a specific size but different mass fraction in Newtonian liquids. For each suspension, a pure liquid with same viscosity was additionally used as reference fluid, which allows for the separation of the effect of the glass mass fraction and dynamic viscosity on atomization.
- A new measurement technique that applies the time-shift method for local measurement of droplet size and velocity in opaque suspension sprays was successfully used. The following effects were observed:
- -
- With increasing gas velocity, the radial Sauter mean diameter distribution decreased.
- -
- With increasing dynamic viscosity, the radial Sauter mean diameter distribution increased.
- -
- With increasing solid mass fraction, an increasing droplet diameter was detected. This effect can be explained by the theory of tensile strength and a force balance.
- The primary breakup analysis of the viscous liquids and suspensions was performed with a high-speed camera. The primary breakup instability varied as a function of the dynamic viscosity, whereas a change in the solid mass fraction led to the same instability mode.
- The dependencies of droplet size on gas velocity, dynamic viscosity and solid mass fraction revealed by the SpraySpy® system were confirmed by a commonly applied laser diffraction system.
Author Contributions
Funding
Conflicts of Interest
Abbreviations
| ATMO | Atmospheric Spray Test Rig |
| CWS | Coal-water Slurry |
| EFG | Entrained Flow Gasification |
| GLR | Gas-to-Liquid Ratio |
| LDS | Laser Diffraction System |
| LED | Light-emitting Diode |
References
- Andreussi, P.; Tognotti, L.; Graziadio, M.; de Michele, G. Atomization of Coal-Water Fuels by a Pneumatic Nozzle: Characteristics of the Spray. Aerosol Sci. Technol. 1990, 13, 35–46. [Google Scholar] [CrossRef]
- Hai-long, Y.; Chao, Z.; Jian-zhong, L.; Ke-fa, C. Experimental study of the atomizing performance of a new type of nozzle for coal water slurry. Energy Fuels 2008, 22, 1170–1173. [Google Scholar] [CrossRef]
- Smith, C.F.; Sojka, P.E.; Lefebvre, A.H. Plain-Jet Airblast Atomization of Coal-Water Slurry Fuels; SAE International: Warrendale, PA, USA, 1985. [Google Scholar]
- Tsai, S.C.; Vu, T. Atomization of coal-water slurry using twin-fluid jet atomizer. Fuel 1987, 66, 1596–1602. [Google Scholar] [CrossRef]
- Ohene, F.; Bratton, T.; Rhone, Y. Viscoelastic Behavior of Beneficated Coal-Water Slurries and Their Atomization Characteristics; Grambling State University: Grambling, LA, USA, 1996. [Google Scholar]
- Mayer, W. Coaxial atomization of a round liquid jet in a high speed gas stream: A phenomenological study. Exp. Fluids 1994, 16, 401–410. [Google Scholar] [CrossRef]
- Leroux, B.; Delabroy, O.; Lacas, F. Experimental study of coaxial atomizers scaling. Part 2: Diluted zone. Atom. Sprays 2007, 17, 409–430. [Google Scholar] [CrossRef]
- Dumouchel, C. On the experimental investigation on primary atomization of liquid streams. Exp. Fluids 2008, 45, 371–422. [Google Scholar] [CrossRef]
- Hede, P.D.; Bach, P.; Jensen, A.D. Two-fluid spray atomisation and pneumatic nozzles for fluid bed coating/agglomeration purposes: A review. Chem. Eng. Sci. 2008, 63, 3821–3842. [Google Scholar] [CrossRef]
- Chigier, N.; Faragó, Z. Morphological Classification of Disintegration of Round Liquid Jets in a Coaxial Air Stream. Atom. Sprays 1992, 2, 137–153. [Google Scholar] [CrossRef]
- Lasheras, J.C.; Hopfinger, E.J. Liquid Jet Instability and Atomization in a Coaxial Gas Stream. Annu. Rev. Fluid Mech. 2000, 32, 275–308. [Google Scholar] [CrossRef]
- Wachter, S.; Jakobs, T.; Kolb, T. Experimental investigation on the influence of system pressure on resulting spray quality and jet breakup applying pressure adapted twin-fluid nozzles. Int. J. Multi. Flow 2020, 125, 103189. [Google Scholar] [CrossRef]
- Li, L.K.B.; Dressler, D.M.; Green, S.I.; Davy, M.H. Experiments on air-blast atomization of viscoelastic liquids, Part 1: Quiescent conditions. Atom. Sprays 2009, 19, 157–190. [Google Scholar] [CrossRef]
- Sänger, A.D. Zerstäubung hochviskoser Fluide bei variierendem Systemdruck—Grundlagenforschung zur Hochdruck-Flugstromvergasung. Ph.D. Thesis, Karlsruher Institut für Technologie (KIT), Karlsruhe, Germany, 2018. [Google Scholar]
- Muller, F.L.; Davidson, J.F. Rheology of Shear Thinning Polymer Solutions. Indust. Eng. Chem. Res. 1994, 33, 2364–2367. [Google Scholar] [CrossRef]
- Mansour, A.; Chigier, N. Air-blast atomization of non-newtonian liquids. J. Non-Newton. Fluid Mech. 1995, 58, 161–194. [Google Scholar] [CrossRef]
- Aliseda, A.; Hopfinger, E.J.; Lasheras, J.C.; Kremer, D.M.; Berchielli, A.; Connolly, E.K. Atomization of viscous and non-newtonian liquids by a coaxial, high-speed gas jet. Experiments and droplet size modeling. Inter. J. Multiphase Flow 2008, 34, 161–175. [Google Scholar] [CrossRef]
- Gillberg, L.; Larsson, N.; Mathiesen, M.; Nyström, O.; Persson, J.E. Some Rheological Data and Atomization Behaviour of CWMs Containing 68 to 89 Percent Coal. In Proceedings of the International Symposium on Coal Slurry Combustion and Technology, Tampa, FL, USA, 25–27 April 1983; pp. 1229–1243. [Google Scholar]
- Ghezelchi, M.H.; Garcia-Perez, M.; Wu, H. Bioslurry as a Fuel. 7: Spray Characteristics of Bio-Oil and Bioslurry via Impact and Twin-Fluid Atomizers. Energy Fuels 2015, 29, 8058–8065. [Google Scholar] [CrossRef]
- Zhao, H.; Liu, H.F.; Xu, J.L.; Li, W.F.; Cheng, W. Breakup and atomization of a round coal water slurry jet by an annular air jet. Chem. Eng. Sci. 2012, 78, 63–74. [Google Scholar] [CrossRef]
- Cronin, L.; Sojka, P.E.; Lefebvre, A.H. The Effect of Fuel Film Thickness on Coal Water Slurry Atomization; SAE Transactions: Warrendale, PA, USA, 1985; pp. 577–584. [Google Scholar]
- Smith, C.F.; Sojka, P.E.; Thames, J.M. The influence of fluid physical properties on coal-water slurry atomization. J. Eng. Gas Turbines Power 1990, 112, 15–20. [Google Scholar] [CrossRef]
- Mulhem, B.; Fritsching, U.; Schulte, G.; Bauckhage, K. Atomization of suspension in twin-fluid atomizer. In Proceedings of the PARTEC 2001—International Congress for Particle Technology, Nuremberg, Germany, 27–29 March 2001. [Google Scholar]
- Mulhem, B.; Fritsching, U.; Schulte, G.; Bauckhage, K. Effect of solid particle characteristics on suspension atomization. At. Sprays 2003, 13, 321–343. [Google Scholar] [CrossRef]
- Hinze, J.O. Fundamentals of the Hydrodynamic Mechanism of Splitting in Dispersion Dropl. AIChE J. 1955, 1, 289–295. [Google Scholar] [CrossRef]
- Mulhem, B.; Schulte, G.; Fritsching, U. Solid–liquid separation in suspension atomization. Chem. Eng. Sci. 2006, 61, 2582–2589. [Google Scholar] [CrossRef]
- Capes, C. Handbook of Powder Technology: Particle Size Enlargement; Elsevier: Amsterdam, The Netherlands, 1980. [Google Scholar]
- Zhao, H.; Liu, H.F.; Tian, X.S.; Xu, J.L.; Li, W.F.; Lin, K.F. Outer ligament-mediated spray formation of annular liquid sheet by an inner round air stream. Exp. Fluids 2014, 55, 27. [Google Scholar] [CrossRef]
- Jampolski, L.; Sänger, A.; Jakobs, T.; Guthausen, G.; Kolb, T.; Willenbacher, N. Improving the processability of coke water slurries for entrained flow gasification. Fuel 2016, 185, 102–111. [Google Scholar] [CrossRef]
- Chaussonnet, G.; Lieber, C.; Yikang, Y.; Gu, W.; Bartschat, A.; Reischl, M.; Koch, R.; Mikut, R.; Bauer, H.J. Towards DeepSpray: Using Convolutional Neural Network to Post-Process Shadowgraphy Images of Liquid Atomization. arXiv 2019, arXiv:1910.11073. [Google Scholar]
- Schäfer, W.; Rosenkranz, S.; Tropea, C. Validation of the Time-Shift Technique for Spray Characterization. In Proceedings of the ILASS Americas 27th Annual Conference on Liquid Atomization and Spray Systems, Raleigh, NC, USA, 17–20 May 2015. [Google Scholar]
- Tian, X.S.; Zhao, H.; Liu, H.F.; Li, W.F.; Xu, J.L. Effect of central tube thickness on wave frequency of coaxial liquid jet. Fuel Process. Technol. 2014, 119, 190–197. [Google Scholar] [CrossRef]
- Anton Paar GmbH. Available online: https://www.anton-paar.com/corp-de/ (accessed on 6 October 2020).
- Searle, G. A simple viscometer for very viscous liquids. Proc. Cambridge Philos. Soc. 1912. [Google Scholar]
- KRÜSS GmbH. Available online: https://www.kruess.com/ (accessed on 6 October 2020).
- Du Noüy, P.L. An interfacial tensiometer for universal use. J. Gen. Phys. 1925, 7, 625–631. [Google Scholar] [CrossRef]
- Kihm, K.; Son, S. Effect of coal particle size on coal-water-slurry (CWS) atomization. Atom. Sprays 1998, 8, 503–519. [Google Scholar]
- Wachter, S.; Jakobs, T.; Kolb, T. Comparison of spray quality for two different flow configurations: Central liquid jet versus annular liquid sheet. In Proceedings of the 29th European Conference on Liquid Atomization and Spray Systems, Paris, France, 2–4 September 2019. [Google Scholar]
- Damaschke, N.; Nobach, H.; Semidetnov, N.; Tropea, C. Optical particle sizing in backscatter. Appl. Opt. 2002, 41, 5713. [Google Scholar] [CrossRef][Green Version]
- Schäfer, W.; Tropea, C. Time-shift technique for simultaneous measurement of size, velocity, and relative refractive index of transparent droplets or particles in a flow. Appl. Opt. 2014, 53, 588–597. [Google Scholar] [CrossRef]
- Li, L.; Stegmann, P.G.; Rosenkranz, S.; Schäfer, W.; Tropea, C. Simulation of light scattering from a colloidal droplet using a polarized Monte Carlo method: Application to the time-shift technique. Opt. Exp. 2019, 27, 36388. [Google Scholar] [CrossRef]
- Malvern Panalytical GmbH. Available online: https://www.malvernpanalytical.com/de/ (accessed on 6 October 2020).
- Dodge, L.G.; Biaglow, J.A. Effect of elevated temperature and pressure on sprays from simplex swirl atomizers. J. Eng. Gas Turbines Power 1986, 108, 209–215. [Google Scholar] [CrossRef]
- Sigmund Lindner GmbH. Available online: https://www.sigmund-lindner.com/ (accessed on 6 October 2020).
- Lefebvre, A. Twin-fluid atomization: Factors influencing mean drop size. Atom. Sprays 1992, 2, 101–119. [Google Scholar] [CrossRef]
- Rizk, N.; Lefebvre, A. Spray characteristics of plain-jet airblast atomizers. In Proceedings of the ASME 1983 International Gas Turbine Conference and Exhibit, Phoenix, AZ, USA, 27–31 March 1983; p. 634. [Google Scholar]
- Cossali, E.; Hardalupas, Y. Comparison between laser diffraction and phase Doppler velocimeter techniques in high turbidity, small diameter sprays. Exp. Fluids 1992, 13. [Google Scholar] [CrossRef]
- Zaidi, S.H.; Altunbas, A.; Azzopardi, B.J. A comparative study of phase Doppler and laser diffraction techniques to investigate drop sizes in annular two-phase flow. Chem. Eng. J. 1998, 71, 135–143. [Google Scholar] [CrossRef]
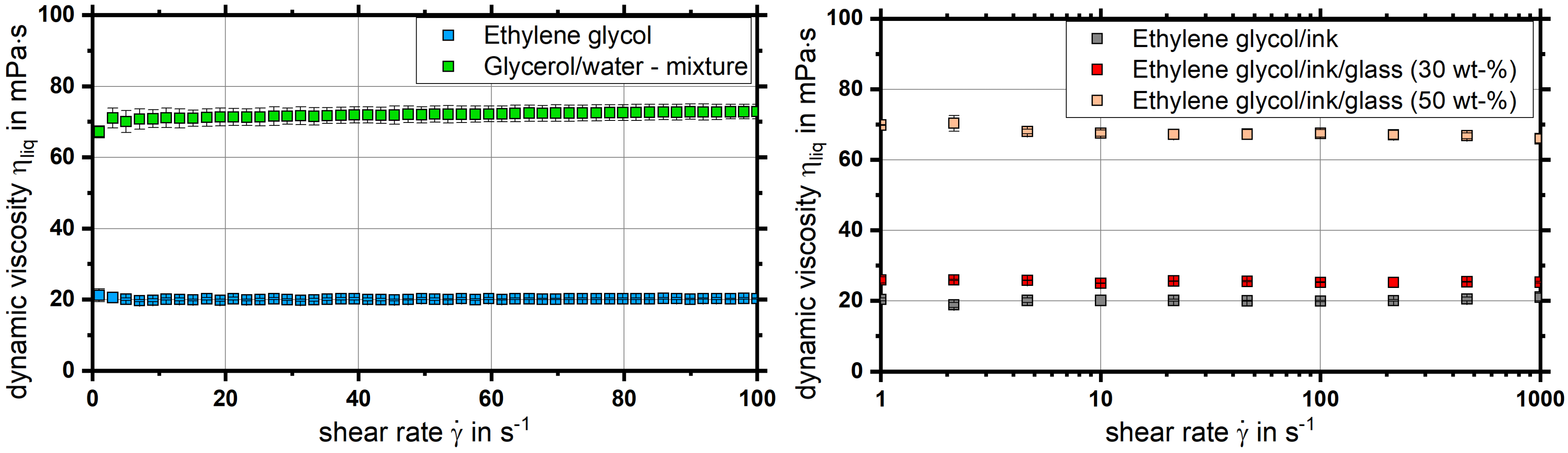

| Glycol | Glycerol/Water | Glycol/Ink/Glass | Glycol/Ink/Glass | |
|---|---|---|---|---|
| in wt−% | 0 | 0 | 30 | 50 |
| in kg·m | 1110 | 1215 | 1332 | 1537 |
| in mPa·s | 21 | 70 | 25 | 68 |
| in mN·m | 48 | 65 | 48 | 48 |
| in − | 1.432 | 1.445 | 1.432 | 1.432 |
| A in − | 0 | 0 | 0 | 0 |
| 0.064 | 0.176 | 0.070 | 0.178 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wachter, S.; Jakobs, T.; Kolb, T. Effect of Solid Particles on Droplet Size Applying the Time-Shift Method for Spray Investigation. Appl. Sci. 2020, 10, 7615. https://doi.org/10.3390/app10217615
Wachter S, Jakobs T, Kolb T. Effect of Solid Particles on Droplet Size Applying the Time-Shift Method for Spray Investigation. Applied Sciences. 2020; 10(21):7615. https://doi.org/10.3390/app10217615
Chicago/Turabian StyleWachter, Simon, Tobias Jakobs, and Thomas Kolb. 2020. "Effect of Solid Particles on Droplet Size Applying the Time-Shift Method for Spray Investigation" Applied Sciences 10, no. 21: 7615. https://doi.org/10.3390/app10217615
APA StyleWachter, S., Jakobs, T., & Kolb, T. (2020). Effect of Solid Particles on Droplet Size Applying the Time-Shift Method for Spray Investigation. Applied Sciences, 10(21), 7615. https://doi.org/10.3390/app10217615






