Regression Equations for Estimating Landslide-Triggering Factors Using Soil Characteristics
Abstract
1. Introduction
2. Methodology
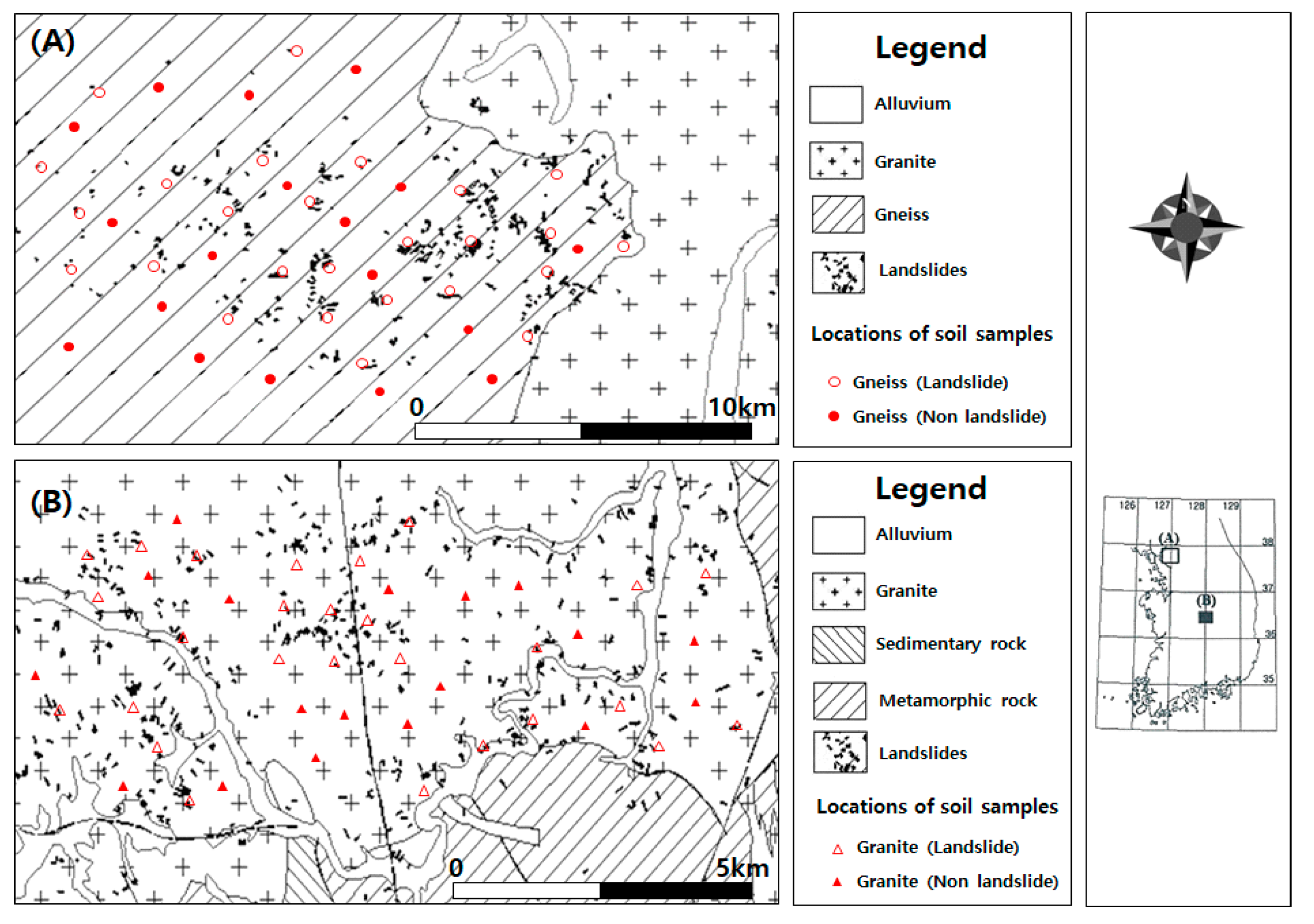
2.1. Soil Properties of Landslide Areas
2.2. Estimation of Landslide-Triggering Factors Using Soil Properties
2.3. Statistical Analysis Using SPSS
3. Results
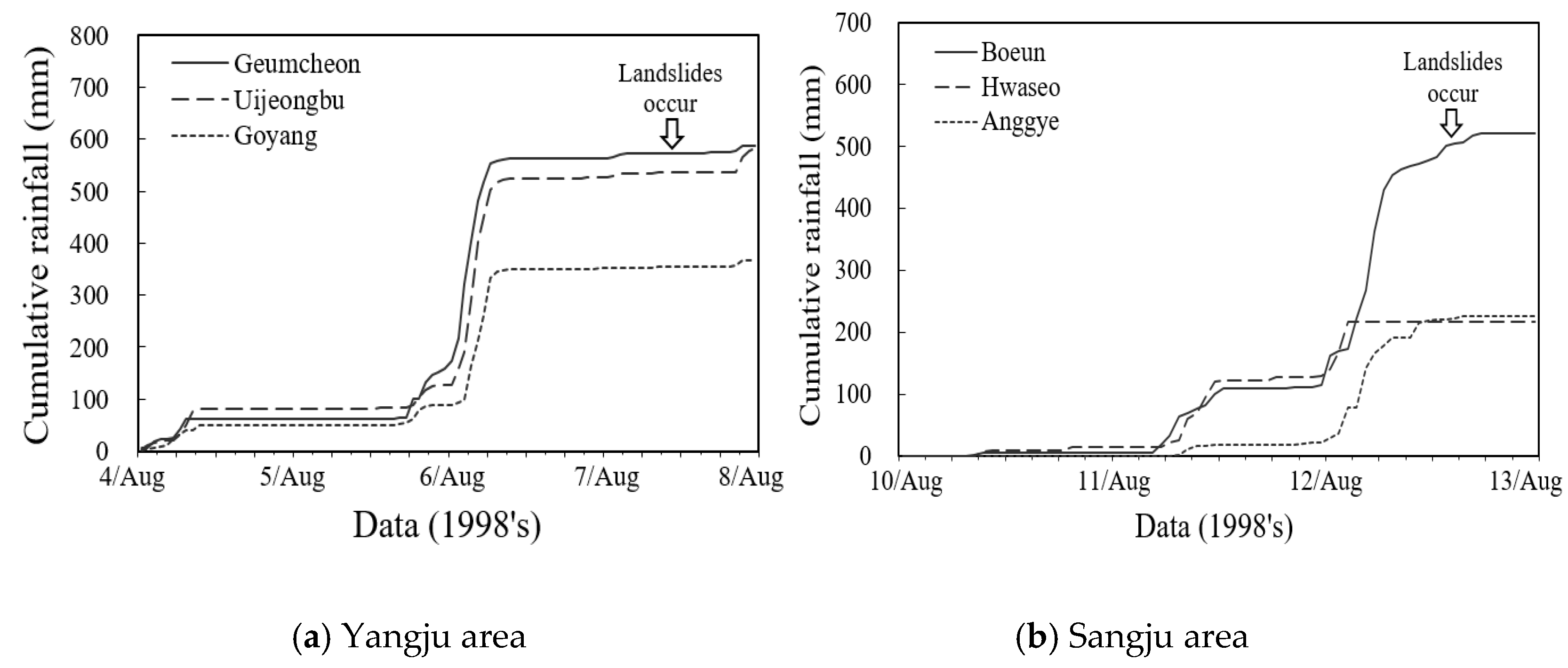
3.1. Rainfall Events
3.2. Landslide Pattern
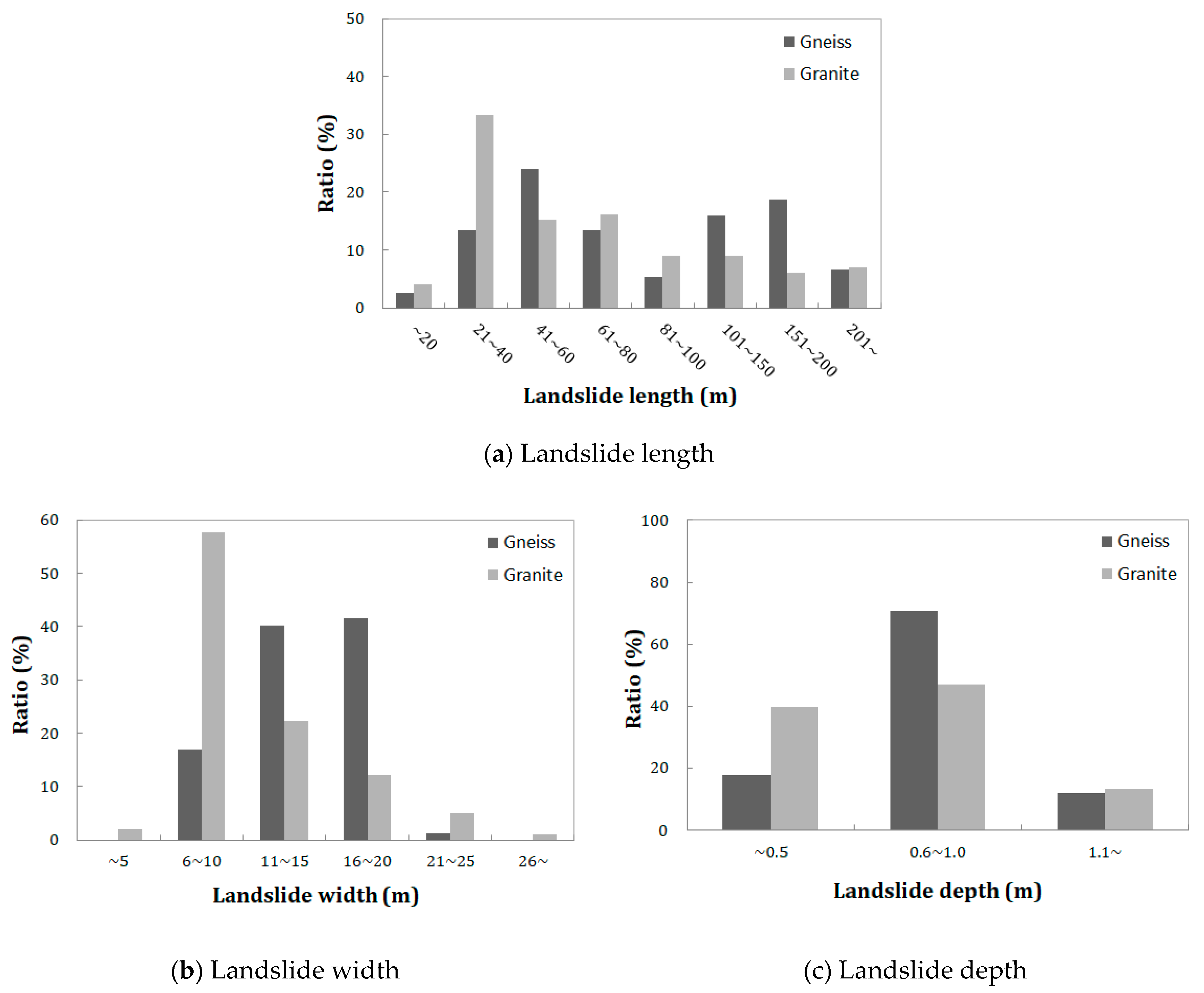
3.3. Landslide Scales
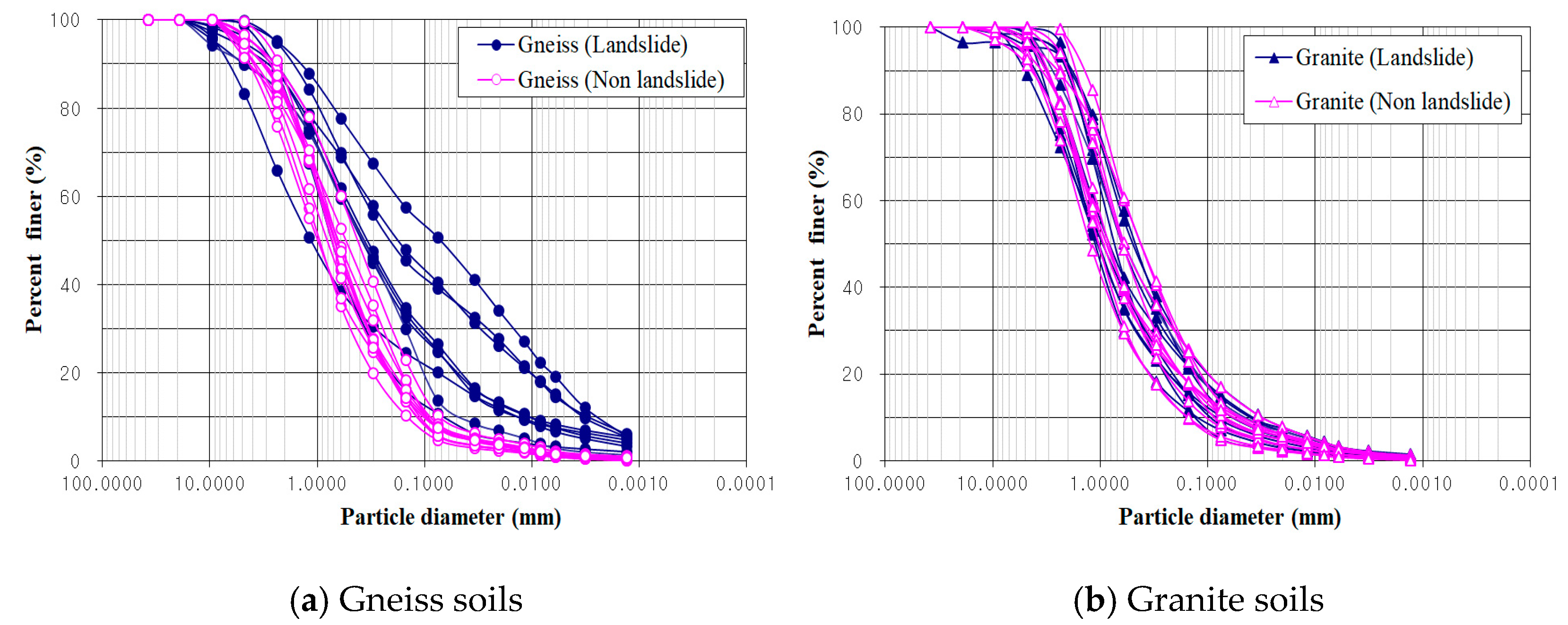
3.4. Soil Properties of Landslide and Non-Landslide Areas
3.5. Derivation of Regression Equations for Evaluating Landslide-Triggering Factors
3.5.1. Regression Equations of Permeability Coefficient
3.5.2. Regression Equations of Shear Strength
4. Discussion
4.1. Validation of Permeability Coefficients
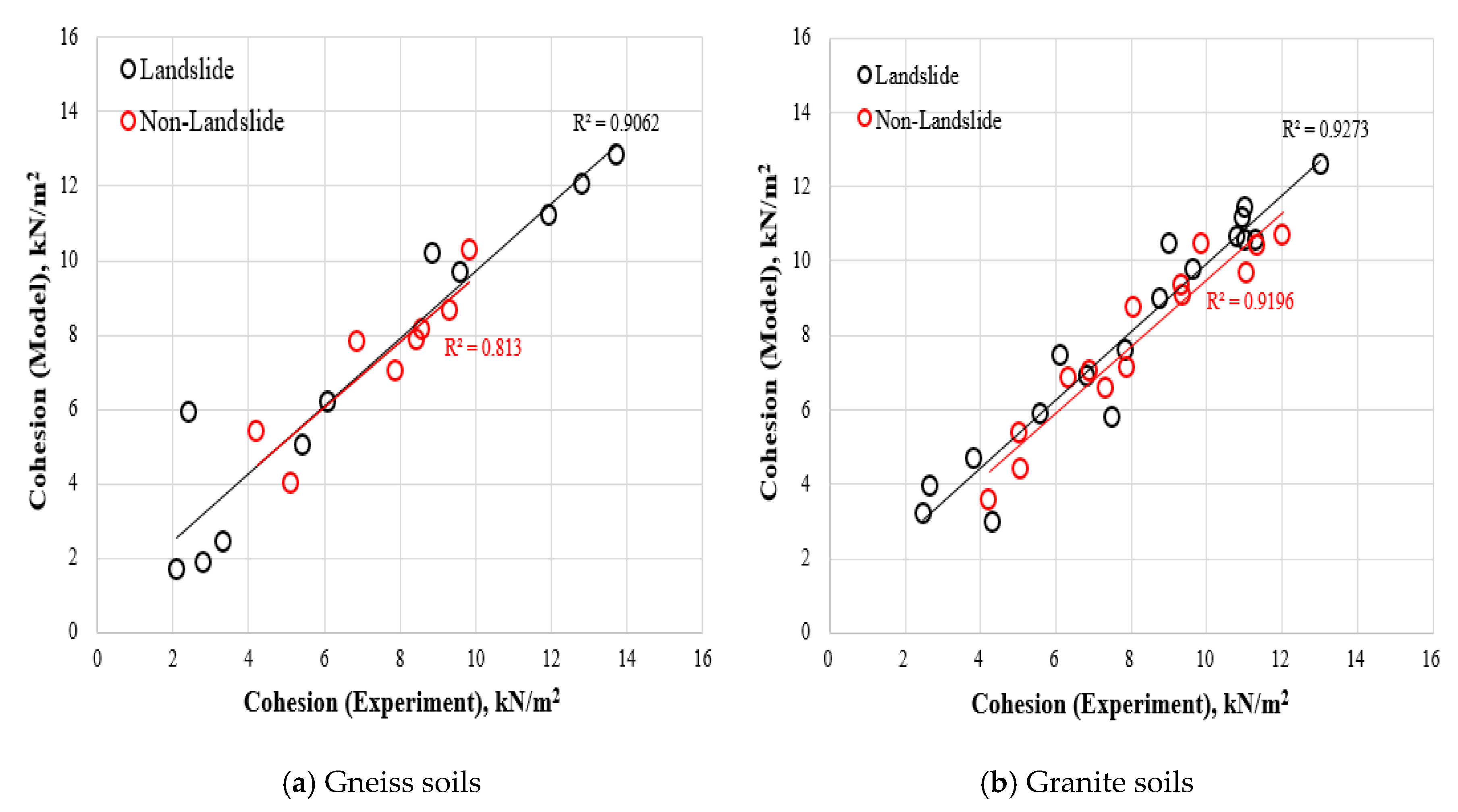
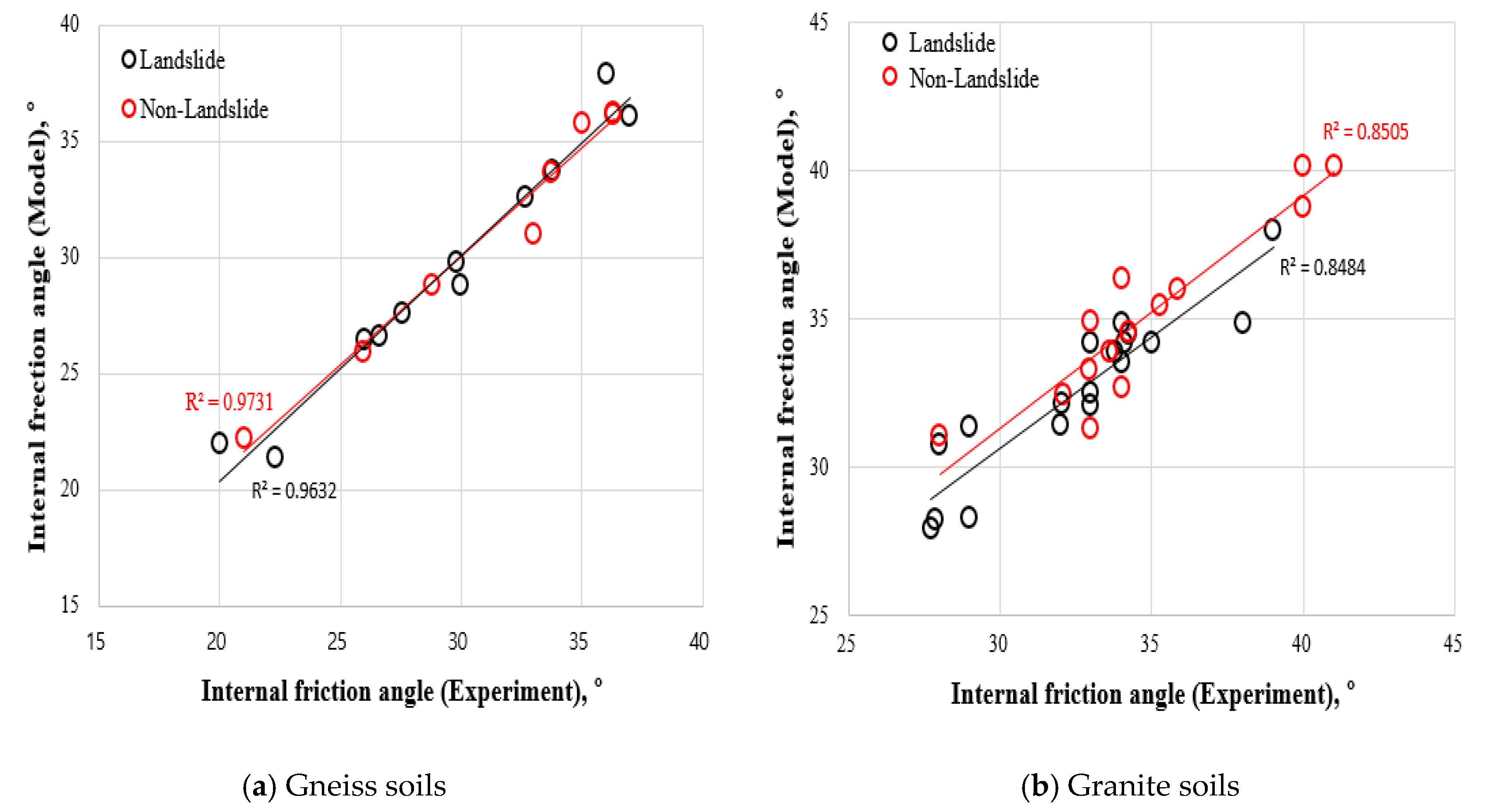
4.2. Validation of Shear Strengths
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Oh, S.; Lee, G.; Bae, W. Estimation of landslide risk based on infinity flow direction. J. Korean Geo-Environ. Soc. 2019, 20, 5–18, (In Korean with English Abstract). [Google Scholar]
- Seoul Metropolitan Government (SMG). The 1st final inspection report on causes and restoration works of the 2011 Umyeonsan landslides. In Korean Geotechnical Society; Seoul Metropolitan Government: Seoul, Korea, 2011; 262p. [Google Scholar]
- Lee, S.-G.; Winter, M.G. The effects of debris flow in the Republic of Korea and some issues for successful risk reduction. Eng. Geol. 2019, 251, 172–189. [Google Scholar] [CrossRef]
- Nam, D.H.; Kim, M.-I.; Kang, D.-H.; Kim, B.S. Debris flow damage assessment by considering debris flow direction and direction angle of structure in South Korea. Water 2019, 11, 328. [Google Scholar] [CrossRef]
- Mathewson, C.C.; Keaton, J.R.; Santi, P.M. Role of bedrock ground water in the initiation of debris flows and sustained post flow stream discharge. Bull. Assoc. Eng. Geol. 1990, 27, 73–83. [Google Scholar] [CrossRef]
- Sitar, N.; Anderson, S.A.; Johnson, K.A. Conditions leading to the initiation of rainfall-induced debris flows. In Proceedings of the Geotechnical Engineering Division Specialty Conference: Stability and Performance of Slopes and Embankments-II, ASCE, New York, NY, USA, June 29–July 1 1992; pp. 834–839. [Google Scholar]
- Montgomery, D.R.; Schmidt, K.M.; Greenberg, H.M.; Dietrich, W.E. Forest clearing and regional landsliding. Geology 2000, 28, 311–314. [Google Scholar] [CrossRef]
- Kim, J.-W.; Shin, H.-S. Slope stability assessment on a landslide risk area in Ulsan during rainfall. J. Korean Geotech. Soc. 2016, 32, 27–40, (In Korean with English Abstract). [Google Scholar] [CrossRef]
- Jeong, S.; Lee, K.; Kim, J.; Kim, Y. Analysis of rainfall-induced landslide on unsaturated soil slopes. Sustainability 2017, 9, 1280. [Google Scholar] [CrossRef]
- Ran, Q.; Hong, Y.; Li, W.; Gao, J. A modelling study of rainfall-induced shallow landslide mechanisms under different rainfall characteristics. J. Hydrol. 2018, 563, 790–801. [Google Scholar] [CrossRef]
- Varnes, D.J. Slope Movement Types and Process; Special Report; National Academy of Science: Washington, DC, USA, 1978; Volume 2, pp. 11–33. [Google Scholar]
- Brand, E.W. Some thoughts on rain-induced slope failures. In Proceedings of the 10th International Conference on Soil Mechanics Foundation Engineering, Stockholm, The Netherlands, 15–19 June 1981; pp. 373–376. [Google Scholar]
- Cho, S.E. Probabilistic stability analysis of rainfall-induced landslides considering spatial variability of permeability. Eng. Geol. 2014, 171, 11–20. [Google Scholar] [CrossRef]
- Hong, H.; Chen, W.; Xu, C.; Youssef, A.M.; Pradha, B.; Bui, D.T. Rainfall-induced landslide susceptibility assessment at the Chongren area (China) using frequency ratio, certainty factor, and index of entropy. Geocarto Int. 2017, 32, 139–154. [Google Scholar] [CrossRef]
- Tang, G.; Huang, J.; Sheng, D.; Sloan, S.W. Stability analysis of unsaturated soil slopes under random rainfall patterns. Eng. Geol. 2018, 245, 322–332. [Google Scholar] [CrossRef]
- Olivier, M.; Bell, F.G.; Jemy, C.A. The effect of rainfall on slope failure, with examples from the Greater Durban area. In Proceedings of the 7th international Congress IAEG, Lisboa, Portugal, 5–9 September 1994; Volume 3, pp. 1629–1636. [Google Scholar]
- Oh, K.D.; Hong, I.P.; Jun, B.H.; Ahn, W.S.; Lee, M.Y. Evaluation of gis-based landslide hazard mapping. J. Korea Water Resour. Assoc. 2006, 39, 23–33, (In Korean with English Abstract). [Google Scholar]
- Cha, A. A comparison on the identification of landslide hazard using geomorphological characteristics. J. Korean Geo-Environ. Soc. 2014, 15, 67–73, (In Korean with English Abstract). [Google Scholar] [CrossRef][Green Version]
- Chae, B.-G.; Choi, J.; Heong, H.K. A feasibility study of a rainfall triggering index model to warn landslides in Korea. J. Eng. Geol. 2016, 26, 235–250, (In Korean with English Abstract). [Google Scholar] [CrossRef][Green Version]
- Hutchinson, J.N. Morphological and geotechnical parameters of landslides in relation to geology and hydrology. In Proceedings of the Landslides Fifth International Symposium on Landslides, Lausanne, Switzerland, 10–15 July 1988; Volume 1, pp. 3–35. [Google Scholar]
- Kwak, J.H.; Kim, M.-I.; Lee, S.-J. Landslide susceptibility assessment considering the saturation depth ratio by rainfall change. J. Eng. Geol. 2018, 28, 687–699, (In Korean with English Abstract). [Google Scholar]
- Highland, L.; Johnson, M. Landslide Types and Processes; Fact Sheet 2004-3072; USGS: Reston, VA, USA, 2004; 40p.
- Lu, N.; Godt, J. Infinite-slope Stability under Steady Unsaturated Seepage Conditions. Water Resour. Res. 2008, 44, 1–13. [Google Scholar] [CrossRef]
- Lee, S.; Jeong, G.; Park, S.J. Evaluating geomorphological classification systems to predict the occurrence of landslides in mountainous region. J. Korean Geogr. Soc. 2015, 50, 485–503, (In Korean with English Abstract). [Google Scholar]
- Scarpelli, G.; Segato, D.; Sakellariadi, E.; Vita, A.; Ruggeri, P.; Fruzzetti, V.M.E. Slope Instability Problems in the Jonica Highway Construction. In Proceedings of the Second World Landslide Forum, Rome, Italy, 3–9 October 2011; pp. 1–6. [Google Scholar]
- Sewell, R.J.; Fletcher, C.J.N. Pilot Study on Regolith Mapping in Hong Kong; Geological Report GR 1/2000; GEO: Nagoya, Japan, 2000; 45p. [Google Scholar]
- Lepore, C.; Kamal, S.A.; Shanahan, P.; Bras, R.L. Rainfall-induced landslide susceptibility zonation of Puerto Rico. Environ. Earth Sci. 2012, 66, 1667–1681. [Google Scholar] [CrossRef]
- Olivares, L.; Picarelli, L. Shallow flowslides triggered by intense rainfalls in natural slopes covered by loose unsaturated pyroclastic soils. Geotechnique 2003, 53, 283–287. [Google Scholar] [CrossRef]
- Springman, S.M.; Jommi, C.; Teysseire, P. Instability on moraine slopes induced by loss of suction: A case history. Geotechnique 2003, 53, 3–10. [Google Scholar] [CrossRef]
- Xue, J.; Gavin, K. Effect of rainfall intensity on infiltration into partly saturated slopes. Geotech. Geol. Eng. 2008, 26, 199–209. [Google Scholar] [CrossRef]
- Rahardjo, H.; Leong, E.C.; Rezaur, R.B. Effect of antecedent rainfall on pore-water pressure distribution characteristics in residual soil slopes under tropical rainfall. Hydrol. Process. 2008, 22, 506–523. [Google Scholar] [CrossRef]
- Ering, P.; Sivakumar Babu, G.L. Probabilistic back analysis of rainfall-induced landslide—A case study of Malin landslide, India. Eng. Geol. 2016, 208, 154–164. [Google Scholar] [CrossRef]
- Fredlund, D.G.; Xing, A.; Huang, S. Predicting the permeability function for unsaturated soils using the soil-water characteristic curve. Can. Geotech. J. 1994, 31, 533–546. [Google Scholar] [CrossRef]
- Fourie, A.B.; Rowe, D.; Blight, G.E. The effect of infiltration on the stability of the slopes of a dry ash dump. Geotechnique 1999, 49, 1–13. [Google Scholar] [CrossRef]
- Rahardjo, H.; Li, X.E.; Toll, D.G.; Leong, E.C. The effect of antecedent rainfall on slope stability. Geotech. Geol. Eng. 2001, 19, 371–399. [Google Scholar] [CrossRef]
- Lee, S.R.; Oh, T.K.; Kim, Y.K.; Kim, H.C. Influence of Rainfall Intensity and Saturated Permeability on Slope Stability during Rainfall Infiltration. J. Korean Geotech. Soc. 2009, 25, 65–76, (In Korean with English Abstract). [Google Scholar]
- Korean Standards Association. Standard Test Method for Particle Size Distribution of Soils; KS F 2302; Korean Standards Association: Seoul, Korea, 2017. [Google Scholar]
- Korean Standards Association. Standard Test Method for Water Content of Soils; KS F 2306; Korean Standards Association: Seoul, Korea, 2015. [Google Scholar]
- Korean Standards Association. Standard Test Method for Density of Soil Particles; KS F 2308; Korean Standards Association: Seoul, Korea, 2016. [Google Scholar]
- Korean Standards Association. Standard Test Method for Density of Soil in Place by the Sand cone Method; KS F 2311; Korean Standards Association: Seoul, Korea, 2016. [Google Scholar]
- Korean Standards Association. Standard Test Method for Permeability of Saturated Soils; KS F 2322; Korean Standards Association: Seoul, Korea, 2015. [Google Scholar]
- Cho, S.E.; Lee, S.R. Slope stability analysis of unsaturated soil slopes due to rainfall infiltration. J. Korean Geotech. Soc. 2000, 16, 51–64, (In Korean with English Abstract). [Google Scholar]
- Kim, J.H.; Jeong, S.S.; Regueiro, R.A. Instability of partially saturated soil slopes due to alteration of rainfall pattern. Eng. Geol. 2012, 147–148, 28–36. [Google Scholar] [CrossRef]
- Alyamani, M.S.; Sen, Z. Determination of hydraulic conductivity from grain size distribution curves. Ground Water 1993, 31, 551–555. [Google Scholar] [CrossRef]
- Odong, J. Evaluation of empirical formulae for determination of hydraulic conductivity based on grain-size analysis. J. Am. Sci. 2008, 4, 1–6. [Google Scholar]
- Nagy, L.S. Permeability of well graded soils. Period. Polytech. Civ. Eng. 2011, 55, 199–204. [Google Scholar] [CrossRef]
- Singh, A.; Noor, S. Estimation of soil permeability using soil index properties. Int. J. Latest Trends Eng. Technol. 2012, 1, 31–33. [Google Scholar]
- Elhakim, A.F. Estimation of soil permeability. Alex. Eng. J. 2016, 55, 2631–2638. [Google Scholar] [CrossRef]
- Hazen, A. Some Physical Properties of Sands and Gravels, with Special Reference to their Use in Filtration; 24th Annual Report; Massachusetts State Board of Health: Boston, MA, USA, 1892; Volume 34, pp. 539–556.
- Carrier, W.D. Goodbye, Hazen; Hello, Kozeny-Carman. J. Geotech. Geoenviron. Eng. 2003, 129, 1054–1056. [Google Scholar] [CrossRef]
- Holtz, R.D.; Kovacks, W.D. An Introduction to Geotechnical Engineering; Prentice-Hall: Upper Saddle River, NJ, USA, 1981; 733p. [Google Scholar]
- Hamidi, A.; Azini, E.; Masoudi, B. Impact of gradation on the shear strength-dilation behavior of well-graded sand-gravel mixtures. Sci. Iran. A 2012, 19, 393–402. [Google Scholar] [CrossRef]
- Kolbuszewski, J.; Frederick, M.R. The significance of particle shape and size on the mechanical behavior of granular materials. In Proceedings of the European Conference on Soil Mechanics and Foundation Engineering, Wiesbaden, Germany, 15–18 October 1963; Volume 4, pp. 253–263. [Google Scholar]
- Chattopadhya, B.C.; Saha, S. Effect of grain size of cohesionless soil on its shear strength characteristics. In Proceedings of the Geomech 81: Proceeding Symposium on Engineering Behaviour of Coarse Grained Soils, Boulders and Rocks, Hyderabad, India, 21–23 December 1981; Volume 1, pp. 291–297. [Google Scholar]
- Sitharam, G.T.; Nimbkar, S.M. Micromechanical Modelling of Granular Materials: Effect of Particle Size and Gradation. Geotech. Geol. Eng. 2000, 18, 91–117. [Google Scholar] [CrossRef]
- Katzenbach, R.; Schmitt, A. Micromechanical modelling of granular materials under triaxial and oedometric loading. In Numerical Modeling in Micromechanics via Particle Methods-2004-Shimizu; Shimizu, Y., Hart, R., Cundall, P., Eds.; CRC Press: London, UK, 2004; pp. 313–322. [Google Scholar]
- Arkkelin, D. Using SPSS to understand research and data analysis. In Psychology Curricular Materials; Book 1; Valparaiso University: Valparaiso, Indiana, 2014; 194p, Available online: http://scholar.valpo.edu/psych_oer/1 (accessed on 2 May 2020).
- Wang, Q.; Wang, W.; He, X.; Zheng, Q.; Wang, H.; Wu, Y.; Zhong, Z. Changes in soil properties, X-ray-mineral diffractions and infrared-functional groups in bulk soil and fractions following afforestation of farmland, Northeast China. Sci. Rep. 2017, 7, 12829. [Google Scholar] [CrossRef]
- Griffiths, J.S.; Hearn, G.J. Engineering geomorphology: A UKuk perspective. Bull. Int. Assoc. Eng. Geol. 1990, 42, 39–44. [Google Scholar] [CrossRef]
- Kim, W.-Y.; Chae, B.-G. Characteristics of rainfall, geology and failure geometry of the landslide areas on natural terrains, Korea. J. Eng. Geol. 2009, 19, 331–344, (In Korean with English Abstract). [Google Scholar]
- Hungr, O.; Leroueil, S.; Picarelli, L. The Varnes classification of landslide types, an update. Landslides 2014. [Google Scholar] [CrossRef]
- Giannecchini, R.; Pochini, A. Geotechnical influence on soil slips in the Apuan Alps (Tuscany): First results in the Cardoso area. In Proceedings of the International Conference on Fast Movements-Prediction and Prevention for Risk Mitigation (IC-FSM 2003), Naples, Italy, 11–13 May 2003; pp. 241–245. [Google Scholar]
- Gallage, C.P.K.; Uchimura, T. Effects of dry density and grain size distribution on soil-water characteristic curves of sandy soils. Soils Found. 2010, 50, 161–172. [Google Scholar] [CrossRef]


| Geology | Density, g/cm3 | Water Content, % | Liquid Limit, % | Plastic Limit, % | ||||
|---|---|---|---|---|---|---|---|---|
| Range | Average | Range | Average | Range | Average | Range | Average | |
| Gneiss | 2.6–2.76 | 2.69 | 8.72–33.47 | 17.38 | 20.15–37.55 | 29.66 | 14.74–26.32 | 19.84 |
| Granite | 2.52–2.72 | 2.62 | 9.49–31.84 | 16.23 | 23.97–37.15 | 30.10 | 13.77–27.00 | 18.97 |
| Geology | Void Ratio | Porosity, % | Dry Unit Weight, kN/m3 | |||
|---|---|---|---|---|---|---|
| Range | Average | Range | Average | Range | Average | |
| Gneiss | 0.75–1.57 | 1.13 | 42.86–61.09 | 52.57 | 10.3–15.3 | 12.8 |
| Granite | 0.76–1.29 | 1.03 | 43.18–56.33 | 50.49 | 11.5–14.7 | 13.0 |
| Geology | Permeability Coefficient, cm/s | Cohesion, kN/m2 | Internal Friction Angle, ° | |||
|---|---|---|---|---|---|---|
| Range | Average | Range | Average | Range | Average | |
| Gneiss | 1.33 × 10−5–2.17×10−4 | 5.43 × 10−5 | 1.6–29.6 | 8.8 | 18–38 | 33 |
| Granite | 1.66 × 10−6–5.16×10−4 | 6.00 × 10−5 | 0.8–13.3 | 5.3 | 30–39 | 34 |
| Soil Variable | Correlation | Permeability Coefficient, cm/s | Soil Variable | Correlation | Permeability Coefficient, cm/s | ||
|---|---|---|---|---|---|---|---|
| Gneiss | Granite | Gneiss | Granite | ||||
| Density | r | 0.293 | 0.118 | Liquid limit, % | r | −0.167 | 0.373 |
| F | 0.063 | 0.544 | F | 0.310 | 0.046 | ||
| Water Content, % | r | 0.045 | −0.003 | Plastic limit, % | r | −0.065 | 0.161 |
| F | 0.781 | 0.987 | F | 0.694 | 0.404 | ||
| Void Ratio | r | 0.353 | 0.551 | Gravel, % | r | 0.100 | 0.067 |
| F | 0.024 | 0.002 | F | 0.535 | 0.729 | ||
| Porosity, % | r | 0.238 | 0.213 | Sand, % | r | 0.139 | 0.124 |
| F | 0.134 | 0.266 | F | 0.387 | 0.523 | ||
| Degree of Saturation, % | r | −0.162 | −0.229 | Fine, % | r | −0.189 | −0.315 |
| F | 0.311 | 0.231 | F | 0.236 | 0.097 | ||
| Wet unit Weight, kN/m3 | r | −0.294 | −0.533 | Effective grain size, D10, cm | r | 0.670 | 0.836 |
| F | 0.062 | 0.003 | F | 0.000 | 0.000 | ||
| Saturation Unit Weight, kN/m3 | r | −0.270 | −0.413 | Coefficient of uniformity, cu | r | −0.627 | −0.591 |
| F | 0.088 | 0.026 | F | 0.000 | 0.001 | ||
| Dry Unit weight, kN/m3 | r | −0.298 | −0.504 | Coefficient of curvature, cg | r | 0.060 | −0.470 |
| F | 0.058 | 0.005 | F | 0.711 | 0.010 | ||
| Geology | Soil Variable | Unstandardized Coefficients | Standardized Coefficients | t-value | Significance Probability, P | |
|---|---|---|---|---|---|---|
| B | Standard Error | Beta | ||||
| Gneiss | (Constant) | −1.893 × 10−2 | 0.004 | −5.004 | 0.000 | |
| Effective grain size, D10 | 1.076 | 0.161 | 0.774 | 6.683 | 0.000 | |
| Coefficient of uniformity, cu | −1.629 × 10−4 | 0.000 | −0.151 | −1.350 | 0.185 | |
| Void ration, e | 1.488 × 10−2 | 0.002 | 0.655 | 9.415 | 0.000 | |
| Granite | (Constant) | −9.907 × 10−3 | 0.001 | −7.831 | 0.000 | |
| Effective grain size, D10 | 8.281 × 10−3 | 0.001 | 0.474 | 9.177 | 0.000 | |
| Coefficient of uniformity, cu | 0.639 | 0.047 | 0.772 | 13.702 | 0.000 | |
| Void ration, e | −2.766 × 10−5 | 0.000 | −0.058 | −0.973 | 0.340 | |
| Soil Variable | Correlation | Cohesion, kN/m2 | Internal Friction Angle, ° | ||
|---|---|---|---|---|---|
| Gneiss | Granite | Gneiss | Granite | ||
| Density | r | 0.634 | 0.297 | 0.635 | 0.219 |
| F | 0.036 | 0.231 | 0.036 | 0.254 | |
| Water Content, % | r | −0.409 | −0.642 | −0.403 | −0.351 |
| F | 0.211 | 0.004 | 0.219 | 0.062 | |
| Void Ratio | r | −0.272 | −0.093 | −0.935 | −0.808 |
| F | 0.418 | 0.713 | 0.000 | 0.000 | |
| Porosity, % | r | 0.146 | 0.546 | −0.337 | −0.287 |
| F | 0.667 | 0.019 | 0.311 | 0.132 | |
| Degree of Saturation, % | r | −0.234 | −0.687 | 0.112 | −0.113 |
| F | 0.489 | 0.002 | 0.743 | 0.559 | |
| Wet Unit Weight, kN/m3 | r | 0.097 | −0.294 | 0.826 | 0.679 |
| F | 0.777 | 0.234 | 0.002 | 0.000 | |
| Saturation Unit Weight, kN/m3 | r | 0.318 | 0.178 | 0.960 | 0.816 |
| F | 0.341 | 0.481 | 0.000 | 0.000 | |
| Dry Unit Weight, kN/m3 | r | 0.071 | 0.136 | 0.925 | 0.844 |
| F | 0.837 | 0.590 | 0.000 | 0.000 | |
| Liquid Limit | r | −0.270 | 0.073 | −0.502 | −0.258 |
| F | 0.423 | 0.775 | 0.115 | 0.177 | |
| Plastic Limit | r | −0.151 | 0.309 | −0.475 | 0.247 |
| F | 0.658 | 0.213 | 0.139 | 0.196 | |
| Gravel, % | r | 0.215 | 0.419 | 0.032 | −0.040 |
| F | 0.525 | 0.084 | 0.927 | 0.835 | |
| Sand, % | r | 0.534 | 0.125 | 0.198 | 0.252 |
| F | 0.091 | 0.621 | 0.559 | 0.188 | |
| Fine, % | r | −0.947 | −0.928 | −0.219 | −0.345 |
| F | 0.000 | 0.000 | 0.518 | 0.067 | |
| Effective Grain Size, D10, cm | r | 0.480 | 0.606 | 0.599 | −0.043 |
| F | 0.135 | 0.008 | 0.052 | 0.826 | |
| Coefficient of Uniformity, cu | r | −0.746 | −0.417 | −0.374 | −0.008 |
| F | 0.008 | 0.086 | 0.257 | 0.966 | |
| Coefficient of Curvature, cg | r | 0.551 | −0.403 | 0.147 | 0.197 |
| F | 0.079 | 0.097 | 0.666 | 0.307 | |
| Geology | Soil Variable | Unstandardized Coefficients | Standardized Coefficients | t-value | Significance Probability, P | ||
|---|---|---|---|---|---|---|---|
| B | Standard Error | Beta | |||||
| Gneiss | Cohesion, kN/m2 | (Constant) | 15.335 | 1.479 | 10.369 | 0.000 | |
| Fine grain content, Fines | −0.712 | 0.130 | −0.851 | −5.457 | 0.001 | ||
| Coefficient of uniformity, cu | −0.131 | 0.151 | −0.135 | −0.863 | 0.413 | ||
| Internal Friction Angle,° | (Constant) | 6.018 | 10.509 | 0.573 | 0.583 | ||
| Void ratio, e | −12.594 | 2.566 | −0.543 | −4.909 | 0.001 | ||
| Dry unit weight, rd | 27.010 | 6.047 | 0.494 | 4.466 | 0.002 | ||
| Granite | Cohesion, kN/m2 | (Constant) | 18.590 | 0.871 | 21.347 | 0.000 | |
| Fine grain content, Fines | −0.698 | 0.072 | −0.779 | −9.701 | 0.000 | ||
| Degree of saturation, S | −7.441 × 10−2 | 0.020 | −0.298 | −3.709 | 0.002 | ||
| Internal Friction Angle,° | (Constant) | −9.685 | 27.740 | −0.349 | 0.730 | ||
| Void ratio, e | −0.875 | 9.138 | −0.033 | −0.096 | 0.924 | ||
| Dry unit weight, rd | 33.640 | 14.381 | 0.813 | 2.339 | 0.027 | ||
| Soil Variable | Type | Gneiss Soil | Granite Soil | ||
|---|---|---|---|---|---|
| MSE | RMSE | MSE | RMSE | ||
| Permeability coefficient, cm/s | Landslide | 2.864 × 10−6 | 1.692 × 10−3 | 1.536 × 10−7 | 3.919 × 10−4 |
| Non-landslide | 2.738 × 10−6 | 1.654 × 10−3 | 1.769 × 10−7 | 4.206 × 10−4 | |
| Soil variable | Type | Gneiss Soil | Granite Soil | ||
|---|---|---|---|---|---|
| MSE | RMSE | MSE | RMSE | ||
| Cohesion, kN/m2 | Landslide | 1.643 | 1.282 | 0.722 | 0.850 |
| Non-landslide | 0.692 | 0.831 | 0.559 | 0.748 | |
| Internal Friction Angle, ° | Landslide | 0.987 | 0.993 | 1.633 | 1.278 |
| Non-landslide | 0.761 | 0.872 | 1.854 | 1.361 | |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kim, K.-S.; Kim, M.-I.; Lee, M.-S.; Hwang, E.-S. Regression Equations for Estimating Landslide-Triggering Factors Using Soil Characteristics. Appl. Sci. 2020, 10, 3560. https://doi.org/10.3390/app10103560
Kim K-S, Kim M-I, Lee M-S, Hwang E-S. Regression Equations for Estimating Landslide-Triggering Factors Using Soil Characteristics. Applied Sciences. 2020; 10(10):3560. https://doi.org/10.3390/app10103560
Chicago/Turabian StyleKim, Kyeong-Su, Man-Il Kim, Moon-Se Lee, and Eui-Soon Hwang. 2020. "Regression Equations for Estimating Landslide-Triggering Factors Using Soil Characteristics" Applied Sciences 10, no. 10: 3560. https://doi.org/10.3390/app10103560
APA StyleKim, K.-S., Kim, M.-I., Lee, M.-S., & Hwang, E.-S. (2020). Regression Equations for Estimating Landslide-Triggering Factors Using Soil Characteristics. Applied Sciences, 10(10), 3560. https://doi.org/10.3390/app10103560





