Seismic Behavior of Triple Tunnel Complex in Soft Soil Subjected to Transverse Shaking
Abstract
1. Introduction
2. Stages of Analysis
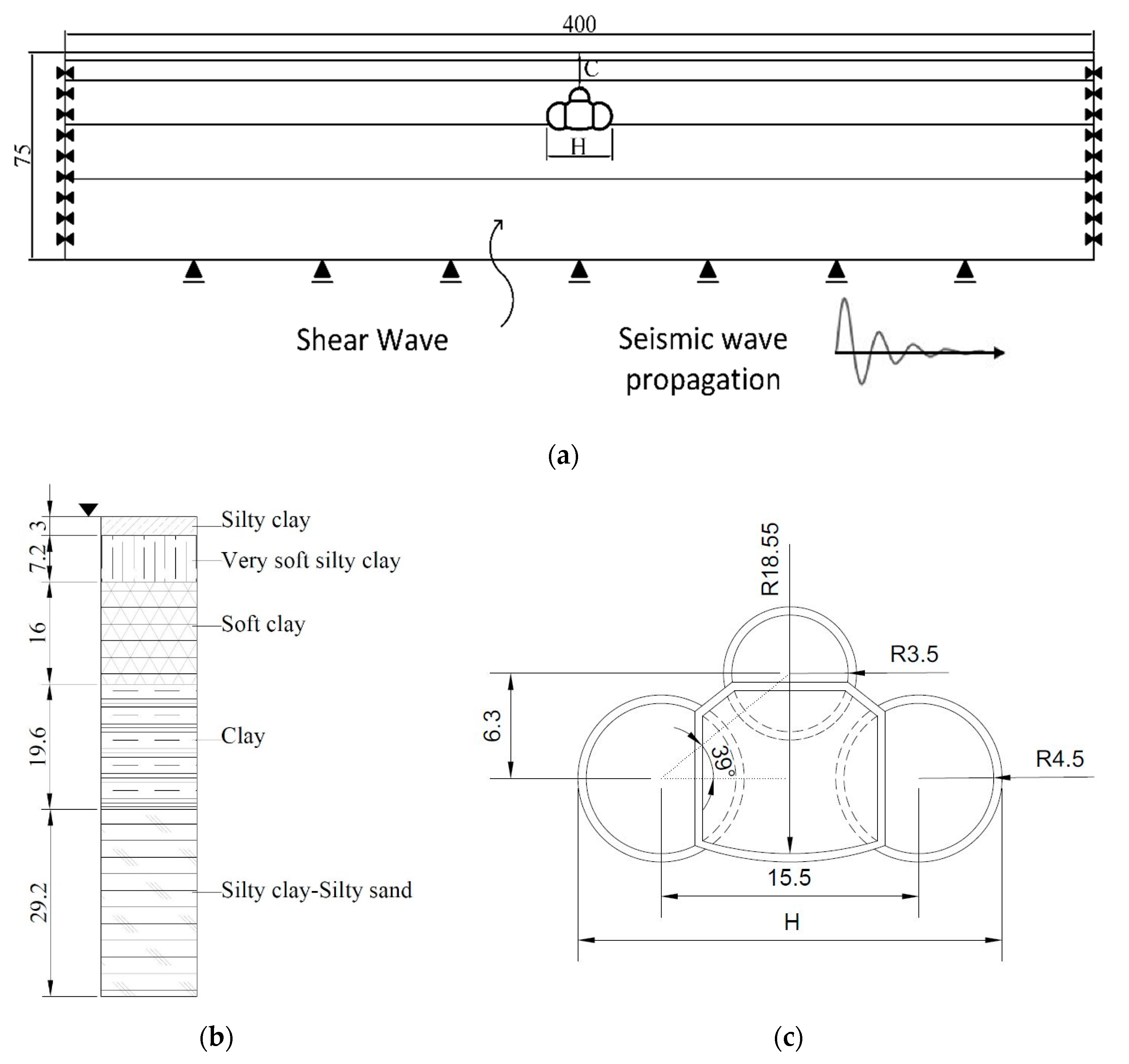
3. Reference Model
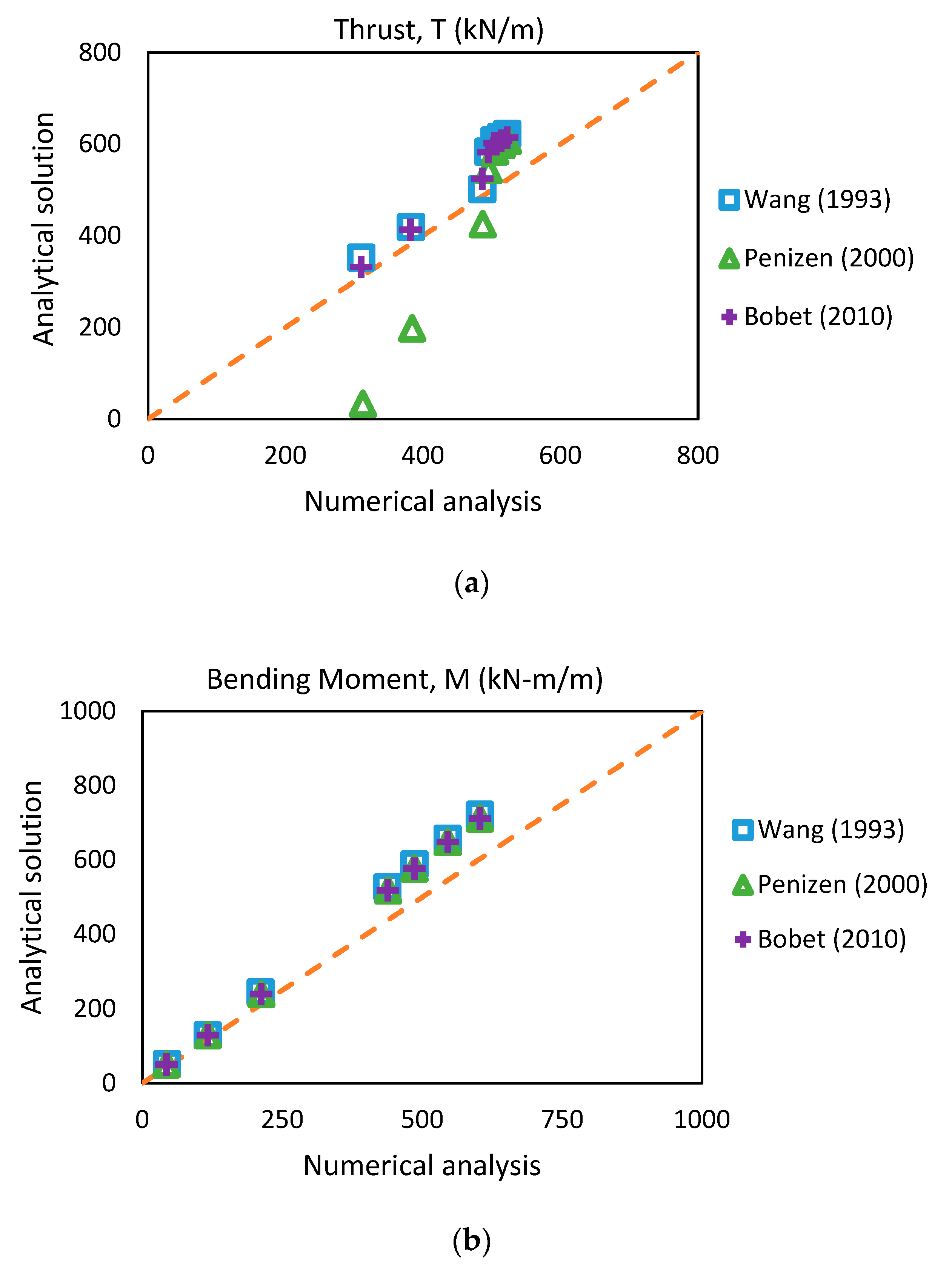
4. Details of the Finite Element Analysis
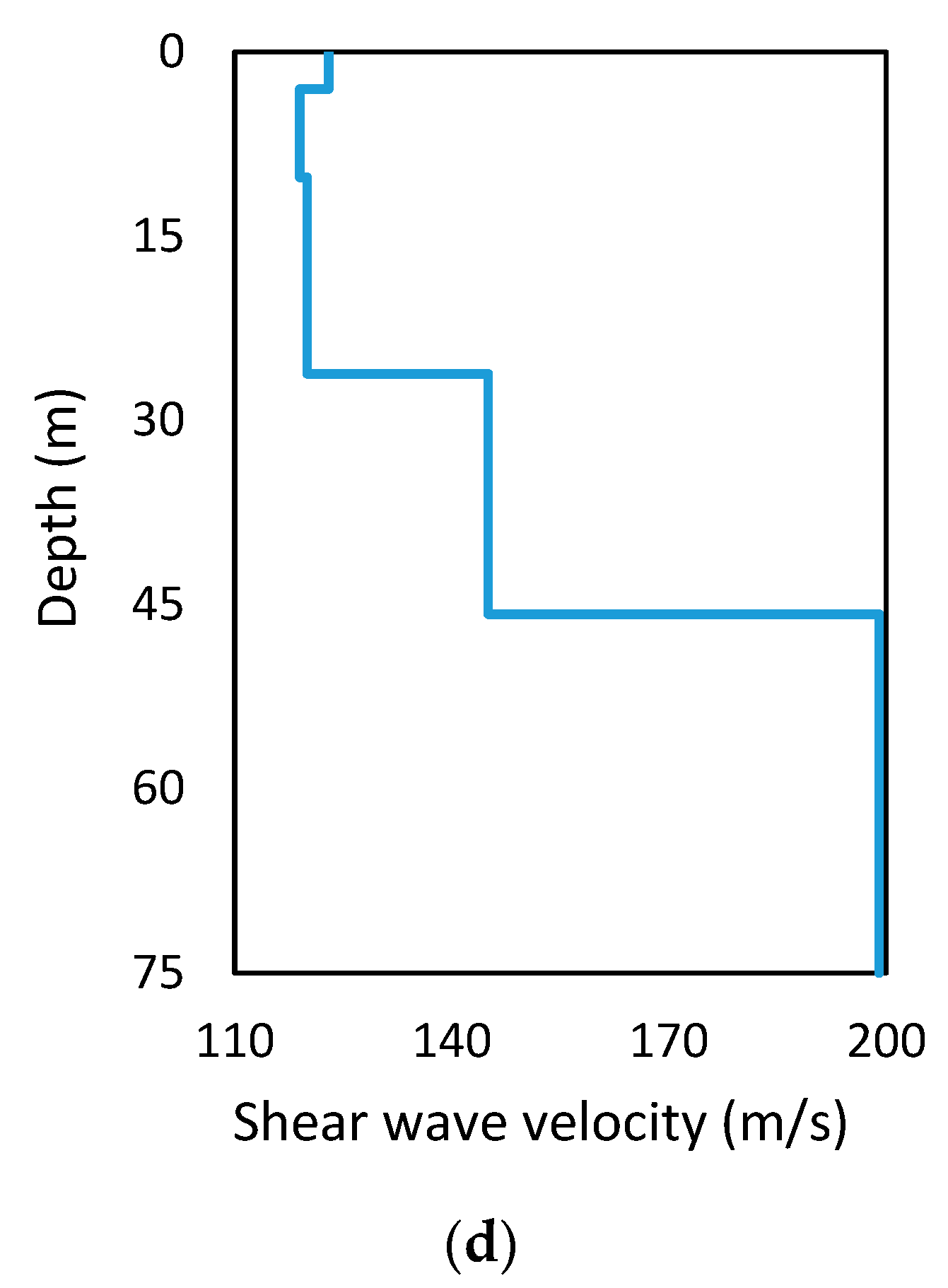
4.1. Software and Soil Parameters
4.2. Constitutive Model and Boundary Conditions
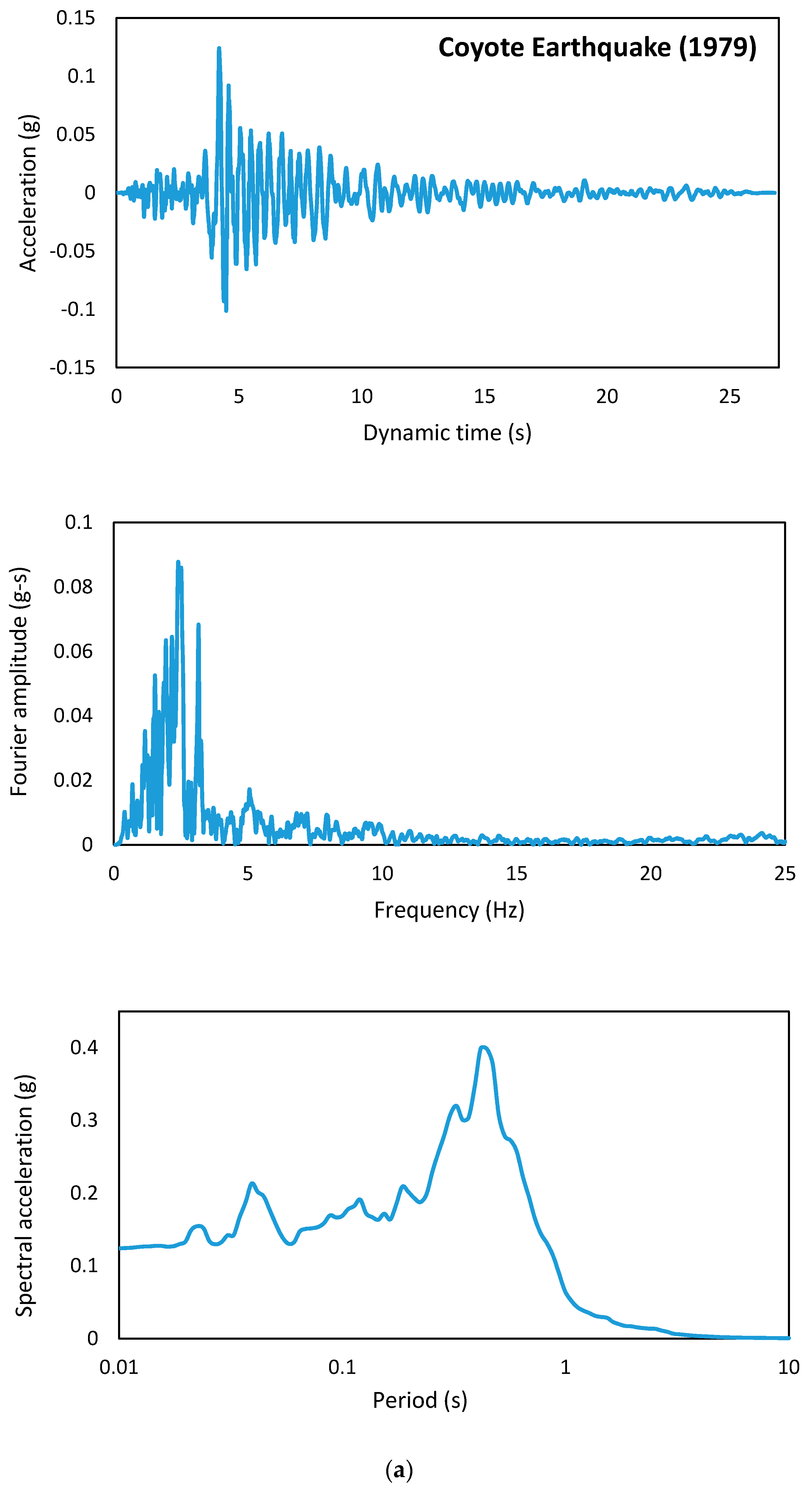
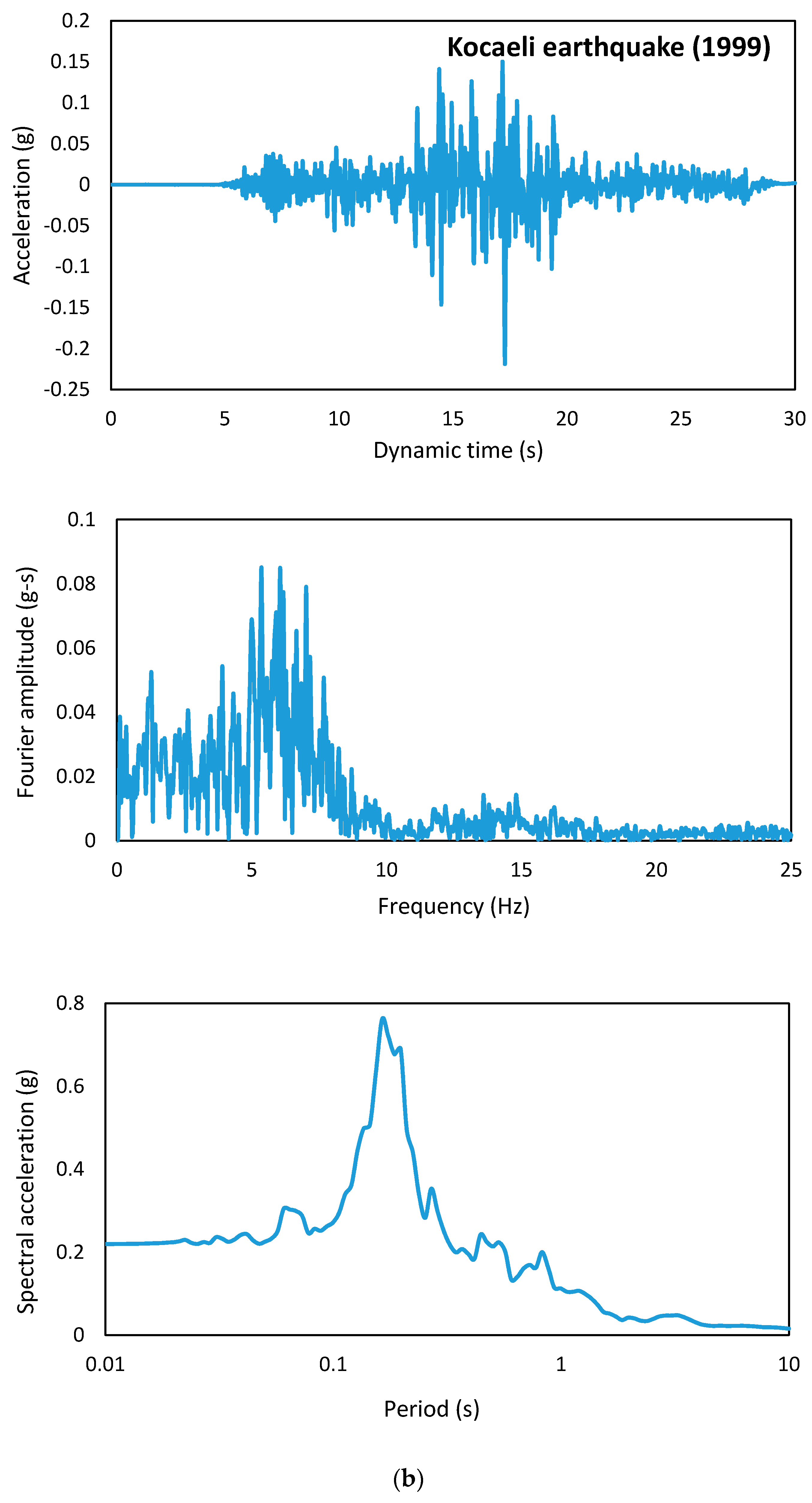
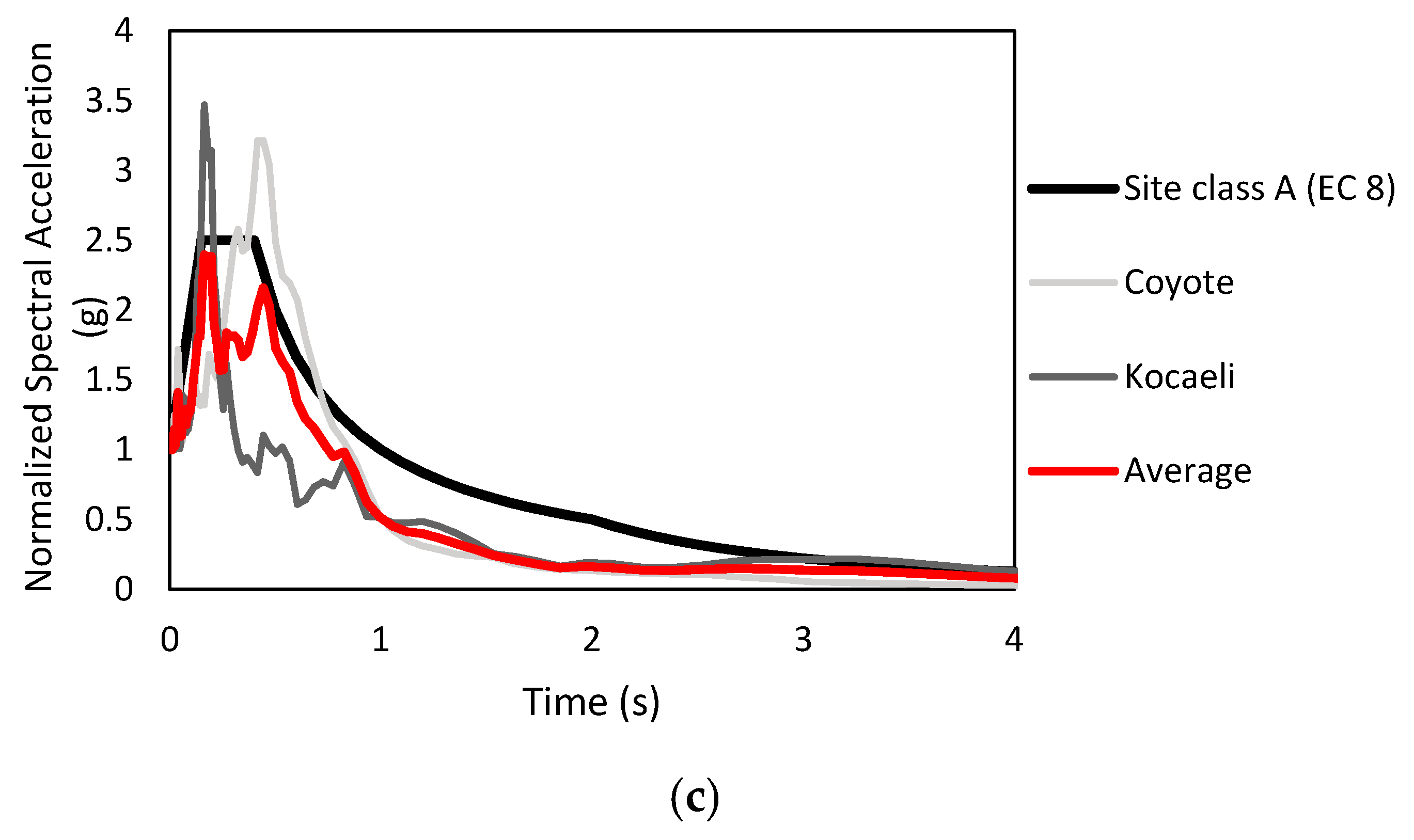
4.3. Input Ground Motion Characteristics
5. Parametric Study
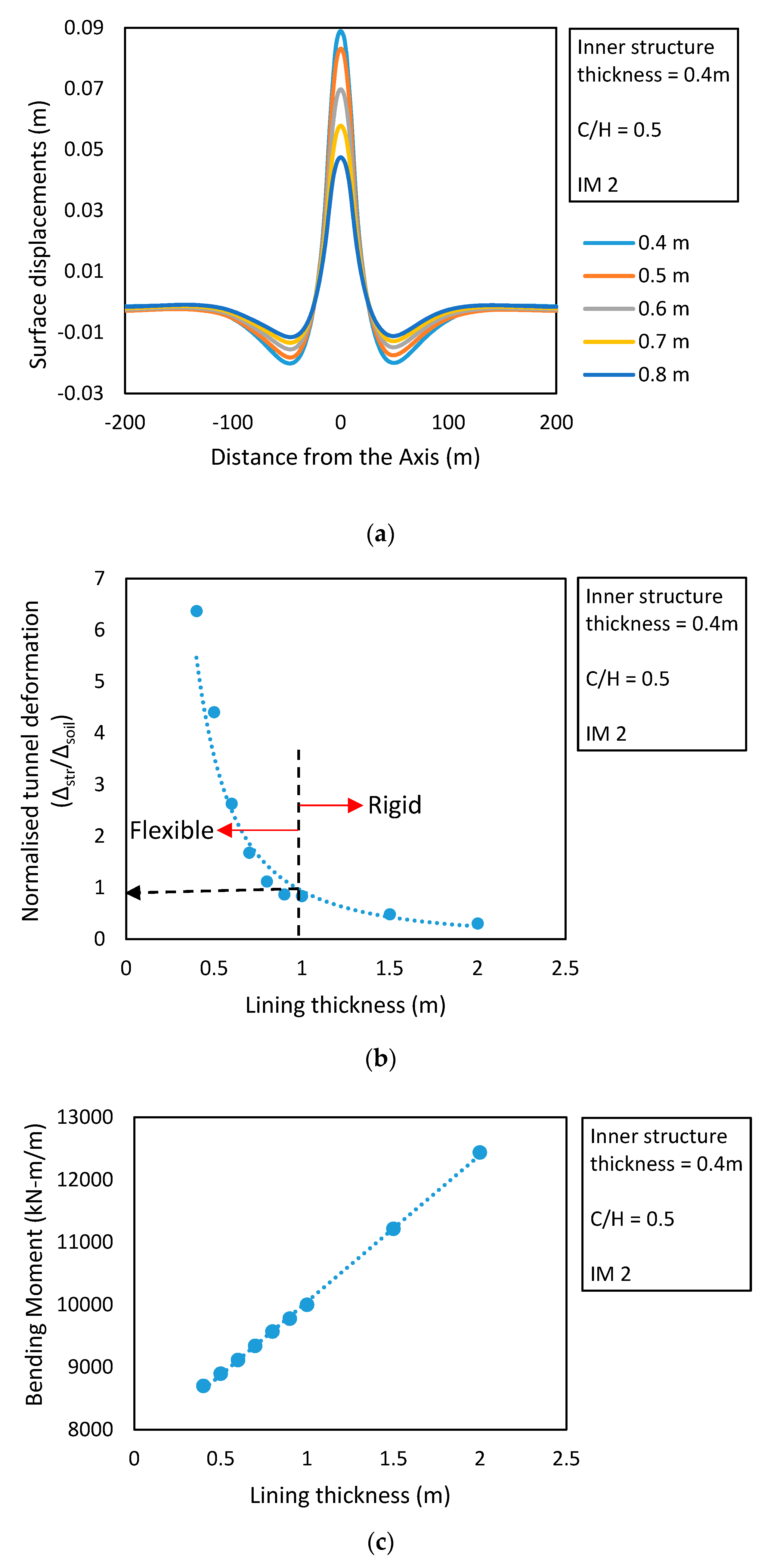
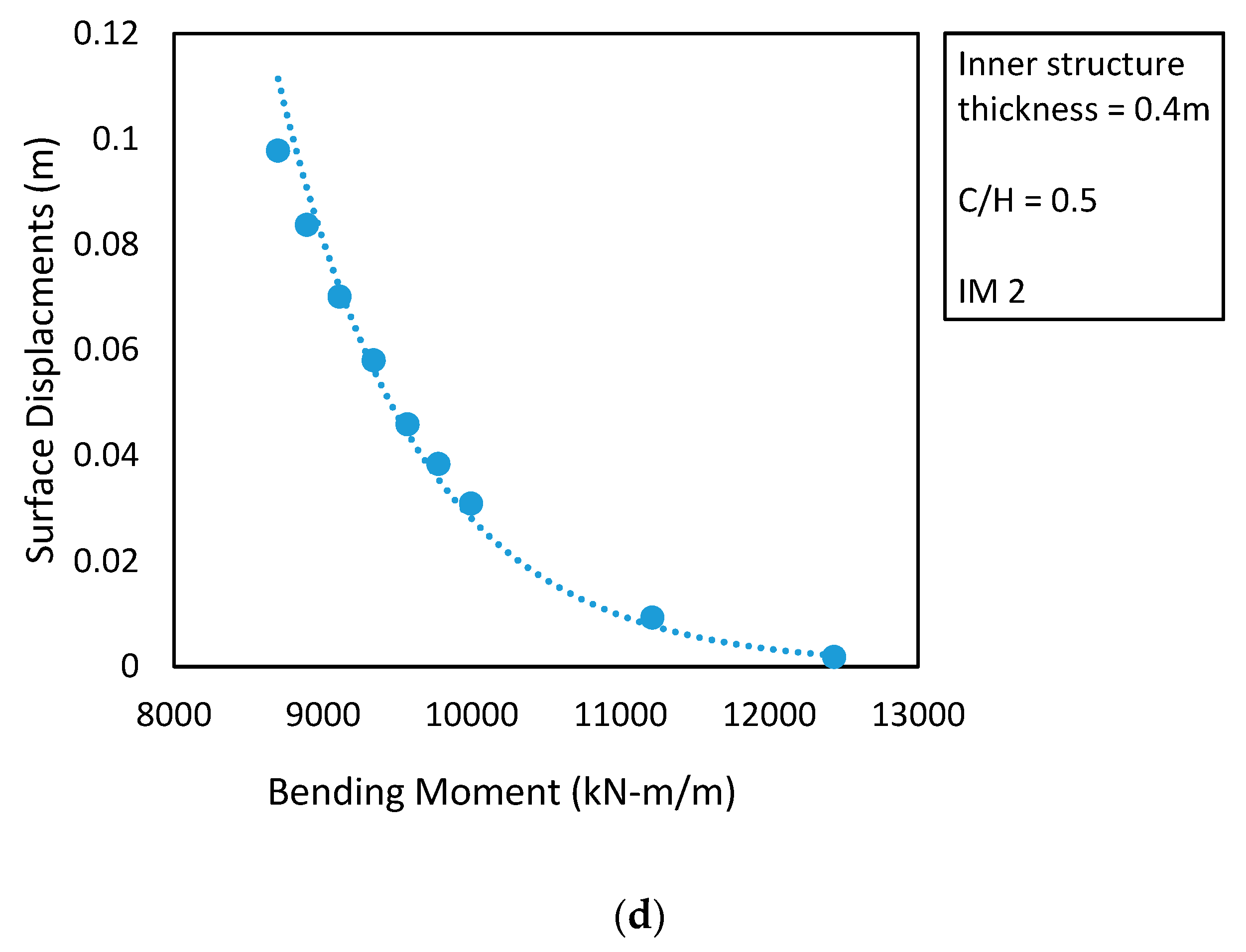
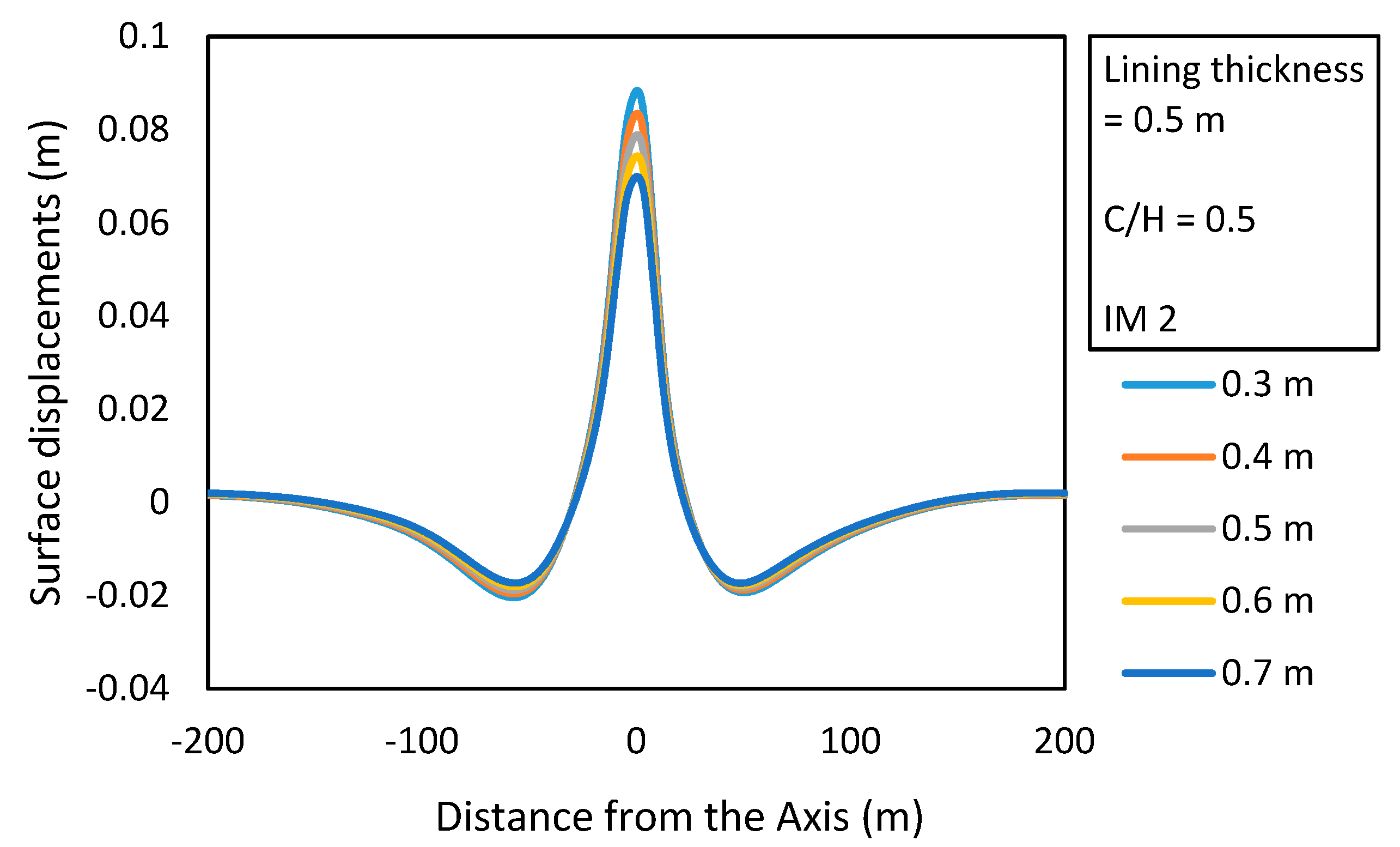
5.1. Effect of the Variation in Lining Thickness
5.2. Effect of Variation in the Inner Structure
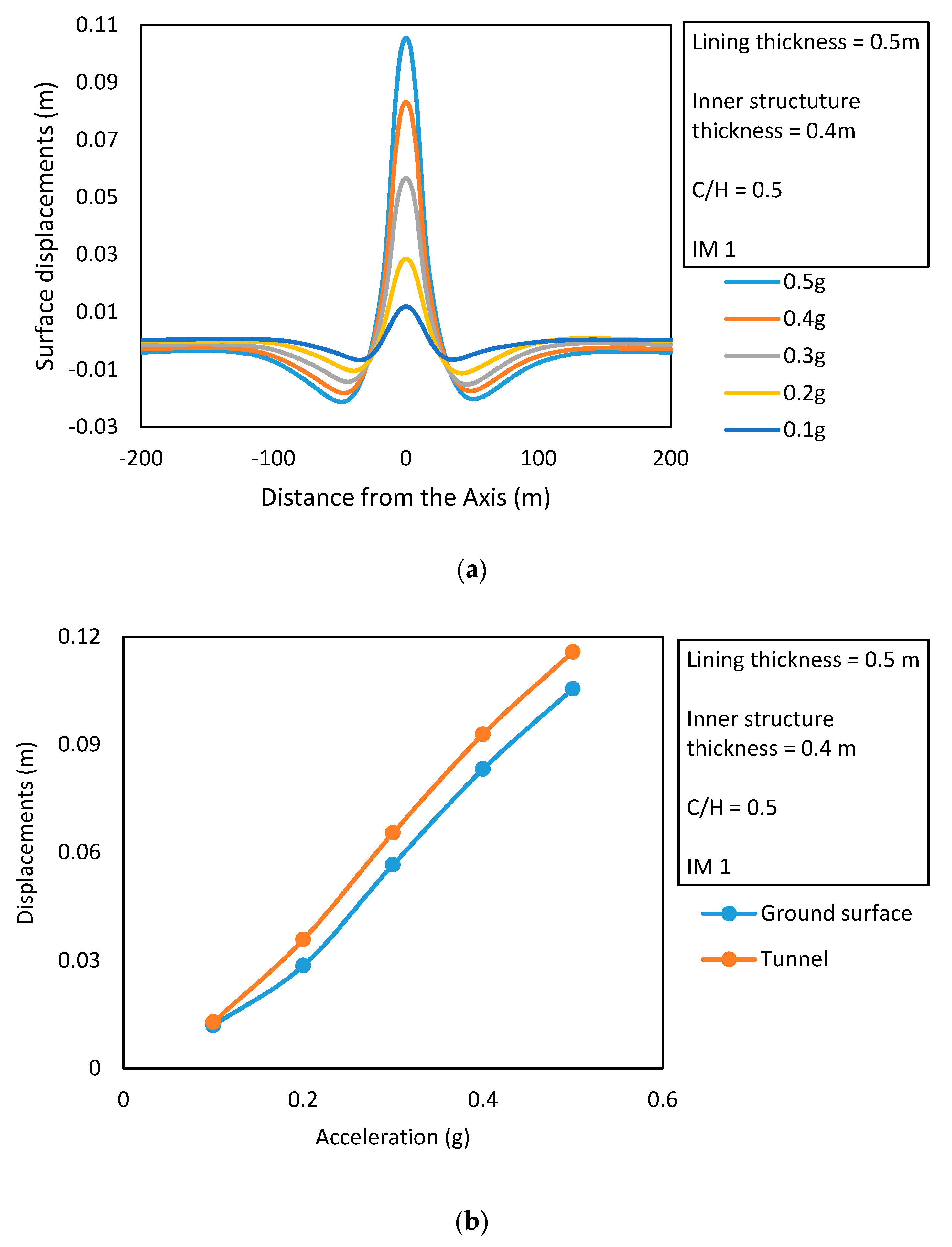
5.3. Effect of the Variation in the Amplitude of the Input Motion
5.4. Effect of Variation in the Embedment Depth
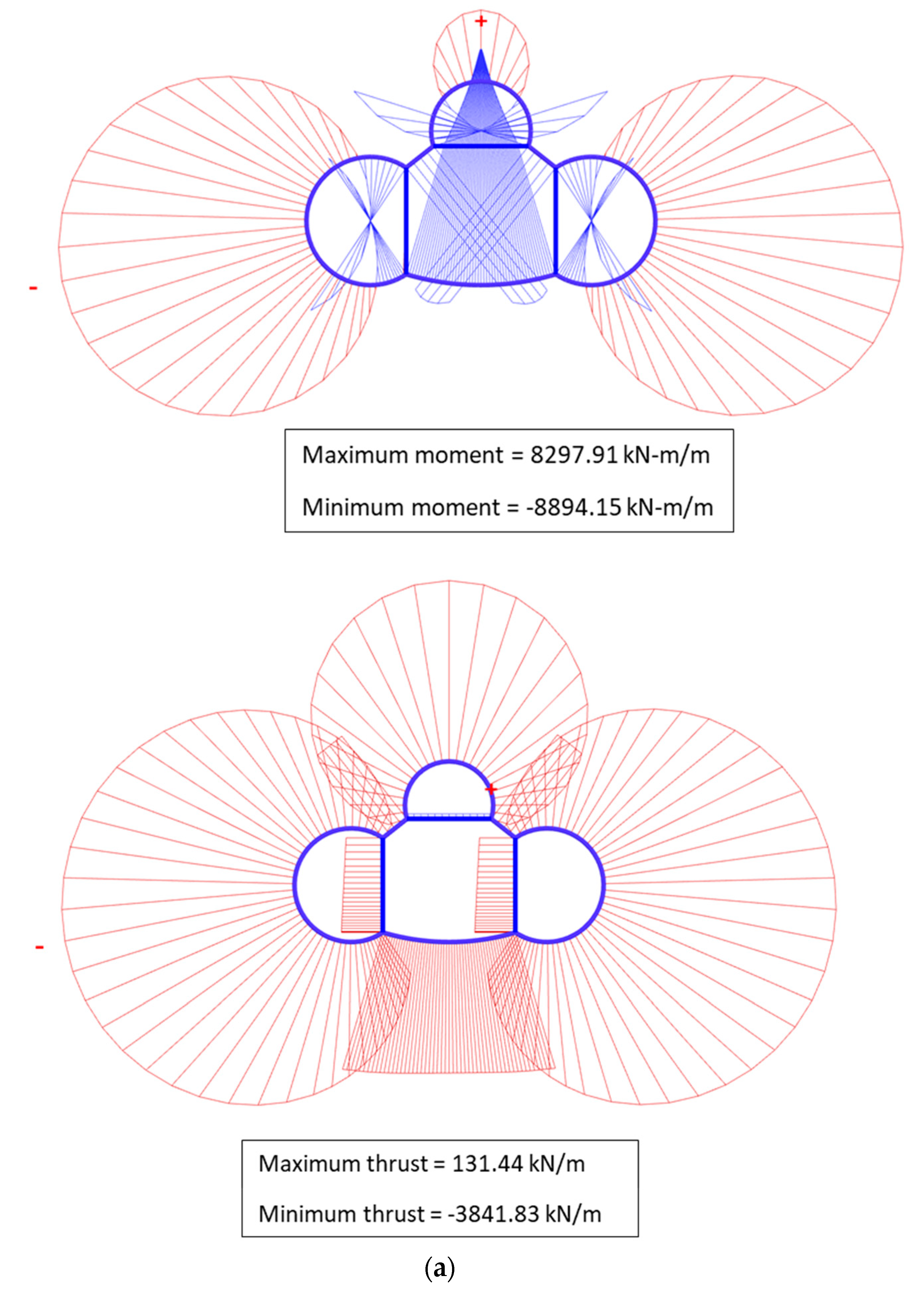
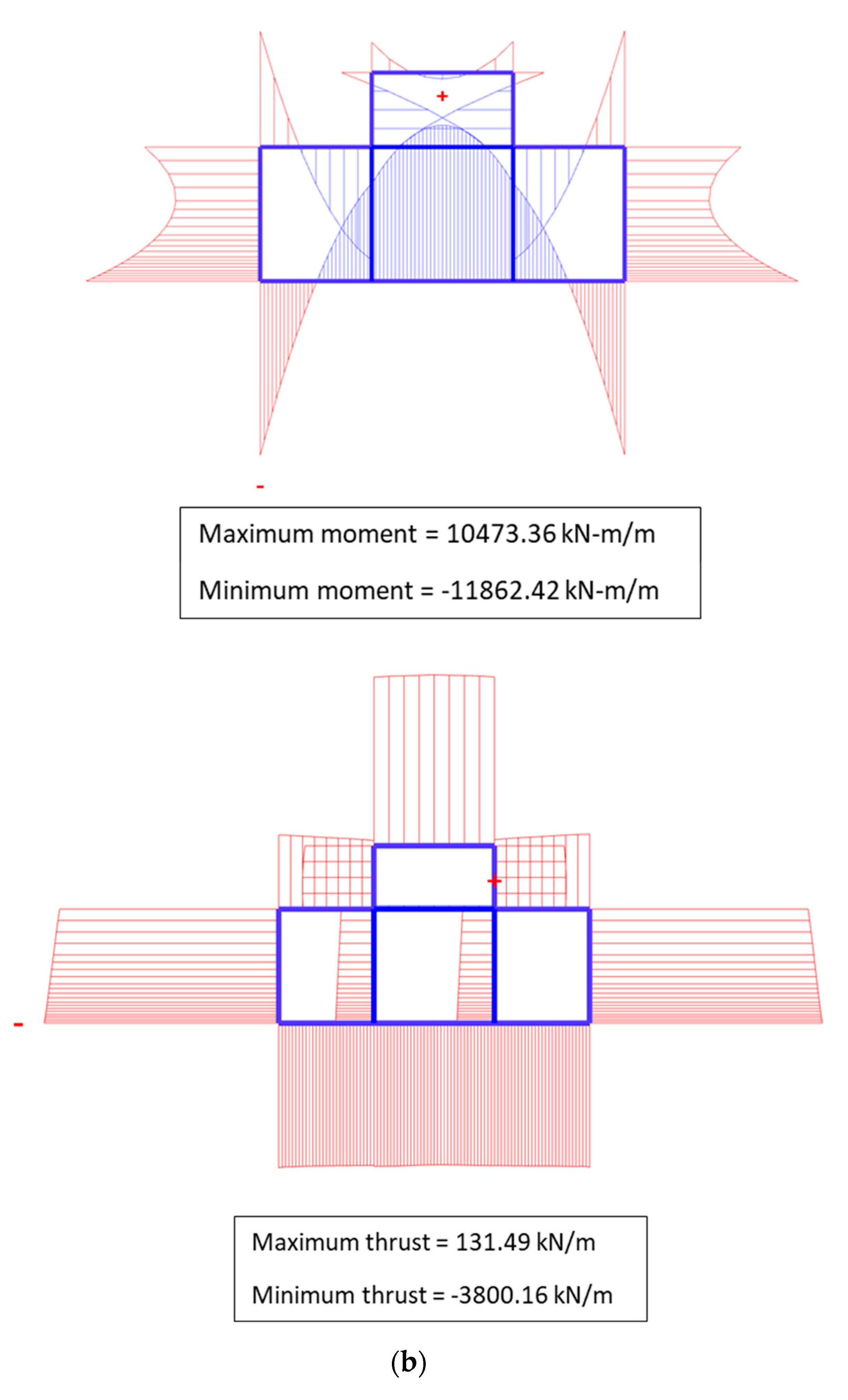
5.5. Effect of Variation in the Shape of the Tunnel Complex
6. Conclusions
- (1)
- The increase in the lining thickness from 0.4 m to 0.8 m decreases the surface displacements from 0.088 m to 0.045 m, reduces the normalized tunnel deformations from 6.37 to 1.12, and the resisted seismic-induced bending moments increase from 8698.27 kN-m/m to 9568.54 kN-m/m, respectively.
- (2)
- The increase in the thickness of the inner structure from 0.3 m to 0.7 m decreases the surface heave from 0.088 m to 0.069 m, respectively.
- (3)
- When the amplitude of the IM increases from 0.1 g to 0.5 g, the surface heave increases from 0.088 m to 0.106 m while the tunnel uplift increases from 0.013 m to 0.116 m.
- (4)
- The change in embedment depth of the tunnel from 0.25 to 2 decreases the surface heave from 0.120 m to 0.051 m while the normalized tunnel deformations are also reduced. In other words, the deeper embedded tunnel would act more rigid in comparison to the shallower embedded tunnel with the same lining thickness.
- (5)
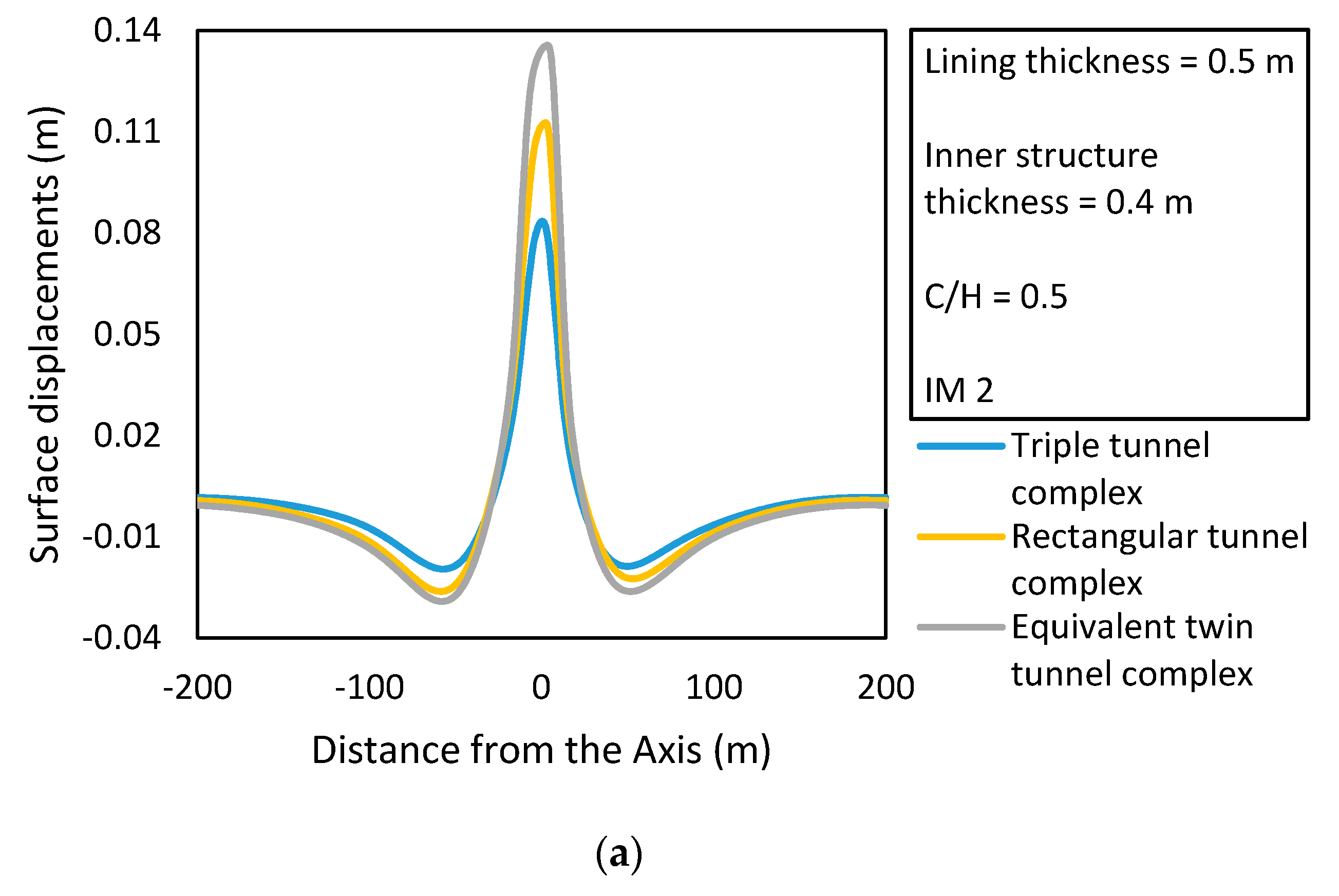
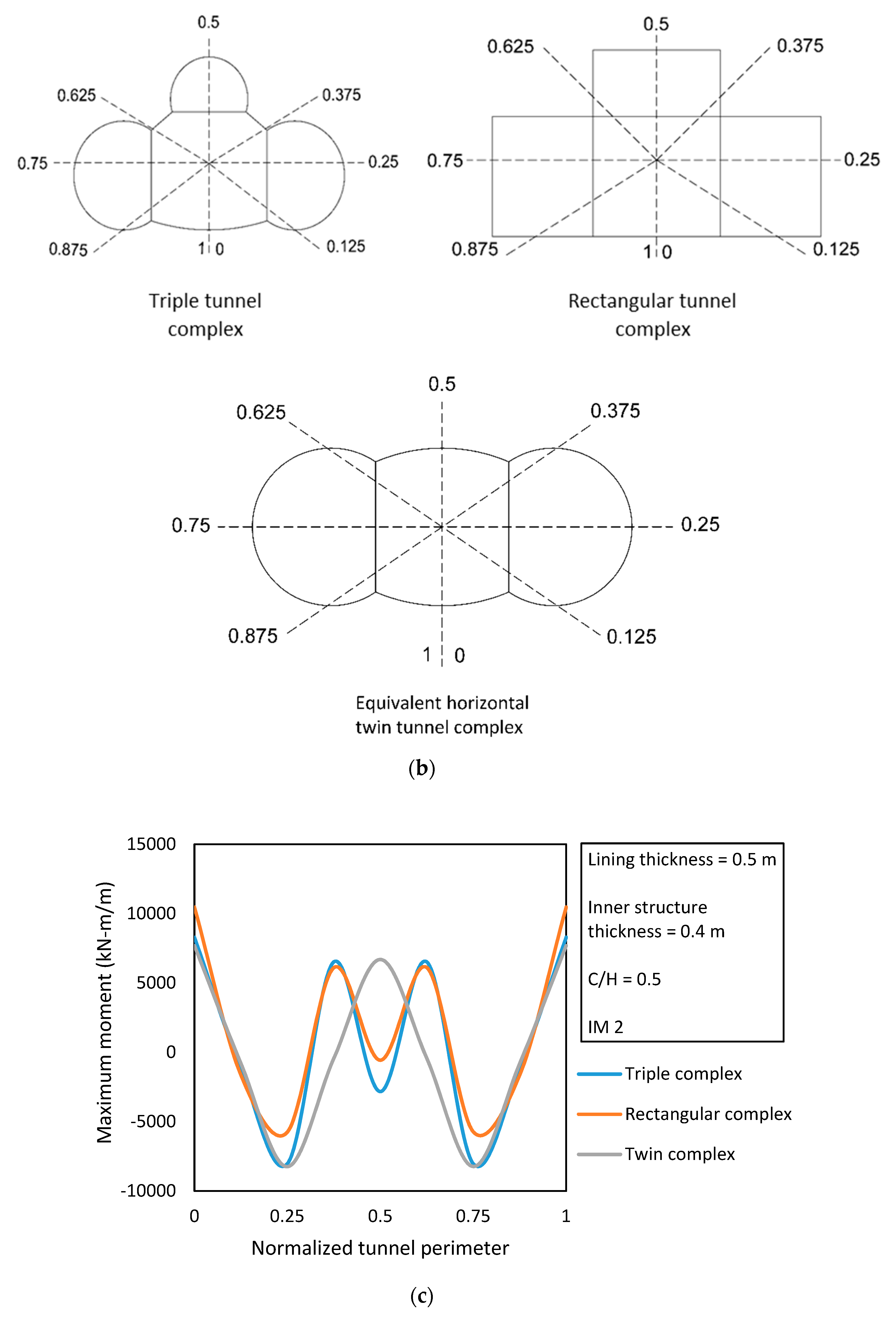
- The shape of the tunnel complex also plays an important role in its seismic behavior. The triple tunnel complex results in a surface heave of 0.083 m as compared to 0.113 m and 0.136 m, which are 36.44% and 63.85% more in the presence of a rectangular tunnel complex and the equivalent horizontal twin tunnel complex, respectively.
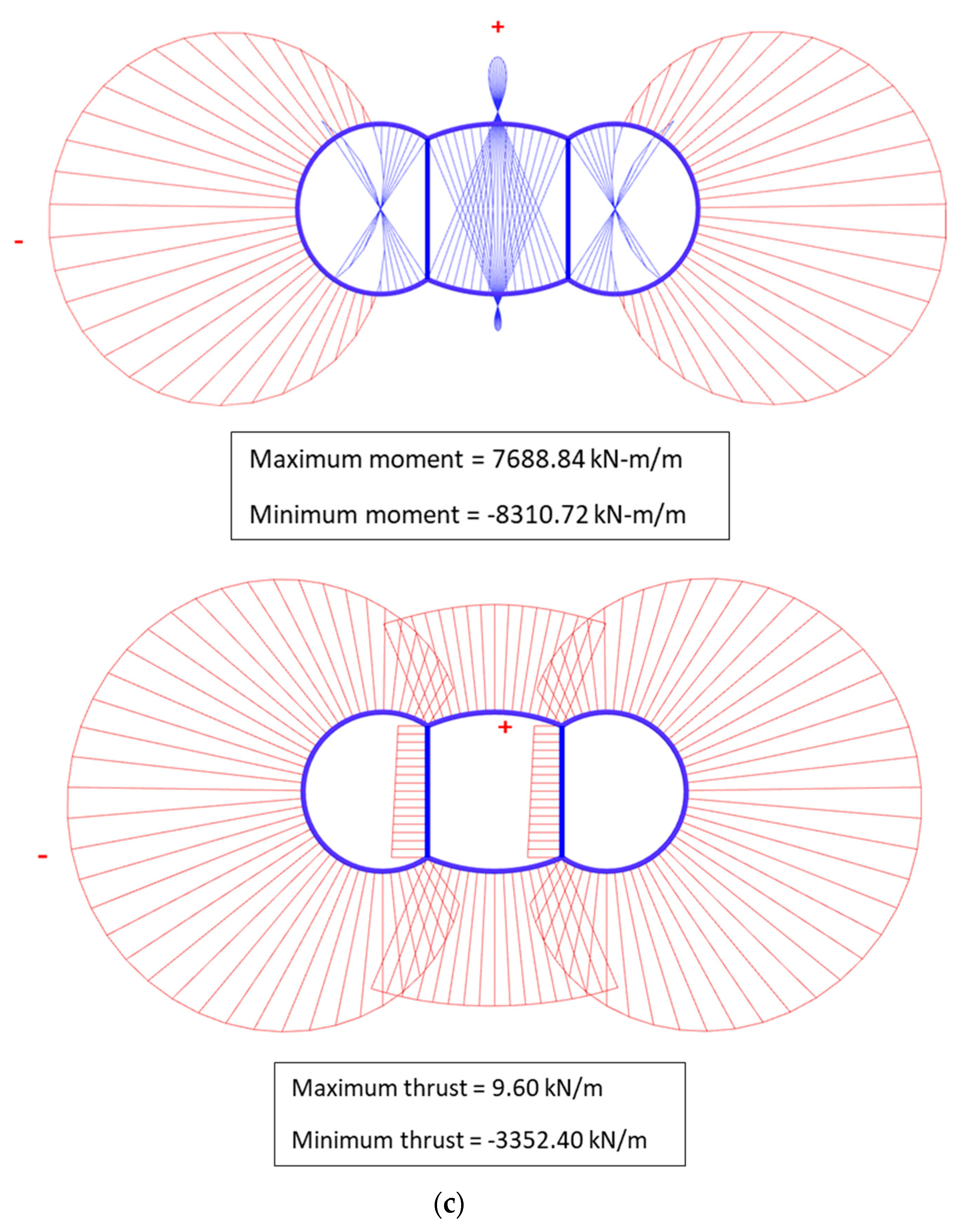
- (6)
- The maximum resisted seismic-induced bending moments by the triple tunnel complex are 33.37% less than that of the rectangular tunnel complex while 6.55% more than the equivalent horizontal twin tunnel complex at the tunnel invert. Looking to the resisted seismic-induced bending moments along the tunnel perimeter, it can be noted that, at the normalized perimeter of about 0.375, 0.5, and 0.625, the resisted moments in case of the triple tunnel complex are about 30.20% more while, at the crown level, about 79.86% more than at the rectangular tunnel complex.
7. Limitations
8. Future Work
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Power, M.; Rosidi, D.; Kaneshiro, J.; Gilstrap, S.; Chiou, S. Summary and Evaluation of Procedures for the Seismic Design of Tunnels; Final report for task; Parsons Brinckerhoff Inc.: New York, NY, USA, 1998. [Google Scholar]
- Wang, J. Seismic Design of Tunnels: A State-of-the-Art Approach, Monograph, Monograph 7; Parsons, Brinckerhoff Quade Douglas Inc.: New York, NY, USA, 1993. [Google Scholar]
- Penzien, J. Seismically induced racking of tunnel linings. Earthq. Eng. Struct. Dyn. 2000, 29, 683–691. [Google Scholar] [CrossRef]
- Bobet, A. Drained and undrained response of deep tunnels subjected to far-field shear loading. Tunn. Undergr. Space Technol. 2010, 25, 21–31. [Google Scholar] [CrossRef]
- Hashash, Y.M.; Hook, J.J.; Schmidt, B.; John, I.; Yao, C. Seismic design and analysis of underground structures. Tunn. Undergr. Space Technol. 2001, 16, 247–293. [Google Scholar] [CrossRef]
- Hashash, Y.M.; Park, D.; John, I.; Yao, C. Ovaling deformations of circular tunnels under seismic loading, an update on seismic design and analysis of underground structures. Tunn. Undergr. Space Technol. 2005, 20, 435–441. [Google Scholar] [CrossRef]
- Liu, H.; Song, E. Seismic response of large underground structures in liquefiable soils subjected to horizontal and vertical earthquake excitations. Comput. Geotech. 2005, 32, 223–244. [Google Scholar] [CrossRef]
- Azadi, M.; Hosseini, S.M.M. Analyses of the effect of seismic behavior of shallow tunnels in liquefiable grounds. Tunn. Undergr. Space Technol. 2010, 25, 543–552. [Google Scholar] [CrossRef]
- Unutmaz, B. 3d liquefaction assessment of soils surrounding circular tunnels. Tunn. Undergr. Space Technol. 2014, 40, 85–94. [Google Scholar] [CrossRef]
- Lanzano, G.; Bilotta, E.; Russo, G.; Silvestri, F.; Madabhushi, S.G. Centrifuge modeling of seismic loading on tunnels in sand. Geotech. Test. J. 2012, 35, 854–869. [Google Scholar] [CrossRef]
- Cilingir, U.; Madabhushi, S.G. Effect of depth on seismic response of circular tunnels. Can. Geotech. J. 2010, 48, 117–127. [Google Scholar] [CrossRef]
- Cilingir, U.; Madabhushi, S.G. Effect of depth on the seismic response of square tunnels. Soils Found. 2011, 51, 449–457. [Google Scholar] [CrossRef]
- Cilingir, U.; Madabhushi, S.G. A model study on the effects of input motion on the seismic behaviour of tunnels. Soil Dyn. Earthq. Eng. 2011, 31, 452–462. [Google Scholar] [CrossRef]
- Qiu, J.; Xie, Y.; Fan, H.; Wang, Z.; Zhang, Y. Centrifuge modelling of twin-tunnelling induced ground movements in loess strata. Arab. J. Geosci. 2017, 10, 493. [Google Scholar] [CrossRef]
- Shahrour, I.; Khoshnoudian, F.; Sadek, M.; Mroueh, H. Elastoplastic analysis of the seismic response of tunnels in soft soils. Tunn. Undergr. Space Technol. 2010, 25, 478–482. [Google Scholar] [CrossRef]
- Patil, M.; Choudhury, D.; Ranjith, P.; Zhao, J. Behavior of shallow tunnel in soft soil under seismic conditions. Tunn. Undergr. Space Technol. 2018, 82, 30–38. [Google Scholar] [CrossRef]
- Tsinidis, G. Response characteristics of rectangular tunnels in soft soil subjected to transversal ground shaking. Tunn. Undergr. Space Technol. 2017, 62, 1–22. [Google Scholar] [CrossRef]
- Chian, S.; Madabhushi, S. Effect of buried depth and diameter on uplift of underground structures in liquefied soils. Soil Dyn. Earthq. Eng. 2012, 41, 181–190. [Google Scholar] [CrossRef]
- Boldini, D.; Graziani, A. Remarks on axisymmetric modelling of deep tunnels in argillaceous formations. Ii: Fissured argillites. Tunn. Undergr. Space Technol 2012, 28, 80–89. [Google Scholar] [CrossRef]
- Chian, S.C.; Tokimatsu, K.; Madabhushi, S.P.G. Soil liquefaction–induced uplift of underground structures: Physical and numerical modeling. J. Geotech. Geoenviron. Eng. 2014, 140, 04014057. [Google Scholar] [CrossRef]
- Abuhajar, O.; El Naggar, H.; Newson, T. Seismic soil–culvert interaction. Can. Geotech. J. 2015, 52, 1649–1667. [Google Scholar] [CrossRef]
- Chian, S.; Tokimatsu, K. Floatation of Underground Structures During the Mw 9.0 tōhoku Earthquake of 11th March 2011. In Proceedings of the 15th World Conference on Earthquake Engineering, Lisbon, Portugal, 24–28 September 2012. [Google Scholar]
- Bobet, A.; Fernandez, G.; Huo, H.; Ramirez, J. A practical iterative procedure to estimate seismic-induced deformations of shallow rectangular structures. Can. Geotech. J. 2008, 45, 923–938. [Google Scholar] [CrossRef]
- Chou, H.; Yang, C.; Hsieh, B.; Chang, S. A study of liquefaction related damages on shield tunnels. Tunn. Undergr. Space Technol. 2001, 16, 185–193. [Google Scholar] [CrossRef]
- Amorosi, A.; Boldini, D. Numerical modelling of the transverse dynamic behaviour of circular tunnels in clayey soils. Soil Dyn. Earthq. Eng. 2009, 29, 1059–1072. [Google Scholar] [CrossRef]
- Baziar, M.H.; Moghadam, M.R.; Kim, D.-S.; Choo, Y.W. Effect of underground tunnel on the ground surface acceleration. Tunn. Undergr. Space Technol. 2014, 44, 10–22. [Google Scholar] [CrossRef]
- Huo, H.; Bobet, A.; Fernández, G.; Ramirez, J. Load transfer mechanisms between underground structure and surrounding ground: Evaluation of the failure of the daikai station. J. Geotech. Geoenviron. Eng. 2005, 131, 1522–1533. [Google Scholar] [CrossRef]
- Nguyen, D.-D.; Lee, T.-H.; Nguyen, V.-Q.; Park, D. Seismic damage analysis of box metro tunnels accounting for aspect ratio and shear failure. Appl. Sci. 2019, 9, 3207. [Google Scholar] [CrossRef]
- Naseem, A.; Schotte, K.; De Pauw, B.; De Backer, H. Ground settlements due to construction of triplet tunnels with different construction arrangements. Adv. Civ. Eng. 2019, 2019, 18. [Google Scholar] [CrossRef]
- Hu, Z.; Yue, Z.; Zhou, J.; Tham, L. Design and construction of a deep excavation in soft soils adjacent to the shanghai metro tunnels. Can. Geotech. J. 2003, 40, 933–948. [Google Scholar] [CrossRef]
- Standard, B. Eurocode 8: Design of structures for earthquake resistance. Part 2005, 1, 1998-1. [Google Scholar]
- Fernández Ruiz, J.; Medina Rodríguez, L. Application of an advanced soil constitutive model to the study of railway vibrations in tunnels through 2d numerical models: A real case in madrid (spain). Rev. Constr. 2015, 14, 55–63. [Google Scholar] [CrossRef]
- Brinkgreve, R.; Swolfs, W.; Engin, E.; Waterman, D.; Chesaru, A.; Bonnier, P.; Galavi, V. Plaxis 2d Reference Manual; Delft University of Technology and PLAXIS bv: Delft, The Netherlands, 2011. [Google Scholar]
- Brinkgreve, R.B.J. Geomaterial Models and Numerical Analysis of Softening. Ph.D. Thesis, Delft University, Delft, The Netherlands, 1996. Available online: https://elibrary.ru/item.asp?id=6868289 (accessed on 2 January 2020).
- Hardin, B.O.; Drnevich, V.P. Shear modulus and damping in soils: Design equations and curves. J. Soil Mech. Found. Div. 1972, 98, 667–692. [Google Scholar]
- Kuhlemeyer, R.L.; Lysmer, J. Finite element method accuracy for wave propagation problems. J. Soil Mech. Found. Div. 1973, 99, 421–427. [Google Scholar]



| No. | Soil Type | Saturated Unit Weight (kN/m3) | Shear Strength (kPa) | Permeability (m/s) | Rayleigh Coefficients | ||
|---|---|---|---|---|---|---|---|
| Horizontal | Vertical | α | β (× 10−3) | ||||
| 1 | Silty clay | 18.4 | 29.9 | 5.5 × 10−7 | 2.50 × 10−9 | 9.660 | 0.776 |
| 2 | Very soft silty clay | 17.5 | 27.4 | 3.5 × 10−6 | 1.70 × 10−8 | 3.893 | 1.926 |
| 3 | Very soft clay | 16.9 | 19.8 | 5.13 × 10−8 | 1.91 × 10−9 | 1.771 | 4.238 |
| 4 | Clay | 18 | 26.3 | 3.40 × 10−6 | 3.51 × 10−8 | 1.744 | 4.301 |
| 5 | Silty clay-silty sand | 18.1 | 30 | 2.13 × 10−5 | 2.67 × 10−6 | 1.706 | 4.397 |
| No. | Earthquake | Station | Year | Magnitude (Mw) | Epicenter Distance (Km) | Peak Ground Acceleration PGA (g) | Peak Ground Velocity PGV (m/s) |
|---|---|---|---|---|---|---|---|
| 1 | Coyote, USA | San Juan Bautista | 1979 | 5.7 | 17.2 | 0.124 | 0.176 |
| 2 | Kocaeli, Turkey | Arcelik | 1999 | 7.4 | 17 | 0.218 | 0.177 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Naseem, A.; Kashif, M.; Iqbal, N.; Schotte, K.; De Backer, H. Seismic Behavior of Triple Tunnel Complex in Soft Soil Subjected to Transverse Shaking. Appl. Sci. 2020, 10, 334. https://doi.org/10.3390/app10010334
Naseem A, Kashif M, Iqbal N, Schotte K, De Backer H. Seismic Behavior of Triple Tunnel Complex in Soft Soil Subjected to Transverse Shaking. Applied Sciences. 2020; 10(1):334. https://doi.org/10.3390/app10010334
Chicago/Turabian StyleNaseem, Ahsan, Muhammad Kashif, Nouman Iqbal, Ken Schotte, and Hans De Backer. 2020. "Seismic Behavior of Triple Tunnel Complex in Soft Soil Subjected to Transverse Shaking" Applied Sciences 10, no. 1: 334. https://doi.org/10.3390/app10010334
APA StyleNaseem, A., Kashif, M., Iqbal, N., Schotte, K., & De Backer, H. (2020). Seismic Behavior of Triple Tunnel Complex in Soft Soil Subjected to Transverse Shaking. Applied Sciences, 10(1), 334. https://doi.org/10.3390/app10010334






