Vegetated Channel Flows: Turbulence Anisotropy at Flow–Rigid Canopy Interface
Abstract
1. Introduction
2. Materials and Methods
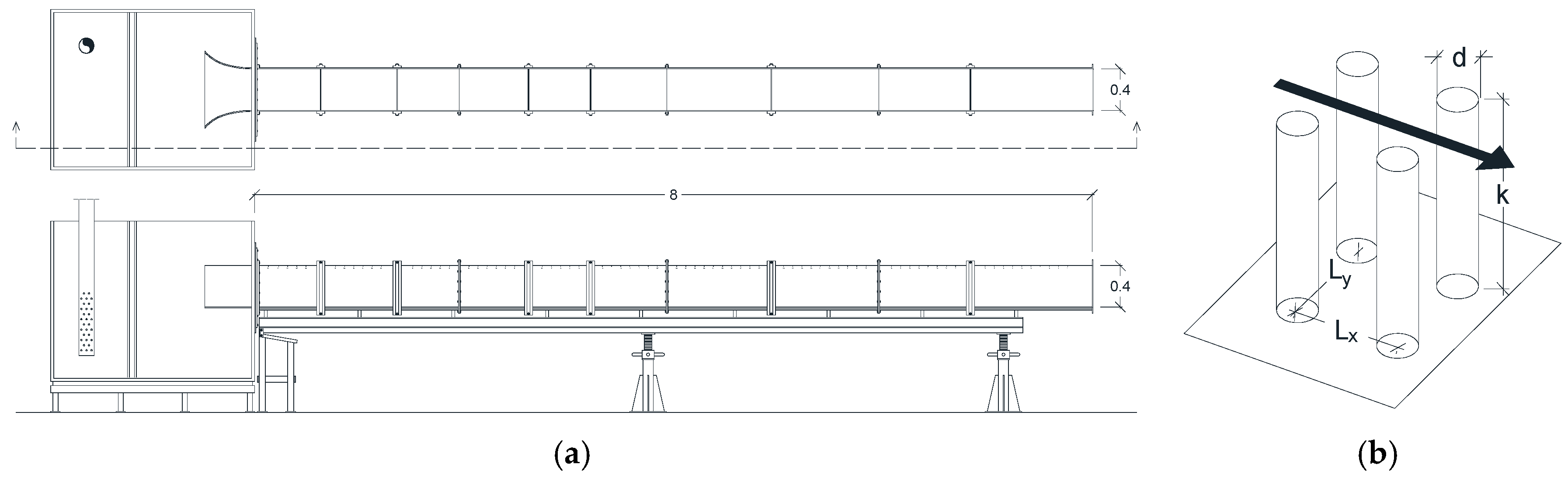
2.1. Laboratory Flume
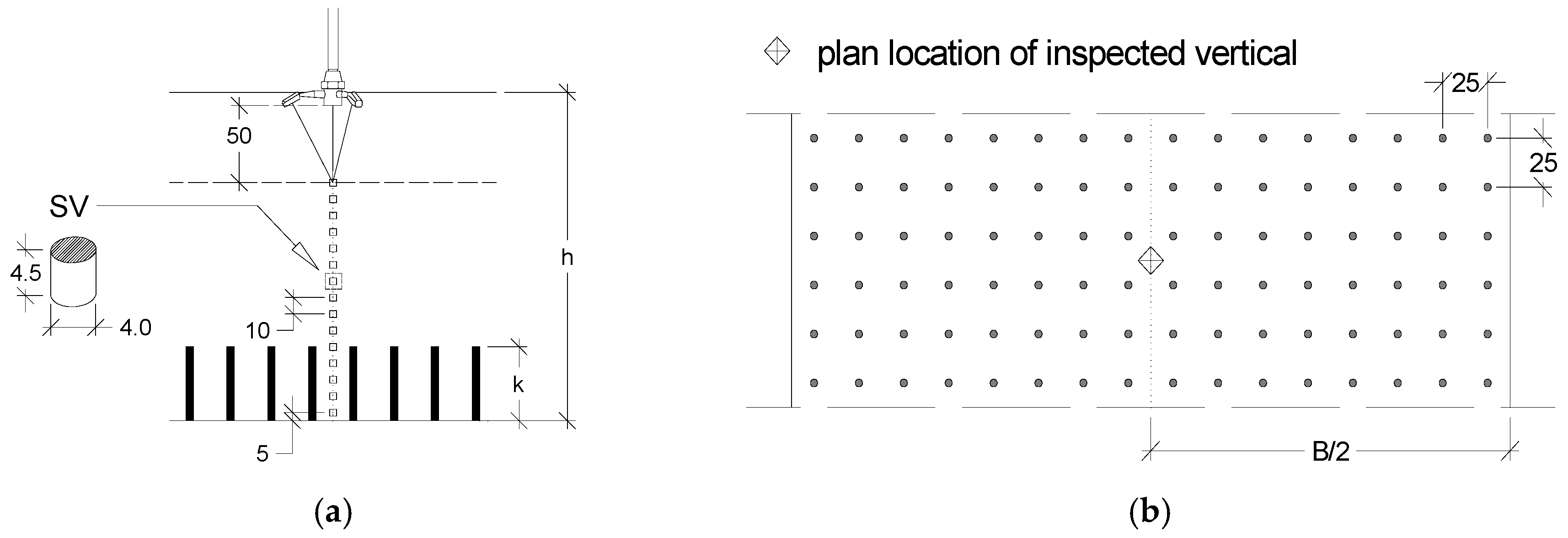
2.2. Rigid Vegetation Model
- the frontal area per canopy volume , equal to 6.4 m−1;
- the frontal area per bed area , also known as the density roughness, computable as for vertically uniform vegetation, and equal to 0.288;
- the solid volume fraction occupied by the canopy elements , evaluable as for cylindrical elements and equal to 0.020.
2.3. Test Cases and Velocity Measurements
3. Results and Discussion
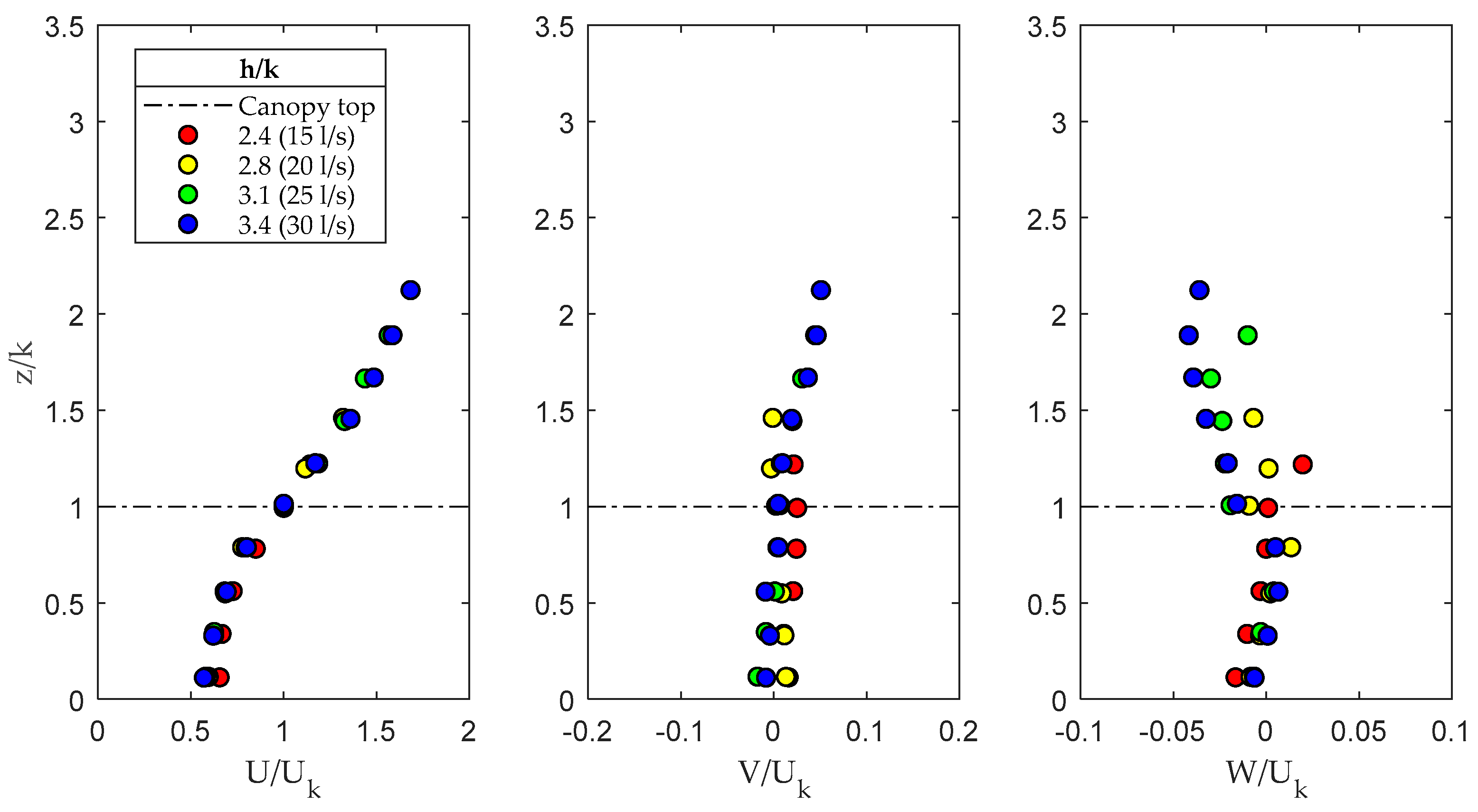
3.1. Mean Velocity Profiles
3.2. Turbulent Intensities
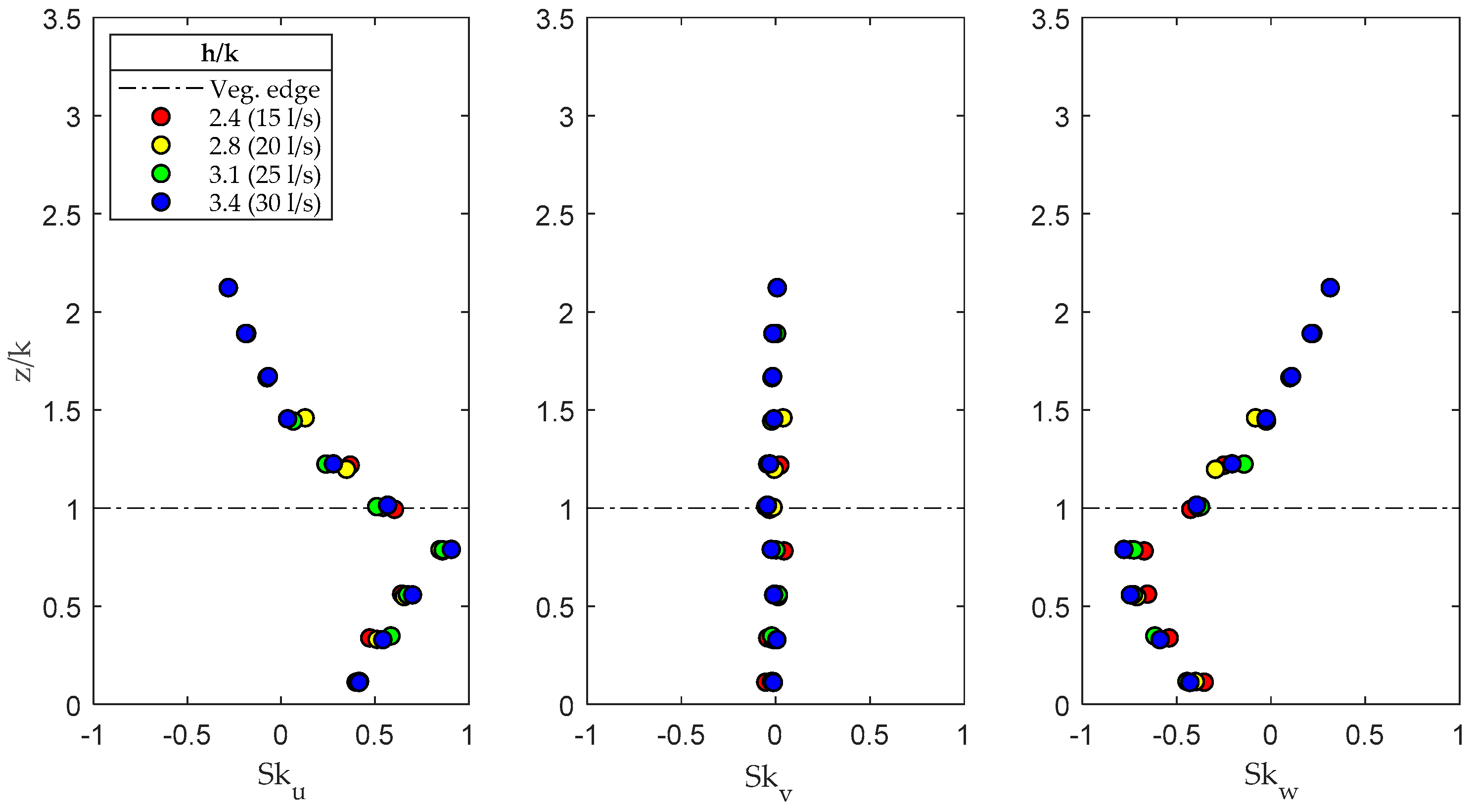
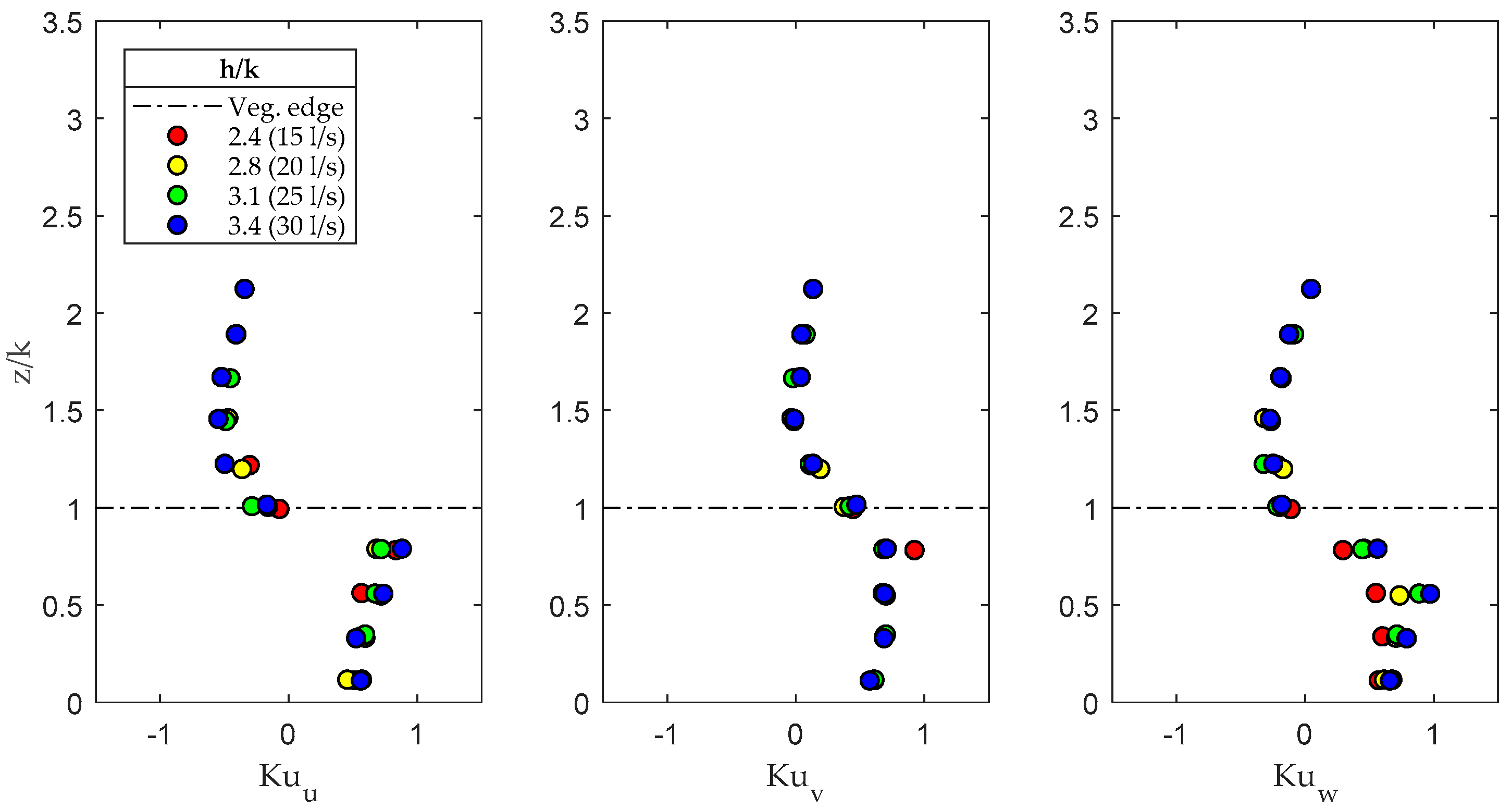
3.3. Skewness and Kurtosis
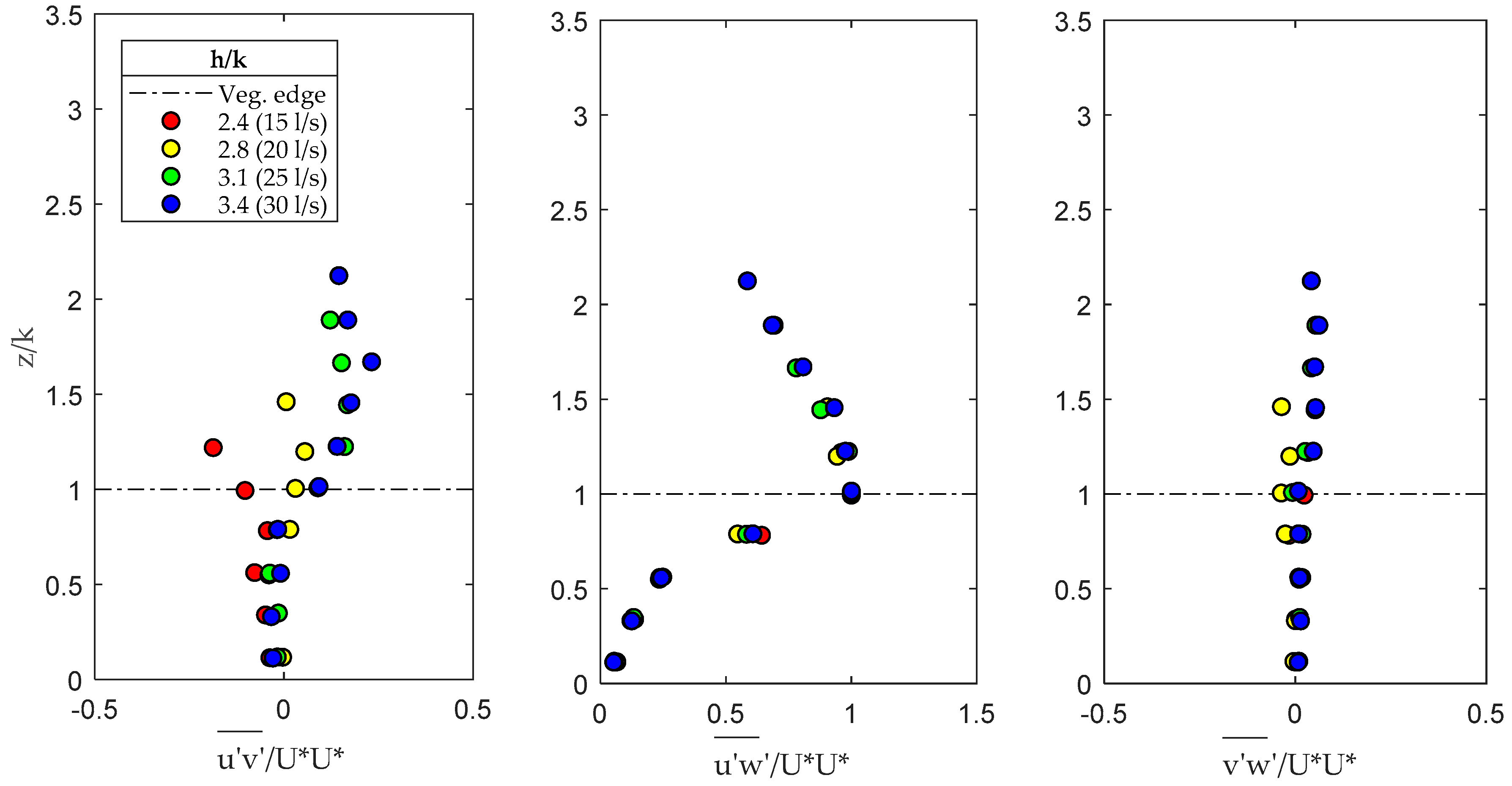
3.4. Reynolds Shear Stress
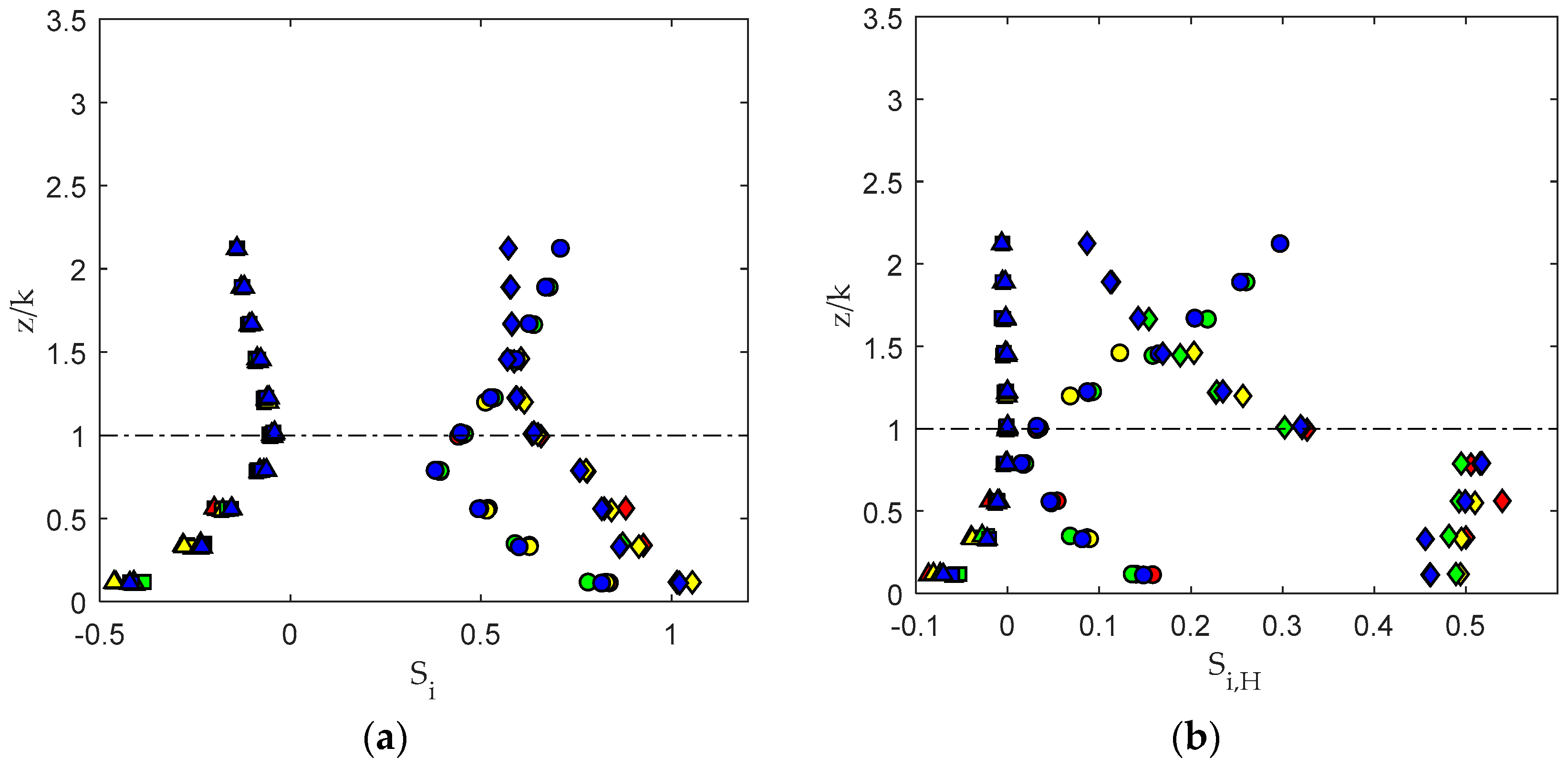
3.5. Quadrant Analysis
- quadrant 1: outward interaction ( > , > );
- quadrant 2: ejection ( < , > );
- quadrant 3: inward interaction ( < , < );
- quadrant 4: sweep ( > , < ).
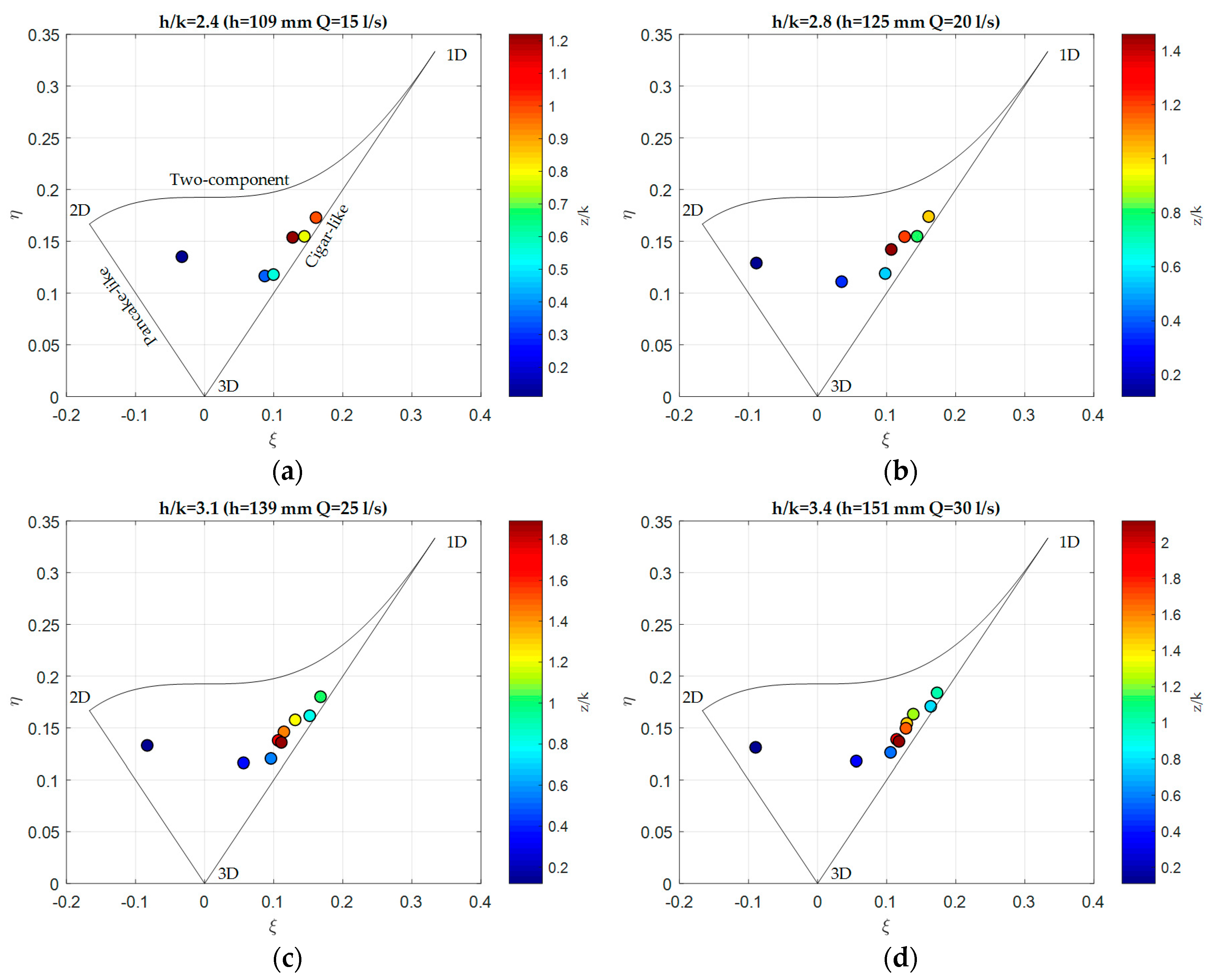
3.6. Turbulence Anisotropy
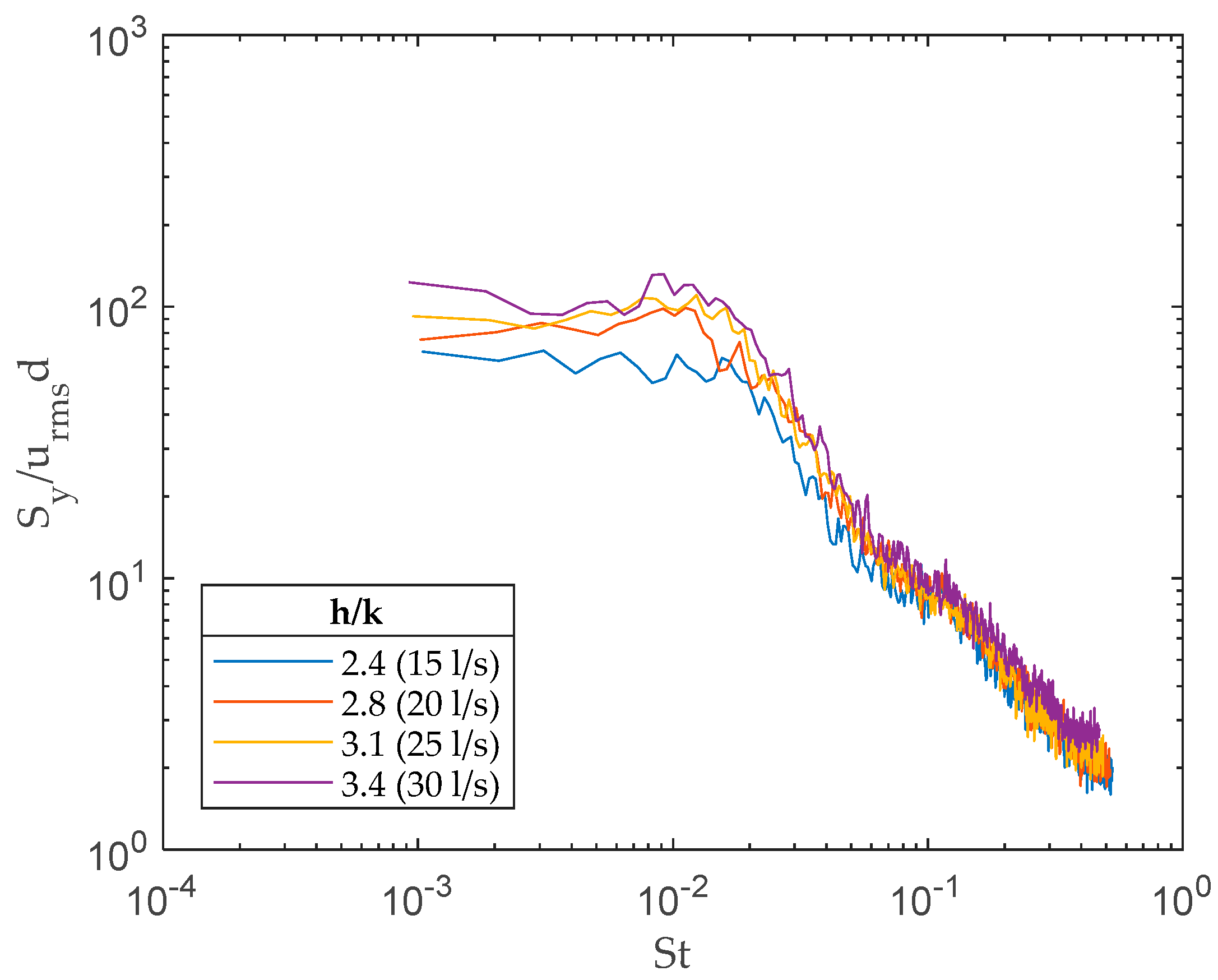
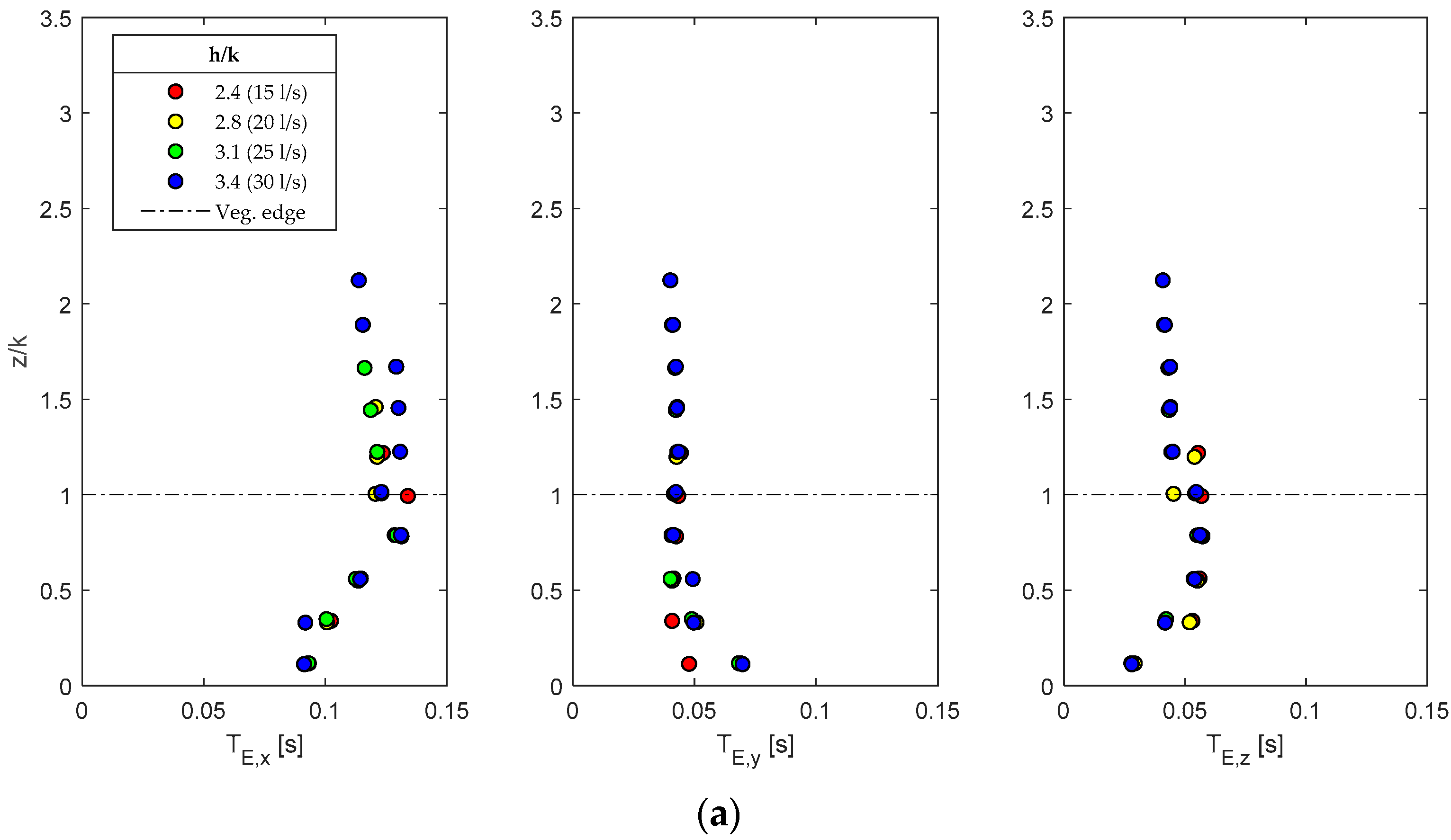
3.7. Integral Scales
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Järvelä, J.; Aberle, J.; Dittrich, A. Flow-vegetation-sediment interaction: Research challenges. In River Flow 2006, Proceedings of the 2006-Third International Conference Fluvial Hydraul, Lisbon, Portugal, 6–8 September 2006; Taylor & Francis: New York, NY, USA, 2006; pp. 2017–2026. [Google Scholar]
- Nepf, H.M. Flow and transport in regions with aquatic vegetation. Annu. Rev. Fluid Mech. 2012, 44, 123–142. [Google Scholar] [CrossRef]
- Tabacchi, E.; Lambs, L.; Guilloy, H. Impacts of riparian vegetation. Hydrol. Process. 2000, 14, 2959–2976. [Google Scholar] [CrossRef]
- Gurnell, A. Plants as river system engineers. Earth Surf. Process. Landf. 2013, 40, 135–137. [Google Scholar] [CrossRef]
- Poggi, D.; Porporato, A.; Ridolfi, L.; Albertson, J.D.; Katul, G.G. The effect of vegetation density on canopy sub-layer turbulence. Boundary-Layer Meteorol. 2004, 111, 565–587. [Google Scholar] [CrossRef]
- Nezu, I.; Sanjou, M. Turburence structure and coherent motion in vegetated canopy open-channel flows. J. Hydro-Environ. Res. 2008, 2, 62–90. [Google Scholar] [CrossRef]
- Rowiński, P.M.; Kubrak, J. A mixing-length model for predicting vertical velocity distribution in flows through emergent vegetation. Hydrol. Sci. J. 2002, 47, 893–904. [Google Scholar] [CrossRef]
- Ghisalberti, M.; Nepf, H.M. Mixing layers and coherent structures in vegetated aquatic flows. J. Geophys. Res. 2002, 107, 1–11. [Google Scholar] [CrossRef]
- Sukhodolova, T.A.; Sukhodolov, A.N. Vegetated mixing layer around a finite-size patch of submerged plants: Part 1. Theory and field experiments. Water Resour. Res. 2012, 48, 1–16. [Google Scholar] [CrossRef]
- Aberle, J.; Järvelä, J. Flow resistance of emergent rigid and flexible floodplain vegetation. J. Hydraul. Res. 2013, 51, 33–45. [Google Scholar] [CrossRef]
- Västilä, K.; Järvelä, J. Modeling the flow resistance of woody vegetation using physically based properties of the foliage and stem. Water Resour. Res. 2014, 50, 229–245. [Google Scholar] [CrossRef]
- Caroppi, G.; Västilä, K.; Järvelä, J.; Rowiński, P.M. Experimental investigation of turbulent flow structure in a partially vegetated channel with natural-like flexible plant stands. In New Challenges in Hydraulic Reseearch and Engineering, Proceedings of the 5th IAHR Europe Congress, Trento, Italy, 12–14 June 2018; Armanini, A., Nucci, E., Eds.; Taylor & Francis: New York, NY, USA, 2018; pp. 615–616. [Google Scholar]
- Vargas-Luna, A.; Crosato, A.; Calvani, G.; Uijttewaal, W.S.J. Representing plants as rigid cylinders in experiments and models. Adv. Water Resour. 2016, 93, 205–222. [Google Scholar] [CrossRef]
- Devi, T.B.; Kumar, B. Experimentation on submerged flow over flexible vegetation patches with downward seepage. Ecol. Eng. 2016, 91, 158–168. [Google Scholar] [CrossRef]
- Peruzzo, P.; de Serio, F.; Defina, A.; Mossa, M. Wave height attenuation and flow resistance due to emergent or near-emergent vegetation. Water 2018, 10, 402. [Google Scholar] [CrossRef]
- Viero, D.P.; Pradella, I.; Defina, A. Free surface waves induced by vortex shedding in cylinder arrays. J. Hydraul. Res. 2017, 55, 16–26. [Google Scholar] [CrossRef]
- Coscarella, F.; Servidio, S.; Ferraro, D.; Carbone, V.; Gaudio, R. Turbulent energy dissipation rate in a tilting flume with a highly rough bed. Phys. Fluids 2017, 29, 085101. [Google Scholar] [CrossRef]
- Raupach, M.R.; Thom, A.S. Turbulence in and above Plant Canopies. Annu. Rev. Fluid Mech. 1981, 13, 97–129. [Google Scholar] [CrossRef]
- Ghannam, K.; Poggi, D.; Porporato, A.; Katul, G.G. The Spatio-temporal Statistical Structure and Ergodic Behaviour of Scalar Turbulence Within a Rod Canopy. Boundary-Layer Meteorol. 2015, 157, 447–460. [Google Scholar] [CrossRef]
- Gualtieri, P.; De Felice, S.; Pasquino, V.; Doria, G.P. Use of conventional flow resistance equations and a model for the Nikuradse roughness in vegetated flows at high submergence. J. Hydrol. Hydromech. 2018, 66, 107–120. [Google Scholar] [CrossRef]
- Ghannam, K.; Katul, G.G.; Bou-Zeid, E.; Gerken, T.; Chamecki, M. Scaling and similarity of the anisotropic coherent eddies in near-surface atmospheric turbulence. J. Atmos. Sci. 2018, 75, 943–964. [Google Scholar] [CrossRef]
- Pope, S.B. Turbulent Flows; Cambridge University Press: Cambridge, UK, 2000; pp. 88–89. [Google Scholar]
- Lumley, J.L.; Newman, G.R. The return to isotropy of homogeneous turbulence. J. Fluid Mech. 1977, 82, 161–178. [Google Scholar] [CrossRef]
- Smalley, R.; Leonardi, S.; Antonia, R.A.; Djenidi, L.; Orlandi, P. Reynolds stress anisotropy of turbulent rough wall layers. Exp. Fluids 2002, 33, 31–37. [Google Scholar] [CrossRef]
- Bomminayuni, S.; Stoesser, T. Turbulence statistics in an open-channel flow over a rough bed. J. Hydraul. Eng. 2011, 137, 1347–1358. [Google Scholar] [CrossRef]
- Ghisalberti, M.; Nepf, H.M. The structure of the shear layer in flows over rigid and flexible canopies. Environ. Fluid Mech. 2006, 6, 277–301. [Google Scholar] [CrossRef]
- Choi, K.S.; Lumley, J.L. The return to isotropy of homogeneous turbulence. J. Fluid Mech. 2001, 436, 161. [Google Scholar] [CrossRef]
- Nepf, H.M. Hydrodynamics of vegetated channels. J. Hydraul. Res. 2012, 50, 262–279. [Google Scholar] [CrossRef]
- Augustijn, D.C.M.; Huthoff, F.; Van Velzen, E.H. Comparison of vegetation roughness descriptions. In Proceedings of the Fourth International Conference Fluvial Hydraul, River Flow 2008, Cesme, Turkey, 3–5 September 2008; p. 9. [Google Scholar]
- Nepf, H.M.; Vivoni, E.R. Flow structure in depth-Iimited, vegetated flow. J. Geophys. Res. 2000, 105, 28547–28557. [Google Scholar] [CrossRef]
- Doroudian, B.; Hurther, D.; Lemmin, U. Discussion of “Turbulence measurements with acoustic doppler velocimeters” by Carlos M. Garcia, Mariano I. Cantero, Yarko Nino, and Marcelo H. Garcia. J. Hydraul. Eng. 2007, 133, 1283–1288. [Google Scholar] [CrossRef]
- Goring, D.G.; Nikora, V.I. Despiking acoustic doppler velocimeter data. J. Hydraul. Eng. 2002, 128, 117–126. [Google Scholar] [CrossRef]
- Wahl, T.L. Discussion of “Despiking acoustic doppler velocimeter data” by Derek G. Goring and Vladimir I. Nikora January. J. Eng. Mech. 2003, 128, 2002–2003. [Google Scholar]
- Raupach, M.R.; Finnigan, J.J.; Brunei, Y. Coherent eddies and turbulence in vegetation canopies: The mixing-layer analogy. Boundary-Layer Meteorol. 1996, 78, 351–382. [Google Scholar] [CrossRef]
- Nepf, H.M.; Ghisalberti, M.; White, B.L.; Murphy, E. Retention time and dispersion associated with submerged aquatic canopies. Water Resour. Res. 2007, 43, 1–10. [Google Scholar] [CrossRef]
- Lu, S.S.; Willmarth, W.W. Measurements of the structure of the Reynolds stress in a turbulent boundary layer. J. Fluid Mech. 1973, 60, 481–511. [Google Scholar] [CrossRef]
- Wallace, J.M.; Brodkey, R.S. Reynolds stress and joint probability density distributions in the u-v plane of a turbulent channel flow. Phys. Fluids 1977, 20, 351–355. [Google Scholar] [CrossRef]
- Nakagawa, H.; Nezu, I. Prediction of the contributions to the Reynolds stress from bursting events in open-channel flows. J. Fluid Mech. 1977, 80, 99. [Google Scholar] [CrossRef]
- Helgeland, A.; Andreassen, O.; Ommundsen, A.; Reif, B.A.P.; Werne, J.; Gaarder, T. Visualization of the Energy-Containing Turbulent Scales. In Proceedings of the IEEE Symposium on Volume Visualization and Graphics, Austin, TX, USA, 12 October 2004; pp. 103–109. [Google Scholar]
- Emory, M.; Iaccarino, G. Visualizing turbulence anisotropy in the spatial domain with componentality contours. Cent. Turbul. Res. Annu. Res. Briefs 2014, 123–138. [Google Scholar]
- Nepf, H.M.; Sullivan, J.A.; Zavistoski, R.A. A model for diffusion within emergent vegetation. Limnol. Oceanogr. 1997, 42, 1735–1745. [Google Scholar] [CrossRef]
- Zong, L.; Nepf, H.M. Vortex development behind a finite porous obstruction in a channel. J. Fluid Mech. 2012, 691, 368–391. [Google Scholar] [CrossRef]
- Beckett, P.M. Physical Fluid Dynamics By D. J. Tritton; Oxford University Press: New York, NY, USA, 1988, pp. 519. ISBN 0–19–854489-8 (hardback); 0–19–854493-6 (paperback). J. Chem. Technol. Biotechnol. 1991, 50, 144. [Google Scholar] [CrossRef]
- O’Neill, P.L.; Nicolaides, D.; Honnery, D.R.; Soria, J. Autocorrelation Functions and the Determination of Integral Length with Reference to Experimental and Numerical Data. In Proceedings of the 15th Australas Fluid Mech Conference, Sydney, Australia, 13–17 December 2004; pp. 1–4. [Google Scholar]
- Gualtieri, P.; De Felice, S.; Pasquino, V.; Pulci Doria, G. Evaluation of integral length scale from experimental data in a flow through submerged vegetation. In Proceedings of the XXXVI IAHR Congress, Delft–The Hague, The Netherlands, 28 June–3 July 2015; pp. 1–9. [Google Scholar]
- Brunet, Y.; Finnigan, J.J.; Raupach, M.R. A wind tunnel study of air flow in waving wheat: Single-point velocity statistics. Boundary-Layer Meteorol. 1994, 70, 95–132. [Google Scholar] [CrossRef]




| Vegetation Type | (mm) | (—) | (m−1) |
|---|---|---|---|
| Current vegetation model | 4 | 0.02 | 6.4 |
| Marsh grasses | 1–10 | 0.001–0.01 | 1–7 |
| Mangroves | 40–90 | ≤0.45 | ≤2 |
| Seagrasses | — | 0.01–0.1 | 1–100 |
| 15 | 10.9 | 2.4 | 29.2 | 5.4 | 34.56 | 13,121 | 37,500 | 218 | 0.34 |
| 20 | 12.5 | 2.8 | 32.3 | 6.3 | 40.00 | 14,517 | 50,000 | 254 | 0.36 |
| 25 | 13.9 | 3.1 | 35.4 | 7.2 | 44.96 | 15,916 | 62,500 | 290 | 0.39 |
| 30 | 15.1 | 3.4 | 37.2 | 7.9 | 49.54 | 16,747 | 75,000 | 316 | 0.41 |
| (—) | (mm) |
|---|---|
| 2.4 | 35 |
| 2.8 | 33 |
| 3.1 | 34 |
| 3.4 | 33 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Caroppi, G.; Gualtieri, P.; Fontana, N.; Giugni, M. Vegetated Channel Flows: Turbulence Anisotropy at Flow–Rigid Canopy Interface. Geosciences 2018, 8, 259. https://doi.org/10.3390/geosciences8070259
Caroppi G, Gualtieri P, Fontana N, Giugni M. Vegetated Channel Flows: Turbulence Anisotropy at Flow–Rigid Canopy Interface. Geosciences. 2018; 8(7):259. https://doi.org/10.3390/geosciences8070259
Chicago/Turabian StyleCaroppi, Gerardo, Paola Gualtieri, Nicola Fontana, and Maurizio Giugni. 2018. "Vegetated Channel Flows: Turbulence Anisotropy at Flow–Rigid Canopy Interface" Geosciences 8, no. 7: 259. https://doi.org/10.3390/geosciences8070259
APA StyleCaroppi, G., Gualtieri, P., Fontana, N., & Giugni, M. (2018). Vegetated Channel Flows: Turbulence Anisotropy at Flow–Rigid Canopy Interface. Geosciences, 8(7), 259. https://doi.org/10.3390/geosciences8070259







