The Influence of Pre-Existing Tension Cracks on the Stability of Unsupported Temporary Excavations in Stratified Hard Clays: Case Study of Corfu Island, Northwestern Greece

Abstract

Slope failures in overconsolidated hard clays present significant geotechnical challenges, particularly in stratified formations prone to pre-existing discontinuities. Despite extensive research on residual shear strength and fissuring in stiff clays, the role of undetected tension cracks and their interaction with hydrogeological conditions in temporary excavations remains underexplored. This study addresses this research gap through a detailed case study of a slope failure during an unsupported residential excavation on Corfu Island, Greece. The investigation aimed to identify the failure mechanism, assess the influence of geological discontinuities and groundwater conditions, and evaluate the contribution of residual shear strength to slope stability. The methodology combined field observations, laboratory testing (including unconfined compression and ring shear tests), and numerical modelling using both finite element (FEM) and limit equilibrium (LEM) approaches. The results revealed that a nearly vertical, pre-existing fissure—acting as a tension crack—and water infiltration along the clay–sandstone interface significantly reduced the factor of safety, triggering a planar slide. Both FEM and LEM analyses indicated that critical conditions for failure were reached with a residual friction angle of 19°, inclined sandstone layers at 15–17°, and hydrostatic pressure from groundwater accumulation. This study demonstrates the compounded destabilizing effects of undetected discontinuities and water pressures in stratified hard clays and underscores the necessity of comprehensive geotechnical assessments for temporary excavations, even in seemingly stable formations.

1. Introduction

Slope failures in overconsolidated hard clays are of great interest due to their impact on numerous infrastructure and geotechnical projects. The Factor of Safety (FS) against sliding is a critical consideration, both in slope design and in the investigation of slope failures. Shear strength (τ) is a fundamental geotechnical parameter essential for analyzing the stability of such slopes. Additionally, drained residual shear strength (τ_r) plays a crucial role in the stability of stratified hard clay slopes when examining pre-existing tension cracks [1,2].

Residual shear strength, along with fully softened shear strength, is used to determine the factor of safety against first-time sliding in stiff plastic clay slopes [3,4,5,6,7]. The studies by Skempton and Hutchinson [8] and Skempton [9] indicate that fissures in overconsolidated hard clays weaken the surrounding soil mass, creating planes of weakness. The presence of these fissures significantly reduces the soil’s shear strength, increasing the risk of slope instability.

Barnes [10] notes that the shear strength of fissured overconsolidated clays can be as low as 10% of their intact strength. This reduction is attributed to soil dilation and increased water content under shear stress. Skempton [9] suggests that shear strength within a fissure corresponds to the fully softened condition. Additionally, Mesri and Shahien [7] and Castellanos et al. [11] propose that first-time slope failures in overconsolidated clays often involve sections of the slip surface reaching the residual condition, particularly in excavated slopes where progressive deformation occurs along horizontal discontinuities.

Residual shear strength is also related by various geotechnical and geological parameters, such as the vertical effective stress on the potential sliding surface [2,12,13,14], soil type and classification [1,2,15,16,17], soil plasticity [17,18,19], mineralogy, the shape and orientation of clay particles [16,17,20,21], the chemistry of the pore water [22,23], the degree of saturation [24], as well as shear deformation rates [25].

From the above, it is clear that understanding the mechanics of slope failures in overconsolidated hard clays with complex stratigraphy is both challenging and essential for the design and safety of excavation projects.

The current study investigates a specific case of slope failure in overconsolidated hard clays during an excavation for a residential construction project on Corfu Island in Northwestern Greece. The excavation reached a depth of up to 4.50 m from the ground surface and a soil mass was detached on the southern slope of the excavation. Prior to this incident, similar temporary excavations were conducted without support, in shallower depths, following standard construction practices.

The excavation was performed in hard, stratified overconsolidated clays interbedded with thin sandstone layers. The local regulatory framework did not mandate a geotechnical assessment for temporary excavations, and the excavation was therefore unsupported. This lack of prior investigation contributed to the unexpected failure, underscoring the importance of understanding the influence of soil strength, groundwater conditions, and excavation depth on slope stability.

Subsequent investigations focused on identifying the failure mechanism were carried out by analyzing various contributing factors, including the role of excavation depth, soil properties, groundwater conditions, and the effect of surcharges from the excavated material deposits. The primary factor influencing stability was the presence of nearly vertical fissures behind the excavated slope, which were not identifiable before excavation. These fissures acted as tension cracks and, when combined with hydrostatic forces along the failure plane—formed at the geological contact between the clay and sandstone layers—led to a planar slide of a soil mass, which, in the analysis performed, is considered a hard soil rigid body.

The main objectives of the current study are to analyze the mechanisms of slope failure in overconsolidated hard clays, particularly within stratified geological formations containing pre-existing fissures; to investigate a specific real-world case of slope failure that occurred during a residential excavation project on Corfu Island, Greece; to assess the role of residual and fully softened shear strength in slope stability, especially under conditions involving fissured and stratified hard clays; to identify key contributing factors to the failure, such as excavation depth, the presence of fissures, groundwater conditions, and surcharges from excavated material; and finally, to highlight the importance of conducting thorough geotechnical investigations prior to excavation, particularly in areas characterized by complex soil and geological conditions.

The research questions under investigation are as follows: What geotechnical and geological factors contributed to the unexpected slope failure in overconsolidated hard clays during the Corfu Island excavation? How do fissures and pre-existing discontinuities influence the shear strength and stability of stratified hard clay soils? And to what extent do excavation depth and the presence of groundwater affect the factor of safety against sliding in stiff clay formations?

The novelty of this study lies in its detailed examination of a real-world case of slope failure in hard clays during a routine residential excavation—an event that is seldom documented in depth within the literature. It brings to attention the critical influence of stratified and fissured clay formations, which are often overlooked in conventional geotechnical practice, particularly in areas with limited regulatory oversight for temporary excavations. This study provides new insights into a combined failure mechanism involving vertical fissures (tension cracks) and hydrostatic pressures, contributing to a more comprehensive understanding of planar slides in stratified hard clays. By bridging the established theoretical frameworks with actual field observations, this study enhances the practical application of residual shear strength concepts and underscores the need for stricter regulatory measures in geotechnical assessments for temporary excavations.

2. Geological and Geotechnical Conditions

2.1. Location and Regional Geological Setting

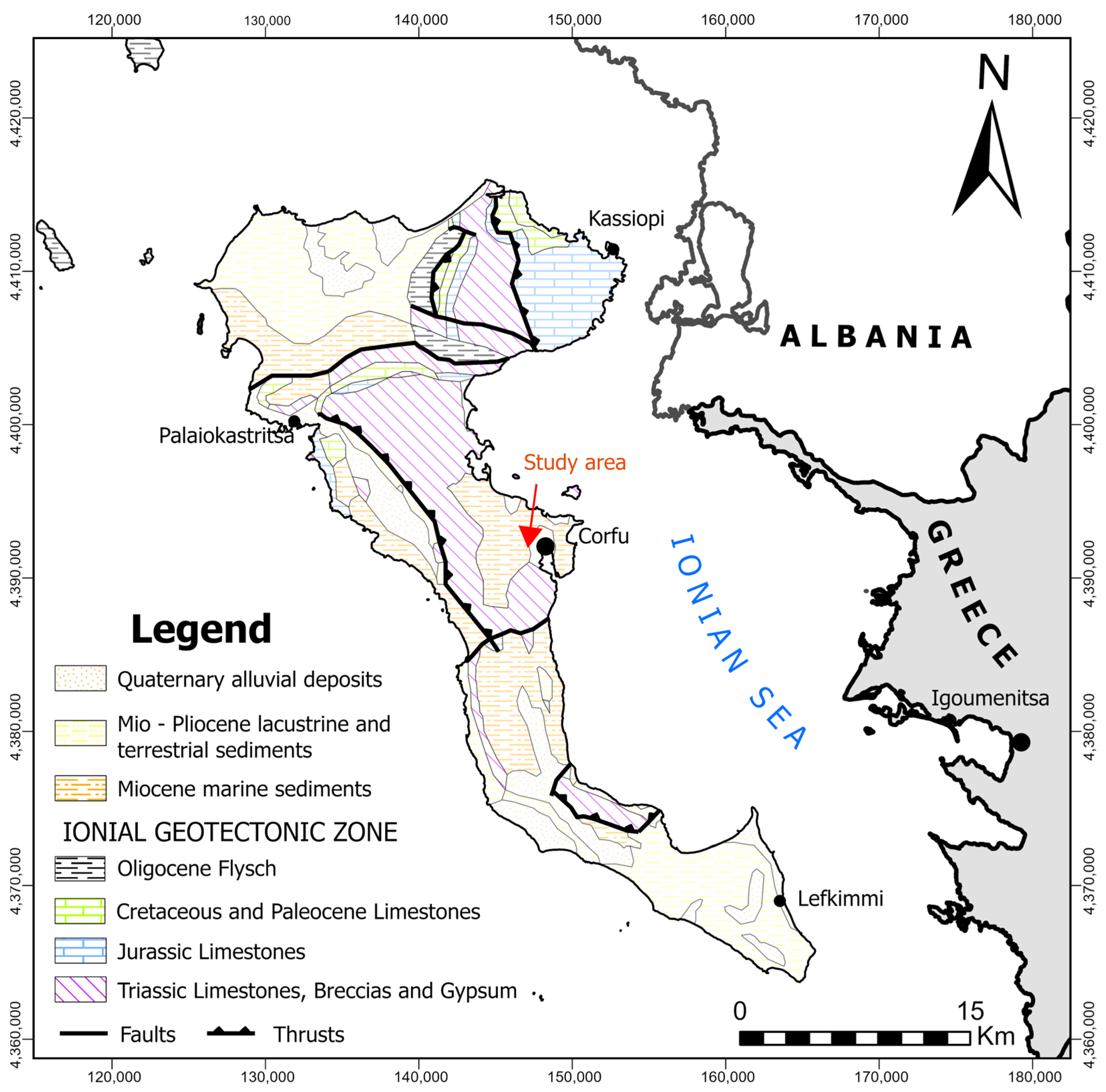
The island of Corfu in Northwestern Greece is mainly covered by post-alpine geological formations (Quaternary deposits and Miocene–Pliocene sediments) as shown in the simplified geological map of Figure 1. The geological map presented in Figure 1 was created after digital processing of the three geological sheets concerning the island of Corfu, which have been published by the Institute of Geological and Mineral Exploration (IGME) of Greece [26,27,28]. The processing and design of the map was performed using the ArcGIS Pro v3.3 software.

In Corfu, the alpine formations of the Ionian geotectonic unit dominate throughout the island, comprising a carbonate series, which starts with the typical Triassic formation of limestones, followed by Triassic breccia and gypsum. The Triassic formations are overlaid by Jurassic–Cretaceous formations of limestones with cherts and shales, and flysch of the Oligocene age. Regarding the post-alpine molassic formations above the Ionian unit, a sequence of marls alternating with breccia, conglomerates, and sandstones can be observed. The base of the breccia is located at the lower horizons of the sequence, while a package of marls with interbedding sandstone and conglomerates overlies them. The sequence of marls continues with sandstones, breccia, and conglomerates until the Pliocene layer. Alluvial deposits are placed in unconformity either on the Miocene and Plio-Pleistocene layers or the Alpine formations [26,27,28].

The study area is located approximately 1 km from Corfu town and its geology consists of Miocene clayey-marly deposits interlayered with sandstone beds which dip gently (up to 15°) towards the N-NE (Figure 1).

2.2. Local Geotechnical and Hydrogeological Conditions

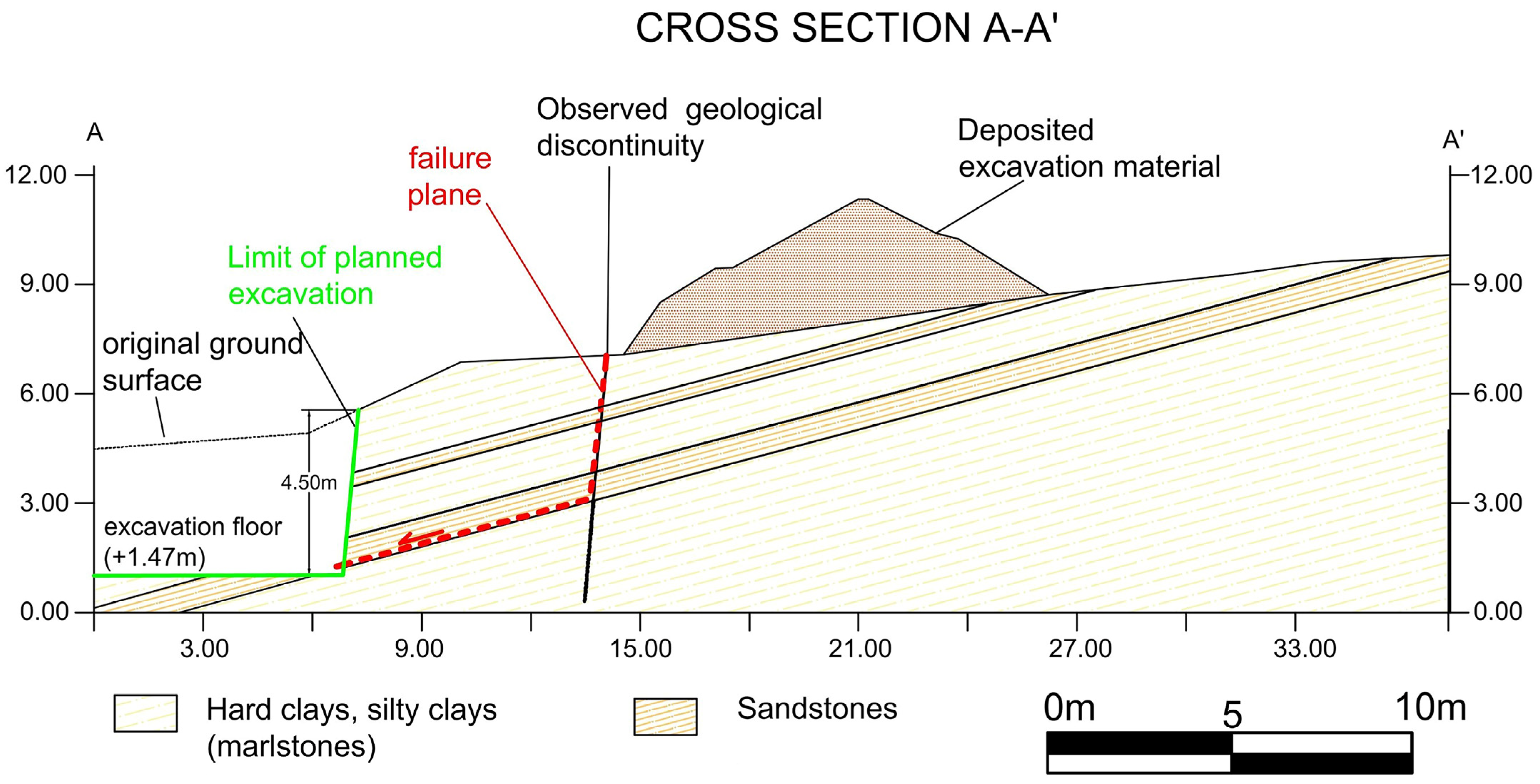
For residential building construction and based on the approved construction drawings, soil excavation from an absolute elevation of +5.35 m to +2.25 m (maximum depth of 3.10 m) was required. The existing ground level within the excavation area exhibits unevenness, with its southern side being 1.70 m higher than its northern side. Towards the end of the excavation works, a translational movement of soil mass from the southern front of the excavation (approximately 25 m long) as well as a slide of the soil mass in a SW-NE direction by 3.80 m, approximately, occurred.

The prevailing geotechnical conditions of the area of study consisted of hard, stratified overconsolidated clays interbedded with thin inclined sandstone layers in the SW-NE direction (the angles of the sandstone layers ranged between 15 and 17° and had a maximum thickness of 20 cm), with no history of soil instability.

A plan view of the study area showing the outline of the excavation area, the boundary of the ground failure, the detached soil mass, the excavated material disposal area, the sampling locations and the photographic positions are shown in Figure 2, while a photograph of the detached soil mass is shown in Figure 3.

The post-failure investigations revealed the presence of nearly vertical fissures, which created an unfavorable discontinuity pattern, significantly reducing soil mass strength. Moreover, a post-failure topographical survey showed that the actual excavation depth exceeded the planned depth by 0.65–0.80 m (an average of 0.73 m, i.e., an elevation of +1.47 m), reaching a maximum depth of 4.5 m below ground level.

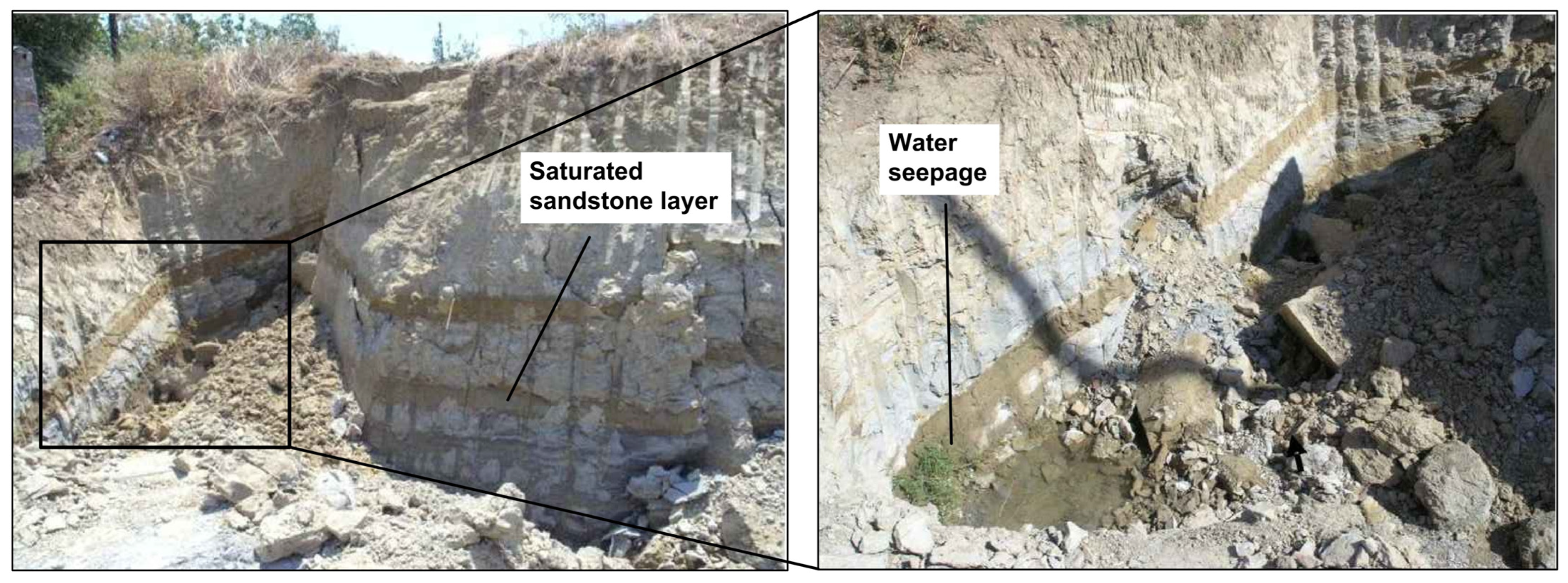
Apart from the unfavorable discontinuity pattern, instability was also influenced by the development of hydrostatic forces along the failure plane. Although no groundwater accumulation was observed during excavation, water ponding (up to 35 cm) was noted five days after the failure. Increased moisture was also recorded in the sandstone layer, 2–3 m below ground level, with saturation increasing with depth. Post-failure groundwater monitoring indicated that the phreatic level was 35 cm above the excavation level (+1.47 m), suggesting that if the excavation depth had remained at the designed level, water inflow might have been avoided (Figure 4).

3. Methods

3.1. Geotechnical Investigation and Laboratory Tests

To support the development of a geotechnical model for the excavation and the failed soil mass, block samples were collected from two locations—on the north and east sides of the excavation area—comprising both hard clay and sandstone layers (Figure 2). Due to the strength of the soil at the sampling locations, block detachment was only possible after loosening with hammering. Sampling was performed following the ASTM D7015 [29]-recommended procedure and soil laboratory tests were carried out. The main aim of the laboratory tests was to define the shear strength parameters in the large deformation (residual strength) of the involved clayey formation.

The soil classification test results are presented in

Table 1. Soil classification test results.

Location/Sample	Depth (m)	w (%)	Atterberg Limits			γb (kN/m3)	γd (kN/m3)	Sieve Analysis			Soil Classification U.S.C.S
			LL (%)	PL (%)	PI (%)			Gravel (%)	Sand (%)	Fines (%) *
Location 1 Sample 1	3.80	16.7	45	23.5	21.5	22.0	19.0	0	2	98 (36)	CL
Location 2 Sample 4	2.50	22.5	47	26	21	20.0	17.0	0	13	87 (35)	CL
Location 2 Sample 5	3.00	23.5	48	29	19	20.0	16.5	0	4	96 (36)	ML
Location 2 Sample 6	3.00	19.0	43	24	19	21.0	17.5	0	0	100 (35)	CL
Location 2 Sample 7	3.00	18.5	35	30	5	21.9	18.48	0	68	32	SM
Location 2 Sample 3	2.50	19.2	43	36	7	20.1	16.86	0	70	30	SM
Location 1 Sample 2	3.80	17.6	45	36	9	21.8	18.54	0	55	45	SM

* Values in () indicate silt and clay content.

, including moisture content (w, %) following the ASTM D2216 [30]-recommended procedure, a grain size analysis following the ASTM D422-63 [31]- and ASTM D7928-21 [32]-recommended procedures, and Atterberg limits following the ASTM D4318-17 [33]-recommended procedure. All samples were classified according to the Unified Soil Classification System (USCS), which revealed inorganic grayish brown to bluish gray clays of low to average plasticity (CL), as well as inorganic silts (ML) and silty sands (SM). Thin yellowish-brown sandstone layers were also interbedded between the clayey strata.

The moisture content in all clayey samples was lower than the Plasticity limit (PL), a typical characteristic of an overconsolidated and very stiff cohesive soil in a solid state. The average bulk unit weight of all clayey samples was equal to γ_b = 20.5 kN/m³, the specific gravity was G_s = 2.75, and the void ratio was e = 0.570.

Three unconfined compressive strength tests were also undertaken in clays, following the ASTM D2166-16 [34]-recommended procedure, for the estimation of the unconfined compressive strength (q_u) of cohesive soils (

Table 2. Unconfined compressive strength test results of the clayey samples.

Location/Sample	Depth (m)	Unconfined Compressive Strength, (qu, kPa)	Strain (ε, %)
Location 2 Sample 4	2.50	512	2.46
Location 2 Sample 5	3.00	610	3.00
Location 2 Sample 6	3.00	512	2.94

). The average value of the unconfined compressive strength of the clayey samples was equal to q_u = 544 kPa, thus their undrained shear strength was equal to c_u = 272 kPa. All samples were retrieved from depths of 2.50 m to 3.0 m below ground level.

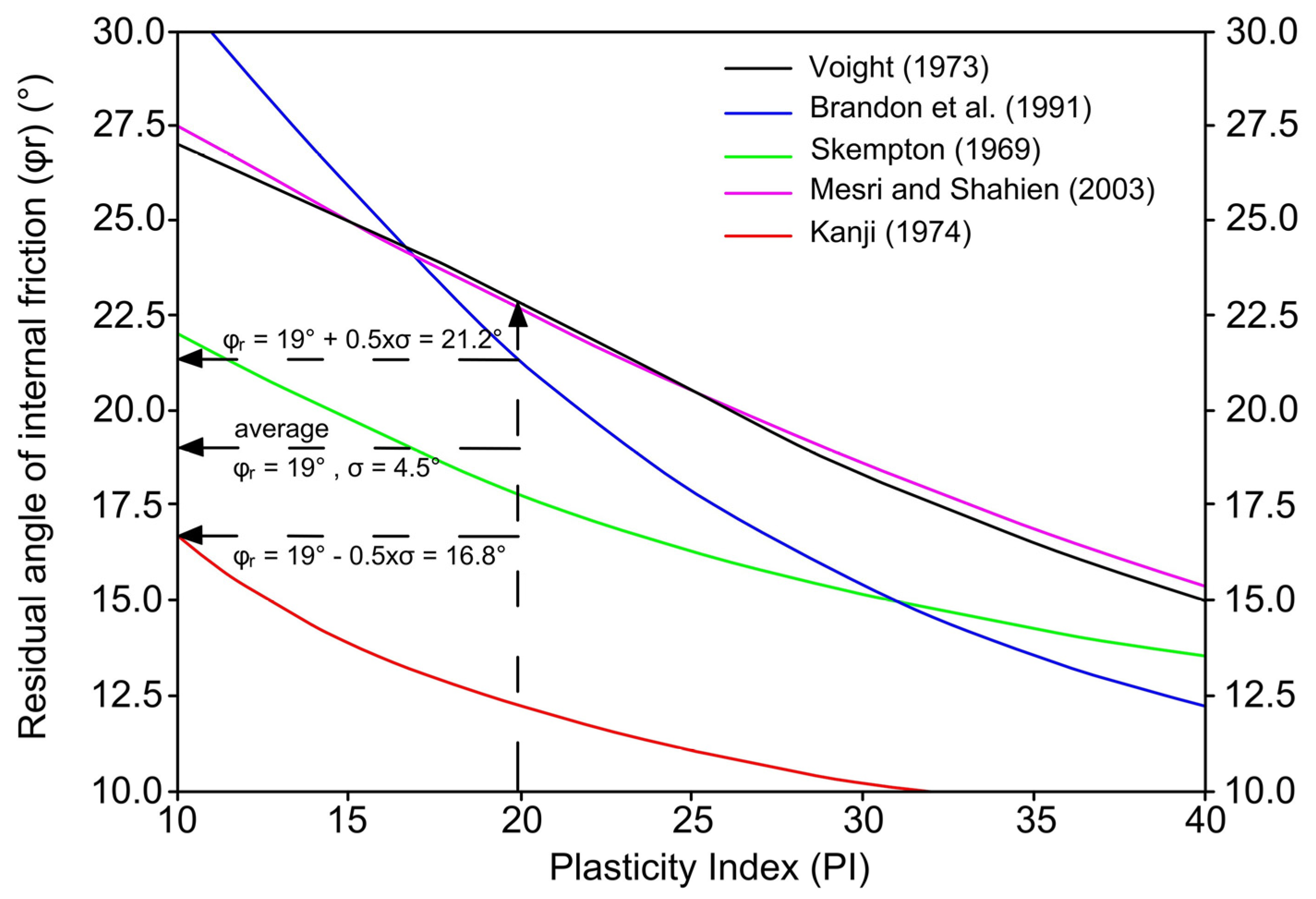
For the determination of the residual shear strength of the clayey samples, ring shear tests were performed using the Bromhead apparatus [35,36]. Three (3) steps of different normal stresses (30, 55, and 90 kPa) were applied in one sample (Location 2/Sample 4). Assuming an effective cohesion, c’ ≈ 0 kPa, the residual friction angle (φ’_r) was calculated with a value of φ’_r = 19°. The calculated residual friction angle was also compared with the residual friction angle values estimated from the empirical relationships using the Plasticity Index (PI) as shown in Figure 5.

By using the average measured PI value of 20%, the residual friction angle [7,8,37,38,39] was empirically estimated between φ’_r ≈ 12.2° and 22.8°. The mean estimated residual friction angle, as calculated from the above empirical relationships, was φ’_r,m = 19° with a standard deviation of σ = 4.5°, which is equal to the corresponding laboratory average value. Therefore, for slope stability analyses, a characteristic residual friction angle φ’_r of 19° was considered.

In addition to the ring shear tests, simple tilt tests were also performed in dry sand material produced from the grated sandstone samples to find the basic friction angle of the sandstones. The tilt tests showed that the basic friction angle of the sandstone was equal to approximately φ_b ≈ 31° (Figure 6).

The main outcomes from the executed laboratory tests were as follows:

• The average dry and bulk unit weight of the clayey material was γ_d = 17.5 kN/m³ and γ_b = 20.5 kN/m³, respectively.
• The undrained shear strength of the clayey material was c_u = 270 kPa, typical of a very stiff to hard overconsolidated cohesive soil [40,41].
• The average residual friction angle of the clayey material was φ_r = 19°, typical of hard overconsolidated clayey soils [40,41].
• The basic friction angle of the sandstone was estimated to be slightly higher than 30°.

3.2. Geotechnical Model for Stabiltiy Analysis

The type of shear failure mechanism (turbulent, transitional, sliding) depends on soil composition (clay percentage) and the value of the residual friction angle, φ’_r [42]. The expected failure mechanism based on the characteristic value of the residual friction angle φ’_r = 19°, and the clay percentage of approximately 35%, indicates a transitional movement of the soil mass. This mechanism is in very good agreement with the observed behavior which is characterized by an almost horizontal and “undisturbed” movement of the soil mass as shown in Figure 2.

To model the failure mechanism and identify the combination of geological, groundwater, and geotechnical parameters that contributed to the activation of the slide, slope stability analyses were carried out. For this purpose, a representative geotechnical cross-section of the affected area was designed (Figure 7), and slope stability analysis was undertaken by utilizing both the limit equilibrium and finite element methods, focusing on determining the failure mechanism and the relative contribution of the shear strength parameters. The material properties used in the analyses are presented in

Table 3. Material properties used in the stability analyses for an interface plane inclined at α = 17⁰.

Material	Unit Weight, (γ, kN/m3)	Friction Angle φ (°)	Undrained Shear Strength, (cu, kPa)
Hard clay prism	20.5	-	270
Clay–sandstone interface	-	19	-

.

3.3. Finite Element Analysis

A slope stability analysis was carried out using the finite element method (FEM), assuming non-linear behavior of the soil materials, using the Mohr–Coulomb failure criterion and plane strain conditions. For this purpose, PLAXIS 2D software, version 2024.1 [43] was used, which allows for the calculation of the safety factor (an indicator of the stability of the soil mass) using the phi/c reduction method, the distribution of stresses and strains in the soil mass, as well as the presentation of the vectors of the movements of the soil elements.

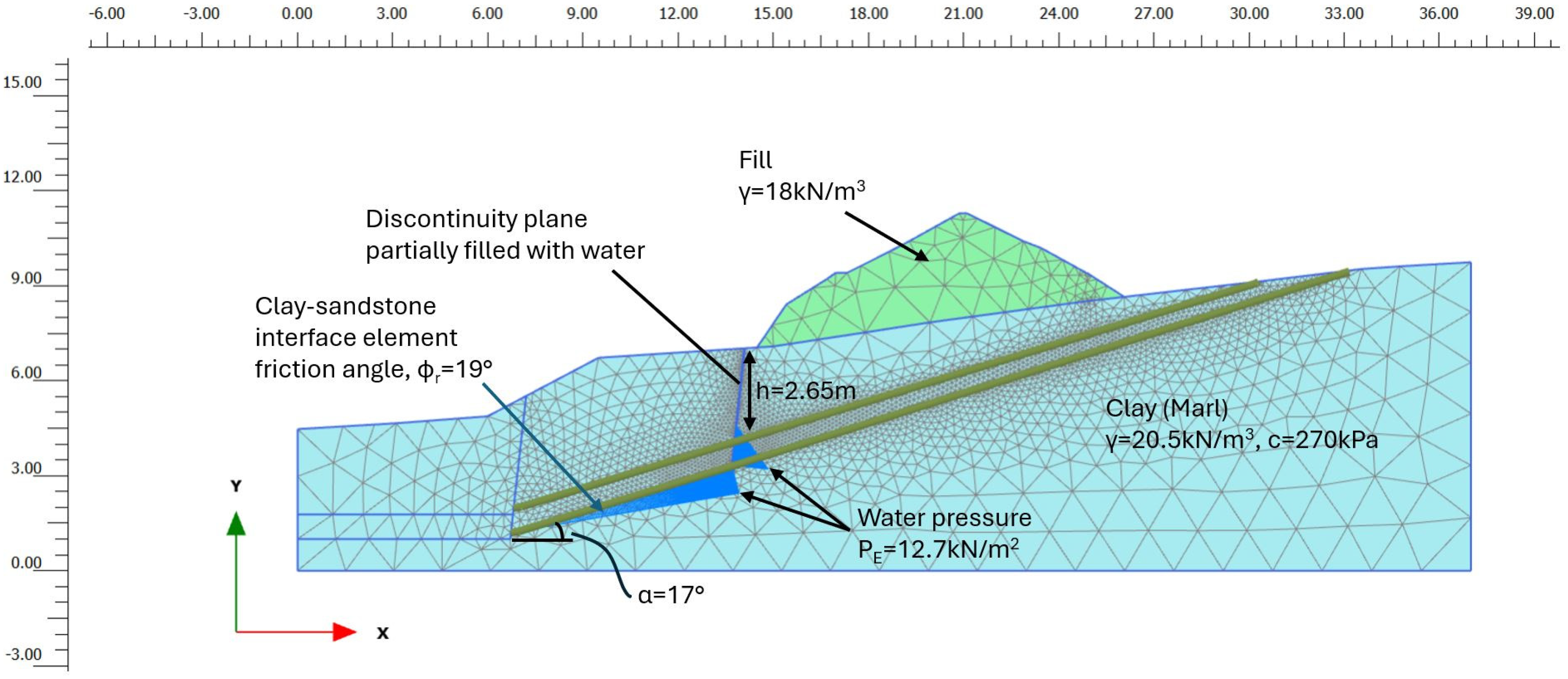
To simulate the relative displacement (slipping) between the sandstone and hard clay cluster, an interface element was used in Plaxis. The level at which slipping occurs is directly controlled by the strength properties and the coefficient R_inter, which is equal to the ${\displaystyle \frac{\mathrm{t}\mathrm{a}\mathrm{n}\left({\phi }_{int.}\right)}{tan{(\phi }_{soil})}}$ or ${\displaystyle \frac{c_{int.}}{c_{soil}}}$ value of the relevant material set. In Plaxis, these parameters are, by default, taken from the material set of the adjacent soil cluster (i.e., hard clay). In this study, a material set entitled “Clay–sandstone interface” was created and directly assigned to the interface allowing for direct control of the strength properties (and thus the interface strength) without changing the properties of the soil cluster (i.e., the hard clay). Therefore, the only input parameter required for the “clay–sandstone” interface element was the friction angle which corresponds to the residual friction of φ_r = 19°, and the coefficient R_inter = 1 was considered. The sliding prism consisted of hard clay for which unit weight (γ), undrained shear strength (c_u), and interface plane (α) parameters are summarized in Table 3.

The sandstone beds and discontinuity plane were considered to be partially filled with water to a depth of h below ground level (bgl). Sensitivity analyses were carried out to assess the water depth, h, that resulted in the sliding of the soil mass, i.e., FoS = 1. Sensitivity analyses indicate that a water depth, h, of 2.65 m bgl (i.e., water pressure at the bottom of the vertical discontinuity plane of 12.7 kN/m²) was required to cause the slope failure. The selection of the physical and mechanical parameters of the soil formations was based on the results of laboratory tests in combination with experience from similar soil formations. The Plaxis 2D model with characteristic soil properties is shown in Figure 8.

To investigate (a) the influence of groundwater within the sandstone beds and discontinuity plane on the stability of the sliding prism and (b) the angle, α, of the inclined sandstone layers ranging between 15° and 17°, the generation of a new mesh grid was required, and the development of several FE models was time consuming. Therefore, limit equilibrium analyses using a simplified model, which takes into account the soil prism as a rigid-plastic body, were carried out as described below.

3.4. Limit Equilibrium Analysis

The Limit Equilibrium Model (LEM) focused on finding the mobilized shear strength for slope failure initiation. The residual shear strength of the overconsolidated hard clay along the bedding contact with the sandstone layers was used, assuming saturated conditions of the hard clayey layers along the contact point with the sandstones as well as the presence of the nearly vertical discontinuity.

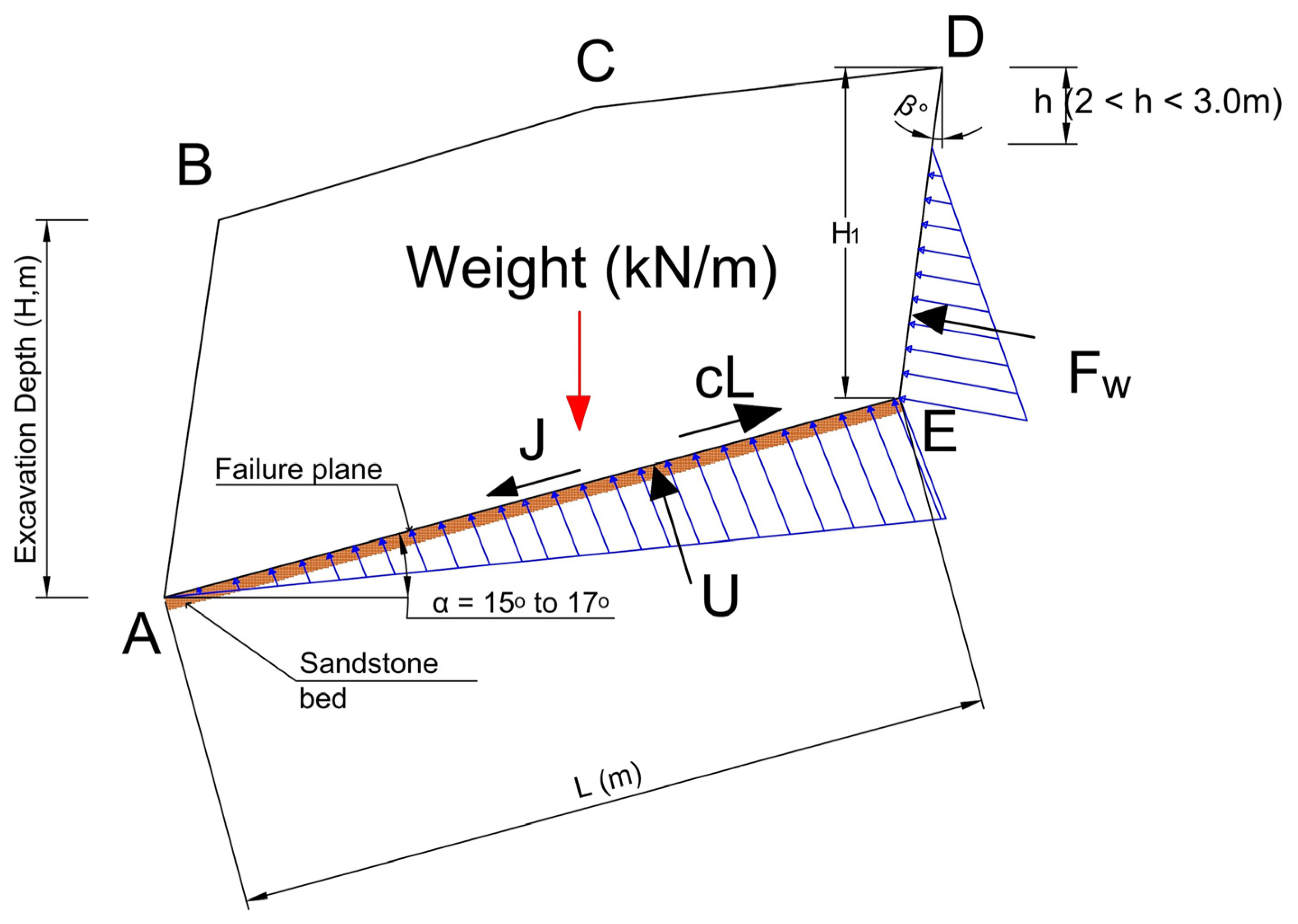
The stratified hard clays were directed parallel to the slope and dipped 15–17° towards the excavation site, satisfying the existing field conditions for triggering a planar failure of a soil block. Additionally, there was evidence that the nearly vertical discontinuity, trending at approximately 7 m south of the designed excavation limit and directing parallel to the slope, acted as a tension crack, which when filled with water influences the stability conditions. It is worth noting that the geological discontinuity was observed after the failure, since it was located outside the excavated property. Given that these preparatory conditions limit equilibrium, stability analyses were performed using the planar failure method with the presence of a tension crack filled with water behind the slope crest by using Equation (1), following the methodology of Hoek and Bray [44]. The incorporation of the vertical discontinuity in the slope model aimed to investigate the influence of the hydrostatic pressure within the sandstone layers on the overall stability of the sliding mass. The analysis assumed residual shear strength parameters at the “clay–sandstone” interface.

The geotechnical slope model considered a planar failure with the presence of a tension crack at 7 m from the slope crest, thus simulating the observed vertical discontinuity which was recorded at the wall of the detached soil mass. In this case, the Factor of Safety was calculated using Equation (1) and considering the model presented in Figure 9.

$$F.S.={\displaystyle \frac{c\times L+\{W\times cosa-\left[F_w\times \sin \left(\beta +a\right)+U\right\}\times tan\phi }{F_w\times \cos \left(b+a\right)+W\times sina+J}}$$

(1)

where

α is the slope failure plane angle;

β is the angle of the tension crack from the vertical fissures;

φ is the friction angle at the failure plane (residual friction angle φ_r of the clayey soil);

c represents cohesion between the failure plane and soil mass (c = 0);

L is the length of the failure plane (between points A and E);

W is the weight of the sliding mass (i.e., area ABCDE × soil unit weight);

U represents the total pore pressure force from the saturated sandstone bed with water outflow towards the slope face (i.e., from point E to point A);

J is the seepage force due to water flow through the sandstone layer;

F_w is the resultant hydrostatic force due to water filling in the tension crack.

4. Results

The presence of seepage forces (J) and pore water pressure (U) reduces stability by decreasing normal stress and increasing driving forces. Moreover, the hydrostatic force (F_w) due to the tension crack can either stabilize or destabilize depending on how much water fills the crack. A deep tension crack filled with water increases hydrostatic pressure (F_w), which adds to the driving force. If the crack is empty or drains freely, it reduces the weight of the wedge, improving stability. Seepage force (J) is due to water flowing through the permeable sandstone layer, exerting a downslope force. Water outflow through sandstone also generates pore water pressure (U), which reduces normal stress and frictional resistance.

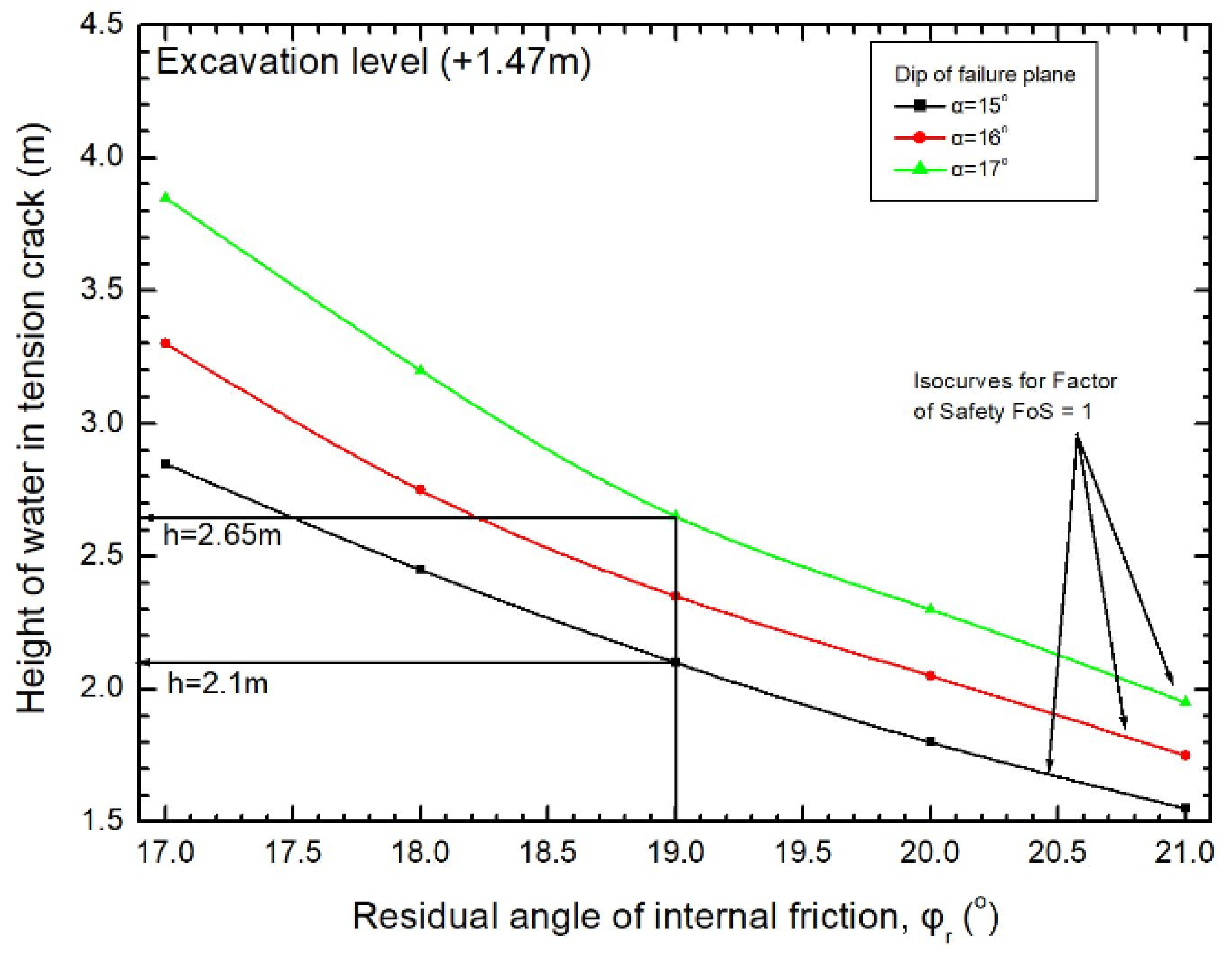
Considering the limit equilibrium analysis performed, the results regarding the relationship between the residual angle of friction, the hydrostatic force, and the angle of the slope failure plane are presented in Figure 10. The isocurves of the Factors of Safety (FS), ≈ 1.0, presenting the critical instability combinations are plotted for the original excavation level at +1.47 m (as-built excavation) with respect to the residual angle of friction (φ_r) and the height of the water (h) inside the tension crack.

As shown in Figure 10, for the +1.47-excavation level, the area shows potential instability combinations for (a) residual friction angles between 18.5° and 19.5° (average of 19°), (b) angles of the slope failure plane between 15° and 17° and (c) a water height in the tension crack between 2.1 m and 2.65 m below ground level (Figure 10). The above critical combination matches the field observations and geotechnical assessment presented in the previous sections.

The slope failure mechanism (i.e., FoS = 1), using Plaxis 2D [43], for the case of the inclined sandstone layers with an angle α = 17°, a residual friction angle of the clay–sandstone interface of φ_r = 19°, and a water depth in the tension crack of h = 2.65 m bgl, is presented in Figure 11. Even though the purpose of the analysis was not to examine any potential post-failure mechanism after the sliding of the hard clay prism, in an additional case including the fill (excavation products) material and removing the sliding prism downstream, vertical discontinuity was examined. The analysis indicated an FoS = 1.11, confirming that the FoS in this case is independent of the weight of the excavation products material.

The results indicate that both the FEM and LEM are identical since the only parameter affecting the results besides the water pressure (upward pore pressure force, U, and hydrostatic lateral force of tension crack, Fw) is the shear resistance along the clay–sandstone interface. It is thus concluded that the presence of a vertical discontinuity, parallel to the excavated slope face and partially filled with water, played a major role in the activation of slope failure. The exceedance of the planned excavation depth together with the presence of the undetected discontinuity, which acted as a tension crack and the seepage force parallel to the clay–sandstone interface reduced the expected factor of safety by 10%, triggering the slope failure.

5. Discussion

This study presents a detailed investigation into a slope failure incident in stratified, overconsolidated hard clays during an unsupported residential excavation on Corfu Island, Greece. The results clearly demonstrate that the mechanism of failure was primarily governed by a pre-existing, nearly vertical discontinuity acting as a tension crack, combined with the presence of hydrostatic pressure along the clay–sandstone interface. This discontinuity, initially hidden and located just outside the original excavation limits, acted as a critical plane of weakness. When filled with water, it significantly reduced the effect of normal stress on the failure surface, thereby lowering the factor of safety to critical levels. The movement of the soil mass was translational, with limited internal deformation, indicating a planar slide along the stratified interface. This behavior aligns well with the characteristics of fissured, overconsolidated clays where residual strength conditions dominate post-yield behavior.

A finite element analysis using PLAXIS 2D and a simplified Limit Equilibrium Method (LEM) produced consistent results. Both analyses confirmed that the critical conditions for failure involved a residual friction angle of approximately 19°, an inclined bedding angle of 15 to 17 degrees, and a water height ranging between 2.10 and 2.65 m below ground level, within the vertical fissure. These parameters closely correspond to the field and laboratory data, reinforcing the validity of the assumed failure mechanism and the robustness of the analytical approach. Moreover, the findings underscore the significant destabilizing influence of geological discontinuities and transient groundwater conditions in such geological settings.

The limit equilibrium method remains a fundamental tool in a slope stability analysis, particularly due to its computational efficiency and conceptual simplicity. In this case, the LEM was highly effective for conducting parametric sensitivity analyses and estimating the threshold conditions that triggered the failure, since the soil mass was considered a rigid body. It allowed for rapid iteration over key variables, including shear strength, water height, and slope angle, facilitating a comprehensive understanding of their combined effects. The translational nature of the observed failure further justified the use of planar sliding models within the LEM analysis.

Despite these advantages, the method exhibits notable limitations. One primary weakness is its reliance on rigid body assumptions, which can oversimplify complex soil behavior, especially in stratified and anisotropic formations. The method does not account for the development of strain or stress redistribution prior to failure and cannot model progressive failure or post-yield deformation. This makes it less suitable for analyzing scenarios where time-dependent behavior or stress–strain interactions are significant. Additionally, the LEM is highly sensitive to input parameters, particularly the residual friction angle and the water level within discontinuities. Given the inherent difficulty in accurately determining these values in the field, the potential for error in safety factor estimations remains a concern. In contrast, finite element methods provide a more rigorous representation of the stress–strain state and fluid–structure interaction. Nevertheless, the LEM remains a valuable tool, when supported by high-quality field and laboratory data and can be used in cases where the soil mass can be modelled as a hard soil with the behavior of a rigid body, as this was the most representative scenario in the present study.

The failure described in this study was precipitated by a previously undetected geological discontinuity that functioned as a tension crack once hydrostatic conditions developed. This highlights a critical gap in the current geotechnical investigation practices for temporary excavations, especially in complex stratified environments. Standard site investigation techniques, such as borehole drilling and classification tests, are sometimes insufficient for identifying subvertical fissures or tension cracks, particularly those located outside the excavation boundary.

To address this limitation, a more comprehensive investigative approach is required. High-resolution geophysical methods such as electrical resistivity tomography and seismic refraction tomography can offer valuable insights into subsurface conditions, particularly when combined with geotechnical interpretation. These methods are capable of detecting moisture anomalies and stiffness contrasts that may indicate the presence of cracks or weak planes. Another method that can be used in shallow, surficial investigations, is drone-based photogrammetry, which can reveal surface discontinuities or gaps, especially when supported by a terrain analysis and historical land-use data. In combination with targeted geological profiling that considers regional tectonic and stratigraphic contexts, these tools can greatly improve the detection of hidden discontinuities.

This case underscores the importance of revisiting the existing regulatory and design practices related to temporary excavations in geologically complex terrains. The observed failure resulted from a combination of overlooked geological conditions and an absence of a mandated geotechnical investigation, reflecting a systemic vulnerability in standard practice. There is a pressing need for stricter enforcement of pre-excavation site assessments, particularly in areas characterized by stratified overconsolidated clays. It is worth noting that according to the relevant provisions of the Greek Seismic Code (EAK 2003) [45] and Eurocode 8 (EC-8) [46], the soil conditions in the area of interest are classified as Category B. For this category, according to EAK 2003 [45], it is not required to conduct a geotechnical investigation for ordinary buildings (i.e., ordinary residential and office buildings, industrial buildings, hotels, etc.). Moreover, based on the Greek legislation [47], for a Category B (hard soil) soil and for the excavation geometry conducted in the study area, no temporary support system was required for the excavation area.

The findings of this case study emphasize the necessity of incorporating detailed geotechnical investigations in construction planning, especially in areas with complex stratigraphy. The presence of tension cracks should be carefully evaluated, and excavation depths should be strictly controlled to prevent the exceeding of critical stability thresholds. This study highlights the need for stricter regulatory measures regarding geotechnical assessments prior to excavation in areas with complex geological conditions. National construction regulations should require pre-excavation geotechnical assessments to mitigate such failures in future projects with similar soil conditions.

For this purpose, geotechnical assessments should include both drained and residual strength measurements, especially in soils suspected of containing fissures or laminated structures. Residual strength parameters must be derived from laboratory tests such as ring shear or direct shear tests under large deformation conditions. Moreover, excavation designs should incorporate conservative depth limits, anticipate possible groundwater inflows, and consider proactive drainage solutions to mitigate pore pressure buildup.

Finally, the combined use of limit equilibrium and finite element methods is encouraged to ensure a robust and multidimensional understanding of slope stability. While the LEM can offer quick preliminary assessments, the FEM should be employed for final designs or forensic analysis, especially in complex soil–structure–water interaction scenarios. This consideration has been studied by various researchers using multiple analytical and numerical methods [48,49], and incorporating the influence, location, and development of tension cracks on the stability of soil and rock slopes. Tension cracks are of critical importance in the analysis of slopes and excavations, particularly in geological formations where pre-existing fissures or other discontinuities may evolve into tension cracks, significantly reducing the overall stability. When such cracks are structurally linked to the inherent features of a slope, especially when their existence is not known in advance, they pose a substantial risk. This highlights the need for further research aimed at informing updates to the building codes and engineering standards governing slope excavations, with particular emphasis on the detection, assessment, and mitigation of tension crack-related failures.

6. Conclusions

This study analyzed a well-documented case of slope failure in stratified, overconsolidated hard clays during an unsupported excavation on Corfu Island, Greece. The investigation identified a nearly vertical pre-existing discontinuity, unrecognized prior to the excavation, as the critical factor that triggered the failure when partially filled with water. Acting as a tension crack, this discontinuity combined with hydrostatic pressure and seepage forces along the clay–sandstone interface significantly reduced the stability of the slope, ultimately lowering the Factor of Safety (FoS) to unity.

Laboratory and field investigations provided significant geotechnical parameters, including a residual friction angle of 19°, which matched empirical estimates and was used in both the Limit Equilibrium Method (LEM) and Finite Element Method (FEM) analyses. Both methods demonstrated consistency in predicting failure conditions, validating the translational failure mechanism observed on site and proved that a limit equilibrium analysis, when supported by accurate field data, remains effective for practical assessments of slope stability, particularly for rigid soil masses.

Another important aspect of this study is the identification of critical parameters that play an important role in the stability of unsupported hard clay slopes. For example, undetected geological discontinuities can be major contributors to excavation failures even in stiff, overconsolidated soils that are considered stable under typical design assumptions. Moreover, transient groundwater conditions and small deviations from design excavation depths can significantly affect slope stability, especially in stratified formations.

The findings of this research underscore the necessity for more rigorous and site-specific geotechnical investigations, especially in areas with complex stratigraphy. The current regulatory frameworks may inadequately address the risks posed by such conditions, as evident in this case where no geotechnical study was legally required.

For future excavation projects in similar geological settings, this study recommends the following: (a) mandatory pre-excavation geotechnical investigations including assessments of residual strength and groundwater conditions, (b) the use of combined analytical and numerical approaches for a comprehensive understanding of potential failure mechanisms, including a 3D analysis, and (c) a re-evaluation of the regulatory criteria for temporary excavations, especially in stratified or fissured terrains.