Pit Lake Slope Stability under Water Level Variations
Abstract
1. Introduction
2. Methodology Adopted in Fully Coupled Flow-Deformation Analysis
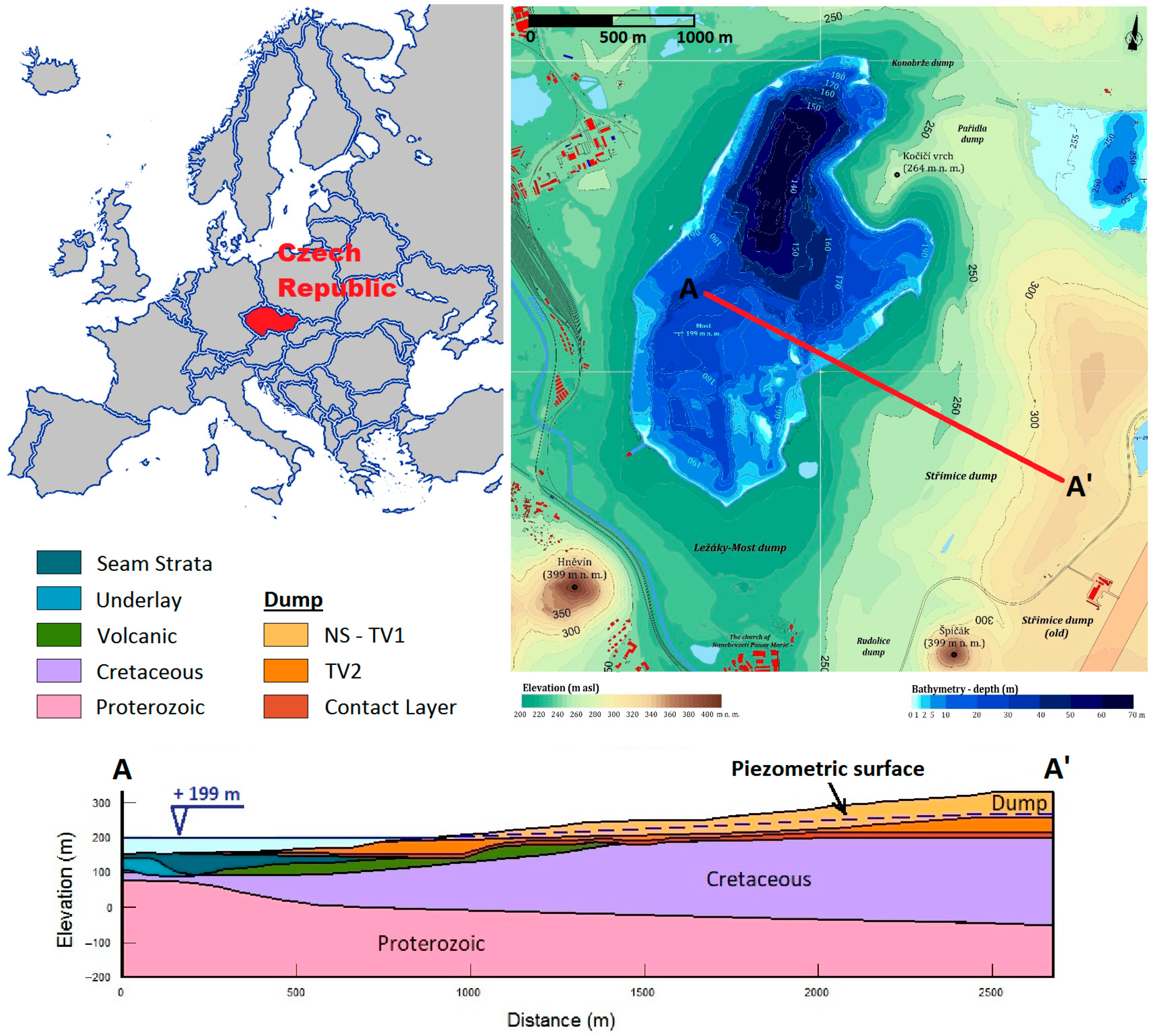
3. Study Area
- NS–TV1 formation is generally considered as a stable loose material. It is at a certain stage of consolidation and it can be considered as a semi-permeable environment.
- The TV2 layer, which shows very similar values to TV1 concerning the internal friction angle. However, it exhibits a relatively significant decrease in cohesion compared to TV1.
- The Contact Layer, located at the base of the dump, has the lowest strength compared to the other layers.
4. Stability of the Inner Dump Slopes
4.1. Numerical Modeling
4.2. Geometry and Material Types of the Geotechnical Model
4.3. Computations
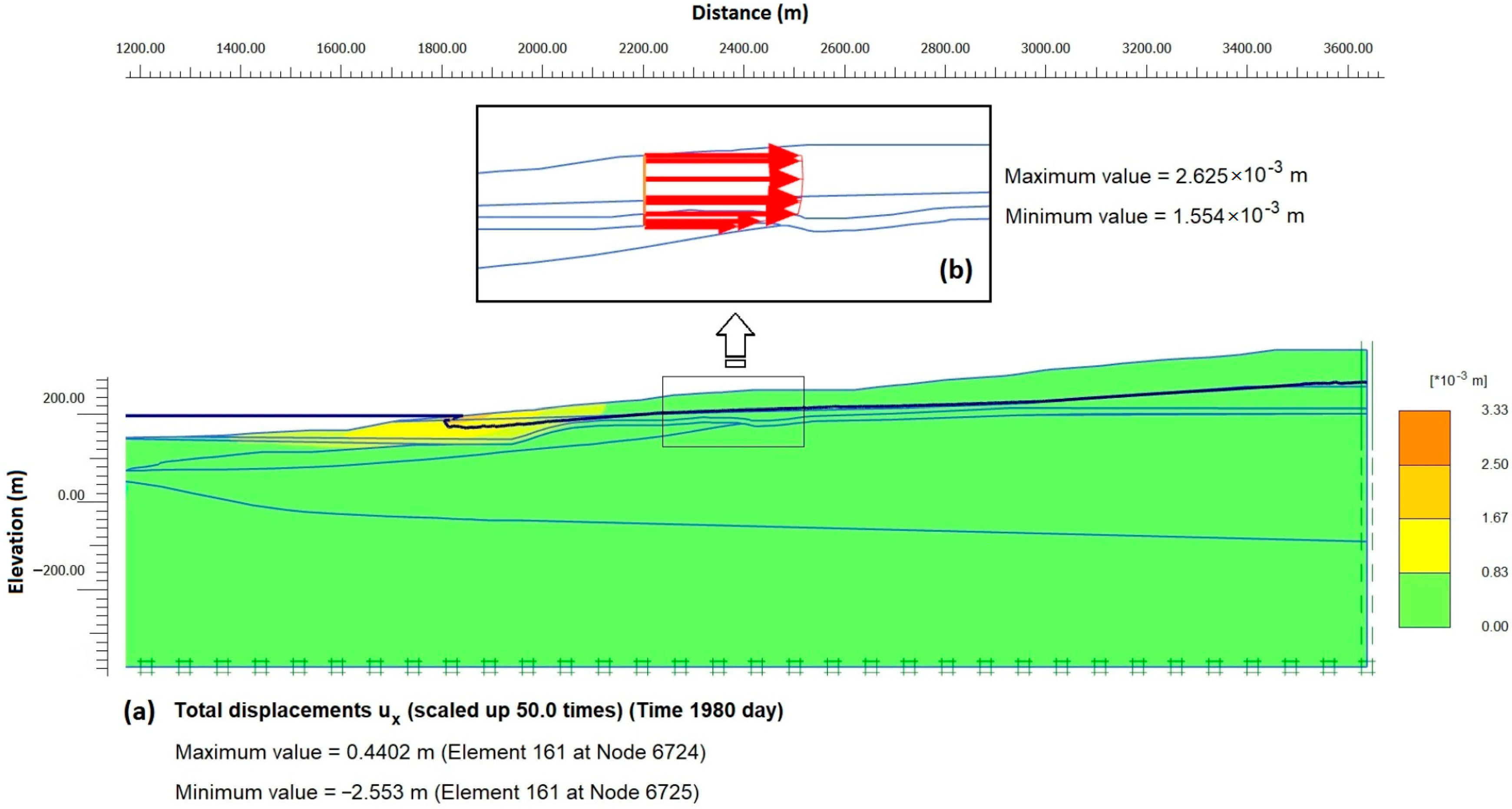
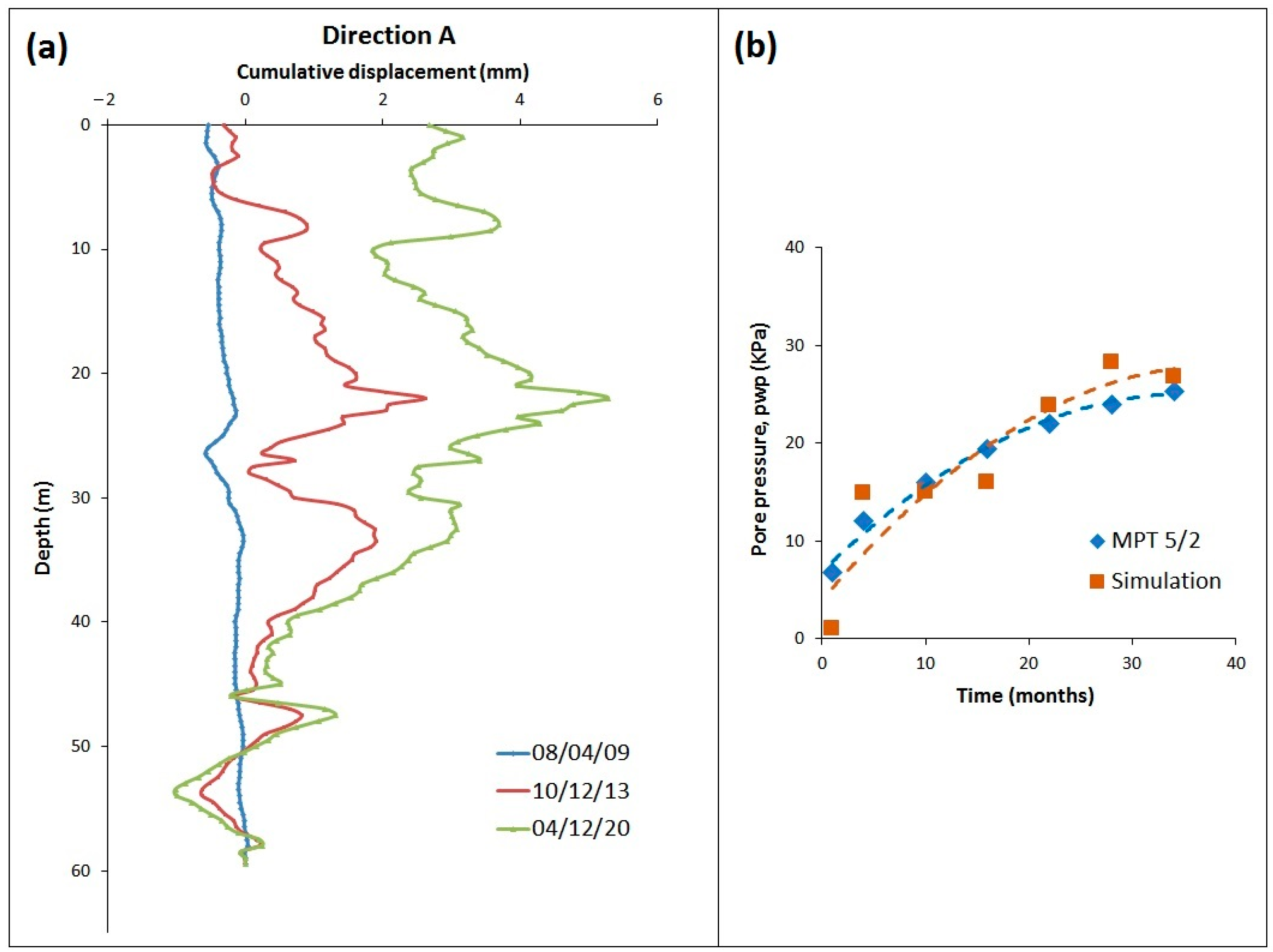
5. Effects of Flooding Process on Slope Stability
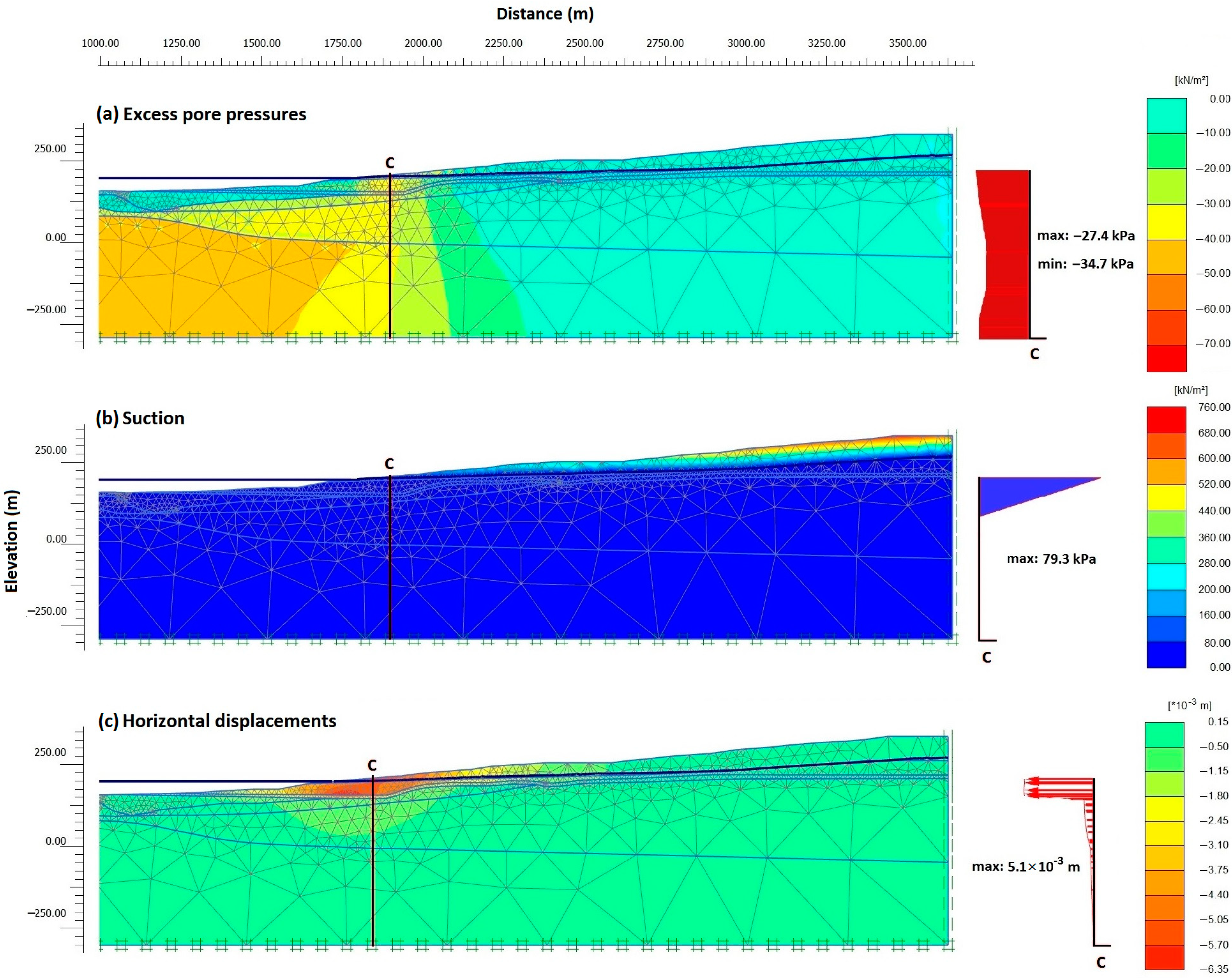
6. Slope Stability under the Impact of Reservoir Water Level
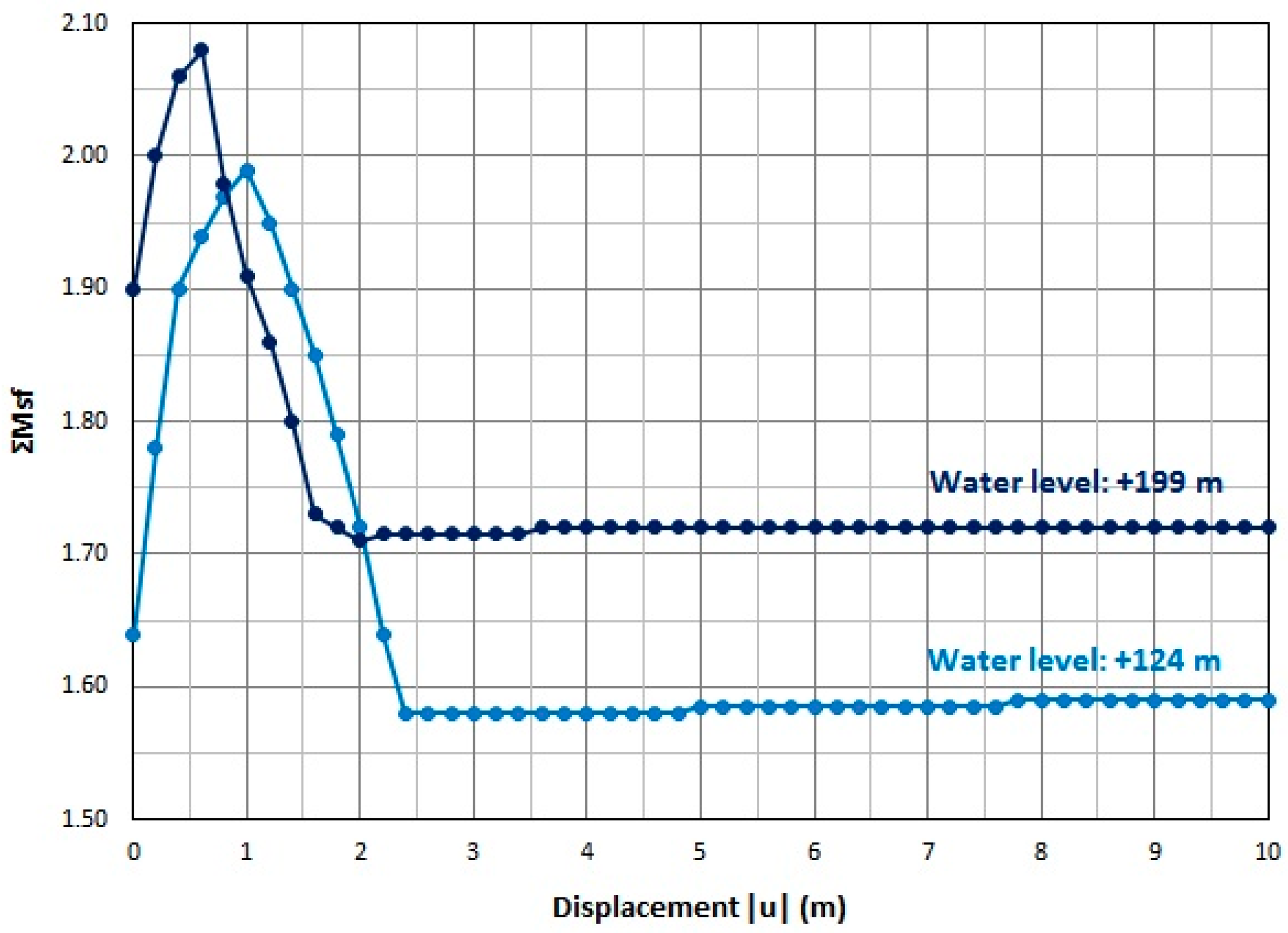
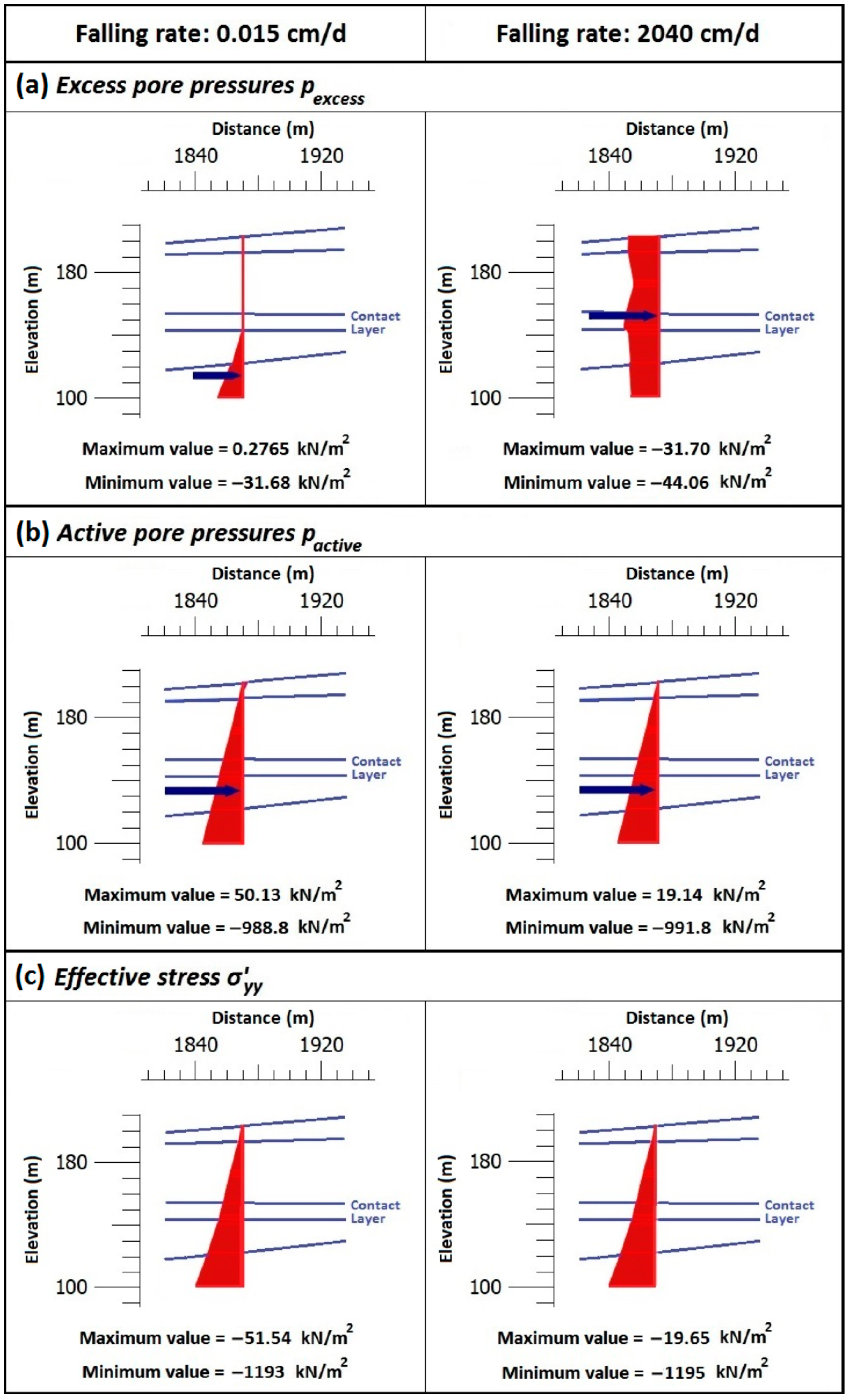
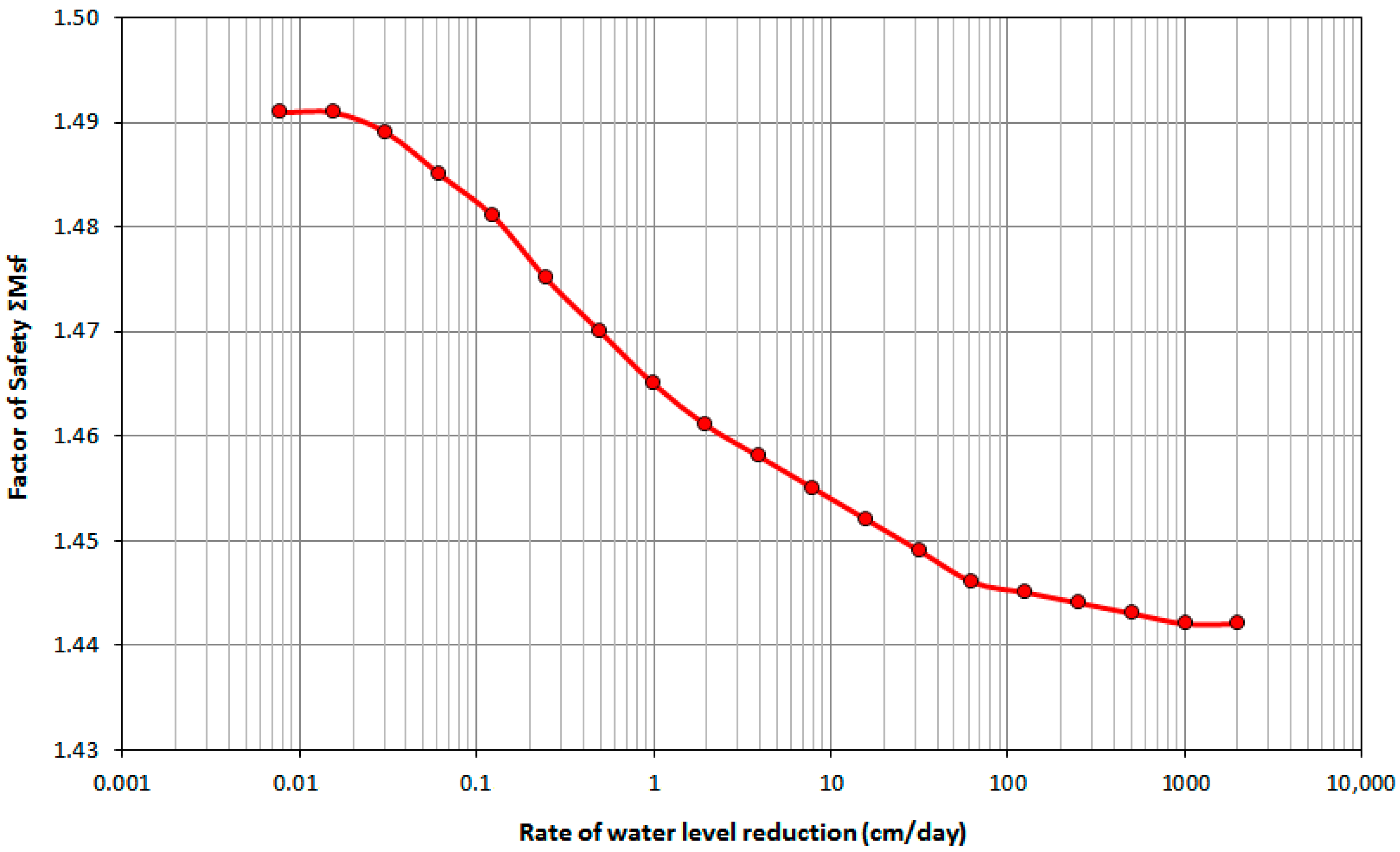
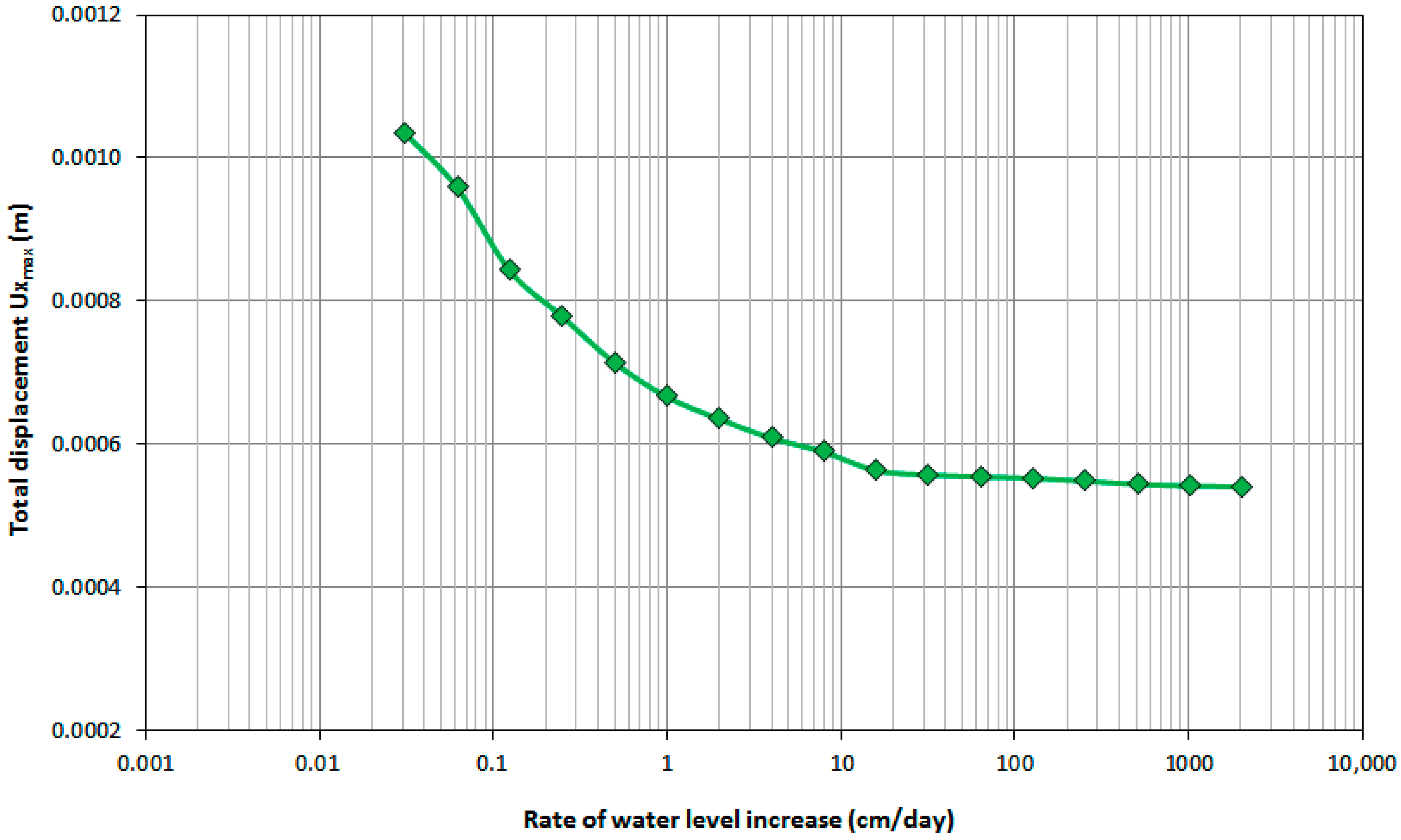
6.1. The Impact of Water Level Drawdown on the Slope (Case 1)
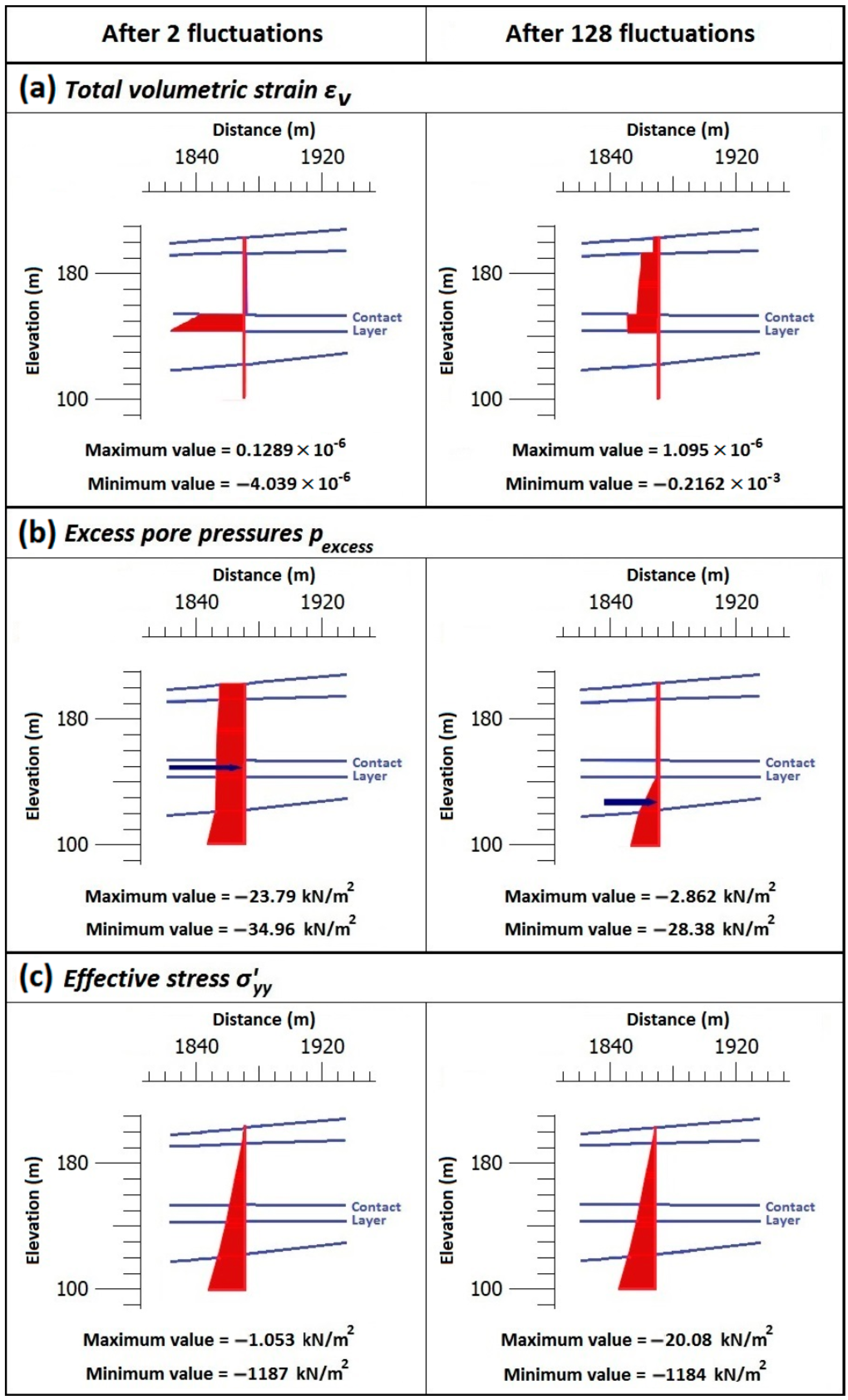
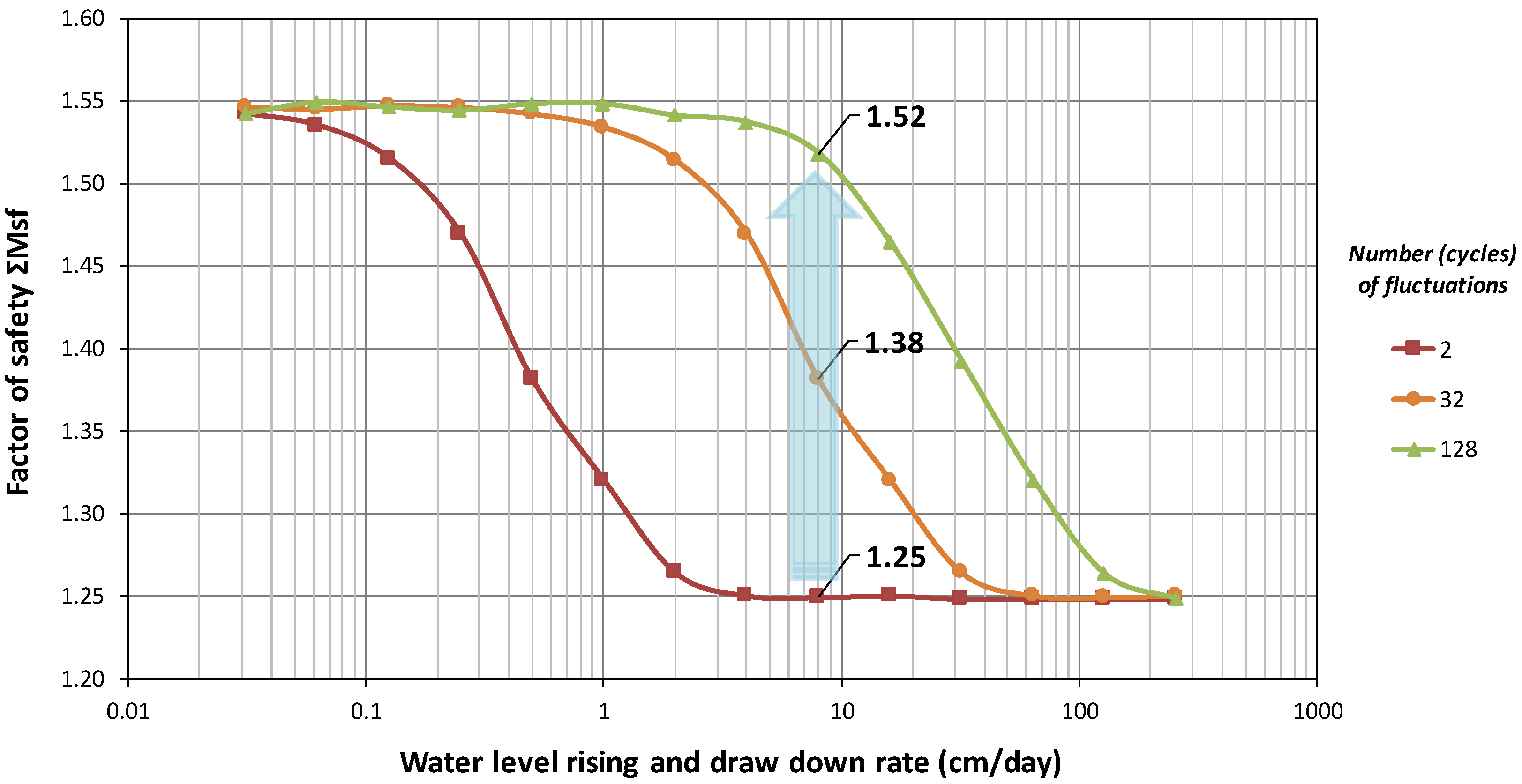
6.2. The Impact of Water Level Fluctuations on the Stability of the Slope (Case 2)
6.3. The Impact of Water Level Rising on the Slope Stability (Case 3)
7. Conclusions
- The slope stability decreases as the water level drops; the stability factor is negatively related to the rate of lowering the water level.
- Increased fluctuations affect slope safety. With a specified number of fluctuations, the soil mass seems to become less stable as the rate of change in the water level increases. However, at a specific speed, the stability grows as the number of fluctuations increases.
- The rise in the water level causes the unsaturated region of the slope to shrink, and may reduce the stability of the reservoir slope. However, the water pressure due to the external water level acts on the slope surface with a positive impact on the stability factor, and as a final result, the rise in water level improves slope stability.
- Furthermore, when comparing the stability of the slope, it is more stable under conditions of gradual water-level rising than water-level drop conditions.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Schultze, M.; Hemm, M.; Geller, W.; Benthaus, F.C. Pit Lakes in Germany: Hydrography, Water Chemistry, and Management. In Acidic Pit Lakes; Springer: Berlin/Heidelberg, Germany, 2013; pp. 265–291. [Google Scholar]
- Schultze, M.; Pokrandt, K.-H.; Hille, W. Pit Lakes of the Central German Lignite Mining District: Creation, Morphometry and Water Quality Aspects. Limnologica 2010, 40, 148–155. [Google Scholar] [CrossRef]
- Oggeri, C.; Fenoglio, T.M.; Godio, A.; Vinai, R. Overburden Management in Open Pits: Options and Limits in Large Limestone Quarries. Int. J. Min. Sci. Technol. 2019, 29, 217–228. [Google Scholar] [CrossRef]
- Redondo-Vega, J.M.; Melón-Nava, A.; Peña-Pérez, S.A.; Santos-González, J.; Gómez-Villar, A.; González-Gutiérrez, R.B. Coal Pit Lakes in Abandoned Mining Areas in León (NW Spain): Characteristics and Geoecological Significance. Environ. Earth Sci. 2021, 80, 792. [Google Scholar] [CrossRef]
- Renaud, V.; Al Heib, M.; Burda, J. 3D Large-Scale Numerical Model of Open-Pit Lake Slope Stability case Study of Lake Most. Bull. Eng. Geol. Environ. 2022, 81, 282. [Google Scholar] [CrossRef]
- Lund, M.A.; Blanchette, M.L. Closing Pit Lakes as Aquatic Ecosystems: Risk, Reality, and Future Uses. Wiley Interdiscip. Rev. Water 2023, 10, e1648. [Google Scholar] [CrossRef]
- Geller, W.; Schultze, M.; Kleinmann, B.; Wolkersdorfer, C. Acidic Pit Lakes: The Legacy of Coal and Metal Surface Mines; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2012. [Google Scholar]
- Vinzelberg, G.; Dahmen, D. The Inden Residual Lake and the Federal Autobahn A44n–Geotechnical Requirements to Be Met by Large-Scale Structures in the Rhenish Lignite Mining Area. In Proceedings of the 12th International Symposium Continuous Surface Mining-Aachen 2014; Springer: Berlin/Heidelberg, Germany, 2015; pp. 91–100. [Google Scholar]
- Graupner, H. Nachterstedt Landslide Raises Worries for Other Former Mine Areas; Deutsche Welle: Bonn, Germany, 2009. [Google Scholar]
- Johansson, J.; Edeskär, T. Effects of External Water-Level Fluctuations on Slope Stability. Electron. J. Geotech. Eng. 2014, 19, 2437–2463. [Google Scholar]
- Johansson, J.; Edeskär, T. Modelling Approaches Considering Impacts of Water-Level Fluctuations on Slope Stability. J. Earth Sci. Geotech. Eng. 2014, 4, 17–34. [Google Scholar]
- Jia, G.W.; Zhan, T.L.T.; Chen, Y.M.; Fredlund, D.G. Performance of a Large-Scale Slope Model Subjected to Rising and Lowering Water Levels. Eng. Geol. 2009, 106, 92–103. [Google Scholar] [CrossRef]
- Wang, H.B.; Xu, W.Y.; Xu, R.C.; Jiang, Q.H.; Liu, J.H. Hazard Assessment by 3D Stability Analysis of Landslides Due to Reservoir Impounding. Landslides 2007, 4, 381–388. [Google Scholar] [CrossRef]
- Fourniadis, I.G.; Liu, J.G.; Mason, P.J. Regional Assessment of Landslide Impact in the Three Gorges Area, China, Using ASTER Data: Wushan-Zigui. Landslides 2007, 4, 267–278. [Google Scholar] [CrossRef]
- Cojean, R.; Caï, Y.J. Analysis and Modeling of Slope Stability in the Three-Gorges Dam Reservoir (China). The Case of Huangtupo Landslide. J. Mt. Sci. 2011, 8, 166–175. [Google Scholar] [CrossRef]
- Hu, X.; He, C.; Zhou, C.; Xu, C.; Zhang, H.; Wang, Q.; Wu, S. Model Test and Numerical Analysis on the Deformation and Stability of a Landslide Subjected to Reservoir Filling. Geofluids 2019, 2019, 5924580. [Google Scholar] [CrossRef]
- Fredlund, D.G.; Rahardjo, H. Soil Mechanics for Unsaturated Soils; John Wiley & Sons: Hoboken, NJ, USA, 1993. [Google Scholar]
- Gavin, K.; Xue, J. A Simple Method to Analyze Infiltration into Unsaturated Soil Slopes. Comput. Geotech. 2008, 35, 223–230. [Google Scholar] [CrossRef]
- Bittelli, M.; Valentino, R.; Salvatorelli, F.; Pisa, P.R. Monitoring Soil-Water and Displacement Conditions Leading to Landslide Occurrence in Partially Saturated Clays. Geomorphology 2012, 173, 161–173. [Google Scholar] [CrossRef]
- Lane, P.A.; Griffiths, D.V. Assessment of Stability of Slopes under Drawdown Conditions. J. Geotech. Geoenviron. Eng. 2000, 126, 443–450. [Google Scholar] [CrossRef]
- Duncan, J.M.; Wright, S.G. Soil Strength and Slope Stability; Wiley: Hoboken, NJ, USA, 2005. [Google Scholar]
- Yang, H.; Rahardjo, H.; Xiao, D. Rapid Drawdown of Water Table in Layered Soil Column. In Geoenvironmental Engineering and Geotechnics: Progress in Modeling and Applications; American Society of Civil Engineers: Reston, VA, USA, 2010; pp. 202–209. [Google Scholar]
- Pinyol, N.M.; Alonso, E.E.; Corominas, J.; Moya, J. Canelles Landslide: Modelling Rapid Drawdown and Fast Potential Sliding. Landslides 2012, 9, 33–51. [Google Scholar] [CrossRef]
- Fan, L.; Zhang, G.; Li, B.; Tang, H. Deformation and Failure of the Xiaochatou Landslide under Rapid Drawdown of the Reservoir Water Level Based on Centrifuge Tests. Bull. Eng. Geol. Environ. 2017, 76, 891–900. [Google Scholar] [CrossRef]
- Sun, G.; Yang, Y.; Jiang, W.; Zheng, H. Effects of an Increase in Reservoir Drawdown Rate on Bank Slope Stability: A Case Study at the Three Gorges Reservoir, China. Eng. Geol. 2017, 221, 61–69. [Google Scholar] [CrossRef]
- Berilgen, M.M. Investigation of Stability of Slopes under Drawdown Conditions. Comput. Geotech. 2007, 34, 81–91. [Google Scholar] [CrossRef]
- Paronuzzi, P.; Rigo, E.; Bolla, A. Influence of Filling–Drawdown Cycles of the Vajont Reservoir on Mt. Toc Slope Stability. Geomorphology 2013, 191, 75–93. [Google Scholar] [CrossRef]
- He, C.; Hu, X.; Xu, C.; Wu, S.; Zhang, H.; Liu, C. Model Test of the Influence of Cyclic Water Level Fluctuations on a Landslide. J. Mt. Sci. 2020, 17, 191–202. [Google Scholar] [CrossRef]
- Zhan, T.L.T.; Zhang, W.J.; Chen, Y.M. Influence of Reservoir Level Change on Slope Stability of a Silty Soil Bank. In Unsaturated Soils 2006; ASCE Press: Reston, VA, USA, 2006; pp. 463–472. [Google Scholar]
- Shen, Y.B.; Zhu, D.Y.; Yao, H.Y. Critical Slip Field of Slope in Process of Reservoir Water Level Fluctuations. Rock Soil Mech. 2010, 31, 179–183. [Google Scholar]
- Oh, S.; Lu, N. Slope Stability Analysis under Unsaturated Conditions: Case Studies of Rainfall-Induced Failure of Cut Slopes. Eng. Geol. 2015, 184, 96–103. [Google Scholar] [CrossRef]
- Liu, Y.; He, L.Q.; Jiang, Y.J.; Sun, M.M.; Chen, E.J.; Lee, F.-H. Effect of in Situ Water Content Variation on the Spatial Variation of Strength of Deep Cement-Mixed Clay. Géotechnique 2019, 69, 391–405. [Google Scholar] [CrossRef]
- Mao, J.-Z.; Guo, J.; Fu, Y.; Zhang, W.-P.; Ding, Y.-N. Effects of Rapid Water-Level Fluctuations on the Stability of an Unsaturated Reservoir Bank Slope. Adv. Civ. Eng. 2020, 2020, 1–10. [Google Scholar] [CrossRef]
- Chen, G.; Li, Q.-Y.; Li, D.-Q.; Wu, Z.-Y.; Liu, Y. Main Frequency Band of Blast Vibration Signal Based on Wavelet Packet Transform. Appl. Math. Model. 2019, 74, 569–585. [Google Scholar] [CrossRef]
- Bishop, A.W.; Blight, G.E. Some Aspects of Effective Stress in Saturated and Partly Saturated Soils. Geotechnique 1963, 13, 177–197. [Google Scholar] [CrossRef]
- Khalili, N.; Khabbaz, M.H. A Unique Relationship for χ for the Determination of the Shear Strength of Unsaturated Soils. Geotechnique 1998, 48, 681–687. [Google Scholar] [CrossRef]
- Plaxis, B.V. Plaxis 2D Reference Manual; Plaxis, B.V.: Delft, The Netherlands, 2018. [Google Scholar]
- Bolzon, G.; Schrefler, B.A.; Zienkiewicz, O.C. Elastoplastic Soil Constitutive Laws Generalized to Partially Saturated States. Géotechnique 1996, 46, 279–289. [Google Scholar] [CrossRef]
- Van Genuchten, M.T. A Closed-Form Equation for Predicting the Hydraulic Conductivity of Unsaturated Soils. Soil Sci. Soc. Am. J. 1980, 44, 892–898. [Google Scholar] [CrossRef]
- Galavi, V. Groundwater Flow, Fully Coupled Flow Deformation and Undrained Analyses in PLAXIS 2D and 3D. Plaxis Rep. 2010. [Google Scholar] [CrossRef]
- Žižka, L.; Burda, J. Post Exploitation Lakes. In Zpravodaj Hnӗdé Uhlí; Brown Coal Research Institute: Budovatelů, Czech Republic, 2021; pp. 11–46. [Google Scholar]
- Burda, J.; Veselý, M.; Řehoř, M.; Vilímek, V. Reconstruction of a Large Runout Landslide in the Krušné Hory Mts. (Czech Republic). Landslides 2018, 15, 423–437. [Google Scholar] [CrossRef]
- Arvanitidis, C.; Steiakakis, E.; Agioutantis, Z. Peak Friction Angle of Soils as a Function of Grain Size. Geotech. Geol. Eng. 2019, 37, 1155–1167. [Google Scholar] [CrossRef]
- Stiakakis, E.; Agioutantis, Z. Stiffness and Strength Parameters of the Ptolemais Calcareous Marls. In Proceedings of the International Workshop in Geoenvironment and Geotechnics, Milos Island, Greece, 12–14 September 2005; pp. 51–57. [Google Scholar]
- Steiakakis, E.; Agioutantis, Z. A Kinetic Behavior Model at a Surface Lignite Mine, Based on Geotechnical Investigation. Simul. Model. Pract. Theory 2010, 18, 558–573. [Google Scholar] [CrossRef]
- Steiakakis, E.; Kavouridis, K.; Monopolis, D. Large Scale Failure of the External Waste Dump at the South Field Lignite Mine, Northern Greece. Eng. Geol. 2009, 104, 269–279. [Google Scholar] [CrossRef]
- Steiakakis, E.; Xiroudakis, G.; Lazos, I.; Vavadakis, D.; Bazdanis, G. Stability Analysis of a Multi-Layered Slope in an Open Pit Mine. Geosciences 2023, 13, 359. [Google Scholar] [CrossRef]
- Xia, M.; Ren, G.M.; Zhu, S.S.; Ma, X.L. Relationship between Landslide Stability and Reservoir Water Level Variation. Bull. Eng. Geol. Environ. 2015, 74, 909–917. [Google Scholar] [CrossRef]





| Geotechnical Parameters | NS–TV1 | TV2 | Contact Layer | Seam Strata | Underlay | Volcanic | Cretaceous | Proterozoic | |
|---|---|---|---|---|---|---|---|---|---|
| Unit weight above phreatic level γunsat | [kN/m3] | 17.6 | 18.9 | 17 | 15 | 14 | 21 | 23 | 23 |
| Unit weight below phreatic level γsat | [kN/m3] | 18.6 | 19.9 | 20 | 18 | 15 | 23 | 25 | 25 |
| Cohesion c′ | [kN/m2] | 22 | 40.6 | 8 | 50 | 35 | 500 | Elastic | Elastic |
| Friction angle φ′ | [°] | 22.1 | 25.9 | 7 | 35 | 38 | 25 | Elastic | Elastic |
| Young’s modulus E′ | [MPa] | 300 | 560 | 70 | 150 | 200 | 3000 | 5000 | 30,000 |
| Poisson’s ratio v′ | 0.4 | 0.4 | 0.30 | 0.3 | 0.30 | 0.35 | 0.35 | 0.35 | |
| NS–TV1 | TV2 | ||||
 | Unsaturated unit weight | γunsat | [kN/m3] | 17.6 | 18.9 |
| Saturated unit weight | γsat | [kN/m3] | 18.6 | 19.9 | |
| Initial void ratio | einit | - | 0.5 | 0.5 | |
| Cohesion | cref′ | [kN/m2] | 4.6 | 7.8 | |
| Friction angle | φ′ | [°] | 22.1 | 25.9 | |
| Dilatancy angle | ψ | [°] | 0 | 8.6 | |
| Groundwater parameters | |||||
| Perneability of saturated soil, x | kx | (m/day) | 0.117 | 0.002 | |
| Perneability of saturated soil, y | ky | (m/day) | 0.117 | 0.002 | |
| Van Genuchten parameters | |||||
| Fitting parameter | gn | - | 1.09 | 1.52 | |
| Fitting parameter | ga | - | 0.80 | 4.30 | |
| Fitting parameter | gc | - | 0.50 | 1.25 | |
| Saturated degree of saturation | Ssat | - | 1 | 1 | |
| Residual degree of saturation | Sres | - | 0.179 | 0.068 | |
| Case | Conditions Research |
|---|---|
| Case 1 | Water level drops from +199 m to +194 m at various rates. |
| Case 2 | Water level fluctuates. The initial water-level position was set to +199 m and the level-change magnitude to 0.6 m (linearly lowered and raised) at various rates. The analysis was repeated for an increasing number of water level fluctuations. |
| Case 3 | Water level rises from +199 m to +204 m at various rates. |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Steiakakis, E.; Syllignakis, G.; Galetakis, M.; Vavadakis, D.; Renaud, V.; Al Heib, M.; Burda, J. Pit Lake Slope Stability under Water Level Variations. Geosciences 2024, 14, 142. https://doi.org/10.3390/geosciences14060142
Steiakakis E, Syllignakis G, Galetakis M, Vavadakis D, Renaud V, Al Heib M, Burda J. Pit Lake Slope Stability under Water Level Variations. Geosciences. 2024; 14(6):142. https://doi.org/10.3390/geosciences14060142
Chicago/Turabian StyleSteiakakis, Emmanouil, Georgios Syllignakis, Michail Galetakis, Dionysios Vavadakis, Vincent Renaud, Marwan Al Heib, and Jan Burda. 2024. "Pit Lake Slope Stability under Water Level Variations" Geosciences 14, no. 6: 142. https://doi.org/10.3390/geosciences14060142
APA StyleSteiakakis, E., Syllignakis, G., Galetakis, M., Vavadakis, D., Renaud, V., Al Heib, M., & Burda, J. (2024). Pit Lake Slope Stability under Water Level Variations. Geosciences, 14(6), 142. https://doi.org/10.3390/geosciences14060142









