A Meteorological Drought Migration Model for Assessing the Spatiotemporal Paths of Drought in the Choushui River Alluvial Fan, Taiwan
Abstract
1. Introduction
2. Materials and Methods
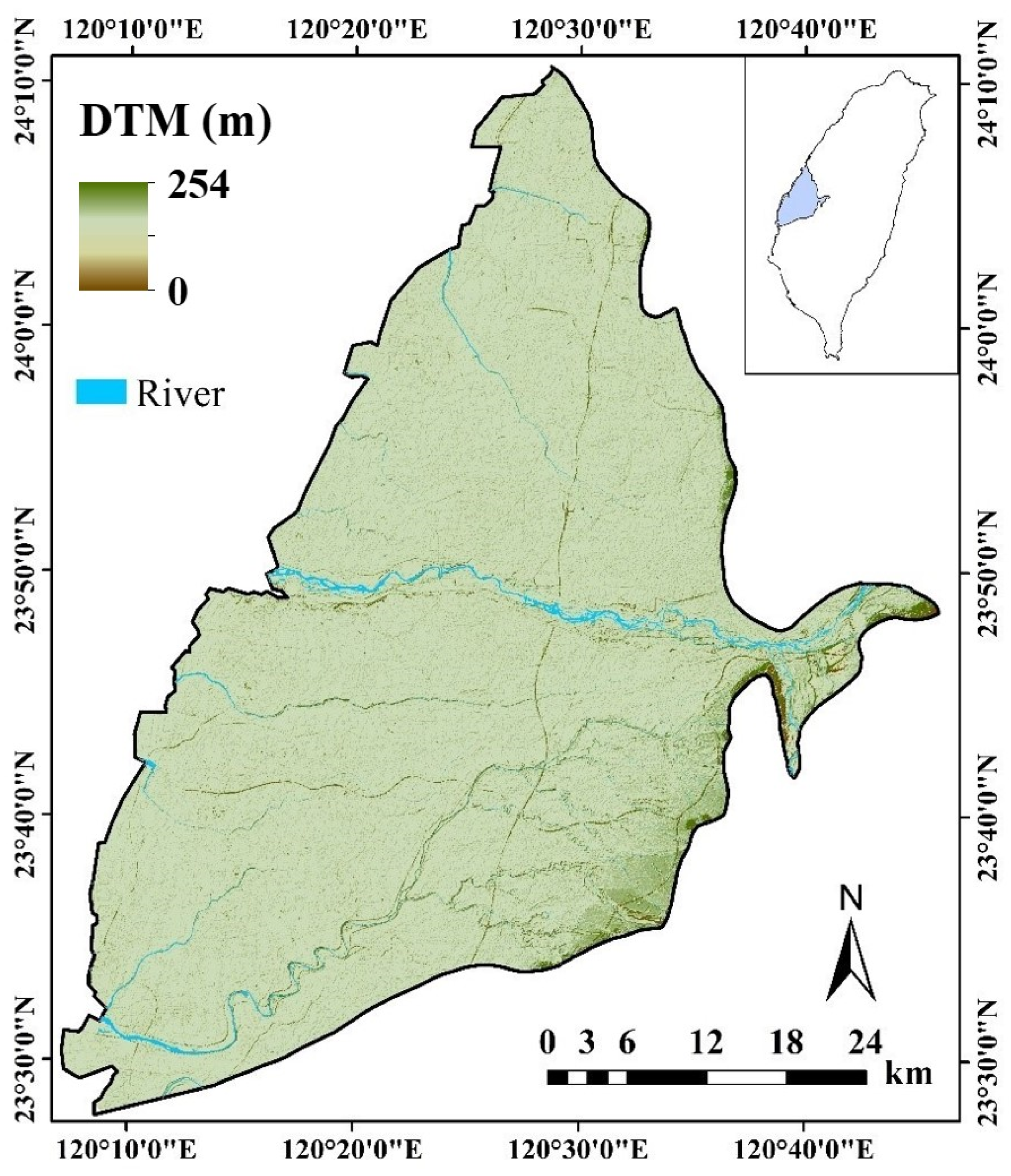
2.1. Study Area and Data
2.2. Standardized Precipitation Index (SPI)
2.3. Drought Coverage (Dc)
2.4. Selection of Major Drought Events
- (1)
- Select drought characteristics as drought variables to assess the magnitude and frequency of drought events.
- (2)
- (3)
- Fit the copula function and estimate its parameters [45].
- (4)
- Perform goodness-of-fit tests to choose the optimal copula function [46].
- (5)
- Determine major drought events through thresholding of joint distribution probabilities.
2.5. Spatiotemporal Paths of Drought Events
- (1)
- Identifying drought events: Drought events are defined as a continuous negative value of SPI for a period of time, including the occurrence of an SPI less than −1 [13]. In this study, the minimum drought area threshold was set at 10%.
- (2)
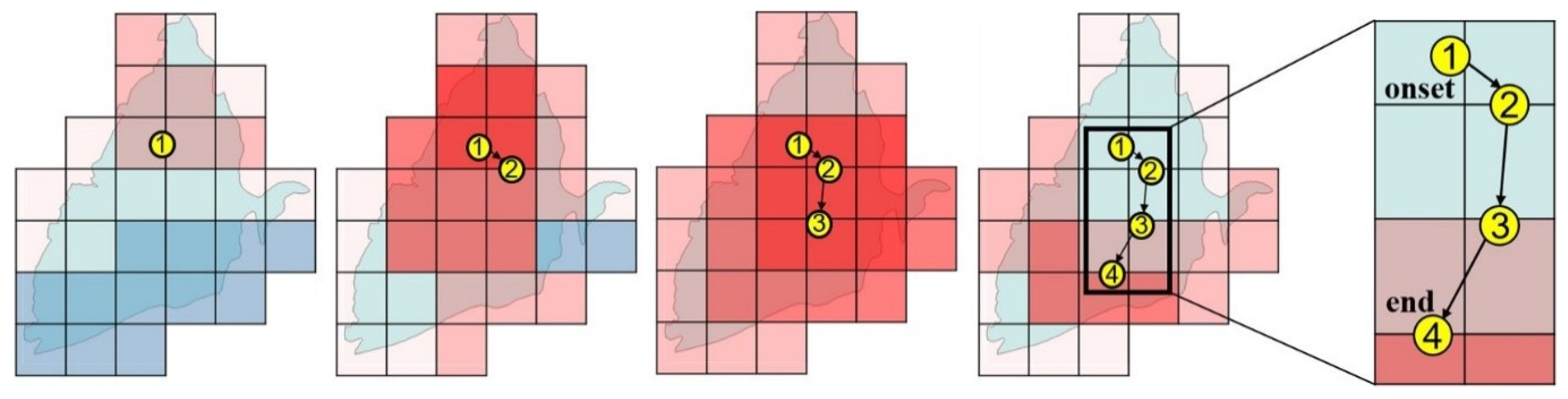
- Screening of the grid: The study area is divided into multiple grids for analysis. Each grid is evaluated based on the drought index to determine if it experiences drought (SPI < 0). If drought occurs, the drought state (Ds) is assigned a value of 1; otherwise, it is assigned a value of 0, as shown in Equation (2):
- (3)
- Determining the location of the drought centroids: The drought area is determined by grids where Ds is equal to 1. To account for the different weights of the SPI, the SPI values within each drought grid are recorded. The centroid coordinates are used to represent the spatial location of the drought event in that month, as shown in Equation (3):
- (4)
- Connecting centroids: By connecting the centroids of drought events, it is possible to comprehensively describe the path, length, and velocity characteristics of drought events.
2.6. Spatial Distribution of Drought
3. Results and Discussion
3.1. Direction of Drought Events
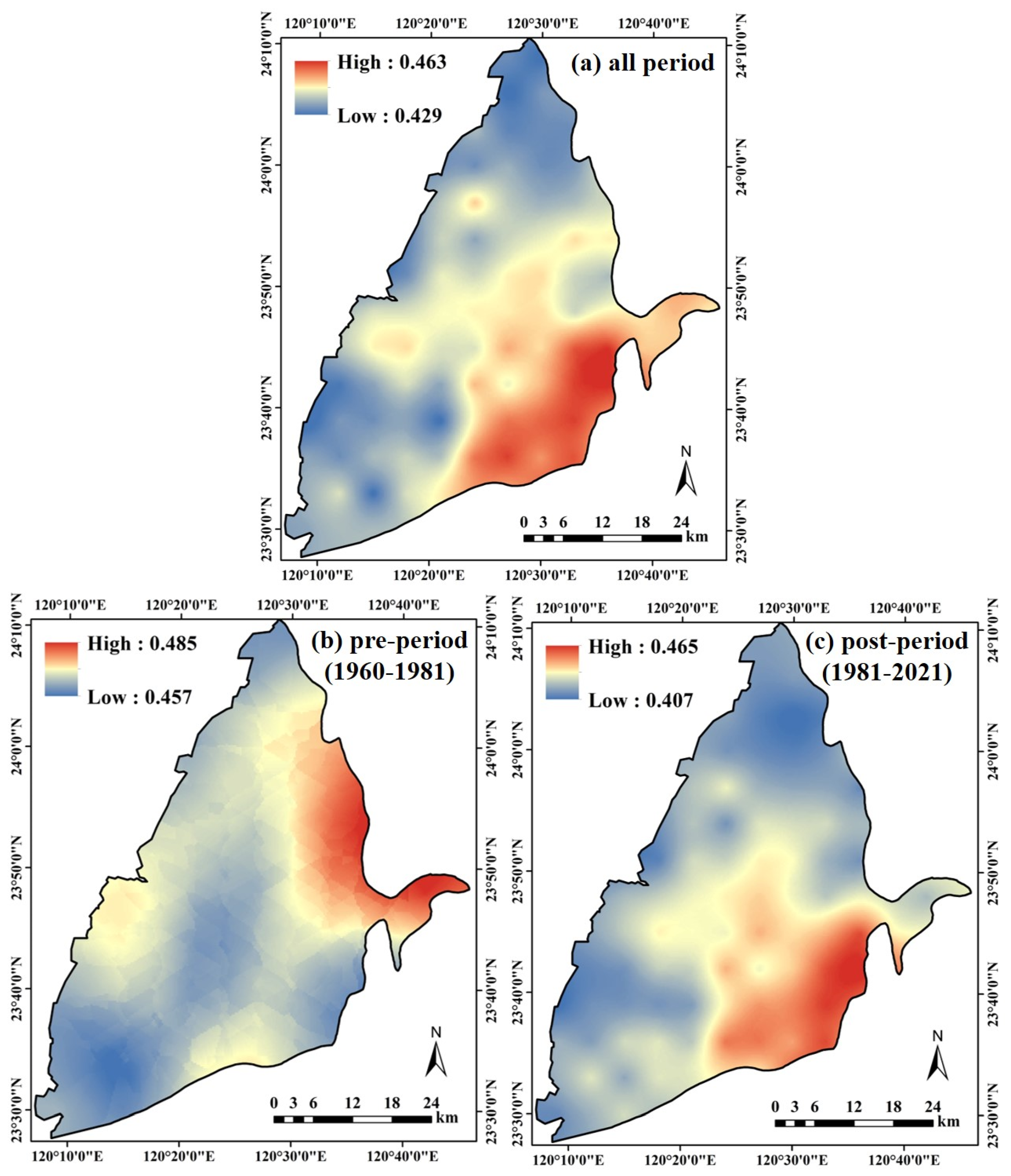
3.2. Spatial Distribution of Drought Frequency in Different Periods
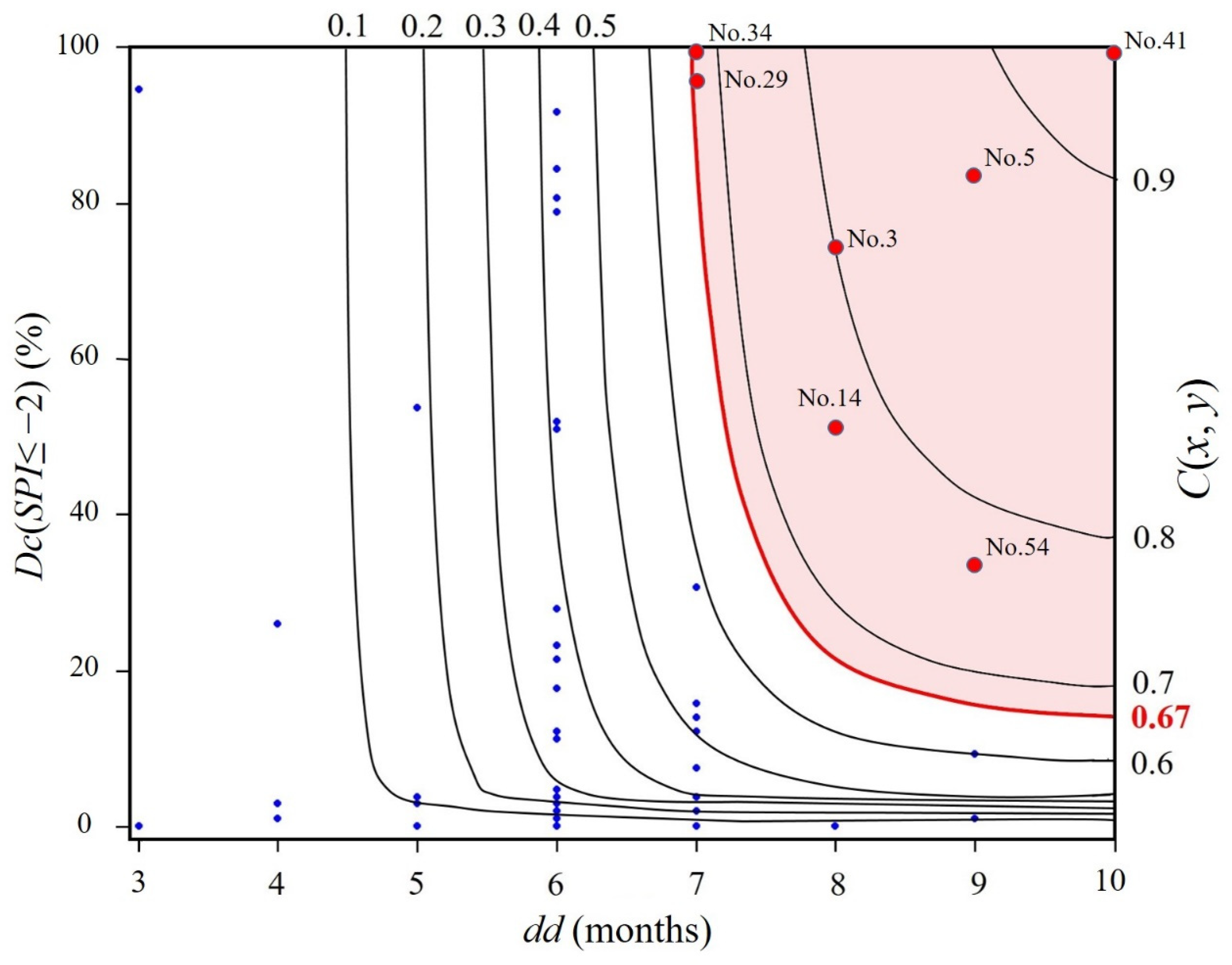
3.3. Joint Probability Distribution and Major Drought Events
3.4. Spatial Migration Process of Major Drought Events
3.5. Links between Rainfall Patterns and Drought Paths
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- UNEP. UNEP Emissions Gap Report; UNEP: Nairobi, Kenya, 2022. [Google Scholar]
- Qing, Y.; Wang, S.; Yang, Z.-L.; Gentine, P. Soil moisture−atmosphere feedbacks have triggered the shifts from drought to pluvial conditions since 1980. Commun. Earth Environ. 2023, 4, 254. [Google Scholar] [CrossRef]
- Beniston, M.; Stephenson, D.B. Extreme climatic events and their evolution under changing climatic conditions. Glob. Planet. Change 2004, 44, 1–9. [Google Scholar] [CrossRef]
- Smith, A.B.; Matthews, J.L. Quantifying uncertainty and variable sensitivity within the US billion-dollar weather and climate disaster cost estimates. Nat. Hazards 2015, 77, 1829–1851. [Google Scholar] [CrossRef]
- Pokhrel, Y.; Felfelani, F.; Satoh, Y.; Boulange, J.; Burek, P.; Gädeke, A.; Gerten, D.; Gosling, S.N.; Grillakis, M.; Gudmundsson, L.; et al. Global terrestrial water storage and drought severity under climate change. Nat. Clim. Chang. 2021, 11, 226–233. [Google Scholar] [CrossRef]
- Liu, X.; Ma, Q.; Yu, H.; Li, Y.; Li, L.; Qi, M.; Wu, W.; Zhang, F.; Wang, Y.; Zhou, G.; et al. Climate warming-induced drought constrains vegetation productivity by weakening the temporal stability of the plant community in an arid grassland ecosystem. Agric. For. Meteorol. 2021, 307, 108526. [Google Scholar] [CrossRef]
- Ji, Y.; Li, Y.; Yao, N.; Biswas, A.; Zou, Y.; Meng, Q.; Liu, F. The lagged effect and impact of soil moisture drought on terrestrial ecosystem water use efficiency. Ecol. Indic. 2021, 133, 108349. [Google Scholar] [CrossRef]
- Mehdipour, S.; Nakhaee, N.; Zolala, F.; Okhovati, M.; Foroud, A.; Haghdoost, A.A. A systematized review exploring the map of publications on the health impacts of drought. Nat. Hazards 2022, 113, 35–62. [Google Scholar] [CrossRef]
- Wilhite, D.; Glantz, M. Understanding: The drought phenomenon: The role of definitions. . Water Int. 1985, 10, 111–120. [Google Scholar] [CrossRef]
- Mishra, A.K.; Singh, V.P. A review of drought concepts. J. Hydrol. 2010, 391, 202–216. [Google Scholar] [CrossRef]
- Wood, E.F.; Schubert, S.D.; Wood, A.W.; Peters-Lidard, C.D.; Mo, K.C.; Mariotti, A.; Pulwarty, R.S. Prospects for Advancing Drought Understanding, Monitoring, and Prediction. J. Hydrometeorol. 2015, 16, 1636–1657. [Google Scholar] [CrossRef]
- Guo, H.; Bao, A.; Ndayisaba, F.; Liu, T.; Jiapaer, G.; El-Tantawi, A.M.; Maeyer, P.D. Space-time characterization of drought events and their impacts on vegetation in Central Asia. J. Hydrol. 2018, 564, 1165–1178. [Google Scholar] [CrossRef]
- McKee, T.B.; Doesken, N.J.; Kleist, J. The relationship of drought frequency and duration to time scales. In Proceedings of the 8th Conference on Applied Climatology, Anaheim, CA, USA, 17–22 January 1993; Volume 17, pp. 179–183. [Google Scholar]
- Palmer, W.C. Meteorological Drought; US Department of Commerce, Weather Bureau.: Silver Spring, MD, USA, 1965; Volume 30.
- Vicente-Serrano, S.M.; Beguería, S.; López-Moreno, J.I. A Multiscalar Drought Index Sensitive to Global Warming: The Standardized Precipitation Evapotranspiration Index. J. Clim. 2010, 23, 1696–1718. [Google Scholar] [CrossRef]
- Ashraf, M.; Routray, J.K. Spatio-temporal characteristics of precipitation and drought in Balochistan Province, Pakistan. Nat. Hazards 2015, 77, 229–254. [Google Scholar] [CrossRef]
- Damberg, L.; AghaKouchak, A. Global trends and patterns of drought from space. Theor. Appl. Climatol. 2014, 117, 441–448. [Google Scholar] [CrossRef]
- Allen, R.; Pereira, L.; Raes, D.; Smith, M. Crop Evapotranspiration-Guidelines for Computing Crop Water Requirements-FAO Irrigation and Drainage Paper 56; FAO: Rome, Italy, 1998; Volume 300.
- Xu, K.; Yang, D.; Yang, H.; Li, Z.; Qin, Y.; Shen, Y. Spatio-temporal variation of drought in China during 1961–2012: A climatic perspective. J. Hydrol. 2015, 526, 253–264. [Google Scholar] [CrossRef]
- Yeh, H.-F. Spatiotemporal Variation of the Meteorological and Groundwater Droughts in Central Taiwan. Front. Water 2021, 3, 636792. [Google Scholar] [CrossRef]
- Yeh, H.-F. Using integrated meteorological and hydrological indices to assess drought characteristics in southern Taiwan. Hydrol. Res. 2019, 50, 901–914. [Google Scholar] [CrossRef]
- Yeh, H.-F.; Hsu, H.-L. Stochastic Model for Drought Forecasting in the Southern Taiwan Basin. Water 2019, 11, 2041. [Google Scholar] [CrossRef]
- Li, L.; She, D.; Zheng, H.; Lin, P.; Yang, Z.-L. Elucidating Diverse Drought Characteristics from Two Meteorological Drought Indices (SPI and SPEI) in China. J. Hydrometeorol. 2020, 21, 1513–1530. [Google Scholar] [CrossRef]
- Naz, F.; Dars, G.H.; Ansari, K.; Jamro, S.; Krakauer, N.Y. Drought Trends in Balochistan. Water 2020, 12, 470. [Google Scholar] [CrossRef]
- Lloyd-Hughes, B.; Saunders, M.A. A drought climatology for Europe. Int. J. Climatol. A J. R. Meteorol. Soc. 2002, 22, 1571–1592. [Google Scholar] [CrossRef]
- Mishra, A.K.; Singh, V.P. Drought modeling—A review. J. Hydrol. 2011, 403, 157–175. [Google Scholar] [CrossRef]
- Genest, C.; Favre, A.-C. Everything You Always Wanted to Know about Copula Modeling but Were Afraid to Ask. J. Hydrol. Eng. 2007, 12, 347–367. [Google Scholar] [CrossRef]
- Goel, N.K.; Seth, S.M.; Chandra, S. Multivariate Modeling of Flood Flows. J. Hydraul. Eng. 1998, 124, 146–155. [Google Scholar] [CrossRef]
- Yue, S. Applying bivariate normal distribution to flood frequency analysis. Water Int. 1999, 24, 248–254. [Google Scholar] [CrossRef]
- Yue, S.; Ouarda, T.B.M.J.; Bobée, B. A review of bivariate gamma distributions for hydrological application. J. Hydrol. 2001, 246, 1–18. [Google Scholar] [CrossRef]
- Singh, K.; Singh, V.P. Derivation of bivariate probability density functions with exponential marginals. Stoch. Hydrol. Hydraul. 1991, 5, 55–68. [Google Scholar] [CrossRef]
- Yue, S.; Ouarda, T.B.M.J.; Bobée, B.; Legendre, P.; Bruneau, P. The Gumbel mixed model for flood frequency analysis. J. Hydrol. 1999, 226, 88–100. [Google Scholar] [CrossRef]
- Shiau, J.T. Return period of bivariate distributed extreme hydrological events. Stoch. Environ. Res. Risk Assess. 2003, 17, 42–57. [Google Scholar] [CrossRef]
- Le, H.M.; Corzo, G.; Medina, V.; Diaz, V.; Nguyen, B.L.; Solomatine, D.P. A Comparison of Spatial–Temporal Scale between Multiscalar Drought Indices in the South Central Region of Vietnam. In Spatiotemporal Analysis of Extreme Hydrological Events; Elsevier: Amsterdam, The Netherlands, 2019; pp. 143–169. [Google Scholar] [CrossRef]
- Diaz, V.; Perez, G.A.C.; Lanen, H.A.J.V.; Solomatine, D.; Varouchakis, E.A. Characterisation of the dynamics of past droughts. Sci. Total Environ. 2020, 718, 134588. [Google Scholar] [CrossRef]
- Herrera-Estrada, J.E.; Satoh, Y.; Sheffield, J. Spatiotemporal dynamics of global drought. Geophys. Res. Lett. 2017, 44, 2254–2263. [Google Scholar] [CrossRef]
- Zhou, H.; Liu, Y.; Liu, Y. An Approach to Tracking Meteorological Drought Migration. Water Resour. Res. 2019, 55, 3266–3284. [Google Scholar] [CrossRef]
- Han, Z.; Huang, Q.; Huang, S.; Leng, G.; Bai, Q.; Liang, H.; Wang, L.; Zhao, J.; Fang, W. Spatial-temporal dynamics of agricultural drought in the Loess Plateau under a changing environment: Characteristics and potential influencing factors. Agric. Water Manag. 2021, 244, 106540. [Google Scholar] [CrossRef]
- Wang, Y.-L. Characterizing Subsurface Hydraulic Characteristics at Zhuoshui River Alluvial Fan, Taiwan; The University of Arizona ProQuest Dissertations Publishing: Tucson, AZ, USA, 2016. [Google Scholar]
- Yeh, H.-F.; Chang, C.-F. Using Standardized Groundwater Index and Standardized Precipitation Index to Assess Drought Characteristics of the Kaoping River Basin, Taiwan. Water Resour. 2019, 46, 670–678. [Google Scholar]
- Sklar, A. Random variables, joint distribution functions, and copulas. Kybernetika 1973, 9, 449–460. [Google Scholar]
- Guo, Y.; Zhang, J.; Li, K.; Aru, H.; Feng, Z.; Liu, X.; Tong, Z. Quantifying hazard of drought and heat compound extreme events during maize (Zea mays L.) growing season using Magnitude Index and Copula. Weather Clim. Extrem. 2023, 40, 100566. [Google Scholar] [CrossRef]
- Brunner, M.I. Floods and droughts: A multivariate perspective. Hydrol. Earth Syst. Sci. 2023, 27, 2479–2497. [Google Scholar] [CrossRef]
- Pandey, V.; Pandey, P.K.; Lalrammawii, H.P. Characterization and return period analysis of meteorological drought under the humid subtropical climate of Manipur, northeast India. Nat. Hazards Res. 2023, 3, 546–555. [Google Scholar] [CrossRef]
- Nelsen, R.B. An Introduction to Copulas; Springer: Berlin/Heidelberg, Germany, 2006. [Google Scholar]
- Genest, C.; Rémillard, B.; Beaudoin, D. Goodness-of-fit tests for copulas: A review and a power study. Insur. Math. Econ. 2009, 44, 199–213. [Google Scholar] [CrossRef]
- Tsakiris, G.; Kordalis, N.; Tigkas, D.; Tsakiris, V.; Vangelis, H. Analysing Drought Severity and Areal Extent by 2D Archimedean Copulas. Water Resour. Manag. 2016, 30, 5723–5735. [Google Scholar] [CrossRef]
- Friedman, M. On the analysis and solution of certain geographical optimal covering problems. Comput. Oper. Res. 1976, 3, 283–294. [Google Scholar] [CrossRef]
- Johnston, K.; Hoef, J.V.; Krivoruchko, K.; Lucas, N. Using ArcGIS Geostatistical Analyst; Esri Press: Washington, DC, USA, 2001. [Google Scholar]
- Dai, M.; Huang, S.; Huang, Q.; Leng, G.; Guo, Y.; Wang, L.; Fang, W.; Li, P.; Zheng, X. Assessing agricultural drought risk and its dynamic evolution characteristics. Agric. Water Manag. 2020, 231, 106003. [Google Scholar] [CrossRef]
- Kao, S.-C.; Govindaraju, R.S. A copula-based joint deficit index for droughts. J. Hydrol. 2010, 380, 121–134. [Google Scholar] [CrossRef]
- Hung, C.-w.; Hsu, H.-H.; Lu, M.-M. Decadal oscillation of spring rain in northern Taiwan. Geophys. Res. Lett. 2004, 31. [Google Scholar] [CrossRef]
- Hau, N.-X.; Sano, M.; Nakatsuka, T.; Chen, S.-H.; Chen, I.-C. The modulation of Pacific Decadal Oscillation on ENSO-East Asian summer monsoon relationship over the past half-millennium. Sci. Total Environ. 2022, 857, 159437. [Google Scholar] [CrossRef] [PubMed]
- Weng, S.-P. Constructing a 1-km Gridded Multi-Scalar Drought Index Dataset (1960–2012) in Taiwan Based on the Standardized Precipitation Evapotranspiration Index-SPEI. Terr. Atmos. Ocean. Sci. 2016, 27, 625–648. [Google Scholar] [CrossRef]
- Jiang, Z.; Chen, G.T.-J.; Wu, M.-C. Large-Scale Circulation Patterns Associated with Heavy Spring Rain Events over Taiwan in Strong ENSO and Non-ENSO Years. Mon. Weather Rev. 2003, 131, 1769–1782. [Google Scholar] [CrossRef]


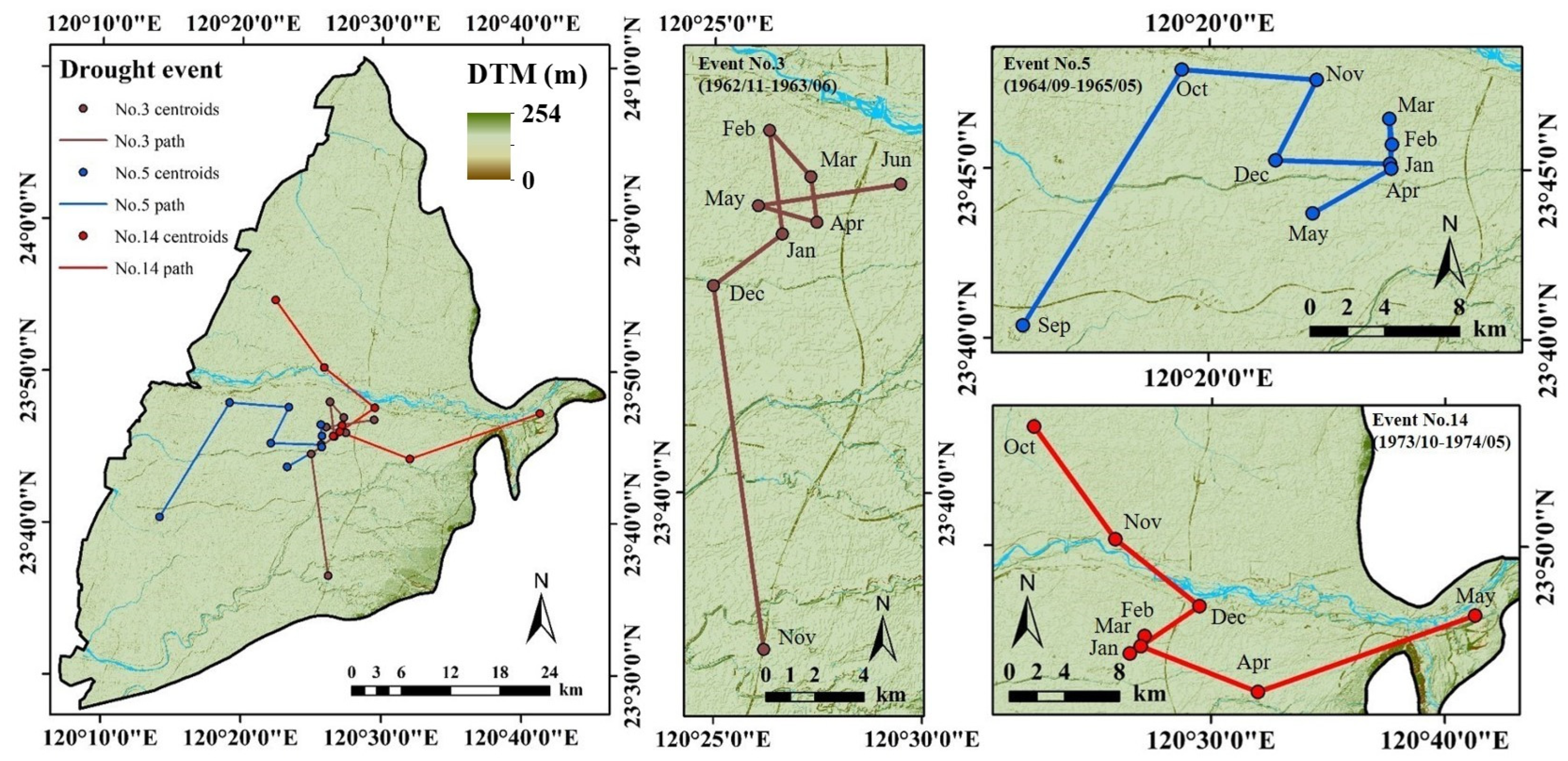
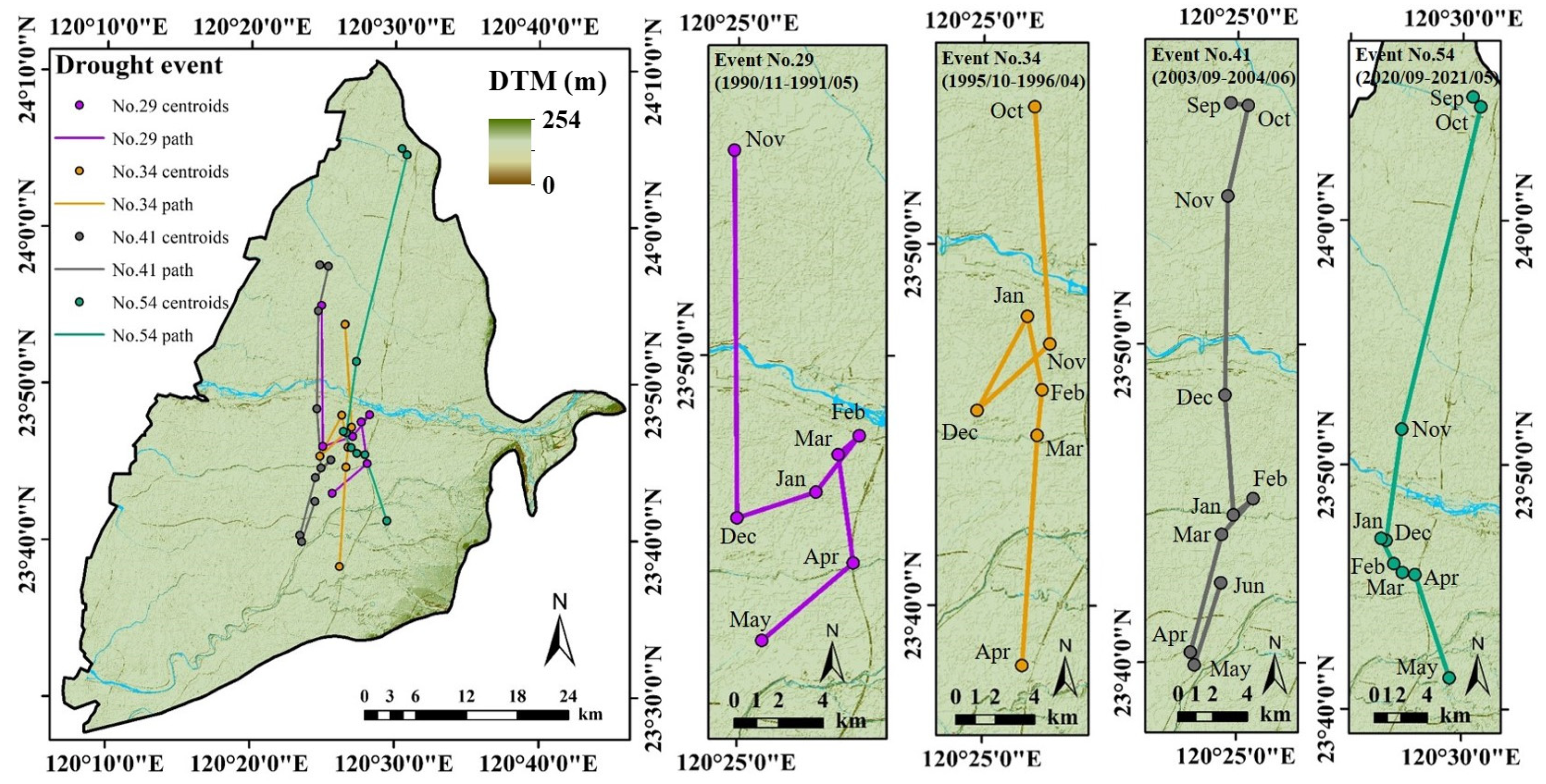
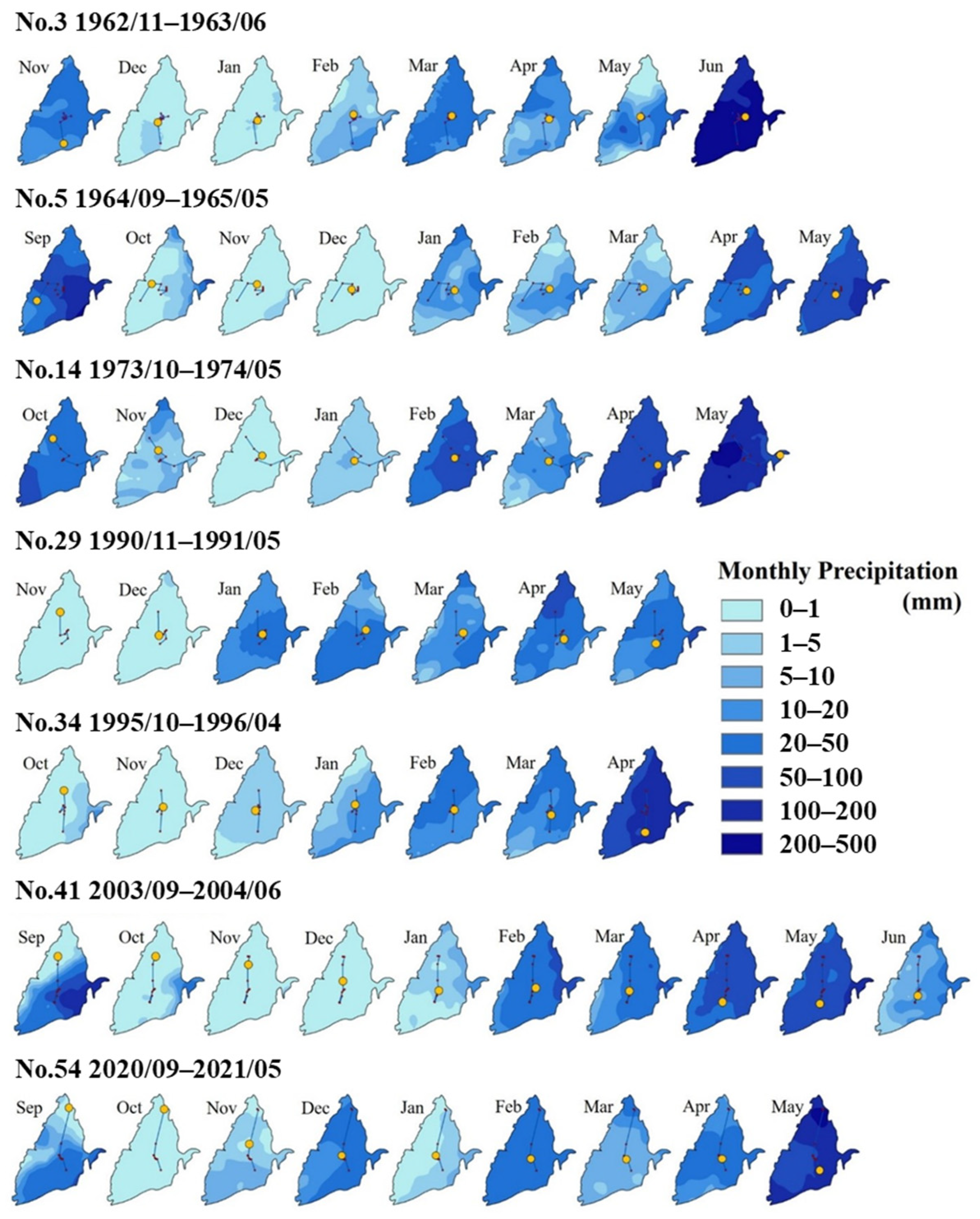
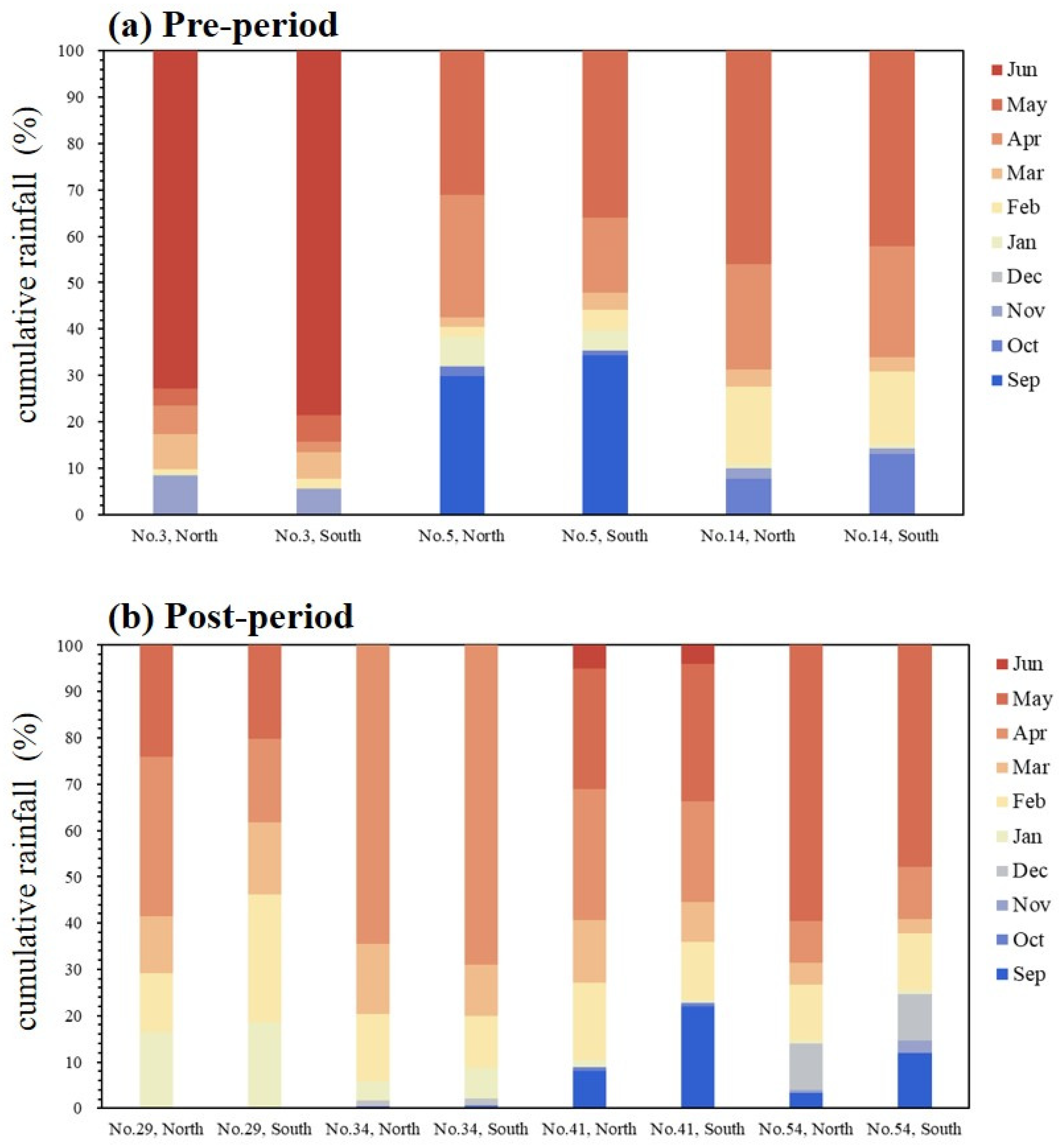
| Time | S | E | SE | SW | Others |
|---|---|---|---|---|---|
| all | 38.9% | 14.8% | 14.8% | 14.8% | 16.7% |
| pre-period (1960–1981) | 23.8% | 23.8% | 23.8% | 9.5% | 19.0% |
| post-period (1981–2021) | 48.5% | 9.1% | 9.1% | 18.2% | 15.2% |
| dd | |||
|---|---|---|---|
| Distributions | Loglikelihood | AIC | BIC |
| exponential | −153.358 | 308.7158 | 310.7048 |
| lognormal | −98.49 | 200.97 | 204.95 |
| gamma | −97.08 | 198.16 | 202.14 |
| Weibull | −97.08 | 198.16 | 202.14 |
| Dc(SPI ≤ −2) | |||
| Distributions | Loglikelihood | AIC | BIC |
| exponential | −229.61 | 461.21 | 463.20 |
| lognormal | −167.55 | 339.09 | 343.07 |
| gamma | −143.38 | 290.76 | 294.73 |
| Weibull | −153.32 | 310.63 | 314.61 |
| Clayton Copula | Gumbel Copula | Frank Copula | |
|---|---|---|---|
| θ | 0.32 | 1.22 | 1.3 |
| AIC | 0.47 | −2.58 | 0.11 |
| BIC | 2.44 | −0.61 | 2.08 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yeh, H.-F.; Lin, X.-Y.; Huang, C.-C.; Chen, H.-Y. A Meteorological Drought Migration Model for Assessing the Spatiotemporal Paths of Drought in the Choushui River Alluvial Fan, Taiwan. Geosciences 2024, 14, 106. https://doi.org/10.3390/geosciences14040106
Yeh H-F, Lin X-Y, Huang C-C, Chen H-Y. A Meteorological Drought Migration Model for Assessing the Spatiotemporal Paths of Drought in the Choushui River Alluvial Fan, Taiwan. Geosciences. 2024; 14(4):106. https://doi.org/10.3390/geosciences14040106
Chicago/Turabian StyleYeh, Hsin-Fu, Xin-Yu Lin, Chia-Chi Huang, and Hsin-Yu Chen. 2024. "A Meteorological Drought Migration Model for Assessing the Spatiotemporal Paths of Drought in the Choushui River Alluvial Fan, Taiwan" Geosciences 14, no. 4: 106. https://doi.org/10.3390/geosciences14040106
APA StyleYeh, H.-F., Lin, X.-Y., Huang, C.-C., & Chen, H.-Y. (2024). A Meteorological Drought Migration Model for Assessing the Spatiotemporal Paths of Drought in the Choushui River Alluvial Fan, Taiwan. Geosciences, 14(4), 106. https://doi.org/10.3390/geosciences14040106








