Physically Based and Empirical Ground Motion Prediction Equations for Multiple Intensity Measures (PGA, PGV, Ia, FIV3, CII, and Maximum Fourier Acceleration Spectra) on Sakhalin Island
Abstract
1. Introduction
2. Materials and Methods
2.1. Materials
2.1.1. Study Area and Tectonic Settings
2.1.2. Strong Motion Database
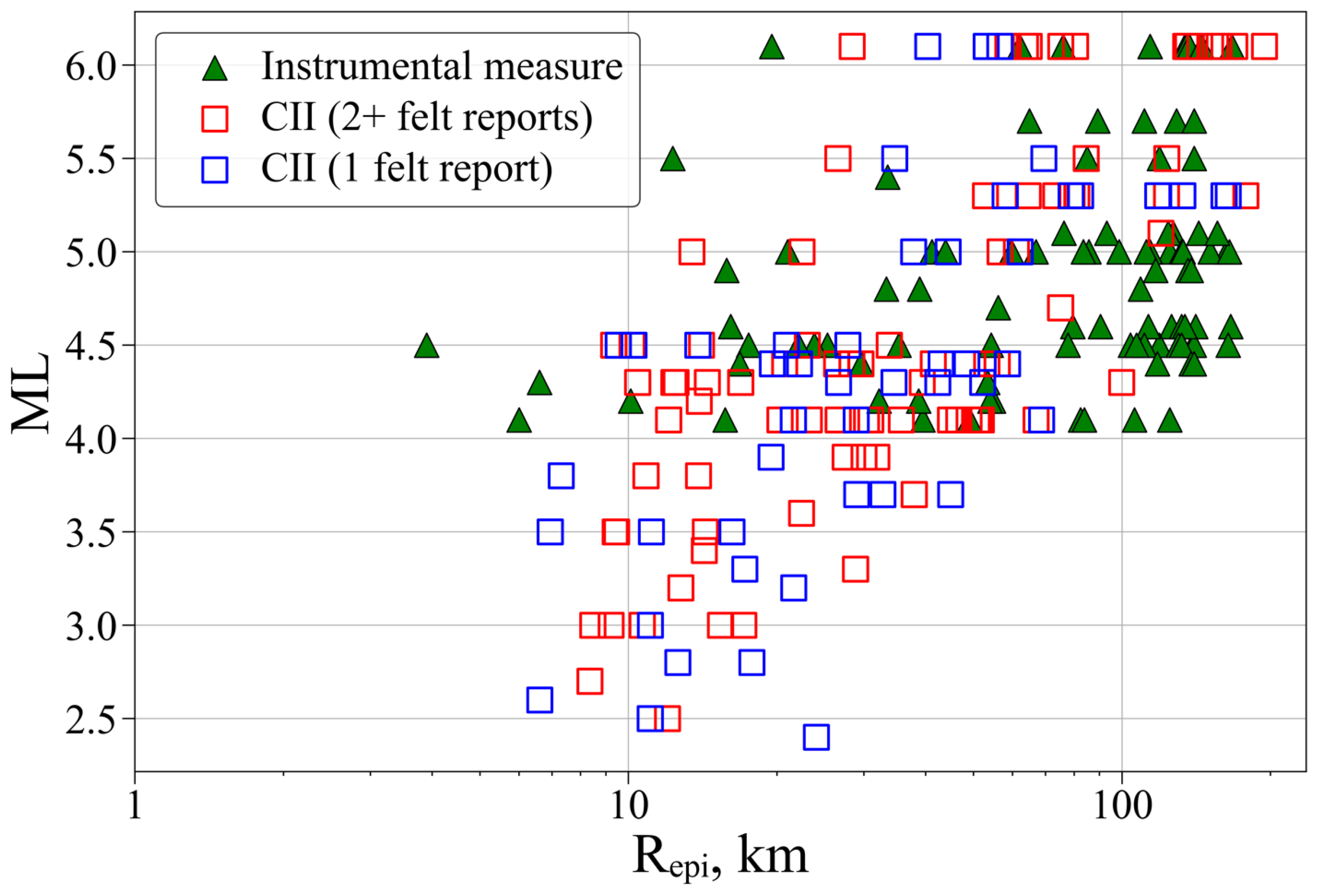
2.1.3. Felt Reports
2.2. Methods
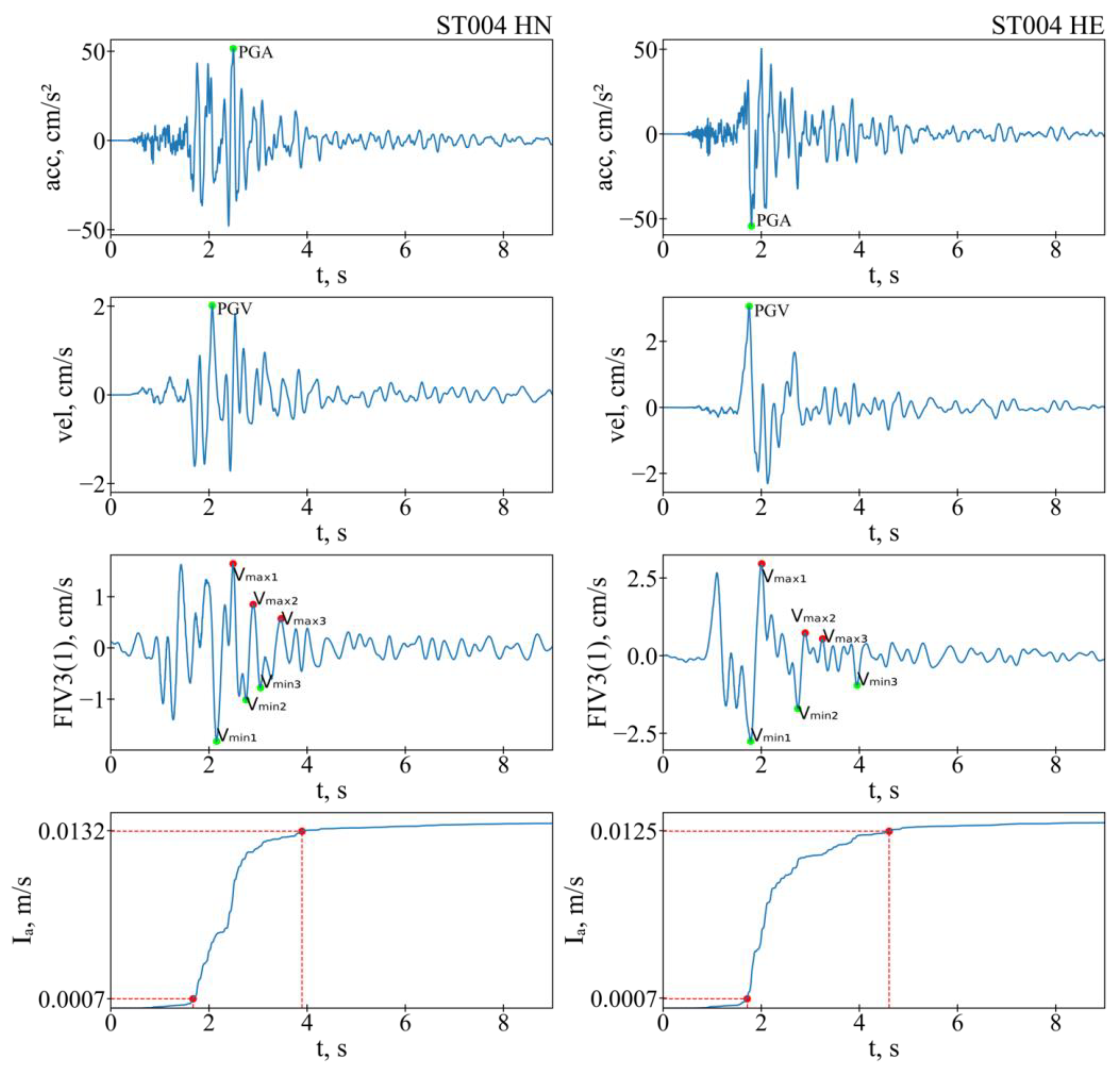
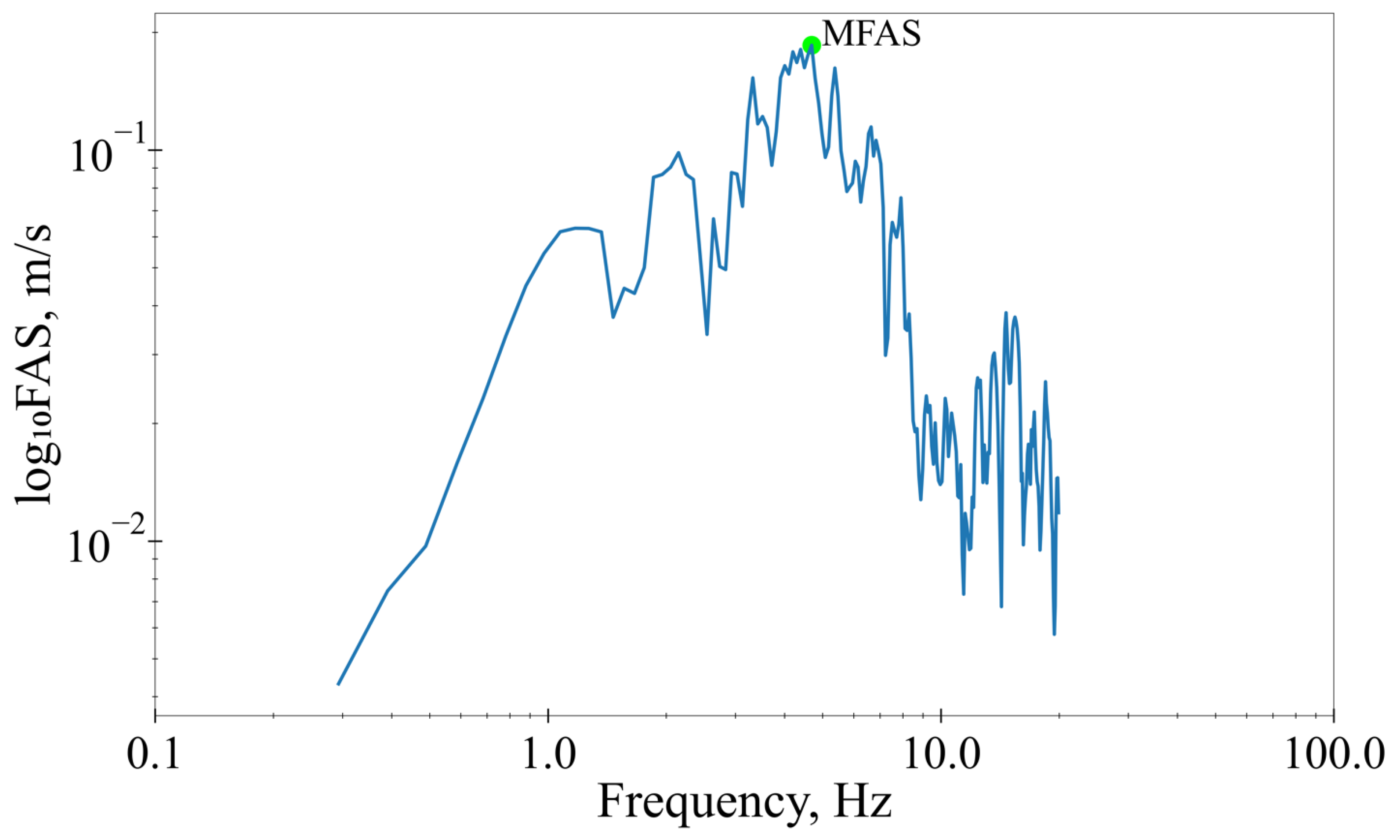
2.2.1. Intensity Measures
2.2.2. Attenuation Model
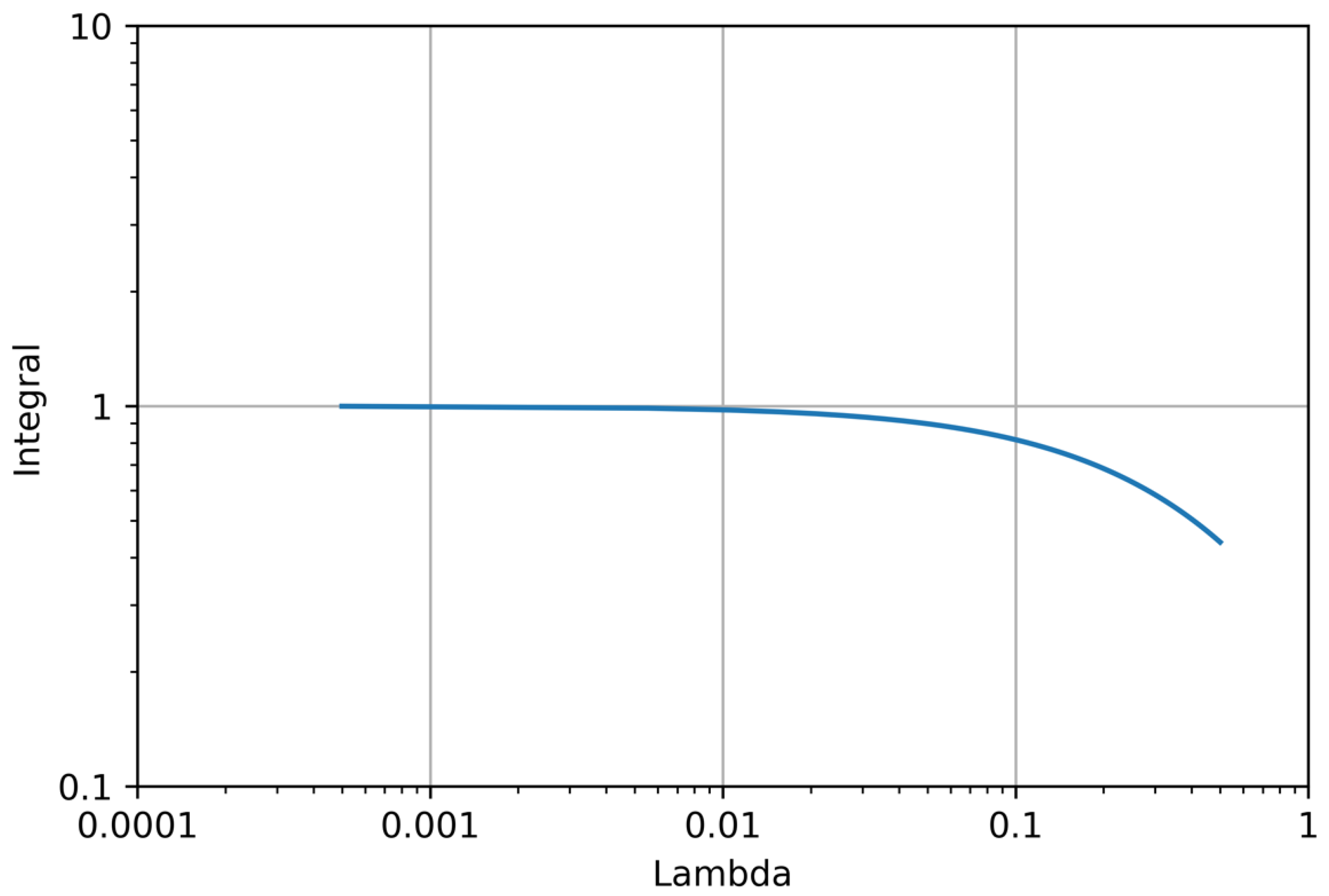
2.2.3. Physical Representation of High-Frequency Measures
3. Results
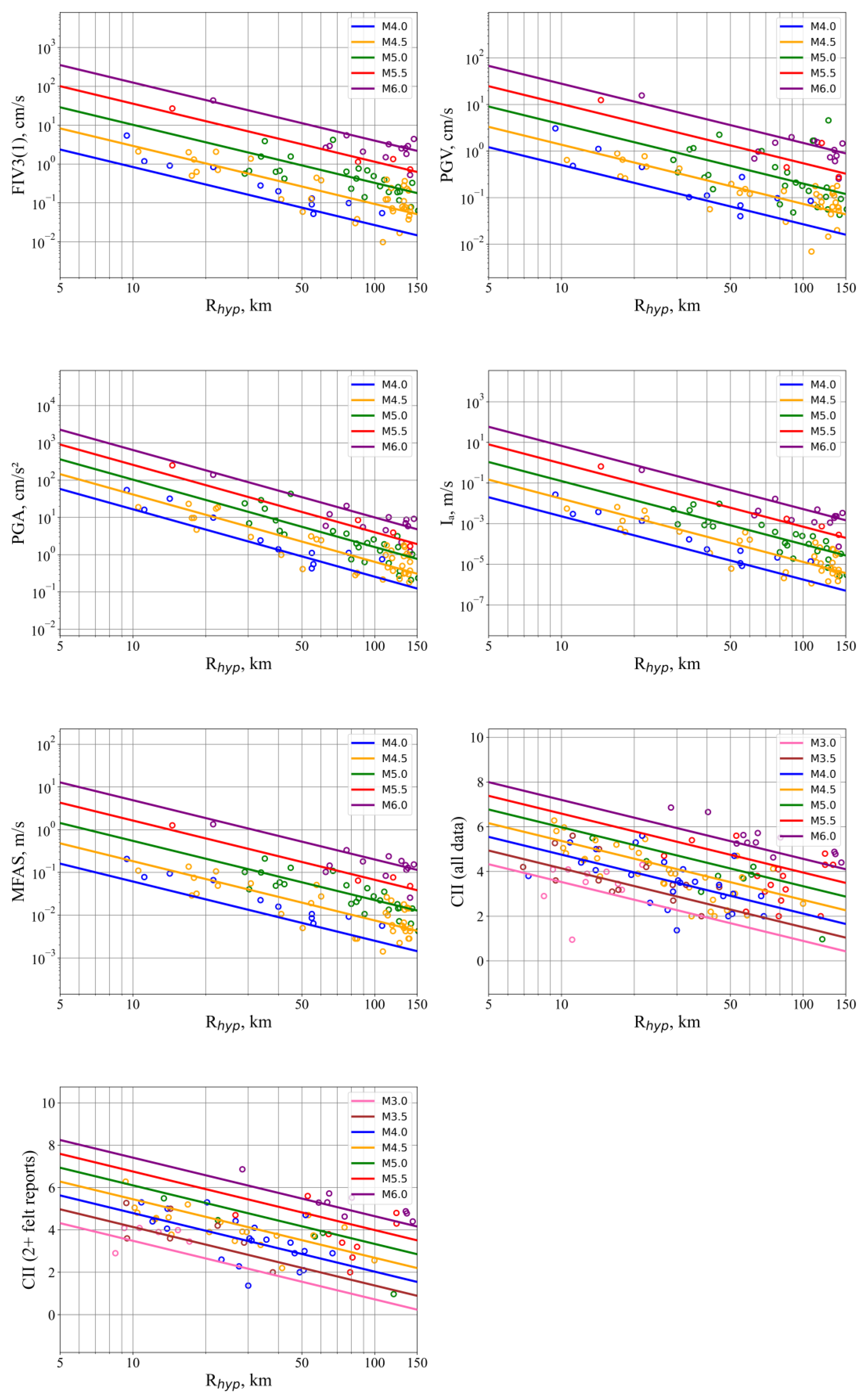
3.1. Performance Metrics of Attenuation Models
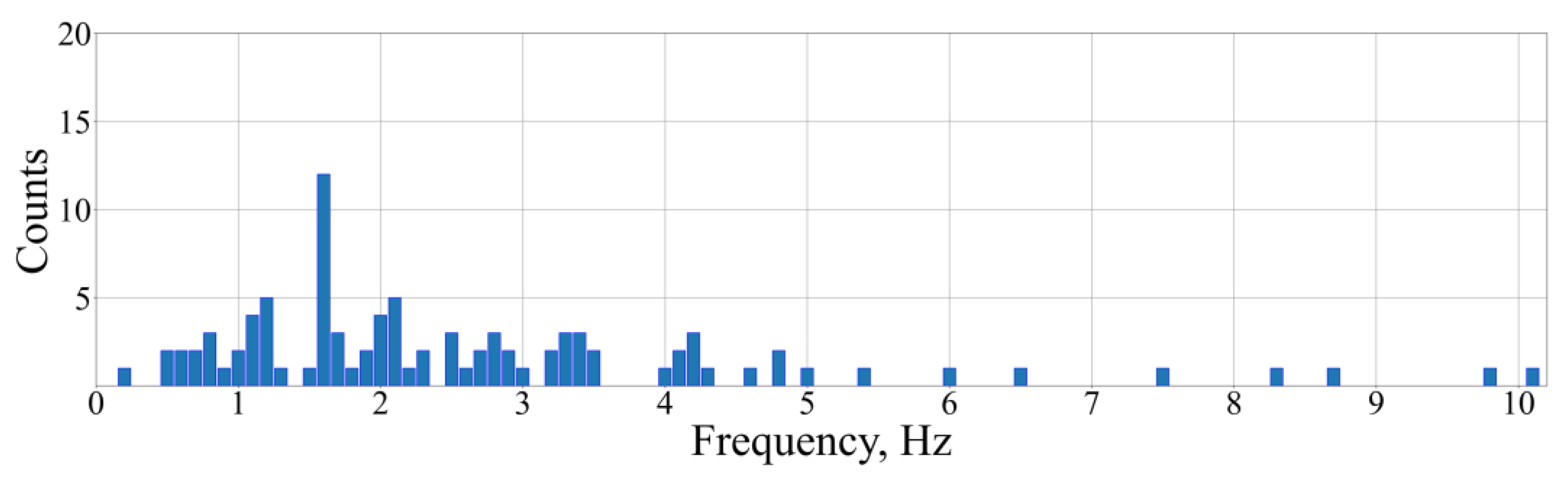
3.2. Average Inner-Fault Parameters and the Physical Interpretation
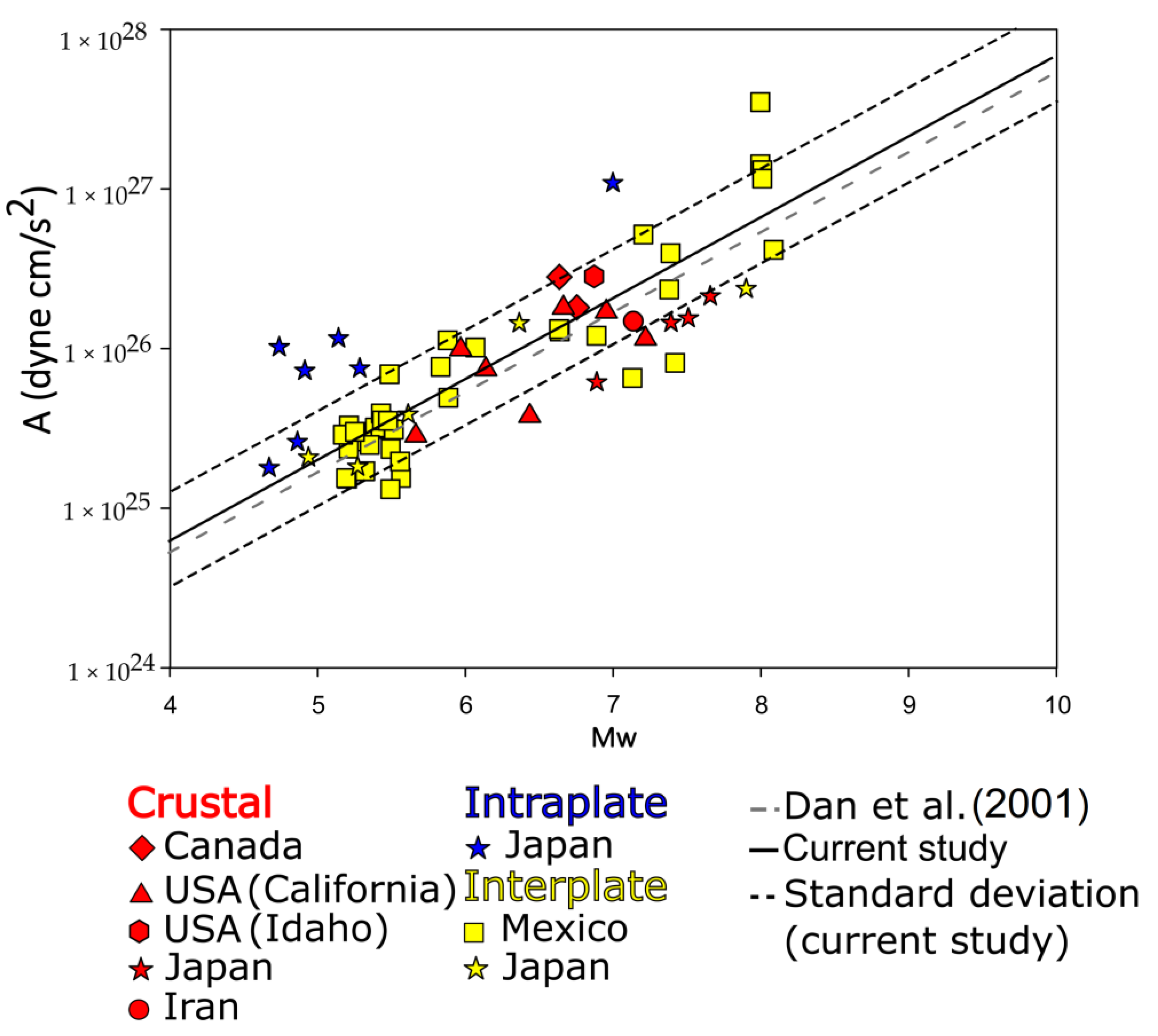
3.3. Acceleration Source Spectral Level
4. Discussion
4.1. Applicability of Spectral Metrics
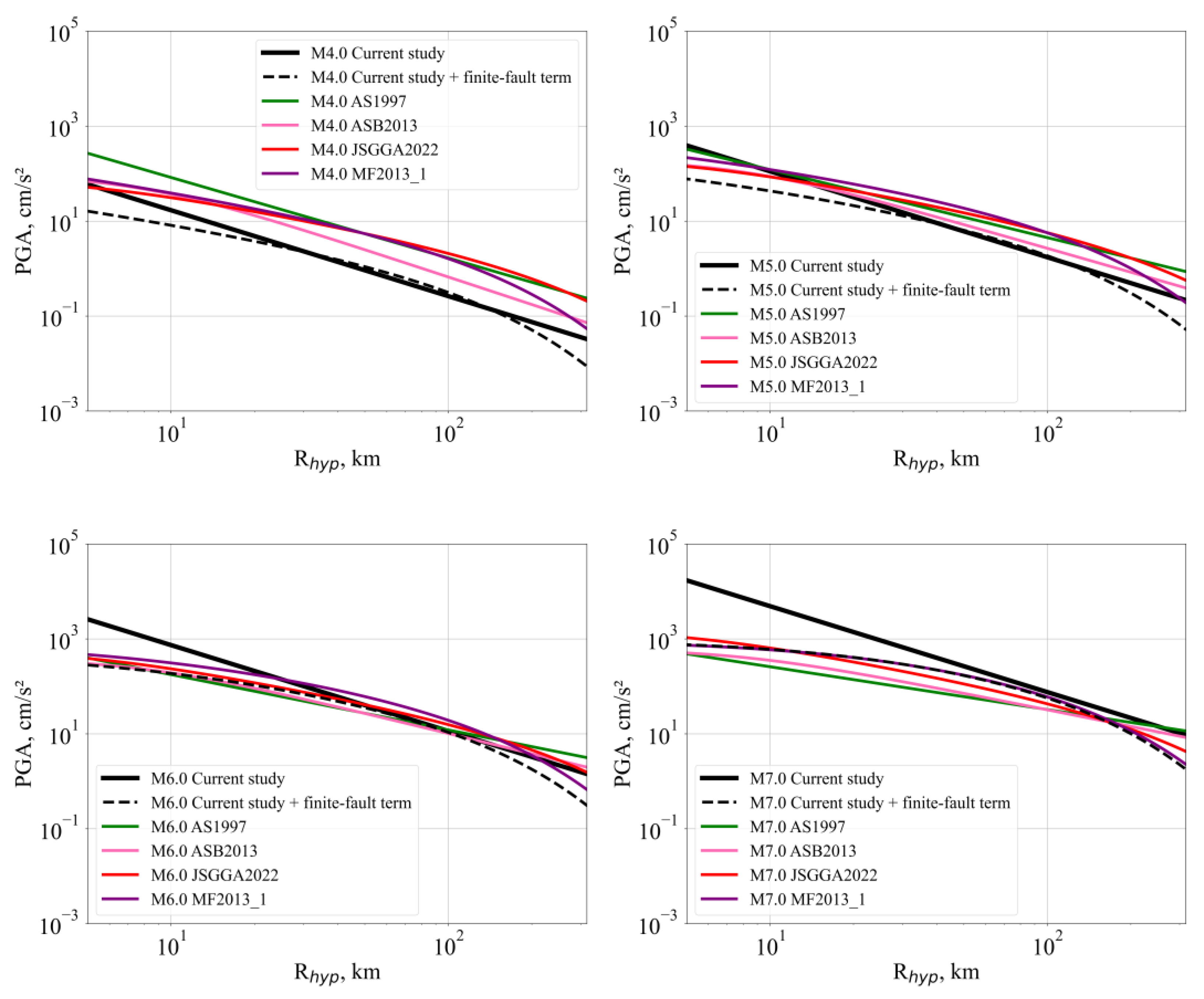
4.2. Comparison of GMPEs and Finite-Fault Effects
4.3. Selection of Point-Source Distance Metrics
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Guaman, J.; Kirkner, D.; Kurama, Y. Empirical ground motion attenuation relationships for maximum incremental velocity. In Proceedings of the 9th US National and 10th Canadian Conference on Earthquake Engineering 2010, Including Papers from the 4th International Tsunami Symposium, Toronto, ON, Canada, 25 July 2010. [Google Scholar]
- Earthquake Research Committee. Seismic Activity in Japan: Regional Perspectives on the Characteristics of Destructive Earthquakes; Science and Technology Agency: Tokyo, Japan, 1998. [Google Scholar]
- Kramer, S.L.; Mitchell, R.A. Ground motion intensity measures for liquefaction hazard evaluation. Earthq. Spectra 2006, 22, 413–438. [Google Scholar] [CrossRef]
- Campbell, K.W.; Bozorgnia, Y. Cumulative absolute velocity (CAV) and seismic intensity based on the PEER-NGA database. Earthq. Spectra 2012, 28, 457–485. [Google Scholar] [CrossRef]
- Ji, D.; Liu, J.; Wen, W.; Zhai, C.; Wang, W.; Katsanos, E.I. Prediction of Cumulative Absolute Velocity Based on Refined Second-order Deep Neural Network. J. Earthq. Eng. 2021, 26, 8021–8040. [Google Scholar] [CrossRef]
- Bullock, Z.; Dashti, S.; Liel, A.; Porter, K.; Karimi, Z.; Bradley, B. Ground-motion prediction equations for Arias intensity, cumulative absolute velocity, and peak incremental ground velocity for rock sites in different tectonic environments. Bull. Seismol. Soc. Am. 2017, 107, 2293–2309. [Google Scholar] [CrossRef]
- Jampole, E.; Miranda, E.; Deierlein, G. Effective incremental ground velocity: An IM to estimate sliding isolation displacement. In Proceedings of the 11th National Conference on Earthquake Engineering, NCEE 2018: Integrating Science, Engineering, and Policy, Los Angeles, CA, USA, 25–29 June 2018. [Google Scholar]
- Dávalos, H.; Miranda, E. Filtered incremental velocity: A novel approach in intensity measures for seismic collapse estimation. Earthq. Eng. Struct. Dyn. 2019, 48, 1384–1405. [Google Scholar] [CrossRef]
- Dávalos, H.; Heresi, P.; Miranda, E. A ground motion prediction equation for filtered incremental velocity, FIV3. Soil Dyn. Earthq. Eng. 2020, 139, 1384–1405. [Google Scholar] [CrossRef]
- Wilson, R.C. Relation of Arias Intensity to Magnitude and Distance in California; US Geological Survey: Reston, VA, USA, 1993. [CrossRef]
- Stafford, P.J.; Berrill, J.B.; Pettinga, J.R. New predictive equations for Arias intensity from crustal earthquakes in New Zealand. J. Seismol. 2009, 13, 31–52. [Google Scholar] [CrossRef]
- Keefer, D.K.; Wilson, R.C. Predicting earthquake-induced landslides, with emphasis on arid and semi-arid environments. Landslides A Semi-Arid. Environ. 1989, 2, 118–149. [Google Scholar]
- Li, X.; Xu, W.; Gao, M. Characteristics of Arias intensity and Newmark displacement of strong ground motion in Lushan earthquake. Acta. Seismol. Sin. 2021, 43, 768–786. [Google Scholar] [CrossRef]
- Chernov, Y.K. Strong Ground Motion and Quantitative Assessment of Seismic Hazard; Fan Publishing House: Tashkent, Uzbekistan, 1989. [Google Scholar]
- Sokolov, V.Y. Seismic intensity and Fourier acceleration spectra: Revised relationship. Earthq. Spectra 2002, 18, 161–187. [Google Scholar] [CrossRef]
- Kotha, S.R.; Bindi, D.; Cotton, F. A regionally adaptable ground-motion model for fourier amplitude spectra of shallow crustal earthquakes in Europe. Bull. Earthq. Eng. 2022, 20, 711–740. [Google Scholar] [CrossRef]
- Vemula, S.; Raghukanth, S.T.G. Generation of a Response Spectrum from a Fourier Spectrum Using a Recurrent Neural Network: Application to New Zealand. Pure Appl. Geophys. 2022, 179, 2797–2816. [Google Scholar] [CrossRef]
- Irikura, K.; Miyake, H. Recipe for predicting strong ground motion from crustal earthquake scenarios. Pure Appl. Geophys. 2011, 168, 85–104. [Google Scholar] [CrossRef]
- Feng, T.; Meng, L. A High-Frequency Distance Metric in Ground-Motion Prediction Equations Based on Seismic Array Backprojections. Geophys. Res. Lett. 2018, 45, 11–612. [Google Scholar] [CrossRef]
- Ito, C.; Takahashi, H.; Ohzono, M. Estimation of convergence boundary location and velocity between tectonic plates in northern Hokkaido inferred by GNSS velocity data. Earth Planets Space 2019, 71, 86. [Google Scholar] [CrossRef]
- García, D.; Wald, D.J.; Hearne, M.G. A global earthquake discrimination scheme to optimize ground-motion prediction equation selection. Bull. Seismol. Soc. Am. 2012, 102, 185–203. [Google Scholar] [CrossRef]
- Konovalov, A.V.; Nagornyh, T.V.; Safonov, D.A. Resent study of earthquake source mechanisms in Sakhalin. In Vladivostok: Dal’nauka; Kozhurin, A.I., Ed.; Dal’nauka: Vladivostok, Russia, 2014; 252p. [Google Scholar]
- Konovalov, A.V.; Sychev, A.S. A calibration curve of local magnitude and intermagnitude relations for northern Sakhalin. J. Volcanol. Seismol. 2014, 8, 390–400. [Google Scholar] [CrossRef]
- Gusev, A.A.; Mel’nikova, B.N. Relations between magnitudes—World average and for Kamchatka. Vulkanol Seism. 1990, 6, 55–63. [Google Scholar]
- Federal Emergency Management Agency (FEMA), Edition NEHRP Recommended Provisions for Seismic Regulations for New Buildings and Other Structures, Part 1: Provisions (FEMA 302). 1997. Available online: http://www.ce.memphis.edu/7137/PDFs/fema302a.pdf (accessed on 16 August 2022).
- Wald, D.J.; Quitoriano, V.; Dengler, L.A.; Dewey, J.W. Utilization of the Internet for rapid community intensity maps. Seismol. Res. Lett. 1999, 70, 680–697. [Google Scholar] [CrossRef]
- Konovalov, A.V.; Stepnov, A.A.; Bogdanov, E.S.; Dmitrienko, R.Y.; Orlin, I.D.; Sychev, A.S.; Gavrilov, A.V.; Manaychev, K.A.; Tsoy, A.T.; Stepnova, Y.A. New Tools for Rapid Assessment of Felt Reports and a Case Study on Sakhalin Island. Seism. Instruments. 2022, 58, 676–693. [Google Scholar] [CrossRef]
- Si, H.; Midorikawa, S. New Attenuation Relationships for Peak Ground Acceleration and Velocity Considering Effects of Fault Type and Site Condition. J. Struct. Constr. Eng. 1999, 64, 63–70. [Google Scholar] [CrossRef]
- Morikawa, N.; Fujiwara, H. A new ground motion prediction equation for Japan applicable up to M9 mega-earthquake. J. Disaster Res. 2013, 8, 878–888. [Google Scholar] [CrossRef]
- Fukushima, Y.; Tanaka, T. A new attenuation relation for peak horizontal acceleration of strong earthquake ground motion in Japan. Bull. Seismol. Soc. Am. 1990, 80, 757–783. [Google Scholar] [CrossRef]
- Kanno, T.; Narita, A.; Morikawa, N.; Fujiwara, H.; Fukushima, Y. A New Attenuation Relation for Strong Ground Motion in Japan Based on Recorded Data. Bull. Seismol. Soc. Am. 2006, 96, 879–897. [Google Scholar] [CrossRef]
- Joyner, W.B.; Boore, D.M. Peak horizontal acceleration and velocity from strong-motion records including records from the 1979 imperial valley, California, earthquake. Bull. Seismol. Soc. Am. 1981, 71, 2011–2038. [Google Scholar] [CrossRef]
- Hanks, T.C.; McGuire, R.K. The character of high-frequency strong ground motion. Bull. Seismol. Soc. Am. 1981, 71, 2071–2095. [Google Scholar] [CrossRef]
- Madariaga, R. High-frequency radiation from crack (stress drop) models of earthquake faulting. Geophys. J. Int. 1977, 51, 625–651. [Google Scholar] [CrossRef]
- Gusev, A.A. Multiasperity fault model and the nature of short-period subsources. Pure Appl. Geophys. 1989, 130, 635–660. [Google Scholar] [CrossRef]
- Gusev, A.A. Random kinematics of unbounded earthquake rupture propagation simulated using a cellular model. Geophys. J. Int. 2018, 215, 924–941. [Google Scholar] [CrossRef]
- Boore, D.M. Simulation of Ground Motion Using the Stochastic Method. Pure Appl. Geophys. 2003, 160, 635–676. [Google Scholar] [CrossRef]
- Bansal, B.K.; Singh, S.K.; Suresh, G.; Mittal, H. A source and ground motion study of earthquakes in and near Delhi (the National Capital Region), India. Nat. Hazards 2022, 111, 1885–1905. [Google Scholar] [CrossRef]
- Konovalov, A.V.; Sychev, A.S.; Solov’ev, V.N. Mass estimates of the scalar seismic moments of small earthquake foci on southern Sakhalin. Russ. J. Pacific. Geol. 2011, 5, 225–233. [Google Scholar] [CrossRef]
- Kosuga, M. Dependence of Coda Q on Frequency and Lapse Time in the Western Nagano Region, Central Japan. J. Phys. Earth 1992, 40, 421–445. [Google Scholar] [CrossRef]
- Kostrov, B.V. Mechanics of Tectonic Earthquake Source; Nauka: Moscow, Russia, 1975; 176p. [Google Scholar]
- Hanks, T.C.; Kanamori, H. A moment magnitude scale. J. Geophys. Res. B Solid. Earth 1979, 84, 2348–2350. [Google Scholar] [CrossRef]
- Xu, B.; Rathje, E.M.; Hashash, Y.M.A.; Stewart, J.P.; Campbell, K.W.; Silva, W.J. k0 for soil sites: Observations from KiK-net sites and their use in constraining small-strain damping profiles for site response analysis. Earthq. Spectra 2020, 36, 111–137. [Google Scholar] [CrossRef]
- Satoh, T.; Okazaki, A. Relation Between Stress Drops and Depths of Strong Motion Generation Areas Based on Previous Broadband Source Models for Crustal Earthquakes in Japan. In Earthquakes, Tsunamis and Nuclear Risks, Predicition and Assessment beyond the Fukushima Accident, Part II; Kamae, K., Ed.; OAPEN Library: Hague, The Netherlands, 2016; pp. 77–85. [Google Scholar] [CrossRef]
- Dan, K.; Watanabe, M.; Sato, T.; Ishii, T. Short-period source spectra inferred from variable-slip rupture models and modeling of earthquake faults for strong motion prediction by semi-empirical method. J. Struct. Constr. Eng. 2001, 66, 51–62. [Google Scholar] [CrossRef]
- González, J.; Irikura, K. Source Characterization of Mexican Subduction Earthquakes from Acceleration Source Spectra for the Prediction of Strong Ground Motions. Bull. Seismol. Soc. Am. 2007, 97, 1960–1969. [Google Scholar] [CrossRef]
- Akkar, S.; Sandıkkaya, M.A.; Bommer, J.J. Empirical ground-motion models for point- and extended-source crustal earthquake scenarios in Europe and the Middle East. Bull. Earthq. Eng. 2014, 12, 359–387. [Google Scholar] [CrossRef]
- Yao, Q.; Wang, D.; Fang, L.; Mori, J. Rapid Estimation of Magnitudes of Large Damaging Earthquakes in and around Japan Using Dense Seismic Stations in China. Bull. Seismol. Soc. Am. 2019, 109, 2545–2555. [Google Scholar] [CrossRef]
- Abrahamson, N.A.; Silva, W.J. Empirical response spectral attenuation relations for shallow crustal earthquakes. Seismol. Res. Lett. 1997, 68, 94–109. [Google Scholar] [CrossRef]
- Jorjiashvili, N.; Shengelia, I.; Godoladze, T.; Gunia, I.; Akubardia, D. Ground motion prediction equations based on shallow crustal earthquakes in Georgia and the surrounding Caucasus. Earthq. Sci. 2022, 35, 497–509. [Google Scholar] [CrossRef]
- Allen, R.M.; Melgar, D. Earthquake Early Warning: Advances, Scientific Challenges, and Societal Needs. Annu. Rev. Earth Planet Sci. 2019, 47, 361–388. [Google Scholar] [CrossRef]


| Measure | Equation |
|---|---|
| , peak ground acceleration (cm/s2) | |
| , peak ground velocity (cm/s) | |
| , Arias intensity (m/s) | |
| , modified Arias intensity (m/s) | |
| , modified Arias intensity (m/s) | |
| , filtered incremental velocity (cm/s) | , where |
| , maximum Fourier acceleration spectra (m/s) |
| Parameter | Value |
|---|---|
| 2 | |
| 1 | |
| 2 | |
| 0.03 s | |
| 2700 kg/m3 | |
| 3300 m/s | |
| 1000 m | |
| 9.81 m/s2 | |
| 3 × 106 Pa |
| (a) | ||||||
| IM | a | k | b | c | σ | |
| lg FIV3(0.01) (cm/s)], Mw | 0.94 ± 0.08 | 1.64 ± 0.11 | 0 * | −2.89 ± 0.37 | 0.328 | 0.757 |
| lg FIV3(0.2) (cm/s), Mw | 0.98 ± 0.08 | 1.55 ± 0.11 | 0 * | −2.36 ± 0.38 | 0.34 | 0.736 |
| lg FIV3(1) (cm/s), Mw | 1.12 ± 0.08 | 1.5 ± 0.12 | 0 * | −3.08 ± 0.39 | 0.349 | 0.744 |
| lg FIV3(3) (cm/s), Mw | 1.12 ± 0.08 | 1.47 ± 0.11 | 0 * | −3.13 ± 0.38 | 0.338 | 0.753 |
| lg PGV (cm/s), Mw | 0.9 ± 0.09 | 1.28 ± 0.13 | 0 * | −2.64 ± 0.44 | 0.391 | 0.612 |
| lg PGA (cm/s2), Mw | 0.82 ± 0.08 | 1.81 ± 0.12 | 0 * | −0.24 ± 0.38 | 0.344 | 0.75 |
| lg Iₐ (m/s), Mw | 1.79 ± 0.14 | 3.12 ± 0.19 | 0 * | −6.68 ± 0.65 | 0.58 | 0.782 |
| lg Iₐ(1) (m/s), Mw | 1.43 ± 0.14 | 3.15 ± 0.2 | 0 * | −5.21 ± 0.67 | 0.6 | 0.75 |
| lg Iₐ(3) (m/s), Mw | 1.2 ± 0.16 | 3.23 ± 0.23 | 0 * | −4.73 ± 0.77 | 0.69 | 0.69 |
| lg MFAS (m/s), Mw | 0.98 ± 0.07 | 1.39 ± 0.1 | 0 * | −3.76 ± 0.34 | 0.306 | 0.754 |
| CII(all data), Mw | 1.22 ± 0.14 | 2.64 ± 0.32 | 0 * | 2.5 ± 0.43 | 0.917 | 0.398 |
| CII(2+ felt reports), Mw | 1.31 ± 0.16 | 2.76 ± 0.35 | 0 * | 2.33 ± 0.48 | 0.788 | 0.487 |
| lg FIV3(0.01) (cm/s), ML | 0.88 ± 0.04 | 1.63 ± 0.07 | 0 * | −2.61 ± 0.21 | 0.216 | 0.895 |
| lg FIV3(0.2) (cm/s), ML | 0.91 ± 0.04 | 1.54 ± 0.07 | 0 * | −2.06 ± 0.22 | 0.226 | 0.883 |
| lg FIV3(1) (cm/s), ML | 1.02 ± 0.04 | 1.48 ± 0.08 | 0 * | −2.64 ± 0.22 | 0.23 | 0.889 |
| lg FIV3(3) (cm/s), ML | 1.01 ± 0.04 | 1.31 ± 0.24 | 0.001 ± 0.0017 | −2.82 ± 0.36 | 0.227 | 0.888 |
| lg PGV (cm/s), ML | 0.82 ± 0.06 | 1.2 ± 0.35 | 0.0004 ± 0.0024 | −2.35 ± 0.52 | 0.33 | 0.723 |
| lg PGA (cm/s2), ML | 0.77 ± 0.05 | 1.81 ± 0.09 | 0 * | −0.03 ± 0.25 | 0.263 | 0.854 |
| lg Iₐ (m/s), ML | 1.63 ± 0.08 | 2.87 ± 0.42 | 0.0016 ± 0.0029 | −6.25 ± 0.63 | 0.401 | 0.896 |
| lg Iₐ(1) (m/s), ML | 1.33 ± 0.09 | 2.98 ± 0.49 | 0.0012 ± 0.0034 | −4.99 ± 0.74 | 0.47 | 0.847 |
| lg Iₐ(3) (m/s), ML | 1.11 ± 0.12 | 2.87 ± 0.65 | 0.0025 ± 0.0045 | −4.75 ± 0.98 | 0.621 | 0.749 |
| lg MFAS (m/s), ML | 0.89 ± 0.04 | 1.18 ± 0.22 | 0.0014 ± 0.0015 | −3.58 ± 0.33 | 0.211 | 0.883 |
| CII(all data), ML | 1.09 ± 0.12 | 2.62 ± 0.32 | 0 * | 3.07 ± 0.4 | 0.917 | 0.398 |
| CII(2+ felt reports), ML | 1.15 ± 0.14 | 2.71 ± 0.35 | 0 * | 2.96 ± 0.44 | 0.801 | 0.47 |
| (b) | ||||||
| IM | a | k | b | c | σ | |
| lg FIV3(0.01) (cm/s), Mw | 0.5 * | 1.44 ± 0.12 | 0 * | −1.1 ± 0.2 | 0.382 | 0.669 |
| lg FIV3(0.2) (cm/s), Mw | 0.5 * | 1.34 ± 0.13 | 0 * | −0.4 ± 0.2 | 0.4 | 0.633 |
| lg FIV3(1) (cm/s), Mw | 0.5 * | 1.22 ± 0.14 | 0 * | −0.6 ± 0.3 | 0.445 | 0.584 |
| lg FIV3(3) (cm/s), Mw | 0.5 * | 1.19 ± 0.14 | 0 * | −0.6 ± 0.3 | 0.435 | 0.589 |
| lg PGV (cm/s), Mw | 0.5 * | 1.09 ± 0.14 | 0 * | −1.0 ± 0.3 | 0.429 | 0.532 |
| lg PGA (cm/s2), Mw | 0.5 * | 1.67 ± 0.12 | 0 * | 1.0 ± 0.2 | 0.372 | 0.709 |
| lg Iₐ (m/s), Mw | 1 * | 2.76 ± 0.21 | 0 * | −3.5 ± 0.4 | 0.677 | 0.703 |
| lg Iₐ(1) (m/s), Mw | 1 * | 2.96 ± 0.2 | 0 * | −3.5 ± 0.4 | 0.63 | 0.725 |
| lg Iₐ(3) (m/s), Mw | 1 * | 3.14 ± 0.22 | 0 * | −3.9 ± 0.4 | 0.696 | 0.685 |
| lg MFAS (m/s), Mw | 0.5 * | 1.17 ± 0.12 | 0 * | −1.8 ± 0.2 | 0.373 | 0.634 |
| (c) | ||||||
| IM | a | k | b | c | σ | |
| lg FIV3(0.01) (cm/s), Mw | 0.91 ± 0.08 | 1 * | 0.0038 ± 0.001 | −3.58 ± 0.38 | 0.344 | 0.732 |
| lg FIV3(0.2) (cm/s), Mw | 0.95 ± 0.08 | 1 * | 0.0032 ± 0.001 | −2.96 ± 0.38 | 0.352 | 0.717 |
| lg FIV3(1) (cm/s), Mw | 1.1 ± 0.08 | 1 * | 0.0029 ± 0.001 | −3.62 ± 0.39 | 0.36 | 0.729 |
| lg FIV3(3) (cm/s), Mw | 1.1 ± 0.08 | 1 * | 0.0028 ± 0.001 | −3.64 ± 0.38 | 0.345 | 0.741 |
| lg PGV (cm/s), Mw | 0.89 ± 0.09 | 1 * | 0.0016 ± 0.001 | −2.93 ± 0.43 | 0.394 | 0.606 |
| lg PGA (cm/s2), Mw | 0.78 ± 0.08 | 1 * | 0.0049 ± 0.001 | −1.13 ± 0.4 | 0.364 | 0.72 |
| lg Iₐ (m/s), Mw | 1.74 ± 0.14 | 2 * | 0.0069 ± 0.001 | −7.91 ± 0.66 | 0.601 | 0.766 |
| lg Iₐ(1) (m/s), Mw | 1.38 ± 0.14 | 2 * | 0.0072 ± 0.001 | −6.48 ± 0.68 | 0.62 | 0.733 |
| lg Iₐ(3) (m/s), Mw | 1.16 ± 0.16 | 2 * | 0.0079 ± 0.002 | −6.1 ± 0.77 | 0.704 | 0.678 |
| lg MFAS (m/s), Mw | 0.97 ± 0.07 | 1 * | 0.0024 ± 0.001 | −4.19 ± 0.34 | 0.311 | 0.746 |
| (d) | ||||||
| IM | a | k | b | c | σ | |
| lg FIV3(0.01) (cm/s), Mw | 0.5 * | 1 * | 0.0026 ± 0.0008 | −1.69 ± 0.08 | 0.38 | 0.673 |
| lg FIV3(0.2) (cm/s), Mw | 0.5 * | 1 * | 0.002 ± 0.0009 | −0.9 ± 0.09 | 0.395 | 0.643 |
| lg FIV3(1) (cm/s), Mw | 0.5 * | 1 * | 0.0012 ± 0.001 | −0.88 ± 0.1 | 0.438 | 0.598 |
| lg FIV3(3) (cm/s), Mw | 0.5 * | 1 * | 0.0011 ± 0.0009 | −0.91 ± 0.1 | 0.426 | 0.606 |
| lg PGV (cm/s), Mw | 0.5 * | 1 * | 0.0005 ± 0.0009 | −1.17 ± 0.09 | 0.423 | 0.546 |
| lg PGA (cm/s2), Mw | 0.5 * | 1 * | 0.0042 ± 0.0008 | 0.15 ± 0.08 | 0.377 | 0.7 |
| lg Iₐ (m/s), Mw | 1 * | 2 * | 0.0049 ± 0.0015 | −4.52 ± 0.15 | 0.666 | 0.712 |
| lg Iₐ(1) (m/s), Mw | 1 * | 2 * | 0.0062 ± 0.0014 | −4.73 ± 0.14 | 0.627 | 0.727 |
| lg Iₐ(3) (m/s), Mw | 1 * | 2 * | 0.0075 ± 0.0015 | −5.38 ± 0.16 | 0.699 | 0.682 |
| lg MFAS (m/s), Mw | 0.5 * | 1 * | 0.0011 ± 0.0008 | −2.06 ± 0.08 | 0.364 | 0.651 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Konovalov, A.; Orlin, I.; Stepnov, A.; Stepnova, Y. Physically Based and Empirical Ground Motion Prediction Equations for Multiple Intensity Measures (PGA, PGV, Ia, FIV3, CII, and Maximum Fourier Acceleration Spectra) on Sakhalin Island. Geosciences 2023, 13, 201. https://doi.org/10.3390/geosciences13070201
Konovalov A, Orlin I, Stepnov A, Stepnova Y. Physically Based and Empirical Ground Motion Prediction Equations for Multiple Intensity Measures (PGA, PGV, Ia, FIV3, CII, and Maximum Fourier Acceleration Spectra) on Sakhalin Island. Geosciences. 2023; 13(7):201. https://doi.org/10.3390/geosciences13070201
Chicago/Turabian StyleKonovalov, Alexey, Ilia Orlin, Andrey Stepnov, and Yulia Stepnova. 2023. "Physically Based and Empirical Ground Motion Prediction Equations for Multiple Intensity Measures (PGA, PGV, Ia, FIV3, CII, and Maximum Fourier Acceleration Spectra) on Sakhalin Island" Geosciences 13, no. 7: 201. https://doi.org/10.3390/geosciences13070201
APA StyleKonovalov, A., Orlin, I., Stepnov, A., & Stepnova, Y. (2023). Physically Based and Empirical Ground Motion Prediction Equations for Multiple Intensity Measures (PGA, PGV, Ia, FIV3, CII, and Maximum Fourier Acceleration Spectra) on Sakhalin Island. Geosciences, 13(7), 201. https://doi.org/10.3390/geosciences13070201







