Global Evidence of Obliquity Damping in Climate Proxies and Sea-Level Record during the Last 1.2 Ma: A Missing Link for the Mid-Pleistocene Transition
Abstract
:1. Introduction
1.1. Obliquity–Oblateness Feedback
- Searching worldwide for new observational evidence for the link between obliquity damping and short eccentricity amplification from global and regional (Antarctic, Pacific, Atlantic, Mediterranean, Indian) climate-related proxies (Section 3.1);
- Discussing the role of the long-term cooling trend in the MPT debate and the relationships between orbital forcings and proxies (Section 3.2 and Section 3.3);
- Critically reviewing the requisite theoretical constraints of the ODH to establish that the obliquity–oblateness feedback could be the driving mechanism of the interglacial/glacial damping observed in Mid-Late Pleistocene obliquity responses (Section 3.4);
- Refreshing by new cross-spectral data the role of the short eccentricity forcing in the context of Milankovitch’s theory (Section 3.5).
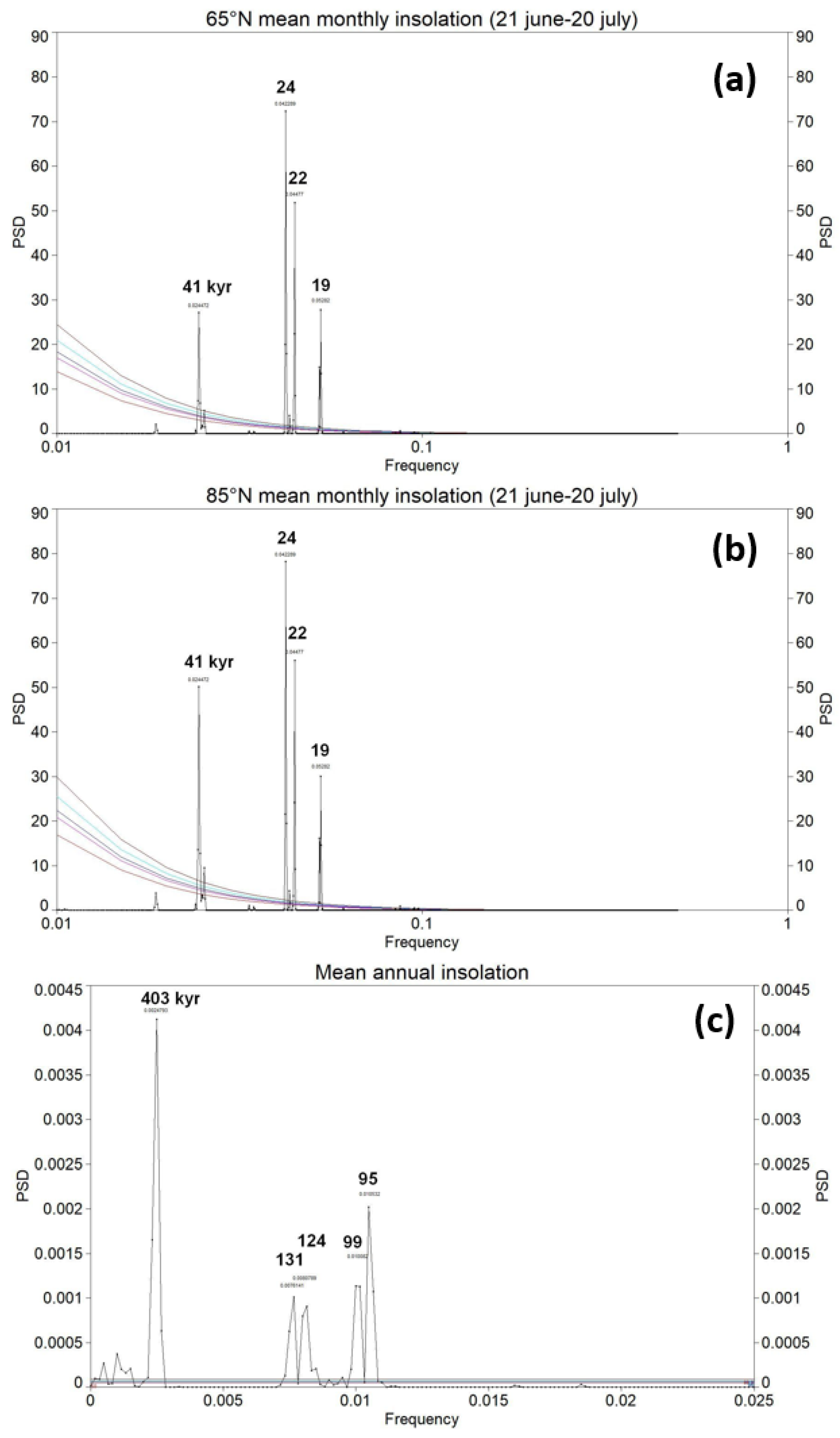
1.2. Key role of Obliquity Forcing on the Earth’s Climate System
2. Materials and Methods
2.1. Materials
2.2. Statistical Methods
3. Results and Discussion
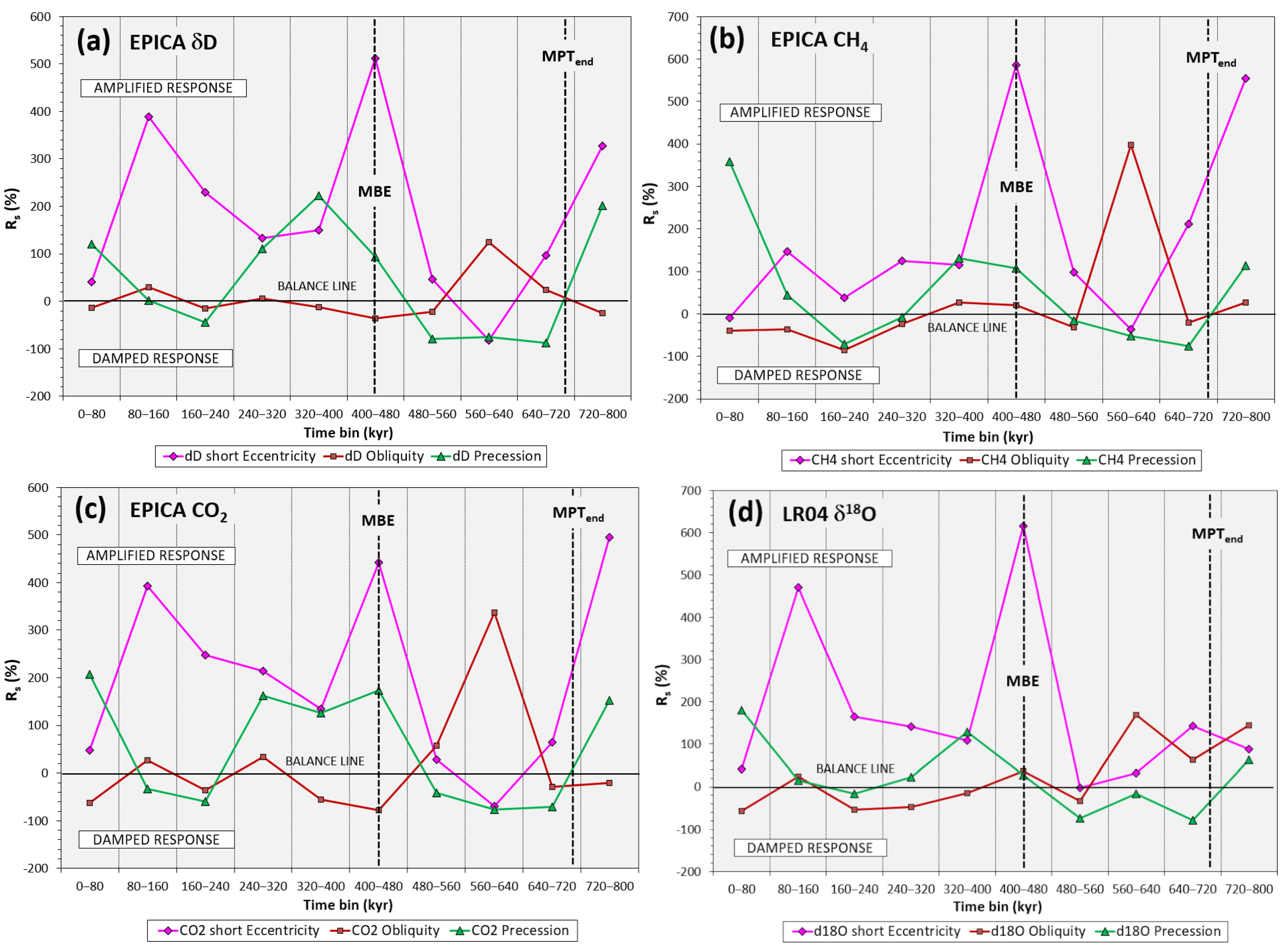
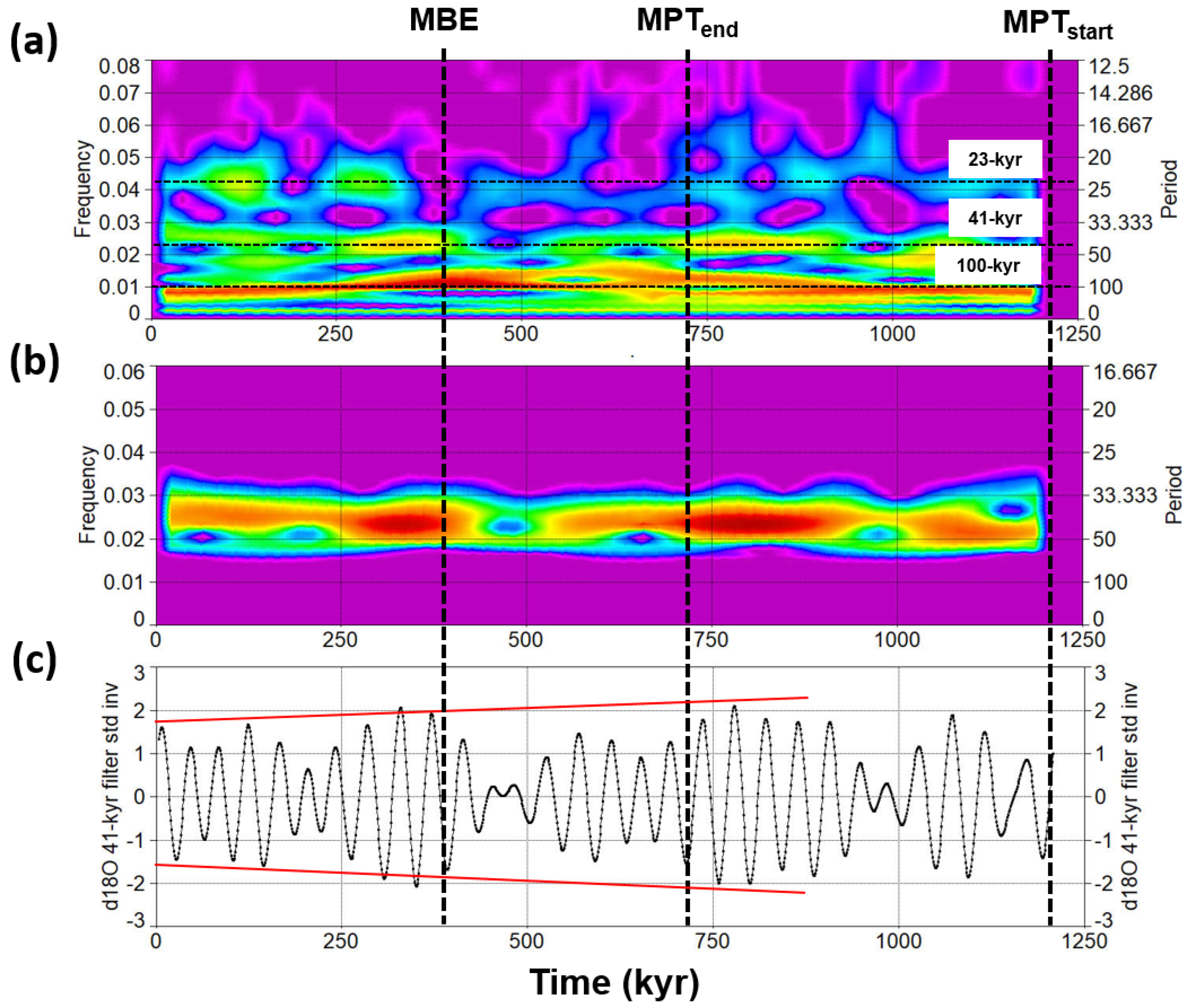
3.1. Evidence of Post-MPT Obliquity Damping
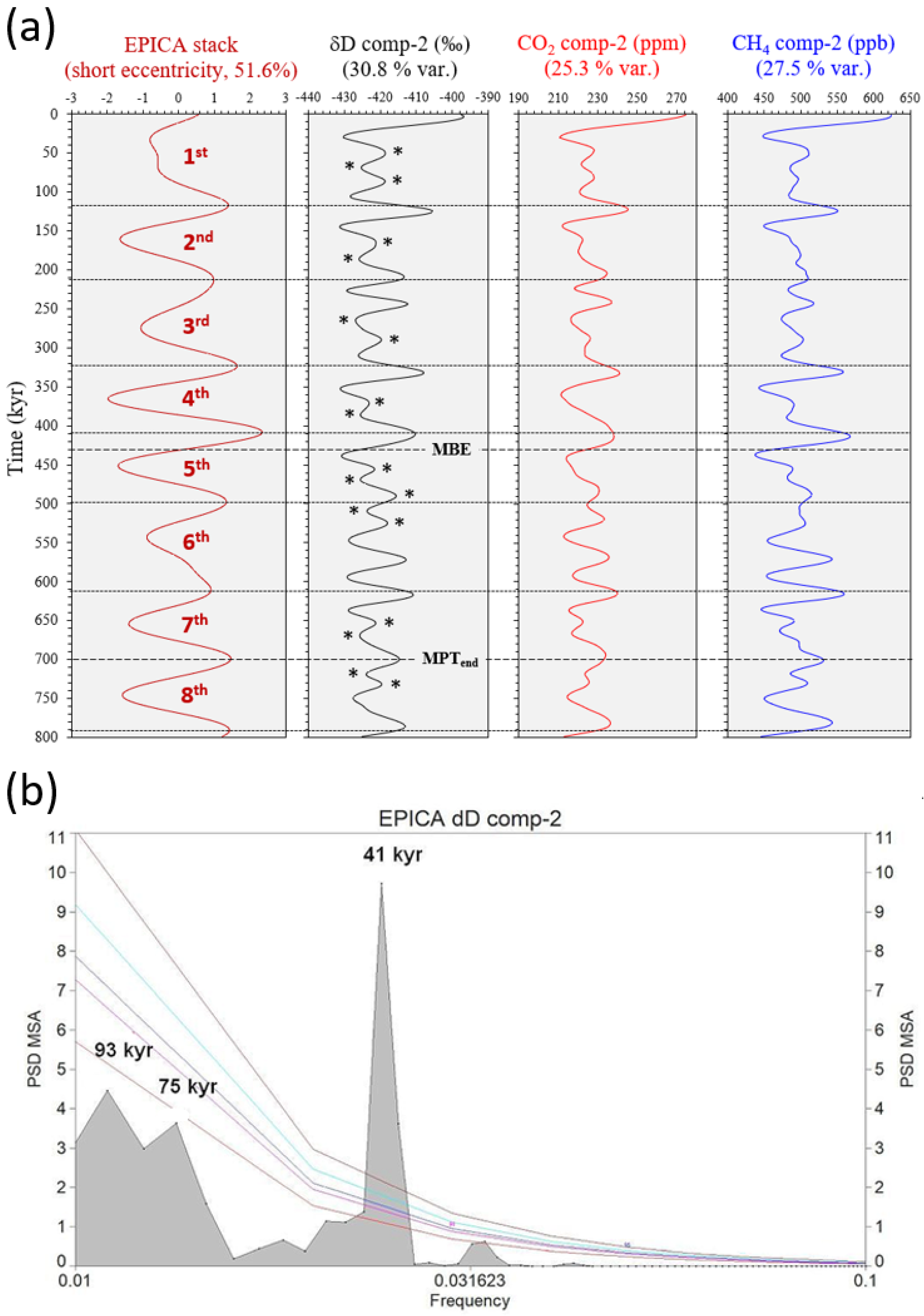
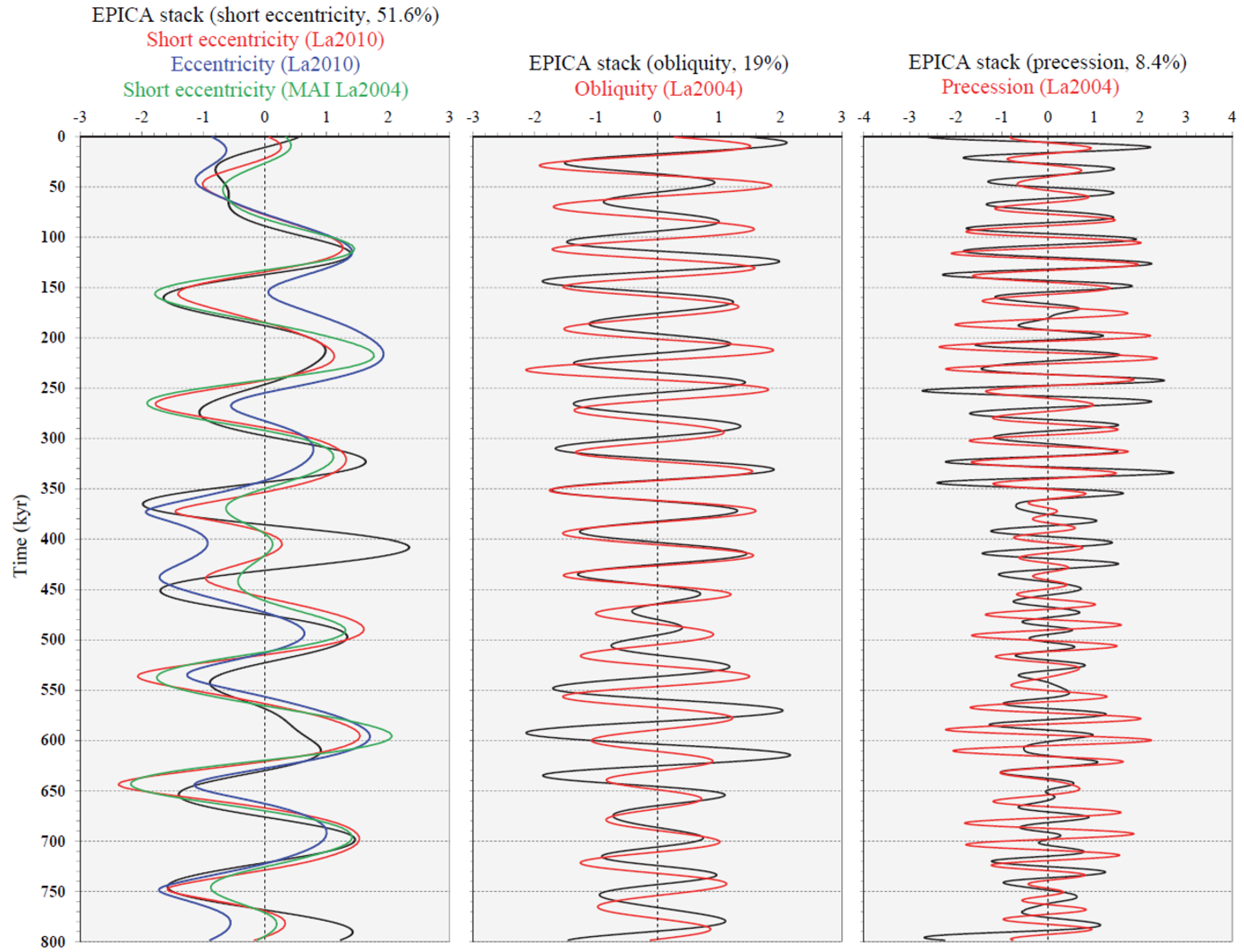
3.1.1. EPICA Record
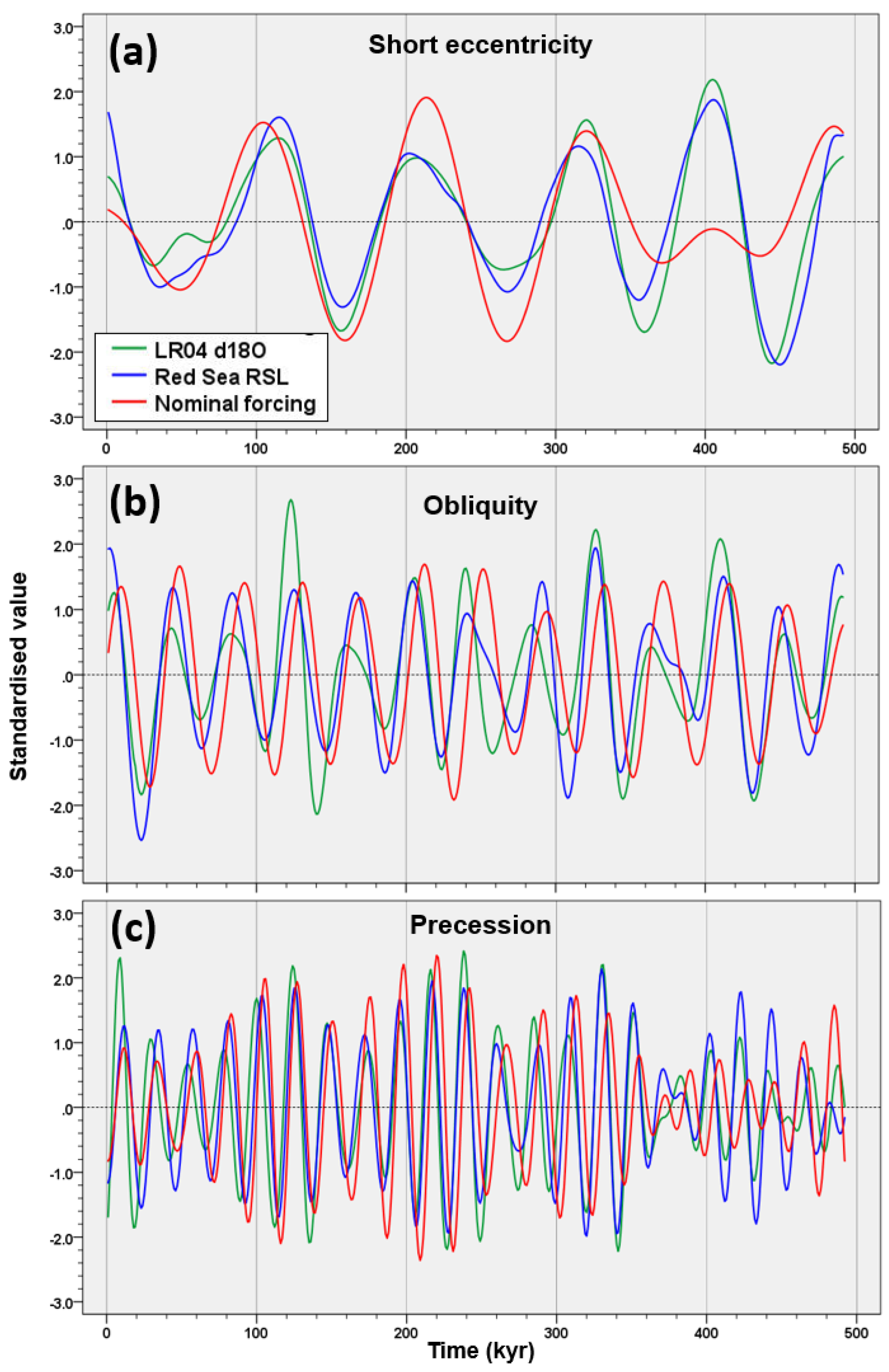
3.1.2. Sea-Level Record of the Red Sea
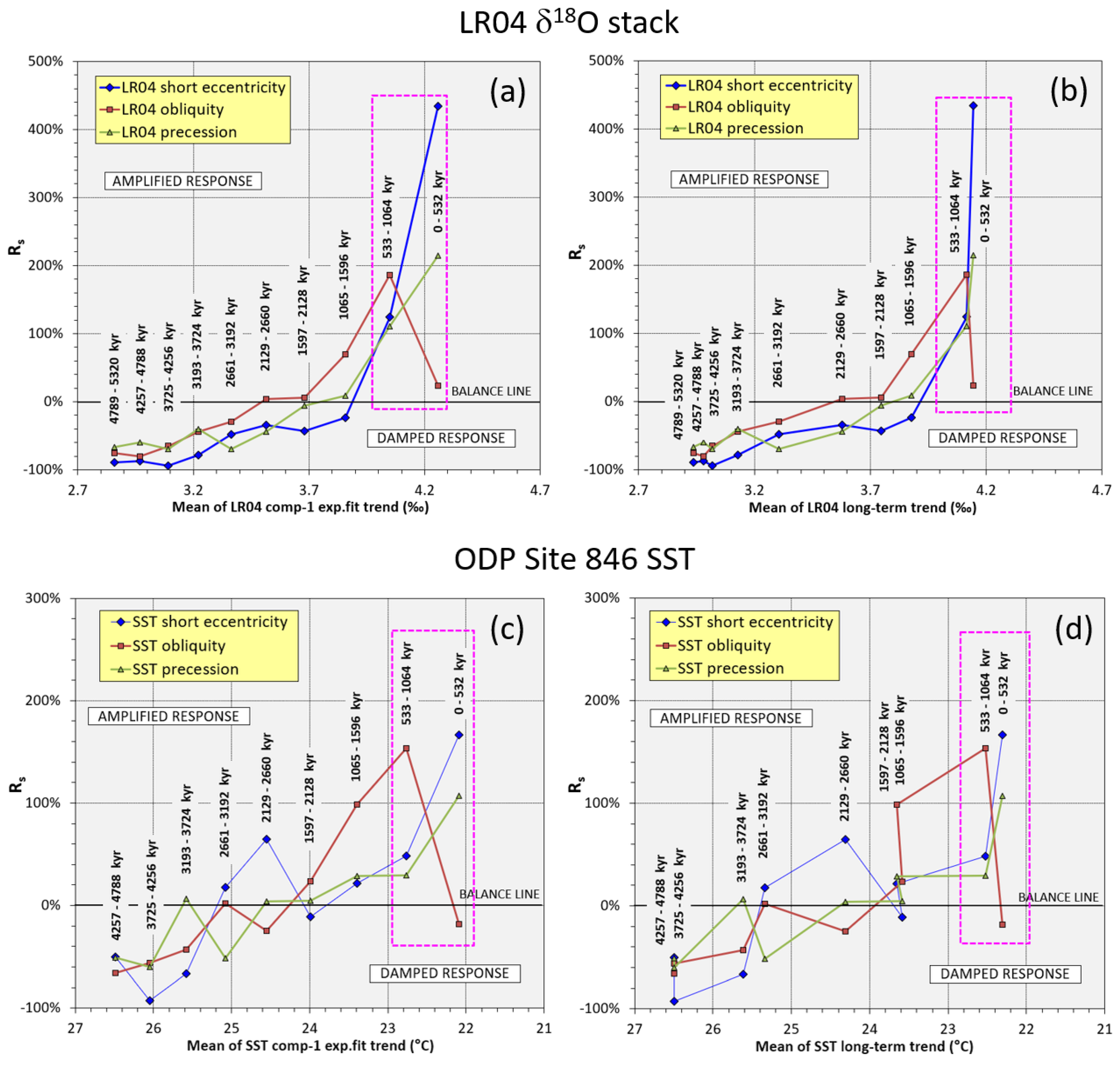
3.1.3. LR04 δ18O and Equatorial ODP Site 846 SST
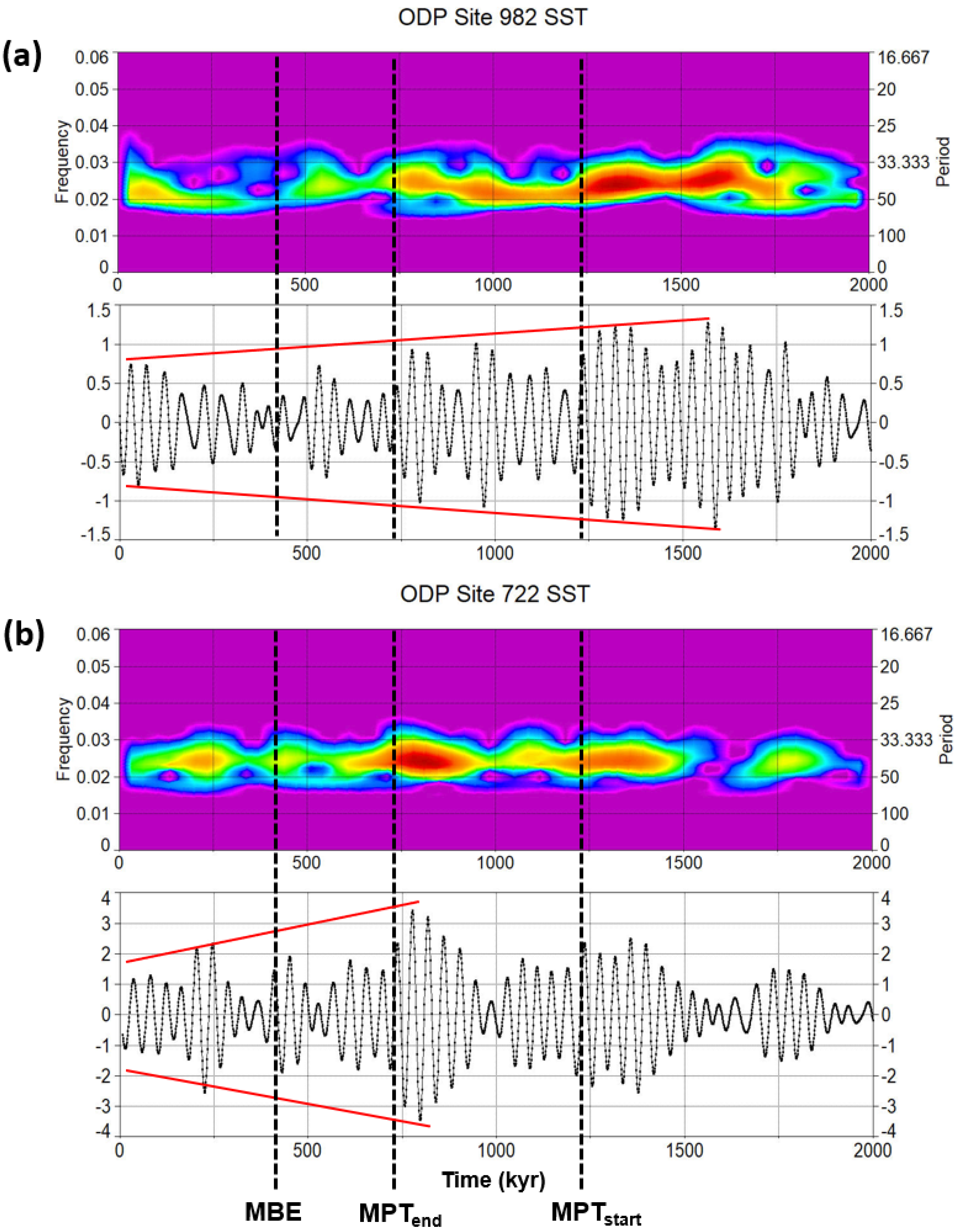
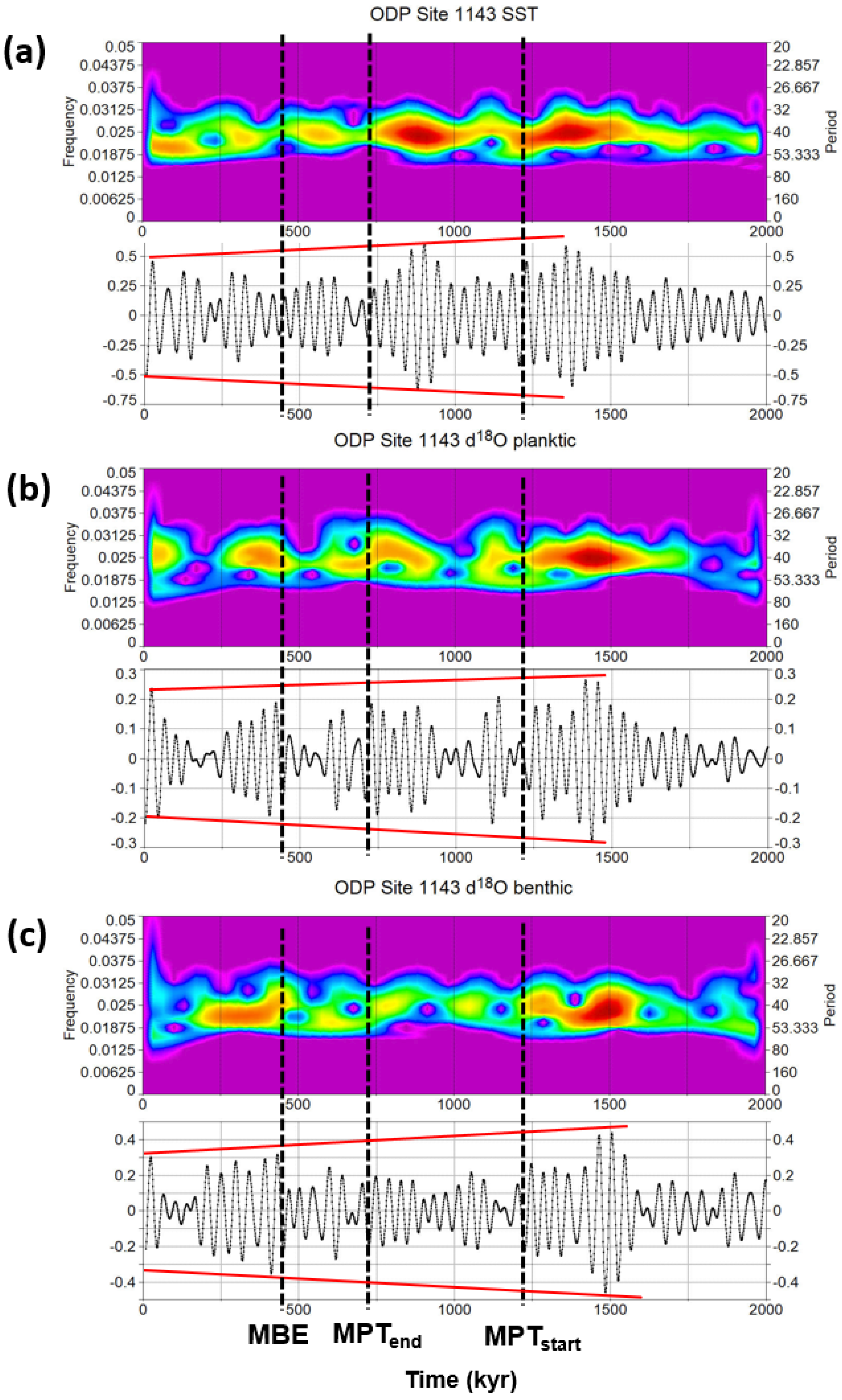
3.1.4. Atlantic, Pacific, Mediterranean, and Indian Proxies
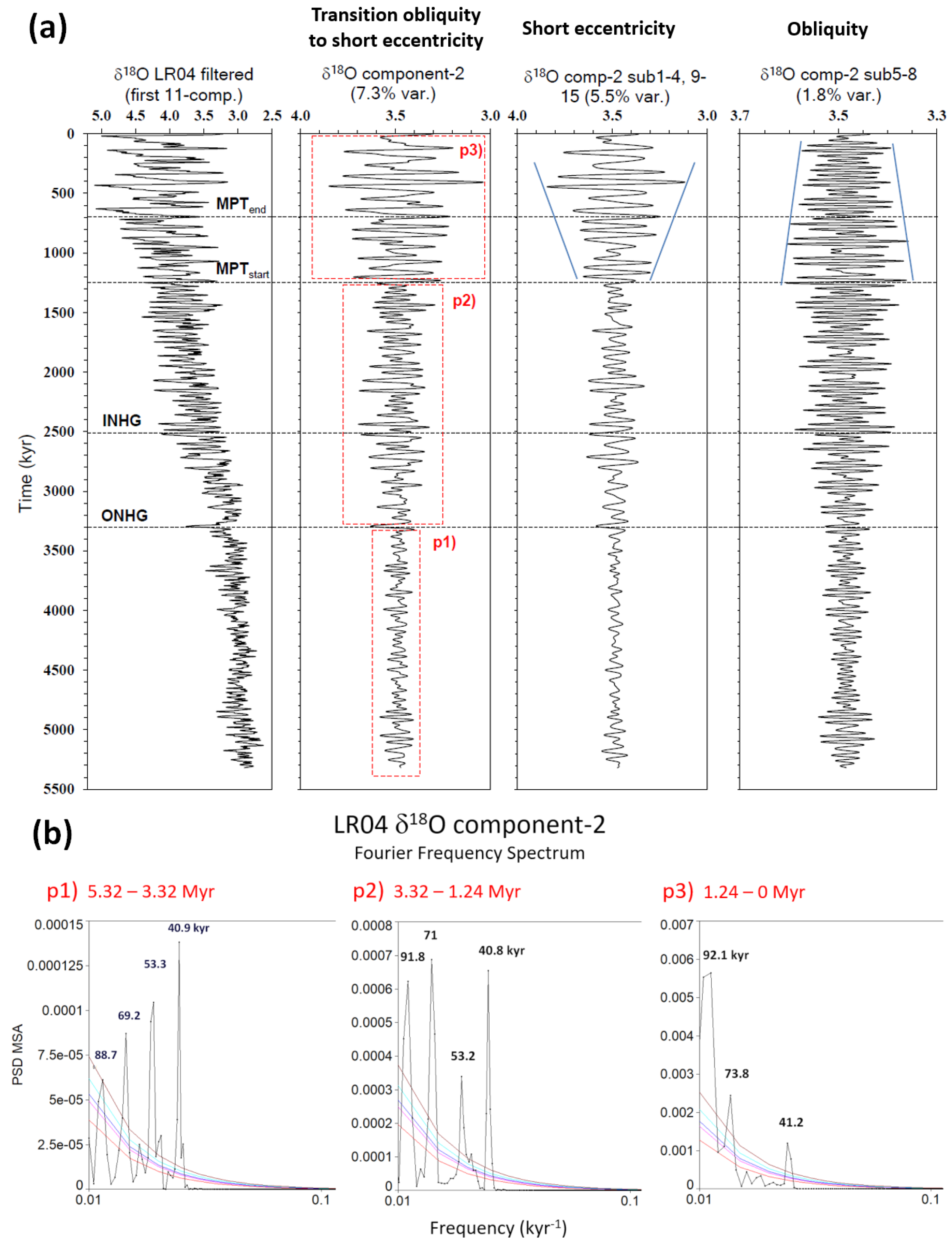
3.2. Long-Term Cooling Sets Boundary Conditions for Glacial/Interglacial Cycles
3.3. Amplitude Relationships between Orbital Forcings and Proxies
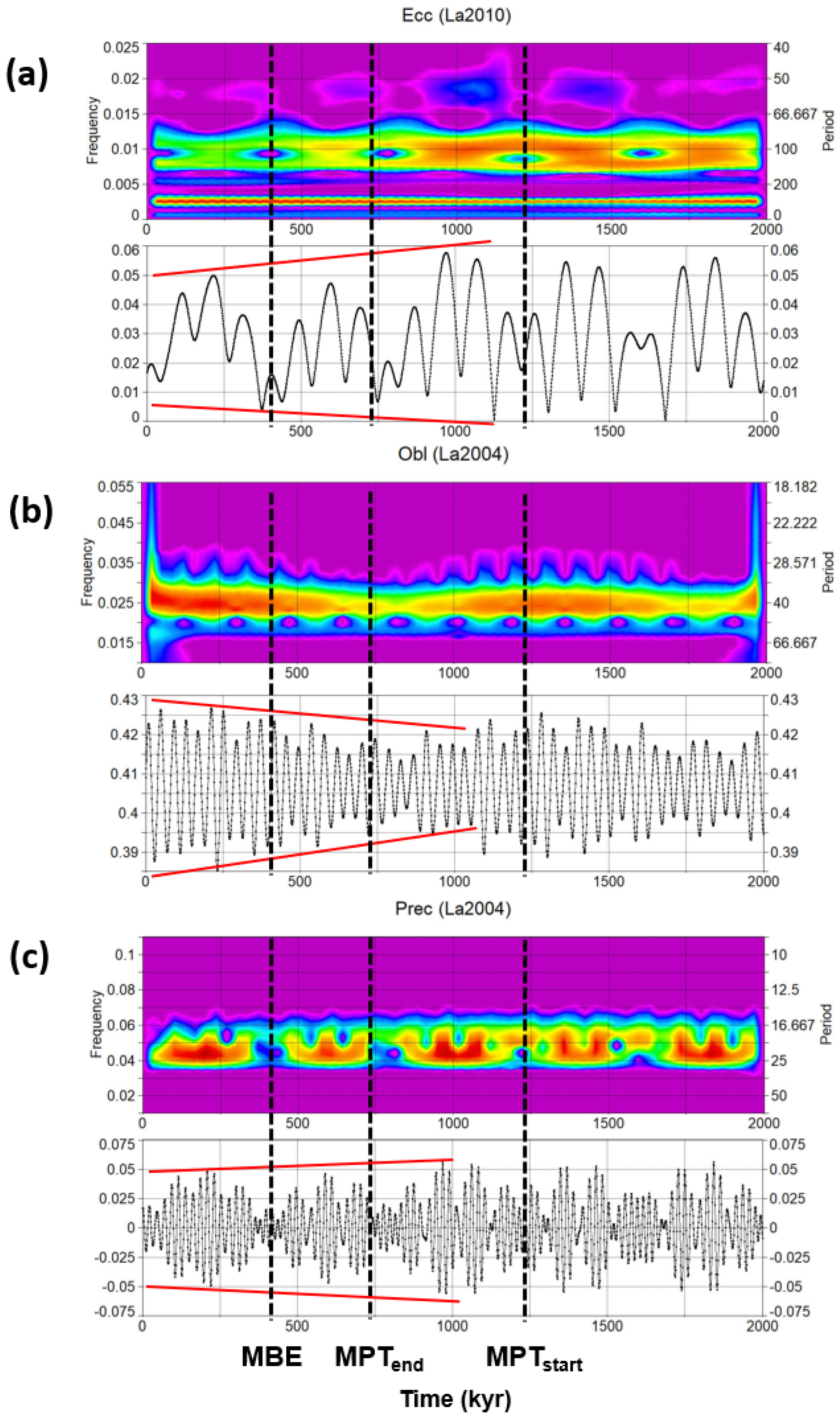
3.4. Why the Dampening of the Obliquity Response?
3.4.1. Remarks on Obliquity Phase Lag and Temperature/Ice-Volume Proxies
| Obliquity Component | Cross-Spectrum Freq. (kyr−1) | Cross-Spectrum Period (kyr) | Coherency | Phase Shift (Deg, kyr) | |
|---|---|---|---|---|---|
| EPICA δD (0–800 kyr) 1 | 0.02500 | 40.0 | 0.93 | −37 | −4.1 |
| EPICA CO2 (0–800 kyr) 1 | 0.02500 | 40.0 | 0.23 a | −63 | −7.0 |
| EPICA CH4 (0–800 kyr) 1 | 0.02500 | 40.0 | 0.76 | −29 | −3.2 |
| EPICA stack (0–800 kyr) 1 | 0.02500 | 40.0 | 0.79 | −38 | −4.2 |
| SST East Equatorial Pacific ODP 846 (6–800 kyr) 2 | 0.02500 | 40.0 | 0.59 | −9 | −1.0 |
| SST North Atlantic DSDP 607 (250–2000 kyr) *,3 | 0.02444 | 40.9 | 0.64 | −34 | −3.9 |
| SST West Tropical Pacific IODP 1146 (6–800 kyr) *,4 | 0.02500 | 40.0 | 0.86 | −52 | −5.7 |
| SST Arabian Sea ODP 722 (8–800 kyr) *,4 | 0.02500 | 40.0 | 0.83 | −34 | –3.8 |
| δ18O benthic LR04 global stack (0–800 kyr) 2 | 0.02500 | 40.0 | 0.88 | –50 | −5.5 |
| δ18O benthic Atlantic stack (0–800) *,5 | 0.02500 | 40.0 | 0.86 | −52 | −5.8 |
| δ18O benthic Pacific stack (0–800) *,5 | 0.02500 | 40.0 | 0.88 | −51 | −5.7 |
| δ18O benthic East Mediterr. ODP 967/968 (0–800 kyr) *,6 | 0.02500 | 40.0 | 0.69 | −40 | −4.4 |
| Mean (EPICA stack + SST) | 0.02489 | 40.2 | 0.74 | −33 ± 15 | −3.7 ± 1.7 |
| Mean (benthic δ18O) | 0.02500 | 40.0 | 0.83 | −48 ± 6 | −5.3 ± 0.6 |
3.4.2. Observations of Orbital Phase Lags between δ18O and Red Sea RSL Records
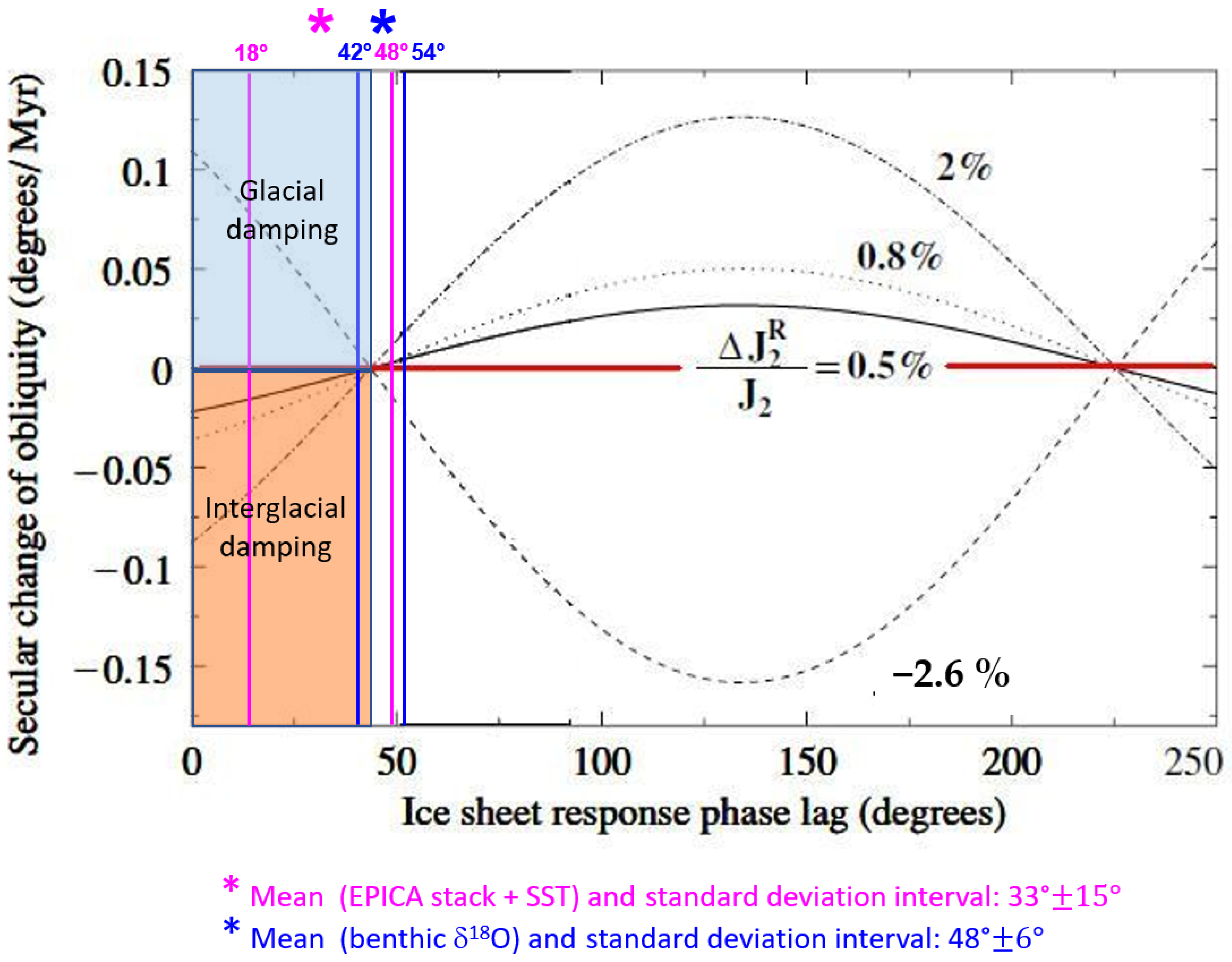
3.4.3. Changes in Earth’s Oblateness
3.5. Earth’s Eccentricity and the 100,000-Year Issue
4. Summary and Conclusions
4.1. Main Results
- The Antarctic orbital Rs demonstrates that, since 560 kyr, a strong amplification of the short eccentricity signals has been occurring (up to 400%, 600%) coupled with the damping of obliquity responses (up to −80%, −60%), confirming the marked asymmetry of the climate responses to orbital forcing. The PCA model of Rs data (EPICA, LR04 δ18O) suggests PC-1 to be a latent factor, indicating a post-MPT anticorrelation among obliquity and short eccentricity/precession Rs that is related to the long-term growth of the cryosphere volume.
- The PCA model integrating Plio–Pleistocene orbital Rs data and the long-term components of both LR04 δ18O and Site 846 SST records identify two PCs that are strictly related to the δ18O/SST short eccentricity/precession (PC-1) and obliquity (PC-2) amplification. Both are linked to the long-term δ18O enrichment and SST reduction. PC-2 factor highlights the post-MPT anomalous depletion of the obliquity Rs. These factors corroborate the latent link among the increasing amplitude of all orbital climate responses, the obliquity damping, and the Earth’s icy state developed through four stages of step-wise growth (subtrend I to IV).
- The spread of Plio–Pleistocene PCs exhibits two anticorrelation patterns of increasing absolute magnitude among forcing responses through time: a positive spread showing high obliquity associated with low short eccentricity/precession Rs, and a negative spread showing low obliquity linked to high short eccentricity/precession Rs. These response configurations are associated with three transition patterns of positive-to-negative spread including ONHG (Transition-1), INHG (Transition-2), and MBE (Transition-3), the latter being characterised by an extremely high magnitude and containing the MPT.
- EPICA orbital SSA stacks, which are rescaled on Plio–Pleistocene variance, exhibit a short eccentricity estimate of 12.2%, which is very high compared to the global δ18O value of 6.5%. In addition, an obliquity estimate of 4.5% is very low compared to the δ18O value of 9.9%. This is in agreement with the results of the Rs analysis, indicating a post-MPT short eccentricity amplification vs. obliquity damping.
- Orbital SSA components from the RSL record of the Red Sea exhibit a Plio–Pleistocene rescaled variance consistent with that of EPICA: short eccentricity (14.5%), obliquity (3.1%), and precession (2.8%). These data suggest post-MPT short eccentricity amplification vs. obliquity damping even in ESL fluctuations.
- Antarctic SSA structural signal observations of two or three low-amplitude 41 kyr obliquity peaks (glacial/interglacial) embedded in a weak ~93/75 kyr short eccentricity framework determined from δD, CO2, and CH4 component-2s are very similar in shape to the global LR04 δ18O and Site 846 equatorial Pacific SST component-3–4s during the MPT. These shapes may be further evidence of an obliquity attenuation phenomenon linked to the short eccentricity, and seem observationally reminiscent of the ‘obliquity-cycle skipping’ model.
- Additional evidence of MPT and post-MPT anticorrelation between obliquity damping and short eccentricity amplification is highlighted from a variety of global and regional (Atlantic, Pacific, Mediterranean, Indian) climate-related records, hinting that they are a widespread feature of the Pleistocene climate system. However, this feature does not seem to be consistent with the nominal solutions.
- Studies on Greenland and Antarctica indicate a fast response of the cryosphere mass balance to recent atmospheric and SST changes. The EPICA stacks better approximate the global benthic δ18O, where the latter probably represents the most lagged signal in the climate chain and may be considered a proxy of the global atmospheric temperature averaged by GHGs. Thus, the phase lags averaged among surface temperature proxies approximate the phase lag of the ice volume better, overcoming the deep temperature lag bias of the benthic δ18O.
- Assuming a fast response of the ice volume to surface temperature changes (EPICA stack, SST), the mean value of obliquity lag <5.0 kyr (3.7 ± 1.7 kyr) has been documented during the last 800 kyr. Also, considering the benthic δ18O records, the obliquity mean phase lag is 5.3 ± 0.6 kyr, which is very close to the theoretical threshold of 5.0 kyr and is significantly lower than the range of 6–10 kyr considered in previous studies.
- Cross-coherency data demonstrate that the Red Sea RSL record approximates extremely well the glacio-eustatic sea-level fluctuations linked to ice volume and paced by orbital forcings, also in the short eccentricity band. Global benthic δ18O lags Red Sea ESL by ~2.0 kyr in the obliquity band, suggesting a delay bias that could be attributed to the benthic δ18O deep-water temperature signal. Thus, the benthic δ18O obliquity mean phase lag of 5.3 kyr could be corrected to 3.3 kyr, close to the EPICA stack/SST mean of 3.7 kyr, which is significantly lower than the theoretical threshold of 5 kyr.
- Recent studies on variations in present-day satellite temporal gravity suggest the high sensitivity of the Earth to oblateness and highlight concurrent slow moderate negative (GIA rebound) and fast robust positive (water mass redistribution) J2 changes during the recent postglacial and global warming context, resulting in a J2 positive net change. Cross-spectral results from the Red Sea RSL over the last 500 kyr suggest a rapid and coherent oscillation in the obliquity band of the water layer component of the Earth’s oblateness.
- It is hypothesised that the fast and robust J2 water mass redistribution component in the obliquity band, which is reasonably related to the glacio-eustatic variation in the sea level, could be a crucial element in determining +ΔJ2 ⟹ −Δε during obliquity maxima (interglacial damping), and −ΔJ2 ⟹ +Δε during obliquity minima (glacial damping). This explains the fact that obliquity damping in climate proxies is basically symmetrical.
4.2. Obliquity Damping Hypothesis
- The widespread evidence from proxy records of anticorrelation between obliquity and short eccentricity/precession has been interpreted as an effect of the obliquity–oblateness feedback by critically reviewing its theoretical constraints, which support negative/positive secular change in obliquity for both low ice-volume phase lag (<5.0 kyr) and positive/negative net change in oblateness, respectively, in the obliquity band, that are likely dominated by the J2 water mass redistribution component.
- Obliquity damping during the interglacial stages might have contributed to the strengthening of the short eccentricity response by mitigating the obliquity’s ice killing, favouring the obliquity-cycle skipping, and a ~100 kyr long-life feedback amplified ice growth in the context of the global icy state.
- Orbitals, including short eccentricity, may pace the frequency beat of the climate response. The phase-locked feedback mechanisms might have nonlinearly transferred most of the system energy depending on the long-term climate state and the cycle duration, overcoming the energy excess ‘paradox’, especially with respect to the eccentricity band.
- The observed transition patterns (TRA-1,-2,-3) of the orbitals Rs and the early onset of the short eccentricity response suggest the traditional notion of the MPT to be the final, high-magnitude, nonlinear transitional stage of a complex competing interaction between obliquity and short eccentricity forcing under the influence of both long-term cooling and obliquity–oblateness feedback that had already started during the Piacenzian. The maximum expression of this mechanism occurs during TRA-3, which is associated with the maximum ice-volume development (subtrend IV) and a strong amplification of the obliquity response until the termination of the MPT (TH4).
- The Plio–Pleistocene long-term cooling is a relevant background forcing in setting boundary conditions to orbital climate responses, and is characterised by four step-wise subtrends (I to IV), where a mild curvilinear shape is broken by slope changes representing four thresholds (TH1 to TH4) of mean climate state change.
- The role of the long-term cooling is outlined as follows:
- The nonlinearity of the orbital responses is increased by the scale effect of the ice-sheet growth on feedback mechanisms, which are sensitive to long orbital periods. Specifically, the amplitude increases in the obliquity band of the ice-volume changes. It is hypothesised that the glacio-eustatic oscillations in the obliquity band inducing oblateness variations by the dominant/fast water mass redistribution component could have led to the overcoming of the thresholds sensitive to secular changes in obliquity. This mechanism would explain why the 100 kyr cycle reached its maximum expression post-MPT, albeit after a period of early/weak manifestations as early as in the Piacenzian.
- The icy state may have increased the sensitivity of the polar climate system to the minima of MAI by triggering a strong nonlinear energy transfer in the short eccentricity band by feedback mechanisms (primarily albedo and GHGs).
4.3. Outlook
- Unbiased estimates of the ice-volume lag in the obliquity band.
- Modelling the changes in Earth’s oblateness in the obliquity band and its effects on the forcing, determined especially by considering the dominant/fast water mass redistribution component. This could make the difficult and uncertain solid Earth’s oblateness component less stringent among the theoretical constraints.
- Modelling the hypothesis with full observational evidence control.
- Determining the role of the MAI and feedback mechanisms in the context of the Earth’s long-term icy state.
Supplementary Materials
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
References
- Head, M.J.; Gibbard, P.L. Early–Middle Pleistocene transitions: An overview and recommendation for the defining boundary. Geol. Soc. Lond. Spec. Publ. 2005, 247, 1–18. [Google Scholar] [CrossRef]
- Clark, P.U.; Archer, D.; Pollard, D.; Blum, J.D.; Rial, J.A.; Brovkin, V.; Mix, A.C.; Pisias, N.G.; Roy, M. The middle Pleistocene transition: Characteristics, mechanisms, and implications for long-term changes in atmospheric pCO2. Quat. Sci. Rev. 2006, 25, 3150–3184. [Google Scholar] [CrossRef]
- Head, M.J.; Pillans, B.; Farquhar, S.A. The Early–Middle Pleistocene transition: Characterization and proposed guide for the defining boundary. Episodes 2008, 31, 255–259. [Google Scholar] [CrossRef]
- Imbrie, J.; Berger, A.; Boyle, E.A.; Clemens, S.C.; Duffy, A.; Howard, W.R.; Kukla, G.; Kutzbach, J.; Martinson, D.G.; Mcintyre, A.; et al. On the structure and origin of major glaciation cycles 2. The 100,000-year cycle. Paleoceanography 1993, 8, 699–735. [Google Scholar] [CrossRef]
- Berger, A.; Loutre, M.F.; Li, X.S. Modeling northern hemisphere ice volume over the last 3 Ma. Quat. Sci. Rev. 1999, 18, 1–11. [Google Scholar] [CrossRef]
- Berger, A.; Loutre, M. Modeling the 100-kyr glacial–interglacial cycles. Glob. Planet. Change 2010, 72, 275–281. [Google Scholar] [CrossRef]
- Lisiecki, L.E. Links between eccentricity forcing and the 100,000-year glacial cycle. Nat. Geosci. 2010, 3, 349–352. [Google Scholar] [CrossRef]
- Viaggi, P. δ18O and SST signal decomposition and dynamic of the Pliocene-Pleistocene climate system: New insights on orbital nonlinear behavior vs. long-term trend. Prog. Earth Planet. Sci. 2018, 5, 81. [Google Scholar] [CrossRef]
- Bintanja, R.; van de Wal, R.S.W. North American ice-sheet dynamics and the onset of 100,000-year glacial cycles. Nature 2008, 454, 869–872. [Google Scholar] [CrossRef]
- Ellis, R.; Palmer, M. Modulation of ice ages via precession and dust-albedo feedbacks. Geosci. Front. 2016, 7, 891–909. [Google Scholar] [CrossRef]
- Chalk, T.B.; Hain, M.P.; Foster, G.L.; Rohling, E.J.; Sexton, P.F.; Badger, M.P.S.; Cherry, S.G.; Hasenfratz, A.P.; Haug, G.H.; Jaccard, S.L.; et al. Causes of ice age intensification across the Mid-Pleistocene Transition. Proc. Natl. Acad. Sci. USA 2017, 114, 13114–13119. [Google Scholar] [CrossRef] [PubMed]
- Köhler, P.; van de Wal, R.S.W. Interglacials of the Quaternary defined by northern hemispheric land ice distribution outside of Greenland. Nat. Commun. 2020, 11, 1–10. [Google Scholar] [CrossRef] [PubMed]
- Imbrie, J.; Imbrie-Moore, A.; Lisiecki, L.E. A phase-space model for Pleistocene ice volume. Earth Planet Sci. Lett. 2011, 307, 94–102. [Google Scholar] [CrossRef]
- Crucifix, M. Oscillators and relaxation phenomena in Pleistocene climate theory. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2012, 370, 1140–1165. [Google Scholar] [CrossRef] [PubMed]
- Ditlevsen, P.D.; Ashwin, P. Complex Climate Response to Astronomical Forcing: The Middle-Pleistocene Transition in Glacial Cycles and Changes in Frequency Locking. Front. Phys. 2018, 6, 62. [Google Scholar] [CrossRef]
- Quinn, C.; Sieber, J.; von der Heydt, A.S.; Lenton, T.M. The Mid-Pleistocene Transition induced by delayed feedback and bistability. Dyn. Stat. Clim. Syst. 2018, 3, dzy005. [Google Scholar] [CrossRef]
- Mukhin, D.; Gavrilov, A.; Loskutov, E.; Kurths, J.; Feigin, A. Bayesian Data Analysis for Revealing Causes of the Middle Pleistocene Transition. Sci. Rep. 2019, 9, 7328. [Google Scholar] [CrossRef]
- Nyman, K.H.M.; Ditlevsen, P.D. The middle Pleistocene transition by frequency locking and slow ramping of internal period. Clim. Dynam. 2019, 53, 3023–3038. [Google Scholar] [CrossRef]
- Berends, C.J.; Köhler, P.; Lourens, L.J.; van de Wal, R.S.W. On the Cause of the Mid-Pleistocene Transition. Rev. Geophys. 2021, 59, e2020RG000727. [Google Scholar] [CrossRef]
- Huybers, P. Combined obliquity and precession pacing of late Pleistocene deglaciations. Nature 2011, 480, 229–232. [Google Scholar] [CrossRef]
- Huybers, P. Glacial variability over the last two million years: An extended depth-derived agemodel, continuous obliquity pacing, and the Pleistocene progression. Quat. Sci. Rev. 2007, 26, 37–55. [Google Scholar] [CrossRef]
- Liu, Z.; Cleaveland, L.C.; Herbert, T.D. Early onset and origin of 100-kyr cycles in Pleistocene tropical SST records. Earth Planet. Sci. Lett. 2008, 265, 703–715. [Google Scholar] [CrossRef]
- Raymo, M.E. The timing of major climate terminations. Paleoceanography 1997, 12, 577–585. [Google Scholar] [CrossRef]
- Shackleton, N.J. The 100,000-Year Ice-Age Cycle Identified and Found to Lag Temperature, Carbon Dioxide, and Orbital Eccentricity. Science 2000, 289, 1897–1902. [Google Scholar] [CrossRef] [PubMed]
- Lisiecki, L.E.; Raymo, M.E. Plio–Pleistocene climate evolution: Trends and transitions in glacial cycle dynamics. Quat. Sci. Rev. 2007, 26, 56–69. [Google Scholar] [CrossRef]
- Viaggi, P. Quantitative impact of astronomical and sun-related cycles on the Pleistocene climate system from Antarctica records. Quat. Sci. Adv. 2021, 4, 100037. [Google Scholar] [CrossRef]
- Abe-Ouchi, A.; Saito, F.; Kawamura, K.; Raymo, M.E.; Okuno, J.; Takahashi, K.; Blatter, H. Insolation-driven 100,000-year glacial cycles and hysteresis of ice-sheet volume. Nature 2013, 500, 190–193. [Google Scholar] [CrossRef]
- Zachos, J.C.; Pagani, M.; Sloan, L.; Thomas, E.; Billups, K. Trends, rhythms, and aberrations in global climate 65 Myr to present. Science 2001, 292, 686–693. [Google Scholar] [CrossRef]
- Zachos, J.C.; Dickens, G.R.; Zeebe, R.E. An early Cenozoic perspective on greenhouse warming and carbon-cycle dynamics. Nature 2008, 451, 279–283. [Google Scholar] [CrossRef]
- Kender, S.; Ravelo, A.C.; Worne, S.; Swann, G.E.A.; Leng, M.J.; Asahi, H.; Becker, J.; Detlef, H.; Aiello, I.W.; Andreasen, D.; et al. Closure of the Bering Strait caused Mid-Pleistocene Transition cooling. Nat. Commun. 2018, 9, 5386. [Google Scholar] [CrossRef]
- Shoenfelt, E.M.; Winckler, G.; Lamy, F.; Anderson, R.F.; Bostick, B.C. Highly bioavailable dust-borne iron delivered to the Southern Ocean during glacial periods. Proc. Natl. Acad. Sci. USA 2018, 115, 11180–11185. [Google Scholar] [CrossRef]
- Farmer, J.R.; Hönisch, B.; Haynes, L.L.; Kroon, D.; Jung, S.; Ford, H.L.; Raymo, M.E.; Jaume-Seguí, M.; Bell, D.B.; Goldstein, S.L.; et al. Deep Atlantic Ocean carbon storage and the rise of 100,000-year glacial cycles. Nat. Geosci. 2019, 12, 355–360. [Google Scholar] [CrossRef]
- Hasenfratz, A.P.; Jaccard, S.L.; Martínez-García, A.; Sigman, D.M.; Hodell, D.A.; Vance, D.; Bernasconi, S.M.; Kleiven, H.F.; Haumann, F.A.; Haug, G.H. The residence time of Southern Ocean surface waters and the 100,000-year ice age cycle. Science 2019, 363, 1080–1084. [Google Scholar] [CrossRef]
- Jicha, B.R.; Scholl, D.W.; Rea, D.K. Circum-Pacific arc flare-ups and global cooling near the Eocene-Oligocene boundary. Geology 2009, 37, 303–306. [Google Scholar] [CrossRef]
- Steinberger, B.; Spakman, W.; Japsen, P.; Torsvik, T.H. The key role of global solid-Earth processes in preconditioning Greenland’s glaciation since the Pliocene. Terra Nova 2015, 27, 1–8. [Google Scholar] [CrossRef]
- Daradich, A.; Huybers, P.; Mitrovica, J.; Chan, N.-H.; Austermann, J. The influence of true polar wander on glacial inception in North America. Earth Planet. Sci. Lett. 2017, 461, 96–104. [Google Scholar] [CrossRef]
- Laskar, J.; Robutel, P.; Joutel, F.; Gastineau, M.; Correia, A.C.M.; Levrard, B. A long term numerical solution for the insolation quantities of the Earth. Astron. Astrophys. 2004, 428, 261–285. [Google Scholar] [CrossRef]
- Laskar, J.; Fienga, A.; Gastineau, M.; Manche, H. La2010: A new orbital solution for the long-term motion of the Earth. Astron. Astrophys. 2011, 532, A89. [Google Scholar] [CrossRef]
- Lisiecki, L.E.; Raymo, M.E. A Pliocene-Pleistocene stack of 57 globally distributed benthic δ18O records. Paleoceanography 2005, 20, PA1003. [Google Scholar] [CrossRef]
- Herbert, T.D.; Peterson, L.C.; Lawrence, K.T.; Liu, Z. Tropical Ocean Temperatures Over the Past 3.5 Million Years. Science 2010, 328, 1530–1534. [Google Scholar] [CrossRef]
- Rubincam, D.P. The obliquity of Mars and climate friction. J. Geophys. Res. 1993, 98, 10827–10832. [Google Scholar] [CrossRef]
- Rubincam, D.P. Has climate changed the Earth’s tilt? Paleoceanography 1995, 10, 365–372. [Google Scholar] [CrossRef]
- Bills, B.G. Obliquity-oblateness feedback: Are climatically sensitive values of obliquity dynamically unstable? Geophys. Res. Lett. 1994, 21, 177–180. [Google Scholar] [CrossRef]
- Bills, B.G. An oblique view of climate. Nature 1998, 396, 405–406. [Google Scholar] [CrossRef]
- Williams, D.M.; Kasting, J.F.; Frakes, L.A. Low-latitude glaciations and rapid changes in the Earth’s obliquity explained by obliquity–oblateness feedback. Nature 1998, 396, 453–455. [Google Scholar] [CrossRef] [PubMed]
- Levrard, B.; Laskar, J. Climate friction and the Earth’s obliquity. Geophys. J. Int. 2003, 154, 970–990. [Google Scholar] [CrossRef]
- Skinner, L.; Shackleton, N. An Atlantic lead over Pacific deep-water change across Termination I: Implications for the application of the marine isotope stage stratigraphy. Quat. Sci. Rev. 2005, 24, 571–580. [Google Scholar] [CrossRef]
- Lisiecki, L.E.; Raymo, M.E. Diachronous benthic δ18O responses during late Pleistocene terminations. Paleoceanography 2009, 24, PA3210. [Google Scholar] [CrossRef]
- Lisiecki, L.E. Atlantic overturning responses to obliquity and precession over the last 3 Myr. Paleoceanography 2014, 29, 71–86. [Google Scholar] [CrossRef]
- Huybers, P. Early Pleistocene Glacial Cycles and the Integrated Summer Insolation Forcing. Science 2006, 313, 508–511. [Google Scholar] [CrossRef]
- Raymo, M.E.; Nisancioglu, K.H. The 41 kyr world: Milankovitch’s other unsolved mystery. Paleoceanography 2003, 18, 1–15. [Google Scholar] [CrossRef]
- Bajo, P.; Drysdale, R.N.; Woodhead, J.D.; Hellstrom, J.C.; Hodell, D.; Ferretti, P.; Voelker, A.H.L.; Zanchetta, G.; Rodrigues, T.; Wolff, E.; et al. Persistent influence of obliquity on ice age terminations since the Middle Pleistocene transition. Science 2020, 367, 1235–1239. [Google Scholar] [CrossRef]
- Elderfield, H.; Ferretti, P.; Greaves, M.; Crowhurst, S.; McCave, I.N.; Hodell, D.; Piotrowski, A.M. Evolution of Ocean Temperature and Ice Volume Through the Mid-Pleistocene Climate Transition. Science 2012, 337, 704–709. [Google Scholar] [CrossRef] [PubMed]
- Laskar, J.; Joutel, F.; Boudin, F. Orbital, precessional and insolation quantities for the Earth from −20 Myr to +10 Myr. Astron. Astrophys. 1993, 270, 522–533. [Google Scholar]
- Jouzel, J.; Masson-Delmotte, V.; Cattani, O.; Dreyfus, G.; Falourd, S.; Hoffmann, G.; Minster, B.; Nouet, J.; Barnola, J.M.; Chappellaz, J.; et al. Orbital and Millennial Antarctic Climate Variability over the Past 800,000 Years. Science 2007, 317, 793–796. [Google Scholar] [CrossRef] [PubMed]
- Lüthi, D.; Le Floch, M.; Bereiter, B.; Blunier, T.; Barnola, J.-M.; Siegenthaler, U.; Raynaud, D.; Jouzel, J.; Fischer, H.; Kawamura, K.; et al. High-resolution carbon dioxide concentration record 650,000–800,000 years before present. Nature 2008, 453, 379–382. [Google Scholar] [CrossRef]
- Loulergue, L.; Schilt, A.; Spahni, R.; Masson-Delmotte, V.; Blunier, T.; Lemieux, B.; Barnola, J.-M.; Raynaud, D.; Stocker, T.F.; Chappellaz, J. Orbital and millennial-scale features of atmospheric CH4 over the past 800,000 years. Nature 2008, 453, 383–386. [Google Scholar] [CrossRef]
- Bereiter, B.; Eggleston, S.; Schmitt, J.; Nehrbass-Ahles, C.; Stocker, T.F.; Fischer, H.; Kipfstuhl, S.; Chappellaz, J. Revision of the EPICA Dome C CO2 record from 800 to 600 kyr before present. Geophys. Res. Lett. 2015, 42, 542–549. [Google Scholar] [CrossRef]
- Past Interglacials Working Group of PAGES. Interglacials of the last 800,000 years. Rev. Geophys. 2016, 54, 162–219. [Google Scholar] [CrossRef]
- Bazin, L.; Landais, A.; Lemieux-Dudon, B.; Kele, H.T.M.; Veres, D.; Parrenin, F.; Martinerie, P.; Ritz, C.; Capron, E.; Lipenkov, V.; et al. An optimized multi-proxy, multi-site Antarctic ice and gas orbital chronology (AICC2012): 120–800 ka. Clim. Past 2013, 9, 1715–1731. [Google Scholar] [CrossRef]
- Jouzel, J.; Alley, R.B.; Cuffey, K.M.; Dansgaard, W.; Grootes, P.; Hoffmann, G.; Johnsen, S.J.; Koster, R.D.; Peel, D.; Shuman, C.A.; et al. Validity of the temperature reconstruction from water isotopes in ice cores. J. Geophys. Res. 1997, 102, 26471–26487. [Google Scholar] [CrossRef]
- Hodell, D.A.; Channell, J.E.T.; Curtis, J.H.; Romero, O.E.; Röhl, U. Onset of “Hudson Strait” Heinrich events in the eastern North Atlantic at the end of the middle Pleistocene transition (∼640 ka)? Paleoceanography 2008, 23, PA4218. [Google Scholar] [CrossRef]
- Hodell, D.; Lourens, L.; Crowhurst, S.; Konijnendijk, T.; Tjallingii, R.; Jiménez-Espejo, F.; Skinner, L.; Tzedakis, P.; Abrantes, F.; Acton, G.D.; et al. A reference time scale for Site U1385 (Shackleton Site) on the SW Iberian Margin. Glob. Planet. Chang. 2015, 133, 49–64. [Google Scholar] [CrossRef]
- Lawrence, K.T.; Herbert, T.D.; Brown, C.M.; Raymo, M.E.; Haywood, A.M. High-amplitude variations in North Atlantic sea surface temperature during the early Pliocene warm period. Paleoceanography 2009, 24, PA2218. [Google Scholar] [CrossRef]
- Lawrence, K.; Sosdian, S.; White, H.; Rosenthal, Y. North Atlantic climate evolution through the Plio-Pleistocene climate transitions. Earth Planet. Sci. Lett. 2010, 300, 329–342. [Google Scholar] [CrossRef]
- Russon, T.; Elliot, M.; Sadekov, A.; Cabioch, G.; Corrège, T.; De Deckker, P. The mid-Pleistocene transition in the subtropical southwest Pacific. Paleoceanography 2011, 26, A1211. [Google Scholar] [CrossRef]
- Cheng, X.; Tian, J.; Wang, P. Data report: Stable isotopes from Site 1143. In Proceedings of the Ocean Drilling Program, Scientific Results; Prell, W.L., Wang, P., Blum, P., Rea, D.K., Clemens, S.C., Eds.; Ocean Drilling Program: College Station, TX, USA, 2004; Volume 184, pp. 1–8. [Google Scholar] [CrossRef]
- Ao, H.; Dekkers, M.J.; Qin, L.; Xiao, G. An updated astronomical timescale for the Plio-Pleistocene deposits from South China Sea and new insights into Asian monsoon evolution. Quat. Sci. Rev. 2011, 30, 1560–1575. [Google Scholar] [CrossRef]
- Li, L.; Li, Q.; Tian, J.; Wang, P.; Wang, H.; Liu, Z. A 4-Ma record of thermal evolution in the tropical western Pacific and its implications on climate change. Earth Planet. Sci. Lett. 2011, 309, 10–20. [Google Scholar] [CrossRef]
- Konijnendijk, T.; Ziegler, M.; Lourens, L. On the timing and forcing mechanisms of late Pleistocene glacial terminations: Insights from a new high-resolution benthic stable oxygen isotope record of the eastern Mediterranean. Quat. Sci. Rev. 2015, 129, 308–320. [Google Scholar] [CrossRef]
- Grant, K.M.; Rohling, E.J.; Ramsey, C.B.; Cheng, H.; Edwards, R.L.; Florindo, F.; Heslop, D.; Marra, F.; Roberts, A.P.; Tamisiea, M.E.; et al. Sea-level variability over five glacial cycles. Nat. Commun. 2014, 5, 5076. [Google Scholar] [CrossRef]
- Hays, J.D.; Imbrie, J.; Shackleton, N.J. Variations in the Earth’s Orbit: Pacemaker of the Ice Ages. Science 1976, 194, 1121–1132. Available online: http://www.jstor.org/stable/1743620 (accessed on 5 January 2009). [CrossRef] [PubMed]
- Steinhilber, F.; Abreu, J.A.; Beer, J.; Brunner, I.; Christl, M.; Fischer, H.; Heikkilä, U.; Kubik, P.W.; Mann, M.; McCracken, K.G.; et al. 9400 years of cosmic radiation and solar activity from ice cores and tree rings. Proc. Natl. Acad. Sci. USA 2012, 109, 5967–5971. [Google Scholar] [CrossRef] [PubMed]
- Zharkova, V.V.; Shepherd, S.J.; Zharkov, S.I. Principal component analysis of background and sunspot magnetic field variations during solar cycles 21–23. Mon. Not. R Astron. Soc. 2012, 424, 2943–2953. [Google Scholar] [CrossRef]
- Viaggi, P.; Scotti, P.; Previde Massara, E.; Knezaurek, G.; Menichetti, E.; Piva, A.; Torricelli, S.; Gambacorta, G. Paleoceanographic Evolution of mid-Cretaceous Paleobioproductivity and Paleoredox Chemometric Signals. Link to Cretaceous Long-term Eustatic Climax from a Central Atlantic Well Sequence. In Proceedings of the 3rd International Congress on Stratigraphy, Conference Presentation, STRATI 2019, Milan, Italy, 2–5 July 2019. [Google Scholar] [CrossRef]
- Vautard, R.; Ghil, M. Singular spectrum analysis in nonlinear dynamics, with applications to paleoclimatic time series. Phys. D 1989, 35, 395–424. [Google Scholar] [CrossRef]
- Elsner, J.B.; Tsonis, A.A. Singular Spectrum Analysis: A New Tool in Time Series Analysis; Springer: Berlin/Heidelberg, Germany, 1996. [Google Scholar]
- Ghil, M.; Allen, R.M.; Dettinger, M.D.; Ide, K.; Kondrashov, D.; Mann, M.E.; Robertson, A.; Saunders, A.; Tian, Y.; Varadi, F.; et al. Advanced spectral methods for climatic time series. Rev. Geophys. 2002, 40, 3-1–3-41. [Google Scholar] [CrossRef]
- Hassani, H. Singular spectrum analysis: Methodology and comparison. J. Data Sci. 2007, 5, 239–257. [Google Scholar] [CrossRef]
- Lang, N.; Wolff, E.W. Interglacial and glacial variability from the last 800 ka in marine, ice and terrestrial archives. Clim. Past 2011, 7, 361–380. [Google Scholar] [CrossRef]
- Barth, A.M.; Clark, P.U.; Bill, N.S.; He, F.; Pisias, N.G. Climate evolution across the Mid-Brunhes Transition. Clim. Past 2018, 14, 2071–2087. [Google Scholar] [CrossRef]
- Berger, A.; Yin, Q. Modeling the interglacials of the last 1 million years. In Climate Change: Inferences from Paleoclimate and Regional Aspects; Berger, A., Mesinger, F., Sijacki, D., Eds.; Springer: Vienna, Austria, 2012. [Google Scholar]
- Meyers, S.R.; Hinnov, L.A. Northern Hemisphere glaciation and the evolution of Plio-Pleistocene climate noise. Paleoceanography 2010, 25, A3207. [Google Scholar] [CrossRef]
- McClymont, E.L.; Sosdian, S.M.; Rosell-Melé, A.; Rosenthal, Y. Pleistocene sea-surface temperature evolution: Early cooling, delayed glacial intensification, and implications for the mid-Pleistocene climate transition. Earth-Sci. Rev. 2013, 123, 173–193. [Google Scholar] [CrossRef]
- Feng, F.; Bailer-Jones, C. Obliquity and precession as pacemakers of Pleistocene deglaciations. Quat. Sci. Rev. 2015, 122, 166–179. [Google Scholar] [CrossRef]
- Viaggi, P. Evidence of Climate Mitigation Feedbacks by Global Forest Cover Expansion during the Pliocene. Int. J. Environ. Sci. Nat. Resour. 2021, 28, 556249. [Google Scholar] [CrossRef]
- Berger, A.; Loutre, M.F. Long-term variations in insolation and their effects on climate, the LLN experiments. Surv. Geophys. 1997, 18, 147–161. [Google Scholar] [CrossRef]
- Ruddiman, W.F. Orbital insolation, ice volume, and greenhouse gases. Quat. Sci. Rev. 2003, 22, 1597–1629. [Google Scholar] [CrossRef]
- Archer, D.; Martin, P.; Buffett, B.; Brovkin, V.; Rahmstorf, S.; Ganopolski, A. The importance of ocean temperature to global biogeochemistry. Earth Planet. Sci. Lett. 2004, 222, 333–348. [Google Scholar] [CrossRef]
- Rial, J.A.; Pielke, R.A., Sr.; Beniston, M.; Claussen, M.; Canadell, J.; Cox, P.; Held, H.; de Noblet-Ducoudré, N.; Prinn, R.; Reynolds, J.F.; et al. Nonlinearities, feedbacks and critical thresholds within the Earth’s climate system. Clim. Change 2004, 65, 11–38. [Google Scholar] [CrossRef]
- Brovkin, V.; Ganopolski, A.; Archer, D.; Rahmstorf, S. Lowering of glacial atmospheric CO2 in response to changes in oceanic circulation and marine biogeochemistry. Paleoceanography 2007, 22, PA4202. [Google Scholar] [CrossRef]
- Hansen, J.; Sato, M.; Kharecha, P.; Russell, G.; Lea, D.W.; Siddall, M. Climate change and trace gases. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2007, 365, 1925–1954. [Google Scholar] [CrossRef]
- Köhler, P.; Bintanja, R.; Fischer, H.; Joos, F.; Knutti, R.; Lohmann, G.; Masson-Delmotte, V. What caused Earth’s temperature variations during the last 800,000 years? Data-based evidence on radiative forcing and constraints on climate sensitivity. Quat. Sci. Rev. 2010, 29, 129–145. [Google Scholar] [CrossRef]
- Hansen, J.; Sato, M.; Kharecha, P.; von Schuckmann, K. Earth’s energy imbalance and implications. Atmos. Meas. Tech. 2011, 11, 13421–13449. [Google Scholar] [CrossRef]
- Miller, G.H.; Alley, R.B.; Brigham-Grette, J.; Fitzpatrick, J.J.; Polyak, L.; Serreze, M.C.; White, J.W. Arctic amplification: Can the past constrain the future? Quat. Sci. Rev. 2010, 29, 1779–1790. [Google Scholar] [CrossRef]
- Box, J.E.; Hubbard, A.; Bahr, D.B.; Colgan, W.T.; Fettweis, X.; Mankoff, K.D.; Wehrlé, A.; Noël, B.; Broeke, M.R.v.D.; Wouters, B.; et al. Greenland ice sheet climate disequilibrium and committed sea-level rise. Nat. Clim. Chang. 2022, 12, 808–813. [Google Scholar] [CrossRef]
- Wunsch, C. Quantitative estimate of the Milankovitch-forced contribution to observed Quaternary climate change. Quat. Sci. Rev. 2004, 23, 1001–1012. [Google Scholar] [CrossRef]
- Berger, A.; Mélice, J.L.; Loutre, M.F. On the origin of the 100-kyr cycles in the astronomical forcing. Paleoceanography 2005, 20, PA4019. [Google Scholar] [CrossRef]
- Berger, A.; Yin, Q.Z.; Herold, N. MIS-11 and MIS-19, analogs of our Holocene interglacial. In Proceedings of the 3rd International Conference on Earth System Modelling, Hamburg, Germany, 17–21 September 2012; Available online: https://meetingorganizer.copernicus.org/3ICESM/3ICESM-11.pdf (accessed on 1 January 2016).
- Martínez-Botí, M.A.; Foster, G.L.; Chalk, T.B.; Rohling, E.J.; Sexton, P.F.; Lunt, D.J.; Pancost, R.D.; Badger, M.P.S.; Schmidt, D.N. Plio-Pleistocene climate sensitivity evaluated using high-resolution CO2 records. Nature 2015, 518, 49–54. [Google Scholar] [CrossRef]
- Hönisch, B.; Hemming, N.G.; Archer, D.; Siddall, M.; McManus, J.F. Atmospheric Carbon Dioxide Concentration Across the Mid-Pleistocene Transition. Science 2009, 324, 1551–1554. [Google Scholar] [CrossRef]
- Seki, O.; Foster, G.L.; Schmidt, D.N.; Mackensen, A.; Kawamura, K.; Pancost, R.D. Alkenone and boron-based Pliocene pCO2 records. Earth Planet. Sci. Lett. 2010, 292, 201–211. [Google Scholar] [CrossRef]
- Bartoli, G.; Hönisch, B.; Zeebe, R.E. Atmospheric CO2 decline during the Pliocene intensification of Northern Hemisphere glaciations. Paleoceanography 2011, 26, PA4213. [Google Scholar] [CrossRef]
- Huybers, P.; Tziperman, E. Integrated summer insolation forcing and 40,000-year glacial cycles: The perspective from an ice-sheet/energy-balance model. Paleoceanography 2008, 23, PA1208. [Google Scholar] [CrossRef]
- Yehudai, M.; Kim, J.; Pena, L.D.; Jaume-Seguí, M.; Knudson, K.P.; Bolge, L.; Malinverno, A.; Bickert, T.; Goldstein, S.L. Evidence for a Northern Hemispheric trigger of the 100,000-y glacial cyclicity. Proc. Natl. Acad. Sci. USA 2021, 118, e2020260118. [Google Scholar] [CrossRef]
- Waelbroeck, C.; Levi, C.; Duplessy, J.-C.; Labeyrie, L.; Michel, E.; Cortijo, E.; Bassinot, F.; Guichard, F. Distant origin of circulation changes in the Indian Ocean during the last deglaciation. Earth Planet. Sci. Lett. 2006, 243, 244–251. [Google Scholar] [CrossRef]
- Yin, Q.Z.; Berger, A. Individual contribution of insolation and CO2 to the interglacial climates of the past 800,000 years. Clim. Dyn. 2011, 38, 709–724. [Google Scholar] [CrossRef]
- Masson-Delmotte, V.; Kageyama, M.; Braconnot, P.; Charbit, S.; Krinner, G.; Ritz, C.; Guilyardi, E.; Jouzel, J.; Abe-Ouchi, A.; Crucifix, M.; et al. Past and future polar amplification of climate change: Climate model intercomparisons and ice-core constraints. Clim. Dyn. 2005, 26, 513–529. [Google Scholar] [CrossRef]
- Cuffey, K.M.; Clow, G.D. Temperature, accumulation, and ice sheet elevation in central Greenland through the last deglacial transition. J. Geophys. Res. 1997, 102, 26383–26396. [Google Scholar] [CrossRef]
- Lemke, P.; Ren, J.; Alley, R.B.; Allison, I.; Carrasco, J.; Flato, G.; Fujii, Y.; Kaser, G.; Mote, P.; Thomas, R.H.; et al. Observations: Changes in Snow, Ice and Frozen Ground. In Climate Change 2007: The Physical Science Basis. Contribution of Working Group I to the Fourth Assessment Report of the Intergovernmental Panel on Climate Change; Solomon, S., Qin, D., Manning, M., Chen, Z., Marquis, M., Averyt, K.B., Tignor, M., Miller, H.L., Eds.; Cambridge University Press: Cambridge, UK; New York, NY, USA, 2007. [Google Scholar]
- Hanna, E.; Huybrechts, P.; Steffen, K.; Cappelen, J.; Huff, R.; Shuman, C.; Irvine-Fynn, T.; Wise, S.; Griffiths, M. Increased Runoff from Melt from the Greenland Ice Sheet: A Response to Global Warming. J. Clim. 2008, 21, 331–341. [Google Scholar] [CrossRef]
- Lawrence, D.M.; Slater, A.G.; Tomas, R.A.; Holland, M.M.; Deser, C. Accelerated Arctic land warming and permafrost degradation during rapid sea ice loss. Geophys. Res. Lett. 2008, 35, L11506. [Google Scholar] [CrossRef]
- Ettema, J.; van den Broeke, M.R.; van Meijgaard, E.; van de Berg, W.J.; Bamber, J.L.; Box, J.E.; Bales, R.C. Higher surface mass balance of the Greenland ice sheet revealed by high-resolution climate modeling. Geophys. Res. Lett. 2009, 36, L12501. [Google Scholar] [CrossRef]
- Tarnocai, C.; Canadell, J.G.; Schuur, E.A.G.; Kuhry, P.; Mazhitova, G.; Zimov, S. Soil organic carbon pools in the northern circumpolar permafrost region. Glob. Biogeochem. Cycles 2009, 23, GB2023. [Google Scholar] [CrossRef]
- Yin, J.; Overpeck, J.T.; Griffies, S.M.; Hu, A.; Russell, J.L.; Stouffer, R.J. Different magnitudes of projected subsurface ocean warming around Greenland and Antarctica. Nat. Geosci. 2011, 4, 524–528. [Google Scholar] [CrossRef]
- Pattyn, F.; Ritz, C.; Hanna, E.; Asay-Davis, X.; DeConto, R.; Durand, G.; Favier, L.; Fettweis, X.; Goelzer, H.; Golledge, N.R.; et al. The Greenland and Antarctic ice sheets under 1.5 °C global warming. Nat. Clim. Change 2018, 8, 1053–1061. [Google Scholar] [CrossRef]
- King, M.D.; Howat, I.M.; Candela, S.G.; Noh, M.J.; Jeong, S.; Noël, B.P.Y.; van den Broeke, M.R.; Wouters, B.; Negrete, A. Dynamic ice loss from the Greenland Ice Sheet driven by sustained glacier retreat. Commun. Earth Environ. 2020, 1, 1. [Google Scholar] [CrossRef]
- Tesi, T.; Asioli, A.; Previde Massara, E.; Montagna, P.; Pellegrini, C.; Nogarotto, A.; Cipriani, A.; Piva, A.; Muschitiello, F.; Rovere, M.; et al. Abrupt and persistent shutdown of the thermohaline forcing during MIS5e in the Adriatic Sea: Insights from shallow-water sapropel sediments. Quat. Sci. Adv. 2023, 13, 100134. [Google Scholar] [CrossRef]
- Hilgen, F.J.; Lourens, L.J.; Berger, A.; Loutre, M.F. Evaluation of the astronomically calibrated time scale for the Late Piocene and the earliest Pleistocene. Paleoceanography 1993, 8, 549–565. [Google Scholar] [CrossRef]
- Shackleton, N.J. Oxygen isotope analysis and pleistocene temperature reassessed. Nature 1967, 215, 15–17. [Google Scholar] [CrossRef]
- Chao, B.F. Earth’s oblateness and its temporal variations. Comptes. Rendus Geosci. 2006, 338, 1123–1129. [Google Scholar] [CrossRef]
- Nerem, R.S.; Wahr, J. Recent changes in the Earth’s oblateness driven by Greenland and Antarctic ice mass loss. Geophys. Res. Lett. 2011, 38, L13501. [Google Scholar] [CrossRef]
- Whitehouse, P.L. Glacial isostatic adjustment modelling: Historical perspectives, recent advances, and future directions. Earth Surf. Dyn. 2018, 6, 401–429. [Google Scholar] [CrossRef]
- Mitrovica, J.X.; Peltier, W.R. Present-day secular variations in the zonal harmonics of the Earth’s geopotential. J. Geophys. Res. 1993, 98, 4509–4526. [Google Scholar] [CrossRef]
- Cheng, M.; Tapley, B.D. Variations in the Earth’s oblateness during the past 28 years. J. Geophys. Res. 2004, 109, B09402. [Google Scholar] [CrossRef]
- Cheng, M.; Tapley, B.D.; Ries, J.C. Deceleration in the Earth’s oblateness. J. Geophys. Res. Solid Earth 2013, 118, 740–747. [Google Scholar] [CrossRef]
- Seo, K.-W.; Chen, J.; Wilson, C.R.; Lee, C.-K. Decadal and quadratic variations of Earth’s oblateness and polar ice mass balance from 1979 to 2010. Geophys. J. Int. 2015, 203, 475–481. [Google Scholar] [CrossRef]
- Nakada, M.; Okuno, J. Secular variations in zonal harmonics of Earth’s geopotential and their implications for mantle viscosity and Antarctic melting history due to the last deglaciation. Geophys. J. Int. 2017, 209, 1660–1676. [Google Scholar] [CrossRef]
- Sun, Y.; Riva, R.; Ditmar, P.; Rietbroek, R. Using GRACE to Explain Variations in the Earth’s Oblateness. Geophys. Res. Lett. 2019, 46, 158–168. [Google Scholar] [CrossRef]
- Sun, Y.; Riva, R.E.M. A global semi-empirical glacial isostatic adjustment (GIA) model based on Gravity Recovery and Climate Experiment (GRACE) data. Earth Syst. Dyn. 2020, 11, 129–137. [Google Scholar] [CrossRef]
- Govin, A.; Braconnot, P.; Capron, E.; Cortijo, E.; Duplessy, J.C.; Jansen, E.; Labeyrie, L.; Landais, A.; Marti, O.; Michel, E.; et al. Persistent influence of ice sheet melting on high northern latitude climate during the early Last Interglacial. Clim. Past 2012, 8, 483–507. [Google Scholar] [CrossRef]
- Masson-Delmotte, V.; Braconnot, P.; Hoffmann, G.; Jouzel, J.; Kageyama, M.; Landais, A.; Lejeune, Q.; Risi, C.; Sime, L.; Sjolte, J.; et al. Sensitivity of interglacial Greenland temperature and δ18O: Ice core data, orbital and increased CO2 climate simulations. Clim. Past 2011, 7, 1041–1059. [Google Scholar] [CrossRef]
- Berger, A.; Loutre, M.; Tricot, C. Insolation and Earth’s orbital periods. J. Geophys. Res. Atmos. 1993, 98, 10341–10362. [Google Scholar] [CrossRef]
- Rial, J.A. Pacemaking the Ice Ages by frequency modulation of Earth’s orbital eccentricity. Science 1999, 285, 564–568. [Google Scholar] [CrossRef]



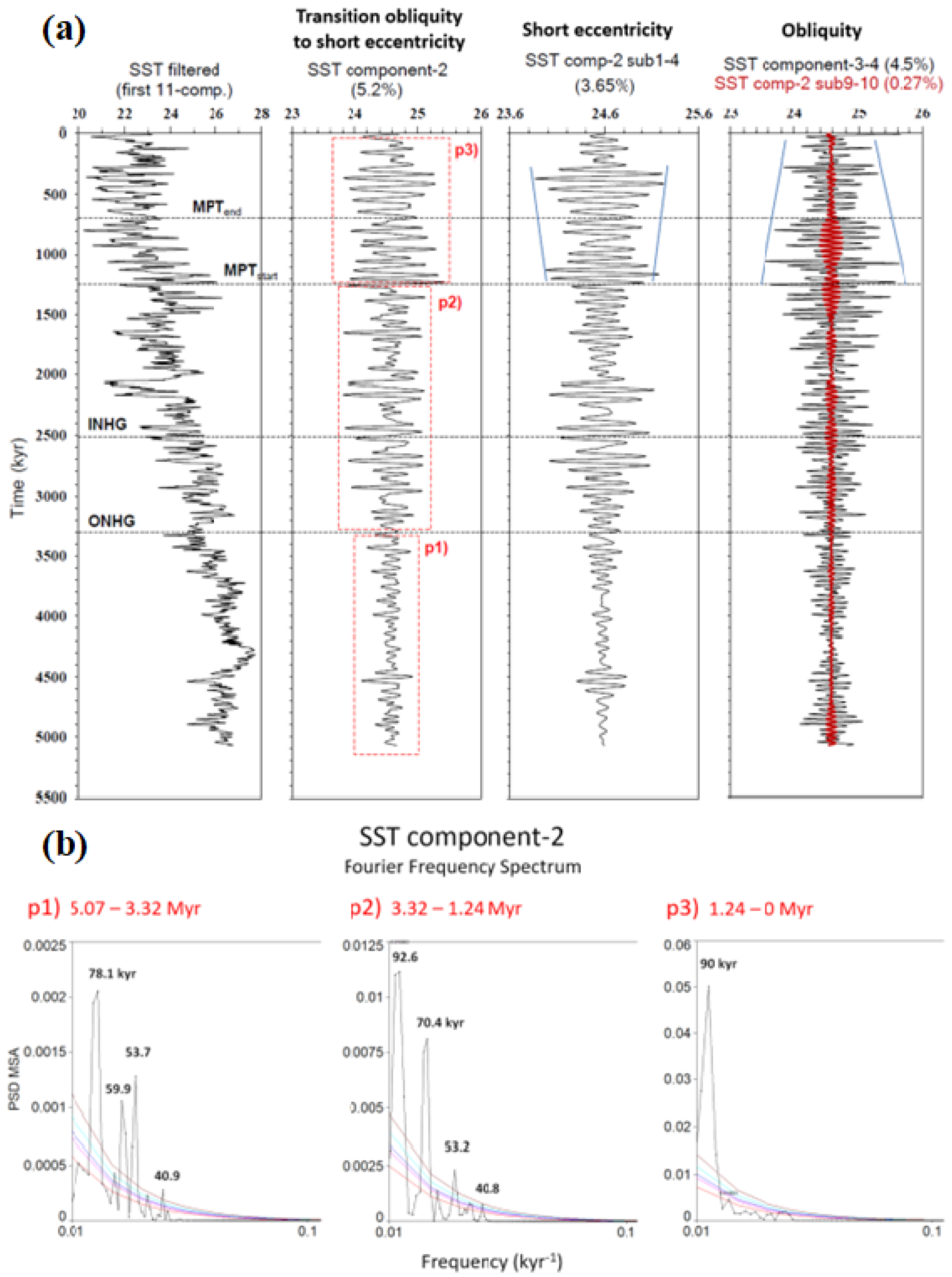

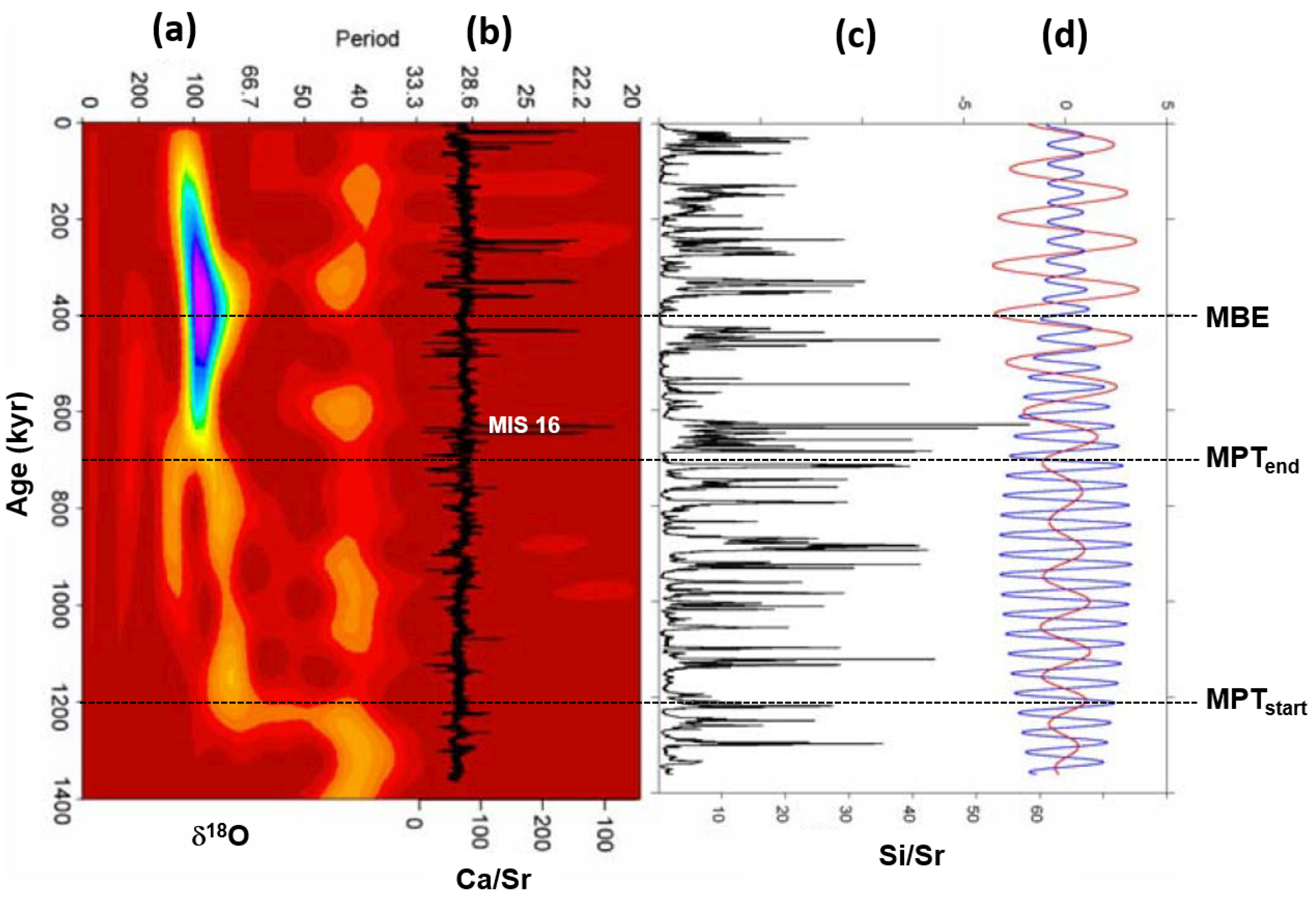

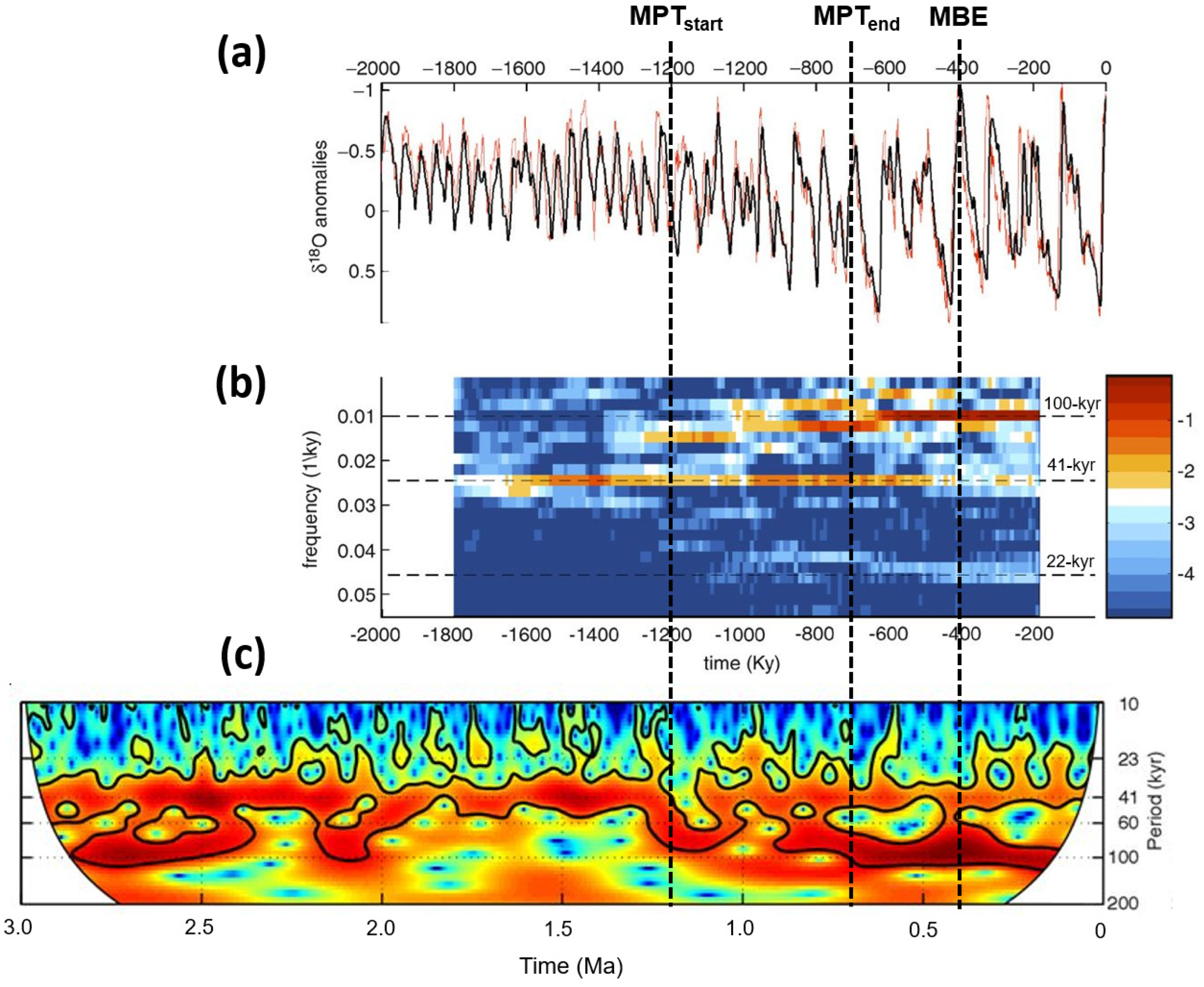
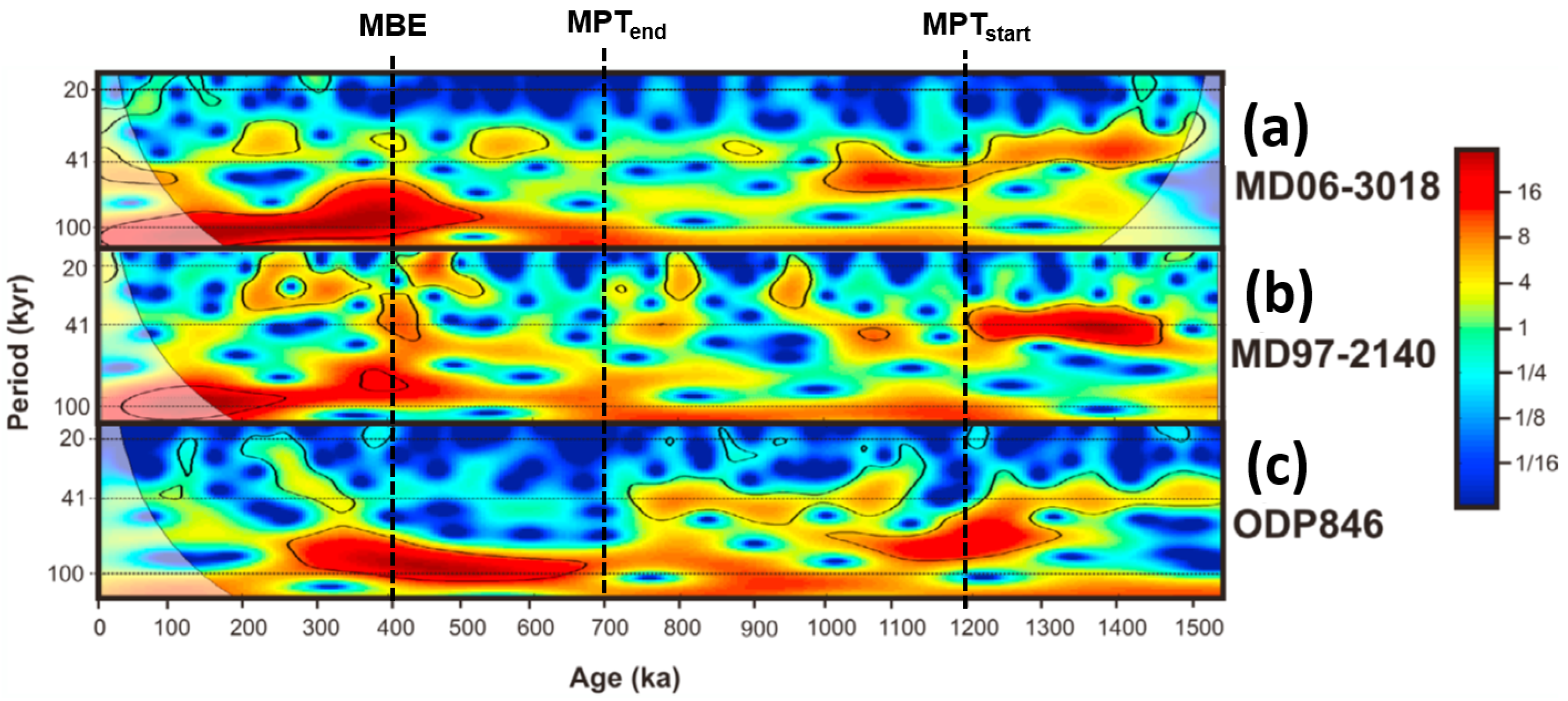



| Rs statistic | Signal | Short Eccentricity | Obliquity | Precession |
|---|---|---|---|---|
| Max | δD | 6.40 | 1.56 | 2.78 |
| CO2 | 6.18 | 4.21 | 2.58 | |
| CH4 | 7.32 | 4.97 | 4.48 | |
| δ18O | 7.70 | 2.11 | 2.25 | |
| Min | δD | −1.02 | −0.45 | –1.10 |
| CO2 | −0.88 | −0.97 | −0.96 | |
| CH4 | −0.46 | −1.08 | −0.96 | |
| δ18O | −0.04 | −0.70 | −0.97 |
| RSL Component Rank | RSL Component Variance | Frequency (kyr−1) | TISA Power (%) | Period (kyr) | Forcing |
|---|---|---|---|---|---|
| 1–2; 7–10 | 61.4% | 0.01001973 | 100.0 | 100 | Short eccentricity |
| 3–4; 13–14 | 13.1% | 0.02460637 | 84.8 | 41 | Obliquity |
| 0.03244360 | 15.2 | 31 | |||
| 5–6; 11–12 | 11.7% | 0.04393214 | 80.9 | 23 | Precession |
| 0.05342083 | 19.1 | 19 | |||
| 15–200 | 13.8% | Suborbital + noise |
| Forcing | Red Sea 1 | Antarctica 1 | LR04 | ||
|---|---|---|---|---|---|
| RSL (%) | Δσ (%) | EPICA (%) | Δσ (%) | δ18O (%) | |
| Short eccentricity | 14.5 | 8.0 | 12.2 | 5.7 | 6.5 |
| Obliquity | 3.1 | −6.8 | 4.5 | −5.4 | 9.9 |
| Precession | 2.8 | 0.8 | 2.0 | 0.0 | 2.0 |
| Forcing | Signal | Cross-Spectrum Freq. (kyr−1) | Cross-Spectrum Period (kyr) | Coherency | Phase Shift (Deg, kyr) | ||
|---|---|---|---|---|---|---|---|
| Short eccentricity * | RSL | 0.010 | 100.0 | 0.78 | −12.6 | −3.50 | RSL lags short ecc. |
| δ18O | 0.010 | 100.0 | 0.75 | −9.8 | −2.72 | δ18O lags short ecc. | |
| RSL vs. δ18O | 0.010 | 100.0 | 0.94 | 2.8 | 0.78 | δ18O leads RSL | |
| Obliquity | RSL | 0.024 | 41.7 | 0.88 | −48.8 | −5.65 | RSL lags obl. |
| δ18O | 0.024 | 41.7 | 0.86 | −66.1 | −7.65 | δ18O lags obl. | |
| RSL vs. δ18O | 0.024 | 41.7 | 0.83 | −17.3 | −2.00 | δ18O lags RSL | |
| Precession | RSL | 0.044 | 22.7 | 0.92 | −46.3 | −2.92 | RSL lags prec. |
| δ18O | 0.044 | 22.7 | 0.88 | −62.7 | −3.96 | δ18O lags prec. | |
| RSL vs. δ18O | 0.044 | 22.7 | 0.92 | −16.5 | −1.04 | δ18O lags RSL | |
| Forcing | EPICA Signal | Cross-Spectrum Freq. (kyr−1) | Cross-Spectrum Period (kyr) | Coherency | Phase Shift (Deg, kyr) | ||
|---|---|---|---|---|---|---|---|
| Eccentricity | Short ecc. stack | 0.01125 | 88.9 | 0.94 | 17.4 | 4.30 | EPICA signal leads |
| Short eccentricity 1 | Short ecc. stack | 0.01125 | 88.9 | 0.94 | 18.3 | 4.52 | EPICA signal leads |
| Mean annual insolation (short eccentricity 1) | Short ecc. stack | 0.01 | 100.0 | 0.72 | 13.8 | 3.83 | EPICA signal leads |
| Obliquity | Obliquity stack | 0.025 | 40.0 | 0.98 | −37.9 | −4.21 | EPICA signal lags |
| Precession | Precession stack | 0.0425 | 23.5 | 0.67 | −60.8 | −3.97 | EPICA signal lags |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Viaggi, P. Global Evidence of Obliquity Damping in Climate Proxies and Sea-Level Record during the Last 1.2 Ma: A Missing Link for the Mid-Pleistocene Transition. Geosciences 2023, 13, 354. https://doi.org/10.3390/geosciences13120354
Viaggi P. Global Evidence of Obliquity Damping in Climate Proxies and Sea-Level Record during the Last 1.2 Ma: A Missing Link for the Mid-Pleistocene Transition. Geosciences. 2023; 13(12):354. https://doi.org/10.3390/geosciences13120354
Chicago/Turabian StyleViaggi, Paolo. 2023. "Global Evidence of Obliquity Damping in Climate Proxies and Sea-Level Record during the Last 1.2 Ma: A Missing Link for the Mid-Pleistocene Transition" Geosciences 13, no. 12: 354. https://doi.org/10.3390/geosciences13120354
APA StyleViaggi, P. (2023). Global Evidence of Obliquity Damping in Climate Proxies and Sea-Level Record during the Last 1.2 Ma: A Missing Link for the Mid-Pleistocene Transition. Geosciences, 13(12), 354. https://doi.org/10.3390/geosciences13120354








