Changes in Unfrozen Water Contents in Warming Permafrost Soils
Abstract
:1. Introduction
2. Materials for Estimating Unfrozen Water Content in Permafrost
3. Water Potential Method and Its Advantages
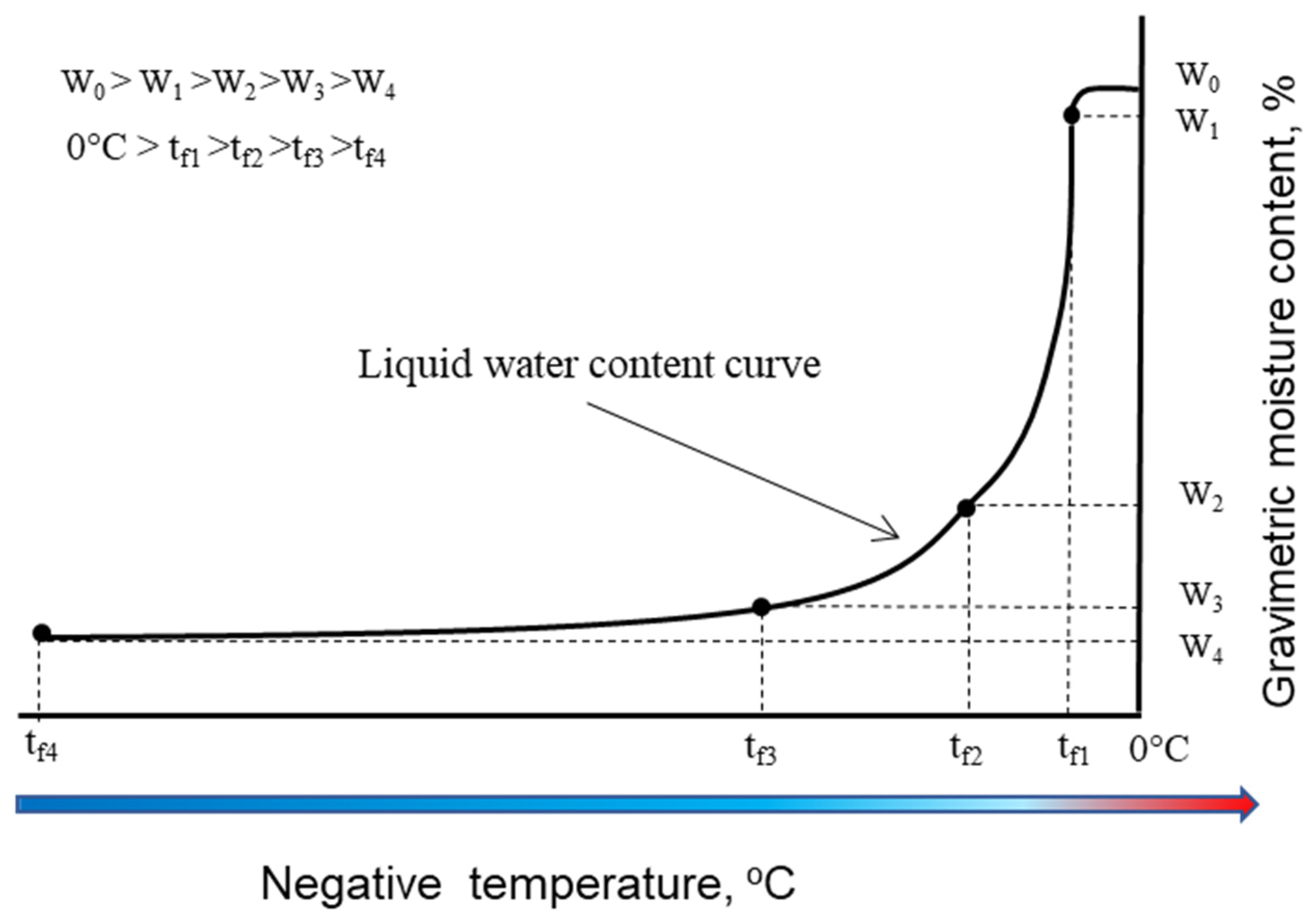
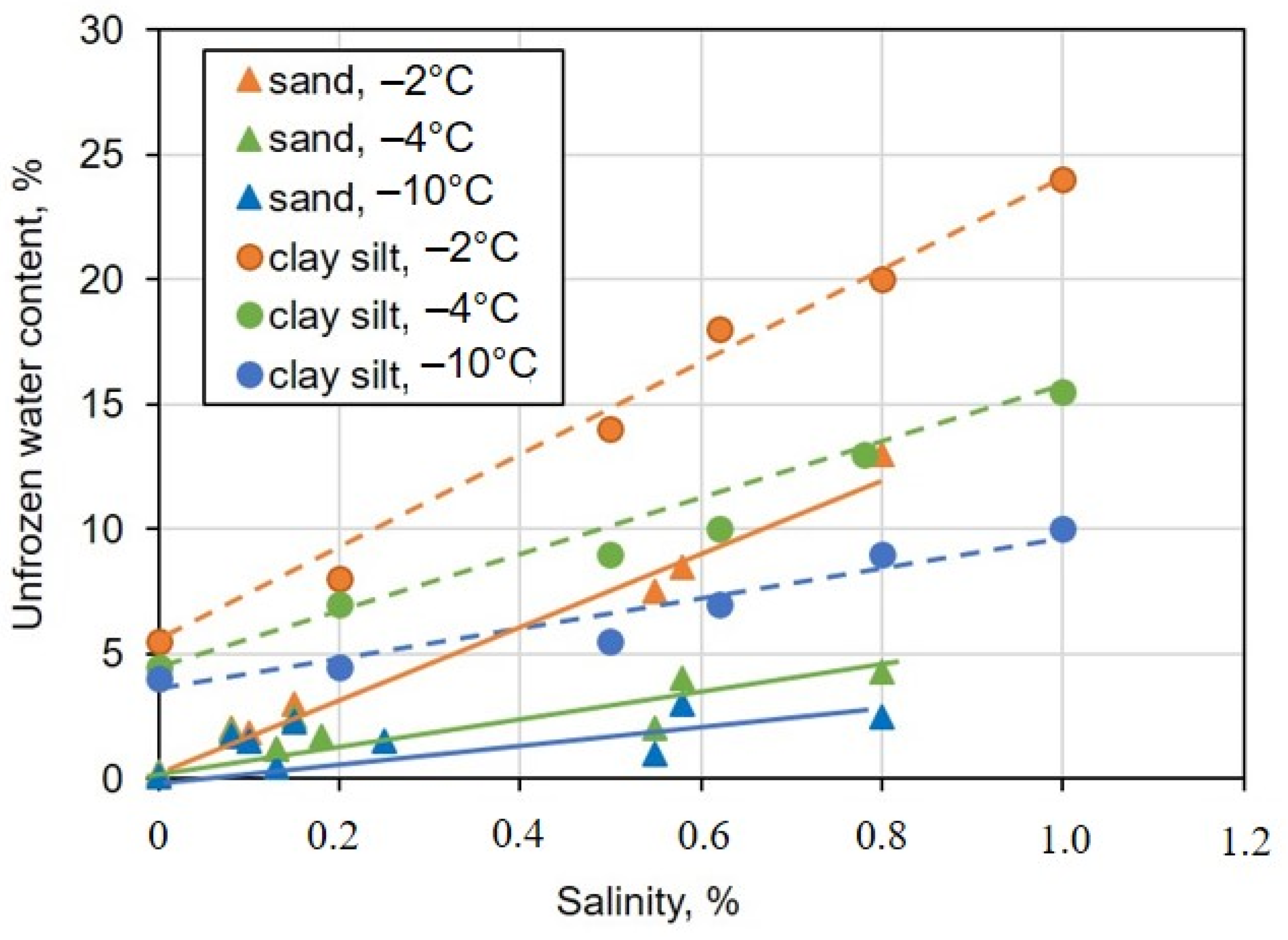
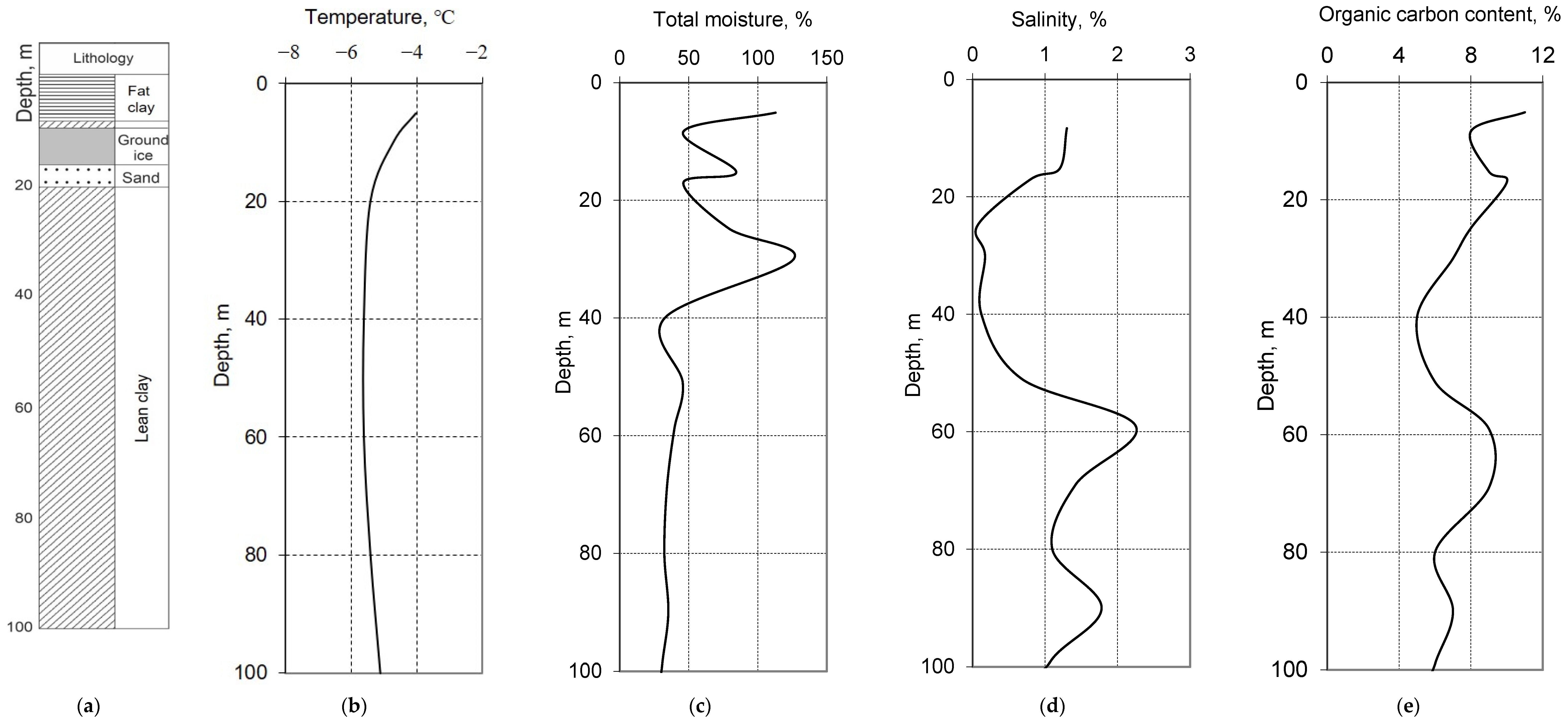
4. Main Controls of Unfrozen Water Content in Permafrost
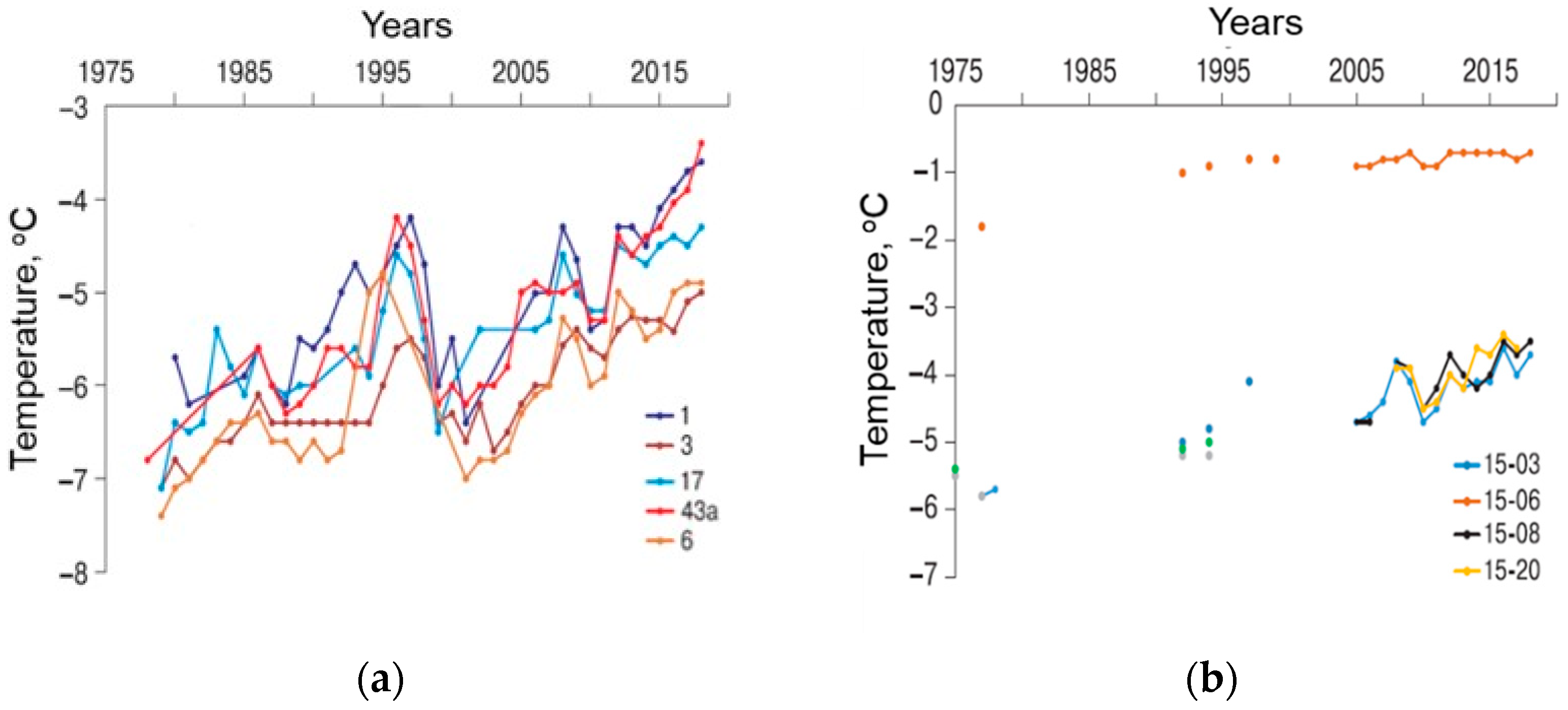
5. Discussion
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Jorgenson, M.T.; Racine, C.H.; Walters, J.C.; Osterkamp, T.E. Permafrost degradation and ecological changes associated with a warming climate in Central Alaska. Clim. Chang. 2001, 48, 551–579. [Google Scholar] [CrossRef]
- Wang, D.; Tighe, S.L.; Yin, S. Preliminary analysis of permafrost degradation in Ingraham Trail, Northwest Territories. In Proceedings of the Canadian Society of Civil Engineering Annual Conference 2021; CSCE 2021; Lecture Notes in Civil Engineering, 240; Springer: Singapore, 2023. [Google Scholar] [CrossRef]
- Maadani, O.; Shafiee, M.; Egorov, I. Climate change challenges for flexible pavement in Canada: An overview. J. Cold Reg. Eng. 2021, 35, 03121002. [Google Scholar] [CrossRef]
- Melnikov, V.P.; Osipov, V.I.; Brouchkov, A.V.; Falaleeva, A.A.; Badina, S.V.; Zheleznyak, M.N.; Sadurtdinov, M.R.; Ostrakov, N.A.; Drozdov, D.S.; Osokin, A.B.; et al. Climate warming and permafrost thaw in the Russian Arctic: Potential economic impacts on public infrastructure by 2050. Nat. Hazards 2022, 112, 231–251. [Google Scholar] [CrossRef]
- Hjort, J.; Karjalainen, O.; Aalto, J.; Westermann, S.; Romanovsky, V.E.; Nelson, F.E.; Etzelmüller, B.; Luoto, M. Degrading permafrost puts Arctic infrastructure at risk by mid-century. Nat. Commun. 2018, 9, 5147. [Google Scholar] [CrossRef] [PubMed]
- Romanovsky, V.E.; Drozdov, D.S.; Oberman, N.G.; Malkova, G.V.; Kholodov, A.L.; Marchenko, S.S.; Moskalenko, N.G.; Sergeev, D.O.; Ukraintseva, N.G.; Abramov, A.A.; et al. Thermal state of permafrost in Russia. Permafr. Periglac. Processes 2010, 21, 136–155. [Google Scholar] [CrossRef]
- Harris, S.A.; Brouchkov, A.; Goudong, C. Geocryology. Characteristics and Use of Frozen Ground and Permafrost Landforms; CRC Press, Taylor & Francis Group: London, UK, 2018; 765p. [Google Scholar]
- Macdonald, R.W.; Harner, T.; Fyfe, J. Recent climate change in the Arctic and its impact on contaminant pathways and interpretation of temporal trend data. Sci. Total Environ. 2005, 342, 5–86. [Google Scholar] [CrossRef]
- Yu, W.; Zhang, T.; Lu, Y.; Han, F.; Zhou, Y.; Hu, D. Engineering risk analysis in cold regions: State of the art and perspectives. Cold Reg. Sci. Technol. 2020, 171, 102963. [Google Scholar] [CrossRef]
- Peng, H.; Ma, W.; Mu, Y.L.; Jin, L. Impact of permafrost degradation on embankment deformation of Qinghai-Tibet Highway in permafrost regions. J. Cent. South Univ. 2015, 22, 1079–1086. [Google Scholar] [CrossRef]
- Kong, X.; Doré, G. Thermal stabilization of embankments built on thaw-sensitive permafrost. J. Cold Reg. Eng. 2019, 35, 04021010. [Google Scholar] [CrossRef]
- Buslaev, G.; Tsvetkov, P.; Lavrik, A.; Kunshin, A.; Loseva, E.; Sidorov, D. Ensuring the sustainability of Arctic industrial facilities under conditions of global climate change. Resources 2021, 10, 128. [Google Scholar] [CrossRef]
- Khrustalev, L.N. Fundamentals of Geotechnics in Cryolithozone; MSU Press: Moscow, Russia, 2005; 544p. (In Russian) [Google Scholar]
- Boyoucous, G.J. Degree of temperature to which soils can be cooled without freezing. J. Agric. Res. 1920, 20, 267–269. [Google Scholar] [CrossRef]
- Andrianov, P.I. The freezing point of soils. Akad. Nauk SSSR 1936, 1, 17–54. (In Russian) [Google Scholar]
- Sumgin, M.I.; Kachurin, S.P.; Tolstikhin, N.I.; Tumel, V.F. General permafrost science. Akad. Nauk SSSR 1940, 340. (In Russian) [Google Scholar]
- Tsytovich, N.A. On the theory of water equilibrium in frozen soils. Izv. AN SSSR Ser. Geogr. Geofiz. 1945, 9, 493–502. [Google Scholar]
- Are, F.E. Thermal aspects of Tsytovich principle of water and ice equilibrium state in frozen ground. Kriosf. Zemli 2014, XVIII, 47–56. [Google Scholar]
- Yershov, E.D.; Akimov, Y.P.; Cheverev, V.G.; Kuchukov, E.Z. Phase Composition of Pore Moisture in Permafrost; Moscow University Press: Moscow, Russia, 1979; 189p. (In Russian) [Google Scholar]
- Williams, P.J.; Smith, M.W. The Frozen Earth. Fundamentals of Geocryology; Carleton University: Ottawa, ON, Canada, 1989; 306p. [Google Scholar]
- Cheverev, V.G. Properties of bound water in cryogenic soils. Kriosf. Zemli 2003, VII, 30–41. [Google Scholar]
- Yershov, E.D. (Ed.) Fundamentals of Geocryology. Part 1. Physico-Chemical Geocryology; Moscow University Press: Moscow, Russia, 1995; 368p. (In Russian) [Google Scholar]
- Yershov, E.D. General Geocryology; Cambridge University Press: Cambridge, UK, 1998; 580p. [Google Scholar]
- Frolov, A.D. Electrical and Elastic Properties of Frozen Rocks and Ice; ONTI PNC RAN: Pushchino, Russia, 1998; 515p. (In Russian) [Google Scholar]
- Roman, L.T. Mechanic Properties of Frozen Ground; Nauka/Interperiodika: Moscow, Russia, 2002; 426p. (In Russian) [Google Scholar]
- Andersland, O.B.; Ladanyi, B. Frozen Ground Engineering; John Wiley: New York, NY, USA, 2003; 363p. [Google Scholar]
- Cheverev, V.G. Cryogenic Properties of Soils; Nauchniy Mir: Moscow, Russia, 2004; 234p. (In Russian) [Google Scholar]
- Sun, Q.J.; Li, D.W.; Wang, S.Y. Research on uniaxial compressive strength of frozen sand containing salt with different water contents. Coal Technol. 2015, 34, 86–88. [Google Scholar]
- Xu, X.T.; Wang, Y.B.; Bai, R.Q.; Fan, C.X.; Hua, S.G. Comparative studies on mechanical behavior of frozen natural saline silty sand and frozen desalted silty sand. Cold Reg. Sci. Technol. 2016, 132, 81–88. [Google Scholar] [CrossRef]
- Zhao, X.; Zhou, G.; Lu, G. Strain responses of frozen clay with thermal gradient under triaxial creep. Acta Geotech. 2017, 12, 183–193. [Google Scholar] [CrossRef]
- Tang, R.; Zhou, G.; Wang, J.; Zhao, G.; Lai, Z.; Jiu, F. A new method for estimating salt expansion in saturated saline soils during cooling based on electrical conductivity. Cold Reg. Sci. Technol. 2020, 170, 102943. [Google Scholar] [CrossRef]
- Liu, J.; Yang, P.; Yang, Z. Electrical properties of frozen saline clay and their relationship with unfrozen water content. Cold Reg. Sci. Technol. 2020, 178, 103127. [Google Scholar] [CrossRef]
- Grechishchev, S.E.; Chistotinov, L.V.; Shur, Y.L. Cryogenic Physical and Geological Processes and their Prediction; Nedra: Moscow, Russia, 1980; 384p. (In Russian) [Google Scholar]
- Komarov, I.A. Thermodynamics and Heat and Mass Transfer in Fine-Grained Porous Rocks; Nauchnyi Mir: Moscow, Russia, 2003; 608p. (In Russian) [Google Scholar]
- Starostin, E.G. Determination of the amount of unfrozen water, based on the crystallization kinetics. Kriosf. Zemli 2008, XII, 60–64. [Google Scholar]
- Liao, M.; Lai, Y.; Liu, E.; Wan, X. A fractional order creep constitutive model of warm frozen silt. Acta Geotech. 2017, 12, 377–389. [Google Scholar] [CrossRef]
- Kong, L.; Wang, Y.; Sun, W.; Qi, J. Influence of plasticity on unfrozen water content of frozen soils as determined by nuclear magnetic resonance. Cold Reg. Sci. Technol. 2020, 172, 102993. [Google Scholar] [CrossRef]
- Wang, L.; Liu, J.; Yu, X.; Wang, T.; Feng, R. A simplified model for the phase composition curve of saline soils considering the second phase transition. Water Resour. Res. 2021, 57, e2020WR028556. [Google Scholar] [CrossRef]
- Design Standards #II-B.6-66; Basements and Foundations of Buildings and Structures on Permafrost. Gosstroyizdat: Moscow, Russia, 1967; 33p. (In Russian)
- Working Document SNIP 2.02.04-88; Design Standards. Basements and Foundations on Permafrost. Gosstroy: Moscow, Russia, 2005; 52p. (In Russian)
- Koopmans, R.W.R.; Miller, R. Soil Freezing and Soil Water Characteristic Curves 1. Soil Sci. Soc. Am. J. 1966, 30, 680–685. [Google Scholar] [CrossRef]
- Dillon, H.B.; Andersland, O.B. Predicting unfrozen water contents in frozen soils. Can. Geotech. J. 1966, 3, 53–60. [Google Scholar] [CrossRef]
- Anderson, D.M.; Tice, A.R. Predicting unfrozen water contents in frozen soils from surface area measurements. Highw. Res. Rec. 1972, 393, 12–18. [Google Scholar]
- Anderson, D.M.; Tice, A.R.; McKim, H.L. The unfrozen water and the apparent specific heat capacity of frozen soils. In Proceedings of the Second International Conference on Permafrost, Jakutsk, Russia, 16–28 July 1973; North American Contribution National Academy of Science: Washington, DC, USA, 1973; pp. 289–294. [Google Scholar]
- Tice, A.R.; Anderson, D.M.; Banin, A. The Prediction of Unfrozen Water Contents in Frozen Soils from Liquid Limit Determinations; CRREL Report 79-8; Cold Regions Research and Engineering Laboratory: Hanover, NH, USA, 1976; 13p. [Google Scholar]
- Low, P.F.; Anderson, D.M.; Duwayne, M.; Hoekstra, P. Some thermodynamic relationships for soils at or below the freezing point. 1. Freezing point depression and heat capacity. Water Resour. Res. 1968, 4, 379–394. [Google Scholar] [CrossRef] [Green Version]
- Roman, L.T. Frozen Peat-Bearing Foundation Soils; Nauka: Novosibirsk, Russia, 1987; 222p. (In Russian) [Google Scholar]
- Sheikin, I.V. Calculated relationships between water-physical variables of permafrost. In Formation of Frozen Ground and Prediction of Cryogenic Processes; Nauka: Moscow, Russia, 1986; pp. 151–156. (In Russian) [Google Scholar]
- Kozlowski, T. A semi-empirical model for phase composition of water in clay-water systems. Cold Reg. Sci. Technol. 2007, 49, 226–236. [Google Scholar] [CrossRef]
- Qin, Y.; Li, G.; Qu, G. A formula for the unfrozen water content and temperature of frozen soils. In Proceedings of the 14th Conference on Cold Regions Engineering, Duluth, MN, USA, 31 August–2 September 2009; pp. 155–161. [Google Scholar] [CrossRef]
- Istomin, V.; Chuvilin, E.; Makhonina, N.; Bukhanov, B. Temperature dependence of unfrozen water content in sediments on the water potential measurements. Kriosf. Zemli 2009, XIII, 35–43. [Google Scholar]
- Yershov, E.D. (Ed.) Methods of Geocryological Research; Moscow University Press: Moscow, Russia, 2004; 512p. (In Russian) [Google Scholar]
- Nersesova, Z.A. Phase composition of Pore Moisture in Freezing and Thawing Soils. In Laboratory Studies of Frozen Soils; Idatel’stvo AN SSSR: Moscow, Russia, 1953; Volume 1, pp. 37–51. (In Russian) [Google Scholar]
- Nersesova, Z.A.; Tsytovich, H.A. Unfrozen water in frozen ground. In Proceedings of the International Conference on Permafrost, Lafayette, IN, USA, 11–15 November 1963; Section 4. Phase Equilibrium and Transitions. Izd. AN SSSR: Moscow, Russia, 1963; pp. 62–70. (In Russian). [Google Scholar]
- Williams, P.J. Specific Heat and Unfrozen Water Contents of Frozen Soils; Technical Memorandum 76; Natural Research Council of Canada, Associate Committee on Soil and Snow Mechanics: Ottawa, ON, Canada, 1963; pp. 109–126. [Google Scholar]
- Williams, P.J. Experimental determination of apparent specific heat of frozen soils. Geotechnique 1964, 14, 133–142. [Google Scholar] [CrossRef] [Green Version]
- Sargsyan, R.M.; Nersesova, Z.A.; Vyalov, S.S.; Zazarnaya, A.G. (Eds.) Guidelines for the Estimation of Physical, Thermal, and Mechanic Properties of Frozen Soils; Gosstoyizdat: Moscow, Russia, 1973; 194p. (In Russian) [Google Scholar]
- Kvlividze, V.I.; Kiselev, V.F.; Ushakova, L.A. NMR of the mobile water phase on the ice surface. Bull. Mosc. State Univ. 1974, 6, 736–738. [Google Scholar]
- Ananyan, A.A. Phase composition of water in frozen fine-grained rocks determined by NMR relaxometry. In Frozen Soils and Snow Cover; Nauka: Moscow, Russia, 1977; pp. 82–91. (In Russian) [Google Scholar]
- Tice, A.R.; Barrous, C.M.; Anderson, D.M. Phase composition measurements on soils at very high water contents by the pulsed Nuclear Magnetic Resonance technique. Transp. Res. Rec. 1978, 675, 11–14. [Google Scholar] [CrossRef]
- Tice, A.R.; Anderson, D.M.; Sterrett, K.F. Unfrozen water contents of submarine permafrost determined by nuclear magnetic resonance. Eng. Geol. 1981, 18, 135–146. [Google Scholar] [CrossRef]
- Oliphant, J.L.; Tice, A.R. Comparison of unfrozen water contents measured by DSC and NMR. In Proceedings of the 3rd International Symposium on Ground Freezing, Hanover, NH, USA, 22–24 June 1982; pp. 115–121. [Google Scholar]
- Tice, A.R.; Oliphant, J.L. The effect of magnetic particles on the unfrozen water contents of frozen soils determined by Nuclear Magnetic Resonance. Soil Sci. 1984, 138, 63–73. [Google Scholar] [CrossRef]
- Votyakov, I.N. Mechanic Properties of Permafrost in Yakutia; Nauka: Novosibirsk, Russia, 1975; 176p. (In Russian) [Google Scholar]
- Frolov, A.D. Electrical and Elastic Properties of Permafrost; Nedra: Moscow, Russia, 1976; 254p. (In Russian) [Google Scholar]
- Paterson, D.E.; Smith, M.W. The use of time domain reflectometry for the measurement of unfrozen water content in frozen soils. Cold Reg. Sci. Technol. 1980, 3, 205–210. [Google Scholar] [CrossRef]
- Paterson, D.E.; Smith, M.W. The measurement of unfrozen water content by time domain reflectometry: Results from laboratory tests. Can. Geotech. J. 1981, 18, 131–144. [Google Scholar] [CrossRef]
- Gurov, V.V. Methodology and some experimental data on dielectric properties of frozen soils. Merzlotnye Issled. 1983, 21, 170–178. [Google Scholar]
- Stein, J.; Kane, D.L. Monitoring the unfrozen water content of soil and snow using time domain reflectometry. Water Resour. Res. 1983, 19, 1573–1584. [Google Scholar] [CrossRef]
- Spaans, E.J.A.; Baker, J.M. The soil freezing characteristics: Its measurement and similarity to the soil moisture characteristic. Soil Sci. Soc. Am. J. 1996, 60, 13–19. [Google Scholar] [CrossRef]
- Starostin, E.G.; Petrov, E.E. Crystallization kinetic studies of unfrozen water contents in soils. Nauka i Obraz. 2013, 1, 19–23. [Google Scholar]
- Yershov, E.D.; Gurov, V.V.; Kuchukov, E.Z.; Komarov, I.A. Sublimation as a method for studying the properties of ice and permafrost. Merzlotnye Issled. 1976, 15, 243–247. [Google Scholar]
- Cheverev, V.G.; Vidyapin IYu Motenko, R.G.; Kondakov, M.V. Determination of unfrozen water content in soils by sorption-desorption isotherms. Kriosf. Zemli 2005, IX, 29–33. [Google Scholar]
- Istomin, V.A.; Chuvilin, E.M.; Makhonina, N.A.; Bukhanov, B.A. A method for calculating the unfrozen water curve from moisture potential. In Proceedings of the International Conference Cryogenic Resources of the Polar and Mountainous Regions: State of the Art and Prospects of Permafrost Engineering, Tyumen, Russia, 21–24 April 2008; pp. 398–401. (In Russian). [Google Scholar]
- Istomin, V.A.; Chuvilin, E.M.; Bukhanov, B.A. Fast estimation of unfrozen water content in frozen soils. Earth’s Cryosphere 2017, XXI, 134–139. [Google Scholar]
- Istomin, V.; Chuvilin, E.; Bukhanov, B.; Uchida, T. Pore water content in equilibrium with ice or gas hydrate in sediments. Cold Reg. Sci. Technol. 2017, 137, 60–67. [Google Scholar] [CrossRef]
- Chuvilin, E.M.; Sokolova, N.S.; Bukhanov, B.A.; Shevchik, F.A.; Istomin, V.A.; Mukhametdinova, A.Z.; Alekseev, A.G.; Grechishcheva, E.S. The water potential method for determination of unfrozen water contents in frozen soils of different compositions. Earth’s Cryosphere 2020, XXIV, 16–28. [Google Scholar]
- Chuvilin, E.M.; Bukhanov, B.A.; Mukhametdinova, A.Z.; Grechishcheva, E.S.; Sokolova, N.S.; Alekseev, A.G.; Istomin, V.A. Freezing point and unfrozen water contents of permafrost soils: Estimation by the water potential method. Cold Reg. Sci. Technol. 2022, 196, 103488. [Google Scholar] [CrossRef]
- Campbell, G.S.; Smith, D.M.; Teare, B.L. Application of a Dew Point Method to obtain the soil water characteristic. In Experimental Unsaturated Soil Mechanics; Springer Proceedings in Physics; Springer: Berlin/Heidelberg, Germany, 2007; Volume 112, pp. 71–77. [Google Scholar]
- Istomin, V.A.; Chuvilin, E.M.; Bukhanov, B.A. A Method for Determination of Unfrozen Water Content in Soils. Russian Federation. Patent No. 2654832, 22 May 2018. (In Russian). [Google Scholar]
- Chuvilin, E.M.; Sokolova, N.S.; Bukhanov, B.A.; Istomin, V.A.; Mingareeva, G.R. Determination of the freezing point of soils based on measurements of pore water potential. Earth’s Cryosphere 2020, XXIV, 9–16. [Google Scholar] [CrossRef]
- Aleksyutina, D.M.; Motenko, R.G. Effect of soil salinity and organic matter content on thermal properties and unfrozen water content of frozen soils in the western coast of Baydara Bay. Bull. Mosc. Univ. 2016, 4, 59–63. [Google Scholar] [CrossRef]
- ASTM D 2487–06; Standard Practice for Classification of Soils for Engineering Purposes (Unified Soil Classification System). ASTM: West Conshohocken, PA, USA, 2010; 12p.
- Roman, L.T.; Tsarapov, M.N.; Kotov, P.I.; Volokhov, S.S.; Motenko, R.G.; Cherkasov, A.M.; Shteyn, A.I.; Kostousov, A.I. A Guide for Estimation of Mechanic Properties of Freezing, Frozen and Thawing Fine-Grained Soils; Knizhnyi Dom Universitet: Moscow, Russia, 2018; 188p. (In Russian) [Google Scholar]
- Vasiliev, A.A.; Gravis, A.G.; Gubarkov, A.A.; Drozdov, D.S.; Korostelev, Y.V.; Malkova, G.V.; Oblogov, G.E.; Ponomareva, O.E.; Sadurtdinov, M.R.; Streletskaya, I.D.; et al. Permafrost degradation: Results of the long-term geocryological monitoring in the Western sector of Russian Arctic. Earth’s Cryosphere 2020, XXIV, 14–26. [Google Scholar] [CrossRef]
- Osipov, V.; Aksyutin, O.; Sergeev, D.; Tipenko, G.; Ishkov, A. Using the data of geocryological monitoring and geocryological forecast for risk assessment and adaptation to climate change. Energies 2022, 15, 879. [Google Scholar] [CrossRef]
- Chuvilin, E.M.; Perlova, E.V.; Baranov, Y.B.; Kondakov, V.V.; Osokin, A.B.; Yakushev, V.S. Structure and Properties of Permafrost in the Southern Bovanenkovo Gas-Condensate Field; GEOS: Moscow, Russia, 2007; 137p. (In Russian) [Google Scholar]



| Experiment | Combined (Experiments + Modeling) | Modeling Using Empirical Relationships with Other Soil Variables |
|---|---|---|
| Cryoscopy [15] | Calorimetry [53,54,55,56,57] | Plasticity, temperature, and salinity [39,40,53] |
| Nuclear Magnetic Resonance (NMR) [58,59,60,61,62,63] | Total moisture and grain size [50] | |
| Hygroscopic absorption [64] | Time domain reflectometry (TDR) [65,66,67,68,69,70] | Pore pressure [41] |
| Thermometry [35,71] | Specific surface area [42,43,44] | |
| Sublimation [72] | Desorption [27,73] | Total moisture and heat capacity [46] |
| Water potential [74,75,76,77,78] | Total moisture, ground temperature, and freezing point [47,48] | |
| Contact [19] | Freezing point and unfreezable water content [49] |
| Sample | Soil Type * | Salinity, % | Organic Matter Content **, % |
|---|---|---|---|
| 1 | Lean clay (CL) | 0.05 | 0.4 |
| 2 | Sandy silty clay (CL-ML) | 0.04 | 2.7 |
| 3 | Lean clay (CL) | 0.06 | 3.1 |
| 4 | Silt (ML) | 0.06 | 6.2 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chuvilin, E.; Sokolova, N.; Bukhanov, B. Changes in Unfrozen Water Contents in Warming Permafrost Soils. Geosciences 2022, 12, 253. https://doi.org/10.3390/geosciences12060253
Chuvilin E, Sokolova N, Bukhanov B. Changes in Unfrozen Water Contents in Warming Permafrost Soils. Geosciences. 2022; 12(6):253. https://doi.org/10.3390/geosciences12060253
Chicago/Turabian StyleChuvilin, Evgeny, Natalia Sokolova, and Boris Bukhanov. 2022. "Changes in Unfrozen Water Contents in Warming Permafrost Soils" Geosciences 12, no. 6: 253. https://doi.org/10.3390/geosciences12060253
APA StyleChuvilin, E., Sokolova, N., & Bukhanov, B. (2022). Changes in Unfrozen Water Contents in Warming Permafrost Soils. Geosciences, 12(6), 253. https://doi.org/10.3390/geosciences12060253







