Carbon Storage Potential of North American Oil & Gas Produced Water Injection with Surface Dissolution
Abstract
1. Introduction
2. Economic Analysis
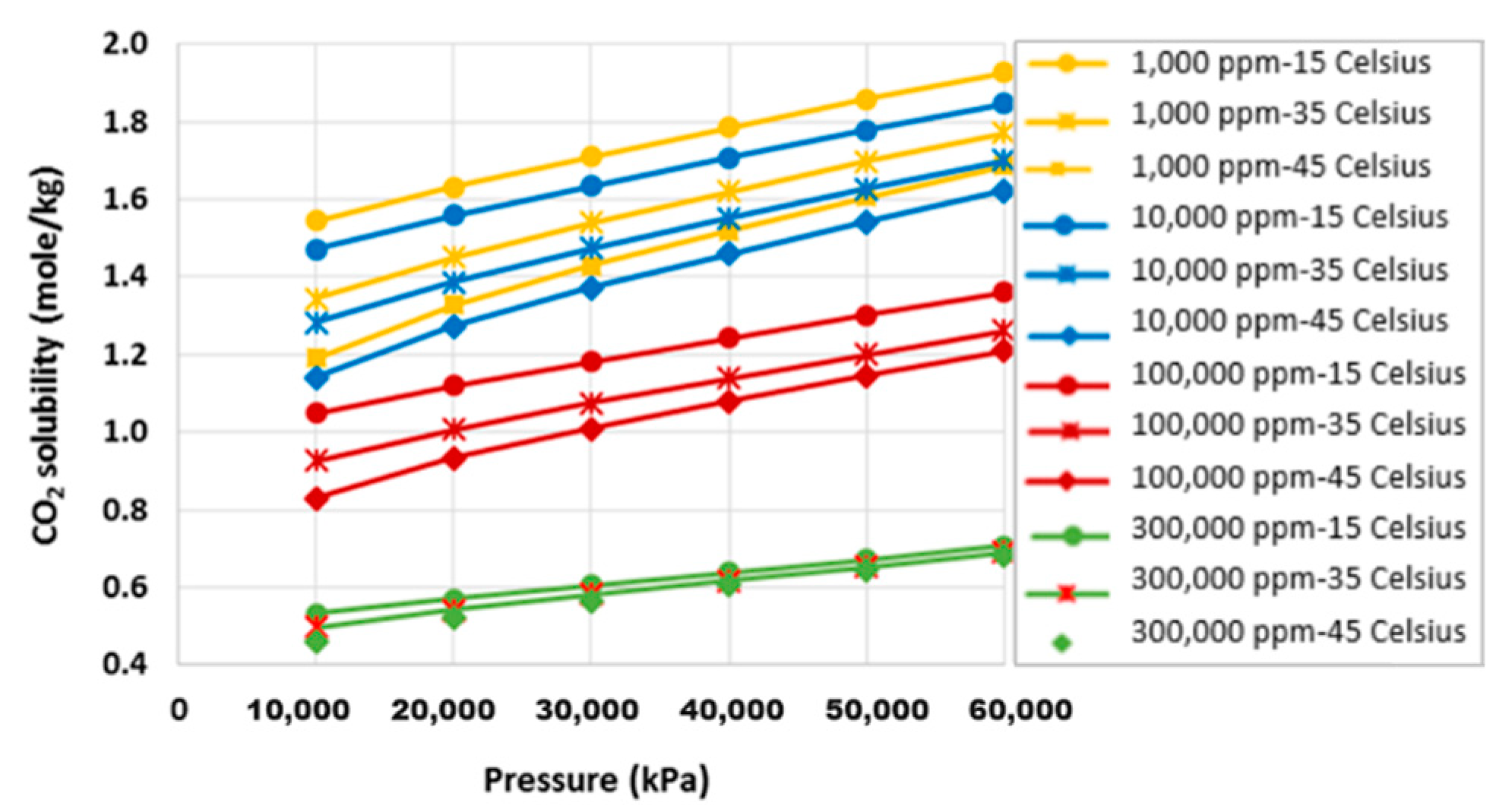
2.1. Surface Dissolution
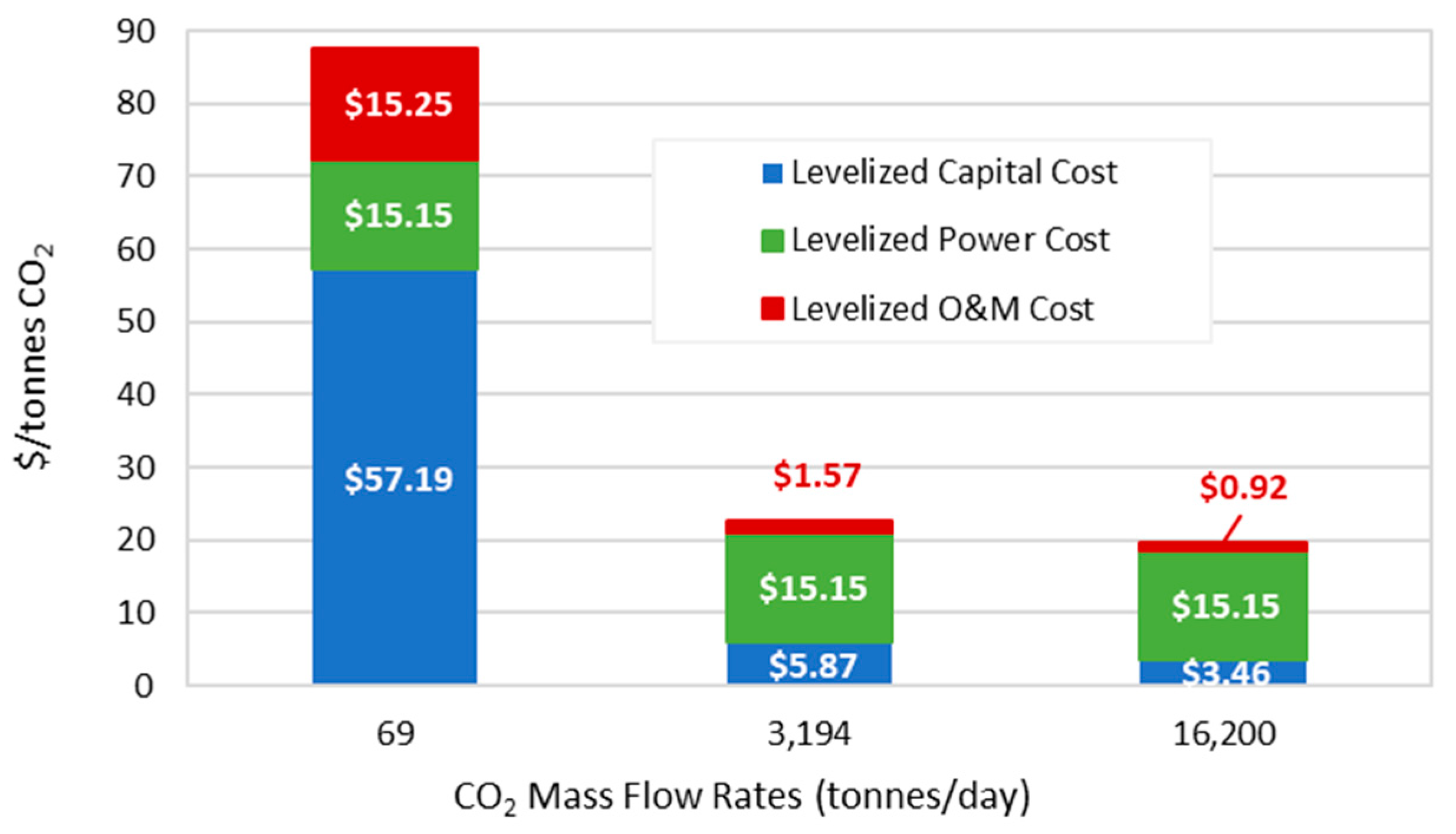
2.2. Compression Cost
2.3. Transportation Cost
3. Economic Analysis
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- IPCC. IPCC Special Report on Carbon Dioxide Capture and Storage. Prepared by Working Group III of the Intergovernmental Panel on Climate Change; Cambridge University Press: Cambridge, UK; New York, NY, USA, 2005; 442p. [Google Scholar]
- Hitchon, B.; Gunter, W.; Gentzis, T. The Serendipitous Association of Sedimentary Basins and Greenhouse Gases; American Chemical Society, Division of Fuel Chemistry: Washington, DC, USA, 1996; Volume 41, pp. 1428–1432. [Google Scholar]
- Khan, C.; Ge, L.; Rudolph, V. Reservoir Simulation Study for CO2 Sequestration in Saline Aquifers. Int. J. Appl. Sci. Technol. 2015, 5, 30–45. [Google Scholar]
- U.S. Geological Survey Geologic Carbon Dioxide Storage Resources Assessment Team. 2013a, National Assessment of Geologic Carbon Dioxide Storage Resources—Data: U.S. Geological Survey Data Series 774, 13 p., Plus 2 Appendixes and 2 Large Tables in Separate Files. Available online: http://pubs.usgs.gov/ds/774/ (accessed on 15 March 2018).
- Prevost, H.; Fuller, R.; Altevogt, A.; Bruant, R.; Scherer, G. Numerical modelling of carbon dioxide injection and transport in deep saline aquifers. Greenh. Gas Control Technol. 2005, 11, 2189–2193. [Google Scholar]
- Bennion, B.; Bachu, S. Supercritical CO2 and H2S—Brine drainage and imbibition relative permeability relationships for intercrystallite sandstone and carbonate formations. In Proceedings of the SPE Europec/EAGE Annual Conference and Exhibition, Vienna, Austria, 12–15 June 2006. SPE 99326. [Google Scholar]
- Benson, S.; Pini, R.; Calvo, R.; Niemi, A. Relative Permeability for Multi-Phase Flow in CO2 Storage Reservoirs. Part II: Resolving Fundamental Issues and Filling Data Gaps; Global CCS Institute: Melbourne, Australia; Stanford University: Stanford, CA, USA, 2015. [Google Scholar]
- Benson, S.; Pini, R.; Reynolds, C.; Krevor, M. Relative Permeability Analysis to Describe Multiphase Flow in CO2 Storage Reservoirs; Report No.2; Global CCS Institute: Melbourne, Australia; Stanford University: Stanford, CA, USA, 2013. [Google Scholar]
- Gaspar, A.; Lima, G.; Suslick, S. CO2 capture and storage in mature oil reservoir: Physical description, EOR and economic evaluation of a case of a Brazilian mature field. In Proceedings of the SPE Europe/EAGE Annual Conference, Madrid, Spain, 13–16 June 2005. SPE 94181. [Google Scholar]
- Bachu, S. CO2 storage in geological media: Role, means, status and barriers to deployment. Prog. Energy Combust. Sci. 2008, 34, 254–273. [Google Scholar] [CrossRef]
- Burton, M.; Bryant, S. Eliminating buoyant migration of sequestered CO2 through surface dissolution: Implementation costs and technical challenges. SPE Reserv. Eval. Eng. 2009, 12, 399–407. [Google Scholar] [CrossRef]
- Eke, P.; Naylor, M.; Haszeldine, S.; Curtis, A. CO2-Brine surface dissolution and injection: CO2 storage enhancement. In Proceedings of the SPE Offshore Europe Oil and Gas Conference and Exhibition, Aberdeen, UK, 8–11 September 2009. SPE 124700. [Google Scholar]
- Tao, Q.; Bryant, S. Optimal control of injection/extraction wells for the surface dissolution CO2 storage strategy. In Proceedings of the Carbon Management Technology Conference, Orlando, FL, USA, 7–9 February 2012. SPE 151370. [Google Scholar]
- Nghiem, L.; Shrivastava, V.; Kohse, B. Modelling aqueous phase behavior and chemical reactions in compositional simulation. In Proceedings of the SPE Reservoir Simulation Symposium, Woodlands, TX, USA, 21–23 February 2011. SPE Paper 141417. [Google Scholar]
- Clark, C.; Veil, J. Produced Water Volumes and Management Practices in the United State; Report for U.S. Department of Energy, Office of Fossil Energy, National Energy Technology Laboratory: Washington, DC, USA, 2009. [Google Scholar]
- Veil, J. Produced Water Volumes and Management Practices in 2012; Report for the Groundwater Protection Council; Veil Environmental, LLC.: Annapolis, MD, USA, 2015. [Google Scholar]
- Fossil Water. Scoping Study: Produced Water Beneficial Re-Use–High TDS Waters; Report for PTAC; Government of Alberta: Edmonton, AB, Canada, 2007. [Google Scholar]
- Bachu, S.; Melnikb, A.; Bistrana, R. Approach to evaluating the CO2 storage capacity in Devonian deep saline aquifers for emissions from oil sands operations in the Athabasca area, Canada. Energy Procedia 2014, 63, 5093–5102. [Google Scholar] [CrossRef]
- Ghaderi, S.; Leonenko, Y. Reservoir modeling for Wabamun lake sequestration project. Collect. Energy Sci. Eng. 2017, 3, 98–114. [Google Scholar] [CrossRef]
- Zhao, H.; Fedkin, M.V.; Dilmore, R.M.; Lvov, S.N. Carbon dioxide solubility in aqueous solutions of sodium chloride at geological conditions: Experimental results at 323.15, 373.15, and 423.15 K and 150 bar and modeling up to 573.15 K and 2000 bar. Geochim. Cosmochim. Acta. 2015, 149, 165–189. [Google Scholar] [CrossRef]
- Mao, S.; Zhang, D.; Li, Y.; Liu, N. An improved model for calculating CO2 solubility in aqueous NaCl solutions and the application to CO2–H2O–NaCl fluid inclusions. Chem. Geol. 2013, 347, 43–58. [Google Scholar] [CrossRef]
- Duan, Z.; Sun, R. An improved model calculating CO2 solubility in pure water and aqueous NaCl solutions from 273 to 533 K and from 0 to 2000 bar. Chem. Geol. 2003, 193, 257–271. [Google Scholar] [CrossRef]
- Hangx, S. Behaviour of the CO2-H2O System and Preliminary Mineralisation Model and Experiments; HPT Laboratory, Department of Earth Sciences Utrecht University: Utrecht, The Netherlands, 2005. [Google Scholar]
- McCollum, D.; Ogden, J.M. Techno-Economic Models for Carbon Dioxide Compression, Transport, and Storage and Correlations for Estimating Carbon Dioxide Density and Viscosity; Institute of Transportation Studies, University of California: Davis, CA, USA, 2006. [Google Scholar]
- Dawson, G.; Biddle, D.; Farquhar, S.; Golding, S.; Jiang, X.; Keck, R.; Khan, C.; Law, A.; Li, Q.; Pearce, J.; et al. Geochemical and Geomechanical Testing of Near Wellbore CO2 Injectivity Improvement; ANLEC. Project 7-1110-0101; The University of Queensland: Brisbane, Australia, 2014. [Google Scholar]



| Field Name | Volumes m3/Year | Injection Wells |
|---|---|---|
| Alabama | 4,584,938 | 83 |
| Alaska | 10,095,110 | 64 |
| Arkansas | 16,845,018 | 640 |
| California | 74,288,290 | 970 |
| Colorado | 14,772,648 | 292 |
| Florida | 1,784,466 | 7 |
| Indiana | 1,714,328 | 208 |
| Kansas | 93,570,453 | 3523 |
| Louisiana | 102,238,847 | 3231 |
| Michigan | 11,924,047 | 710 |
| Mississippi | 12,407,716 | 494 |
| Montana | 6,741,390 | no data |
| Nebraska | 2,236,986 | 113 |
| Nevada | 565,538 | 10 |
| New Mexico | 45,449,739 | no data |
| North Dakota | 19,314,300 | 350 |
| South Dakota | 270,841 | 15 |
| Ohio | 1,688,193 | 190 |
| Oklahoma | 129,623,927 | 4021 |
| Pennsylvania | 503,198 | no data |
| Utah | 10,199,134 | 118 |
| West Virginia | 462,171 | 64 |
| Virginia | 385,327 | 4879 |
| Wyoming | 37,315,578 | 335 |
| Total | 598,982,186 | 20,317 |
| Field Name | Volume m3/Year | Injection Wells |
|---|---|---|
| Provost | 22,100,000 | 130 |
| Redwater | 20,900,000 | 50 |
| Hayter | 19,200,000 | 35 |
| Grand Forks | 16,700,000 | 63 |
| Bellshill Lake | 15,600,000 | 19 |
| Jenner | 14,400,000 | 19 |
| Bow Island | 13,500,000 | 27 |
| Bantry | 12,600,000 | 28 |
| Enchant | 12,200,000 | 22 |
| Killam | 11,600,000 | 13 |
| Rainbow | 10,800,000 | 16 |
| Taber North | 9,420,000 | 27 |
| Taber | 8,870,000 | 24 |
| Fenn-Big Valley | 8,340,000 | 18 |
| Hays | 5,970,000 | 14 |
| Lindbergh | 5,170,000 | 14 |
| Mitsue | 4,330,000 | 10 |
| Fort Saskatchewan | 3,400,000 | 3 |
| Sturgeon Lake | 377,000 | 8 |
| Total | 215,477,000 | 130 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Khan, C.; Pearce, J.K.; Golding, S.D.; Rudolph, V.; Underschultz, J.R. Carbon Storage Potential of North American Oil & Gas Produced Water Injection with Surface Dissolution. Geosciences 2021, 11, 123. https://doi.org/10.3390/geosciences11030123
Khan C, Pearce JK, Golding SD, Rudolph V, Underschultz JR. Carbon Storage Potential of North American Oil & Gas Produced Water Injection with Surface Dissolution. Geosciences. 2021; 11(3):123. https://doi.org/10.3390/geosciences11030123
Chicago/Turabian StyleKhan, Chawarwan, Julie K. Pearce, Suzanne D. Golding, Victor Rudolph, and Jim R. Underschultz. 2021. "Carbon Storage Potential of North American Oil & Gas Produced Water Injection with Surface Dissolution" Geosciences 11, no. 3: 123. https://doi.org/10.3390/geosciences11030123
APA StyleKhan, C., Pearce, J. K., Golding, S. D., Rudolph, V., & Underschultz, J. R. (2021). Carbon Storage Potential of North American Oil & Gas Produced Water Injection with Surface Dissolution. Geosciences, 11(3), 123. https://doi.org/10.3390/geosciences11030123








