Analytical and Numerical Investigations Applied to Study the Reflections and Transmissions of a Rectangular Breakwater Placed at the Bottom of a Wave Tank
Abstract
:1. Introduction
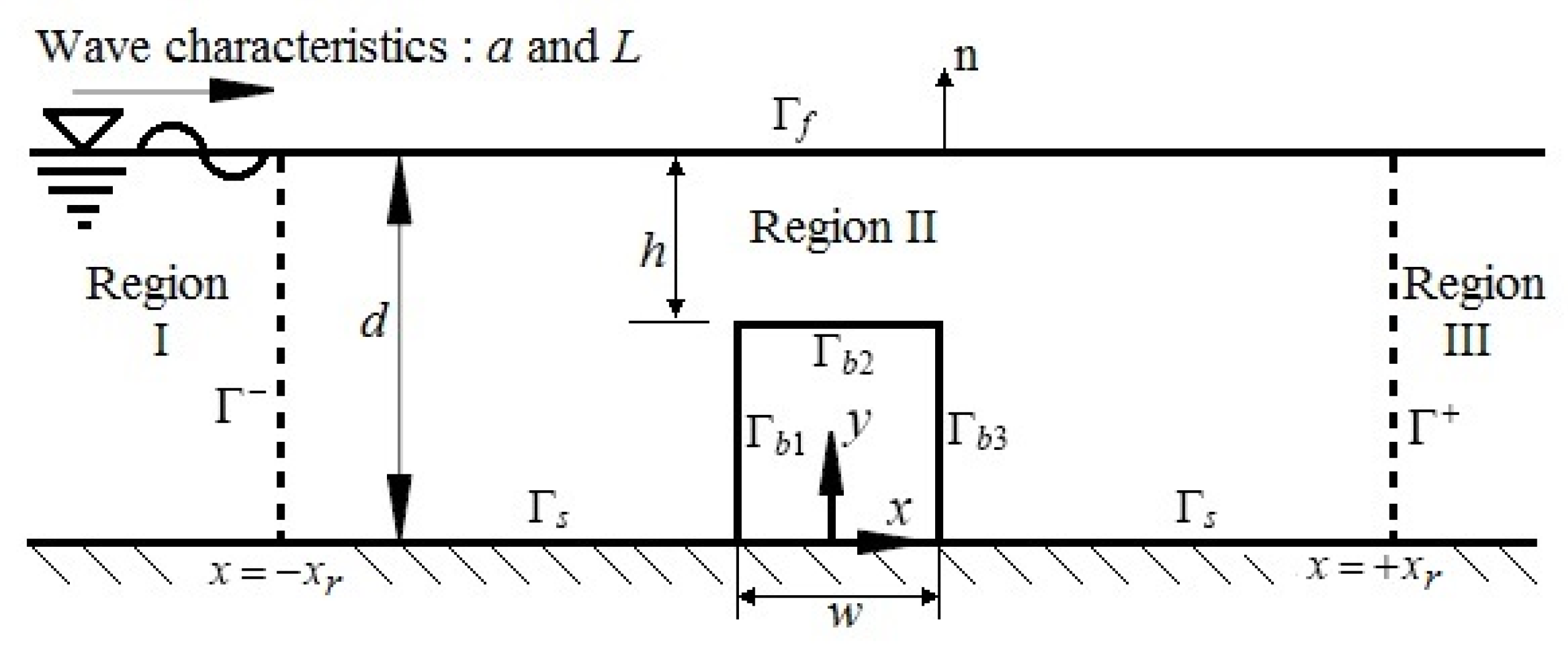
2. Formulation of the Problem
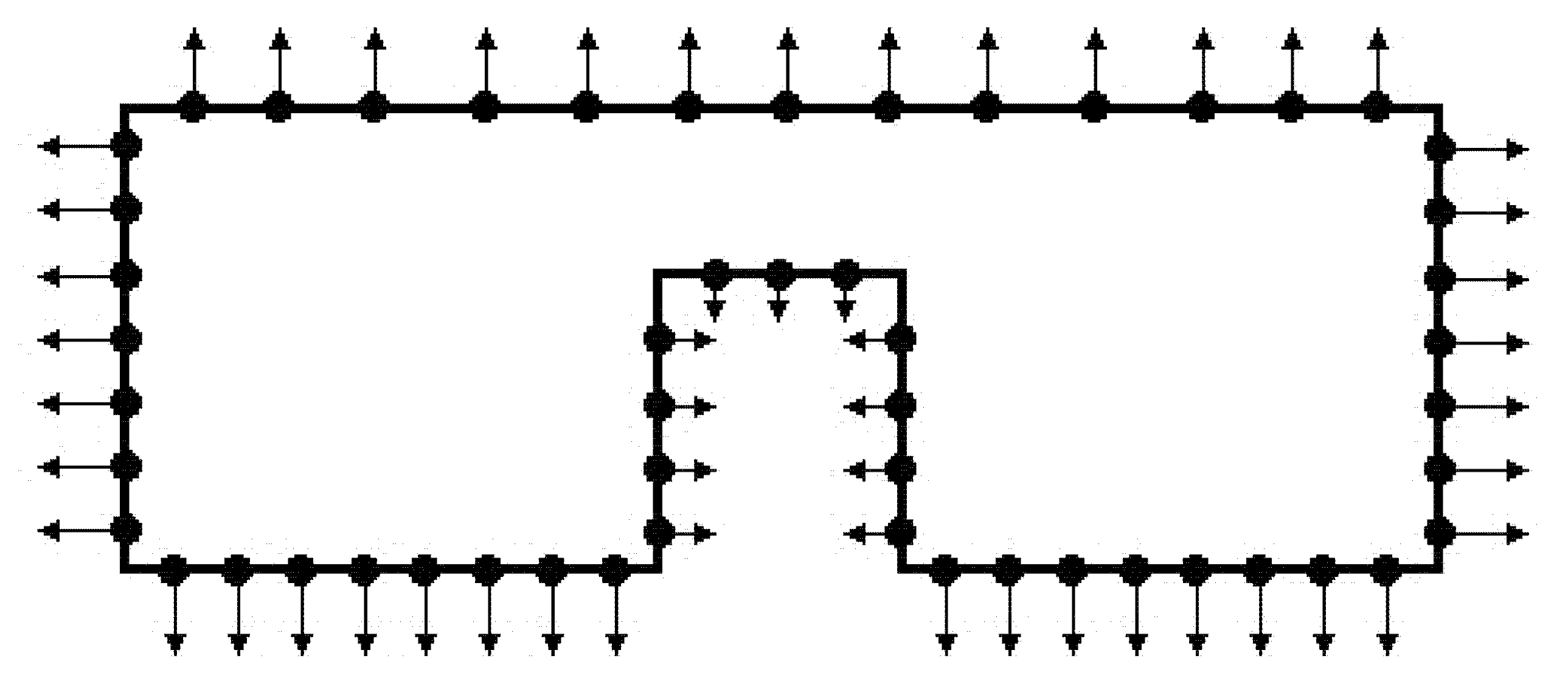
3. Numerical Solution by the ISBM
4. Results and Discussion
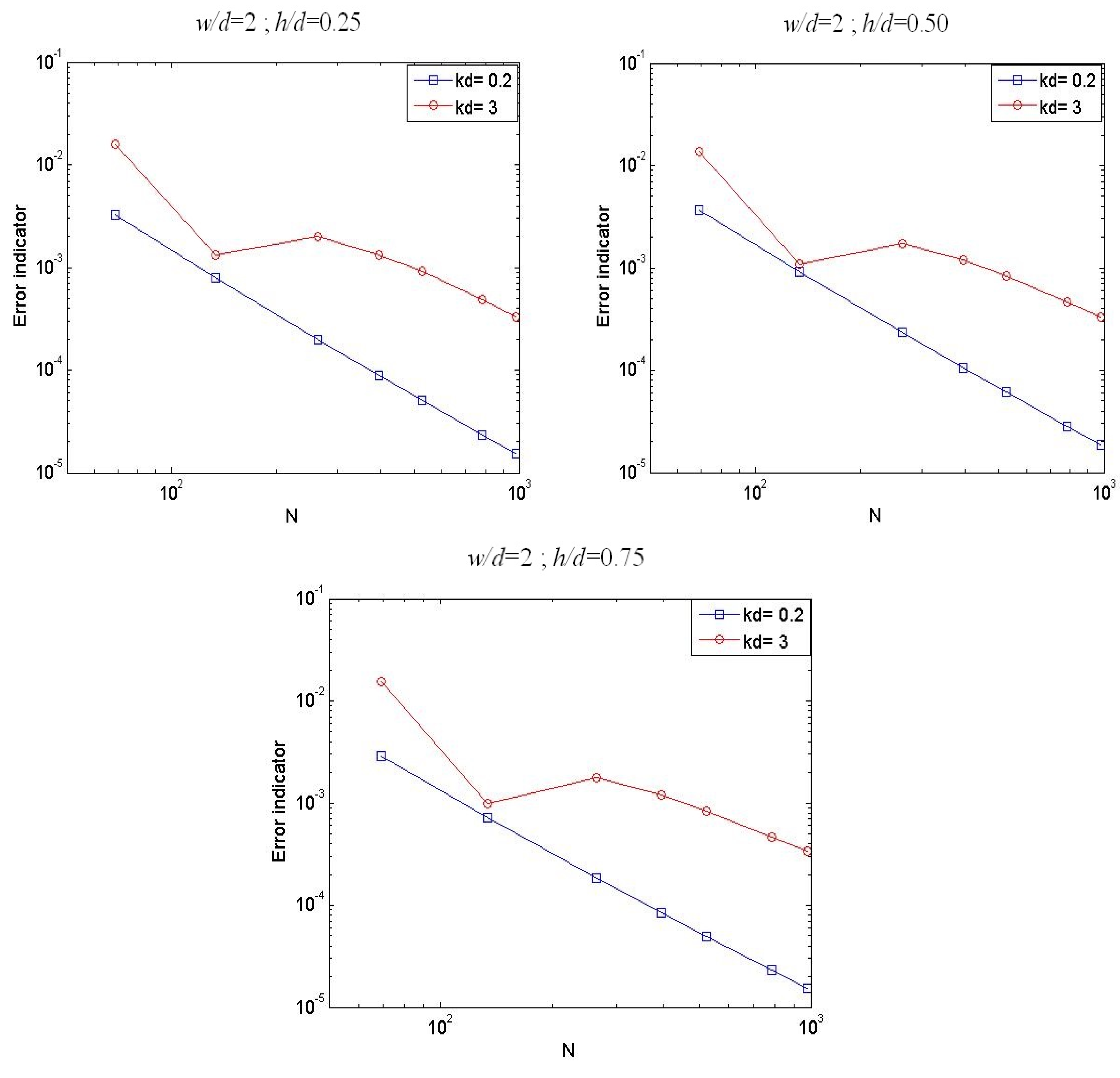
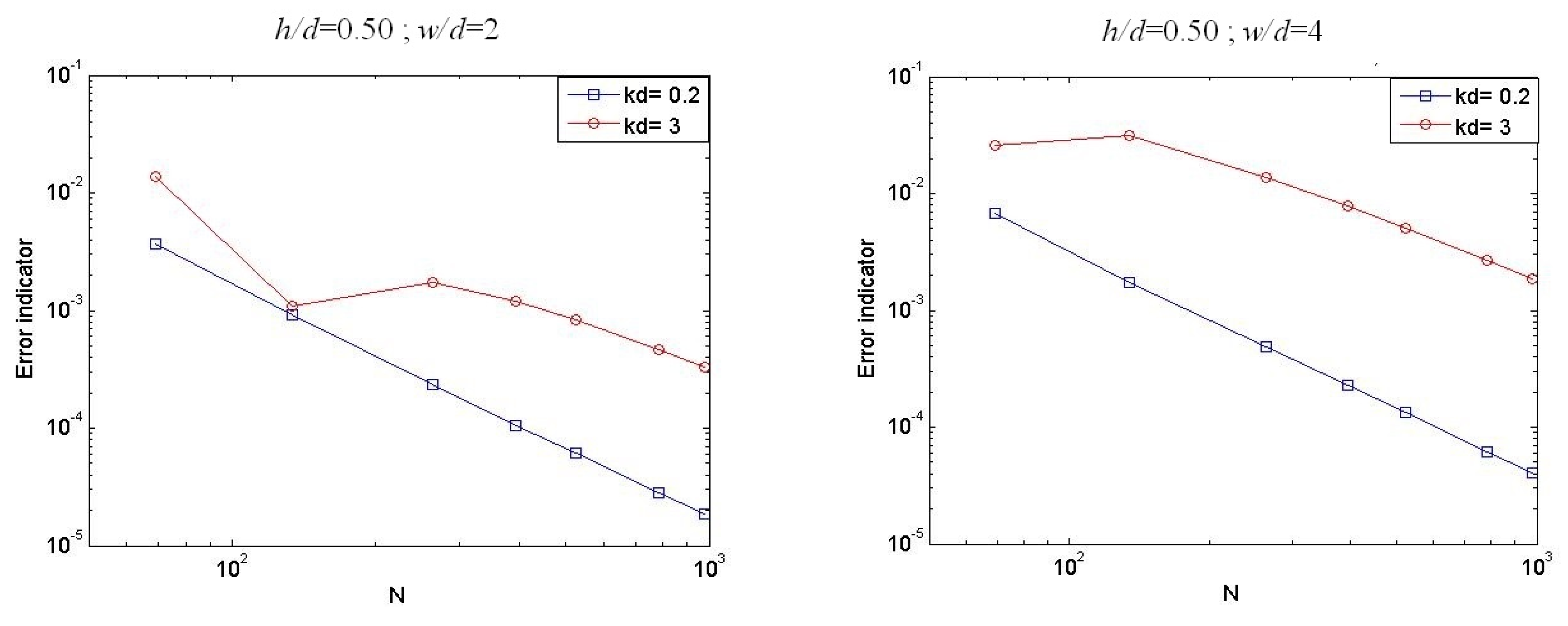
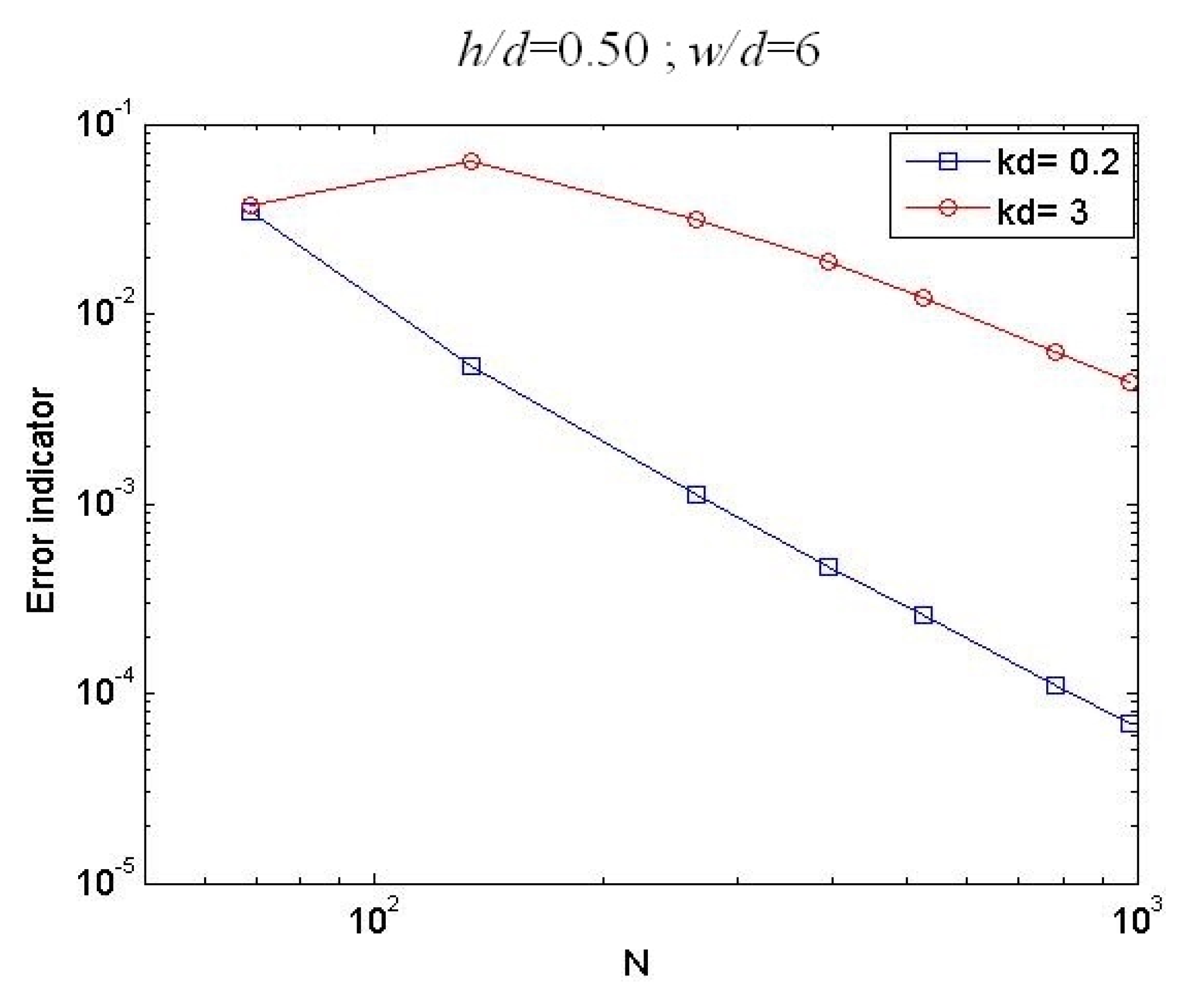
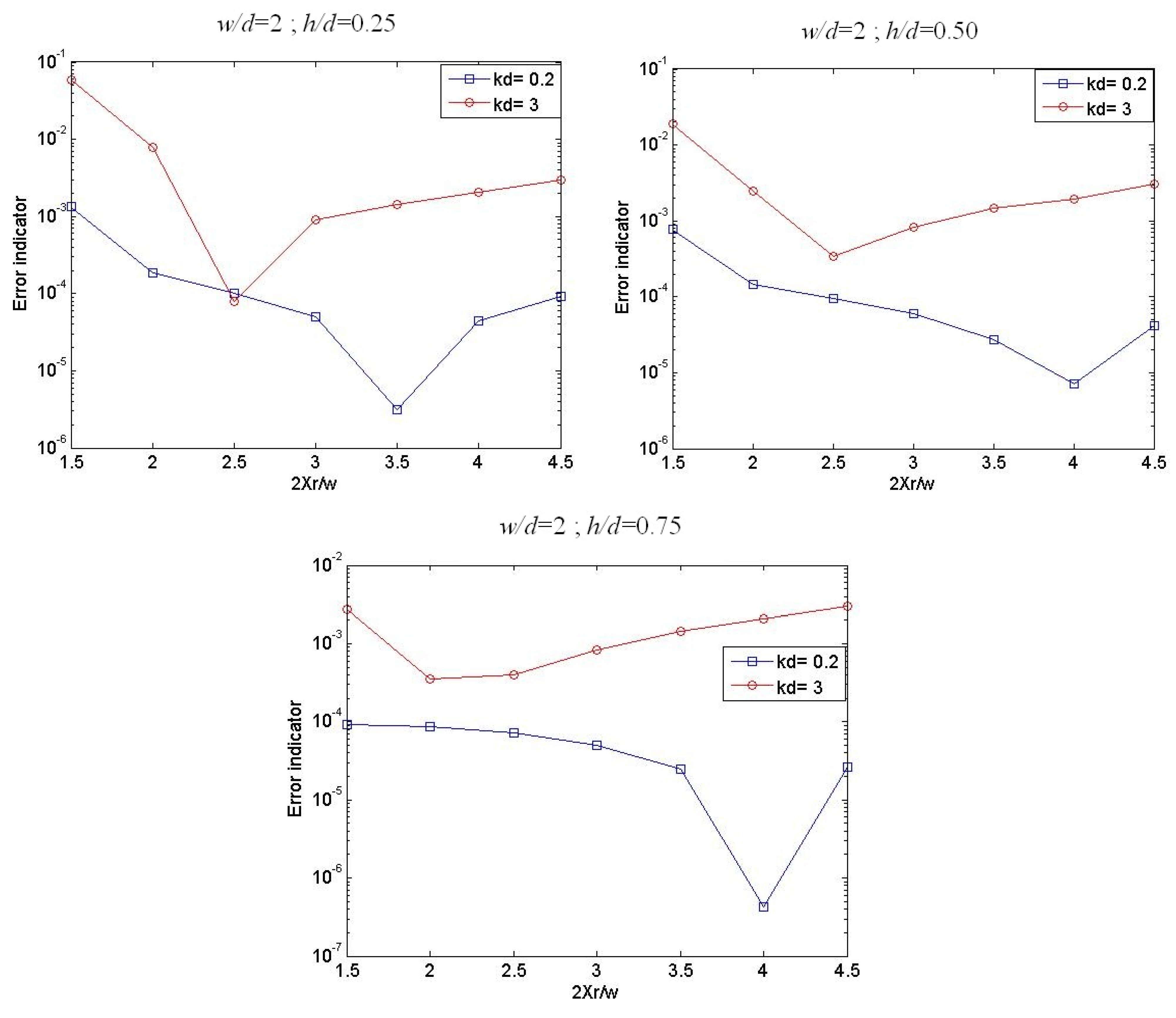
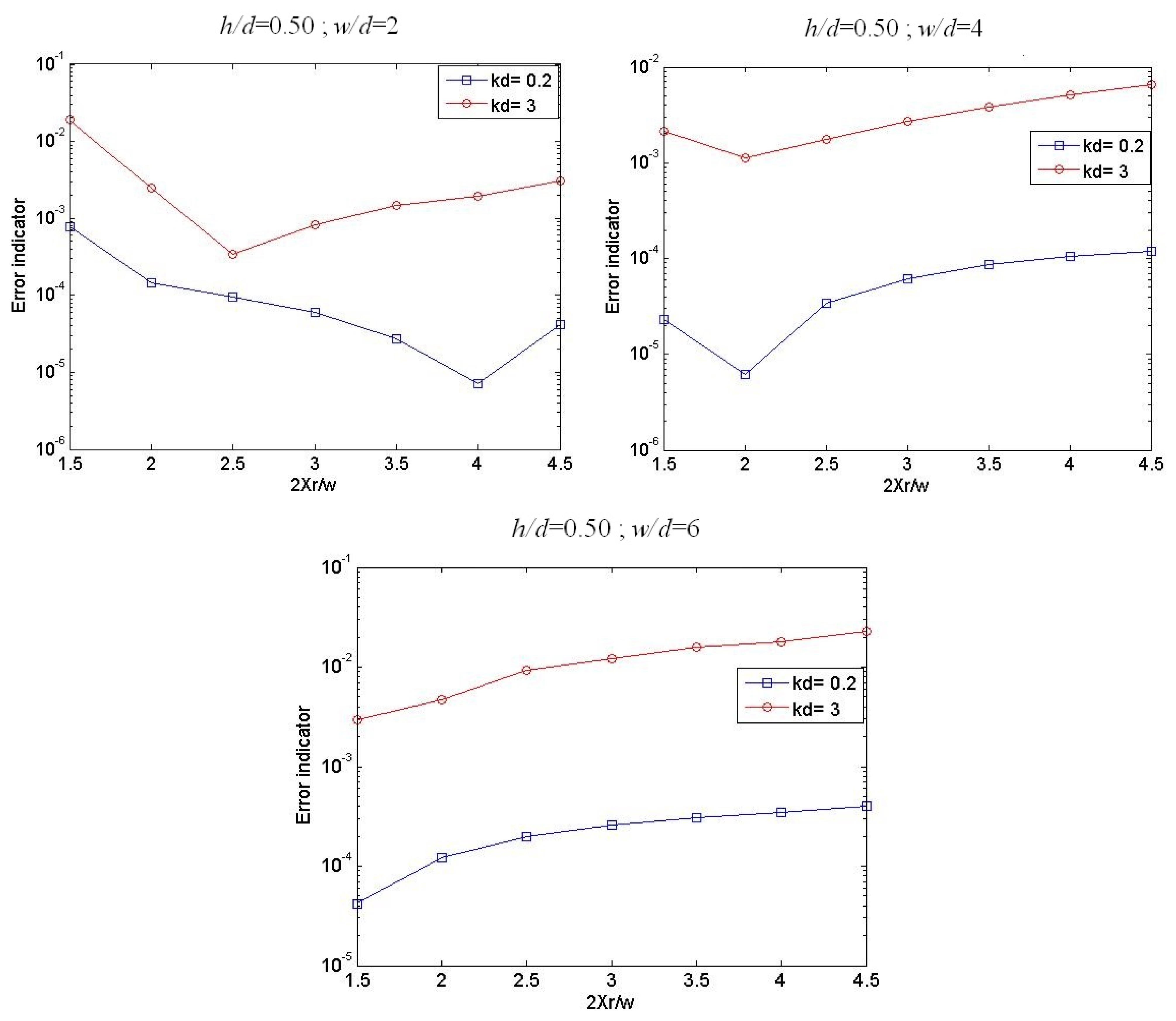
4.1. Error Indicators Analysis
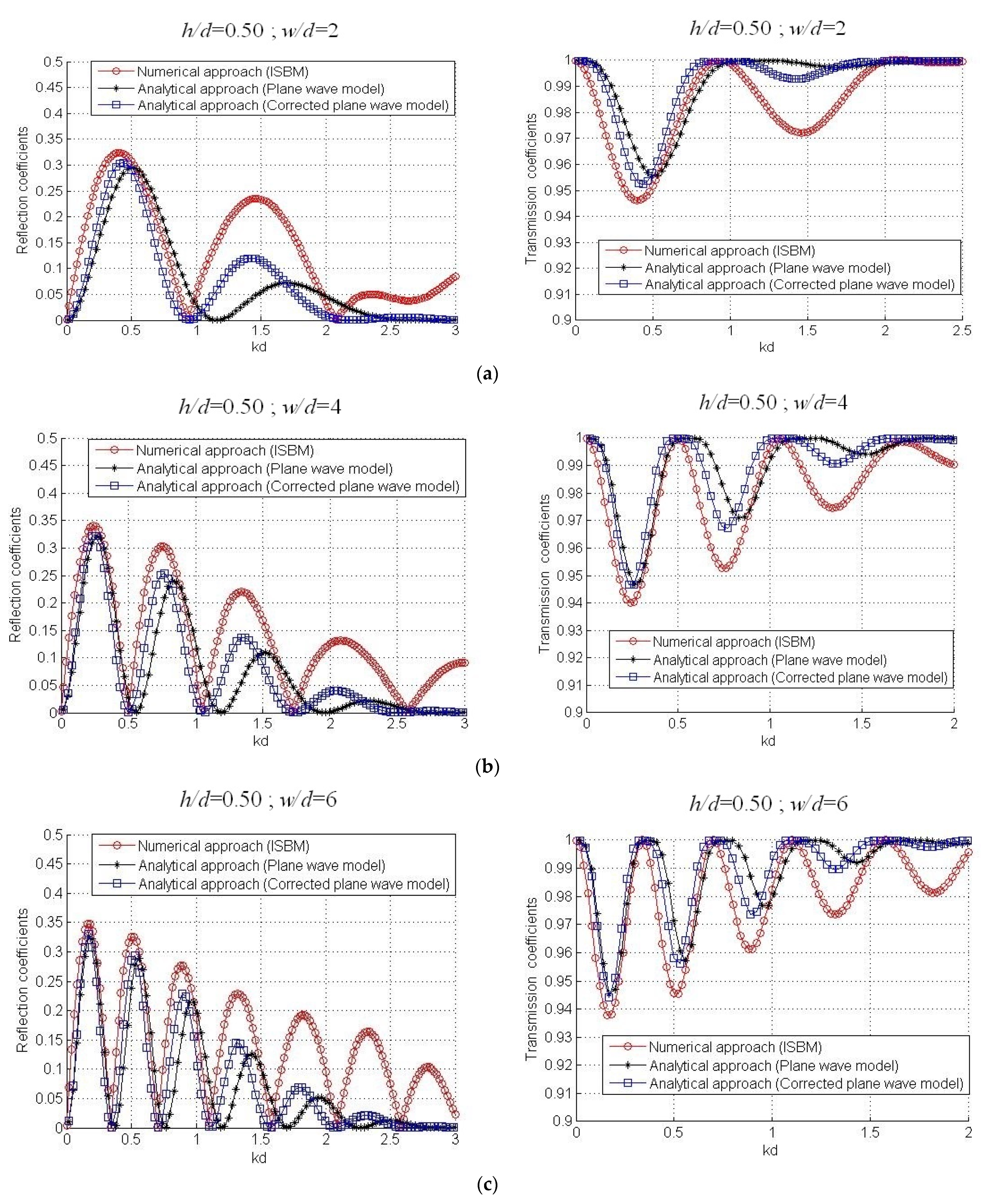
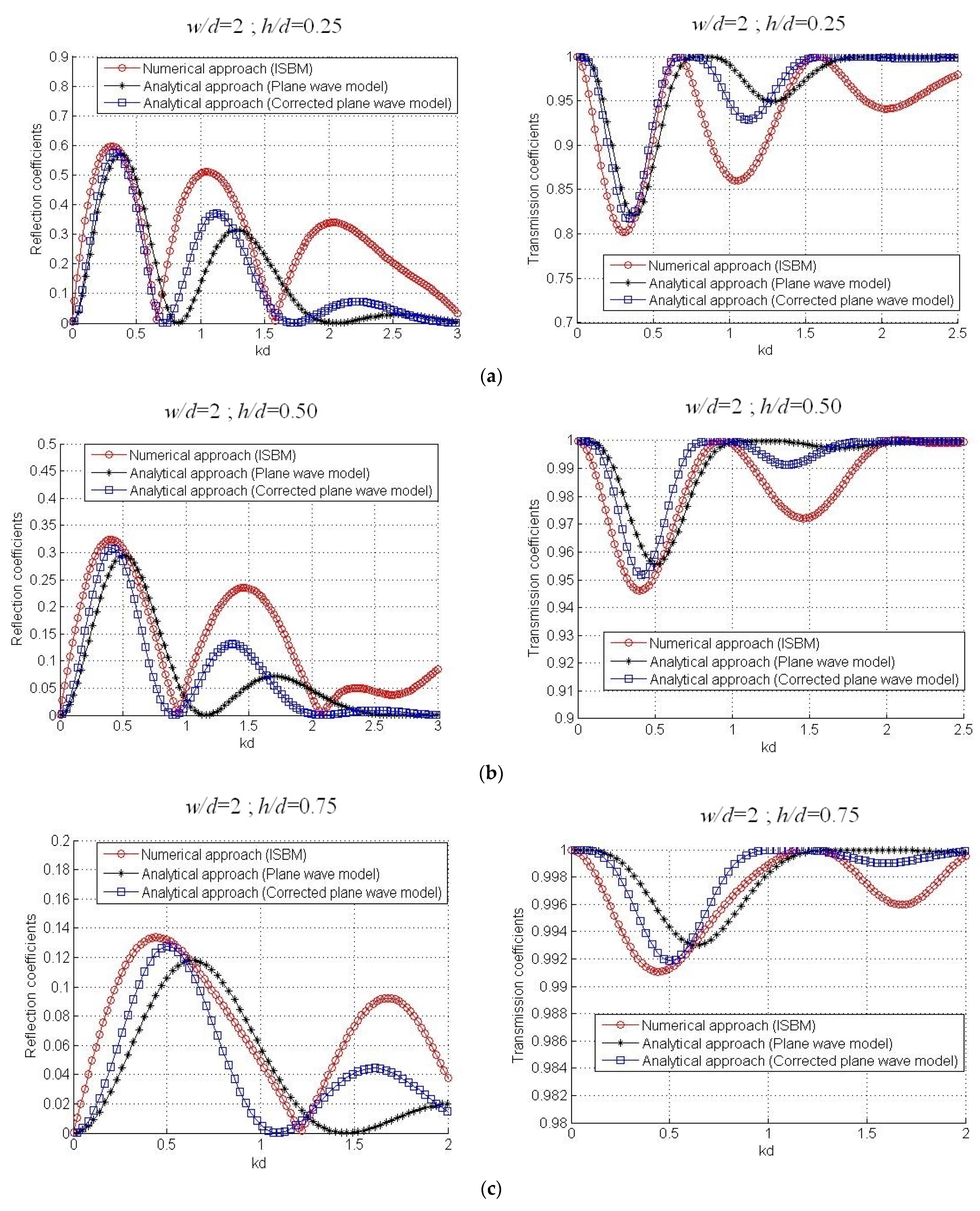
4.2. Reflection and Transmission Coefficients Analysis
5. Conclusions and Perspectives
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| The amplitude of the incident wave | |
| T | The period |
| R | The reflection coefficient |
| The transmission coefficient | |
| d | The water depth |
| h | The water depth above the obstacle |
| w | The length of the obstacle |
| h/d | The immersion ratio |
| w/d | The relative length |
| The wave number that verifies the dispersion relation | |
| The wave number above of the obstacle that verifies the dispersion relation |
Appendix A

- -
- at the subdomain
- -
- at the subdomain
- -
- at the subdomain
- At the position x =
- At the position x=after expressing the connection conditions at the positions x = and x = , we obtain a linear algebraic system aswhere:by combining the system of Equation (8), we obtain
Appendix B
References
- Dean, W.R. On the reflexion of surface waves by a submerged plane barrier. In Mathematical Proceedings of the Cambridge Philosophical Society; Cambridge University Press: Cambridge, UK, 1945; Volume 41, pp. 231–238. [Google Scholar]
- Takano, K. Effets d’un obstacle parallélépipédique sur la propagation de la houle. Houille Blanche 1960, 3, 247–267. [Google Scholar] [CrossRef] [Green Version]
- Patarapanich, M. Forces and moment on a horizontal plate due to wave scattering. Coast. Eng. 1984, 83, 279–301. [Google Scholar] [CrossRef]
- Liu, P.L.F.; Wu, J. Wave transmission through submerged apertures. J. Waterw. Port Coast. Ocean Eng. 1987, 113, 660–671. [Google Scholar] [CrossRef]
- Stamos, D.J.; Hajj, M.R.; Telionis, D.P. Performance of hemi-cylindrical and rectangular submerged breakwaters. Ocean Eng. 2003, 30, 813–828. [Google Scholar] [CrossRef]
- Molin, B.; Kimmoun., O.; Liu., Y.; Remy., F.; Bingham, H.B. Experimental and numerical study of the wave run-up along a vertical plate. J. Fluid Mech. 2010, 654, 363–386. [Google Scholar] [CrossRef]
- Liu, Y.; Li, H.J.; Zhu, L. Bragg reflection of water waves by multiple submerged semi-circular breakwaters. Appl. Ocean Res. 2016, 11, 67–78. [Google Scholar] [CrossRef]
- Scyphers, S.B.; Powers, S.P.; Heck, K.L. Ecological Value of Submerged Breakwaters for Habitat Enhancement on a Residential Scale. Environ. Manag. 2015, 55, 383–391. [Google Scholar] [CrossRef] [PubMed]
- Mei, C.; Black, J. Scattering of surface waves by rectangular obstacles in waters of finite depth. J. Fluid Mech. 1969, 38, 499–511. [Google Scholar] [CrossRef]
- Massel, S. Harmonic generation by waves propagating over a submerged step. Coast. Eng. 1983, 7, 357–380. [Google Scholar] [CrossRef]
- Driscoll, A.; Dalrymple, R.; Grilli, S. Harmonic generation and transmission past a submerged rectangular obstacle. Coast. Eng. 1992, 1142–1152. [Google Scholar] [CrossRef]
- Szmidt, K. Finite difference analysis of surface wave scattering by underwater rectangular obstacles. Arch. Hydro-Eng. Environ. Mech. 2010, 57, 179–198. [Google Scholar]
- Chen, W.; Gu, Y. An improved formulation of singular boundary method. Adv. Appl. Math. Mech. 2012, 4, 543–558. [Google Scholar] [CrossRef]
- Gu, Y.; Chen, W.; Zhang, J.Y. Investigation on near-boundary solutions by singular boundary method. Eng. Anal. Bound. Elem. 2012, 36, 1173–1182. [Google Scholar] [CrossRef]
- Gu, Y.; Chen, W. Infinite domain potential problems by a new formulation of singular boundary method. Appl. Math. Model. 2013, 37, 1638–1651. [Google Scholar] [CrossRef]
- Chioukh, N.; Çevik, E.; Yüksel, Y. Reflection and transmission of regular waves from/through single and double perforated thin walls. China Ocean Eng. 2017, 31, 466–475. [Google Scholar] [CrossRef]
- Bakhti, Y.; Chioukh, N.; Hamoudi, B.; Boukhari, M. A multi-domain boundary element method to analyze the reflection and transmission of oblique waves from double porous thin walls. J. Mar. Sci. Appl. 2017, 16, 276–285. [Google Scholar] [CrossRef]
- Yueh, C.Y.; Chuang, S.H. A boundary element model for a partially piston-type porous wave energy converter in gravity waves. Eng. Anal. Bound. Elem. 2012, 36, 658–664. [Google Scholar] [CrossRef]
- Brebbia, C.A.; Dominguez, J. Boundary Elements: An Introductory Course. In Computational Mechanics Publications; WIT Press: Southampton, UK; Boston, MA, USA, 1992. [Google Scholar]
- Gu, Y.; Chen, W. Recent advances in singular boundary method for ultra-thin structural problems. In Boundary Elements and Other Mesh Reduction Methods XXXVI; WIT Transactions on Modelling and Simulation: Southampton, UK; Boston, MA, USA, 2014; Volume 56, pp. 233–243. [Google Scholar]
- Senouci, F.; Chioukh, N.; Dris, M.E.A. Performance Evaluation of Bottom-Standing Submerged Breakwaters in Regular Waves Using the Meshless Singular Boundary Method. J. Ocean Univ. China 2019, 18, 823–833. [Google Scholar] [CrossRef]
- Loukili, M.; Dutykh, D.; Kotrasova, K.; Ning, D. Numerical Stability Investigations of the Method of Fundamental Solutions Applied to Wave-Current Interactions Using Generating-Absorbing Boundary Conditions. Symmetry 2021, 13, 1153. [Google Scholar] [CrossRef]
- Dingemans, M. Water Wave Propagation Over Uneven Bottoms. Adv. Ser. Ocean Eng. 1997, 13, 1–1016. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Loukili, M.; Dutykh, D.; Nadjib, C.; Ning, D.; Kotrasova, K. Analytical and Numerical Investigations Applied to Study the Reflections and Transmissions of a Rectangular Breakwater Placed at the Bottom of a Wave Tank. Geosciences 2021, 11, 430. https://doi.org/10.3390/geosciences11100430
Loukili M, Dutykh D, Nadjib C, Ning D, Kotrasova K. Analytical and Numerical Investigations Applied to Study the Reflections and Transmissions of a Rectangular Breakwater Placed at the Bottom of a Wave Tank. Geosciences. 2021; 11(10):430. https://doi.org/10.3390/geosciences11100430
Chicago/Turabian StyleLoukili, Mohammed, Denys Dutykh, Chioukh Nadjib, Dezhi Ning, and Kamila Kotrasova. 2021. "Analytical and Numerical Investigations Applied to Study the Reflections and Transmissions of a Rectangular Breakwater Placed at the Bottom of a Wave Tank" Geosciences 11, no. 10: 430. https://doi.org/10.3390/geosciences11100430
APA StyleLoukili, M., Dutykh, D., Nadjib, C., Ning, D., & Kotrasova, K. (2021). Analytical and Numerical Investigations Applied to Study the Reflections and Transmissions of a Rectangular Breakwater Placed at the Bottom of a Wave Tank. Geosciences, 11(10), 430. https://doi.org/10.3390/geosciences11100430









