Experimental Reproducibility and Natural Variability of Hydraulic Transport Properties of Fractured Sandstone Samples
Abstract
1. Introduction
2. Materials and Methods
2.1. Samples and Sample Preparation
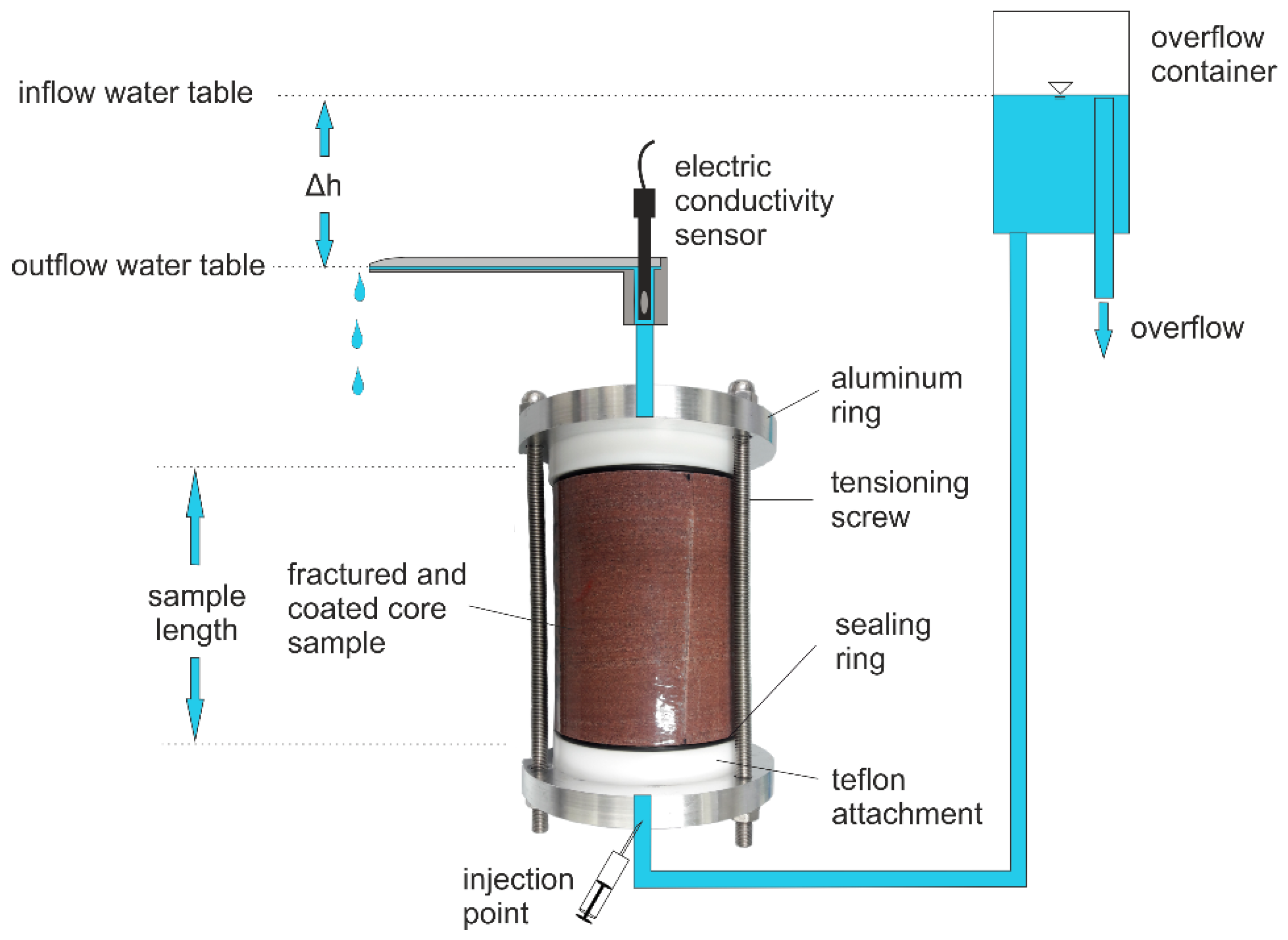
2.2. Experimental Setup and Procedure
2.3. Determination of Hydraulic Parameters
2.4. Determination of Transport Parameters
2.5. Characterization of Fracture Roughness
3. Results
3.1. Determination of Hydraulic Parameters
3.2. Determination of Transport Parameters Using CTRW
3.3. Determination of Transport Parameters Using ADE
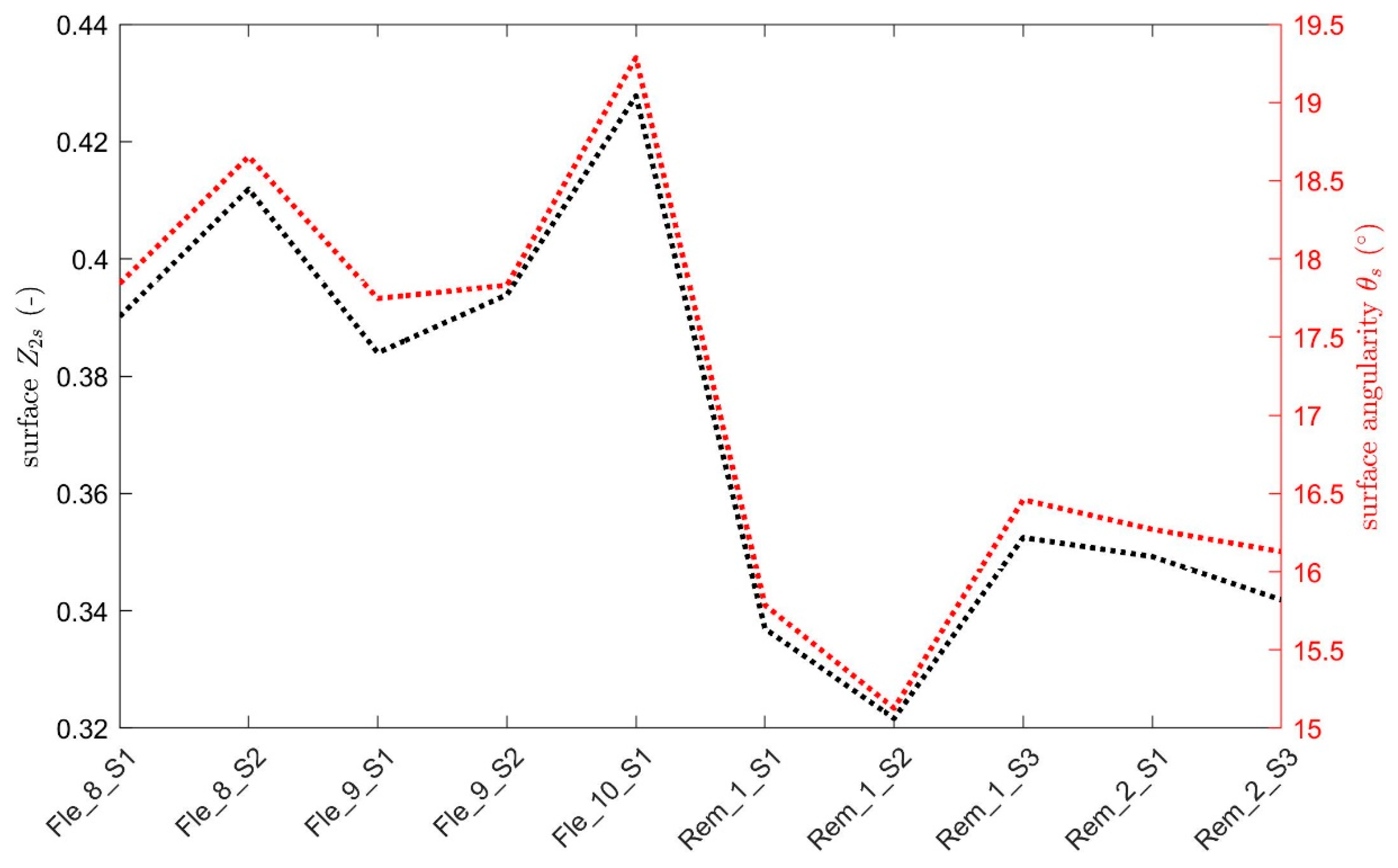
3.4. Comparison of Fracture Surface Morphology
4. Discussion
4.1. Dependence of Rock Type and Spatial Heterogeneity
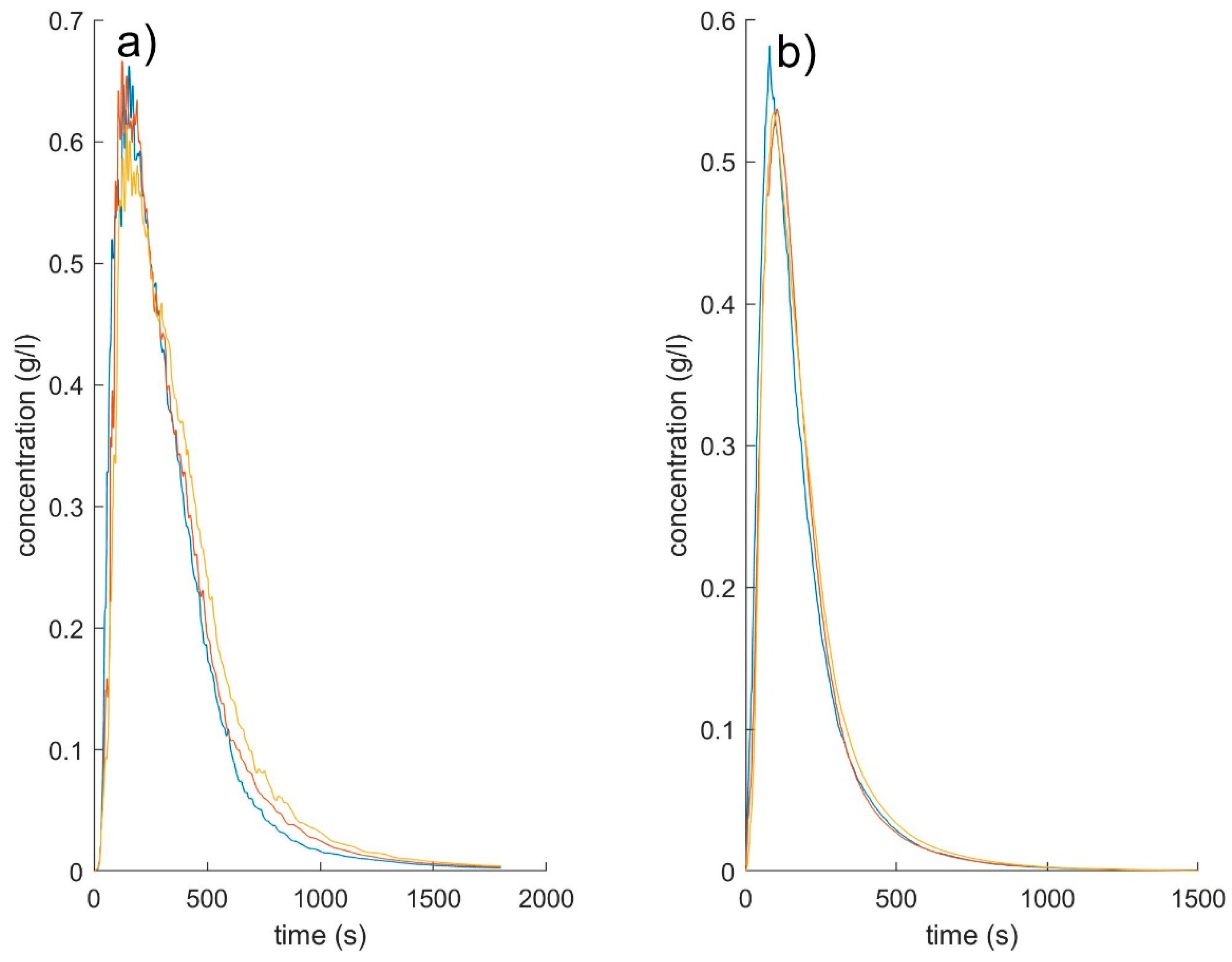
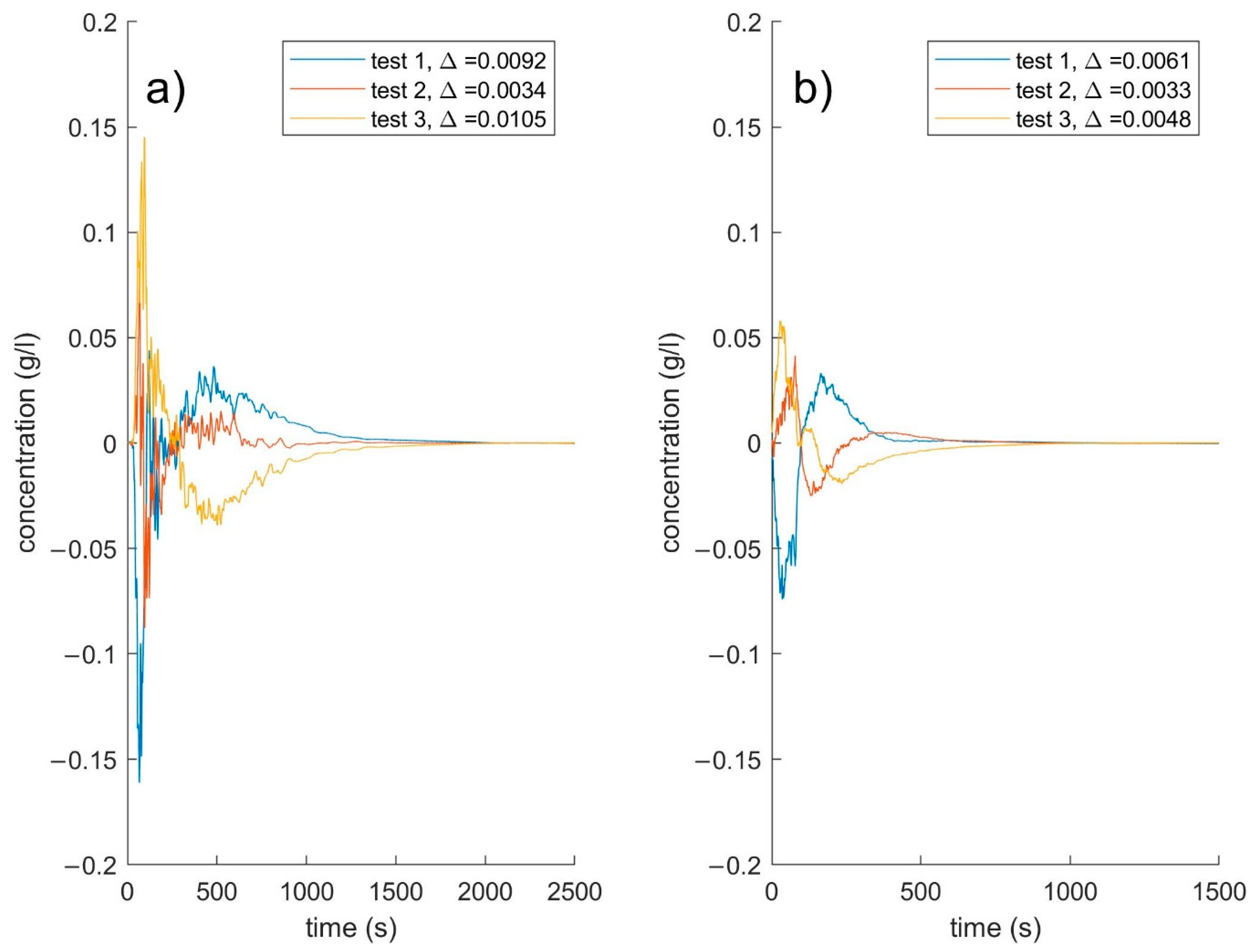
4.2. Reproducibility of Tracer Tests in a Single Rough Fracture
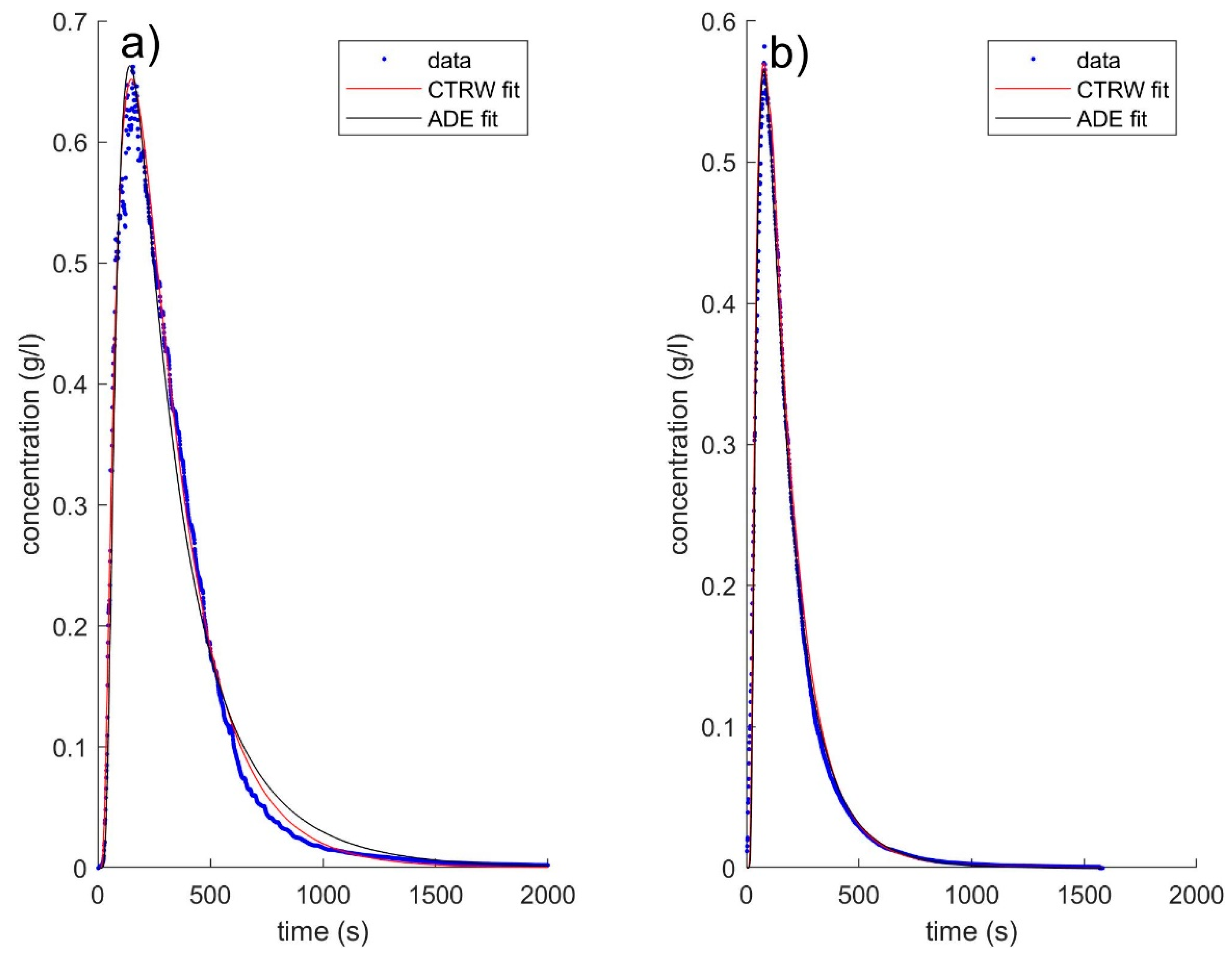
4.3. Comparison of Parameters Determined by CTRW and ADE
4.4. Natural Variability and Parameter Uncertainty in Fracture Flow Modeling
5. Conclusions
- (1)
- When the influence of surface roughness on the flow and transport behavior is significant, there are variations in the transport behavior between repeated measurements, which are beyond experimental control. This limits the experimental reproducibility of tracer tests.
- (2)
- Roughness dominated tracer transport is signaled by high frequency oscillations overlaying the BTCs. In principle, a rougher surface exhibits more of those oscillations, but usually a rougher surface also causes higher effective hydraulic apertures, canceling the effect of the increased roughness.
- (3)
- The effective aperture width correlates with fracture surface roughness and grain size. A smoother surface is observed at samples with smaller grain size, causing a smaller effective aperture width. The transport behavior is largely dominated by the effective aperture width, but dispersivity has no observable dependence on rock type or fracture surface roughness.
- (4)
- For numerical fracture models, fracture aperture width and dispersivity were identified as the most crucial parameters from which velocity and dispersion can be estimated. The smaller the effective aperture width is, the more dominant is the influence of surface roughness on the transport behavior.
- (5)
- The mean values of the transport parameters for the all Flechtinger and Remlinger sandstones are summarized in Table 6. Relatively, the variance of the transport parameters across the Flechtinger samples is around 30% to 50% of the mean values and is in general higher, up to 100%, for the Remlinger samples. However, the relative error of repeated measurements of the Remlinger samples is only around 10%, while it is around 10–30% for the Flechtinger samples (Table 2). The variability of the repeated measurements is therefore significantly smaller than the overall spread in parameters across the separate samples.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Dou, Z.; Sleep, B.; Zhan, H.; Zhou, Z.; Wang, J. Multiscale roughness influence on conservative solute transport in self-affine fractures. Int. J. Heat Mass Transf. 2019, 133, 606–618. [Google Scholar] [CrossRef]
- Briggs, S.; Karney, B.W.; Sleep, B.E. Numerical modeling of the effects of roughness on flow and eddy formation in fractures. J. Rock Mech. Geotech. Eng. 2017, 9, 105–115. [Google Scholar] [CrossRef]
- Chen, Z.; Zhan, H.; Zhao, G.; Huang, Y.; Tan, Y. Effect of Roughness on Conservative Solute Transport through Synthetic Rough Single Fractures. Water 2017, 9, 656. [Google Scholar] [CrossRef]
- Dou, Z.; Zhou, Z.F. Lattice Boltzmann simulation of solute transport in a single rough fracture. Water Sci. Eng. 2014, 7, 277–287. [Google Scholar]
- Bodin, J.; Delay, F.; de Marsily, G. Solute transport in a single fracture with negligible matrix permeability: 1. fundamental mechanisms. Hydrogeol. J. 2003, 11, 418–433. [Google Scholar] [CrossRef]
- Meller, C.; Bremer, J.; Baur, S.; Bergfeldt, T.; Blum, P.; Canic, T.; Eiche, E.; Gaucher, E.; Hagenmeyer, V.; Heberling, F. Integrated research as key to the development of a sustainable geothermal energy technology. Energy Technol. 2017, 5, 965–1006. [Google Scholar] [CrossRef]
- Keller, A.A.; Roberts, P.V.; Blunt, M.J. Effect of fracture aperture variations on the dispersion of contaminants. Water Resour. Res. 1999, 35, 55–63. [Google Scholar] [CrossRef]
- Zimmerman, R.W.; Yeo, I.W. Fluid flow in rock fractures: From the navier-stokes equations to the cubic law. Geophys. Monograph Ameri. Geophys. Union 2000, 122, 213–224. [Google Scholar]
- Kohl, T.; Evans, K.; Hopkirk, R.; Jung, R.; Rybach, L. Observation and simulation of non-darcian flow transients in fractured rock. Water Resour. Res. 1997, 33, 407–418. [Google Scholar] [CrossRef]
- Louis, C. Strömungsvorgänge in klüftigen Medien und ihre Wirkung auf die Standsicherheit von Bauwerken und Böschungen im Fels, 1st ed.; Veröffentlichungen des Institutes für Bodenmechanik und Felsmechanik der Univ. Karlsruhe: Karlsruhe, Germany, 1967; p. 167. (In German) [Google Scholar]
- Unger, A.J.A.; Mase, C.W. Numerical Study of the Hydromechanical Behavior of Two Rough Fracture Surfaces in Contact. Water Resour. Res. 1993, 29, 2101–2114. [Google Scholar] [CrossRef]
- Wang, M.; Chen, Y.F.; Ma, G.W.; Zhou, J.Q.; Zhou, C.B. Influence of surface roughness on nonlinear flow behaviors in 3d self-affine rough fractures: Lattice Boltzmann simulations. Adv. Water Resour. 2016, 96, 373–388. [Google Scholar] [CrossRef]
- Huber, F.; Enzmann, F.; Wenka, A.; Bouby, M.; Dentz, M.; Schäfer, T. Natural micro-scale heterogeneity induced solute and nanoparticle retardation in fractured crystalline rock. J. Contam. Hydrol. 2012, 133, 40–52. [Google Scholar] [CrossRef] [PubMed]
- Boutt, D.F.; Grasselli, G.; Fredrich, J.T.; Cook, B.K.; Williams, J.R. Trapping zones: The effect of fracture roughness on the directional anisotropy of fluid flow and colloid transport in a single fracture. Geophys. Res. Lett. 2006, 33, L21402. [Google Scholar] [CrossRef]
- Plouraboué, F.; Kurowski, P.; Boffa, J.M.; Hulin, J.P.; Roux, S. Experimental study of the transport properties of rough self-affine fractures. J. Contam. Hydrol. 2000, 46, 295–318. [Google Scholar] [CrossRef]
- Zambrano, M.; Pitts, A.D.; Salama, A.; Volatili, T.; Giorgioni, M.; Tondi, E. Analysis of fracture roughness control on permeability using sfm and fluid flow simulations: Implications for carbonate reservoir characterization. Geofluids 2019, 2019, 4132386. [Google Scholar] [CrossRef]
- Wang, L.; Bayani Cardenas, M. Transition from non-Fickian to Fickian longitudinal transport through 3-D rough fractures: Scale-(in)sensitivity and roughness dependence. J. Contam. Hydrol. 2017, 198, 1–10. [Google Scholar] [CrossRef]
- Tian, X.; Deng, Y.; Jing, D.; Peng, X.; Duan, M. Research on the influence of geometry on nonlinear flow in constructed rough fractures by lattice Boltzmann simulation. Arab. J. Geosci. 2020, 13, 1–18. [Google Scholar] [CrossRef]
- Zou, L.; Jing, L.; Cvetkovic, V. Modeling of solute transport in a 3d rough-walled fracture–matrix system. Transp. Porous Media 2017, 116, 1005–1029. [Google Scholar] [CrossRef]
- Singh, K.K.; Singh, D.N.; Gamage, R.P. Effect of sample size on the fluid flow through a single fractured granitoid. J. Rock Mech. Geotech. Eng. 2016, 8, 329–340. [Google Scholar] [CrossRef]
- Zambrano, M.; Tondi, E.; Mancini, L.; Lanzafame, G.; Trias, F.X.; Arzilli, F.; Materazzi, M.; Torrieri, S. Fluid flow simulation and permeability computation in deformed porous carbonate grainstones. Adv. Water Resour. 2018, 115, 95–111. [Google Scholar] [CrossRef]
- Gadelmawla, E.; Koura, M.; Maksoud, T.; Elewa, I.; Soliman, H. Roughness parameters. J. Mater. Process. Technol. 2002, 123, 133–145. [Google Scholar] [CrossRef]
- Bhushan, B. Surface roughness analysis and measurement techniques. In Modern Tribology Handbook: Volume One: Principles of Tribology, 1st ed.; Bhushan, B., Ed.; CRC Press: Washington, DC, USA, 2000. [Google Scholar]
- Tse, R.; Cruden, D.M. Estimating joint roughness coefficients. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1979, 16, 303–307. [Google Scholar] [CrossRef]
- Myers, N. Characterization of surface roughness. Wear 1962, 5, 182–189. [Google Scholar] [CrossRef]
- Belem, T.; Homand-Etienne, F.; Souley, M. Quantitative Parameters for Rock Joint Surface Roughness. Rock Mech. Rock Eng. 2000, 33, 217–242. [Google Scholar] [CrossRef]
- Grasselli, G.; Wirth, J.; Egger, P. Quantitative three-dimensional description of a rough surface and parameter evolution with shearing. Int. J. Rock Mech. Min. Sci. 2002, 39, 789–800. [Google Scholar] [CrossRef]
- Roux, S.; Plouraboué, F.; Hulin, J.P. Tracer dispersion in rough open cracks. Transp. Porous Media 1998, 32, 97–116. [Google Scholar] [CrossRef]
- Dou, Z.; Zhou, Z.; Wang, J.; Huang, Y. Roughness scale dependence of the relationship between tracer longitudinal dispersion and Peclet number in variable-aperture fractures. Hydrol. Proces. 2018, 32, 1461–1475. [Google Scholar] [CrossRef]
- Frank, S.; Heinze, T.; Wohnlich, S. Comparison of Surface Roughness and Transport Processes of Sawed, Split and Natural Sandstone Fractures. Water 2020, 12, 2530. [Google Scholar] [CrossRef]
- Tan, Y.; Lu, B.; Wu, T.; Wu, S.; Sha, H.; Zhou, P. Flow and solute transport through a single fracture during the biodegradation of residual toluene. J. Appl. Fluid Mech. 2017, 10, 1669–1677. [Google Scholar] [CrossRef]
- Chen, Z.; Qian, J.Z.; Qin, H. Experimental study of the non-darcy flow and solute transport in a channeled single fracture. J. Hydrodyn. 2011, 23, 745–751. [Google Scholar] [CrossRef]
- Neretnieks, I.; Eriksen, T.; Tahtinen, P. Tracer movement in a single fissure in granitic rock: Some experimental results and their interpretation. Water Resour. Res. 1982, 18, 849–858. [Google Scholar] [CrossRef]
- Yan, X.; Qian, J.; Ma, L.; Wang, M.; Hu, A. Non-Fickian solute transport in a single fracture of marble parallel plate. Geofluids 2018, 2018, 7418140. [Google Scholar] [CrossRef]
- Nowamooz, A.; Radilla, G.; Fourar, M.; Berkowitz, B. Non-fickian transport in transparent replicas of rough-walled rock fractures. Transp. Porous Media 2013, 98, 651–682. [Google Scholar] [CrossRef]
- Bauget, F.; Fourar, M. Non-fickian dispersion in a single fracture. J. Contam. Hydrol. 2008, 100, 137–148. [Google Scholar] [CrossRef]
- Jiménez-Hornero, F.; Giráldez, J.; Laguna, A.; Pachepsky, Y. Continuous time random walks for analyzing the transport of a passive tracer in a single fissure. Water Resour. Res. 2005, 41, W04009. [Google Scholar] [CrossRef]
- Noetinger, B.; Roubinet, D.; Russian, A.; Le Borgne, T.; Delay, F.; Dentz, M.; Gouze, P. Random walk methods for modeling hydrodynamic transport in porous and fractured media from pore to reservoir scale. Trans. Porous Media 2016, 115, 345–385. [Google Scholar] [CrossRef]
- Albinal, A.; Holy, R.; Sarak, H.; Ozkan, E. Modeling of 1D Anomalous Diffusion in Fractured Nanoporous Media. Oil Gas Sci. Technol. Rev. IFP Energ. Nouv. 2016, 71, 56. [Google Scholar] [CrossRef]
- Stoll, M.; Huber, F.; Trumm, M.; Enzmann, F.; Meinel, D.; Wenka, A.; Schill, E.; Schäfer, T. Experimental and numerical investigations on the effect of fracture geometry and fracture aperture distribution on flow and solute transport in natural fractures. J. Contam. Hydrol. 2019, 221, 82–97. [Google Scholar] [CrossRef]
- Tenchine, S.; Gouze, P. Density contrast effects on tracer dispersion in variable aperture fractures. Adv. Water Res. 2005, 28, 273–289. [Google Scholar] [CrossRef]
- Zimmerman, M.D.; Bennett, P.C.; Sharp, J.M., Jr.; Choi, W.J. Experimental determination of sorption in fractured flow systems. J. Contam. Hydrol. 2002, 58, 51–77. [Google Scholar] [CrossRef]
- Hassanzadegan, A.; Blöcher, G.; Zimmermann, G.; Milsch, H. Thermoporoelastic properties of Flechtinger sandstone. Int. J. Rock Mech. Min. 2012, 49, 94–104. [Google Scholar] [CrossRef]
- Heiland, J. Permeability of Triaxially Compressed Sandstone: Influence of Deformation and Strain-rate on permeability. Pure Appl. Geophys. 2003, 160, 889–908. [Google Scholar] [CrossRef]
- Stanchits, S.; Mayr, S.; Shapiro, S.; Dresen, G. Fracturing of porous rock induced by fluid injection. Tectonophysics 2011, 503, 94–104. [Google Scholar] [CrossRef]
- Blöcher, G.; Zimmermann, G.; Milsch, H. Impact of poroelastic response of sandstones on geothermal power production. Pure Appl. Geophys. 2009, 166, 1089–1106. [Google Scholar] [CrossRef]
- Tsang, Y.W. Usage of “Equivalent Apertures” for Rock Fractures as Derived from Hydraulic and Tracer Tests. Water Resour. Res. 1992, 28, 1451–1455. [Google Scholar] [CrossRef]
- Becker, T.A. Zur Strömungssimulation in Einzelklüften: Gegenüberstellung von Numerischen Methoden und Experiment; Dissertation; Rheinisch-Westfälische Technische Hochschule Aachen: Aachen, Germany, 2008. [Google Scholar]
- De Marsily, G. Quantitative Hydrogeology-Groundwater Hydrology for Engineers, 1st ed.; Academic Press: Orlando, FL, USA, 1986. [Google Scholar]
- Appelo, C.A.J.; Postma, D. Geochemistry, Groundwater and Pollution, 2nd ed.; Balkema: Leiden, The Netherlands, 2005. [Google Scholar]
- Bear, J. Hydraulics of Groundwater, 1st ed.; McGraw-Hill: New York, NY, USA, 1979. [Google Scholar]
- Darcy, H. Exposition et Application des Principes a Suivre et des Formulesa Employer dans les Questions de Distribution d’Eau. In Les Fontaines Publiques de la Ville de Dijon, 1st ed.; Dalmont, V., Ed.; Libraire des Corps Imperiaux des Ponts et Chausseés et des Mines: Paris, France, 1856. (In French) [Google Scholar]
- Berkowitz, B.; Cortis, A.; Dentz, M.; Scher, H. Modeling Non-fickian transport in geological formations as a continuous time random walk. Rev. Geophys. 2006, 44, 1–49. [Google Scholar] [CrossRef]
- Hu, Y.; Xu, W.; Zhan, L.; Ye, Z.; Chen, Y. Non-Fickian Solute Transport in Rough-Walled Fractures: The Effect of Contact Area. Water 2020, 12, 2049. [Google Scholar] [CrossRef]
- Cortis, A.; Berkowitz, B. Computing “anomalous” contaminant transport in porous media: The CTRW MATLAB toolbox. Ground Water 2005, 43, 947–950. [Google Scholar] [CrossRef]
- Brown, S.R.; Stockman, H.W.; Reevs, S.J. Applicability of the Reynolds Equation for Modeling Fluid Flow between Rough Surfaces. Geophys. Res. Lett. 1995, 22, 2537–2540. [Google Scholar] [CrossRef]
- Drazer, G.; Koplik, J. Transport in rough self-affine fractures. Phys. Rev. E 2002, 66, 026303. [Google Scholar] [CrossRef]

| Material | Name | aeff (μm) | K (m2) |
|---|---|---|---|
| Flechtinger Sandstone | Fle_8_100_S1 | 143.94 ± 0.62 | 1.73 × 10−9 ± 1.49 × 10−11 |
| Fle_8_100_S2 | 122.62 ± 0.85 | 1.25 × 10−9 ± 1.74 × 10−11 | |
| Fle_9_100_S1 | 139.84 ± 0.40 | 1.63 × 10−9 ± 9.32 × 10−12 | |
| Fle_9_100_S2 | 124.59 ± 2.61 | 1.29 × 10−9 ± 2.71 × 10−11 | |
| Fle_10_100_S1 | 121.11 ± 0.95 | 1.22 × 10−9 ± 1.92 × 10−11 | |
| Remlinger Sandstone | Rem_1_100_S1 | 102.66 ± 1.61 | 8.79 × 10−10 ± 2.75 × 10−11 |
| Rem_1_100_S2 | 93.66 ± 1.25 | 7.31 × 10−10 ± 1.95 × 10−11 | |
| Rem_1_100_S3 | 101.94 ± 4.15 | 8.67 × 10−10 ± 7.01 × 10−11 | |
| Rem_2_100_S1 | 83.06 ± 0.92 | 5.75 × 10−10 ± 1.27 × 10−11 | |
| Rem_2_100_S3 | 72.70 ± 2.57 | 4.41 × 10−10 ± 3.14 × 10−11 |
| Name | v (m/s) | DL (m2/s) | α (m) | Err (–) |
|---|---|---|---|---|
| Fle_8_100_S1 | 9.09 × 10−4 ± 1.70 × 10−5 | 1.72 × 10−5 ± 3.11 × 10−6 | 1.90 × 10−2 ± 3.73 × 10−3 | 0.57 |
| Fle_8_100_S2 | 1.35 × 10−4 ± 5.00 × 10−5 | 8.76 × 10−6 ± 3.33 × 10−6 | 6.49 × 10−2 ± 1.24 × 10−3 | 0.62 |
| Fle_9_100_S1 | 5.95 × 10−4 ± 2.16 × 10−5 | 1.75 × 10−5 ± 1.78 × 10−6 | 2.96 × 10−2 ± 4.11 × 10−3 | 0.32 |
| Fle_9_100_S2 | 1.13 × 10−3 ± 2.53 × 10−4 | 1.53 × 10−5 ± 5.08 × 10−7 | 1.44 × 10−2 ± 2.86 × 10−3 | 0.94 |
| Fle_10_100_S1 | 1.08 × 10−3 ± 7.01 × 10−4 | 2.44 × 10−5 ± 1.55 × 10−5 | 2.29 × 10−2 ± 2.26 × 10−3 | 0.28 |
| Rem_1_100_S1 | 3.08 × 10−4 ± 3.16 × 10−5 | 1.13 × 10−5 ± 1.42 × 10−6 | 3.72 × 10−2 ± 7.12 × 10−3 | 0.22 |
| Rem_1_100_S2 | 7.53 × 10−5 ± 7.82 × 10−7 | 3.94 × 10−6 ± 3.08 × 10−7 | 5.22 × 10−2 ± 4.27 × 10−3 | 0.30 |
| Rem_1_100_S3 | 6.42 × 10−5 ± 1.20 × 10−5 | 1.51 × 10−6 ± 3.06 × 10−7 | 2.35 × 10−2 ± 2.70 × 10−3 | 0.29 |
| Rem_2_100_S1 | 1.14 × 10−4 ± 1.06 × 10−5 | 3.58 × 10−6 ± 3.71 × 10−7 | 3.16 × 10−2 ± 2.09 × 10−3 | 0.15 |
| Rem_2_100_S3 | 6.10 × 10−6 ± 8.73 × 10−7 | 4.17 × 10−7 ± 7.12 × 10−8 | 6.81 × 10−2 ± 4.35 × 10−3 | 0.04 |
| Name | Test | v (m/s) | DL (m2/s) | α (m) | β | Err (–) |
|---|---|---|---|---|---|---|
| Fle_9_100_S1 | 1 | 5.65 × 10−4 | 2.02 × 10−5 | 3.58 × 10−2 | 2.56 | 0.44 |
| 2 | 6.27 × 10−4 | 1.67 × 10−5 | 2.67 × 10−2 | 2.42 | 0.37 | |
| 3 | 5.93 × 10−4 | 1.57 × 10−5 | 2.64 × 10−2 | 2.44 | 0.17 | |
| Rem_1_100_S1 | 1 | 2.80 × 10−4 | 1.34 × 10−5 | 4.79 × 10−2 | 2.76 | 0.20 |
| 2 | 3.56 × 10−4 | 1.04 × 10−5 | 2.92 × 10−2 | 2.36 | 0.24 | |
| 3 | 2.90 × 10−4 | 1.00 × 10−5 | 3.45 × 10−2 | 2.48 | 0.21 |
| Name | v (m/s) | DL (m2/s) | α (m) | Err (–) |
|---|---|---|---|---|
| Fle_8_100_S1 | 1.16 × 10−3 ± 5.85 × 10−5 | 4.31 × 10−5 ± 4.47 × 10−6 | 3.77 × 10−2 ± 5.82 × 10−3 | 0.19 |
| Fle_8_100_S2 | 4.74 × 10−4 ± 1.85 × 10−5 | 2.86 × 10−5 ± 9.65 × 10−7 | 6.04 × 10−2 ± 4.52 × 10−3 | 0.99 |
| Fle_9_100_S1 | 7.86 × 10−4 ± 1.97 × 10−5 | 3.50 × 10−5 ± 3.85 × 10−6 | 4.55 × 10−2 ± 4.30 × 10−3 | 0.19 |
| Fle_9_100_S2 | 1.29 × 10−3 ± 1.47 × 10−4 | 4.95 × 10−5 ± 4.02 × 10−6 | 3.87 × 10−2 ± 2.71 × 10−3 | 0.47 |
| Fle_10_100_S1 | 4.82 × 10−4 ± 1.97 × 10−5 | 2.45 × 10−5 ± 3.16 × 10−6 | 5.11 × 10−2 ± 7.67 × 10−3 | 0.11 |
| Rem_1_100_S1 | 3.99 × 10−4 ± 2.27 × 10−5 | 1.99 × 10−5 ± 1.61 × 10−6 | 4.97 × 10−2 ± 2.22 × 10−3 | 0.62 |
| Rem_1_100_S2 | 2.70 × 10−4 ± 4.19 × 10−5 | 1.55 × 10−5 ± 1.46 × 10−6 | 5.85 × 10−2 ± 5.98 × 10−3 | 1.31 |
| Rem_1_100_S3 | 3.25 × 10−4 ± 5.03 × 10−5 | 8.39 × 10−6 ± 2.14 × 10−6 | 2.54 × 10−2 ± 3.79 × 10−3 | 0.69 |
| Rem_2_100_S1 | 2.49 × 10−4 ± 3.09 × 10−5 | 1.26 × 10−5 ± 8.69 × 10−7 | 5.15 × 10−2 ± 5.53 × 10−3 | 7.17 |
| Rem_2_100_S3 | 5.89 × 10−5 ± 1.11 × 10−5 | 3.53 × 10−6 ± 2.88 × 10−7 | 6.13 × 10−2 ± 5.91 × 10−3 | 1.14 |
| Name | Test | v (m/s) | DL (m2/s) | α (m) | Err (–) |
|---|---|---|---|---|---|
| Fle_9_100_S1 | 1 | 7.85 × 10−4 | 4.07 × 10−5 | 5.19 × 10−2 | 0.26 |
| 2 | 7.82 × 10−4 | 3.18 × 10−5 | 4.07 × 10−2 | 0.25 | |
| 3 | 7.39 × 10−4 | 3.23 × 10−5 | 4.37 × 10−2 | 0.06 | |
| Rem_1_100_S1 | 1 | 4.20 × 10−4 | 2.23 × 10−5 | 5.31 × 10−2 | 0.87 |
| 2 | 4.12 × 10−4 | 1.97 × 10−5 | 4.79 × 10−2 | 0.46 | |
| 3 | 3.65 × 10−4 | 1.76 × 10−5 | 4.83 × 10−2 | 0.52 |
| Name | Fit | v (m/s) | DL (m2/s) | α (m) |
|---|---|---|---|---|
| Flechtinger | CTRW | 7.70 × 10−4 ± 3.68 × 10−4 | 1.66 × 10−5 ± 5.00 × 10−6 | 3.02 × 10−2 ± 1.81 × 10−2 |
| ADE | 8.38 × 10−4 ± 3.38 × 10−4 | 3.61 × 10−5 ± 9.17 × 10−6 | 4.67 × 10−2 ± 8.41 × 10−3 | |
| Remlinger | CTRW | 1.14 × 10−4 ± 1.03 × 10−4 | 4.15 × 10−6 ± 3.81 × 10−6 | 4.25 × 10−2 ± 1.59 × 10−2 |
| ADE | 2.60 × 10−4 ± 1.13 × 10−4 | 1.20 × 10−5 ± 5.65 × 10−6 | 4.93 × 10−2 ± 1.27 × 10−2 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Frank, S.; Heinze, T.; Ribbers, M.; Wohnlich, S. Experimental Reproducibility and Natural Variability of Hydraulic Transport Properties of Fractured Sandstone Samples. Geosciences 2020, 10, 458. https://doi.org/10.3390/geosciences10110458
Frank S, Heinze T, Ribbers M, Wohnlich S. Experimental Reproducibility and Natural Variability of Hydraulic Transport Properties of Fractured Sandstone Samples. Geosciences. 2020; 10(11):458. https://doi.org/10.3390/geosciences10110458
Chicago/Turabian StyleFrank, Sascha, Thomas Heinze, Mona Ribbers, and Stefan Wohnlich. 2020. "Experimental Reproducibility and Natural Variability of Hydraulic Transport Properties of Fractured Sandstone Samples" Geosciences 10, no. 11: 458. https://doi.org/10.3390/geosciences10110458
APA StyleFrank, S., Heinze, T., Ribbers, M., & Wohnlich, S. (2020). Experimental Reproducibility and Natural Variability of Hydraulic Transport Properties of Fractured Sandstone Samples. Geosciences, 10(11), 458. https://doi.org/10.3390/geosciences10110458





