Proof of Principle of a Rotating Actuator Based on Magnetostrictive Material with Simultaneous Vibration Amplitude
Abstract
1. Introduction
1.1. Challenges for Dynamic Additional Axes for Rotating Tools
1.2. Basics and Applications of Magnetostrictive Materials
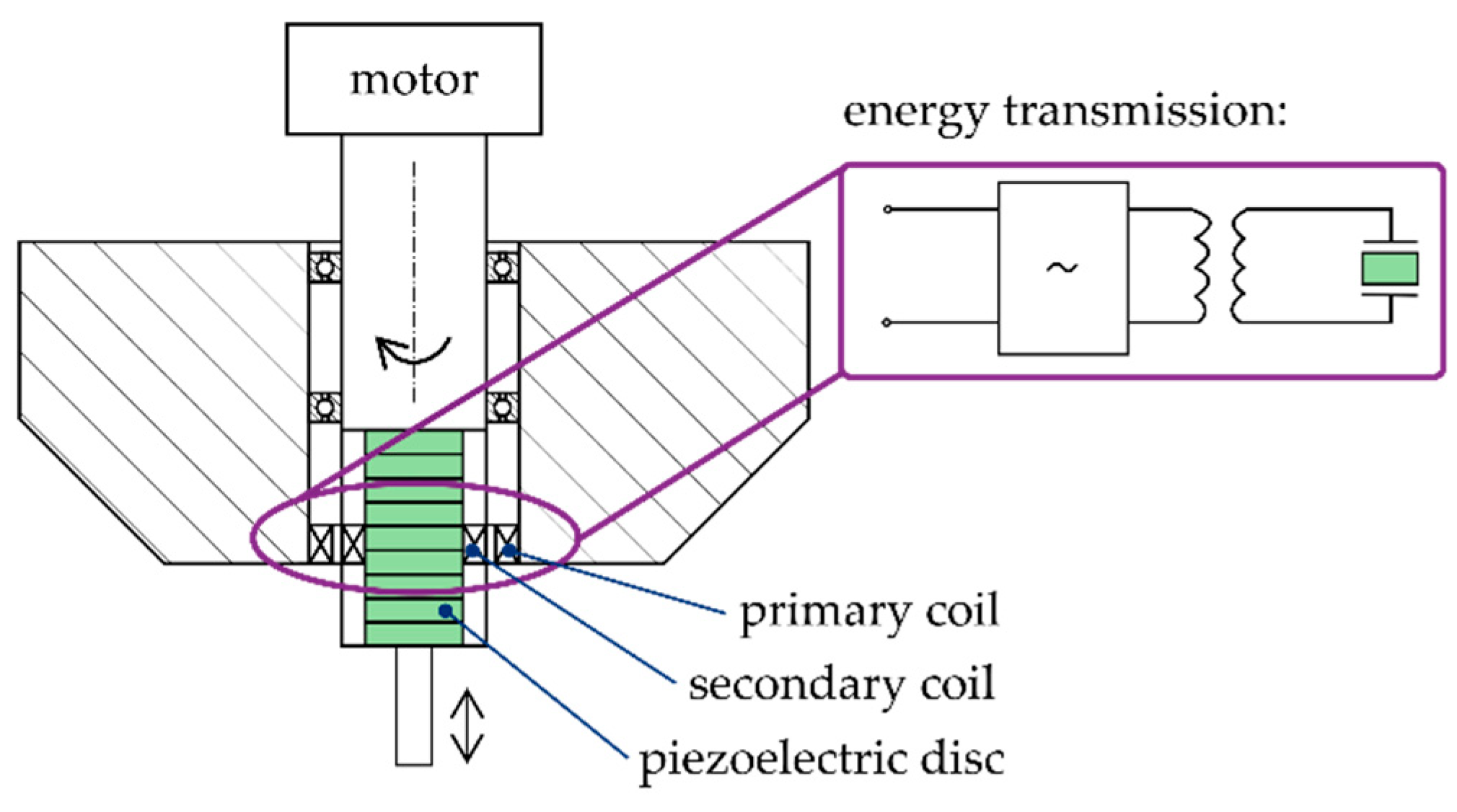
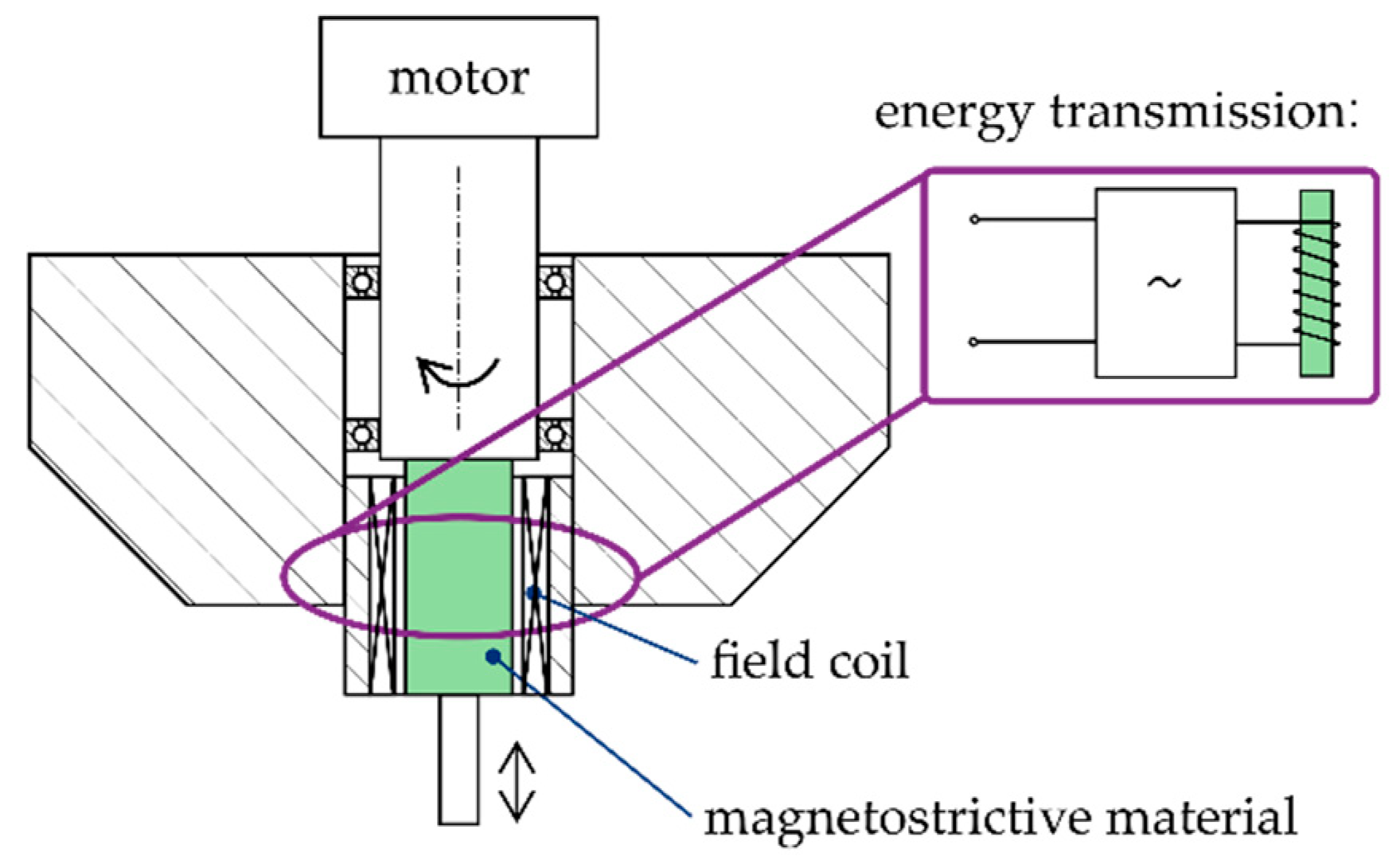
1.3. Comparison of Piezoeletric and Magnetostrictive Material and Rotional Actuator Design
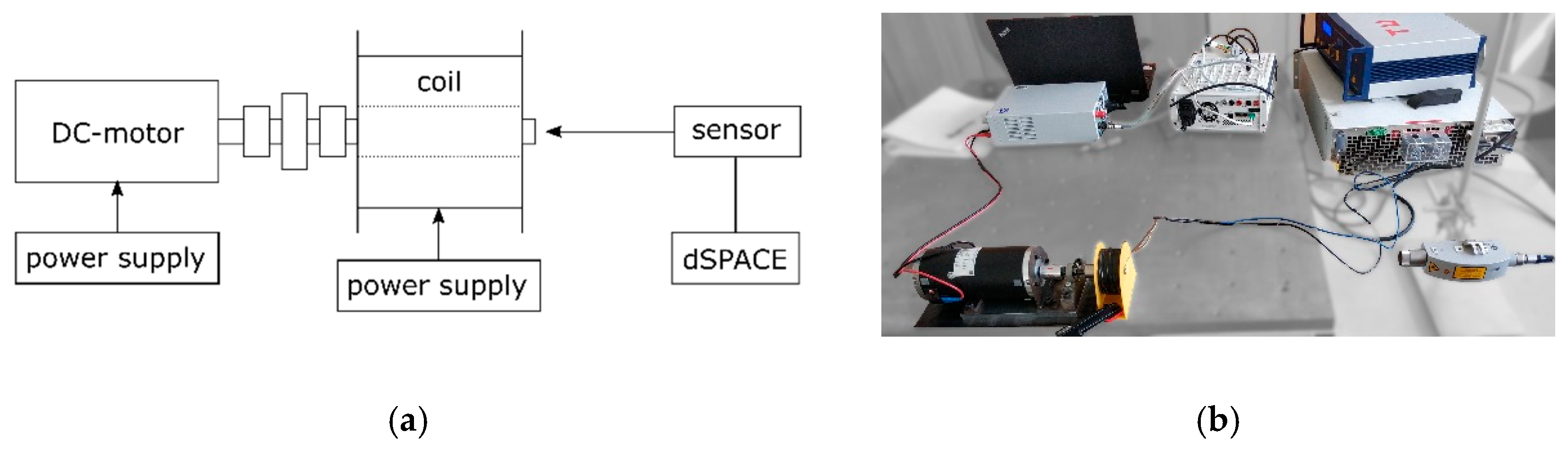
2. Materials, Test Stand, Tests and Design of Experiments
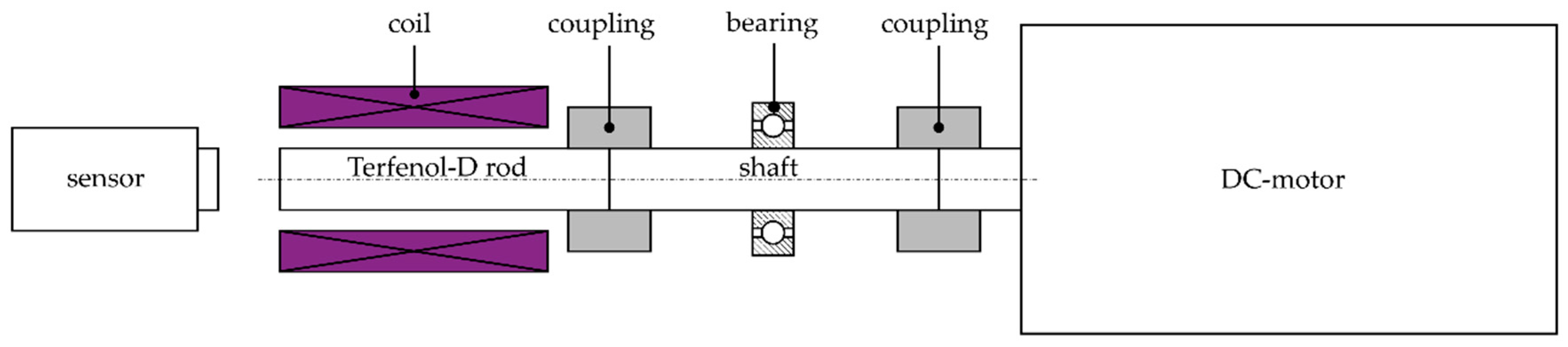
2.1. Materials and Test Stand
- the generation of a rotary movement (target value 2000 rpm),
- the generation of a switching magnetic field, which is high enough for the actuation of the sample (20 kA/m based on [19]),
- a system to measure displacements in µm-range, which is insensitive to the magnetic flux of the sample.
2.2. Tests and Validation
3. Results
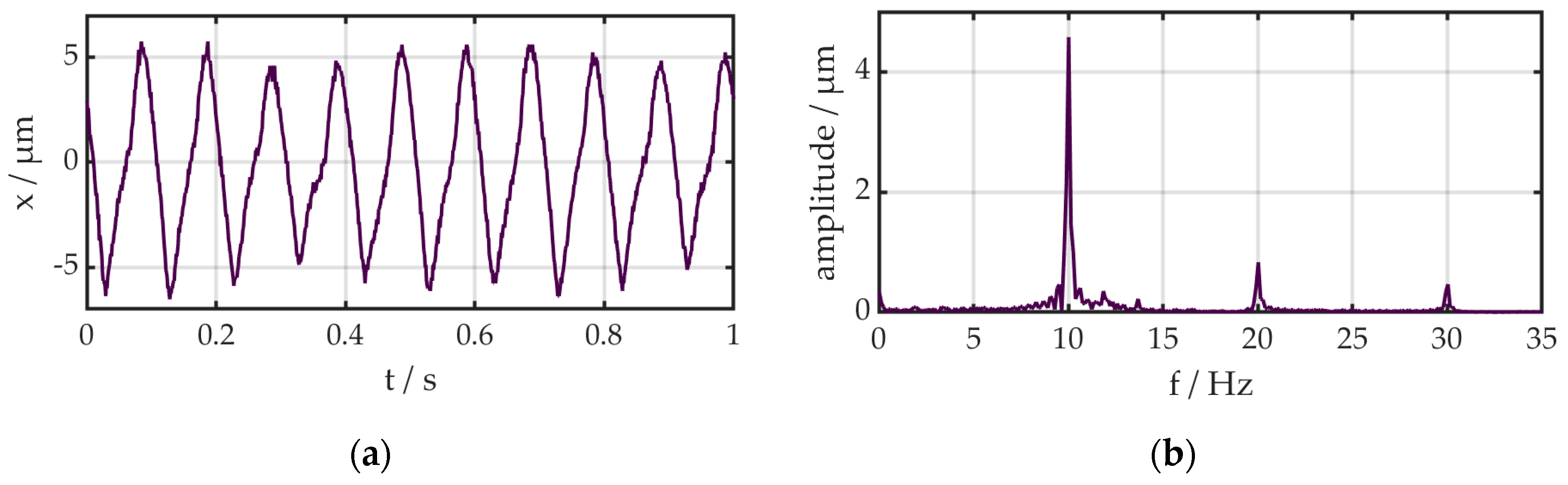
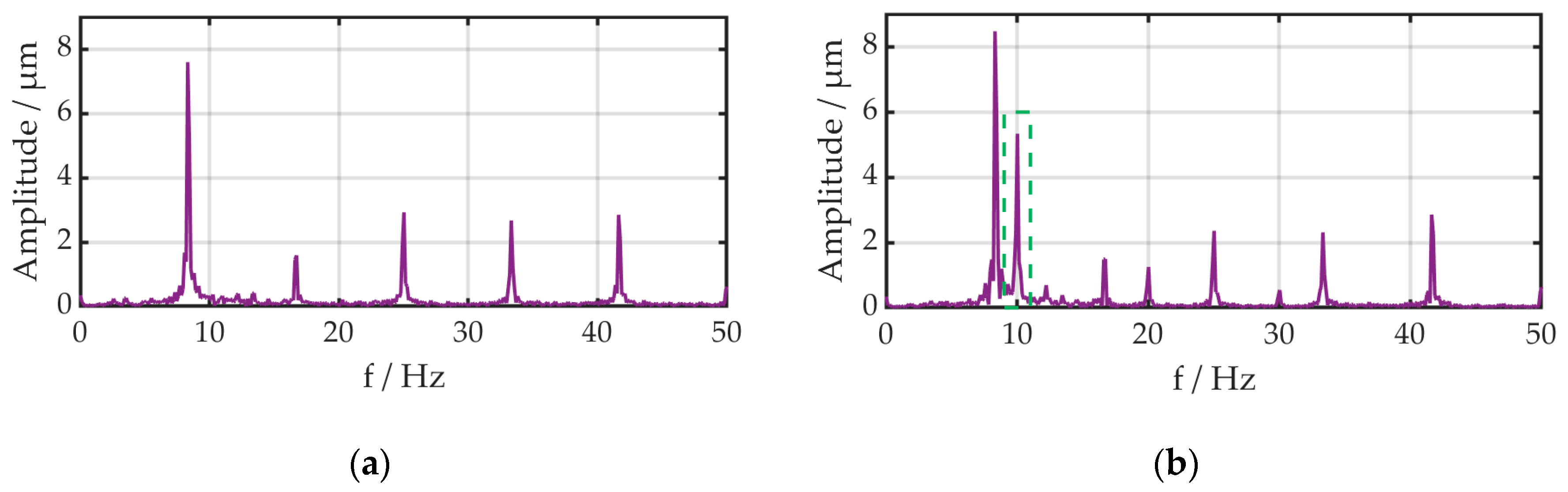
3.1. Influence of Rotation Speed
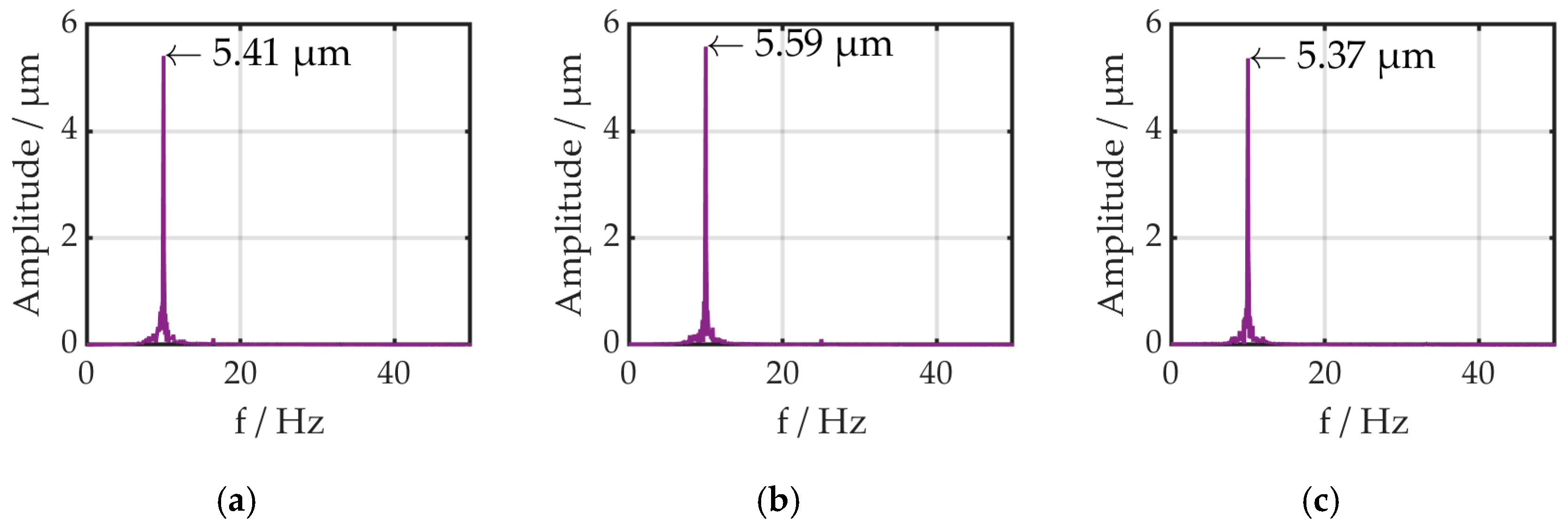
3.2. Influence of Currents Amplitude
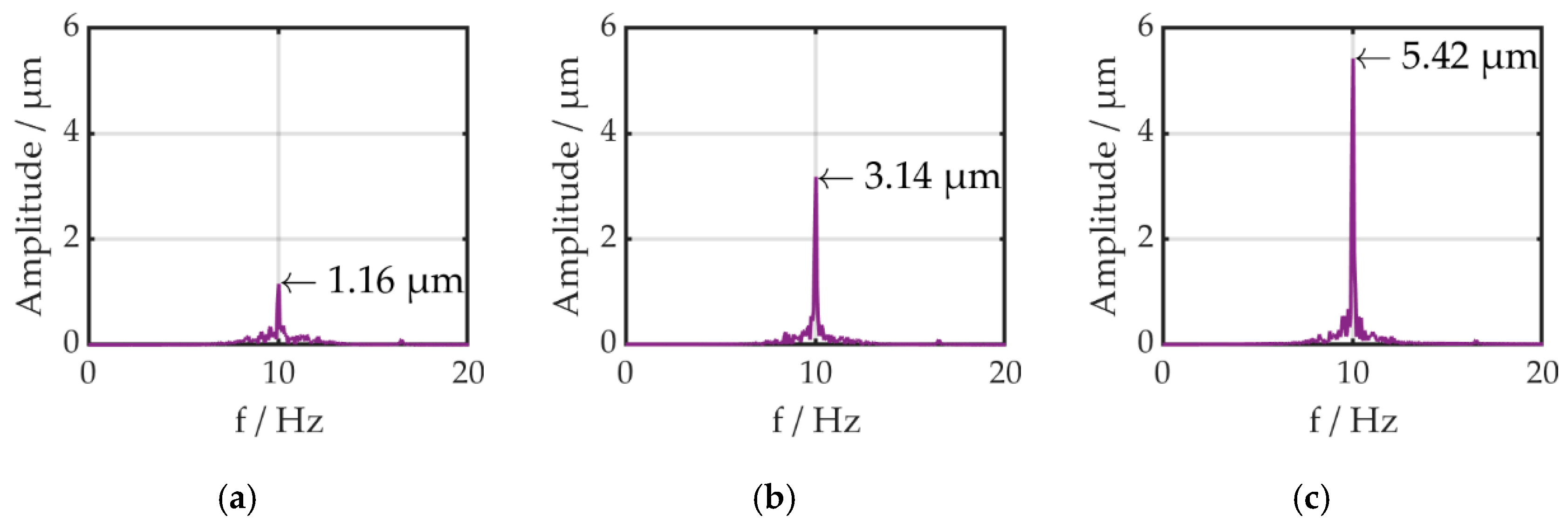
3.3. Influence of the Current Frequency
4. Discussion
5. Conclusions
- The rotation speed has only a minimal influence on the amplitude of the displacement (Variation 0.22 µm).
- The displacement amplitude increases almost linearly with an increasing amplitude of the current up to 5.42 µm.
- The displacement amplitude decreases with an increasing current frequency due to the reduction of the magnetic field (during 1000 Hz an amplitude less than 0.1 µm).
Author Contributions
Funding
Conflicts of Interest
References
- Greco, A.; Raphaelson, S.; Ehmann, K.; Wang, Q.J.; Lin, C. Surface texturing of tribological interfaces using the vibromechanical texturing method. J. Manuf. Sci. Eng. 2009, 131, 061005. [Google Scholar] [CrossRef]
- Xu, W.X.; Zhang, L.C. Ultrasonic vibration-assisted machining: Principle, design and application. Adv. Manuf. 2015, 3, 173–192. [Google Scholar] [CrossRef]
- Brehl, D.E.; Dow, T.A. Review of vibration-assisted machining. Precis. Eng. 2008, 32, 153–172. [Google Scholar] [CrossRef]
- Gallego-Juárez, J.A.; Graff, K.F. Power Ultrasonics: Applications of High-Intensity Ultrasound; Elsevier: Amsterdam, The Netherlands, 2014. [Google Scholar]
- Hemsel, T.; Bornmann, P.; Morita, T.; Sondermann-Woelke, C.; Sextro, W. Reliability analysis of ultrasonic power transducers. Arch. Appl. Mech. 2016, 86, 1707–1713. [Google Scholar] [CrossRef]
- Zhu, X.M.; Lin, B.; Liu, L.P. Review on the development of the accessory rotary ultrasonic machining tools. In Applied Mechanics and Materials; Trans Tech Publications Ltd.: Stafa-Zurich, Switzerland, 2013; Volume 268, pp. 1464–1468. [Google Scholar]
- Apicella, V.; Clemente, C.S.; Davino, D.; Leone, D.; Visone, C. Review of modeling and control of magnetostrictive actuators. In Actuators; Multidisciplinary Digital Publishing Institute: Basel, Switzerland, 2019; Volume 8, p. 45. [Google Scholar]
- Olabi, A.G.; Grunwald, A. Design and application of magnetostrictive materials. Mater. Des. 2008, 29, 469–483. [Google Scholar] [CrossRef]
- Quandt, E.; Ludwig, A. Magnetostrictive actuation in microsystems. Sens. Actuators A Phys. 2000, 81, 275–280. [Google Scholar] [CrossRef]
- Albach, T.S. Magnetostriktive Mikroaktoren und deren Anwendung als Mikrolautsprecher. Ph.D. Thesis, Universitätsbibliothek der Universität Erlangen-Nürnberg, Erlangen, Germany, 2013. [Google Scholar]
- Hiller, M.W.; Bryant, M.D.; Umegaki, J. Attenuation and transformation of vibration through active control of magnetostrictive Terfenol. J. Sound Vib. 1989, 134, 507–519. [Google Scholar] [CrossRef]
- Zhang, T.; Jiang, C.; Zhang, H.; Xu, H. Giant magnetostrictive actuators for active vibration control. Smart Mater. Struct. 2004, 13, 473. [Google Scholar] [CrossRef]
- Shaw, J. Adaptive vibration control by using magnetostrictive actuator. J. Intell. Mater. Syst. Struct. 1998, 9, 87–94. [Google Scholar] [CrossRef]
- Karafi, M.R.; Hojjat, Y.; Sassani, F. A new hybrid longitudinal–torsional magnetostrictive ultrasonic transducer. Smart Mater. Struct. 2013, 22, 065013. [Google Scholar] [CrossRef]
- Karafi, M.R.; Korivand, S. Design and fabrication of a novel vibration-assisted drilling tool using a torsional magnetostrictive transducer. Int. J. Adv. Manuf. Technol. 2019, 102, 2095–2106. [Google Scholar] [CrossRef]
- TdVibLLC. Available online: http://tdvib.com/cu18a-ultrasonic-actuator/ (accessed on 5 December 2019).
- Calkins, F.T.; Flatau, A.B.; Dapino, M.J. Overview of magnetostrictive sensor technology. J. Intell. Mater. Syst. Struct. 2007, 18, 1057–1066. [Google Scholar] [CrossRef]
- Janocha, H. Magnetostriktive Aktoren. In Unkonventionelle Aktoren: Eine Einführung; Walter de Gruyter: Berlin, Germany, 2013; pp. 71–96. [Google Scholar]
- TdVibLLC. Available online: http://www.tdvib.com/wp-content/uploads/2015/09/Terfenol-D-Magnetostriction.png (accessed on 11 December 2019).
- Ansys Inc.: Ansys Maxwell, 19.1, Computer Software. 2020. Available online: https://www.ansys.com/de-de/products/electronics/ansys-maxwell (accessed on 16 June 2020).
- The MathWorks, Inc.: Matlab, R2019b, Computer Software. 2020. Available online: https://www.mathworks.com/products/new_products/release2019b.html (accessed on 16 June 2020).
- Matlab. Available online: https://de.mathworks.com/help/signal/ref/designfilt.html#bt7zd56 (accessed on 11 December 2019).
- TdVibLLC. Available online: http://tdvib.com/terfenol-d/ (accessed on 11 December 2019).
- Börner, R.; Winkler, S.; Junge, T.; Titsch, C.; Schubert, A.; Drossel, W.G. Generation of functional surfaces by using a simulation tool for surface prediction and micro structuring of cold-working steel with ultrasonic vibration assisted face milling. J. Mater. Process. Technol. 2018, 255, 749–759. [Google Scholar] [CrossRef]
- Saslow, W.M. Maxwell’s theory of eddy currents in thin conducting sheets, and applications to electromagnetic shielding and MAGLEV. Am. J. Phys. 1992, 60, 693–711. [Google Scholar] [CrossRef]



| Magnetostrictive Material (Terfenol-D) | Piezoelectric Material (PXE 52) | |
|---|---|---|
| Maximum strain (10−3 m/m) | … 1.5 | … 1.5 |
| Coupling factor | … 0.75 | … 0.75 |
| Modulus of elasticity (103 N/mm2) | 25 … 35 (cH) 50 … 55 (cB) | ≈110 (cD) 60 … 90 (cE) |
| Speed of sound (m/s) | 1700 () 2500 () | 2800 () 3800 () |
| Energy density (103 J/m3) | 10 … 25 | 20 … 30 |
| Curie temperature (°C) | 380 | 165 … 300 |
| Density (103 kg/m3) | 9.25 | 7.8 |
| Control | current | voltage |
| Field | magnetic field | electric field |
| Name of the Equipment | Used for |
|---|---|
| CLV-2534 compact laser vibrometer from Polytec GmbH | Measurement of elongation of the sample |
| Air sensor type K from VOLTCRAFT | Measurement of temperature |
| Magnetic field strength meter FH 52 from MAGNET-PHYSIK Dr. Steingroever GmbH | Measurement of magnetic field |
| MicroLabBox from dSPACE GmbH | Data collection |
| EA-PSI 9750-20 WR 3U from EA Elektro-Automatik GmbH & Co. KG | Power supply coil |
| Experiment | Frequency/Hz | Amplitude/A | Rotation Speed/rpm | ||||||
|---|---|---|---|---|---|---|---|---|---|
| Influence of rotation speed | 10 | 1.4 | 1000 | 1500 | 2000 | ||||
| Influence of currents amplitude | 10 | 0.6 | 1.0 | 1.4 | 1000 | ||||
| Influence of currents frequency | 10 | 100 | 1000 | 1.4 | 1000 | ||||
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Titsch, C.; Li, Q.; Kimme, S.; Drossel, W.-G. Proof of Principle of a Rotating Actuator Based on Magnetostrictive Material with Simultaneous Vibration Amplitude. Actuators 2020, 9, 81. https://doi.org/10.3390/act9030081
Titsch C, Li Q, Kimme S, Drossel W-G. Proof of Principle of a Rotating Actuator Based on Magnetostrictive Material with Simultaneous Vibration Amplitude. Actuators. 2020; 9(3):81. https://doi.org/10.3390/act9030081
Chicago/Turabian StyleTitsch, Christian, Qiang Li, Simon Kimme, and Welf-Guntram Drossel. 2020. "Proof of Principle of a Rotating Actuator Based on Magnetostrictive Material with Simultaneous Vibration Amplitude" Actuators 9, no. 3: 81. https://doi.org/10.3390/act9030081
APA StyleTitsch, C., Li, Q., Kimme, S., & Drossel, W.-G. (2020). Proof of Principle of a Rotating Actuator Based on Magnetostrictive Material with Simultaneous Vibration Amplitude. Actuators, 9(3), 81. https://doi.org/10.3390/act9030081




