A Novel Adjustable Damper Design for a Hybrid Passive Ankle Prosthesis
Abstract
:1. Introduction
2. Methods
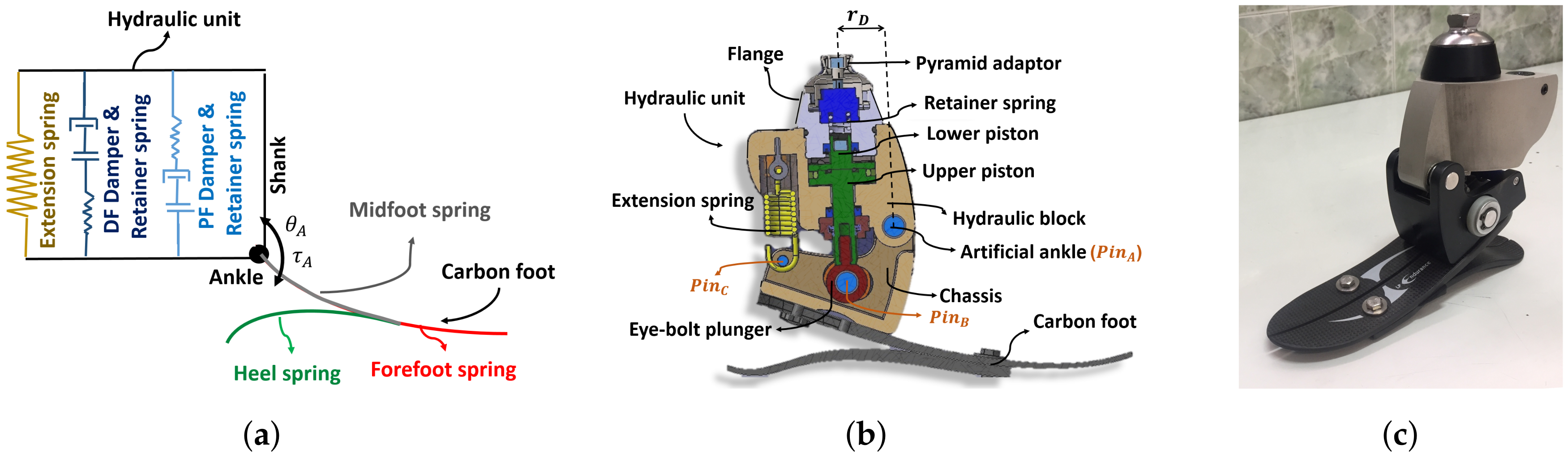
2.1. Hybrid-Hydraulic Ankle Prosthetic (H2AP) Foot
2.1.1. Ankle Biomechanics
2.1.2. Mechanical Representation
- During LD, the ankle mechanics can be described as linear springs [37].
2.1.3. Mechanical Design
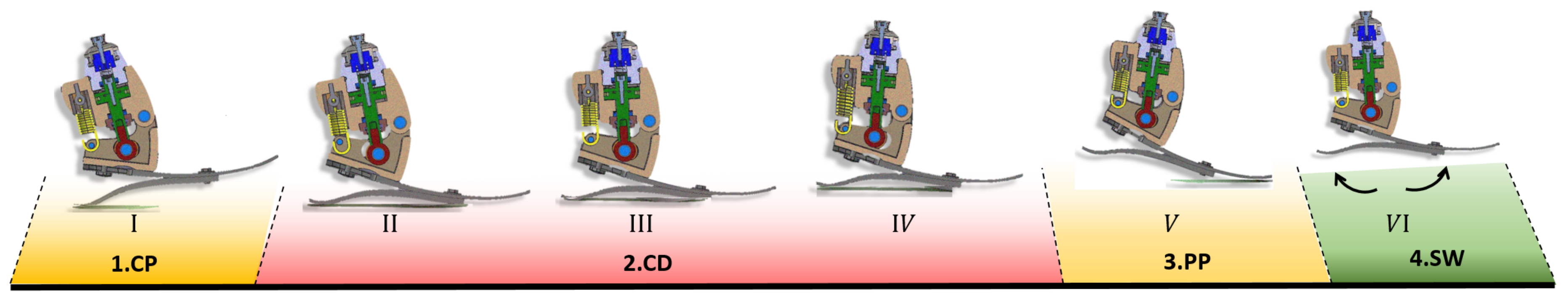
- Chassis: The chassis includes a hybrid railway mechanism to engage and disengage the piston movement (latching/unlatching the damper) during different moments of the gait cycle. The rotary motion of the artificial ankle is converted to the piston’s reciprocating linear movement by damper arm (Figure 1b). A chassis is screwed to the midfoot spring.
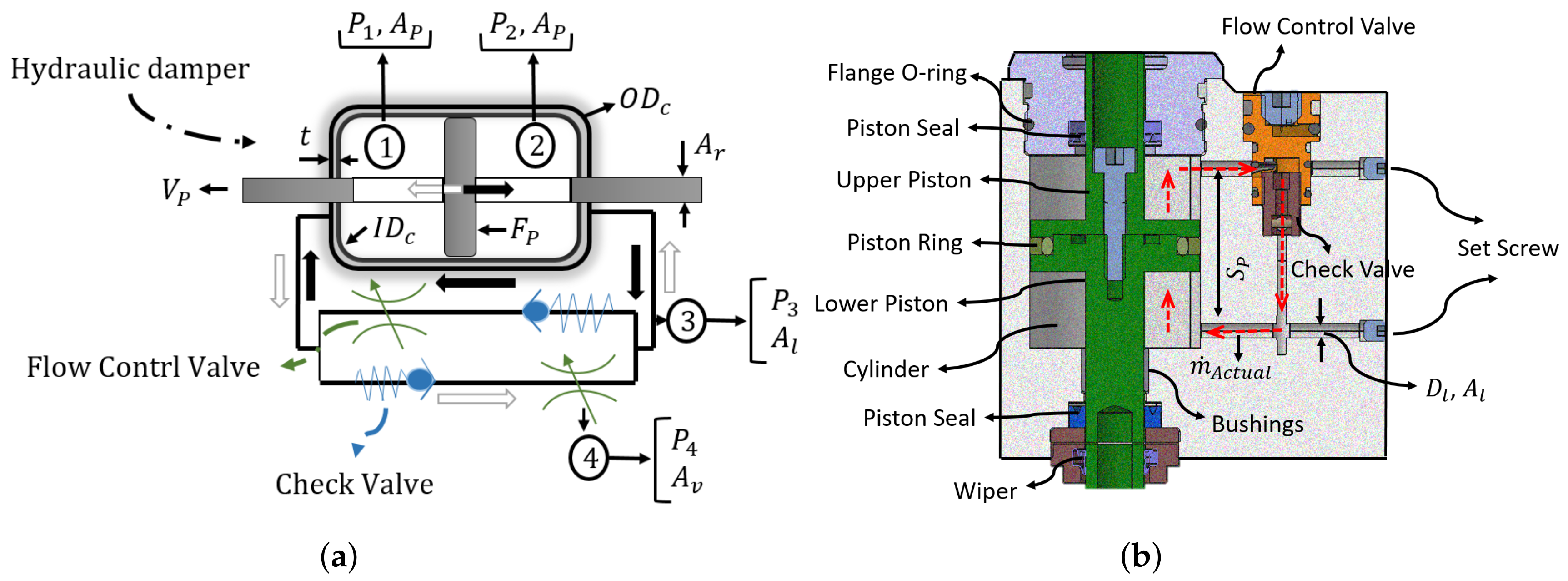
- Hydraulic block: The hydraulic block acts as a housing for the piston and the rotary flow control valves. The piston is reciprocally embedded in the hydraulic block and comprises the upper piston and the lower piston. The piston divides the cylinder, located in the hydraulic block, into upper and lower chambers on the opposite side of the piston. The cover and flange screwed to the hydraulic block to maintain the components’ location inside the hydraulic block.
- Rotary Flow Control Valves: Two rotary flow control valves integrated with check-valves (Figure 3) are manually adjusting the damping coefficient of the plantar flexion (PF) and dorsiflexion (DF) dampers, independently. The upper cylinder chamber connects to the lower cylinder chamber by two independent hydraulic lines. Each hydraulic line has the rotary flow control valve integrated with a check-valve. The presence of the check-valve in each hydraulic line allows the fluid to flow through only one line. Screws are used to keep the rotary flow control valves in their housing and prevent them from being fully closed.
2.2. Experiment-Based Design
- The maximum angular velocity of the artificial ankle joint is determined based on the intact ankle biomechanical behavior during the first 25% of the gait cycle (when the damper is active) [11].
- The maximum allowable pressure specified based on the industry sealing standards [43].
- The ultimate safety factors determined by contemplating the following factors: 1. material, 2. stress, 3. geometry, 4. failure analysis, and 5. reliability [44].
2.3. Hydraulic Damper Specification
2.3.1. Damper RoM
2.3.2. Valve Optimal Diameter
2.3.3. Hydraulic Damper Performance
2.3.4. Hydraulic Damper Material
- Piston stress: The piston and rod diameters were determined using Equation (5) and the Parker Hydraulics Corporation catalog of metric rod wipers and piston seals [47]. The piston stress was calculated based on the mechanical handbook design [48]. The eye-bolt plunger, the piston thread-locks, and the edge of the piston, where the piston seal is located, identified as the critical parts of the piston. The force exerted to the eye-bolt plunger is equal to the force considered to design .
- Hydraulic block stress: The hydraulic block sizing (the cylinder diameters and thickness and the hydraulic line diameter) was determined to perform the stress analysis. The hydraulic cylinder’s internal diameter was considered to be equal to the piston ring’s diameter (Figure 5b). Then, the lame’s equation (Equation (9), [49]) was utilized to define the outside diameter of the cylinder. In addition, the cylinder thickness was computed using Equation (10):The hydraulic line size was calculated exploiting the standard nomogram (industry standards for sizing hydraulic lines [50]), as presented in Equation (11):The hydraulic bock critical stress was specified with the help of ABAQUS [51]. The ABAQUS model was assumed to be symmetric and pretty simplified due to the complexity of the hydraulic block for partitioning and meshing. The mesh independence study (evaluate the stress convergence) was performed by implementing several simulations with different meshes’ size to determine proper mesh size. The top end (the flange location, Figure 1a) and the hinge joint (the artificial ankle location, Figure 1a) of the block were considered cantilever and pinned support, respectively. The maximum operating pressure (derived within the simulation study) and the critical forces exerted by at the artificial ankle joint served as inputs to the model. Block static stress analysis was performed with a safety factor of 1.88 (Table 1) to include the dynamic conditions (the dynamic pressure and forces applied to the hydraulic block during 25% of the gait cycle).
2.4. Pilot Level-Ground Walking Experiment
3. Results
3.1. Hydraulic Damper Specification
3.1.1. Damper RoM
3.1.2. Valve Optimal Diameter
3.1.3. Hydraulic Damper Performance
3.1.4. Hydraulic Damper Material
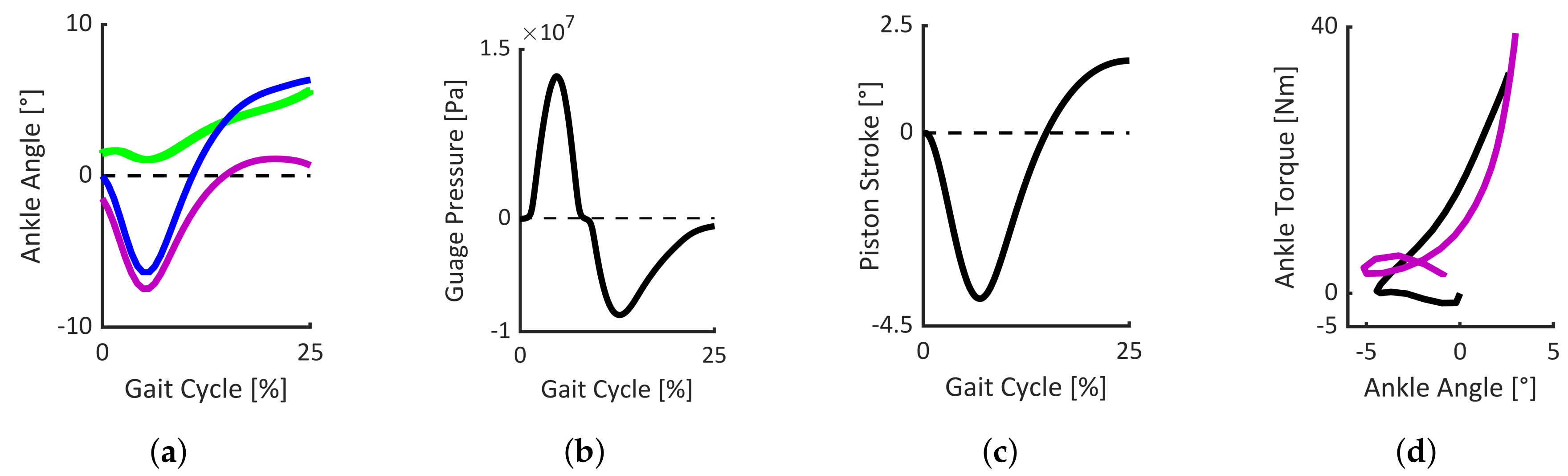
3.2. Pilot Level-Ground Walking Experiment
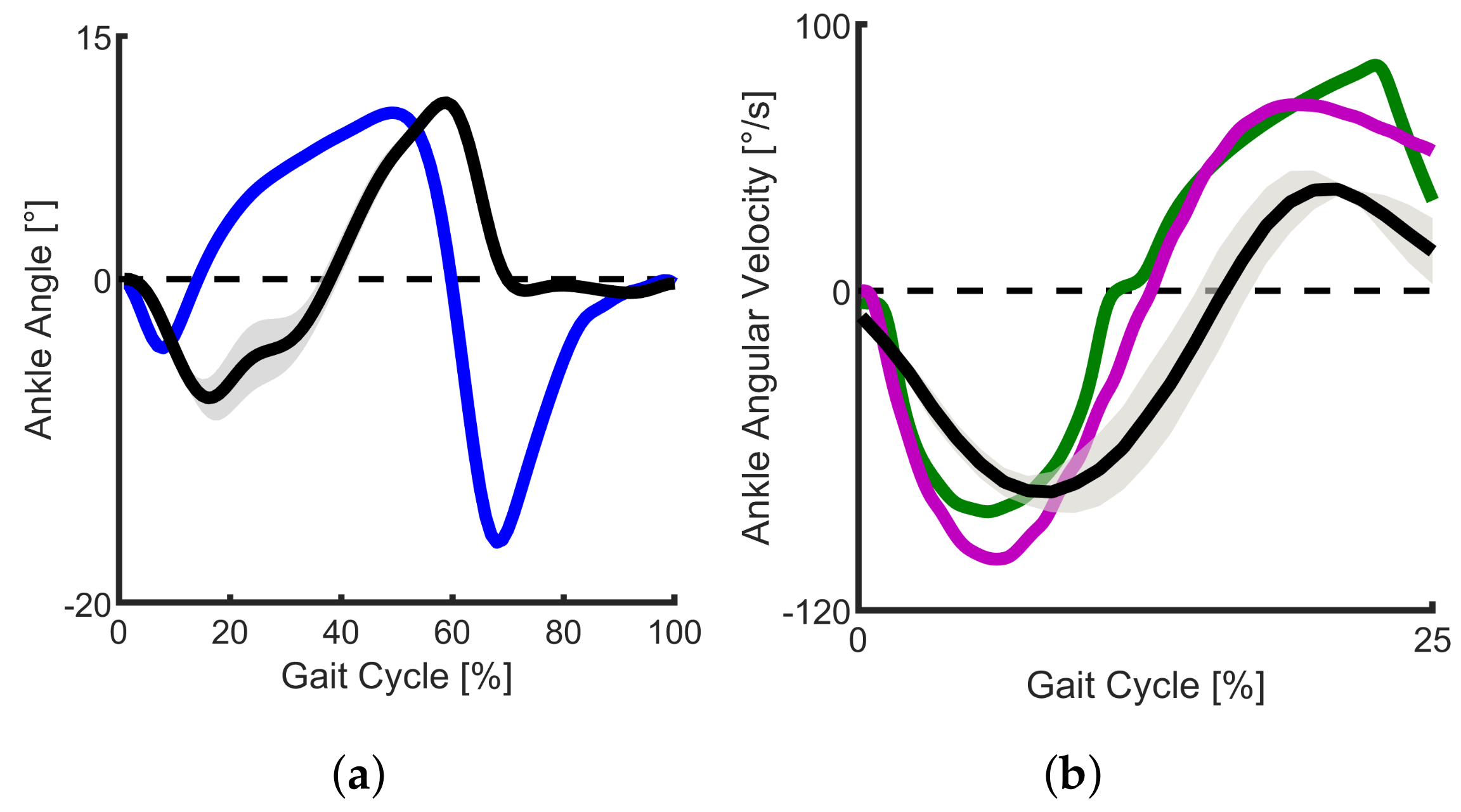
3.2.1. Ankle Angle
3.2.2. Ankle Angular Velocity
4. Discussion
- The contrived hybrid railway mechanism (four-bar linkage) latches and unlatches the dampers in different sub-phases of the gait cycle without exploiting any additional elements such as clutches, sensors, motors, and batteries. Since employing dampers disrupts energy-returning during late stance, this mechanism can provide significant advantages compared to other passive hydraulic prosthetic feet.
- The mechanism for converting the ankle joint’s rotary motion to the linear movement of the piston raises the issue of fluid leakage in the hydraulic feet. As the piston should be aligned with the cylinder, the hydraulic sealing parts’ placement has to be carefully designed. Utilizing a curved cylinder can remove the potential of fluid leakage [54].
- The multiple sealing components were considered for the flow control valves, embedded in the hydraulic damper, not only guarantee the valves’ functionality but also provide frictional torque (between the valves and their housing) to restrict any sudden movement of the control valves.
- The design of flow control valves, customized for the hydraulic damper, may result in developing light and optimized prosthetic foot. The usage of flow control valves rather than the piston orifice (hollows carved on the piston surface) results in an ease of valve changing for the maintenance purpose. In addition, this mechanism makes it possible to couple motorized components to the valves and produce a variable damper.
- The subject preferred to set a low damping coefficient for the PF damper to have a more compliant heel strike. However, this low coefficient can cause an excessive and prolonged negative angle in CP compared to the reference data. Although the pilot experiment was performed based on the damper coefficients’ subject-specific tuning, a higher-viscosity fluid or an increased damping ratio (manually tuned) can be used for the PF damper to increase the resistance during CP.
- A possible reason for the delay in dorsiflexion increase (in ED) may belong to the high damping coefficient for the DF damper. This damping ratio was adjusted to increase resistance (preventing forward fall over), and provide smooth rollover (smooth transition between CP and ED).
- The reason for the increased ankle dorsiflexion during late stance (compared to the initial design, 7 in dorsiflexion) can be due to the incorrect static alignment of the prosthesis in the anterior-posterior direction. A methodological approach (e.g., 3D L.A.S.A.R Posture from Otto Bock) should be used to check and optimize the individual static alignment in anterior-posterior.
- A possible reason that can explain the observed hump in ED may be due to fluid leakage. As the fluid can be lost during the experiment, which can change the H2AP damper’s behavior, the fill level of the dampers should be checked to ensure the intended performance within the tests.
5. Conclusions and Future Work
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Fletcher, D.D.; Andrews, K.L.; Hallett, J.W., Jr.; Butters, M.A.; Rowland, C.M.; Jacobsen, S.J. Trends in rehabilitation after amputation for geriatric patients with vascular disease: Implications for future health resource allocation. Arch. Phys. Med. Rehabil. 2002, 83, 1389–1393. [Google Scholar] [CrossRef]
- Gardiner, J.; Bari, A.Z.; Howard, D.; Kenney, L. Transtibial amputee gait efficiency: Energy storage and return versus solid ankle cushioned heel prosthetic feet. J. Rehabil. Res. Dev. 2017, 53, 1133–1138. [Google Scholar] [CrossRef]
- Casillas, J.M.; Dulieu, V.; Cohen, M.; Marcer, I.; Didier, J.P. Bioenergetic comparison of a new energy-storing foot and SACH foot in traumatic below-knee vascular amputations. Arch. Phys. Med. Rehabil. 1995, 76, 39–44. [Google Scholar] [CrossRef]
- Kulkarni, J.; Wright, S.; Toole, C.; Morris, J.; Hirons, R. Falls in patients with lower limb amputations: Prevalence and contributing factors. Physiotherapy 1996, 2, 130–136. [Google Scholar] [CrossRef]
- Curtze, C.; Hof, A.L.; Postema, K.; Otten, B. Over rough and smooth: Amputee gait on an irregular surface. Gait Posture 2011, 33, 292–296. [Google Scholar] [CrossRef]
- LaPrè, A.K.; Sup, F. Simulation of a slope adapting ankle prosthesis provided by semi-active damping. In Proceedings of the 2011 Annual International Conference of the IEEE Engineering in Medicine and Biology Society, Boston, MA, USA, 30 August–3 September 2011; pp. 587–590. [Google Scholar]
- Silverman, A.K.; Neptune, R.R. Muscle and prosthesis contributions to amputee walking mechanics: A modeling study. J. Biomech. 2012, 45, 2271–2278. [Google Scholar] [CrossRef]
- Zmitrewicz, R.J.; Neptune, R.R.; Walden, J.G.; Rogers, W.E.; Bosker, G.W. The effect of foot and ankle prosthetic components on braking and propulsive impulses during transtibial amputee gait. Arch. Phys. Med. Rehabil. 2006, 87, 1334–1339. [Google Scholar] [CrossRef]
- Miller, W.C.; Speechley, M.; Deathe, B. The prevalence and risk factors of falling and fear of falling among lower extremity amputees. Arch. Phys. Med. Rehabil. 2001, 82, 1031–1037. [Google Scholar] [CrossRef]
- Mills, P.M.; Barrett, R.S. Swing phase mechanics of healthy young and elderly men. Hum. Mov. Sci. 2001, 20, 427–446. [Google Scholar] [CrossRef]
- Sup, F.; LaPrè, A.K. A semi-active damper design for use in a terrain adaptive ankle prosthesis. In Proceedings of the ASME 2011 International Mechanical Engineering Congress and Exposition, American Society of Mechanical Engineers Digital Collection, Denver, CO, USA, 13–17 November 2011; pp. 1289–1294. [Google Scholar]
- Koehler-McNicholas, S.R.; Nickel, E.A.; Medvec, J.; Barrons, K.; Mion, S.; Hansen, A.H. The influence of a hydraulic prosthetic ankle on residual limb loading during sloped walking. PLoS ONE 2017, 12, e0173423. [Google Scholar] [CrossRef] [Green Version]
- Mauch, H.A. Control Mechanism for Artificial Ankle. U.S. Patent 2,843,853, 22 July 1958. [Google Scholar]
- Moser, D.; Abimosleh, F.; Zahedi, M.S.; Harris, G.; Lang, S.T.; Sykes, A.J. Prosthetic Ankle Joint Mechanism. U.S. Patent 8,740,991, 3 June 2014. [Google Scholar]
- Alimusaj, M.; Fradet, L.; Braatz, F.; Gerner, H.J.; Wolf, S.I. Kinematics and kinetics with an adaptive ankle foot system during stair ambulation of transtibial amputees. Gait Posture 2009, 30, 356–363. [Google Scholar] [CrossRef]
- Poulson, A.M., III; Christensen, R.; Heath, S.; Hsu, H. Hydraulic Prosthetic Ankle. U.S. Patent 8,986,398, 7 August 2014. [Google Scholar]
- Au, S.; Berniker, M.; Herr, H. Powered ankle-foot prosthesis to assist level-ground and stair-descent gaits. Neural Netw. 2008, 21, 654–666. [Google Scholar] [CrossRef]
- New Elan. Available online: http://www.endolite.com/products/elan (accessed on 16 July 2020).
- Motion Foot MX. Available online: http://fillauer.eu/feet/motion-foot (accessed on 16 July 2020).
- Gharini, M.; Mohammadi Moghaddam, M.; Farahmand, F. Investigating the effect of viscoelastic ankle foot prosthesis on below-knee amputee gait cycle: Modeling and simulation. Modares Mech. Eng. 2017, 17, 311–321. [Google Scholar]
- De Asha, A.R.; Johnson, L.; Munjal, R.; Kulkarni, J.; Buckley, J.G. Attenuation of centre-of-pressure trajectory fluctuations under the prosthetic foot when using an articulating hydraulic ankle attachment compared to fixed attachment. Clin. Biomech. 2013, 28, 218–224. [Google Scholar] [CrossRef] [Green Version]
- Johnson, L.; De Asha, A.R.; Munjal, R.; Kulkarni, J.; Buckley, J.G. Toe Clearance When Walking in People with Unilateral Transtibial Amputation: Effects of Passive Hydraulic Ankle. J. Rehabil. Res. Dev. 2014, 51, 429–437. [Google Scholar] [CrossRef]
- De Asha, A.R.; Askew, G.; Buckley, J.G. Mechanical and Physiological Energetics When Using an Echelon Hydraulic Ankle-Foot Device in Unilateral Trans-Tibial Amputees; American Orthotics and Prosthetics Association National Assembly: Las Vegas, NV, USA, 2014. [Google Scholar]
- Vrieling, A.; Van Keeken, H.; Schoppen, T.; Otten, E.; Halbertsma, J.; Hof, A.; Postema, K. Uphill and downhill walking in unilateral lower limb amputees. Gait Posture 2008, 28, 235–242. [Google Scholar] [CrossRef] [Green Version]
- Williams, R.J.; Hansen, A.H.; Gard, S.A. Prosthetic ankle-foot mechanism capable of automatic adaptation to the walking surface. J. Biomech. Eng. 2009, 131, 035002. [Google Scholar] [CrossRef]
- Ward, J.A.; Holgate, R.; Hollander, K. Quasi-Active Prosthetic Joint System. U.S. Patent 9,289,316, 22 March 2016. [Google Scholar]
- Naseri, A.; Mohammadi Moghaddam, M.; Ahmad sharbafi, M.; Gharini, M. Hybrid-Hydraulic Prosthetic Foot. U.S. Patent 62/933,376, 2020; under review,
- Sharbafi, M.; Naseri, A.; Seyfarth, A.; Grimmer, M. Neural control in prostheses and exoskeletons. In Powered Prostheses; Elsevier: Amsterdam, The Netherlands, 2020; pp. 153–178. [Google Scholar]
- Gates, D.H. Characterizing Ankle Function During Stair Ascent, Descent, and Level Walking for Ankle Prosthesis and Orthosis Design. Ph.D. Thesis, Boston University, Boston, MA, USA, 2004. [Google Scholar]
- Palmer, M.L. Sagittal Plane Characterization of Normal Human Ankle Function Across a Range of Walking Gait Speeds. Ph.D. Thesis, Massachusetts Institute of Technology, Cambridge, MA, USA, 2002. [Google Scholar]
- Fatone, S.; Hansen, A.H. Effect of ankle-foot orthosis on roll-over shape in adults with hemiplegia. J. Rehabil. Res. Dev. 2007, 44, 11. [Google Scholar] [CrossRef]
- Hansen, A.H.; Childress, D.S.; Miff, S.C.; Gard, S.A.; Mesplay, K.P. The human ankle during walking: Implications for design of biomimetic ankle prostheses. J. Biomech. 2004, 37, 1467–1474. [Google Scholar] [CrossRef]
- Lipfert, S.W.; Günther, M.; Renjewski, D.; Seyfarth, A. Impulsive ankle push-off powers leg swing in human walking. J. Exp. Biol. 2014, 217, 1218–1228. [Google Scholar] [CrossRef] [Green Version]
- Hof, A.; Geelen, B.; Van den Berg, J. Calf muscle moment, work and efficiency in level walking; role of series elasticity. J. Biomech. 1983, 16, 523–537. [Google Scholar] [CrossRef]
- Lipfert, S. Kinematic and Dynamic Similarities between Walking and Running; Kovac: Hamburg, Germany, 2010. [Google Scholar]
- Gharini, M.; Mohammadi Moghaddam, M.; Farahmand, F. Personalized design of ankle-foot prosthesis based on computer modeling of amputee locomotion. Assist. Technol. 2020, 32, 100–108. [Google Scholar] [CrossRef]
- Bhat, S.G. Design and Development of a Passive Prosthetic Ankle; Arizona State University: Tempe, AR, USA, 2017. [Google Scholar]
- Collins, S.H.; Kuo, A.D. Recycling energy to restore impaired ankle function during human walking. PLoS ONE 2010, 5, e9307. [Google Scholar] [CrossRef] [Green Version]
- Hansen, A.H.; Nickel, E.A. Ankle-Foot Prosthesis for Automatic Adaptation to Sloped Walking Surfaces. U.S. Patent 9,549,827, 24 January 2017. [Google Scholar]
- Zahedi. A Step Change. 2010. Available online: https://www.ingenia.org.uk/getattachment/Ingenia/Issue-45/A-step-change/Zahedi.pdf (accessed on 20 January 2020).
- Nilsson, J.; Thorstensson, A. Ground reaction forces at different speeds of human walking and running. Acta Physiol. Scand. 1989, 136, 217–227. [Google Scholar] [CrossRef]
- Goldberg, S.R.; Stanhope, S.J. Sensitivity of joint moments to changes in walking speed and body-weight-support are interdependent and vary across joints. J. Biomech. 2013, 46, 1176–1183. [Google Scholar] [CrossRef] [Green Version]
- Parker. Parker Sealing Solution. 2015. Available online: https://www.parker.com/literature/Praedifa/Catalogs/Catalog_HydrSeals_PTD3350-EN.pdf (accessed on 20 January 2020).
- Ullman, D.G. The Mechanical Design Process; McGraw-Hill: New York, NY, USA, 1992. [Google Scholar]
- Ziese, A. Effects of Varying Power on Gait with an Active Ankle Prosthesis. Bachelor’s Thesis, Technical University of Darmstadt, Darmstadt, Germany, 2016. [Google Scholar]
- Fox, R.W.; Mc Donald, A.T. Introduction to Fluid Mechanics; John Wiley & Sons: Hoboken, NJ, USA, 1985. [Google Scholar]
- Fluid Power Seal Design Guide, Catalog EPS 5370; Parker Hannifin Corporation: Cleveland, OH, USA, 2007.
- Avallone, U.A.; Baumeister, H.; Sadegh, L. Standard Handbook for Mechanical Engineers; Mechanics of Solids: New York, NY, USA, 1987; Volume 3. [Google Scholar]
- Riley, W.F.; Sturges, L.D.; Morris, D.H.; Riley, W.F. Statics and Mechanics of Materials: An Integrated Approach; John Wiley & Sons: Hoboken, NJ, USA, 2002. [Google Scholar]
- Parker. Three Key Words in Hydraulic Hose Sizing: I.D., Dash Size and Nomogram. 2017. Available online: http://blog.parker.com/three-key-words-in-hydraulic-hose-sizing-id-dash-size-and-nomogram (accessed on 20 January 2012).
- Systèmes, D. ABAQUS, Analysis User’s Manual, Version 6.10-1. Dassault SystÈMes Simulia Corp. 2010, 16, 2017. [Google Scholar]
- Grimmer, M.; Seyfarth, A. Mimicking human-like leg function in prosthetic limbs. In Neuro-Robotics; Springer: Berlin/Heidelberg, Germany, 2014; pp. 105–155. [Google Scholar]
- Stigler, S.M. Francis Galton’s account of the invention of correlation. Stat. Sci. 1989, 4, 73–79. [Google Scholar] [CrossRef]
- Grissom, M. Universal Open Power, Communications, and Control for Assistive Devices; Technical Report; KCF Technologies, Inc.: State College, PA, USA, 2017. [Google Scholar]
- Winter, D.A. Biomechanics and Motor Control of Human Movement; John Wiley & Sons: Hoboken, NJ, USA, 2009. [Google Scholar]
Sample Availability: The datasets generated during and/or analyzed during the current study are available from the corresponding author on reasonable request. |



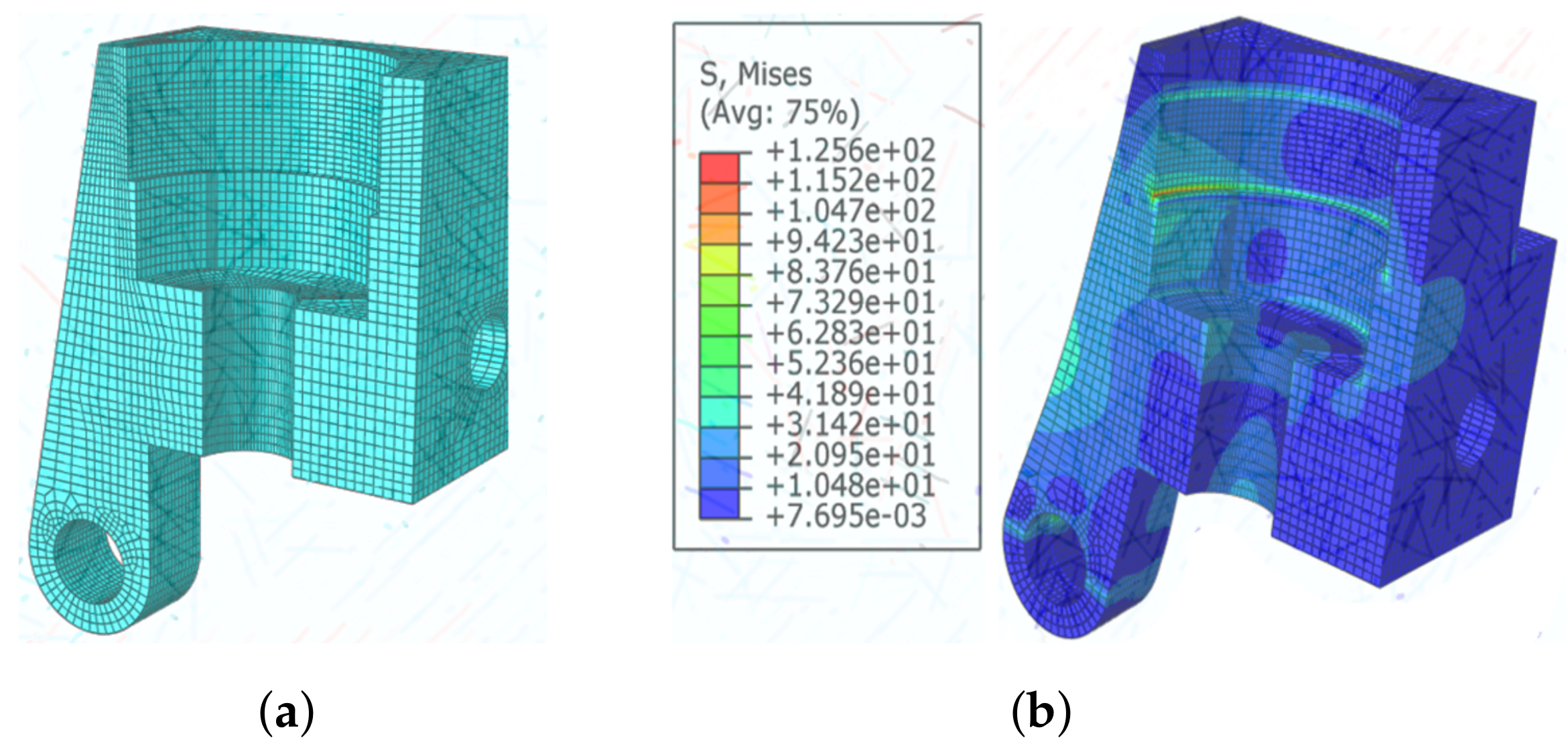
| Criterion | Definition | Value |
|---|---|---|
| Maximum allowable pressure | 20 [MPa] | |
| Maximum ankle moment | 1.2 | |
| Vertical ground reaction force | 1839 [N] | |
| Horizontal ground reaction force | 368 [N] | |
| Maximum angular velocity | 150 | |
| Hydraulic block safety factor | 2.4 | |
| Hydraulic damper components safety factor | 1.88 |
| Symbol | Description | Unit | Symbol | Description | Unit |
|---|---|---|---|---|---|
| Ankle torque | [Nm] | Fluid density | [] | ||
| Artificial ankle angle | [rad] | Cylinder inner diameter | [mm] | ||
| Carbon foot deflection | [rad] | Cylinder outer diameter | [mm] | ||
| Damper angle | [rad] | t | Cylinder wall thickness | [mm] | |
| Damper arm | [mm] | Hydraulic line Area/Diameter | []/[mm] | ||
| Piston stroke | [mm] | Control valve Area/Diameter | []/[mm] | ||
| Piston Force | [N] | Piston/Rod area | [mm] | ||
| Piston Velocity | [] | Actual mass flow rate | [] | ||
| Piston pressure in region 1,2 | [Pa] | C | Empirical discharge coefficient |
| Critical Components | Critical Stress (Von Mises) [MPa] | Critical Stress (Considering Safety Factor) [MPa] |
|---|---|---|
| 263.5 | 495 | |
| 223 | 419 | |
| 190.5 | 398 | |
| Eye_bolt plunger | 156.1 | 293.5 |
| Piston | 133.6 | 251.2 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Naseri, A.; Mohammadi Moghaddam, M.; Gharini, M.; Ahmad Sharbafi, M. A Novel Adjustable Damper Design for a Hybrid Passive Ankle Prosthesis. Actuators 2020, 9, 74. https://doi.org/10.3390/act9030074
Naseri A, Mohammadi Moghaddam M, Gharini M, Ahmad Sharbafi M. A Novel Adjustable Damper Design for a Hybrid Passive Ankle Prosthesis. Actuators. 2020; 9(3):74. https://doi.org/10.3390/act9030074
Chicago/Turabian StyleNaseri, Amirreza, Majid Mohammadi Moghaddam, Mohammad Gharini, and Maziar Ahmad Sharbafi. 2020. "A Novel Adjustable Damper Design for a Hybrid Passive Ankle Prosthesis" Actuators 9, no. 3: 74. https://doi.org/10.3390/act9030074
APA StyleNaseri, A., Mohammadi Moghaddam, M., Gharini, M., & Ahmad Sharbafi, M. (2020). A Novel Adjustable Damper Design for a Hybrid Passive Ankle Prosthesis. Actuators, 9(3), 74. https://doi.org/10.3390/act9030074






