Development of a Piezoelectric Actuator for Separation and Purification of Biological Microparticles
Abstract
1. Introduction
2. Materials and Methods
2.1. Numerical Simulation
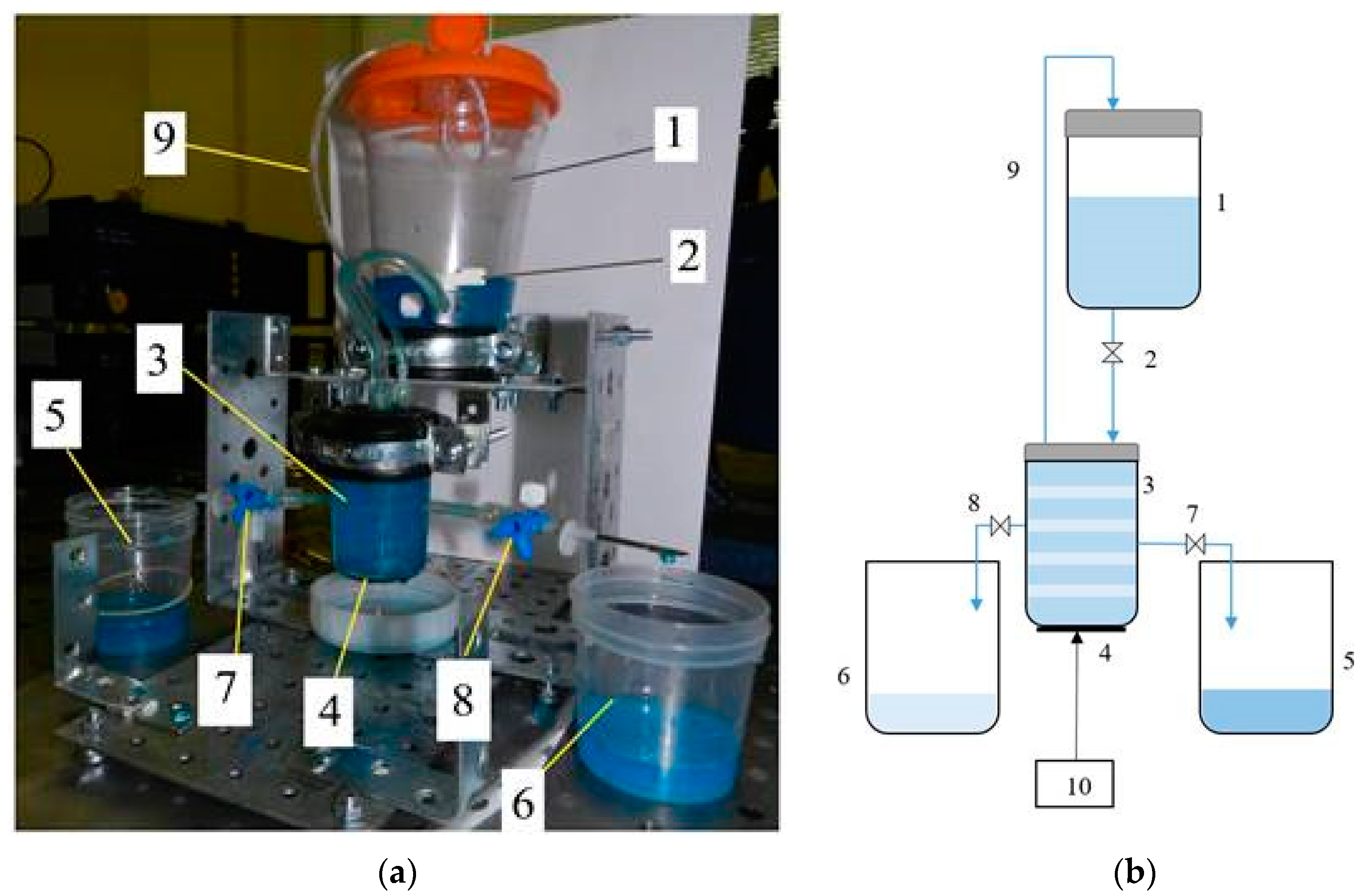
2.2. Experimental Validation
3. Results
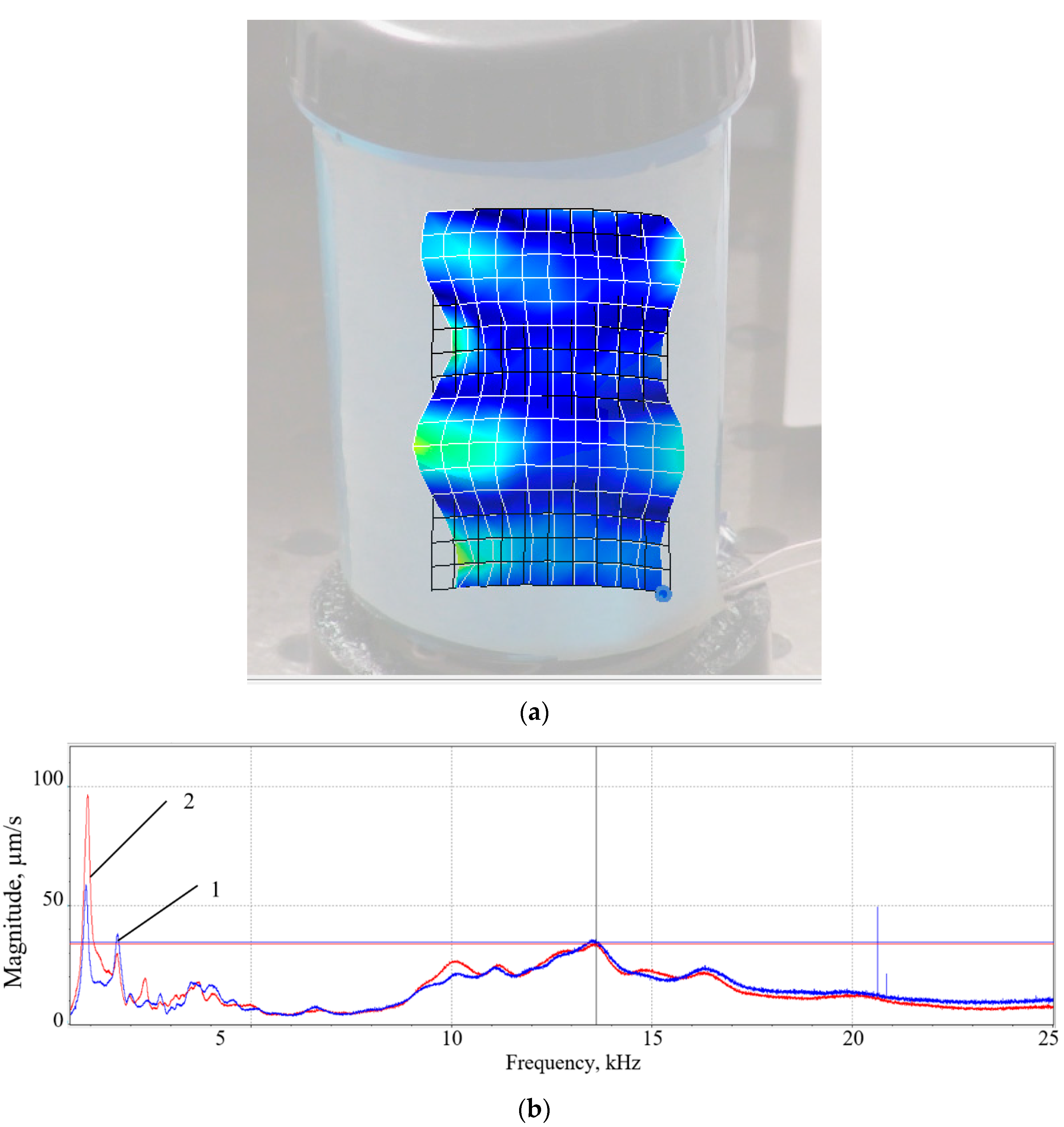
3.1. Simulation Results
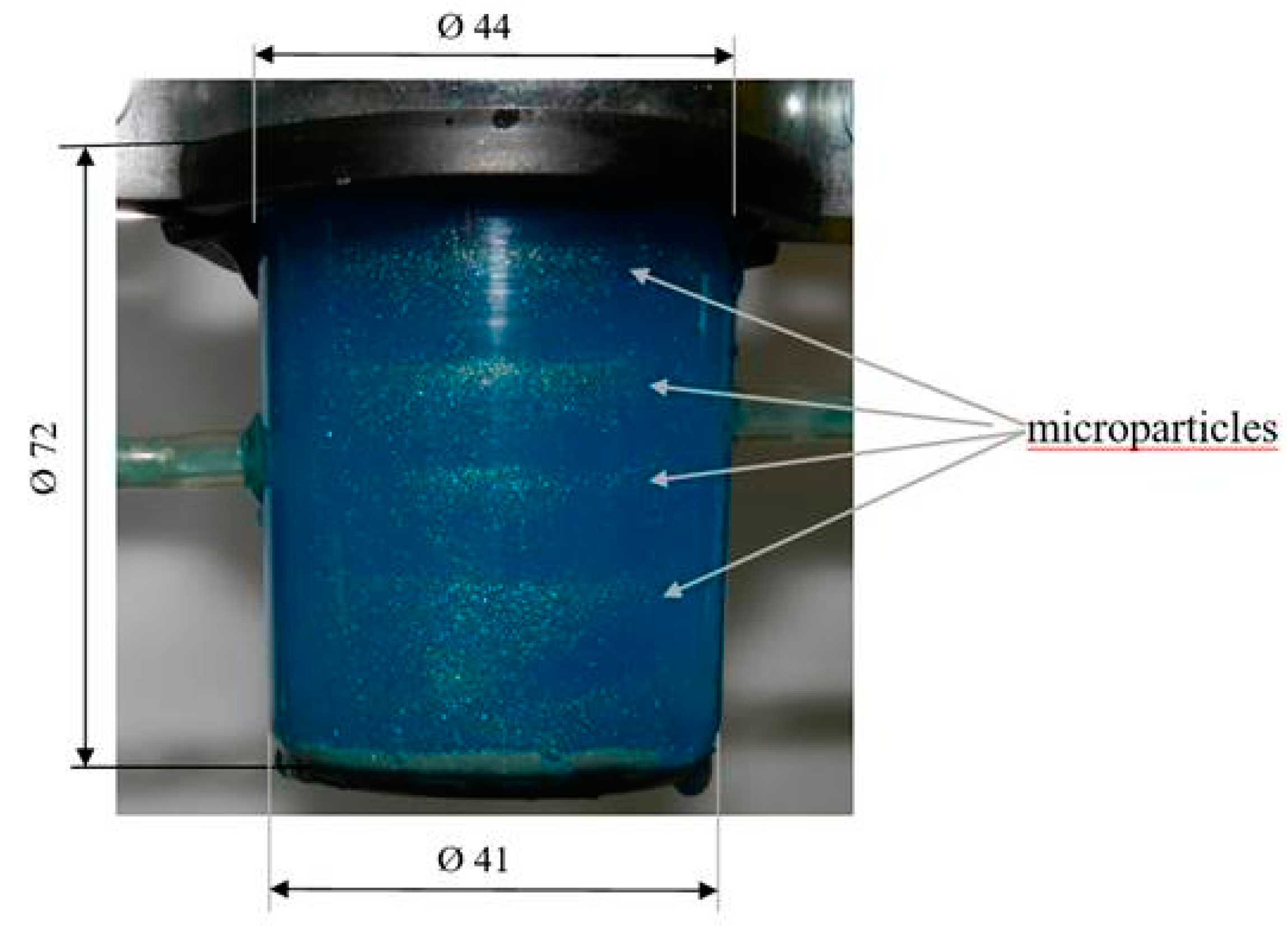
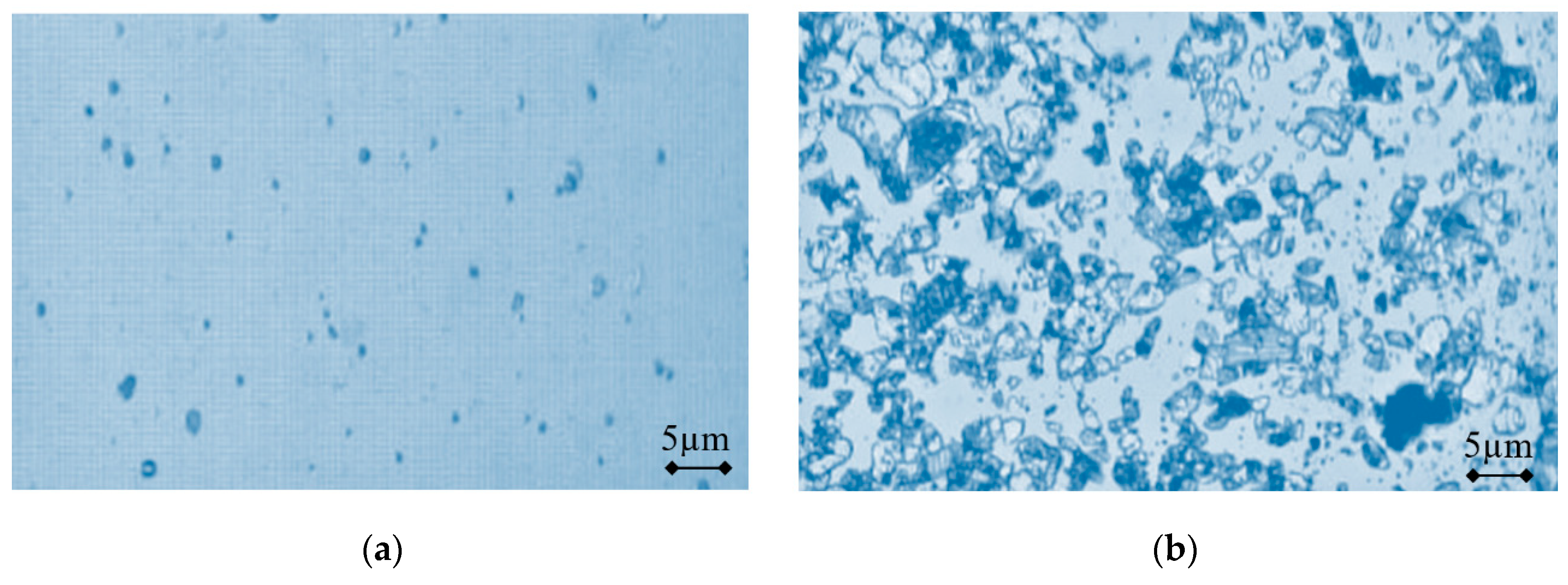
3.2. Experimental Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Gosse, C.; Croquette, V. Magnetic tweezers: Micromanipulation and force measurement at the molecular level. Biophys. J. 2002, 82, 3314–3329. [Google Scholar] [CrossRef]
- Murray, C.; Pao, E.; Tseng, P.; Aftab, S.; Kulkarni, R.; Rettig, M.; Di Carlo, D. Quantitative magnetic separation of particles and cells using gradient magnetic ratcheting. Small 2016, 12, 1891–1899. [Google Scholar] [CrossRef]
- Ding, X.; Lin, S.-C.S.; Kiraly, B.; Yue, H.; Li, S.; Chiang, I.-K.; Shi, J.; Benkovic, S.-J.; Huang, T.-J. On-chip manipulation of single microparticles, cells, and organisms using surface acoustic waves. Proc. Nat. Acad. Sci. USA 2012, 109, 11105–11109. [Google Scholar] [CrossRef] [PubMed]
- Ozcelik, A.; Rufo, J.; Guo, F.; Gu, Y.; Li, P.; Lata, J.; Huang, T.-J. Acoustic tweezers for the life sciences. Nat. Methods 2018, 15, 1021–1028. [Google Scholar] [CrossRef] [PubMed]
- Wu, M.; Ozcelik, A.; Rufo, J.; Wang, Z.; Fang, R.; Huang, T.-J. Acoustofluidic separation of cells and particles. Microsyst. Nanoeng. 2019, 5, 32. [Google Scholar] [CrossRef] [PubMed]
- Courtney, C.R.P.; Drinkwater, B.W.; Demore, C.E.M.; Cohran, S.; Grinenko, A. Dexterous manipulation of microparticles using Bessel-function acoustic pressure fields. Appl. Phys. Lett. 2013, 102, 5. [Google Scholar] [CrossRef]
- Whitehill, J.; Neild, A.; Wah, N.T.; Stokes, M. Collection of suspended particles in a drop using low frequency vibration. Appl. Phys. Lett. 2010, 96, 3. [Google Scholar] [CrossRef]
- Oberti, S.; Neild, A.; Quach, R.; Dual, J. The use of acoustic radiation forces to position particles within fluid droplets. Ultrasonics 2010, 49, 47–52. [Google Scholar] [CrossRef]
- Nam, J.; Lee, Y.; Shin, S. Size-dependent microparticles separation through standing surface acoustic waves. Microfluid Nanofluidics 2011, 11, 317–326. [Google Scholar] [CrossRef]
- Park, K.; Park, J.; Jung, J.H.; Destgeer, G.; Ahmed, H.; Sung, H.J. In-droplet microparticle separation using travelling surface acoustic wave. Biomicrofluidics 2017, 11, 64112. [Google Scholar] [CrossRef]
- Rogers, P.; Gralinski, I.; Galtry, C.; Neild, A. Selective particle and cell clustering at air–liquid interfaces within ultrasonic microfluidic systems. Microfluid. Nanofluidics 2013, 14, 469–477. [Google Scholar] [CrossRef]
- Kanazaki, T.; Okada, T. Two-Dimensional particle separation in coupled acoustic-gravity-flow field vertically by composition and laterally by size. Anal. Chem. 2012, 84, 10750–10755. [Google Scholar] [CrossRef]
- Soliman, A.M.; Eldosoky, M.A.; Taha, T.E. The Separation of Blood Components Using Standing Surface Acoustic Waves (SSAWs) Microfluidic Devices: Analysis and Simulation. Bioengineering 2017, 2, 28. [Google Scholar] [CrossRef] [PubMed]
- Mishra, P.; Martyn, H.; Glynne-Jones, P. Deformation of red blood cells using acoustic radiation forces. Biomicrofluidics 2014, 8, 11. [Google Scholar] [CrossRef] [PubMed]
- Korobtsov, A.; Kotova, S.; Losevsky, N.; Mayorova, A.; Patlan, V.; Timchenko, E.; Lysov, N.; Zarubina, E. Optical tweezers technique for the study of red blood cells deformation ability. Laser Phys. 2012, 22, 1265. [Google Scholar] [CrossRef]
- Lenshof, A.; Magnusson, C.; Laurell, T. Acoustofluidics 8: Applications of acoustophoresis in continuous flow microsystems. Lab Chip 2012, 12, 1210. [Google Scholar] [CrossRef]
- Wiklund, M. Acoustofluidic 12: Biocompatibility and cell viability in microfluidic acoustic resonators. Lab Chip 2012, 12, 2018–2028. [Google Scholar] [CrossRef]
- Bazou, D.; Kearney, R.; Mansergh, F.; Bourdon, C.; Farrar, J.; Wride, M. Gene expression analysis of mouse embrionic stem cells following levitation in an ultrasound standing wave trap. Ultrasound Med. Biol. 2011, 37, 321–330. [Google Scholar] [CrossRef]
- Zoueshtiagh, F.; Thomas, P.-J.; Thomy, V.; Merlen, A. Micrometric granular ripple patterns in a capillary tube. Phys. Rev. Lett. 2008, 100, 54501. [Google Scholar] [CrossRef]
- Lerche, D.; Sobisch, T. Consolidation of concentrated dispersions of nano- and microparticles determined by analytical centrifugation. Powder Technol. 2007, 16, 46–49. [Google Scholar] [CrossRef]
- Dijkshoorn, J.P.; Schutyser, M.A.I.; Wagterveld, R.-M.; Schroën, C.-G.-P.-H.; Boom, R.M. A comparison of microfiltration and inertia-based microfluidics for large scale suspension separation. Sep. Purif. Technol. 2017, 173, 86–92. [Google Scholar] [CrossRef]
- Saxena, A.; Bijay, P.; Tripathi, B.P.; Kumar, M.; Shahi, V.K. Membrane-based techniques for the separation and purification of proteins: An overview. Adv. Colloid Interface Sci. 2009, 145, 1–22. [Google Scholar] [CrossRef] [PubMed]
- Devendran, C.; Carthew, J.; Frith, J.E.; Neild, A. Cell Adhesion, Morphology, and Metabolism Variation via Acoustic Exposure within Microfluidic Cell Handling Systems. Adv. Sci. 2019, 6, 1902326. [Google Scholar] [CrossRef] [PubMed]
- Belling, J.N.; Heidenreich, L.K.; Tian, Z.; Mendoza, A.M.; Chiou, T.T.; Gong, Y.; Chen, N.Y.; Young, T.D.; Wattanatorn, N.; Park, J.H.; et al. Acoustofluidic sonoporation for gene delivery to human hematopoietic stem and progenitor cells. Proc. Natl. Acad. Sci. USA 2020, 117, 10976–10982. [Google Scholar] [CrossRef] [PubMed]
- Collins, D.J.; Morahan, B.; Garcia-Bustos, J.; Doerig, C.; Plebanski, M.; Neild, A. Two-dimensional single-cell patterning with one cell per well driven by surface acoustic waves. Nat. Commun. 2015, 6, 8686. [Google Scholar] [CrossRef]
- Mutafopulos, K.; Spink, P.; Lofstrom, C.D.; Lu, P.J.; Lu, H.; Sharpe, J.C.; Franke, T.; Weitz, D.A. Traveling surface acoustic wave (TSAW) microfluidic fluorescence activated cell sorter (μFACS). Lab Chip 2019, 14, 2435–2443. [Google Scholar] [CrossRef]
- Link, A.; Franke, T. Acoustic erythrocytometer for mechanically probing cell viscoelasticity. Lab Chip 2020, 20, 1991–1998. [Google Scholar] [CrossRef]
- Ostasevicius, V.; Jurenas, V.; Golinka, E.; Gaidys, R.; Aleksa, A. Separation of microparticles from suspension utilizing ultrasonic standing waves in a piezoelectric cylinder actuator. Actuators 2018, 7, 14. [Google Scholar] [CrossRef]
- Settnes, M.; Bruus, H. Forces acting on a small particle in an acoustical field in a viscous fluid. Phys. Rev. E 2012, 85, 16327. [Google Scholar] [CrossRef]
- Gor’kov, L.P. On the forces acting on a small particle in an acoustical field in an ideal fluid. Sov. Phys. Dokl. 1962, 6, 773. [Google Scholar]
- Ostasevicius, V.; Jurenas, V.; Gaidys, R.; Kizauskiene, L. Acoustic Suspension Separation Device. LT Patent Unpublished Patent. 2021. [Google Scholar]
- Otterstedt, J.E.; Brandreth, D.A. Small Particles Technology; Springer: New York, NY, USA, 2010; p. 524. [Google Scholar]
- Turchiano, M.; Nguyen, C.; Fierman, A.; Lifshitz, M.; Convit, A. Impact of blood sample collection and processing methods on glucose levels in community outreach studies. J. Environ. Public Health 2013, 2013, 256151. [Google Scholar] [CrossRef] [PubMed]
- Stegmayr, B.G. A survey of blood purification techniques. Transfus. Apheresis Sci. 2005, 32, 209–220. [Google Scholar] [CrossRef] [PubMed]
- Muller, P.B.; Barnkob, R.; Jensen, M.J.H.; Bruus, H. A numerical study of microparticle acoustophoresis driven by acoustic radiation forces and streaming-induced drag forces. Lab Chip 2012, 22, 4617–4627. [Google Scholar] [CrossRef] [PubMed]
- Devendran, C.; Albrecht, T.; Brenker, J.; Alan, T.; Neild, A. The importance of travelling wave components in standing surface acoustic wave (SSAW) systems. Lab Chip 2016, 16, 3756–3766. [Google Scholar] [CrossRef] [PubMed]




| Resonant Frequency, kHz | 2.8 ± 0.5 kHz |
|---|---|
| Resonant Impedance, Ω | 200 max. |
| Capacitance, pF | 30.0 ± 30% [1 kHz] |
| Operational voltage (RMS), V | 30 |
| Plate/piezo diameters, mm | 35/25 |
| Plate/piezo thickness, mm | 0.30/0.23 |
| Plate material | Brass |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ostasevicius, V.; Jurenas, V.; Gaidys, R.; Golinka, I.; Kizauskiene, L.; Mikuckyte, S. Development of a Piezoelectric Actuator for Separation and Purification of Biological Microparticles. Actuators 2020, 9, 61. https://doi.org/10.3390/act9030061
Ostasevicius V, Jurenas V, Gaidys R, Golinka I, Kizauskiene L, Mikuckyte S. Development of a Piezoelectric Actuator for Separation and Purification of Biological Microparticles. Actuators. 2020; 9(3):61. https://doi.org/10.3390/act9030061
Chicago/Turabian StyleOstasevicius, Vytautas, Vytautas Jurenas, Rimvydas Gaidys, Ievgeniia Golinka, Laura Kizauskiene, and Sandra Mikuckyte. 2020. "Development of a Piezoelectric Actuator for Separation and Purification of Biological Microparticles" Actuators 9, no. 3: 61. https://doi.org/10.3390/act9030061
APA StyleOstasevicius, V., Jurenas, V., Gaidys, R., Golinka, I., Kizauskiene, L., & Mikuckyte, S. (2020). Development of a Piezoelectric Actuator for Separation and Purification of Biological Microparticles. Actuators, 9(3), 61. https://doi.org/10.3390/act9030061




