Electromechanical Actuation for Morphing Winglets
Abstract
1. Introduction
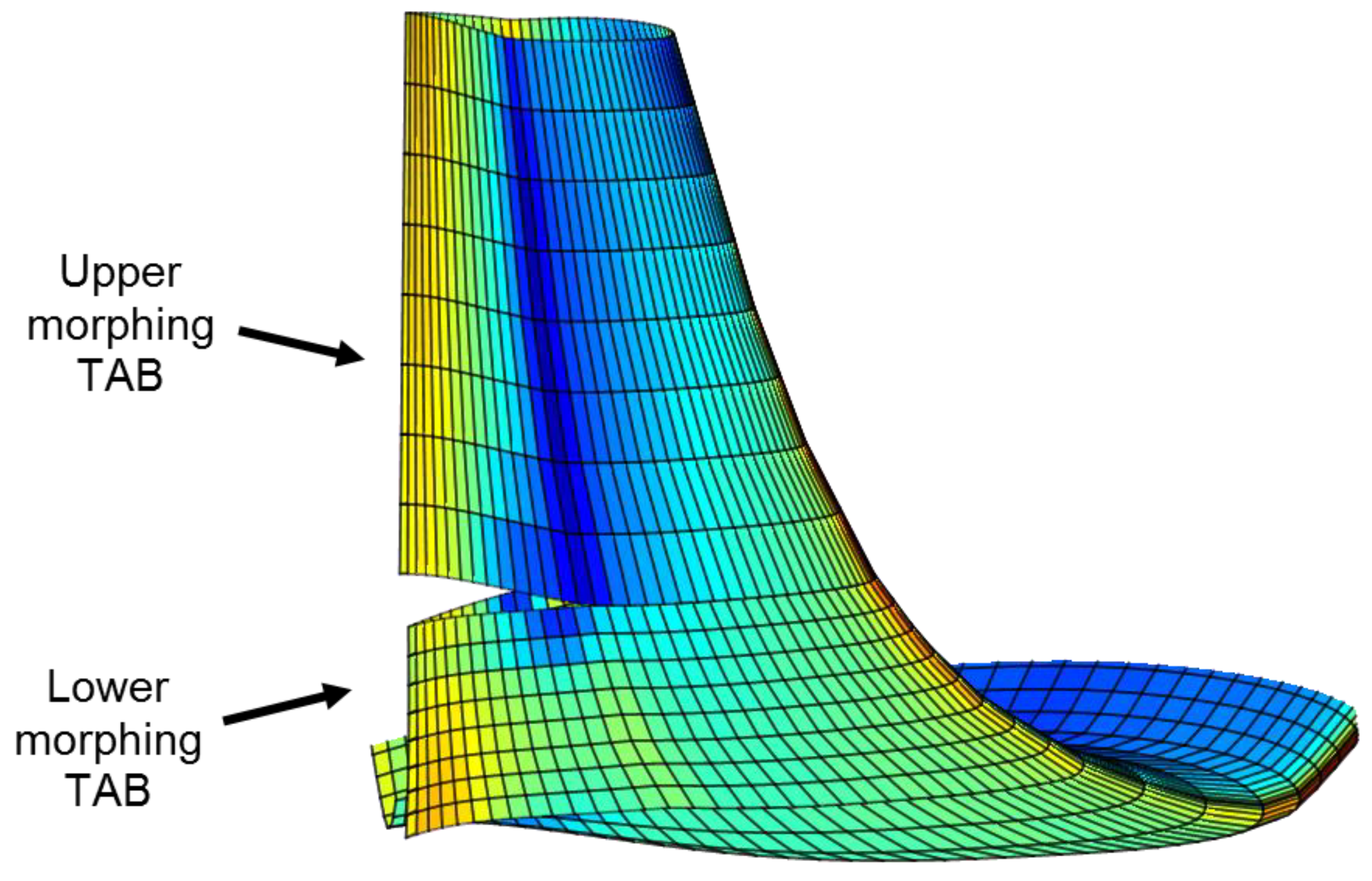
2. Morphing Winglet Concept
Morphing Tabs Mechanisms
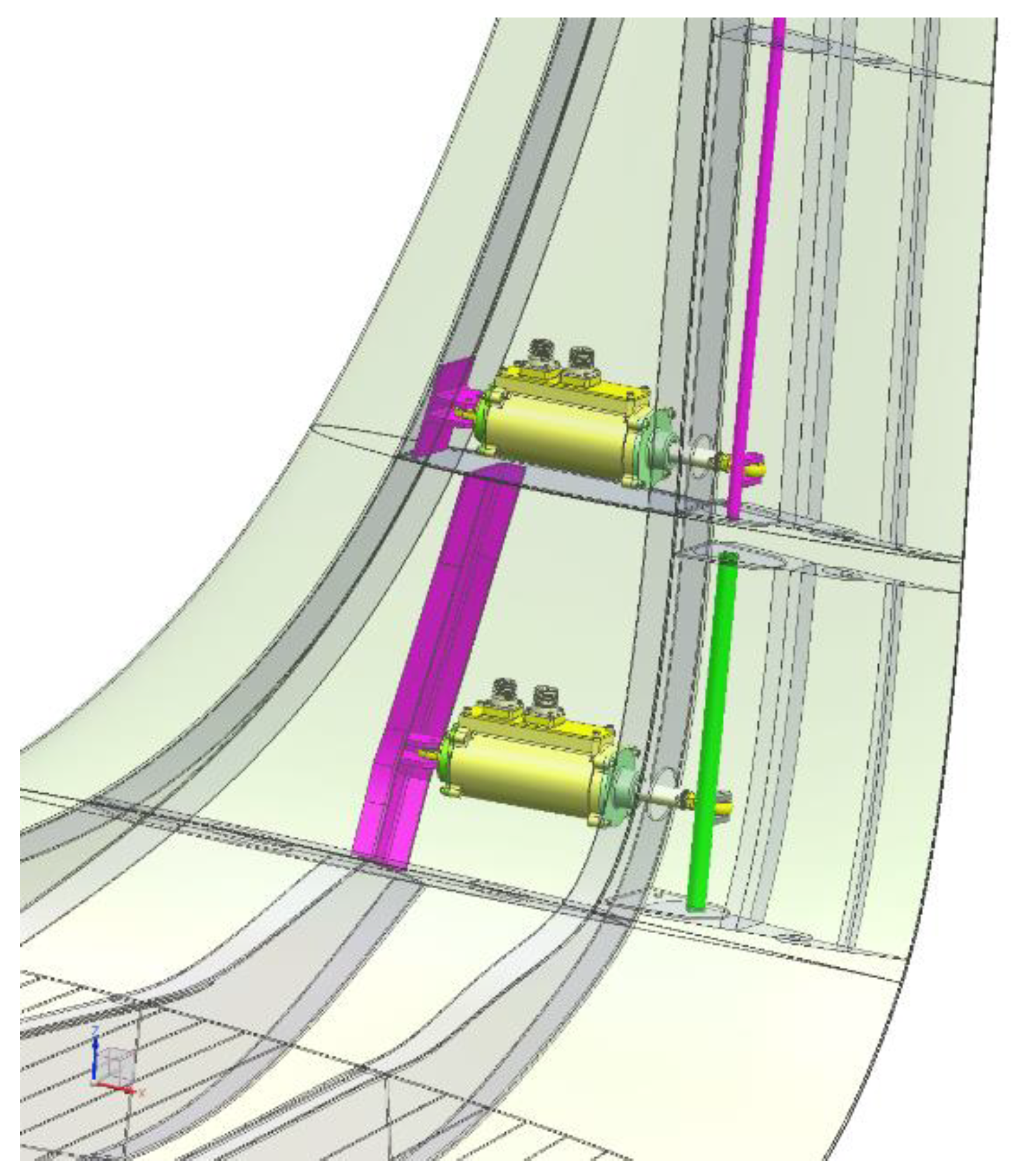
3. Actuators and Kinematics Integration
3.1. Electromechanical Actuation Concept
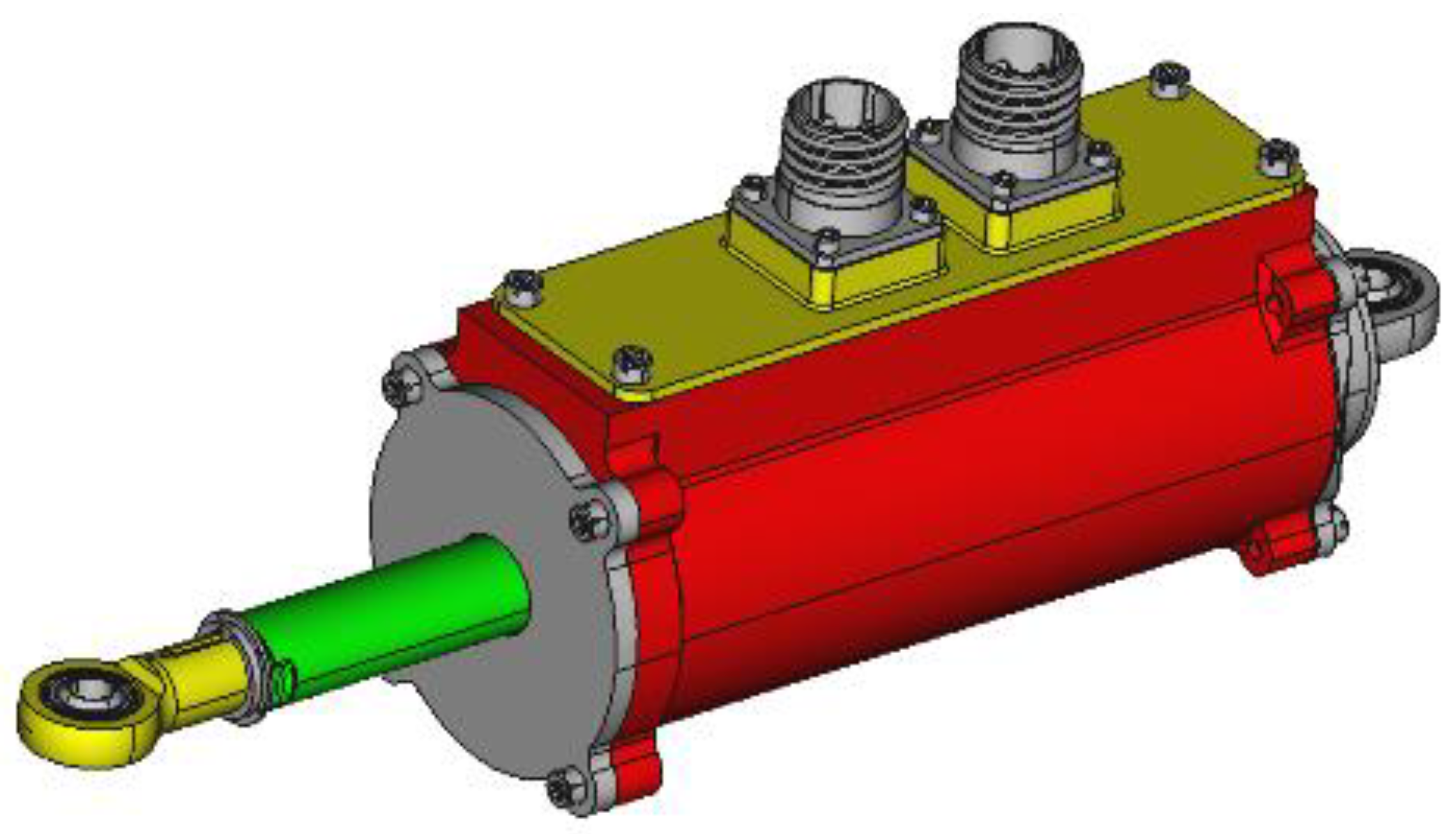
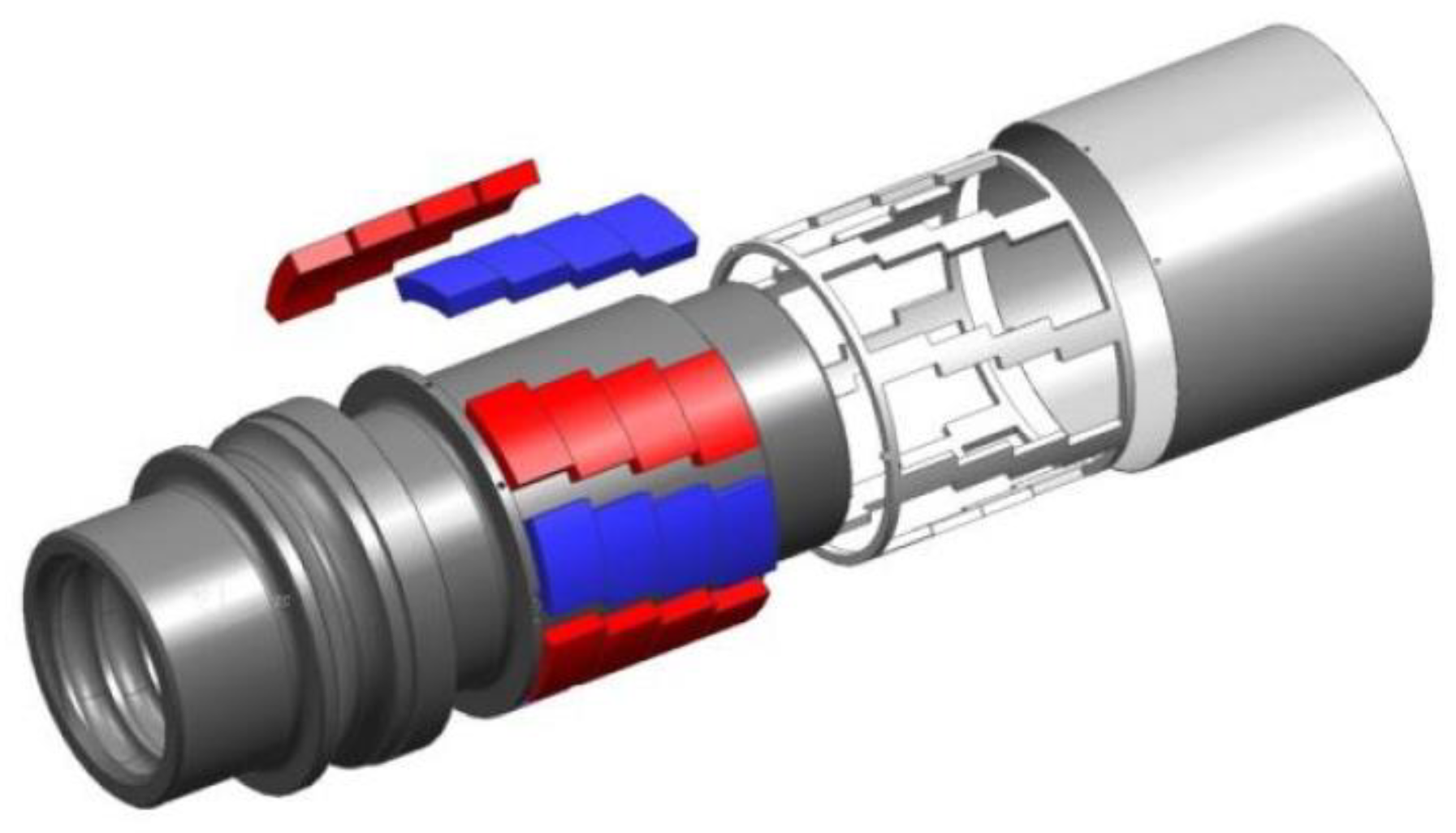
3.2. Electromechanical Actuators’ Components
- ■
- Low reflected inertia from the motor rotor to the load due to the low gear ratio;
- ■
- Small number of components for greater reliability and easier assembly;
- ■
- High efficiency due to the lower number of transmission stages;
- ■
- Thermal stability;
- ■
- Reduced maintenance;
- ■
- Reduced noise due to absence of gears and reduced friction between the ball-nut, balls; and screw shaft;
- ■
- Increased accuracy due to the lower number of transmission stages;
- ■
- Reduced irreversibility load;
- ■
- Lower backlash.
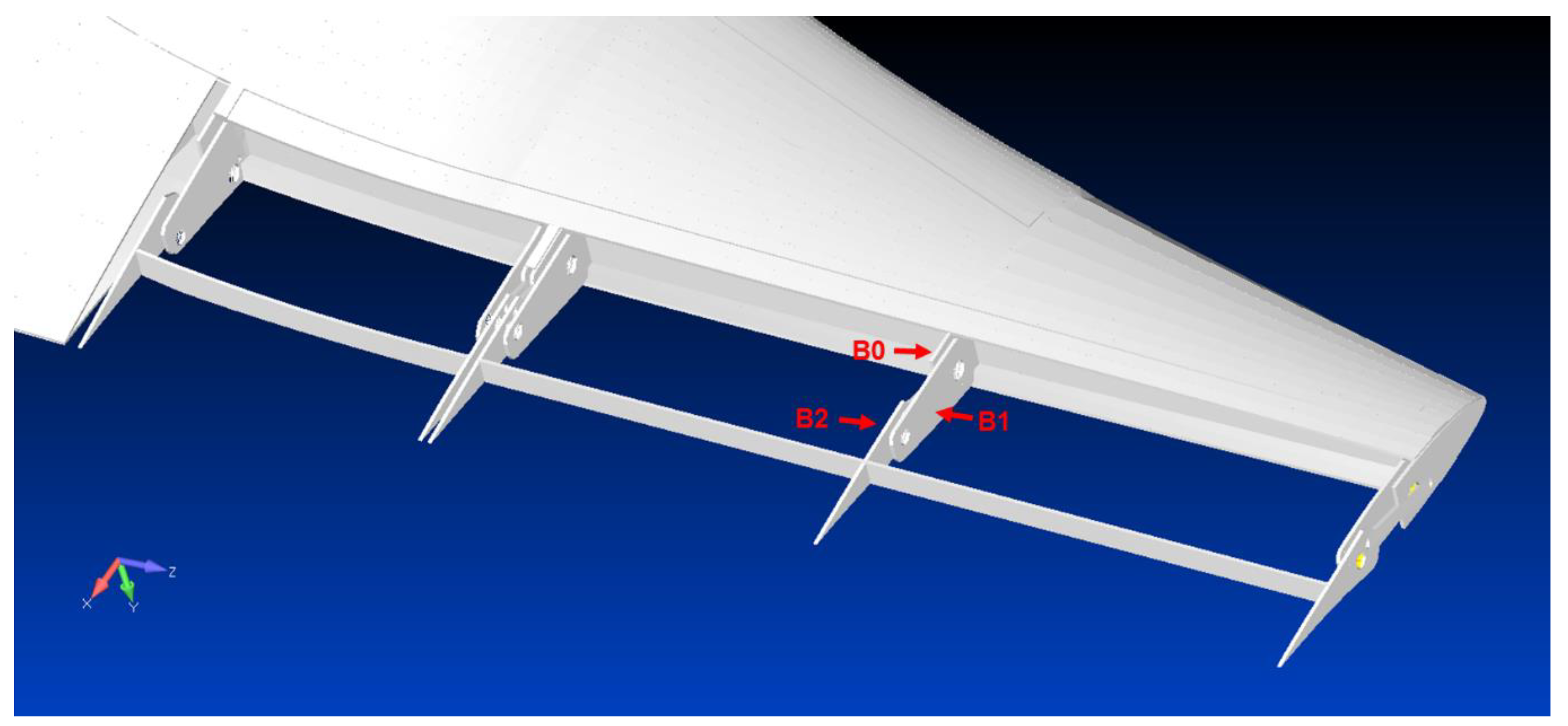
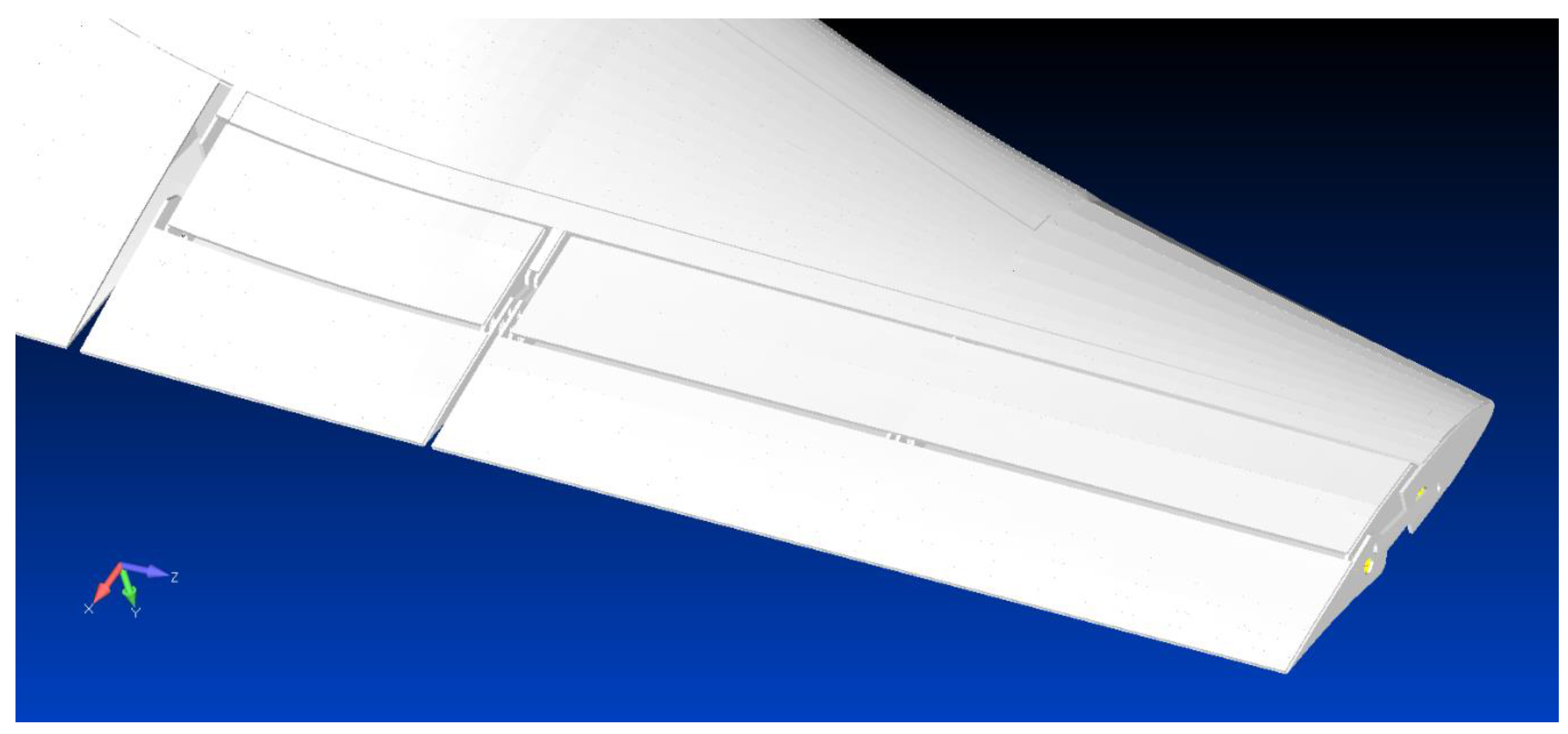
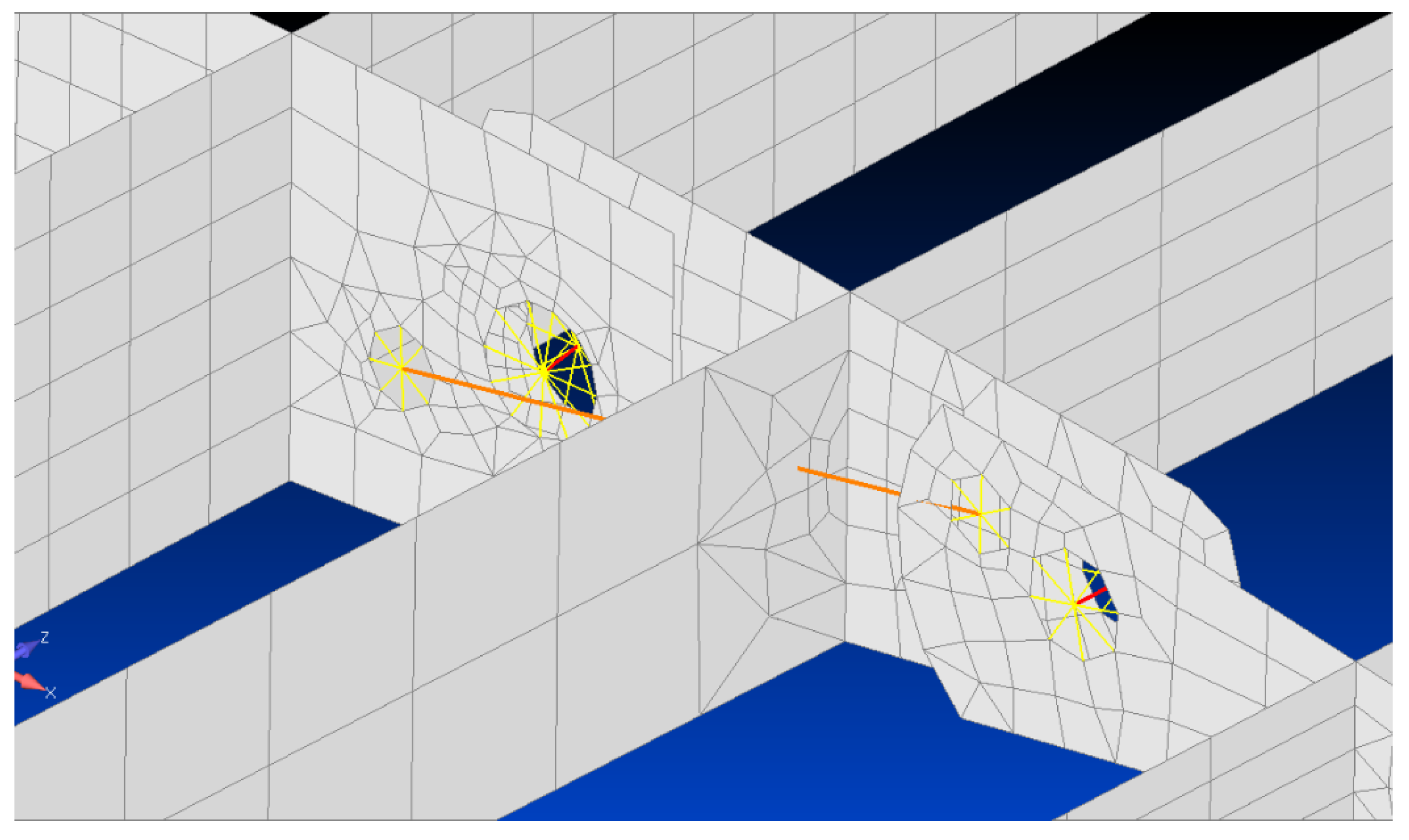
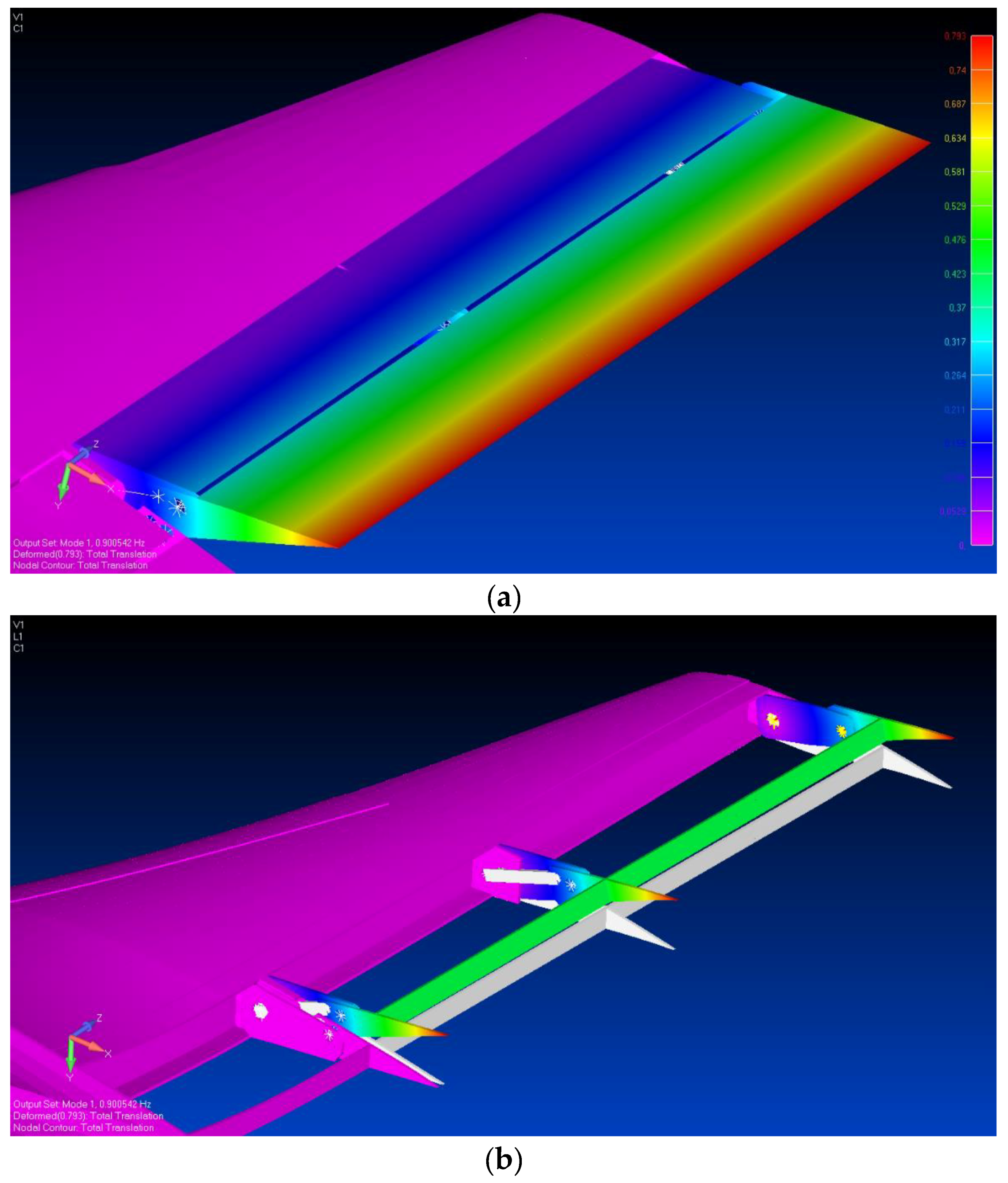
4. Actuators and Kinematics Integration into the FE Model
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Concilio, A.; Dimino, I.; Lecce, L.; Pecora, R. Morphing Wings Technology for Large Commercial Aircraft and Helicopter Scenario; Butterworth-Heinemann: Oxford, UK, 2017; 978p, ISBN 978-0-08-100964-2. [Google Scholar] [CrossRef]
- Woelcken, P.C.; Papadopoulos, M. Smart Intelligent Aircraft Structures (SARISTU); Springer International Publishing: Cham, Switzerland, 2016; ISBN 978-3-319-22413-8. [Google Scholar]
- Barbarino, S.; Bilgen, O.; Ajaj, R.M.; Friswell, M.I.; Inman, D.J. A Review of Morphing Aircraft. J. Intell. Mater. Syst. Struct. 2011, 22, 823–877. [Google Scholar] [CrossRef]
- Bishay, P.L.; Burg, E.; Akinwunmi, A.; Phan, R.; Sepulveda, K. Development of a new span-morphing wing core design. Designs 2019, 3, 12. [Google Scholar] [CrossRef]
- Fichera, S.; Isnardi, I.; Mottershead, J.E. High-Bandwidth Morphing Actuator for Aeroelastic Model Control. Aerospace 2019, 6, 13. [Google Scholar] [CrossRef]
- Mills, J.; Ajaj, R. Flight Dynamics and Control Using Folding Wingtips: An Experimental Study. Aerospace 2017, 4, 19. [Google Scholar] [CrossRef]
- Bendsøe, M.P.; Sigmund, O. Topology Optimization—Theory, Methods, and Applications; Springer: Heidelberg/Berlin, Germany, 2003. [Google Scholar]
- Amendola, G.; Dimino, I.; Concilio, A.; Andreutti, G.; Pecora, R.; Lo Cascio, M. Preliminary design process for an adaptive winglet. Int. J. Mech. Eng. Robot. Res. 2018, 7, 83–92. [Google Scholar] [CrossRef]
- Pecora, R.; Amoroso, F.; Magnifico, M.; Dimino, I.; Concilio, A. KRISTINA: Kinematic rib-based structural system for innovative adaptive trailing edge. In Proceedings of the SPIE—The International Society for Optical Engineering, Las Vegas, NV, USA, 20–24 March 2016. [Google Scholar]
- Zhao, A.; Zou, H.; Jin, H.; Wen, D. Structural design and verification of an innovative whole adaptive variable camber wing. Aerosp. Sci. Technol. 2019, 89, 11–18. [Google Scholar] [CrossRef]
- Aage, N.; Andreassen, E.; Lazarov, B.S.; Sigmund, O. Giga-voxel computational morphogenesis for structural design. Nature 2017, 550, 84–86. [Google Scholar] [CrossRef]
- Arena, M.; Nagel, C.; Pecora, R.; Schorsch, O.; Concilio, A.; Dimino, I. Static and Dynamic Performance of a Morphing Trailing Edge Concept with High-Damping Elastomeric Skin. Aerospace 2019, 6, 22. [Google Scholar] [CrossRef]
- Dimino, I.; Concilio, A.; Pecora, R. Safety and Reliability Aspects of an Adaptive Trailing Edge Device (ATED). In Proceedings of the 24th AIAA/AHS Adaptive Structures Conference, San Diego, CA, USA, 4–8 January 2016. [Google Scholar]
- Amendola, G.; Dimino, I.; Magnifico, M.; Pecora, R. Distributed Actuation Concepts for a Morphing Aileron device. Aeronaut. J. 2016, 120, 1365–1385. [Google Scholar] [CrossRef]
- Amendola, G.; Dimino, I.; Amoroso, F.; Pecora, R. Experimental characterization of an Adaptive Aileron: Lab tests and FE correlation. In Sensors and Smart Structures Technologies for Civil, Mechanical, and Aerospace Systems; SPIE Smart Structures/NDE: Las Vegas, NV, USA, 2016; Volume 9803, 98034p. [Google Scholar] [CrossRef]
- Della Vecchia, P.; Corcione, S.; Pecora, R.; Nicolosi, F.; Dimino, I.; Concilio, A. Design and integration sensitivity of a morphing trailing edge on a reference airfoil: The effect on high-altitude long-endurance aircraft performance. J. Intell. Mater. Syst. Struct. 2017, 28, 2933–2946. [Google Scholar] [CrossRef]
- Dimino, I.; Diodati, G.; Concilio, A.; Volovick, A.; Zivan, L. Distributed electromechanical actuation system design for a morphing trailing edge wing. In Industrial and Commercial Applications of Smart Structures Technologies; SPIE Smart Structures/NDE: Las Vegas, NV, USA, 2016; Volume 9801, p. 980108. [Google Scholar] [CrossRef]
- Dimino, I.; Flauto, D.; Diodati, G.; Concilio, A.; Pecora, R. Actuation System Design for a Morphing Wing Trailing Edge. Recent Pat. Mech. Eng. 2014, 7, 138–148. [Google Scholar] [CrossRef]
- Diodati, G.; Concilio, A.; Ricci, S.; De Gaspari, A.; Huvelin, F.; Dumont, A.; Godard, J.-L. Estimated Performances of an Adaptive Trailing Edge Device Aimed at Reducing Fuel Consumption on a Medium-Size Aircraft. In Proceedings of the SPIE 20th Annual Symposium on Smart Structures and Materials, San Diego, CA, USA, 10–14 March 2013. [Google Scholar]
- Pecora, R.; Dimino, I. SMA for Aeronautics. In Shape Memory Alloy Engineering for Aerospace, Structural and Biomedical Applications; Butterworth-Heinemann: Oxford, UK, 2015; pp. 275–304. [Google Scholar] [CrossRef]
- Bishay, P.L.; Finden, R.; Recinos, S.; Alas, C.; Lopez, E.; Aslanpour, D.; Flores, D.; Gonzalez, E. Development of an SMA-based camber morphing UAV tail core design. In Smart Materials and Structures; IOP Publishing: Bristol, UK, 2019. [Google Scholar] [CrossRef]
- Systems. Available online: https://www.cleansky.eu/systems (accessed on 5 May 2019).
- Gavrilovic, N.; Rasuo, B.; Dulikravich, G.; Parezanovic, V. Commercial Aircraft Performance Improvement Using Winglets. FME Trans. 2015, 43, 1–8. [Google Scholar] [CrossRef]
- Allen, J.B. Articulating Winglets. US patent 5,988,563 A, 23 November 1999. [Google Scholar]
- Irving, J.; Davies, R. Wing Tip Device. US patent 7,275,722 B2, 2 October 2007. [Google Scholar]
- Wildschek, A.; Storm, S.; Herring, M.; Drezga, D.; Korian, V.; Roock, O. Design, Optimization, Testing, Verification, and Validation of the Wingtip Active Trailing Edge. In Smart Intelligent Aircraft Structures (SARISTU); Springer: Cham, Switzerland, 2016; pp. 219–255. [Google Scholar]
- Amendola, G.; Dimino, I.; Concilio, A.; Magnifico, M.; Pecora, R. Numerical design of an adaptive aileron. In Proceedings of SPIE—The International Society for Optical Engineering; SPIE: Las Vegas, NV, USA, 2016; Volume 9803, p. 98032A. ISBN 9781510600447. [Google Scholar] [CrossRef]
- Amendola, G.; Dimino, I.; Concilio, A.; Amoroso, F.; Pecora, R. Preliminary design of an adaptive aileron for the next generation regional aircraft. J. Theor. Appl. Mech. 2017, 55, 307–316. [Google Scholar] [CrossRef]
- Abraham, T. Magnets and magnetic materials: A technical economic analysis. J. Miner. Met. Mater. Soc. 1995, 47, 16–18. [Google Scholar] [CrossRef]
- Kouvel, J.S. Intermetallic Compounds; Westbrook, J.H., Ed.; Wiley: New Jersey, NJ, USA, 1967; pp. 529–568. [Google Scholar]
- Stadelmaier, H.H.; Reinsch, B. Intermetallic Compounds—Magnetic, Electrical and Optical Properties and Applications of Intermetallic Compounds; Westbrook, J.H., Fleischer, R.L., Eds.; John Wiley & Sons: Hoboken, NJ, USA, 2000; pp. 31–50. [Google Scholar]
- Sundar, R.S.; Deevi, S.C. Soft Magnetic FeCo Alloys: Alloy Development, Processing, and Properties. Int. Mater. Rev. 2005, 50, 157–192. [Google Scholar] [CrossRef]
- Zetterstrom, S. Electromechanical steering, suspension, drive and brake modules. In Proceedings of the IEEE 56th Vehicular Technology Conference, Vancouver, BC, Canada, 24–28 September 2002; Volume 3, pp. 1856–1863. [Google Scholar]
- Maron, C.; Dieckmann, T.; Hauck, S.; Prinzler, H. Electromechanical Brake System: Actuator Control Development System; SAE Technical Paper; SAE International: Michigan, MI, USA, 1997. [Google Scholar]
- Schwarz, R.; Isermann, R.; Böhm, J.; Nell, J.; Rieth, P. Modeling and Control of an Electromechanical Disk Brake; SAE Technical Paper 980600; SAE International: Michigan, MI, USA, 1998. [Google Scholar]
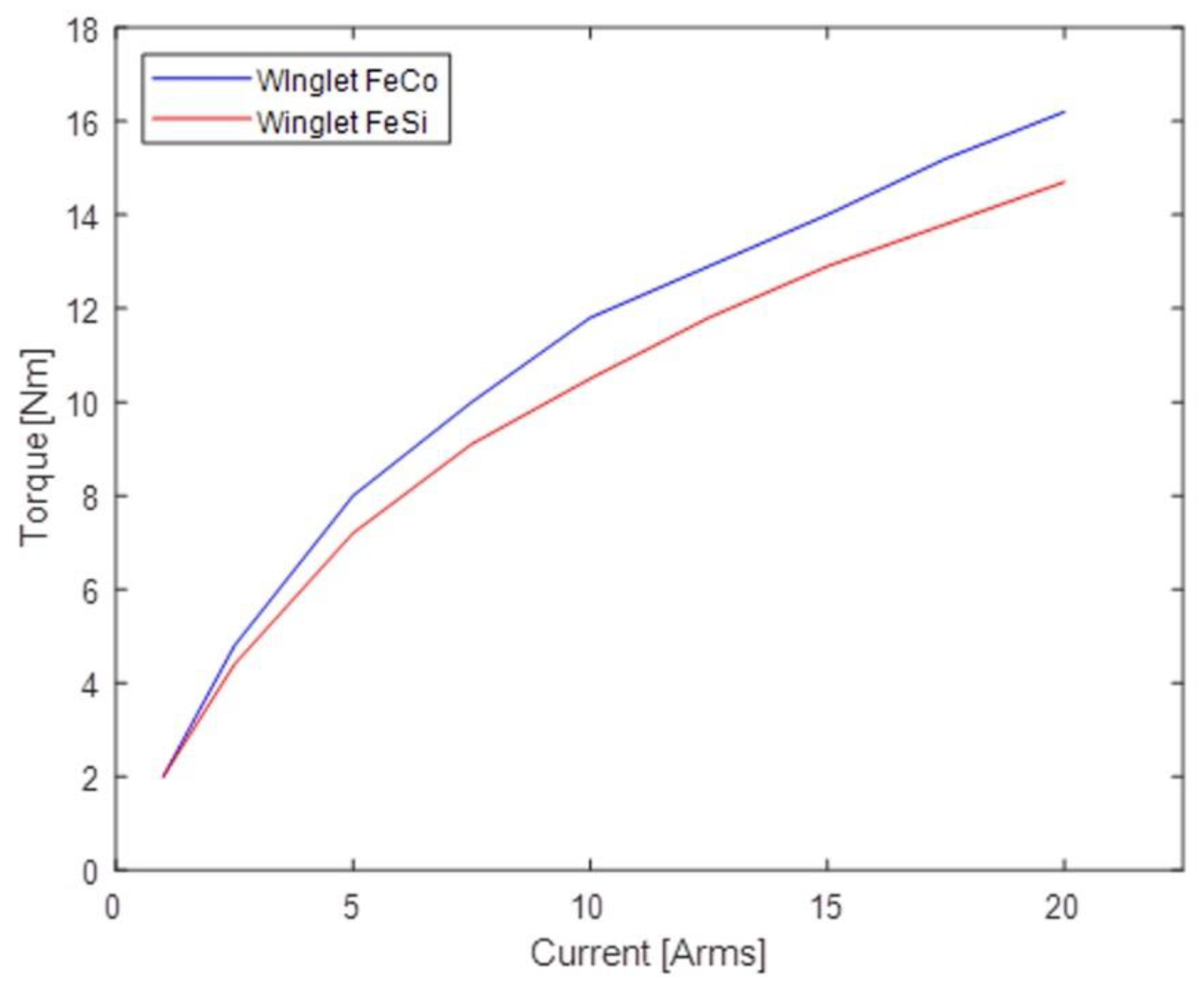

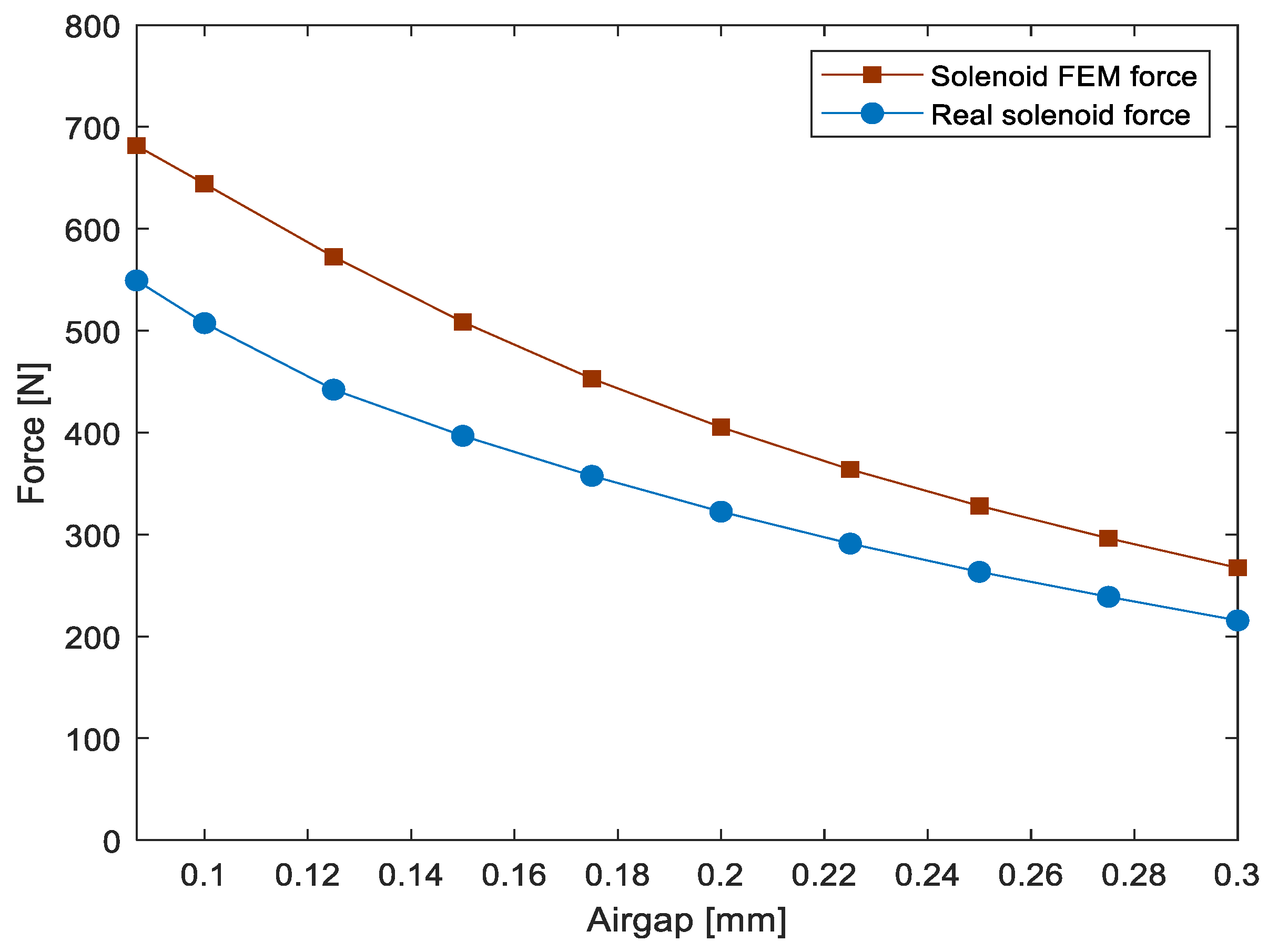
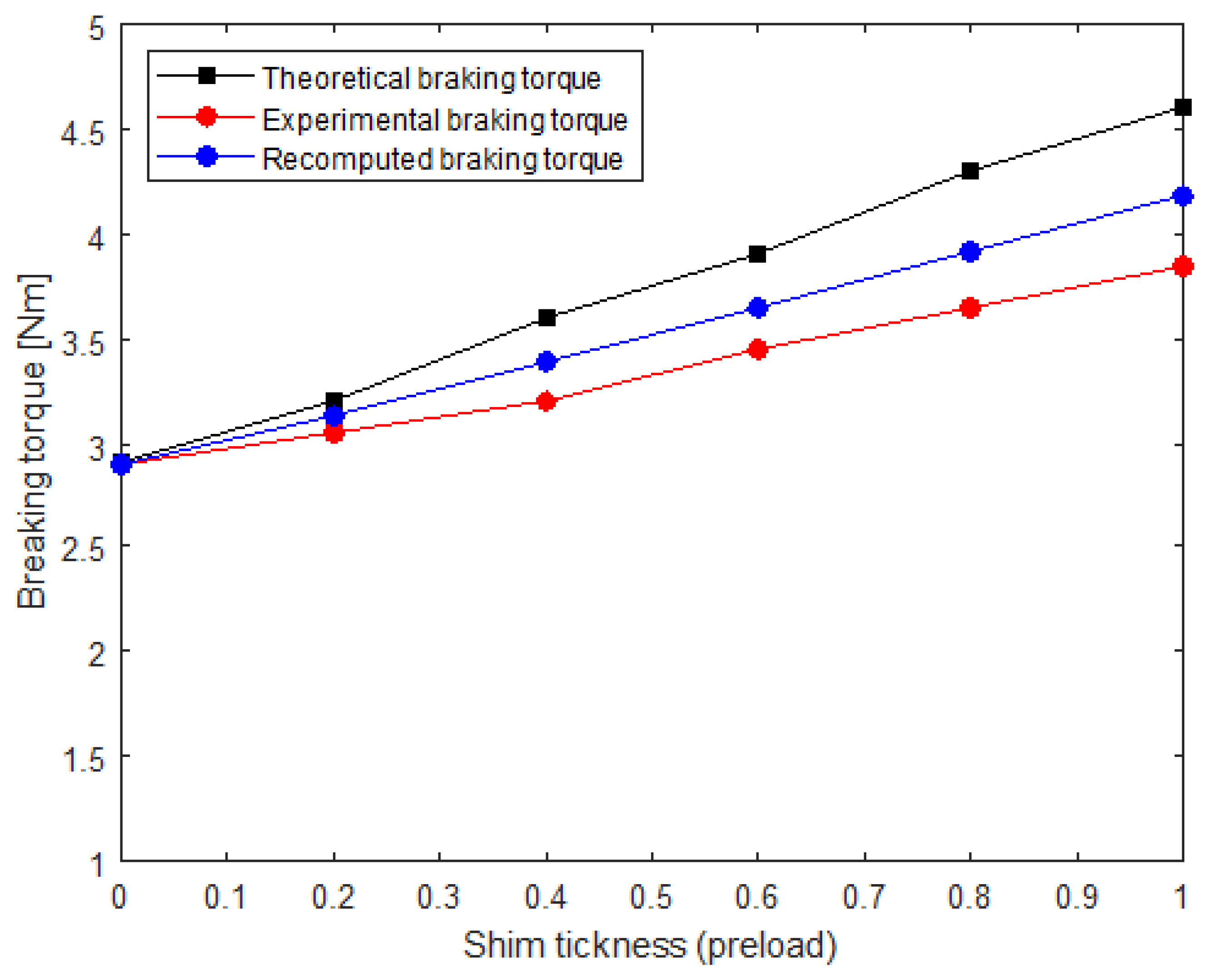
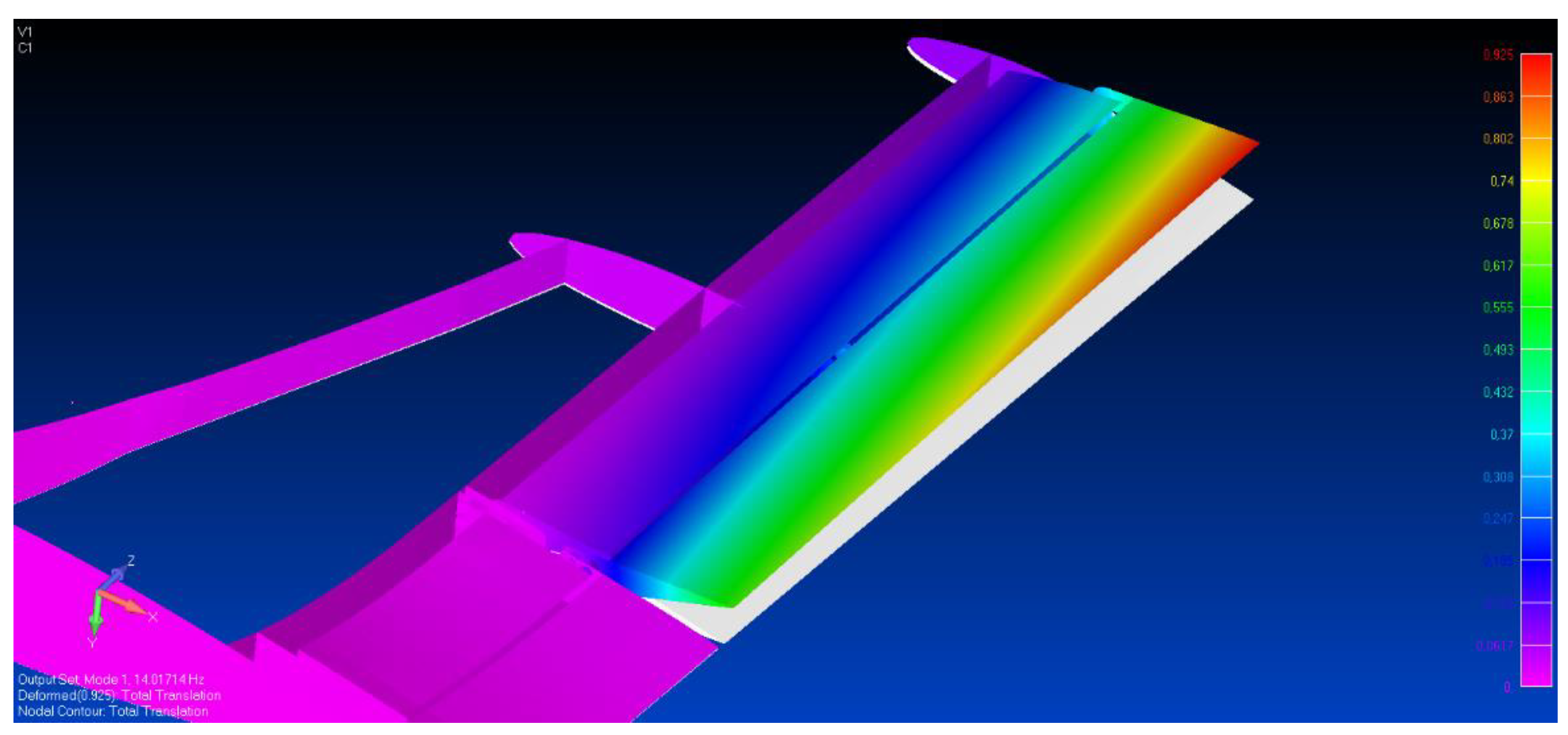

| Parameter | Value |
|---|---|
| Maximum operating axial load | 3500 N |
| Maximum static axial load | 5000 N |
| Total operating stroke | 10 mm for the lower surface 20 mm for the upper surface |
| Max speed | 5 mm/s |
| Feature | Standard Direct-Drive Configuration | Winglet Direct-Drive Configuration | % Increase |
|---|---|---|---|
| Power-to-weight ratio in discontinuous operation point | 175 W/kg | 205 W/kg | 17% |
| Power-to-volume ratio in discontinuous operation point | 925 W/L | 1700 W/L | 84% |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dimino, I.; Gallorini, F.; Palmieri, M.; Pispola, G. Electromechanical Actuation for Morphing Winglets. Actuators 2019, 8, 42. https://doi.org/10.3390/act8020042
Dimino I, Gallorini F, Palmieri M, Pispola G. Electromechanical Actuation for Morphing Winglets. Actuators. 2019; 8(2):42. https://doi.org/10.3390/act8020042
Chicago/Turabian StyleDimino, Ignazio, Federico Gallorini, Massimiliano Palmieri, and Giulio Pispola. 2019. "Electromechanical Actuation for Morphing Winglets" Actuators 8, no. 2: 42. https://doi.org/10.3390/act8020042
APA StyleDimino, I., Gallorini, F., Palmieri, M., & Pispola, G. (2019). Electromechanical Actuation for Morphing Winglets. Actuators, 8(2), 42. https://doi.org/10.3390/act8020042






