Electromagnetic Levitation Control for Bending Flexible Steel Plate: Experimental Consideration on Disturbance Cancellation Control
Abstract
:1. Introduction
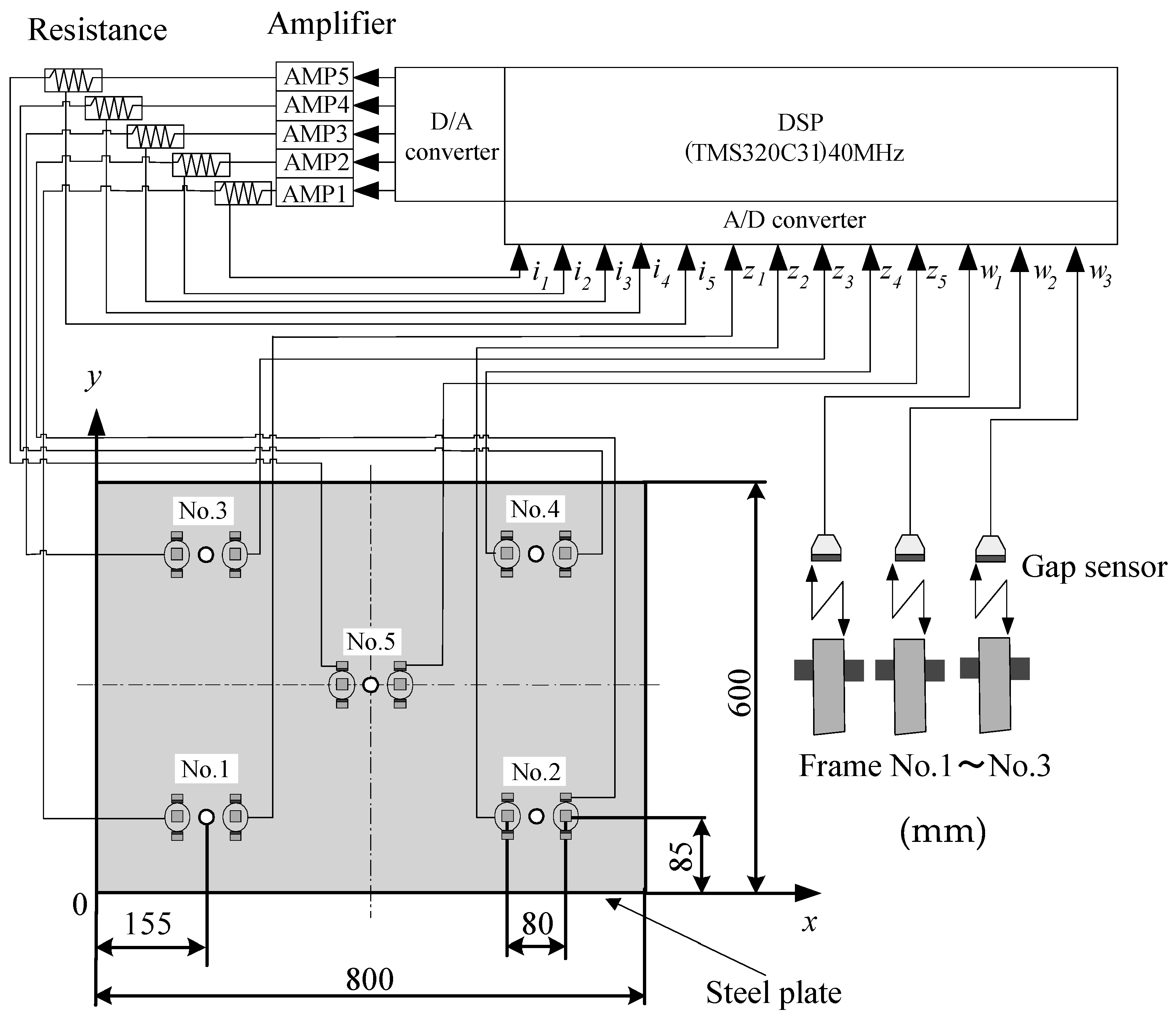
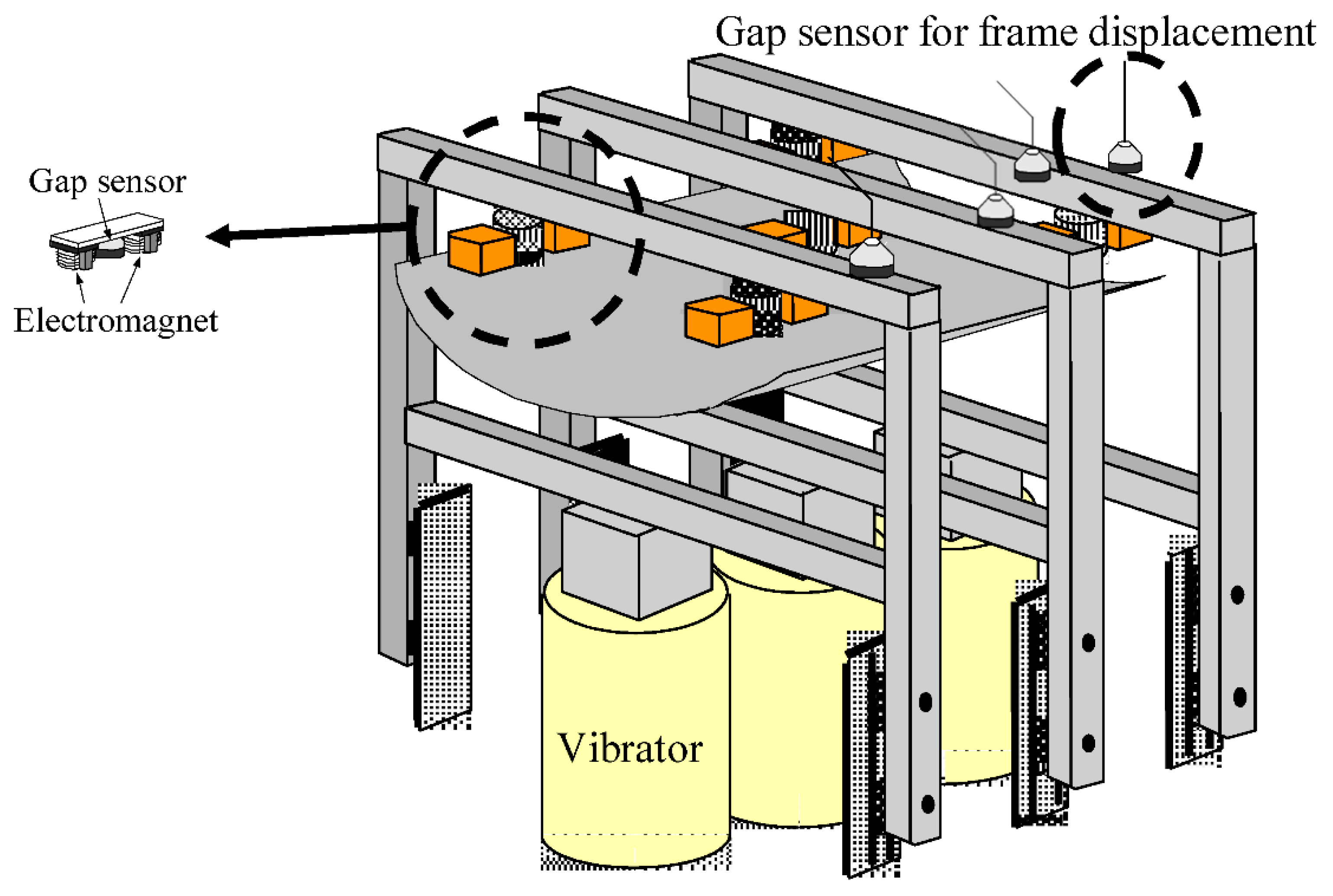
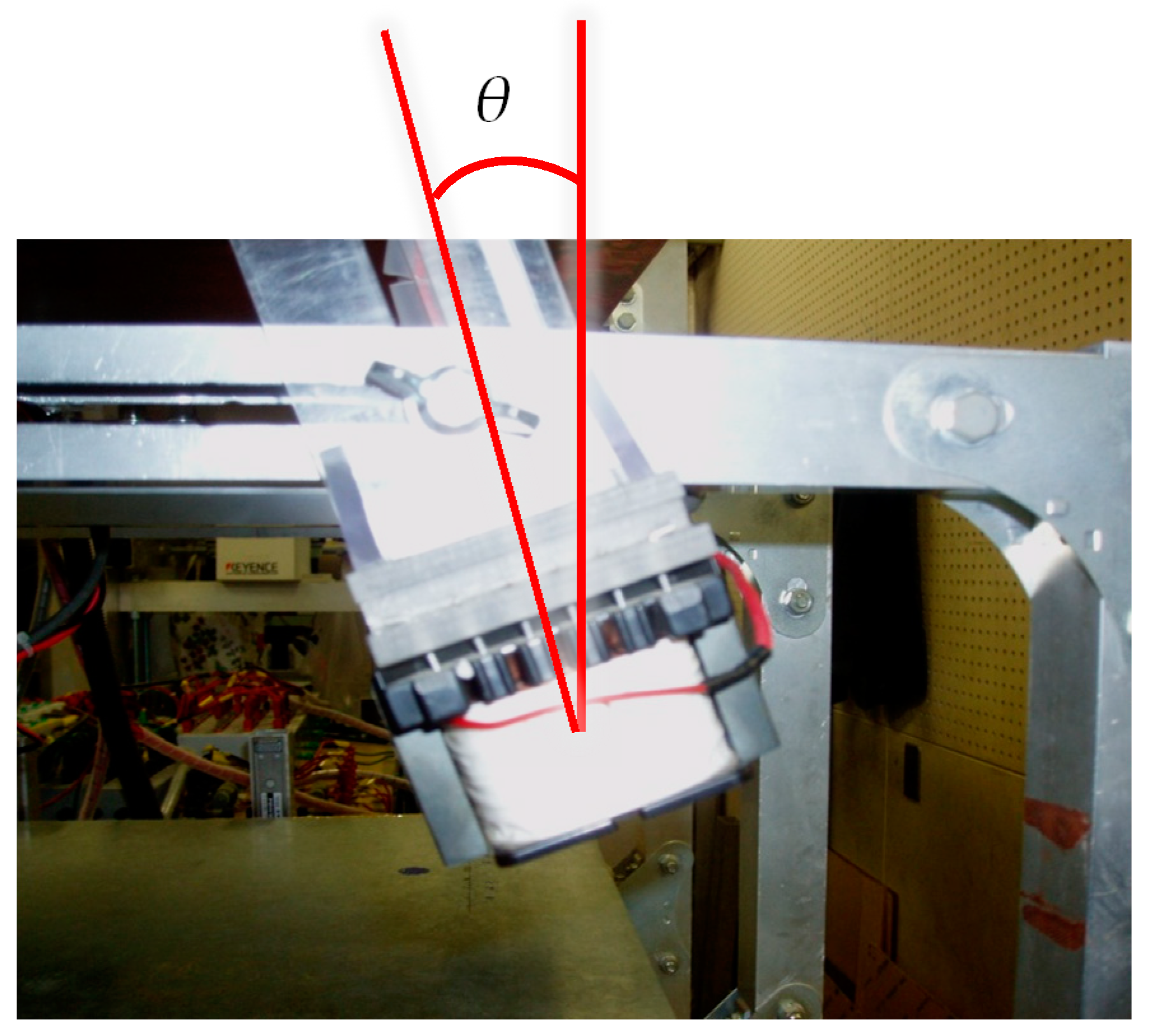
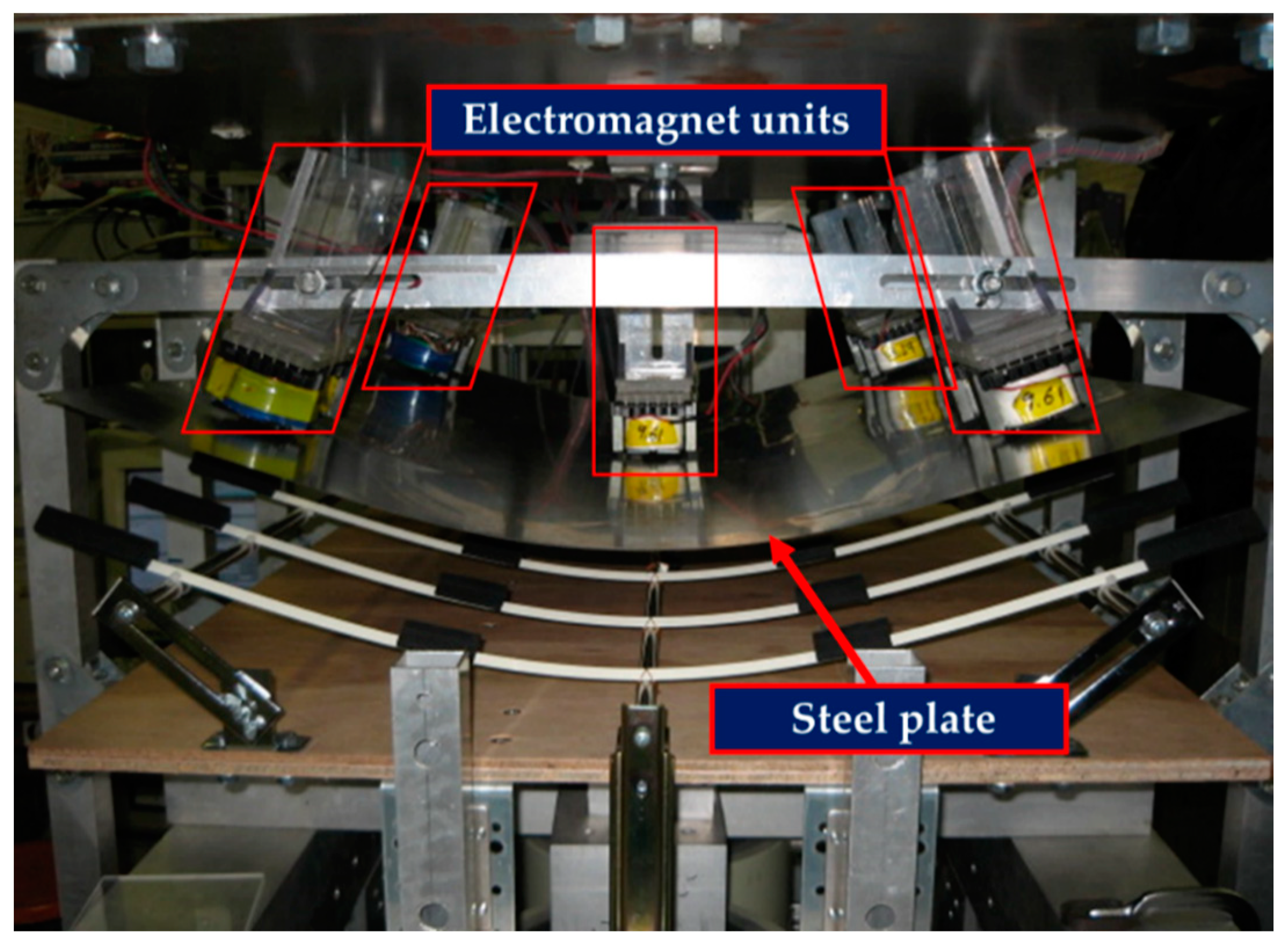
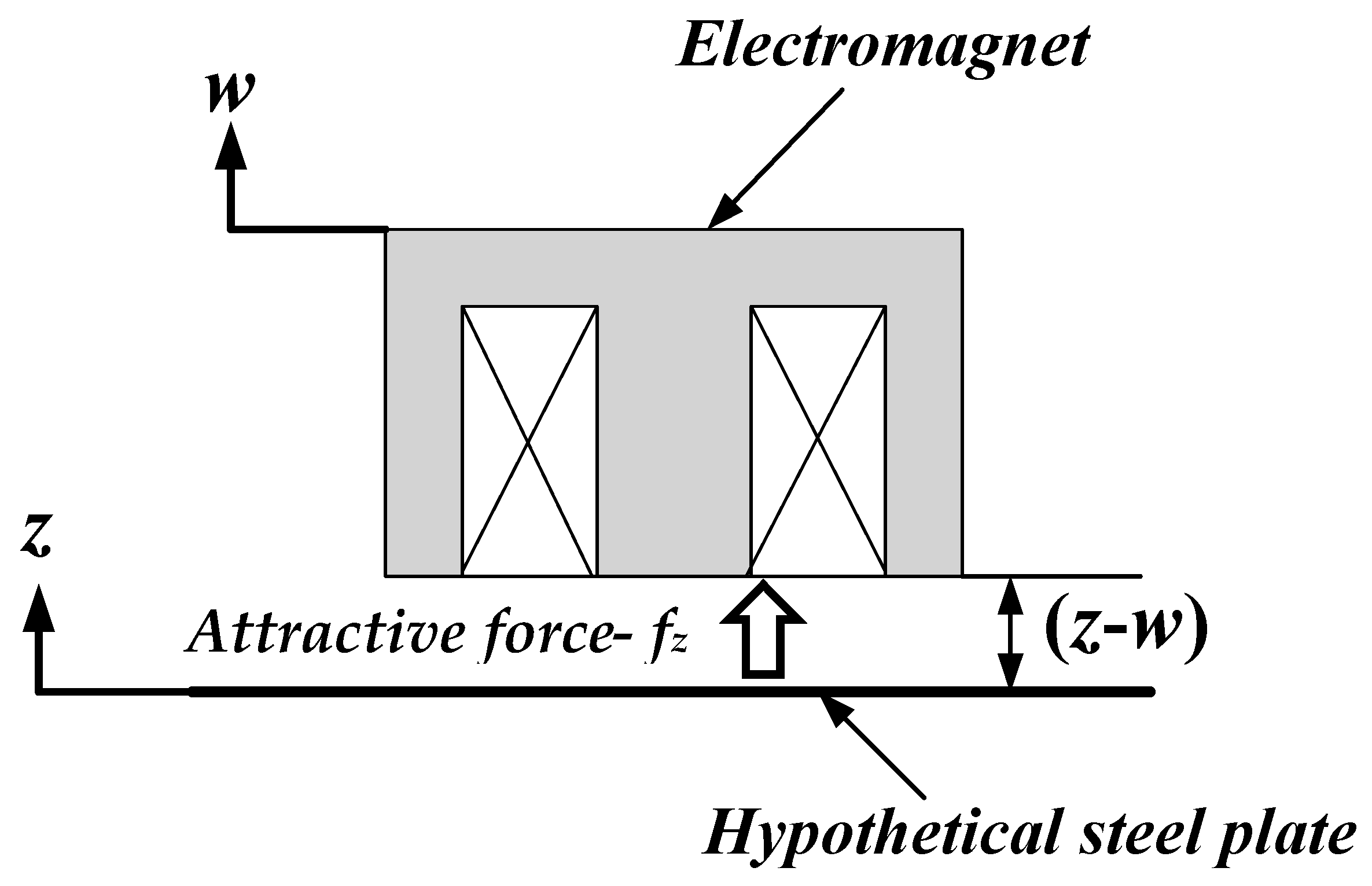
2. System for Control Experiment
3. Equation of Motion
4. State Equation
5. Control Theory
5.1. Optimal Control
5.2. Disturbance Cancellation Control
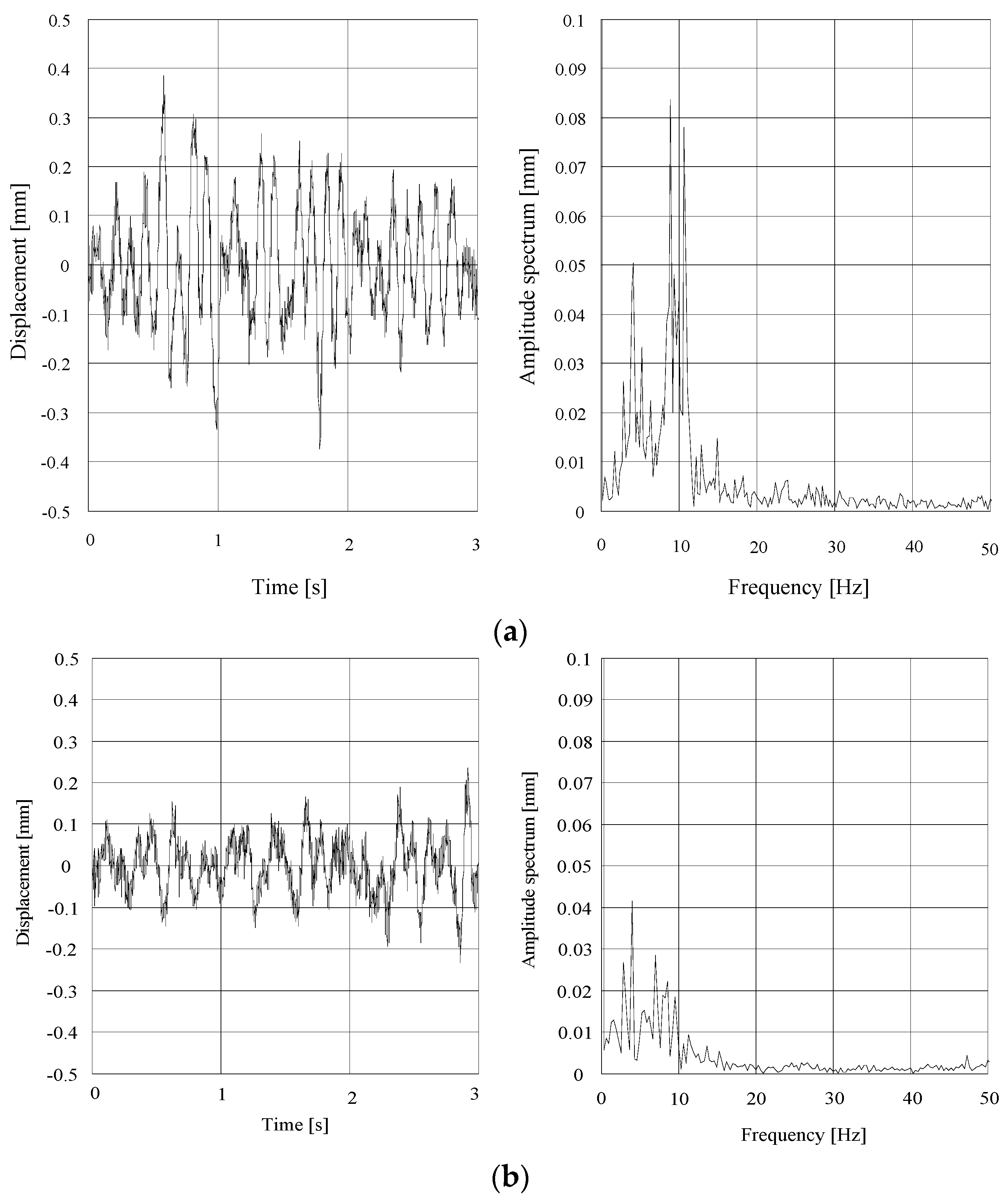
6. Levitation Experiment
7. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Carl, R.K.; Samir, M.T. Robust Adaptive Control of Unbalance Response for a Flexible Rotor. JSME Int. J. Ser. C Mech. Syst. Mach. Elem. Manuf. 1997, 40, 599–606. [Google Scholar]
- Kim, C.; Kim, K.; Yu, J.; Cho, H. Dynamic performance evaluation of 5-DOF magnetic levitation and guidance device by using equivalent magnetic circuit model. IEEE Trans. Magn. 2013, 49, 4156–4159. [Google Scholar] [CrossRef]
- Perini, E.; Giunchi, G.; Geri, M.; Morandi, A. Experomental and Numerical Investigation of the Levitaton Force between Bilk Permanent Magnet and Mg B2 Disk. IEEE Trans. Appl. Supercond. 2009, 19, 2124–2128. [Google Scholar] [CrossRef]
- Javed, A.; Mizuno, T.; Takasaki, M.; Ishino, Y.; Hara, M.; Yamaguchi, D. Lateral Vibration Suppression by Varying Stiffness Control in a Vertically Active Magnetic Suspension System. Actuators 2018, 7, 21. [Google Scholar] [CrossRef]
- Matsumoto, S.; Arai, Y.; Nakagawa, T. Noncontact Levitation and Conveyance Characteristics of a very Thin Steel Plate Magnetically Levitated by a LIM-Driven Cart. IEEE Trans. Magn. 2014, 50, 8600304. [Google Scholar] [CrossRef]
- Namerikawa, T.; Mizutani, D.; Kuroki, S. Robust H.INF. DIA Control of Levitated Steel Plates. IEEJ Trans. Ind. Appl. 2016, 126, 1319–1324. [Google Scholar] [CrossRef]
- Yonezawa, H.; Marumori, H.; Narita, T.; Hasegawa, S.; Oshinoya, Y. Bending Magnetic Levitation Control for Thin Steel Plate (Experimental Consideration Using Sliding Mode Control). In Proceedings of the International Power Electronics Conference -ECCE ASIA-, Hiroshima, Japan, 18–21 May 2014. [Google Scholar]
- Tada, M.; Yonezawa, H.; Marumori, H.; Narita, T.; Kato, H. Evaluation of levitation performance of bending flexible steel plate considering elastic mode. Trans. Magn. Soc. Jpn. (Spec. Issues) 2017, 1, 70–75. [Google Scholar]
- Tada, M.; Yonezawa, H.; Marumori, H.; Narita, T.; Kato, H. Integrated control of bending levitation for flexible steel plate using sliding mode control. J. Jpn. Soc. Appl. Electromagn. Mech. 2017, 25, 82–87. [Google Scholar] [CrossRef]
- Tada, M.; Yonezawa, H.; Marumori, H.; Narita, T.; Kato, H.; Moriyama, H. Basic Study on Maglev System for Flexible Steel Plate with Curvature: Effect on Levitation Performance under Disturbance. In Proceedings of the 18th International Symposium on Applied Electromagnetics and Mechanics, Chamonix Mont Blanc, France, 3–6 September 2017. [Google Scholar]
- Hasegawa, S.; Ohta, N.; Oshinoya, Y.; Ishibashi, K. Disturbance Cancellation Control of Electromagnetic Levitation System for Thin Steel Plate (Basic Research on Rigid Steel Plate). Proc. Sch. Eng. Tokai Univ. Ser. E 2004, 44, 67–73. [Google Scholar]



| Measurement range | 0–15 mm |
| Straightness | ±1% of F.S |
| Degradability | 0.03% of F.S |
| Responsiveness | DC-10 kHz (−3 dB) |
| A/D Converter | D/A Converter | ||
|---|---|---|---|
| Input voltage range | ±10 V | Output voltage range | ±10 V |
| Resolution | 12 bit | Resolution | 12 bit |
| Conversion time | 3 μs/ch | Conversion time | 5 μs/ch |
| Output voltage | ±20 V |
| Output current | ±5.5A |
| Frequency range | 2–3 kHz |
| Maximum excitation force | 10 kgf ± 5% |
| Maximum acceleration | 28 G ± 5% |
| Frequency range | 3 Hz–13 kHz |
| Maximum input current | 5.5 A ± 5% |
| Maximum speed | 1.77 m/s |
| Maximum amplitude | 10 mm |
| Optimal control | 0.129 mm |
| Optimal control with disturbance cancellation control | 0.092 mm |
| Optimal control | 86% |
| Optimal control with disturbance cancellation control | 96% |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ogawa, K.; Tada, M.; Narita, T.; Kato, H. Electromagnetic Levitation Control for Bending Flexible Steel Plate: Experimental Consideration on Disturbance Cancellation Control. Actuators 2018, 7, 43. https://doi.org/10.3390/act7030043
Ogawa K, Tada M, Narita T, Kato H. Electromagnetic Levitation Control for Bending Flexible Steel Plate: Experimental Consideration on Disturbance Cancellation Control. Actuators. 2018; 7(3):43. https://doi.org/10.3390/act7030043
Chicago/Turabian StyleOgawa, Kazuki, Makoto Tada, Takayoshi Narita, and Hideaki Kato. 2018. "Electromagnetic Levitation Control for Bending Flexible Steel Plate: Experimental Consideration on Disturbance Cancellation Control" Actuators 7, no. 3: 43. https://doi.org/10.3390/act7030043
APA StyleOgawa, K., Tada, M., Narita, T., & Kato, H. (2018). Electromagnetic Levitation Control for Bending Flexible Steel Plate: Experimental Consideration on Disturbance Cancellation Control. Actuators, 7(3), 43. https://doi.org/10.3390/act7030043





