A System Identification Technique Using Bias Current Perturbation for the Determination of the Magnetic Axes of an Active Magnetic Bearing
Abstract
:1. Introduction
Literature Review
2. Materials and Methods
2.1. The Multi-Point Method System Identification Approach
2.2. Experimental Approach
2.3. Experimental Signal Flow
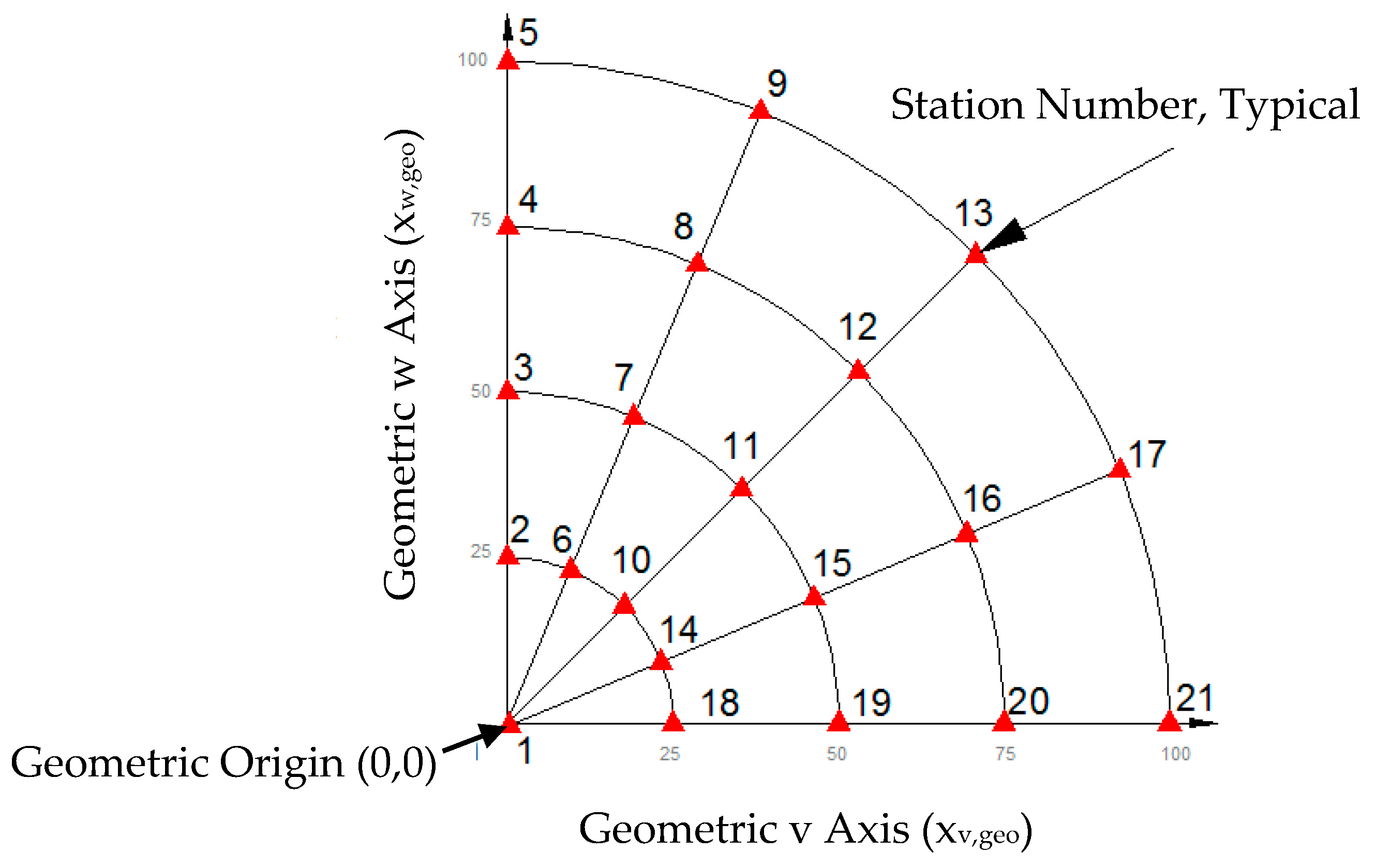
2.4. Bearing Rotor Space Geometry
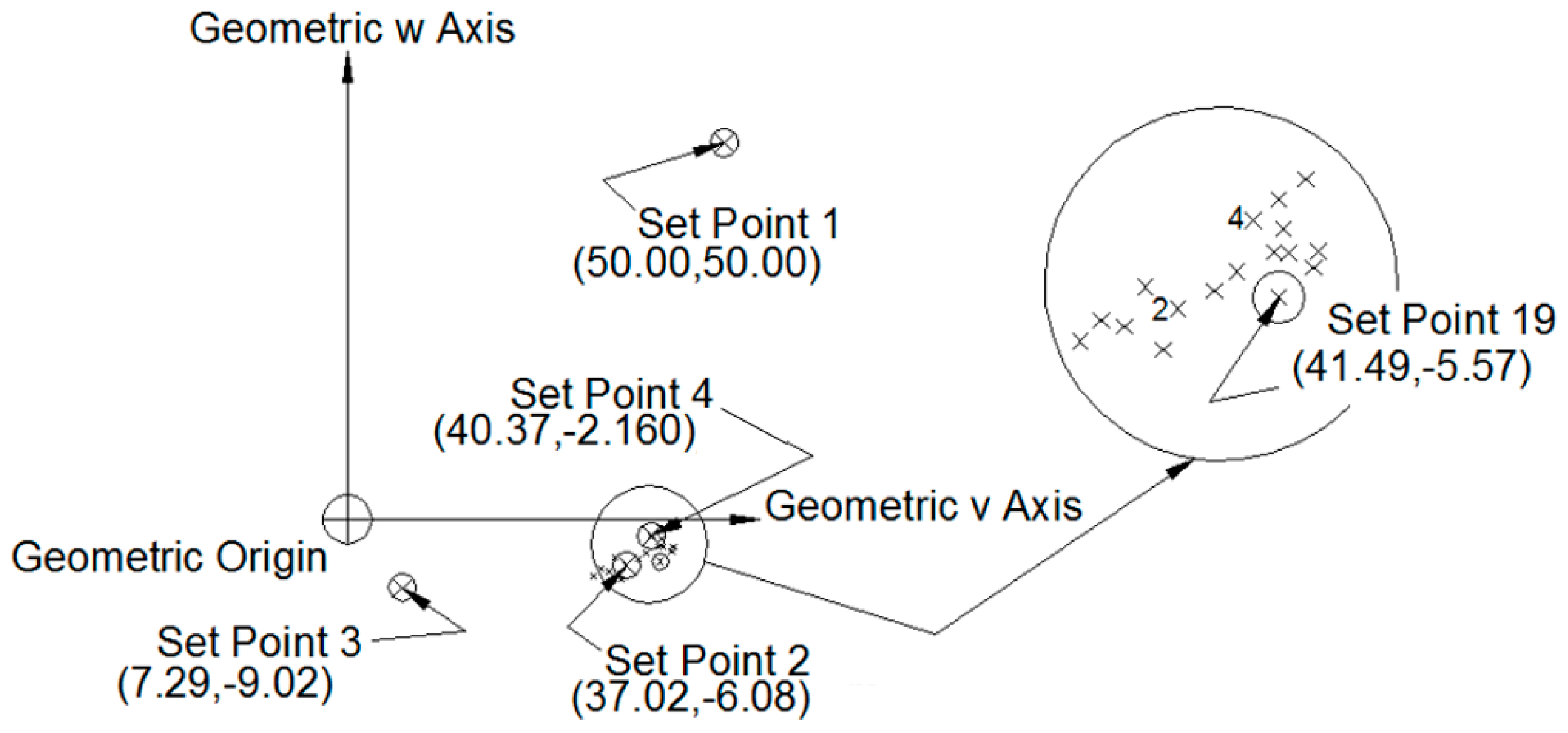
2.5. Reaction Measurement Using Geometric Coordinates and Geometric Set Points
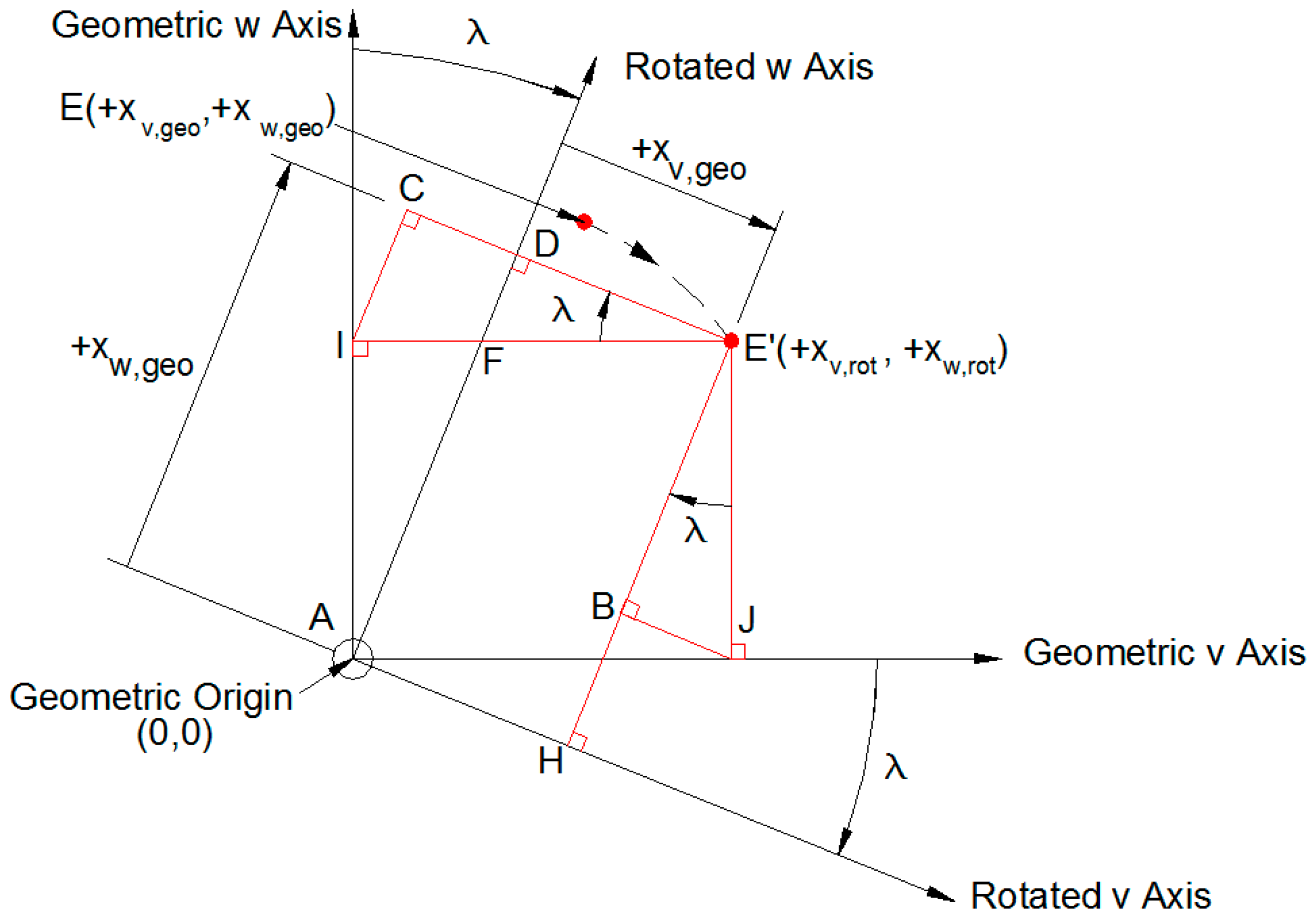
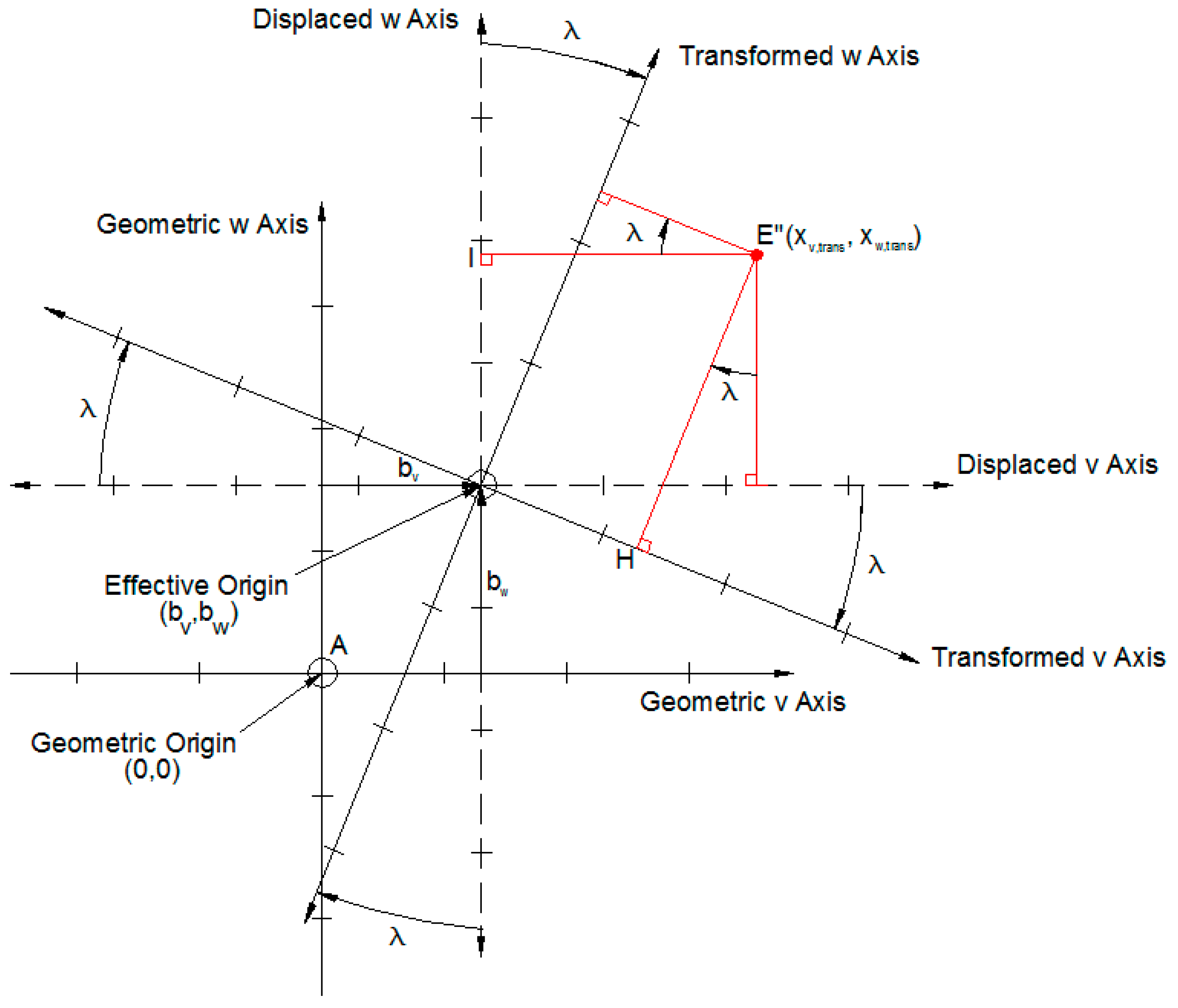
2.6. Transformation Equations
2.7. Rotational Transformation
2.8. Scale and Displacement Transformation
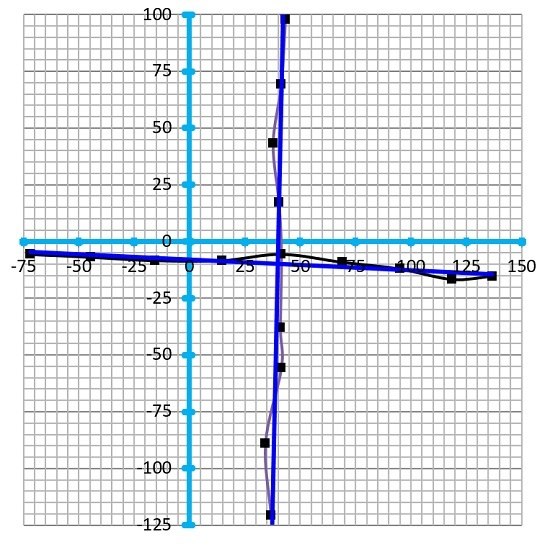
3. Results
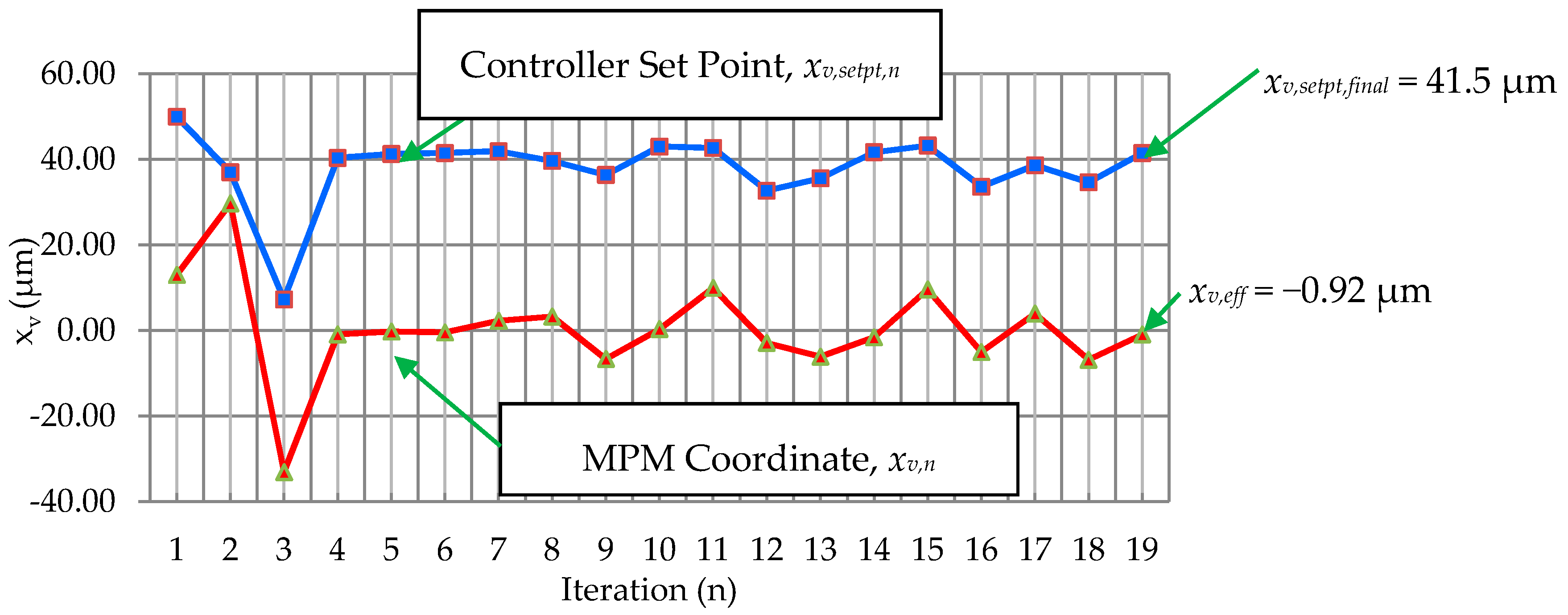
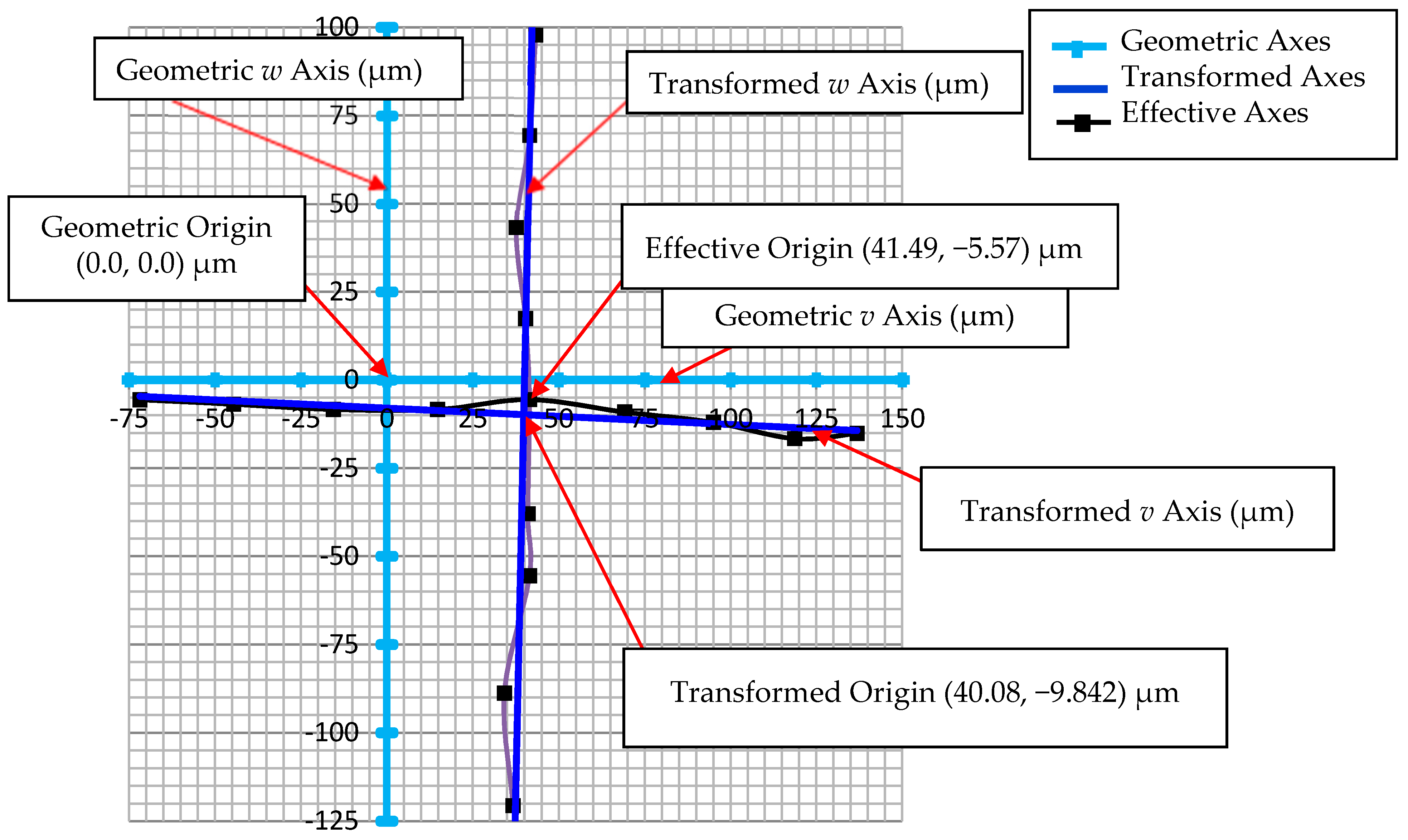
3.1. Locating the Effective Origin
3.2. Effective Coordinate Axes
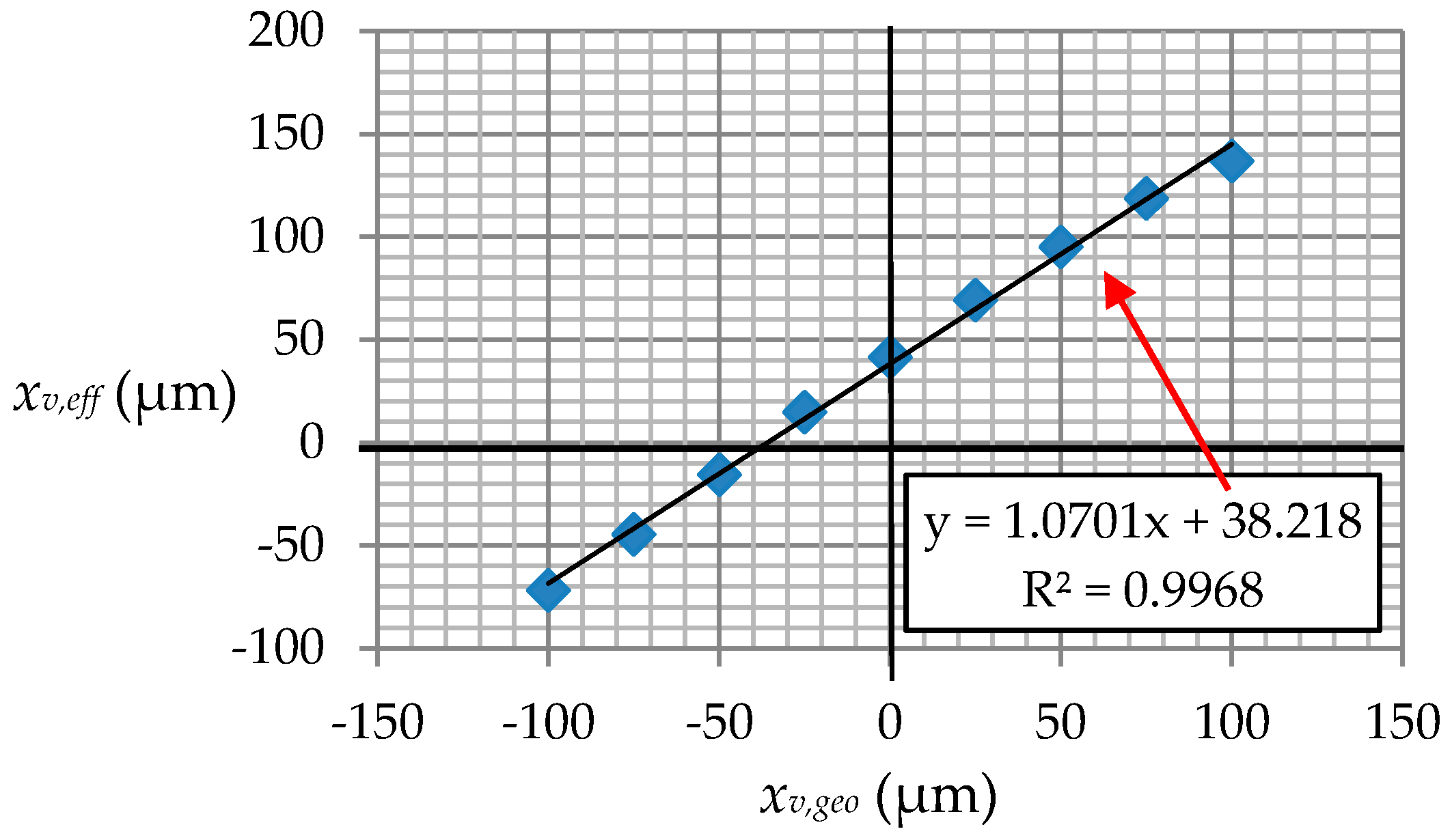
4. Empirical Transformation Equations
Reaction Measurement using Corrected Set Points
5. Conclusions
Author Contributions
Conflicts of Interest
Nomenclature
| A | area of two pole faces (2 faces per horseshoe) = 2.992 × 10−4 m2 |
| Fmagnetic | force due to magnetic source (N) |
| Li | flux path through rotor and stator (0.045 m) [2] |
| N | number of wire turns for two actuator coils (two coils per horseshoe) = 248 |
| b | Li/μr = manufacturer’s equivalent air gap based on magnetic reluctance of magnetic material (15.0 × 10−6 m) |
| ε | parameter to quantify the error between the bearing force obtained from force transducers and the force obtained from an MPM iteration |
| go | air gap between rotor and magnetic horseshoe when rotor and stator geometric centers are concentric |
| gtop | air gap between top of rotor and inside face or stator |
| gbottom | air gap between bottom of rotor and inside face or stator |
| i | total current in single magnetic horseshoe |
| itop | total current at top horseshoe |
| ibottom | total current at bottom horseshoe |
| λ | angle between the geometric v and w axes and the transformed v and w axes, respectively |
| R | Magnitude of the vector resultant of the magnetic forces along the v and w axes |
| θ | angle between the vertical axis and the v or w magnetic axes |
| θ′ | angle between the v or w magnetic axes and the axes of the position sensor |
| xv,n | v axis coordinate returned from MPM iteration n |
| xv,eff | desired v axis effective coordinate |
| xw,n | w axis coordinate returned from MPM iteration n |
| xw,eff | desired w axis effective coordinate |
| xv,geo, xw,geo | measured from the geometric center of the bearing located at the intersection of v and w sensor axes. Geometric coordinates are based on the assumption that the magnetic field is in perfect alignment with the rotor geometric center, magnetic axes and positional sensor axes. |
References
- Marshall, J.; Kasarda, M.; Imlach, J. A Multi-Point Measurement Technique for the Enhancement of Force Measurement with Active Magnetic Bearings. ASME J. Eng. Gas Turbine Power 2003, 125, 90–94. [Google Scholar] [CrossRef]
- Prins, R. System Identification and Calibration Techniques for Force Measurement in Active Magnetic Bearing. Ph.D. Thesis, Virginia Tech., Blacksburg, VA, USA, 2005, Section 1.1 (unpublished). [Google Scholar]
- Prins, R.J.; Kasarda, M.E.F.; Bates, S.C. A System Identification Technique Using Bias Current Perturbation for Determining Effective Rotor Origin of Active Magnetic Bearings. ASME J. Vib. Acoust. 2007, 129, 317–322. [Google Scholar] [CrossRef]
- Kasarda, M. An Overview of Active Magnetic Bearing Technology and Applications. Shock Vib. Dig. 2000, 32, 91–99. [Google Scholar] [CrossRef]
- Gahler, C.; Forch, P. A Precise Magnetic Bearing Exciter for Rotordynamic Experiments. In Proceedings of the Fourth International Symposium on Magnetic Bearings, Zurich, Switzerland, 23–26 August 1994. [Google Scholar]
- Rantatalo, M.; Aidanpaa, J.; Goransson, B.; Norman, P. Milling Machine Spindle Analysis Using FEM and Non-Contact Spindle Excitation and Response Measurement. Int. J. Mach. Tools Manuf. 2007, 47, 1034–1045. [Google Scholar] [CrossRef]
- Auchet, S.; Chevrier, P.; Lacour, M.; Lipinski, P. A New Method of Cutting Force Measurement Based on Command Voltages of Active Electro-Magnetic Bearings. Int. J. Mach. Tools Manuf. 2004, 44, 1441–1449. [Google Scholar] [CrossRef]
- Aenis, M.; Knopf, E.; Nordmann, R. Active Magnetic Bearings for the Identification and Fault Diagnosis in Turbomachinery. Mechatronics 2002, 12, 1011–1021. [Google Scholar] [CrossRef]
- Hussien, A.A.; Yamada, S.; Iwahara, M.; Okada, T.; Ohji, T. Application of the Repulsive-Type Magnetic Bearing for Manufacturing Micromass Measurement Balance Equipment. IEEE Trans. Magn. 2005, 41, 3802–3804. [Google Scholar] [CrossRef]
- Plonus, M.A. Applied Electromagnetics; Section 10.7; McGraw-Hill: New York, NY, USA, 1978. [Google Scholar]
- Spangler, D. Application of a Bias Current Perturbation Method for Determining Effective Gaps in Magnetic Bearings Utilizing an Error Vector Concept; Mechanical Engineering Master of Engineering Final Report; Virginia Tech: Blacksburg, VA, USA, 2011. [Google Scholar]
- MBRotor Research Test Stand (no. 893-0005-001-05/98). In Hardware User’s Guide, version 1.0; Section 2.4; Revolve Magnetic Bearings Inc.: Calgary, AB, Canada, 1998.



| Run | Sum of AMB Outboard Reaction (N) |
|---|---|
| 1 | 19.4 |
| 2 | 19.5 |
| 3 | 19.7 |
| 4 | 19.3 |
| 5 | 20.6 |
| 6 | 20.1 |
| 7 | 19.5 |
| 8 | 19.8 |
| 9 | 19.8 |
| 10 | 19.8 |
| Average | 19.8 |
| Bias Current (Amp) | iv,top | iw,top | iv,bottom | iw,bottom | Bearing Reaction R (N) |
|---|---|---|---|---|---|
| 1.5 | 1.768 | 1.670 | 1.228 | 1.305 | 22.28 |
| Station | Quadrant | Polar Coordinate R (μm) | Polar Coordinate Θ (°) | xv (μm) | xw (μm) | xv,setpoint (μm) | xw,setpoint (μm) | Reaction R (N) | Percent Difference |
|---|---|---|---|---|---|---|---|---|---|
| 1-origin | 1 | 0 | 90 | 0.00 | 0.00 | 0.00 | 0.00 | 22.02 | 11.5 |
| 3-positive (pos) w axis | 1 | 50 | 90 | 0.00 | 50 | 0.00 | 50 | 22.51 | 14 |
| 5-pos w axis | 1 | 100 | 90 | 0.00 | 100 | 0.00 | 100 | 22.95 | 16.2 |
| 11 (from Table 2) | 1 | 50 | 45 | 35.36 | 35.36 | 35.36 | 35.36 | 22.28 | 12.8 |
| 13 | 1 | 100 | 45 | 70.71 | 70.71 | 70.71 | 70.71 | 22.46 | 13.7 |
| 19-pos v axis | 1 | 50 | 0 | 50.0 | 0.00 | 50.0 | 0.00 | 22.06 | 11.7 |
| 21-pos v axis | 1 | 100 | 0 | 100 | 0.00 | 100 | 0.00 | 22.28 | 12.8 |
| 51-negative (neg) v axis | 2 | 50 | 180 | −50.0 | 0.00 | −50.0 | 0.00 | 21.84 | 10.6 |
| 53-neg v axis | 2 | 100 | 180 | −100 | 0.00 | −100 | 0.00 | 22.37 | 13.3 |
| 59 | 2 | 50 | 135 | −35.36 | 35.36 | −35.36 | 35.36 | 22.24 | 12.6 |
| 61 | 2 | 100 | 135 | −70.71 | 70.71 | −70.71 | 70.71 | 22.60 | 14.4 |
| 35-neg w axis | 3 | 50 | 270 | 0.00 | −50 | 0.00 | −50 | 21.44 | 8.56 |
| 37-neg w axis | 3 | 100 | 270 | 0.00 | −100 | 0.00 | −100 | 21.22 | 7.43 |
| 43 | 3 | 50 | 225 | −35.36 | −35.36 | −35.36 | −35.36 | 21.75 | 10.1 |
| 45 | 3 | 100 | 225 | −70.71 | −70.71 | −70.71 | −70.71 | 21.35 | 8.11 |
| 27 | 4 | 50 | 315 | 35.36 | −35.36 | 35.36 | −35.36 | 21.66 | 9.68 |
| 29 | 4 | 100 | 315 | 70.71 | −70.71 | 70.71 | −70.71 | 21.57 | 9.23 |
| Average | 22.02 | 11.6% |
| Bias Current (Amp) | iv,top | iw,top | iv,bottom | iw,bottom |
|---|---|---|---|---|
| 1.3 | 1.579 | 1.500 | 1.015 | 1.086 |
| 1.5 | 1.738 | 1.646 | 1.259 | 1.331 |
| 1.7 | 1.904 | 1.797 | 1.495 | 1.569 |
| Iteration (n) | xv,setpt,n (μm) | xw,setpt,n (μm) | xv,n Returned from MPM Iteration, n (μm) | xw,n Returned from MPM Iteration, n (μm) | εn |
|---|---|---|---|---|---|
| 1 | 50.00 | 50.00 | 12.98 | 56.08 | 57.54 |
| 2 | 37.02 | −6.08 | 29.73 | 2.94 | 29.88 |
| 3 | 7.29 | −9.02 | −33.08 | −6.86 | 33.78 |
| 4 | 40.37 | −2.16 | −0.9 | 1.39 | 1.66 |
| 5 | 41.27 | −3.55 | −0.23 | −2.32 | 2.33 |
| 6 | 41.5 | −1.23 | −0.43 | 2.37 | 2.41 |
| 7 | 41.93 | −3.6 | 2.28 | 0.81 | 2.42 |
| 8 | 39.65 | −4.41 | 3.27 | 3.49 | 4.78 |
| 9 | 36.38 | −7.9 | −6.64 | −3.66 | 7.58 |
| 10 | 43.02 | −4.24 | 0.34 | −4.57 | 4.58 |
| 11 | 42.68 | 0.33 | 9.98 | 7.84 | 12.69 |
| 12 | 32.7 | −7.51 | −2.89 | −2.39 | 3.75 |
| 13 | 35.59 | −5.12 | −6.09 | −2.6 | 6.62 |
| 14 | 41.68 | −2.52 | −1.57 | 1.01 | 1.87 |
| 15 | 43.25 | −3.53 | 9.62 | 3.04 | 10.09 |
| 16 | 33.63 | −6.57 | −5 | −1.28 | 5.16 |
| 17 | 38.63 | −5.29 | 3.97 | 1.56 | 4.27 |
| 18 | 34.66 | −6.85 | −6.83 | −1.28 | 6.95 |
| 19 | 41.49 | −5.57 | −0.92 | −0.67 | 1.14 |
| Bias Current (Amp) | iv,top | iw,top | iv,bottom | iw,bottom | Bearing Reaction R (N) |
|---|---|---|---|---|---|
| 1.3 | 1.590 | 1.588 | 1.008 | 0.998 | 19.37 |
| 1.5 | 1.751 | 1.744 | 1.251 | 1.232 | 19.28 |
| 1.7 | 1.923 | 1.909 | 1.482 | 1.458 | 19.25 |
| Station | Quad. | xv (μm) | xw (μm) | xv,setpoint (μm) | xw,setpoint (μm) | Reaction R (N) | Percent Diff. |
|---|---|---|---|---|---|---|---|
| 1-origin | 1 | 0.00 | 0.00 | 44.11 | −6.92 | 19.26 | −2.48 |
| 3-pos w axis | 1 | 0.00 | 50 | 46.59 | 46.51 | 19.13 | −3.15 |
| 5-pos w axis | 1 | 0.00 | 100 | 49.07 | 99.94 | 19.22 | −2.7 |
| 11 | 1 | 35.36 | 35.36 | 83.69 | 30.04 | 18.90 | −4.28 |
| 13 | 1 | 70.71 | 70.71 | 123.27 | 66.99 | 19.04 | −3.6 |
| 19-pos v axis | 1 | 50.0 | 0.00 | 97.6 | −8.08 | 18.90 | −4.28 |
| 21-pos v axis | 1 | 100 | 0.00 | 151.1 | −9.25 | 20.91 | 5.86 |
| 51-neg v axis | 2 | −50.0 | 0.00 | −9.38 | −5.75 | 19.30 | −2.25 |
| 53-neg v axis | 2 | −100 | 0.00 | −62.87 | −4.59 | 19.75 | 0.00 |
| 59 | 2 | −35.36 | 35.36 | 8.04 | 31.69 | 19.17 | −2.93 |
| 61 | 2 | −70.71 | 70.71 | −28.03 | 70.29 | 19.70 | −0.23 |
| 35-neg w axis | 3 | 0.00 | −50 | 41.63 | −60.35 | 19.17 | −2.93 |
| 37-neg w axis | 3 | 0.00 | −100 | 39.15 | −113.78 | 19.17 | −2.93 |
| 43 | 3 | −35.36 | −35.36 | 4.53 | −43.88 | 19.48 | −1.35 |
| 45 | 3 | −70.71 | −70.71 | −35.05 | −80.83 | 19.53 | −1.13 |
| 27 | 4 | 35.36 | −35.36 | 80.18 | −45.52 | 19.13 | −3.15 |
| 29 | 4 | 70.71 | −70.71 | 116.25 | −84.13 | 18.99 | −3.83 |
| Average | 19.34 | −2.77% |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Spangler, D.; Prins, R.; Kasarda, M. A System Identification Technique Using Bias Current Perturbation for the Determination of the Magnetic Axes of an Active Magnetic Bearing. Actuators 2017, 6, 13. https://doi.org/10.3390/act6020013
Spangler D, Prins R, Kasarda M. A System Identification Technique Using Bias Current Perturbation for the Determination of the Magnetic Axes of an Active Magnetic Bearing. Actuators. 2017; 6(2):13. https://doi.org/10.3390/act6020013
Chicago/Turabian StyleSpangler, Dewey, Robert Prins, and Mary Kasarda. 2017. "A System Identification Technique Using Bias Current Perturbation for the Determination of the Magnetic Axes of an Active Magnetic Bearing" Actuators 6, no. 2: 13. https://doi.org/10.3390/act6020013
APA StyleSpangler, D., Prins, R., & Kasarda, M. (2017). A System Identification Technique Using Bias Current Perturbation for the Determination of the Magnetic Axes of an Active Magnetic Bearing. Actuators, 6(2), 13. https://doi.org/10.3390/act6020013