Modeling and Realization of a Bearingless Flux-Switching Slice Motor
Abstract
:1. Introduction
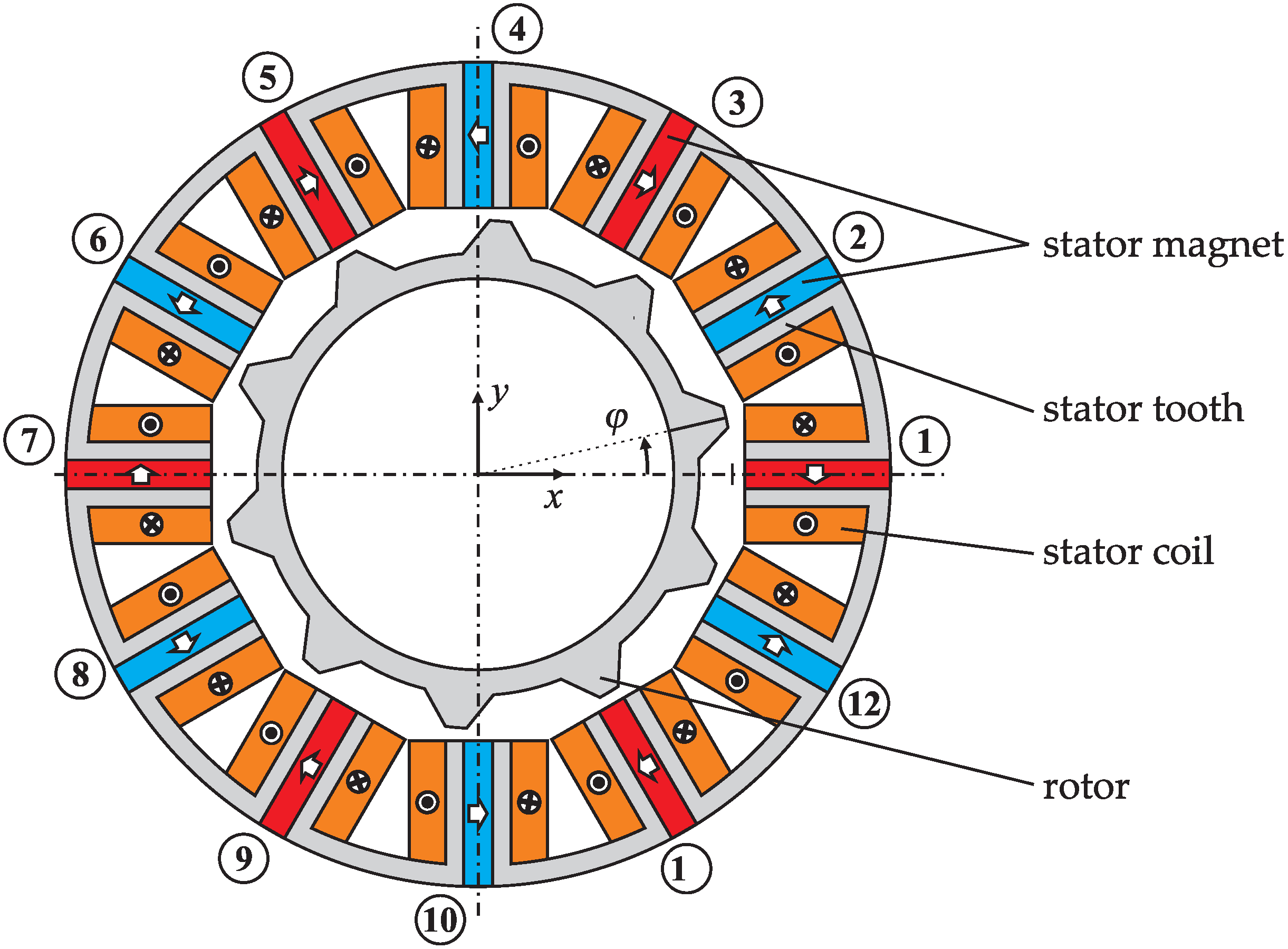
2. Bearingless Flux-Switching Slice Motor
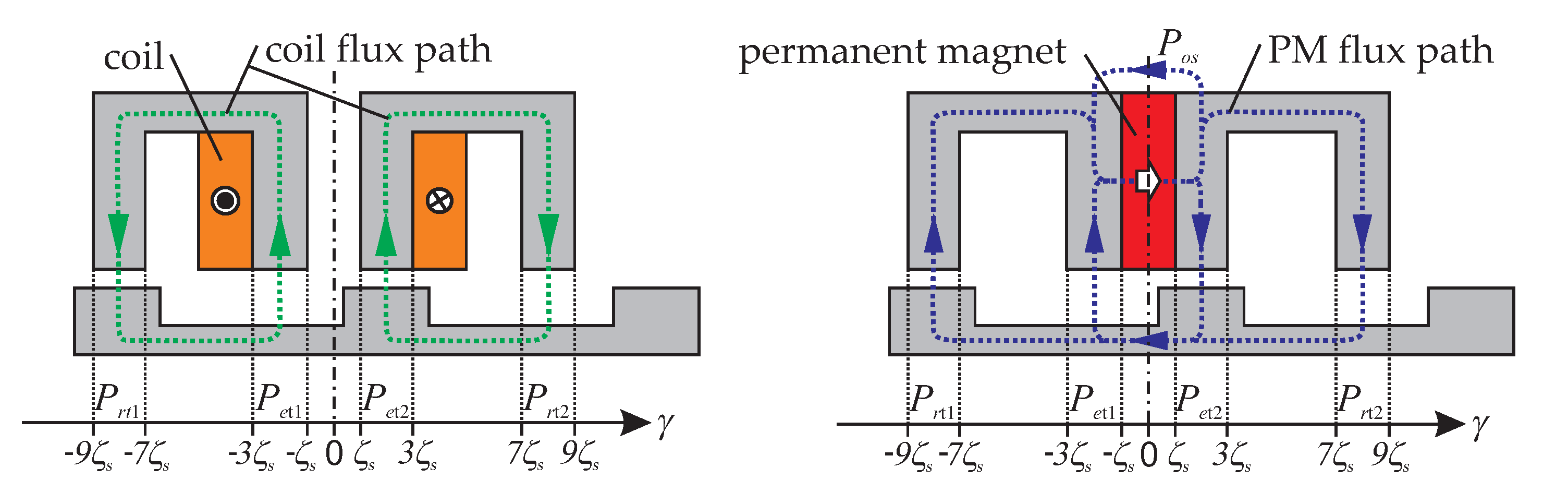
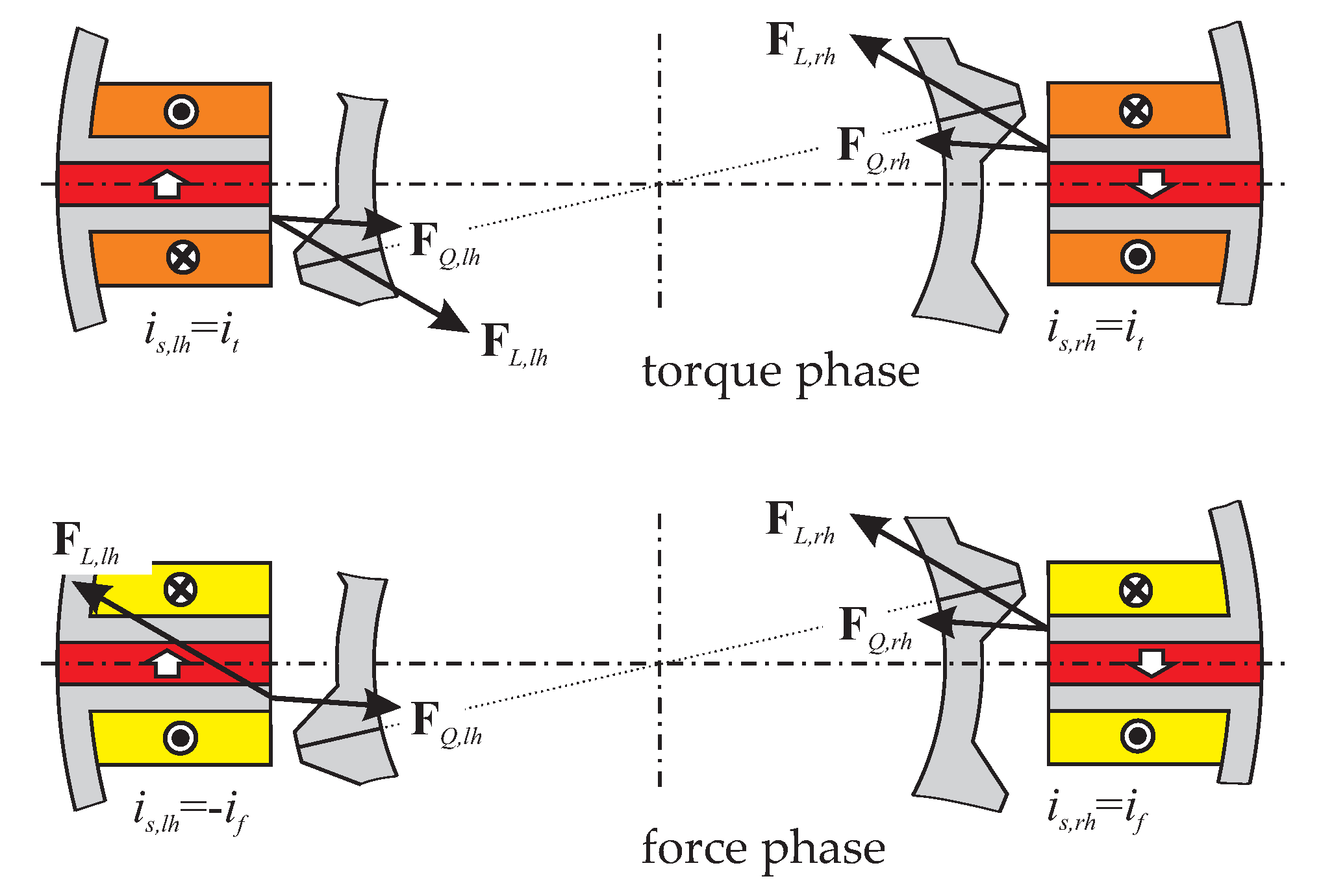
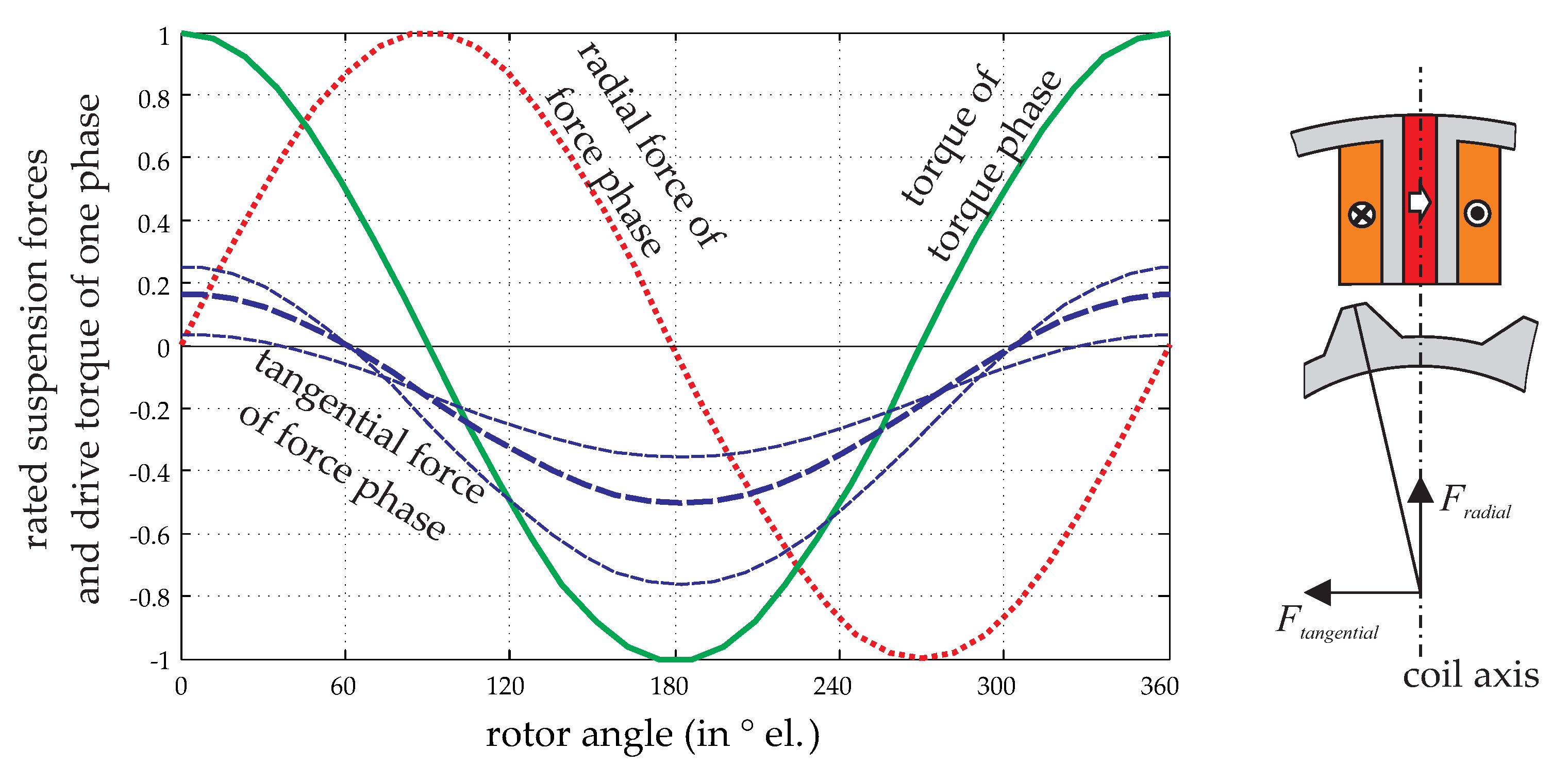
3. Modeling of the Single-Phase Characteristic
4. Overall Performance of the Bearingless Flux-Switching Motor
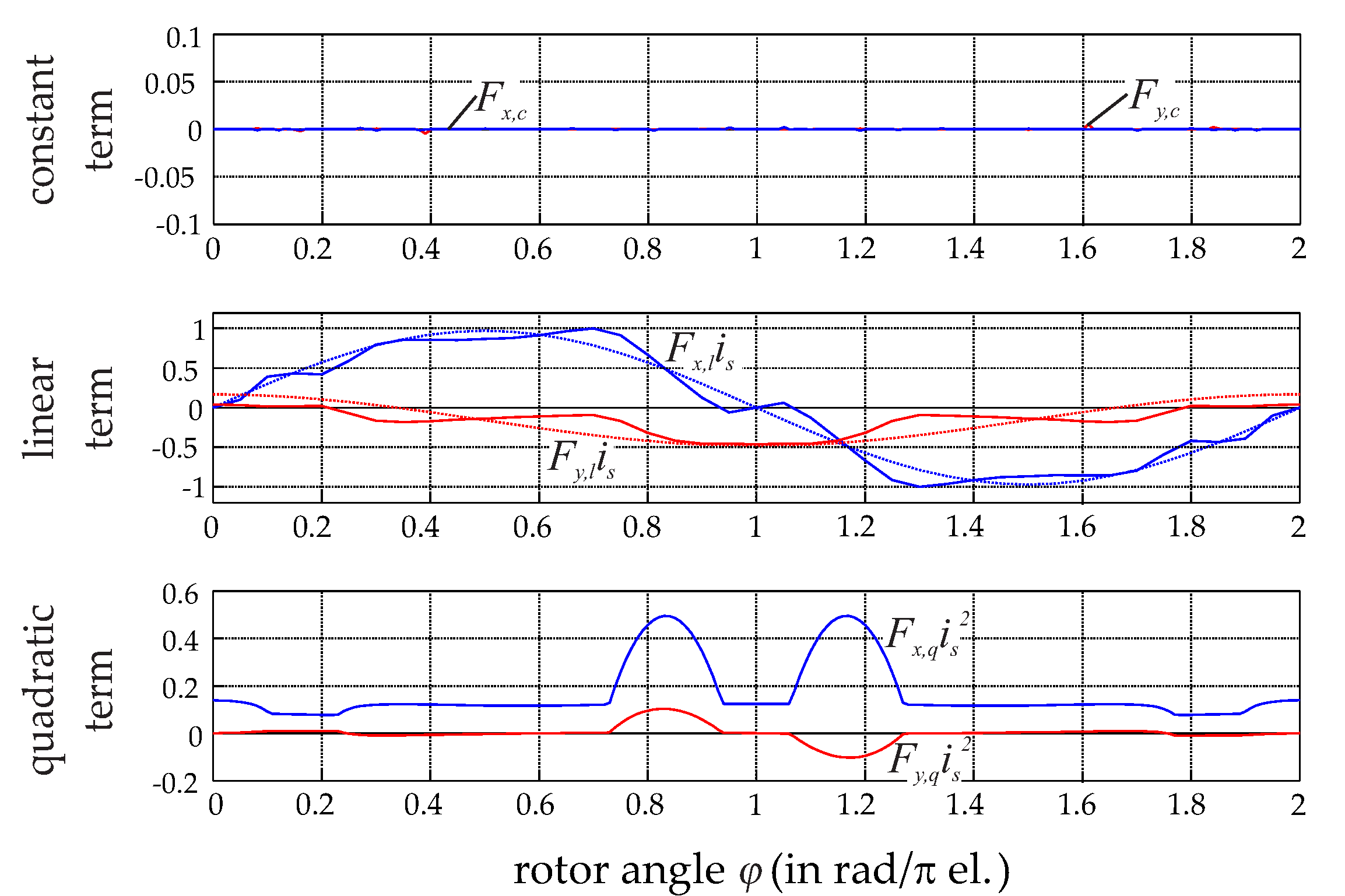
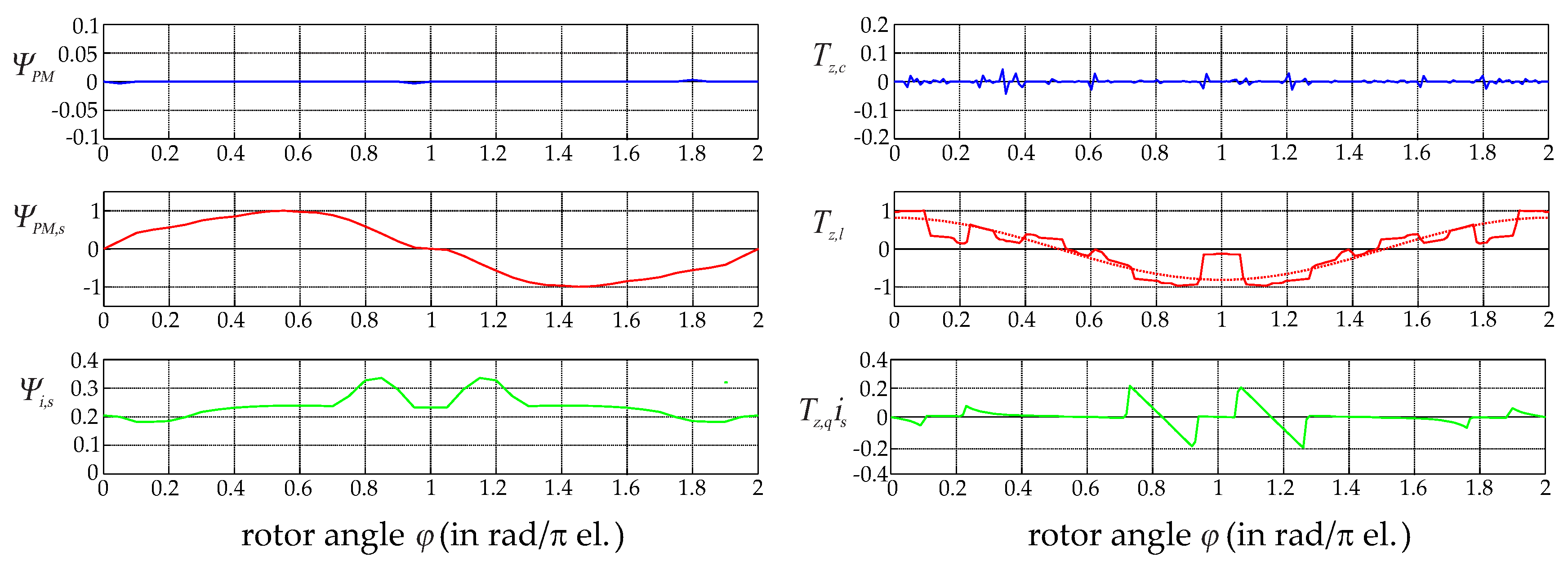
4.1. Linearization and Decoupling
4.2. Topology Evaluation
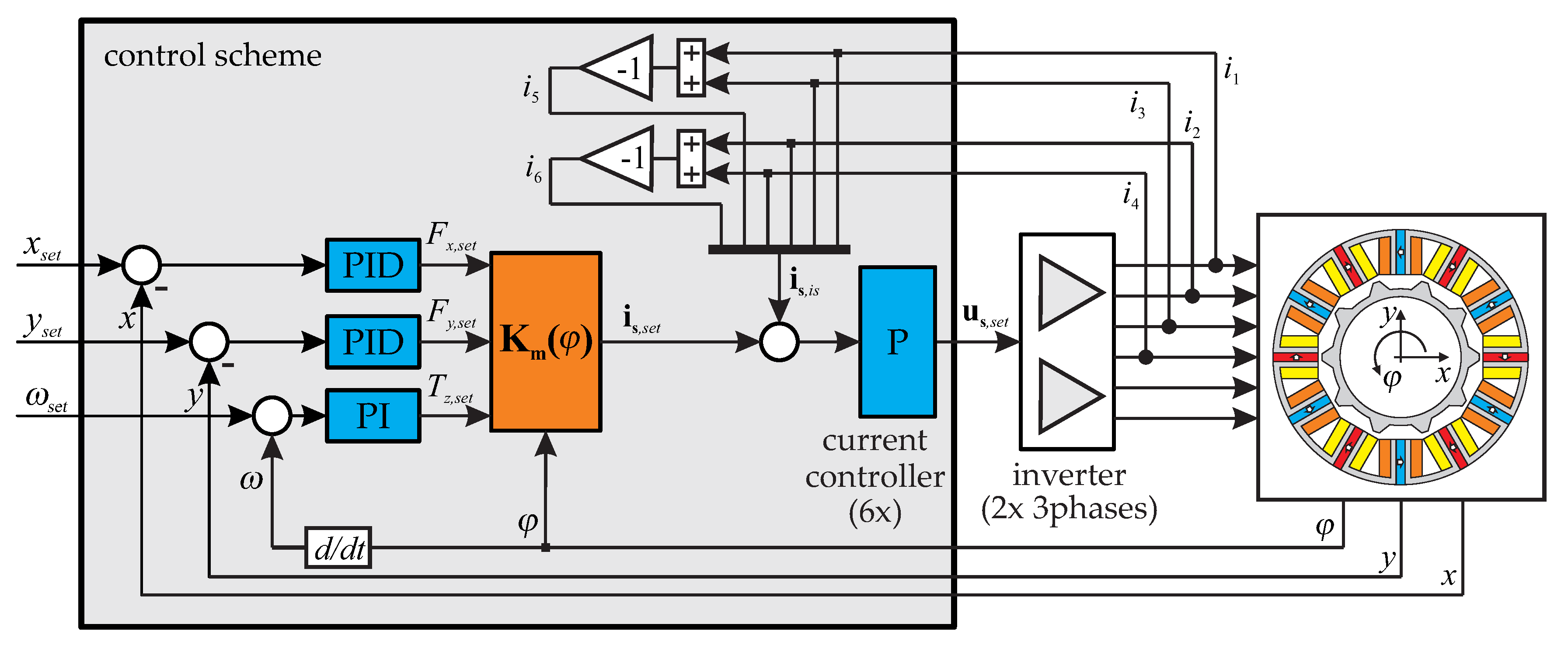
4.3. Control Scheme
5. Optimization and Design of the Prototype
6. Measurement Results
6.1. Static Measurements
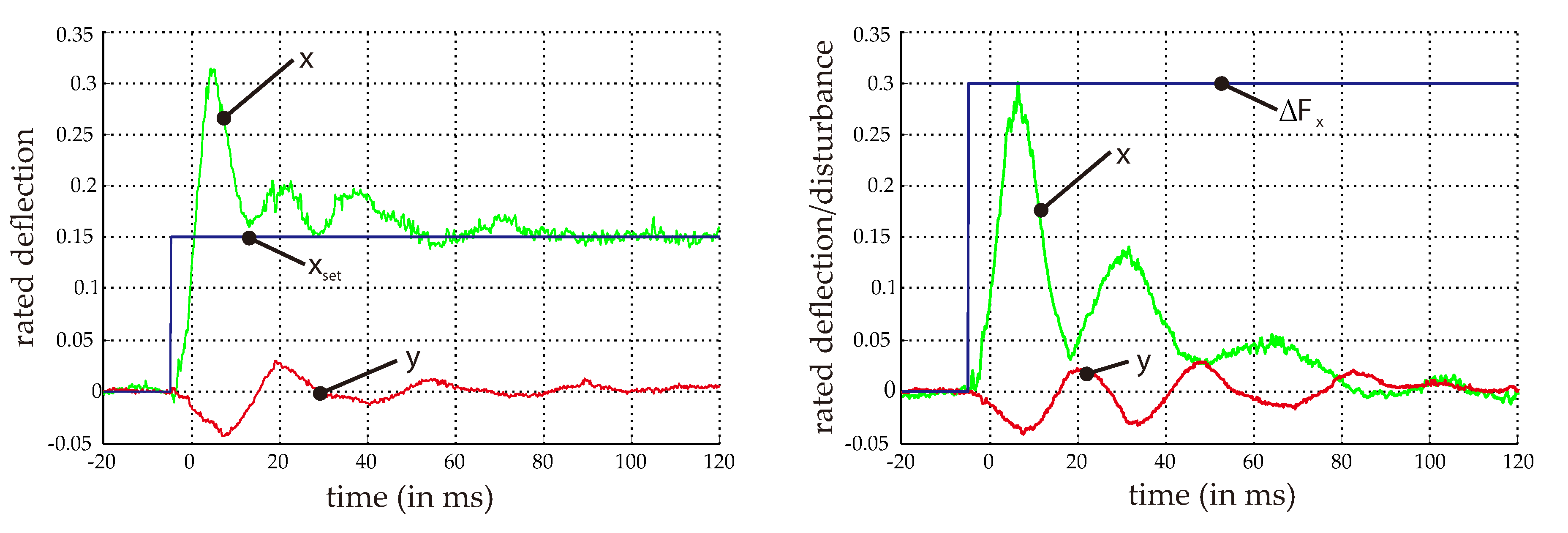
6.2. Dynamic Suspension Measurements
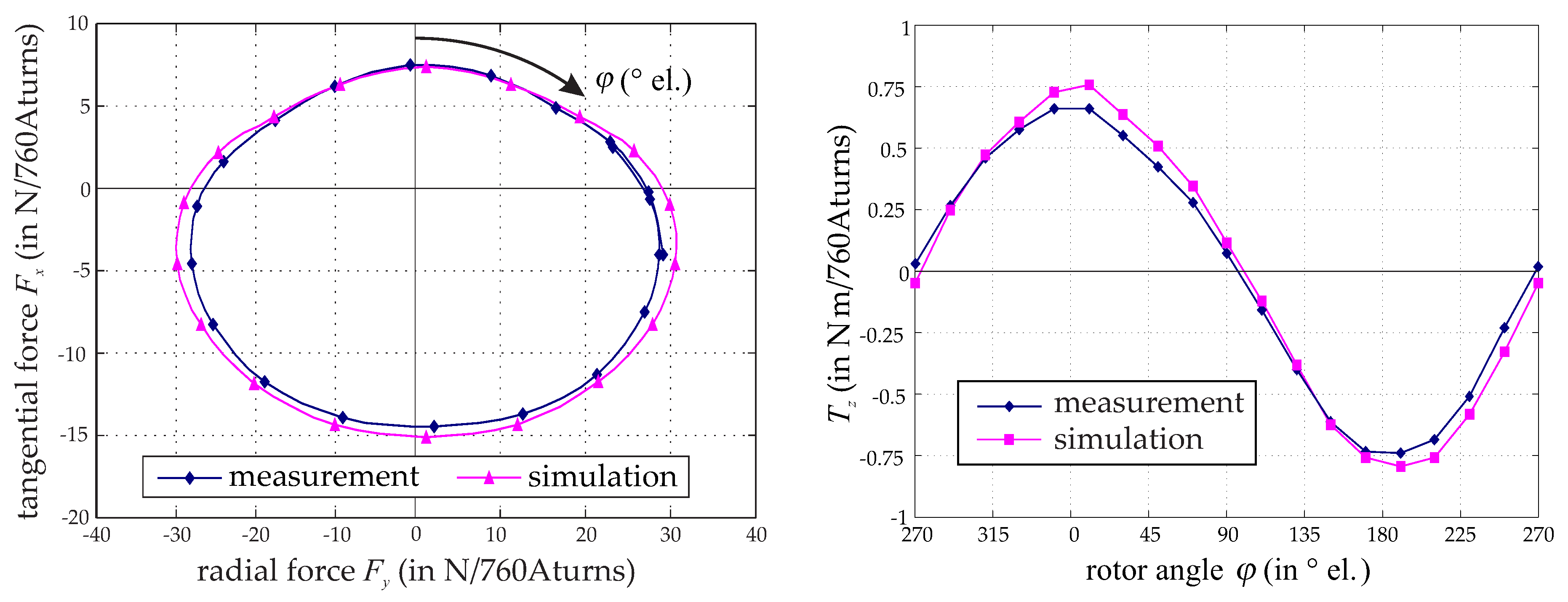
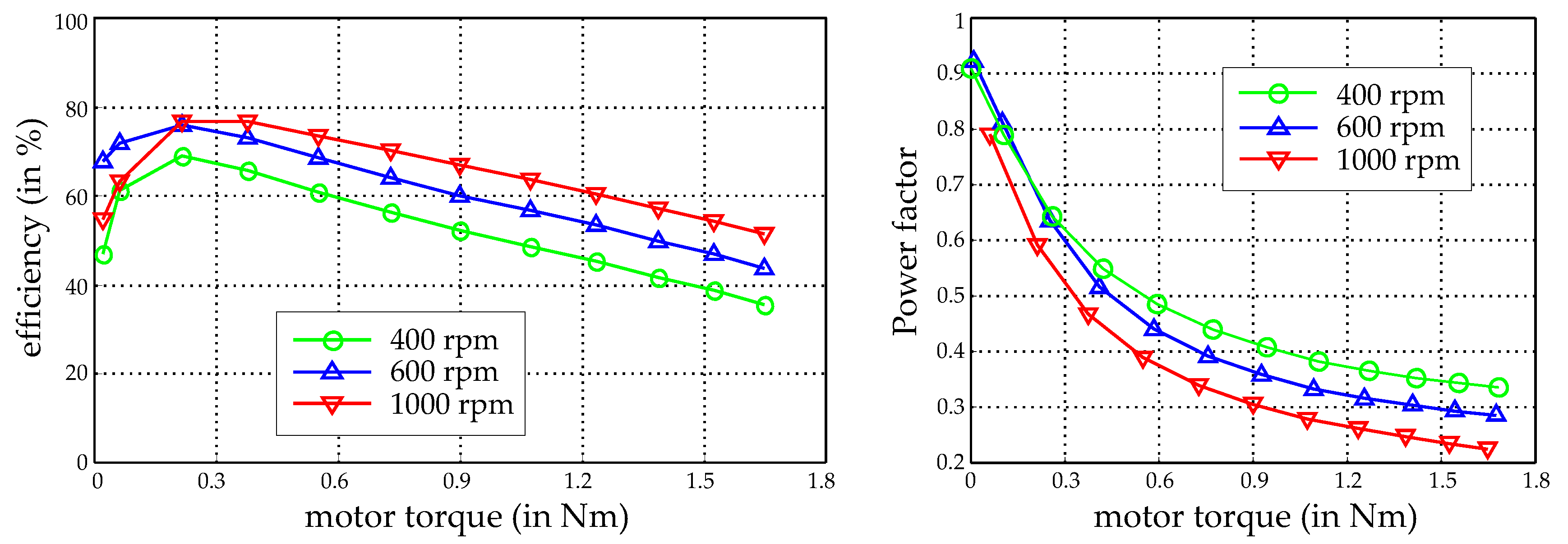
6.3. Motor Measurements
7. Discussion
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Schöb, R.; Barletta, N. Principle and application of a bearingless slice motor. In Proceedings of the 5th International Symposium on Magnetic Bearings (ISMB), Kanazawa, Japan, 28–30 August 1996; pp. 333–338. [Google Scholar]
- Asama, J.; Fukao, T.; Chiba, A.; Rahman, M.A.; Oiwa, T. A design consideration of a novel bearingless disk motor for artificial hearts. In Proceedings of the 1st IEEE Energy Conversion Congress and Exposition (ECCE), San Jose, CA, USA, 20–24 September 2009; pp. 1693–1699. [Google Scholar]
- Nussbaumer, T.; Raggl, K.; Bösch, P.; Kolar, J.W. Trends in integration for magnetically levitated pump systems. In Proceedings of the Power Conversion Conference (PCC), Nagoya, Japan, 2–5 April 2007; pp. 1551–1558. [Google Scholar]
- Nussbaumer, T.; Karutz, P.; Zürcher, F.; Kolar, J.W. Magnetically levitated slice motors—An overview. IEEE Trans. Ind. Appl. 2011, 47, 754–766. [Google Scholar] [CrossRef]
- Warberger, B.; Kaelin, R.; Nussbaumer, T.; Kolar, J.W. 50-Nm/2500-W bearingless motor for high-purity pharmaceutical mixing. IEEE Trans. Ind. Electron. 2012, 59, 2236–2247. [Google Scholar] [CrossRef]
- Mitterhofer, H.; Mrak, B.; Amrhein, W. Suitability investigation of a bearingless disk drive for micro turbine applications. In Proceedings of the IEEE Energy Conversion Congress and Exposition (ECCE), Denver, CO, USA, 15–19 Sept. 2013; pp. 2480–2485. [Google Scholar]
- Zingerli, C.M.; Coray, I.; Weber, J.; Nussbaumer, T.; Kolar, J.W. Scaling of magnetically levitated homopolar hollow-shaft machines. In Proceedings of the 10th IEEE International Conference on Power Electronics and Drive Systems (PEDS), Kitakyushu, Japan, 22–25 April 2013; pp. 54–59. [Google Scholar]
- Huwyler, S.; Schrag, D.; Gilbert, R.; Thorsen, T.; Schöb, R.; Hahn, J. Bearingless in-line viscometer for the semiconductor industry. Proc. IEEE Sens. 2003, 2, 1077–1081. [Google Scholar]
- Asami, K.; Chiba, A.; Rahman, M.A.; Hoshino, T.; Nakajima, A. Stiffness analysis of a magnetically suspended bearingless motor with permanent magnet passive positioning. IEEE Trans. Magn. 2005, 41, 3820–3822. [Google Scholar] [CrossRef]
- Sugimoto, H.; Miyoshi, M.; Chiba, A. Low speed test in two-axis actively positioned bearingless machines with non-collocated structure for wind power applications. In Proceedings of the IEEE Energy Conversion Congress and Exposition (ECCE), Montreal, QC, Canada, 20–24 September 2015; pp. 799–804. [Google Scholar]
- Gruber, W.; Briewasser, W.; Rothböck, W.; Schöb, R. Bearingless slice motor concepts without permanent magnets in the rotor. In Proceedings of the IEEE International Conference on Industrial Technology (ICIT), Cape Town, South Africa, 25–28 February 2013; pp. 259–265. [Google Scholar]
- Gruber, W.; Rothböck, M.; Schöb, R. Design of a novel homopolar bearingless slice motor with reluctance rotor. IEEE Trans. Ind. Appl. 2015, 51, 1456–1464. [Google Scholar] [CrossRef]
- Rao, J.; Hijikata, W.; Shinshi, T. A permanent magnet free bearingless motor for disposable centrifugal blood pump. In Proceedings of the 14th International Symposium on Magnetic Bearing (ISMB), Linz, Austria, 11–14 August 2014; pp. 183–186. [Google Scholar]
- Zhu, Z.Q.; Howe, D. Electrical machines and drives for electric, hybrid, and fuel cell vehicles. Proc. IEEE 2007, 95, 746–765. [Google Scholar] [CrossRef]
- Gruber, W.; Radman, K.; Schöb, R. Design of a bearingless flux-switching slice motor. In Proceedings of the Power Electronics Conference (IPEC—ECCE ASIA), Hiroshima, Japan, 18–21 May 2014; pp. 1691–1696. [Google Scholar]
- Radman, K.; Bulic, N.; Gruber, W. Loss analysis of a bearingless flux-switching slice motor. In Proceedings of the 14th International Symposium on Magnetic Bearings (ISMB), Linz, Austria, 11–14 August 2014; pp. 210–215. [Google Scholar]
- Hoang, E.; Ahmed, H.B.; Lucidarme, J. Switching flux permanent magnet poly-phased synchronous machines. In Proceedings of the 7th European Conference on Power Electronics and Applications (EPE), Trondheim, Norway, 8–10 September 1997; Volume 3, pp. 903–908. [Google Scholar]
- Rauch, S.E.; Johnson, L.J. Design principles of flux-switch alternators. Trans. Am. Inst. Electr. Eng. Part III Power Appar. Syst. 1955, 74, 1261–1268. [Google Scholar]
- Gruber, W.; Briewasser, W.; Rothböck, M.; Schöb, R.; Amrhein, W. Bearingless reluctance slice motors. In Proceedings of the 13th International Symposium on Magnetic Bearings (ISMB), Arlington, TX, USA, 6–9 August 2012. [Google Scholar]
- Gruber, W.; Bauer, W.; Radman, K.; Amrhein, W.; Schöb, R. Considerations Regarding Bearingless Flux-Switching Slice Motors. In Proceedings of the 1st Brazilian Workshop on Magnetic Bearings, Rio de Janeiro, Brazil, 25–26 October 2013; Available online: www.magneticbearings.org (accessed on 28 November 2016).
- Jia, H.; Fang, C.; Zhang, T. Finite element analysis of a novel bearingless flux-switching permanent magnet motor with the single winding. In Proceedings of the 17th International Conference on Electrical Machines and Systems (ICEMS), Hangzhou, China, 22–25 October 2014; pp. 2315–2318. [Google Scholar]
- Li, H.; Zhu, H. Design of bearingless flux-switching permanent-magnet motor. IEEE Trans. Appl. Superconduct. 2016, 26, 1–5. [Google Scholar] [CrossRef]
- Ni, T.; Wang, X.; Ding, Q.; Wang, Y. Novel structure of bearingless flux-switching motor for improvement of levitation force characteristics. In Proceedings of the 8th International Power Electronics and Motion Control Conference (IPEMC-ECCE Asia), Hefei, China, 22–26 May 2016; pp. 1929–1933. [Google Scholar]
- Jastrzbeski, R.P.; Jaatinen, P.; Pyrhönen, O. Modelling and control design simulations of permanent magnet flux-switching linear bearingless motor. In Proceedings of the 15th International Symposium on Magnetic Bearing (ISMB), Kitykyushu, Japan, 3–6 August 2016; pp. 296–303. [Google Scholar]
- Silber, S. Power optimal current control scheme for bearingless pm motors. In Proceedings of the 7th International Symposium on Magnetic Bearings (ISMB), Zurich, Switzerland, 23–25 August 2000; pp. 401–406. [Google Scholar]
- Zhu, Z.Q.; Pang, Y.; Howe, D.; Iwasaki, S.; Deodhar, R.; Pride, A. Analysis of electromagnetic performance of flux-switching permanent-magnet machines by non-linear adaptive lumped parameter magnetic circuit model. IEEE Trans. Magn. 2005, 41, 4277–4287. [Google Scholar] [CrossRef]
- Chen, J.T.; Zhu, Z.Q. Winding configurations and optimal stator and rotor pole combination of flux-switching pm brushless ac machines. IEEE Trans. Energy Conv. 2010, 25, 293–302. [Google Scholar] [CrossRef]
- Wei, H.; Ming, C.; Zhu, Z.Q.; Howe, D. Analysis and optimization of back EMF waveform of a flux-switching permanent magnet motor. IEEE Trans. Energy Conv. 2008, 23, 727–733. [Google Scholar] [CrossRef]
- He, W.; Hantong, W.; Fei, R.; Zhou, Y. Research on control strategy for a double-winding bearingless flux-switching machine with alternating excited orthogonal suspension windings. In Proceedings of the 8th IEEE International Power Electronics and Motion Control Conference (IPEMC-ECCE Asia), Hefei, China, 22–26 May 2016; pp. 821–826. [Google Scholar]
- Mitterhofer, H.; Mrak, B.; Gruber, W. Comparison of high-speed bearingless drive topologies with combined windings. IEEE Trans. Ind. Appl. 2015, 51, 2116–2122. [Google Scholar] [CrossRef]
- Zhu, Z.Q.; Pang, Y.; Chen, J.; Xia, Z.P.; Howe, D. Influence of design parameters on output torque of flux-switching permanent magnet machines. In Proceedings of the IEEE Vehicle Power and Propulsion Conference (VPPC), Harbin, China, 3–5 September 2008; pp. 1–6. [Google Scholar]
- Radman, K.; Bulic, N.; Gruber, W. Geometry optimization of a bearingless flux-switching slice motor. In Proceedings of the IEEE International Electric Machines & Drives Conference (IEMDC), Coeur d’Alene, ID, USA, 10–13 May 2015; pp. 1695–1701. [Google Scholar]
- Hua, W.; Cheng, M.; Zhu, Z.Q.; Howe, D. Analysis and optimization of back emf waveform of a flux-switching permanent magnet motor. IEEE Trans. Energy Conv. 2008, 23, 727–733. [Google Scholar] [CrossRef]
- Grabner, H. Dynamik und Ansteuerkonzepte Lagerloser Drehfeld-Scheibenläufermotoren in radialer Bauform. Ph.D. Thesis, Johannes Kepler University (JKU) Linz, Linz, Austria, 2006. (In German). [Google Scholar]
- Karutz, P. Magnetically Levitated 2-Level Slice Motor for Application in High Purity Process Environments. Ph.D. Thesis (No. 18809), Eidgenössische Technische Hochschule (ETH) Zurich, Zurich, Switzerland, 2010. [Google Scholar]
- Steinert, D. Der Nutenlose Lagerlose Scheibenläufermotor. Ph.D. Thesis (No. 22961), Eidgenössische Technische Hochschule (ETH) Zurich, Zurich, Switzerland, 2015. (In German). [Google Scholar]
Sample Availability: All ISMB papers are available online at www.magneticbearings.org (accessed on 28 November 2016). |


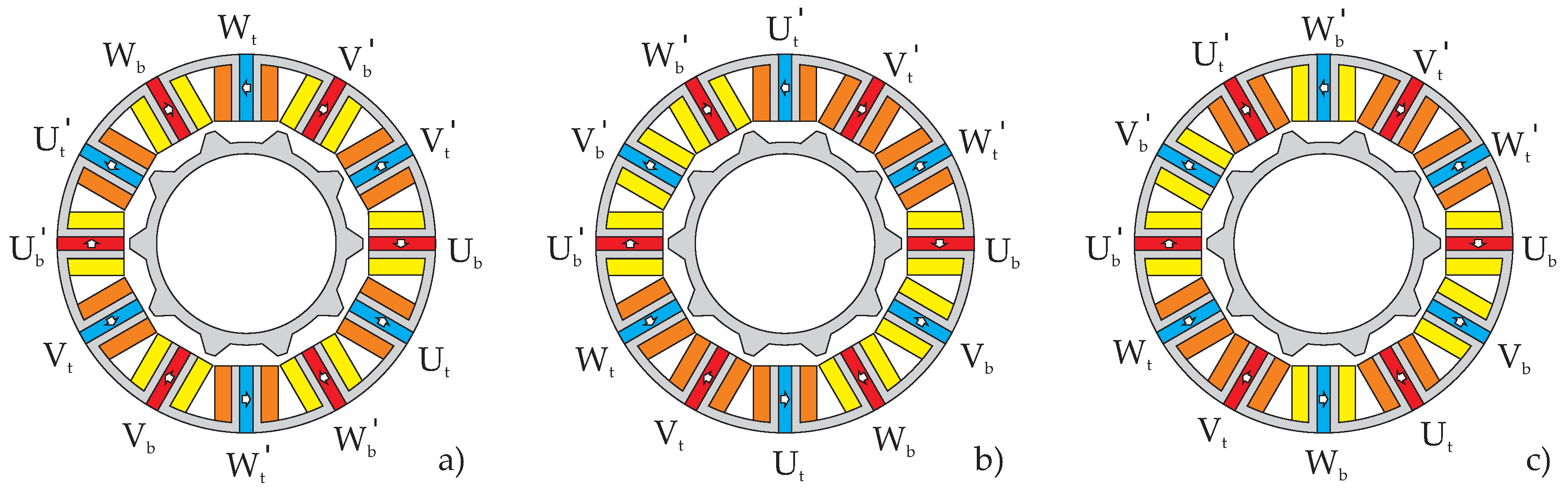
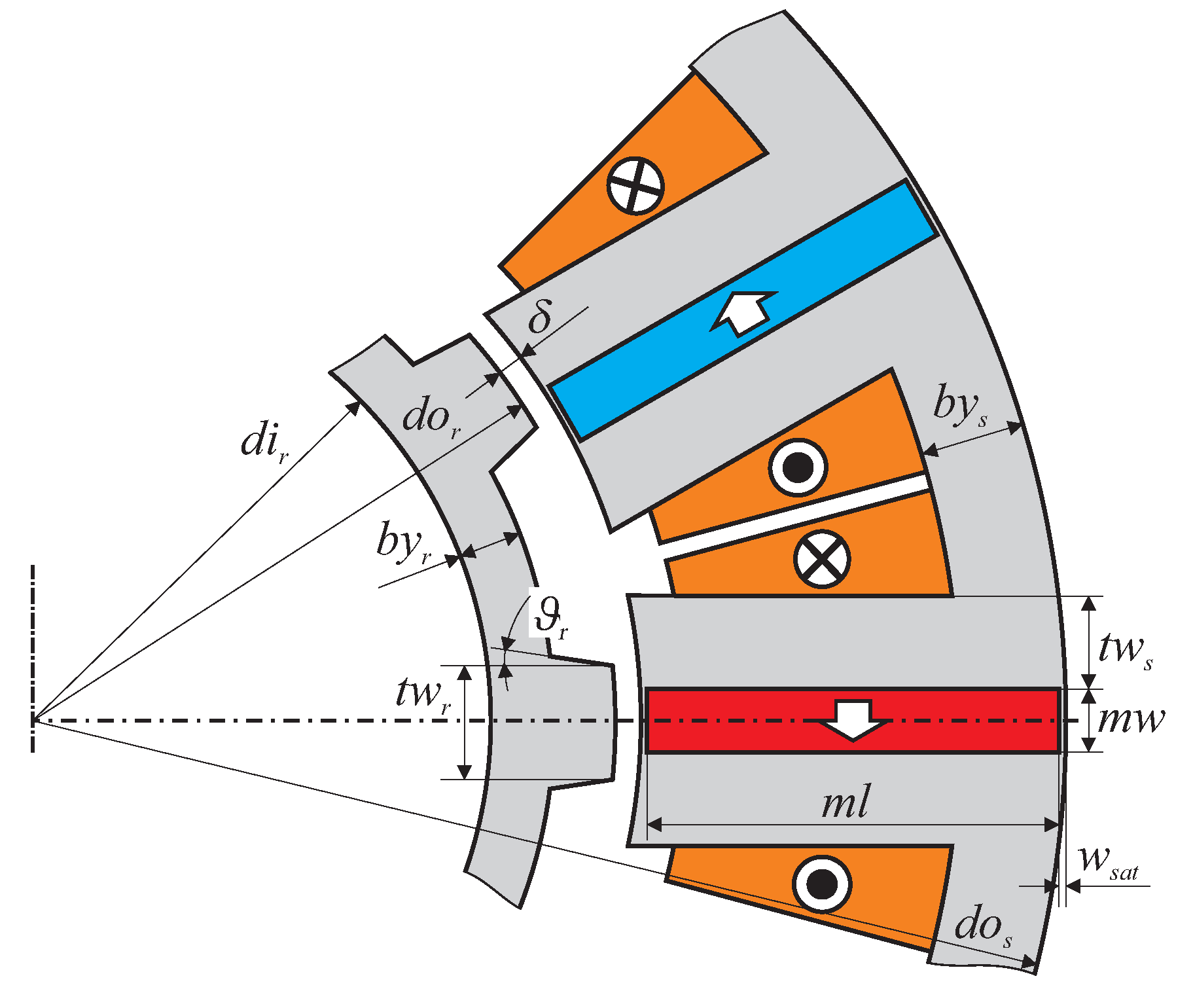

| Winding Scheme (Corresponding to Figure 9) | Number of Stator Teeth Ns = 12 | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Nr = 8 | Nr = 10 | Nr = 12 | Nr = 14 | Nr = 16 | ||||||
| (a) double three-phase | cf = 0 | ct = 1 | cf = 0.5 | ct = 1 | cf = 0.2 | ct = 0 * | cf = 0 | ct = 1 | cf = 0.5 | ct = 1 |
| CF↓ | CT− | CF↓ | CT− | CF↓ | CT↑ | CF↓ | CT− | CF↓ | CT− | |
| (b) double three-phase | cf = 0.2 | ct = 1 | cf = 0.1 | ct = 1 | cf = 0.2 | ct = 0 * | cf = 0.4 | ct = 1 | cf = 0.3 | ct = 1 |
| CF↓ | CT− | CF↓ | CT− | CF↓ | CT↑ | CF↓ | CT− | CF↓ | CT− | |
| (c) double three-phase | cf = 0.2 | ct = 0.6 | cf = 0.3 | ct = 0.6 | cf = 0.2 | ct = 0 * | cf = 0.3 | ct = 0.6 | cf = 0.2 | ct = 0.6 |
| CF↓ | CT− | CF↓ | CT− | CF↓ | CT↑ | CF↓ | CT− | CF↓ | CT− | |
| Variable | Description | Value | Unit |
|---|---|---|---|
| dos | stator iron outer diameter | 266 | mm |
| dor | rotor outer diameter | 150 | mm |
| hz | axial rotor and stator length | 10 | mm |
| δ | magnetic air gap | 3 | mm |
| mw | magnet width | 8.5 | mm |
| ml | magnet length | 54 | mm |
| laminated iron material | M330-35A | ||
| permanent magnet material | NdFeB N38 | ||
| Variable | Description | Value | Unit |
|---|---|---|---|
| kr | radial stiffness | −41.6 | N/mm |
| kz | axial stiffness | 7.3 | N/mm |
| kϕ | tilt stiffness | 21.4 | Nm/rad |
| Jmax | maximum current density | 6 | A/mm2 |
| Fmax | maximum bearing force | 10 | N |
| Tmax | maximum drive torque | 1.5 | Nm |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gruber, W.; Radman, K. Modeling and Realization of a Bearingless Flux-Switching Slice Motor. Actuators 2017, 6, 12. https://doi.org/10.3390/act6020012
Gruber W, Radman K. Modeling and Realization of a Bearingless Flux-Switching Slice Motor. Actuators. 2017; 6(2):12. https://doi.org/10.3390/act6020012
Chicago/Turabian StyleGruber, Wolfgang, and Karlo Radman. 2017. "Modeling and Realization of a Bearingless Flux-Switching Slice Motor" Actuators 6, no. 2: 12. https://doi.org/10.3390/act6020012
APA StyleGruber, W., & Radman, K. (2017). Modeling and Realization of a Bearingless Flux-Switching Slice Motor. Actuators, 6(2), 12. https://doi.org/10.3390/act6020012





