Dynamic Analysis of Vibration Attenuation in Dual-Stage Cascade Spring-Mass System (DCSMS) for High-Precision Instrumentation
Abstract
1. Introduction
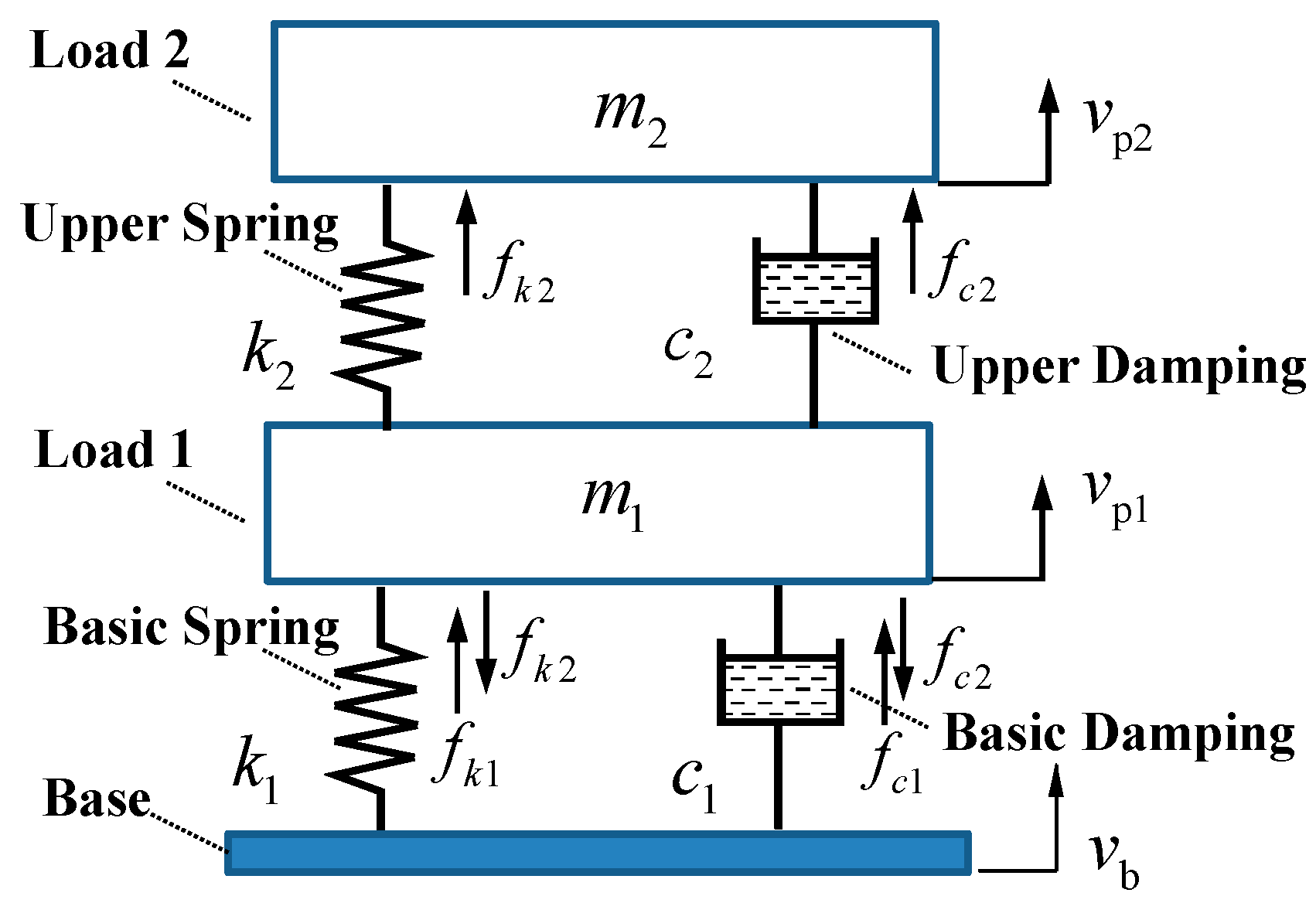
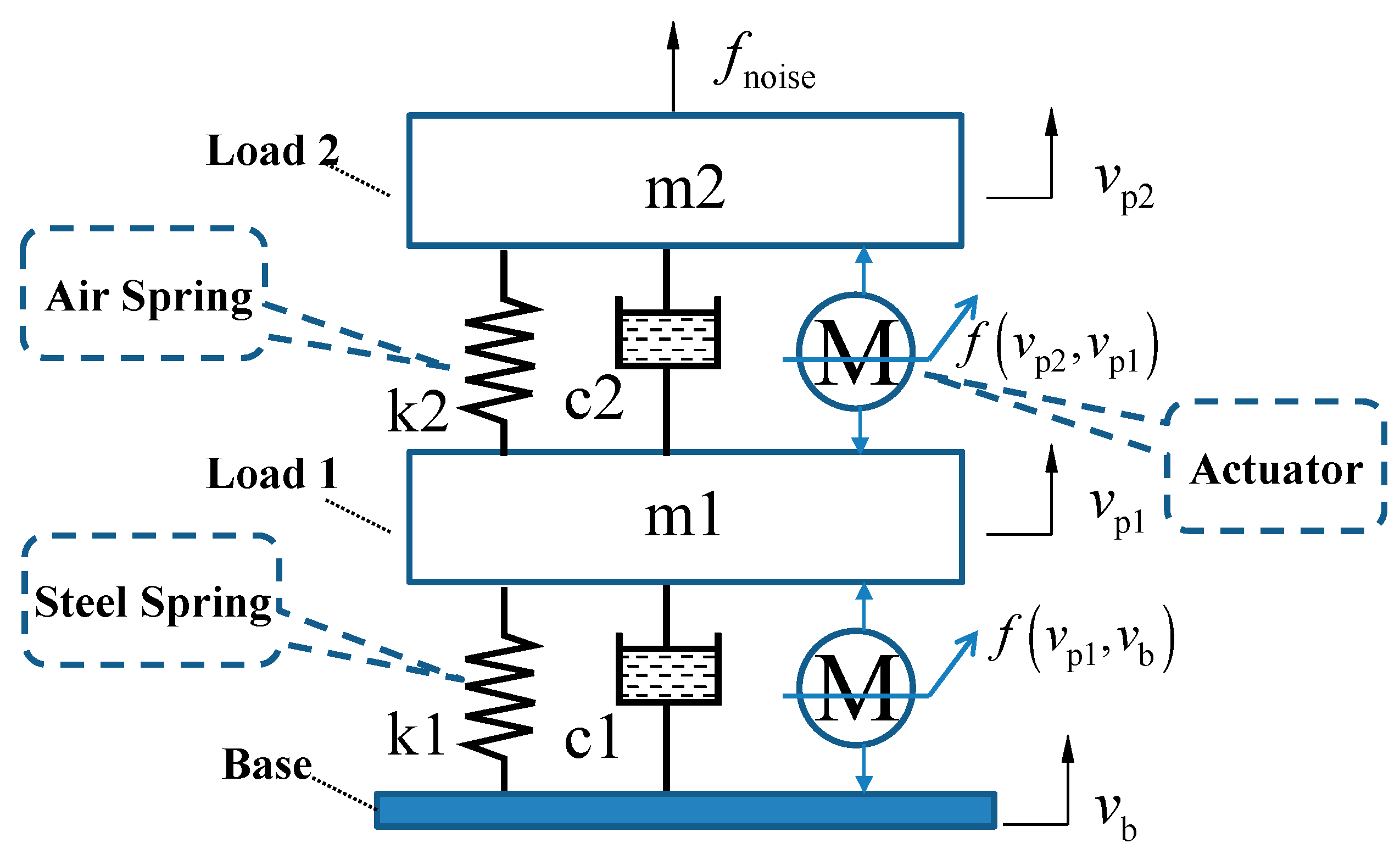
2. Passive Characteristics of the DCSMS
2.1. Analysis of the Vibration Propagation Characteristics of the DCSMS
2.2. DCSMS Stability Analysis
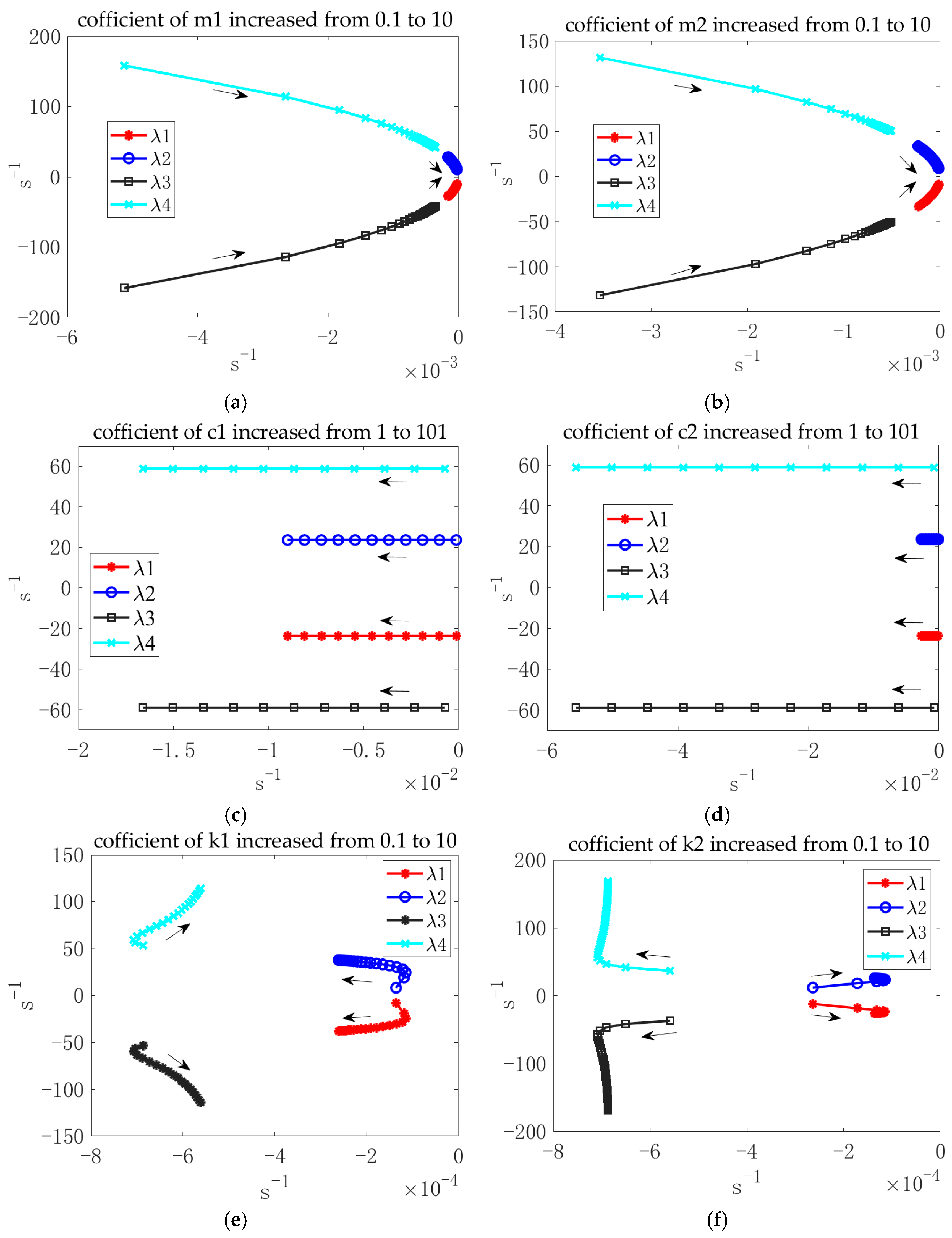
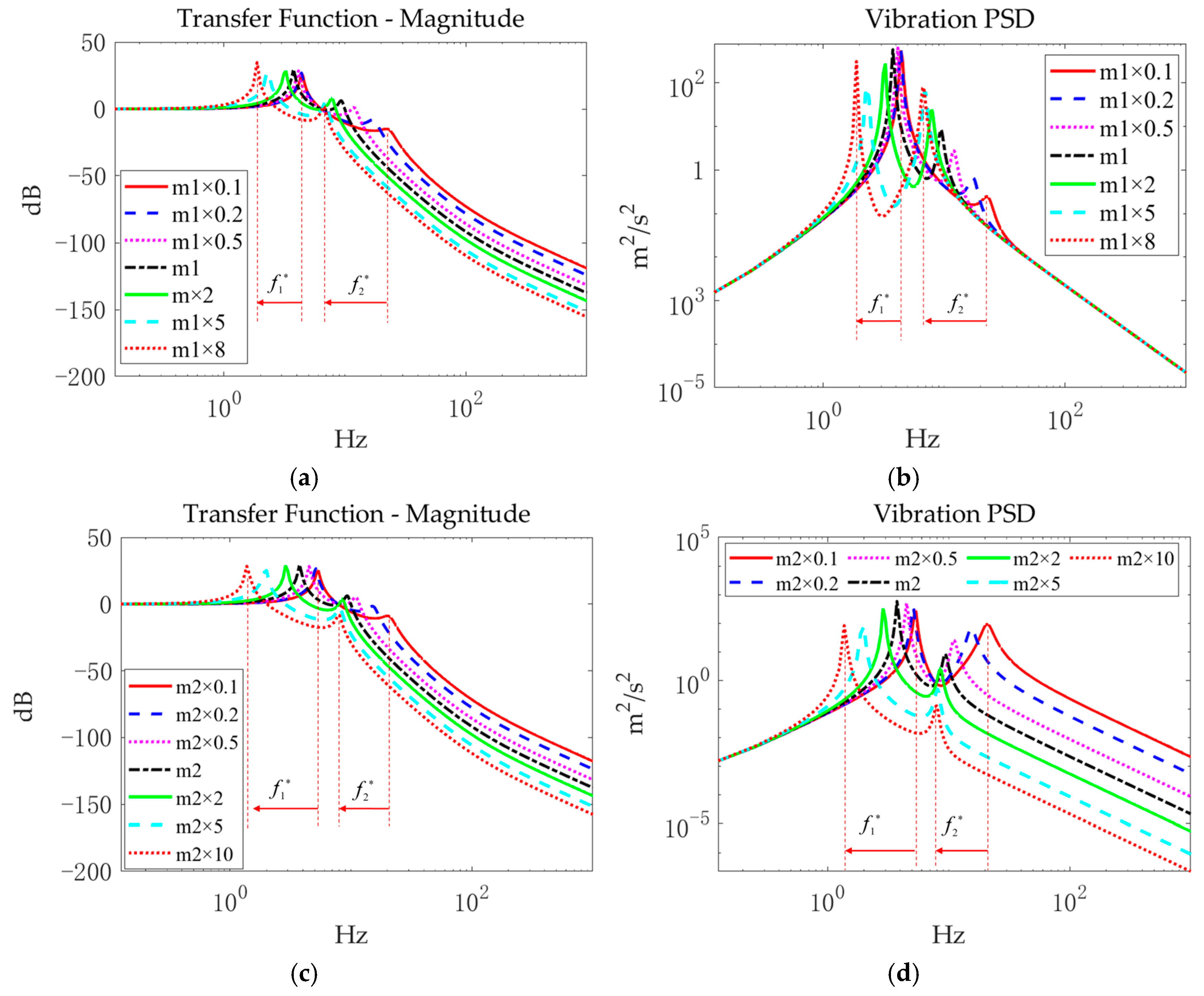
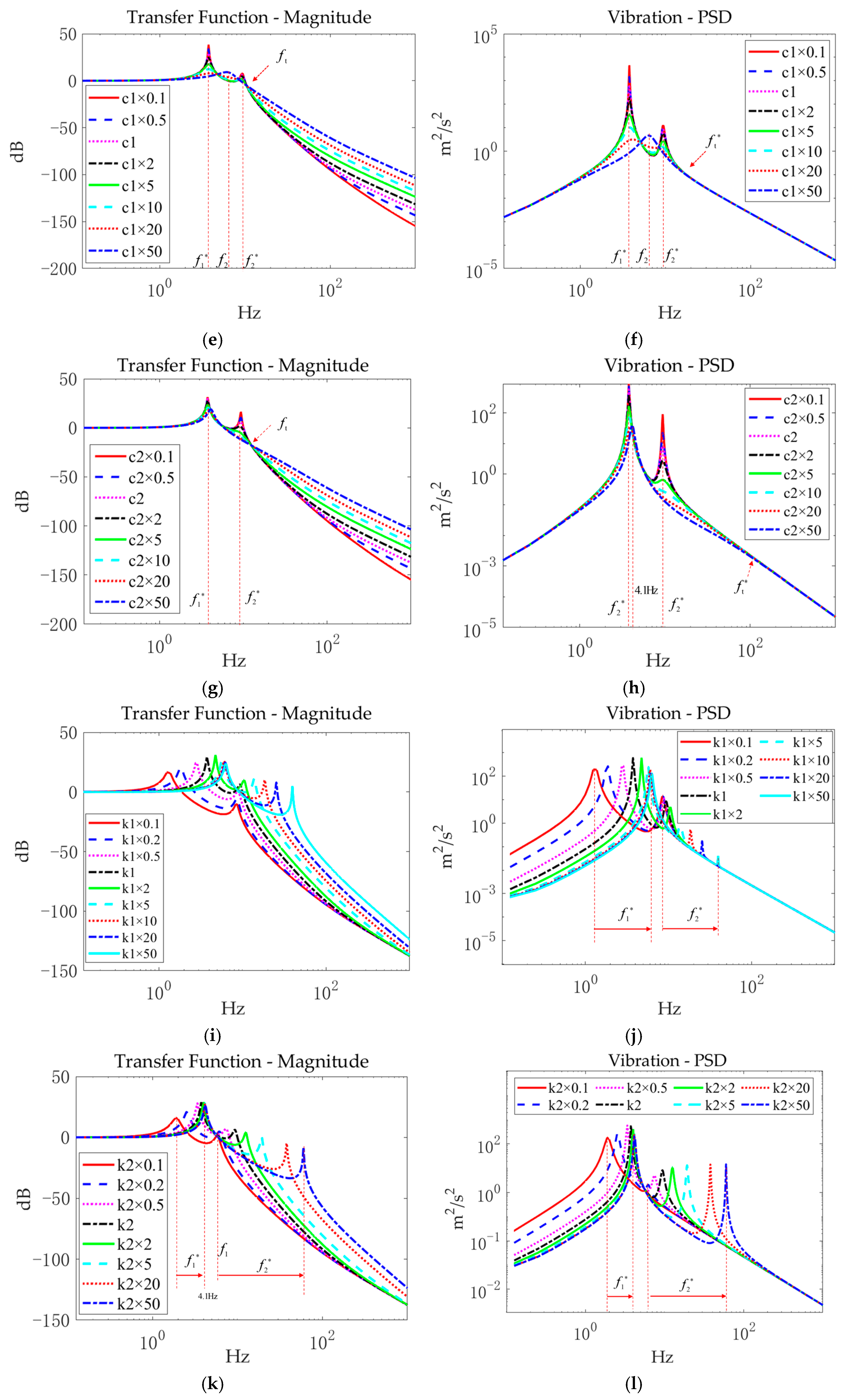
2.3. Influence of Parameters on DCSMS Vibration Isolation Performance
- (1)
- Parametric Influence Analysis of the Characteristic Roots of DCSMS
- (2)
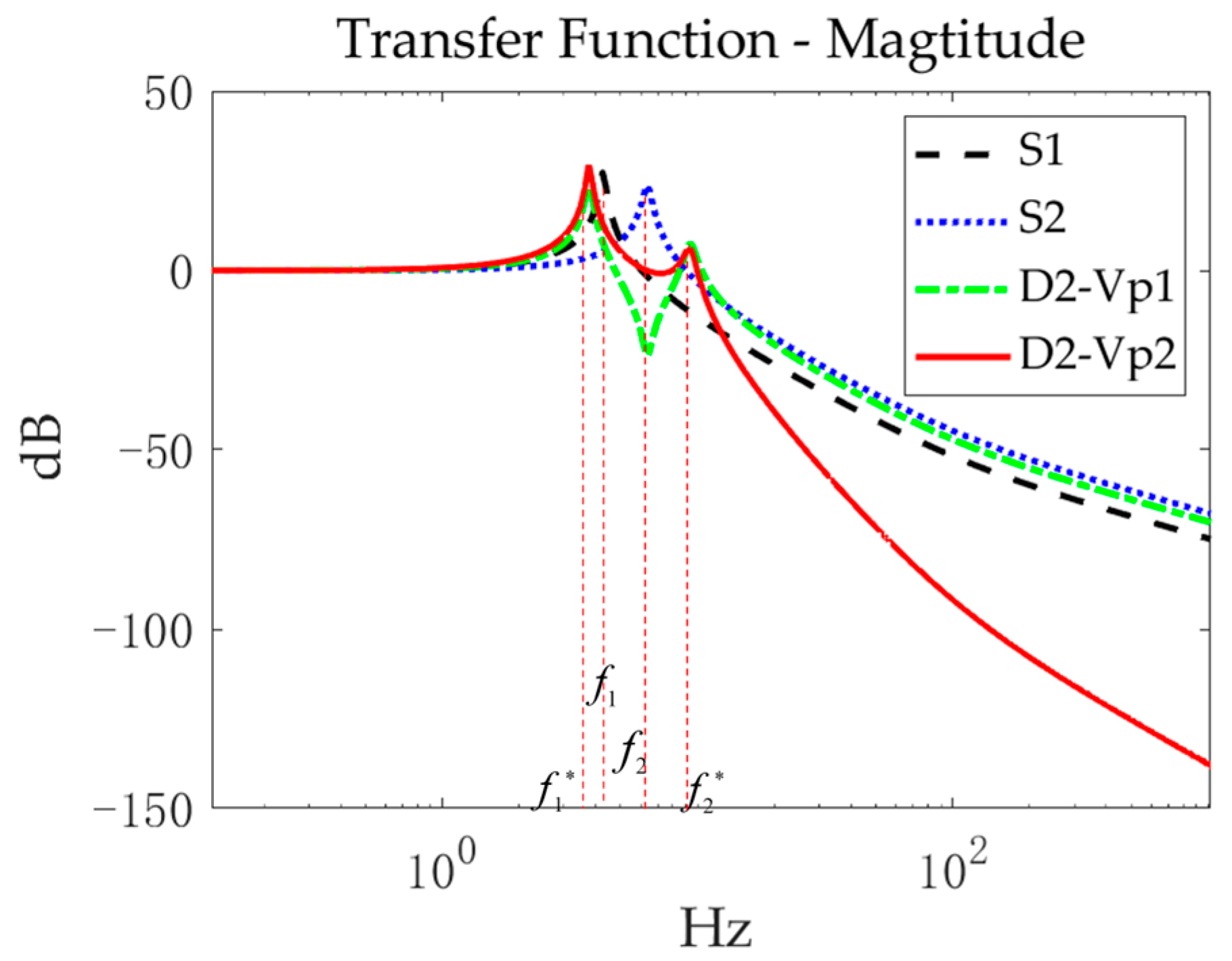
- Frequency Response Characteristics and Power Spectral Density Analysis of DCSMS
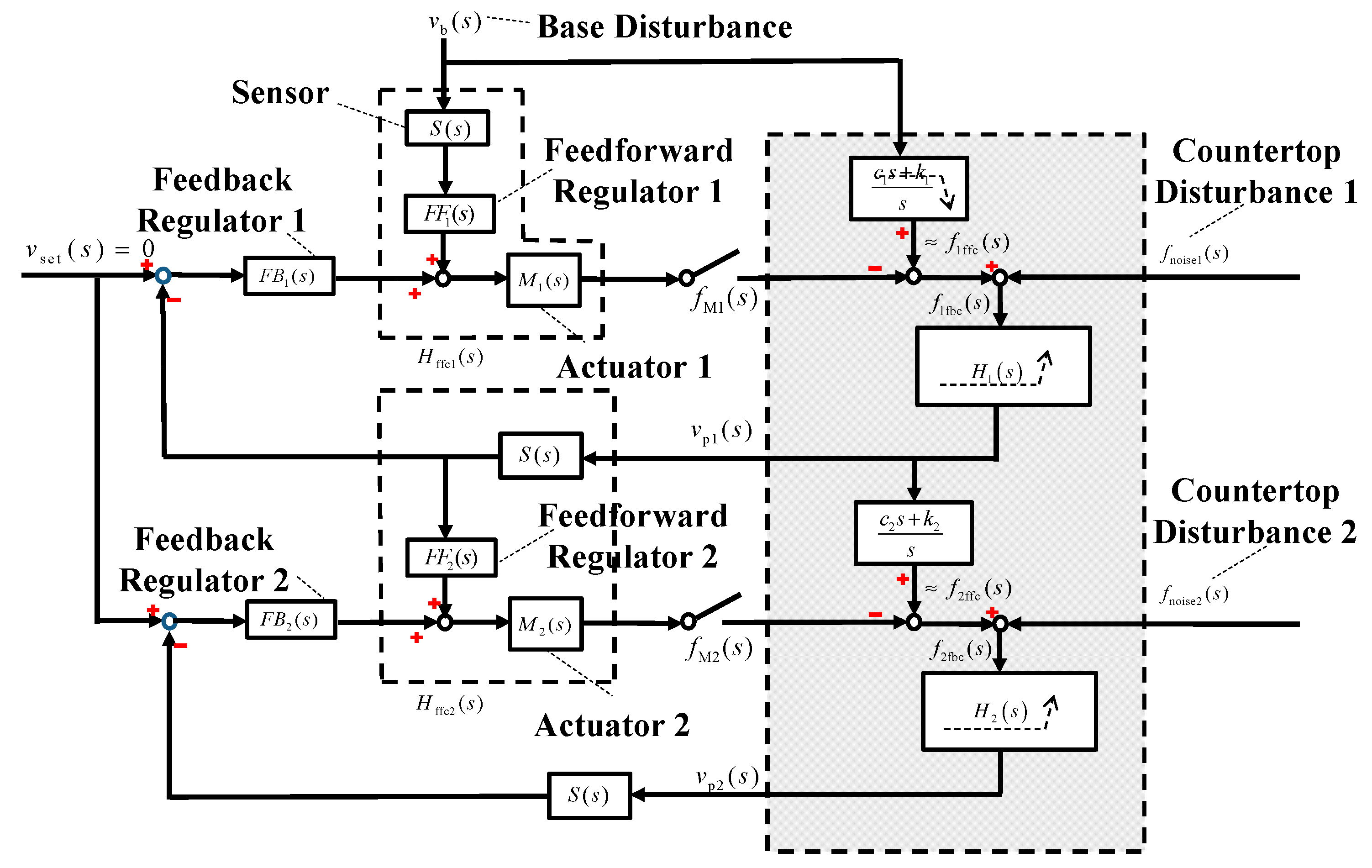
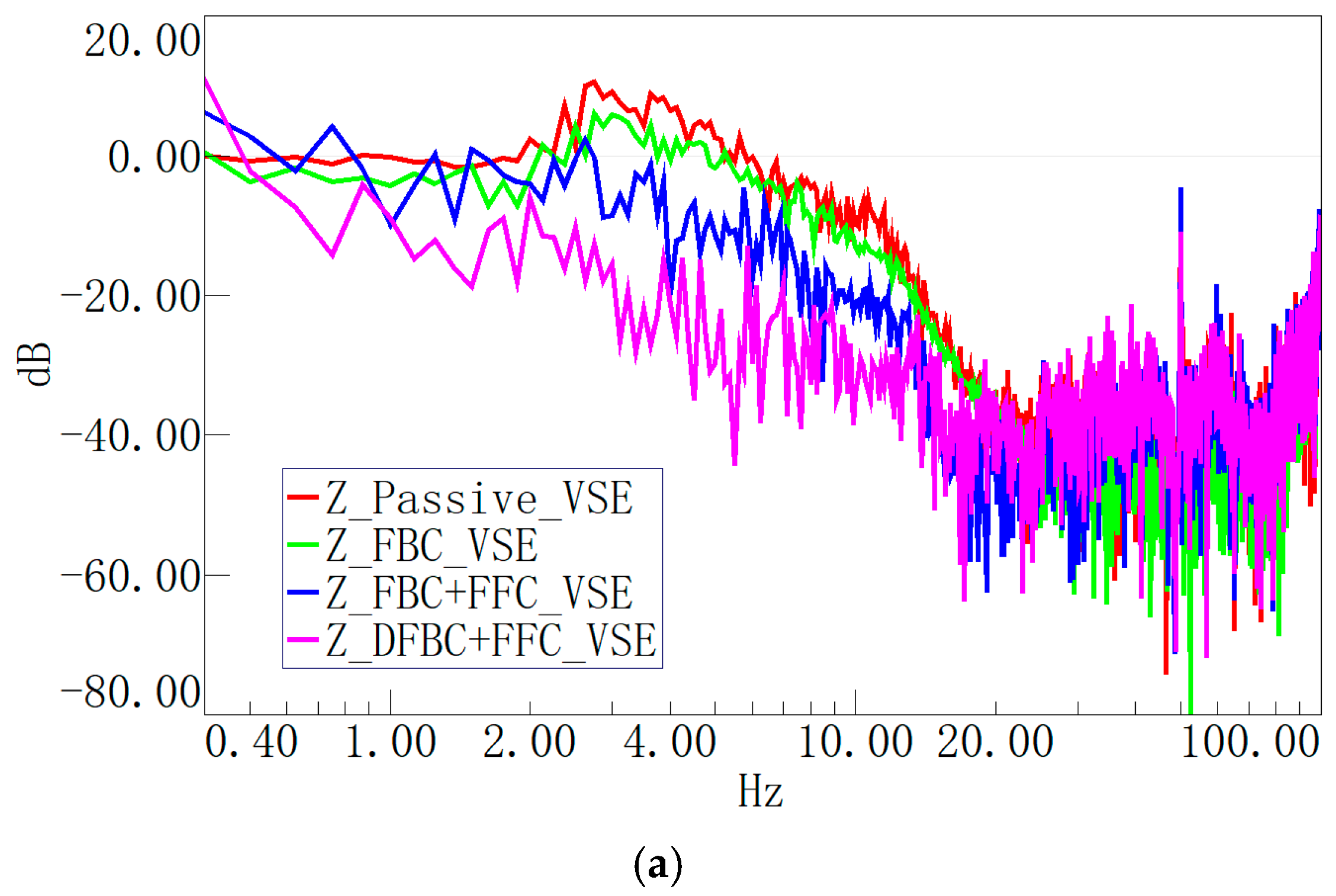
2.4. Analysis of DCSMS Control Characteristics
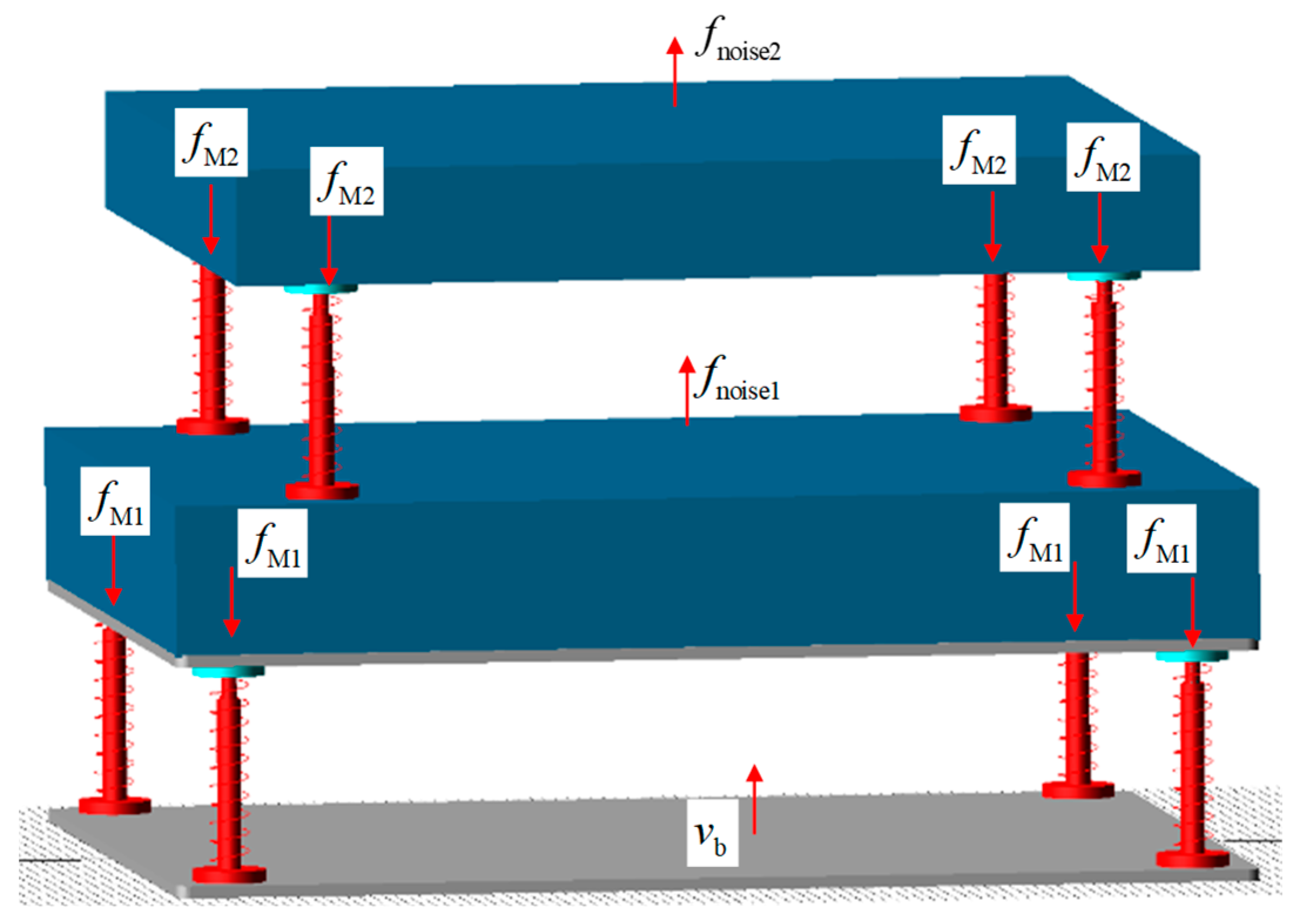
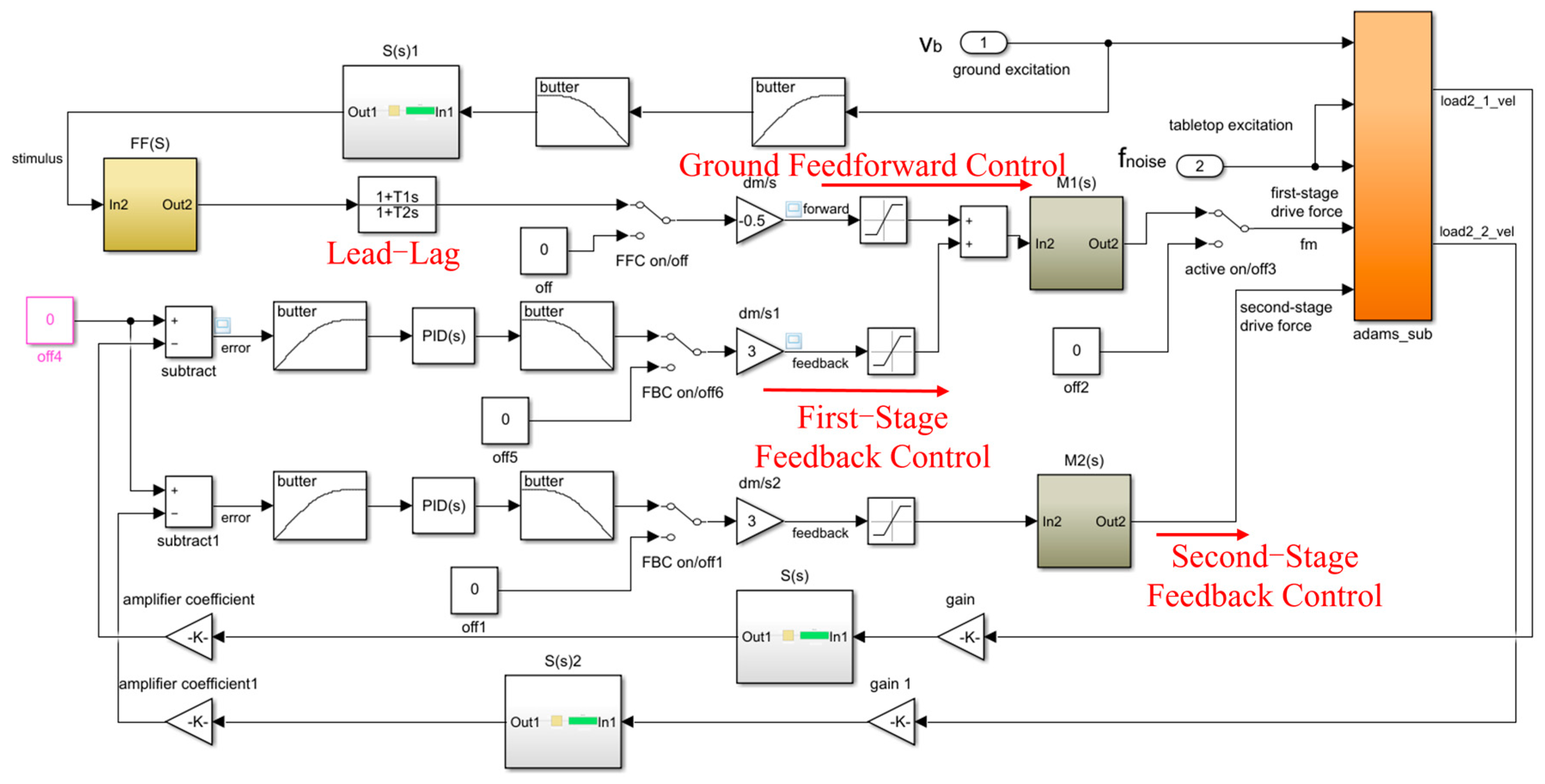
3. Modeling and Simulation of Active Vibration Control in DCSMS
ADAMS and MATLAB DCSMS Co-Simulation
4. Control Experiment and DCSMS Application
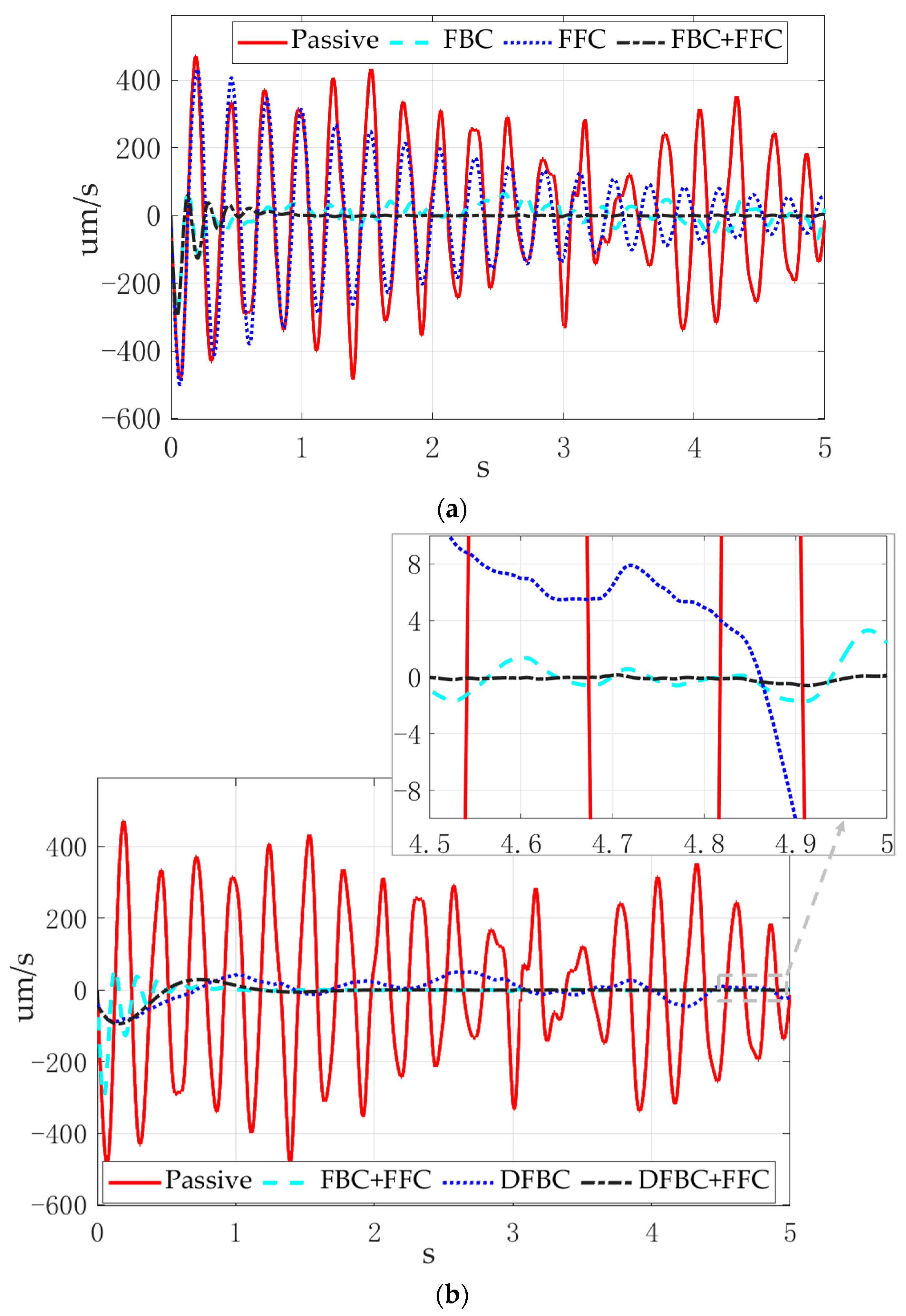
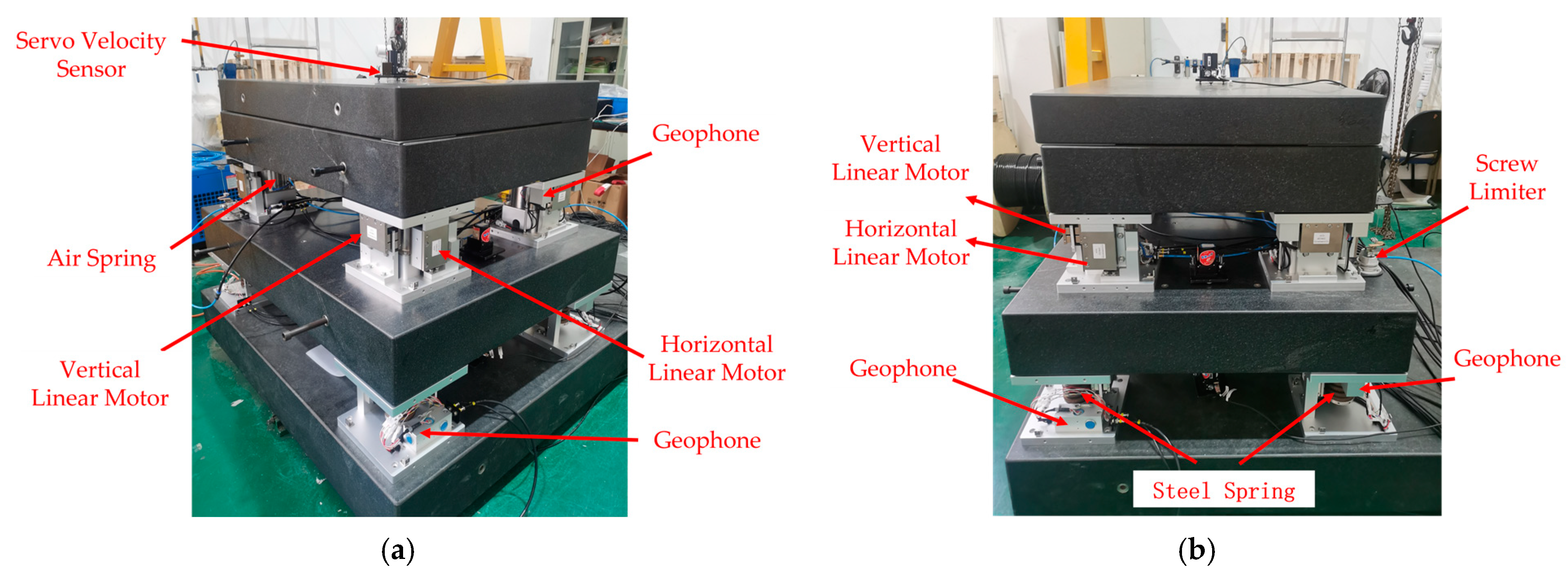
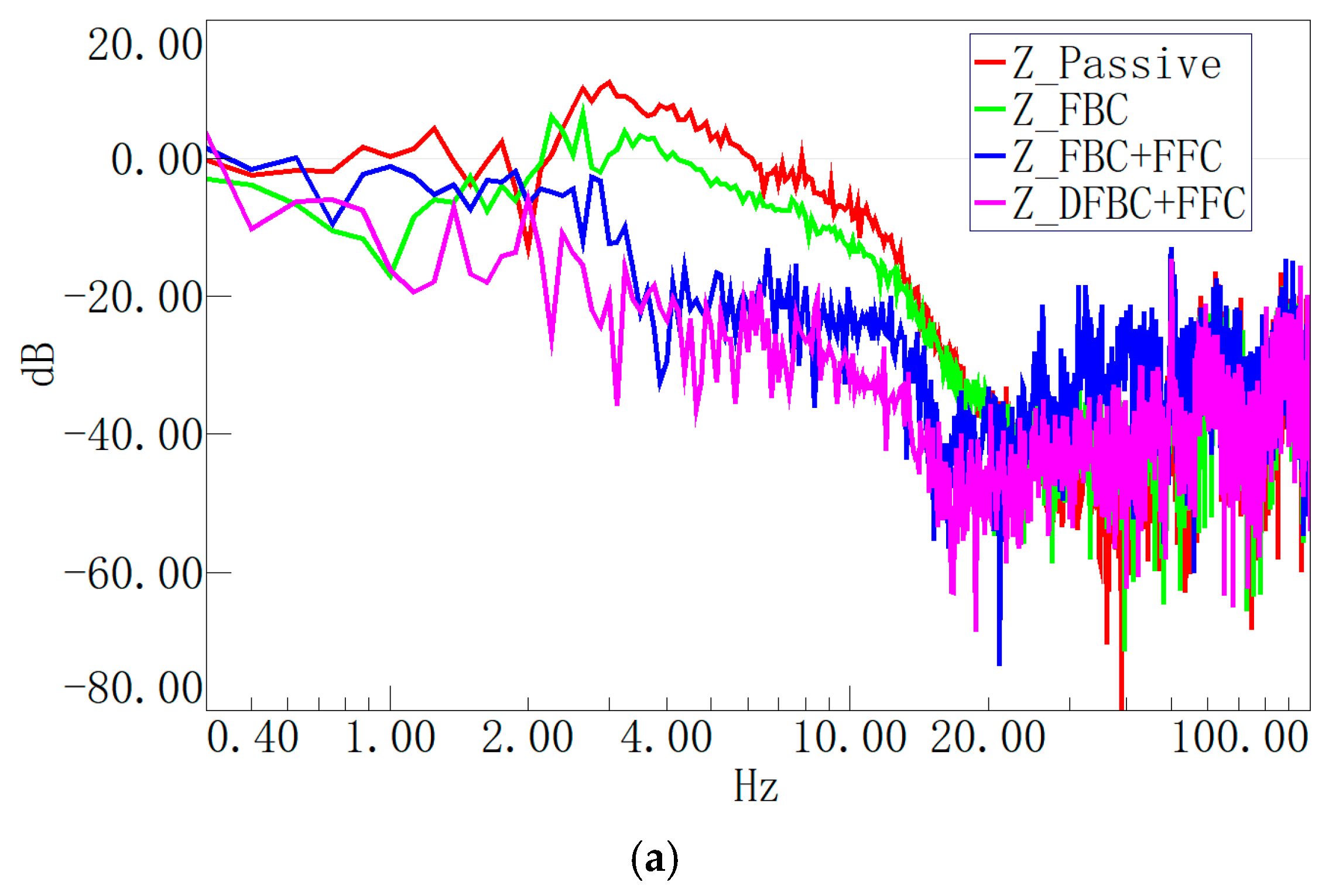
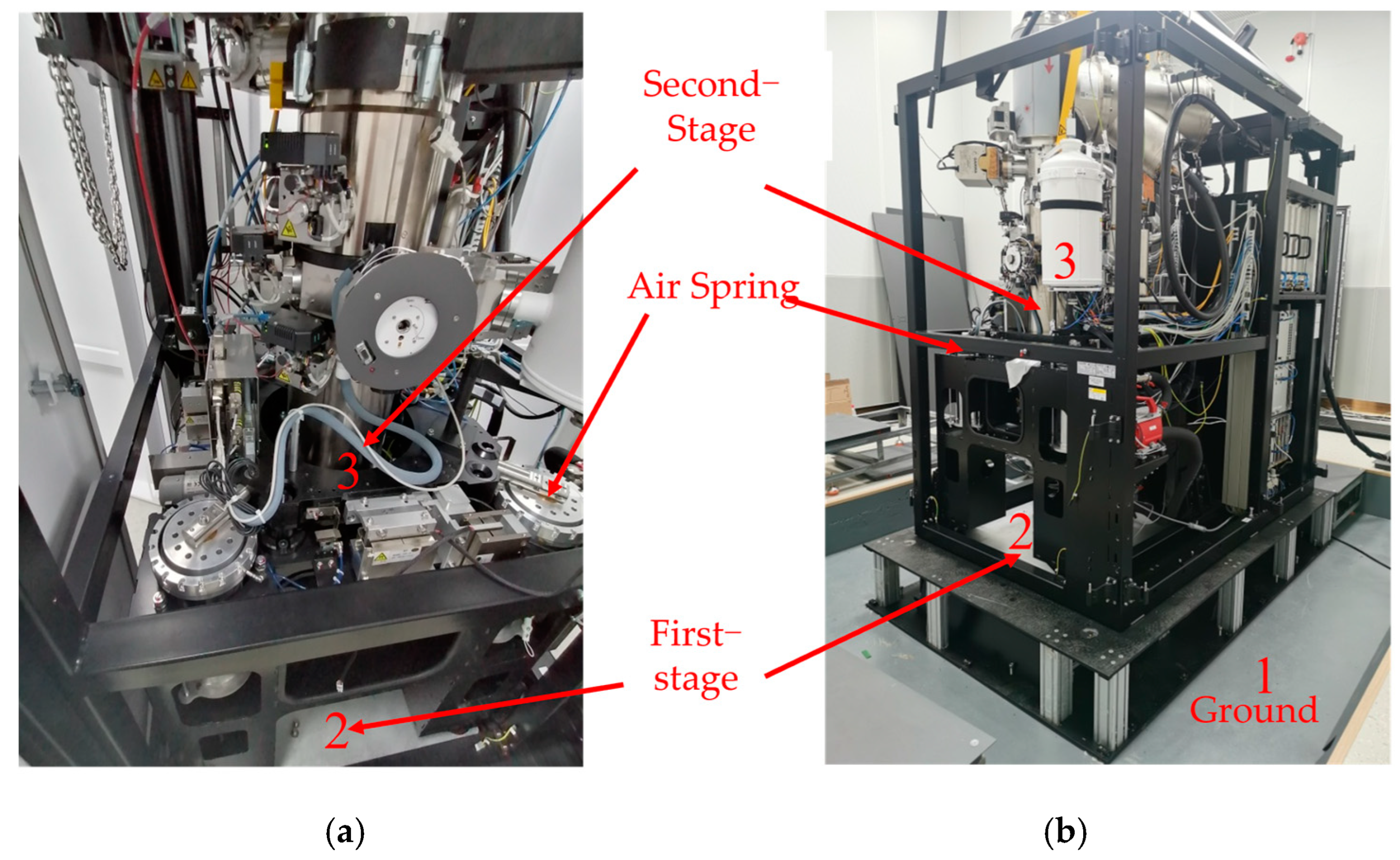
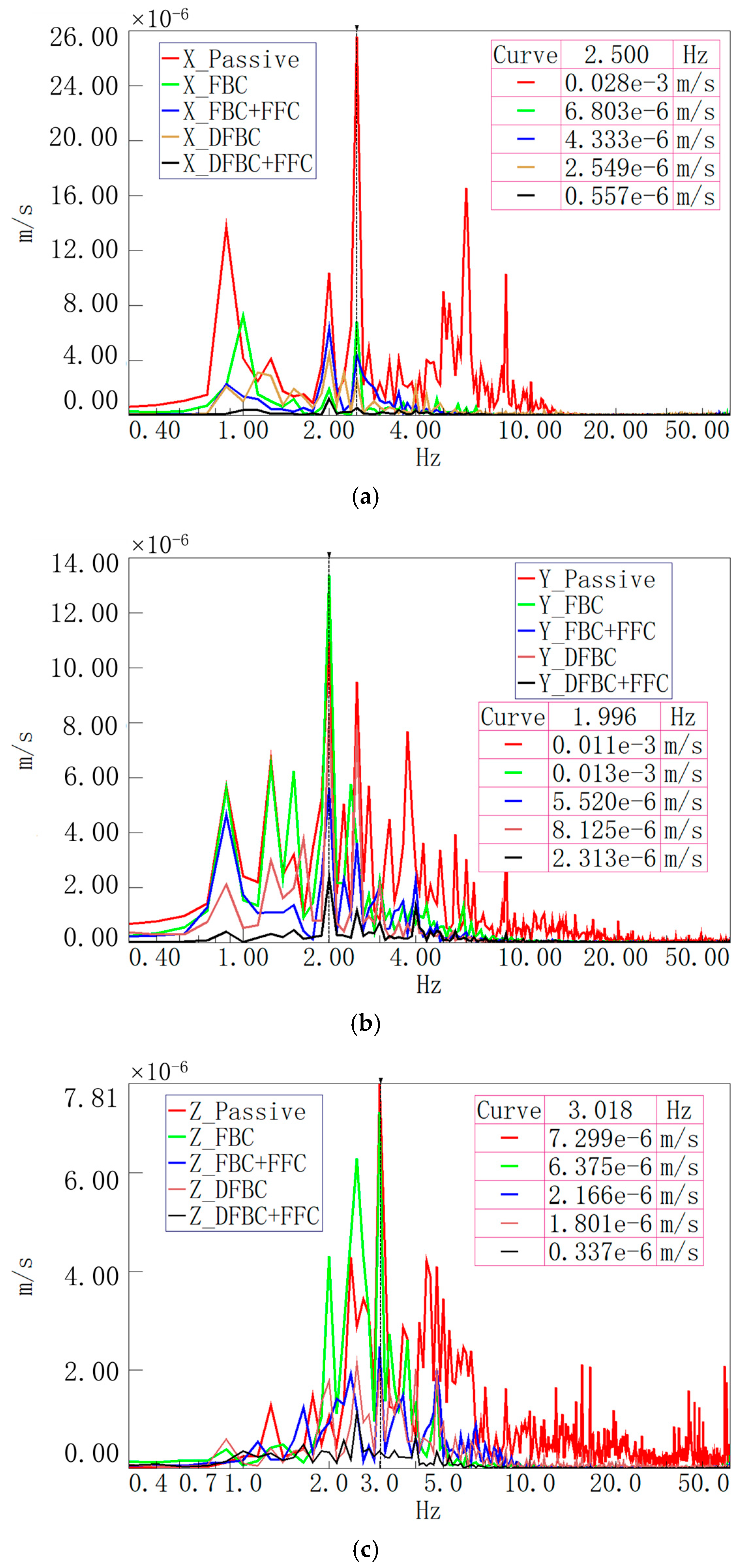
4.1. Control Experiment of DCSMS Prototype
- Sensor measurement accuracy and linearity
- 2.
- Voice-coil actuator drive capability
- 3.
- Controller specifications
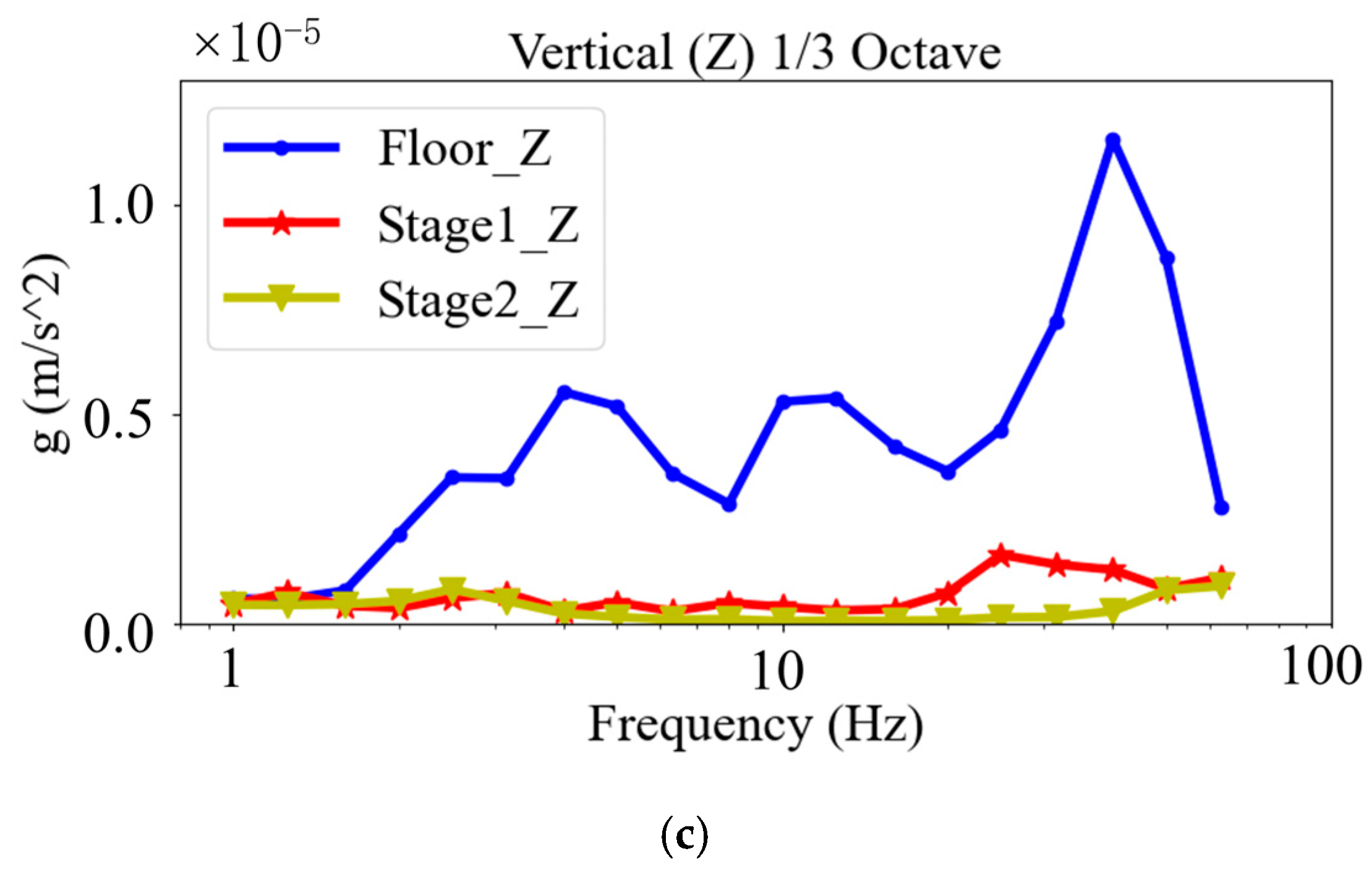
4.2. DCSMS Applications
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Kato, T.; Kawashima, K.; Funaki, T.; Tadano, K.; Kagawa, T. A new, high precision, quick response pressure regulator for active control of pneumatic vibration isolation tables. Precis. Eng. 2010, 34, 43–48. [Google Scholar] [CrossRef]
- Li, Y.; He, L.; Shuai, C.; Wang, C. Improved hybrid isolator with maglev actuator integrated in air spring for active-passive isolation of ship machinery vibration. J. Sound Vib. 2017, 407, 226–239. [Google Scholar] [CrossRef]
- Wu, W.; Chen, X.; Shan, Y. Analysis and experiment of a vibration isolator using a novel magnetic spring with negative stiffness. J. Sound Vib. 2014, 333, 2958–2970. [Google Scholar] [CrossRef]
- Shan, J.; Shi, Z.; Gong, N.; Shi, W. Performance improvement of base isolation systems by incorporating eddy current damping and magnetic spring under earthquakes. Struct. Control Health Monit. 2020, 27, e2524. [Google Scholar] [CrossRef]
- Karnopp, D.; Crosby, M.J.; Harwood, R.A. Vibration Control Using Semi-Active Force Generators. J. Eng. Ind. 1974, 96, 619–626. [Google Scholar] [CrossRef]
- Gordon, C.G. Generic Vibration Criteria or Vibration-Sensitive Equipment. In Proceedings of the SPIE International Symposium on Optical Science, Engineering and Instrumentation, Denver, CO, USA, 18–23 July 1999. [Google Scholar] [CrossRef]
- Yang, F.; Sedaghati, R.; Esmailzadeh, E. Vibration suppression of structures using tuned mass damper technology: A state-of-the-art review. J. Vib. Control 2022, 28, 812–836. [Google Scholar] [CrossRef]
- Shen, Y.-J.; Wang, L.; Yang, S.-P.; Gao, G.-S. Nonlinear dynamical analysis and parameters optimization of four semi-active on-off dynamic vibration absorbers. J. Vib. Control 2013, 19, 143–160. [Google Scholar] [CrossRef]
- Zuo, L.; Slotine, J.-J.E. Robust vibration isolation via frequency-shaped sliding control and modal decomposition. J. Sound Vib. 2005, 285, 1123–1149. [Google Scholar] [CrossRef]
- Soubhia, A.L.; Serpa, A.L. Discrete optimization for actuator and sensor positioning for vibration control using genetic algorithms. J. Vib. Control 2018, 24, 4050–4064. [Google Scholar] [CrossRef]
- Jin, X.; Ma, H.; Tang, J.; Kang, Y. A Self-Adaptive Vibration Reduction Method Based on Deep Deterministic Policy Gradient (DDPG) Reinforcement Learning Algorithm. Appl. Sci. 2022, 12, 9703. [Google Scholar] [CrossRef]
- Yun, H.; Kong, D.; Aoyagi, M. Development of a multi-drive-mode piezoelectric linear actuator with parallel-arrangement dual stator. Precis. Eng. 2022, 77, 127–140. [Google Scholar] [CrossRef]
- Ouyang, H.; Zhang, J. Passive modifications for partial assignment of natural frequencies of mass-spring systems. Mech. Syst. Signal Process. 2015, 50–51, 214–226. [Google Scholar] [CrossRef]
- Zhu, S.J.; Zheng, Y.F.; Fu, Y.M. Analysis of non-linear dynamics of a two-degree-of-freedom vibration system with non-linear damping and non-linear spring. J. Sound Vib. 2004, 271, 15–24. [Google Scholar] [CrossRef]
- Griffin, S.; Gussy, J.; Lane, S.A.; Henderson, B.K.; Sciulli, D. Virtual Skyhook Vibration Isolation System. J. Vib. Acoust. 2002, 124, 63–67. [Google Scholar] [CrossRef]
- Wang, Q.; Zhou, J.; Wang, K.; Lin, Q.; Tan, D.; Xu, D.; Wen, G. Design and experimental study of a two-stage nonlinear vibration isolator with quasi-zero stiffness. Commun. Nonlinear Sci. Numer. Simul. 2023, 122, 107246. [Google Scholar] [CrossRef]
- Liu, Z.; Xie, Q.; Li, C. Further results on inverse eigenvalue problem for mass-spring-inerter systems. Mech. Syst. Signal Process. 2023, 204, 110719. [Google Scholar] [CrossRef]
- Hu, Y.; Chen, M.Z.Q.; Smith, M.C. Natural frequency assignment for mass-chain systems with inerters. Mech. Syst. Signal Process. 2018, 108, 126–139. [Google Scholar] [CrossRef]
- Li, H.; Chen, M.Z.Q. Achievable dynamic responses of general damped mass-chain systems. J. Frankl. Inst. 2024, 361, 341–356. [Google Scholar] [CrossRef]
- Li, P.; Lam, J.; Cheung, K.C. H ∞ control of periodic piecewise vibration systems with actuator saturation. J. Vib. Control 2017, 23, 3377–3391. [Google Scholar] [CrossRef]
- Uyar, M.; Ergün, M. Investigation of vibration performance of pure active single and multi-feedback controls for a tall RC building. Structures 2023, 50, 615–635. [Google Scholar] [CrossRef]
- Beijen, M.A.; Heertjes, M.F.; Van Dijk, J.; Hakvoort, W.B.J. Self-tuning MIMO disturbance feedforward control for active hard-mounted vibration isolators. Control Eng. Pract. 2018, 72, 90–103. [Google Scholar] [CrossRef]
- Beijen, M.A.; Heertjes, M.F.; Butler, H.; Steinbuch, M. Mixed feedback and feedforward control design for multi-axis vibration isolation systems. Mechatronics 2019, 61, 106–116. [Google Scholar] [CrossRef]
- Zheng, Y.; Shangguan, W.-B.; Rakheja, S. Modeling and analysis of time-domain nonlinear characteristics of air spring with an auxiliary chamber. Mech. Syst. Signal Process. 2022, 176, 109161. [Google Scholar] [CrossRef]




| (kg) | (kg) | (N/mm) | (N/mm) | ||
|---|---|---|---|---|---|
| 988.8 | 748.8 | 0.49 | 0.49 | 300 | 300 |
| Mode Number | Undamped Natural Frequency (Hz) | Damping Ratio | Real | Imaginary |
|---|---|---|---|---|
| 2 | 3.764679 | 1.931758 × 10−2 | −7.272447 × 10−2 | +/−3.763976 |
| 3 | 9.362550 | 4.804175 × 10−2 | −4.497933 × 10−1 | +/−9.351740 |
| Component | Parameter | Value |
|---|---|---|
| Air Spring | Membrane radius | 0.03 m |
| Maximum pressure | 0.8 MPa | |
| Working pressure | 0.4 MPa | |
| Maximum working stroke | 0.075 m | |
| Maximum load (four isolators) | 2000 kg | |
| Module weight | 27 kg | |
| Helical Spring | Stiffness | 310 N/mm |
| Maximum load | 500 kg | |
| Outside diameter | 88 mm | |
| Inside diameter | 55 mm | |
| Voice Coil Actuator | Force sensitivity | 80.3 V/m/s |
| Peak force | 100 N | |
| Total stroke | 2 mm | |
| Response bandwidth | DC~200 Hz | |
| Marble 1 | Weight | 700 Kg |
| Marble 2 | Weight | 750 Kg |
| Geophone | Natural frequency | 4.5 Hz |
| Sensitivity | 100.4 V/m/s | |
| Open circuit damping | 0.42 |
| Normalization Factor | X | Y | Z |
|---|---|---|---|
| Passive | 1.000 | 1.000 | 1.000 |
| FBC | 0.243 | 1.182 | 0.873 |
| FBC + FFC | 0.155 | 0.504 | 0.297 |
| DFBC | 0.091 | 0.751 | 0.144 |
| DFBC + FFC | 0.021 | 0.214 | 0.052 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jin, X.; Kang, Y.; Huang, Z. Dynamic Analysis of Vibration Attenuation in Dual-Stage Cascade Spring-Mass System (DCSMS) for High-Precision Instrumentation. Actuators 2025, 14, 179. https://doi.org/10.3390/act14040179
Jin X, Kang Y, Huang Z. Dynamic Analysis of Vibration Attenuation in Dual-Stage Cascade Spring-Mass System (DCSMS) for High-Precision Instrumentation. Actuators. 2025; 14(4):179. https://doi.org/10.3390/act14040179
Chicago/Turabian StyleJin, Xin, Yihua Kang, and Zhiwei Huang. 2025. "Dynamic Analysis of Vibration Attenuation in Dual-Stage Cascade Spring-Mass System (DCSMS) for High-Precision Instrumentation" Actuators 14, no. 4: 179. https://doi.org/10.3390/act14040179
APA StyleJin, X., Kang, Y., & Huang, Z. (2025). Dynamic Analysis of Vibration Attenuation in Dual-Stage Cascade Spring-Mass System (DCSMS) for High-Precision Instrumentation. Actuators, 14(4), 179. https://doi.org/10.3390/act14040179






