Performance Enhancement of MRAC via Generalized Dynamic Inversion
Abstract
1. Introduction
2. Generalized Dynamic Inversion-Based Model Reference Control
3. Generalized Dynamic Inversion-based Model Reference Adaptive Control
3.1. Classical MRAC
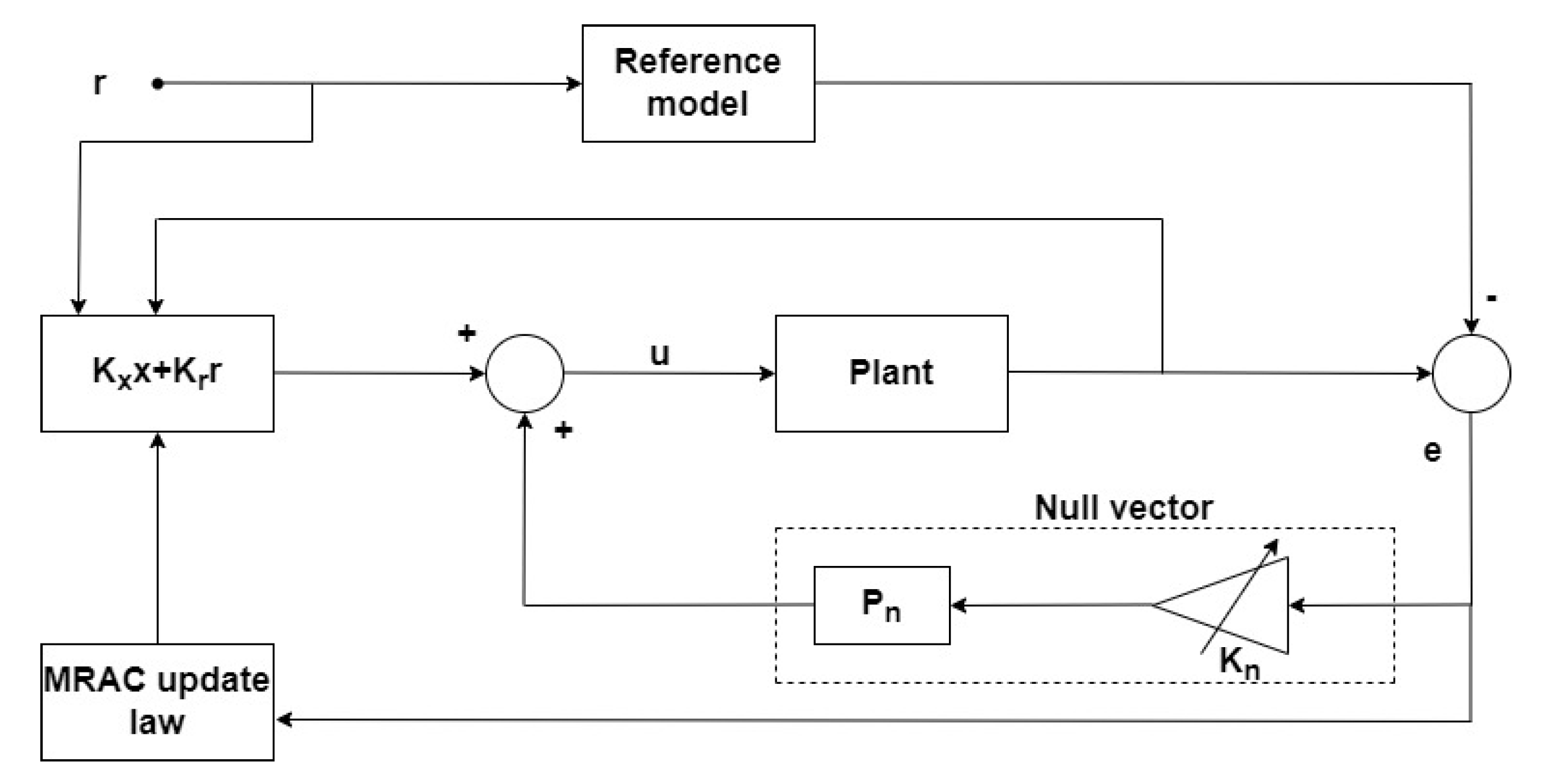
3.2. Modified MRAC
4. Application to Aircraft Longitudinal and Lateral Directional Control
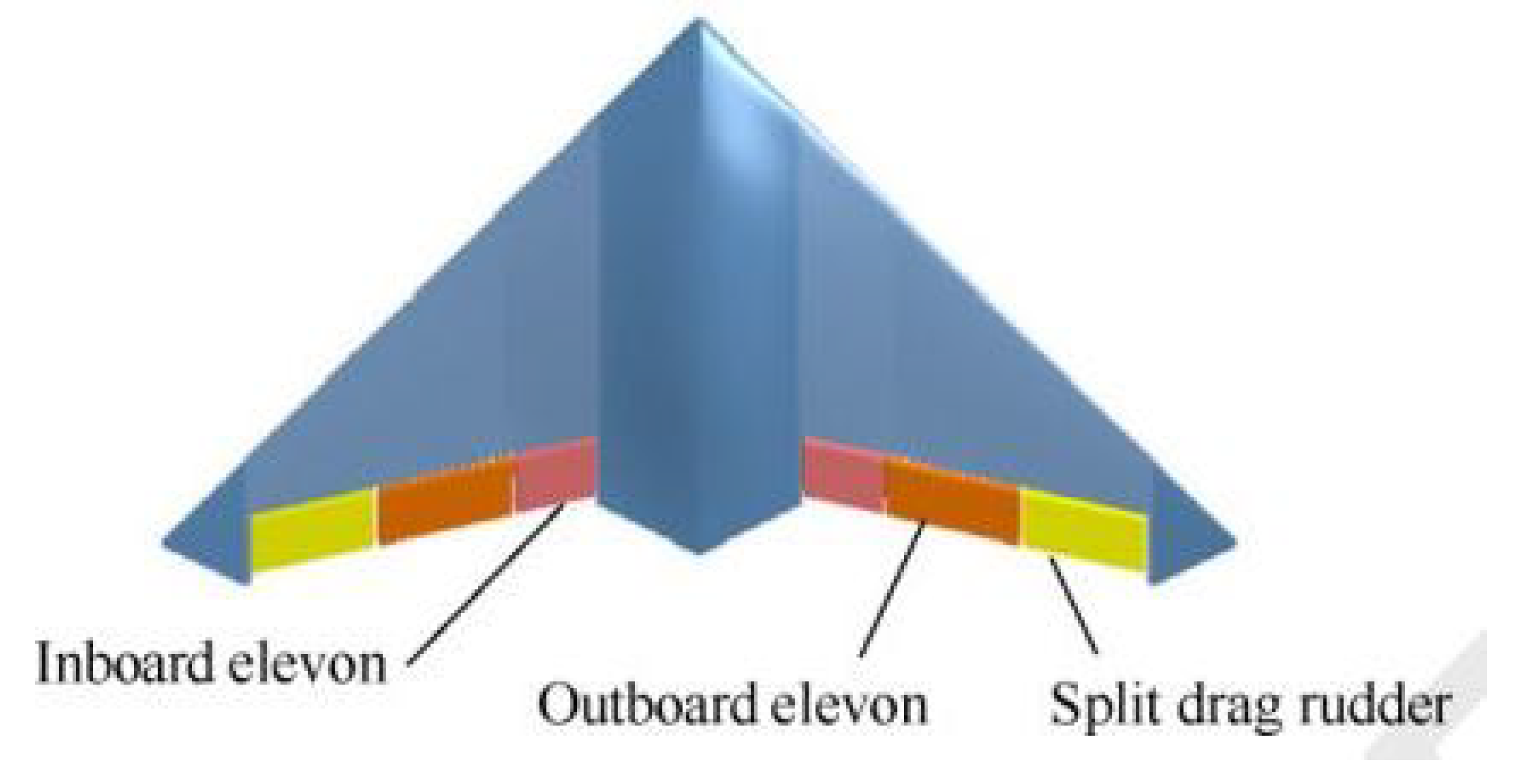
4.1. Aircraft Dynamic Model
4.2. Reference Model
4.2.1. First Constraint {r} Configuration
4.2.2. Second Constraint {q} Configuration
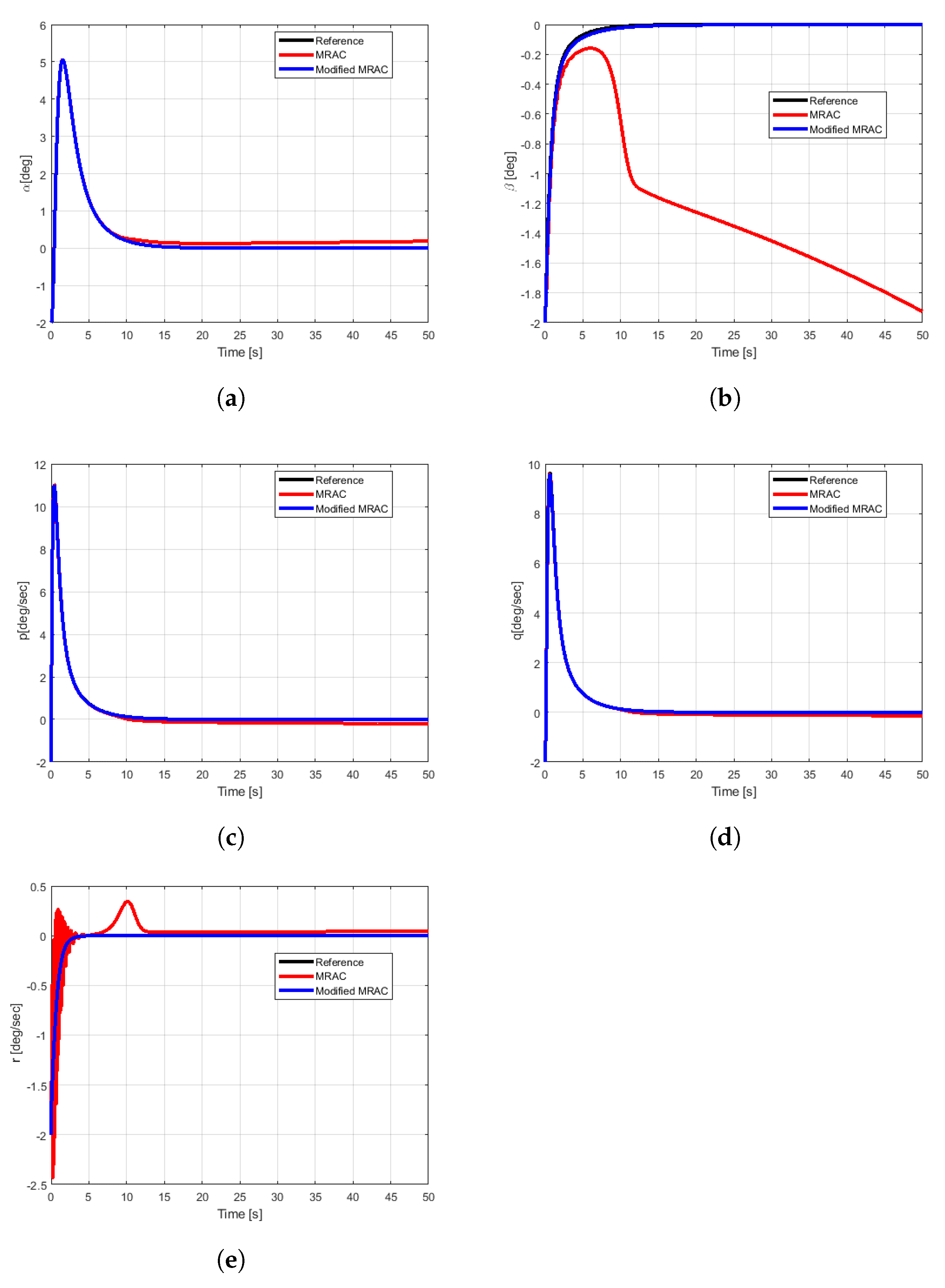
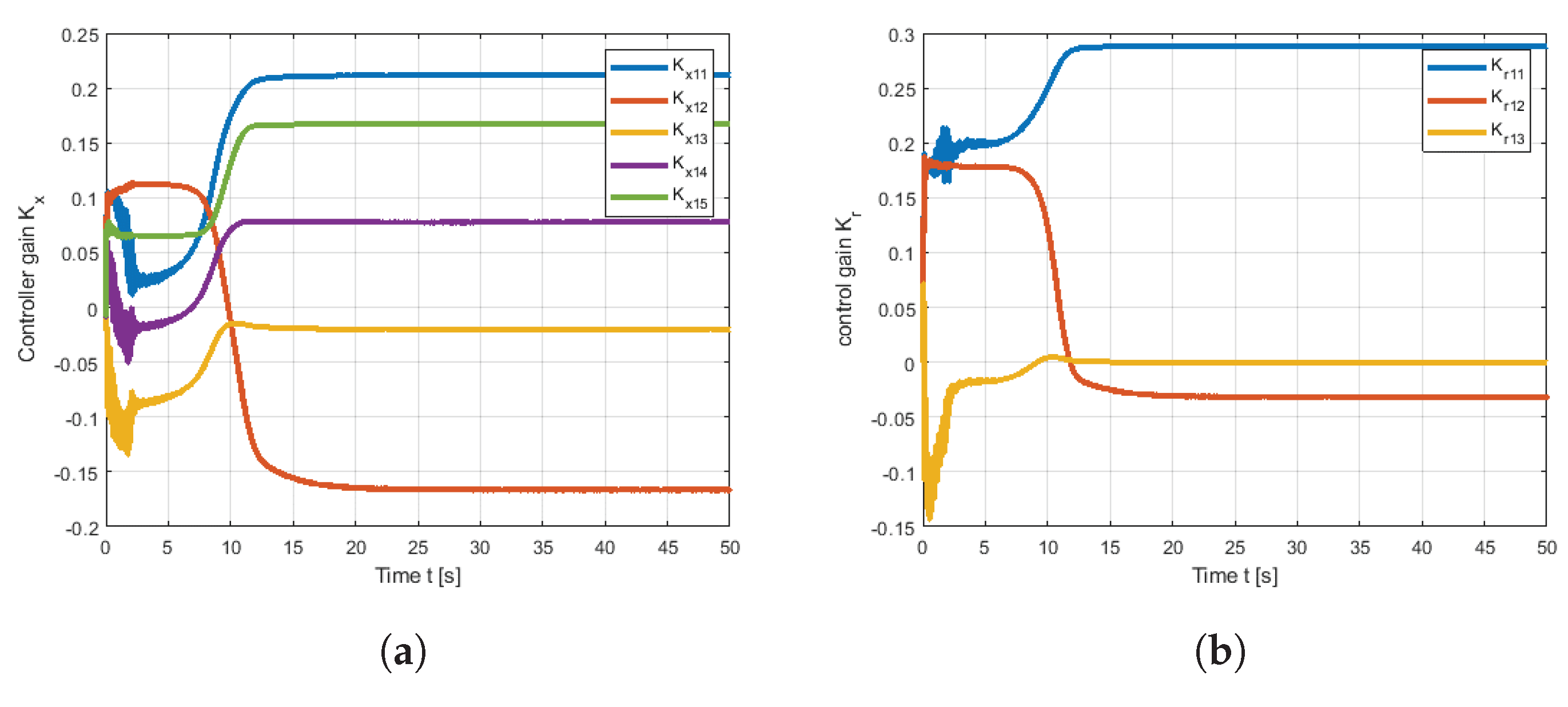
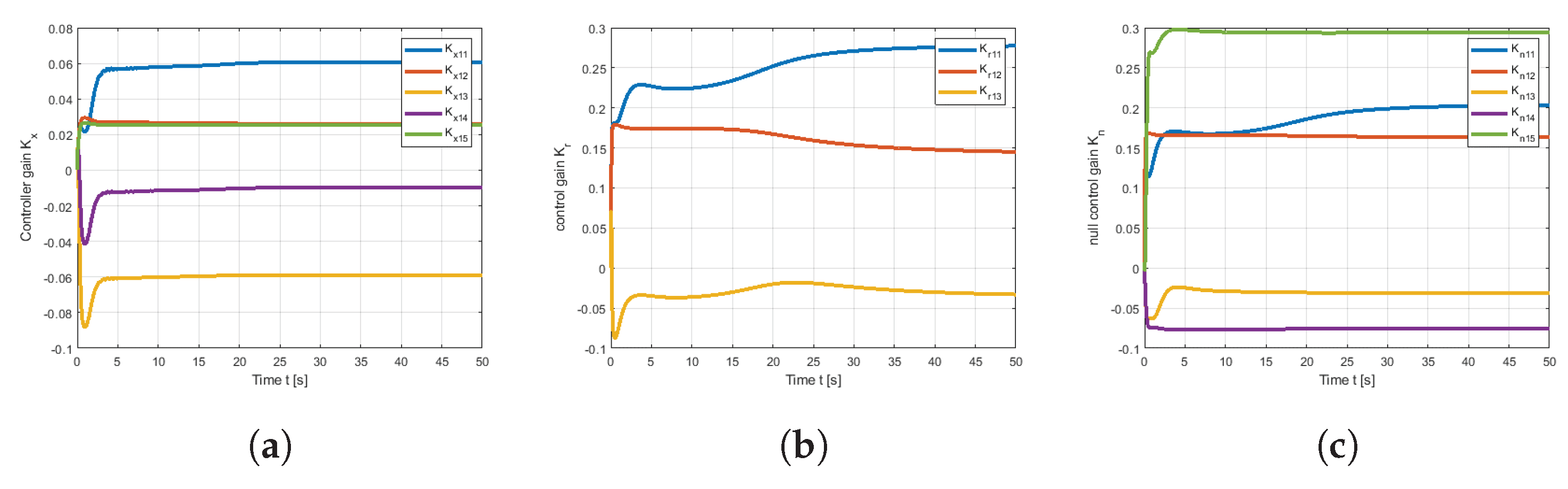
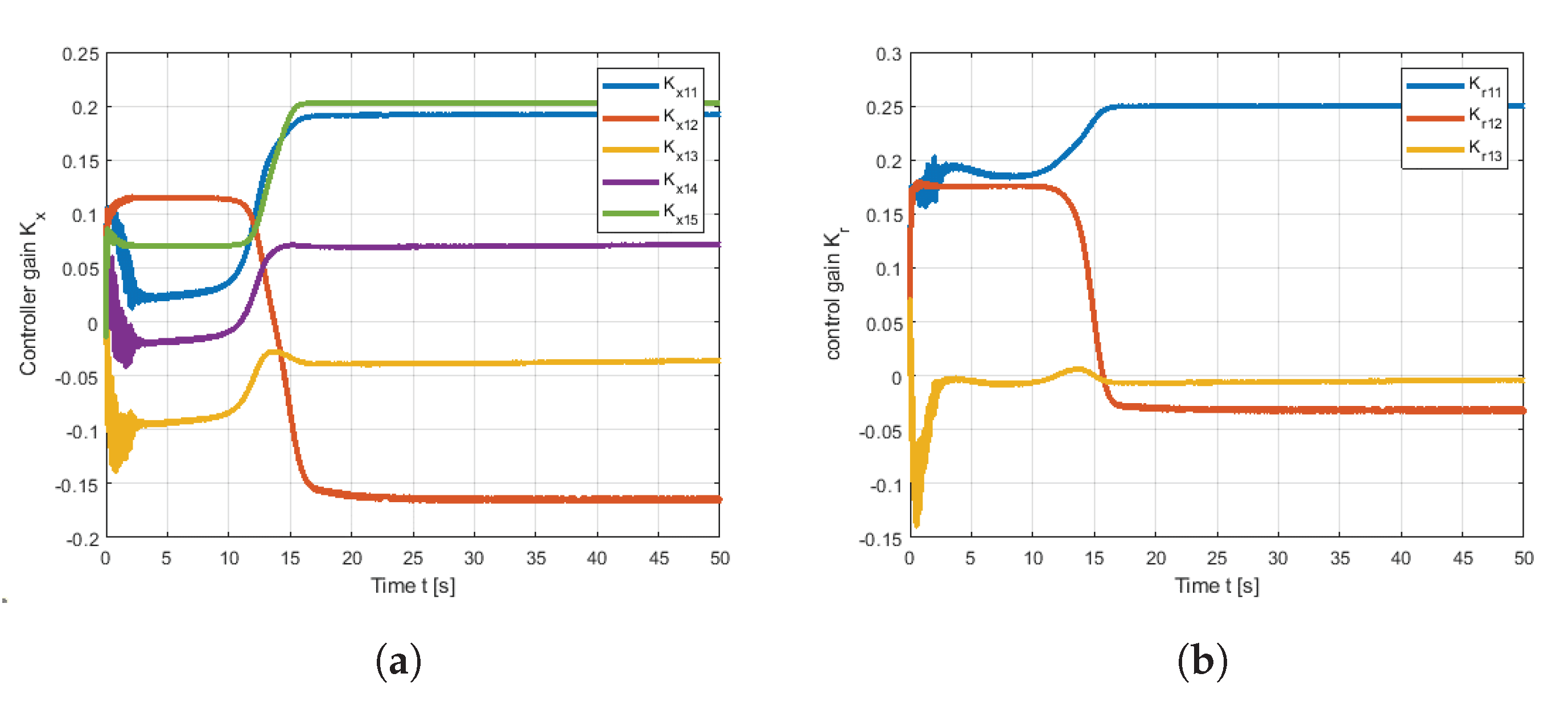
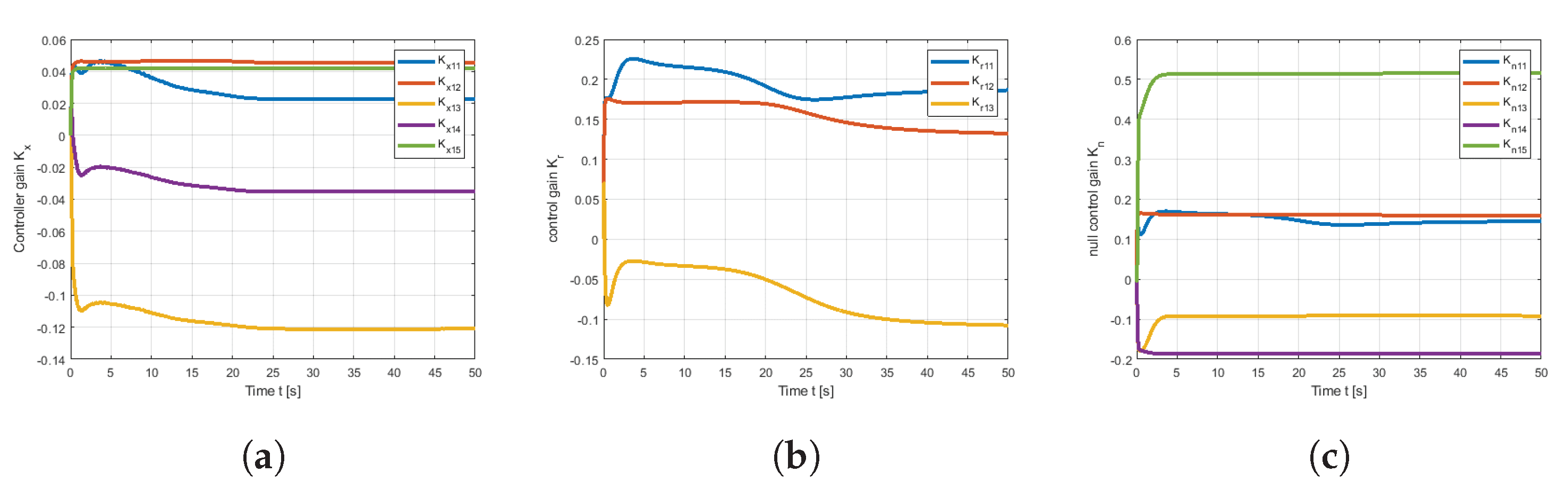
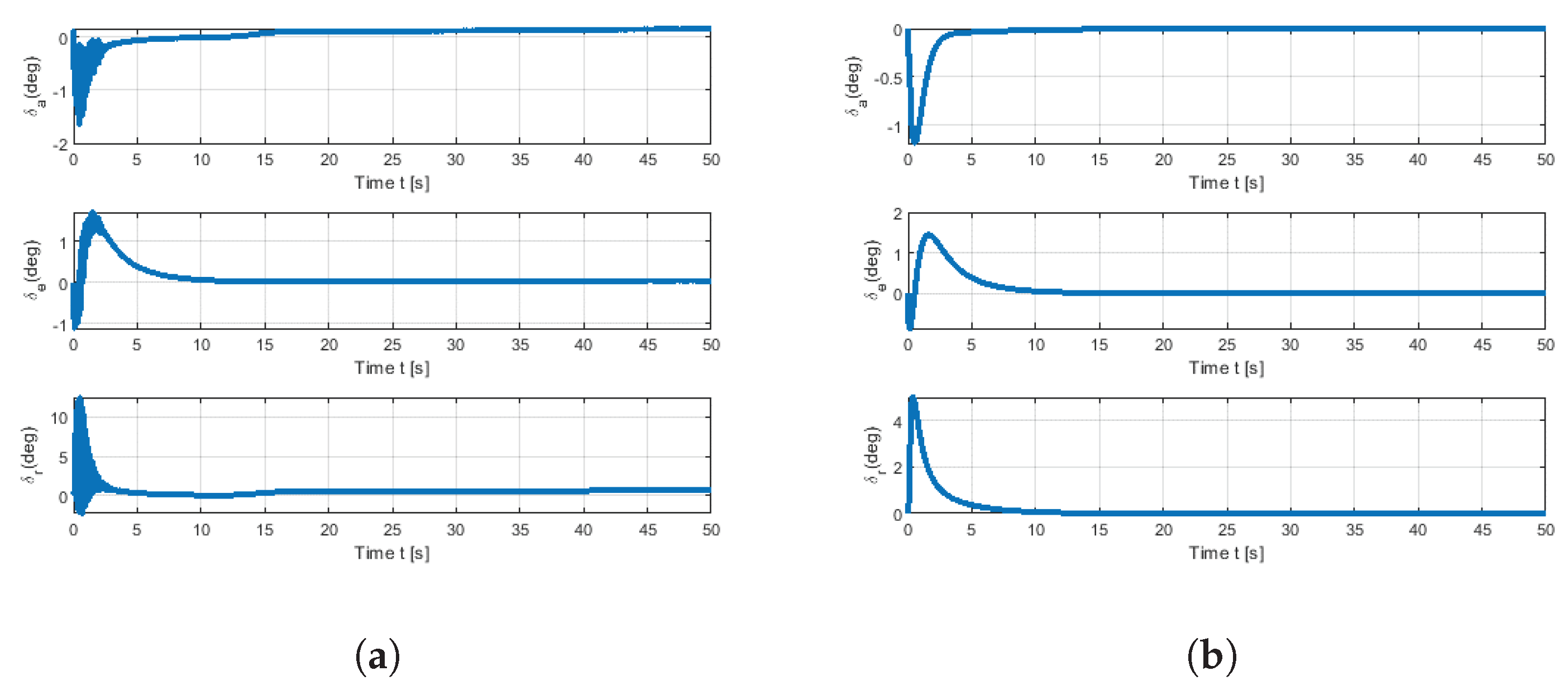
4.3. Adaptive System Simulation
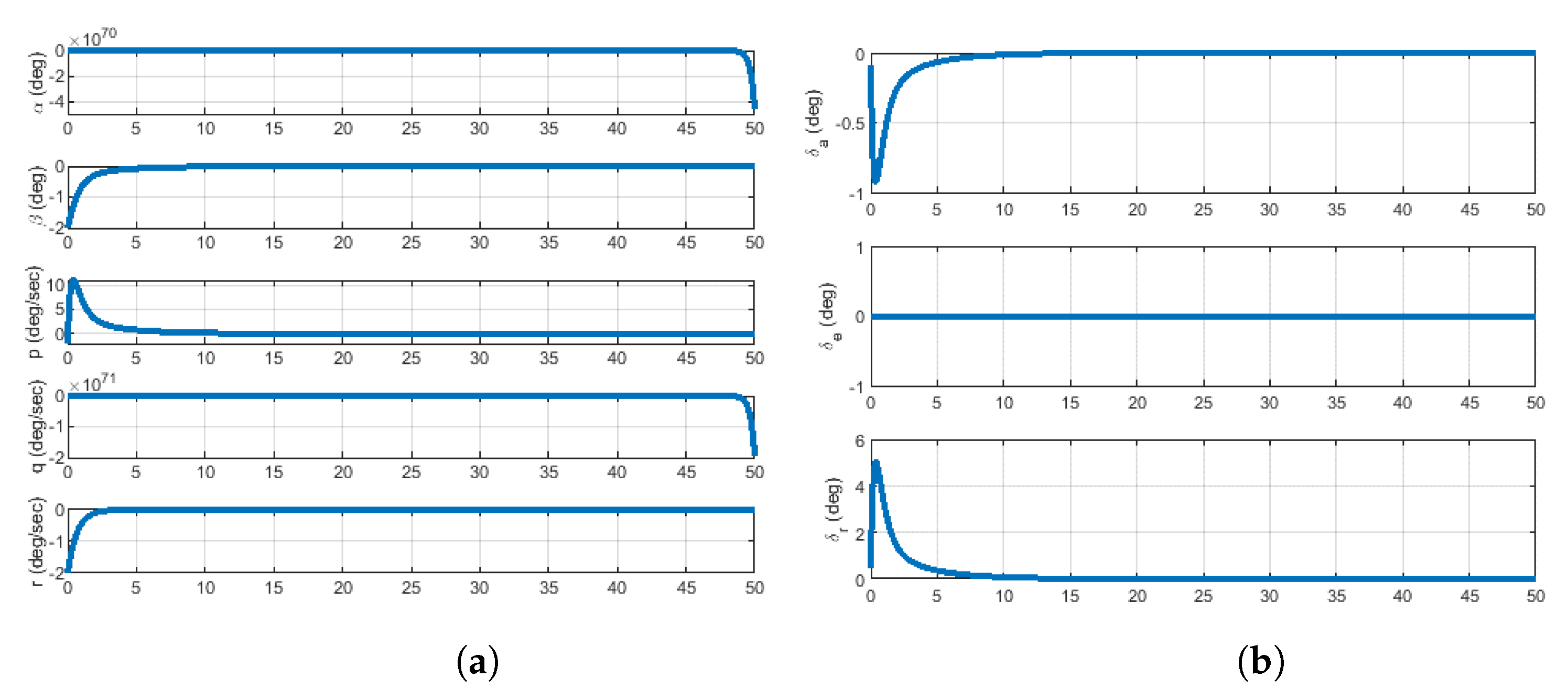
4.3.1. First Case Study
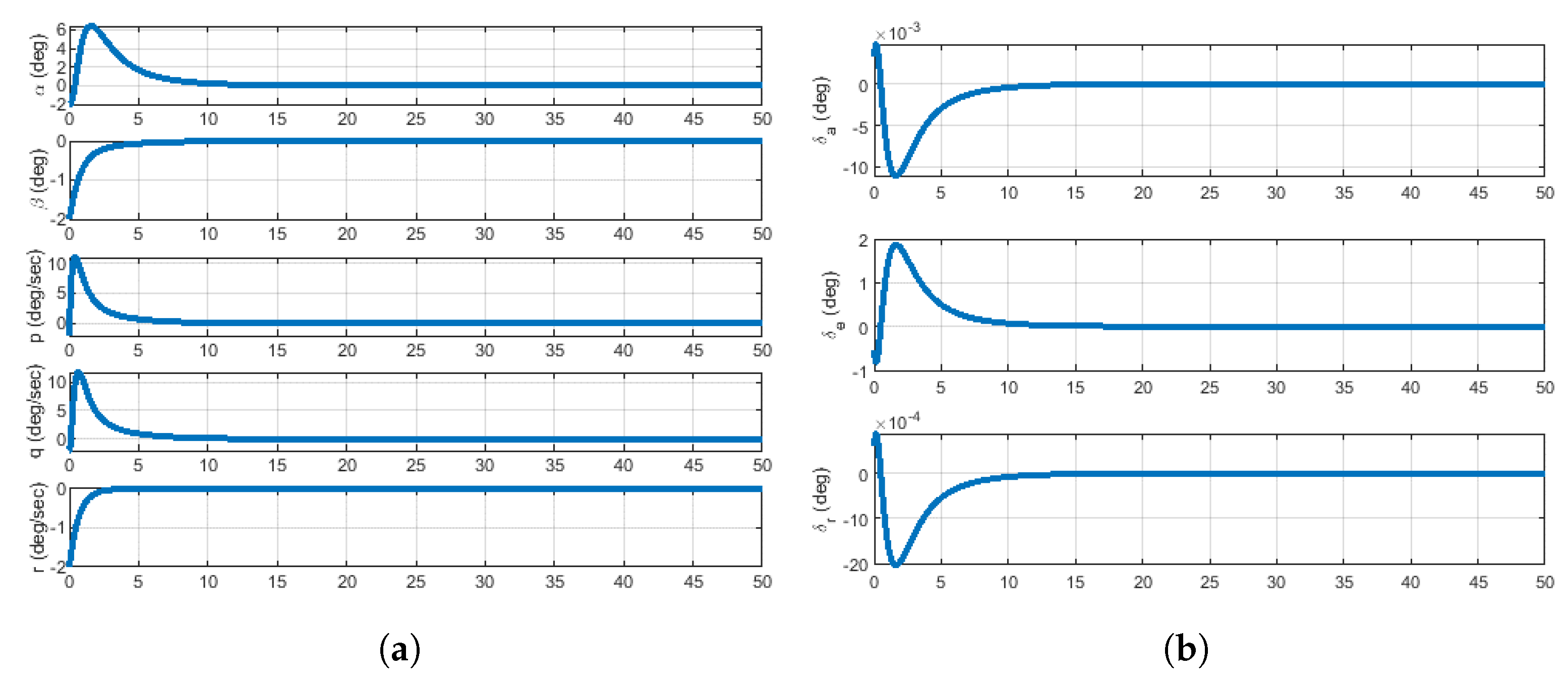
4.3.2. Second Case Study
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Narendra, K.S.; Annaswamy, A.M. Stable Adaptive Systems; Courier Corporation: Chelmsford, MA, USA, 2012. [Google Scholar]
- Åström, K.J.; Wittenmark, B. Adaptive Control; Courier Corporation: Chelmsford, MA, USA, 2013. [Google Scholar]
- Sastry, S.; Bodson, M. Adaptive Control: Stability, Convergence and Robustness; Courier Corporation: Chelmsford, MA, USA, 2011. [Google Scholar]
- Nguyen, N.T. Model-Reference Adaptive Control: A Primer; Springer International Publishing: Cham, Switzerland, 2018. [Google Scholar]
- Lavretsky, E.; Wise, K.A. Robust and Adaptive Control with Aerospace Applications; Springer International Publishing: Cham, Switzerland, 2024. [Google Scholar]
- Duarte, M.A.; Narendra, K.S. Combined direct and indirect approach to adaptive control. IEEE Trans. Autom. Control 1989, 34, 1071–1075. [Google Scholar] [CrossRef]
- Nakanishi, J.; Farrell, J.A.; Schaal, S. Composite adaptive control with locally weighted statistical learning. Neural Netw. 2005, 18, 71–90. [Google Scholar] [CrossRef] [PubMed]
- Lavretsky, E. Combined/composite model reference adaptive control. IEEE Trans. Autom. Control 2009, 54, 2692–2697. [Google Scholar] [CrossRef]
- Patre, P.M.; MacKunis, W.; Johnson, M.; Dixon, W.E. Composite adaptive control for Euler–Lagrange systems with additive disturbances. Automatica 2010, 46, 140–147. [Google Scholar] [CrossRef]
- Gibson, T.E.; Annaswamy, A.M.; Lavretsky, E. On adaptive control with closed-loop reference models: Transients, oscillations, and peaking. IEEE Access 2013, 1, 703–717. [Google Scholar] [CrossRef]
- Gibson, T.E.; Qu, Z.; Annaswamy, A.M.; Lavretsky, E. Adaptive output feedback based on closed-loop reference models. IEEE Trans. Autom. Control 2015, 60, 2728–2733. [Google Scholar] [CrossRef]
- Cao, C.; Hovakimyan, N. Design and analysis of a Novel L1 adaptive control architecture with guaranteed transient performance. IEEE Trans. Autom. Control 2008, 53, 586–591. [Google Scholar] [CrossRef]
- Boyd, S.; Sastry, S.S. Necessary and sufficient conditions for parameter convergence in adaptive control. Automatica 1986, 22, 629–639. [Google Scholar] [CrossRef]
- Loria, A. Explicit convergence rates for MRAC-type systems. Automatica 2004, 40, 1465–1468. [Google Scholar] [CrossRef]
- Krstić, M.; Kokotović, P.V.; Kanellakopoulos, I. Transient-performance improvement with a new class of adaptive controllers. Syst. Control Lett. 1993, 21, 451–461. [Google Scholar] [CrossRef]
- Datta, A.; Ioannou, P.A. Performance analysis and improvement in model reference adaptive control. IEEE Trans. Autom. Control 1994, 39, 2370–2387. [Google Scholar] [CrossRef]
- Sun, J. A modified model reference adaptive control scheme for improved transient performance. IEEE Trans. Autom. Control 1993, 38, 1255–1259. [Google Scholar] [CrossRef]
- Miller, D.E.; Davison, E.J. An adaptive controller which provides an arbitrarily good transient and steady-state response. IEEE Trans. Autom. Control 1991, 36, 68–81. [Google Scholar] [CrossRef]
- Huang, J.T. Sufficient conditions for parameter convergence in linearizable systems. IEEE Trans. Autom. Control 2003, 48, 878–880. [Google Scholar] [CrossRef]
- Landau, I. A survey of model reference adaptive techniques—Theory and applications. Automatica 1974, 10, 353–379. [Google Scholar] [CrossRef]
- Slotine, J.J.E.; Li, W. Composite adaptive control of robot manipulators. Automatica 1989, 25, 509–519. [Google Scholar] [CrossRef]
- Bechlioulis, C.P.; Rovithakis, G.A. Adaptive control with guaranteed transient and steady state tracking error bounds for strict feedback systems. Automatica 2009, 45, 532–538. [Google Scholar] [CrossRef]
- Na, J.; Chen, Q.; Ren, X.; Guo, Y. Adaptive prescribed performance motion control of servo mechanisms with friction compensation. IEEE Trans. Ind. Electron. 2013, 61, 486–494. [Google Scholar] [CrossRef]
- Annaswamy, A.; Lavretsky, E.; Dydek, Z.; Gibson, T.; Matsutani, M. Recent results in robust adaptive flight control systems. Int. J. Adapt. Control Signal Process. 2013, 27, 4–21. [Google Scholar] [CrossRef]
- Morse, A.S. High-order parameter tuners for the adaptive control of linear and nonlinear systems. In Systems, Models and Feedback: Theory and Applications; Springer: Berlin/Heidelberg, Germany, 1992; pp. 339–364. [Google Scholar]
- Bajodah, A.H. Generalised dynamic inversion spacecraft control design methodologies. IET Control Theory Appl. 2009, 3, 239–251. [Google Scholar] [CrossRef]
- Bajodah, A.H. Asymptotic generalised dynamic inversion attitude control. IET Control Theory Appl. 2010, 4, 827–840. [Google Scholar] [CrossRef]
- Bajodah, A.H. Asymptotic perturbed feedback linearisation of underactuated Euler’s dynamics. Int. J. Control 2009, 82, 1856–1869. [Google Scholar] [CrossRef]
- Bajodah, A.H. Servo-constraint generalized inverse dynamics for robot manipulator control design. In Proceedings of the 2009 IEEE International Conference on Control and Automation, Christchurch, New Zealand, 9–11 December 2009; IEEE: Piscataway, NJ, USA, 2009; pp. 1019–1026. [Google Scholar]
- Greville, T. The pseudoinverse of a rectangular or singular matrix and its application to the solution of systems of linear equations. SIAM Rev. 1959, 1, 38–43. [Google Scholar] [CrossRef]
- Ben-Israel, A.; Greville, T.N. Generalized Inverses: Theory and Applications; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2003; Volume 15. [Google Scholar]
- Bajodah, A.H.; Hodges, D.H.; Chen, Y.H. Inverse dynamics of servo-constraints based on the generalized inverse. Nonlinear Dyn. 2005, 39, 179–196. [Google Scholar] [CrossRef]
- Mibar, H.; Bajodah, A.H. Mutual direct controllability of MIMO systems and application to longitudinal aircraft control. In Proceedings of the 2020 IEEE International Conference on System Theory, Control and Computing (ICSTCC), Sinaia, Romania, 8–10 October 2020; IEEE: Piscataway, NJ, USA, 2020; pp. 1618–2372. [Google Scholar]
- Mibar, H.; Bajodah, A.H. Mutual direct state controllability analysis of multivariable underactuated LTI systems. In Proceedings of the 2020 IEEE Australian and New Zealand Control Conference (ANZCC), Gold Coast, QLD, Australia, 26–27 November 2020; IEEE: Piscataway, NJ, USA, 2020; pp. 18–23. [Google Scholar]
- Wang, L.; Zhang, N.; Yue, T.; Liu, H.; Zhu, J.; Jia, X. Three-axis coupled flight control law design for flying wing aircraft using eigenstructure assignment method. Chin. J. Aeronaut. 2020, 33, 2510–2526. [Google Scholar] [CrossRef]





| Mode | Eigenvalue |
|---|---|
| Short period | −9.4949 |
| 3.2511 | |
| Dutch roll | −3.6965 |
| 2.4777 | |
| Roll subsidence | −12.0279 |
| Mode | Eigenvalue |
|---|---|
| Short period | 3.2511 |
| −9.4949 | |
| Dutch roll | −0.3139 |
| −1.5 | |
| Roll subsidence | −4.6623 |
| Mode | Eigenvalue |
|---|---|
| Short period | −5.0931 + 0.53939i |
| −5.0931 − 0.53939i | |
| Dutch roll | −0.3485 |
| −1.5 | |
| Roll subsidence | −0.8783 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mahmoud, A.; Bajodah, A.H. Performance Enhancement of MRAC via Generalized Dynamic Inversion. Actuators 2025, 14, 18. https://doi.org/10.3390/act14010018
Mahmoud A, Bajodah AH. Performance Enhancement of MRAC via Generalized Dynamic Inversion. Actuators. 2025; 14(1):18. https://doi.org/10.3390/act14010018
Chicago/Turabian StyleMahmoud, Alharith, and Abdulrahman H. Bajodah. 2025. "Performance Enhancement of MRAC via Generalized Dynamic Inversion" Actuators 14, no. 1: 18. https://doi.org/10.3390/act14010018
APA StyleMahmoud, A., & Bajodah, A. H. (2025). Performance Enhancement of MRAC via Generalized Dynamic Inversion. Actuators, 14(1), 18. https://doi.org/10.3390/act14010018





