Development and Experimental Study of a Mixed-Mode Vibration Isolator Using Magnetorheological Elastomer
Abstract
1. Introduction
2. The Structural Design of the Mixed-Mode MRE Isolator
2.1. Structure Design
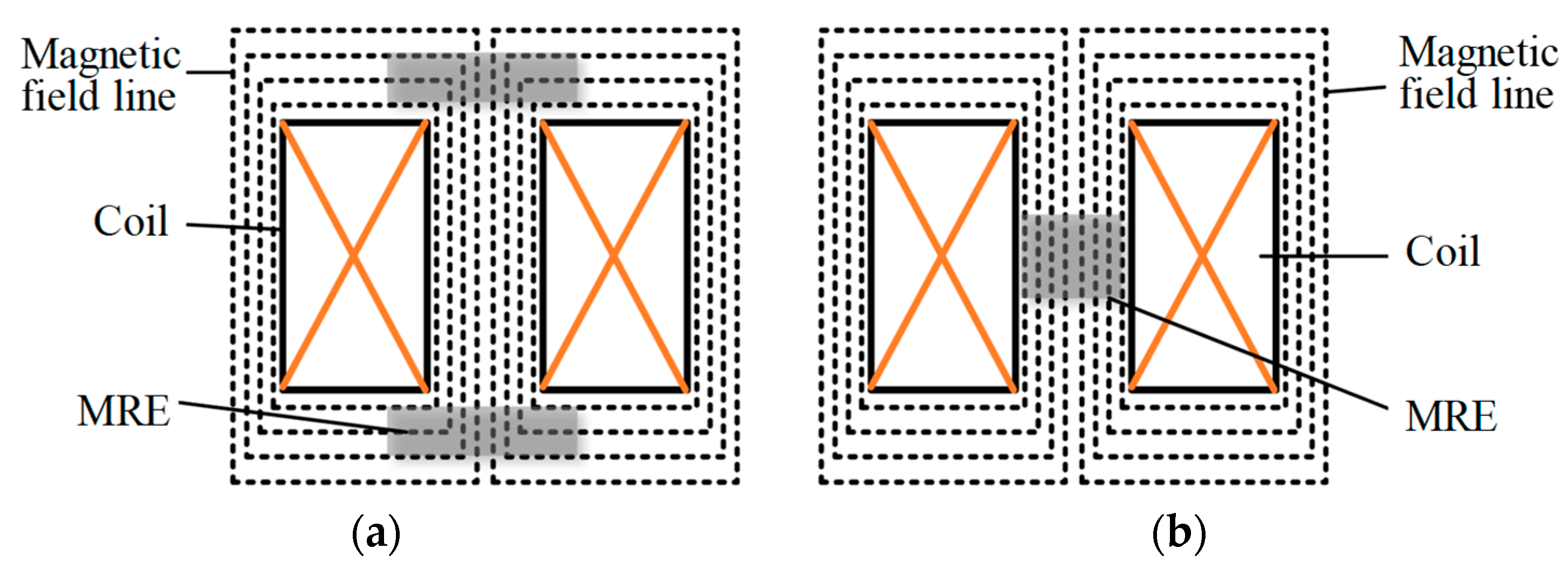
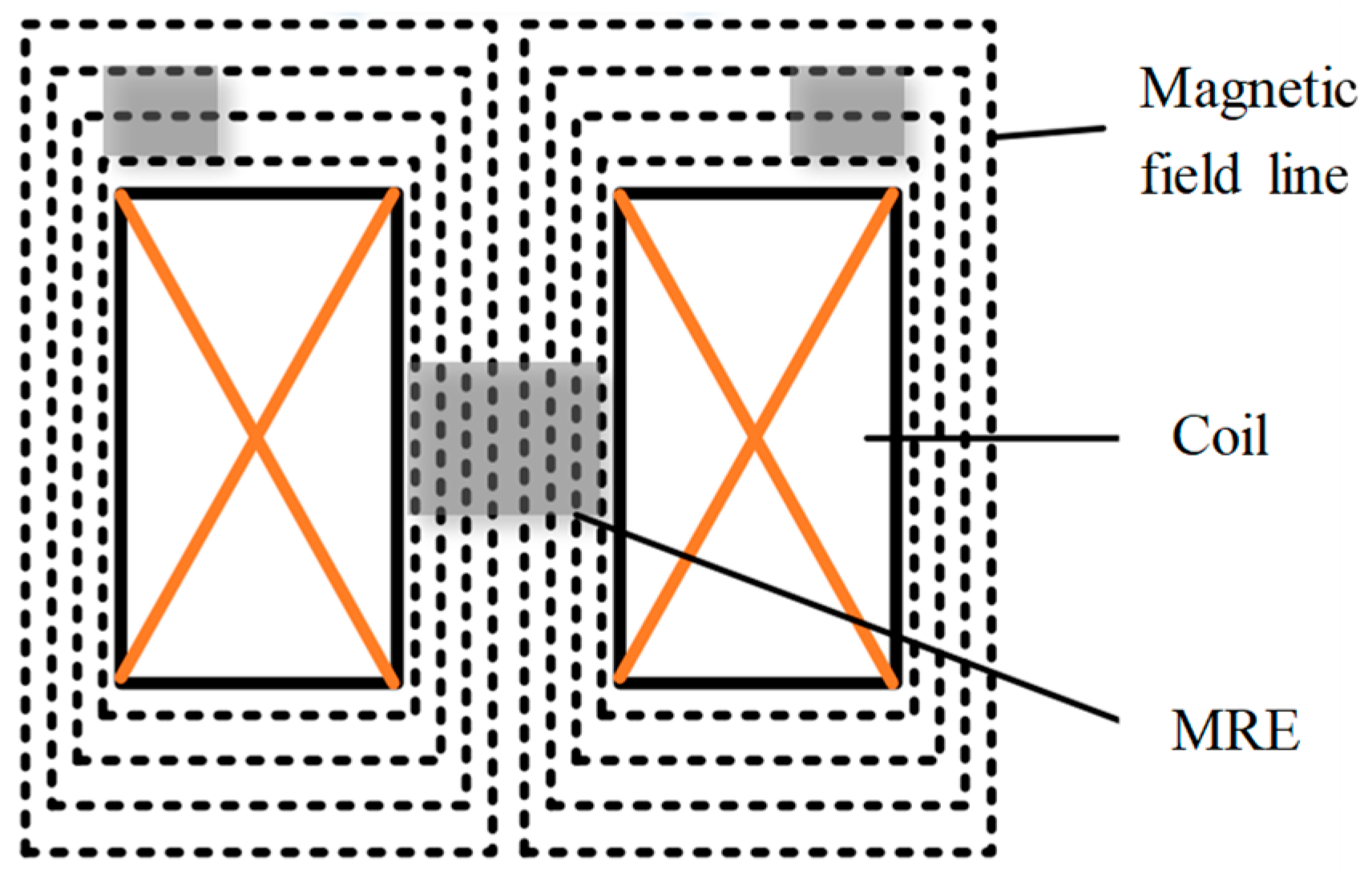
2.2. Magnetic Circuit Design
3. The Simulation Analysis of the Mixed-Mode MRE Isolator
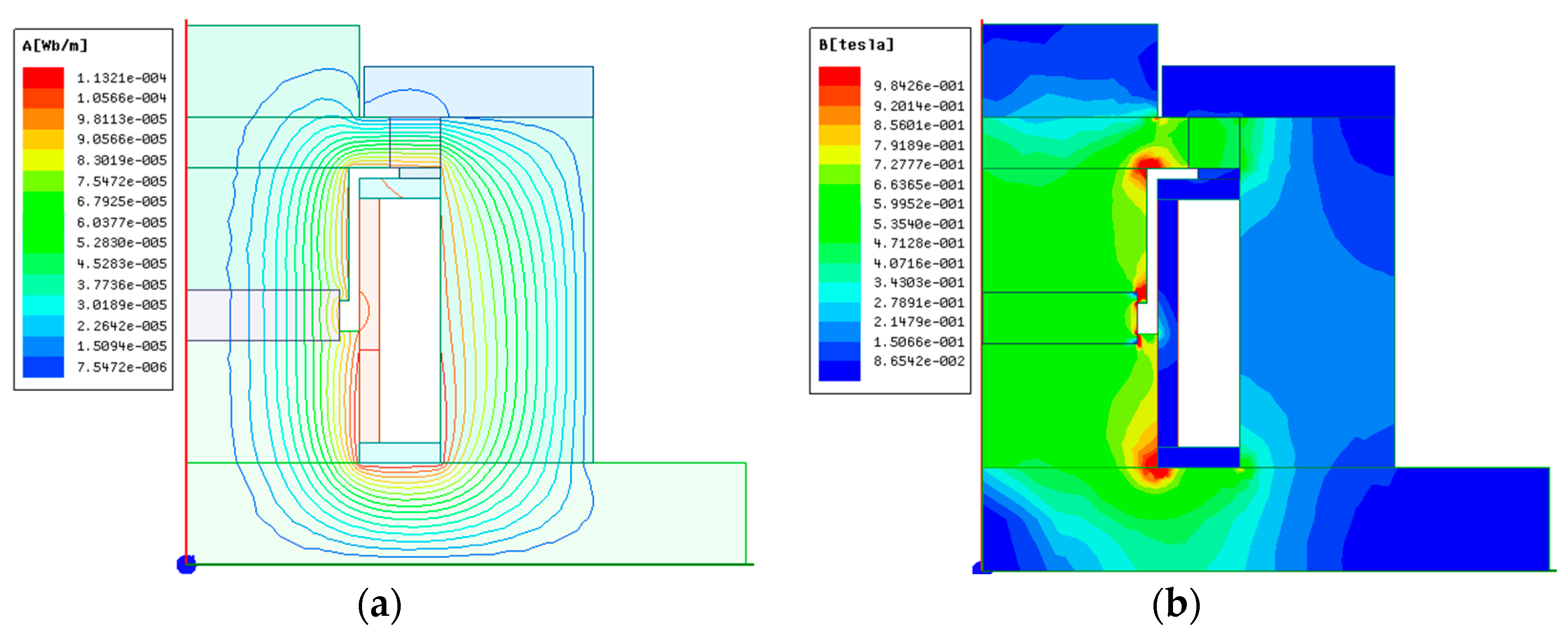
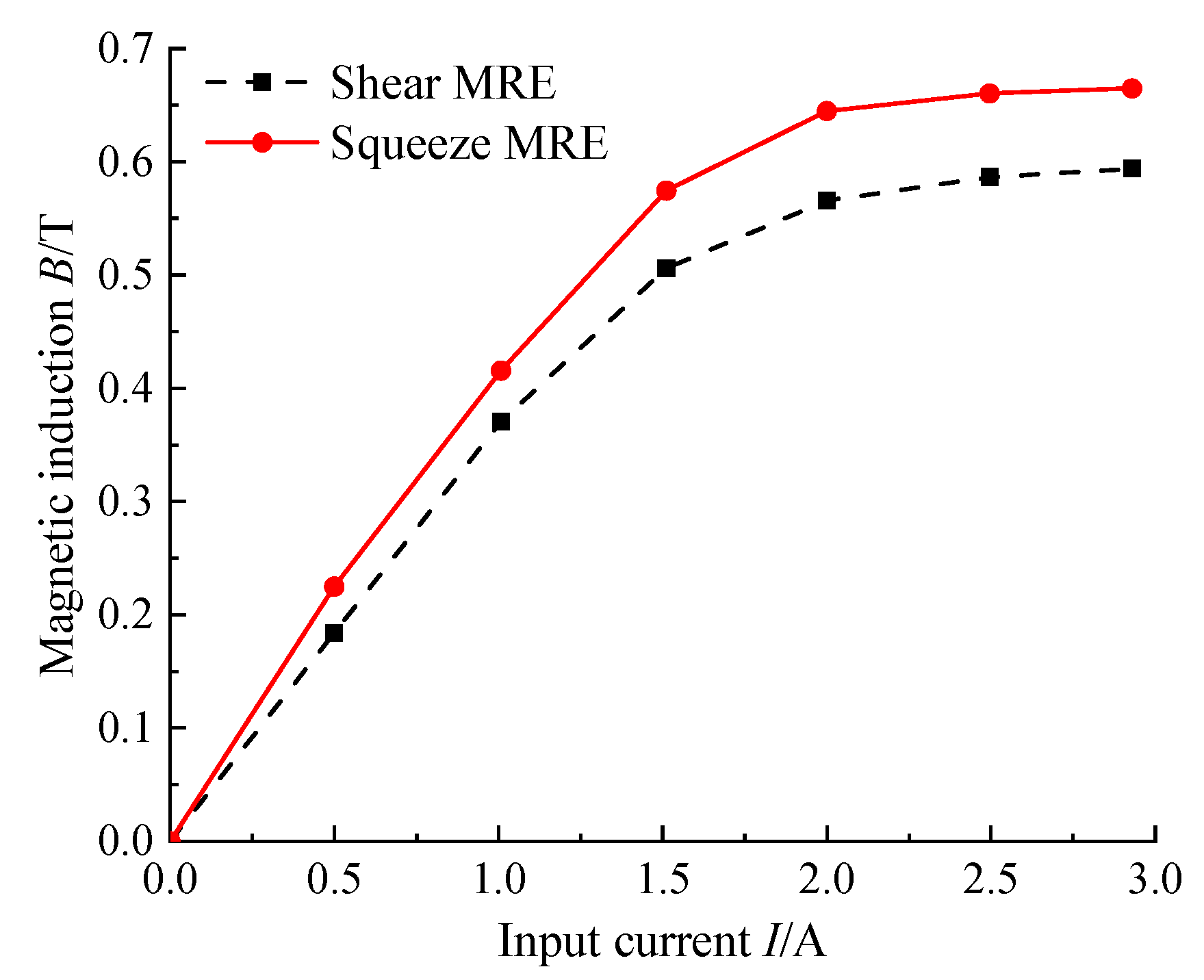
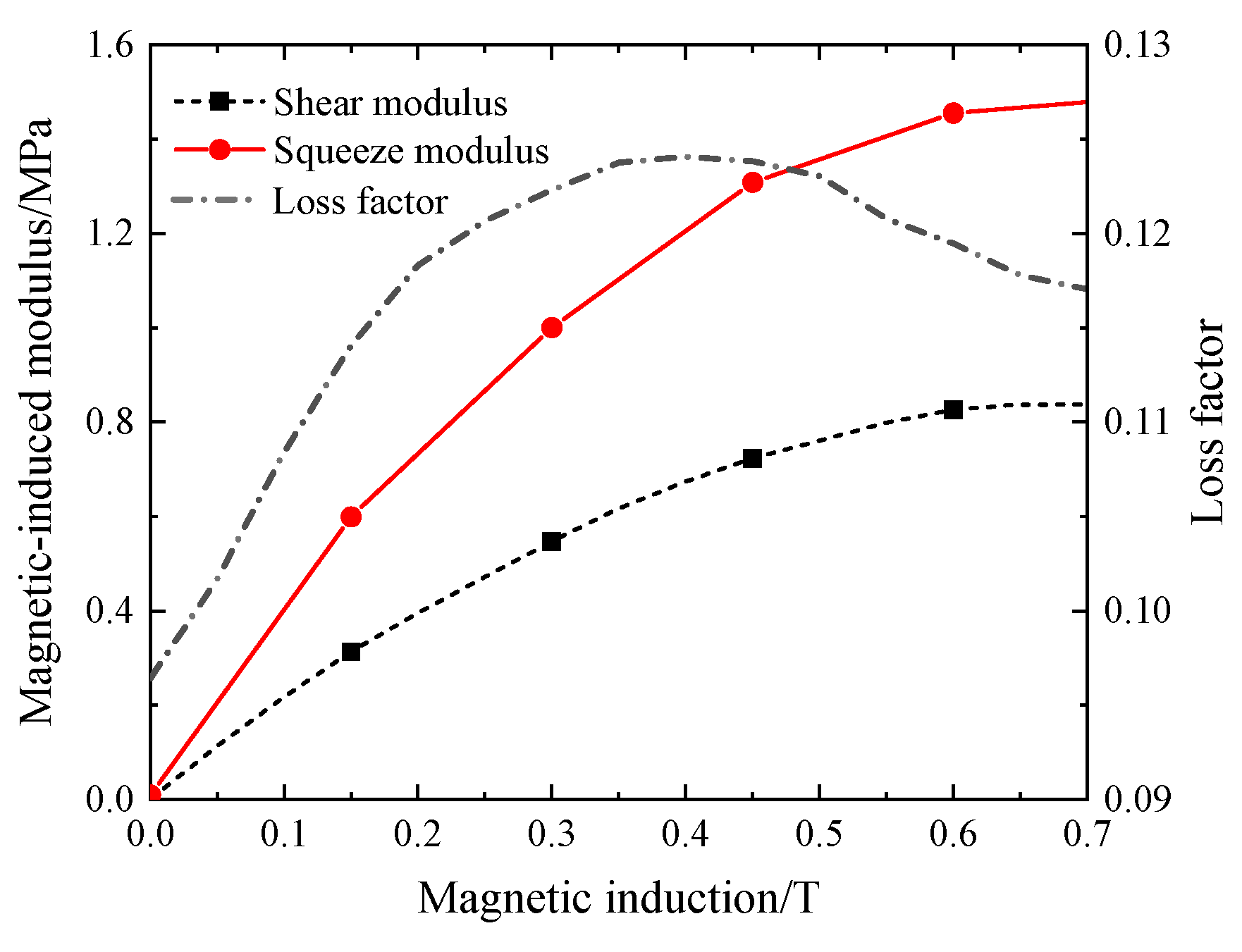
3.1. Magnetic Field Simulation Analysis
3.2. Dynamics Simulation Analysis
4. The Experimental Analysis of the Mixed-Mode MRE Isolator
4.1. Experimental System
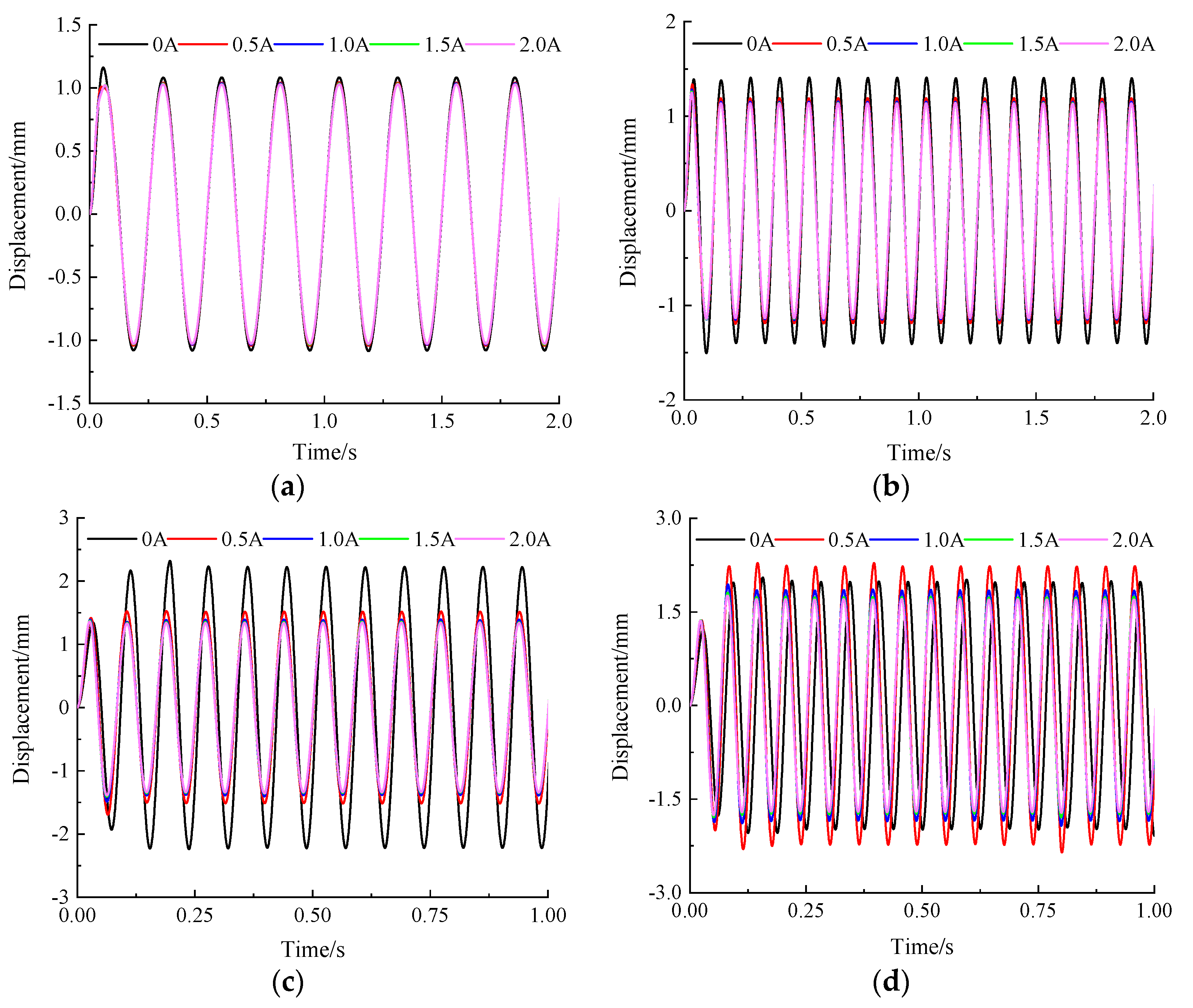
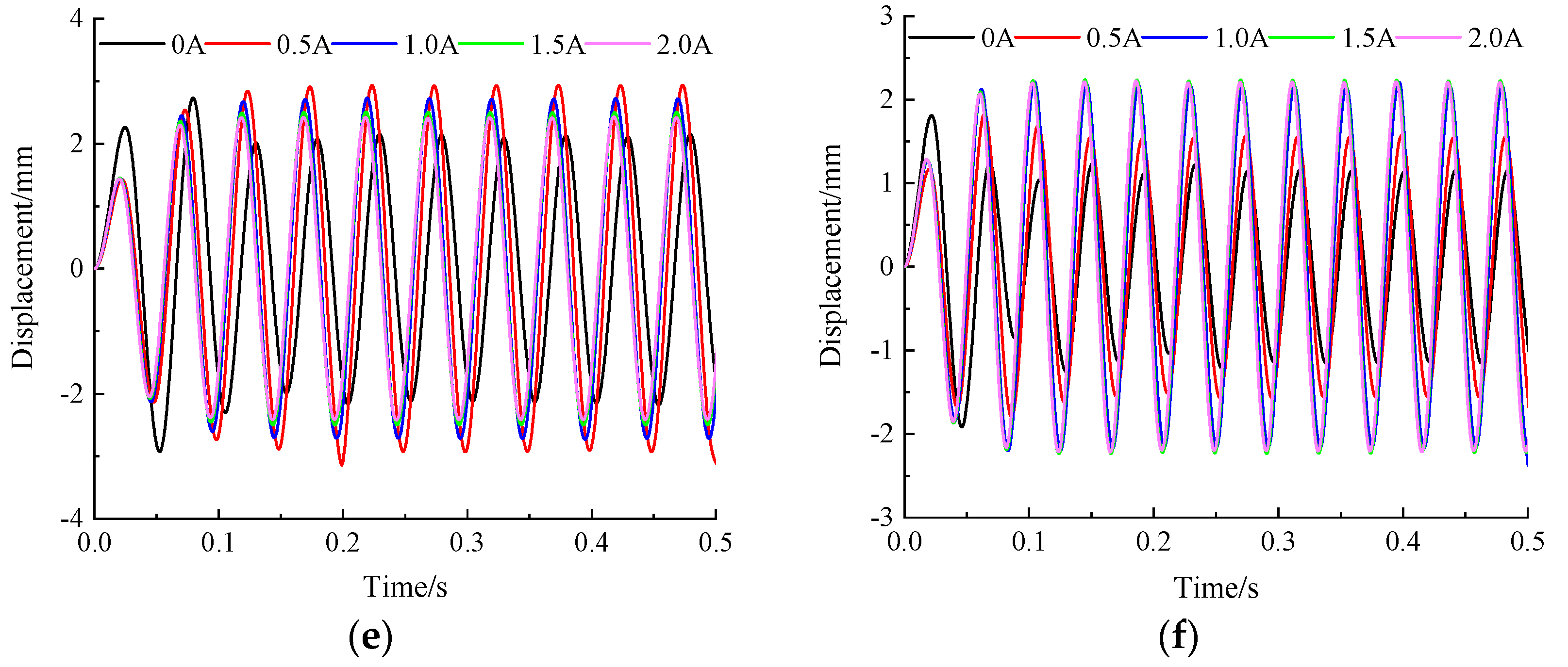
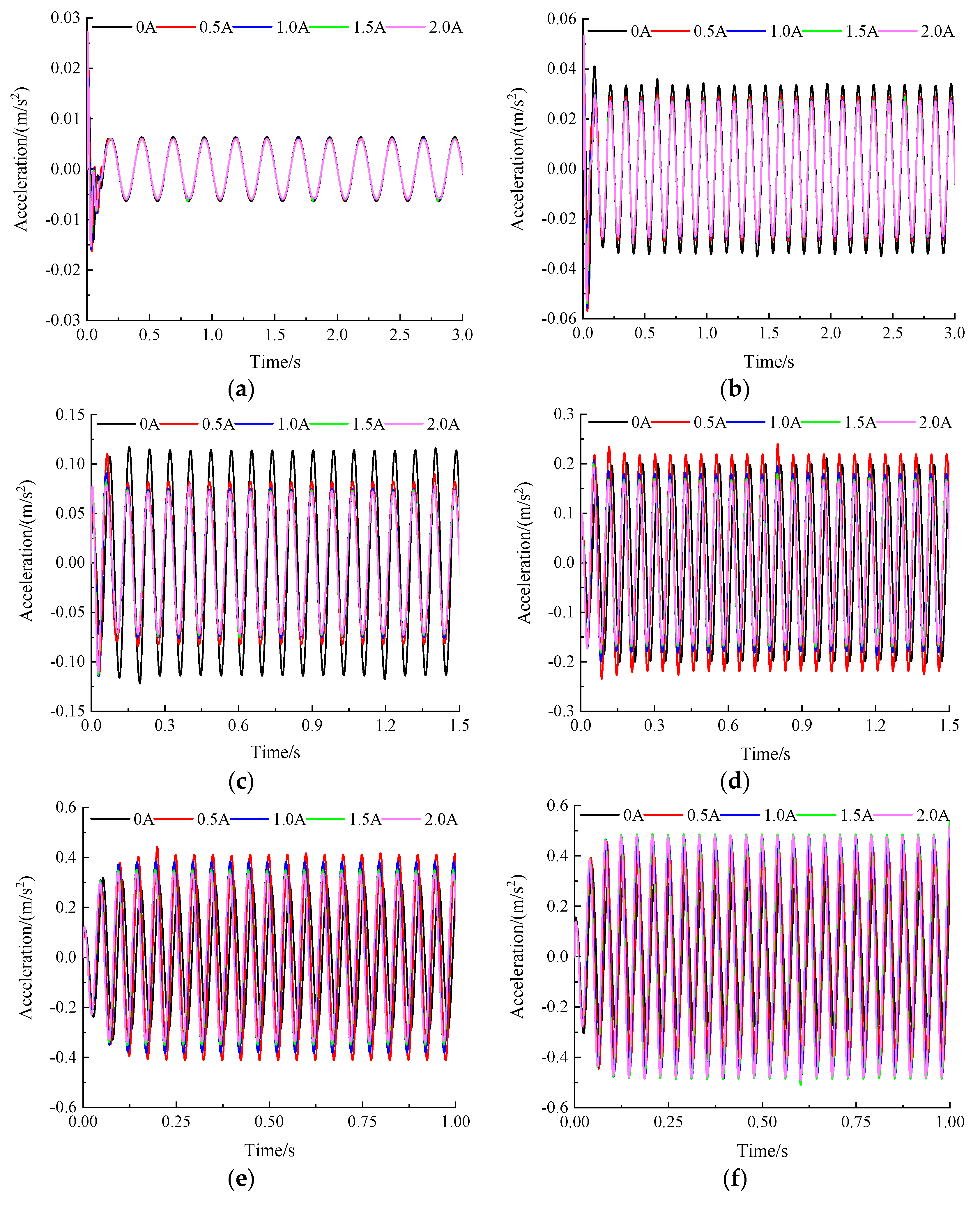
4.2. The Comparison of Experimental and Simulation Results
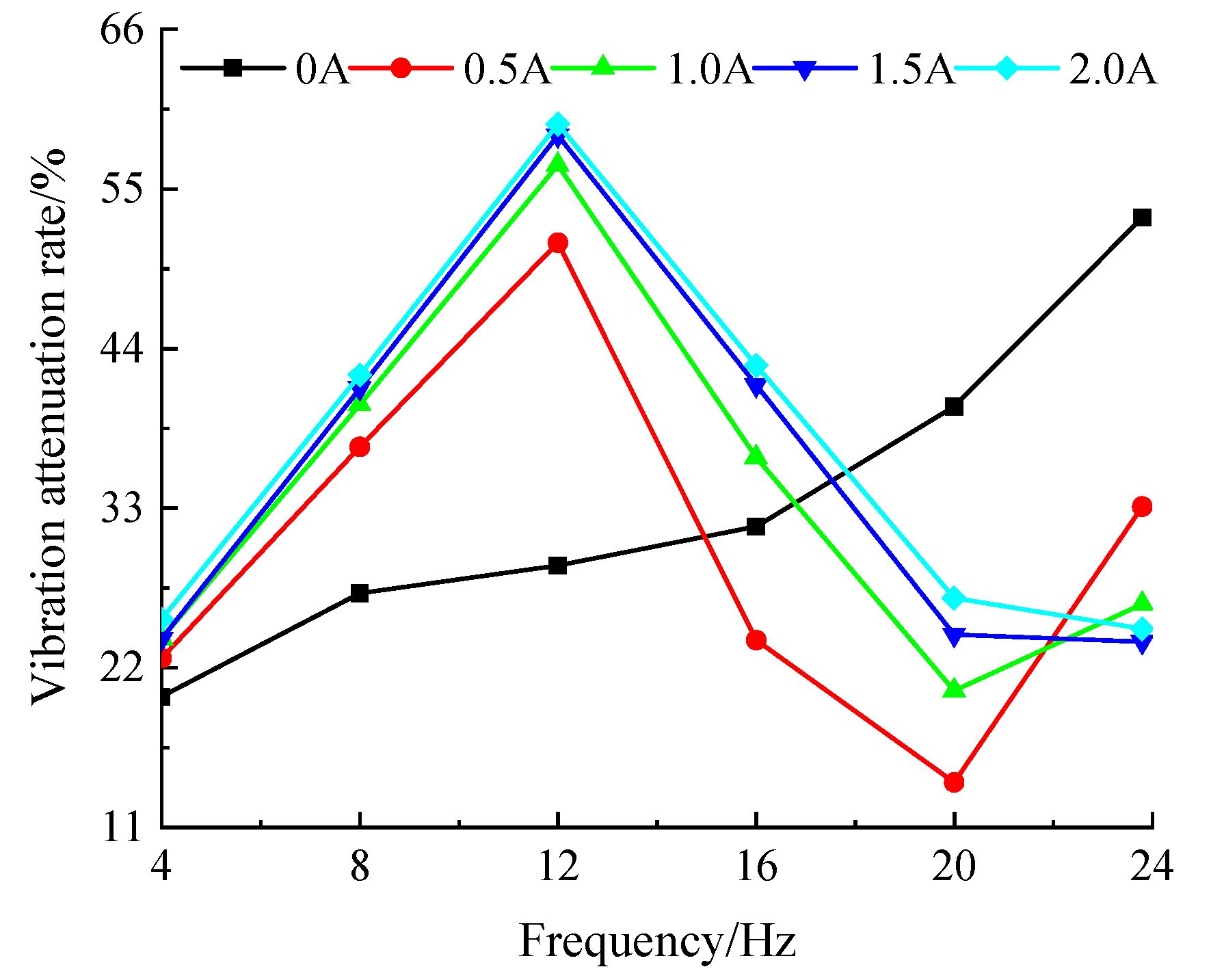
4.3. Experimental Result
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Bastola, A.K.; Paudel, M.; Li, L.; Li, W.H. Recent progress of magnetorheological elastomers: A review. Smart Mater. Struct. 2020, 29, 123002. [Google Scholar] [CrossRef]
- Zhang, Z.; Zhou, Y.S.; Shen, H.C.; Sun, M.; Chai, H.; Wu, H.P.; Jiang, S.F. Experimental study of orthogonal bistable laminated composite shell driven by magnetorheological elastomer. Compos. Struct. 2021, 271, 114119. [Google Scholar] [CrossRef]
- Liu, Q.J.; Chen, W.; Hu, H.S.; Hu, G.L.; Zhu, Q.Y. Effect of magnetorheological damper parameters on dynamic responses of a full-vehicle suspension system. Int. J. Appl. Electromagn. Mech. 2020, 63, 483–503. [Google Scholar] [CrossRef]
- Jin, S.D.; Sun, S.S.; Yang, J.; Deng, L.; Du, H.P.; Li, W.H. A hybrid MRE isolation system integrated with ball-screw inerter for vibration control. Smart Mater. Struct. 2021, 31, 025009. [Google Scholar] [CrossRef]
- Erenchun, A.; Kari, L.; Blanco, B.; Wang, B.C.; Irazu, L.; Gil-Negrete, N. Modeling and design of magnetorheological elastomer isolator system for an active control solution to reduce the vibration transmission in elevator context. J. Intell. Mater. Syst. Struct. 2024, 35, 29–48. [Google Scholar] [CrossRef]
- Yu, J.Q.; Dong, X.M.; Qi, S.; Wang, T.; Liang, Y.X. Development of a magnetorheological isolator with variable damping and variable stiffness for broadband vibration suppression. Smart Mater. Struct. 2021, 30, 025023. [Google Scholar] [CrossRef]
- Choi, Y.; Wereley, N.M. Vibration isolation performance of an adaptive magnetorheological elastomer-based dynamic vibration absorber. Actuators 2022, 11, 157. [Google Scholar] [CrossRef]
- Liao, G.J.; Gong, X.L.; Xuan, S.H.; Kang, C.J.; Zong, L.H. Development of a real-time tunable stiffness and damping vibration isolator based on magnetorheological elastomer. J. Intell. Mater. Syst. Struct. 2012, 23, 25–33. [Google Scholar] [CrossRef]
- Du, G.L.; Huang, X.G.; Li, Y.C.; Ouyang, Q.; Wang, J. Performance of a semi-active/passive integrated isolator based on a magnetorheological elastomer and spring. Smart Mater. Struct. 2017, 26, 095024. [Google Scholar] [CrossRef]
- Zhu, M.; Qi, S.; Xie, Y.P.; Fu, J.; Yu, M. Transient responses of magnetorheological elastomer and isolator under shear mode. Smart Mater. Struct. 2019, 28, 044002. [Google Scholar] [CrossRef]
- Jalali, A.; Dianati, H.; Norouzi, M.; Vatandoost, H.; Ghatee, M. A novel bi-directional shear mode magneto-rheological elastomer vibration isolator. J. Intell. Mater. Syst. Struct. 2020, 31, 2002–2019. [Google Scholar] [CrossRef]
- Syam, T.M.I.; Muthalif, A.G.A. Magnetorheological elastomer based torsional vibration isolator for application in a prototype drilling shaft. J. Low Freq. Noise Vib. Act. Control 2022, 41, 676–700. [Google Scholar] [CrossRef]
- Li, Y.C.; Li, J.C.; Li, W.H.; Du, H.P. A state-of-the-art review on magnetorheological elastomer devices. Smart Mater. Struct. 2014, 23, 123001. [Google Scholar] [CrossRef]
- Fu, J.; Li, P.D.; Liao, G.Y.; Lai, J.J.; Yu, M. Development and dynamic characterization of a mixed mode magnetorheological elastomer isolator. IEEE Trans. Magn. 2017, 53, 2800104. [Google Scholar] [CrossRef]
- Vatandoost, H.; Sedaghati, R.; Rakheja, S.; Hemmatian, M. Effect of pre-strain on compression mode properties of magnetorheological elastomers. Polym. Test. 2021, 93, 106888. [Google Scholar] [CrossRef]
- Du, H.P.; Li, W.H.; Zhang, N. Semi-active variable stiffness vibration control of vehicle seat suspension using an MR elastomer isolator. Smart Mater. Struct. 2011, 20, 105003. [Google Scholar] [CrossRef]
- Li, W.H.; Zhang, X.Z.; Du, H.P. Development and simulation evaluation of a magnetorheological elastomer isolator for seat vibration control. J. Intell. Mater. Syst. Struct. 2012, 23, 1041–1048. [Google Scholar] [CrossRef]
- Yang, F.F.; Tao, Y. Magnetorheological elastomer isolator in compression mode for IMU vibration isolation. J. Mech. Mater. Struct. 2020, 15, 565–583. [Google Scholar] [CrossRef]
- Fu, J.; Huang, Z.; Li, W.; Wang, W.; Zhong, C.; Qi, S.; Yu, M. A bidirectional-controllable magnetorheological elastomer-based quasi-zero-stiffness isolator. Smart Mater. Struct. 2024, 33, 085009. [Google Scholar] [CrossRef]
- Rustighi, E.; Ledezma-Ramirez, D.F.; Tapia-Gonzalez, P.E.; Ferguson, N.; Zakaria, A. Modelling and experimental characterisation of a compressional adaptive magnetorheological elastomer isolator. J. Vib. Control 2022, 28, 3093–3107. [Google Scholar] [CrossRef]
- Niu, C.G.; Dong, X.F.; Xiong, X.Y.; Ren, J.Q.; Niu, L.K.; Li, C.M.; Zhang, D.G.; Guo, J.B. Development of an electrorheological elastomer isolator working in shear-squeeze mixed mode. Smart Mater. Struct. 2023, 32, 065004. [Google Scholar] [CrossRef]
- Gao, W.; Lu, J.Y.; Han, W.H.; Chen, G.L.; Wang, X.Z. Improving the performance of magnetorheological elastomer-based adaptive isolator through integrated compression-torsion structure. Smart Mater. Struct. 2024, 33, 075022. [Google Scholar] [CrossRef]
- Saber, A.; Sedaghati, R. The modeling of magnetorheological elastomers: A state-of-the-art review. Adv. Eng. Mater. 2023, 25, 2300182. [Google Scholar] [CrossRef]
- Hu, Z.J.; Xia, L.L.; Sun, L.Z. Multiscale magneto-mechanical coupling of magnetorheological elastomer isolators. Finite Elem. Anal. Des. 2023, 224, 104003. [Google Scholar] [CrossRef]
- Hu, G.L.; Zhou, F.; Yang, X.; Wang, N.B.; Yu, L.F.; Li, G. Analysis of pressure drop and response characteristics of an enhanced radial magnetorheological valve based on magneto-fluidic coupling. J. Magn. Magn. Mater. 2024, 589, 171589. [Google Scholar] [CrossRef]
- Zhang, J.W.; Hu, G.L.; Qian, C.; Zhu, W.C. Analysis of braking performance and heat dissipation characteristics of multi-disc magnetorheological brake with an inner water-cooling mechanism. J. Magn. Magn. Mater. 2024, 604, 172313. [Google Scholar] [CrossRef]
- Tao, Y.; Rui, X.T.; Yang, F.F. Investigation of the impacts on magnetic permeability of MREs. J. Magn. Magn. Mater. 2019, 477, 269–274. [Google Scholar] [CrossRef]
- Leng, D.X.; Xiao, H.Y.; Sun, L.; Liu, G.J.; Wang, X.J.; Sun, L.Y. Study on a magnetorheological elastomer-base device for offshore platform vibration control. J. Intell. Mater. Syst. Struct. 2019, 30, 243–255. [Google Scholar] [CrossRef]
- Sui, G.D.; Hou, S.; Zhang, X.F.; Shan, X.B.; Hou, C.W.; Song, H.A.; Hou, W.J.; Li, J.M. A bio-inspired spider-like structure isolator for low-frequency vibration. Appl. Math. Mech. 2023, 44, 1263–1286. [Google Scholar] [CrossRef]




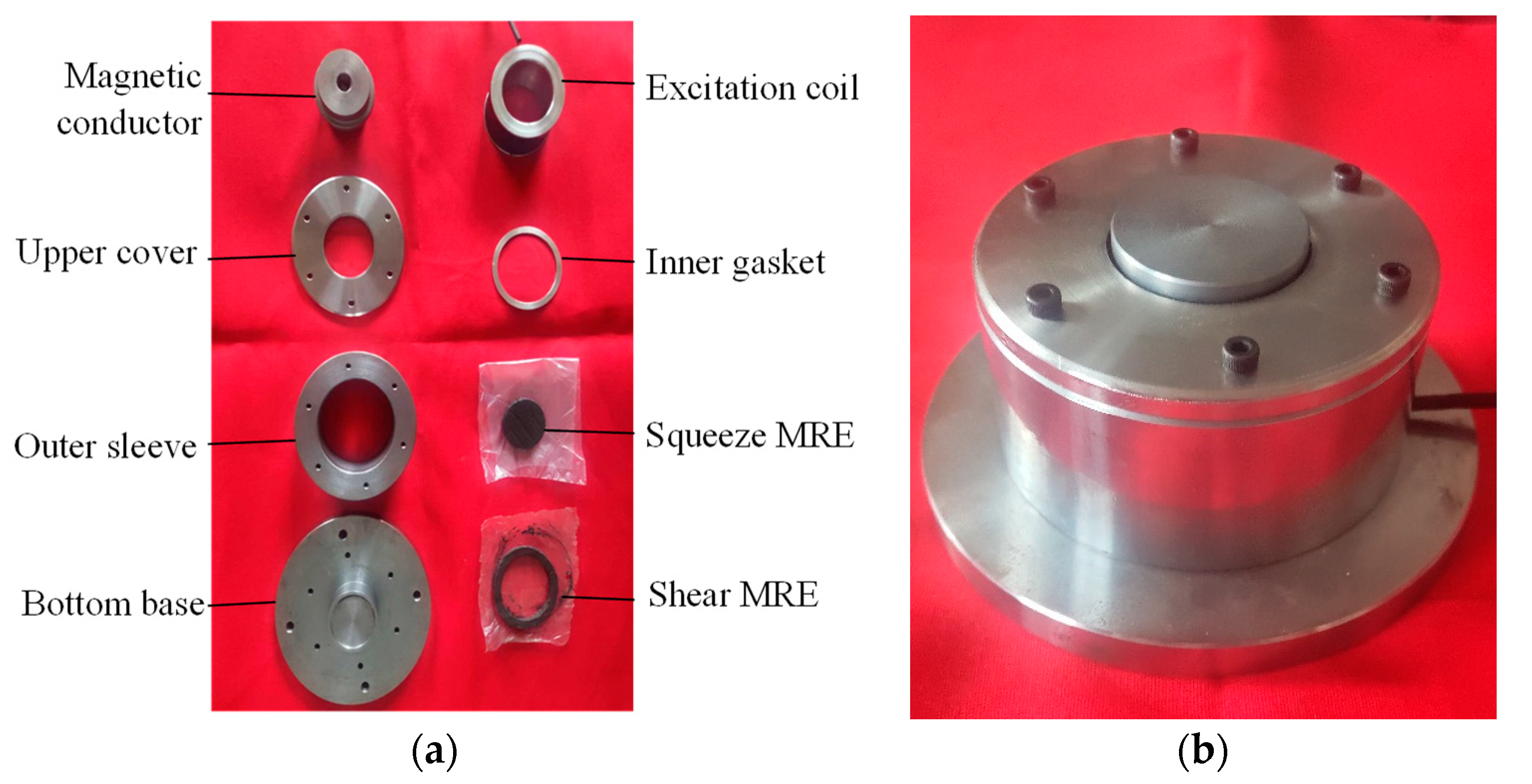
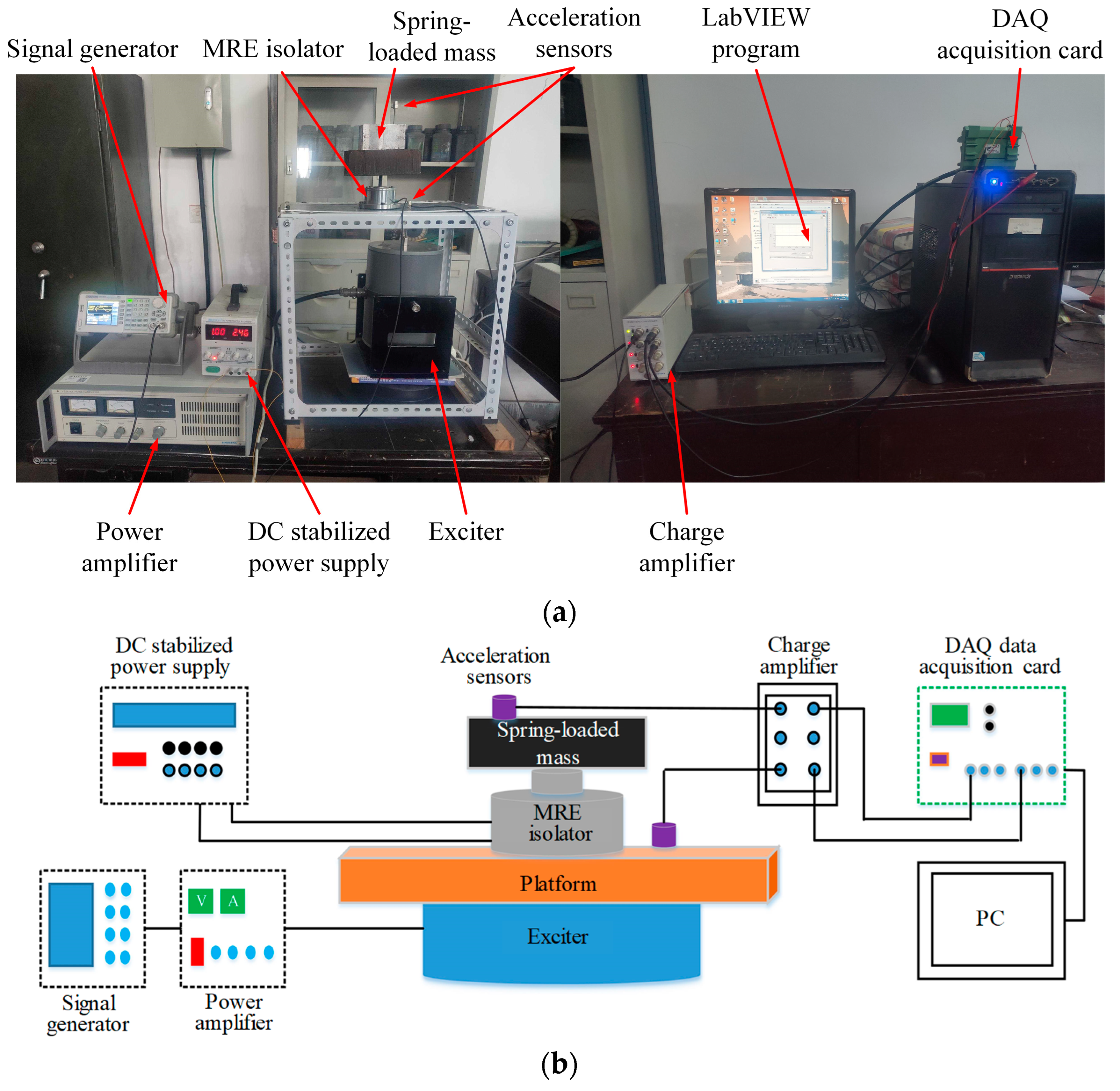
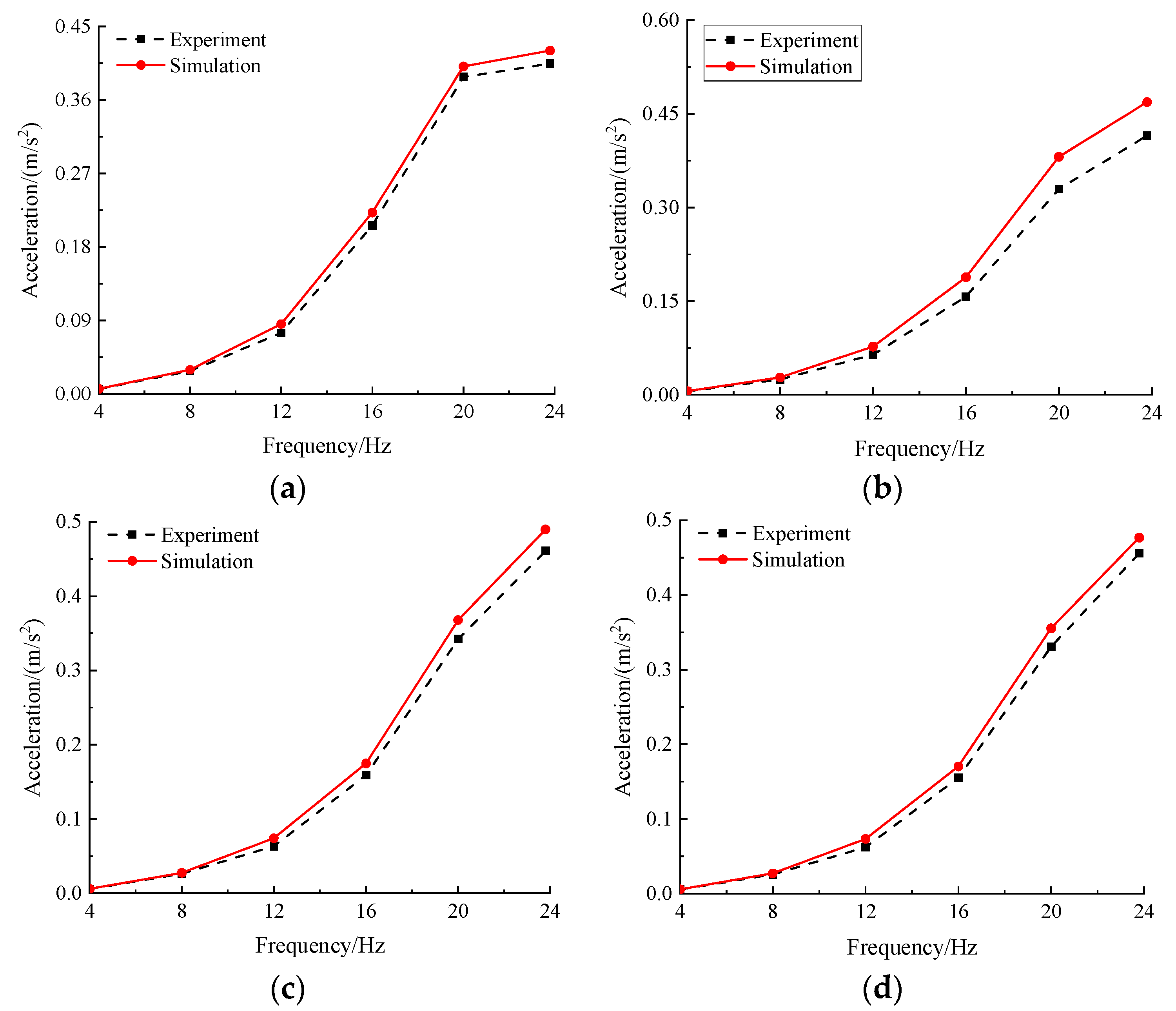

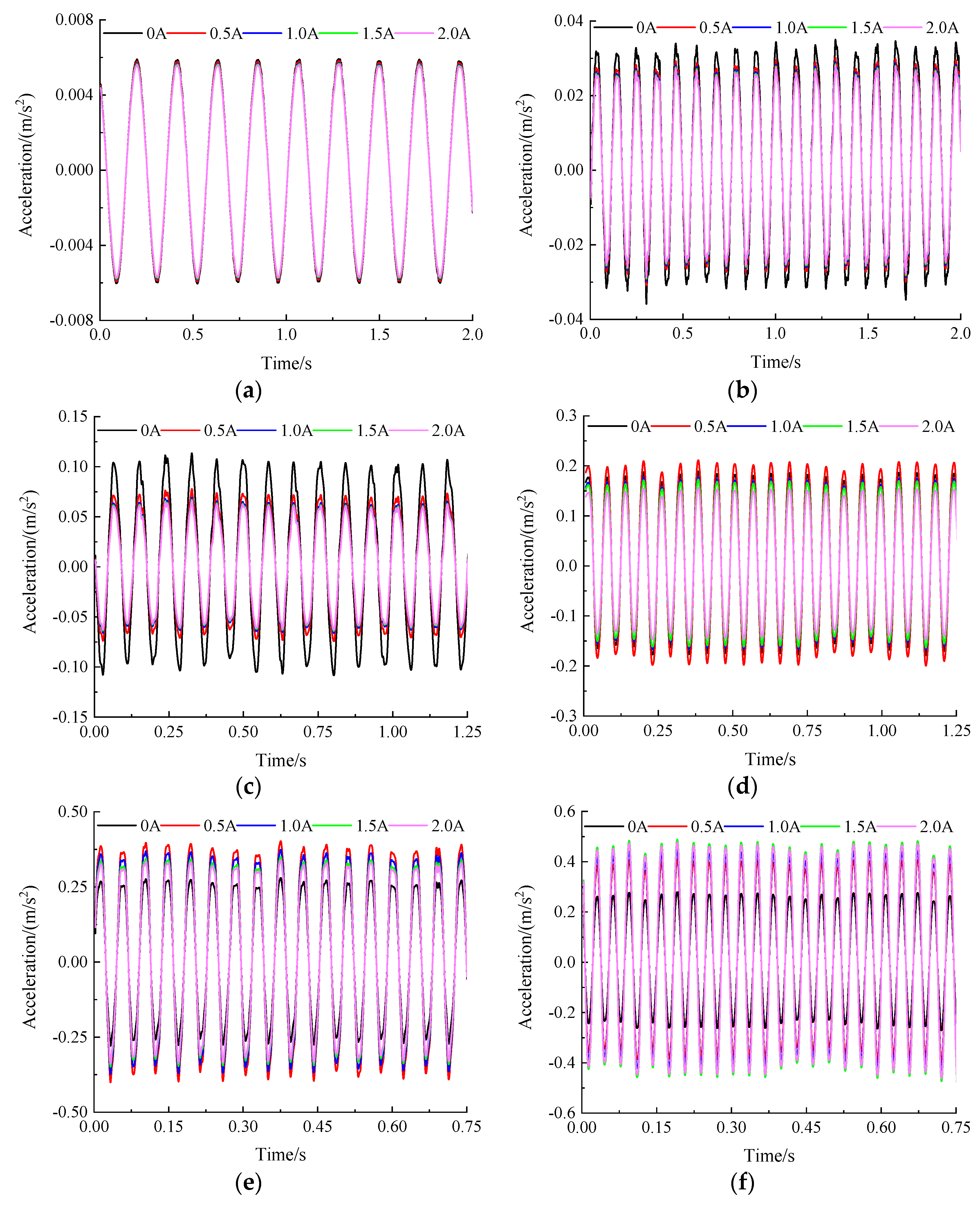
| Parameter | Value (mm) | Parameter | Value (mm) |
|---|---|---|---|
| radius of squeeze MRE r1 | 15 | thickness of the bottom base h1 | 10 |
| radius of lower end of the magnetic conductor r2 | 16 | thickness of the bottom base protrusion h2 | 12 |
| radius of upper end of the magnetic conductor r3 | 17 | thickness of squeeze MRE h3 | 5 |
| inner radius of shear MRE r4 | 20 | thickness of lower end of the magnetic conductor h4 | 12 |
| outer radius of shear MRE r5 | 25 | thickness of shear MRE h5 | 5 |
| radius of the outer sleeve r6 | 40 | thickness of upper cover h6 | 5 |
| radius of the bottom base r7 | 55 | thickness of upper end of the magnetic conductor h7 | 9 |
| Components | Material | Magnetic Property |
|---|---|---|
| MRE | carbonyl iron powder and silicone rubber | magnetic |
| upper cover | stainless steel | non-magnetic |
| magnetic conductor | low-carbon steel | magnetic |
| inner gasket | stainless steel | non-magnetic |
| outer sleeve | low-carbon steel | magnetic |
| excitation coil | copper | non-magnetic |
| winding tube | stainless steel | non-magnetic |
| bottom base | low-carbon steel | magnetic |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, Q.; Guo, Z.; Liu, W.; Li, G.; Jin, S.; Yu, L.; Hu, G. Development and Experimental Study of a Mixed-Mode Vibration Isolator Using Magnetorheological Elastomer. Actuators 2024, 13, 352. https://doi.org/10.3390/act13090352
Liu Q, Guo Z, Liu W, Li G, Jin S, Yu L, Hu G. Development and Experimental Study of a Mixed-Mode Vibration Isolator Using Magnetorheological Elastomer. Actuators. 2024; 13(9):352. https://doi.org/10.3390/act13090352
Chicago/Turabian StyleLiu, Qianjie, Zhirong Guo, Wei Liu, Gang Li, Shengzhi Jin, Lei Yu, and Guoliang Hu. 2024. "Development and Experimental Study of a Mixed-Mode Vibration Isolator Using Magnetorheological Elastomer" Actuators 13, no. 9: 352. https://doi.org/10.3390/act13090352
APA StyleLiu, Q., Guo, Z., Liu, W., Li, G., Jin, S., Yu, L., & Hu, G. (2024). Development and Experimental Study of a Mixed-Mode Vibration Isolator Using Magnetorheological Elastomer. Actuators, 13(9), 352. https://doi.org/10.3390/act13090352







