Analysis Method Development of Hybrid Linear Motor Considering Cogging Force Effect †
Abstract
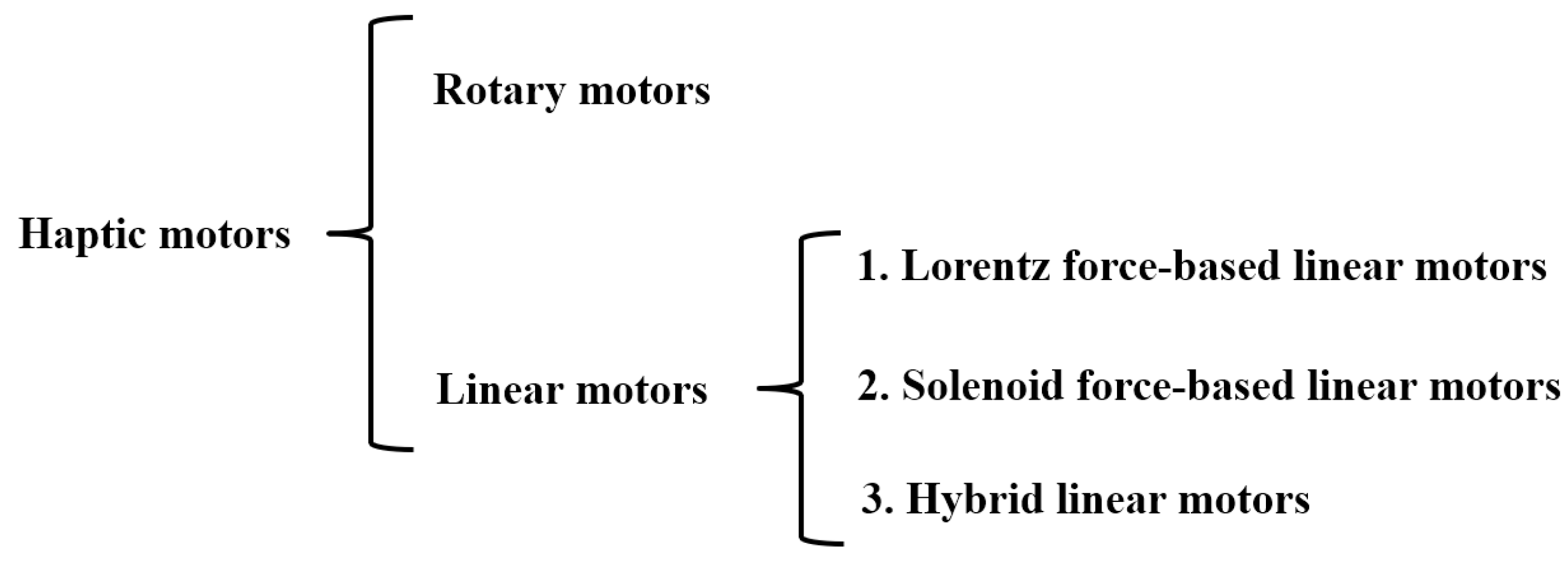
1. Introduction
2. Material Properties
3. Analysis Method
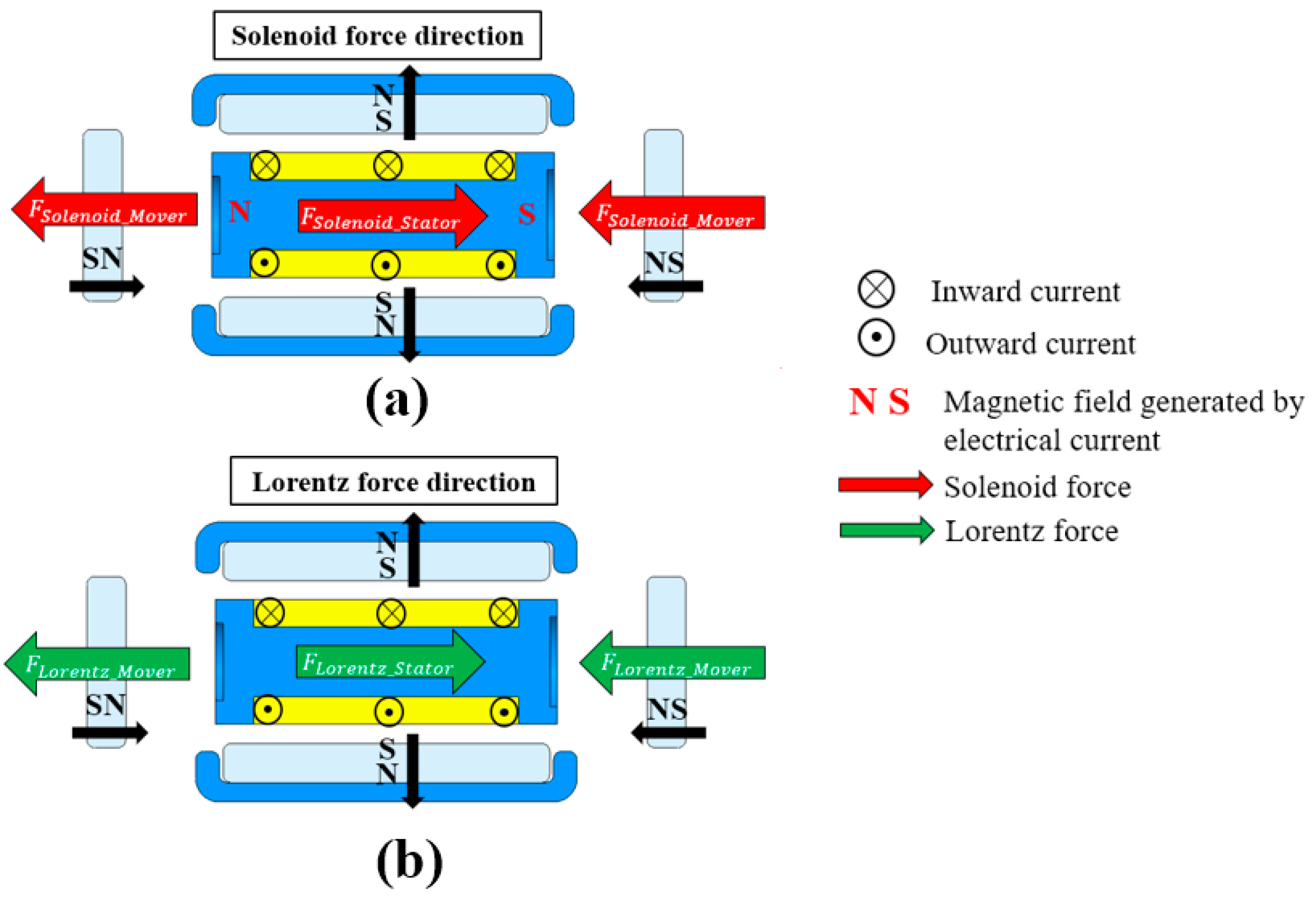
3.1. Force Profile Analysis
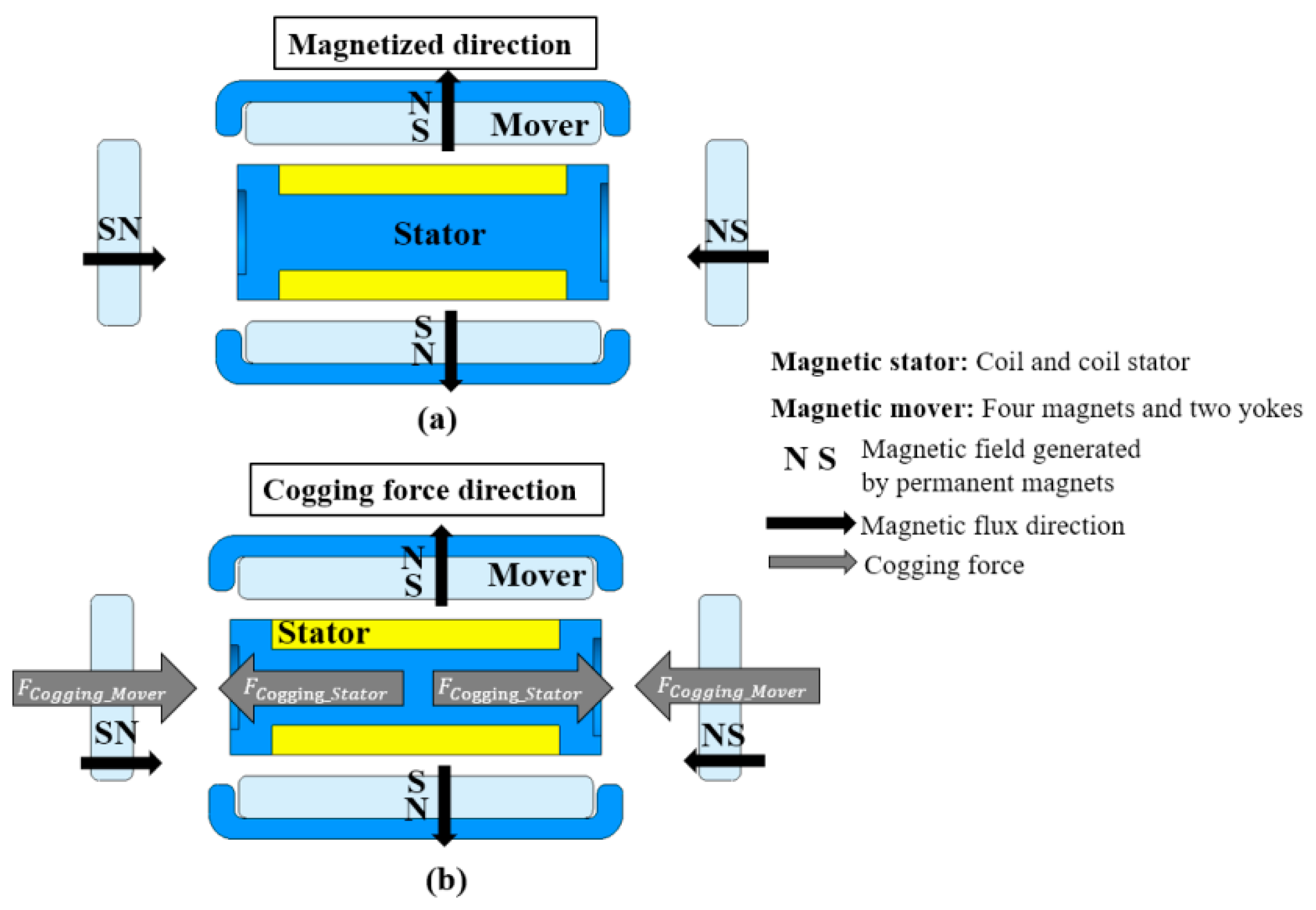
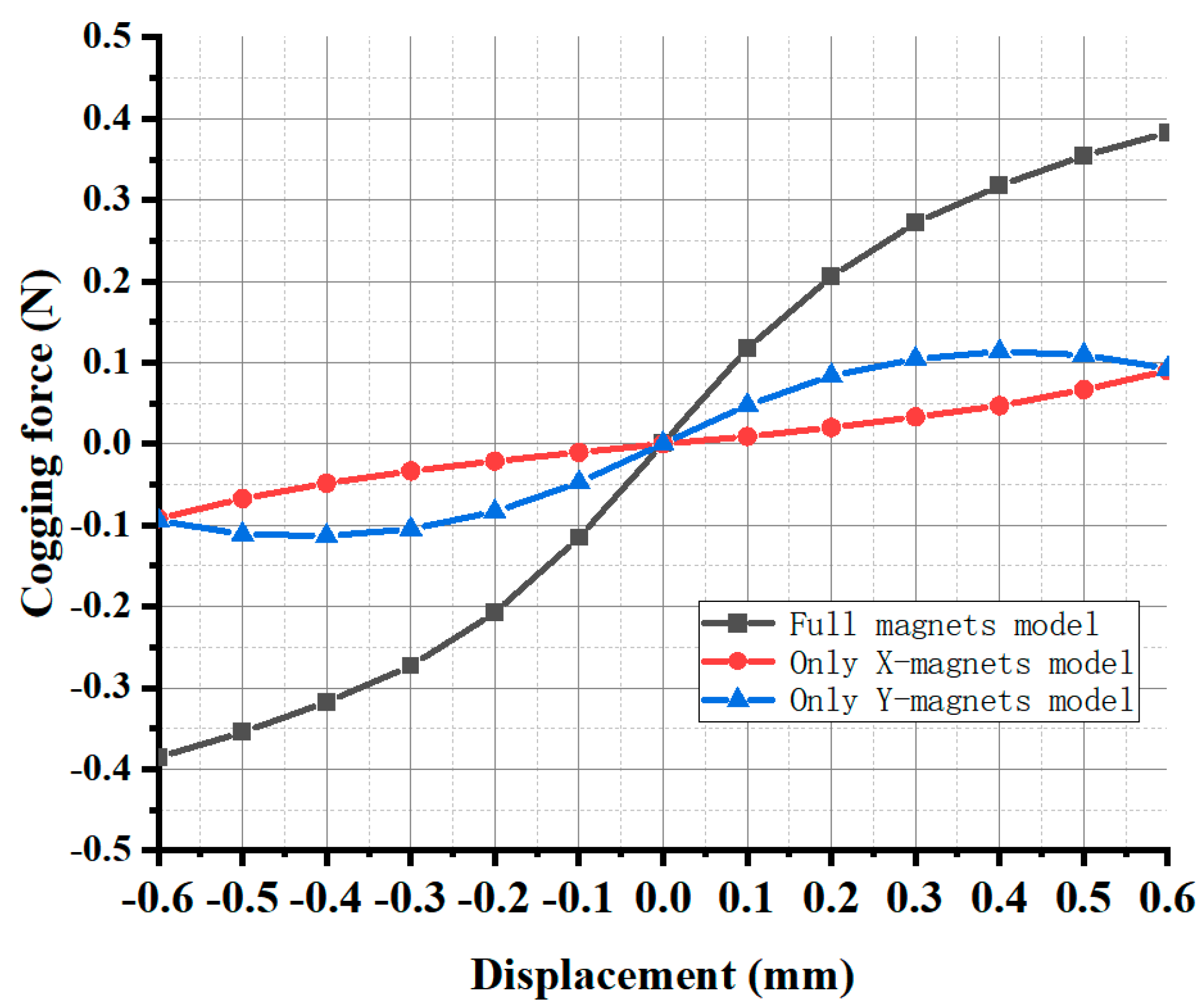
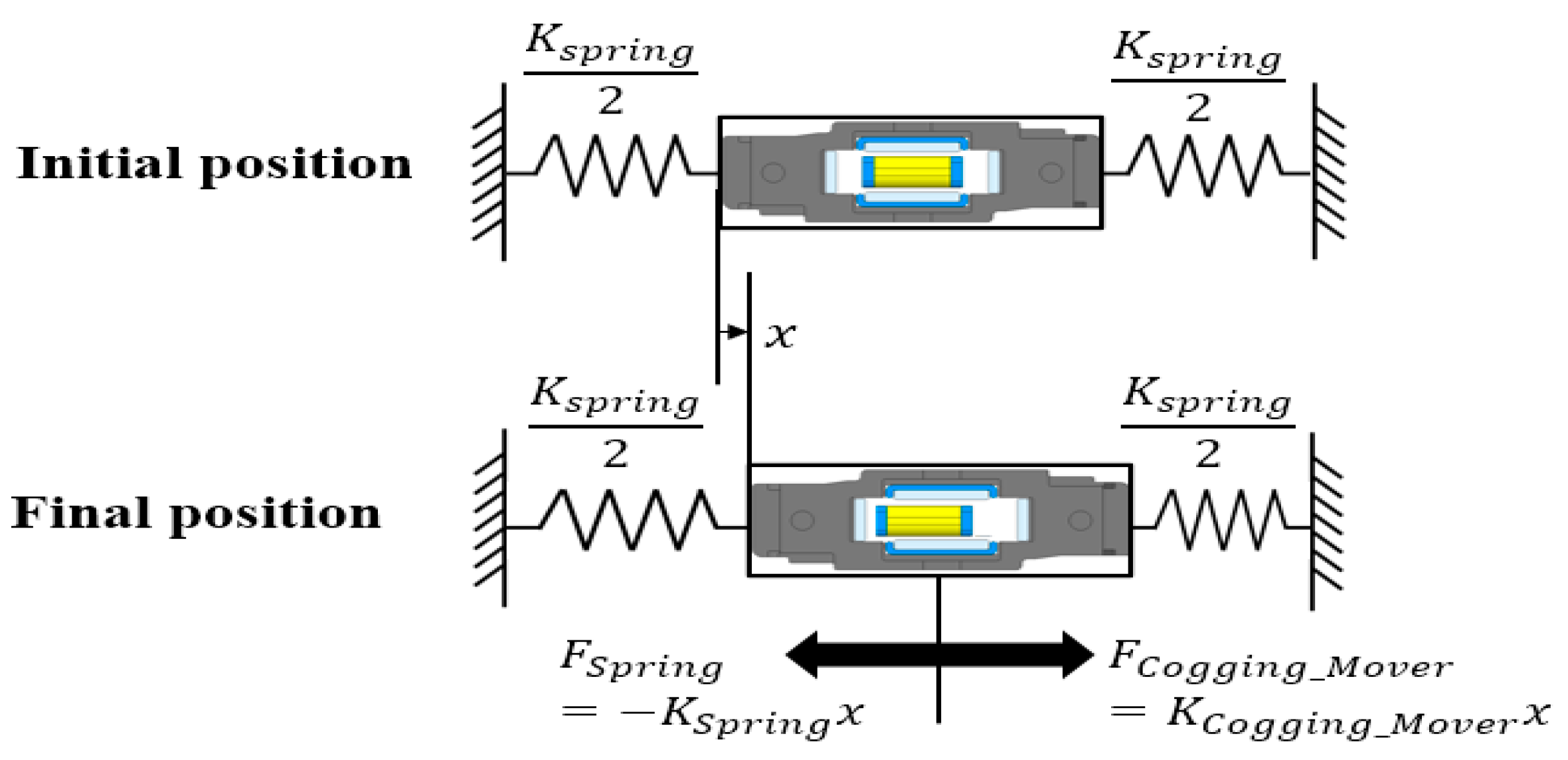
3.2. Cogging Stiffness Effect
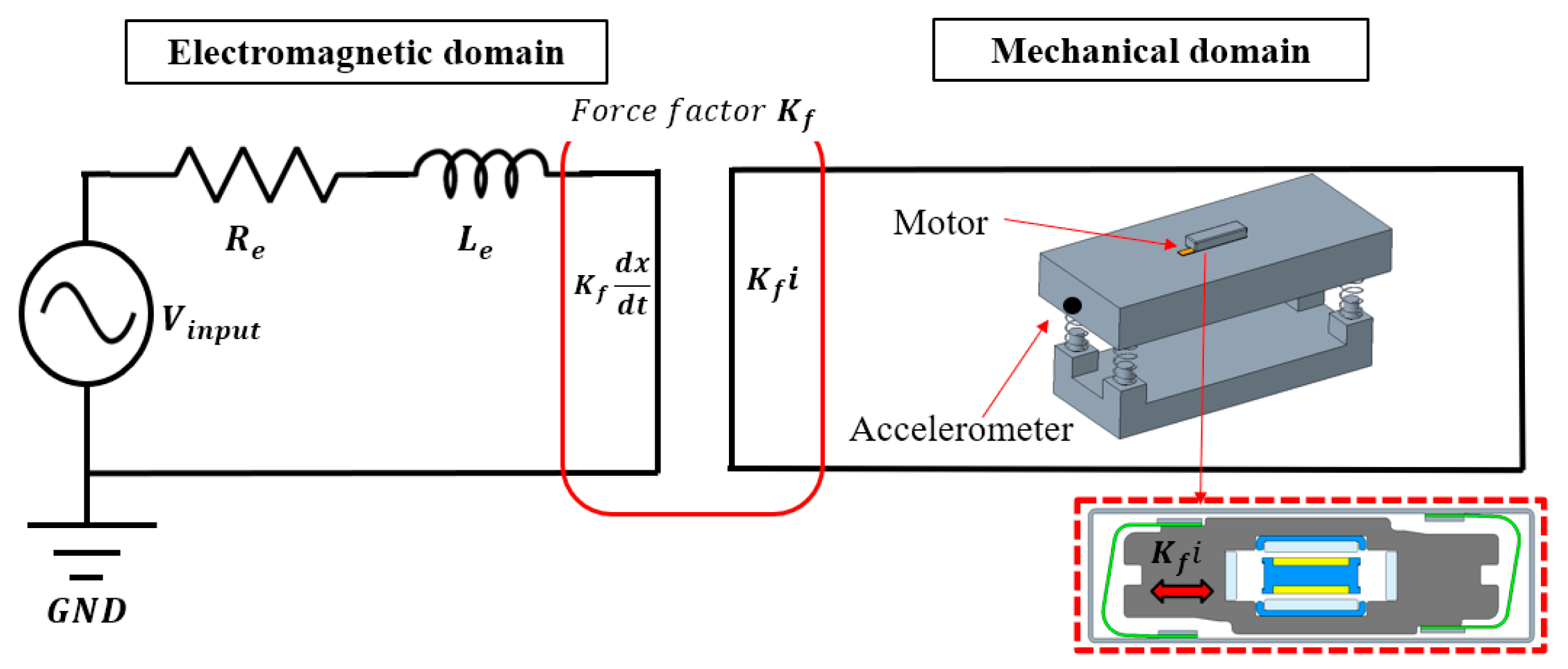
3.3. Electromagnetic–Mechanical Coupling Analysis Method
4. Experiments and Results
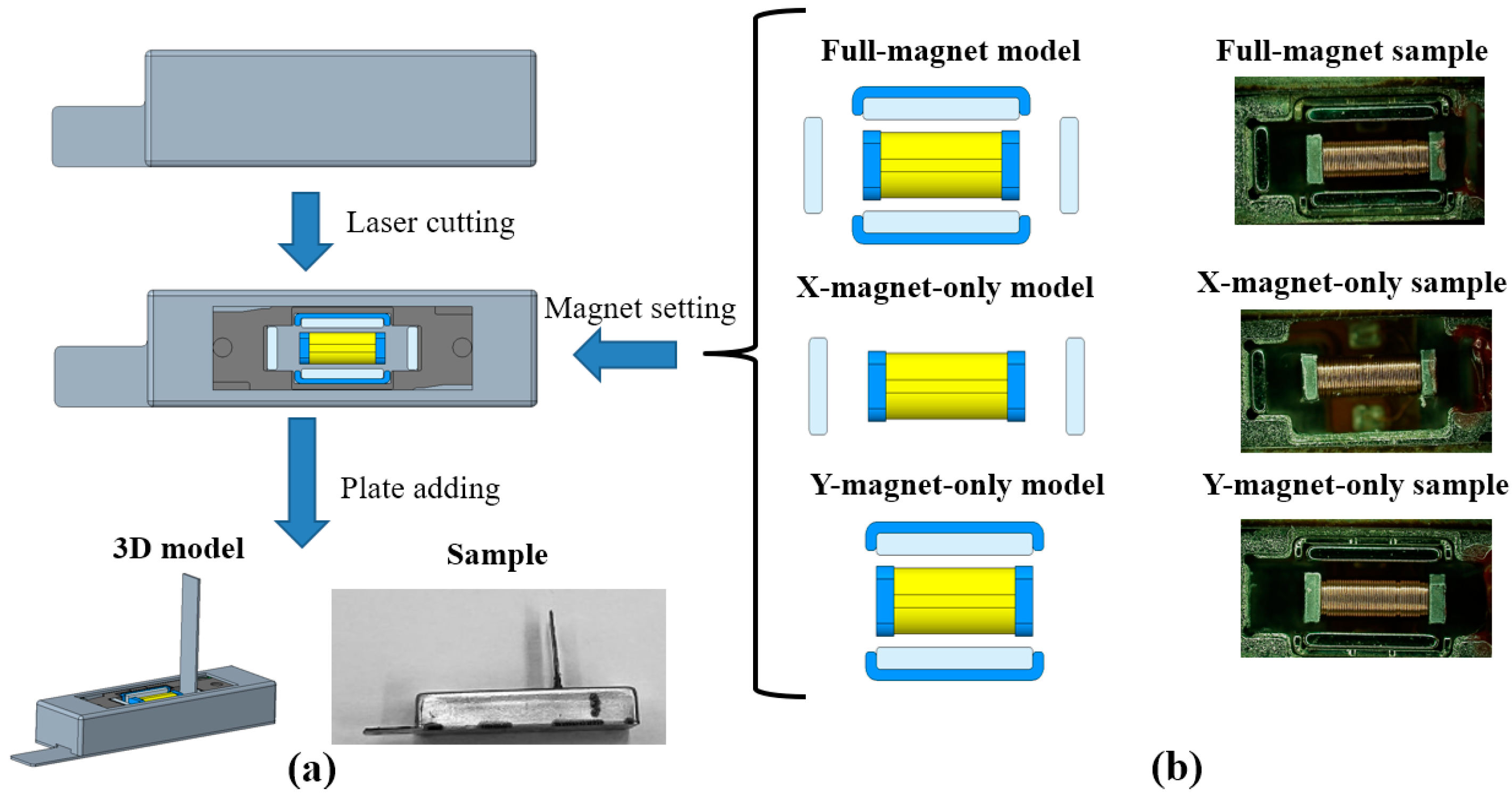
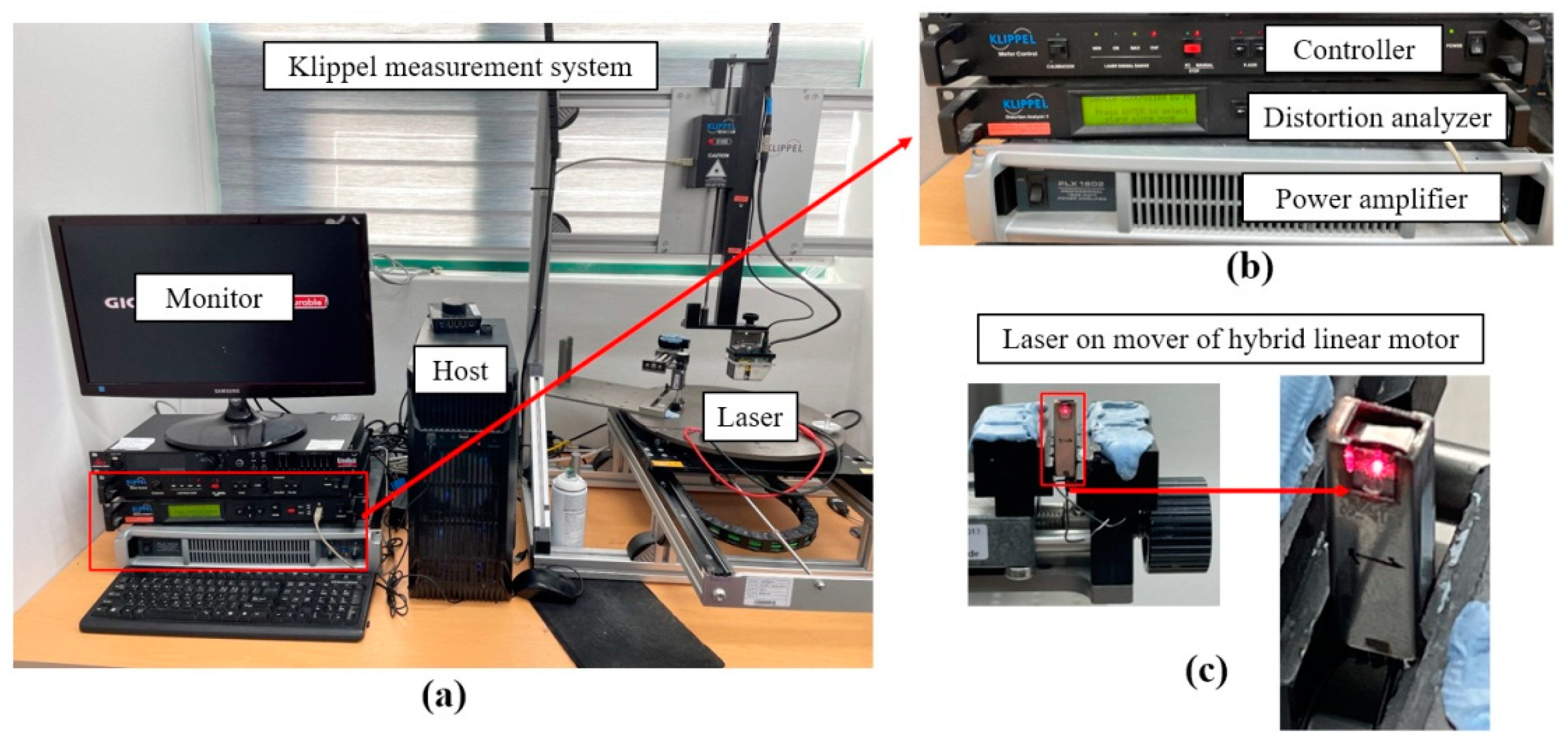
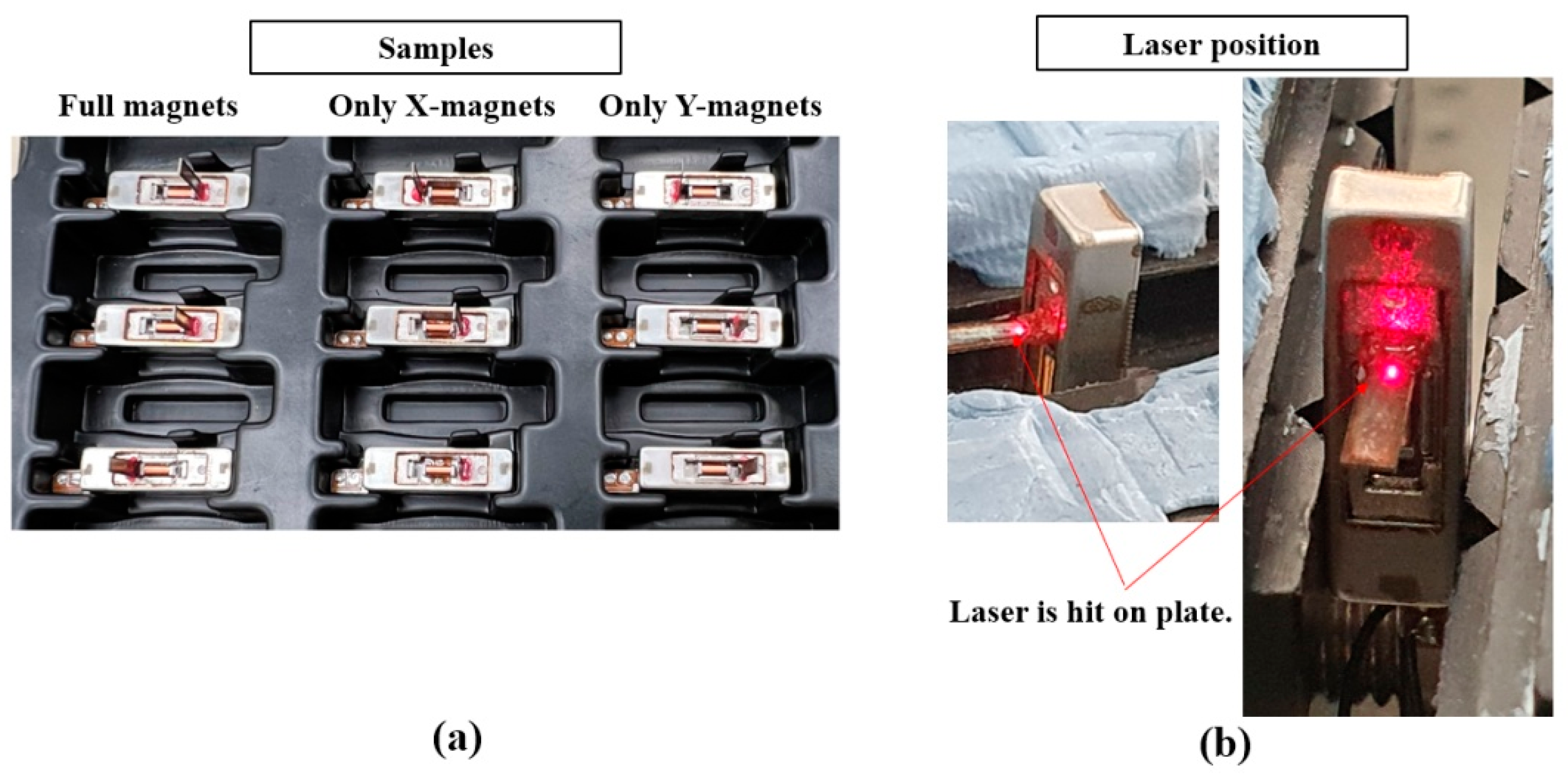
4.1. Experimental Setups
4.2. Total Stiffness Results
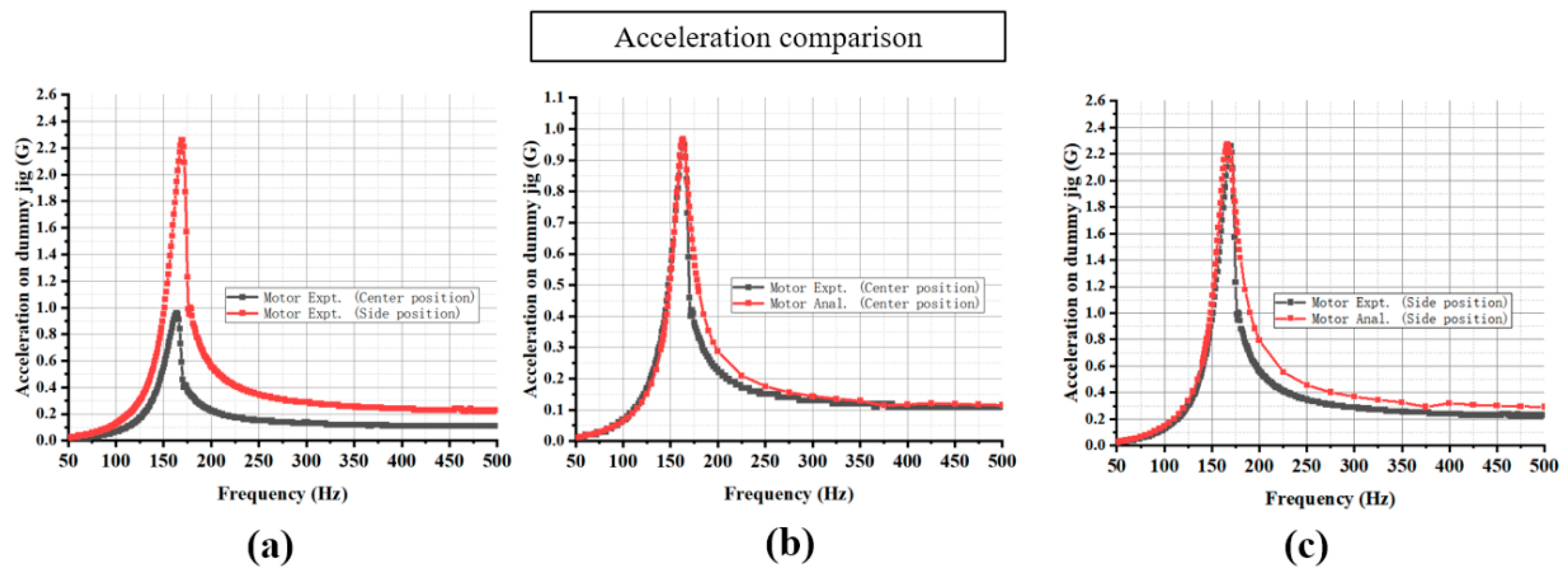
4.3. Displacement and Acceleration Results
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Sreelakshmi, M.; Subash, T.D. Haptic technology: A comprehensive review on its applications and future prospects. Mater. Today Proc. 2017, 4, 4182–4187. [Google Scholar] [CrossRef]
- Chung, S.U.; Hwang, G.Y.; Hwang, S.M.; Kang, B.S.; Kim, H.G. Development of brushless and sensorless vibration motor used for mobile phone. IEEE Trans. Magn. 2002, 38, 3000–3002. [Google Scholar] [CrossRef]
- Won, S.H.; Lee, J. Analysis of flat-type vibration motor for mobile phone. IEEE Trans. Magn. 2005, 41, 4018–4020. [Google Scholar]
- Hwang, S.M.; Lee, H.J.; Chung, S.U.; Hwang, G.Y.; Kang, B.S. Development of solenoid-type vibrators used for mobile phones. IEEE Trans. Magn. 2003, 39, 3262–3264. [Google Scholar] [CrossRef]
- Jiang, Z.X.; Park, K.H.; Kim, J.H.; Jiang, Y.W.; Xu, D.P.; Hwang, S.M. Analysis and design of a new linear vibration motor used to reduce magnetic flux leakage in in-vehicle infotainment. Appl. Sci. 2020, 10, 3370. [Google Scholar] [CrossRef]
- Jiang, Z.X.; Park, K.H.; Hwang, S.M. Design of a Width Slim Linear Vibration Motor Used for Automotive LCD Display Panel. IEEE Trans. Magn. 2022, 58, 8200405. [Google Scholar] [CrossRef]
- Fuchs, P. Virtual Reality Headsets—A Theoretical and Pragmatic Approach, 1st ed.; CRC Press: London, UK, 2017. [Google Scholar]
- Jiang, Z.X.; Park, J.H.; Xu, D.P.; Hwang, S.M. Electromagnetic-Mechanical Coupling Analysis of Linear Haptic Motor Considering Cogging Force Effect. In Proceedings of the 2022 IEEE 20th Biennial Conference on Electromagnetic Field Computation (CEFC), Virtual, Denver, CO, USA, 24–26 October 2022; pp. 1–2. [Google Scholar]
- Guarnieri, M. The development of ac rotary machines. IEEE Ind. Electron. Mag. 2018, 12, 28–32. [Google Scholar] [CrossRef]
- Amiri, M.S.; Ibrahim, M.F.; Ramli, R. Optimal parameter estimation for a DC motor using genetic algorithm. Int. J. Power Electron. Drive Syst. 2020, 11, 1047. [Google Scholar] [CrossRef]
- Zhu, X.; Jiang, M.; Xiang, Z.; Quan, L.; Hua, W.; Cheng, M. Design and optimization of a flux-modulated permanent magnet motor based on an airgap-harmonic-orientated design methodology. IEEE Trans. Ind. Electron. 2019, 67, 5337–5348. [Google Scholar] [CrossRef]
- Bendjedia, M.; Ait-Amirat, Y.; Walther, B.; Berthon, A. Position control of a sensorless stepper motor. IEEE Trans. Power Electron. 2011, 27, 578–587. [Google Scholar] [CrossRef]
- Lee, K.B.; Kim, J.H.; Kim, J.H. Horizontal linear vibrating actuator to reduce smart phone thickness. J. Vibroengineering. 2013, 15, 2003–2011. [Google Scholar]
- Jiang, Z.; Park, K.; Hwang, S. Novel Magnetic Circuit Design and Acceleration Calculation of Horizontal Linear Vibration Motor. Actuators 2022, 11, 149. [Google Scholar] [CrossRef]
- Sun, J.; Tao, Z.; Li, H.; Zhu, K.; Wang, D.; Wu, H.; Xu, T. A MEMS Voice Coil Motor with a 3D Solenoid Coil. In Proceedings of the 2021 IEEE 16th International Conference on Nano/Micro Engineered and Molecular Systems (NEMS), Xiamen, China, 25–29 April 2021; pp. 1745–1748. [Google Scholar]
- Meng, B.; Xu, H.; Liu, B.; Dai, M.; Zhu, C.; Li, S. Novel magnetic circuit topology of linear force motor for high energy utilization of permanent magnet: Analytical modelling and experiment. Actuators 2021, 10, 32. [Google Scholar] [CrossRef]
- Tong, W.; Li, S.; Pan, X.; Wu, S.; Tang, R. Analytical model for cogging torque calculation in surface-mounted permanent magnet motors with rotor eccentricity and magnet defects. IEEE Trans. Energy Convers. 2020, 35, 2191–2200. [Google Scholar] [CrossRef]
- Xu, D.P.; Jiang, Y.W.; Kwon, J.H.; Hwang, S.M. Analysis of the effects of electromagnetic circuit variables on sound pressure level and total harmonic distortion in a balanced armature receiver. IEEE Trans. Magn. 2017, 53, 8001504. [Google Scholar] [CrossRef]
- Meessen, K.J.; Paulides, J.J.H.; Lomonova, E.A. Force calculations in 3-D cylindrical structures using Fourier analysis and the Maxwell stress tensor. IEEE Trans. Magn. 2012, 49, 536–545. [Google Scholar] [CrossRef]
- Yan, X.K.; Koh, C.S.; Ryu, J.S.; Xie, D. Comparison of Force Calculation Methods in 2D and 3D Finite Element Method. KIEE Int. Trans. Electr. Mach. Energy Convers. Syst. 2001, 11, 137–145. [Google Scholar]
- Zaouia, M.; Benamrouche, N.; Rachek, M. Electromagnetic-mechanical coupled model of tubular linear stepping motors. Electromotion 2011, 18, 331–339. [Google Scholar]
- Kim, J.H.; Jiang, Y.W.; Hwang, S.M. Analysis of a vibrating motor considering electrical, magnetic, and mechanical coupling effect. Appl. Sci. 2019, 9, 1434. [Google Scholar] [CrossRef]

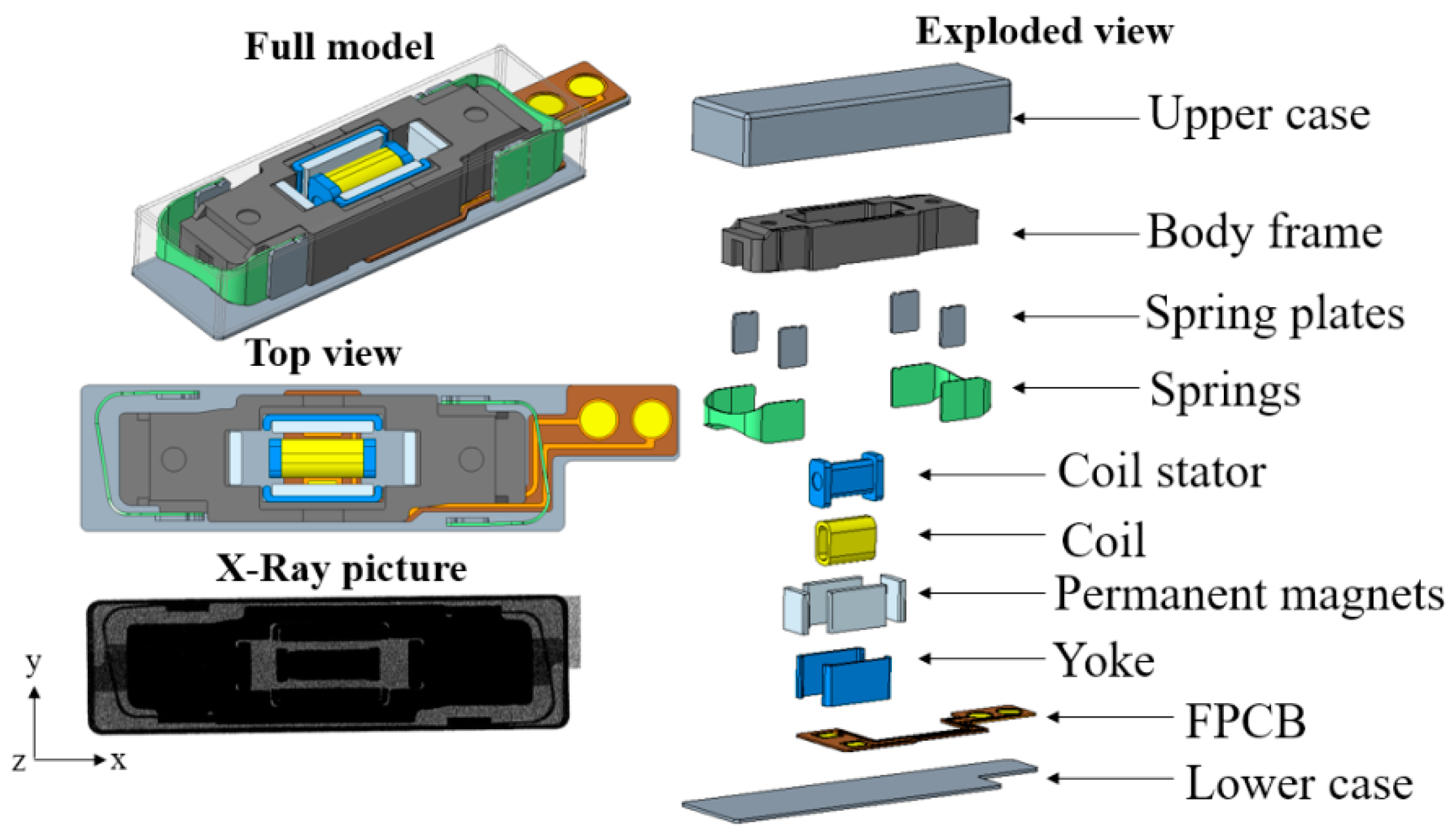
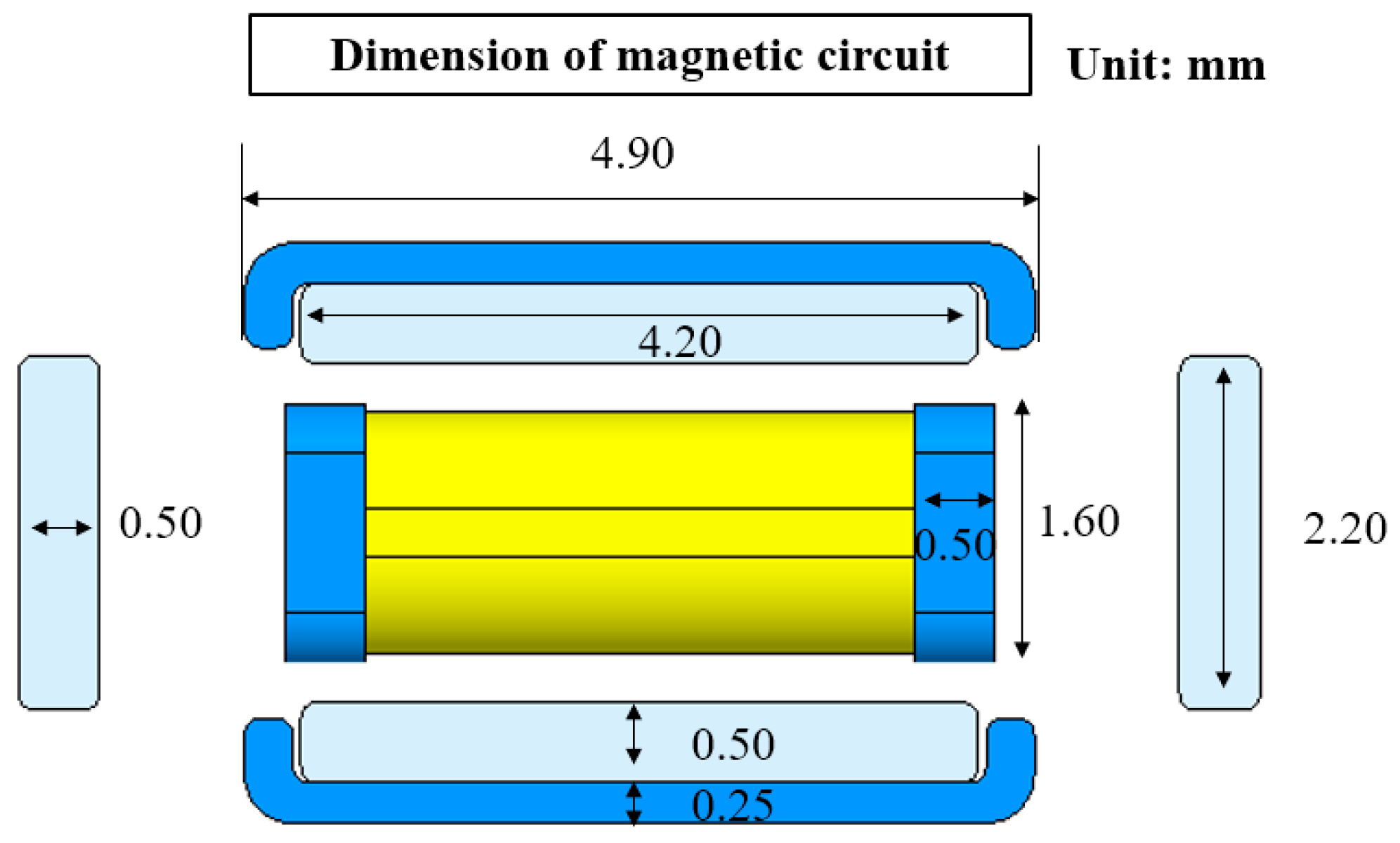




| Part | Material | Density [kg/m3] | Young’s Modulus [MPa] | Poisson’s Ratio |
|---|---|---|---|---|
| Spring and spring plate | Stainless steel | 7551 | 200 × 103 | 0.30 |
| Yoke and coil stator | Cold-rolled carbon steel | 6286 | 207 × 103 | 0.29 |
| Permanent magnet | Neodymium iron boron | 6988 | 41.4 × 103 | 0.30 |
| Body frame | Tungsten alloy | 17,156 | 250 × 103 | 0.29 |
| Coil | Daikoku high-tension wire | 6101 | 126 × 103 | 0.34 |
| Model | Moving Mass [kg] | [N/m] | [N/m] | (Anal.) [N/A] | [N/A] |
|---|---|---|---|---|---|
| Full-magnet | 2.45 × 10−6 | 3339.0 | 812.0 | 2527.0 | 2584.8 |
| X-magnet-only | 2.33 × 10−6 | 3339.0 | 129.5 | 3209.5 | 3264.9 |
| Y-magnet-only | 2.41 × 10−6 | 3339.0 | 370.8 | 2968.2 | 2996.7 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jiang, Z.; Park, J.; Xu, D.; Hwang, S. Analysis Method Development of Hybrid Linear Motor Considering Cogging Force Effect. Actuators 2023, 12, 99. https://doi.org/10.3390/act12030099
Jiang Z, Park J, Xu D, Hwang S. Analysis Method Development of Hybrid Linear Motor Considering Cogging Force Effect. Actuators. 2023; 12(3):99. https://doi.org/10.3390/act12030099
Chicago/Turabian StyleJiang, Zhixiong, Jihun Park, Danping Xu, and Sangmoon Hwang. 2023. "Analysis Method Development of Hybrid Linear Motor Considering Cogging Force Effect" Actuators 12, no. 3: 99. https://doi.org/10.3390/act12030099
APA StyleJiang, Z., Park, J., Xu, D., & Hwang, S. (2023). Analysis Method Development of Hybrid Linear Motor Considering Cogging Force Effect. Actuators, 12(3), 99. https://doi.org/10.3390/act12030099






