Mechanism Analysis and Control of Lateral Instability of 4WID Vehicle Based on Phase Plane Analysis Considering Front Wheel Angle
Abstract
:1. Introduction
2. Distributed Drive Electric Vehicle Dynamics Model
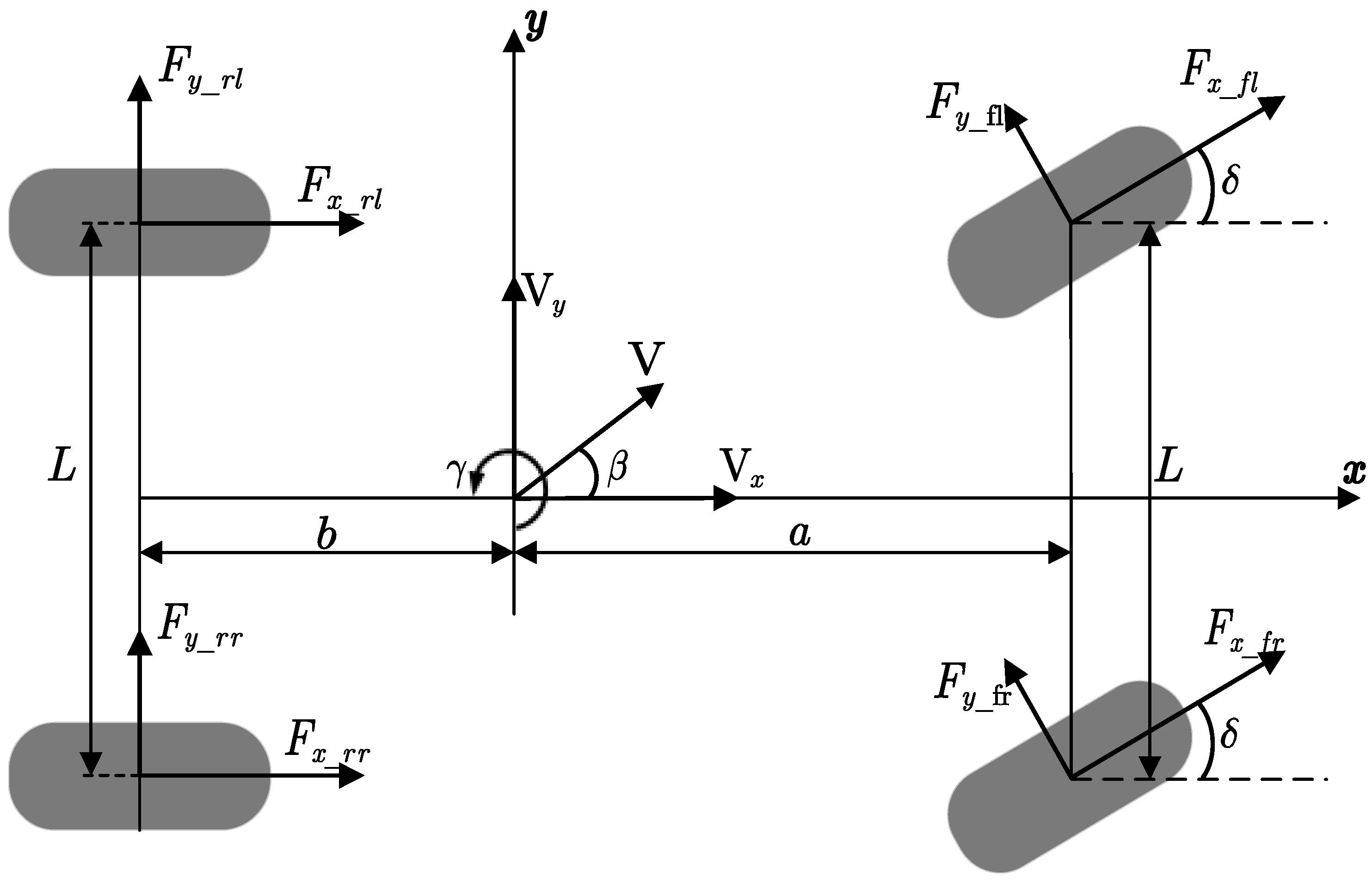
2.1. Distributed Drive Vehicle Seven-Degree-of-Freedom Dynamics Model
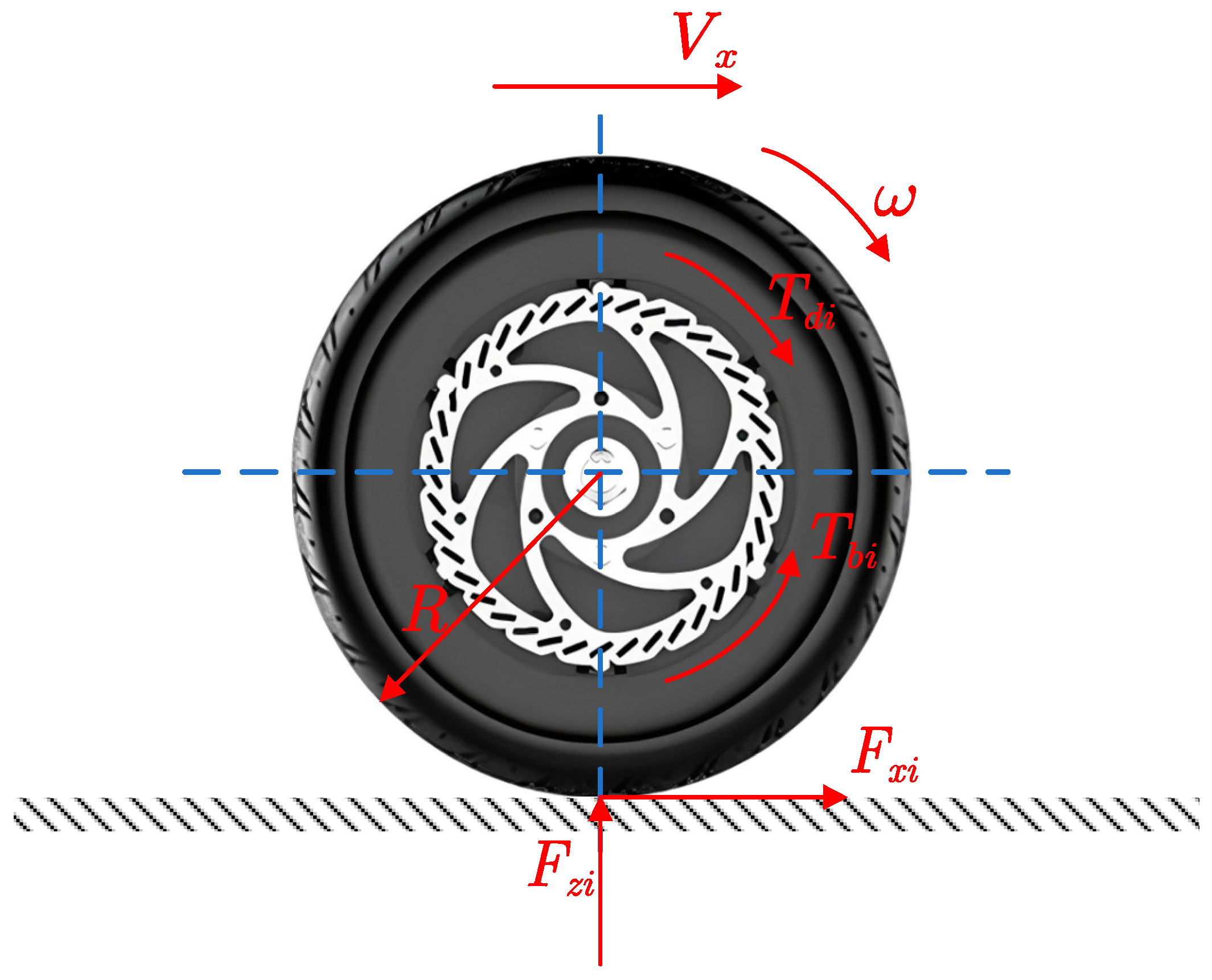
2.2. Wheel Models
2.3. Magic Formula Tyre Model
2.4. In-Wheel Motor Model
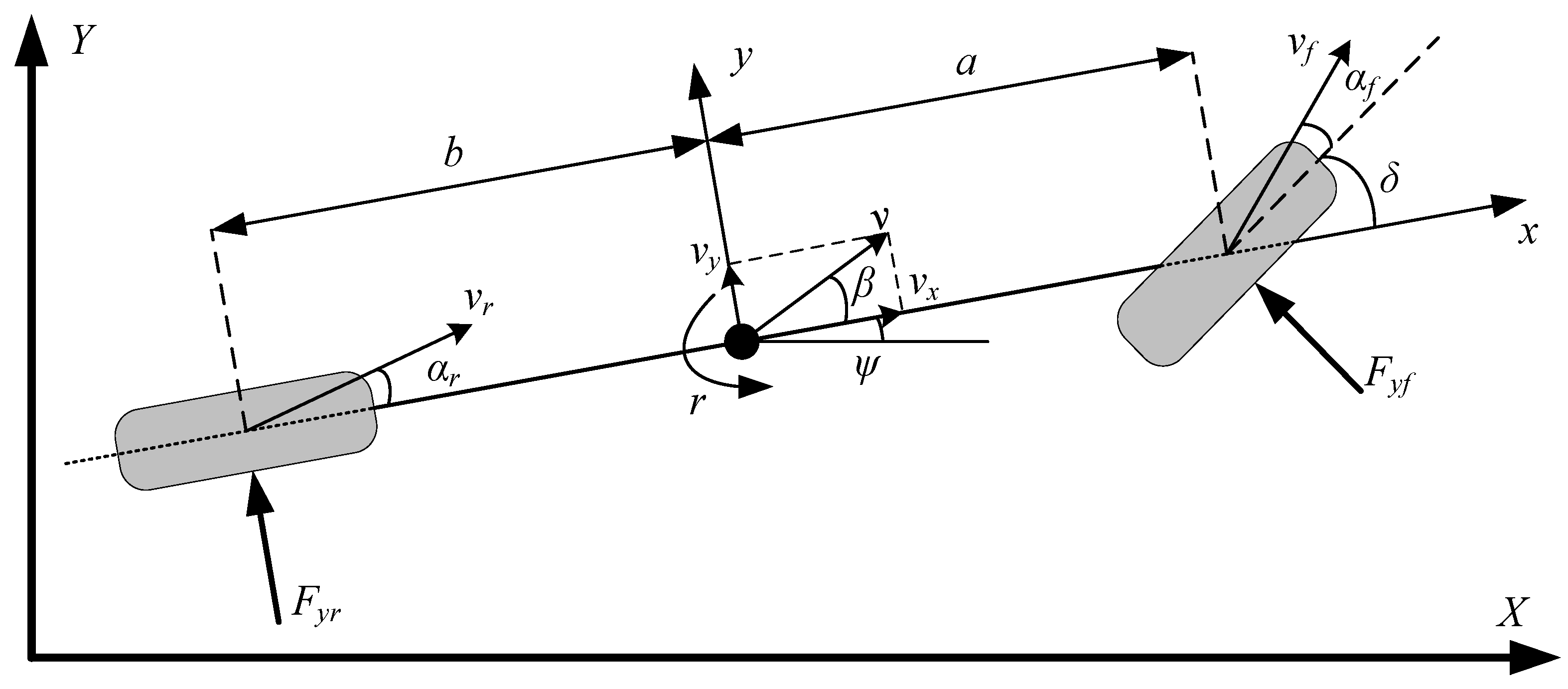
2.5. 2-DOF Vehicle Reference Model
3. Establishment of Phase Plane and Stability Boundary Libraries
3.1. Establishment of Phase Plane
3.2. Analysis of Influencing Factors of Phase Plane Stability Region
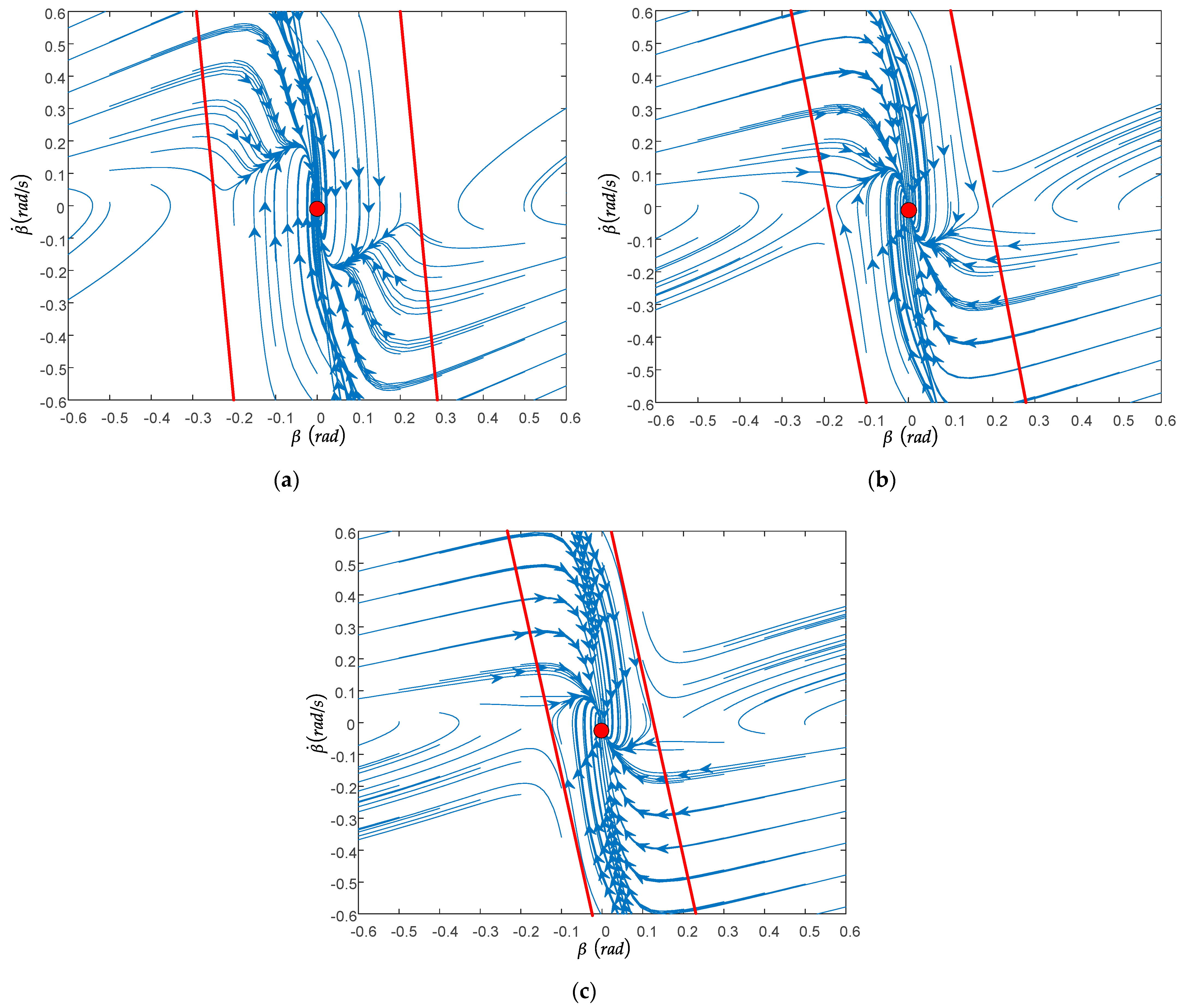
3.2.1. Influence of Longitudinal Speed on the Phase Plane
3.2.2. The Influence of the Road Adhesion Coefficient on the Phase Plane
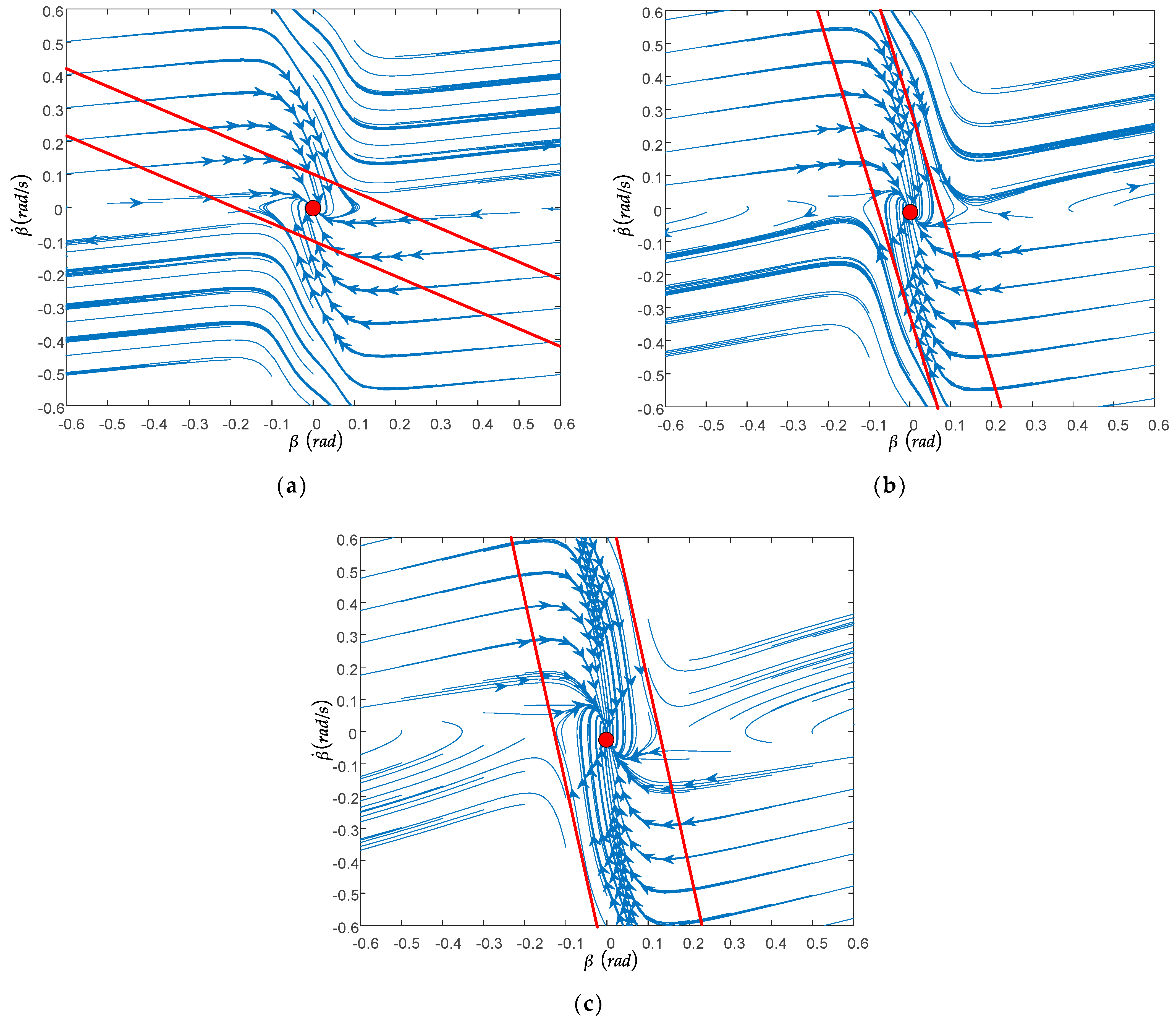
3.2.3. The Influence of the Front Wheel Angle on the Phase Plane
3.3. Design of Phase Plane Stability Region Boundary
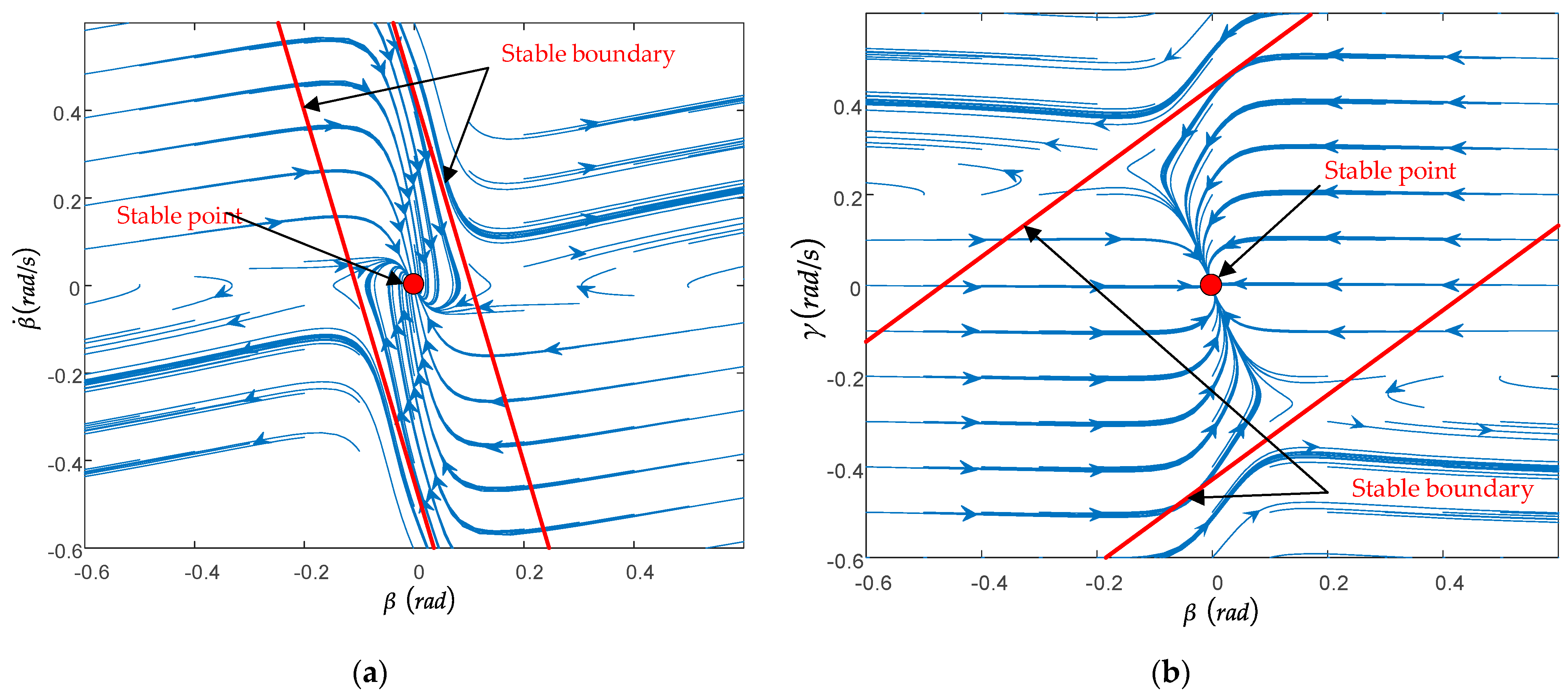
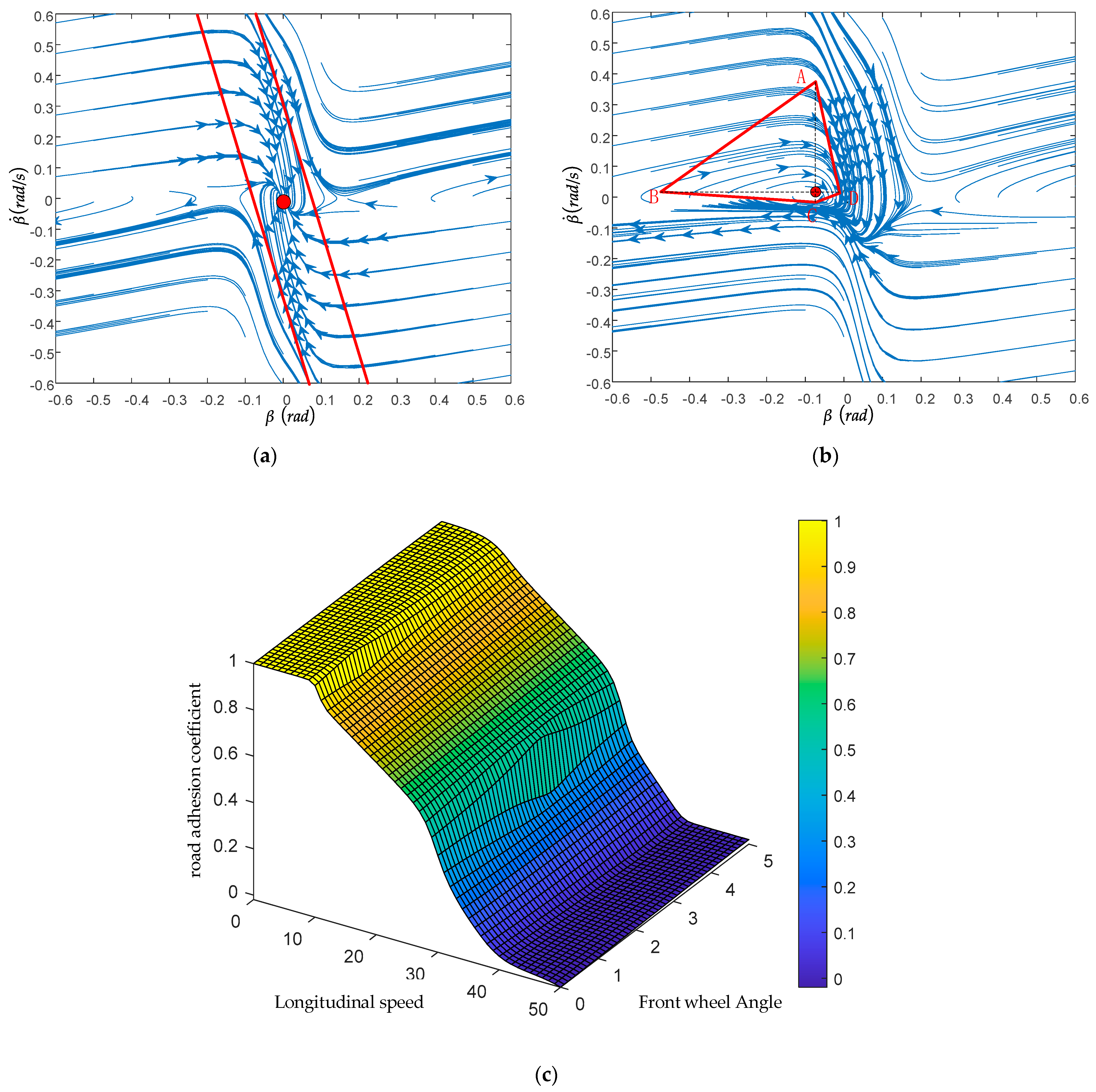
3.3.1. Judgment of Boundary Type of Stable Region
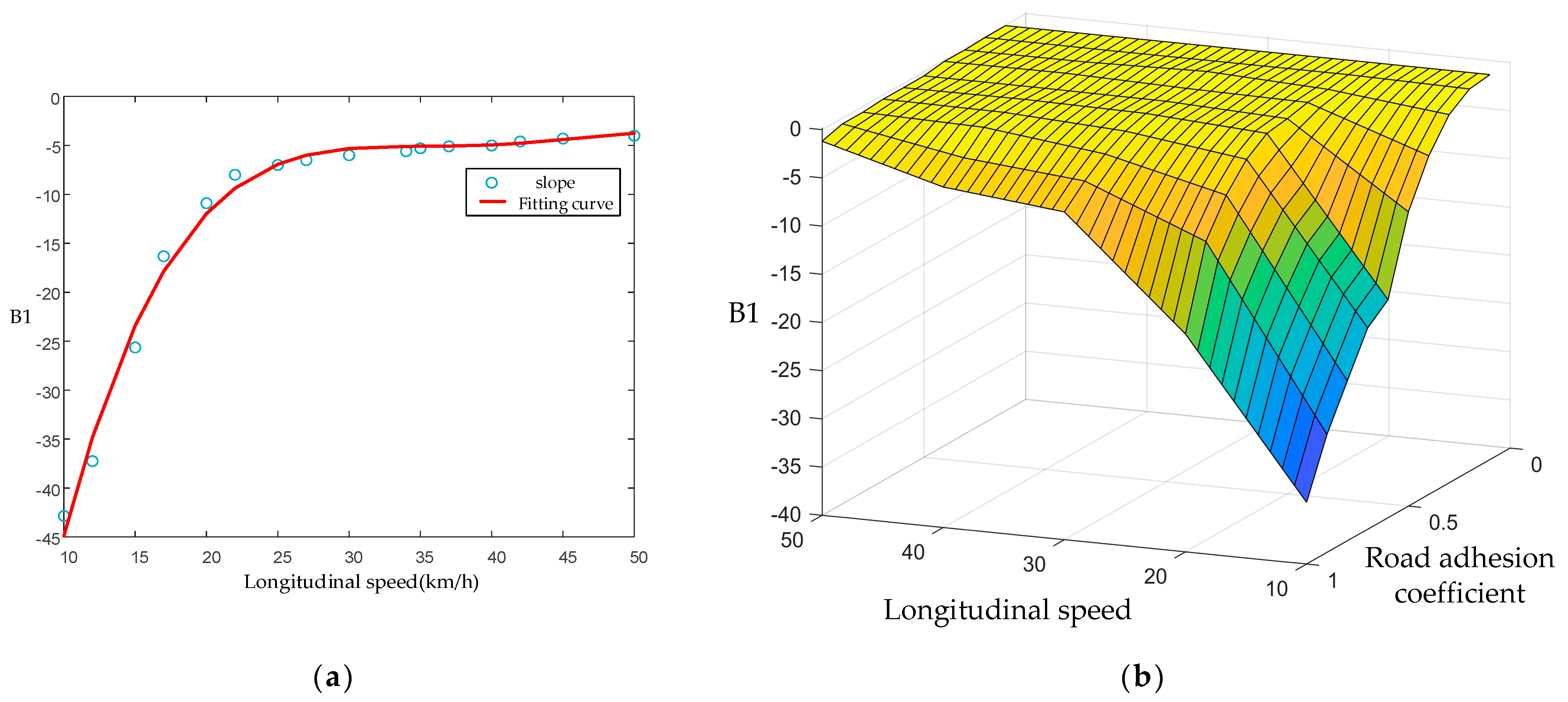
3.3.2. The Boundary Design of the First Type of Stability Region
3.3.3. The Boundary Design of the Second Type of Stability Region
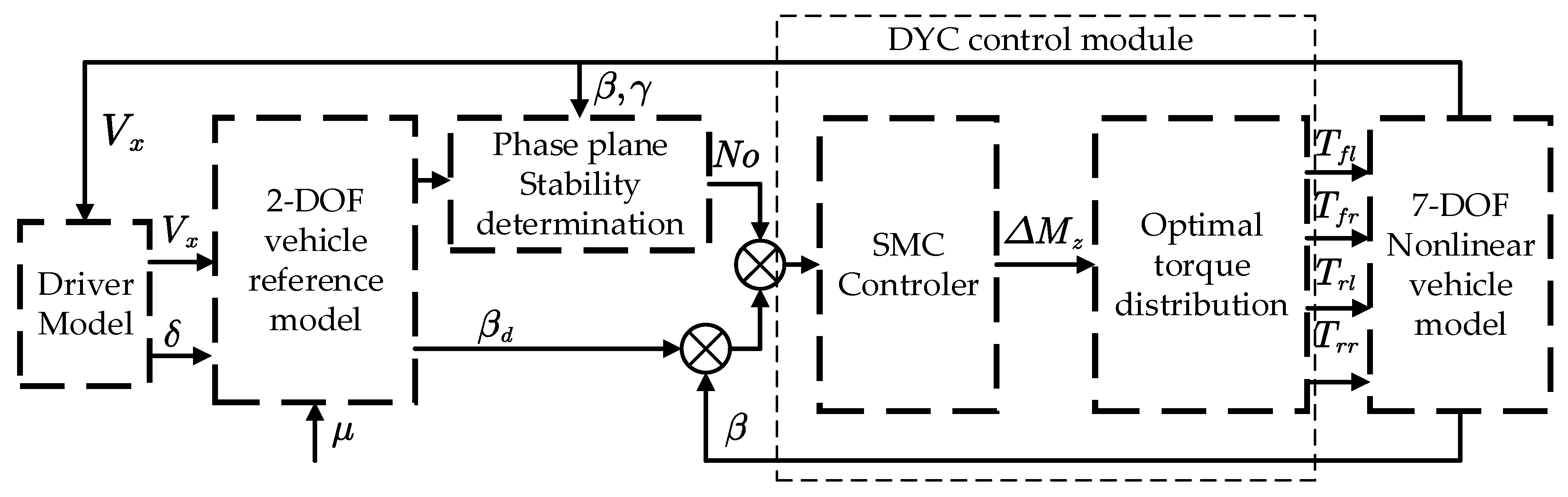
4. Lateral Stability Controller Design
4.1. Upper Controller Design
4.1.1. Yaw Moment Calculation Based on Sideslip Angle Error
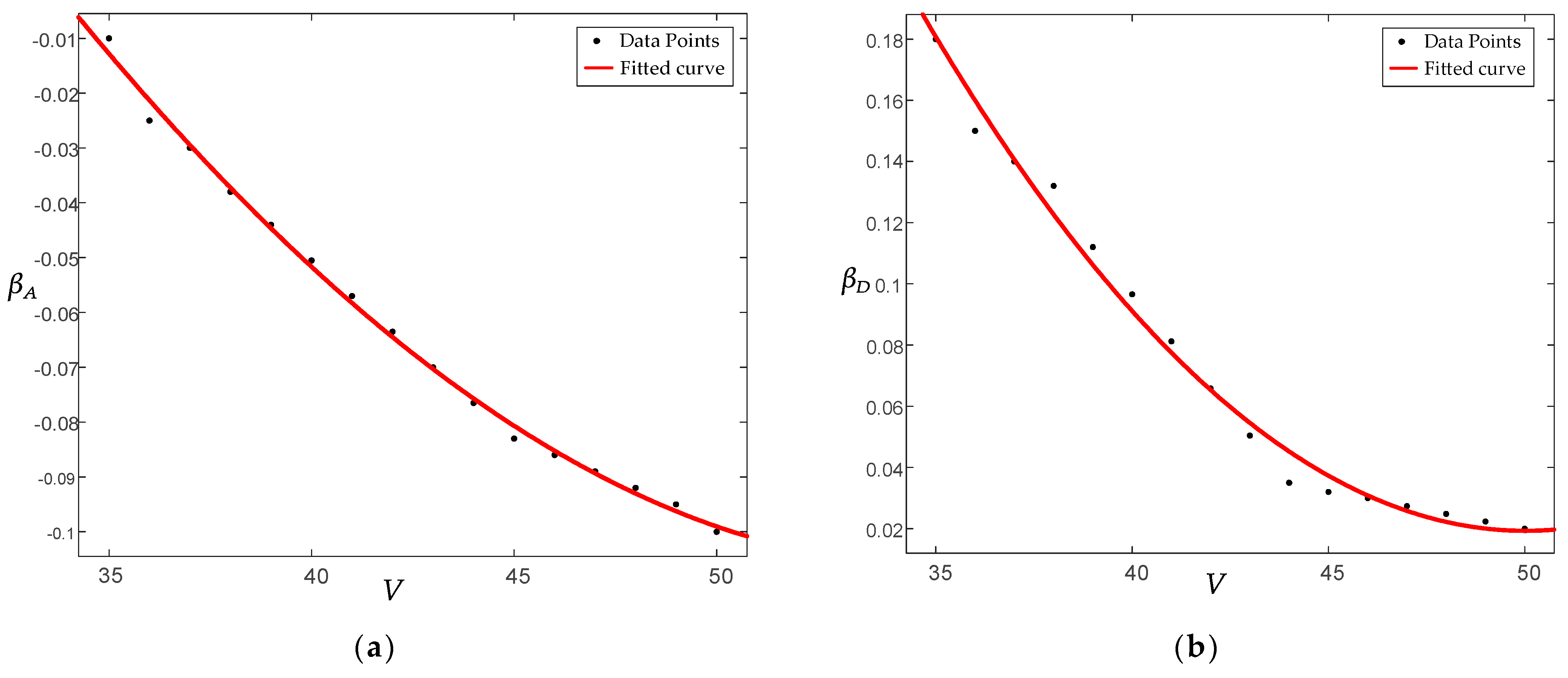
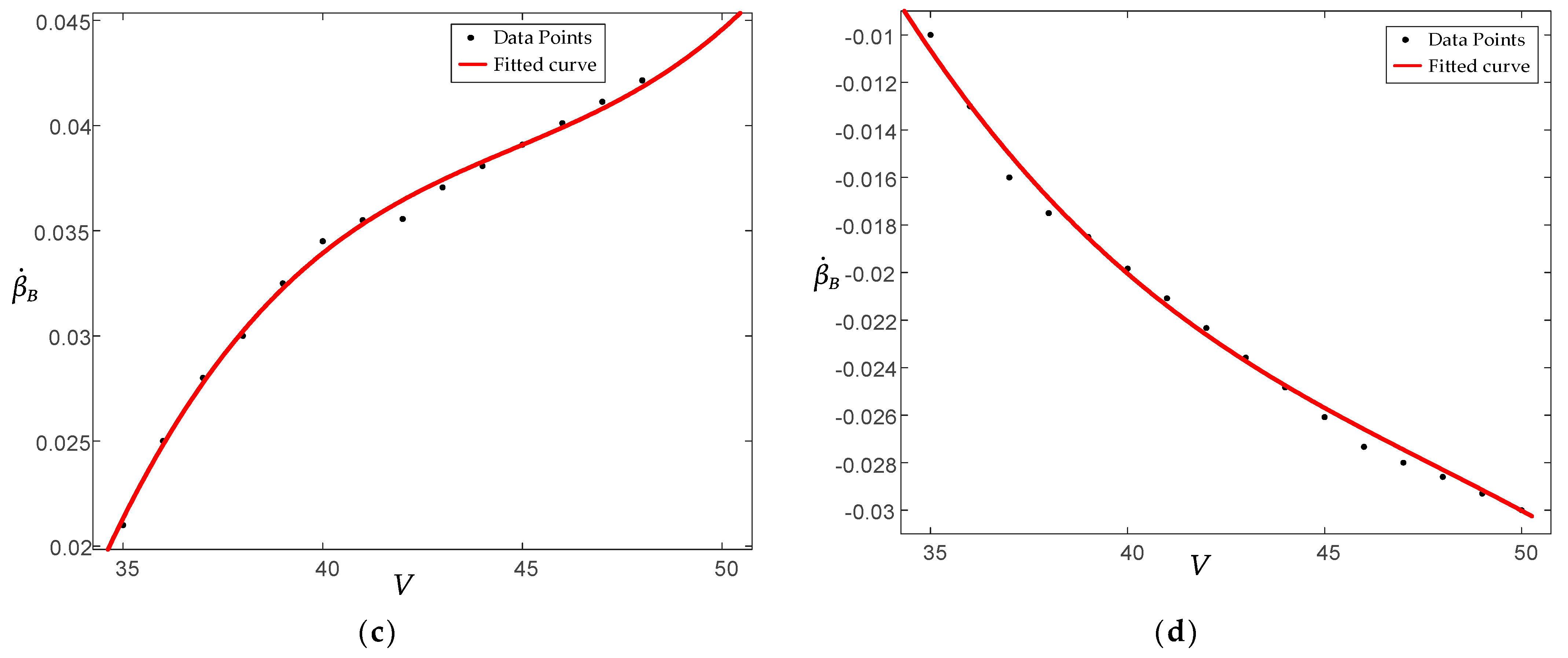
4.1.2. Controller Stability Verification
4.2. Lower Controller Design
5. Simulation Verification
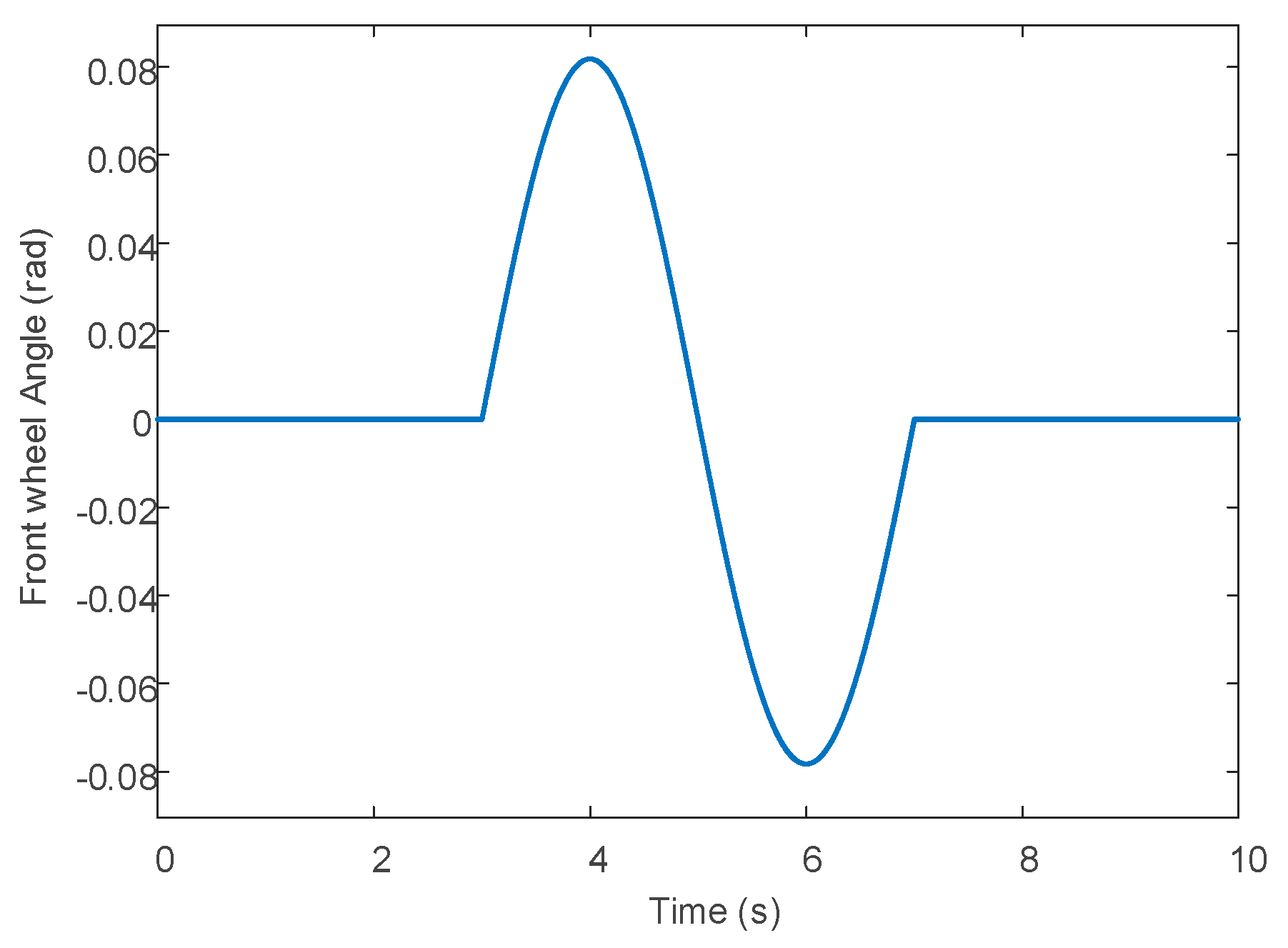
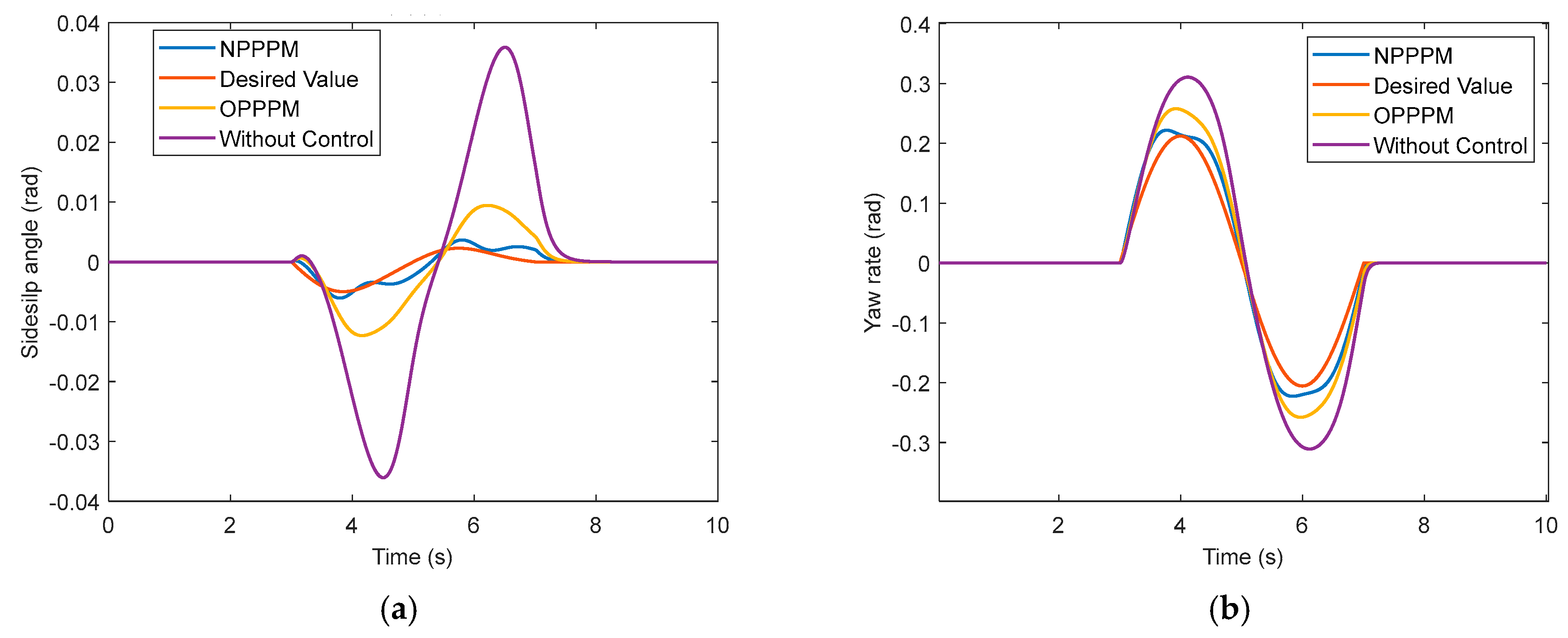
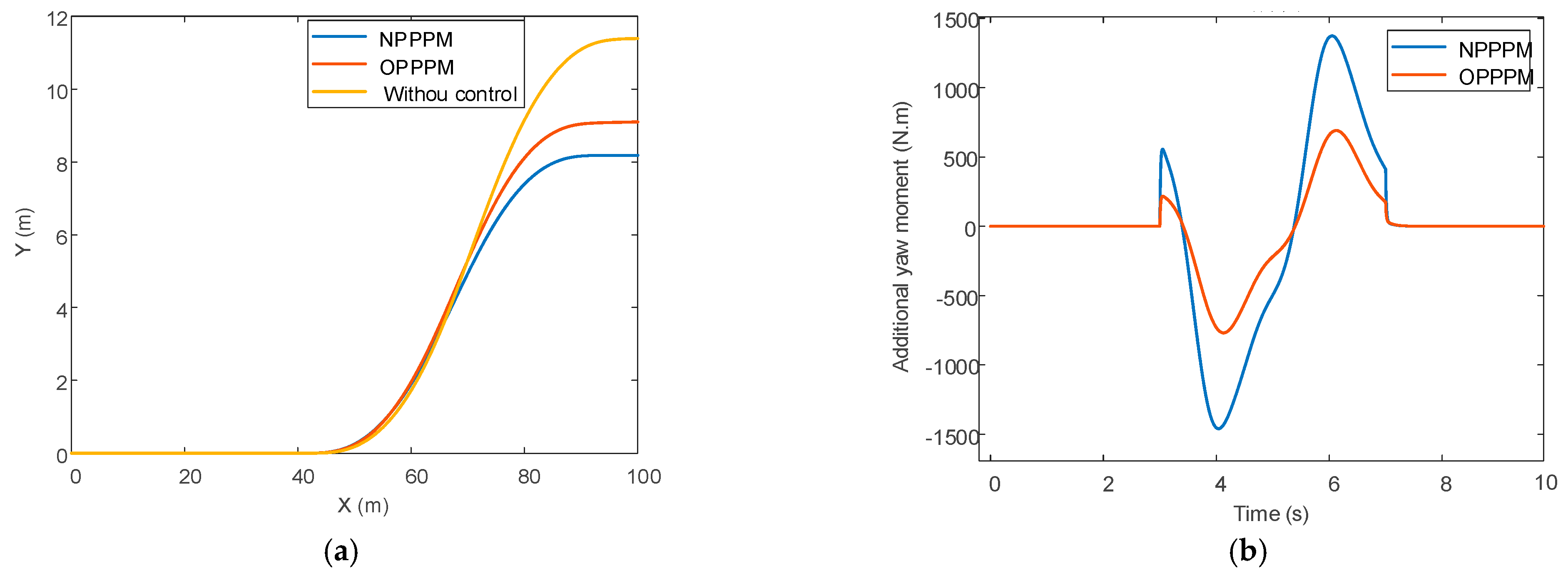
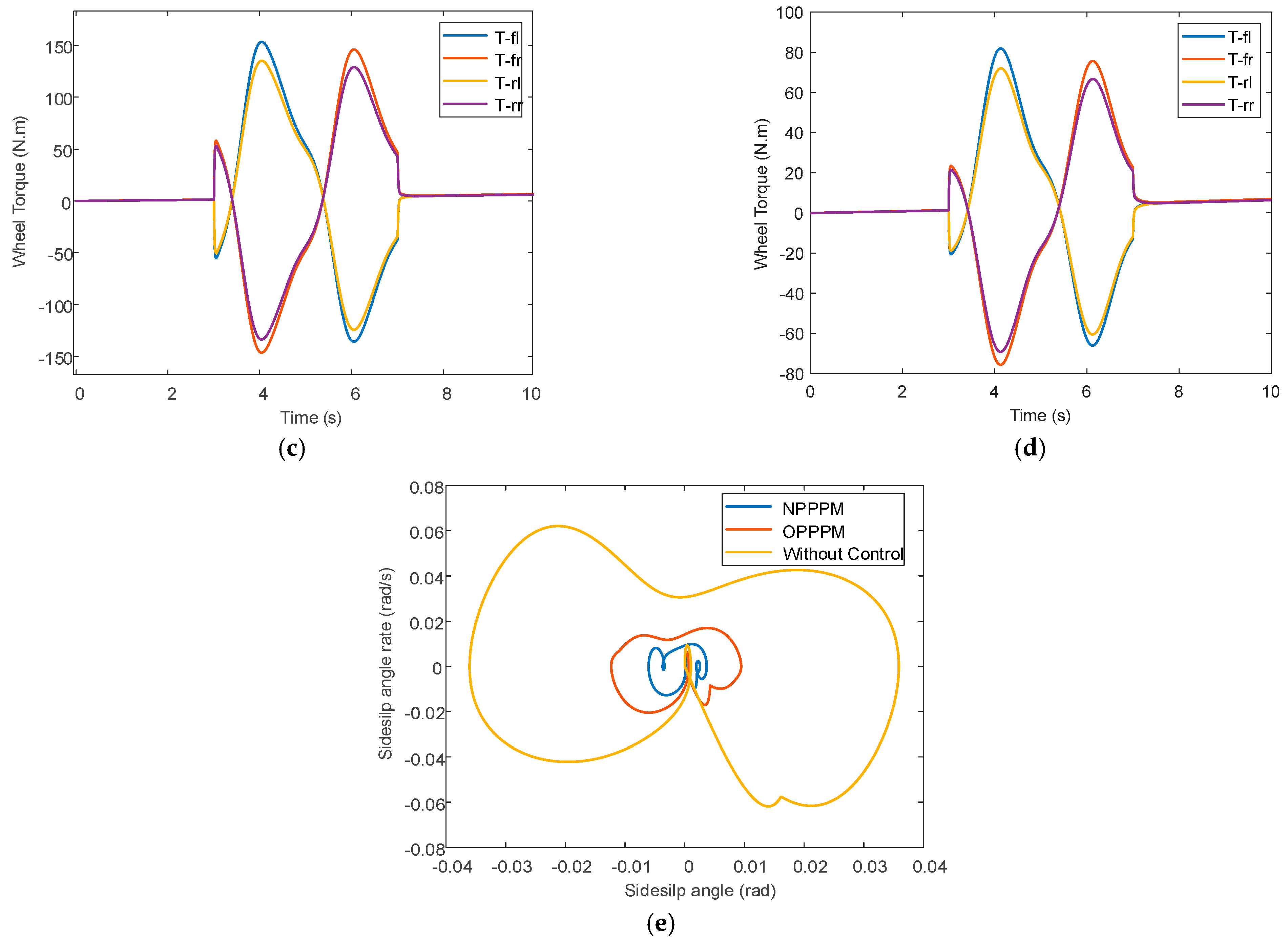
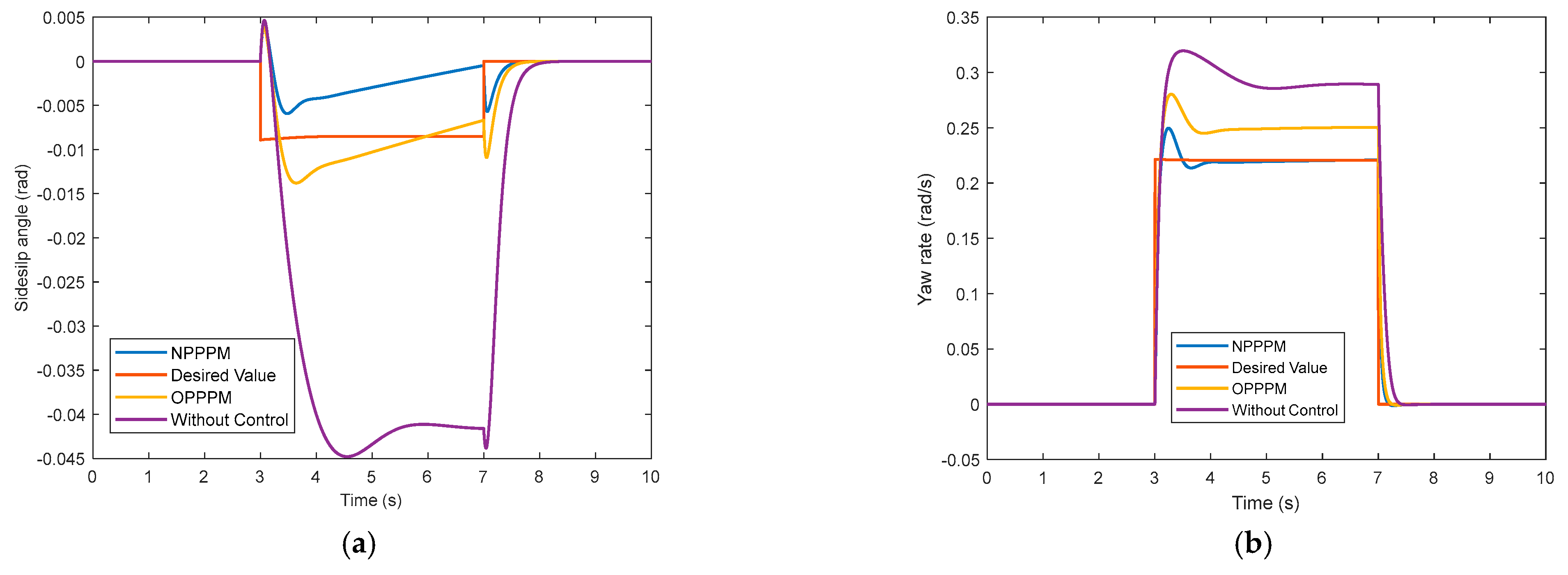
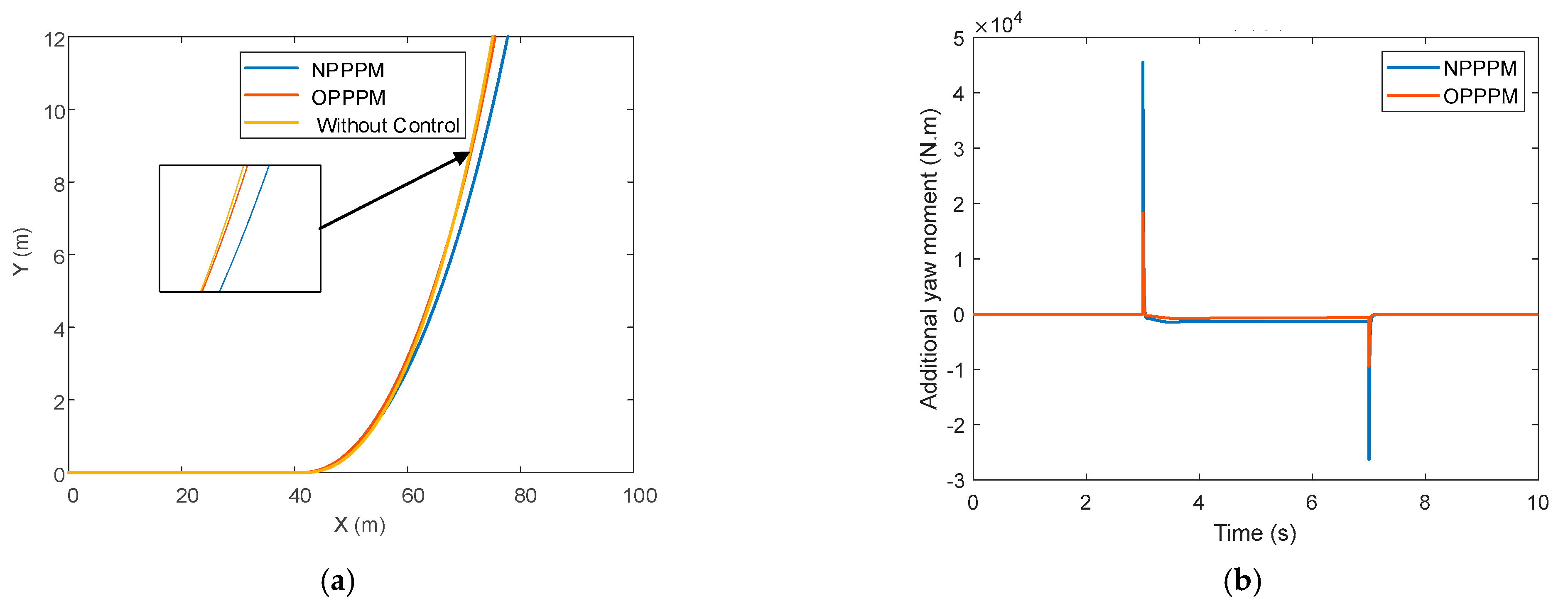
5.1. Sine Input of the Front Wheel Angle
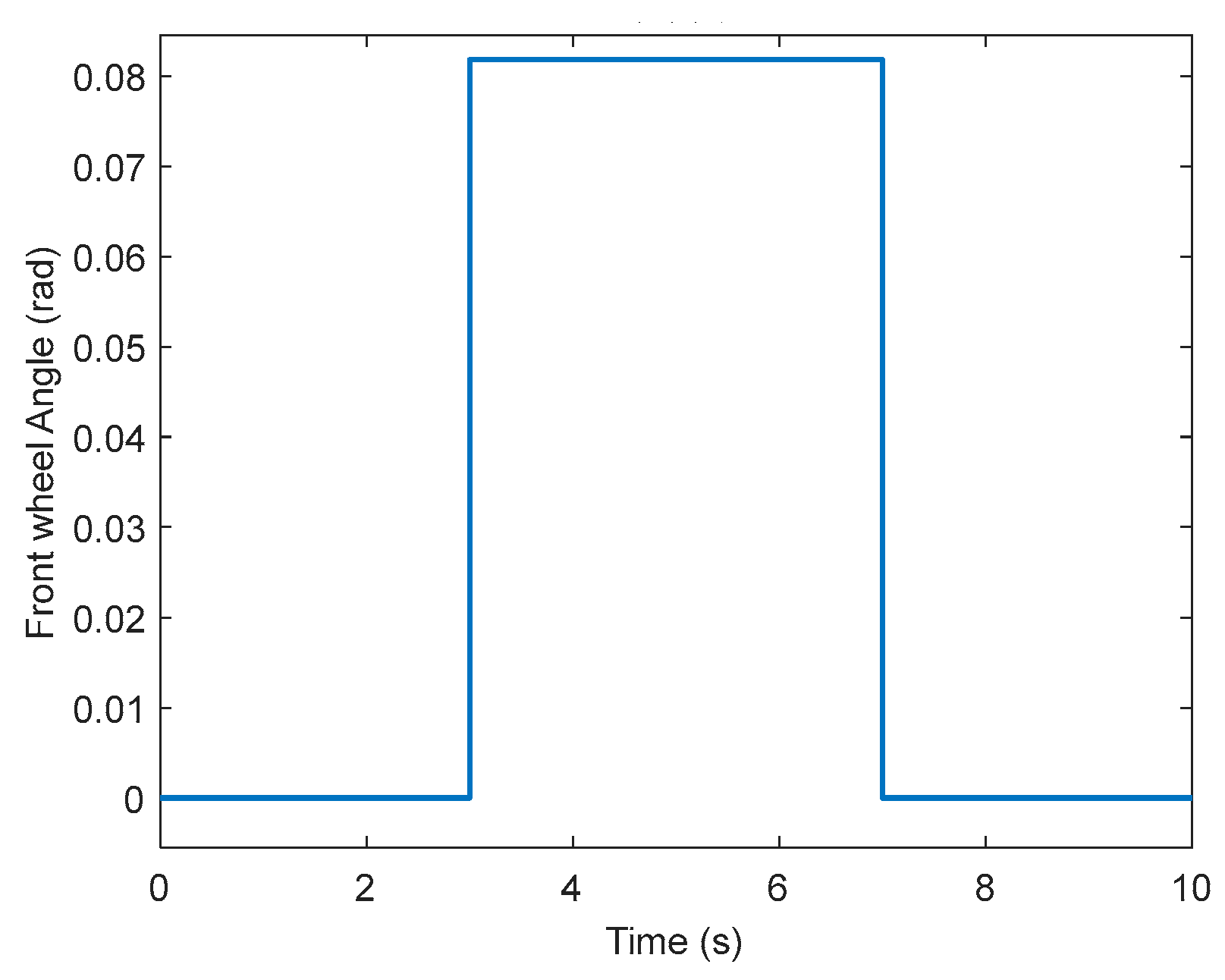
5.2. Step Input of the Front Wheel Angle
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Jiang, W.; Zhang, L. A Review on Stability Control Technology of Distributed Drive Electric Vehicle. Automob. Appl. Technol. 2021, 46, 210–211, 217. [Google Scholar]
- Chen, G. A review of stability control for distributed drive electric vehicles. China-Arab. States Sci. Technol. 2021, 32, 64–66. [Google Scholar]
- Huang, Y.; Guo, G. A Review of Torque Distribution Strategies for Distributed Drive Electric Vehicles. Automob. Appl. Technol. 2020, 45, 230–236, 248. [Google Scholar]
- Yan, S.; Zhang, H.; Li, Q.; Gao, C. Stability Research of Four-wheel-driven Electric Vehicle Based on Co-Simulink of CarSim/Simulink. Agric. Equip. Veh. Eng. 2020, 58, 16–21. [Google Scholar]
- Li, Y.; Xu, X. Review and Future Development of In-Wheel Motor Drive Technology. Mot. Control Appl. 2017, 44, 1–7, 18. [Google Scholar]
- Su, L.; Zhang, F. Research Progress and Trend of Integrated Dynamics Control of Distributed Driven Electric Vehicles. Chin. J. Automot. Eng. 2022, 12, 715–733. [Google Scholar]
- Wu, J.; Kong, Q.; Yang, K.; Liu, Y.; Cao, D.; Li, Z. Research on the Steering Torque Control for Intelligent Vehicles Co-Driving With the Penalty Factor of Human–Machine Intervention. IEEE Trans. Syst. Man Cybern. Syst. 2023, 53, 59–70. [Google Scholar]
- Wu, J.; Zhang, J.; Nie, B.; Liu, Y.; He, X. Adaptive Control of PMSM Servo System for Steering-by-Wire System with Disturbances Observation. IEEE Trans. Transp. Electrif. 2022, 8, 2015–2028. [Google Scholar] [CrossRef]
- Li, X. Research on Instability Mechanism and Handling Stability Control of Distributed Drive Electric Vehicles under Combined Slip Condition; Jilin University: Ji Lin, China, 2020. [Google Scholar]
- Sachs, H.K.; Singh, M. Automobile Stability—A Study of the Domain of Attraction. Veh. Syst. Dyn. 1977, 6, 169–177. [Google Scholar]
- Inagaki, S.; Kushiro, I.; Yamamoto, M. Analysis on Vehicle Stability in Critical Cornering Using Phase-Plane Method. JSAE Rev. 1994, 16, 216. [Google Scholar]
- Gao, Y. Stability Control Study Based on the Stability Boundary of Phase Plane for Light Vehicle; Jilin University: Ji Lin, China, 2013. [Google Scholar]
- Chen, Y.; Chen, Y. Study on Vehicle Instability Mechanism Based on Lyapunov First Method and Phase Plane. Veh. Power Technol. 2021, 164, 11–14, 22. [Google Scholar]
- Zheng, X.; Zhao, Y. Design of Path Tracking Controller Based on Lyapunov Stability Theory. Automob. Technol. 2020, 539, 1–5. [Google Scholar]
- Guo, K. Principles of Vehicle-Handling Dynamics; Science and Technology Press: Nanjing, China, 2011; p. 42. [Google Scholar]
- Sadri, S.; Christine Qiong, W. Lateral stability analysis of on-roadvehicles using the concept of Lyapunov exponents. In Proceedings of the IEEE Intelligent Vehicles Symposium (IV), Madrid, Spain, 3–7 June 2012; pp. 450–455. [Google Scholar]
- Sadri, S.; Christine Qiong, W. Lateral stability analysis of on-road vehicles using Lyapunov’s direct method. In Proceedings of the IEEE Intelligent Vehicles Symposium, Madrid, Spain, 3–7 June 2012; pp. 821–826. [Google Scholar]
- Sadri, S.; Christine, W. Stability analysis of a nonlinear vehicle model in plane motion using the concept of Lyapunov exponents. Veh. Syst. Dyn. 2013, 51, 906–924. [Google Scholar]
- Luo, Y.; Lai, E. Research on vehicle stability judgment based on energy method. Automot. Eng. 2014, 36, 1534–1538. [Google Scholar]
- Guo, Y.; Lu, Y.; Fu, R. Simulation analysis of lateral stability of large passenger cars. China J. Highw. Transp. 2018, 31, 156–164, 230. [Google Scholar]
- Fei, L.; Lu, X. Vehicle Stability Criterion Based on Phase Plane Method. J. South China Univ. Technol. 2014, 42, 63–70. [Google Scholar]
- Liu, J.; Song, J.; Li, H.; Huang, H. Direct yaw-moment control of vehicles based on phase plane analysis. Proc. Inst. Mech. Eng. Part D J. Automob. Eng. 2022, 236, 2459–2474. [Google Scholar]
- Liu, X.; Liu, J. Research on direct yaw moment control of vehicle based on phase plane method. J. Hefei Univ. Technol. 2019, 42, 1455–1461. [Google Scholar]
- Lai, F.; Huang, C.; Ye, X. Analysis of Vehicle Driving Stability based on Longitudinal-lateral and Vertical Unified Dynamics Model. Int. J. Automot. Technol. 2022, 23, 73–87. [Google Scholar]
- Huang, L. Study on Stability Control Strategy of 4WID Electric Vehicle Driven by Hub Moto; Hunan University: Changsha, China, 2018. [Google Scholar]
- Zhong, L.; Peng, Y.; Jiang, M. Stability Control of Distributed Driven Electric Vehicle Based on Phase Plane. Automot. Eng. 2021, 43, 721–729, 738. [Google Scholar]
- Xiao, F. Study on State Estimation And Direct Yaw Moment Control for In-Wheel Motor Electric Vehicles; Jilin University: Ji Lin, China, 2016. [Google Scholar]
- Lu, M.; Xu, Z. Integrated Handling and Stability Control with AFS and DYC for 4WID–EVs via Dual Sliding Mode Control. Autom. Control Comput. Sci. 2021, 55, 243–252. [Google Scholar]
- Gong, T.; Xie, X. A Control Strategy of Vehicle Electronic Stability Based on Phase Plane Method. J. Transp. Inf. Saf. 2019, 37, 83–90. [Google Scholar]
- Song, Y.; Shu, H.; Chen, X. Direct-yaw-moment control of four-wheel-drive electrical vehicle based on lateral tyre–road forces and sideslip angle observer. IET Intell. Transp. Syst. 2019, 13, 303–312. [Google Scholar]
- Wang, H.; Han, J.; Zhang, H. Lateral Stability Analysis of 4WID Electric Vehicle Based on Sliding Mode Control and Optimal Distribution Torque Strategy. Actuators 2022, 11, 244. [Google Scholar]
- Sun, X.; Wang, Y.; Cai, Y.; Wong, P.K.; Chen, L.; Bei, S. Nonsingular Terminal Sliding Mode based Direct Yaw Moment Control for Four-Wheel Independently Actuated Autonomous Vehicle. IEEE Trans. Transp. Electrif. 2022, in press.
- Ji, Q. Research on Yaw-Roll Stability Control of Distributed Drive Electric Vehicle; Xihua University: Cheng Du, China, 2021. [Google Scholar]
- Lan, S. Research on Coordinated Control of Roll Stability and Braking Stability Performance of Distributed Electric Vehicle; Liaoning University of Technology: Jin Zhou, China, 2021. [Google Scholar]
- Wang, J. Steering Stability Control of Four-Wheel Drive Vehicle with Hub Motors; Beijing Institute of Technology: Beijing, China, 2015. [Google Scholar]



| Description | Symbol | Value |
|---|---|---|
| Total mass of the car vehicle | m | 1560 (kg) |
| Distance from front axle to the center of centroid | a | 1.617 (m) |
| Distance from rear axle to the center of centroid | b | 1.683 (m) |
| Moment of inertia of the wheel | J | 2.1 () |
| Yaw moment of inertia of vehicle | 1523 () | |
| Distance from the center of centroid to ground | h | 0.556 (m) |
| Track width | L | 1.82 (m) |
| Rolling radius of the tire | R | 0.354 (m) |
| Front wheel cornering stiffness | 16,000 (N/rad) | |
| Rear wheel cornering stiffness | 16,000 (N/rad) | |
| Rolling resistance coefficient | f | 0.015 |
| Description | Symbol | Value |
|---|---|---|
| Rated power of the motor | 64 | |
| Maximum power of motor | 81 KW | |
| Rated torque of motor | 500 Nm | |
| Maximum torque of motor | 800 Nm | |
| Rated speed of motor | 800 r/min | |
| Maximum speed of motor | 1600 r/min |
| Parameter | Value | Parameter | Value |
|---|---|---|---|
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tang, H.; Bei, S.; Li, B.; Sun, X.; Huang, C.; Tian, J.; Hu, H. Mechanism Analysis and Control of Lateral Instability of 4WID Vehicle Based on Phase Plane Analysis Considering Front Wheel Angle. Actuators 2023, 12, 121. https://doi.org/10.3390/act12030121
Tang H, Bei S, Li B, Sun X, Huang C, Tian J, Hu H. Mechanism Analysis and Control of Lateral Instability of 4WID Vehicle Based on Phase Plane Analysis Considering Front Wheel Angle. Actuators. 2023; 12(3):121. https://doi.org/10.3390/act12030121
Chicago/Turabian StyleTang, Haoran, Shaoyi Bei, Bo Li, Xiaoqiang Sun, Chen Huang, Jing Tian, and Hongzhen Hu. 2023. "Mechanism Analysis and Control of Lateral Instability of 4WID Vehicle Based on Phase Plane Analysis Considering Front Wheel Angle" Actuators 12, no. 3: 121. https://doi.org/10.3390/act12030121
APA StyleTang, H., Bei, S., Li, B., Sun, X., Huang, C., Tian, J., & Hu, H. (2023). Mechanism Analysis and Control of Lateral Instability of 4WID Vehicle Based on Phase Plane Analysis Considering Front Wheel Angle. Actuators, 12(3), 121. https://doi.org/10.3390/act12030121







